Submerged Fixed Floating Structure under the Action of Surface Current
Abstract
:1. Introduction
2. Experimental Configuration and Design
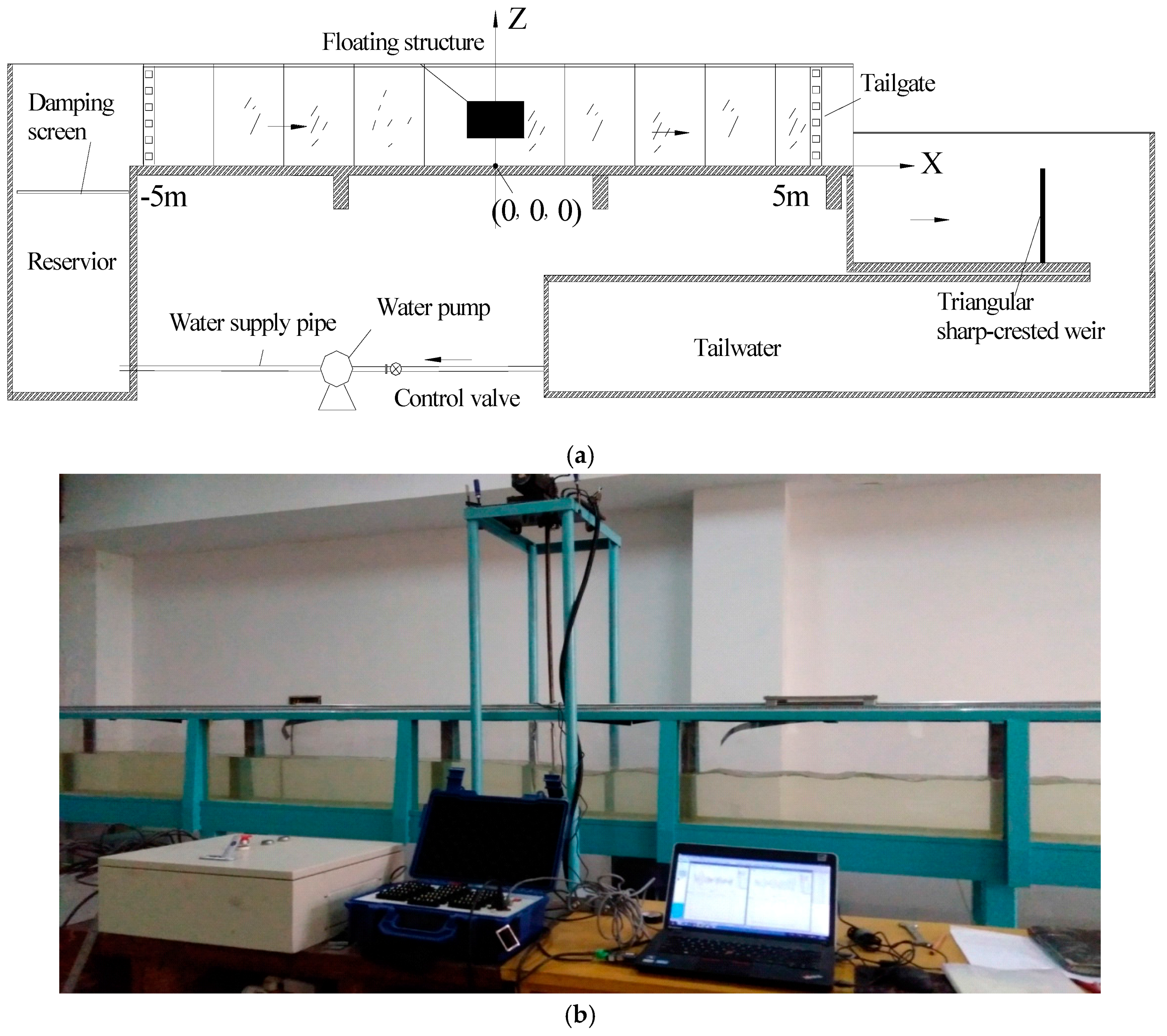
2.1. Experimental Configuration
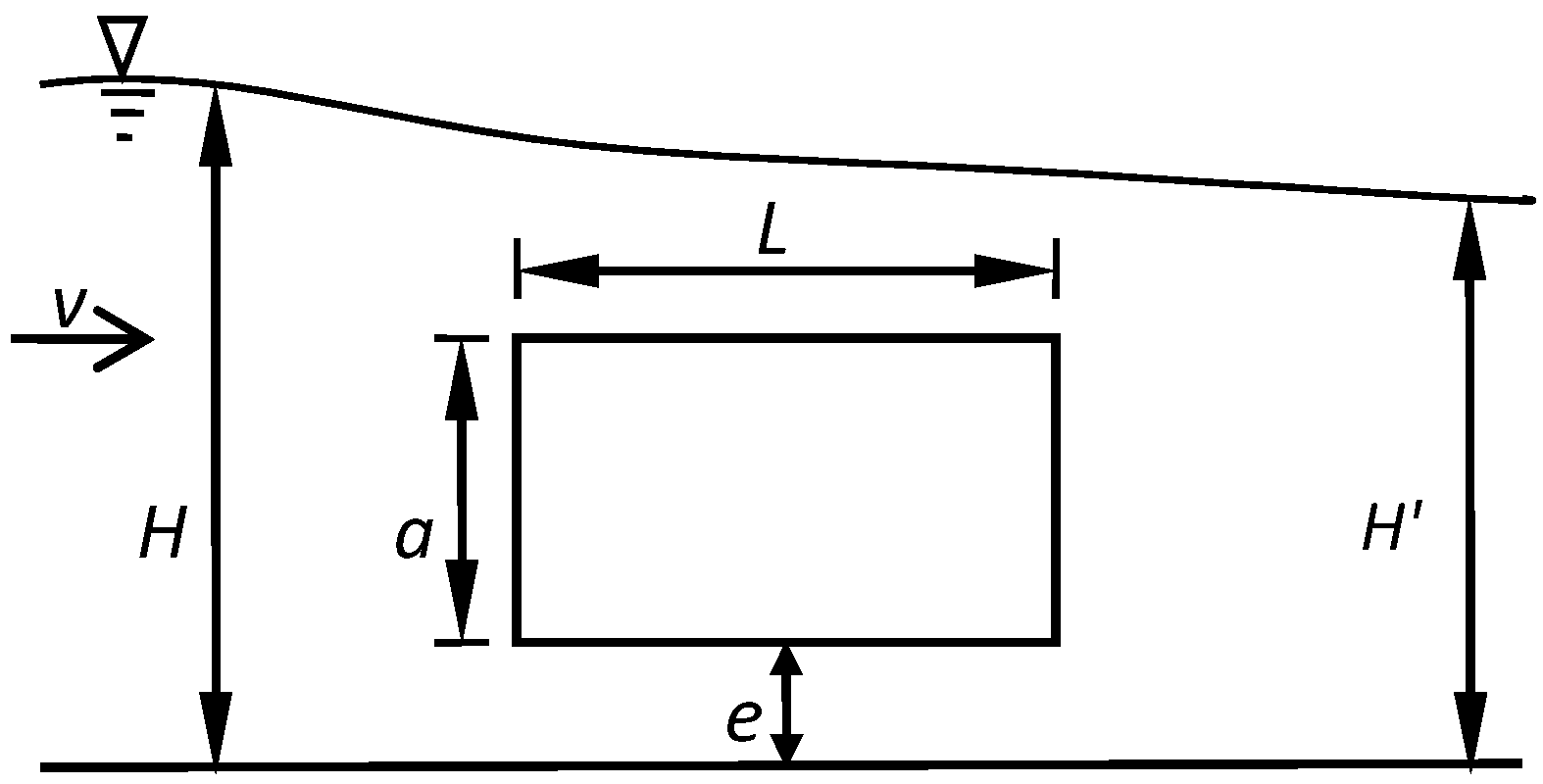
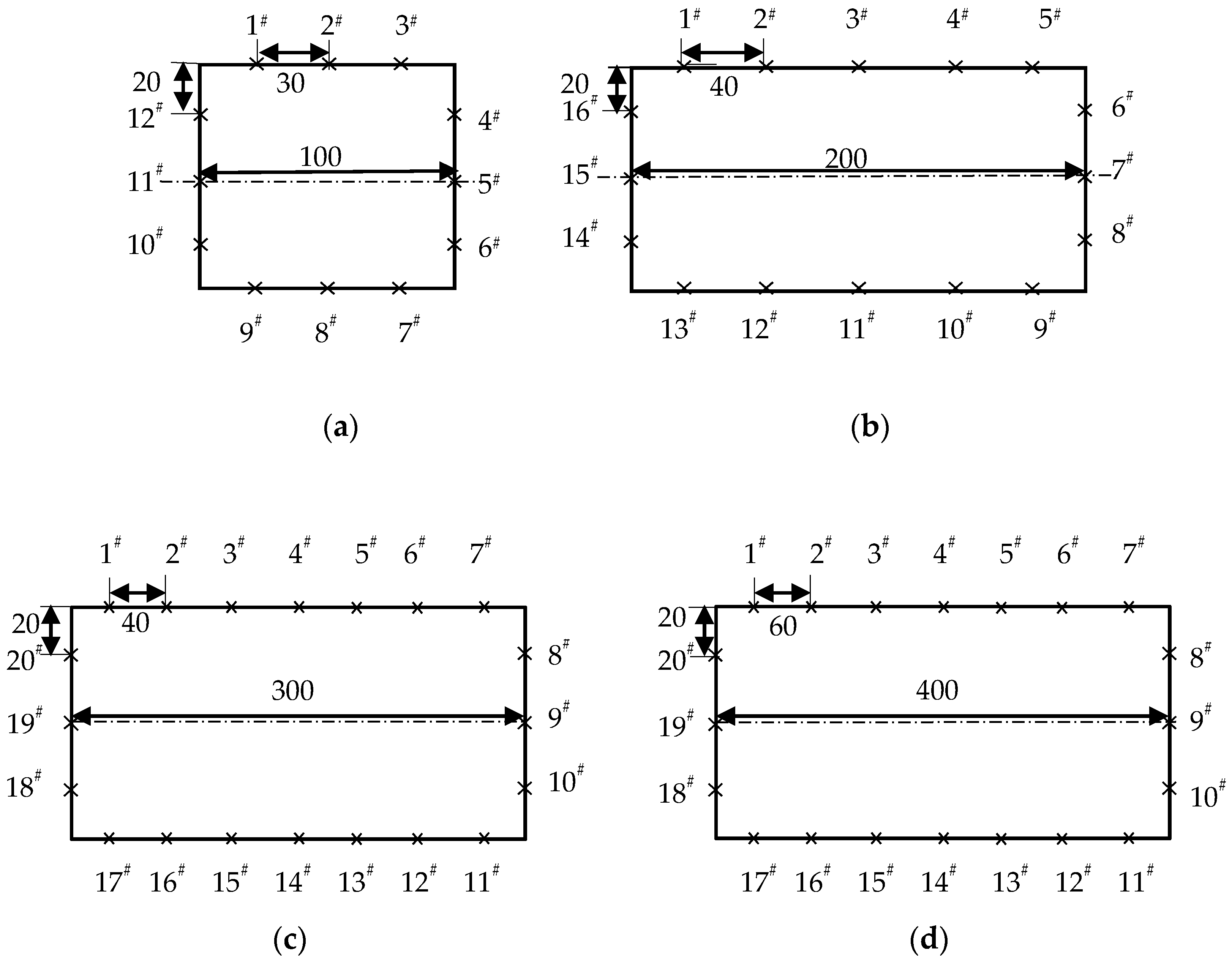
2.2. Design of Experimental Parameters and Test Layout
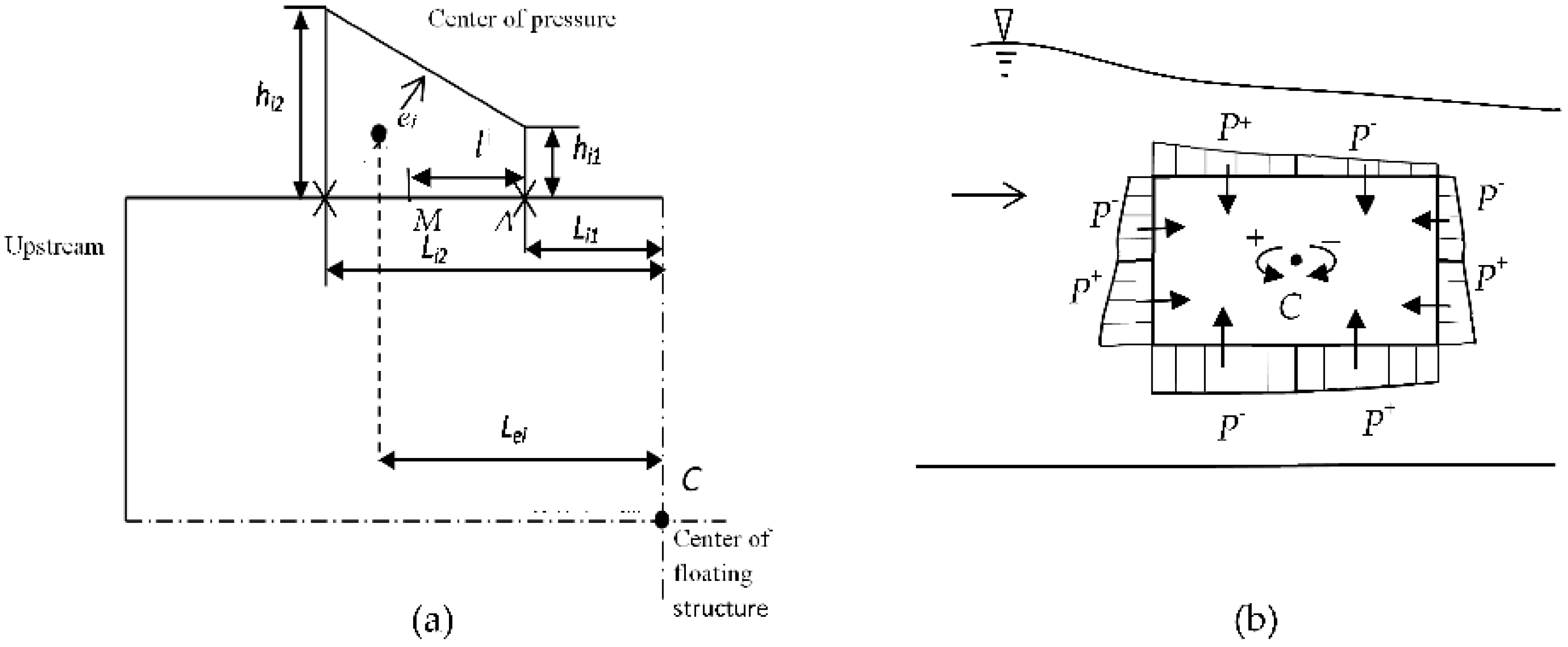
3. Calculation of the Overturning Moment
4. Results and Discussion
4.1. Shape Ratio of the Floating Structure
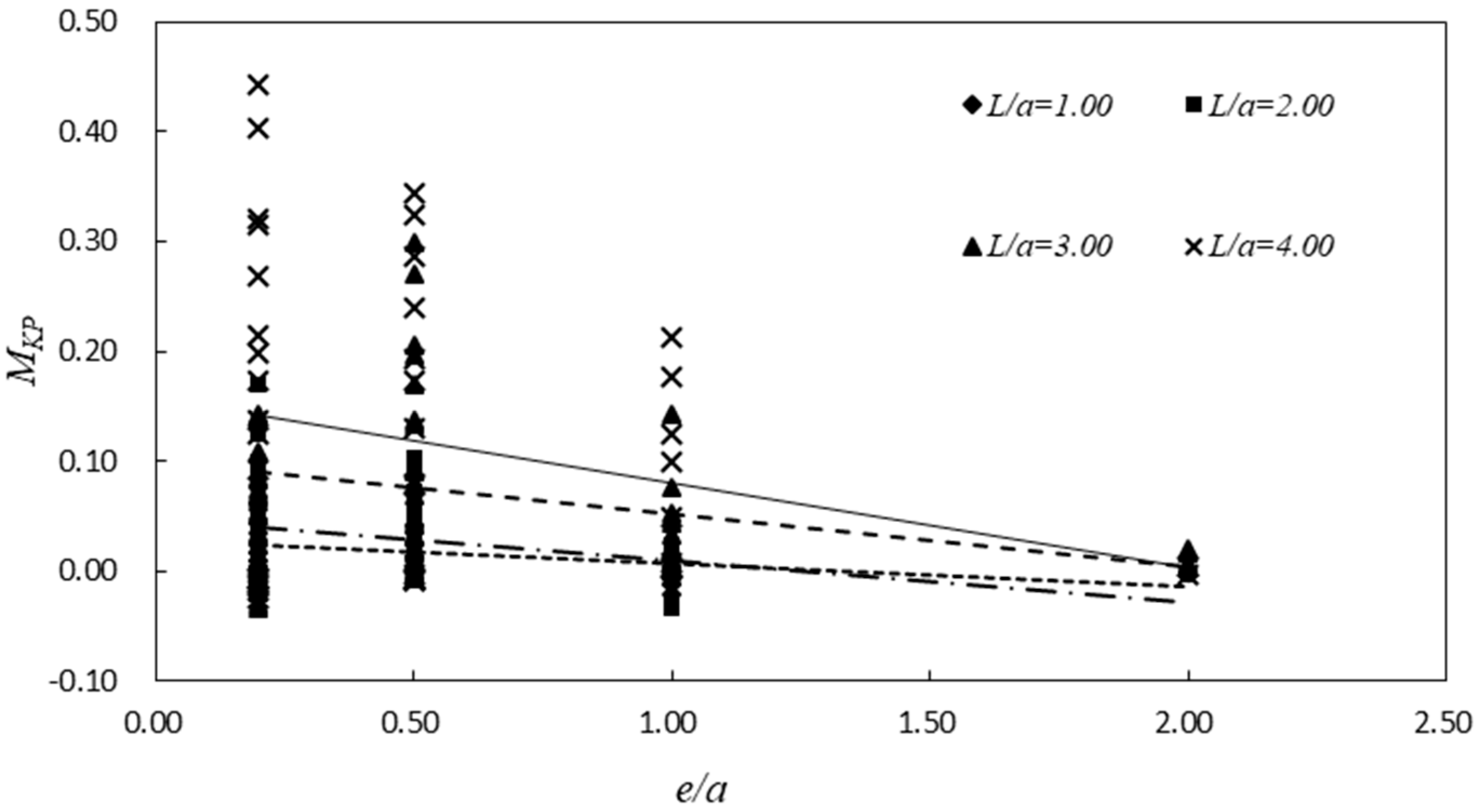
4.2. Vertical Position
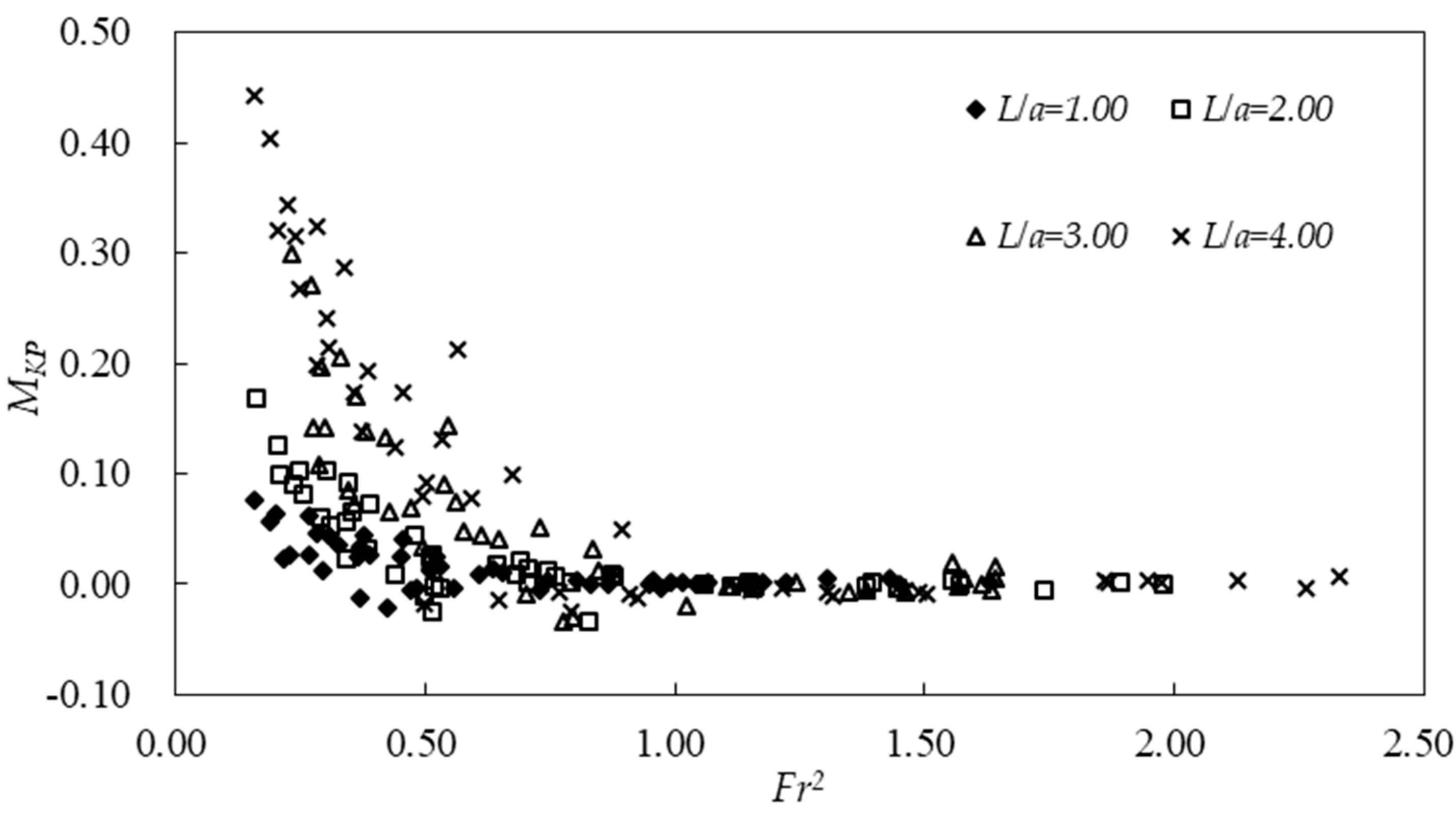
4.3. Froude Number
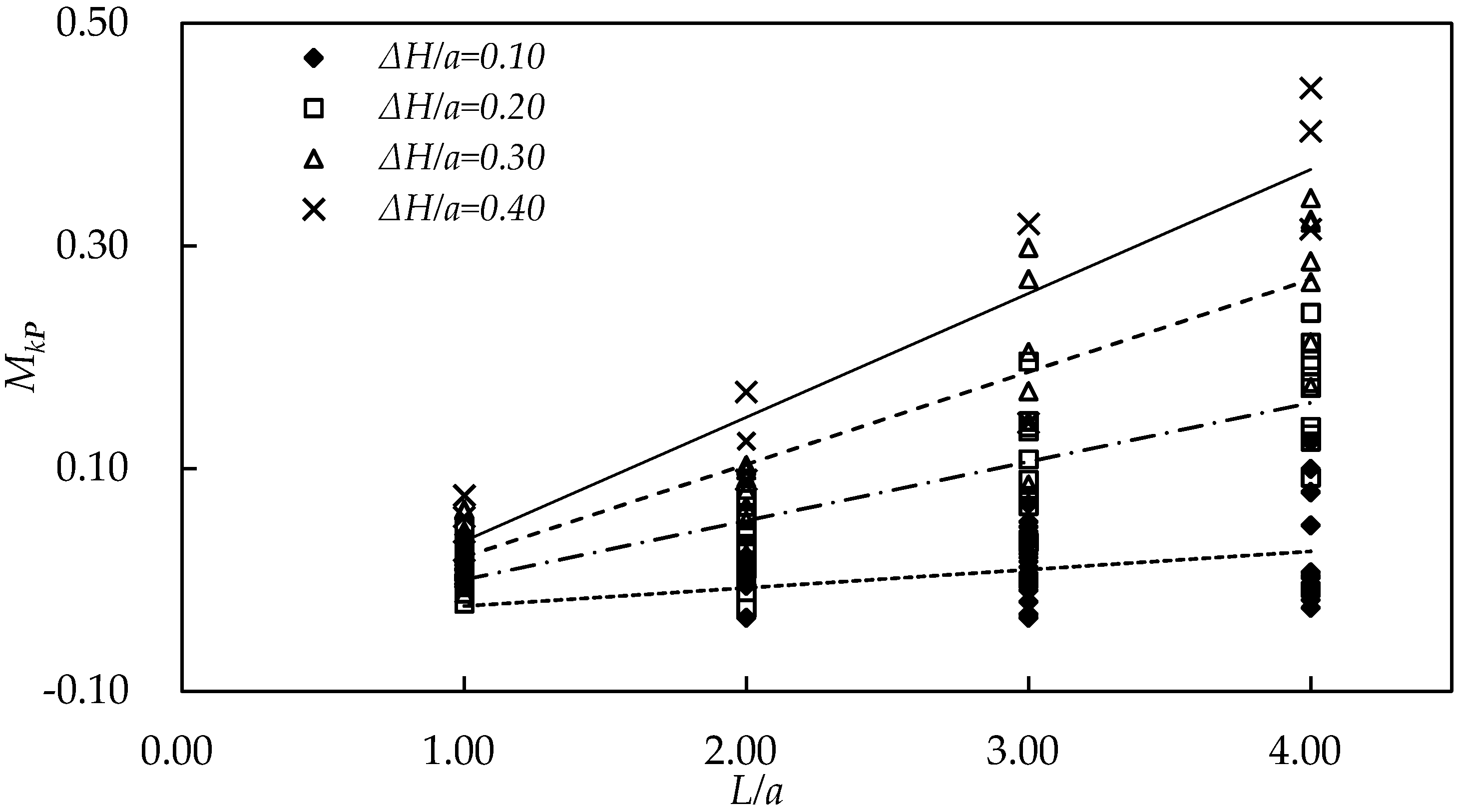
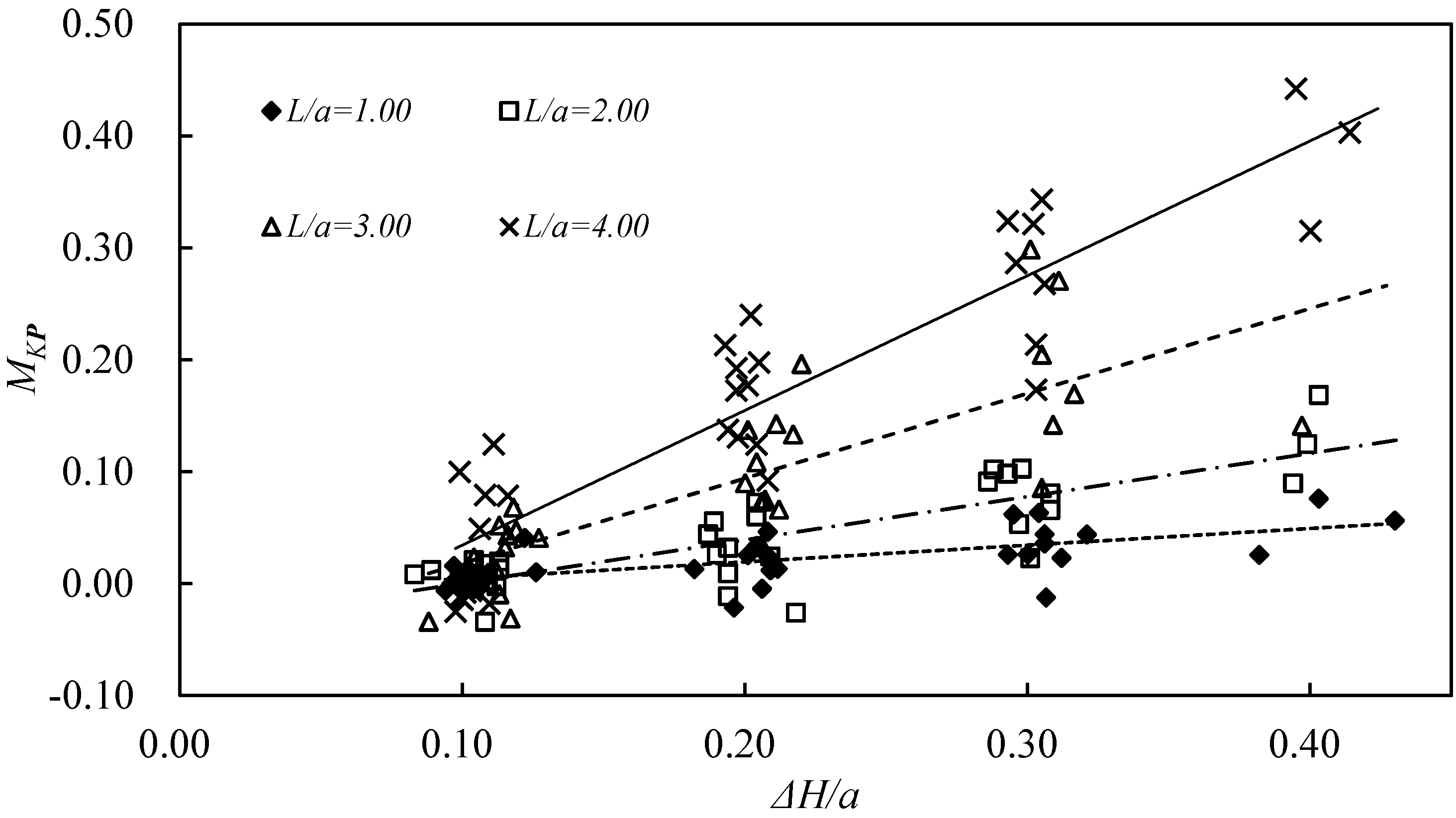
4.4. Water Level
4.5. Calculation Formula of the Overturning Moment
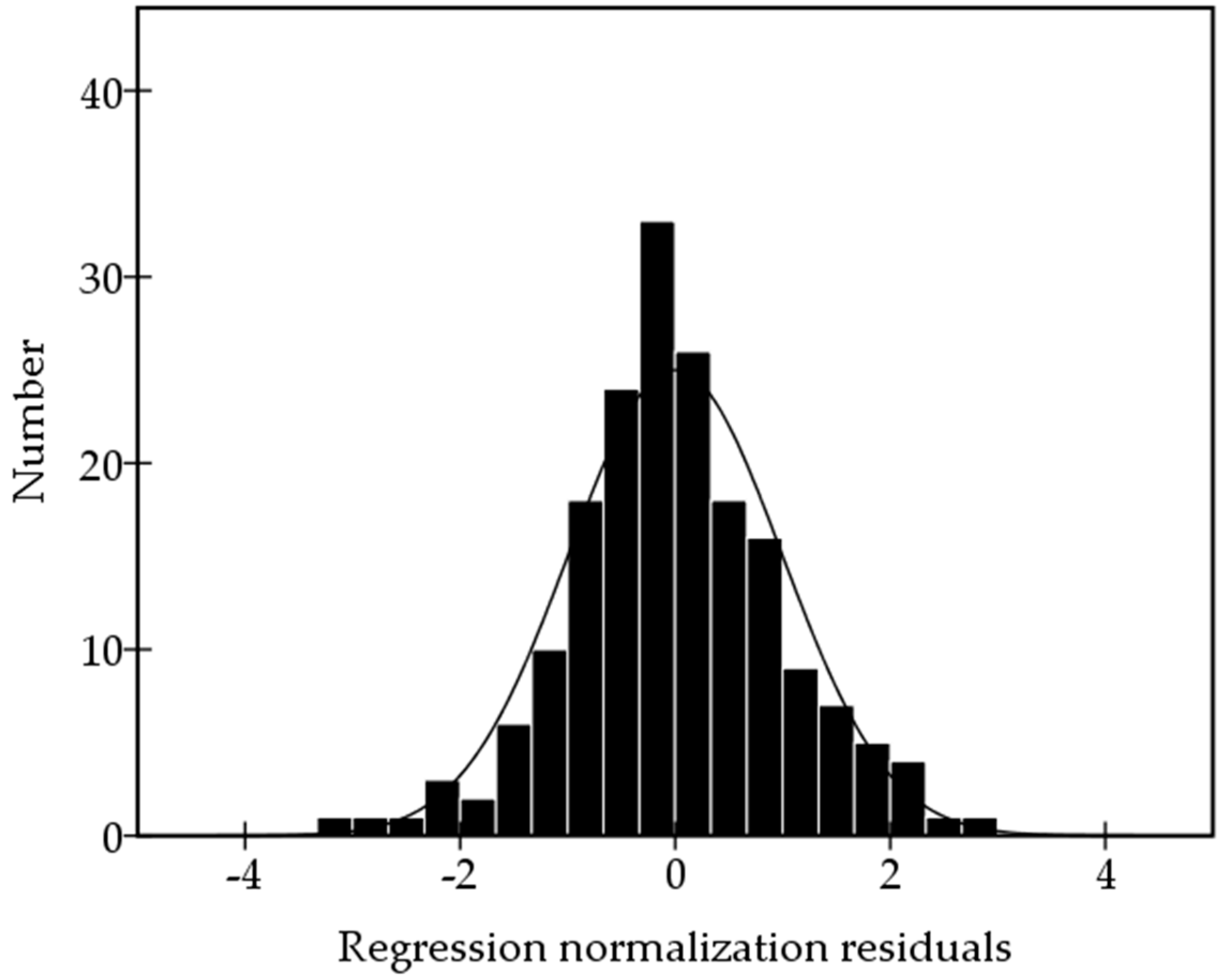
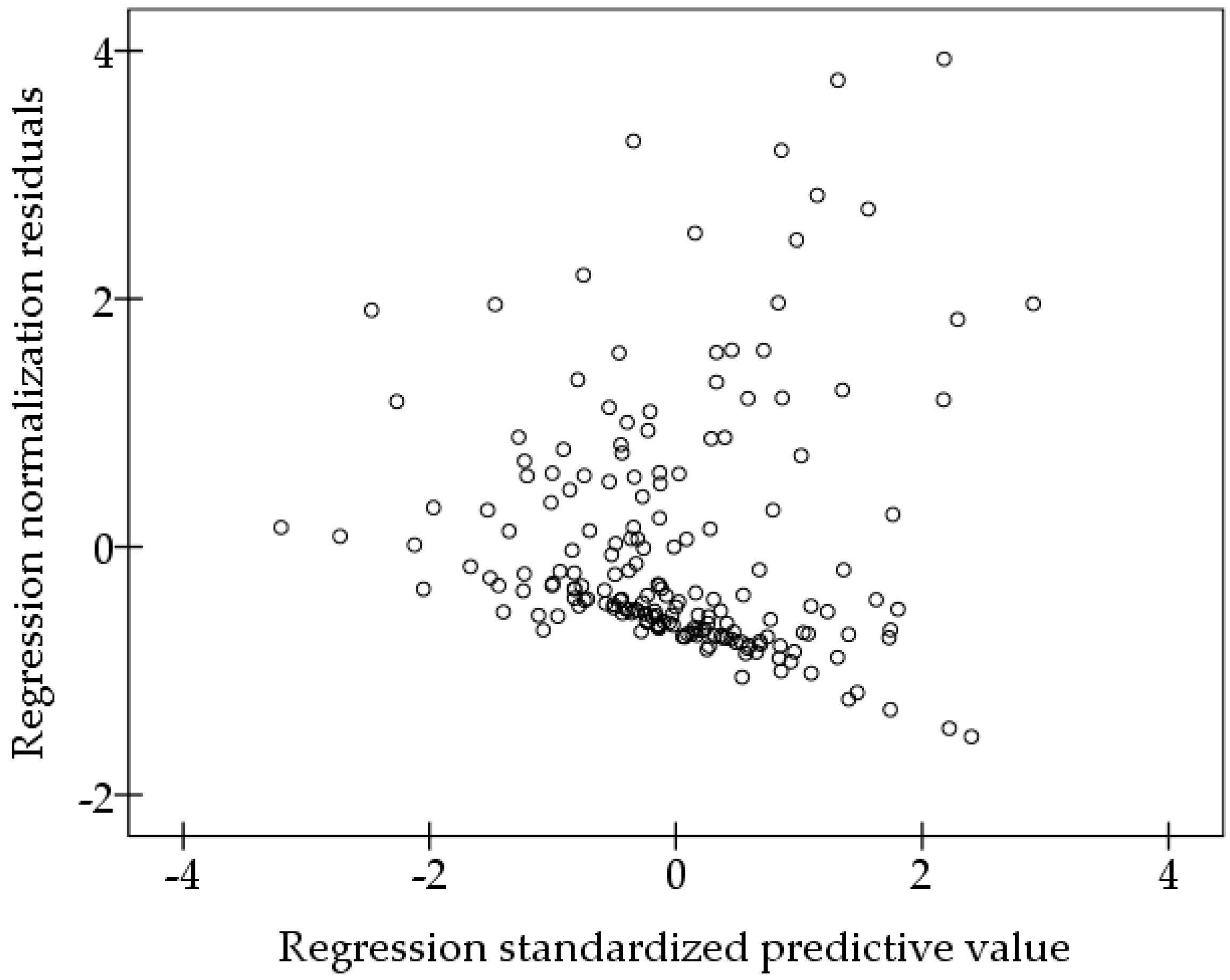
5. Verification and Error Analysis
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Olyaie, E.; Banejad, H.; Chau, K.; Melesse, A.M. A comparison of various artificial intelligence approaches performance for estimating suspended sediment load of river systems: A case study in United States. Environ. Monit. Assess. 2015, 187, 189. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.; Xu, D.; Chau, K.; Lei, G. Assessment of river water quality based on theory of variable fuzzy sets and fuzzy binary comparison method. Water Resour. Manag. 2014, 28, 4183–4200. [Google Scholar] [CrossRef]
- Gholami, V.; Chau, K.W.; Fadaee, F.; Torkaman, J.; Ghaffari, A. Modeling of groundwater level fluctuations using dendrochronology in alluvial aquifers. J. Hydrol. 2015, 529, 1060–1069. [Google Scholar] [CrossRef]
- Chau, K. Use of Meta-Heuristic Techniques in Rainfall-Runoff Modelling. Water 2017, 9, 186. [Google Scholar] [CrossRef]
- Albano, R.; Sole, A.; Mirauda, D.; Adamowski, J. Modelling large floating bodies in urban area flash-floods via a Smoothed Particle Hydrodynamics model. J. Hydrol. 2016, 541, 344–358. [Google Scholar] [CrossRef]
- Chen, L.F.; Sun, L.; Zang, J.; Hillis, A.J.; Plummer, A.R. Numerical study of roll motion of a 2-D floating structure in viscous flow. J. Hydrodyn. Ser. B 2016, 28, 544–563. [Google Scholar] [CrossRef] [Green Version]
- Fu, Z.F.; Yan, Z.M. Stability analysis on a new type of floating sluice. J. Hydraul. Eng. 2005, 8, 1014–1018. [Google Scholar] [CrossRef]
- Roy, P.D.; Ghosh, S. Wave force on vertically submerged circular thin plate in shallow water. Ocean Eng. 2006, 33, 1935–1953. [Google Scholar] [CrossRef]
- Seiffert, B.; Hayatdavoodi, M.; Ertekin, R.C. Experiments and computations of solitary-wave forces on a coastal-bridge deck. Part I: Flat plate. Coast. Eng. 2014, 88, 194–209. [Google Scholar] [CrossRef]
- Hayatdavoodi, M.; Seiffert, B.; Ertekin, R.C. Experiments and computations of solitary-wave forces on a coastal-bridge deck. Part II: Deck with girders. Coast. Eng. 2014, 88, 210–228. [Google Scholar] [CrossRef]
- Hayatdavoodi, M.; Ertekin, R.C. Wave forces on a submerged horizontal plate—Part I: Theory and modelling. J. Fluid Struct. 2015, 54, 566–579. [Google Scholar] [CrossRef]
- Hayatdavoodi, M.; Ertekin, R.C. Wave forces on a submerged horizontal plate—Part II: Solitary and cnoidal waves. J. Fluid Struct. 2015, 54, 580–596. [Google Scholar] [CrossRef]
- Lee, S.M.; Hong, C.B. Characteristics of wave exciting forces on a very large floating structure with submerged plate. J. Mech. Sci. Technol. 2005, 11, 2061–2067. [Google Scholar] [CrossRef]
- Belibassakis, K.A.; Athanassoulis, G.A. A coupled-mode technique for weakly nonlinear wave interaction with large floating structures lying over variable bathymetry regions. Appl. Ocean Res. 2006, 28, 59–76. [Google Scholar] [CrossRef]
- Liu, C.F.; Teng, B.; Gou, Y.; Sun, L. A 3D time-domain method for predicting the wave-induced forces and motions of a floating body. Ocean Eng. 2011, 38, 2142–2150. [Google Scholar] [CrossRef]
- Hadžić, I.; Hennig, J.; Peric, M.; Xing-Kaeding, Y. Computation of flow-induced motion of floating bodies. Appl. Math. Model. 2005, 29, 1196–1210. [Google Scholar] [CrossRef]
- Zhou, B.Z.; Wu, G.X.; Meng, Q.C. Interactions of fully nonlinear solitary wave with a freely floating vertical cylinder. Eng. Anal. Bound. Elem. 2016, 69, 119–131. [Google Scholar] [CrossRef]
- Xing, D.L.; Deng, Y.P.; Zhou, M. Experimental research for added mass of cylinders with reflected boundary condition. J. Dalian Univ. Technol. 1998, 38, 107–111. [Google Scholar] [CrossRef]
- Xing, D.L. Research on hydrodynamic performance of floating bodies in confined zone. J. Dalian Univ. Technol. 1993, 3, 351–355. [Google Scholar] [CrossRef]
- Lu, Y. Stability Analysis and Experimental Study of Large Floating Box Door in Dynamic Water. Master’s Thesis, Hohai University, Nanjing, China, 2002. [Google Scholar]
- Fu, Z.F.; Yin, X.J.; Gu, X.F. Hydraulic characteristics of floating sluices subsiding and buoying in flowing water. Adv. Sci. Technol. Water Resour. 2014, 34, 24–27. [Google Scholar] [CrossRef]
- Huang, S.Q.; Huang, S.F.; Huang, G.F.; Xu, J. The research on numerical simulation of on-off process of floating sluice in dynamic water. In Proceedings of the 23rd National Symposium on Water Dynamics and the 10th National Symposium on Water Dynamics, Xi’an, China, 19–24 September 2011; p. 7. [Google Scholar]
- Fan, B.Q.; Suo, L.S.; Zhou, J. Analysis of floating structures for tidal power houses. Water Conserv. Hydropower Sci. Technol. Prog. 2002, 22, 20–22. [Google Scholar]
- Fan, B.Q.; Suo, L.S. Floating Stability Calculation of Floating-caisson of Tidal Power Plants. Water Conserv. Hydropower Sci. Technol. Prog. 2000, 3, 49–52. [Google Scholar]
- Johnson, H.K.; Karambas, T.V.; Avgeris, I.; Zanuttigh, B.; Gonzalez-Marco, D.; Caceres, I. Modelling of Waves and Currents around Submerged Breakwaters. Coast. Eng. 2005, 52, 949–969. [Google Scholar] [CrossRef]
- Rey, V.; Touboul, J. Forces and moment on a horizontal plate due to regular and irregular waves in the presence of current. Appl. Ocean Res. 2011, 33, 88–99. [Google Scholar] [CrossRef]
- Karmakar, D.; Guedes Soares, C. Scattering of gravity waves by a moored finite floating elastic plate. Appl. Ocean Res. 2012, 34, 135–149. [Google Scholar] [CrossRef]
- Cui, Z.; Fu, Z.; Chen, Y. Explore the flow characteristics of floating structure based on the orthogonal design method. J. Wuhan Univ. (Eng. Ed.) 2018. accepted. [Google Scholar]
- Zhen, C.; Zongfu, F.; Yuejun, C.; Shan, W.; Guanggang, M.; Wen, J. Explore the hydrodynamic force on the surface of floating structure in finite flowing water. In Proceedings of the 37th International Association for the History of Religions (IAHR) World Congress, Kuala Lumpur, Malaysia, 13–18 August 2017. [Google Scholar]

| L (cm) | e (cm) | q (m2 s−1) | ΔH (cm) | |||
|---|---|---|---|---|---|---|
| 10/20/30/40 | 2 | 0.05 | 1.00 | 2.00 | 3.00 | 4.00 |
| 0.06 | 1.00 | 2.00 | 3.00 | 4.00 | ||
| 0.07 | 1.00 | 2.00 | 3.00 | 4.00 | ||
| 0.08 | 1.00 | 200 | 3.00 | 4.00 | ||
| 5 | 0.05 | 1.00 | 2.00 | 3.00 | 4.00 | |
| 0.06 | 1.00 | 2.00 | 3.00 | 4.00 | ||
| 0.07 | 1.00 | 2.00 | 3.00 | 4.00 | ||
| 0.08 | 1.00 | 2.00 | 3.00 | 4.00 | ||
| 10 | 0.05 | 1.00 | 2.00 | 3.00 | 4.00 | |
| 0.06 | 1.00 | 2.00 | 3.00 | 4.00 | ||
| 0.07 | 1.00 | 2.00 | 3.00 | 4.00 | ||
| 0.08 | 1.00 | 2.00 | 3.00 | 4.00 | ||
| 20 | 0.05 | 1.00 | 2.00 | / | / | |
| 0.06 | 1.00 | 2.00 | / | / | ||
| 0.07 | 1.00 | 2.00 | / | / | ||
| 0.08 | 1.00 | 2.00 | / | / | ||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cui, Z.; Fu, Z.-f.; Dai, W.-h.; Lai, Z.-q. Submerged Fixed Floating Structure under the Action of Surface Current. Water 2018, 10, 102. https://doi.org/10.3390/w10020102
Cui Z, Fu Z-f, Dai W-h, Lai Z-q. Submerged Fixed Floating Structure under the Action of Surface Current. Water. 2018; 10(2):102. https://doi.org/10.3390/w10020102
Chicago/Turabian StyleCui, Zhen, Zong-fu Fu, Wen-hong Dai, and Zheng-qing Lai. 2018. "Submerged Fixed Floating Structure under the Action of Surface Current" Water 10, no. 2: 102. https://doi.org/10.3390/w10020102
APA StyleCui, Z., Fu, Z.-f., Dai, W.-h., & Lai, Z.-q. (2018). Submerged Fixed Floating Structure under the Action of Surface Current. Water, 10(2), 102. https://doi.org/10.3390/w10020102





