Impact of Drizzle-Sized Cloud Particles on Production of Precipitation in Hailstorms: A Sensitivity Study
Abstract
:1. Introduction
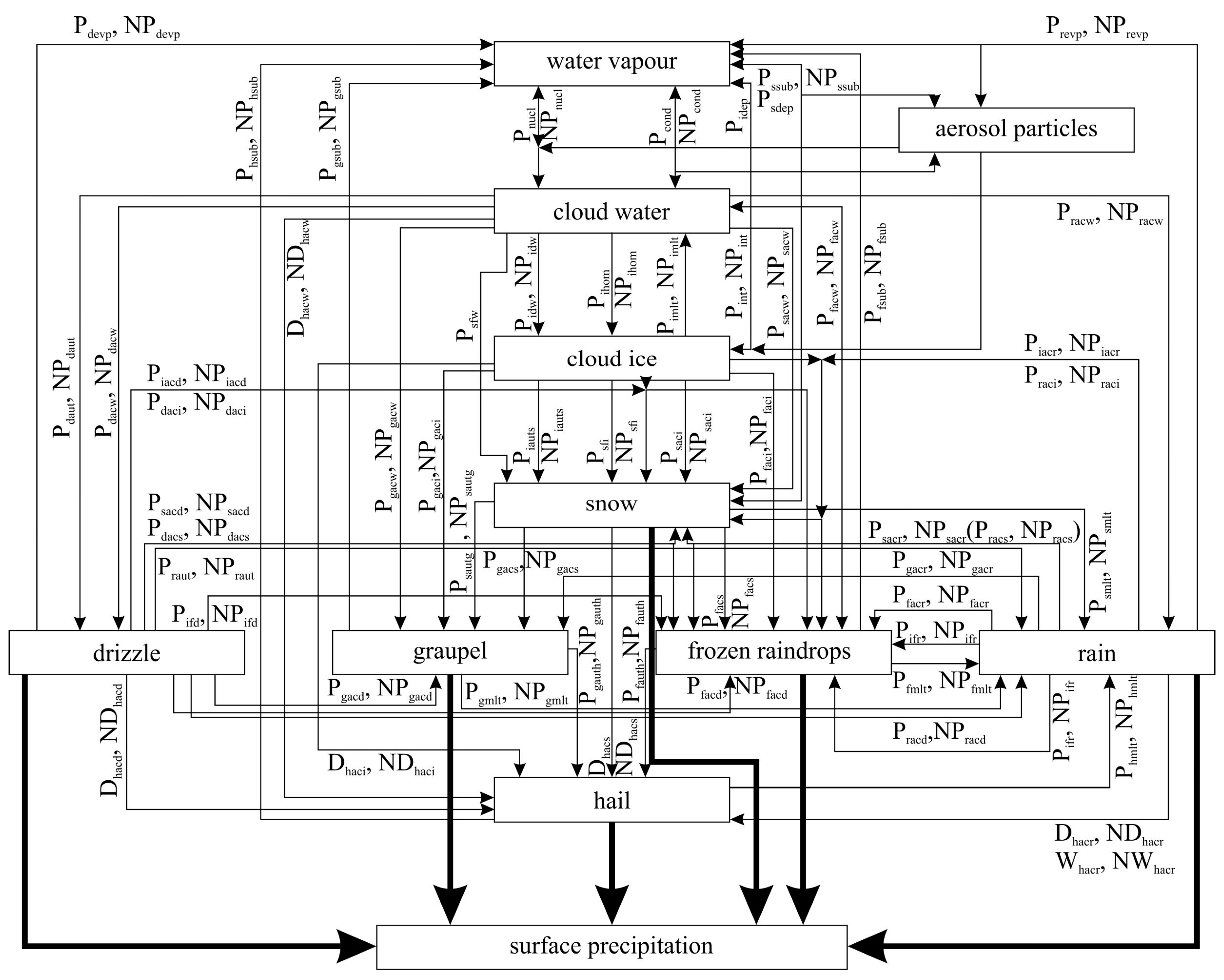
2. Model Description
2.1. General Model Features
2.2. Model Microphysics
2.2.1. Cloud Droplet Activation
2.2.2. Drizzle Representation
3. Model Results and Discussion
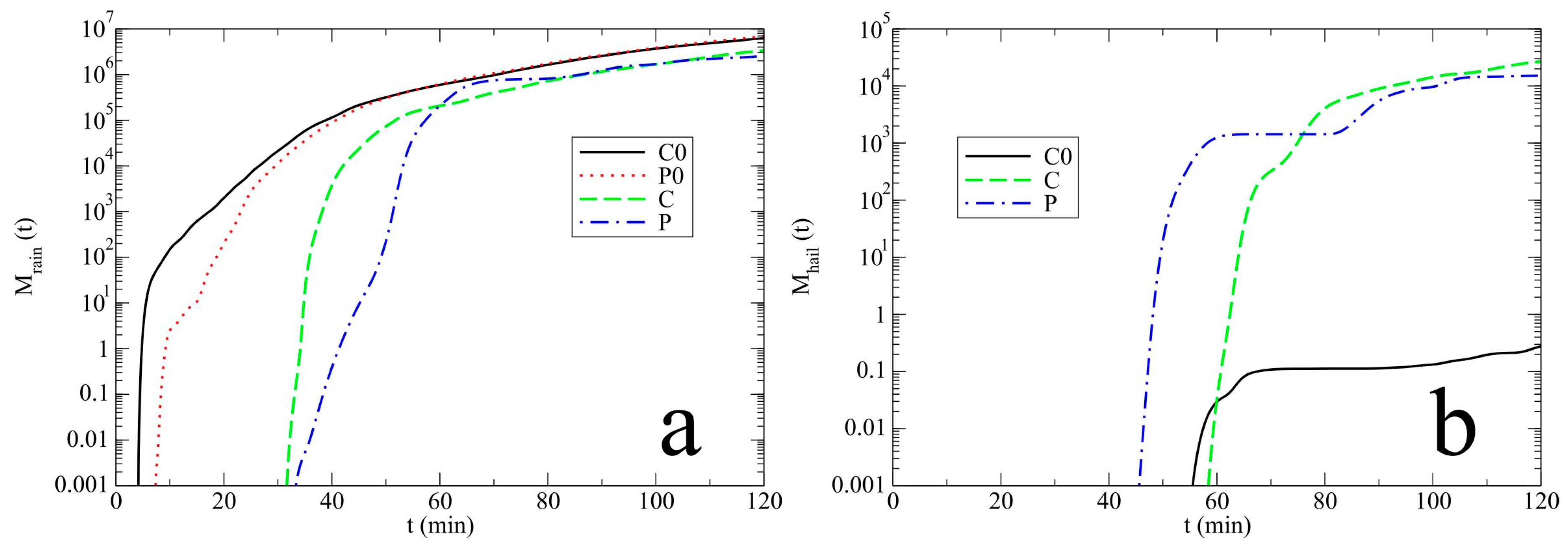
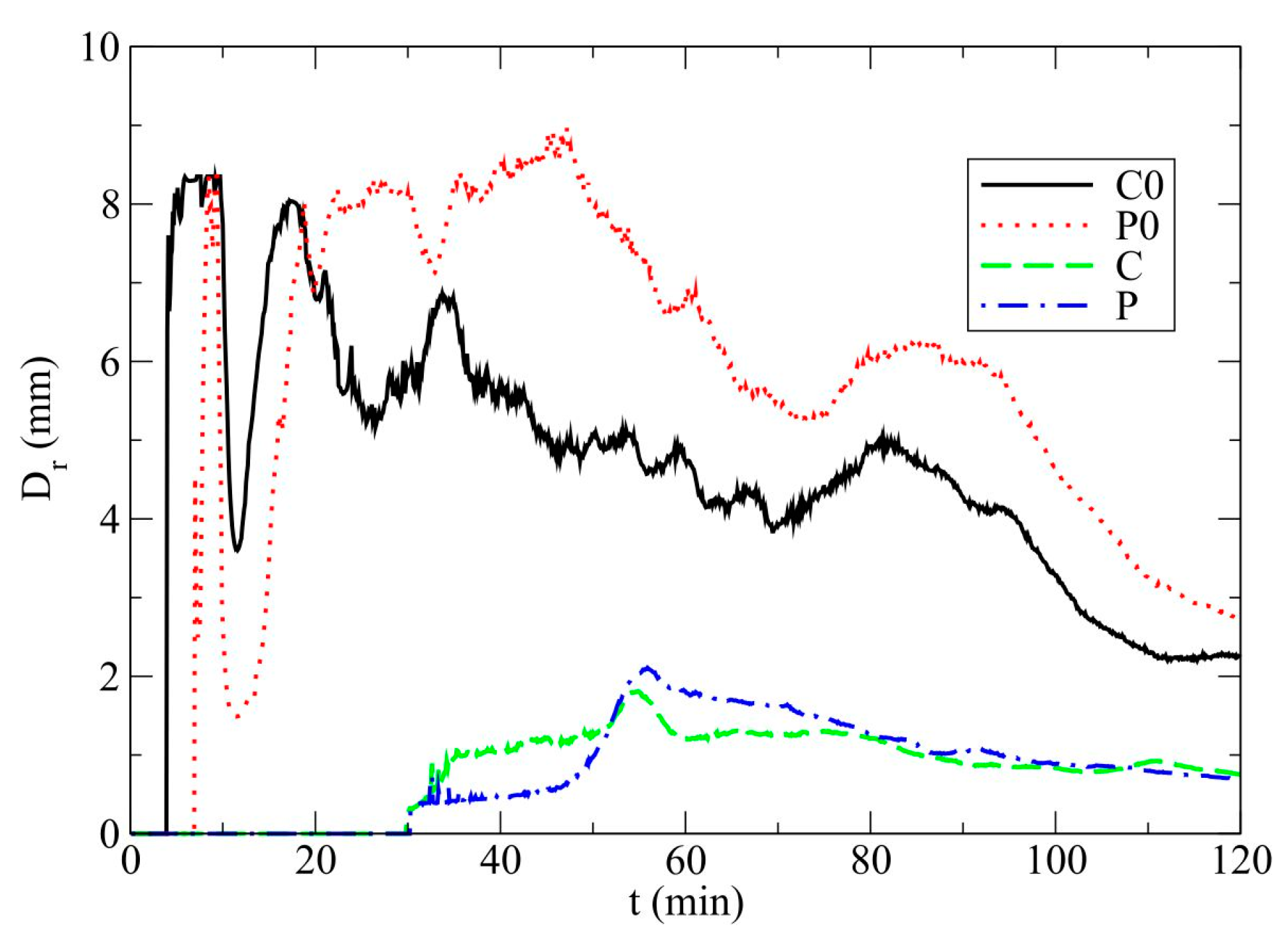
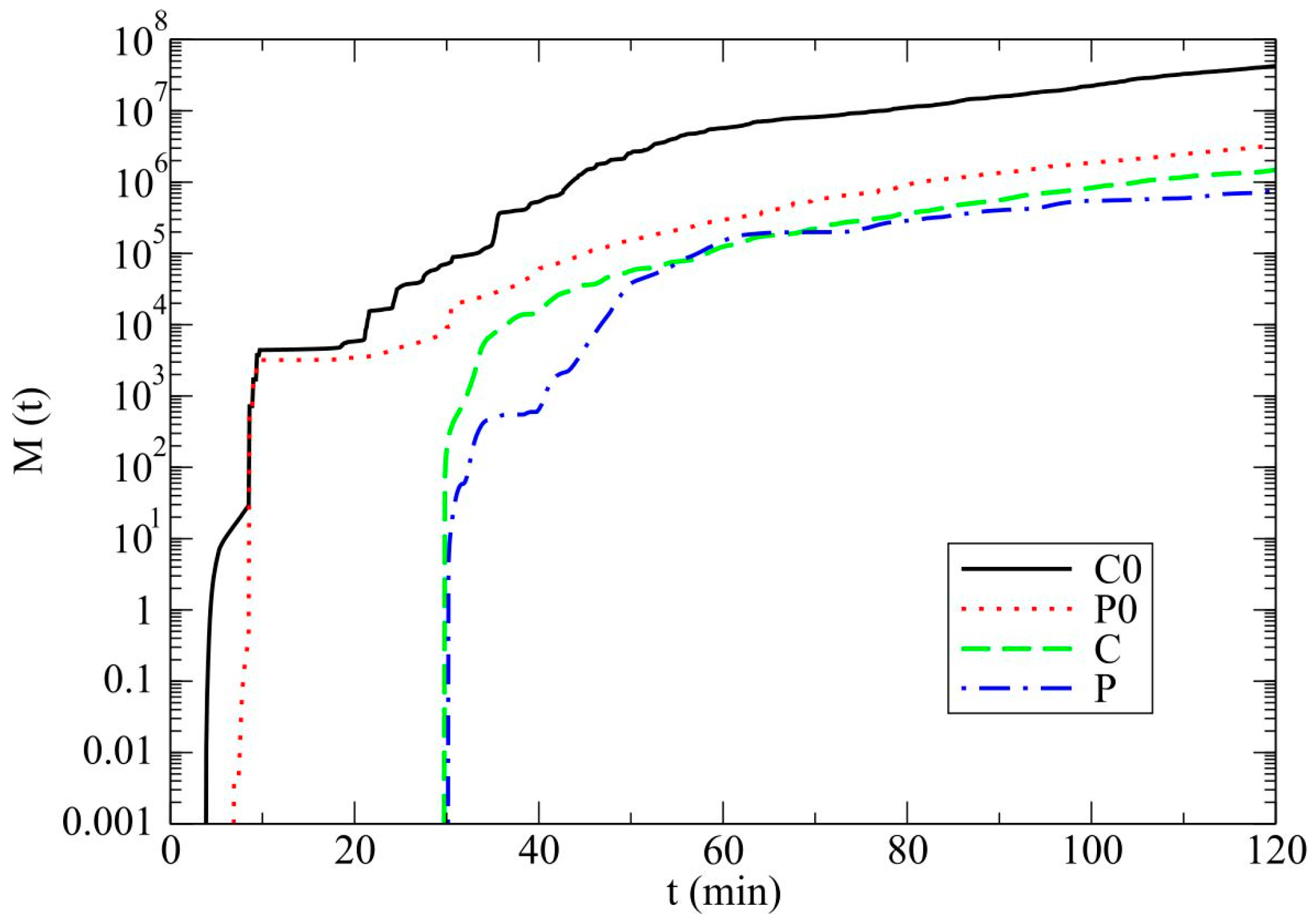
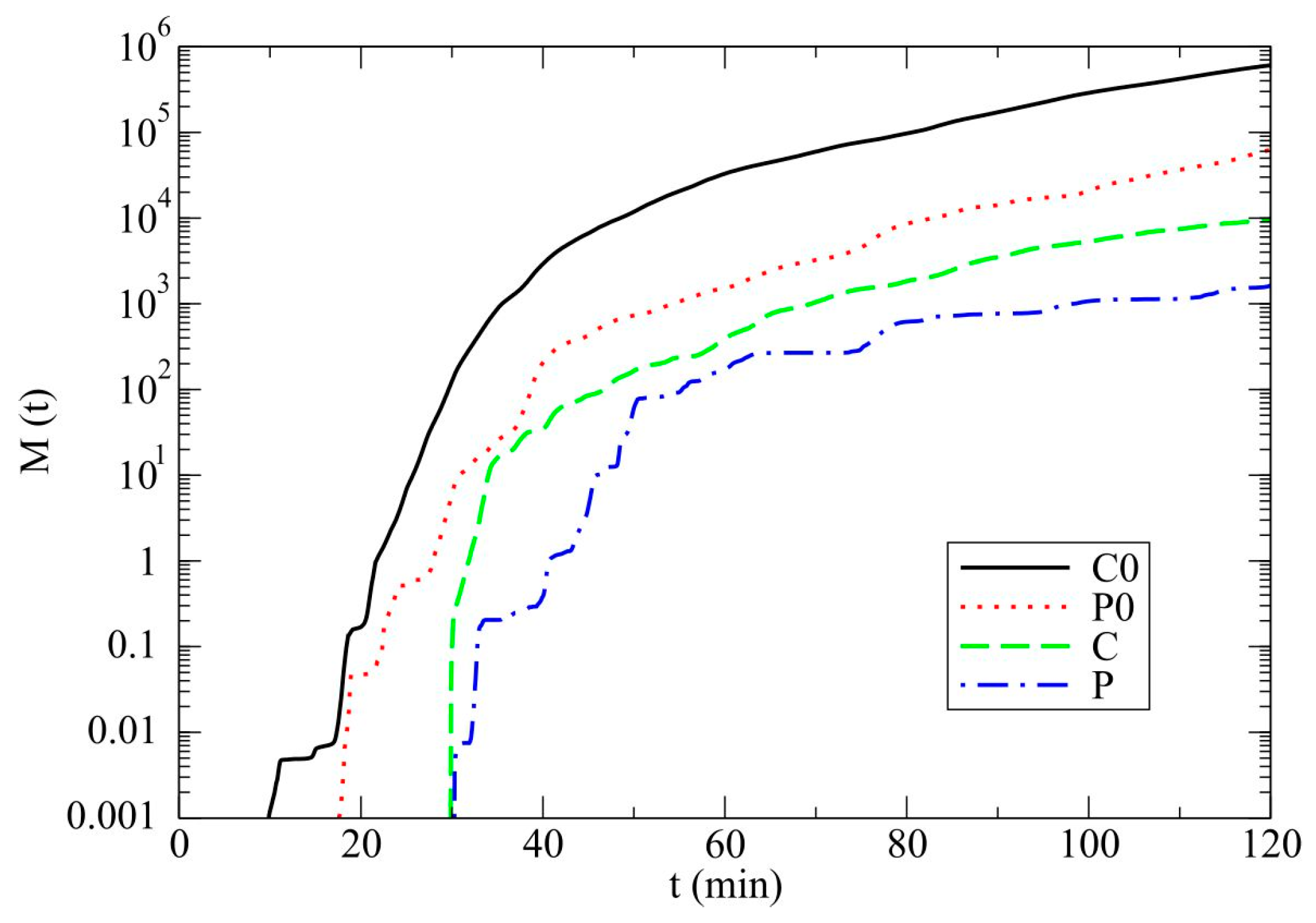
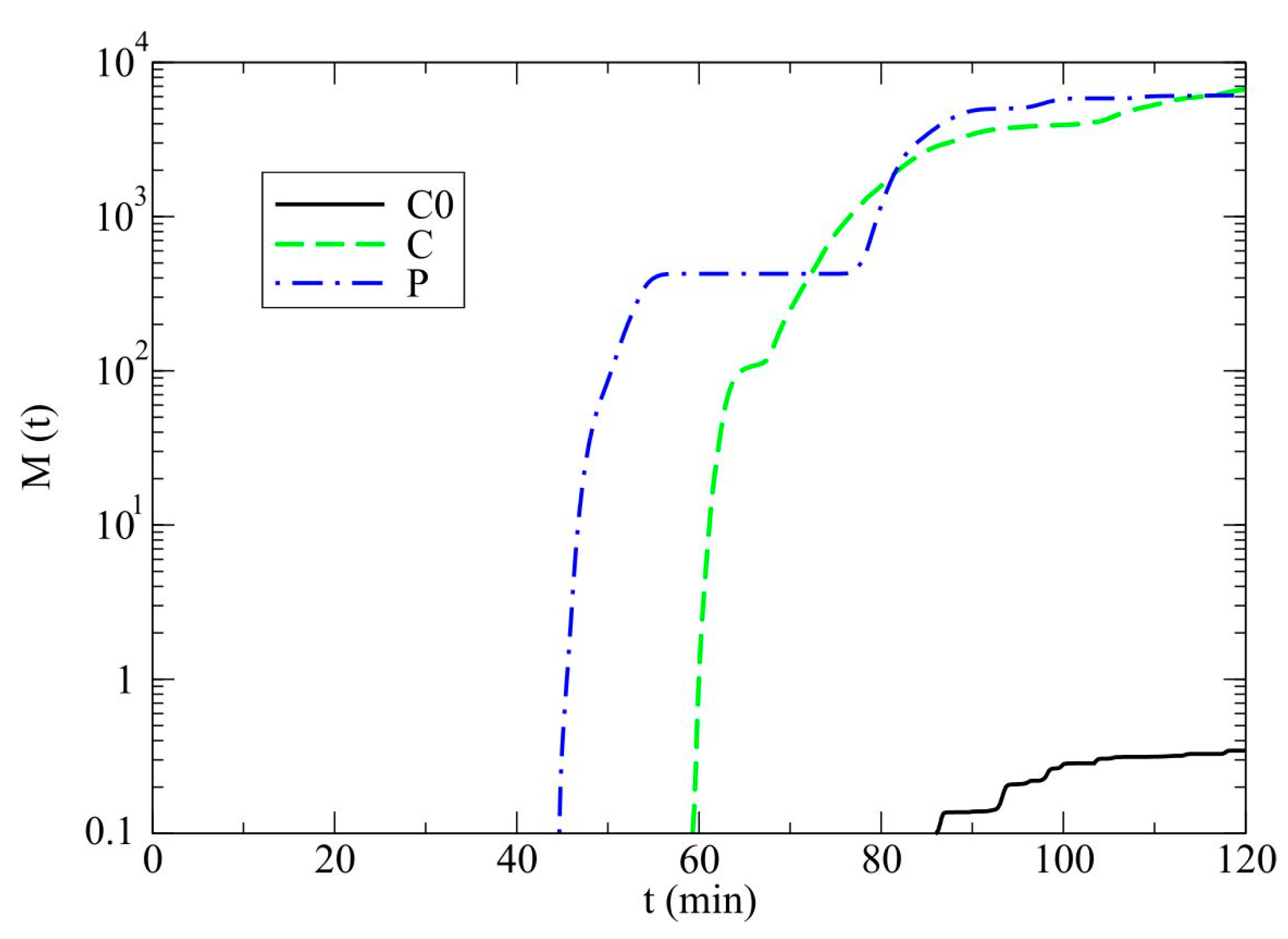
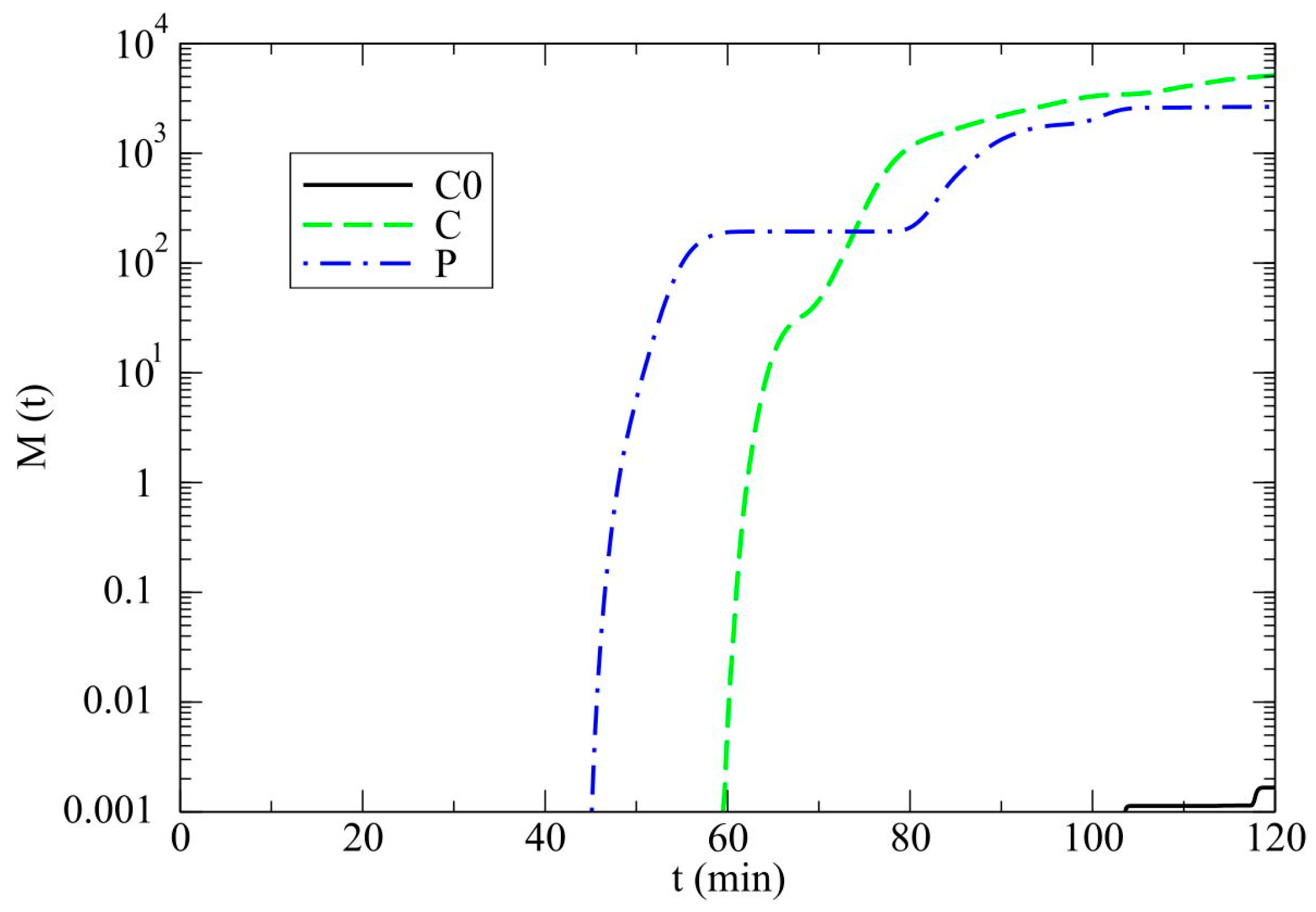
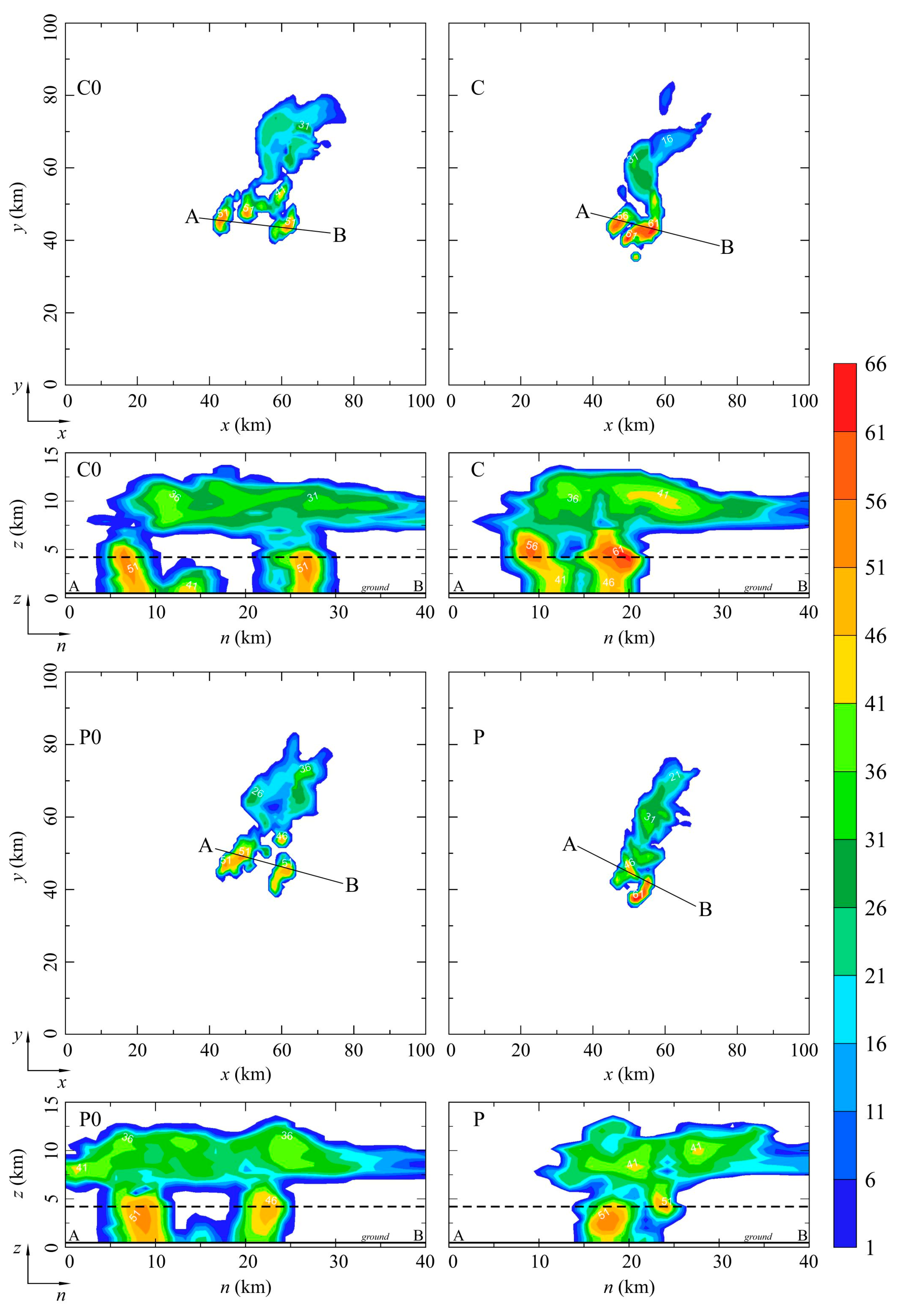
3.1. Model Results
3.2. Discussion
4. Conclusions
- The rain amounts on the ground are slightly lower in the absence of drizzle. In conditions with drizzle, a slower autoconversion of drizzle to rain and smaller accretion of cloud droplets by raindrops leads to weaker development of rain in a hailstorm and narrower rain bands at the surface.
- In simulations of a hailstorm in polluted cases that do not include the drizzle, there is no hail production.
- The mean diameters of raindrops are unrealistically large in non-drizzle cases in comparison with typical observed raindrops. In alternate cases, the values of the mean diameters of raindrops are consistent with common knowledge about size of raindrops.
- The drizzle case generates significantly more hail on the ground due to an excess of cloud droplets (due to slower accretion by rain) which may serve as a source for the hail growth via accretion of cloud droplets.
- Drizzle scheme causes a slightly greater value of radar reflectivity factor due to the fact that the impact of number concentration on the Z-values exceeds the corresponding impact of hydrometeor sizes in comparison with alternate cases. Noteworthy is the slightly faster movement of the hailstorm in simulations without drizzle category.
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
- Collection of cloud water by drizzle is source for drizzle and sink for cloud water:where: F1 = 4, F2 = 4, F3 = 1.
- Collection of drizzle by rain is source for rain and sink for drizzle:where: E1 = 1, E2 = 2, E3 = 1.
- Collection of cloud ice by drizzle is source for frozen raindrops or for snow (if qi, qd < 10−4 kg kg−1) and sink for cloud ice and drizzle:
- Collection of drizzle by cloud ice is source for frozen raindrops or for snow (if qi, qd < 10−4 kg kg−1) and sink for cloud ice and drizzle:
- Collection of snow by drizzle is source for frozen raindrops or for snow (if qs, qd < 10−4 kg kg−1) and sink for snow and drizzle:
- Collection of drizzle by snow is source for frozen raindrops or for snow (if qs, qd < 10−4 kg kg−1) and sink for snow and drizzle:
- Collection of drizzle by graupel is source for graupel and sink for drizzle:
- Collection of drizzle by frozen raindrops is source for frozen raindrops and sink for drizzle:
- Collection of drizzle by hail is source for hail and sink for drizzle:
- Immersion freezing of drizzle is source for frozen raindrops and sink for drizzle:
- Drizzle evaporation is source for water vapour and sink for drizzle:
Appendix B
| Symbol | Description | Value | Units |
| λi | slope parameter for cloud ice | m−1 | |
| λs | slope parameter for snow | m−1 | |
| λg | slope parameter for graupel | m−1 | |
| λf | slope parameter for frozen raindrops | m−1 | |
| λh | slope parameter for hail | m−1 | |
| N0i | intercept parameter for cloud ice | m−5 | |
| N0s | intercept parameter for snow | m−4 | |
| N0g | intercept parameter for graupel | m−4 | |
| N0f | intercept parameter for frozen raindrops | m−4 | |
| N0h | intercept parameter for hail | m−4 | |
| ρ0 | standard air density | 1.225 | kg m−3 |
| ρs | density of snow | 102 | kg m−3 |
| ρh | density of hail | 917 | kg m−3 |
| a | constant for terminal velocity of rain | 842 | m0.2 s−1 |
| c | constant for terminal velocity of snow | 4.836 | m0.75 s−1 |
| e | constant for terminal velocity of cloud water | 3 × 107 | m−1 s−1 |
| Avi | constant for terminal velocity of cloud ice | 3.249 | m2/3 s−1 |
| Avg | constant for terminal velocity of graupel | 124 | M0.36 s−1 |
| Ami | constant in formula for mass of cloud ice | 0.01 | kg m−2 |
| p0 | standard air pressure | 105 | N m−2 |
| p | air pressure | N m−2 | |
| T0 | freezing point of water | 273.16 | K |
| T | air temperature | K | |
| Symbol | Description | Value | Units |
| rd0 | radius of initiated drizzle particles | 100 | µm |
| Edw | collection efficiency of drizzle for cloud water | 1 | |
| Edr | collection efficiency of rain for drizzle | 1 | |
| Edi | collection efficiency of drizzle for cloud ice | 0.1 | |
| Eds | collection efficiency of drizzle for snow | 0.8 | |
| Egd | collection efficiency of graupel for drizzle | 0.8 | |
| Efd | collection efficiency of frozen raindrops for drizzle | 0.8 | |
| Ehd | collection efficiency of hail for drizzle | 0.8 | |
| A’ | constant in formula for immersion freezing | 0.66 | K−1 |
| B’ | constant in formula for immersion freezing | 100 | m−3 s−1 |
| g | gravity acceleration | 9.8 | m s−2 |
| CD | drag coefficient for hail | 0.6 | |
| Fk | (Lv/(RvT) − 1)Lvρw/(KaT) | m−2 s | |
| Fd | ρRvT/(eswDv) | m−2 s | |
| υ | kinematic viscosity of air | m2 s−1 | |
| Dv | diffusivity of water vapour in air | m2 s−1 | |
| Ka | thermal conductivity of air | W m−1 K−1 | |
| Lv | latent heat of evaporation | 2.5 × 106 | J kg−1 |
| esw | pressure of saturated water vapour | N m−2 |
References
- Leroy, D.; Wobrock, W.; Flossmann, A.I. The role of boundary layer aerosol particles for the development of deep convective clouds: A high-resolution 3D model with detailed (bin) microphysics applies to CRYSTAL-FACE. Atmos. Res. 2009, 91, 62–78. [Google Scholar] [CrossRef]
- Khain, A.; Rosenfeld, D.; Pokrovsky, A.; Blahak, U.; Ryzhkov, A. The role of CCN in precipitation and hail in a mid-latitude storm as seen in simulations using a spectral (bin) microphysics model in a 2D dynamic frame. Atmos. Res. 2011, 99, 129–146. [Google Scholar] [CrossRef]
- Phillips, V.T.J.; Donner, L.J.; Garner, S.T. Nucleation processes in deep convection simulated by a cloud-resolving model with double-moment bulk microphysics. J. Atmos. Sci. 2007, 64, 738–761. [Google Scholar] [CrossRef]
- Milbrandt, J.A.; Morrison, H. Prediction of graupel density in a bulk microphysics scheme. J. Atmos. Sci. 2013, 70, 410–429. [Google Scholar] [CrossRef]
- Loftus, A.M.; Cotton, W.R.; Carrió, G.G. A triple-moment hail bulk microphysics scheme. Part I: Description and initial evaluation. Atmos. Res. 2014, 149, 35–57. [Google Scholar] [CrossRef]
- Ćurić, M.; Janc, D.; Vučković, V.; Kovačević, N. The impact of the choice of the entire drop size distribution function on Cumulonimbus characteristics. Meteorol. Z. 2009, 18, 207–222. [Google Scholar] [CrossRef]
- Kovačević, N.; Ćurić, M. Influence of drop size distribution function on simulated ground precipitation for different cloud droplet number concentrations. Atmos. Res. 2015, 158–159, 36–49. [Google Scholar] [CrossRef]
- Berry, E.X.; Reinhardt, R.L. An analysis of cloud drop growth by collection: Part II. Single initial distributions. J. Atmos. Sci. 1974, 31, 1825–1831. [Google Scholar] [CrossRef]
- Meyers, M.P.; Walko, R.L.; Harrington, J.Y.; Cotton, W.R. New RAMS cloud microphysics parameterization. Part II: The two-moment scheme. Atmos. Res. 1997, 45, 3–39. [Google Scholar] [CrossRef]
- Berry, E.X. Cloud droplet growth by collection. J. Atmos. Sci. 1967, 24, 688–701. [Google Scholar] [CrossRef]
- Kessler, E. On the distribution and continuity of water substance in atmospheric circulation. Meteorol. Monogr. 1969, 10, 88. [Google Scholar]
- Glickman, T.S. Glossary of Meteorology, 2nd ed.; American Meteorological Society: New York, NY, USA, 2000; p. 855. [Google Scholar]
- Wang, J.; Geerts, B. Identifying drizzle within marine stratus with W-band radar reflectivity. Atmos. Res. 2003, 69, 1–27. [Google Scholar] [CrossRef]
- Van Zanten, M.C.; Stevens, B.; Vali, G.; Lenschow, D.H. Observations of drizzle in nocturnal marine stratocumulus. J. Atmos. Sci. 2005, 62, 88–106. [Google Scholar] [CrossRef]
- Min, L.; Gong, W.; Liu, G.; Min, Q. Understanding the synoptic variability of stratocumulus cloud liquid water path over the Southeastern Pacific. Meteorol. Atmos. Phys. 2015, 127, 625–634. [Google Scholar] [CrossRef]
- Frisch, A.S.; Fairall, C.W.; Snider, J.B. Measurement of stratus cloud and drizzle parameters in ASTEX with a K-band Doppler radar and a microwave radiometer. J. Atmos. Sci. 1995, 52, 2788–2799. [Google Scholar] [CrossRef]
- Feingold, G.; Stevens, B.; Cotton, W.R.; Frisch, A.S. The relationship between drop in–cloud residence time and drizzle production in numerically simulated stratocumulus clouds. J. Atmos. Sci. 1996, 53, 1108–1122. [Google Scholar] [CrossRef]
- Gerber, H. Microphysics of marine stratocumulus clouds with two drizzle modes. J. Atmos. Sci. 1996, 53, 1649–1662. [Google Scholar] [CrossRef]
- Sears-Collins, A.L.; Schultz, D.M.; Johns, R.H. Spatial and temporal variability of nonfreezing drizzle in the United States and Canada. J. Clim. 2006, 19, 3629–3639. [Google Scholar] [CrossRef]
- Khain, A.; Pinsky, M.; Magaritz, I.; Krasnov, O.; Russchenberg, H.W.J. Combined observational and model investigations of the Z–LWC relationship in stratocumulus clouds. J. Appl. Meteorol. Clim. 2008, 47, 591–606. [Google Scholar] [CrossRef]
- Magaritz-Ronen, L.; Pinsky, M.; Khain, A. Drizzle formation in stratocumulus clouds: Effects of turbulent mixing. Atmos. Chem. Phys. 2016, 6, 1849–1862. [Google Scholar] [CrossRef]
- Hudson, J.G.; Yum, S.S. Maritime-Continental drizzle contrasts in small cumuli. J. Atmos. Sci. 2001, 58, 915–926. [Google Scholar] [CrossRef]
- Pujol, O.; Georgis, J.-F.; Sauvageot, H. Influence of drizzle on Z–M relationships in warm clouds. Atmos. Res. 2007, 86, 297–314. [Google Scholar] [CrossRef]
- Gerber, H.; Frick, G. Drizzle rates and large sea-salt nuclei in small cumulus. J. Geophys. Res. 2012, 117, D01205. [Google Scholar] [CrossRef]
- Gilmore, M.S.; Straka, J.M. The Berry and Reinhardt autoconversion parameterization: A digest. J. Appl. Meteorol. Clim. 2008, 47, 375–396. [Google Scholar] [CrossRef]
- Ćurić, M.; Janc, D.; Vučković, V. On the sensitivity of cloud microphysics under influence of cloud drop size distribution. Atmos. Res. 1998, 47–48, 1–14. [Google Scholar] [CrossRef]
- Kovačević, N.; Ćurić, M. The impact of the hailstone embryos on simulated surface precipitation. Atmos. Res. 2013, 132–133, 154–163. [Google Scholar] [CrossRef]
- Kovačević, N.; Ćurić, M. Sensitivity study of the influence of cloud droplet concentration on hail suppression effectiveness. Meteorol. Atmos. Phys. 2014, 123, 195–207. [Google Scholar] [CrossRef]
- Pruppacher, H.R.; Klett, J.D. Microphysics of Clouds and Precipitation, 2nd ed.; Kluwer: Dordrecht, The Netherlands, 1997; p. 954. [Google Scholar]
- Hu, Z.; He, G. Numerical simulation of microphysical processes in cumulonimbus—Part I: Microphysical model. Acta Meteorol. Sin. 1988, 2, 471–489. [Google Scholar]
- Lin, Y.-L.; Farley, R.D.; Orville, H.D. Bulk parameterization of the snow field in a cloud model. J. Appl. Meteor. 1983, 22, 1065–1092. [Google Scholar] [CrossRef]
- Murakami, M. Numerical modeling of dynamical and microphysical evolution of an isolated convective cloud—The 19 July 1981 CCOPE cloud. J. Meteorol. Soc. Jpn. 1990, 68, 107–127. [Google Scholar] [CrossRef]
- Wisner, C.; Orville, H.D.; Myers, C. A numerical model of a hail-bearing cloud. J. Atmos. Sci. 1972, 29, 1160–1181. [Google Scholar] [CrossRef]
- Twomey, S. The nuclei of natural cloud formation. Part II: The supersaturation in natural clouds and the variation of cloud droplet concentration. Geophys. Pure Appl. 1959, 43, 243–249. [Google Scholar] [CrossRef]
- Cohard, J.-M.; Pinty, J.-P.; Bedos, C. Extending Twomey’s analytical estimate of nucleated cloud droplet concentrations from CCN spectra. J. Atmos. Sci. 1998, 55, 3348–3357. [Google Scholar] [CrossRef]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series and Products, 7th ed.; Academic Press: New York, NY, USA, 2007; p. 1171. [Google Scholar]
- Cohard, J.-M.; Pinty, J.-P. A comprehensive two-moment warm microphysical bulk scheme. I: Description and tests. Q. J. R. Meteorol. Soc. 2000, 126, 1815–1842. [Google Scholar] [CrossRef]
- Khairoutdinov, M.; Kogan, Y. A new cloud physics parameterization in a large-eddy simulation model of marine stratocumulus. Mon. Weather Rev. 2000, 128, 229–243. [Google Scholar] [CrossRef]




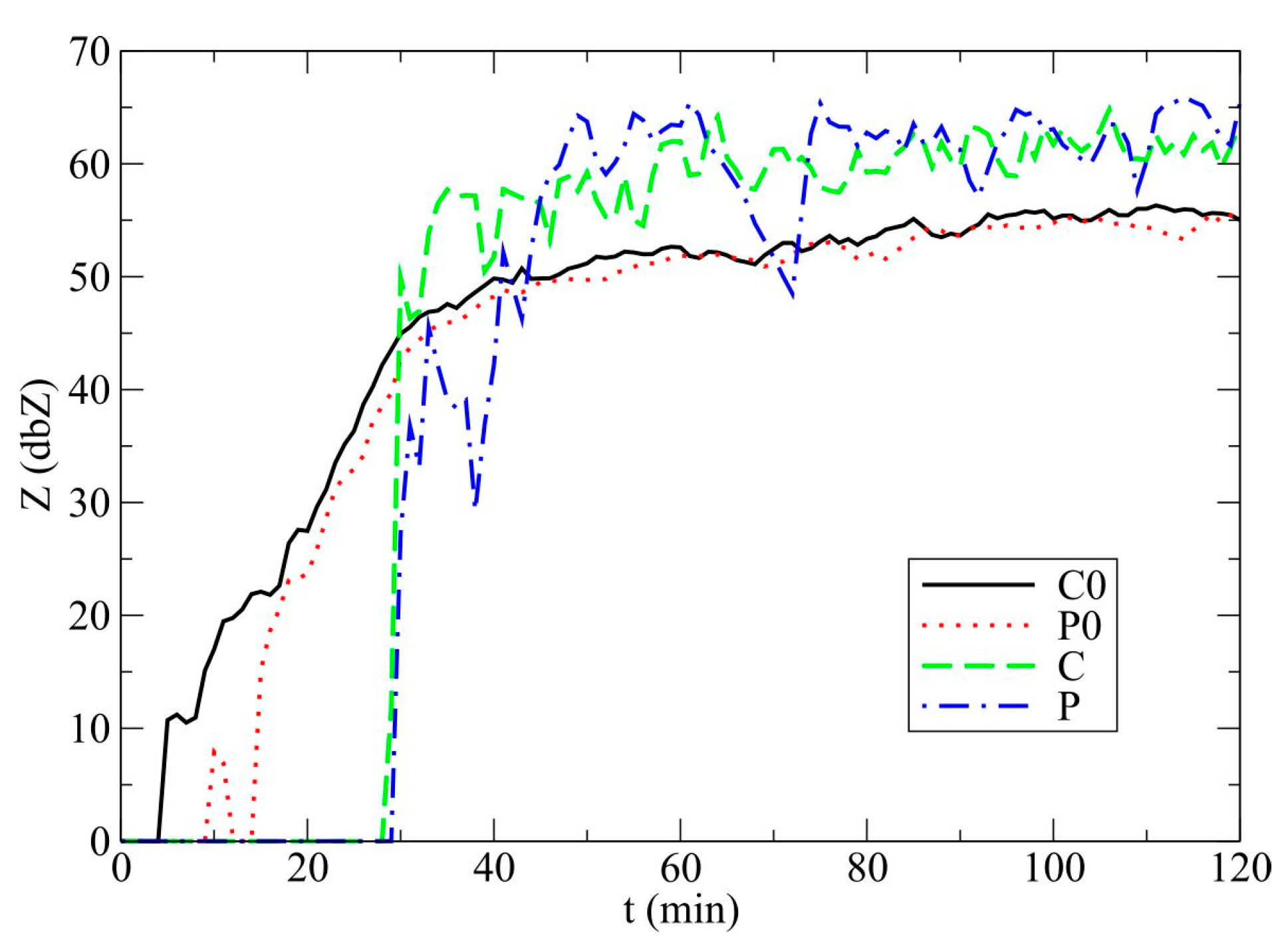
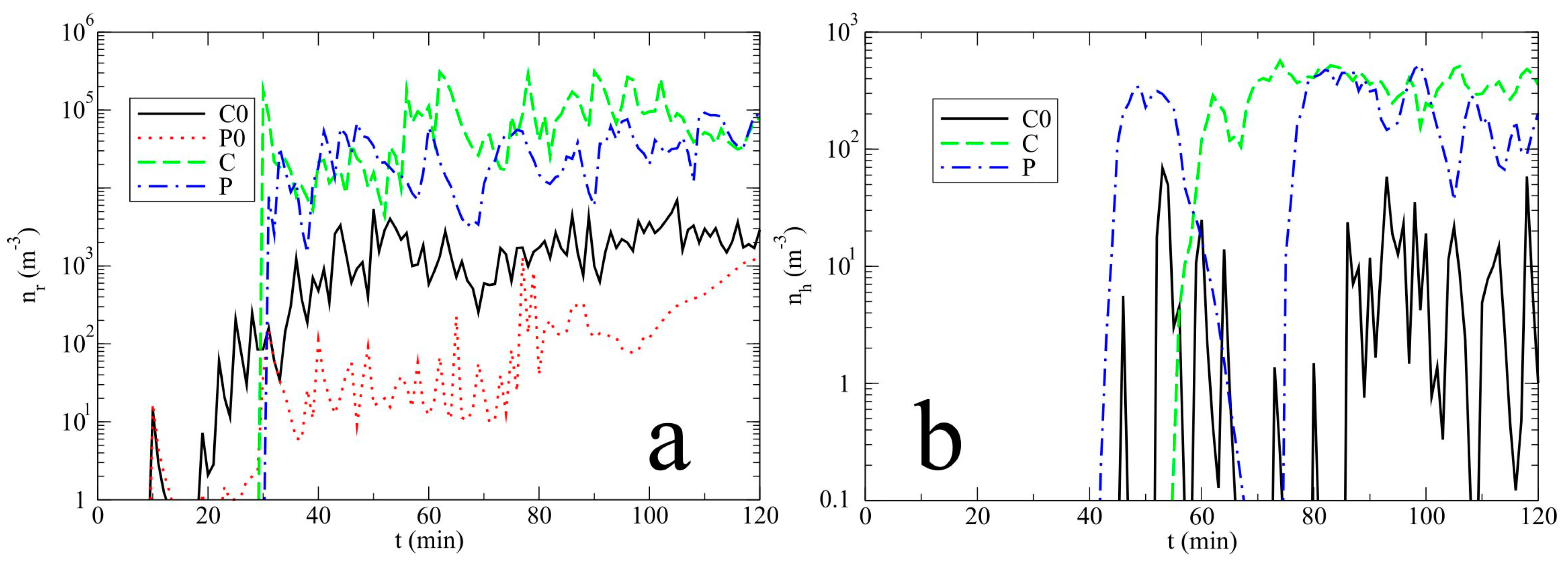
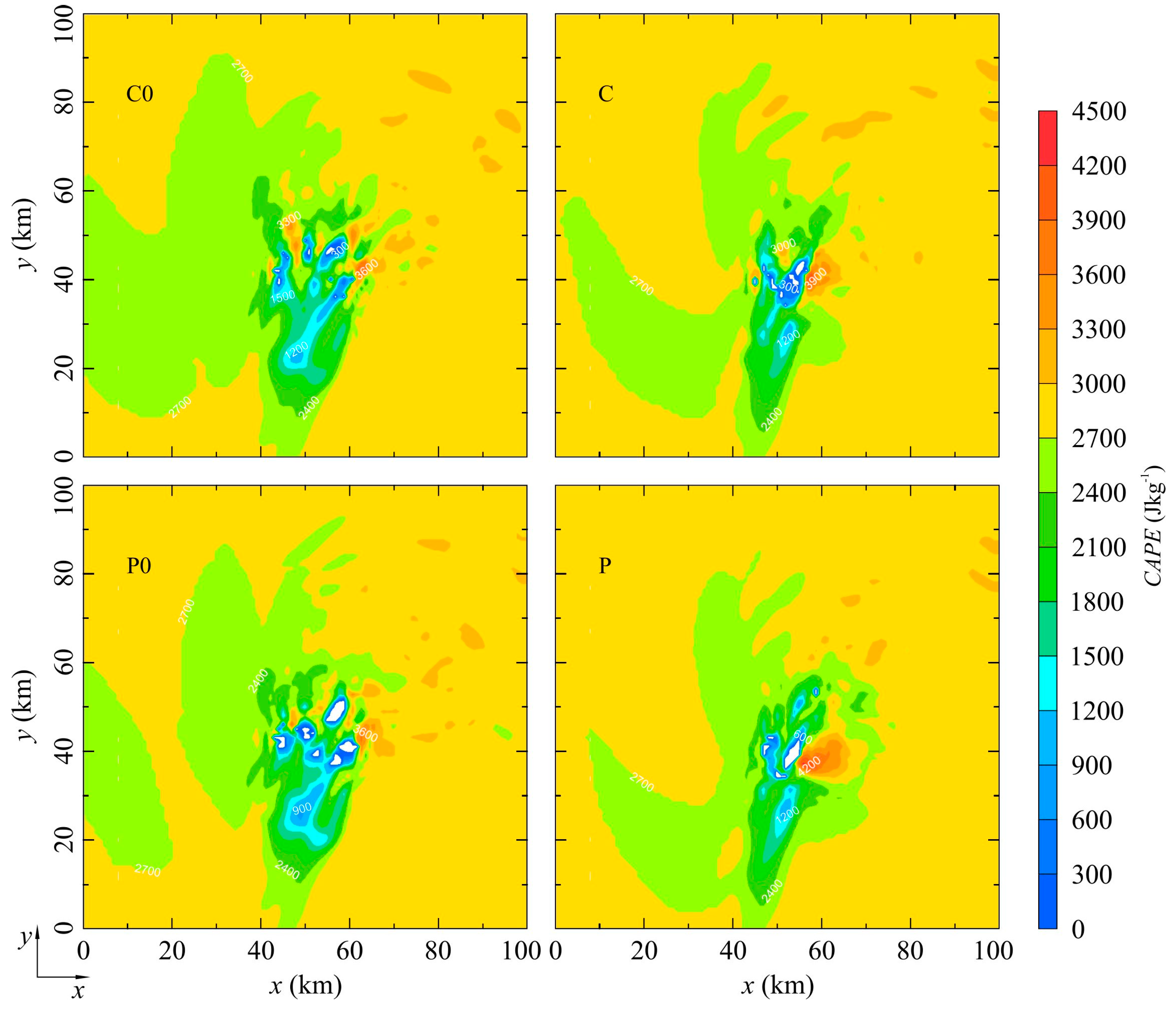
| Hydrometeors | Drizzle Case | Non-Drizzle Case | ||
|---|---|---|---|---|
| DXmin (mm) | DXmax (mm) | DXmin (mm) | DXmax (mm) | |
| Cloud droplets | 0.0 | 0.2 | 0.0 | 0.2 |
| Drizzle | 0.2 | 0.5 | ― | ― |
| Raindrops | 0.5 | 10.0 | 0.2 | 10.0 |
| Environment | C (cm−3) | k | β | µ |
|---|---|---|---|---|
| Clean air | 50.0 | 1.50 | 6.84 | 1.90 |
| Polluted air | 500.0 | 0.86 | 6.80 | 1.50 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kovačević, N.; Veljovic, K. Impact of Drizzle-Sized Cloud Particles on Production of Precipitation in Hailstorms: A Sensitivity Study. Atmosphere 2018, 9, 13. https://doi.org/10.3390/atmos9010013
Kovačević N, Veljovic K. Impact of Drizzle-Sized Cloud Particles on Production of Precipitation in Hailstorms: A Sensitivity Study. Atmosphere. 2018; 9(1):13. https://doi.org/10.3390/atmos9010013
Chicago/Turabian StyleKovačević, Nemanja, and Katarina Veljovic. 2018. "Impact of Drizzle-Sized Cloud Particles on Production of Precipitation in Hailstorms: A Sensitivity Study" Atmosphere 9, no. 1: 13. https://doi.org/10.3390/atmos9010013
APA StyleKovačević, N., & Veljovic, K. (2018). Impact of Drizzle-Sized Cloud Particles on Production of Precipitation in Hailstorms: A Sensitivity Study. Atmosphere, 9(1), 13. https://doi.org/10.3390/atmos9010013





