Comparison of Five Modeling Approaches to Quantify and Estimate the Effect of Clouds on the Radiation Amplification Factor (RAF) for Solar Ultraviolet Radiation
Abstract
1. Introduction
1.1. Development of the EPA’s UVR Monitoring Network
- Improve understanding of the nature and intensity of UVR reaching the Earth’s surface.
- Characterize the physical and chemical parameters that modify UVR flux.
- Obtain better estimates of UVR exposures at multiple times, locations, meteorological conditions, altitudes, terrain characteristics, topologies, and air pollution conditions.
- Evaluate human and ecosystem/environmental exposure to surface UVR across the US.
- Assess the impact of changes in stratospheric ozone and tropospheric pollution on biologically damaging UV radiation in the erythemal action spectrum.
- Assess the effectiveness of control strategies (e.g., Montreal Protocol) designed to reduce the amount of greenhouse gases in the stratosphere, and increase the amount of stratospheric ozone.
- Serve as a component of the EPA’s National Environmental Monitoring Strategy.
1.2. Importance of UVR Monitoring
1.3. Stratospheric Ozone
2. Materials and Methods
2.1. Measurement of the Ultraviolet (UV) Spectrum
2.2. UVR Monitoring Instruments
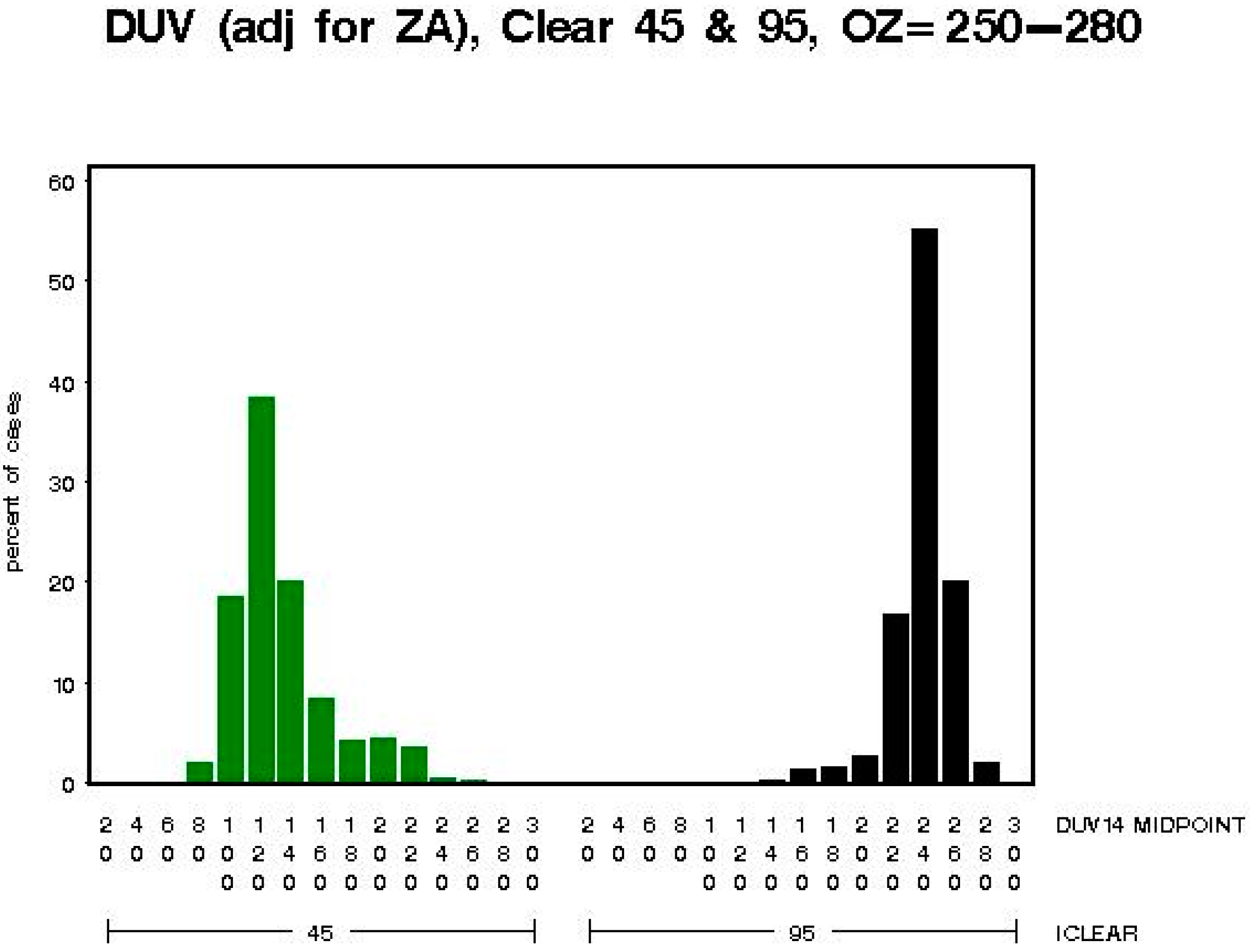
2.3. Determining Cloudiness in a Consistent Manner
2.4. Variability in Biologically Damaging UV Radiation Data
2.5. Relationship between Biologically Damaging UV Radiation, Cloudiness, Column Ozone, and RAF
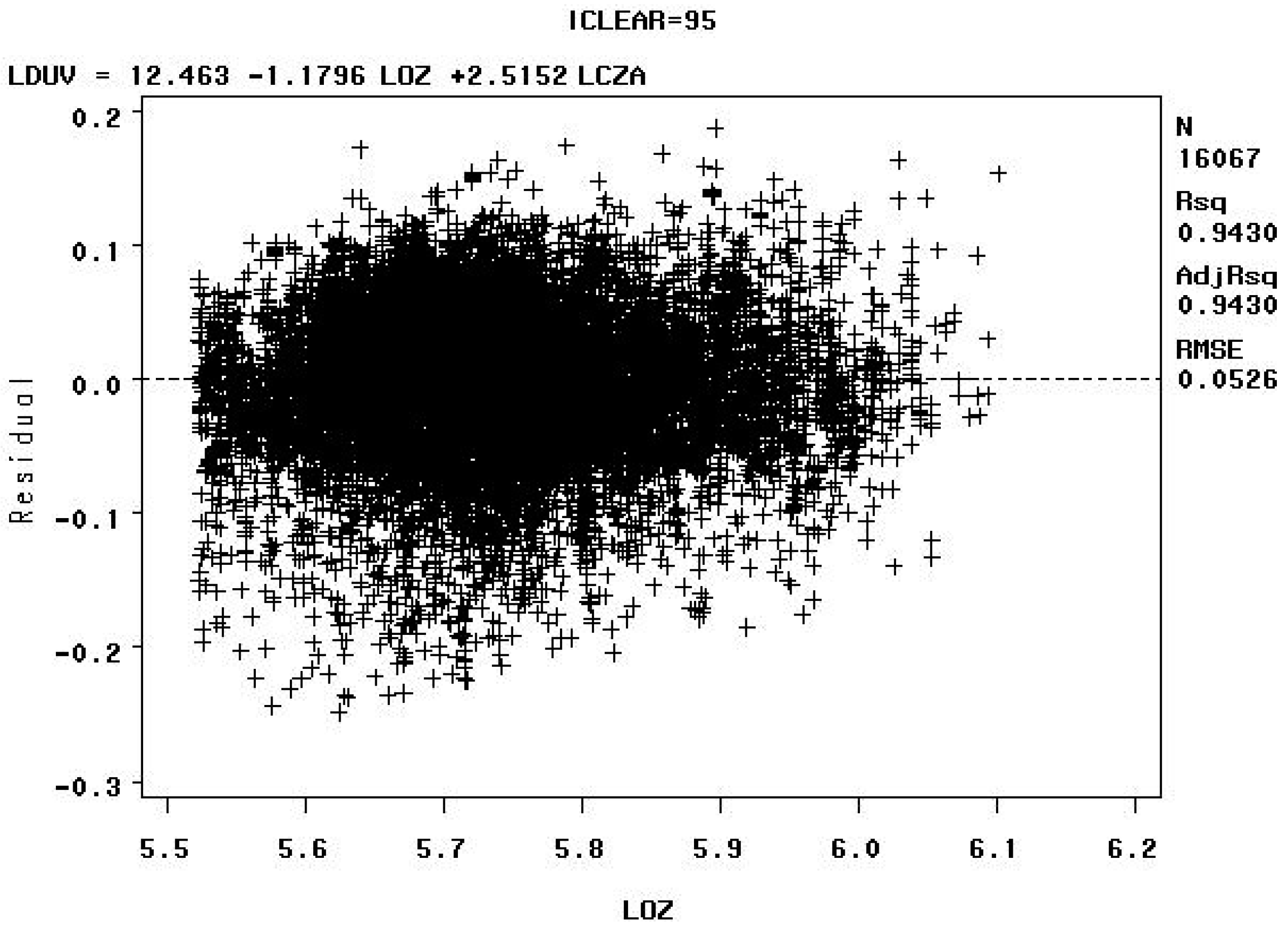
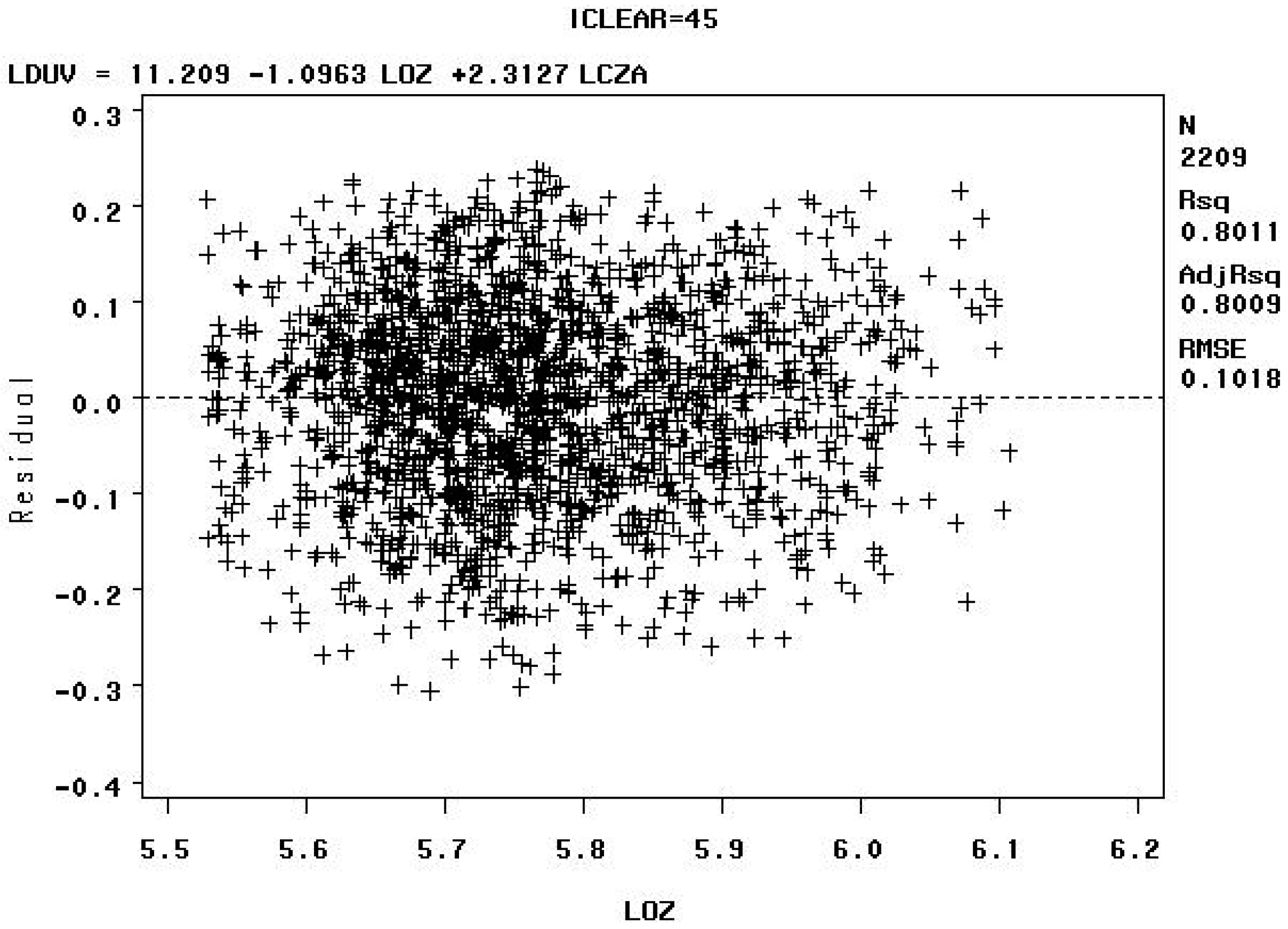
2.6. Experimental Approach Used to Determine Radiation Amplification Factor (RAF)
2.7. Ideal Experiment
- Measure the total column ozone [O3] levels (using either TOMS satellite measurements or ground measurements with spectrophotometers as applicable, etc.) at each fixed SZA.
- Measure the biologically damaging UV radiation levels (using ground measurements with spectrophotometers, etc.) at each fixed SZA at measured:
- Percent clearness = 0% [100% cloud cover] to Percent clearness = 100% [0% cloud cover]
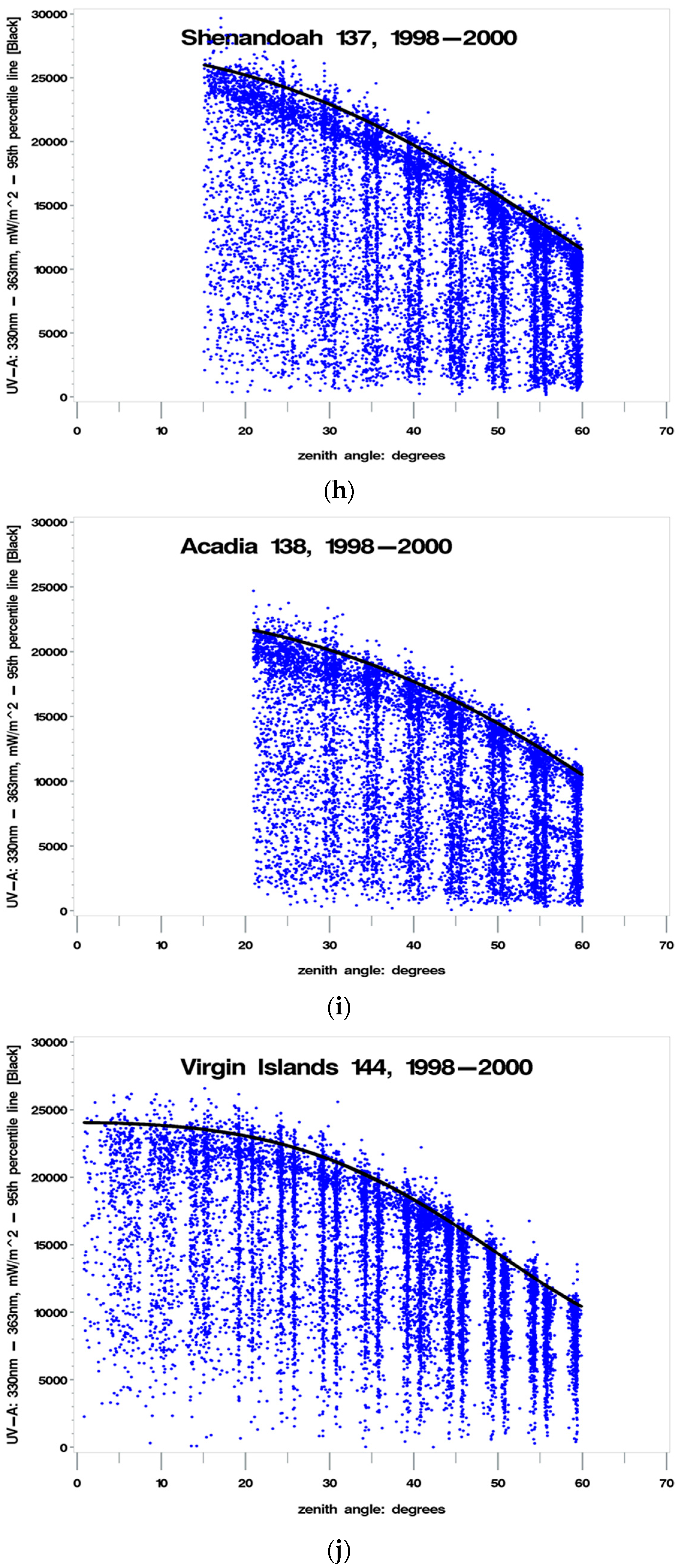
- Percent clearness = 100% to 120% [accounting for cloud “reflections”]—Note: enhancement of UVR by cloud reflections has been measured at up to 30 percent [20], therefore using a 20% enhancement (120%) for maximum biologically damaging UV radiation values provides a reasonable and conservative estimate where the percent clearness (cloudiness) would be measured by satellites and/or from ground-based instruments. Total column ozone was not measured using instruments at the sites, and cloudiness could not be assessed independent of human observation. The instruments could not (and cannot) measure biologically damaging UV radiation at fixed SZAs for extended periods, therefore the ideal experimental setup had to be adjusted to conform to the limitations of real-world constraints. When developing a preliminary methodology for implementing this hypothesis, an examination of the initial set of UV325 versus SZA plots that were generated by the statistical software indicated that “clear” was best defined as a SZA-dependent polynomial approximation of the 95th percentile of the UV325 measurements. This polynomial approximation was determined to be satisfactory, since it captured almost all of the upper values, and allowed some values to exceed clear by at least 20 percent, possibly due to cloud reflections, as noted in the literature [20].
2.8. Realizable Experiment (Driven by Real-World Constraints)
2.9. A Theoretical Definition of Cloudiness (Percent Clearness)
2.10. Measured and Calculated Parameters
- The UVR calculations were normalized with respect to the distance between the sun and the Earth during the year. Note: Earth is closest to sun on 5 January. The formula used to calculate the normalized UVR values is:where jday = 1, 2, …, 365, with jday = 5 being 5 January, [21].UVR = {1−0.034 cos(2π(jday−5)/365))} × Biologically Damaging UV Radiation
- The UVR values were normalized with respect to systematic differences due to site altitude, topography, pollution, aerosol, haze, etc.
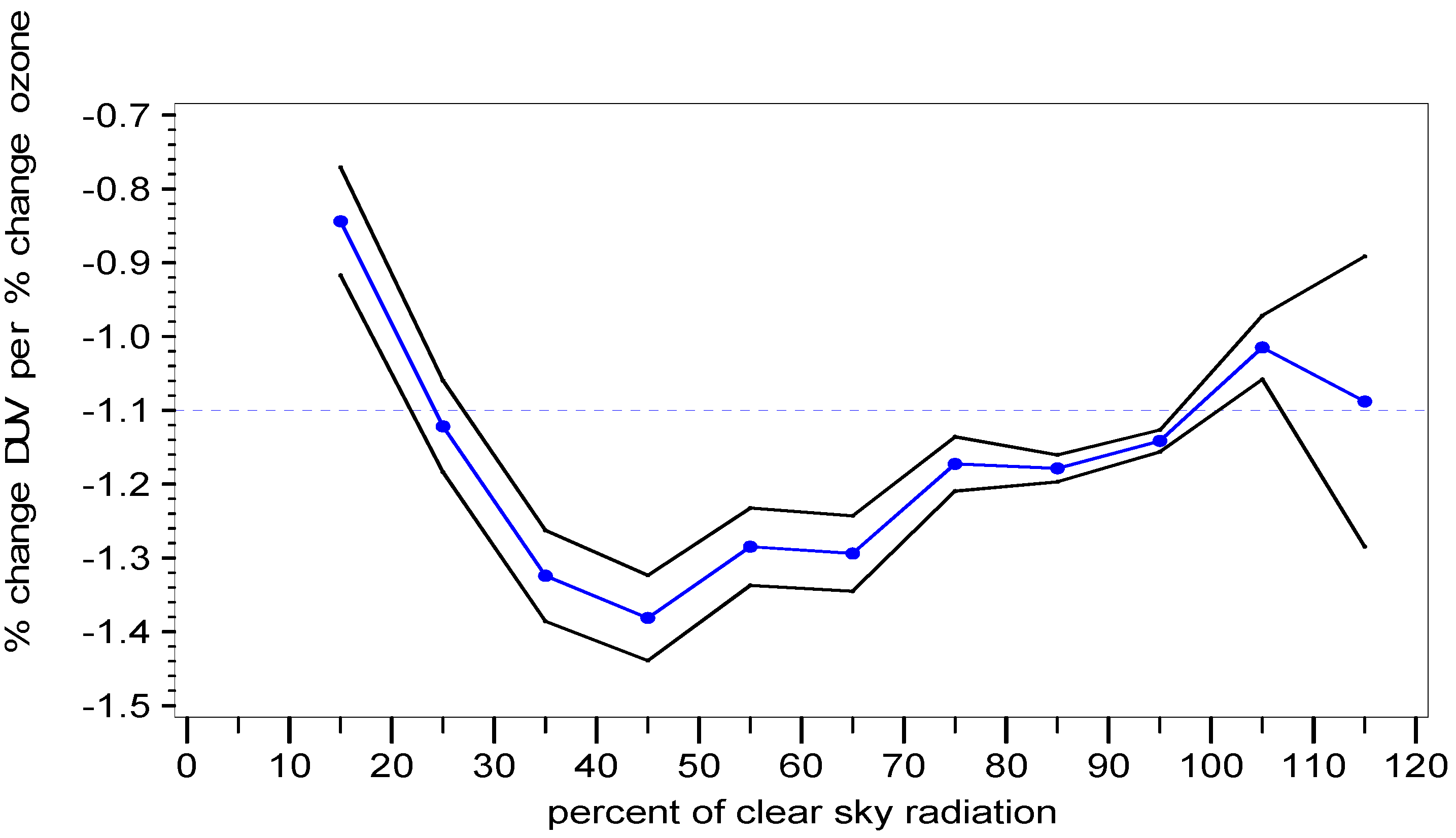
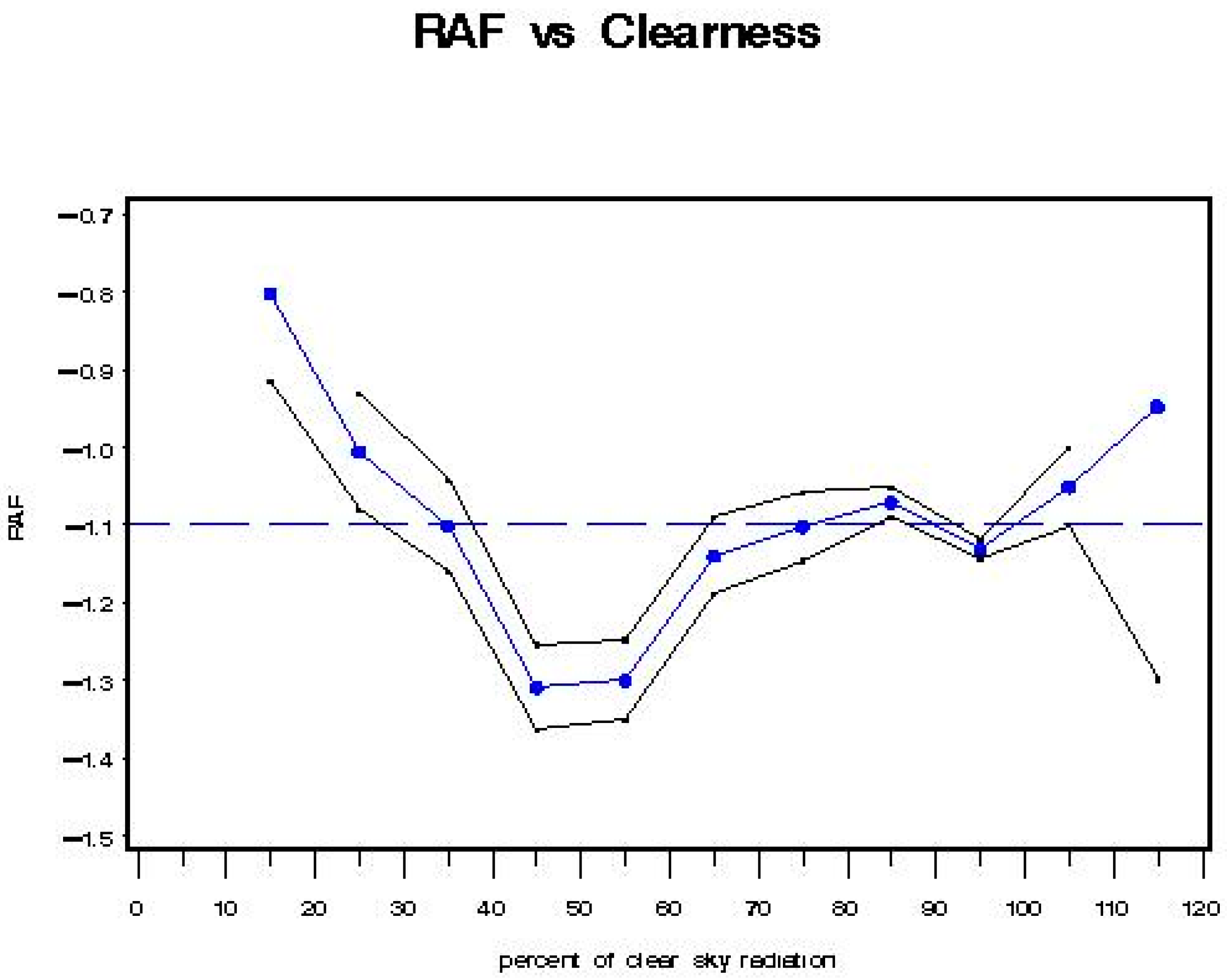
- The data from all ten sites was combined into curves of RAF versus percent clear sky for each of the five modeling approaches, Model A through Model E, (as shown in Figures 6, 7, 13, 16, and 17 respectively).
3. Results
3.1. Experimental Determination of the RAF: Five Modeling Approaches
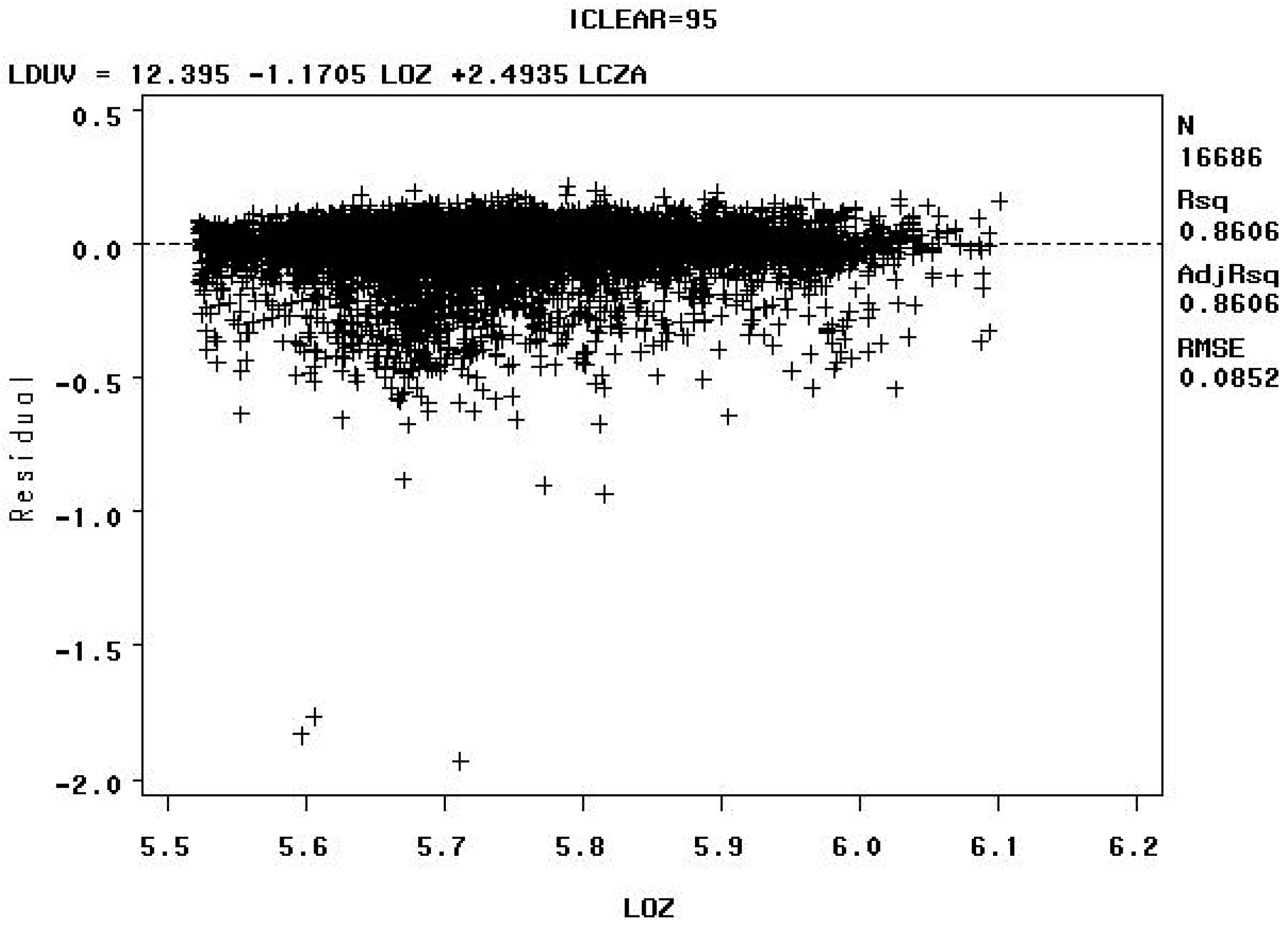
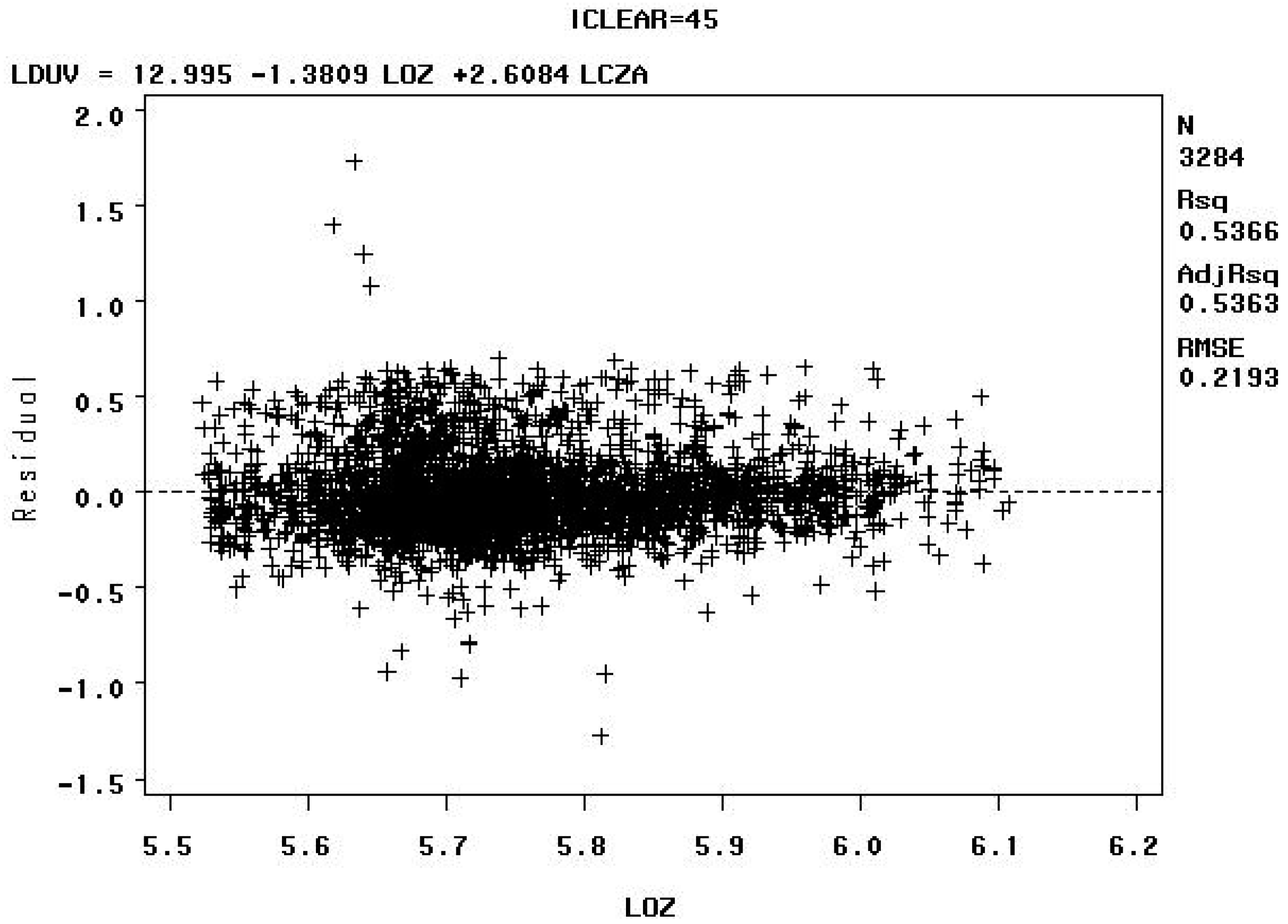
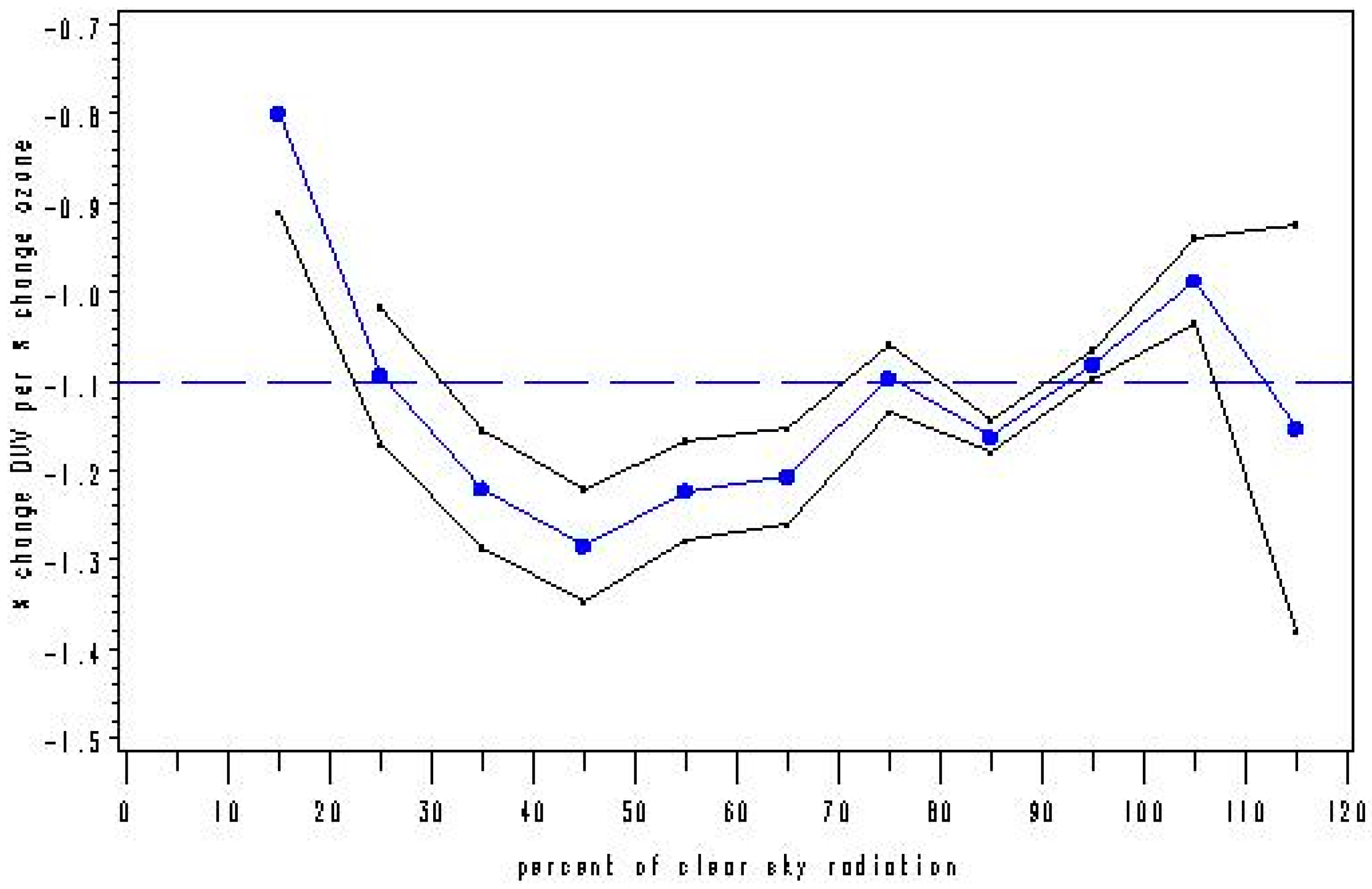
3.1.1. Model A
3.1.2. Model B
3.1.3. Model C
3.1.4. Model D
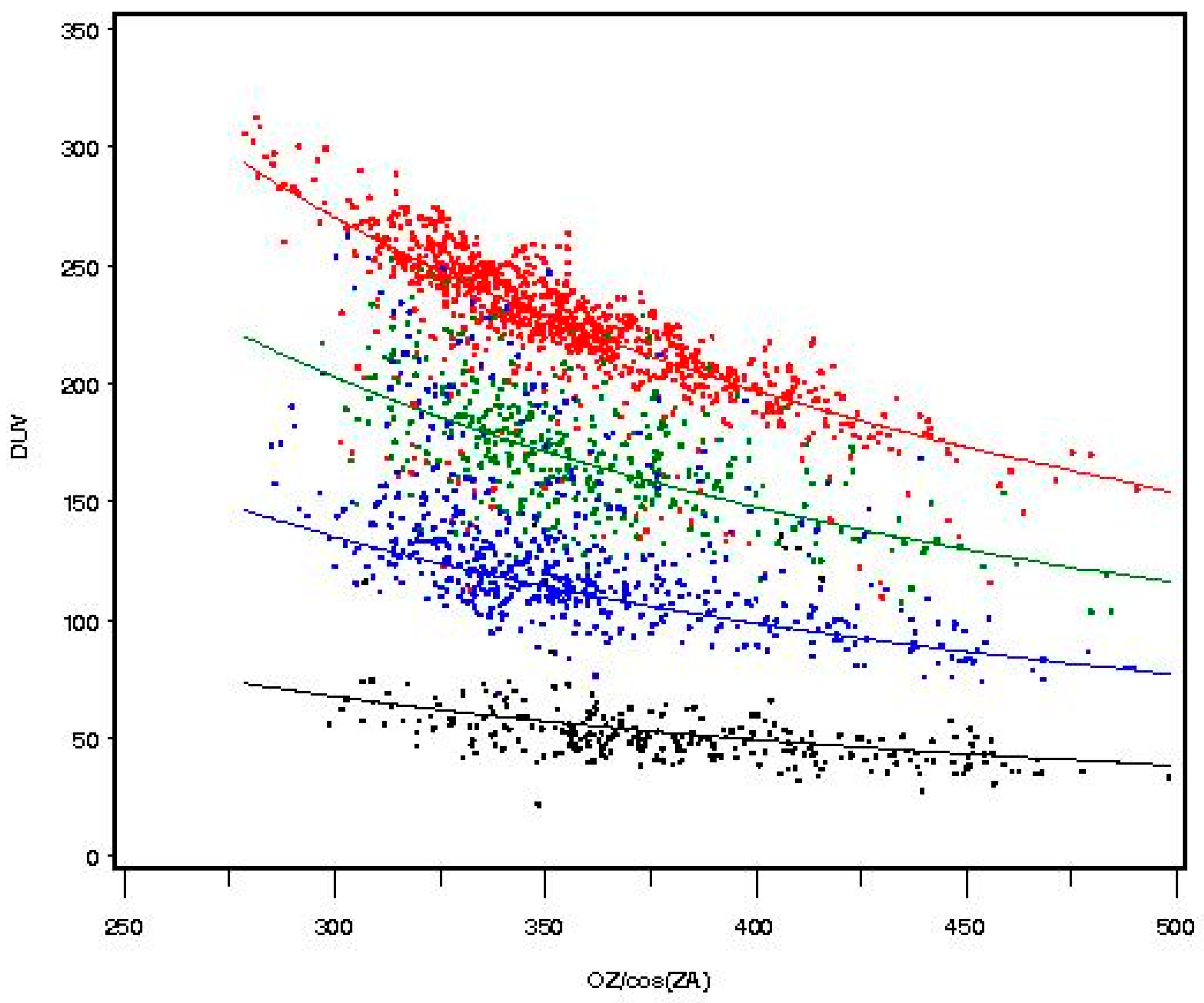
3.1.5. Model E—(A Model Based on an Empirical Relationship: Beer–Lambert’s Law)
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| CCD | charge-coupled device |
| CFC | chlorofluorocarbon |
| CLR | percent clear sky radiation |
| DOI | US Department of the Interior |
| DOS | disk operating system |
| DU | Dobson Unit |
| DUV | biologically damaging UV radiation |
| EPA | US Environmental Protection Agency |
| GMT | Greenwich Mean Time |
| IAG | Interagency Agreement |
| LCZA | natural logarithm of the cosine of the solar zenith angle |
| LDUV | natural logarithm of the biologically damaging UV radiation |
| LOZ | natural logarithm of the total column ozone |
| PCLR | fraction of clear sky radiation |
| NASA | National Aeronautics and Space Administration |
| NERL | EPA National Exposure Research Laboratory |
| NIST | US National Institute for Standards and Technology |
| NOAA | National Oceanographic and Atmospheric Administration |
| NUVMC | National UV Monitoring Center |
| NPS | US National Parks Service |
| O3 | total column ozone |
| ORD | EPA Office of Research and Development |
| RAF | Radiation Amplification Factor |
| SZA | Solar Zenith Angle |
| TOMS | NASA Total Ozone Mapping Spectrometer |
| UGA | University of Georgia at Athens |
| US | United States |
| UV | ultraviolet |
| UVR | ultraviolet radiation |
References
- Gerstl, S.A.W.; Zardecki, A.; Wiser, H.L. Biologically damaging radiation amplified by ozone depletions. Nature 1981, 294, 352–354. [Google Scholar] [CrossRef]
- Smertenko, P.; Stepanov, V.; Ol’khovik, C.; Durzan, D.J. New approach to a radiation amplification factor. In Cell Biology and Instrumentation: UV Radiation, Nitric Oxide, and Cell Death in Plants; Blume, Y., Durzan, D.J., Smertenko, P., Eds.; IOS Press: Amsterdam, The Netherlands, 2006; ISBN 1-58603-574-6. [Google Scholar]
- Parisi, A.V.; Sabburg, J.; Kimlin, M.G. Scattered and Filtered Solar UV Measurements; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2004. [Google Scholar]
- Madronich, S.; McKenzie, R.L.; Bjorn, L.O.; Caldwell, M.M. Changes in biologically active ultraviolet radiation reaching the Earth’s surface. J. Photochem. Photobiol. B 1998, 46, 5–19. [Google Scholar] [CrossRef]
- Pfister, G.; McKenzie, R.L.; Liley, J.B.; Thomas, A.; Forgan, B.W.; Long, C.N. Cloud coverage based on all-sky imaging and its impact on surface solar irradiance. J. Appl. Meteorol. Clim. 2003, 42, 1421–1434. [Google Scholar] [CrossRef]
- Hall, E.S. Ground-Based Measurement of Solar Ultraviolet Radiation. In McGraw-Hill Yearbook of Science and Technology; Kotowski, F., Jr., Ed.; McGraw-Hill Book Co.: New York, NY, USA, 2009; pp. 150–155. [Google Scholar]
- Sabburg, J. EPA/UGA, UV/QA Data Correction Project Specification (v2.3), Unpublished work. 2001.
- Weatherhead, E.C.; Frederick, J.E. Report on Geographic and Seasonal Variability of UV Affecting Human and Ecological Health. Available online: https://cfpub.epa.gov/si/si_public_record_Report.cfm?dirEntryID=133325 (accessed on 17 August 2017).
- Lenoble, J. Atmospheric Radiation Transfer; A. Deepak Publishing: Hampton, VA, USA, 1993. [Google Scholar]
- Warhaft, Z. An Introduction to Thermal-Fluid Engineering: The Engine and the Atmosphere; Cambridge University Press: Cambridge, UK, 1997; ISBN 0-521-58100-1. [Google Scholar]
- Frederick, J.E.; Snell, H.E.; Haywood, E.K. Solar ultraviolet radiation at the earth’s surface. Photochem. Photobiol. 1989, 50, 443–450. [Google Scholar] [CrossRef]
- Crist, K.C.; Carmichael, G.R.; John, K. UV-B exposure and atmospheric ozone: Evaluation of radiative flux to changes in ambient ozone levels. J. Hazard. Mater. 1994, 37, 527–538. [Google Scholar] [CrossRef]
- Sivasakthivel, T.; Reddy, K.S.K. Ozone Layer Depletion and Its Effects: A Review. Available online: http://www.ijesd.org/papers/93-D500.pdf (accessed on 17 August 2017).
- Rowland, F.S. Stratospheric Ozone Depletion. Philos. T. Roy. Soc. B 2006, 361, 769–790. [Google Scholar] [CrossRef] [PubMed]
- McCulloch, A.; Midgley, P.M.; Ashford, P. Releases of refrigerant gases (CFC-12, HCFC-22, and HFC-134a) to the atmosphere. Atmos. Environ. 2003, 37, 889–902. [Google Scholar] [CrossRef]
- United Nations Environment Programme (UNEP). The 1987 Montreal Protocol on Substances that Deplete the Ozone Layer. Available online: http://ozone.unep.org/new_site/en/Treaties/treaties_decisions-hb.php?sec_id=342&show_all (accessed on 17 August 2017).
- US Environmental Protection Agency (US EPA). Clean Air Act Title VI—Stratospheric Ozone Protection. 42 USC 7671 (Title 42 [The Public Health and Welfare], Chapter 85 [Air Pollution Prevention and Control], Subchapter VI [Stratospheric Ozone Protection]). Available online: https://www.epa.gov/clean-air-act-overview/clean-air-act-title-vi-stratospheric-ozone-protection (accessed on 4 August 2017).
- Brewer MKIV Spectrophotometer Operator’s Manual. Available online: https://www.esrl.noaa.gov/gmd/grad/neubrew/docs/manuals/Brewer_MkIV_service_manual.pdf (accessed on 17 August 2017).
- Binyamin, J.; Davies, J.; McArthur, B. Validation of spectral and broadband UV-B (290–325 nm) irradiance for Canada. Atmos. Clim. Sci. 2011, 1, 69–85. [Google Scholar] [CrossRef][Green Version]
- Feister, U.; Cabrol, N.; Häder, D. UV enhancements by scattering of solar radiation from clouds. Atmosphere 2015, 6, 1211–1228. [Google Scholar] [CrossRef]
- ITACA. The Sun as a Source of Energy, Part 4: Irradiation Calculations. Available online: http://www.itacanet.org/the-sun-as-a-source-of-energy/part-4-irradiation-calculations/ (accessed on 17 August 2017).
- El-Nouby, A.M. Effect of stratospheric ozone in UVB solar radiation reaching the Earth’s surface at Qena, Egypt. Atmos. Pollut. Res. 2010, 1, 155–160. [Google Scholar] [CrossRef]
- Varotsos, C. Airborne measurements of aerosol, ozone, and solar ultraviolet irradiance in the troposphere. J. Geophys. Res. Atmos. 2005, 110, 2156–2202. [Google Scholar] [CrossRef]
- Mckenzie, R.L.; Bjorn, L.O.; Bais, A.; Ilyas, M. Changes in biologically active ultraviolet radiation reaching the Earth’s surface. Photochem. Photobiol. Sci. 2003, 2, 5–15. [Google Scholar] [CrossRef] [PubMed]
- McKenzie, R.L.; Aucamp, P.J.; Bais, A.F.; Björn, L.O.; Ilyas, M. Changes in biologically active ultraviolet radiation reaching the Earth’s surface. Photochem. Photobiol. Sci. 2007, 6, 218–231. [Google Scholar] [CrossRef] [PubMed]
- Ziemke, J.R.; Chandra, S.; Herman, J.; Varotsos, C. Erythemally weighted UV trends over northern latitudes derived from Nimbus 7 TOMS measurements. J. Geophys. Res. Atmos. 2000, 105, 7373–7382. [Google Scholar] [CrossRef]
- Massen, F. Computing the Radiation Amplification Factor RAF Using a Sudden Dip in Total Ozone Column Measured at Diekirch, Luxembourg. Available online: http://meteo.lcd.lu/papers/MASSEN/RAF_from_sudden_TOC_dip.pdf (accessed on 17 August 2017).
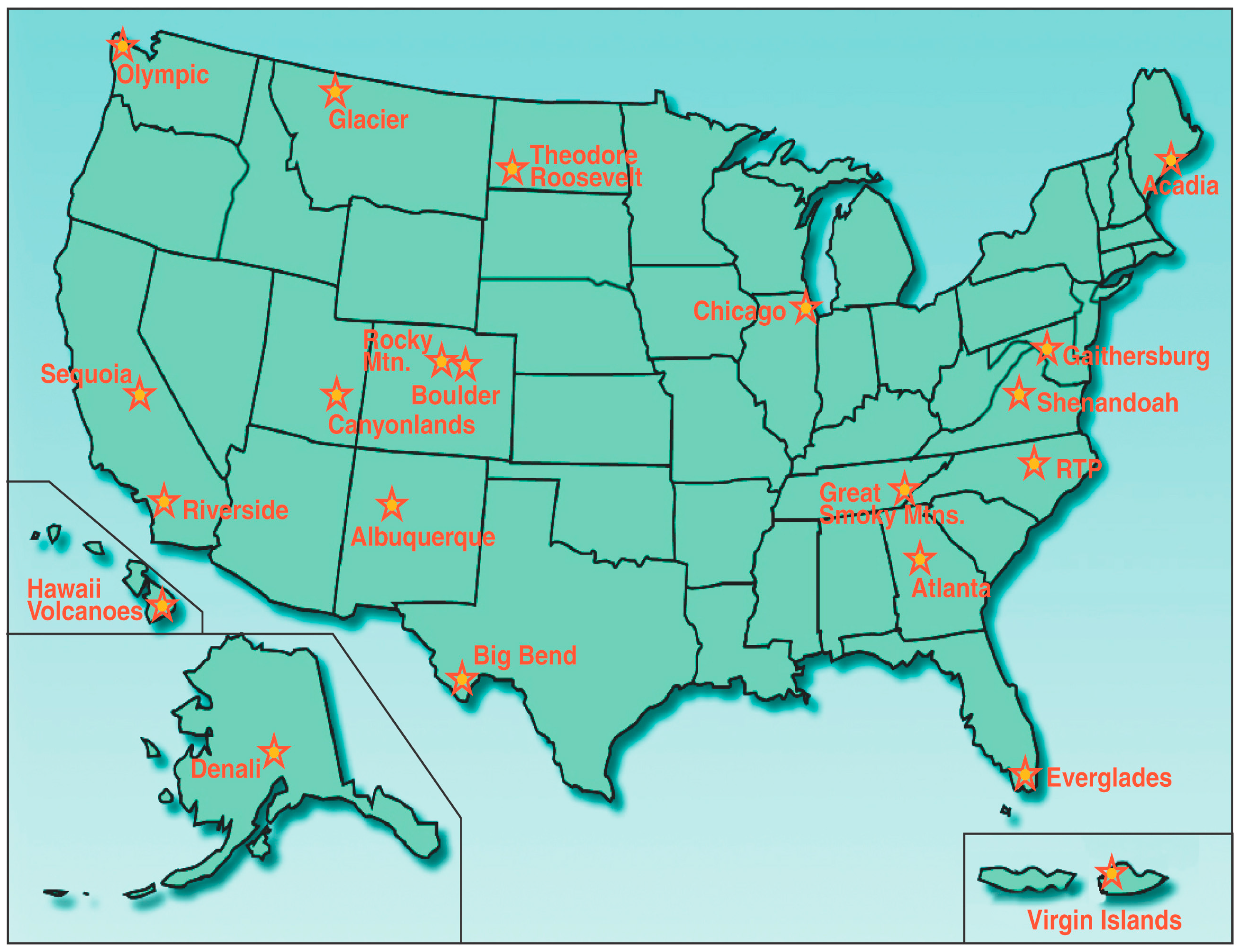


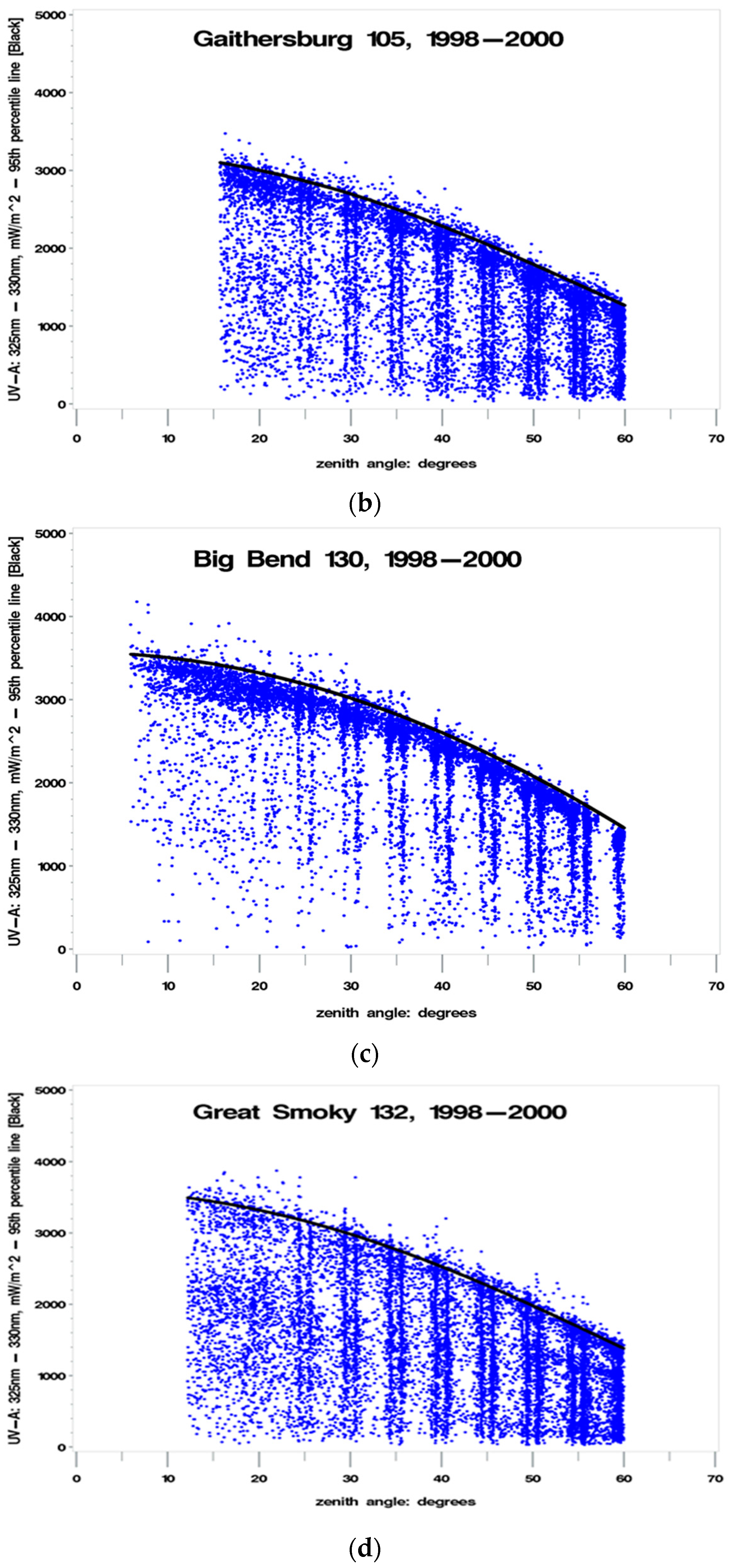
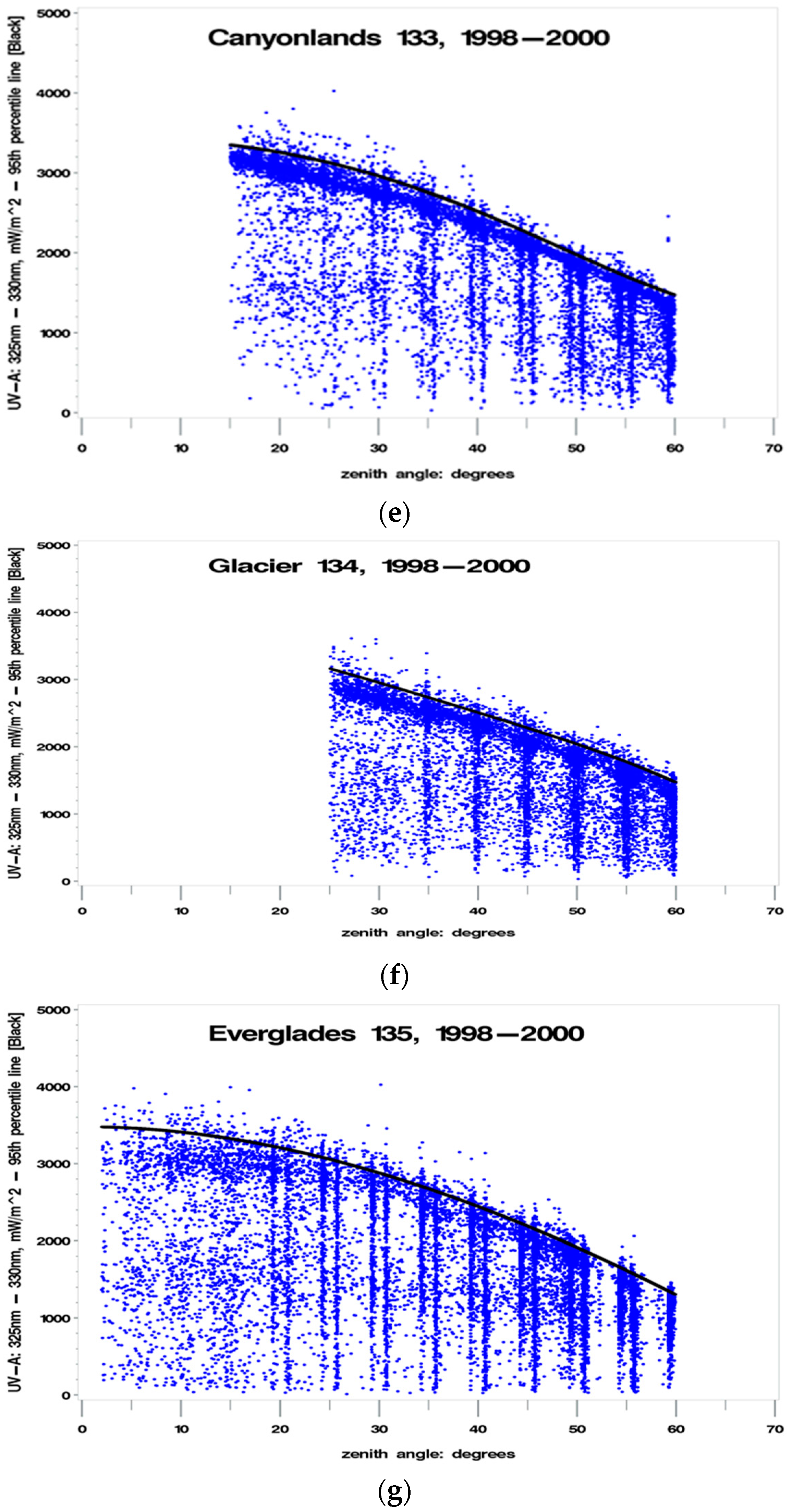
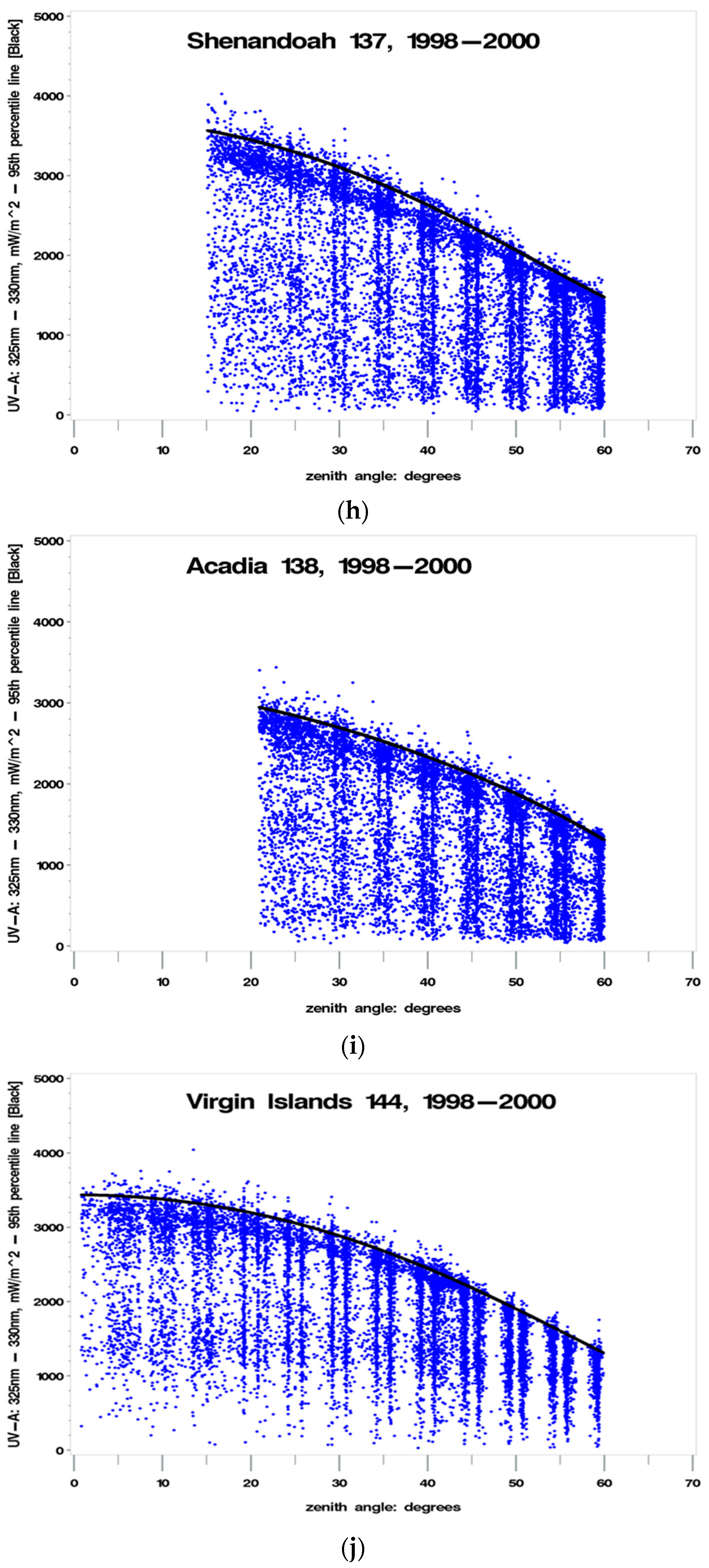
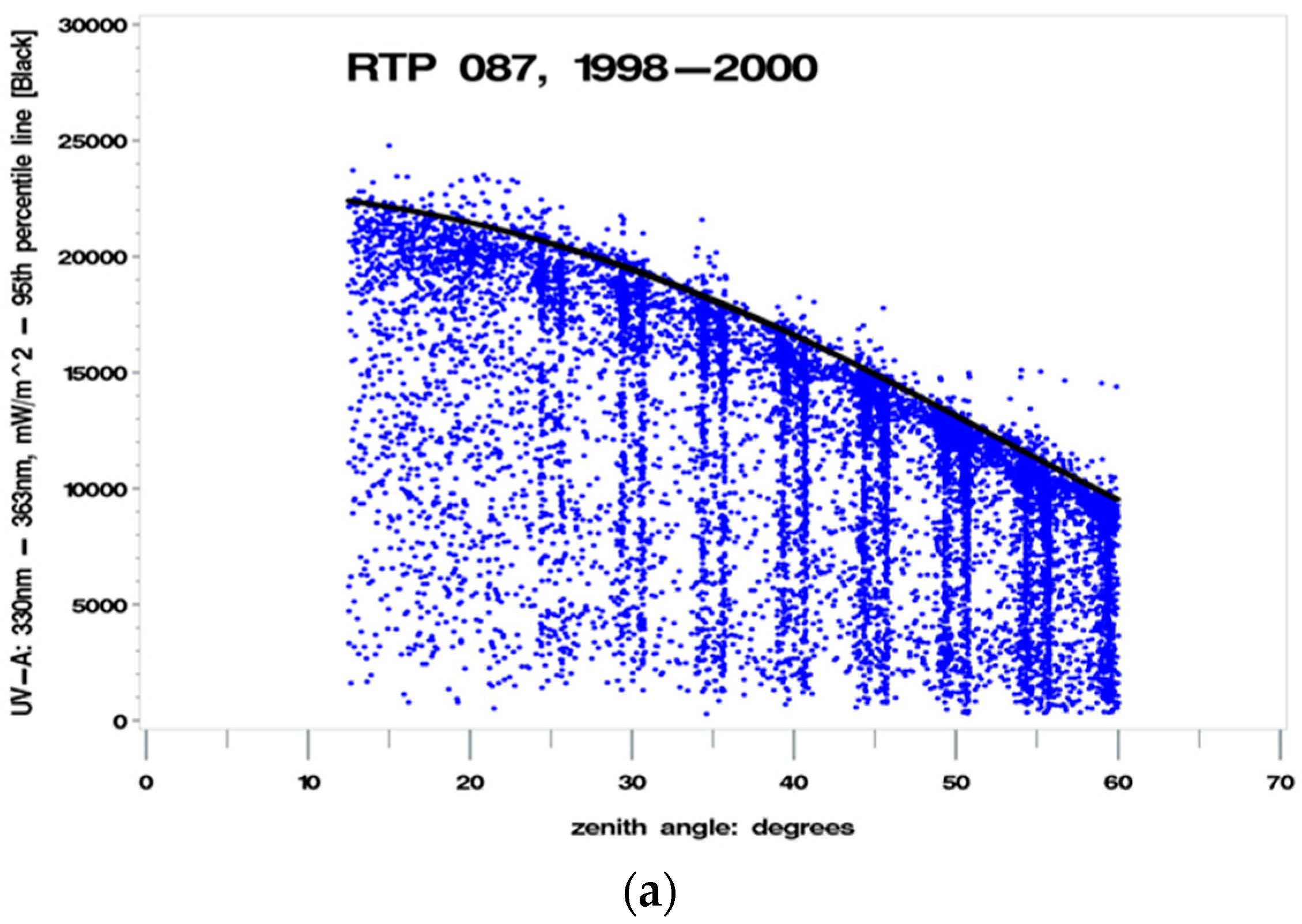
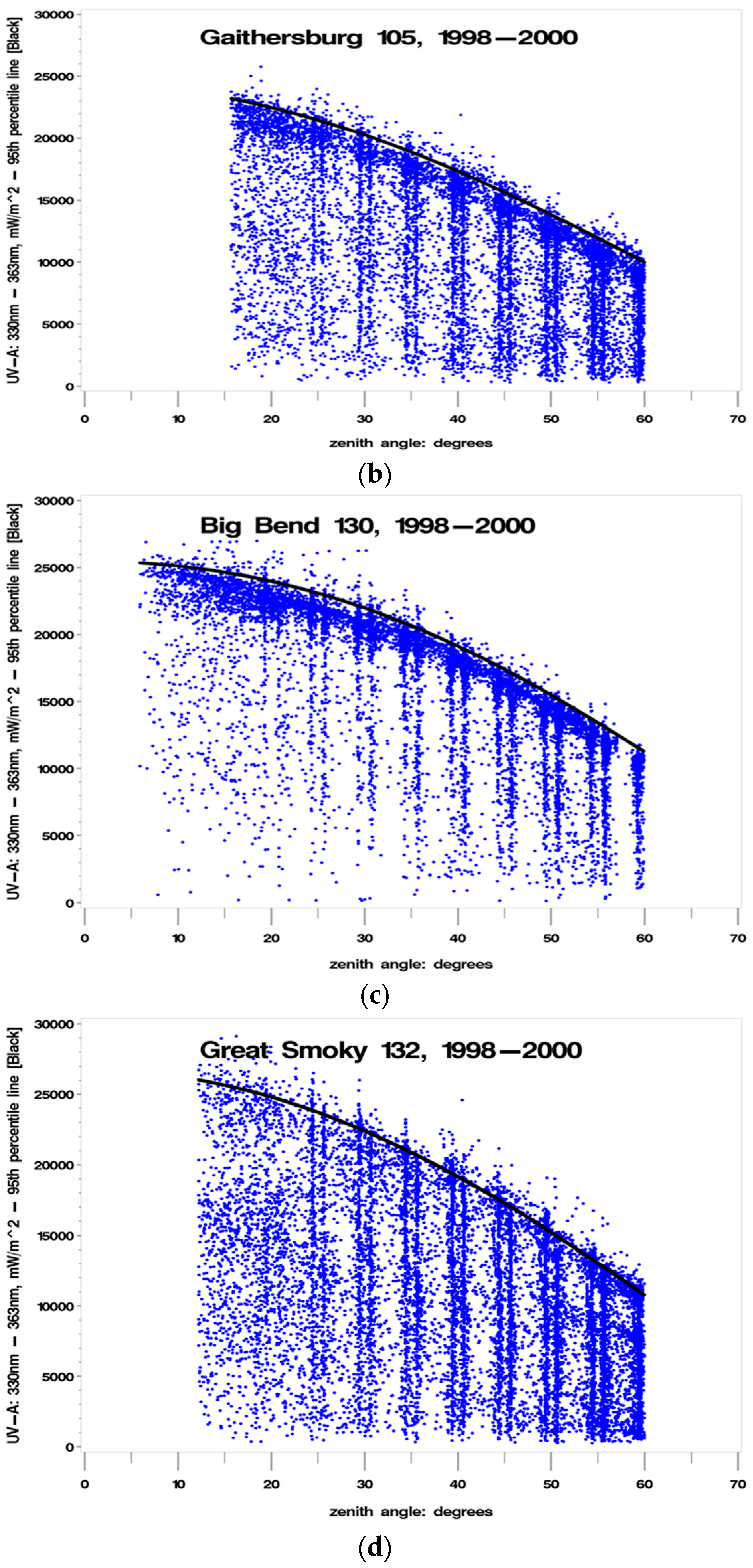
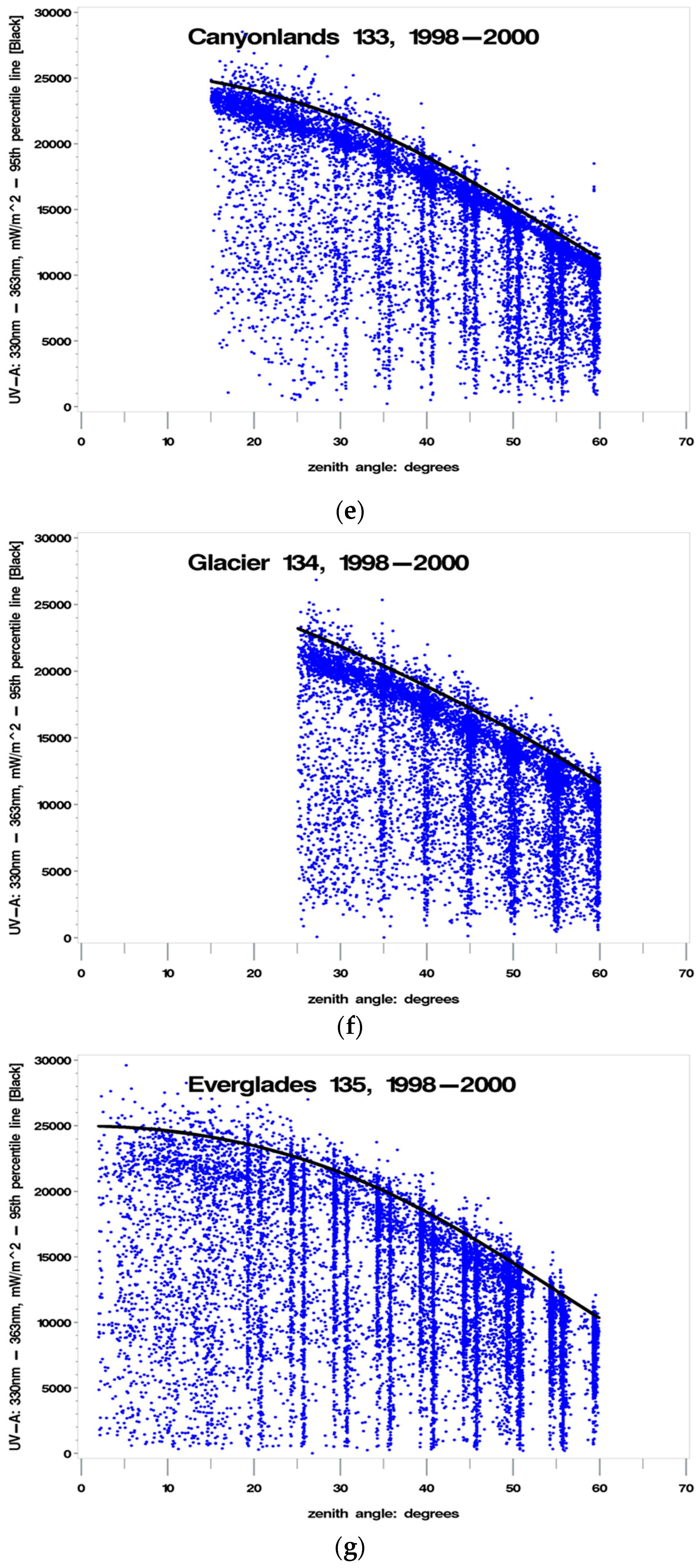




| Brewer Number | Site Location | GPS Latitude | GPS Longitude | Elevation (Meters) | Elevation (Feet) | Start Date | Site Type |
|---|---|---|---|---|---|---|---|
| 087 | Research Triangle Park (RTP), NC | 35.8924 N | 78.8771 W | 104 | 341 | 1995 | Urban |
| 105 | Gaithersburg, MD | 39.1342 N | 77.2167 W | 43 | 141 | 1994 | Urban |
| 130 | Big Bend, TX | 29.3050 N | 103.1770 W | 329 | 1079 | 1997 | National Park |
| 132 | Great Smoky Mountains, TN | 35.6044 N | 83.7829 W | 564 | 1850 | 1996 | National Park |
| 133 | Canyonlands, UT | 38.4584 N | 109.8211 W | 814 | 2671 | 1997 | National Park |
| 134 | Glacier, MT | 48.7409 N | 113.4325 W | 424 | 1391 | 1997 | National Park |
| 135 | Everglades, FL | 25.3906 N | 80.6805 W | 18 | 59 | 1997 | National Park |
| 137 | Shenandoah, VA | 38.5226 N | 78.4349 W | 325 | 1066 | 1997 | National Park |
| 138 | Acadia, ME | 44.3769 N | 68.2609 W | 137 | 449 | 1998 | National Park |
| 144 | St. John, USVI | 18.3360 N | 64.7960 W | 30 | 98 | 1998 | National Park |
| Model | Low RAF | High RAF | Functional Relationship | Calculate RAF—Use Functional Relationship between Biologically Damaging UV Radiation and: |
|---|---|---|---|---|
| A | −0.84 | −1.38 | biologically damaging UV radiation ~ f (O3, CLR, SZA) | total column ozone [O3] |
| CLR [percent (%) clear sky {i.e., percent clearness/cloudiness}] | ||||
| solar zenith angle [SZA] | ||||
| B | −0.88 | −1.375 | biologically damaging UV radiation ~ f (O3, iclear, SZA) | total column ozone [O3] |
| “iclear” [eliminate CLR term, and perform a regression on “classes” of CLR, and replace the parameter CLR with the result of the regression on the “classes” of clear, called iclear] | ||||
| solar zenith angle [SZA] | ||||
| C | −1.00 | −1.18 | biologically damaging UV radiation ~ f (O3, iclear#, SZA)—with data censoring (tails of the biologically damaging UV radiation distribution) | total column ozone [O3] |
| revised “iclear” [eliminate CLR term, and perform a regression on “classes” of CLR, and replace the parameter CLR with the result of the regression on the “classes” of clear, called iclear, and remove the tails of the biologically damaging UV radiation distribution, i.e., censor “bad” data; This is the same as Model B, except with the tails of the biologically damaging UV radiation distribution removed] | ||||
| solar zenith angle [SZA] | ||||
| D | −0.80 | −1.30 | biologically damaging UV radiation ~ f (O3, CLR^, SZA)—CLR is a linear function between 40% and 100% clear skies | total column ozone [O3] |
| CLR [assume a linear function in percent clearness {“classes” of CLR} between 40% and 100% clear skies] | ||||
| solar zenith angle [SZA] | ||||
| E | −0.80 | −1.28 | biologically damaging UV radiation ~ f (O3/cos[SZA], cos[SZA]) | total column ozone [O3]/cos(SZA) |
| cos(SZA) | ||||
| This is known as Beer–Lambert’s Law |
© 2017 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hall, E.S. Comparison of Five Modeling Approaches to Quantify and Estimate the Effect of Clouds on the Radiation Amplification Factor (RAF) for Solar Ultraviolet Radiation. Atmosphere 2017, 8, 153. https://doi.org/10.3390/atmos8080153
Hall ES. Comparison of Five Modeling Approaches to Quantify and Estimate the Effect of Clouds on the Radiation Amplification Factor (RAF) for Solar Ultraviolet Radiation. Atmosphere. 2017; 8(8):153. https://doi.org/10.3390/atmos8080153
Chicago/Turabian StyleHall, Eric S. 2017. "Comparison of Five Modeling Approaches to Quantify and Estimate the Effect of Clouds on the Radiation Amplification Factor (RAF) for Solar Ultraviolet Radiation" Atmosphere 8, no. 8: 153. https://doi.org/10.3390/atmos8080153
APA StyleHall, E. S. (2017). Comparison of Five Modeling Approaches to Quantify and Estimate the Effect of Clouds on the Radiation Amplification Factor (RAF) for Solar Ultraviolet Radiation. Atmosphere, 8(8), 153. https://doi.org/10.3390/atmos8080153





