Atmospheric Response to EEP during Geomagnetic Disturbances
Abstract
1. Introduction
2. Materials and Methods
2.1. Simulation of Ozone Depletion
2.1.1. Model with Standard Parameterization
- ~0.7 molecules of excited nitrogen N(2D), which instantly reacts with neutral oxygen to form NO:
- ~0.55 NO2 molecules
2.1.2. Ion Chemistry Model
3. Results
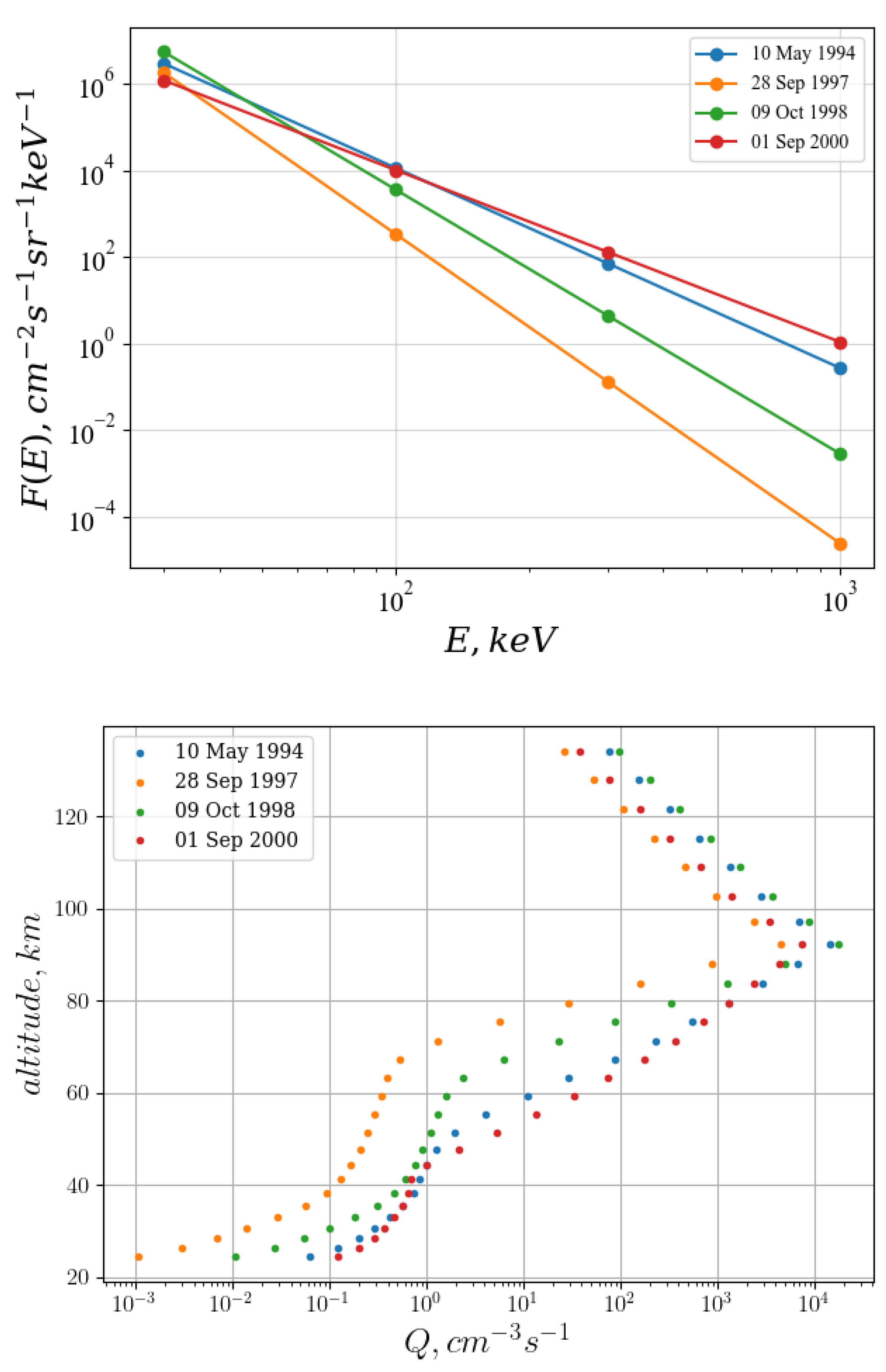
3.1. Obtaining Spectra and Ionization Rates
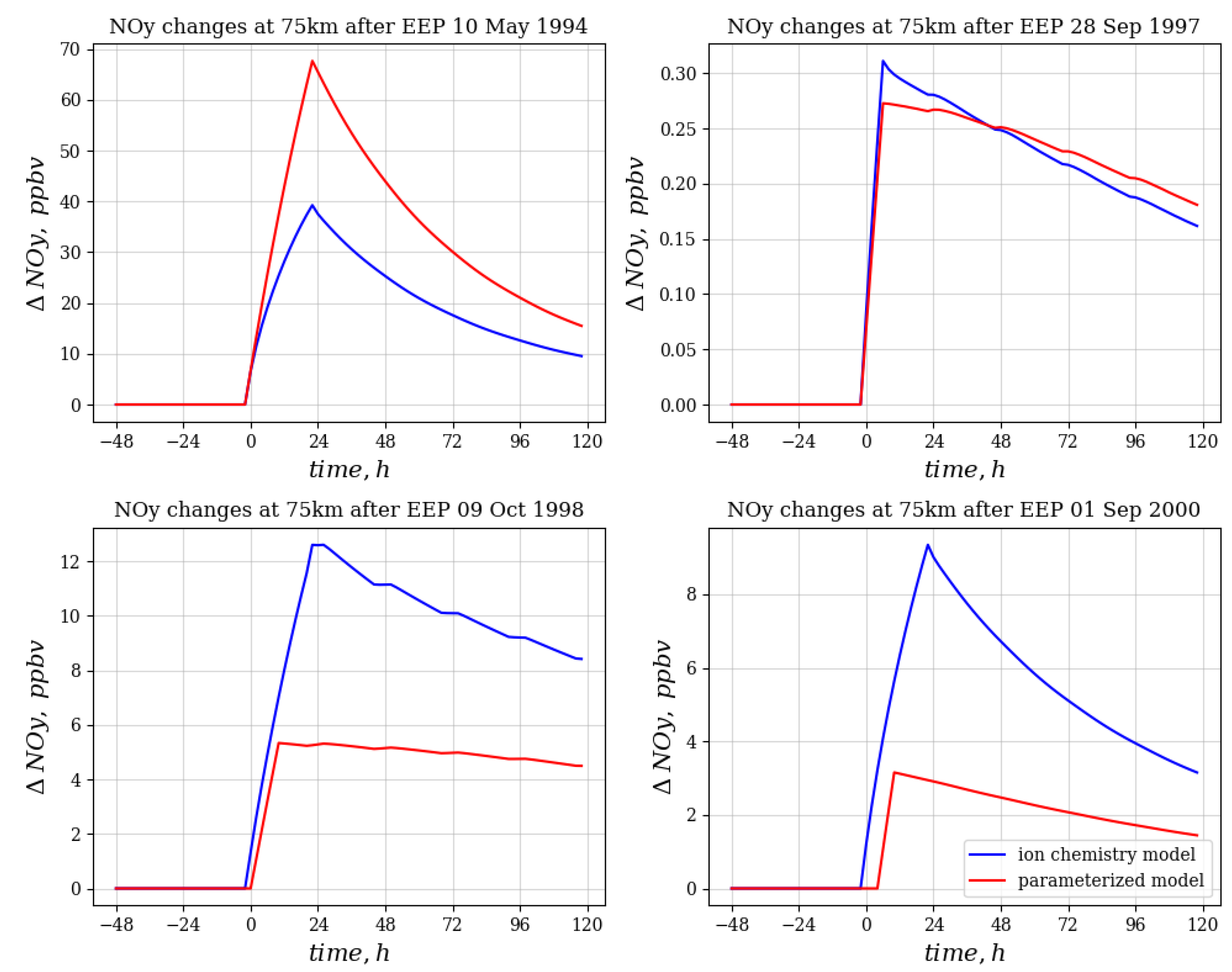
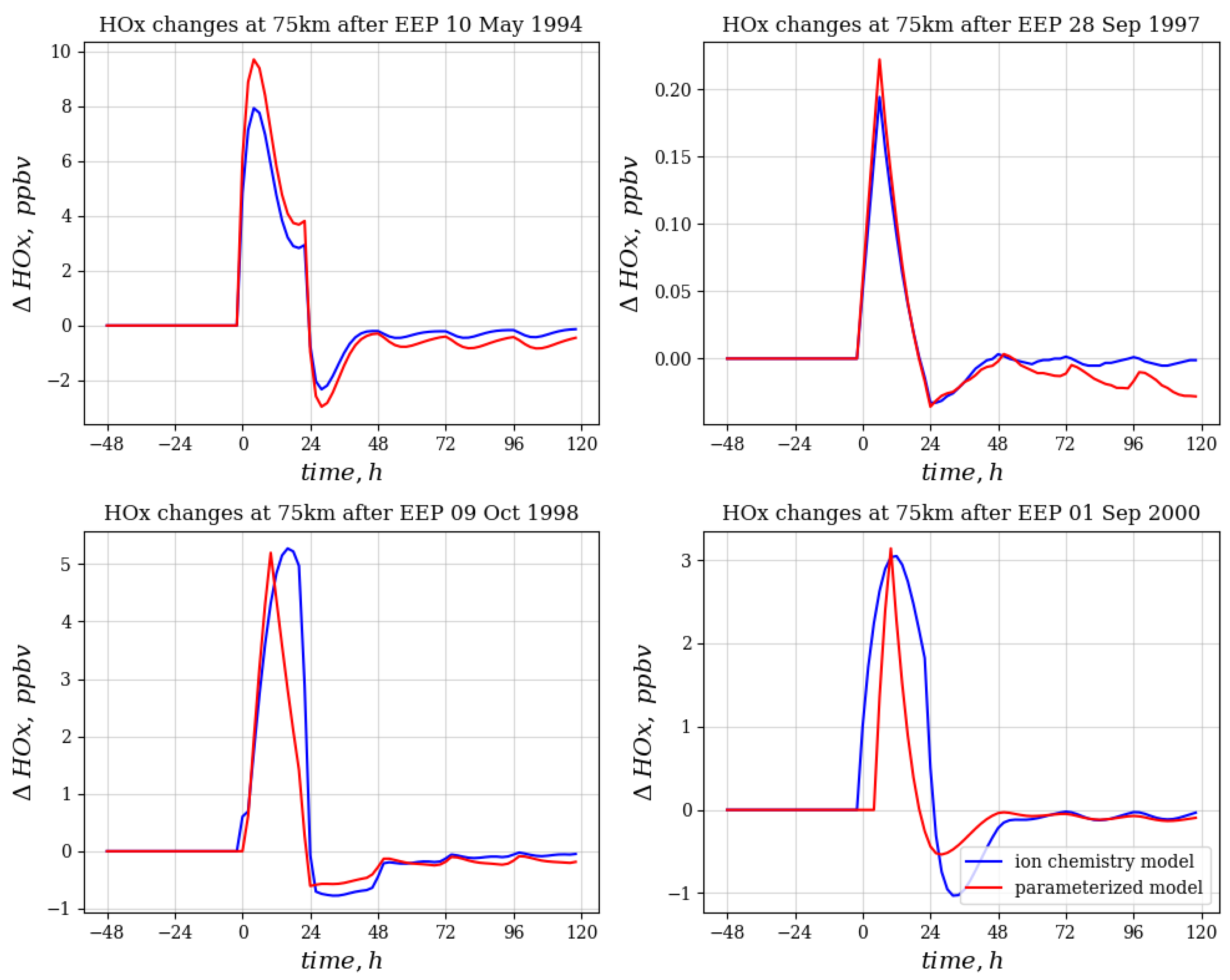
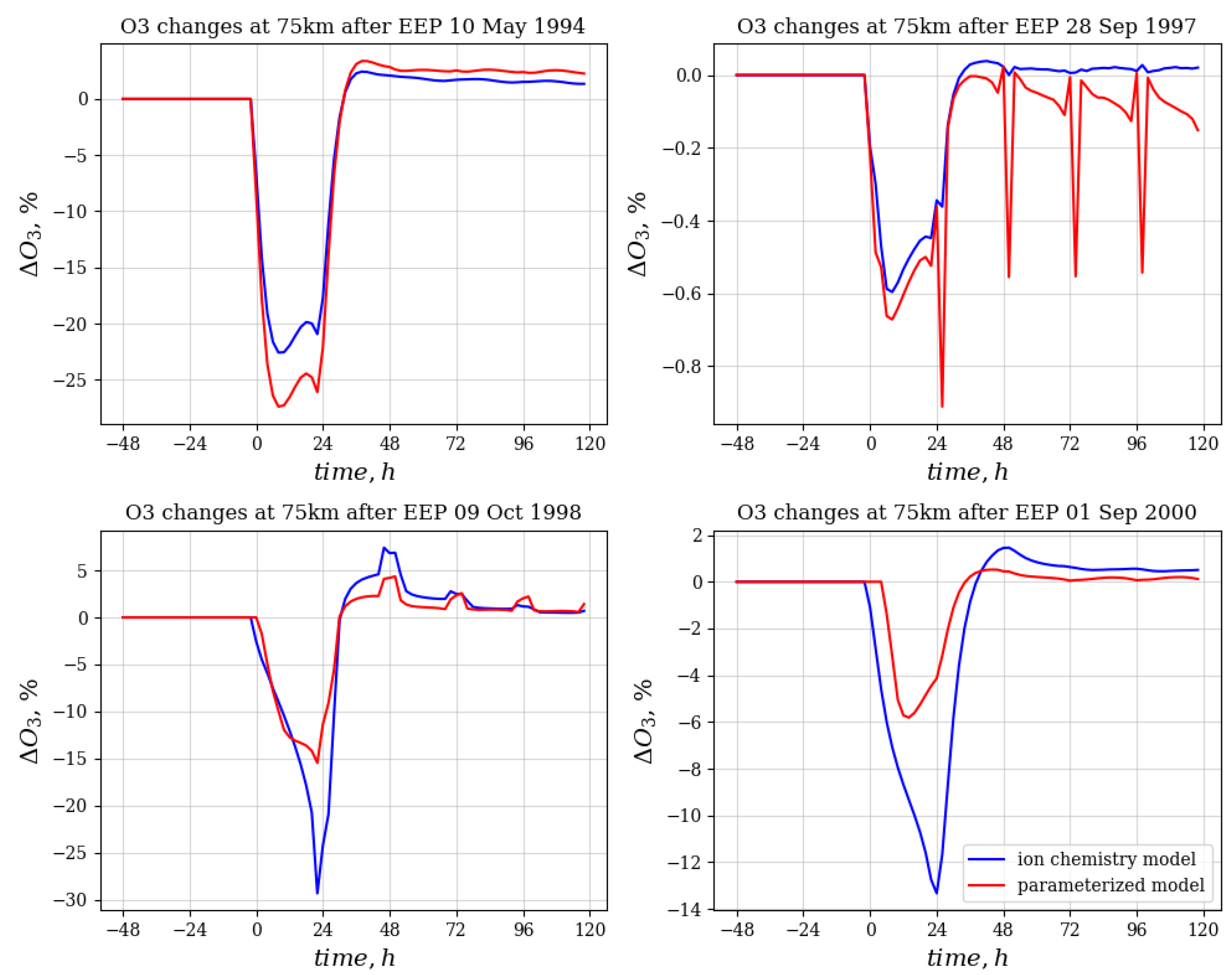
3.2. Model Results
4. Discussions and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mironova, I.A.; Aplin, K.L.; Arnold, F.; Bazilevskaya, G.A.; Harrison, R.G.; Krivolutsky, A.A.; Nicoll, K.A.; Rozanov, E.V.; Turunen, E.; Usoskin, I.G. Energetic Particle Influence on the Earth’s Atmosphere. Space Sci. Rev. 2015, 194, 1–96. [Google Scholar] [CrossRef]
- Rozanov, E.V. Effect of Precipitating Energetic Particles on the Ozone Layer and Climate. Russ. J. Phys. Chem. B 2018, 12, 786–790. [Google Scholar] [CrossRef]
- Cooper, A.; Turney, C.S.M.; Palmer, J.; Hogg, A.; McGlone, M.; Wilmshurst, J.; Lorrey, A.M.; Heaton, T.J.; Russell, J.M.; McCracken, K.; et al. Response to Comment on “A Global Environmental Crisis 42,000 Years Ago”. Science 2021, 374, eabi9756. [Google Scholar] [CrossRef] [PubMed]
- Ball, W.T.; Alsing, J.; Mortlock, D.J.; Staehelin, J.; Haigh, J.D.; Peter, T.; Tummon, F.; Stübi, R.; Stenke, A.; Anderson, J.; et al. Evidence for a Continuous Decline in Lower Stratospheric Ozone Offsetting Ozone Layer Recovery. Atmos. Chem. Phys. 2018, 18, 1379–1394. [Google Scholar] [CrossRef]
- Witze, A. Rare Ozone Hole Opens over Arctic—and It’s Big. Nature 2020, 580, 18–19. [Google Scholar] [CrossRef]
- Timofeyev, Y.M.; Smyshlyaev, S.P.; Virolainen, Y.A.; Garkusha, A.S.; Polyakov, A.V.; Motsakov, M.A.; Kirner, O. Case Study of Ozone Anomalies over Northern Russia in the 2015/2016 Winter: Measurements and Numerical Modelling. Ann. Geophys. 2018, 36, 1495–1505. [Google Scholar] [CrossRef]
- Karagodin, A.; Mironova, I.; Artamonov, A.; Konstantinova, N. Response of the Total Ozone to Energetic Electron Precipitation Events. J. Atmos. Sol. Terr. Phys. 2018, 180, 153–158. [Google Scholar] [CrossRef]
- Szela̧g, M.E.; Marsh, D.R.; Verronen, P.T.; Seppälä, A.; Kalakoski, N. Ozone Impact from Solar Energetic Particles Cools the Polar Stratosphere. Nat. Commun. 2022, 13, 6883. [Google Scholar] [CrossRef] [PubMed]
- George, H.; Reeves, G.; Cunningham, G.; Kalliokoski, M.M.H.; Kilpua, E.; Osmane, A.; Henderson, M.G.; Morley, S.K.; Hoilijoki, S.; Palmroth, M. Contributions to Loss Across the Magnetopause During an Electron Dropout Event. J. Geophys. Res. Space Phys. 2022, 127, 751. [Google Scholar] [CrossRef]
- Wing, S.; Johnson, J.R.; Turner, D.L.; Ukhorskiy, A.Y.; Boyd, A.J. Untangling the Solar Wind and Magnetospheric Drivers of the Radiation Belt Electrons. J. Geophys. Res. Space Phys. 2022, 127, e2021JA030246. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; Tobiska, W.K. A Method for Calculating Atmospheric Radiation Produced by Relativistic Electron Precipitation. Space Weather 2021, 19, e2021SW002735. [Google Scholar] [CrossRef]
- Tobiska, W.K.; Didkovsky, L.; Judge, K.; Weiman, S.; Bouwer, D.; Bailey, J.; Atwell, B.; Maskrey, M.; Mertens, C.; Zheng, Y.; et al. Analytical Representations for Characterizing the Global Aviation Radiation Environment Based on Model and Measurement Databases. Space Weather 2018, 16, 1523–1538. [Google Scholar] [CrossRef] [PubMed]
- Millan, R.M.; McCarthy, M.P.; Sample, J.G.; Smith, D.M.; Thompson, L.D.; McGaw, D.G.; Woodger, L.A.; Hewitt, J.G.; Comess, M.D.; Yando, K.B.; et al. The Balloon Array for RBSP Relativistic Electron Losses (BARREL). Space Sci. Rev. 2013, 179, 503–530. [Google Scholar] [CrossRef]
- Woodger, L.A.; Halford, A.J.; Millan, R.M.; McCarthy, M.P.; Smith, D.M.; Bowers, G.S.; Sample, J.G.; Anderson, B.R.; Liang, X. A Summary of the BARREL Campaigns: Technique for Studying Electron Precipitation. J. Geophys. Res. Space Phys. 2015, 120, 4922–4935. [Google Scholar] [CrossRef] [PubMed]
- Miyoshi, Y.; Hosokawa, K.; Kurita, S.; Oyama, S.I.; Ogawa, Y.; Saito, S.; Shinohara, I.; Kero, A.; Turunen, E.; Verronen, P.T.; et al. Penetration of MeV Electrons into the Mesosphere Accompanying Pulsating Aurorae. Sci. Rep. 2021, 11, 13724. [Google Scholar] [CrossRef] [PubMed]
- Thomson, N.R.; Clilverd, M.A.; Rodger, C.J. Ionospheric D Region: VLF-Measured Electron Densities Compared With Rocket-Based FIRI-2018 Model. J. Geophys. Res. Space Phys. 2022, 127, e2022JA030977. [Google Scholar] [CrossRef]
- Mironova, I.; Bazilevskaya, G. Estimation of Characterized Ionization Rates during Geomagnetic Disturbances with Kp = 4 Based on Balloon Observations; Springer: Saint-Petersburg, Russia, 2023. [Google Scholar]
- Mironova, I.; Sinnhuber, M.; Bazilevskaya, G.; Clilverd, M.; Funke, B.; Makhmutov, V.; Rozanov, E.; Santee, M.L.; Sukhodolov, T.; Ulich, T. Exceptional Middle Latitude Electron Precipitation Detected by Balloon Observations: Implications for Atmospheric Composition. Atmos. Chem. Phys. 2022, 22, 6703–6716. [Google Scholar] [CrossRef]
- Mironova, I.; Kovaltsov, G.; Mishev, A.; Artamonov, A. Ionization in the Earth’s Atmosphere Due to Isotropic Energetic Electron Precipitation: Ion Production and Primary Electron Spectra. Remote Sens. 2021, 13, 4161. [Google Scholar] [CrossRef]
- Mironova, I.A.; Artamonov, A.A.; Bazilevskaya, G.A.; Rozanov, E.V.; Kovaltsov, G.A.; Makhmutov, V.S.; Mishev, A.L.; Karagodin, A.V. Ionization of the Polar Atmosphere by Energetic Electron Precipitation Retrieved From Balloon Measurements. Geophys. Res. Lett. 2019, 46, 990–996. [Google Scholar] [CrossRef]
- Artamonov, A.A.; Mishev, A.L.; Usoskin, I.G. Model CRAC:EPII for Atmospheric Ionization Due to Precipitating Electrons: Yield Function and Applications. J. Geophys. Res. Space Phys. 2016, 121, 1736–1743. [Google Scholar] [CrossRef]
- Mironova, I.; Bazilevskaya, G.; Kovaltsov, G.; Artamonov, A.; Rozanov, E.; Mishev, A.; Makhmutov, V.; Karagodin, A.; Golubenko, K. Spectra of High Energy Electron Precipitation and Atmospheric Ionization Rates Retrieval from Balloon Measurements. Sci. Total. Environ. 2019, 69, 133242. [Google Scholar] [CrossRef] [PubMed]
- Chaston, C.C.; Bonnell, J.W.; Halford, A.J.; Reeves, G.D.; Baker, D.N.; Kletzing, C.A.; Wygant, J.R. Pitch Angle Scattering and Loss of Radiation Belt Electrons in Broadband Electromagnetic Waves. Geophys. Res. Lett. 2018, 45, 9344–9352. [Google Scholar] [CrossRef]
- Turunen, E.; Kero, A.; Verronen, P.T.; Miyoshi, Y.; Oyama, S.I.; Saito, S. Mesospheric Ozone Destruction by High-Energy Electron Precipitation Associated with Pulsating Aurora. J. Geophys. Res. 2016, 121, 11852–11861. [Google Scholar] [CrossRef]
- Matthes, K.; Funke, B.; Andersson, M.E.; Barnard, L.; Beer, J.; Charbonneau, P.; Clilverd, M.A.; Dudok De Wit, T.; Haberreiter, M.; Hendry, A.; et al. Solar Forcing for CMIP6 (v3.2). Geosci. Model. Dev. 2017, 10, 2247–2302. [Google Scholar] [CrossRef]
- Stozhkov, Y.I.; Svirzhevsky, N.S.; Bazilevskaya, G.A.; Kvashnin, A.N.; Makhmutov, V.S.; Svirzhevskaya, A.K. Long-Term (50 Years) Measurements of Cosmic Ray Fluxes in the Atmosphere. Adv. Space Res. 2009, 44, 1124–1137. [Google Scholar] [CrossRef]
- Makhmutov, V.S.; Bazilevskaya, G.A.; Stozhkov, Y.I.; Svirzhevskaya, A.K.; Svirzhevsky, N.S. Catalogue of Electron Precipitation Events as Observed in the Long-Duration Cosmic Ray Balloon Experiment. J. Atmos. Sol. Terr. Phys. 2016, 149, 258–276. [Google Scholar] [CrossRef]
- Bazilevskaya, G.A.; Kalinin, M.S.; Krainev, M.B.; Makhmutov, V.S.; Stozhkov, Y.I.; Svirzhevskaya, A.K.; Svirzhevsky, N.S.; Gvozdevsky, B.B. Temporal Characteristics of Energetic Magnetospheric Electron Precipitation as Observed During Long-Term Balloon Observations. J. Geophys. Res. Space Phys. 2020, 125, e2020JA028033. [Google Scholar] [CrossRef]
- Bernhardt, P.A.; Hua, M.; Bortnik, J.; Ma, Q.; Verronen, P.T.; McCarthy, M.P.; Hampton, D.L.; Golkowski, M.; Cohen, M.B.; Richardson, D.K.; et al. Active Precipitation of Radiation Belt Electrons Using Rocket Exhaust Driven Amplification (REDA) of Man-Made Whistlers. J. Geophys. Res. Space Phys. 2022, 127, e2022JA030358. [Google Scholar] [CrossRef]
- Direct Access to POES SEM Data. Available online: https://www.ngdc.noaa.gov/stp/satellite/poes/dataaccess.html (accessed on 28 January 2022).
- Porter, H.S.; Jackman, C.H.; Green, A.E.S. Efficiencies for Production of Atomic Nitrogen and Oxygen by Relativistic Proton Impact in Air. J. Chem. Phys. 1976, 65, 154–167. [Google Scholar] [CrossRef]
- Sinnhuber, M.; Nieder, H.; Wieters, N. Energetic Particle Precipitation and the Chemistry of the Mesosphere/Lower Thermosphere. Surv. Geophys. 2012, 33, 1281–1334. [Google Scholar] [CrossRef]
- Solomon, S.; Rusch, D.W.; Gitrard, J.-C.; Red, G.C.; Crutzenk, P.J. The Effect Neutral and of Particle Precipitation Events on the Ion Chemistry of the Middle Atmosphere: II. Odd Hydrogen. Planet. Space Sci. 1981, 29, 885–893. [Google Scholar] [CrossRef]
- Verronen, P.T.; Andersson, M.E.; Marsh, D.R.; Kovács, T.; Plane, J.M.C. WACCM-D Whole Atmosphere Community Climate Model with D-Region Ion Chemistry. J. Adv. Model. Earth Syst. 2016, 8, 954–975. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; Fang, X.; Turunen, E.; Kero, A. On the Effects of Bremsstrahlung Radiation During Energetic Electron Precipitation. Geophys. Res. Lett. 2018, 45, 1167–1176. [Google Scholar] [CrossRef]


| Date (DOY) | Start Time (UT) –End Time (UT) | Spectra Parameters Kp (UT) F0 k |
|---|---|---|
| 10 May 1994 (129) | 7 h 10 m–7 h 58 m | 1.94 × 1013 4.62 4 (00–21 h) |
| 28 Sep. 1997 (270) | 7 h 00 m–7 h 32 m | 6.29 × 1016 7.14 4 (00–06 h) |
| 9 Oct. 1998 (281) | 7 h 48 m–8 h 16 m | 5.48 × 1015 6.09 4 (03–09 h) |
| 1 Sep. 2000 (245) | 7 h 42 m–8 h 37 m | 8.68 × 1011 3.97 4 (06–09 h) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grankin, D.; Mironova, I.; Bazilevskaya, G.; Rozanov, E.; Egorova, T. Atmospheric Response to EEP during Geomagnetic Disturbances. Atmosphere 2023, 14, 273. https://doi.org/10.3390/atmos14020273
Grankin D, Mironova I, Bazilevskaya G, Rozanov E, Egorova T. Atmospheric Response to EEP during Geomagnetic Disturbances. Atmosphere. 2023; 14(2):273. https://doi.org/10.3390/atmos14020273
Chicago/Turabian StyleGrankin, Dmitry, Irina Mironova, Galina Bazilevskaya, Eugene Rozanov, and Tatiana Egorova. 2023. "Atmospheric Response to EEP during Geomagnetic Disturbances" Atmosphere 14, no. 2: 273. https://doi.org/10.3390/atmos14020273
APA StyleGrankin, D., Mironova, I., Bazilevskaya, G., Rozanov, E., & Egorova, T. (2023). Atmospheric Response to EEP during Geomagnetic Disturbances. Atmosphere, 14(2), 273. https://doi.org/10.3390/atmos14020273







