Tidal Structures in the Mesosphere and Lower Thermosphere and Their Solar Cycle Variations
Abstract
1. Introduction
2. Dataset and Analysis
2.1. SD-WACCM-X
2.2. Methodology
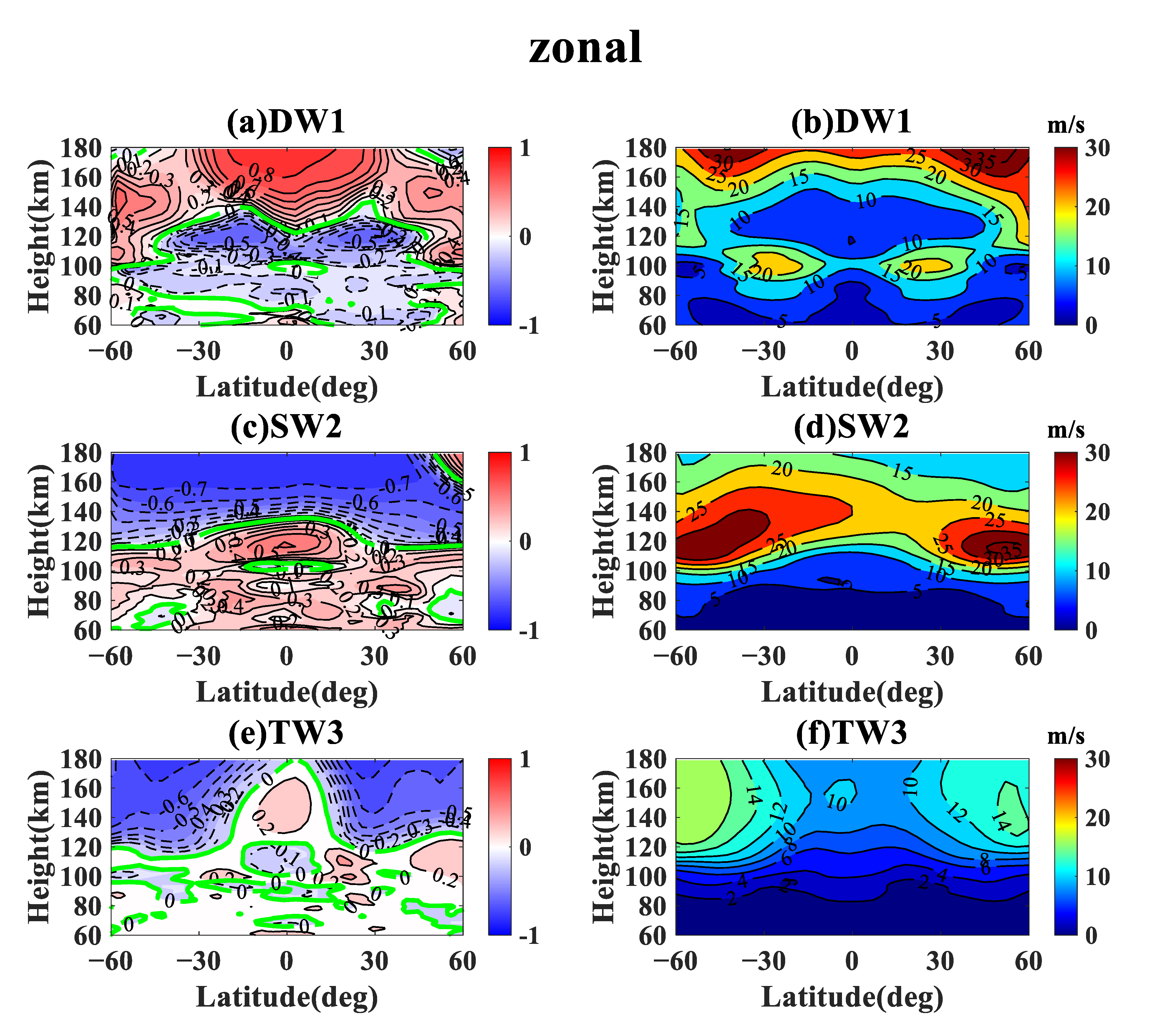
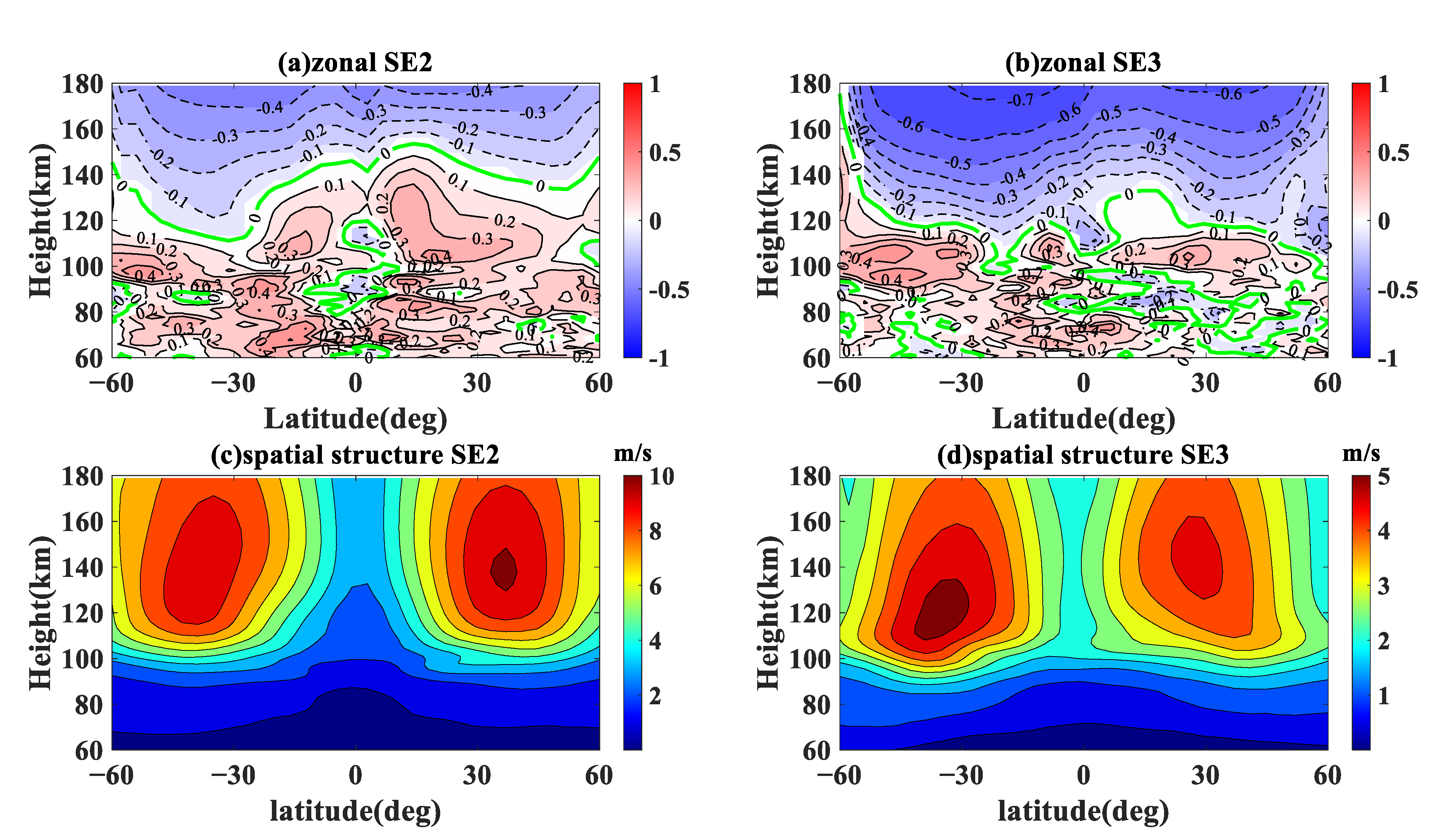
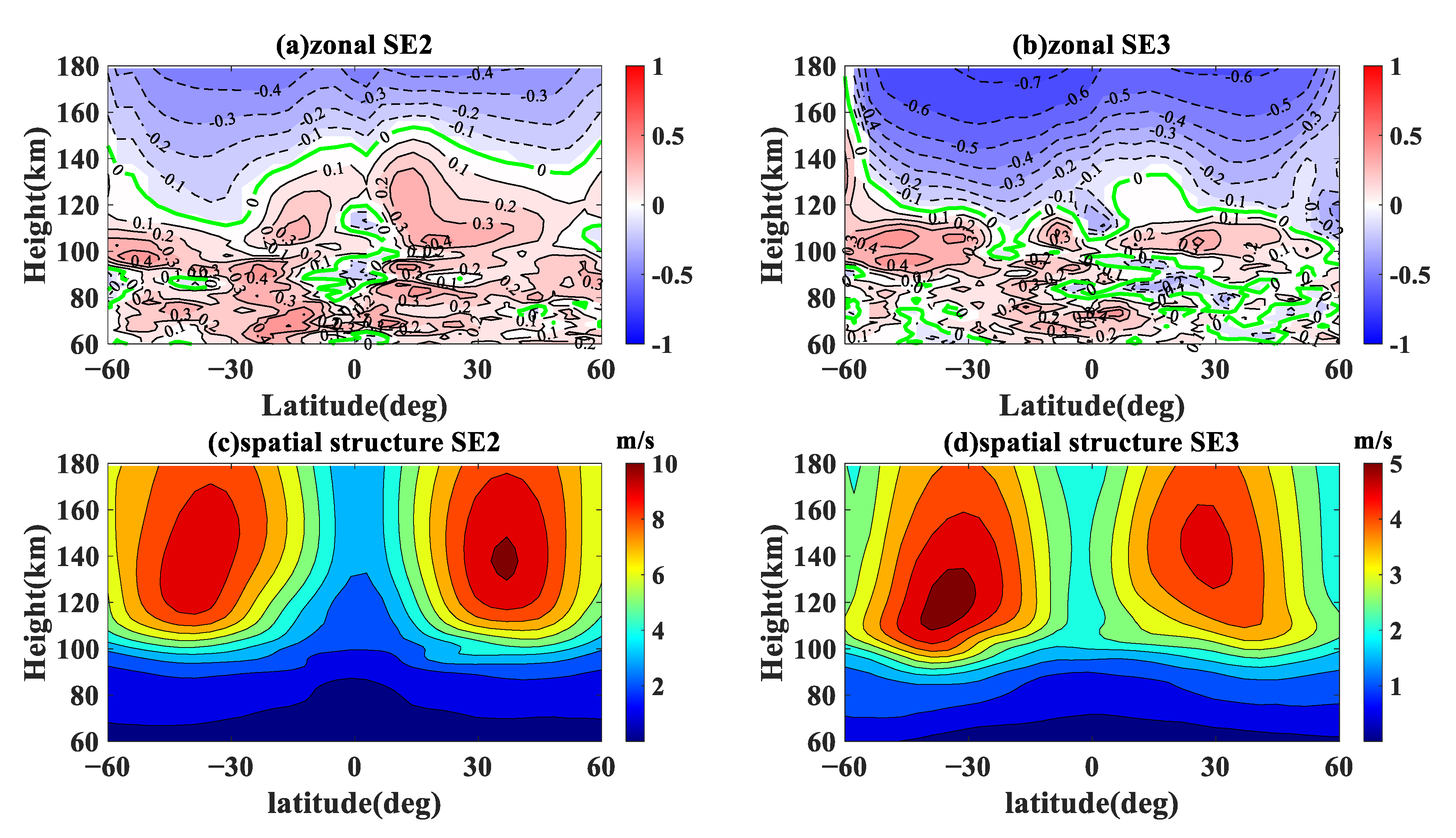
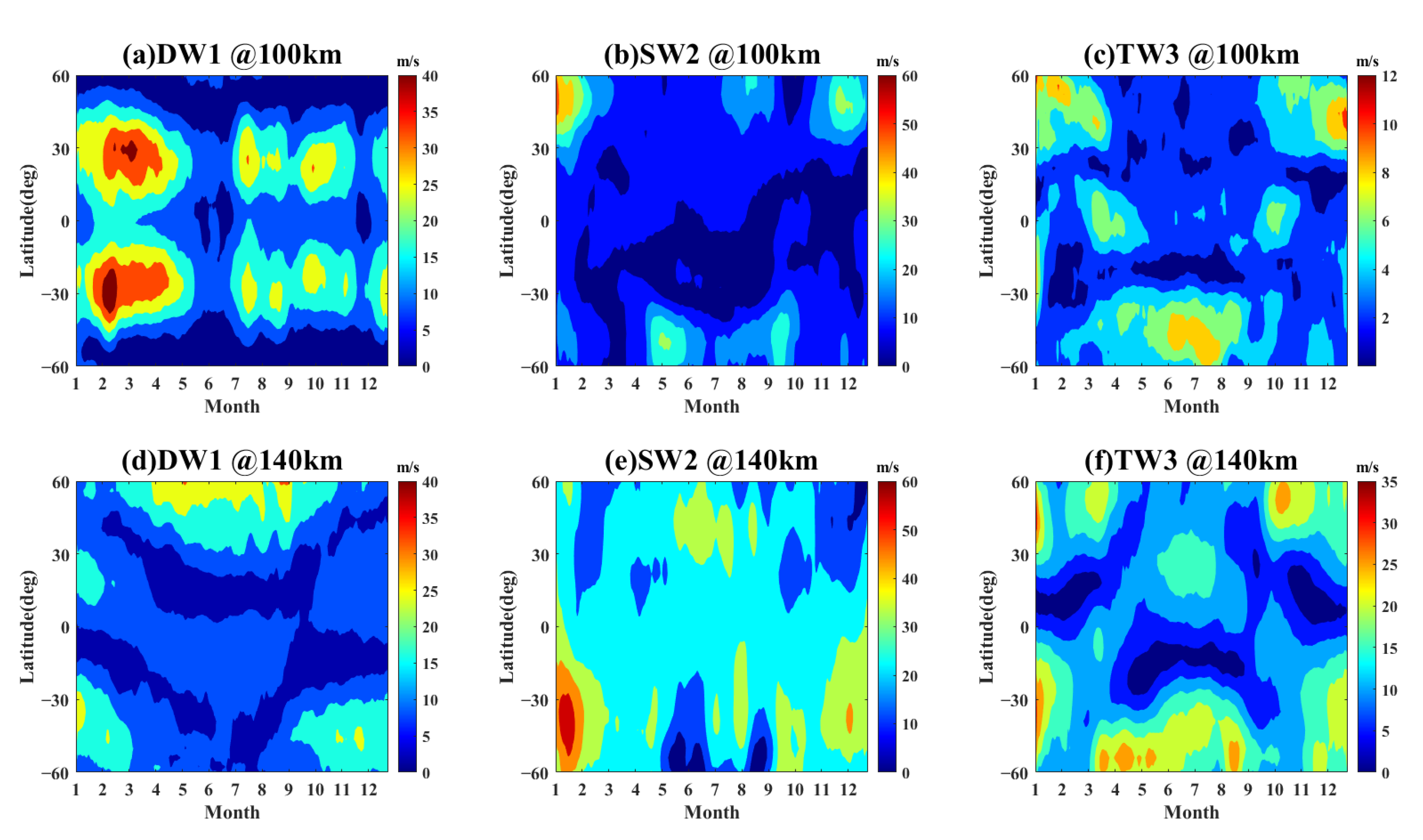
3. Results
3.1. Migrating Tides
3.2. Non-Migrating Tides
3.3. Seasonal Variation
4. Discussion
4.1. Seasonal Variation
4.1.1. Migrating Tides
4.1.2. Non-Migrating Tides
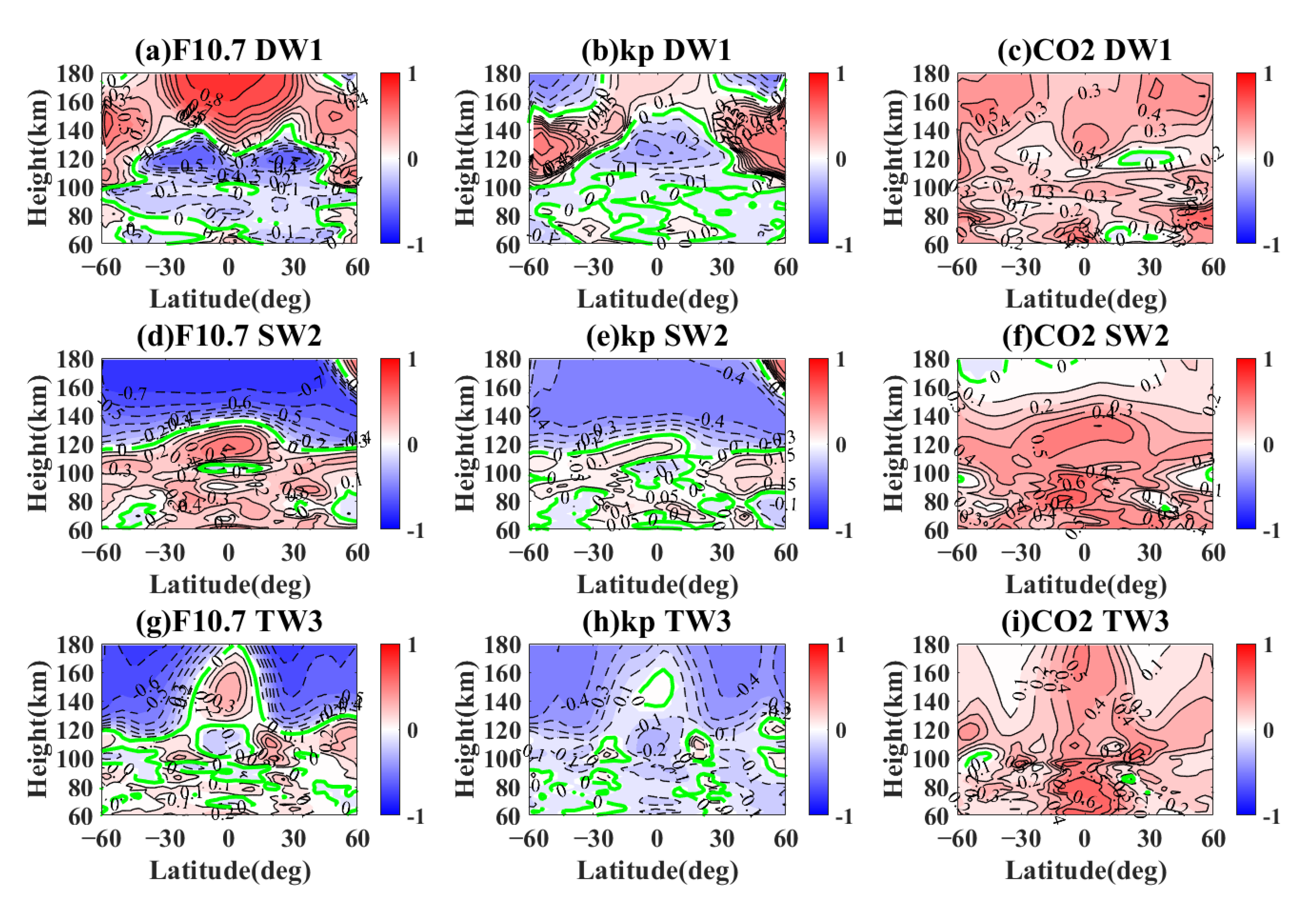
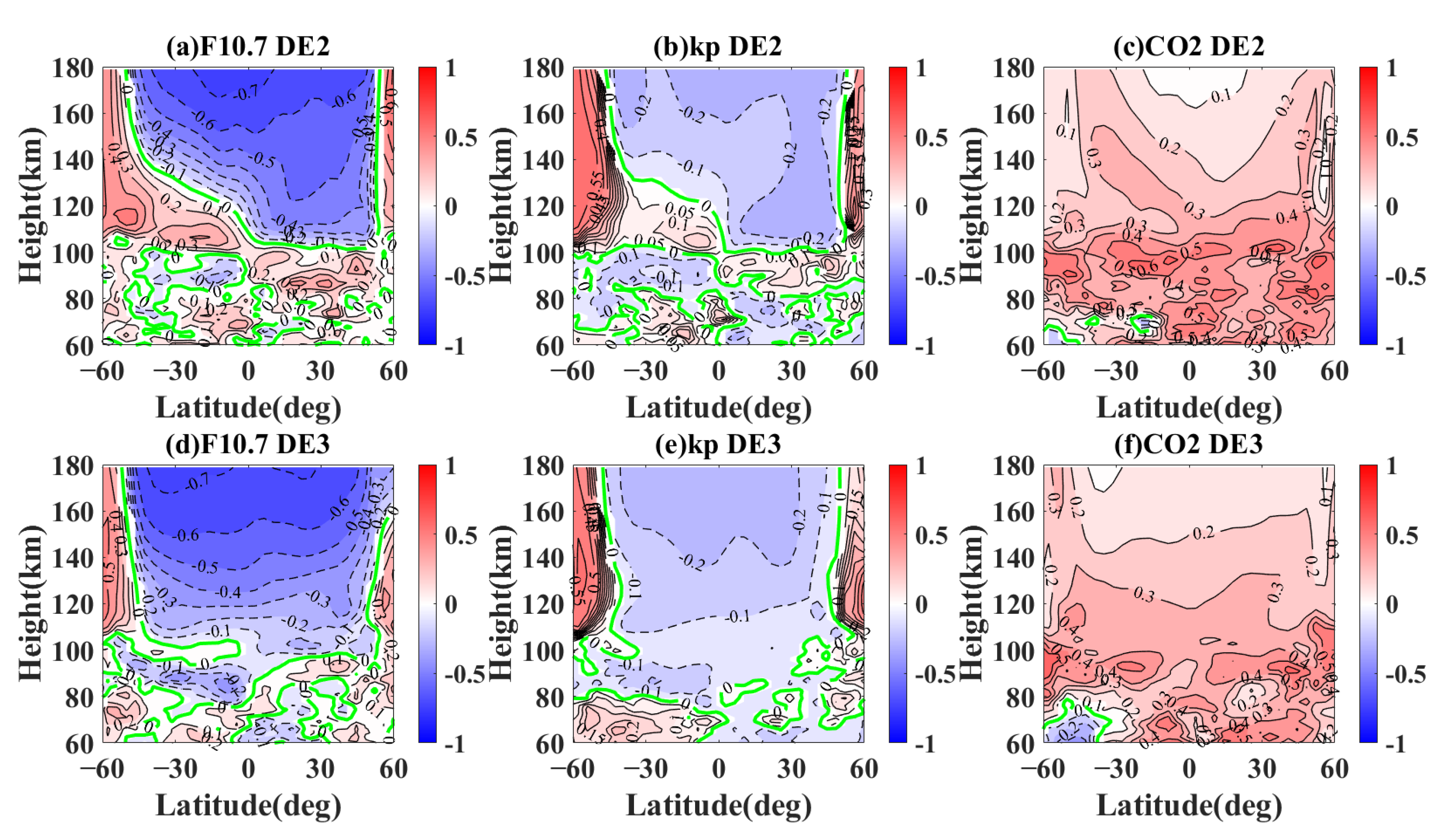
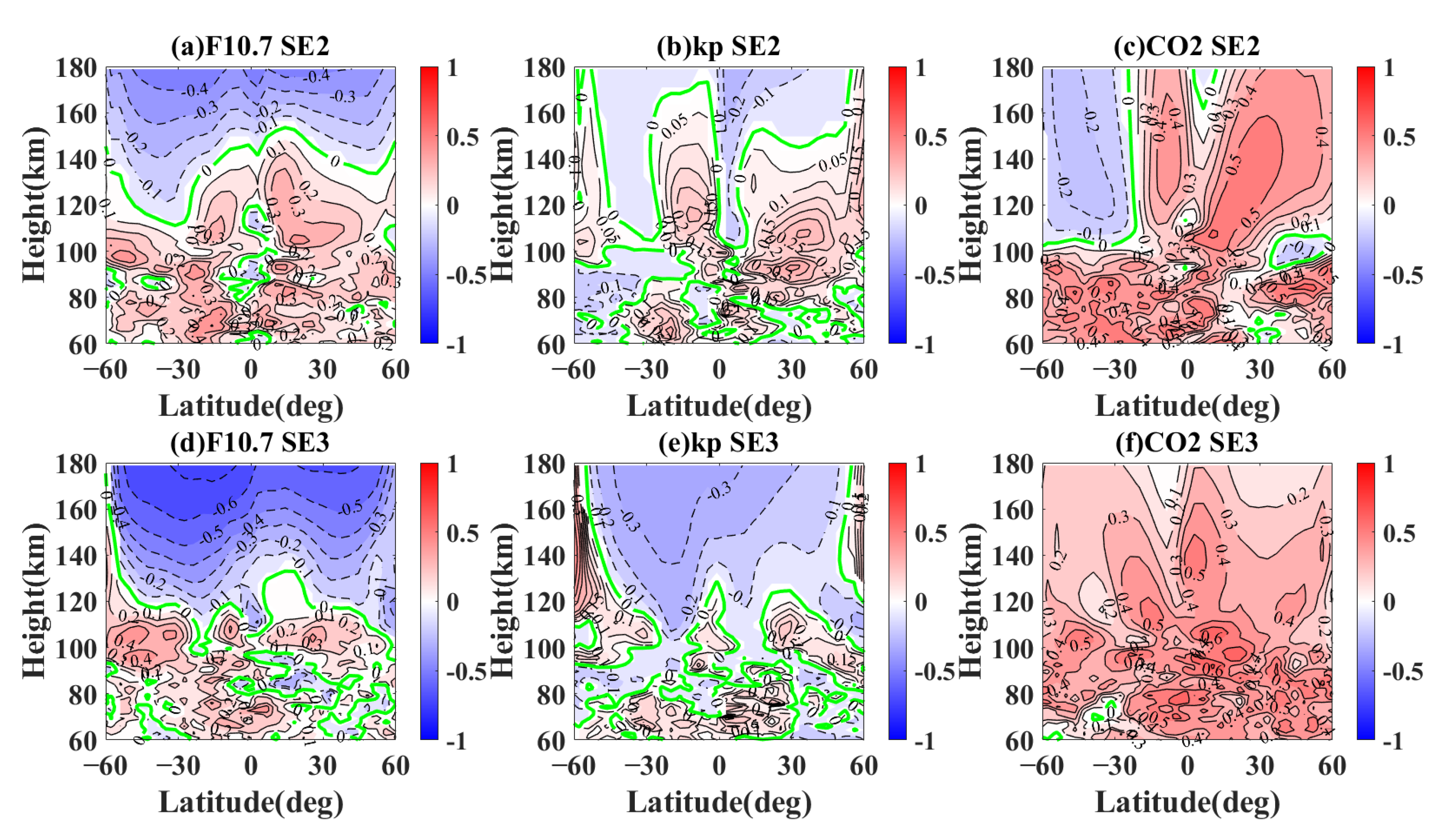
4.2. Impact of CO2 and Geomagnetic Activity
4.2.1. Migrating Tides
4.2.2. Non-Migrating Tides
5. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lindzen, R.S.; Chapman, S. Atmospheric tides. Space Sci. Rev. 1969, 10, 3–188. [Google Scholar] [CrossRef]
- Miyahara, S.; Miyoshi, Y. Migrating and non-migrating atmospheric tides simulated by a middle atmosphere general circulation model. Adv. Space Res. 1997, 20, 1201–1207. [Google Scholar] [CrossRef]
- Hagan, M.E.; Forbes, J.M. Migrating and nonmigrating diurnal tides in the middle and upper atmosphere excited by tropospheric latent heat release. J. Geophys. Res. Atmos. 2002, 107, ACL 6-1–ACL 6-15. [Google Scholar] [CrossRef]
- Häusler, K.; Luhr, H.; Hagan, M.E.; Maute, A.; Roble, R.G. Comparison of CHAMP and TIME-GCM nonmigrating tidal signals in the thermospheric zonal wind. J. Geophys. Res. Atmos. 2010, 115. [Google Scholar] [CrossRef]
- Remsberg, E.; Lingenfelser, G.; Harvey, V.; Grose, W.; Russell, J., III; Mlynczak, M.; Gordley, L.; Marshall, B.T. On the verification of the quality of SABER temperature, geopotential height, and wind fields by comparison with Met Office assimilated analyses. J. Geophys. Res. Atmos. 2003, 108. [Google Scholar] [CrossRef]
- Manson, A.H.; Meek, C.E.; Teitelbaum, H.; Vial, F.; Schminder, R.; Kürschner, D.; Smith, M.J.; Fraser, G.J.; Clark, R.R. Climatologies of semi-diurnal and diurnal tides in the middle atmosphere (70–110 km) at middle latitudes (40–55). J. Atmos. Terr. Phys. 1989, 51, 579–593. [Google Scholar] [CrossRef]
- Sridharan, S. Seasonal Variations of Low-Latitude Migrating and Nonmigrating Diurnal and Semidiurnal Tides in TIMED-SABER Temperature and Their Relationship With Source Variations. J. Geophys. Res. Space Phys. 2019, 124, 3558–3572. [Google Scholar] [CrossRef]
- Yue, J.; Xu, J.; Chang, L.C.; Wu, Q.; Liu, H.-L.; Lu, X.; Russell, J., III. Global structure and seasonal variability of the migrating terdiurnal tide in the mesosphere and lower thermosphere. J. Atmos. Solar-Terr. Phys. 2013, 105, 191–198. [Google Scholar] [CrossRef]
- Liu, H.-L.; Wang, W.; Richmond, A.; Roble, R.G. Ionospheric variability due to planetary waves and tides for solar minimum conditions. J. Geophys. Res. Space Phys. 2010, 115. [Google Scholar] [CrossRef]
- Pedatella, N.M.; Forbes, J.M. Evidence for stratosphere sudden warming-ionosphere coupling due to vertically propagating tides. Geophys. Res. Lett. 2010, 37. [Google Scholar] [CrossRef]
- Chang, L.C.; Palo, S.E.; Liu, H.L. Short-term variation of the s= 1 nonmigrating semidiurnal tide during the 2002 stratospheric sudden warming. J. Geophys. Res. Atmos. 2009, 114. [Google Scholar] [CrossRef]
- Lieberman, R.S.; Oberheide, J.; Talaat, E.R. Nonmigrating diurnal tides observed in global thermospheric winds. J. Geophys. Res. Space Phys. 2013, 118, 7384–7397. [Google Scholar] [CrossRef]
- Häusler, K.; Lühr, H. Nonmigrating tidal signals in the upper thermospheric zonal wind at equatorial latitudes as observed by CHAMP. Ann. Geophys. 2009, 27, 2643–2652. [Google Scholar] [CrossRef]
- Forbes, J.M.; Garrett, H.B. Theoretical studies of atmospheric tides. Rev. Geophys. 1979, 17, 1951–1981. [Google Scholar] [CrossRef]
- Balan, N.; Bailey, G.J. Equatorial plasma fountain and its effects: Possibility of an additional layer. J. Geophys. Res. Space Phys. 1995, 100, 21421–21432. [Google Scholar] [CrossRef]
- Tapping, K.F. The 10.7 cm solar radio flux (F10. 7). Space Weather 2013, 11, 394–406. [Google Scholar] [CrossRef]
- Sprenger, K.; Schminder, R. Solar cycle dependence of winds in the lower ionosphere. J. Atmos. Terr. Phys. 1969, 31, 217–221. [Google Scholar] [CrossRef]
- Namboothiri, S.; Manson, A.; Meek, C. Variations of mean winds and tides in the upper middle atmosphere over a solar cycle, Saskatoon, Canada, 52°N, 107°W. J. Atmos. Terr. Phys. 1993, 55, 1325–1334. [Google Scholar] [CrossRef]
- Bremer, J.; Schminder, R.; Greisiger, K.; Hoffmann, P.; Kürschner, D.; Singer, W. Solar cycle dependence and long-term trends in the wind field of the mesosphere/lower thermosphere. J. Atmos. Solar-Terr. Phys. 1997, 59, 497–509. [Google Scholar] [CrossRef]
- Wilhelm, S.; Stober, G.; Brown, P. Climatologies and long-term changes in mesospheric wind and wave measurements based on radar observations at high and mid latitudes. Ann. Geophys. 2019, 37, 851–875. [Google Scholar] [CrossRef]
- Gong, Y.; Lv, X.; Zhang, S.; Zhou, Q.; Ma, Z. Climatology and seasonal variation of the thermospheric tides and their response to solar activities over Arecibo. J. Atmos. Solar-Terr. Phys. 2021, 215, 105592. [Google Scholar] [CrossRef]
- Andrioli, V.F.; Xu, J.; Batista, P.P.; Resende, L.C.A.; Da Silva, L.A.; Marchezi, J.P.; Li, H.; Wang, C.; Liu, Z.; Guharay, A. New Findings Relating Tidal Variability and Solar Activity in the Low Latitude MLT Region. J. Geophys. Res. Space Phys. 2022, 127. [Google Scholar] [CrossRef]
- Guharay, A.; Batista, P.; Andrioli, V. Investigation of solar cycle dependence of the tides in the low latitude MLT using meteor radar observations. J. Atmos. Solar-Terr. Phys. 2019, 193. [Google Scholar] [CrossRef]
- Dhadly, M.S.; Emmert, J.T.; Drob, D.P.; McCormack, J.P.; Niciejewski, R.J. Short-Term and Interannual Variations of Migrating Diurnal and Semidiurnal Tides in the Mesosphere and Lower Thermosphere. J. Geophys. Res. Space Phys. 2018, 123, 7106–7123. [Google Scholar] [CrossRef]
- Baumgaertner, A.; McDonald, A.; Fraser, G.; Plank, G. Long-term observations of mean winds and tides in the upper mesosphere and lower thermosphere above Scott Base, Antarctica. J. Atmos. Solar-Terr. Phys. 2005, 67, 1480–1496. [Google Scholar] [CrossRef]
- Chang, L.C.; Lin, C.H.; Liu, J.Y.; Balan, N.; Yue, J.; Lin, J.T. Seasonal and local time variation of ionospheric mi-grating tides in 2007–2011 FORMOSAT-3/COSMIC and TIE-GCM total electron content. J. Geophys. Res. Space Phys. 2013, 118, 2545–2564. [Google Scholar] [CrossRef]
- Liu, H.-L.; Foster, B.T.; Hagan, M.E.; McInerney, J.M.; Maute, A.; Qian, L.; Richmond, A.; Roble, R.G.; Solomon, S.; Garcia, R.R.; et al. Thermosphere extension of the Whole Atmosphere Community Climate Model. J. Geophys. Res. Space Phys. 2010, 115. [Google Scholar] [CrossRef]
- Liu, H.; Bardeen, C.G.; Foster, B.T.; Lauritzen, P.; Liu, J.; Lu, G.; Marsh, D.R.; Maute, A.; McInerney, J.M.; Pedatella, N.M.; et al. Development and Validation of the Whole Atmosphere Community Climate Model With Thermosphere and Ionosphere Extension (WACCM-X 2.0). J. Adv. Model. Earth Syst. 2018, 10, 381–402. [Google Scholar] [CrossRef]
- Wu, Q.; Ortland, D.A.; Killeen, T.L.; Roble, R.G.; Hagan, M.E.; Liu, H.-L.; Solomon, S.C.; Xu, J.; Skinner, W.R.; Niciejewski, R.J. Global distribution and interannual variations of mesospheric and lower thermospheric neutral wind diurnal tide: 2. Nonmigrating tide. J. Geophys. Res. Space Phys. 2008, 113. [Google Scholar] [CrossRef]
- Wu, Q.; Ortland, D.; Killeen, T.; Roble, R.; Hagan, M.; Liu, H.L.; Solomon, S.; Xu, J.; Skinner, W.; Niciejewski, R.J. Global distribution and interannual variations of mesospheric and lower thermospheric neutral wind diurnal tide: 1. Migrating tide. J. Geophys. Res. Space Phys. 2008, 113. [Google Scholar] [CrossRef]
- Wu, N.L.; Hays, P.B.; Skinner, W.R. A Least Squares Method for Spectral Analysis of Space-Time Series. J. Atmos. Sci. 1995, 52, 3501–3511. [Google Scholar] [CrossRef]
- Lee Rodgers, J.; Nicewander, W.A. Thirteen ways to look at the correlation coefficient. Am. Stat. 1988, 42, 59–66. [Google Scholar] [CrossRef]
- Huang, C.; Liu, D.-D.; Wang, J.-S. Forecast daily indices of solar activity, F10.7, using support vector regression method. Res. Astron. Astrophys. 2009, 9, 694–702. [Google Scholar] [CrossRef]
- Hathaway, D.H. The Solar Cycle. Living Rev. Sol. Phys. 2015, 12, 1–87. [Google Scholar] [CrossRef]
- Oberheide, J.; Forbes, J.; Zhang, X.; Bruinsma, S.L. Climatology of upward propagating diurnal and semidiurnal tides in the thermosphere. J. Geophys. Res. Space Phys. 2011, 116. [Google Scholar] [CrossRef]
- Hong, S.-S.; Lindzen, R.S. Solar Semidiurnal Tide in the Thermosphere. J. Atmos. Sci. 1976, 33, 135–153. [Google Scholar] [CrossRef]
- Oberheide, J.; Forbes, J.; Häusler, K.; Wu, Q.; Bruinsma, S.L. Tropospheric tides from 80 to 400 km: Propagation, interannual variability, and solar cycle effects. J. Geophys. Res. Atmos. 2009, 114. [Google Scholar] [CrossRef]
- Zhang, X.; Forbes, J.M.; Hagan, M.E. Longitudinal variation of tides in the MLT region: 1. Tides driven by tropospheric net radiative heating. J. Geophys. Res. Space Phys. 2010, 115. [Google Scholar] [CrossRef]
- Zhang, S.; Holt, J.M.; Erickson, P.J.; Goncharenko, L.P.; Nicolls, M.J.; McCready, M.; Kelly, J. Ionospheric ion temperature climate and upper atmospheric long-term cooling. J. Geophys. Res. Space Phys. 2016, 121, 8951–8968. [Google Scholar] [CrossRef]
- Liu, H.; Tao, C.; Jin, H.; Nakamoto, Y. Circulation and Tides in a Cooler Upper Atmosphere: Dynamical Effects of CO2 Doubling. Geophys. Res. Lett. 2020, 47. [Google Scholar] [CrossRef]
- Legrand, J.-P.; Simon, P.J.A. Some solar cycle phenomena related to the geomagnetic activity from 1868 to 1980. I-The shock events, or the interplanetary expansion of the toroidal field. Astron. Astrophys. 1985, 152, 199–204. [Google Scholar]
- Legrand, J.; Simon, P. Solar cycle and geomagnetic activity: A review for geophysicists. Part I. The contributions to geomagnetic activity. Ann. Geophys. 1989, 7, 565–578. [Google Scholar]
- Obridko, V.N.; Kanonidi, K.D.; Mitrofanova, T.A.; Shelting, B.D. Solar activity and geomagnetic disturbances. Geomagn. Aeron. 2013, 53, 147–156. [Google Scholar] [CrossRef]
- Yi, W.; Reid, I.M.; Xue, X.; Murphy, D.J.; Vincent, R.A.; Zou, Z.; Chen, T.; Wang, G.; Dou, X. First Observations of Antarctic Mesospheric Tidal Wind Responses to Recurrent Geomagnetic Activity. Geophys. Res. Lett. 2021, 48, e2020GL089957. [Google Scholar] [CrossRef]
- Kogure, M.; Liu, H.; Tao, C. Mechanisms for Zonal Mean Wind Responses in the Thermosphere to Doubled CO2 Concentration. J. Geophys. Res. Space Phys. 2022, 127. [Google Scholar] [CrossRef]
- Mlynczak, M.G.; Hunt, L.A.; Thomas Marshall, B.; Martin-Torres, F.J.; Mertens, C.J.; Russell, J.M., III; Remsberg, E.E.; López-Puertas, M.; Picard, R.; Winick, J.; et al. Observations of infrared radiative cooling in the thermosphere on daily to mul-tiyear timescales from the TIMED/SABER instrument. J. Geophys. Res. Space Phys. 2010, 115. [Google Scholar] [CrossRef]
- Liu, H.; Tao, C.; Jin, H.; Abe, T. Geomagnetic activity effects on CO2-driven trend in the thermosphere and ionosphere: Ideal model experiments with GAIA. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028607. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, R.; Gu, S.; Dou, X.; Li, N. Tidal Structures in the Mesosphere and Lower Thermosphere and Their Solar Cycle Variations. Atmosphere 2022, 13, 2036. https://doi.org/10.3390/atmos13122036
Sun R, Gu S, Dou X, Li N. Tidal Structures in the Mesosphere and Lower Thermosphere and Their Solar Cycle Variations. Atmosphere. 2022; 13(12):2036. https://doi.org/10.3390/atmos13122036
Chicago/Turabian StyleSun, Ruidi, Shengyang Gu, Xiankang Dou, and Na Li. 2022. "Tidal Structures in the Mesosphere and Lower Thermosphere and Their Solar Cycle Variations" Atmosphere 13, no. 12: 2036. https://doi.org/10.3390/atmos13122036
APA StyleSun, R., Gu, S., Dou, X., & Li, N. (2022). Tidal Structures in the Mesosphere and Lower Thermosphere and Their Solar Cycle Variations. Atmosphere, 13(12), 2036. https://doi.org/10.3390/atmos13122036






