Features of K-Changes Observed in Sri Lanka in the Tropics
Abstract
:1. Introduction
2. Chaotic Pulse Trains and Regular Pulse Trains
3. Experimental Setup
4. Results
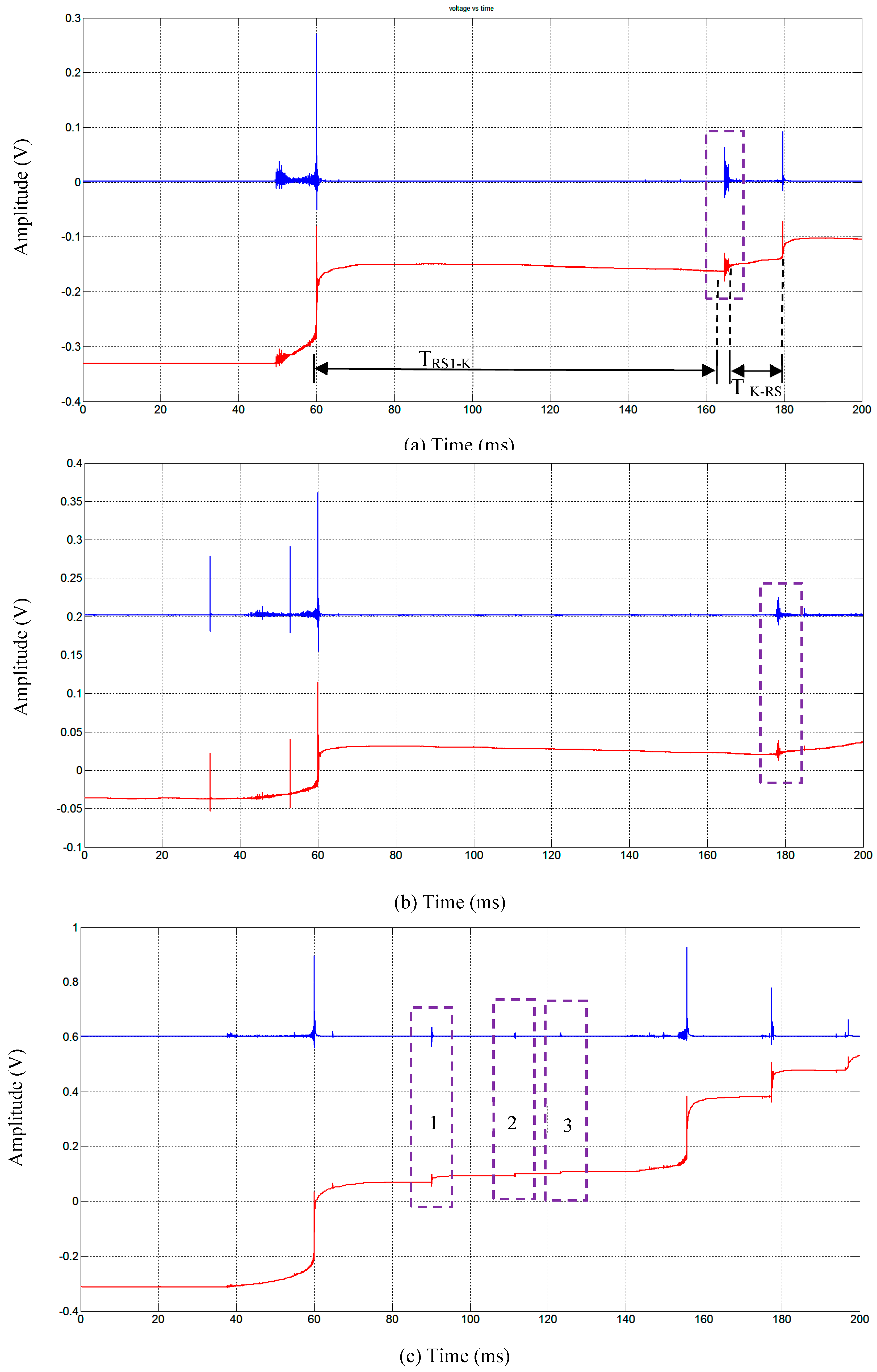
4.1. Characteristics of K-Changes
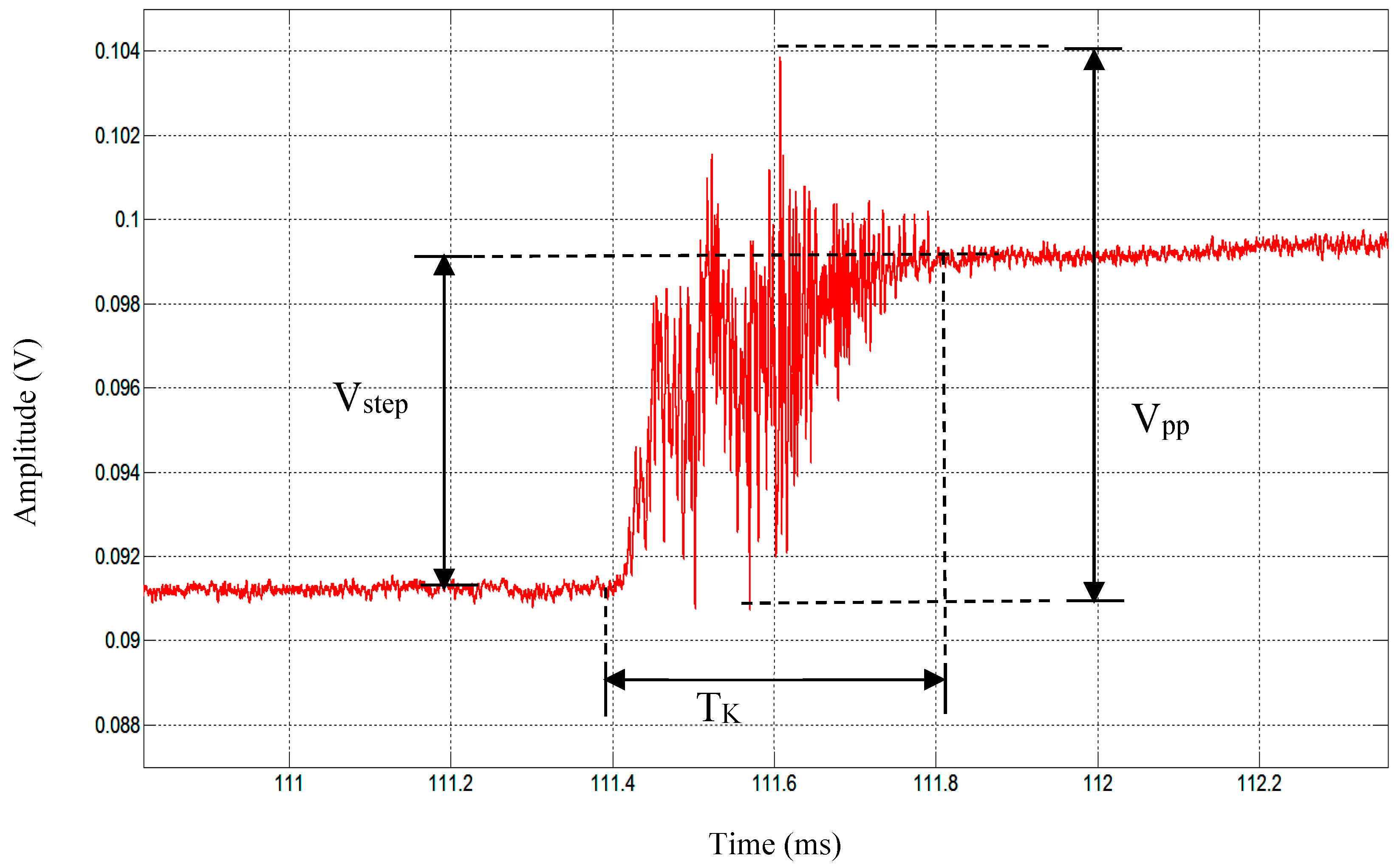
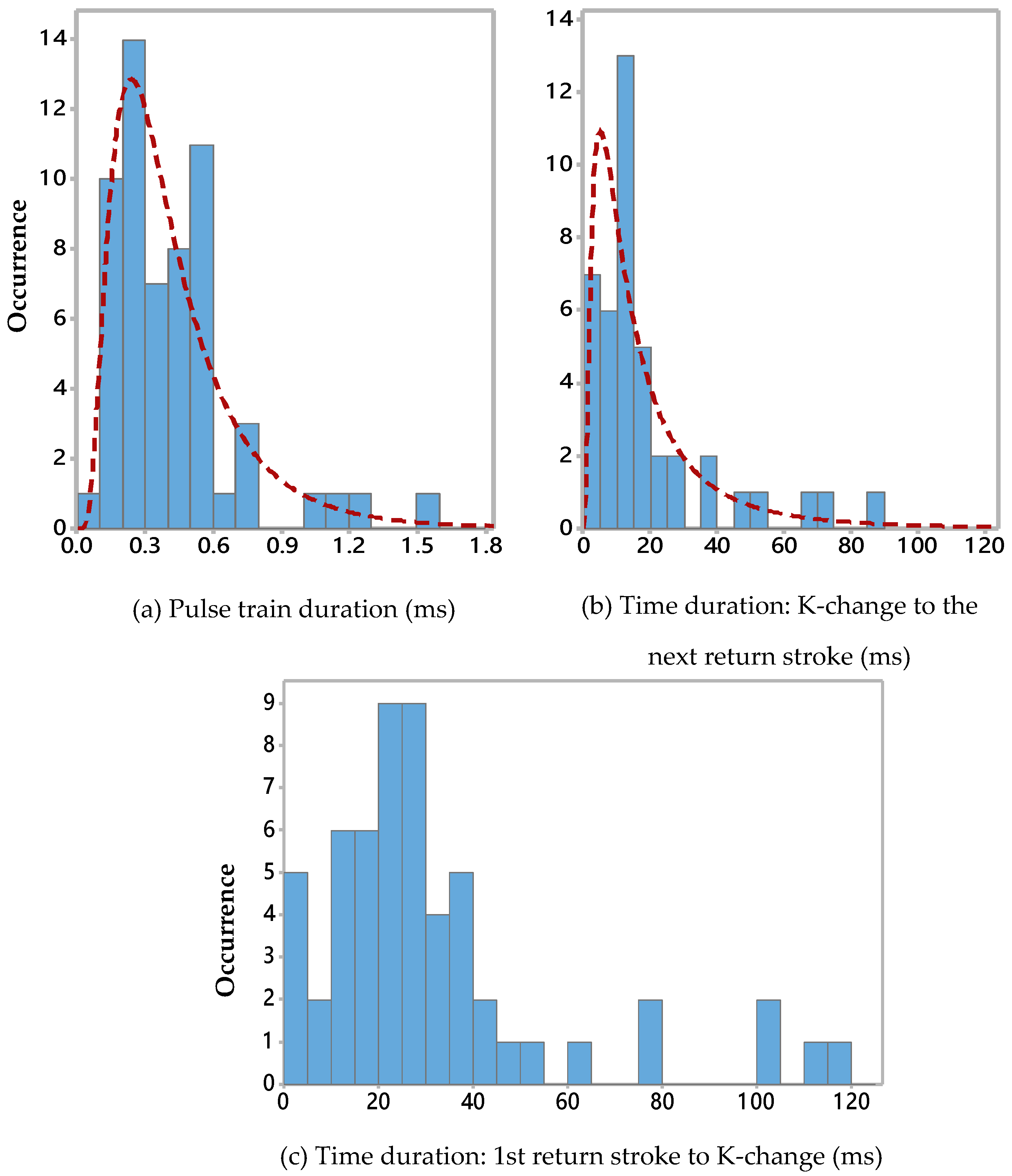
4.2. Parameters of Isolated K-Changes
4.3. Parameters of Consecutive K-Changes
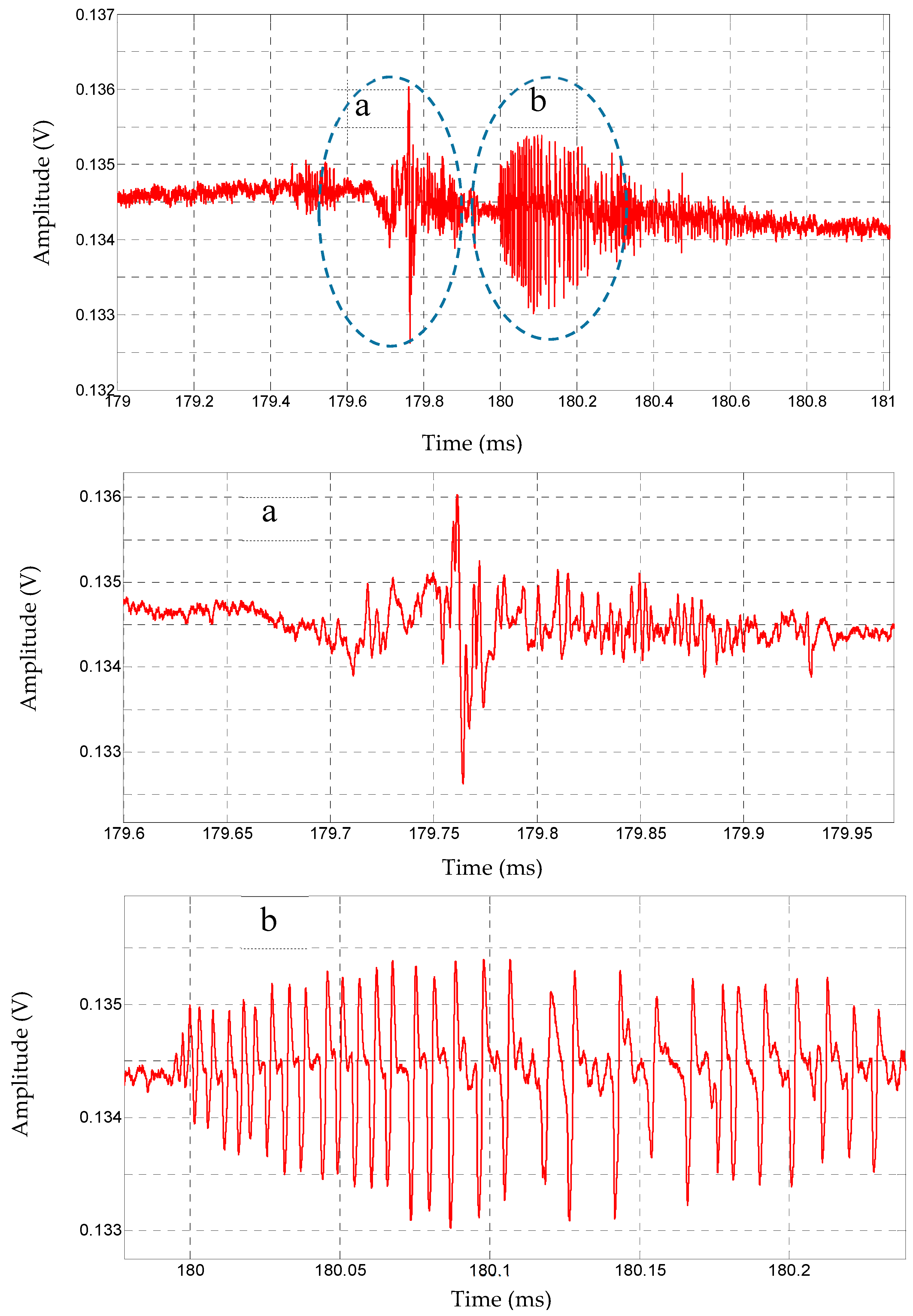
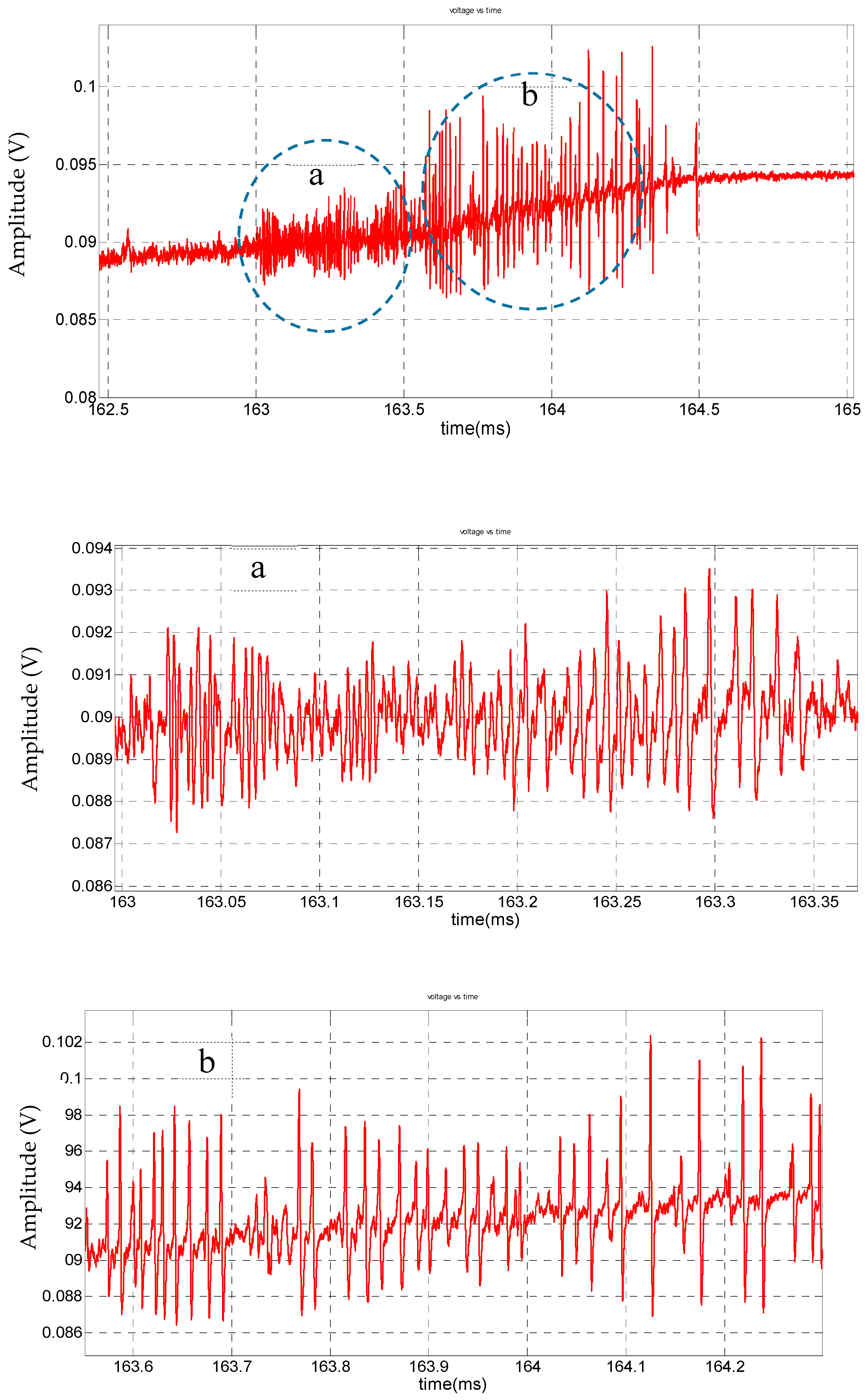
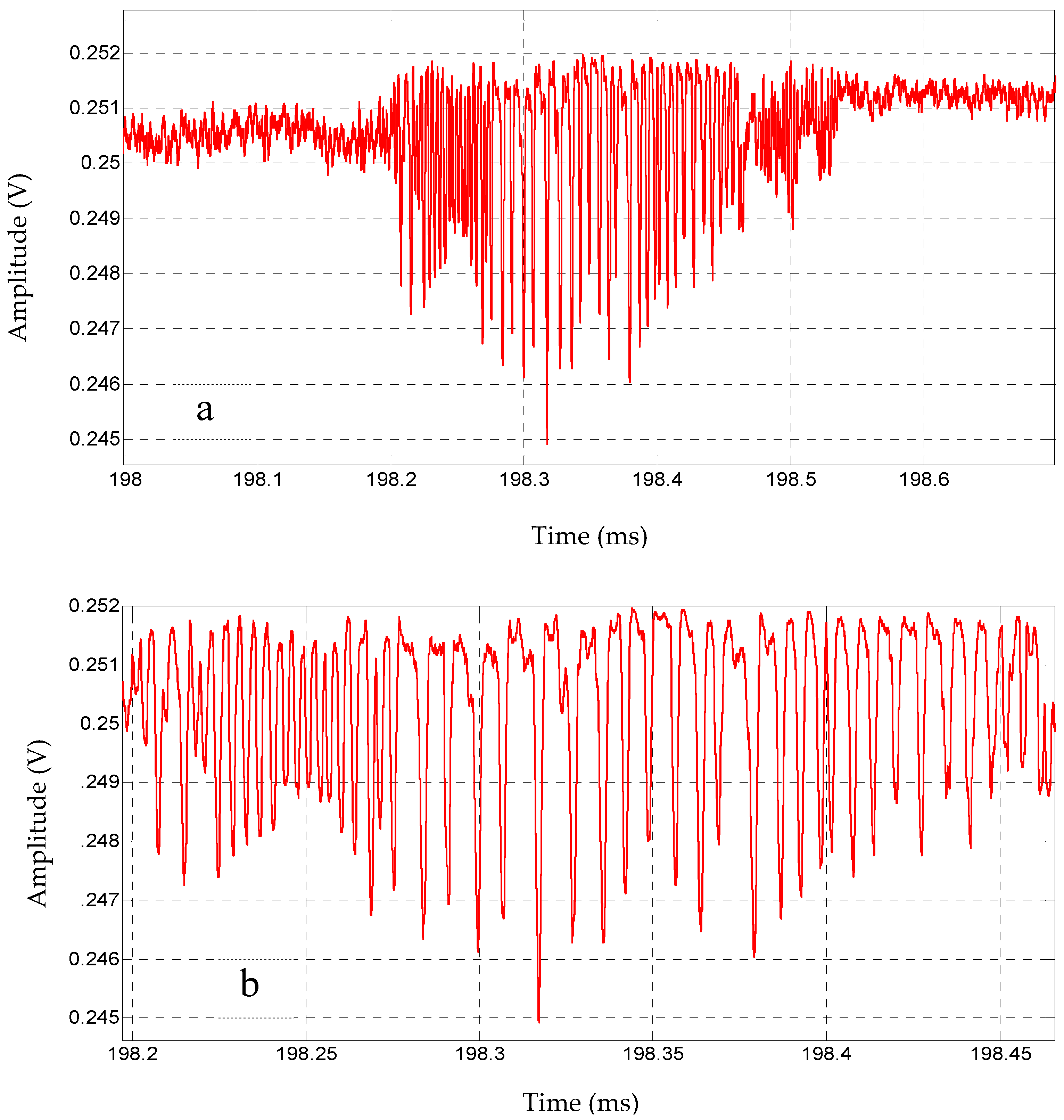
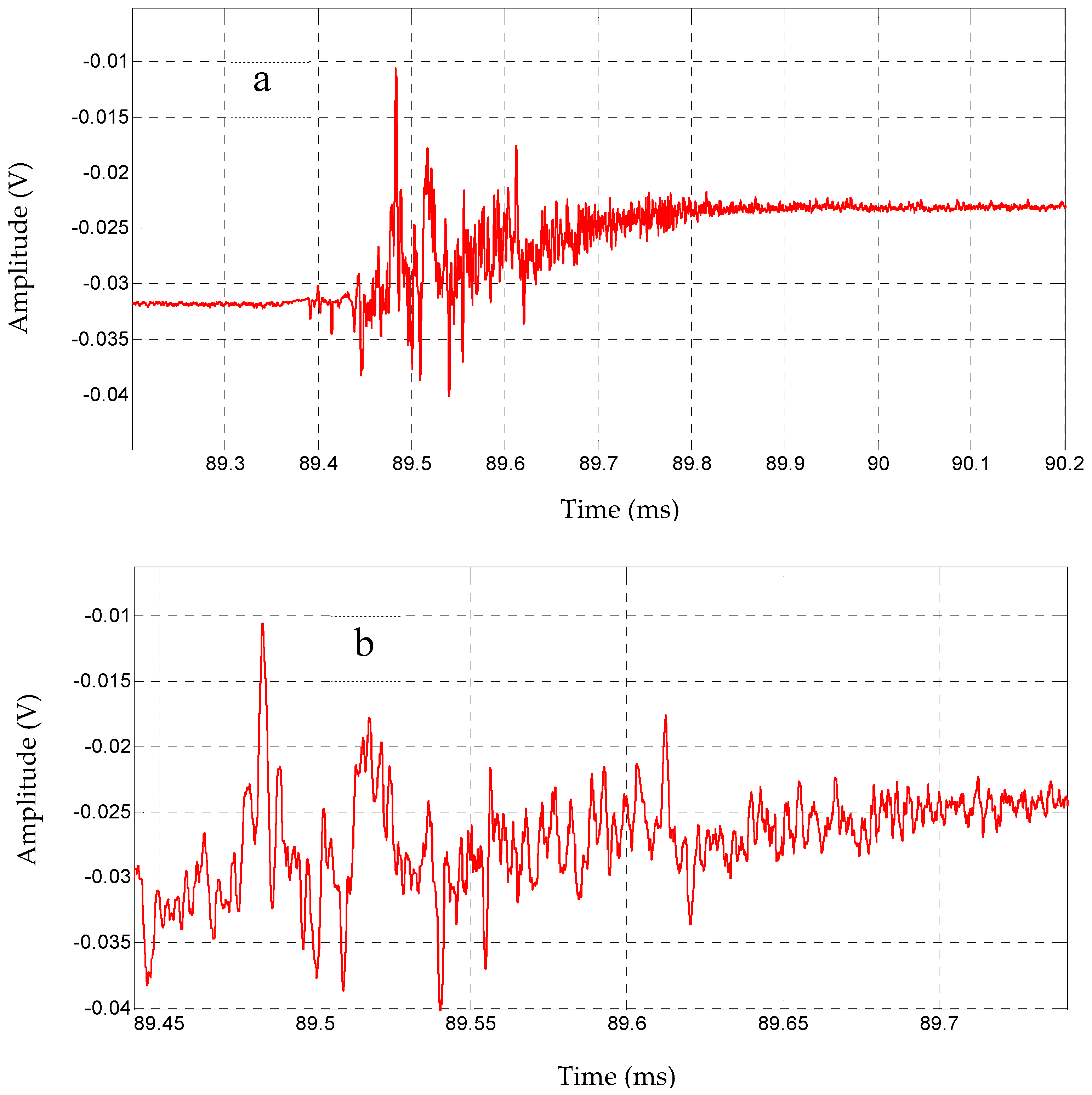
4.4. Fine Structure of K-Change Electric Fields
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kitagawa, N. On the mechanism of cloud flash and junction or final process in flash to ground. Pap. Meteorol. Geophys. 1957, 7, 415–424. [Google Scholar] [CrossRef]
- Kitagawa, N.; Kobayashi, M. Field Changes and Variations of Luminosity due to Lightning Flashes in Recent Advances in Atmospheric Electricity; Smith, L.G., Ed.; Pergamon: Oxford, UK, 1959; pp. 485–501. [Google Scholar]
- Kitagawa, N.; Brook, M.A. Comparison of intracloud and cloud-to-ground lightning discharges. J. Geophys. Res. 1960, 65, 1189–1201. [Google Scholar] [CrossRef]
- Brook, M.; Kitagawa, N. Radiation from lightning discharges in the frequency range 400 to 1000 Mc/s. J. Geophys. Res. 1964, 69, 2431–2434. [Google Scholar] [CrossRef]
- Ogawa, T.; Brook, M. The mechanism of the intracloud lightning discharge. J. Geophys. Res. 1964, 69, 5141–5150. [Google Scholar] [CrossRef]
- Mazur, V.; Ruhnke, L.H.; Warner, T.A.; Orville, R.E. Recoil leader formation and development. J. Electrostat. 2013, 71, 763–768. [Google Scholar] [CrossRef] [Green Version]
- Shao, X.M.; Krehbiel, P.R.; Thomas, R.J.; Rison, W. Radio interferometric observations of cloud-to-ground lightning phenomena in Florida. J. Geophys. Res. 1995, 100, 2749–2783. [Google Scholar] [CrossRef]
- Rakov, V.A.; Thottappillil, R.; Uman, M.A. Electric field pulses in K and M changes of lightning ground flashes. J. Geophys. Res. 1992, 97, 9935–9950. [Google Scholar] [CrossRef]
- Rakov, V.A.; Uman, M.A.; Hoffman, G.R.; Masters, M.W.; Brook, M. Bursts of pulses in lightning electromagnetic radiation observations and implications for lightning test standards. IEEE Trans. 1996, 38, 156–164. [Google Scholar] [CrossRef]
- Krider, E.P.; Radda, G.J.; Noggle, R.C. Regular radiation field pulses produced by intracloud lightning discharges. J. Geophys. Res. 1975, 80, 3801–3804. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, G.; Zhang, T.; Li, Y.; Zhao, Y.; Zhang, X.; Wu, B. The Regular Pulses Bursts in Electromagnetic Radiation from Lightning; APEMC: Beijing, China, 2010. [Google Scholar]
- Davis, S.M. Properties of Lightning Discharges from Multiple Station Wideband Measurements. Ph.D. Thesis, University of Florida, Gainesville, FL, USA, 1999. [Google Scholar]
- Kolmasová, I.; Santolík, O. Properties of unipolar magnetic field pulse trains generated by lightning discharges. Geophys. Res. Lett. 2013, 40, 1637–1641. [Google Scholar] [CrossRef] [Green Version]
- Weidman, C.D. The Sub-Microsecond Structure of Lightning Radiation Fields. Ph.D. Thesis, University of Arizona, Tucson, AZ, USA, 1982. [Google Scholar]
- Gomes, C.; Cooray, V.; Fernando, M.; Montano, R.; Sonnadara, U. Characteristics of chaotic pulse trains generated by lightning flashes. J. Atmos. Sol. Terr. Phys. 2004, 66, 1733–1743. [Google Scholar] [CrossRef]
- Ismail, M.M.; Rahman, M.; Cooray, V.; Fernando, M.; Hettiarachchi, P.; Johari, D. On the Possible Origin of Chaotic Pulse Trains in Lightning Flashes. Atmosphere 2017, 8, 29. [Google Scholar] [CrossRef]
- Galvan, A.; Fernando, M. Operational Characteristics of a Parallel-Plate Antenna to Measure Vertical Electric Fields from Lightning Flashes; Uppsala University: Uppsala, Sweden, 2000; pp. 2–17. [Google Scholar]
- Ye, M.; Cooray, V. Propagation effects caused by a rough ocean surface on the electromagnetic fields generated by lightning return strokes. Radio Sci. 1994, 29, 73–85. [Google Scholar]
- Cooray, V.; Ye, M. Propagation effects on the lightning-generated electromagnetic fields for homogeneous and mixed sea land paths. J. Geophys. Res. 1994, 99, 10641–10652. [Google Scholar] [CrossRef]
- Thottappillil, R.; Rakov, V.A.; Uman, M.A. K and M changes in close lightning ground flashes in Florida. J. Geophys. Res. 1990, 95, 18631–18640. [Google Scholar] [CrossRef]
- Miranda, F.J.; Pinto Jr, O.; Saba, M.M.F. A study of the time-interval between return strokes and K-changes of negative cloud-to-ground lightning flashes in Brazil. Atmos. Sol. Terr. Phys. 2003, 65, 293–297. [Google Scholar] [CrossRef]
- Stolzenburg, M.; Marshall, C.M.; Karunarathne, S.; Karunarathna, N.; Warner, T.A.; Orville, R.E. Stepped-to-dart leaders preceding lightning return strokes. J. Geophys. Res. 2013, 118, 9845–9869. [Google Scholar] [CrossRef] [Green Version]


| Total No. of Flashes | No. of Flashes with K-Changes | Total No. of K-Changes | No. of Flashes with Consecutive K-Changes | No. of Consecutive K-Changes | No. of Isolated K-Changes |
|---|---|---|---|---|---|
| 1106 | 98 | 165 | 53 | 120 | 45 |
| Parameter | Geometric Mean | St. Deviation | Minimum | Maximum | Typical Value |
|---|---|---|---|---|---|
| TK (ms) | 0.43 | 0.3 | 0.08 | 1.599 | 0.2–0.3 |
| TRS1-K (ms) | 24.6 | 27.0 | 1.9 | 118.1 | 20–30 |
| TK-RS (ms) | 19.33 | 19.59 | 0.8 | 87.1 | 10–15 |
| Parameter | Average | Geometric Mean | Standard Deviation | Min. | Max. | Typical Value | Distribution |
|---|---|---|---|---|---|---|---|
| TR1-K1 (ms) | 35.9 | 30.3 | 21.4 | 3.4 | 104.3 | 18–24 | Lognormal |
| TK (ms) | 0.45 | 0.38 | 0.29 | 0.045 | 1.65 | 0.3–0.4 | Lognormal |
| TK-R (ms) | 20.2 | 12.3 | 18.5 | 1.7 | 71.3 | 0–5 | Exponential |
| TK-K (ms) | 20.27 | 11.78 | 26.84 | 0.67 | 198.50 | 0–10 | Lognormal |
| Study | TK (ms) | TK-K (ms) | Distribution | |||
|---|---|---|---|---|---|---|
| Geometric Mean | Typical Value | Average | Geometric Mean | Typical Value | ||
| (Kitagawa & Brook) [3] | - | - | 8.50 | - | 4–6 | Lognormal |
| (Kitagawa 1957) [1] | - | 0.20–0.40 | - | - | 8–16 | - |
| (Brook & Kitagawa 1964) [4] | - | 0.50–0.75 | - | - | - | - |
| (Thottapillil et al.) [20] | 0.70 | 0.40–0.60 | - | 12.50 | 10–15 | - |
| (Miranda et al.) [21] | - | - | 18.50 | 12 | 10–16 | Lognormal |
| This study | 0.38 | 0.3 –0.4 | 20.27 | 11.78 | 0–10 | Lognormal/Exponential |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nanayakkara, S.; Fernando, M.; Cooray, V. Features of K-Changes Observed in Sri Lanka in the Tropics. Atmosphere 2019, 10, 141. https://doi.org/10.3390/atmos10030141
Nanayakkara S, Fernando M, Cooray V. Features of K-Changes Observed in Sri Lanka in the Tropics. Atmosphere. 2019; 10(3):141. https://doi.org/10.3390/atmos10030141
Chicago/Turabian StyleNanayakkara, Sankha, Mahendra Fernando, and Vernon Cooray. 2019. "Features of K-Changes Observed in Sri Lanka in the Tropics" Atmosphere 10, no. 3: 141. https://doi.org/10.3390/atmos10030141
APA StyleNanayakkara, S., Fernando, M., & Cooray, V. (2019). Features of K-Changes Observed in Sri Lanka in the Tropics. Atmosphere, 10(3), 141. https://doi.org/10.3390/atmos10030141





