Estimation of Soil Evaporation in Apple Orchards Based on Hydrogen and Oxygen Isotopes
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area
2.2. Experimental Design
2.3. Data Acquisition
2.3.1. Soil Sample Collection
2.3.2. Precipitation Sample Collection
2.4. Determination of Stable Isotopes
2.5. Linear Conditions’ Excess
2.6. Soil Evaporation Loss Rate
2.7. Water Balance Analysis
3. Results and Analysis
3.1. Effect of Extreme Precipitation on Soil Evaporation in Apple Orchards
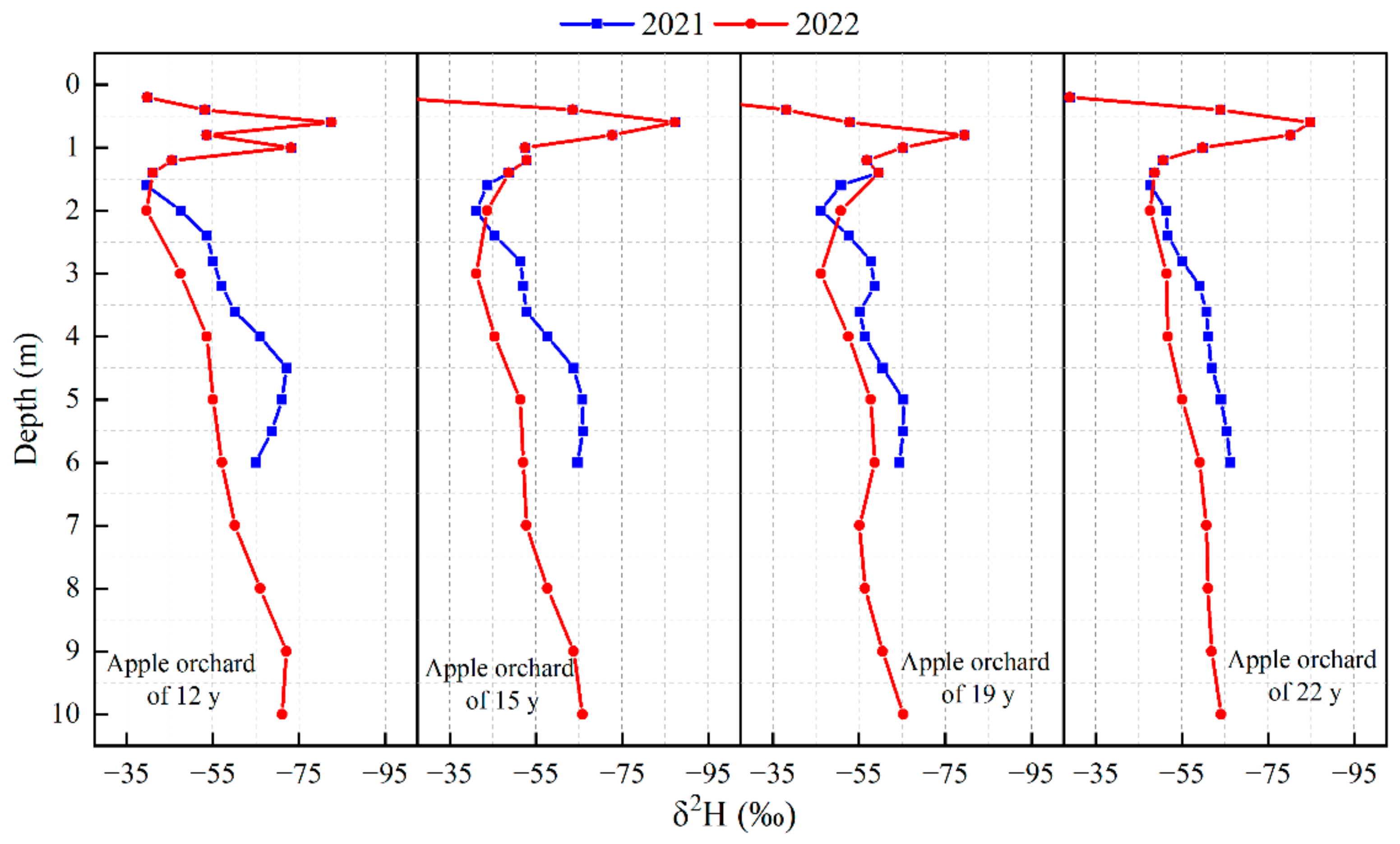
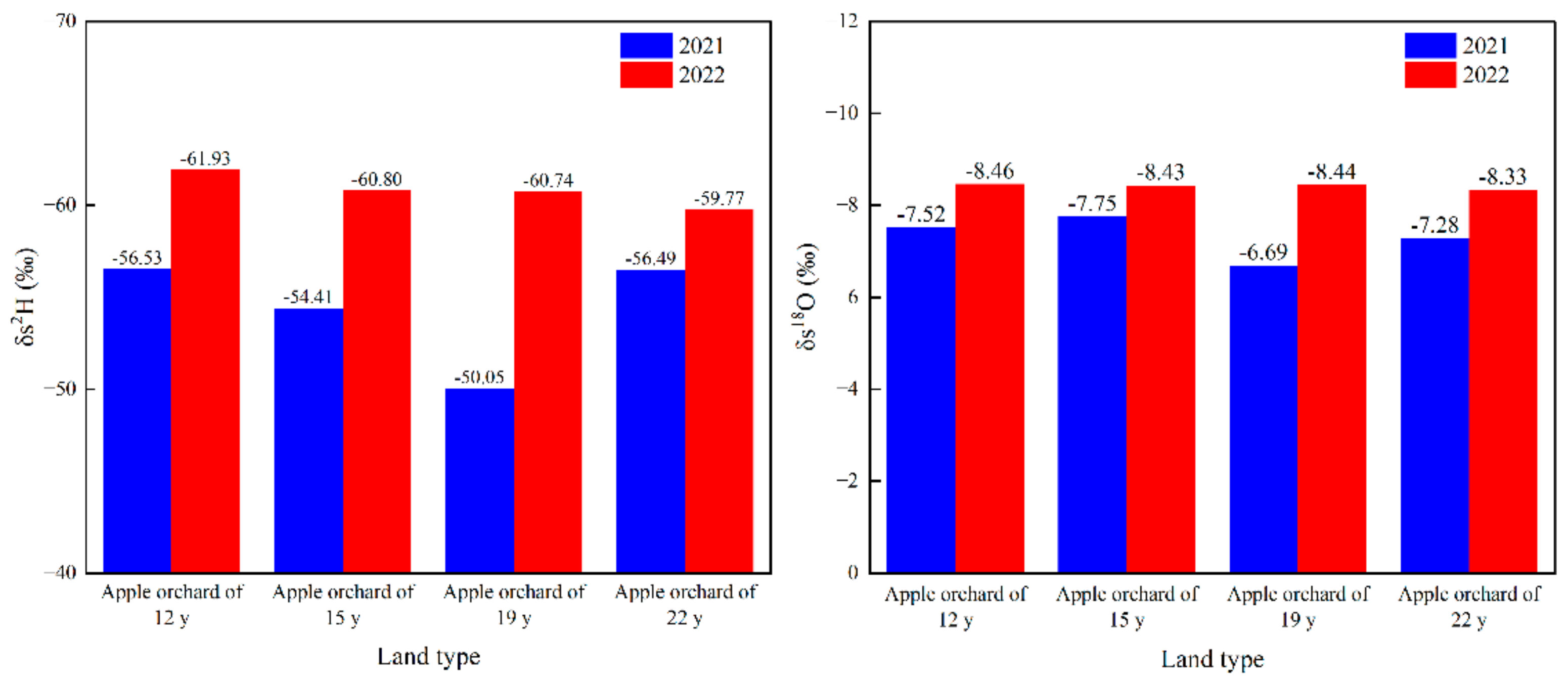
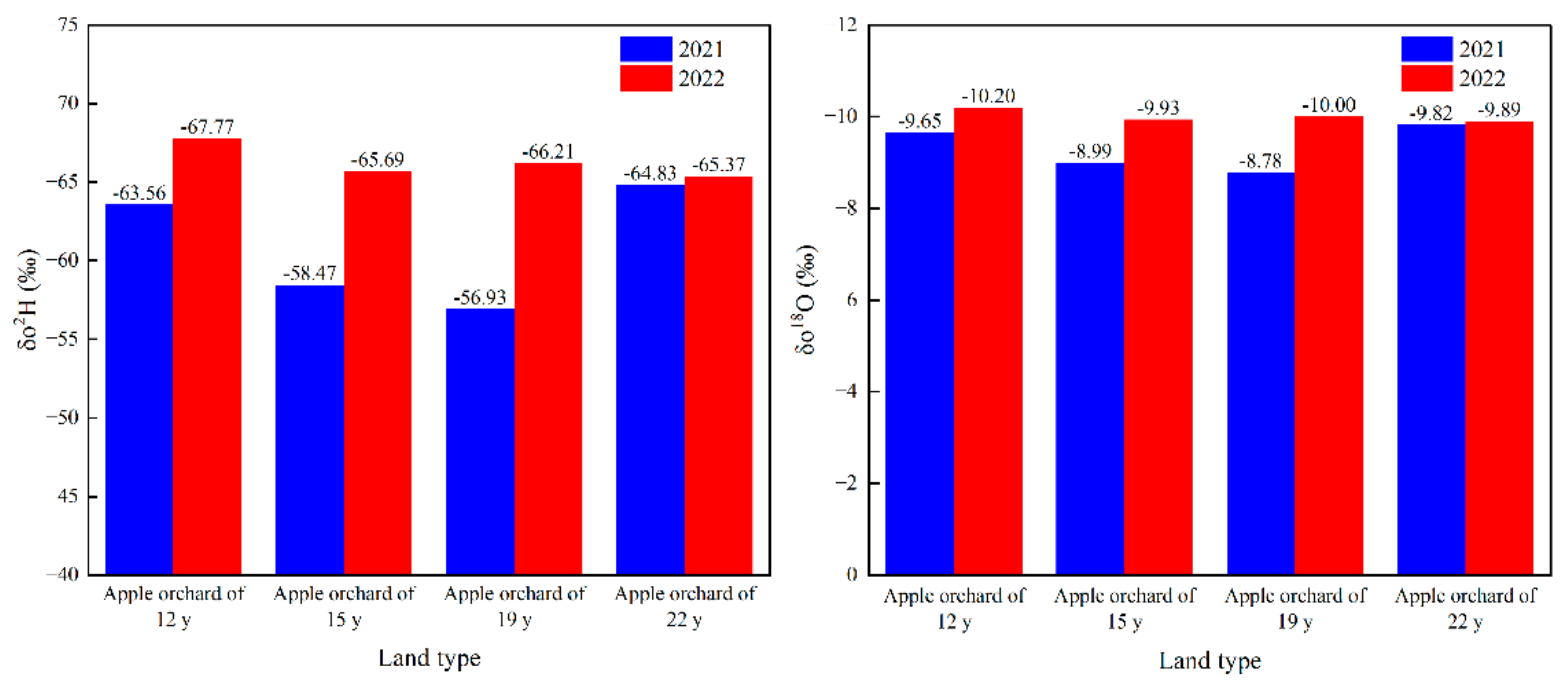
3.1.1. Trend of Stable Isotopes’ Characteristics with Age in Apple Orchards
3.1.2. Characteristics of Evaporative Fractionation of Stable Hydrogen and Oxygen Isotopes and Its Relationship with Age in Apple Orchards
3.2. Estimation of Soil Evaporation Based on Craig–Gordon Model
3.2.1. Soil Evaporation Loss Rate Based on Craig–Gordon Model
3.2.2. Calculation of Soil Evaporation Based on the Soil Evaporation Loss Rate
3.3. Estimation and Analysis of Plant Transpiration and Soil Transpiration
3.3.1. Division of Plant Transpiration According to Water Balance Analysis
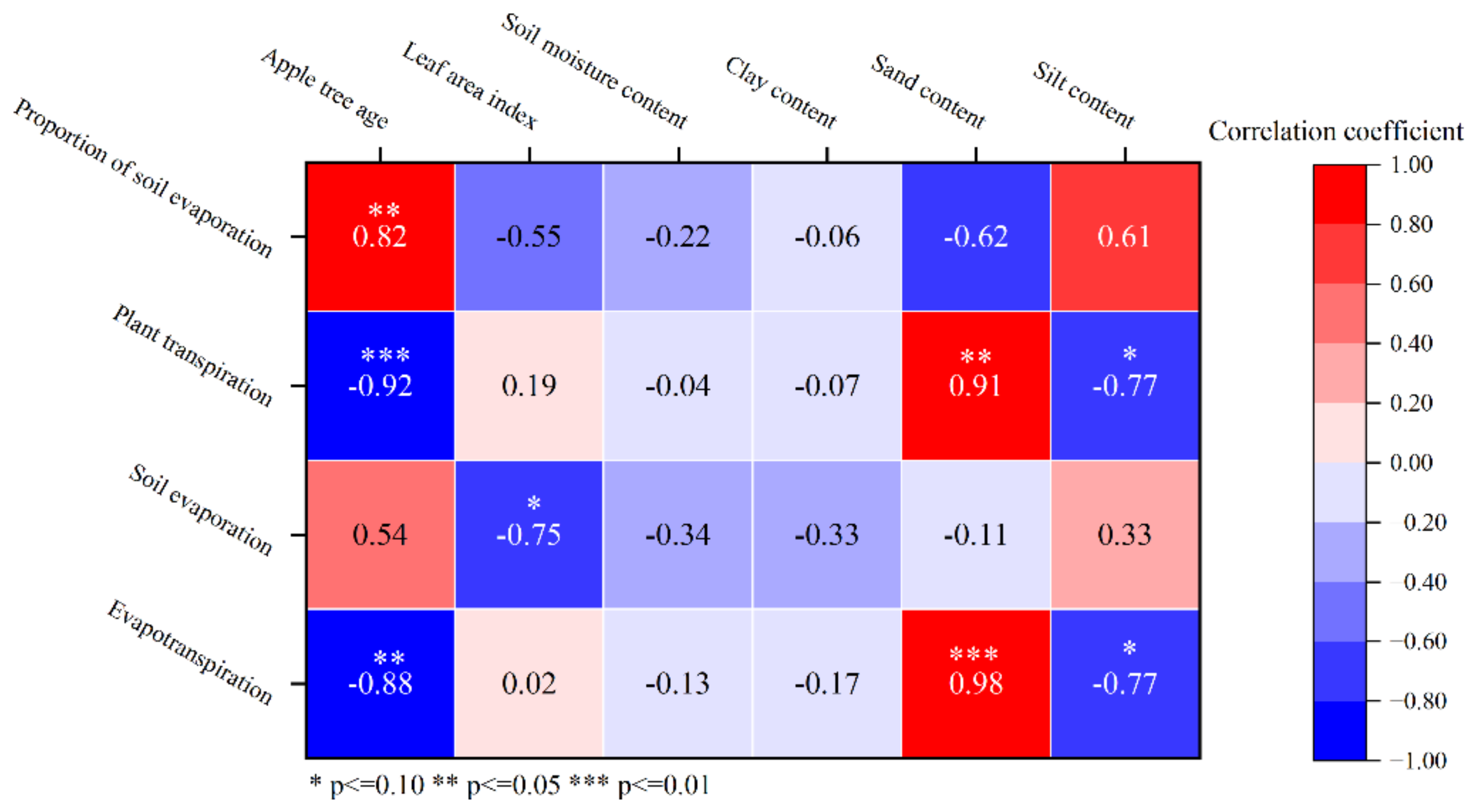
3.3.2. Correlation Analysis
4. Discussion
4.1. Observation Trend Analysis of Soil Evaporation Loss Rate
4.2. Selection of Input Parameters for the Craig–Gordon Model
4.3. Differences in Soil Evaporation Loss Rate Based on δ2H and δ18O
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Coenders-Gerrits, A.M.J.; van der Ent, R.J.; Bogaard, T.A.; Wang-Erlandsson, L.; Hrachowitz, M.; Savenije, H.H.G. Uncertainties in transpiration estimates. Nature 2014, 506, E1–E2. [Google Scholar] [CrossRef] [PubMed]
- Song, X.; Lyu, S.; Wen, X. Limitation of soil moisture on the response of transpiration to vapor pressure deficit in a subtropical coniferous plantation subjected to seasonal drought. J. Hydrol. 2020, 591, 125301. [Google Scholar] [CrossRef]
- Xu, S.; Yu, Z.; Ji, X.; Sudicky, E.A. Comparing three models to estimate transpiration of desert shrubs. J. Hydrol. 2017, 550, 603–615. [Google Scholar] [CrossRef]
- Ge, G.; Chaoying, H. Climate Change and Its Impact on Water Resources in North China. Adv. Atmos. Sci. 2001, 18, 718–732. [Google Scholar] [CrossRef]
- Zhao, M.; A, G.; Liu, Y.; Konings, A.G. Evapotranspiration frequently increases during droughts. Nat. Clim. Change 2022, 12, 1024–1030. [Google Scholar] [CrossRef]
- Chang, X.; Zhao, W.; He, Z. Radial pattern of sap flow and response to microclimate and soil moisture in Qinghai spruce (Picea crassifolia) in the upper Heihe River Basin of arid northwestern China. Agric. For. Meteorol. 2014, 187, 14–21. [Google Scholar] [CrossRef]
- Klapp, I.; Yafin, P.; Oz, N.; Brand, O.; Bahat, I.; Goldshtein, E.; Cohen, Y.; Alchanatis, V.; Sochen, N. Computational end-to-end and super-resolution methods to improve thermal infrared remote sensing for agriculture. Precis. Agric. 2021, 22, 452–474. [Google Scholar] [CrossRef]
- Rafi, Z.; Merlin, O.; Le Dantec, V.; Khabba, S.; Mordelet, P.; Er-Raki, S.; Amazirh, A.; Olivera-Guerra, L.; Hssaine, B.A.; Simonneaux, V.; et al. Partitioning evapotranspiration of a drip-irrigated wheat crop: Inter-comparing eddy covariance-, sap flow-, lysimeter- and FAO-based methods. Agric. For. Meteorol. 2019, 265, 310–326. [Google Scholar] [CrossRef]
- López-López, M.; Espadafor, M.; Testi, L.; Lorite, I.J.; Orgaz, F.; Fereres, E. Water requirements of mature almond trees in response to atmospheric demand. Irrig. Sci. 2018, 36, 271–280. [Google Scholar] [CrossRef]
- Fernández-Martínez, M.; Sardans, J.; Musavi, T.; Migliavacca, M.; Iturrate-Garcia, M.; Scholes, R.J.; Peñuelas, J.; Janssens, I.A. The role of climate, foliar stoichiometry and plant diversity on ecosystem carbon balance. Glob. Change Biol. 2020, 26, 7067–7078. [Google Scholar] [CrossRef]
- Zhang, D.; Zhou, G. Estimation of Soil Moisture from Optical and Thermal Remote Sensing: A Review. Sensors 2016, 16, 1308. [Google Scholar] [CrossRef] [PubMed]
- Seymour, V.; Hsiao, T. A soil surface psychrometer for measuring humidity and studying evaporation. Agric. For. Meteorol. 1984, 32, 61–70. [Google Scholar] [CrossRef]
- Wu, X.; Chen, F.; Liu, X.; Wang, S.; Zhang, M.; Zhu, G.; Zhou, X.; Chen, J. The Significance of Hydrogen and Oxygen Stable Isotopes in the Water Vapor Source in Dingxi Area. Water 2021, 13, 2374. [Google Scholar] [CrossRef]
- Tritschler, F.; Binder, M.; Händel, F.; Burghardt, D.; Dietrich, P.; Liedl, R. Collected Rain Water as Cost-Efficient Source for Aquifer Tracer Testing. Groundwater 2020, 58, 125–131. [Google Scholar] [CrossRef] [PubMed]
- Yong, L.; Zhu, G.; Wan, Q.; Xu, Y.; Zhang, Z.; Sun, Z.; Ma, H.; Sang, L.; Liu, Y.; Guo, H.; et al. The Soil Water Evaporation Process from Mountains Based on the Stable Isotope Composition in a Headwater Basin and Northwest China. Water 2020, 12, 2711. [Google Scholar] [CrossRef]
- Oerter, E.J.; Bowen, G.J. Spatio-temporal heterogeneity in soil water stable isotopic composition and its ecohydrologic implications in semiarid ecosystems. Hydrol. Process. 2019, 33, 1724–1738. [Google Scholar] [CrossRef]
- Li, Y.; Huo, S.; Guo, J.; Sun, J.; Pan, J.; Wang, D.; Tan, Q.; Pei, B. Using hydrogen and oxygen stable isotopes to estimate soil water evaporation loss under continuous evaporation conditions. Hydrol. Process. 2023, 37, e14885. [Google Scholar] [CrossRef]
- Wang, P.; Yamanaka, T.; Li, X.-Y.; Wei, Z. Partitioning evapotranspiration in a temperate grassland ecosystem: Numerical modeling with isotopic tracers. Agric. For. Meteorol. 2015, 208, 16–31. [Google Scholar] [CrossRef]
- Good, S.P.; Soderberg, K.; Guan, K.; King, E.G.; Scanlon, T.M.; Caylor, K.K. δ2H isotopic flux partitioning of evapotranspiration over a grass field following a water pulse and subsequent dry down. Water Resour. Res. 2014, 50, 1410–1432. [Google Scholar] [CrossRef]
- Wei, Z.; Lee, X.; Wen, X.; Xiao, W. Evapotranspiration partitioning for three agro-ecosystems with contrasting moisture conditions: A comparison of an isotope method and a two-source model calculation. Agric. For. Meteorol. 2018, 252, 296–310. [Google Scholar] [CrossRef]
- Ma, Y.; Song, X. Applying stable isotopes to determine seasonal variability in evapotranspiration partitioning of winter wheat for optimizing agricultural management practices. Sci. Total. Environ. 2019, 654, 633–642. [Google Scholar] [CrossRef] [PubMed]
- Ye, S.; Li, J.; Kong, H.; Shen, J.; Wu, D. Effects of varying mulch materials on the photosynthetic characteristics, yield, and soil water use efficiency of wheat in Loess tableland. Sci. Rep. 2023, 13, 18106. [Google Scholar] [CrossRef] [PubMed]
- Zhao, C.; Jia, X.; Zhu, Y.; Shao, M. Long-term temporal variations of soil water content under varying vegetation types in the Loess Plateau, China. CATENA 2017, 158, 55–62. [Google Scholar] [CrossRef]
- Luan, J.; Zhang, Y.; Li, X.; Ma, N.; Naeem, S.; Xu, Z.; He, S.; Miao, P.; Tian, X.; Wang, R. Unexpected consequences of large-scale ecological restoration: Groundwater declines are reversed. Ecol. Indic. 2023, 155, 111008. [Google Scholar] [CrossRef]
- Yang, M.; Wang, S.; Zhao, X.; Gao, X.; Liu, S. Soil properties of apple orchards on China’s Loess Plateau. Sci. Total Environ. 2020, 723, 138041. [Google Scholar] [CrossRef]
- Li, W.; Gao, J.; Zhang, Y.; Ahmad, R.; Gao, Z.; Zhou, F. Effects of Planting Practices on Soil Organic Carbon during Old Apple Orchards’ Reconstruction on the Loess Plateau. Agronomy 2023, 13, 897. [Google Scholar] [CrossRef]
- Lu, Y.; Li, H.; Si, B.; Li, M. Chloride tracer of the loess unsaturated zone under sub-humid region: A potential proxy recording high-resolution hydroclimate. Sci. Total Environ. 2020, 700, 134465. [Google Scholar] [CrossRef]
- Tao, G.; Lei, D.; Liu, L.; Li, Y.; Zhu, X. Prediction of Soil Water Characteristic Curve Based on Soil Water Evaporation. Adv. Civ. Eng. 2021, 2021, 6686442. [Google Scholar] [CrossRef]
- Wei, H.; Lu, Y.; Bai, L.; Niu, J.; Chen, S.; Mojid, M.A.; Yang, Y.; Li, M. Water Uptake Characteristics of Stipa bungeana Trin: Affected by Subsidence in the Coal Mining Areas of Northwest China. Agronomy 2024, 14, 424. [Google Scholar] [CrossRef]
- Chen, K.; Liu, G.; Xia, C.; Meng, Y.; Tetzlaff, D.; Zhong, Q.; Chang, J. Water cycling and partitioning through the soil–plant–atmosphere continuum in a subtropical, urban woodland inferred by water stable isotopes. Hydrol. Process. 2022, 36, e14746. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, J.; Wang, S.; Zhou, T. A simplified method of developing a local meteoric water line equation for Beijing, China. J. Radioanal. Nucl. Chem. 2018, 315, 523–532. [Google Scholar] [CrossRef]
- Sprenger, M.; Tetzlaff, D.; Soulsby, C. Soil water stable isotopes reveal evaporation dynamics at the soil–plant–atmosphere interface of the critical zone. Hydrol. Earth Syst. Sci. 2017, 21, 3839–3858. [Google Scholar] [CrossRef]
- Sprenger, M.; Tetzlaff, D.; Tunaley, C.; Dick, J.; Soulsby, C. Evaporation fractionation in a peatland drainage network affects stream water isotope composition. Water Resour. Res. 2017, 53, 851–866. [Google Scholar] [CrossRef]
- Gonfiantini, R.; Wassenaar, L.I.; Araguas-Araguas, L.J. Stable isotope fractionations in the evaporation of water: The wind effect. Hydrol. Process. 2020, 34, 3596–3607. [Google Scholar] [CrossRef]
- Lu, Y.; Wen, M.; Li, P.; Liang, J.; Wei, H.; Li, M. An Improved Craig–Gordon Isotopic Model: Accounting for Transpiration Effects on the Isotopic Composition of Residual Water during Evapotranspiration. Agronomy 2023, 13, 1531. [Google Scholar] [CrossRef]
- Gibson, J.J.; Birks, S.J.; Edwards, T.W.D. Global prediction of δA and δ2H-δ18O evaporation slopes for lakes and soil water accounting for seasonality. Glob. Biogeochem. Cycles 2008, 22, 191. [Google Scholar] [CrossRef]
- Gonfiantini, R.; Wassenaar, L.I.; Araguas-Araguas, L.; Aggarwal, P.K. A unified Craig-Gordon isotope model of stable hydrogen and oxygen isotope fractionation during fresh or saltwater evaporation. Geochim. Cosmochim. Acta 2018, 235, 224–236. [Google Scholar] [CrossRef]
- Loucos, K.E.; Simonin, K.A.; Song, X.; Barbour, M.M. Observed relationships between leaf H218O Peclet effective length and leaf hydraulic conductance reflect assumptions in Craig-Gordon model calculations. Tree Physiol. 2015, 35, 16–26. [Google Scholar] [CrossRef]
- Smith, D.F.; Saelens, E.; Leslie, D.L.; Carey, A.E. Local meteoric water lines describe extratropical precipitation. Hydrol. Process. 2021, 35, e14059. [Google Scholar] [CrossRef]
- Du, Y.; Berndtsson, R.; An, D.; Zhang, L.; Hao, Z.; Yuan, F. Hydrologic Response of Climate Change in the Source Region of the Yangtze River, Based on Water Balance Analysis. Water 2017, 9, 115. [Google Scholar] [CrossRef]
- Sun, S.; Meng, P.; Zhang, J.; Wan, X.; Zheng, N.; He, C. Partitioning oak woodland evapotranspiration in the rocky mountainous area of North China was disturbed by foreign vapor, as estimated based on non-steady-state 18O isotopic composition. Agric. For. Meteorol. 2014, 184, 36–47. [Google Scholar] [CrossRef]
- Villegas, J.C.; Breshears, D.D.; Zou, C.B.; Royer, P.D. Seasonally Pulsed Heterogeneity in Microclimate: Phenology and Cover Effects along Deciduous Grassland–Forest Continuum. Vadose Zone J. 2010, 9, 537–547. [Google Scholar] [CrossRef]
- Lin, B.B. The role of agroforestry in reducing water loss through soil evaporation and crop transpiration in coffee agroecosystems. Agric. For. Meteorol. 2010, 150, 510–518. [Google Scholar] [CrossRef]
- Tesfuhuney, W.A.; Mengistu, A.G.; Van Rensburg, L.D.; Walker, S. Estimating soil water evaporation as influenced by “dry-and green-mulch” cover beneath maize canopy. Phys. Chem. Earth Parts A/B/C 2022, 128, 103270. [Google Scholar] [CrossRef]
- Di, N.; Wang, Y.; Clothier, B.; Liu, Y.; Jia, L.; Xi, B.; Shi, H. Modeling soil evaporation and the response of the crop coefficient to leaf area index in mature Populus tomentosa plantations growing under varying soil water availabilities. Agric. For. Meteorol. 2019, 264, 125–137. [Google Scholar] [CrossRef]
- Wang, H.X.; Liu, C.M. Soil Evaporation and its Affecting Factors under Crop Canopy. Commun. Soil Sci. Plant Anal. 2007, 38, 259–271. [Google Scholar] [CrossRef]
- Lu, X.; Liang, L.L.; Wang, L.; Jenerette, G.D.; McCabe, M.F.; Grantz, D.A. Partitioning of evapotranspiration using a stable isotope technique in an arid and high temperature agricultural production system. Agric. Water Manag. 2017, 179, 103–109. [Google Scholar] [CrossRef]
- de Vries, J.J.; Simmers, I. Groundwater recharge: An overview of processes and challenges. Hydrogeol. J. 2002, 10, 5–17. [Google Scholar] [CrossRef]
- Dubbert, M.; Cuntz, M.; Piayda, A.; Maguás, C.; Werner, C. Partitioning evapotranspiration—Testing the Craig and Gordon model with field measurements of oxygen isotope ratios of evaporative fluxes. J. Hydrol. 2013, 496, 142–153. [Google Scholar] [CrossRef]
- Braud, I.; Bariac, T.; Gaudet, J.; Vauclin, M. SiSPAT-Isotope, a coupled heat, water and stable isotope (HDO and H218O) transport model for bare soil. Part I. Model description and first verifications. J. Hydrol. 2005, 309, 277–300. [Google Scholar] [CrossRef]
- Mahindawansha, A.; Külls, C.; Kraft, P.; Breuer, L. Investigating unproductive water losses from irrigated agricultural crops in the humid tropics through analyses of stable isotopes of water. Hydrol. Earth Syst. Sci. 2020, 24, 3627–3642. [Google Scholar] [CrossRef]
- Baram, S.; Kurtzman, D.; Dahan, O. Water percolation through a clayey vadose zone. J. Hydrol. 2012, 424–425, 165–171. [Google Scholar] [CrossRef]







| Land Type | Planting Year | Stand Age | Period | Latitude | Longitude |
|---|---|---|---|---|---|
| Apple orchard | 2008 | 12 y | Full bearing | 107°41′05.88″ | 35°14′58.72″ |
| 2005 | 15 y | Full bearing | 107°41′09.01″ | 35°14′52.18″ | |
| 2001 | 19 y | Senescence | 107°40′46.74″ | 35°14′34.12″ | |
| 1998 | 22 y | Senescence | 107°41′03.00″ | 35°14′08.82″ |
| Land Type | Sampling Depth (m) | |
|---|---|---|
| May 2021 | May 2021 | |
| Apple orchard of 12 y | 0~6 | 0~12 |
| Apple orchard of 15 y | 0~6 | 0~10 |
| Apple orchard of 19 y | 0~6 | 0~10 |
| Apple orchard of 22 y | 0~6 | 0~8 |
| Land Type | Leaf Area Index | Soil Moisture Content | Clay Content | Sand Content | Silt Content |
|---|---|---|---|---|---|
| - | % | % | % | % | |
| Apple orchard of 12 y | 2.01 | 0.22 | 18.74 | 11.94 | 69.32 |
| Apple orchard of 15 y | 2.88 | 0.236 | 18.91 | 10.14 | 70.95 |
| Apple orchard of 19 y | 2.59 | 0.24 | 16.91 | 10.89 | 72.2 |
| Apple orchard of 22 y | 2.14 | 0.227 | 18.76 | 8.94 | 72.31 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, J.; Hua, Y.; Zhang, L.; Yu, C.; Chen, K.; Gu, L.; Wu, Y.; Wei, H.; Li, M. Estimation of Soil Evaporation in Apple Orchards Based on Hydrogen and Oxygen Isotopes. Agronomy 2025, 15, 6. https://doi.org/10.3390/agronomy15010006
Huang J, Hua Y, Zhang L, Yu C, Chen K, Gu L, Wu Y, Wei H, Li M. Estimation of Soil Evaporation in Apple Orchards Based on Hydrogen and Oxygen Isotopes. Agronomy. 2025; 15(1):6. https://doi.org/10.3390/agronomy15010006
Chicago/Turabian StyleHuang, Jialiang, Yi Hua, Li Zhang, Chunxiu Yu, Kexin Chen, Linyun Gu, Yi Wu, Haoyan Wei, and Min Li. 2025. "Estimation of Soil Evaporation in Apple Orchards Based on Hydrogen and Oxygen Isotopes" Agronomy 15, no. 1: 6. https://doi.org/10.3390/agronomy15010006
APA StyleHuang, J., Hua, Y., Zhang, L., Yu, C., Chen, K., Gu, L., Wu, Y., Wei, H., & Li, M. (2025). Estimation of Soil Evaporation in Apple Orchards Based on Hydrogen and Oxygen Isotopes. Agronomy, 15(1), 6. https://doi.org/10.3390/agronomy15010006








