Size and Shape of Primary (Bio)Polyelectrolyte Complexes Chitosan/Gelatin: Study Using Small-Angle X-Ray Scattering from Synchrotron Radiation
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Preparation of Chitosan/Gelatin (Bio)Polyelectrolyte Complex Dispersions
2.3. Small-Angle X-Ray Scattering of Synchrotron Radiation
2.4. Determination of the Molecular Weight of Biopolymers Based on SAXS Data
2.5. Ab Initio Modelling
2.6. Dynamic Light Scattering
3. Results
3.1. Analysis of Conformation and Macromolecular Sizes of Individual Chitosan and Gelatin Solutions Using SAXS
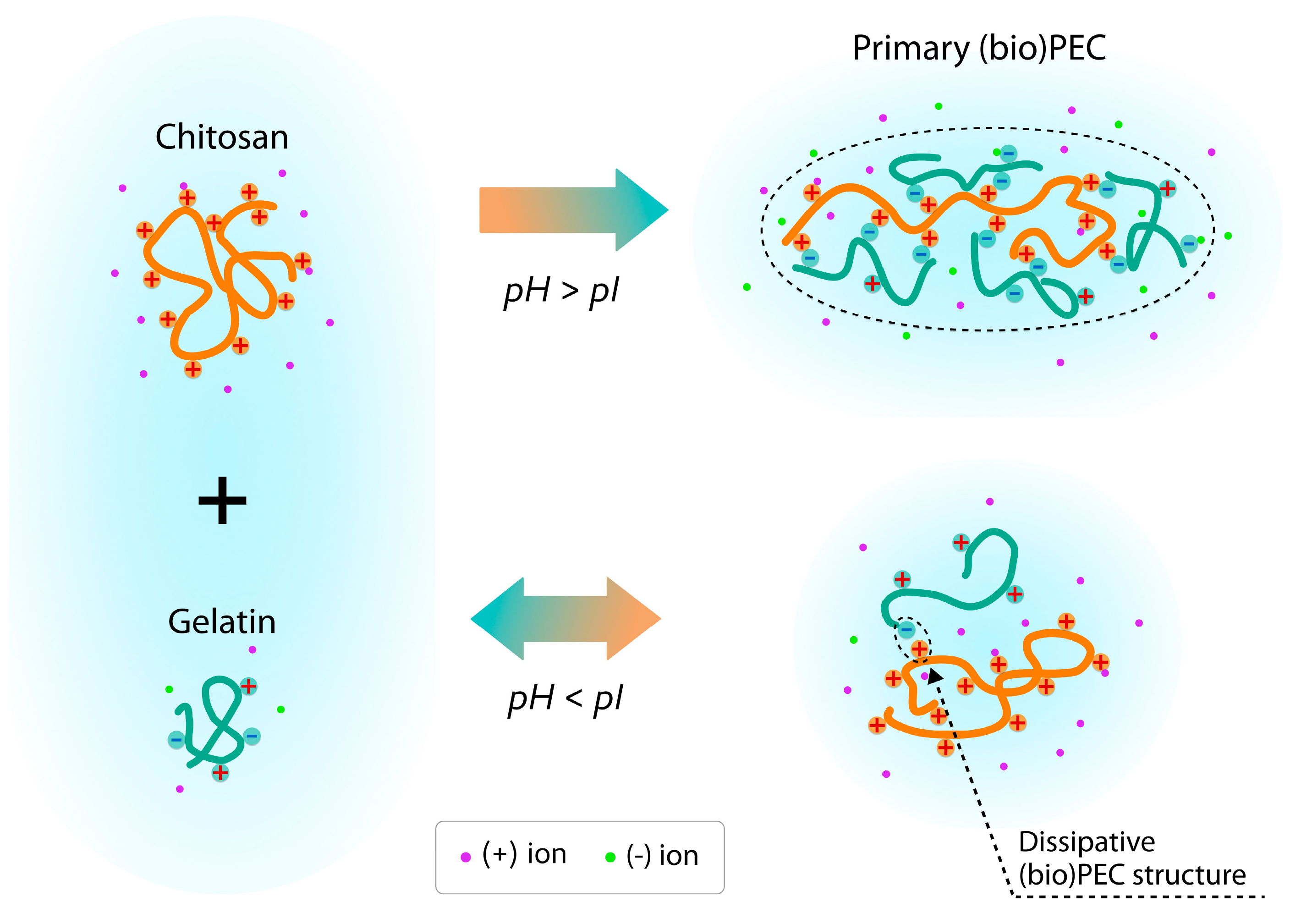
3.2. Estimation of Self-Assembly of Primary PEC Structures Using SAXS
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Timilsena, Y.P.; Akanbi, T.O.; Khalid, N.; Adhikari, B.; Barrow, C.J. Complex Coacervation: Principles, Mechanisms and Applications in Microencapsulation. Int. J. Biol. Macromol. 2019, 121, 1276–1286. [Google Scholar] [CrossRef] [PubMed]
- Margossian, K.O.; Brown, M.U.; Emrick, T.; Muthukumar, M. Coacervation in Polyzwitterion-Polyelectrolyte Systems and Their Potential Applications for Gastrointestinal Drug Delivery Platforms. Nat. Commun. 2022, 13, 2250. [Google Scholar] [CrossRef] [PubMed]
- Black, K.A.; Priftis, D.; Perry, S.L.; Yip, J.; Byun, W.Y.; Tirrell, M. Protein Encapsulation via Polypeptide Complex Coacervation. ACS Macro Lett. 2014, 3, 1088–1091. [Google Scholar] [CrossRef] [PubMed]
- Blocher, W.C.; Perry, S.L. Complex Coacervate-Based Materials for Biomedicine. Wiley Interdiscip. Rev. Nanomed. Nanobiotechnol. 2017, 9, e1442. [Google Scholar] [CrossRef]
- Wang, Q.; Schlenoff, J.B. The Polyelectrolyte Complex/Coacervate Continuum. Macromolecules 2014, 47, 3108–3116. [Google Scholar] [CrossRef]
- Takahashi, R.; Narayanan, T.; Sato, T. Growth Kinetics of Polyelectrolyte Complexes Formed from Oppositely-Charged Homopolymers Studied by Time-Resolved Ultra-Small-Angle X-Ray Scattering. J. Phys. Chem. Lett. 2017, 8, 737–741. [Google Scholar] [CrossRef]
- Bakeev, K.N.; Izumrudov, V.A.; Kuchanov, S.I.; Zezin, A.B.; Kabanov, V.A. Kinetics and Mechanism of Interpolyelectrolyte Exchange and Addition Reactions. Macromolecules 1992, 25, 4249–4254. [Google Scholar] [CrossRef]
- Malay, Ö.; Bayraktar, O.; Batigün, A. Complex Coacervation of Silk Fibroin and Hyaluronic Acid. Int. J. Biol. Macromol. 2007, 40, 387–393. [Google Scholar] [CrossRef]
- Elmer, C.; Karaca, A.C.; Low, N.H.; Nickerson, M.T. Complex Coacervation in Pea Protein Isolate-Chitosan Mixtures. Food Res. Int. 2011, 44, 1441–1446. [Google Scholar] [CrossRef]
- Zou, W.; Mourad, F.K.; Zhang, X.; Ahn, D.U.; Cai, Z.; Jin, Y. Phase Separation Behavior and Characterization of Ovalbumin and Propylene Glycol Alginate Complex Coacervates. Food Hydrocoll. 2020, 108, 105978. [Google Scholar] [CrossRef]
- Luo, W.; Huang, H.; Zhang, Y.; Wang, F.; Yu, J.; Liu, Y.; Li, X. Complex Coacervation Behavior and the Mechanism between Rice Glutelin and Gum Arabic at PH 3.0 Studied by Turbidity, Light Scattering, Fluorescence Spectra and Molecular Docking. LWT 2021, 150, 112084. [Google Scholar] [CrossRef]
- Archut, A.; Klost, M.; Drusch, S.; Kastner, H. Complex Coacervation of Pea Protein and Pectin: Contribution of Different Protein Fractions to Turbidity. Food Hydrocoll. 2023, 134, 108032. [Google Scholar] [CrossRef]
- Liu, X.; Haddou, M.; Grillo, I.; Mana, Z.; Chapel, J.P.; Schatz, C. Early Stage Kinetics of Polyelectrolyte Complex Coacervation Monitored through Stopped-Flow Light Scattering. Soft Matter 2016, 12, 9030–9038. [Google Scholar] [CrossRef] [PubMed]
- Amann, M.; Diget, J.S.; Lyngsø, J.; Pedersen, J.S.; Narayanan, T.; Lund, R. Kinetic Pathways for Polyelectrolyte Coacervate Micelle Formation Revealed by Time-Resolved Synchrotron SAXS. Macromolecules 2019, 52, 8227–8237. [Google Scholar] [CrossRef]
- Xu, A.Y.; Melton, L.D.; Ryan, T.M.; Mata, J.P.; Rekas, A.; Williams, M.A.K.; McGillivray, D.J. Effects of Polysaccharide Charge Pattern on the Microstructures of β-Lactoglobulin-Pectin Complex Coacervates, Studied by SAXS and SANS. Food Hydrocoll. 2018, 77, 952–963. [Google Scholar] [CrossRef]
- Spruijt, E.; Leermakers, F.A.M.; Fokkink, R.; Schweins, R.; Van Well, A.A.; Cohen Stuart, M.A.; Van Der Gucht, J. Structure and Dynamics of Polyelectrolyte Complex Coacervates Studied by Scattering of Neutrons, X-Rays, and Light. Macromolecules 2013, 46, 4596–4605. [Google Scholar] [CrossRef]
- Takahashi, R.; Narayanan, T.; Yusa, S.I.; Sato, T. Kinetics of Morphological Transition between Cylindrical and Spherical Micelles in a Mixture of Anionic-Neutral and Cationic-Neutral Block Copolymers Studied by Time-Resolved SAXS and USAXS. Macromolecules 2018, 51, 3654–3662. [Google Scholar] [CrossRef]
- Takahashi, R.; Narayanan, T.; Yusa, S.I.; Sato, T. Formation Kinetics of Polymer Vesicles from Spherical and Cylindrical Micelles Bearing the Polyelectrolyte Complex Core Studied by Time-Resolved USAXS and SAXS. Macromolecules 2022, 55, 684–695. [Google Scholar] [CrossRef]
- Jing, B.; Ferreira, M.; Lin, K.; Li, R.; Yavitt, B.M.; Qiu, J.; Fukuto, M.; Zhu, Y. Ultrastructure of Critical-Gel-like Polyzwitterion-Polyoxometalate Complex Coacervates: Effects of Temperature, Salt Concentration, and Shear. Macromolecules 2020, 53, 10972–10980. [Google Scholar] [CrossRef]
- Remuñán-López, C.; Bodmeier, R. Effect of Formulation and Process Variables on the Formation of Chitosan-Gelatin Coacervates. Int. J. Pharm. 1996, 135, 63–72. [Google Scholar] [CrossRef]
- Basu, S.K.; Kavitha, K.; Rupeshkumar, M. Evaluation of Ketorolac Tromethamine Microspheres by Chitosan/Gelatin B Complex Coacervation. Sci. Pharm. 2010, 78, 79–92. [Google Scholar] [CrossRef] [PubMed]
- Roy, J.C.; Giraud, S.; Ferri, A.; Mossotti, R.; Guan, J.; Salaün, F. Influence of Process Parameters on Microcapsule Formation from Chitosan—Type B Gelatin Complex Coacervates. Carbohydr. Polym. 2018, 198, 281–293. [Google Scholar] [CrossRef] [PubMed]
- Cheng, C.; Tu, Z.; Wang, H. PH-Induced Complex Coacervation of Fish Gelatin and Carboxylated Chitosan: Phase Behavior and Structural Properties. Food Res. Int. 2023, 167, 112652. [Google Scholar] [CrossRef] [PubMed]
- Prata, A.S.; Grosso, C.R.F. Production of Microparticles with Gelatin and Chitosan. Carbohydr. Polym. 2015, 116, 292–299. [Google Scholar] [CrossRef]
- Wang, X.Y.; Wang, C.S.; Heuzey, M.C. Complexation of Chitosan and Gelatin: From Soluble Complexes to Colloidal Gel. Int. J. Polym. Mater. Polym. Biomater. 2016, 65, 96–104. [Google Scholar] [CrossRef]
- Abdul Aziz, F.R.; Jai, J.; Raslan, R.; Subuki, I. Microencapsulation of Citronella Oil by Complex Coacervation Using Chitosan-Gelatin (b) System: Operating Design, Preparation and Characterization. In Proceedings of the MATEC Web of Conferences, Amsterdam, The Netherlands, 23–25 March 2016; Volume 69. [Google Scholar] [CrossRef]
- Silva, M.C.; Andrade, C.T. Evaluating Conditions for the Formation of Chitosan/Gelatin Microparticles. Polimeros 2009, 19, 133–137. [Google Scholar] [CrossRef]
- Yin, Y.; Li, Z.; Sun, Y.; Yao, K. A Preliminary Study on Chitosan/Gelatin Polyelectrolyte Complex Formation. J. Mater. Sci. 2005, 40, 4649–4652. [Google Scholar] [CrossRef]
- Litvinov, M.; Kashurin, A.; Podshivalov, A. Study of the Influence of the Composition and PH of the Solution on the Structure and Morphology of Particle Dispersions of a (Bio)Polyelectrolyte Complex between Chitosan and Gelatin. Colloid. Polym. Sci. 2025, 303, 33–49. [Google Scholar] [CrossRef]
- Litvinov, M.; Podshivalov, A.; Kovalev, K. Morphological Study of the Particle-to-Fiber Transition Threshold during Electrohydrodynamic Processing of Chitosan Solution. J. Macromol. Sci. Part A Pure Appl. Chem. 2021, 58, 804–810. [Google Scholar] [CrossRef]
- Peters, G.S.; Gaponov, Y.A.; Konarev, P.V.; Marchenkova, M.A.; Ilina, K.B.; Volkov, V.V.; Pisarevsky, Y.V.; Kovalchuk, M.V. Upgrade of the BioMUR Beamline at the Kurchatov Synchrotron Radiation Source for Serial Small-Angle X-Ray Scattering Experiments in Solutions. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2022, 1025, 166170. [Google Scholar] [CrossRef]
- Hammersley, A.P. FIT2D: A Multi-Purpose Data Reduction, Analysis and Visualization Program. J. Appl. Crystallogr. 2016, 49, 646–652. [Google Scholar] [CrossRef]
- Konarev, P.V.; Petoukhov, M.V.; Volkov, V.V.; Svergun, D.I. ATSAS 2.1, a Program Package for Small-Angle Scattering Data Analysis. J. Appl. Crystallogr. 2006, 39, 277–286. [Google Scholar] [CrossRef]
- Neves, S.; Fonseca, C.P. Determination of Fractal Dimension of Polyaniline Composites by SAXS and Electrochemical Techniques. Electrochem. Commun. 2001, 3, 36–43. [Google Scholar] [CrossRef]
- Schmidt, P.W. Small-Angle Scattering Studies of Disordered, Porous and Fractal Systems. J. Appl. Crystallogr. 1991, 24, 414–435. [Google Scholar] [CrossRef]
- Svergun, D.I. Determination of the Regularization Parameter in Indirect-Transform Methods Using Perceptual Criteria. J. Appl. Crystallogr. 1992, 25, 495–503. [Google Scholar] [CrossRef]
- Rambo, R.P.; Tainer, J.A. Accurate Assessment of Mass, Models and Resolution by Small-Angle Scattering. Nature 2013, 496, 477–481. [Google Scholar] [CrossRef]
- Mertens, H.D.T.; Svergun, D.I. Structural Characterization of Proteins and Complexes Using Small-Angle X-Ray Solution Scattering. J. Struct. Biol. 2010, 172, 128–141. [Google Scholar] [CrossRef]
- Volkov, V.V.; Svergun, D.I. Uniqueness of Ab Initio Shape Determination in Small-Angle Scattering. J. Appl. Crystallogr. 2003, 36, 860–864. [Google Scholar] [CrossRef]
- Svergun, D.I. Restoring Low Resolution Structure of Biological Macromolecules from Solution Scattering Using Simulated Annealing. Biophys. J. 1999, 76, 2879–2886. [Google Scholar] [CrossRef]
- Swain, P.; Ronghe, A.; Bhutani, U.; Majumdar, S. Physicochemical Response of Gelatin in a Coulombic Soup of Monovalent Salt: A Molecular Simulation and Experimental Study. J. Phys. Chem. B 2019, 123, 1186–1194. [Google Scholar] [CrossRef]
- Lin, W.; Yan, L.; Mu, C.; Li, W.; Zhang, M.; Zhu, Q. Effect of PH on Gelatin Self-Association Investigated by Laser Light Scattering and Atomic Force Microscopy. Polym. Int. 2002, 51, 233–238. [Google Scholar] [CrossRef]
- Popa-Nita, S.; Alcouffe, P.; Rochas, C.; David, L.; Domard, A. Continuum of Structural Organization from Chitosan Solutions to Derived Physical Forms. Biomacromolecules 2010, 11, 6–12. [Google Scholar] [CrossRef] [PubMed]
- Korchagina, E.V.; Philippova, O.E. Multichain Aggregates in Dilute Solutions of Associating Polyelectrolyte Keeping a Constant Size at the Increase in the Chain Length of Individual Macromolecules. Biomacromolecules 2010, 11, 3457–3466. [Google Scholar] [CrossRef] [PubMed]
- Blagodatskikh, I.V.; Bezrodnykh, E.A.; Abramchuk, S.S.; Muranov, A.V.; Sinitsyna, O.V.; Khokhlov, A.R.; Tikhonov, V.E. Tikhonov Short Chain Chitosan Solutions: Self-Assembly and Aggregates Disruption Effects. J. Polym. Res. 2013, 20, 1–10. [Google Scholar] [CrossRef]
- Kwok, J.J.; Park, K.S.; Patel, B.B.; Dilmurat, R.; Beljonne, D.; Zuo, X.; Lee, B.; Diao, Y. Understanding Solution State Conformation and Aggregate Structure of Conjugated Polymers via Small Angle X-Ray Scattering. Macromolecules 2022, 55, 4353–4366. [Google Scholar] [CrossRef]
- Whittaker, J.L.; Dutta, N.K.; Knott, R.; McPhee, G.; Voelcker, N.H.; Elvin, C.; Hill, A.; Choudhury, N.R. Tunable Thermoresponsiveness of Resilin via Coassembly with Rigid Biopolymers. Langmuir 2015, 31, 8882–8891. [Google Scholar] [CrossRef]
- Korchagina, E.V.; Philippova, O.E. Effects of Hydrophobic Substituents and Salt on Core-Shell Aggregates of Hydrophobically Modified Chitosan: Light Scattering Study. Langmuir 2012, 28, 7880–7888. [Google Scholar] [CrossRef]
- Pa, J.H.; Yu, T.L. Light Scattering Study of Chitosan in Acetic Acid Aqueous Solutions. Macromol. Chem. Phys. 2001, 202, 985–991. [Google Scholar] [CrossRef]
- Li, Y.I.; Cheng, R. Viscometric Study of Gelatin in Dilute Aqueous Solutions. J. Polym. Sci. Part B Polym. Phys. 2006, 44, 1804–1812. [Google Scholar] [CrossRef]
- Rambo, R.P.; Tainer, J.A. Characterizing Flexible and Intrinsically Unstructured Biological Macromolecules by SAS Using the Porod-Debye Law. Biopolymers 2011, 95, 559–571. [Google Scholar] [CrossRef]
- Dainese, E.; Sabatucci, A.; Cozzani, I. Small Angle X-Ray Scattering: A Powerful Tool to Analyze Protein Conformation in Solution. Curr. Org. Chem. 2005, 9, 1781–1800. [Google Scholar] [CrossRef]
- Prior, C.; Davies, O.R.; Bruce, D.; Pohl, E. Obtaining Tertiary Protein Structures by the Ab Initio Interpretation of Small Angle X-Ray Scattering Data. J. Chem. Theory Comput. 2020, 16, 1985–2001. [Google Scholar] [CrossRef] [PubMed]
- Balu, R.; Mata, J.P.; Knott, R.; Elvin, C.M.; Hill, A.J.; Choudhury, N.R.; Dutta, N.K. Effects of Crowding and Environment on the Evolution of Conformational Ensembles of the Multi-Stimuli-Responsive Intrinsically Disordered Protein, Rec1-Resilin: A Small-Angle Scattering Investigation. J. Phys. Chem. B 2016, 120, 6490–6503. [Google Scholar] [CrossRef] [PubMed]
- Blobel, J.; Bernadó, P.; Svergun, D.I.; Tauler, R.; Pons, M. Low-Resolution Structures of Transient Protein-Protein Complexes Using Small-Angle X-Ray Scattering. J. Am. Chem. Soc. 2009, 131, 4378–4386. [Google Scholar] [CrossRef]

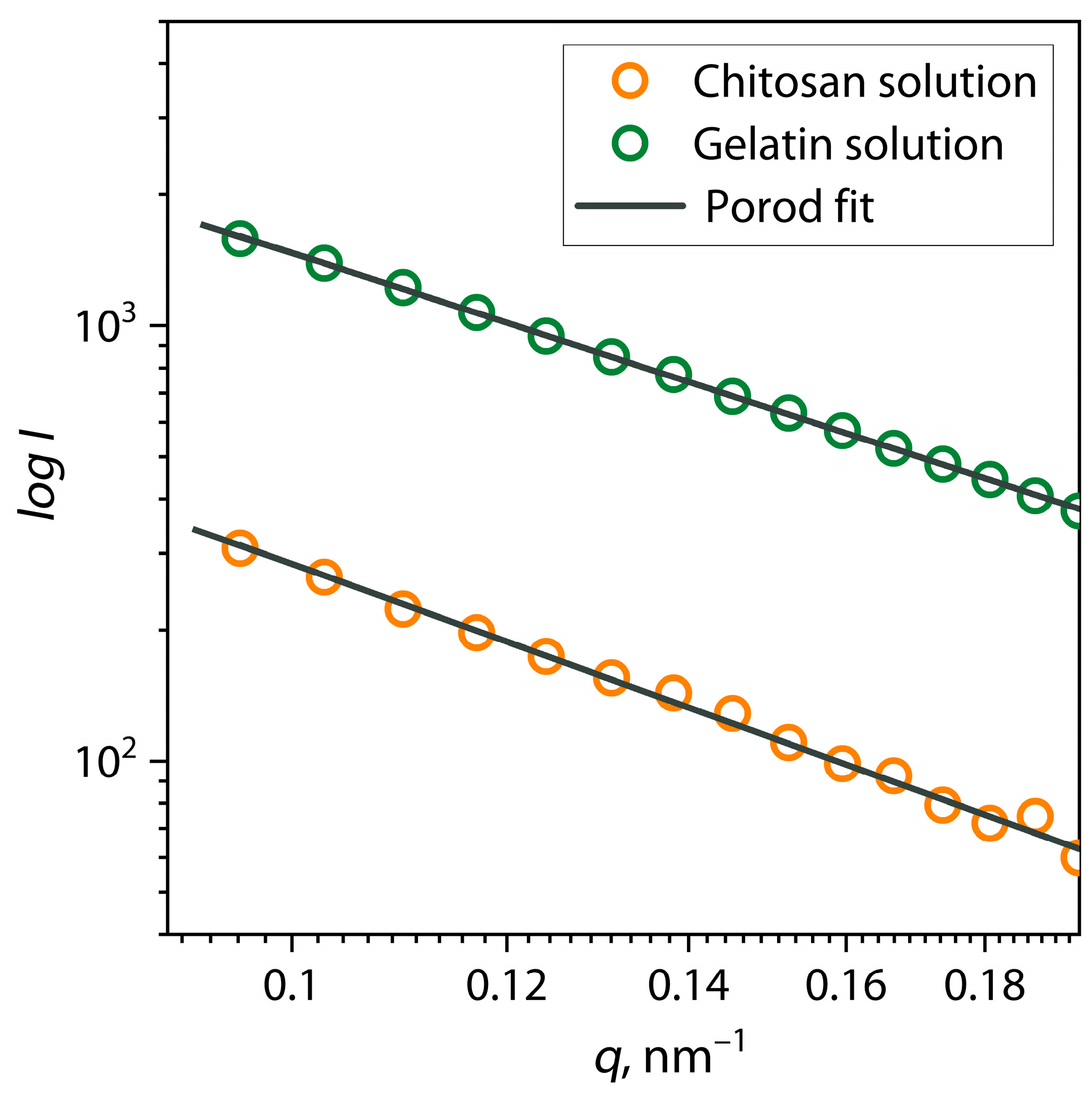
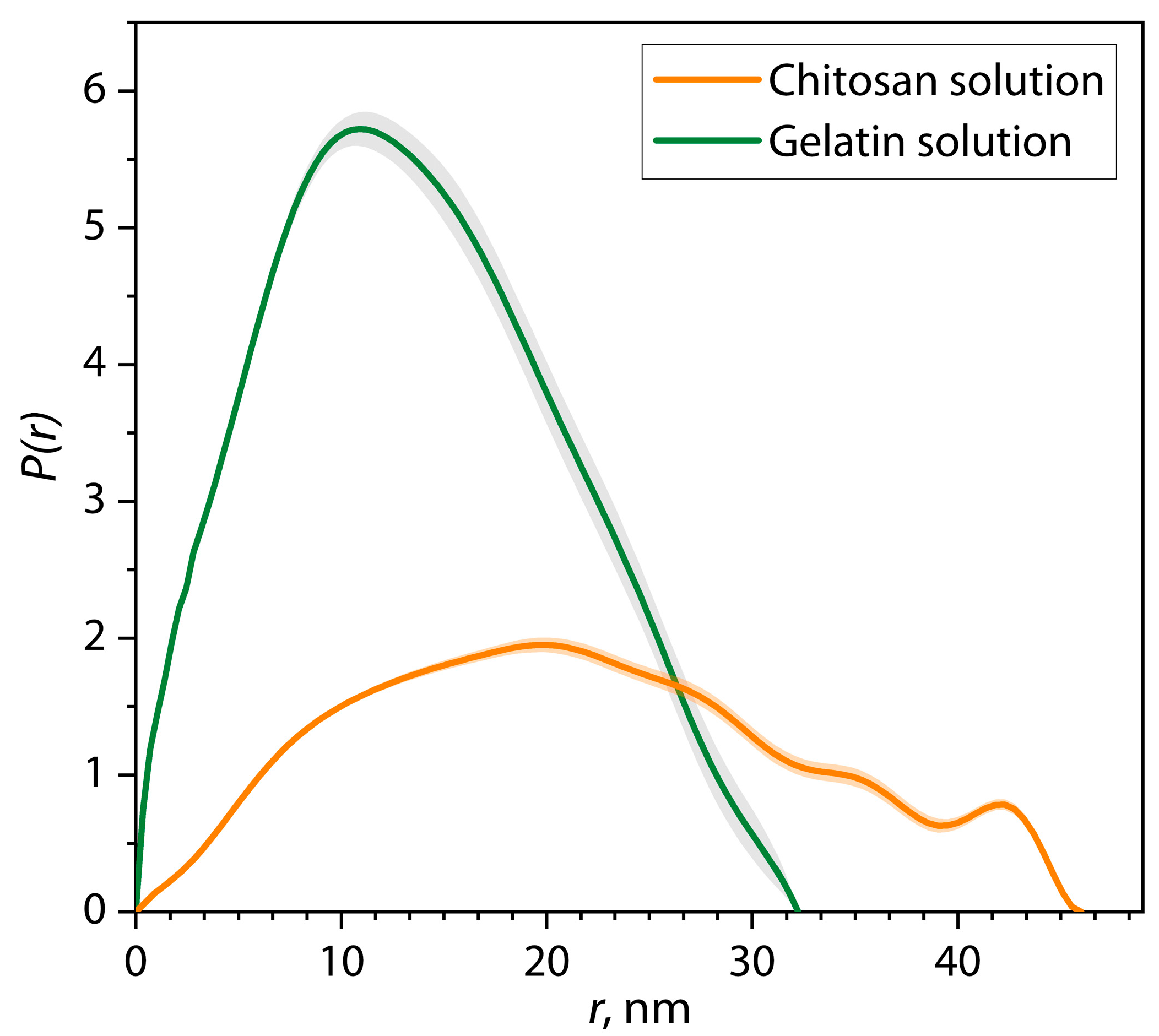


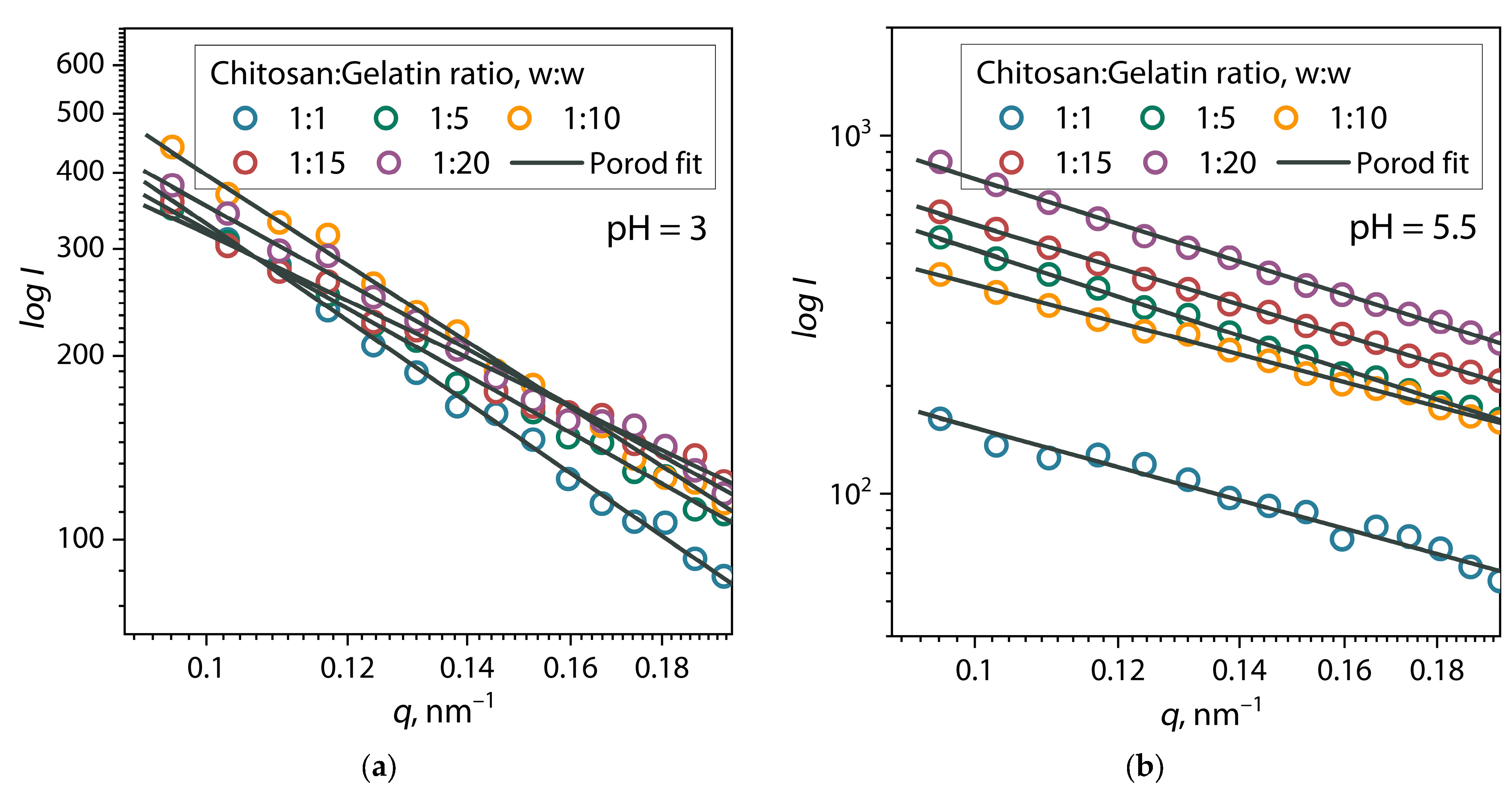
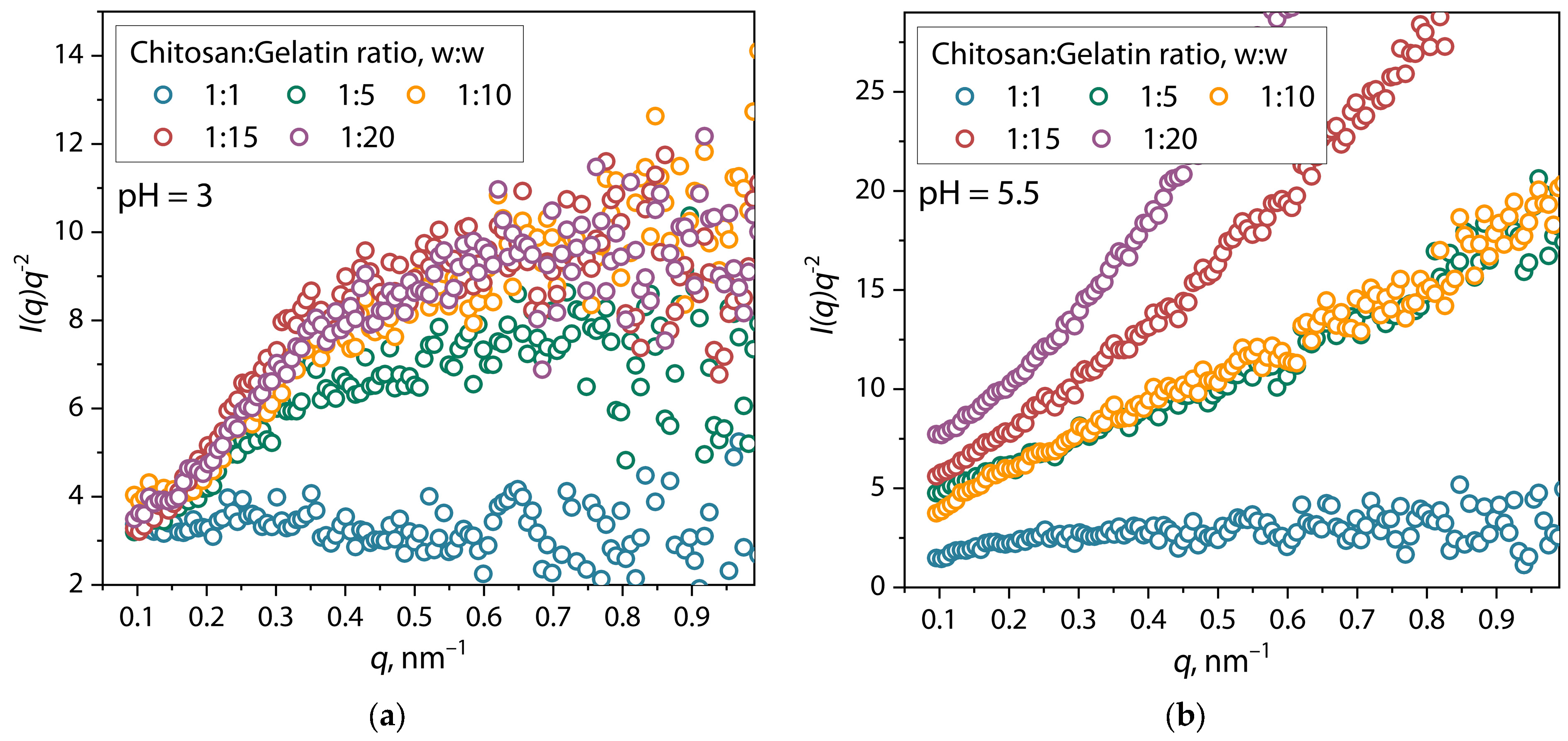
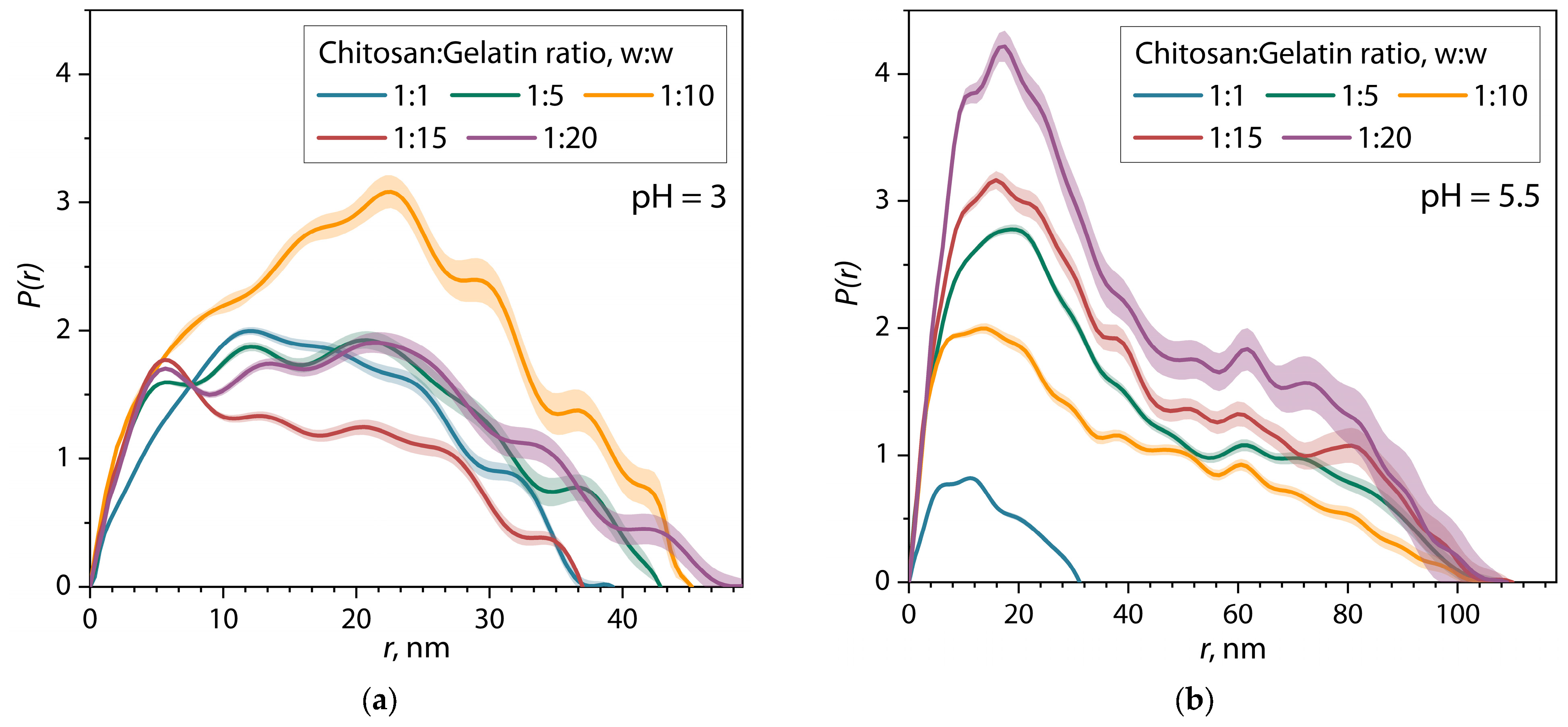

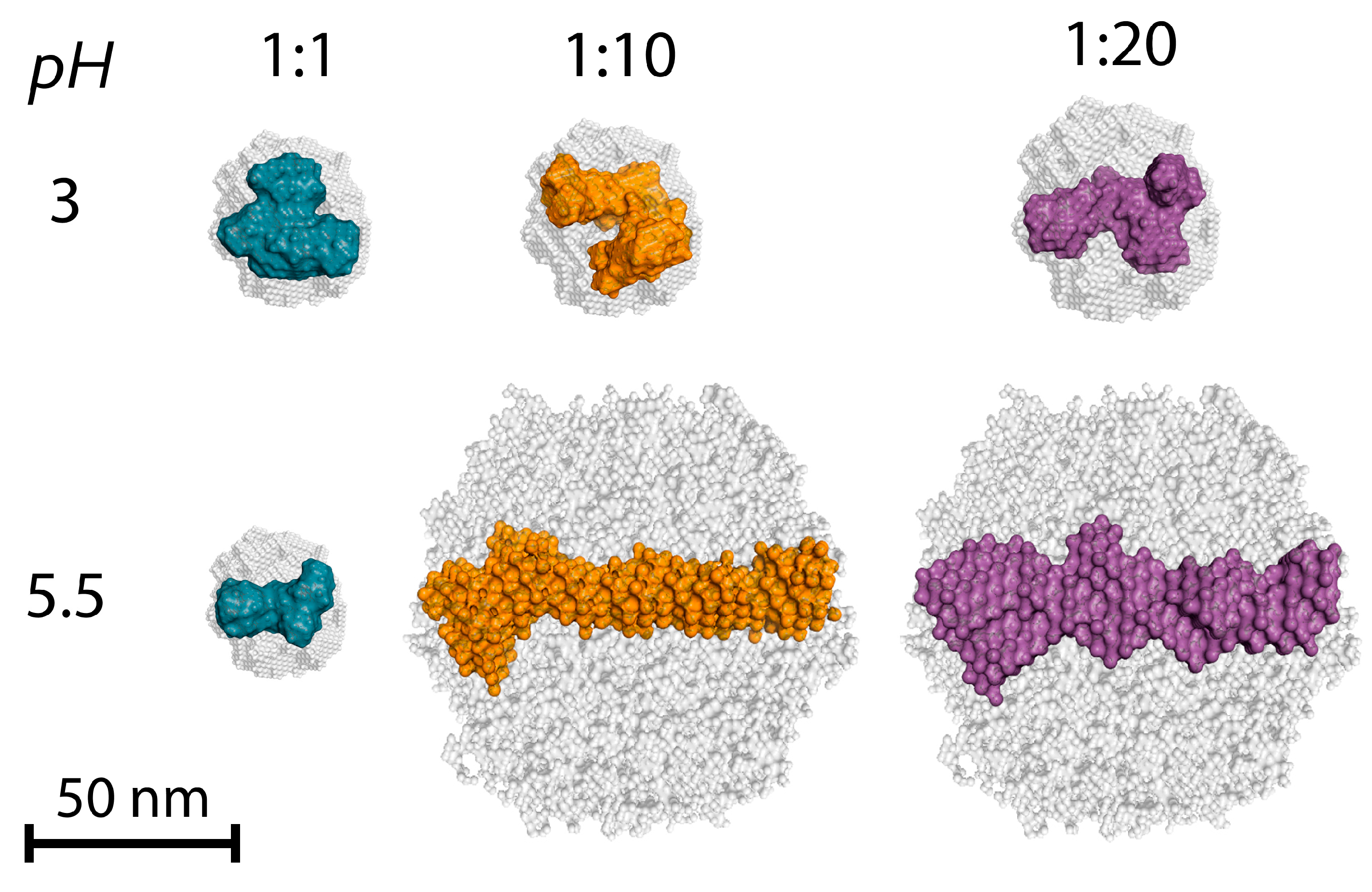
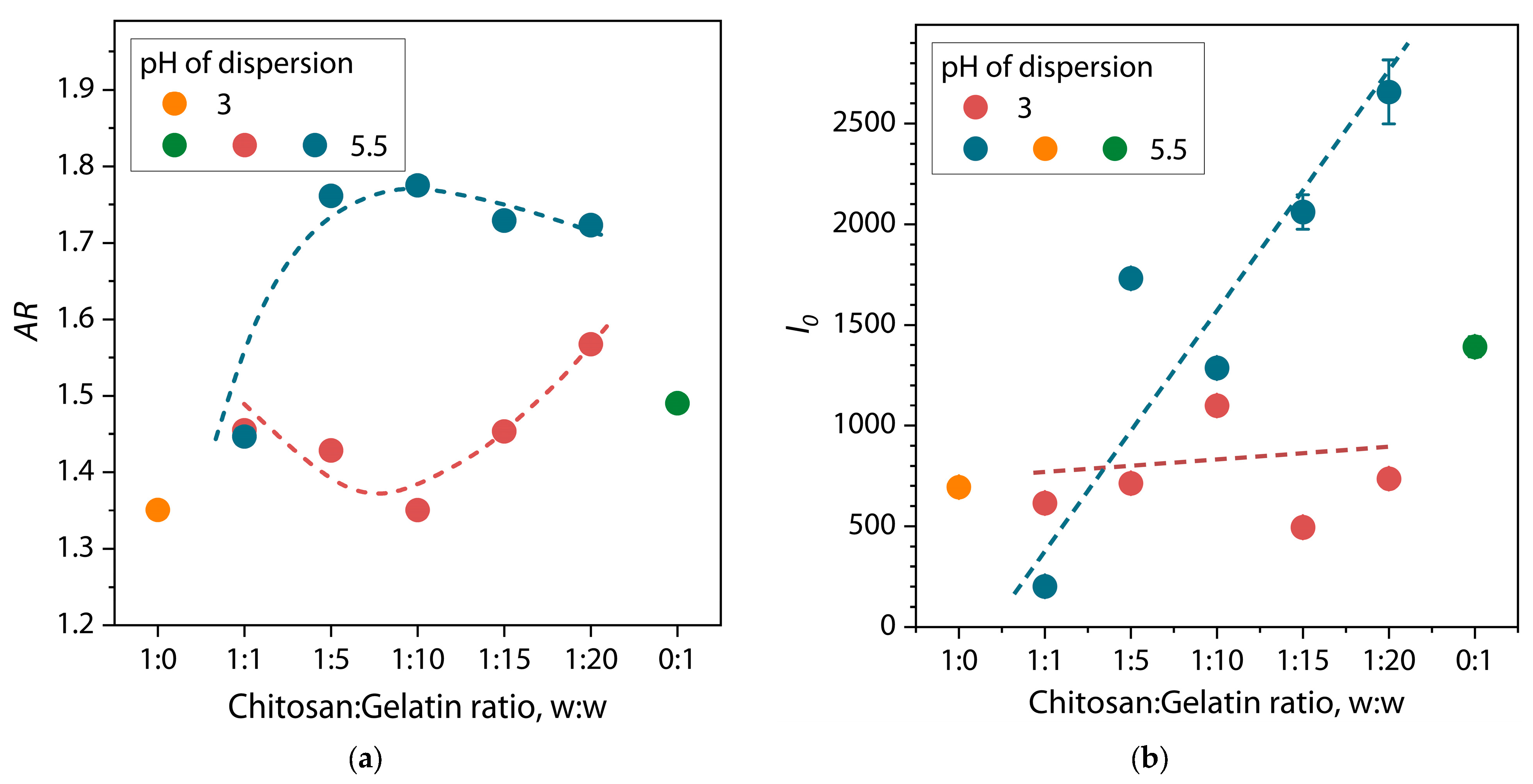
| Sample | α | I0 | Dmax, nm | Estimate (PDDF) | Rg (PDDF), nm | Rg (Viscometric), nm | AR |
|---|---|---|---|---|---|---|---|
| Gelatin | 2.00 | 1391 ± 50 | 32.2 | 0.8332 | 10.8 ± 0.2 | 7.5 ± 3.9 | 1.49 |
| Chitosan | 2.26 | 693 ± 21 | 46.0 | 0.6776 | 17.0 ± 0.1 | 24.2 ± 4.7 | 1.35 |
| Chitosan/Gelatin Ratio, w/w | α | I0 | Dmax, nm | Estimate (PDDF) | Rg (PDDF), nm |
|---|---|---|---|---|---|
| pH = 3 | |||||
| 1:1 | 2.00 | 615 ± 17 | 39.3 | 0.8751 | 13.5 ± 0.2 |
| 1:5 | 1.63 | 713 ± 28 | 42.8 | 0.7990 | 15.0 ± 0.3 |
| 1:10 | 1.87 | 1099 ± 41 | 44.0 | 0.8092 | 16.3 ± 0.2 |
| 1:15 | 1.39 | 495 ± 15 | 37.0 | 0.7202 | 12.7 ± 0.2 |
| 1:20 | 1.61 | 736 ± 38 | 50.0 | 0.8609 | 15.9 ± 0.5 |
| pH = 5.5 | |||||
| 1:1 | 1.38 | 201 ± 4 | 31 | 0.7988 | 10.7 ± 0.1 |
| 1:5 | 1.33 | 1731 ± 38 | 110 | 0.7391 | 31.2 ± 0.7 |
| 1:10 | 1.63 | 1286 ± 38 | 108 | 0.7786 | 30.4 ± 0.7 |
| 1:15 | 1.52 | 2061 ± 85 | 110 | 0.8683 | 31.8 ± 1.1 |
| 1:20 | 1.58 | 2657 ± 159 | 109 | 0.8078 | 31.6 ± 1.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Podshivalov, A.; Litvinov, M.; Kashurin, A.; Danilova, K. Size and Shape of Primary (Bio)Polyelectrolyte Complexes Chitosan/Gelatin: Study Using Small-Angle X-Ray Scattering from Synchrotron Radiation. Polymers 2025, 17, 1236. https://doi.org/10.3390/polym17091236
Podshivalov A, Litvinov M, Kashurin A, Danilova K. Size and Shape of Primary (Bio)Polyelectrolyte Complexes Chitosan/Gelatin: Study Using Small-Angle X-Ray Scattering from Synchrotron Radiation. Polymers. 2025; 17(9):1236. https://doi.org/10.3390/polym17091236
Chicago/Turabian StylePodshivalov, Aleksandr, Mikhail Litvinov, Aleksandr Kashurin, and Ksenia Danilova. 2025. "Size and Shape of Primary (Bio)Polyelectrolyte Complexes Chitosan/Gelatin: Study Using Small-Angle X-Ray Scattering from Synchrotron Radiation" Polymers 17, no. 9: 1236. https://doi.org/10.3390/polym17091236
APA StylePodshivalov, A., Litvinov, M., Kashurin, A., & Danilova, K. (2025). Size and Shape of Primary (Bio)Polyelectrolyte Complexes Chitosan/Gelatin: Study Using Small-Angle X-Ray Scattering from Synchrotron Radiation. Polymers, 17(9), 1236. https://doi.org/10.3390/polym17091236






