Orthotropic Constitutive Modeling and Tsai–Wu Failure Criterion for Carbon Fiber-Reinforced PEEK Composites
Abstract
1. Introduction
2. Theoretical Principles and Experimental Preparation
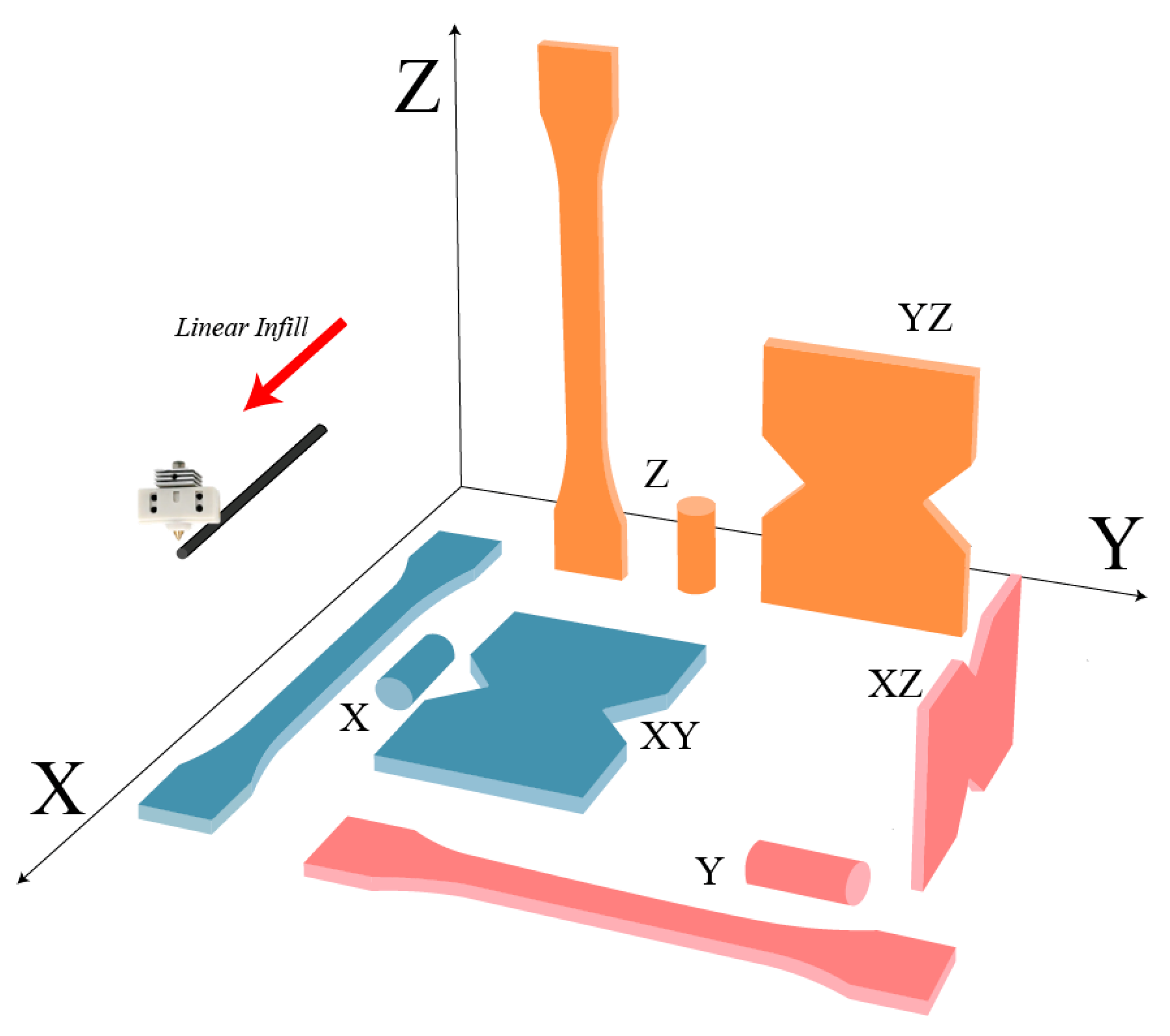
2.1. Characteristics of the Material Extrusion AM Process
- -
- The X-direction represents the filament deposition direction, corresponding to the printing path.
- -
- The Y-direction is perpendicular to the filament deposition direction that reflects the intralayer bonding between fibers.
- -
- The Z-direction represents the build direction that indicates the interlayer bonding.
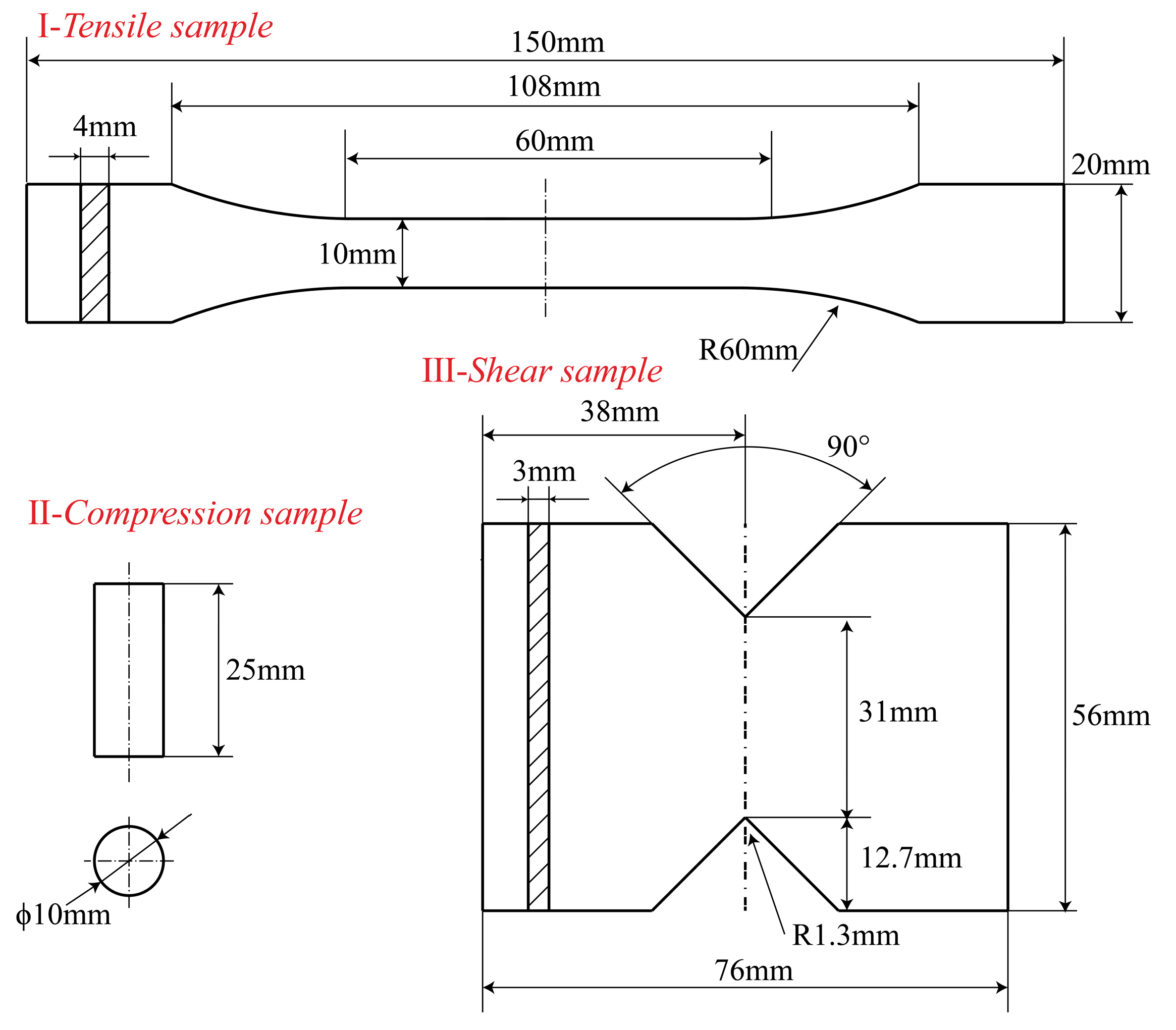
2.2. Sample Preparation
2.3. Experiment Design
3. Experimental Results and Discussion
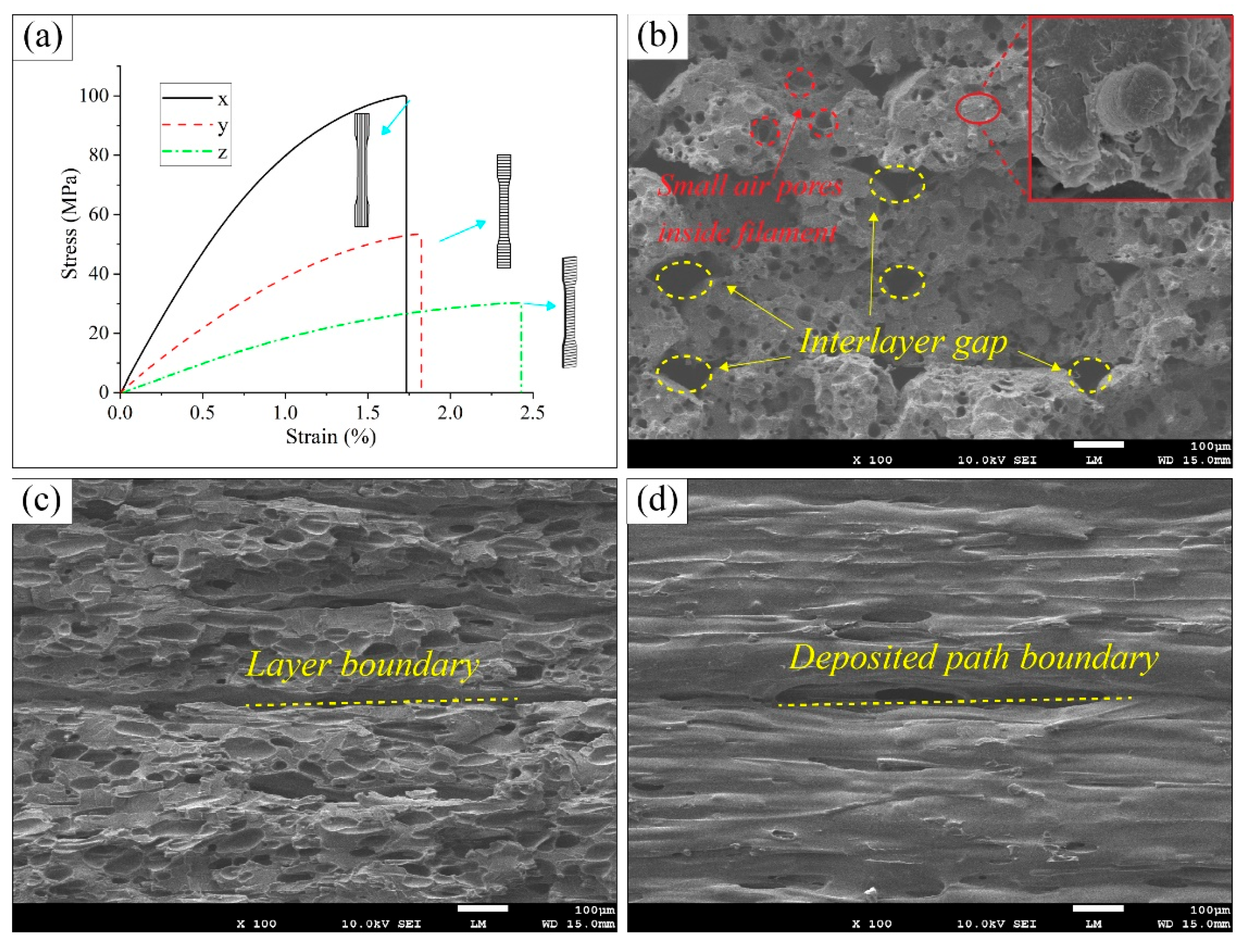
3.1. Tensile Test Result Analysis
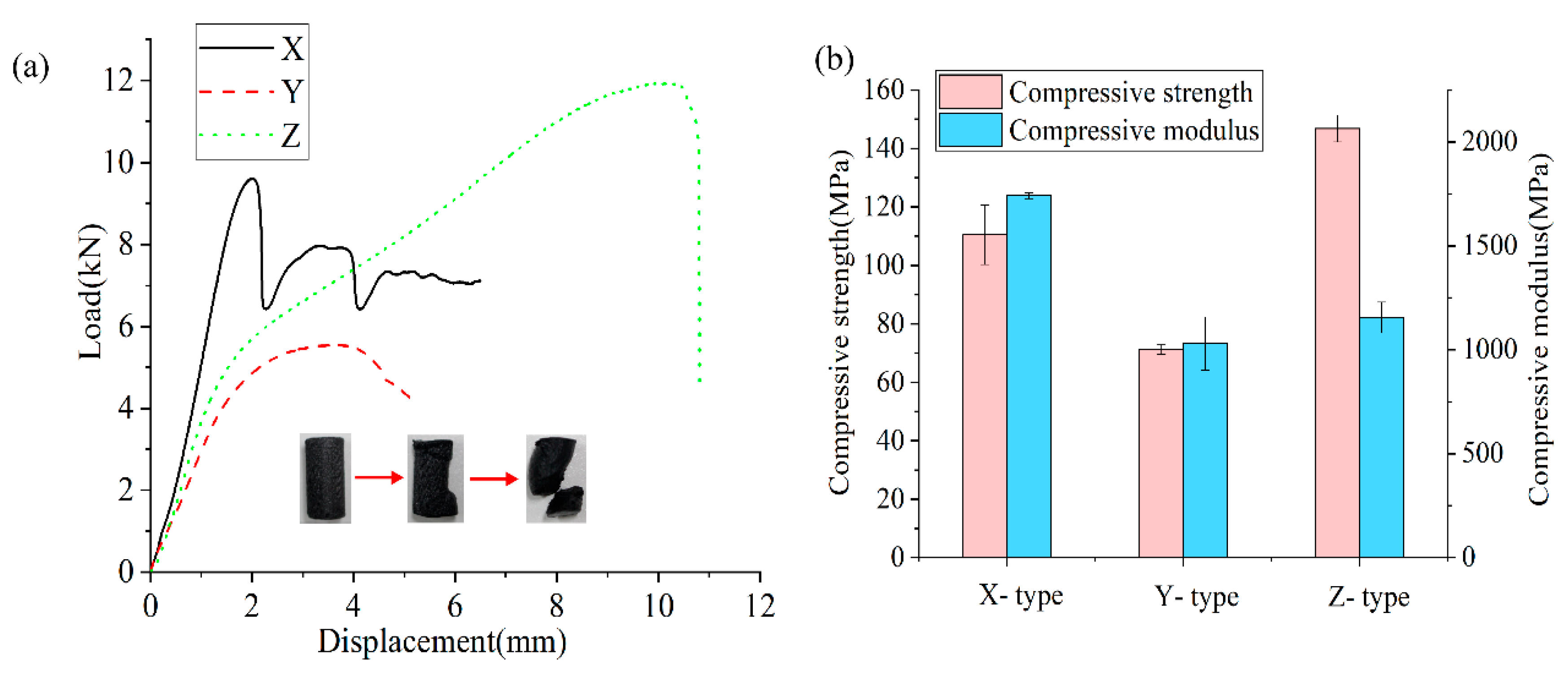
3.2. Compression Test Result Analysis
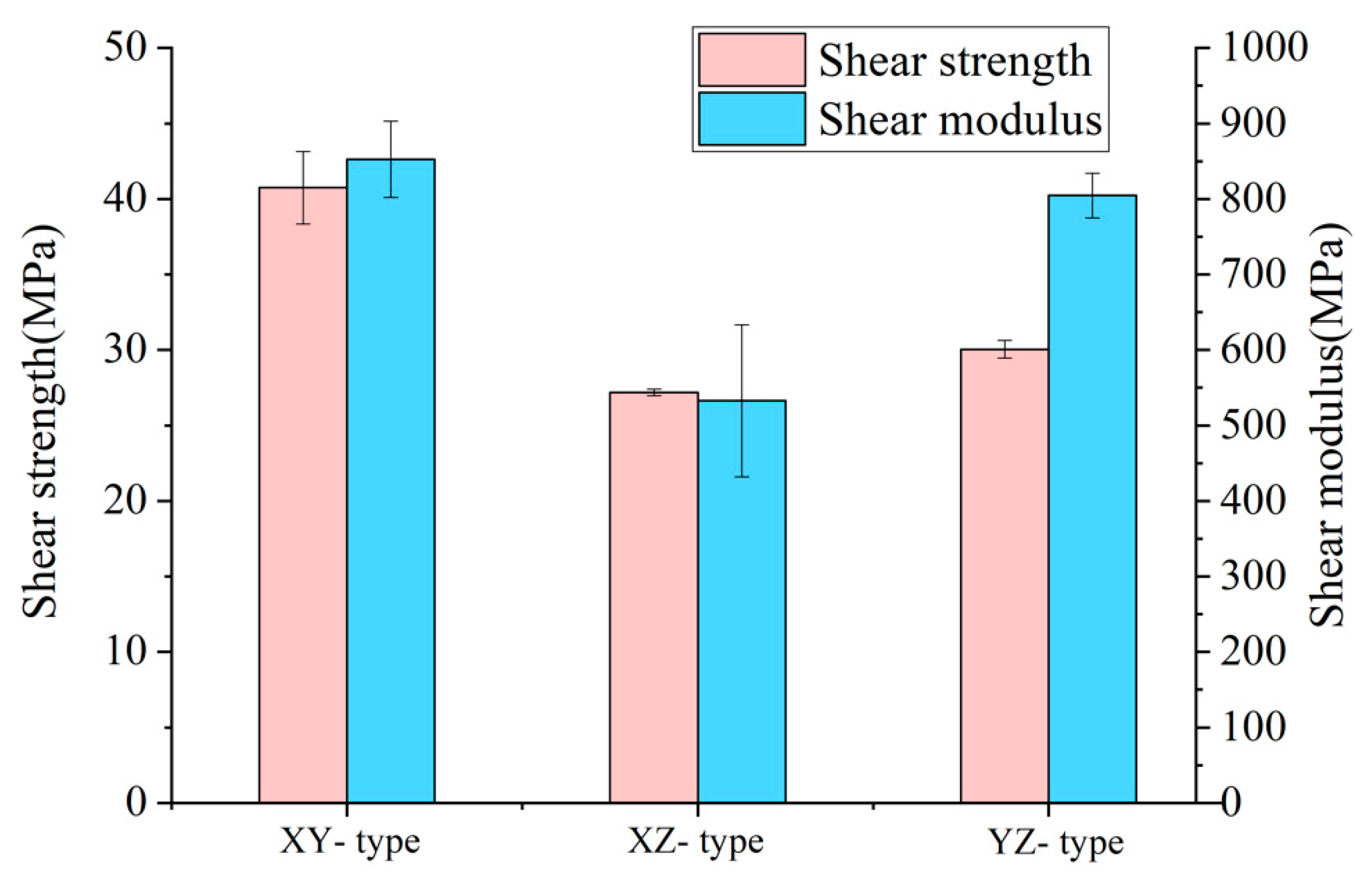
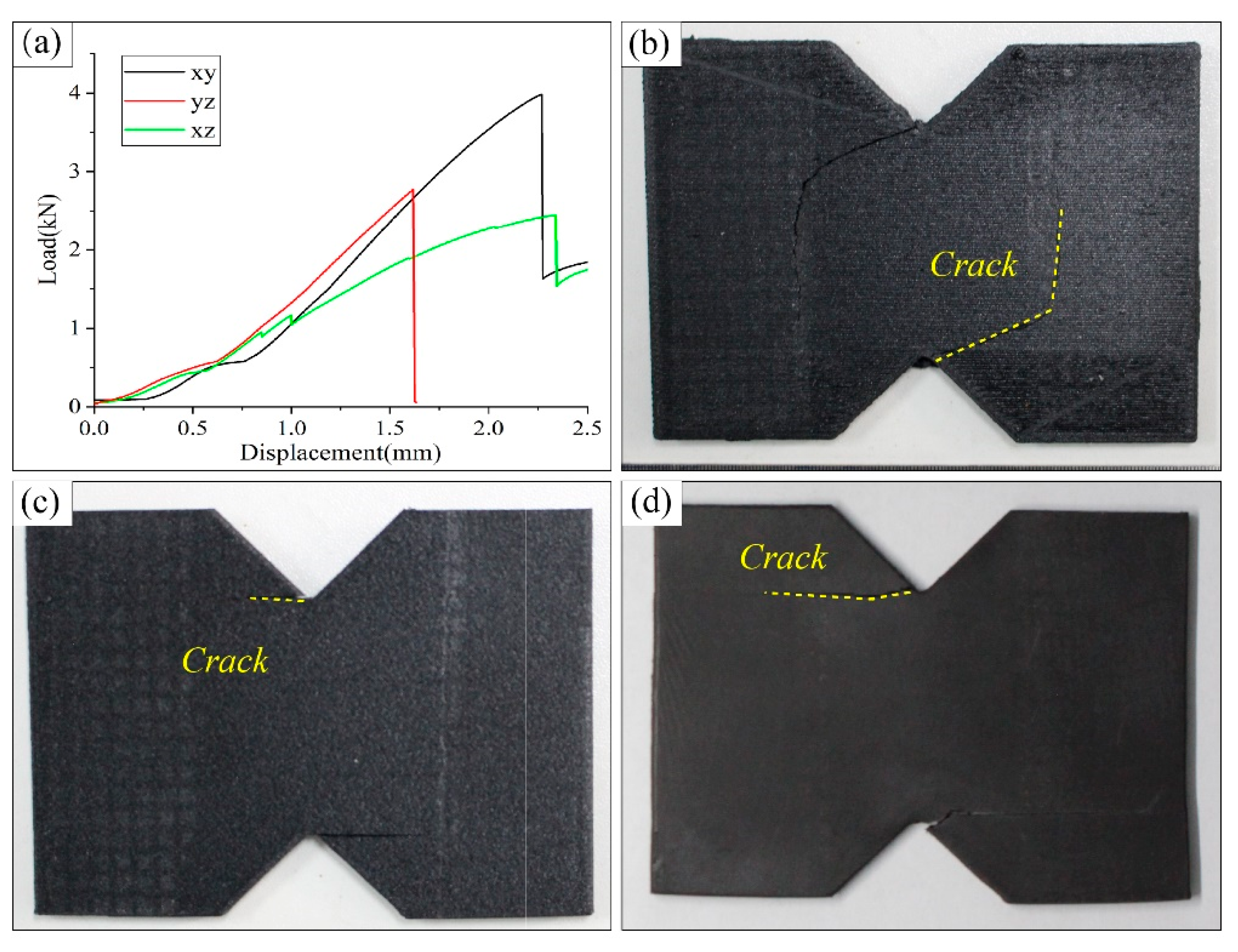
3.3. Shear Test Result Analysis
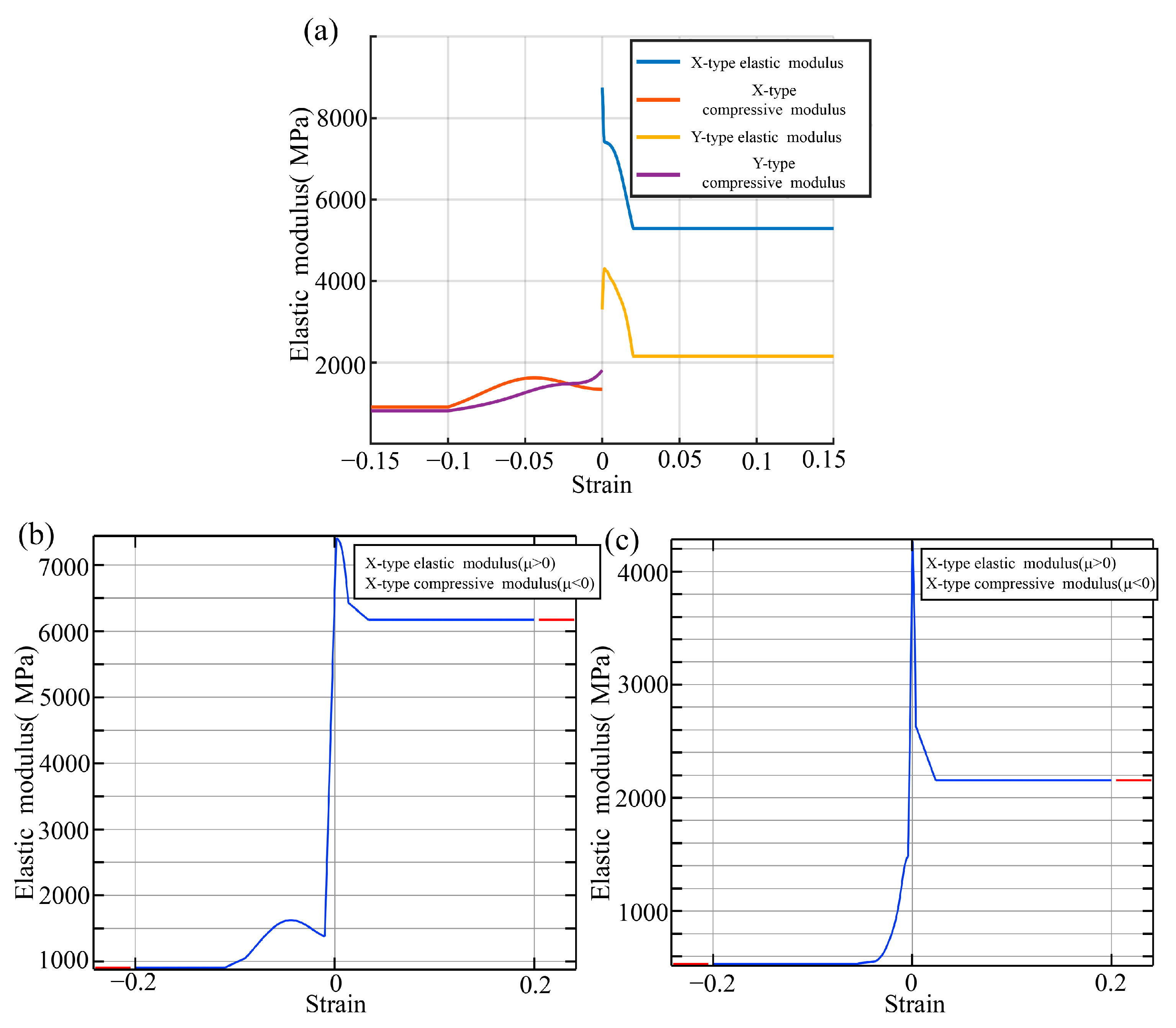
3.4. Elastic Modulus and Poisson’s Ratio
4. Establishment of the Simulation Model and Experimental Comparison
4.1. Failure Evaluation Model for CF-PEEK
4.2. Simulation Model Establishment
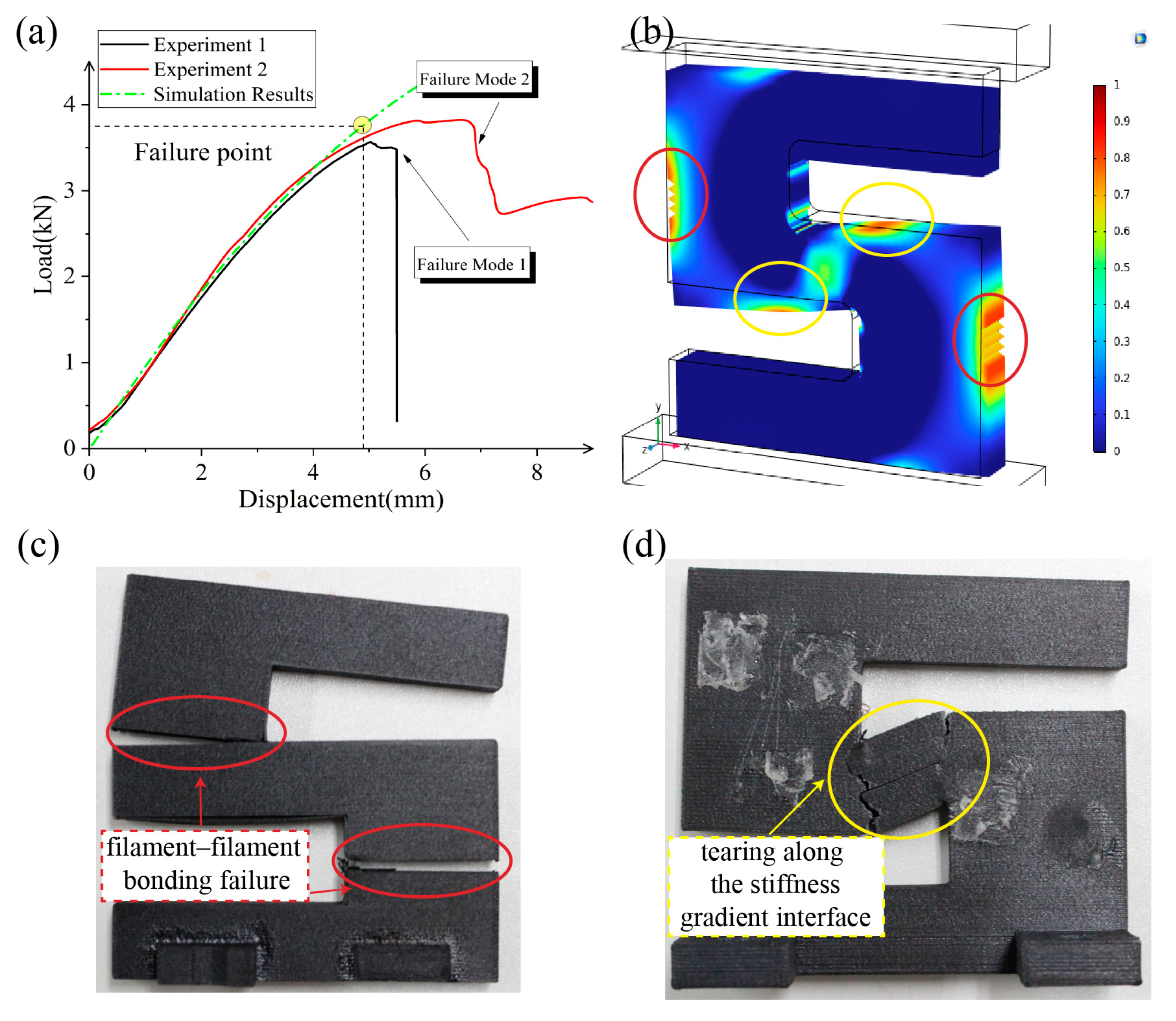
4.3. Comparison of Experimental and Simulation Results
5. Topology Optimization Based on Orthogonal Anisotropy with Tsai–Wu Failure Constraint
5.1. Material Interpolation Method
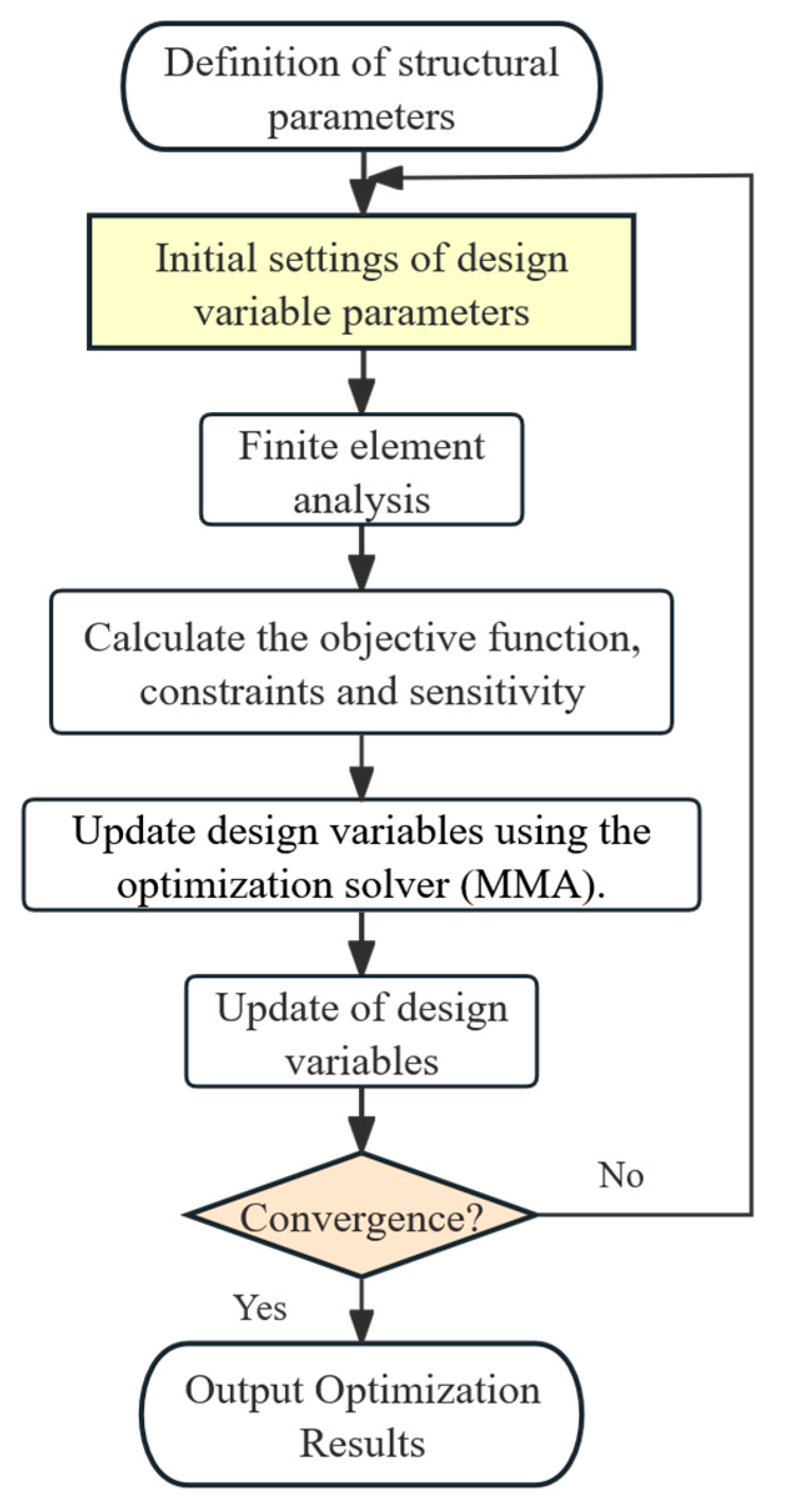
5.2. Optimization Problem and Sensitivity Analysis
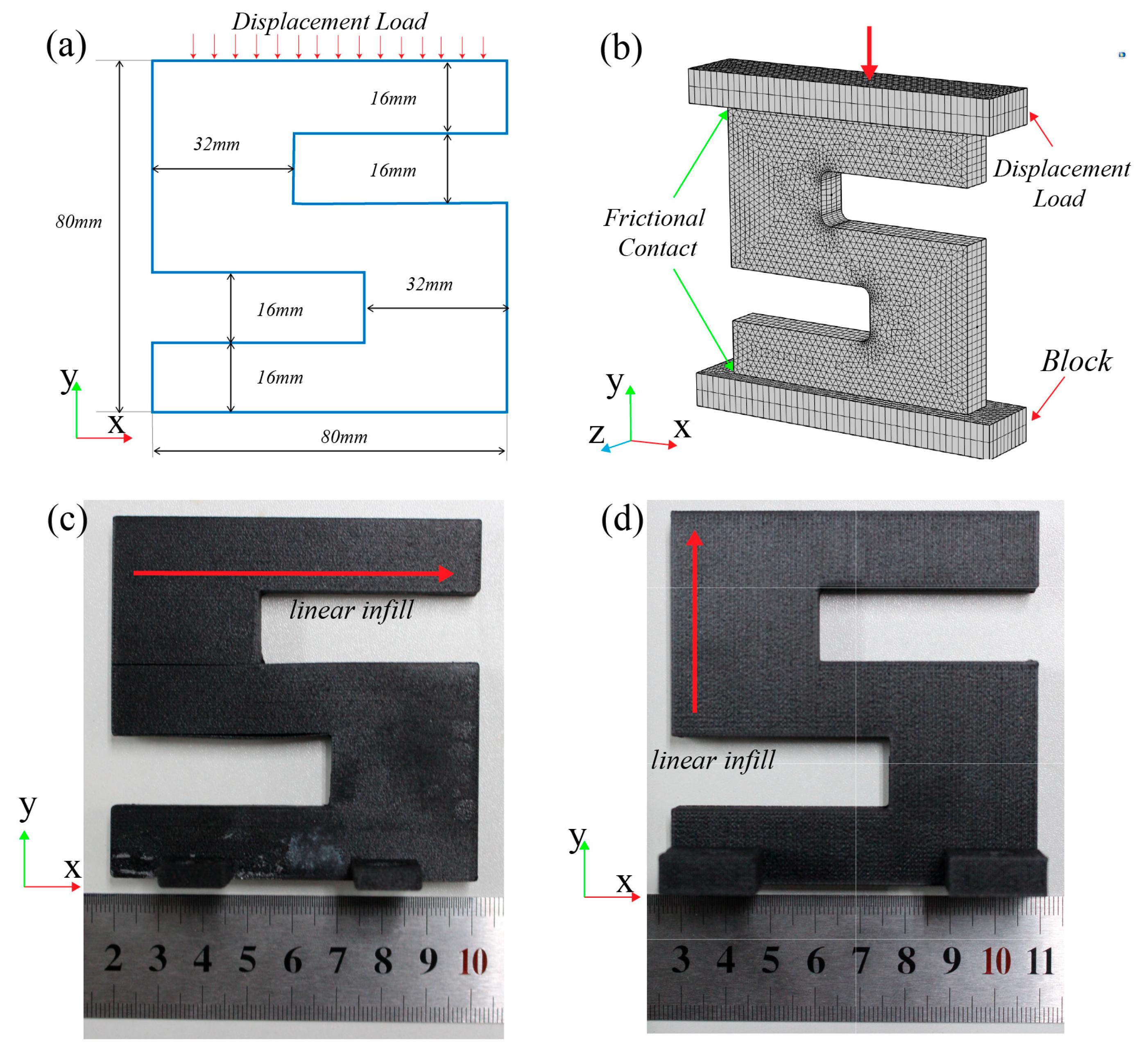
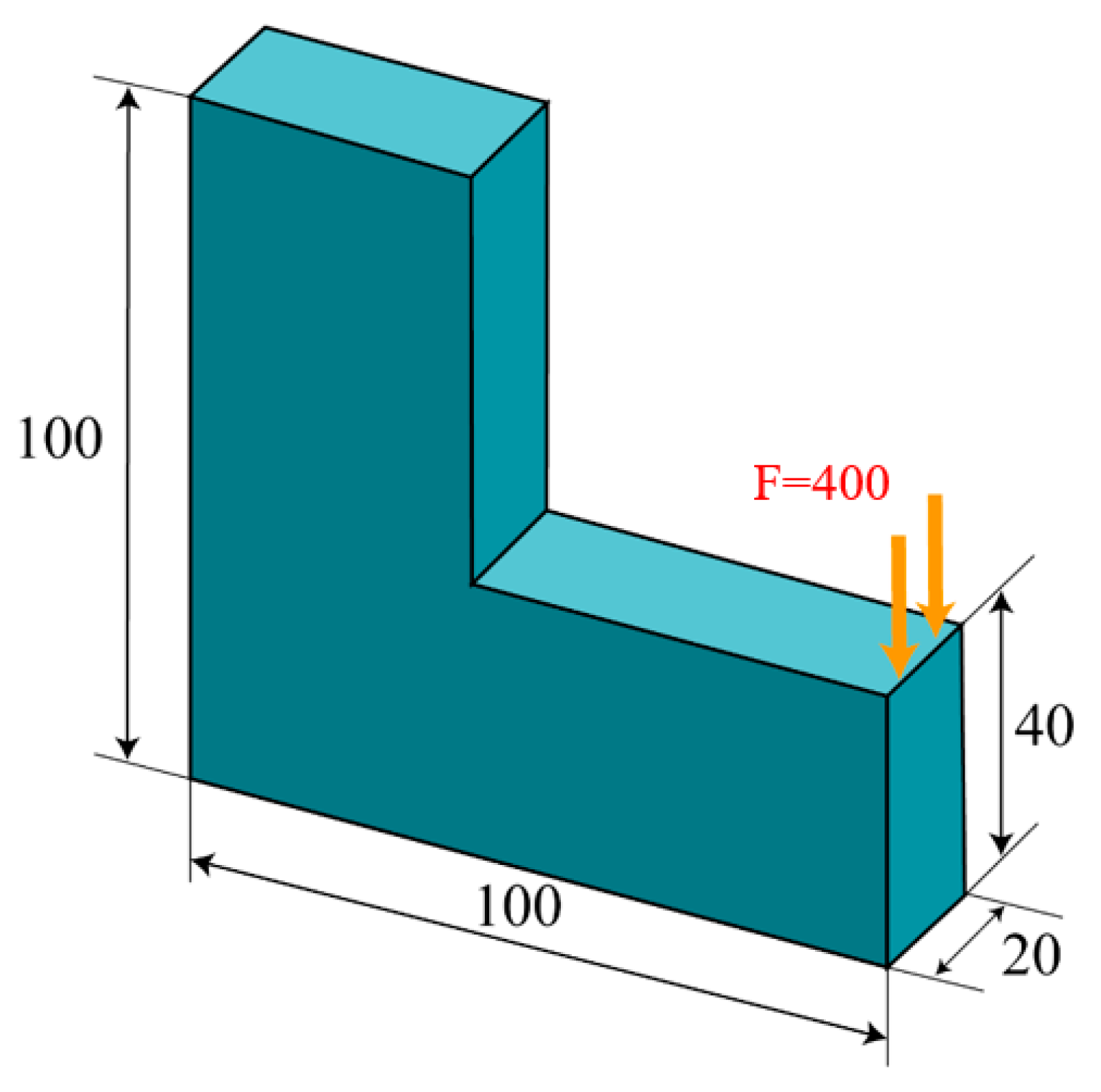
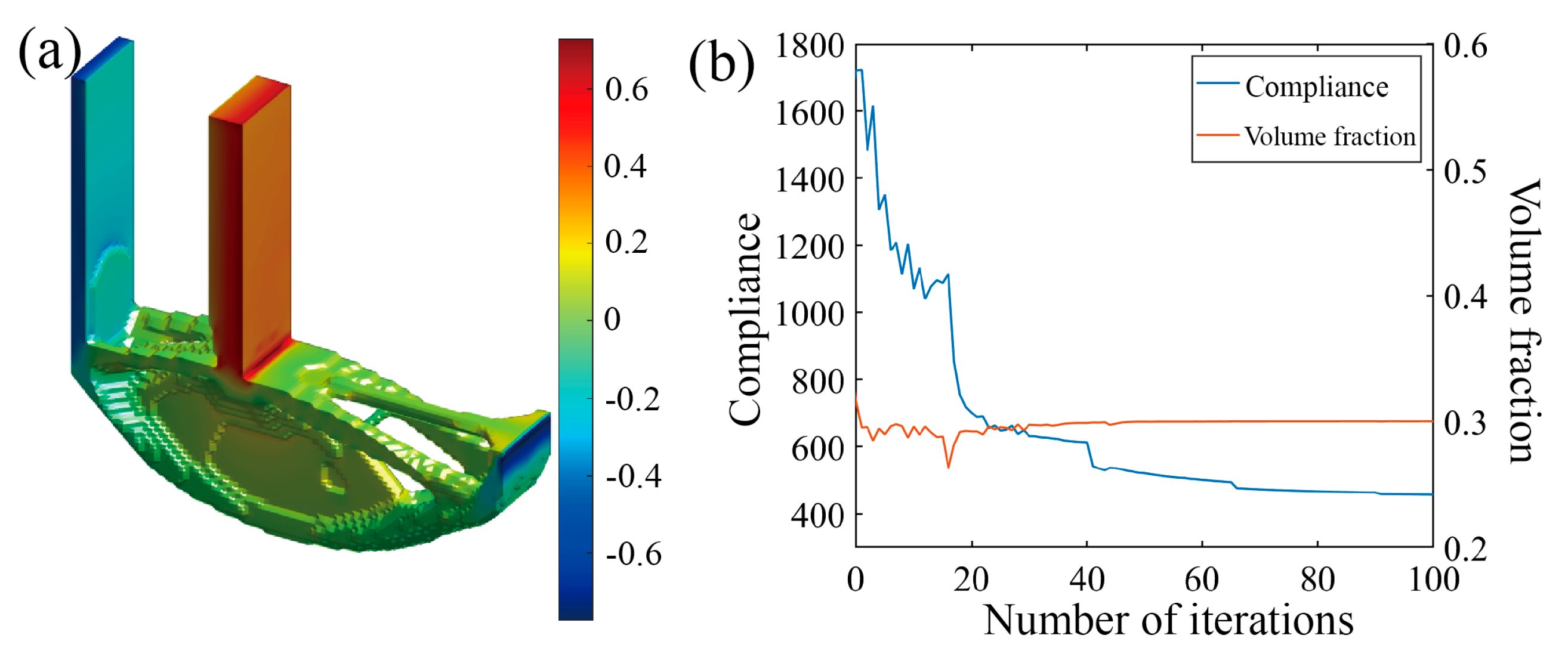
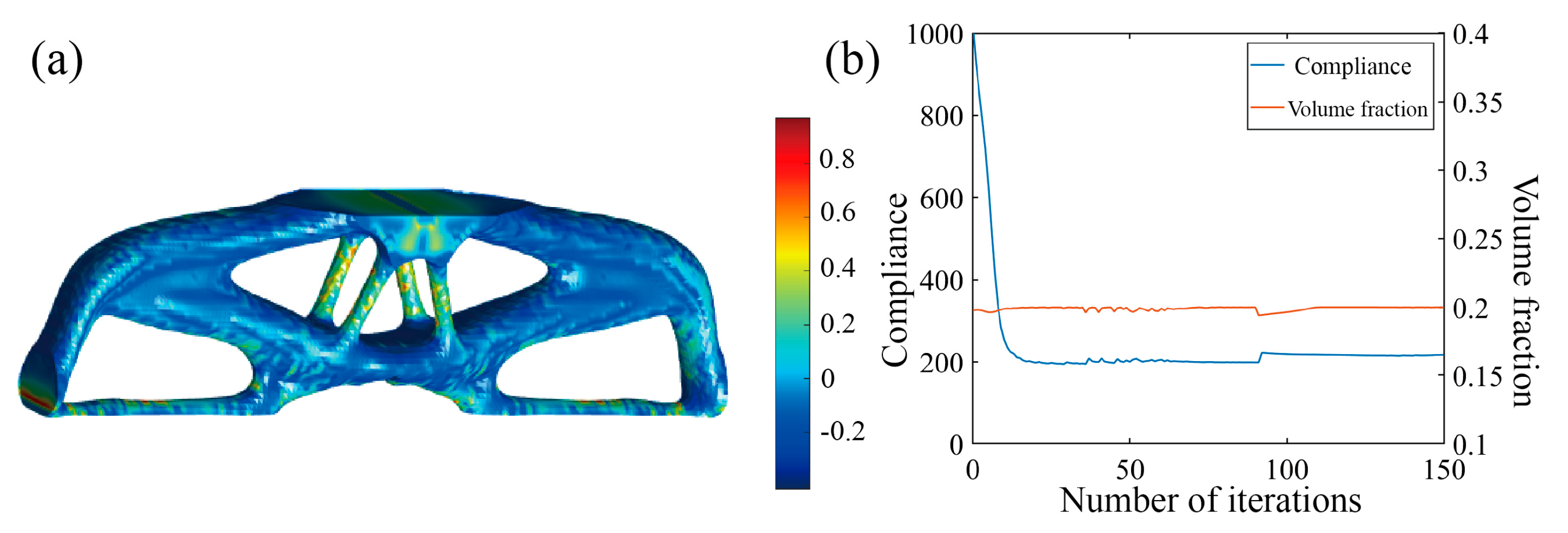
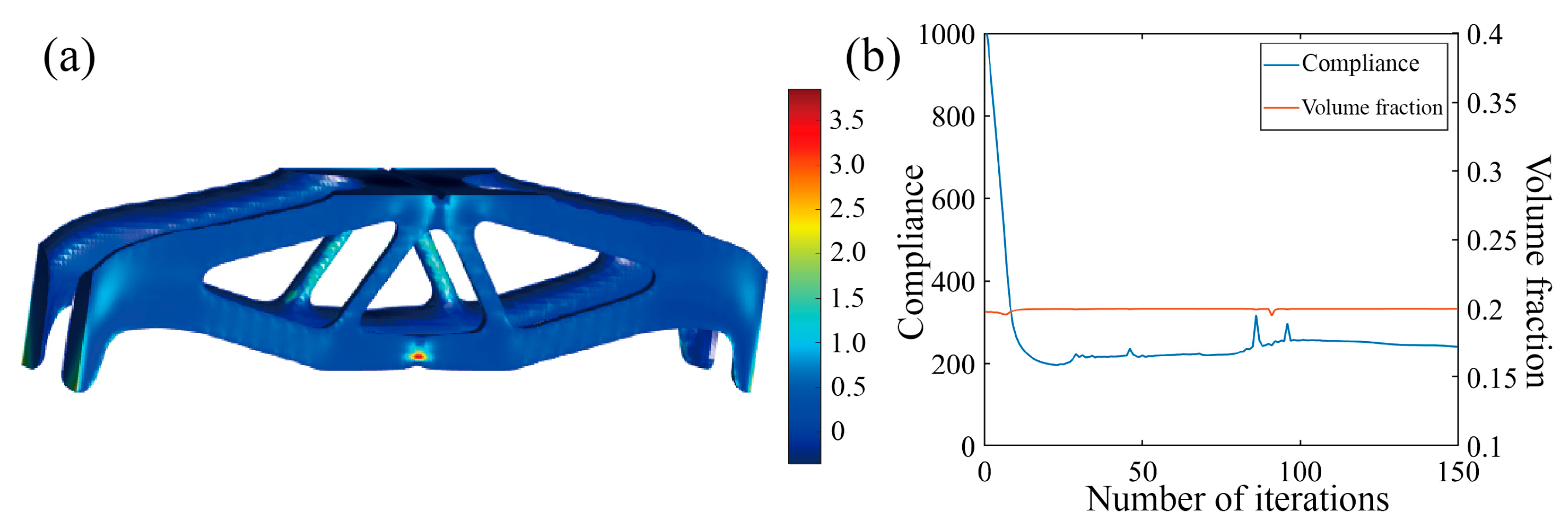
5.3. L-Shaped Bracket Structure
5.4. The MBB Beam with a Notch
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| , , , , | Linear fitting parameters of X-type specimen, index i varies from 1 to 6 |
| , , , , | Linear fitting parameters of Y-type specimen, index i varies from 1 to 5 |
| , , | Tensile yield strength in the X, Y, and Z directions |
| , , | Compressive yield strength in the X, Y, and Z directions |
| , , | Shear strengths in the XY, YZ, and XZ planes |
| , , | Shear modulus in the XY, YZ, and XZ planes |
| , , | Poisson’s ratio in the XY, YZ, and XZ planes |
| , , | Linear stress term coefficients |
| , , | Quadratic normal stress term coefficients |
| , , | Quadratic shear stress term coefficients |
| , , | Cross stress term coefficients |
| , , | Tensile modulus in X/Y-direction |
| , , | Compressive modulus in X/Y-direction |
| Elastic modulus in Z-direction | |
| Element design variable (density) | |
| Stiffness interpolation penalty factor | |
| Stress interpolation penalty factor | |
| Stiffness of solid material | |
| Tsai–Wu failure index for element | |
| Global P-norm stress aggregation | |
| Compliance (strain energy) | |
| Strain–displacement matrix | |
| Global-to-local displacement transformation matrix | |
| Element stiffness matrix , where is solid element stiffness. | |
| Global stiffness matrix | |
| Global load vector | |
| Material elasticity matrix | |
| λ | Adjoint variable |
| Volume fraction constraint | |
| P-norm aggregation parameter |
References
- Ngo, T.D.; Kashani, A.; Imbalzano, G.; Nguyen, K.T.Q.; Hui, D. Additive manufacturing (3D printing): A review of materials, methods, applications and challenges. Compos. Part B Eng. 2018, 143, 172–196. [Google Scholar] [CrossRef]
- Huang, J.; Chen, Q.; Jiang, H.; Zou, B.; Li, L.; Liu, J.; Yu, H. A survey of design methods for material extrusion polymer 3D printing. Virtual Phys. Prototyp. 2020, 15, 148–162. [Google Scholar] [CrossRef]
- Magri, A.E.; Vanaei, S.; Vaudreuil, S. An overview on the influence of process parameters through the characteristic of 3D-printed PEEK and PEI parts. High Perform. Polym. 2021, 33, 862–880. [Google Scholar] [CrossRef]
- Huang, C.; Lv, D.; Zhu, Y.; Chen, G.; Chen, M.; Zhang, Y.; Han, Y.; Wu, H. Influences of heat treatment on mechanical properties of SCF/PEEK composites in FDM-3D printing process with UV laser assistance. Polym. Compos. 2024, 45, 12597–12610. [Google Scholar] [CrossRef]
- Wang, P.; Zou, B.; Xiao, H.; Ding, S.; Huang, C. Effects of printing parameters of fused deposition modeling on mechanical properties, surface quality, and microstructure of PEEK. J. Mater. Process. Technol. 2019, 271, 62–74. [Google Scholar] [CrossRef]
- Stepashkin, A.A.; Chukov, D.I.; Senatov, F.S.; Salimon, A.I.; Korsunsky, A.M.; Kaloshkin, S.D. 3D-printed PEEK-carbon fiber (CF) composites: Structure and thermal properties. Compos. Sci. Technol. 2018, 164, 319–326. [Google Scholar] [CrossRef]
- Ding, S.; Zou, B.; Wang, P.; Ding, H. Effects of nozzle temperature and building orientation on mechanical properties and microstructure of PEEK and PEI printed by 3D-FDM. Polym. Test. 2019, 78, 105948. [Google Scholar] [CrossRef]
- Liu, L.; Qu, D.; Wang, J.; Zhang, J.; Cao, H.; Dong, X. Thermal-field analytical modeling of machined surface layer in high-speed-dry milling UD-CF/PEEK considering thermal anisotropy and nonlinear thermal conductivity. Compos. Part Appl. Sci. Manuf. 2024, 176, 107864. [Google Scholar] [CrossRef]
- Yan, X.; Zhao, H.; Wen, Z.; Wang, Y.; Li, P.; Ding, H. Investigation on grinding temperature characteristics of CF/PEEK and material removal mechanism under temperature treatment. Compos. Sci. Technol. 2024, 252, 110621. [Google Scholar] [CrossRef]
- Lei, M.; Hamel, C.M.; Chen, K.; Zhao, Z.; Lu, H.; Yu, K.; Qi, H.J. Thermomechanical behaviors of polyether ether ketone (PEEK) with stretch-induced anisotropy. J. Mech. Phys. Solids 2021, 148, 104271. [Google Scholar] [CrossRef]
- Liu, W.; Liu, J.; Li, X.; Huang, J.; Chen, Y.; Li, Y.; Ma, J.; Cui, H. Anisotropic elastoplasticity and fracture of SCFR-PEEK composites in complex biaxial loading: Experiments and modelling. Compos. Sci. Technol. 2024, 251, 110569. [Google Scholar] [CrossRef]
- Zhang, P.; Liu, J.; To, A.C. Role of anisotropic properties on topology optimization of additive manufactured load bearing structures. Scr. Mater. 2017, 135, 148–152. [Google Scholar] [CrossRef]
- Somireddy, M.; Czekanski, A. Anisotropic material behavior of 3D printed composite structures—Material extrusion additive manufacturing. Mater. Des. 2020, 195, 108953. [Google Scholar] [CrossRef]
- Liu, J.; Yu, H. Concurrent deposition path planning and structural topology optimization for additive manufacturing. Rapid Prototyp. J. 2017, 23, 930–942. [Google Scholar] [CrossRef]
- Liu, J.; To, A.C. Deposition path planning-integrated structural topology optimization for 3D additive manufacturing subject to self-support constraint. Comput.-Aided Des. 2017, 91, 27–45. [Google Scholar] [CrossRef]
- Dapogny, C.; Estevez, R.; Faure, A.; Michailidis, G. Shape and topology optimization considering anisotropic features induced by additive manufacturing processes. Comput. Methods Appl. Mech. Eng. 2019, 344, 626–665. [Google Scholar] [CrossRef]
- Meng, K.; Fu, J.; Qu, D.; Li, L.; Liu, J. Dynamic topology optimization incorporating the material anisotropy feature for 3D printed fiber composite structures. Finite Elem. Anal. Des. 2025, 243, 104281. [Google Scholar] [CrossRef]
- Ibitoye, F.A.; Radford, D.W. Experimental and statistical study on the effect of process parameters on the quality of continuous fiber composites made via additive manufacturing. J. Thermoplast. Compos. Mater. 2024, 37, 3860–3889. [Google Scholar] [CrossRef]
- Wang, Z.; Fang, Z.; Xie, Z.; Smith, D.E. A Review on Microstructural Formations of Discontinuous Fiber-Reinforced Polymer Composites Prepared via Material Extrusion Additive Manufacturing: Fiber Orientation, Fiber Attrition, and Micro-Voids Distribution. Polymers 2022, 14, 4941. [Google Scholar] [CrossRef]
- Abas, M.; Awadh, M.A.; Habib, T.; Noor, S. Analyzing Surface Roughness Variations in Material Extrusion Additive Manufacturing of Nylon Carbon Fiber Composites. Polymers 2023, 15, 3633. [Google Scholar] [CrossRef]
- Rueschhoff, L.M.; Baldwin, L.A.; Hardin, J.O.; Kaufman, J. Future directions in ceramic additive manufacturing: Fiber reinforcements and artificial intelligence. J. Am. Ceram. Soc. 2023, 107, 1505–1522. [Google Scholar] [CrossRef]
- Liu, P.; Lu, L.; Liu, J. Path-driven shell lattices designed for continuous fiber composite 3D printing. Addit. Manuf. 2023, 78, 103838. [Google Scholar] [CrossRef]
- Pascual-González, C.; Caraballo, J.G.-M.; Lizarralde, I.; Gómez, D.G.; Fernández-Blázquez, J.P. Additive manufacturing and microstructure effects on thermal and mechanical properties of ply-hybrid carbon and glass fiber composites. Compos. Part B Eng. 2024, 279, 111446. [Google Scholar] [CrossRef]
- Luo, M.; Tian, X.; Shang, J.; Zhu, W.; Li, D.; Qin, Y. Impregnation and interlayer bonding behaviours of 3D-printed continuous carbon-fiber-reinforced poly-ether-ether-ketone composites. Compos. Part Appl. Sci. Manuf. 2019, 121, 130–138. [Google Scholar] [CrossRef]
- Sun, Q.; Wen, X.; Yin, G.; Jia, Z.; Yang, X. Thermodynamic Coupling Forming Performance of Short Fiber-Reinforced PEEK by Additive Manufacturing. Polymers 2024, 16, 1789. [Google Scholar] [CrossRef]
- Wang, P.; Zou, B. Improvement of Heat Treatment Process on Mechanical Properties of FDM 3D-Printed Short- and Continuous-Fiber-Reinforced PEEK Composites. Coatings 2022, 12, 827. [Google Scholar] [CrossRef]
- Ayten, A.İ.; Şahbaz, M.; Morkavuk, S.; Polat, Y.; Seyhan, A. Effect of solution-blown polyamide-6 nanofiber interlayer on drilling performance of carbon fiber reinforced composites. J. Reinf. Plast. Compos. 2025. (Online First). Available online: https://api.semanticscholar.org/CorpusID:276217523 (accessed on 22 February 2025).
- Barocio, E.; Brenken, B.; Favaloro, A.; Pipes, R.B. Interlayer fusion bonding of semi-crystalline polymer composites in extrusion deposition additive manufacturing. Compos. Sci. Technol. 2022, 230, 109334. [Google Scholar] [CrossRef]
- Dialami, N.; Chiumenti, M.; Cervera, M.; Chasco, U.; Reyes-Pozo, G.; Pérez, M.A. A hybrid numerical-experimental strategy for predicting mechanical response of components manufactured via FFF. Compos. Struct. 2022, 298, 115998. [Google Scholar] [CrossRef]
- Tang, R.; Zhang, C.; Liu, J. Concurrent Topological Structure and Cross-Infill Angle Optimization for Material Extrusion Polymer Additive Manufacturing with Microstructure Modeling. Micromachines 2022, 13, 852. [Google Scholar] [CrossRef]
- Alaimo, G.; Marconi, S.; Costato, L.; Auricchio, F. Influence of meso-structure and chemical composition on FDM 3D-printed parts. Compos. Part B Eng. 2017, 113, 371–380. [Google Scholar] [CrossRef]
- Li, M.; Xu, Y.; Fang, J. Orthotropic mechanical properties of PLA materials fabricated by fused deposition modeling. Thin-Walled Struct. 2024, 199, 111800. [Google Scholar] [CrossRef]
- Luca, A.D.; Caputo, F. A review on analytical failure criteria for composite materials. AIMS Mater. Sci. 2017, 4, 1165–1185. [Google Scholar] [CrossRef]
- Pang, S.S.; Pandian, A.; Bradshaw, R.D. Modified Tsai-Wu failure criterion for fiber-reinforced composite laminates. Polym. Compos. 1992, 13, 273–277. [Google Scholar] [CrossRef]
- Tefera, G.; Adali, S.; Bright, G. Flexural failure properties of fiber-reinforced hybrid laminated beam subject to three-point bending. Sci. Rep. 2024, 14, 9792. [Google Scholar] [CrossRef]
- Luo, J.; Zou, K.; Luo, Q.; Li, Q.; Sun, G. On asymmetric failure in additively manufactured continuous carbon fiber reinforced composites. Compos. Part B Eng. 2024, 284, 111605. [Google Scholar] [CrossRef]
- Ding, S.; Kong, L.; Jin, Y.; Lin, J.; Chang, C.; Li, H.; Liu, E.; Liu, H. Influence of the molding angle on tensile properties of FDM parts with orthogonal layering. Polym. Adv. Technol. 2020, 31, 873–884. [Google Scholar] [CrossRef]
- Jain, N.; Koch, D. Prediction of Failure in Ceramic Matrix Composites Using Damage-Based Failure Criterion. J. Compos. Sci. 2020, 4, 183. [Google Scholar] [CrossRef]
- Xiao, Z.; Yang, Y.; Xiao, R.; Bai, Y.; Song, C.; Wang, D. Evaluation of topology-optimized lattice structures manufactured via selective laser melting. Mater. Des. 2018, 143, 27–37. [Google Scholar] [CrossRef]
- Liu, J.; Yan, J.; Yu, H. Stress-constrained topology optimization for material extrusion polymer additive manufacturing. J. Comput. Des. Eng. 2021, 8, 979–993. [Google Scholar] [CrossRef]
- Bendsøe, M.P.; Sigmund, O. Material interpolation schemes in topology optimization. Arch. Appl. Mech. 1999, 69, 635–654. [Google Scholar] [CrossRef]
- Querin, O.M.; Steven, G.P.; Xie, Y.M. Evolutionary structural optimisation using an additive algorithm. Finite Elem. Anal. Des. 2000, 34, 291–308. [Google Scholar] [CrossRef]
- Sethian, J.A.; Wiegmann, A. Structural Boundary Design via Level Set and Immersed Interface Methods. J. Comput. Phys. 2000, 163, 489–528. [Google Scholar] [CrossRef]
- Fu, Y.-F.; Long, K.; Zolfagharian, A.; Bodaghi, M.; Rolfe, B. Topological design of cellular structures for maximum shear modulus using homogenization. Mater. Today Proc. 2024, 101, 38–42. [Google Scholar] [CrossRef]
- Noman, A.A.; Shaari, M.S.; Mehboob, H.; Azman, A.H. Recent advancements in additively manufactured hip implant design using topology optimization technique. Results Eng. 2025, 25, 103932. [Google Scholar] [CrossRef]
- Xu, S.; Liu, J.; He, D.; Tang, K.; Yaji, K. Self-support structure topology optimization for multi-axis additive manufacturing incorporated with curved layer slicing. Comput. Methods Appl. Mech. Eng. 2025, 438, 117841. [Google Scholar] [CrossRef]
- Li, P.; Wu, Y.; Yvonnet, J. A SIMP-phase field topology optimization framework to maximize quasi-brittle fracture resistance of 2D and 3D composites. Theor. Appl. Fract. Mech. 2021, 114, 102919. [Google Scholar] [CrossRef]
- Jiang, H.; He, Z.; Tan, H.; Li, E. Robust topology optimization of structures considering additive manufacturing-induced material anisotropy and uncertainty. Appl. Math. Model. 2024, 136, 115635. [Google Scholar] [CrossRef]
- ISO 527-4:2023; Plastics—Determination of Tensile Properties—Part 4: Test Conditions for Isotropic and Orthotropic Fibre-Reinforced Plastic Composites. International Organization for Standardization: Geneva, Switzerland, 2023.
- ASTM D7078-2020; Standard Test Method for Shear Properties of Composite Materials by V-Notched Rail Shear Method. ASTM International: West Conshohocken, PA, USA, 2020.
- GB/T 1448-2005; Fiber-Reinforced Plastics—Determination of Compressive Properties. Standardization Administration of the People’s Republic of China: Beijing, China, 2005.
- He, M.; Chen, X.; Guo, Z.; Qiu, X.; Yang, Y.; Su, C.; Jiang, N.; Li, Y.; Sun, D.; Zhang, L. Super tough graphene oxide reinforced polyetheretherketone for potential hard tissue repair applications. Compos. Sci. Technol. 2019, 174, 194–201. [Google Scholar] [CrossRef]
- Li, J.; Yan, S.; Kong, W.; Li, S. Validation of the fully rationalized Tsai-Wu failure criterion for unidirectional laminates under multiaxial stress states through a ring-on-ring test. Compos. Sci. Technol. 2024, 257, 110813. [Google Scholar] [CrossRef]
- Bendsøe, M.P.; Sigmund, O. Topology Optimization; Springer Berlin Heidelberg: Berlin, Heidelberg, 2004; Available online: http://link.springer.com/10.1007/978-3-662-05086-6 (accessed on 27 May 2016).
- Xu, S.; Liu, J.; Zou, B.; Li, Q.; Ma, Y. Stress constrained multi-material topology optimization with the ordered SIMP method. Comput. Methods Appl. Mech. Eng. 2021, 373, 113453. [Google Scholar] [CrossRef]






| Printing Parameters | Value |
|---|---|
| Nozzle diameter (mm) | 0.4 |
| Nozzle temperature (℃) | 450 |
| Platform temperature (℃) | 180 |
| Layer thickness (mm) | 0.2 |
| Printing speed (mm/s) | 10 |
| Infill density (%) | 100 |
| Wall thickness (mm) | 0.4 |
| Infill pattern | Lines |
| Overlap interval (mm) | 0 |
| Parameter | Values | Parameter | Values | Parameter | Values |
|---|---|---|---|---|---|
| 1.34 × 103 | 9.25 × 10−6 | 6.57 × 10−4 | |||
| 7.66 × 102 | −9.04 × 10−3 | 1.07 × 10−2 | |||
| 4.65 × 102 | 7.94 × 10−3 | 8.28 × 10−3 | |||
| 6.86 × 103 | 1.49 × 10−3 | 3.56 × 10−2 | |||
| 2.76 × 102 | −2.17 × 10−2 | 5.73 × 10−3 | |||
| −2.34 × 103 | −8.87 × 10−4 | 4.81 × 10−2 | |||
| 1.98 × 102 | −3.78 × 100 | 7.44 × 100 | |||
| 1.58 × 103 | −9.20 × 10−2 | 9.13 × 10−2 | |||
| −7.51 × 103 | −1.81 × 10−2 | 9.42 × 10−2 | |||
| 1.02 × 104 | −6.85 × 10−3 | 8.13 × 10−2 | |||
| 5.61 × 102 | 1.25 × 10−1 | 9.07 × 10−2 | |||
| 6.28 × 102 | 7.48 × 10−4 | 1.14 × 10−3 | |||
| 1.06 × 10³ | 1.58 × 10−3 | 2.66 × 10−3 | |||
| 1.79 × 102 | 3.82 × 10−3 | 5.66 × 10−3 | |||
| 3.33 × 103 | 1.25 × 10−2 | 1.14 × 10−2 | |||
| 1.51 × 1016 | 7.02 × 10−0 | 1.27 × 10−1 | |||
| 1.27 × 103 | −2.51 × 10−2 | 4.29 × 10−2 | |||
| 1.29 × 102 | −7.33 × 10−2 | 3.07 × 10−2 | |||
| 4.34 × 102 | −9.49 × 10−2 | 4.96 × 10−2 | |||
| 5.34 × 102 | −1.79 × 10−1 | 9.40 × 10−2 |
| Parameter | Values/MPa | Parameter | Values/MPa | Parameter | Values |
|---|---|---|---|---|---|
| 103 | 110 | 0.29 | |||
| 54 | 71 | 0.40 | |||
| 30 | 146 | 0.35 | |||
| 40 | 852 | ||||
| 27 | 532 | ||||
| 30 | 804 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ye, Y.; Yang, Z.; Qu, D.; Hu, B.; Li, L. Orthotropic Constitutive Modeling and Tsai–Wu Failure Criterion for Carbon Fiber-Reinforced PEEK Composites. Polymers 2025, 17, 1076. https://doi.org/10.3390/polym17081076
Ye Y, Yang Z, Qu D, Hu B, Li L. Orthotropic Constitutive Modeling and Tsai–Wu Failure Criterion for Carbon Fiber-Reinforced PEEK Composites. Polymers. 2025; 17(8):1076. https://doi.org/10.3390/polym17081076
Chicago/Turabian StyleYe, Yu, Zixin Yang, Dianwei Qu, Bingyin Hu, and Lei Li. 2025. "Orthotropic Constitutive Modeling and Tsai–Wu Failure Criterion for Carbon Fiber-Reinforced PEEK Composites" Polymers 17, no. 8: 1076. https://doi.org/10.3390/polym17081076
APA StyleYe, Y., Yang, Z., Qu, D., Hu, B., & Li, L. (2025). Orthotropic Constitutive Modeling and Tsai–Wu Failure Criterion for Carbon Fiber-Reinforced PEEK Composites. Polymers, 17(8), 1076. https://doi.org/10.3390/polym17081076





