Research on Curing Reaction Kinetics and Curing Process of Nitrate Ester Plasticized Polyether (NEPE) Propellants
Abstract
1. Introduction
2. Experiment Setup
2.1. Material
2.2. Sample Preparation
2.3. Curing Experiment
2.4. Non-Isothermal DSC Method
3. Theoretical Models
3.1. Thermo-Chemical Model
3.2. Finite Element Modeling
4. Results and Discussion
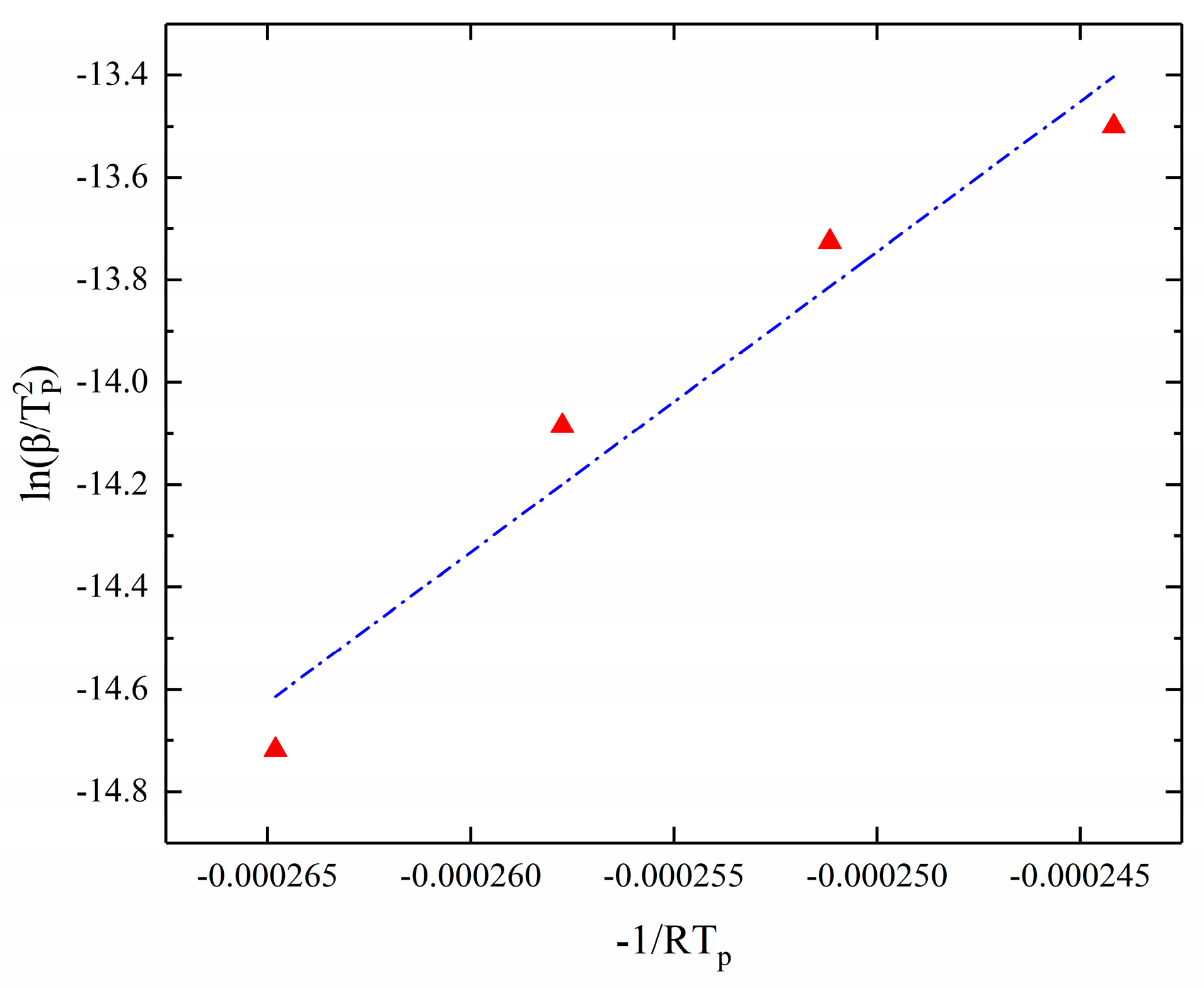
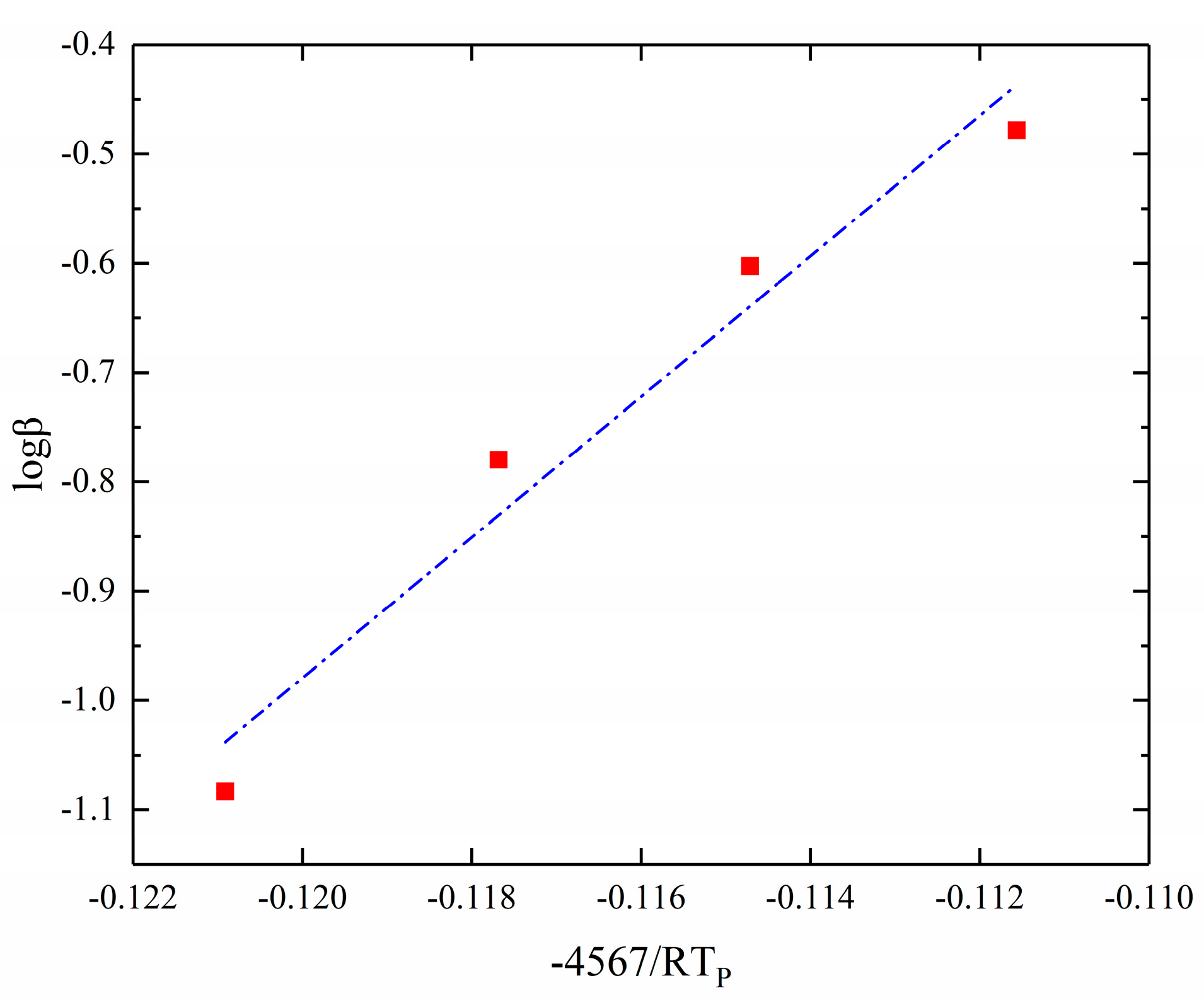
4.1. Curing Reaction Kinetics Based on Non-Theermal DSC Method
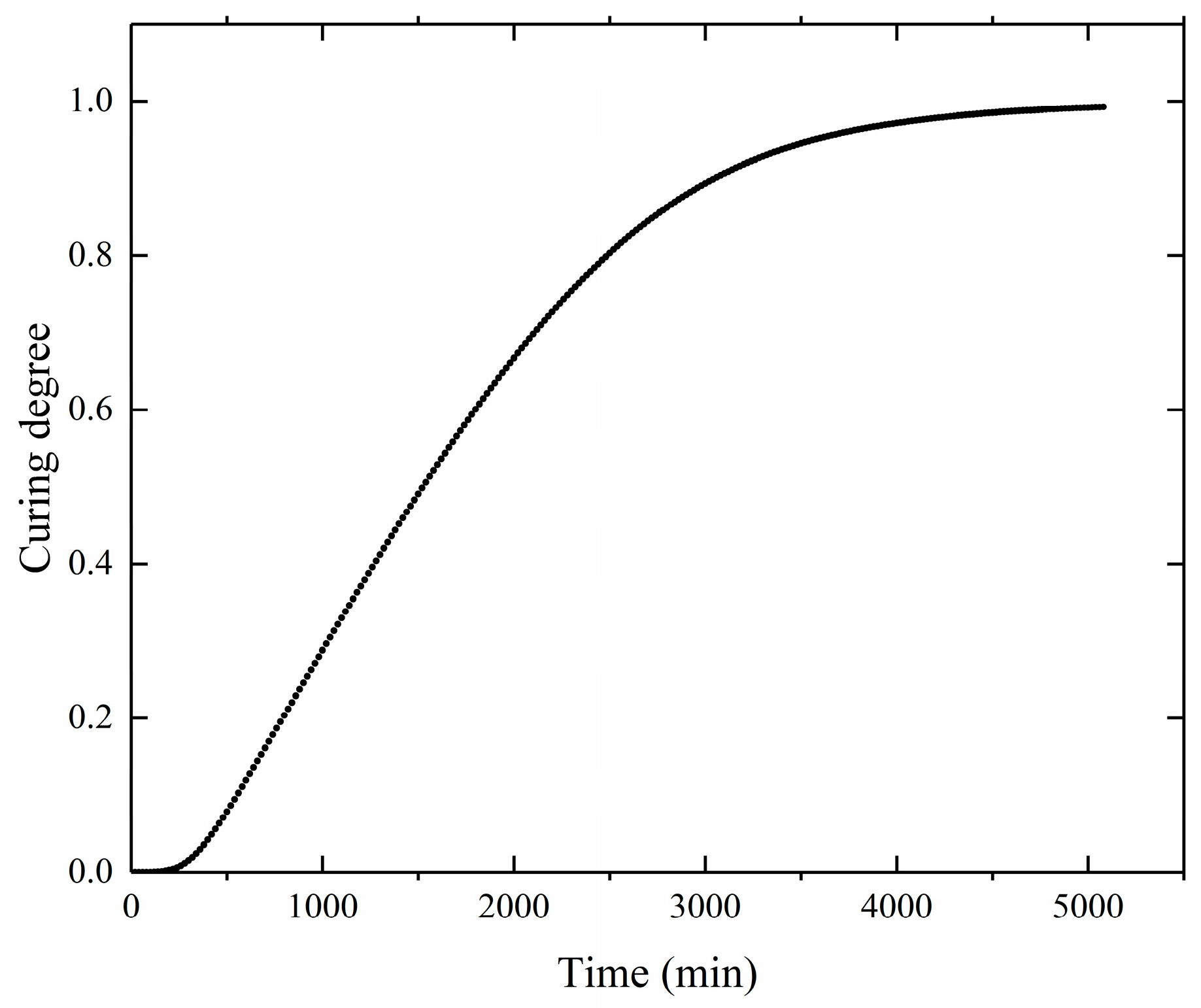
4.2. Temperature and Curing Degree During Curing Process
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Tao, T.; Sui, X.; Li, S.; Wang, N. A Study on Consumption and Characterization of Stabilizer Content of NEPE Propellant via FTIR. Propellants Explos. Pyrotech. 2019, 44, 889–895. [Google Scholar] [CrossRef]
- Liu, H.; Wang, J.; Fu, X. Research on Crack Propagation of Nitrate Ester Plasticized Polyether Propellant: Experiments and Simulation. Materials 2024, 17, 2180. [Google Scholar] [CrossRef]
- Abdillah, L.; Winardi, S.; Sumarno, S.; Nurtono, T. Effect of Mixing Time to Homog. of Propellant Slurry. IPTEK J. Proc. Ser. 2018, 4, 94. [Google Scholar] [CrossRef]
- Guo, Y.; Jiang, B.; Liu, L.; Ma, Y.; Li, J.; Ao, W.; Sun, Q.; Wang, Z.; Liu, P.; Zhao, J. In Situ Monitoring of Curing Reaction in Solid Composite Propellant with Fiber-Optic Sensors. ACS Sens. 2023, 8, 2664–2672. [Google Scholar] [CrossRef] [PubMed]
- Zhang, P.; Yuan, J.; Pang, A.; Tang, G.; Deng, J. A Novel UV-Curing Liner for NEPE Propellant: Insight from Molecular Simulations. Compos. Part. B Eng. 2020, 195, 108087. [Google Scholar] [CrossRef]
- Simon, S.L.; Mckenna, G.B.; Sindt, O. Modeling the Evolution of the Dynamic Mechanical Properties of a Commercial Epoxy during Cure after Gelation. J. Appl. Polym. Sci. 2000, 76, 495–508. [Google Scholar] [CrossRef]
- Gui, D.; Zong, Y.; Ding, S.; Li, C.; Zhang, Q.; Wang, M.; Liu, J.; Chi, X.; Ma, X.; Pang, A. In–Situ Characterization and Cure Kinetics in NEPE Propellant/HTPB Liner Interface by Microscopic FT-IR. Propellants Explos. Pyrotech. 2017, 42, 410–416. [Google Scholar] [CrossRef]
- Zhang, X.; Chang, X.L.; Ma, R.L.; Zhang, L.; Chen, X.D.; Zhang, Q. Numerical Simulation of Temperature Distribution in the Glass Fiber Epoxy Composites during Microwave Curing. MATEC Web Conf. 2018, 206, 03001. [Google Scholar] [CrossRef][Green Version]
- Pang, W.; Fan, X.; Yi, J.; Zhao, F.; Xu, H.; Li, J.; Wang, B.; Li, Y. Thermal Behavior and Non-Isothermal Decomposition Reaction Kinetics of NEPE Propellant with Ammonium Dinitramide. Chin. J. Chem. 2010, 28, 687–692. [Google Scholar] [CrossRef]
- Olalla, B.; Carrot, C.; Fulchiron, R.; Boudimbou, I.; Peuvrel-disdier, E. Analysis of the Influence of Polymer Viscosity on the Dispersion of Magnesium Hydroxide in a Polyolefin Matrix. Rheol. Acta 2012, 51, 235–247. [Google Scholar] [CrossRef]
- Fu, X.; Fan, X. Curing Reaction Kinetics of HTPE Polymer Studied by Simultaneous Rheometry and FTIR Measurements. J. Therm. Anal. Calorim. 2016, 125, 977–982. [Google Scholar] [CrossRef]
- Zhongliang, M.; Le, Q.; Wei, H.; Liming, H. A Novel Approach on the Study of Cure Kinetics for Rheological Isothermal and Non-Isothermal Methods. Compos. Part. B Eng. 2019, 162, 242–249. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Y.; Song, X.; An, C.; Li, F. Rheological Properties and Curing Kinetics of GAP-Based Propellant Slurries. FirePhysChem 2024, 4, 80–86. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, Y.; Chai, T.; Ma, Z.; Jia, K. Curing Reaction Kinetics of the EHTPB-Based PBX Binder System and Its Mechanical Properties. Coatings 2020, 10, 1266. [Google Scholar] [CrossRef]
- Tao, Q.; Pinter, G.; Antretter, T.; Krivec, T.; Fuchs, P. Model Free Kinetics Coupled with Finite Element Method for Curing Simulation of Thermosetting Epoxy Resins. J. Appl. Polym. Sci. 2018, 135, 46408. [Google Scholar] [CrossRef]
- Wei, H.; Liming, H.; Zhongliang, M.; Yanli, G. Using Rheometry to Study the Curing Kinetics of Glycidyl Azide Polymer Spherical Propellant by Non-Isothermal Method. Rheol. Acta 2016, 55, 365–371. [Google Scholar] [CrossRef]
- Rueda, M.M.; Auscher, M.-C.; Fulchiron, R.; Périé, T.; Martin, G.; Sonntag, P.; Cassagnau, P. Rheology and Applications of Highly Filled Polymers: A Review of Current Understanding. Prog. Polym. Sci. 2017, 66, 22–53. [Google Scholar] [CrossRef]
- Restasari, A.; Abdillah, L.H.; Ardianingsih, R.; Prianto, B.; Pinalia, A.; Sitompul, H.R.D.; Kurdianto; Arisandi, E.D.; al Rasyid, H.; Budi, R.S.; et al. Particle Packing Models to Determine Time-Dependent Slip Flow Properties of Highly Filled Polyurethane-Based Propellant. J. Rubber Res. 2022, 25, 157–170. [Google Scholar] [CrossRef]
- Qu, W.; Chen, J.; Li, Z.; Luo, M.; Lu, H.; Hu, X.; Zhu, Q. Rheological Modeling and Simulation of Semi-Solid Slurry Based on Experimental Study. Scr. Mater. 2022, 220, 114932. [Google Scholar] [CrossRef]
- Moghimi Rad, H.; Tavangar Roosta, S.; Motamed Shariati, S.H.; Ghorban Hosseini, S. Numerical Simulation of HTPB Resin Curing Process Using OpenFOAM and Study the Effect of Different Conditions on Its Curing Time. Propellants Explos. Pyrotech. 2021, 46, 1447–1457. [Google Scholar] [CrossRef]
- Yang, W.; Liu, W.; Jia, Y.; Chen, W. Coupled Filling-Curing Simulation and Optimized Design of Cure Cycle in Liquid Composite Molding. Int. J. Adv. Manuf. Technol. 2024, 132, 2489–2501. [Google Scholar] [CrossRef]
- Liu, X.; Xie, X.; Zhou, D.; Wang, R. Numerical Analysis of Curing Residual Stress and Strain in NEPE Propellant Grain. Polymers 2023, 15, 1019. [Google Scholar] [CrossRef] [PubMed]
- Molecular Dynamics Simulation on the Binder of Ethylene Oxide–Tetrahydrofuran Copolyether Cross-Linked with N100. Ind. Eng. Chem. Res. 2015, 54, 3563–35669. [CrossRef]
- Chiumenti, M.; Cervera, M.; Salsi, E.; Zonato, A. A Phenomenological Model for the Solidification of Eutectic and Hypoeutectic Alloys Including Recalescence and Undercooling. J. Heat Transf. 2018, 140. [Google Scholar] [CrossRef]
- Hoque, E.; Ghosal, S.; Patil, R.S.; Jagadeeswar Rao, T.V. Study of Curing Kinetics of 4-(Dimethylsilyl) Butyl Ferrocene Grafted HTPB and Effect of Catalysts by Differential Scanning Calorimetry. Propellants Explos. Pyrotech. 2024, 49, e202400110. [Google Scholar] [CrossRef]






| Material | P(E-CO-T) | Bu-NENA | Al | HMX | AP | Others |
|---|---|---|---|---|---|---|
| Content/% | 5~10 | 10~15 | 10~20 | 10~15 | 40~50 | 1~2 |
| Parameter | Value | Unit |
|---|---|---|
| Density, | 1888 | |
| Specific heat, c | 1256 | |
| Heat conductivity, s | 0.5 | |
| Exothermal heat generation, L | 10,000 | j/kg |
| Ea/(kJ/mol) | R2 | |
| Kissinger | 59.378 | 0.9538 |
| Ozawa | 61.352 | 0.9641 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Y.; Guo, Z.; Yu, H.; Fu, X. Research on Curing Reaction Kinetics and Curing Process of Nitrate Ester Plasticized Polyether (NEPE) Propellants. Polymers 2025, 17, 464. https://doi.org/10.3390/polym17040464
Wu Y, Guo Z, Yu H, Fu X. Research on Curing Reaction Kinetics and Curing Process of Nitrate Ester Plasticized Polyether (NEPE) Propellants. Polymers. 2025; 17(4):464. https://doi.org/10.3390/polym17040464
Chicago/Turabian StyleWu, Yuheng, Zhiming Guo, Hongjian Yu, and Xiaolong Fu. 2025. "Research on Curing Reaction Kinetics and Curing Process of Nitrate Ester Plasticized Polyether (NEPE) Propellants" Polymers 17, no. 4: 464. https://doi.org/10.3390/polym17040464
APA StyleWu, Y., Guo, Z., Yu, H., & Fu, X. (2025). Research on Curing Reaction Kinetics and Curing Process of Nitrate Ester Plasticized Polyether (NEPE) Propellants. Polymers, 17(4), 464. https://doi.org/10.3390/polym17040464






