Dielectric and Ultrasonic Properties of PDMS/TiO2 Nanocomposites
Abstract
1. Introduction
2. Materials and Methods
3. Results
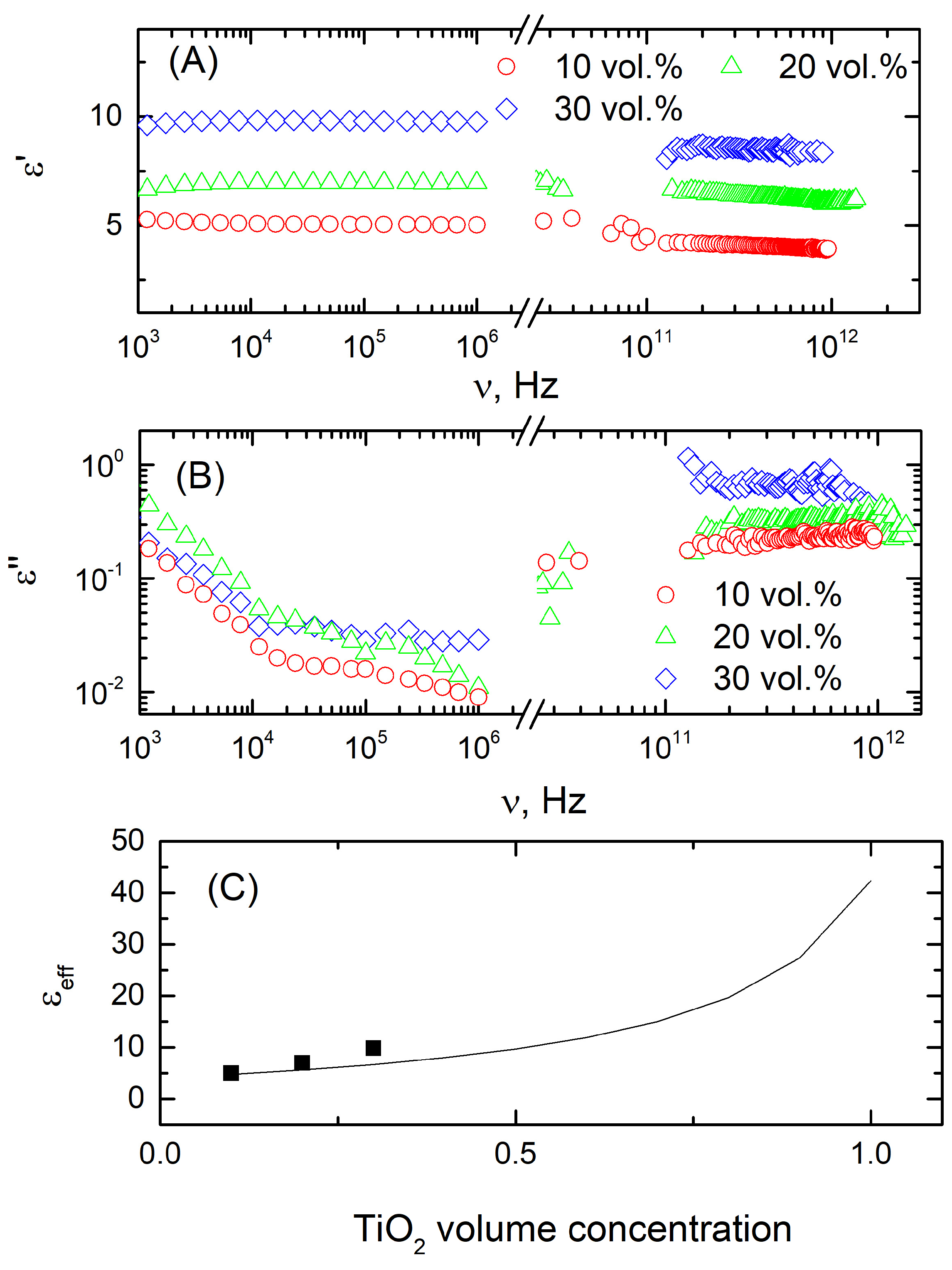
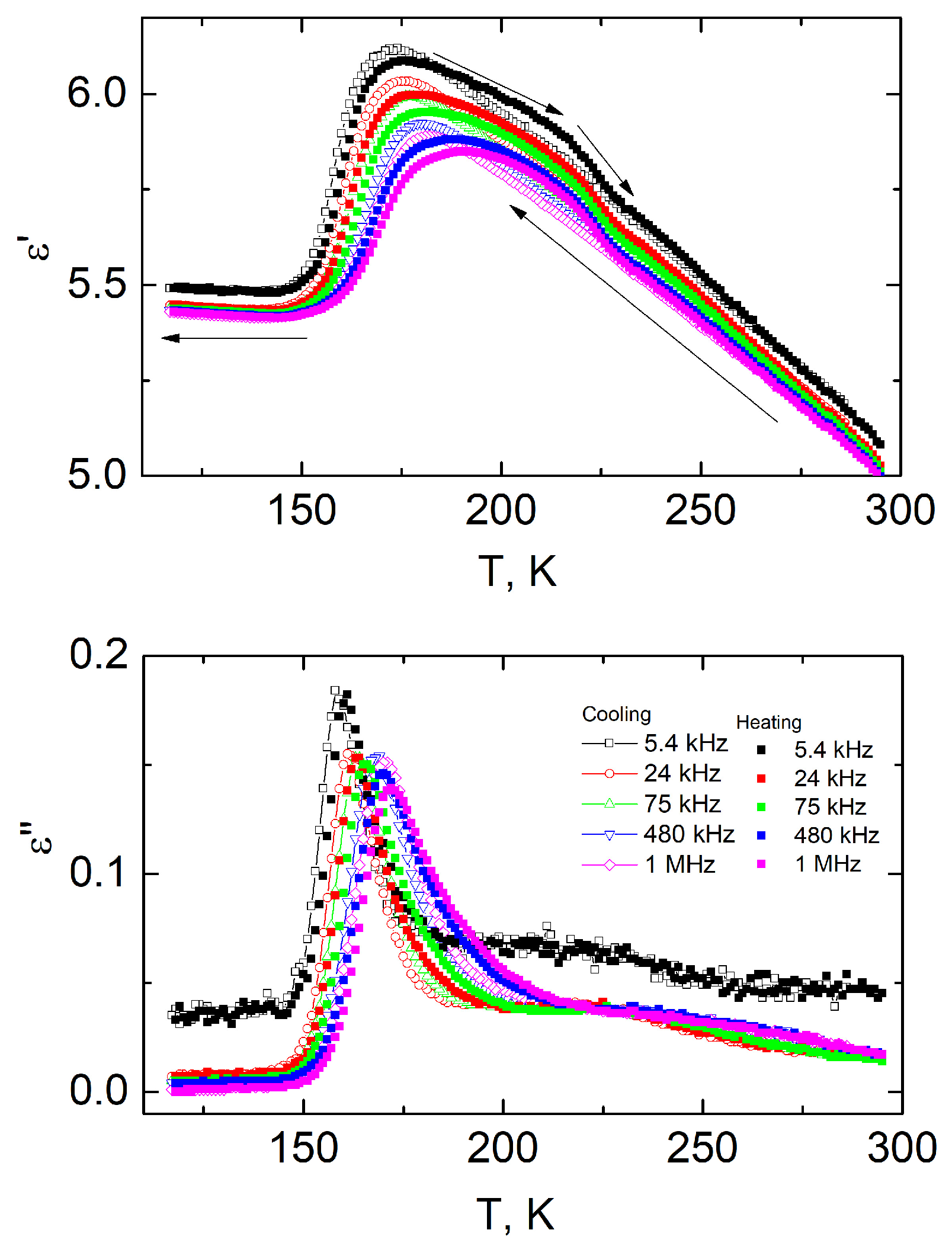
3.1. Structure and Dielectric Properties of Composites
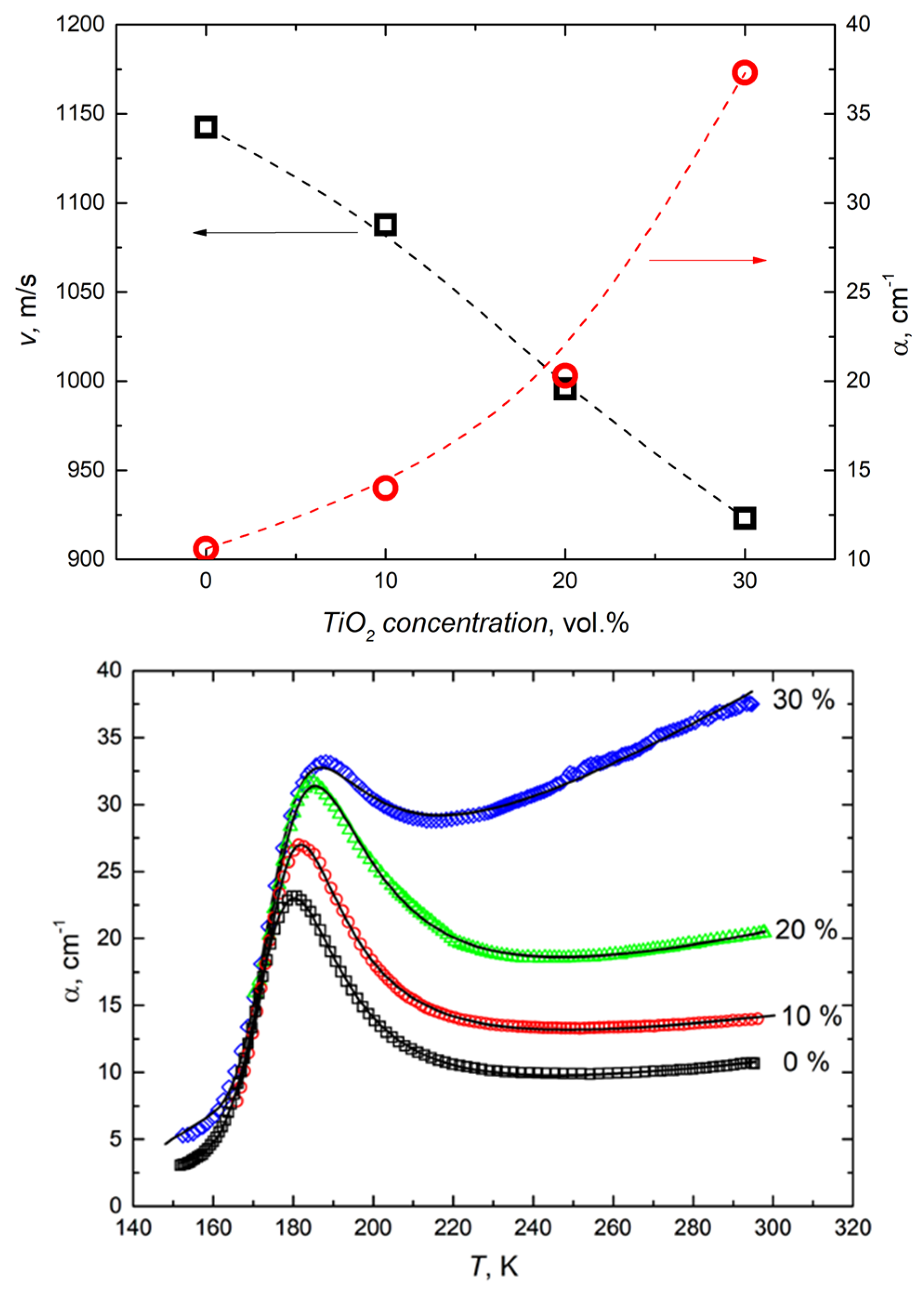
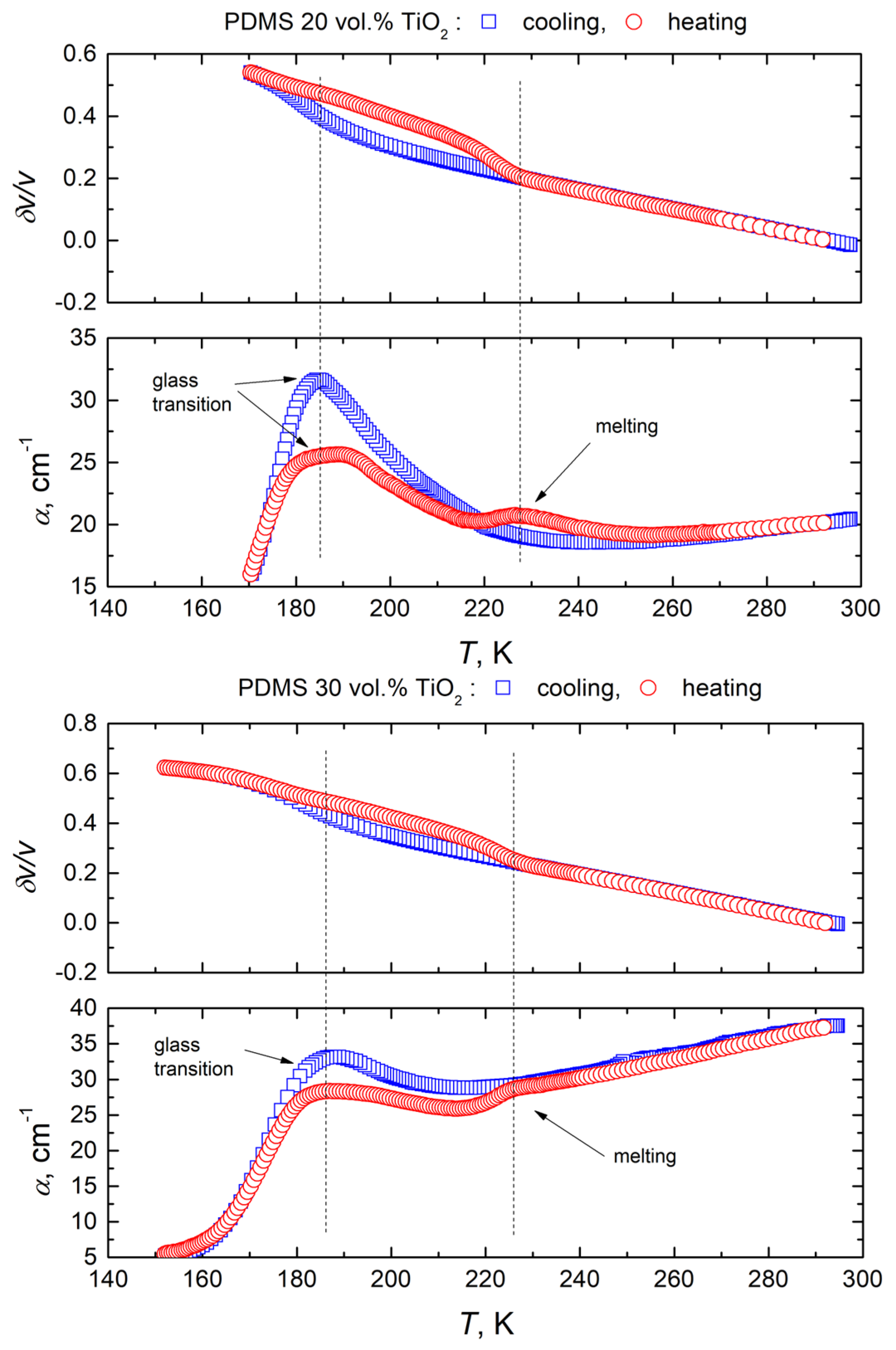
3.2. Ultrasonic Investigations
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Andrews, R.; Weisenberger, M.C. Carbon nanotube polymer composites. Curr. Opin. Solid State Mater. Sci. 2004, 8, 31–37. [Google Scholar] [CrossRef]
- Shahrubudin, N.; Lee, T.C.; Ramlan, R. An overview on 3D printing technology: Technological, materials, and applications. Procedia Manuf. 2019, 35, 1286–1289. [Google Scholar] [CrossRef]
- Maiti, M.; Bhattacharya, M.; Bhowmick, A.K. Elastomer nanocomposites. Rubber Chem. Technol. 2008, 81, 384–469. [Google Scholar] [CrossRef]
- Sia, S.K.; Whitesides, G.M. Microfluidic devices fabricated in poly(dimethylsiloxane) for biological studies. Electrophoresis 2003, 24, 3563. [Google Scholar] [CrossRef]
- Merkel, T.; Bondar, V.; Nagai, K.; Freeman, B.D.; Pinnau, I. Gas sorption, diffusion, and permeation in poly(dimethylsiloxane). J. Polym. Sci. Part B Polym. Phys. 2000, 38, 415–434. [Google Scholar] [CrossRef]
- Chen, D.Z.; Chen, F.X.; Hu, X.Y.; Zhang, H.W.; Yin, X.Z.; Zhou, Y.S. Thermal stability, mechanical and optical properties of novel addition cured PDMS composites with nano-silica sol and MQ silicone resin. Compos. Sci. Technol. 2015, 117, 307–314. [Google Scholar] [CrossRef]
- Lotters, J.C.; Olthuis, W.; Veltinik, P.H.; Bergveld, P. The mechanical properties of the ruber elastic polymer poly(dimethylsiloxane). J. Micromech. Microeng. 1997, 7, 145–147. [Google Scholar] [CrossRef]
- Schonhals, A.; Goernik, H.; Schick, C.; Frick, B.; Zorn, R. Polymers in nanoconfinement: What can be learned from relaxation and scattering experiments? J. Non-Cryst. Solids 2005, 351, 2668–2677. [Google Scholar] [CrossRef]
- Souza, A.; Souza, M.; Pinho, D. 3D manufacturing of intracranial aneurysm biomodels for flow visualizations: Low cost fabrication processes. Mech. Res. Commun. 2020, 107, 103535. [Google Scholar] [CrossRef]
- Carvalho, V.; Maia, I.; Souza, A.; Ribeiro, J.; Costa, P.; Puga, H.; Teixera, S.; Lima, R.A. In vitro biomodels in stenotic arteries to perform blood analogues flow visualizations and measurements: A review. Open Biomed. Eng. J. 2020, 14, 87–102. [Google Scholar] [CrossRef]
- Pinho, D.; Munos-Sanczez, B.N.; Anes, C.F.; Vega, E.J.; Lima, R. Flexible PDMS microparticles to mimic RBC in blood particulate analogue fluids. Mech. Res. Commun. 2019, 100, 103399. [Google Scholar] [CrossRef]
- He, X.; Wang, T.; Huang, J.; Chen, J.; Li, J. Fabrication and characterization of superhydrophobic PDMS composite membranes for efficient ethanol recovery via pervaporation. Sep. Purif. Technol. 2020, 241, 116675. [Google Scholar] [CrossRef]
- Brown, X.O.; Ookawa, K.; Wong, J.Y. Evaluation of poly(dimethylsiloxane) scaffolds with physiologically-relevant elastic moduli: Interplay of substrate mechanics and surface chemistry effects on vascular smooth muscle cell response. Biomaterials 2005, 26, 3123. [Google Scholar] [CrossRef]
- Reyes-Coronado, D.; Rodriguez-Gattorno, G.; Espinosa-Pesqueira, M.E.; Cab, C.; de Coss, R.; Oskam, G. Phase-pure TiO2 nanoparticles: Anatase, brookite, and rutile. Nanotechnology 2008, 19, 145605. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Mao, S.S. Titanium dioxide nanomaterials: Synthesis, properties and applications. Chem. Rev. 2007, 107, 2891–2959. [Google Scholar] [CrossRef] [PubMed]
- Ziental, D.; Czraczynska-Goslinska, B.; Mlynarczyk, D.T.; Glowacka-Sobotta, A.; Stanisz, B.; Goslinski, T.; Sobotta, L. Titanium dioxide nanoparticles: Prospects and applications in medicine. Nanomaterials 2020, 10, 387. [Google Scholar] [CrossRef] [PubMed]
- Wold, A. Photocatalytic properties of TiO2. Chem. Mater. 1993, 5, 280–283. [Google Scholar] [CrossRef]
- Huang, N.; Yang, P.; Leng, Y.X.; Chen, J.Y.; Sun, H.; Wang, J.; Wang, G.J.; Ding, P.D.; Xi, T.F.; Leng, Y. Hemocompatibility of titanium oxide films. Biomaterials 2003, 24, 2177–2188. [Google Scholar] [CrossRef] [PubMed]
- Batzill, M.; Morales, E.H.; Diebold, U. Influence of nitrogen doping on the defect formation and surface properties of TiO2 rutile and anatase. Phys. Lev. Lett. 2006, 96, 026103. [Google Scholar] [CrossRef] [PubMed]
- Nayak, S.; Rahaman, M.; Pandey, A.K.; Setua, D.K.; Chaki, T.K.; Khastgir, D. Development of polydimethylsiloxane-titania nanocomposites with contolled dielectric properties: Effect of heat treatment of titania on electrical properties. J. Appl. Polym. Sci. 2013, 127, 784–796. [Google Scholar] [CrossRef]
- Kashani, M.R.; Javadi, S.; Gharavi, N. Dielectric properties of silicone rubber-titanium dioxide composites prepared dielectrophoretic assembly of filler particles. Smart Mater. Struct. 2010, 19, 035019. [Google Scholar] [CrossRef]
- Abdelmalik, A.A.; Ogbodo, M.O.; Abubakar, Y.M.; Galadima, A.I.; Aliyu, A.; Jonah, S.A. Influence of neutron irradiation on mechanical and dielectric properties of epoxy/titanium oxide nanocomposite. Radiat. Phys. Chem. 2022, 198, 110230. [Google Scholar] [CrossRef]
- Khan, M.; Khurram, A.A.; Li, T.; Zhao, T.; Subhani, T.; Gul, I.H.; Ali, Z.; Patel, V. Synergistic effect of organic and inorganic nano fillers on the dielectric and mechanical properties of epoxy composites. J. Mater. Sci. Technol. 2018, 34, 2424–2430. [Google Scholar] [CrossRef]
- Jaber, M.H.; Aziz, G.A.; Mohammed, A.J.; Al-Alkawi, H.J. Electrical conductivity, magnetic and fatigue properties of aluminum matrix composites reinforced with nano-titanium dioxide (TiO2). Nanocomposites 2020, 6, 47–55. [Google Scholar] [CrossRef]
- Manjunath, S.; Anilkumar, K.R.; Revanasidappa, M.; Prasad, M.V.N. Frequency-dependent and dielectric permittivity of polyaniline/TiO2 composites. Ferroelectr. Lett. 2008, 35, 36–48. [Google Scholar] [CrossRef]
- Ko, S.H.; Song, Y.A.; Kim, S.J.; Kim, M.; Han, J.; Kang, K.H. Nanofluidic preconcentration device in a straight microchannel using ion concentration polarization. Lab Chip 2012, 12, 4472–4482. [Google Scholar] [CrossRef]
- Lu, D.; Mo, Z.; Liang, B.; Yang, L.; He, Z.; Zhu, H.; Tang, Z.; Gui, X. Flexible, lightweight carbon nanotube sponges and composites for high-performance electromagnetic interference shielding. Carbon 2018, 133, 457–463. [Google Scholar] [CrossRef]
- Macutkevic, J.; Kranauskaite, I.; Banys, J.; Moseenkov, S.; Kuznetsov, V.; Shenderova, O. Metal-insulator transition and size dependent electrical percolation in onion-like carbon/polydymethylsiloxane composites. J. Appl. Phys. 2014, 115, 213702. [Google Scholar] [CrossRef]
- Noimark, R.J.; Colchester, R.K.; Poduval, E.; Maneas, E.J.; Alles, T.; Zhao, E.Z.; Zhang, M.; Ashworth, E.; Tsolaki, A.; Chester, H.; et al. Polydimethilsiloxane composites for optical ultrasound generation and multimodality imaging. Adv. Funct. Mater. 2018, 28, 1704919. [Google Scholar] [CrossRef]
- Sosnin, I.M.; Vlassov, S.; Dorogin, L.M. Application of polydimethylsiloxane in photocatalyst composite materials: A review. React. Funct. Polym. 2021, 158, 104781. [Google Scholar] [CrossRef]
- Li, Y.Q.; Zhu, W.B.; Yu, X.G.; Huang, P.; Fu, S.Y.; Hu, N.; Liao, K. Multifunctional wearable device based on flexible and conductive carbon sponge/polydimethylsiloxane composite. ACS Appl. Mater. Interfaces 2016, 8, 33189–33196. [Google Scholar] [CrossRef]
- Wang, Z.; Gao, W.; Zhang, Q.; Zheng, K.; Xu, J.; Xu, W.; Shang, E.; Jiang, J.; Zhang, J.; Liu, Y. 3d-printed graphene/polydimethylsiloxane composites for stretchable and strain-insensitive temperature sensors. ACS Appl. Mater. Interfaces 2019, 11, 1344–1352. [Google Scholar] [CrossRef] [PubMed]
- Wietzke, S.; Jansen, C.; Reuter, M.; Jung, T.; Kraft, D.; Chatterjee, S.; Fischer, B.M.; Koch, M. Terahertz spectroscopy on polymers: A review of morphological studies. J. Mol. Struct. 2011, 1006, 41–51. [Google Scholar] [CrossRef]
- Yang, X.; Vorobiev, A.; Generalov, A.; Andersson, M.A.; Stake, J. A flexible graphene terahertz detector. Appl. Phys. Lett. 2017, 111, 021102. [Google Scholar] [CrossRef]
- Li, J.; Shah, C.M.; Withayachumankul, W.; Ung, B.S.; Mitchell, A.; Sriram, S.; Bhaskaran, M.; Chang, S.; Abbott, D. Mechanically tunable terahertz metamaterials. Appl. Phys. Lett. 2013, 102, 121101. [Google Scholar] [CrossRef]
- Grigas, J. Microwave Dielectric Spectroscopy of Ferroelectric and Related Materials; Gordon and Science Publications, OPA: Amsterdam, The Netherlands, 1996. [Google Scholar]
- Radhanpura, K.; Hargreaves, S.; Lewis, R.A.; Henini, M. The role of optical rectification in the generation of terahertz radiation from GaBiAs. Appl. Phys. Lett. 2009, 94, 251115. [Google Scholar] [CrossRef]
- Pupeza, I.; Wilk, R.; Koch, M. Highly accurate optical material parameter determination with THz time-domain spectroscopy. Opt. Express. 2007, 15, 4335. [Google Scholar] [CrossRef]
- Samulionis, V.; Banys, J.; Sanchez-Ferrer, A.; Mezzenga, R. Ultrasonic characterization of dynamic elastic properties of polymer composites with inorganic nanotubes. Sens. Transducers J. 2011, 12, 66–70. [Google Scholar]
- Samulionis, V.; Banys, J.; Vysochanskii, Y. Ultrasonic and piezoelectric studies of phase transitions in two-dimensional CuInP2S6 type crystals. Ferroelectrics 2009, 379, 69–76. [Google Scholar] [CrossRef]
- Cai, D.; Neyer, A.; Kuckuk, R.; Heise, H.M. Raman, mid-infrared, near-infrared and ultraviolet-visible spectroscopy of PDMS silicone rubber for characterization of polymer optical waveguide materials. J. Mol. Struct. 2010, 976, 274–281. [Google Scholar] [CrossRef]
- Kisilev, A.V.; Uvarov, A.V. Infrared spectra and electron spin resonance of aluminum, silicon and titanium oxides and of absorbed substances. Surf. Sci. 1967, 6, 399–421. [Google Scholar] [CrossRef]
- Leavy, O.; Stroud, D. Maxwell Garnett theory for mixtures of anisotropic inclusions: Applications to conducting polymers. Phys. Rev. B 1997, 56, 8035. [Google Scholar] [CrossRef]
- Wypych, A.; Bobowska, I.; Tracz, M.; Opasinska, A.; Kadlubowski, S.; Krzywania-Kaliszewska, A.; Grobelny, J.; Wojciechowski, P. Dielectric properties and characterization of titanium dioxide obtained by different methods. J. Nanomater. 2014, 2014, 124814. [Google Scholar] [CrossRef]
- Youseff, M.H. Influence of sulfur content and curing time on the glass transition parameters in SBR vulcanizates: An ultrasonic study. Polym. Test. 2003, 22, 235–242. [Google Scholar] [CrossRef]
- Fragiadakis, D.; Pissis, P.; Bokobza, L. Glass transition and molecular dynamics in poly(dimethylsiloxane)/silica nanocomposites. Polymer 2005, 46, 6001–6008. [Google Scholar] [CrossRef]
- Ouyang, J.; Pan, Y.; Zhou, S.; Goh, S.H. Supramolecular assembled C60-containing carboxylated poly(dimethylsiloxane) composites. Polymer 2006, 47, 6140–6148. [Google Scholar] [CrossRef]
- Vulcher, G.S. Analysis of recent measurements of the viscosity of glasses. J. Am. Ceram. Soc. 1925, 8, 339. [Google Scholar] [CrossRef]
- Belovickis, J.; Macutkevic, J.; Svirskas, S.; Samulionis, V.; Banys, J.; Shenderova, O.; Borjanovic, V. Ultrasonic and dielectric relaxations in PDMS/ZnO nanocomposite. Phys. Status Solidi B 2015, 252, 2778–2783. [Google Scholar] [CrossRef]
- Valevicius, V.; Samulionis, V.; Banys, J. Ultrasonic dispersion in the phase transition region of ferroelectric materials. J. Alloys Compd. 1994, 369, 211–212. [Google Scholar]
- Dollase, T.; Wilhelm, M.; Spiess, H.W.; Yagen, Y.; Yerushalmi-Rozen, R.; Gottlieb, M. Effect of interfaces on the crystalization behavior of PDMS. Interface Sci. 2003, 11, 199–209. [Google Scholar] [CrossRef]
- Valle-Carrandi, L.; Alegria, A.; Colmenero, J. PDMS behavior under confinement in strongly segregated mesophases of PS-PDMS diblock copolymers. Eur. Phys. J. Spec. Top. 2010, 189, 257–261. [Google Scholar] [CrossRef]
- Alfihed, S.; Holzman, J.F.; Foulds, I.G. Developments in the integration and application of terahertz spectroscopy with microfluidics. Biosens. Bioelectron. 2020, 165, 112393. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.; Xiang, C.; Zhu, Q.; Zhong, W.; Li, M.; Yan, K.; Wang, D. Multistimulus responsive actuator with GO and carbon nanotube/PDMS bilayer structure for flexible and smart devices. ACS Appl. Mater. Interfaces 2018, 10, 27215–27223. [Google Scholar] [CrossRef] [PubMed]
- Paddubskaya, A.; Demidenko, M.; Batrakov, K.; Valusis, G.; Kaplas, T.; Svirko, Y.; Kuzhir, P. Tunable perfect THz absorber based on a streatchable ultrathin carbon-polymer bilayer. Materials 2019, 12, 143. [Google Scholar] [CrossRef]
- Zhang, H.; Lan, Y.; Qiu, S.; Min, S.; Jang, H.; Park, J.; Gong, S.; Ma, Z. Flexible and stretchable microwave electronics: Past, present and future perspective. Adv. Mater. Technol. 2021, 6, 2000759. [Google Scholar] [CrossRef]

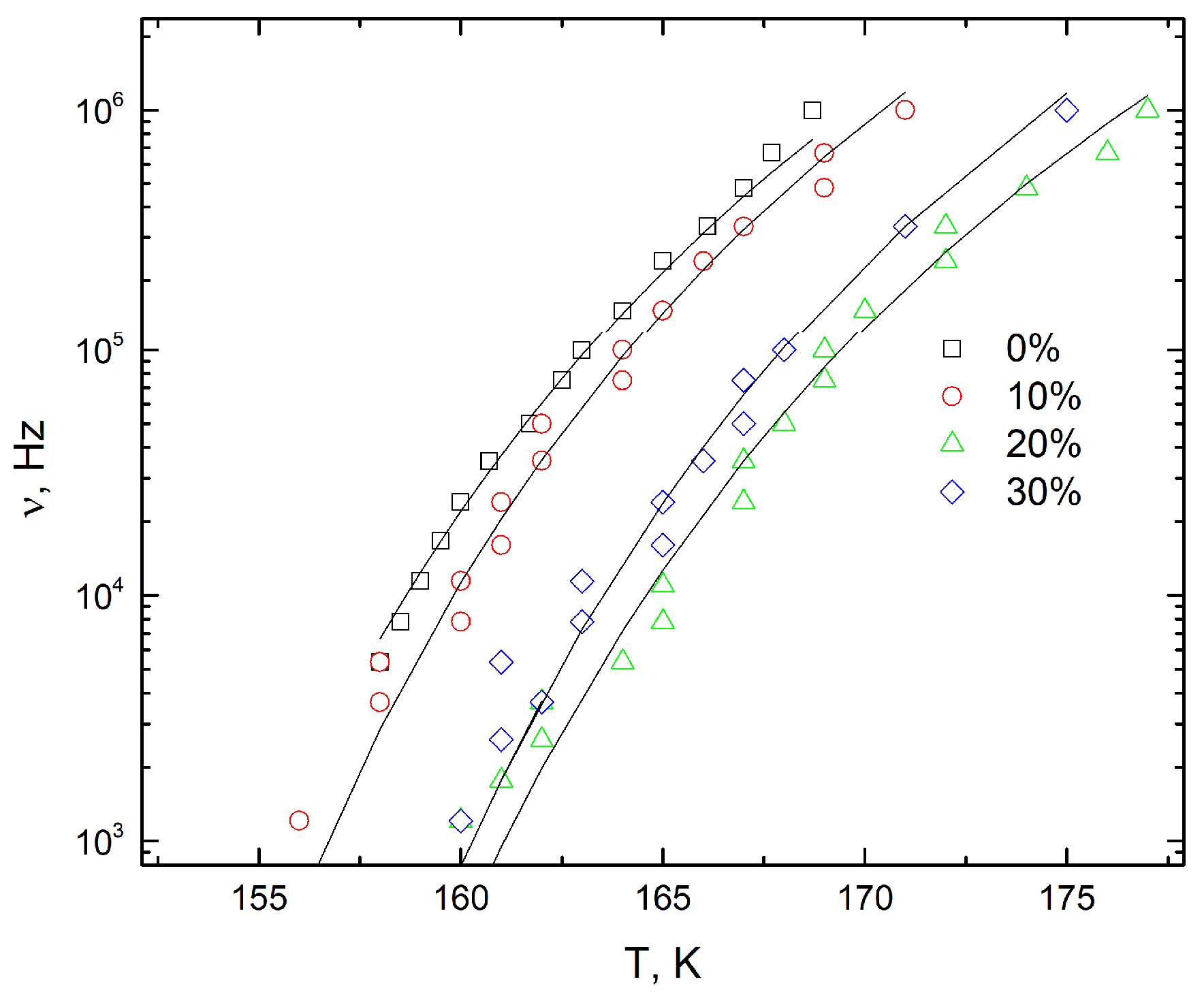

| Concentration (vol.%) | E/kB, K | T0, K |
|---|---|---|
| 0 | 343 | 135 |
| 10 | 331 | 137 |
| 20 | 371 | 139 |
| 30 | 341 | 140 |
| Concentration | τ0, ns | E/kB, K | T02, K |
|---|---|---|---|
| 0 | 1 | 208.9 | 133.6 |
| 10 | 2.3 | 139.2 | 144.6 |
| 20 | 2.9 | 161.3 | 139.3 |
| 30 | 8.3 | 85.8 | 150 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vanskeviče, I.; Kinka, M.; Banys, J.; Macutkevič, J.; Schaefer, S.; Selskis, A.; Fierro, V.; Celzard, A. Dielectric and Ultrasonic Properties of PDMS/TiO2 Nanocomposites. Polymers 2024, 16, 603. https://doi.org/10.3390/polym16050603
Vanskeviče I, Kinka M, Banys J, Macutkevič J, Schaefer S, Selskis A, Fierro V, Celzard A. Dielectric and Ultrasonic Properties of PDMS/TiO2 Nanocomposites. Polymers. 2024; 16(5):603. https://doi.org/10.3390/polym16050603
Chicago/Turabian StyleVanskeviče, Ieva, Martynas Kinka, Jūras Banys, Jan Macutkevič, Sebastien Schaefer, Algirdas Selskis, Vanessa Fierro, and Alain Celzard. 2024. "Dielectric and Ultrasonic Properties of PDMS/TiO2 Nanocomposites" Polymers 16, no. 5: 603. https://doi.org/10.3390/polym16050603
APA StyleVanskeviče, I., Kinka, M., Banys, J., Macutkevič, J., Schaefer, S., Selskis, A., Fierro, V., & Celzard, A. (2024). Dielectric and Ultrasonic Properties of PDMS/TiO2 Nanocomposites. Polymers, 16(5), 603. https://doi.org/10.3390/polym16050603









