An Anisotropic Damage-Plasticity Constitutive Model of Continuous Fiber-Reinforced Polymers
Abstract
1. Introduction
2. Modeling Methodology
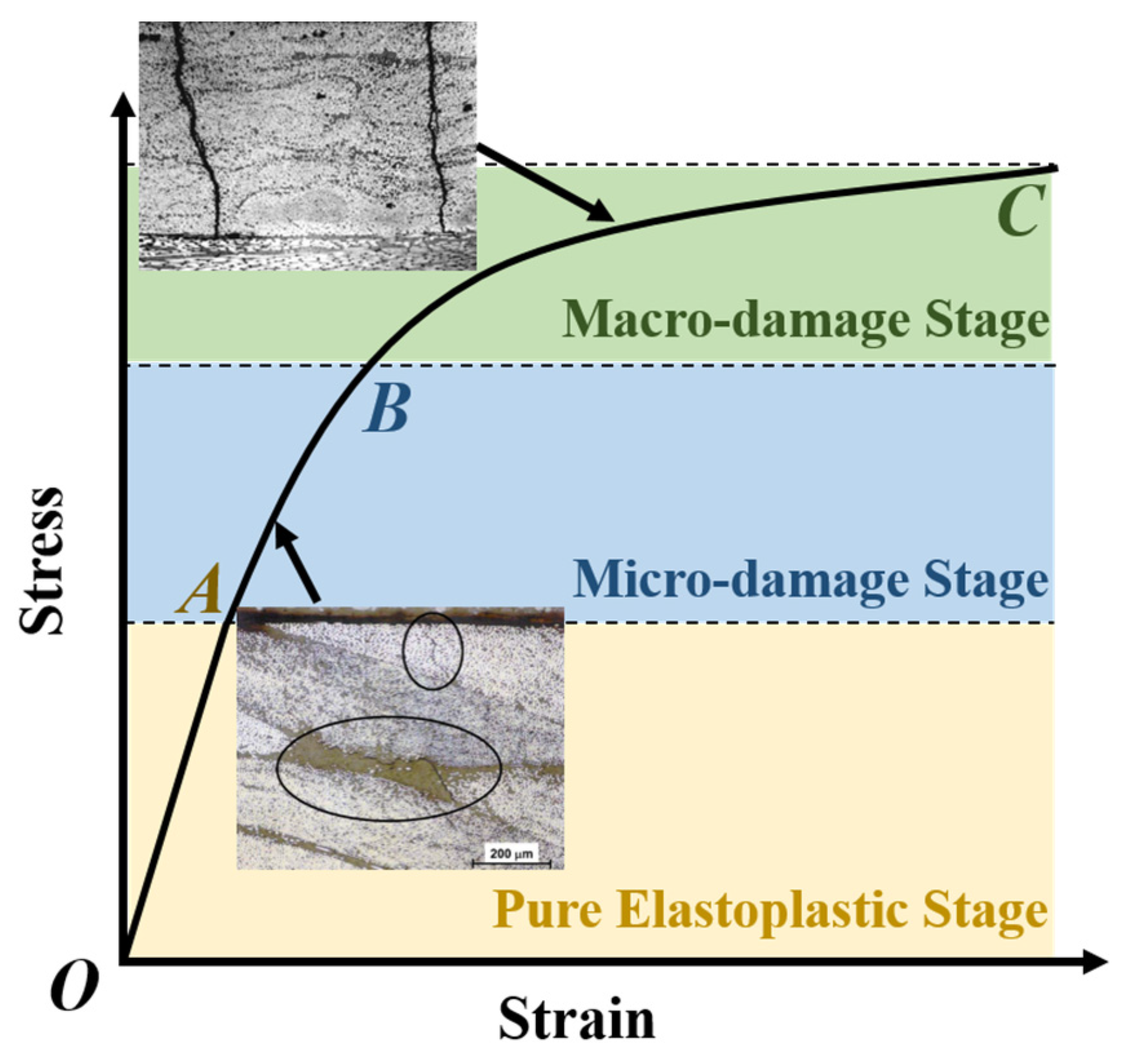
- Pure elastoplastic stage (OA segment): in this initial stage, the matrix remains devoid of any damage, and the nonlinearity is exclusively attributed to plasticity.
- Micro-damage stage (AB segment): As the loading progresses, micro-damages gradually emerge, introducing a subtle deviation in the elastic stress–strain response. The nonlinear behavior of FRPs contributes to both plasticity and internal micro-damages.
- Macro-damage stage (BC segment): Advancing to the final stage, macro-damages emerge, resulting in a noticeable deviation in the elastic stress–strain response. The nonlinear behavior of FRPs contributes to both plasticity and macro-damages.
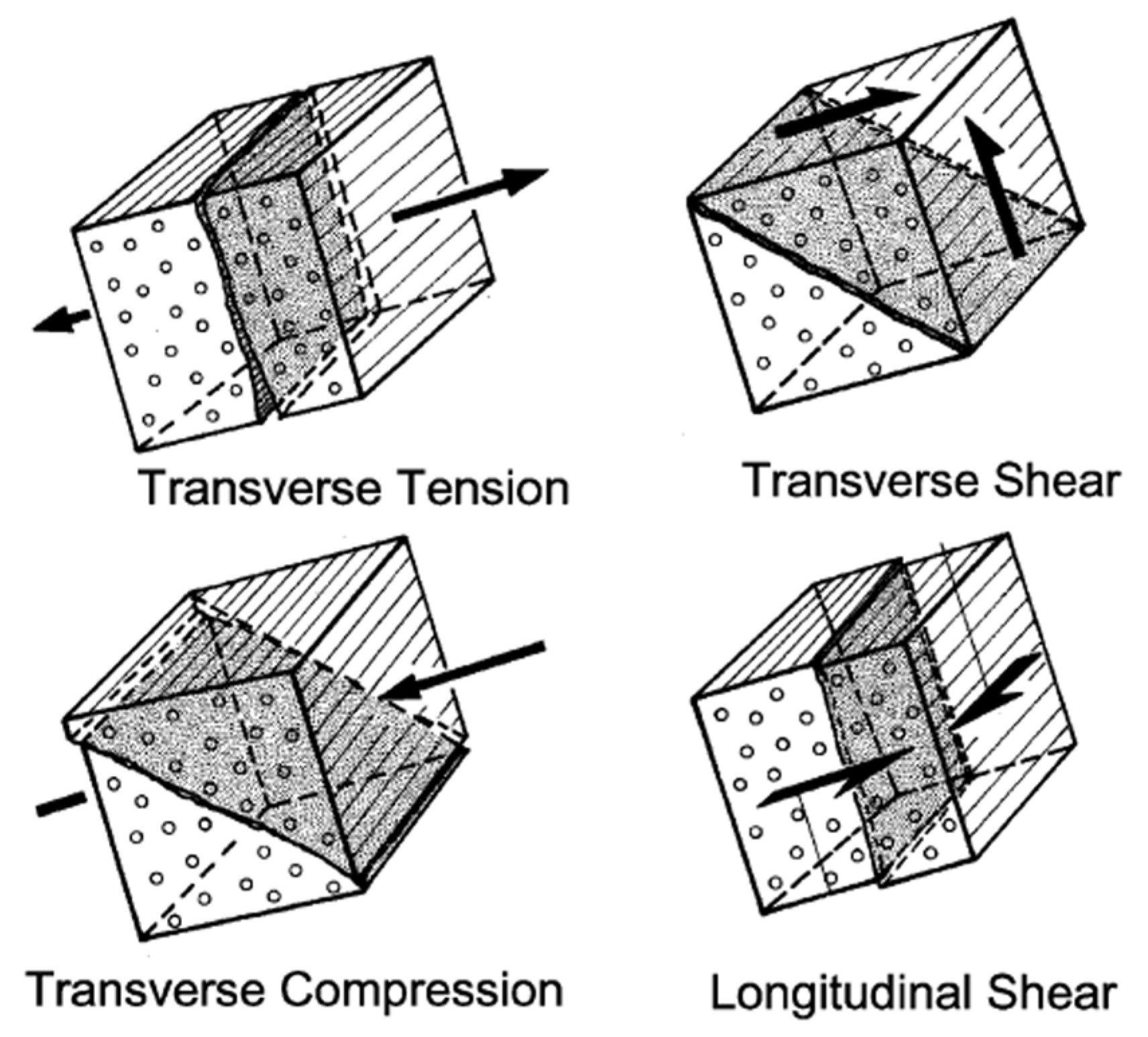
2.1. Damage Determination
2.2. Damage Evolution
2.3. Plasticity Evolution
- (a)
- Elastoplastic strain decomposition
- (b)
- Elastic law
- (c)
- Yield criterion
- (d)
- Plastic flow rule
- (e)
- Hardening law
3. Numerical Implementation Methodology
3.1. Cauchy Stress Solution
3.2. Consistent Tangent Stiffness Solution
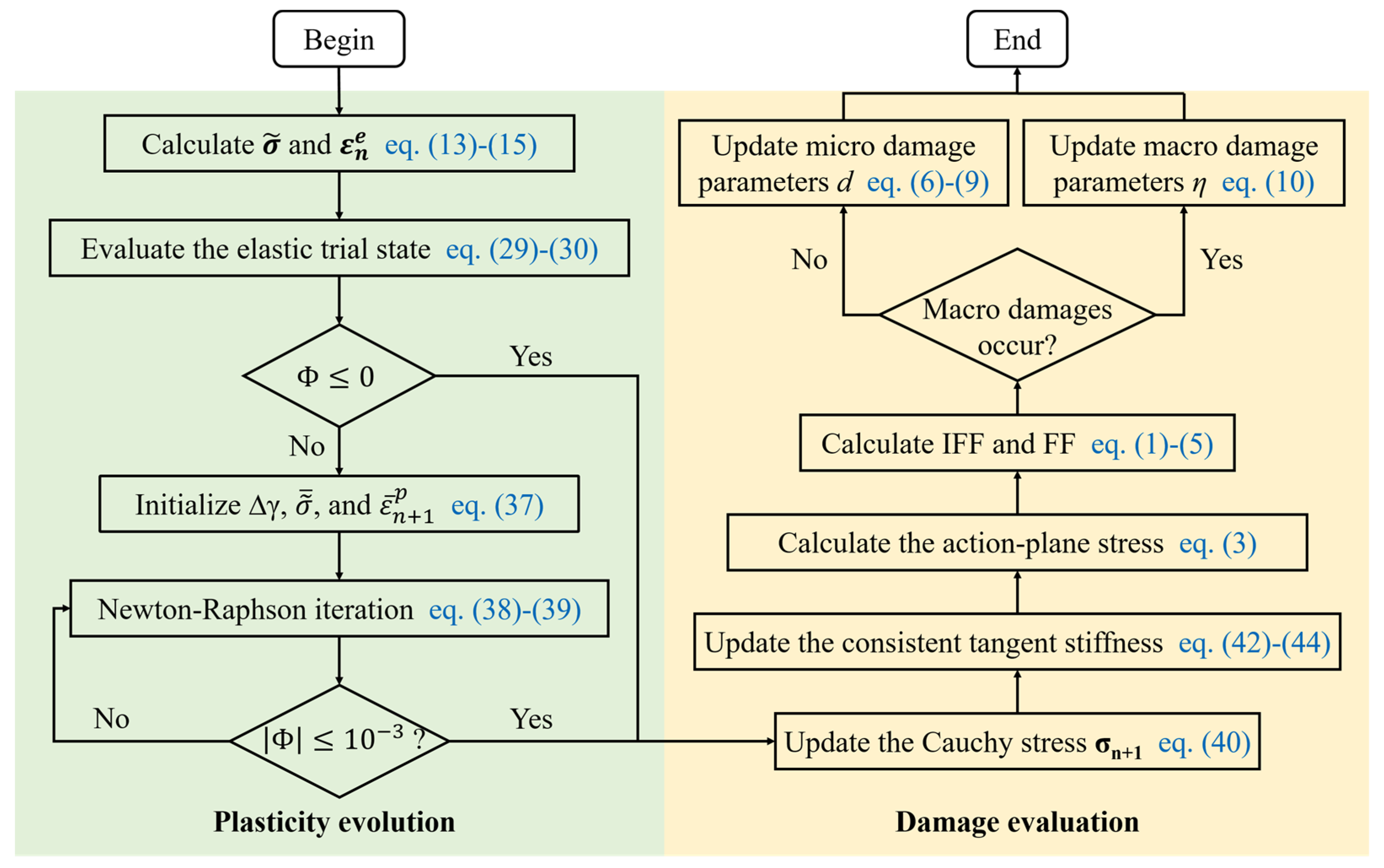
3.3. Numerical Algorithm
4. Results and Discussion
4.1. Materials Tested and Work Method
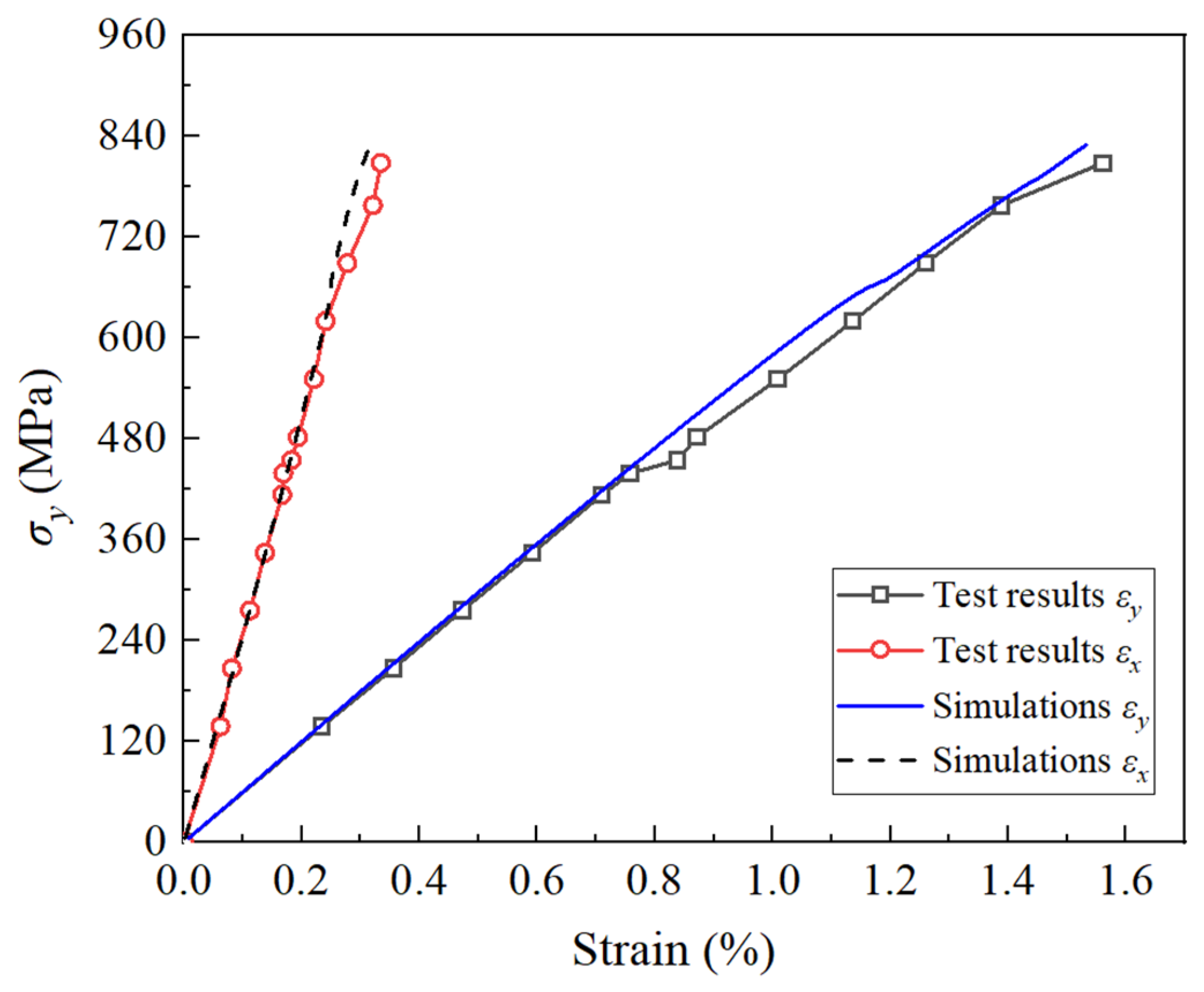
- Deformation prediction. This segment involved the analysis of stress–strain curves of (90°/+45°/−45°/0°)s AS4-carbon/epoxy laminates under a stress ratio of : = 1:20 and 1:2. The x-direction was aligned along the direction of the 0° lay-up direction. Christoforou [47] and Trask [48] conducted these tests at the University of Utah, respectively.
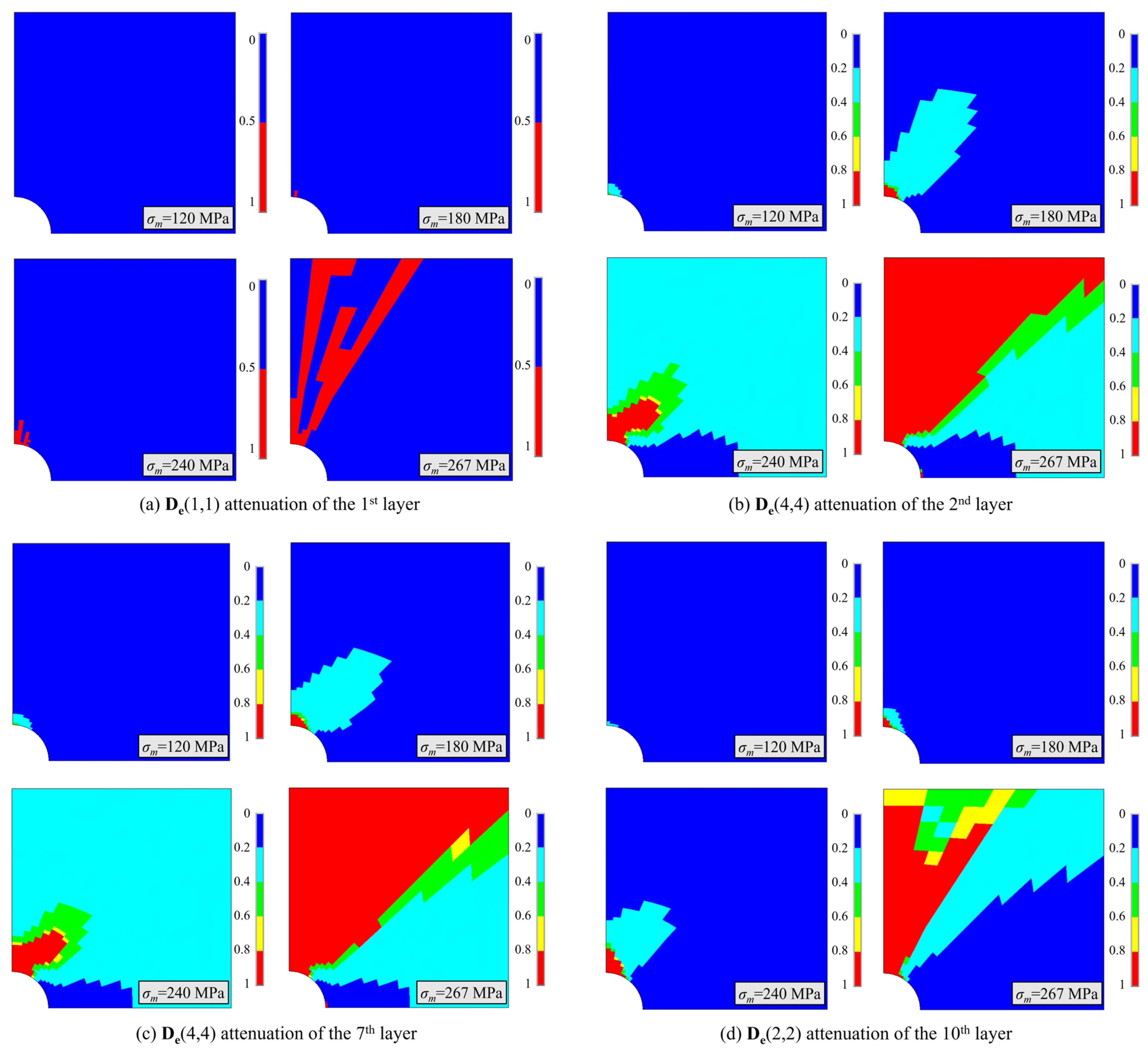
- Open-hole-tension (OHT) strength prediction. In this case, OHT tests of T300-carbon/epoxy laminates conducted by Chang [49,50] were chosen as a reference. These tests encompass three different lay-ups ([0/(±45°)3/90°3]s, [0/(±45°)2/90°5]s, and [0/(±45°)1/90°7]s), each with four different geometric configurations.
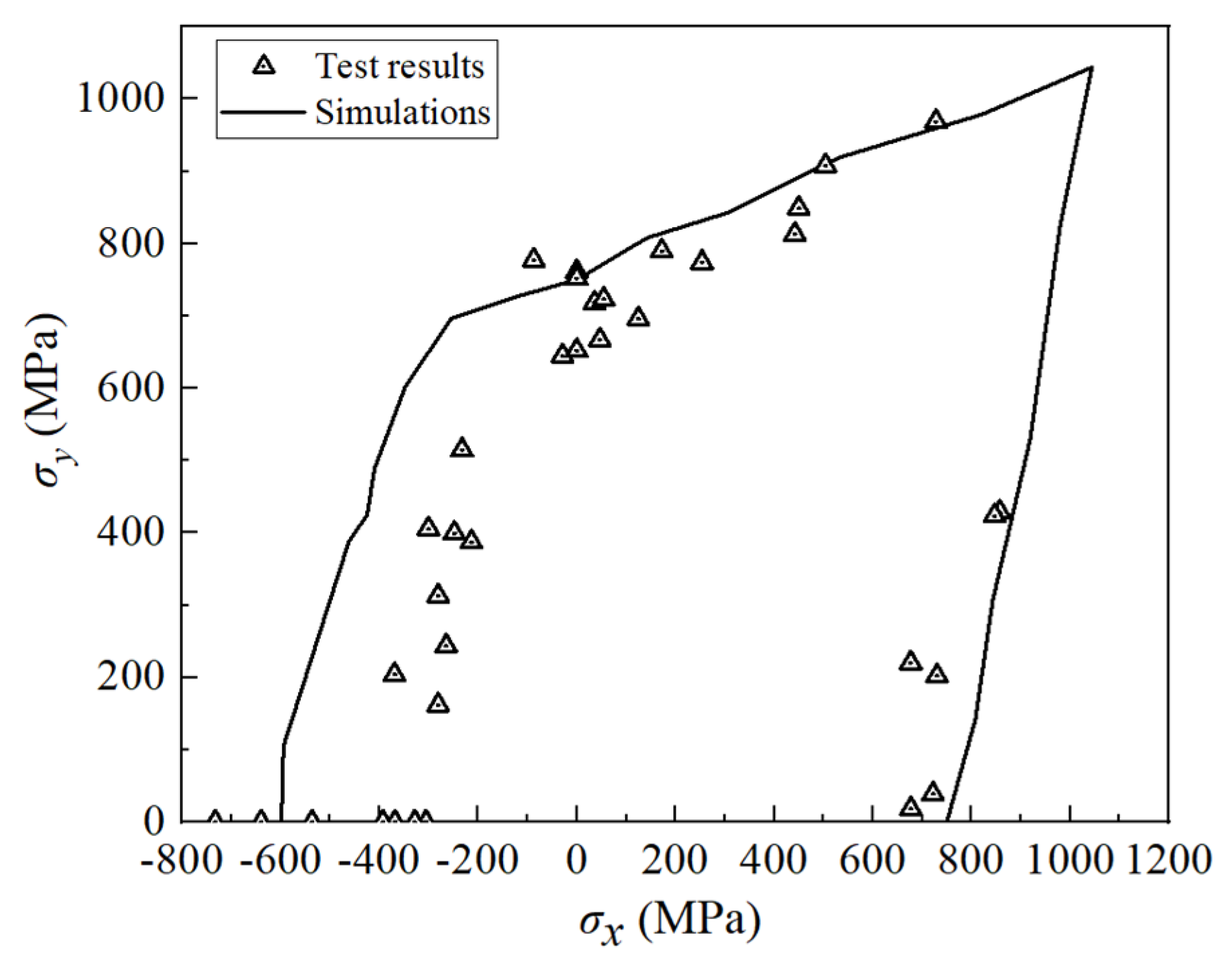
4.2. Biaxial Tension Failure Envelope
4.3. Biaxial Tension Deformation
4.4. Open-Hole-Tension Strength
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Notations
| Latin characters | Greek characters | ||
| a | Plastic coefficients | α, β | Plastic coefficients |
| b, , , , | Parameters relating to micro-damages | γ | Lagrange’s plastic multiplier |
| , | Micro-damage coefficients reflecting transverse and in-plane shear micro-damages | Strain | |
| Voigt form of the four-order stiffness tensor | Elastic-strain-coupled damage | ||
| Consistent tangent stiffness | Plastic strain | ||
| , , | Elastic modulus in 1-, 2-, and 3-directions | Elastic trial strain | |
| , | Transverse and in-plane shear modulus when macro-damages first occur | Equivalent plastic trial strain | |
| Maximum allowable stress exposure for inter-fiber fracture | , , | Strain in 1, 2, and 3 directions | |
| In-plane shear modulus | , , | Strain in 12, 13, and 23 directions | |
| H | Hardening modulus | , | Degradation of the transverse and in-plane shear modulus due to macro-damages |
| M | Damage matrix | , | Residual stiffness of transverse and in-plane shear modulus |
| N | Flow vector | θ | Action-plane angle |
| , | Puck’s inclination parameters | , | Major and minor Poisson’s ratio |
| , | Action-plane resistance against and | Engineering stress | |
| , | Action-plane resistance against tensile and compression | Effective stress | |
| Strength at which the deviation of the secant in-plane shear modulus reaches 7% | Equivalent stress | ||
| In-plane shear strength of unidirectional lamina | Effective trial stress | ||
| Damaged strain energy density | , , | Stress in 1-direction (aligned to the fibers), and 2- and 3-directions (normal to the fibers) | |
| Strength at which the deviation from the linear zone of transverse tension reaches 0.5 MPa | , , | Shear stress in 12, 13, and 23 directions | |
| , | Longitudinal tensile and compression strength | ; , | Action-plane normal and shear stress |
| , | Transverse tensile and compression strength of unidirectional lamina | Φ | Yield function |
| Y | A quantity governing the micro-damage development | ||
| , | Release rate of damaged strain energy | ||
References
- Alabtah, F.G.; Mahdi, E.; Eliyan, F.F. The use of fiber reinforced polymeric composites in pipelines: A review. Compos. Struct. 2021, 276, 114595. [Google Scholar] [CrossRef]
- Rubino, F.; Nisticò, A.; Tucci, F.; Carlone, P. Marine application of fiber reinforced composites: A review. J. Mar. Sci. Eng. 2020, 8, 26. [Google Scholar] [CrossRef]
- Rajak, D.; Pagar, D.; Menezes, P.; Linul, E. Fiber-reinforced polymer composites: Manufacturing, properties, and applications. Polymers 2019, 11, 1667. [Google Scholar] [CrossRef] [PubMed]
- Xie, J.F.; Zhang, S.Z.; Shi, J.T.; Wang, J.F.; Li, L.; Han, X.T. Realisation of the reconfigurable pulsed high magnetic field facility and its scientific application at Wuhan National Pulsed High Magnetic Field Centre. High Volt. 2023, 8, 898–906. [Google Scholar] [CrossRef]
- Knops, M. Analysis of Failure in Fiber Polymer Laminates, 2nd ed.; Springer: New York, NY, USA, 2008; pp. 5–19. [Google Scholar]
- Talreja, R.; Waas, A.M. Concepts and definitions related to mechanical behavior of fiber reinforced composite materials. Compos. Sci. Technol. 2022, 217, 109081. [Google Scholar] [CrossRef]
- Soden, P.D.; Hinton, M.J.; Kaddour, A.S. Biaxial test results for strength and deformation of a range of E-glass and carbon fibre reinforced composite laminates: Failure exercise benchmark data. Compos. Sci. Technol. 2002, 62, 1489–1514. [Google Scholar] [CrossRef]
- Hinton, M.J.; Kaddour, A.S.; Soden, P.D. A further assessment of the predictive capabilities of current failure theories for composite laminates: Comparison with experimental evidence. Compos. Sci. Technol. 2004, 64, 549–588. [Google Scholar] [CrossRef]
- Schuecker, C.; Pettermann, H.E. Fiber reinforced laminates: Progressive damage modeling based on failure mechanisms. Arch. Comput. Methods. Eng. 2008, 15, 163–184. [Google Scholar] [CrossRef]
- Talreja, R. Failure of unidirectional fiber reinforced composites: A case study in strength of materials. Mech. Compos. Mater. 2023, 59, 173–192. [Google Scholar] [CrossRef]
- Guo, Q.; Yao, W.; Li, W.; Gupta, N. Constitutive models for the structural analysis of composite materials for the finite element analysis: A review of recent practices. Compos. Struct. 2021, 260, 113267. [Google Scholar] [CrossRef]
- Jones, R.M. Mechanics of Composite Materials, 2nd ed.; Taylor and Francis: New York, NY, USA, 1999; pp. 11–17. [Google Scholar]
- Pai, A.; Suri, R.; Bhave, A.K.; Verma, P.; Padmaraj, N.H. Puck’s criterion for the tensile response of composite laminates: A numerical approach. Adv. Eng. Softw. 2023, 175, 103364. [Google Scholar] [CrossRef]
- Naghdinasab, M.; Farrokhabadi, A.; Madadi, H. A numerical method to evaluate the material properties degradation in composite RVEs due to fiber-matrix debonding and induced matrix cracking. Finite Elem. Anal. Des. 2018, 146, 84–95. [Google Scholar] [CrossRef]
- Onodera, S.; Okabe, T. Three-dimensional analytical model for effective elastic constants of transversely isotropic plates with multiple cracks: Application to stiffness reduction and steady-state cracking of composite laminates. Eng. Fract. Mech. 2019, 219, 106595. [Google Scholar] [CrossRef]
- Rajaneesh, A.; Ponthot, J.P.; Bruyneel, M. High velocity impact response of composite laminates using modified meso-scale damage models. Int. J. Impact Eng. 2021, 147, 103701. [Google Scholar] [CrossRef]
- Almeida, J.H.S.; Tonatto, M.L.P.; Ribeiro, M.L.; Tita, V.; Amico, S.C. Buckling and post-buckling of filament wound composite tubes under axial compression: Linear, nonlinear, damage and experimental analyses. Compos. Part B Eng. 2018, 149, 227–239. [Google Scholar] [CrossRef]
- Pulungan, D.; Yudhanto, A.; Lubineau, G. Characterizing and modeling the progressive damage of off-axis thermoplastic plies: Effect of ply confinement. Compos. Struct. 2020, 246, 112397. [Google Scholar] [CrossRef]
- Yi, T. The progressive failure analysis of uni-directional fibre reinforced composite laminates. J. Mech. 2020, 36, 159–166. [Google Scholar] [CrossRef]
- Abisset, E.; Daghia, F.; Ladevèze, P. On the validation of a damage mesomodel for laminated composites by means of open-hole tensile tests on quasi-isotropic laminates. Compos. Part A Appl. Sci. Manuf. 2011, 42, 1515–1524. [Google Scholar] [CrossRef]
- Ladeveze, P.; LeDantec, E. Damage modelling of the elementary ply for laminated composites. Compos. Sci. Technol. 1992, 43, 257–267. [Google Scholar] [CrossRef]
- Lee, C.; Kim, J.; Kim, S.; Ryu, D.; Lee, J. Initial and progressive failure analyses for composite laminates using Puck failure criterion and damage-coupled finite element method. Compos. Struct. 2015, 121, 406–419. [Google Scholar] [CrossRef]
- Ahmadi, J.M.; Heidari-Rarani, M. Development of Abaqus WCM plugin for progressive failure analysis of type IV composite pressure vessels based on Puck failure criterion. Eng. Fail. Anal. 2022, 131, 105851. [Google Scholar] [CrossRef]
- Liu, P.F.; Zheng, J.Y. Recent developments on damage modeling and finite element analysis for composite laminates: A review. Mater. Des. 2010, 31, 3825–3834. [Google Scholar] [CrossRef]
- Pinho, S.T.; Vyas, G.M.; Robinson, P. Material and structural response of polymer-matrix fibre-reinforced composites: Part B. J. Compos. Mater. 2013, 47, 679–696. [Google Scholar] [CrossRef]
- He, G.; Liu, Y.; Lacy, T.E.; Horstemeyer, M.F. A historical review of the traditional methods and the internal state variable theory for modeling composite materials. Mech. Adv. Mater. Struc. 2022, 29, 2617–2638. [Google Scholar] [CrossRef]
- Hu, C.; Sang, L.; Jiang, K.; Xing, J.; Hou, W. Experimental and numerical characterization of flexural properties and failure behavior of CFRP/Al laminates. Compos. Struct. 2022, 281, 115036. [Google Scholar] [CrossRef]
- Han, W.; Hu, K.; Shi, Q.; Zhu, F. Damage evolution analysis of open-hole tensile laminated composites using a progress damage model verified by AE and DIC. Compos. Struct. 2020, 247, 112452. [Google Scholar] [CrossRef]
- Fallahi, H.; Taheri-Behrooz, F.; Asadi, A. Nonlinear mechanical response of polymer matrix composites: A review. Polym. Rev. 2020, 60, 42–85. [Google Scholar] [CrossRef]
- Gilat, A.; Goldberg, R.K.; Roberts, G.D. Strain rate sensitivity of epoxy resin in tensile and shear loading. J. Aerosp. Eng. 2007, 20, 75–89. [Google Scholar] [CrossRef]
- Tan, W.; Falzon, B.G. Modelling the nonlinear behaviour and fracture process of AS4/PEKK thermoplastic composite under shear loading. Compos. Sci. Technol. 2016, 126, 60–77. [Google Scholar] [CrossRef]
- Fallahi, H.; Taheri-Behrooz, F. Phenomenological constitutive modeling of the non-linear loading-unloading response of UD fiber-reinforced polymers. Compos. Struct. 2022, 292, 115671. [Google Scholar] [CrossRef]
- Bru, T.; Olsson, R.; Gutkin, R.; Vyas, G.M. Use of the Iosipescu test for the identification of shear damage evolution laws of an orthotropic composite. Compos. Struct. 2017, 174, 319–328. [Google Scholar] [CrossRef]
- Virendra, R.; Jadhav, S.S. Micromechanical analysis of nonlinear response of unidirectional composites: A fundamental approach. In Proceedings of the ASME 2001 International Mechanical Engineering Congress and Exposition, New York, NY, USA, 11–16 November 2001. [Google Scholar]
- Varna, J.; Joffe, R.; Akshantala, N.V.; Talreja, R. Damage in composite laminates with off-axis plies. Compos. Sci. Technol. 1999, 59, 2139–2147. [Google Scholar] [CrossRef]
- Puck, A.; Schürmann, H. Failure analysis of FRP laminates by means of physically based phenomenological models. Compos. Sci. Technol. 2002, 62, 1633–1662. [Google Scholar] [CrossRef]
- Soden, P.D.; Hinton, M.J.; Kaddour, A.S. Lamina properties, lay-up configurations and loading conditions for a range of fibre-reinforced composite laminates. Compos. Sci. Technol. 1998, 58, 1011–1022. [Google Scholar] [CrossRef]
- Hinton, M.J.; Kaddour, A.S.; Soden, P.D. A comparison of the predictive capabilities of current failure theories for composite laminates, judged against experimental evidence. Compos. Sci. Technol. 2002, 62, 1725–1797. [Google Scholar] [CrossRef]
- Higgins, R.M.; McCarthy, C.T.; McCarthy, M.A. Effects of shear-transverse coupling and plasticity in the formulation of an elementary ply composites damage model, part II: Material characterisation. Strain 2012, 48, 59–67. [Google Scholar] [CrossRef]
- Puck, A.; Mannigel, M. Physically based non-linear stress–strain relations for the inter-fibre fracture analysis of FRP laminates. Compos. Sci. Technol. 2007, 67, 1955–1964. [Google Scholar] [CrossRef]
- De Souza Neto, E.A.; Peric, D.; Owen, D.R. Computational Methods for Plasticity: Theory and Applications, 1st ed.; John Wiley and Sons: Singapore, 2008; pp. 168–185. [Google Scholar]
- Chen, J.F.; Morozov, E.V.; Shankar, K. A combined elastoplastic damage model for progressive failure analysis of composite materials and structures. Compos. Struct. 2012, 94, 3478–3489. [Google Scholar] [CrossRef]
- Hütter, U.; Schelling, H.; Krauss, H. An experimental study to determine failure envelope of composite materials with tubular specimen under combined loads and comparison between several classical criteria. In Proceedings of the Failure Modes of Composite Materials with Organic Matrices and Other Consequences on Design, NATO, AGRAD, Conference Proceedings No. 163, Munich, Germany, 13–19 October 1974. [Google Scholar]
- Swanson, S.R.; Christoforou, A.P. Response of quasi-isotropic carbon/epoxy laminates to biaxial stress. J. Compos. Mater. 1986, 20, 457–471. [Google Scholar] [CrossRef]
- Colvin, G.E.; Swanson, S.R. In-situ compressive strength of carbon/epoxy AS4/3501-6 laminates. J. Eng. Mater. Tech. 1993, 115, 122–128. [Google Scholar] [CrossRef]
- Swanson, S.R.; Trask, B.C. Strength of quasi-isotropic laminates under off-axis loading. Compos. Sci. Technol. 1989, 34, 19–34. [Google Scholar] [CrossRef]
- Christoforou, A.P. An Investigation of Composite Material Response under Tension-Tension Biaxial Stresses. Master’s Thesis, Department of Mechanical and Industrial Engineering, The University of Utah, Salt Lake City, UT, USA, 1984. [Google Scholar]
- Trask, B.N. Response of Carbon/Epoxy Laminates to Biaxial Stress. Master’s Thesis, Department of Mechanical and Industrial Engineering, The University of Utah, Salt Lake City, UT, USA, 1987. [Google Scholar]
- Chang, F.K.; Scott, R.A.; Springer, G.S. Strength of Bolted Joints in Laminated Composites; Technical Report AFWAL-TR-84-4029; Air Force Wright Aeronautical Laboratories: San Francisco, CA, USA, 1984. [Google Scholar]
- Chang, F.K.; Chang, K.Y. A progressive damage model for laminated composites containing stress concentrations. J. Compos. Mater. 1987, 21, 834–855. [Google Scholar] [CrossRef]
- Sun, C.T.; Chen, J.T. A simply flow rule for characterizing nonlinear behavior of fiber composites. J. Compos. Mater. 1989, 23, 1009–1020. [Google Scholar] [CrossRef]
- Tan, S.C. A Progressive Failure Model for Composite Laminates Containing Openings. J. Compos. Mater. 1991, 25, 556–577. [Google Scholar] [CrossRef]



| Fiber Type | E-Glass 21 × K43 Gevetex | AS4-Carbon | T300-Carbon | Remark |
|---|---|---|---|---|
| Matrix | LY556/HT907/DY063 | 3501-6 | 1034-C | - |
| Specification | Filament winding | Preprg | Preprg | |
| Fiber volume fraction | 0.62 | 0.60 | 0.53 | |
| (GPa) | 53.48 | 126 | 146.86 | Elastic characteristics, referenced from [37,50] |
| (GPa) | 17.7 | 11 | 11.38 | |
| (GPa) | 5.83 | 6.6 | 6.14 | |
| 0.278 | 0.28 | 0.30 | ||
| 0.4 | 0.4 | 0.4 | ||
| (MPa) | 1140 | 1950 | 1730.6 | Strength, referenced from [37,50] |
| (MPa) | 570 | 1480 | 1379 | |
| (MPa) | 22.9 | 31.5 | 43.8 | |
| (MPa) | 35 | 48 | 66.5 | |
| (MPa) | 114 | 200 | 268.2 | |
| (MPa) | 37.71 | 45 | 56.5 | |
| (MPa) | 72 | 79 | 93 | |
| 0.2 | 0.3 | 0.3 | Parameters relating to damage determination, referenced from [5] | |
| 0.25 | 0.3 | 0.3 | ||
| 0.3 | 0.35 | 0.35 | ||
| 0.25 | 0.3 | 0.3 | ||
| b | 4.4 | 2.5 | 2.5 | Parameters relating to damage evolution, referenced from [21,40] |
| 0.014 | 0.24 | 0.24 | ||
| 1 | 3.78 | 3.78 | ||
| 0.01 | 0.15 | 0.15 | ||
| 3.24 | 2.77 | 2.77 | ||
| 5.0 | 5.0 | 5.0 | ||
| a | 2.0 | 1.25 | 1.25 | Parameters relating to plasticity evolution. a was referenced from [51], and α and β were deduced through in-plane shear tests. |
| α | 0.24 | 0.2 | 0.08 | |
| β (MPa) | 1050 | 1200 | 3000 |
| Laminate Lay-Up | Tensile Strength (MPa) | Error (%) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Test | Present | Chang | Tan | Chen | Present | Chang | Tan | Chen | ||
| [0/(±45°)3/90°3]s | A | 277.2 | 282.0 | 227.5 | 275.8 | 293.1 | 1.8 | −17.9 | −0.5 | 5.7 |
| B | 256.5 | 267.6 | 206.8 | 275.8 | 252.2 | 4.3 | −19.4 | 7.5 | −1.7 | |
| C | 226.2 | 236.8 | 206.8 | 262.0 | 269.1 | 4.7 | −8.5 | 15.9 | 19.0 | |
| D | 235.8 | 238.0 | 179.3 | 248.2 | 238.3 | 0.8 | −24.0 | 5.3 | 1.1 | |
| [0/(±45°)2/90°5]s | A | 236.5 | 238.0 | 193.1 | 186.2 | 239.1 | −0.6 | −18.4 | −21.3 | 1.1 |
| B | 204.1 | 230.5 | 172.4 | 186.2 | 214.3 | 13.0 | −15.5 | −8.8 | 5.0 | |
| C | 177.9 | 197.2 | 165.5 | 172.4 | 216.3 | 10.9 | −7.0 | −3.1 | 21.6 | |
| D | 185.5 | 188.1 | 151.7 | 158.6 | 205.8 | 1.4 | −18.2 | −14.5 | 11.0 | |
| [0/(±45°)1/90°7]s | A | 191.0 | 179.9 | 144.8 | 227.5 | 171.0 | −5.8 | −24.2 | 19.1 | −10.5 |
| B | 158.6 | 167.1 | 124.1 | 227.5 | 150.4 | 5.4 | −21.7 | 43.5 | −5.2 | |
| C | 134.5 | 157.5 | 124.1 | 213.7 | 155.0 | 17.1 | −7.7 | 59.0 | 15.3 | |
| D | 160.0 | 151.5 | 103.4 | 200.0 | 135.7 | −5.3 | −35.3 | 25.0 | −15.2 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, S.; Li, L. An Anisotropic Damage-Plasticity Constitutive Model of Continuous Fiber-Reinforced Polymers. Polymers 2024, 16, 334. https://doi.org/10.3390/polym16030334
Chen S, Li L. An Anisotropic Damage-Plasticity Constitutive Model of Continuous Fiber-Reinforced Polymers. Polymers. 2024; 16(3):334. https://doi.org/10.3390/polym16030334
Chicago/Turabian StyleChen, Siyuan, and Liang Li. 2024. "An Anisotropic Damage-Plasticity Constitutive Model of Continuous Fiber-Reinforced Polymers" Polymers 16, no. 3: 334. https://doi.org/10.3390/polym16030334
APA StyleChen, S., & Li, L. (2024). An Anisotropic Damage-Plasticity Constitutive Model of Continuous Fiber-Reinforced Polymers. Polymers, 16(3), 334. https://doi.org/10.3390/polym16030334






