Analysis of Tensile Failure Behavior of Metal Fiber Laminates Under Different Temperature Environments
Abstract
1. Introduction
2. Preparation of Samples and Experimental Development
2.1. FML Preparation
2.2. Methods of Experimental Investigation
2.2.1. Tensile Test Experiment
2.2.2. Digital Imaging Correlation Technology (DIC)
3. Finite Element Modeling and Damage Criteria
3.1. Metal Damage Modeling
3.2. Fiber Failure Model
3.3. Hierarchical Model
4. Finite Element Modeling
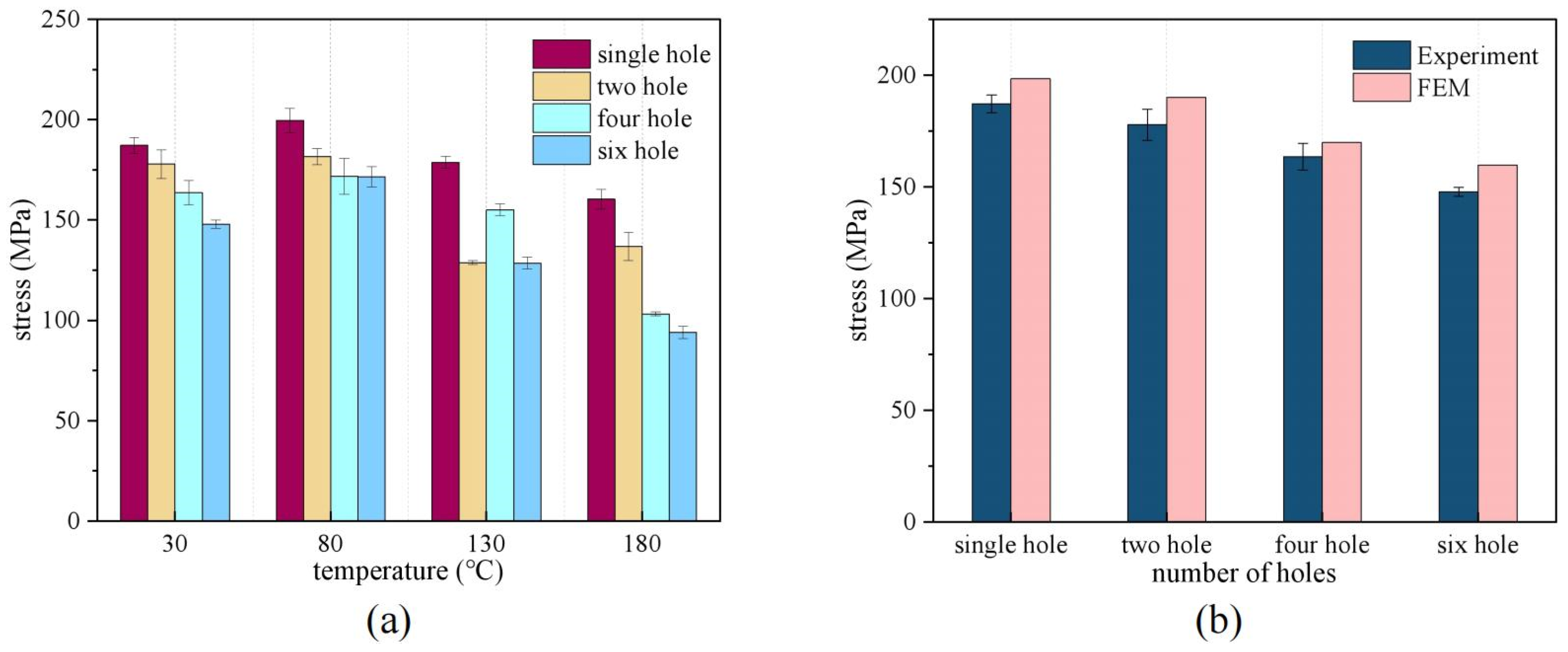
4.1. Examination of Finite Element Simulation Results
4.2. Comparison of Finite Element Simulation Results with Experimental Results
5. Analysis of Experimental Results
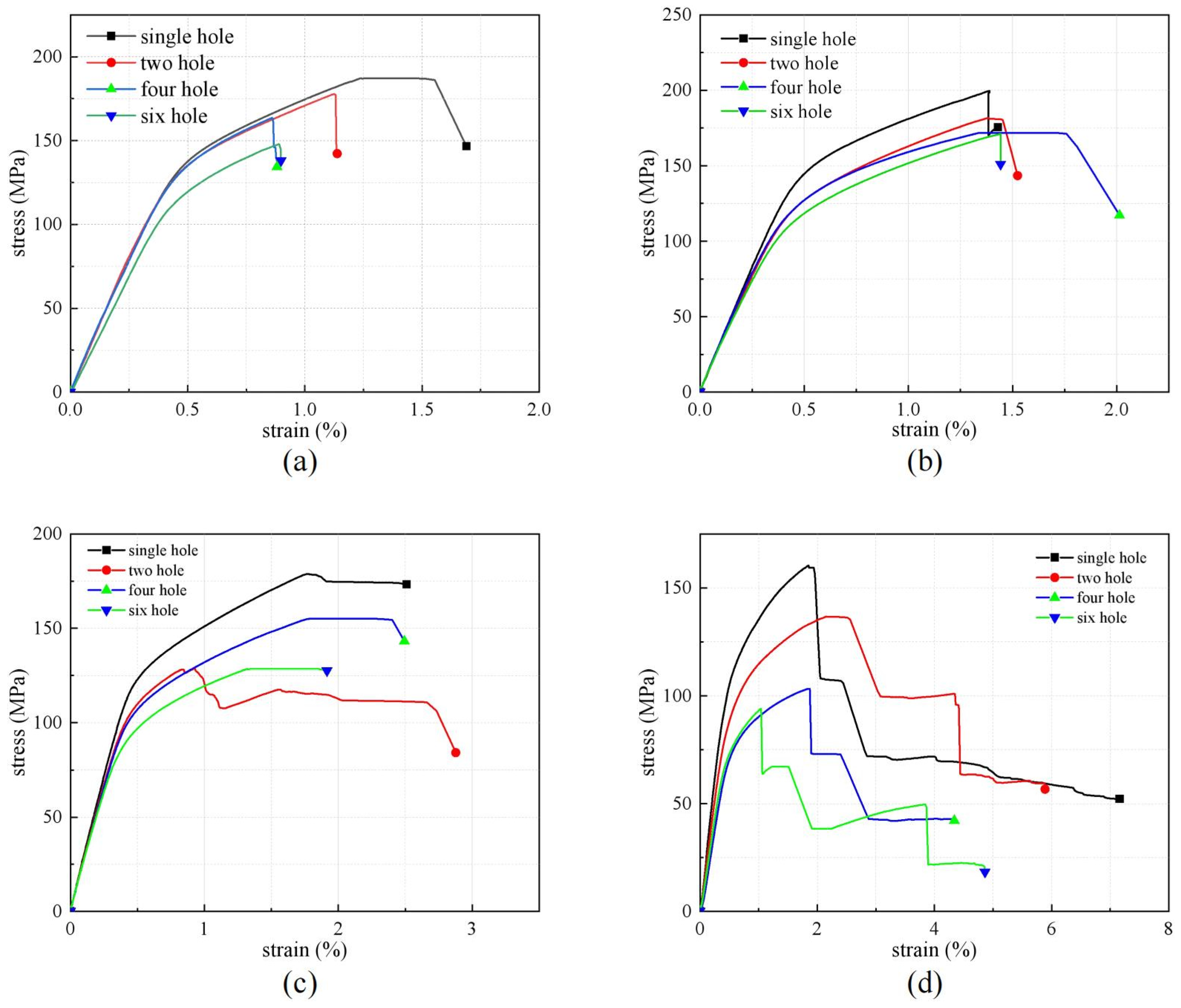
5.1. Tensile Response Analysis
5.2. Tensile Damage Mechanism
5.3. Microscopic Damage Mechanism at the Fracture Site
5.4. Digital Image Correlation Analysis
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- He, W.; Wang, L.; Liu, H.; Wang, C.; Yao, L.; Li, Q.; Sun, G. On Impact Behavior of Fiber Metal Laminate (FML) Structures: A State-of-the-Art Review. Thin-Walled Struct. 2021, 167, 108026. [Google Scholar] [CrossRef]
- Sadighi, M.; Alderliesten, R.C.; Benedictus, R. Impact Resistance of Fiber-Metal Laminates: A Review. Int. J. Impact Eng. 2012, 49, 77–90. [Google Scholar] [CrossRef]
- Tamrakar, S.; Kiziltas, A.; Mielewski, D.; Zander, R. Characterization of Kenaf and Glass Fiber Reinforced Hybrid Composites for Underbody Shield Applications. Compos. Part B Eng. 2021, 216, 108805. [Google Scholar] [CrossRef]
- Wang, M.; Zhang, J.; Yuan, H.; Guo, H.; Zhuang, W. The Plastic Behavior in the Large Deflection Response of Fiber Metal Laminate Sandwich Beams under Transverse Loading. Materials 2022, 15, 439. [Google Scholar] [CrossRef] [PubMed]
- Najafi, M.; Ansari, R.; Darvizeh, A.F. Effect of Cryogenic Aging on Nanophased Fiber Metal Laminates and Glass/Epoxy Composites. Polym. Compos. 2019, 40, 2523–2533. [Google Scholar] [CrossRef]
- Ucan, H.; Apmann, H.; Gral, G.; Krombholz, C.; Fortkamp, K.; Nieberl, D.; Schmick, F.; Nguyen, C.; Akin, D.N. Production Technologies for Lightweight Structures Made from Fibre–Metal Laminates in Aircraft Fuselages. CEAS Aeronaut. J. 2019, 10, 479–489. [Google Scholar] [CrossRef]
- Sun, G.; Zuo, W.; Chen, D.; Luo, Q.; Pang, T.; Li, Q. On the Effects of Temperature on Tensile Behavior of Carbon Fiber Reinforced Epoxy Laminates. Thin-Walled Struct. 2021, 164, 107769. [Google Scholar] [CrossRef]
- Cao, S.; Zhis, W.U.; Wang, X. Tensile Properties of CFRP and Hybrid FRP Composites at Elevated Temperatures. J. Compos. Mater. 2009, 43, 315–330. [Google Scholar] [CrossRef]
- Bhoominathan, R.; Arumugam, V.; Ashok, T.; Jefferson, A.J. Residual Strength Estimation of CFRP Laminates Subjected to Impact at Different Velocities and Temperatures. Polym. Compos. 2015, 38, 2182–2191. [Google Scholar] [CrossRef]
- Ahmed, A.; Liu, S.; Zhu, D.; Guo, S. A Review on the Tensile Behavior of Fiber-Reinforced Polymer Composites under Varying Strain Rates and Temperatures. Constr. Build. Mater. 2021, 294, 123565. [Google Scholar] [CrossRef]
- Ou, Y.; Zhu, D.; Zhang, H.; Yao, Y.; Mobasher, B.; Huang, L. Mechanical Properties and Failure Characteristics of CFRP under Intermediate Strain Rates and Varying Temperatures. Compos. Part B Eng. 2016, 95, 123–136. [Google Scholar] [CrossRef]
- Yao, L.; Zhang, S.; Cao, X.; Gu, Z.; Wang, C.; He, W. Tensile Mechanical Behavior and Failure Mechanisms of Fiber Metal Laminates under Various Temperature Environments. Compos. Struct. 2022, 284, 115142. [Google Scholar] [CrossRef]
- Askari, M.; Javadi, M.; Eslami-Farsani, R.; Geranmayeh, A. The Effect of Thermal and Cryogenic Environments on the Impact Performance of Aluminum-Glass Fibers/Epoxy Laminated Composites. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2023, 237, 5066–5078. [Google Scholar] [CrossRef]
- Cheng, Z.-Q.; Zhong, Y.-Z.; Tan, W.; Zhu, Z.-W.; Xiong, J.-J.; Liu, H. Experimental Investigation on the Low-Velocity Impact Responses of Fibre Metal Laminates with Various Internal and External Factors. Thin-Walled Struct. 2024, 201, 112004. [Google Scholar] [CrossRef]
- Seoane-Rivero, R.; German, L.; Santos, F.; Gondra, K. Development of New Hybrid Composites for High-Temperature Applications. Polymers 2023, 15, 4380. [Google Scholar] [CrossRef]
- Jiang, S.; Gao, L.; Xie, B.; Li, K.; Wu, C. Open-Hole Tensile Behavior and Progressive Damage of Hybrid Fiber Metal Laminates. Appl. Compos. Mater. 2022, 29, 547–563. [Google Scholar] [CrossRef]
- Kazemahvazi, S.; Kiele, J.; Zenkert, D. Tensile Strength of UD-Composite Laminates with Multiple Holes. Compos. Sci. Technol. 2010, 70, 1280–1287. [Google Scholar] [CrossRef]
- Gerendt, C.; Dean, A.; Mahrhol, T.; Rolfes, R. On the Progressive Failure Simulation and Experimental Validation of Fiber Metal Laminate Bolted Joints. Compos. Struct. 2019, 229, 111368. [Google Scholar] [CrossRef]
- Lin, Z.; Sheng, D.; Fang, Y.; Xiong, K.; Song, Y. Experimental and Numerical Investigation of the Tensile and Failure Response of Multiple-Hole-Fiber-Reinforced Magnesium Alloy Laminates under Various Temperature Environments. Materials 2023, 16, 5573. [Google Scholar] [CrossRef]
- Cortes, P.; Cantwell, W.J. The Fracture Properties of a Fibre-Metal Laminate Based on Magnesium Alloy. Compos. Part B Eng. 2006, 37, 163–170. [Google Scholar] [CrossRef]
- Sun, J.; Daliri, A.; Lu, G.; Liu, D.; Gong, A. Tensile Behaviour of Titanium-Based Carbon-Fibre/Epoxy Laminate. Constr. Build. Mater. 2021, 281, 122633. [Google Scholar] [CrossRef]
- Sarasini, F.; Tirillo, J.; Ferrante, L.; Sergi, C.; Sbardella, F.; Russo, P.; Simeoli, G.; Mellier, D.; Calzolari, A. Effect of Temperature and Fiber Type on Impact Behavior of Thermoplastic Fiber Metal Laminates. Compos. Struct. 2019, 223, 110961. [Google Scholar] [CrossRef]
- Moussavi-Torshizi, S.E.; Dariushi, S.; Sadighi, M.; Safarpour, P. A Study on Tensile Properties of a Novel Fiber/Metal Laminates. Mater. Sci. Eng. A 2010, 527, 4920–4925. [Google Scholar] [CrossRef]
- Singh, S.; Angra, S. Experimental Evaluation of Hygrothermal Degradation of Stainless Steel Fibre Metal Laminate. Eng. Sci. Technol. Int. J. 2018, 21, 170–179. [Google Scholar] [CrossRef]
- Ali, A.; Pan, L.; Duan, L.; Zheng, Z.; Sapkota, B. Characterization of Seawater Hygrothermal Conditioning Effects on the Properties of Titanium-Based Fiber-Metal Laminates for Marine Applications. Compos. Struct. 2016, 158, 199–207. [Google Scholar] [CrossRef]
- Xia, Y.; Wang, Y.; Zhou, Y. Effect of Strain Rate on Tensile Behavior of Carbon Fiber Reinforced Aluminum Laminates. Mater. Lett. 2007, 61, 213–215. [Google Scholar] [CrossRef]
- He, W.; Wang, C.; Wang, S.; Yao, L.; Xie, D. Characterizing and Predicting the Tensile Mechanical Behavior and Failure Mechanisms of Notched FMLs—Combined with DIC and Numerical Techniques. Compos. Struct. 2020, 254, 112893. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, Y.; Zhang, J.; Zhou, Z.; Fang, G.; Zhao, Y.; Zu, S. Characterizing the Off-Axis Dependence of Failure Mechanism in Notched Fiber Metal Laminates. Compos. Struct. 2018, 185, 148–160. [Google Scholar] [CrossRef]
- Kubit, A.; Trzepiecinski, T.; Konica, M.; Hebda, M.; Pytel, M.P. The Influence of Temperature Gradient Thermal Shock Cycles on the Interlaminar Shear Strength of Fibre Metal Laminate Composite Determined by the Short Beam Test. Compos. Part B Eng. 2019, 176, 107217. [Google Scholar] [CrossRef]
- Abdullah, A.S.; Kuntjoro, W.; Yamin, A.F.M. Finite Element Modelling of Aluminum Alloy 2024-T3 under Transverse Impact Loading. In Advanced Materials for Sustainability and Growth, Proceedings of the 3rd Advanced Materials Conference 2016 (3rd AMC 2016), Langkawi, Malaysia, 28–29 November 2016; Ibrahim, S.M., Noorsal, K., Eds.; AIP Publishing: Melville, NY, USA, 2017; Volume 1901, p. 050005. [Google Scholar]
- Tsai, S.W.; Wu, E.M. A General Theory of Strength for Anisotropic Materials. J. Compos. Mater. 1971, 5, 58–80. [Google Scholar] [CrossRef]
- Chang, F.K.; Chang, K.Y. A Progressive Damage Model for Laminated Composites Containing Stress Concentrations. J. Compos. Mater. 1987, 21, 834–855. [Google Scholar] [CrossRef]
- Hashin, Z. Failure Criteria for Unidirectional Fiber Composites. J. Appl. Mech. 1980, 47, 329–334. [Google Scholar] [CrossRef]
- Yeh, H.-Y.; Kim, C.H. The Yeh-Stratton Criterion for Composite Materials. J. Compos. Mater. 1994, 28, 926–939. [Google Scholar] [CrossRef]
- Vo, T.P.; Guan, Z.W.; Cantwell, W.J.; Schleyer, G.K. Low-Impulse Blast Behaviour of Fibre-Metal Laminates. Compos. Struct. 2012, 94, 954–965. [Google Scholar] [CrossRef]
- Benzeggagh, M.L.; Kenane, M. Measurement of Mixed-Mode Delamination Fracture Toughness of Unidirectional Glass/Epoxy Composites with Mixed-Mode Bending Apparatus. Compos. Sci. Technol. 1996, 56, 439–449. [Google Scholar] [CrossRef]

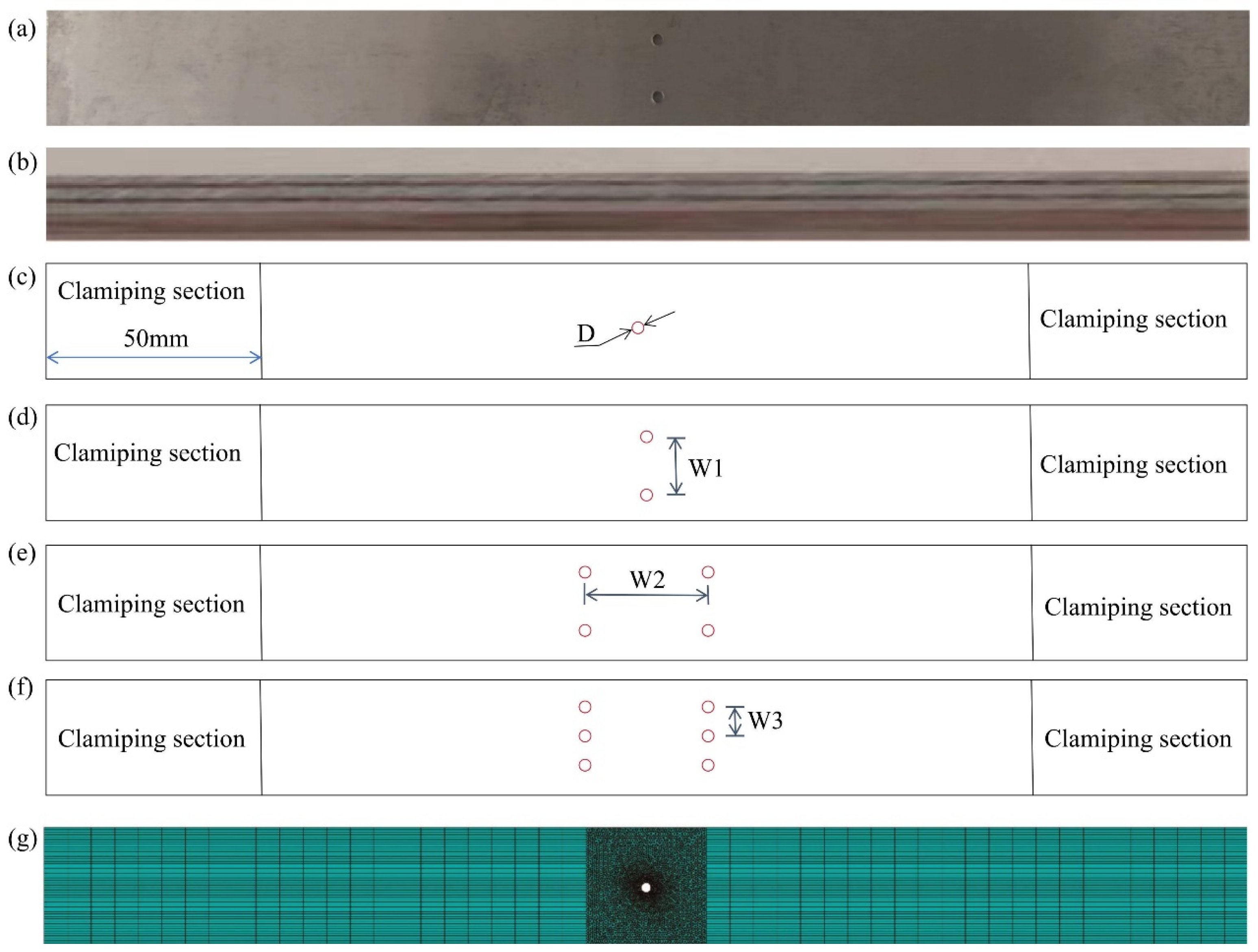
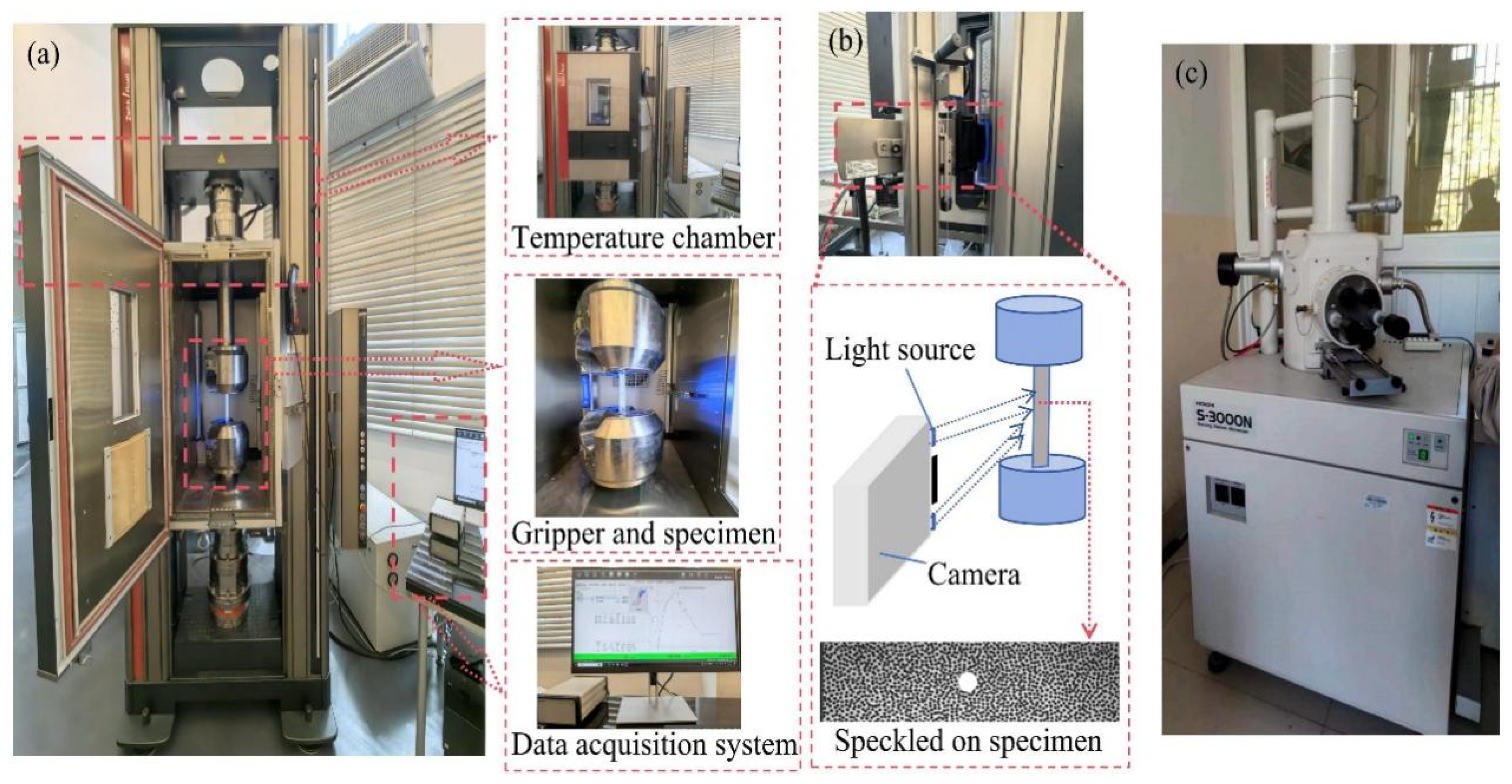





| Mechanical Property | Parameters | Mechanical Property | Parameters |
|---|---|---|---|
| 28.5 | 41.38 | ||
| 6.4 | 500 | ||
| 6.4 | 6.5 | ||
| 2.5 | 60 | ||
| 1.3 | 0.3 | ||
| 41.38 | 0.3 |
| 2700 kg/m3 | Yield Strength | 292 MPa | Fracture Energy | 10,200 J/m2 | ||
| E | 72 GPa | Poisson’s Ration | 0.33 | Fracture Strain | 0.15 | |
| Isotropic Hardening Data | ||||||
| stress (MPa) | 292 | 300 | 313 | 322 | 335 | 342 |
| strain (%) | 0 | 0.098 | 0.29 | 0.47 | 0.78 | 0.97 |
| stress (MPa) | 354 | 360 | 376 | 392 | 406 | 428 |
| strain (%) | 1.47 | 1.65 | 2.48 | 3.49 | 4.82 | 7.18 |
| Johnson–Cook Parameterizations of an Isomorphic Model | ||||
|---|---|---|---|---|
| A | B | C | n | m |
| 369 | 684 | 0.001 | 0.73 | 2.75 |
| Crack model parameters | ||||
| D1 | D2 | D3 | D4 | D5 |
| 0.112 | 0.123 | −1.5 | 0.007 | 0 |
| E (GPa) | t0 (MPa) | Gc (N/mm) | Density (kg/mm3) | Final Temperature | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 0.75 | 0.75 | 65 | 38 | 38 | 2 | 4 | 4 | 920 | 180 |
| Cases | Sequence | Ultimate Tensile Stress (MPa) | Ultimate Tensile Stress (MPa) | Ultimate Tensile Stress (MPa) | Ultimate Tensile Stress (MPa) |
|---|---|---|---|---|---|
| FMLs (single hole) | FMLs (two hole) | FMLs (four hole) | FMLs (six hole) | ||
| 30 °C | 1 | 190.78 | 177.77 | 163.60 | 149.34 |
| 2 | 187.17 | 170.92 | 169.42 | 147.85 | |
| 3 | 182.33 | 182.41 | 162.19 | 148.84 | |
| Average value | 186.76 | 177.03 | 165.07 | 148.68 | |
| 80 °C | 1 | 199.57 | 181.62 | 171.81 | 166.77 |
| 2 | 193.44 | 185.36 | 180.10 | 171.59 | |
| 3 | 204.46 | 182.09 | 174.80 | 169.29 | |
| Average value | 199.16 | 183.02 | 175.57 | 169.21 | |
| 130 °C | 1 | 178.75 | 129.18 | 152.09 | 128.71 |
| 2 | 181.03 | 128.76 | 155.11 | 128.90 | |
| 3 | 172.98 | 128.01 | 159.90 | 125.54 | |
| Average value | 177.59 | 128.65 | 155.70 | 127.72 | |
| 180 °C | 1 | 160.37 | 136.70 | 103.23 | 93.97 |
| 2 | 164.97 | 129.56 | 103.10 | 96.66 | |
| 3 | 168.45 | 139.51 | 102.37 | 92.90 | |
| Average value | 164.60 | 135.26 | 102.9 | 94.51 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, H.; Sheng, D.; Fang, Y.; Yu, H.; Yang, F. Analysis of Tensile Failure Behavior of Metal Fiber Laminates Under Different Temperature Environments. Polymers 2024, 16, 3319. https://doi.org/10.3390/polym16233319
Lu H, Sheng D, Fang Y, Yu H, Yang F. Analysis of Tensile Failure Behavior of Metal Fiber Laminates Under Different Temperature Environments. Polymers. 2024; 16(23):3319. https://doi.org/10.3390/polym16233319
Chicago/Turabian StyleLu, Hongbin, Dongfa Sheng, Yuting Fang, Hongquan Yu, and Fan Yang. 2024. "Analysis of Tensile Failure Behavior of Metal Fiber Laminates Under Different Temperature Environments" Polymers 16, no. 23: 3319. https://doi.org/10.3390/polym16233319
APA StyleLu, H., Sheng, D., Fang, Y., Yu, H., & Yang, F. (2024). Analysis of Tensile Failure Behavior of Metal Fiber Laminates Under Different Temperature Environments. Polymers, 16(23), 3319. https://doi.org/10.3390/polym16233319






