Evaluation of U-Notch and V-Notch Geometries on the Mechanical Behavior of PVDF: The DIC Technique and FEA Approach
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Methodology
2.1.1. Material and Specimen Preparation
2.1.2. Experimental Setup of DIC
2.1.3. FE Model Implementation
3. Results and Discussion
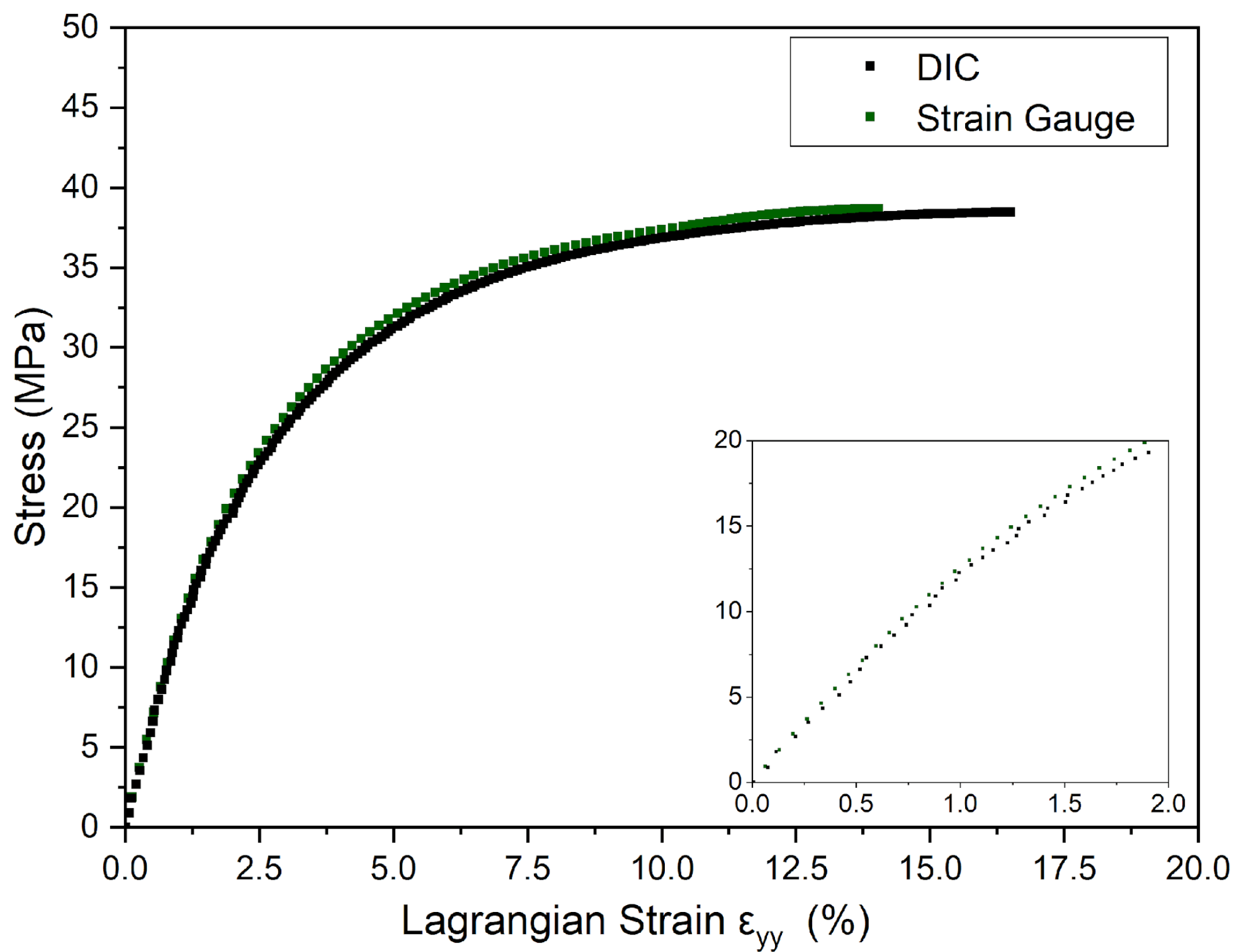
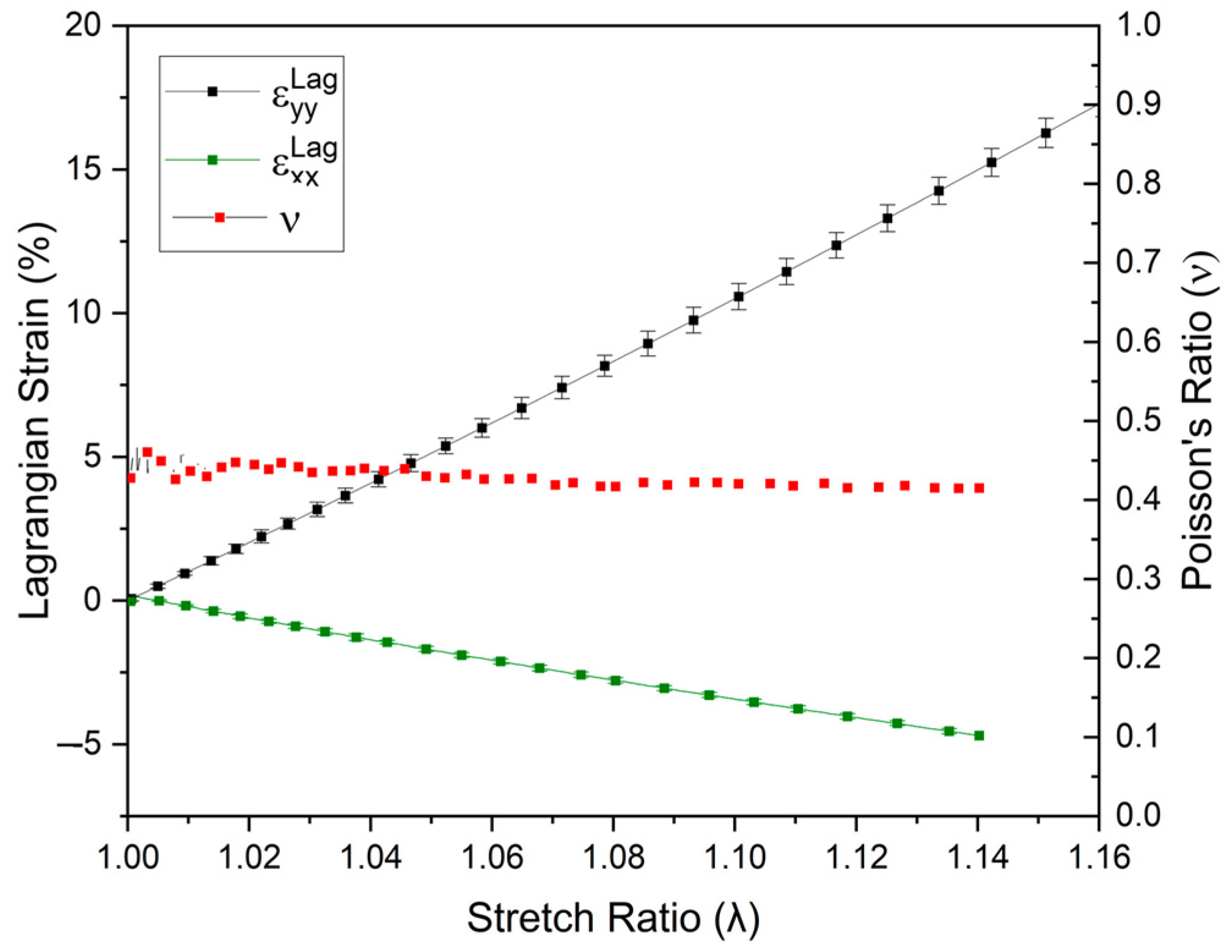
3.1. Tensile Behavior of Unnotched Specimens: DIC and Extensometer
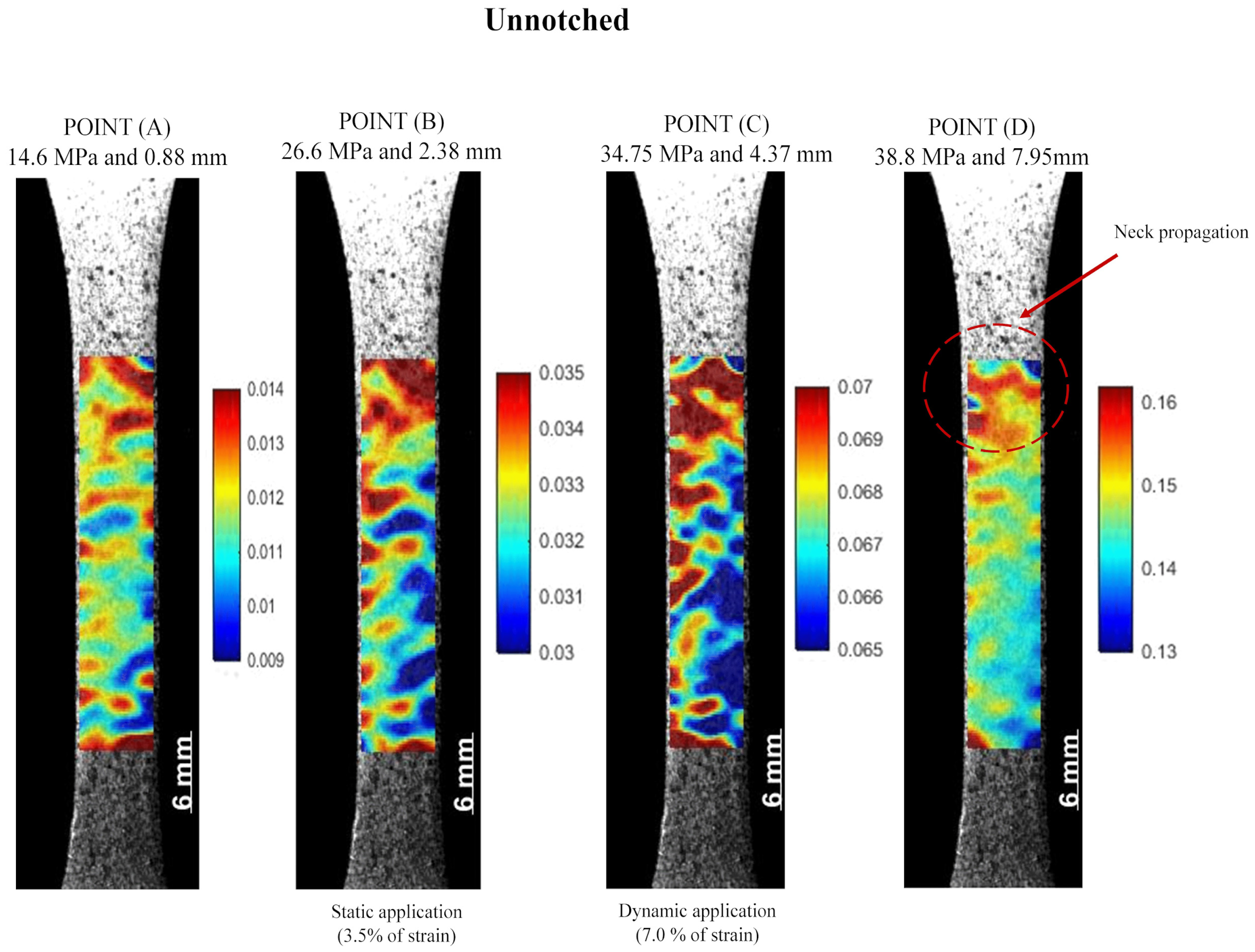
3.2. Strain Map Distribution for Unnotched Specimens
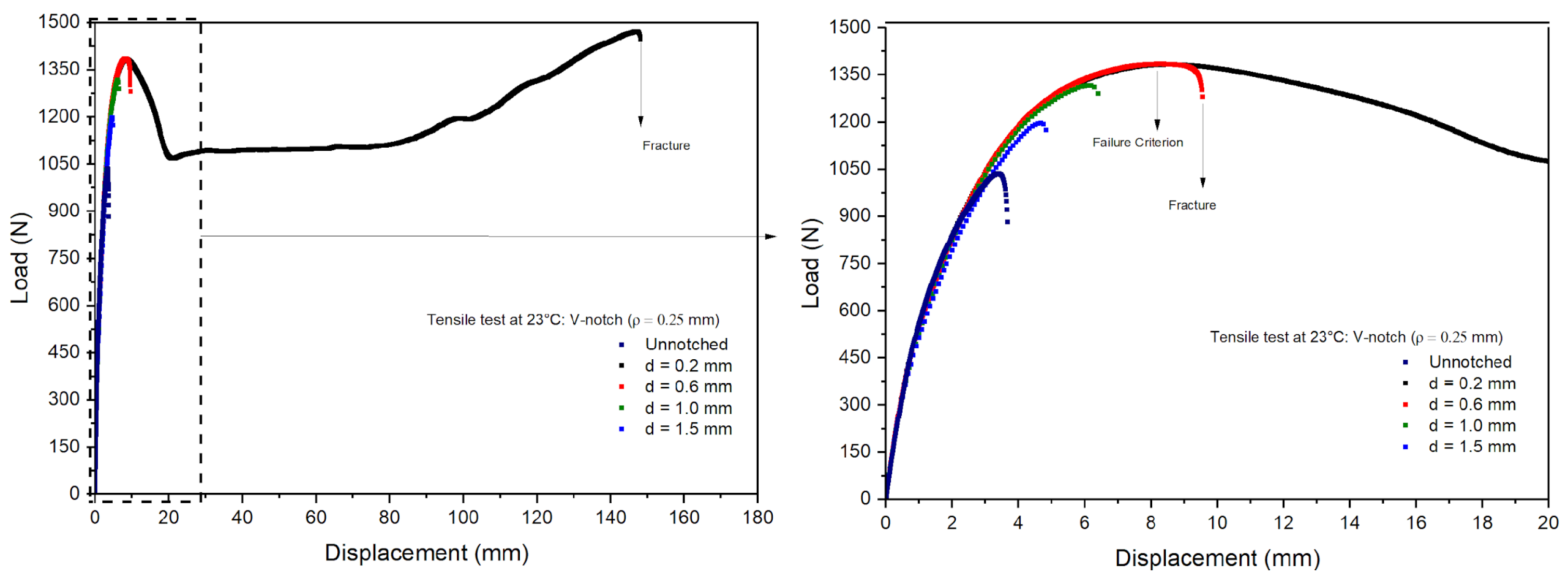
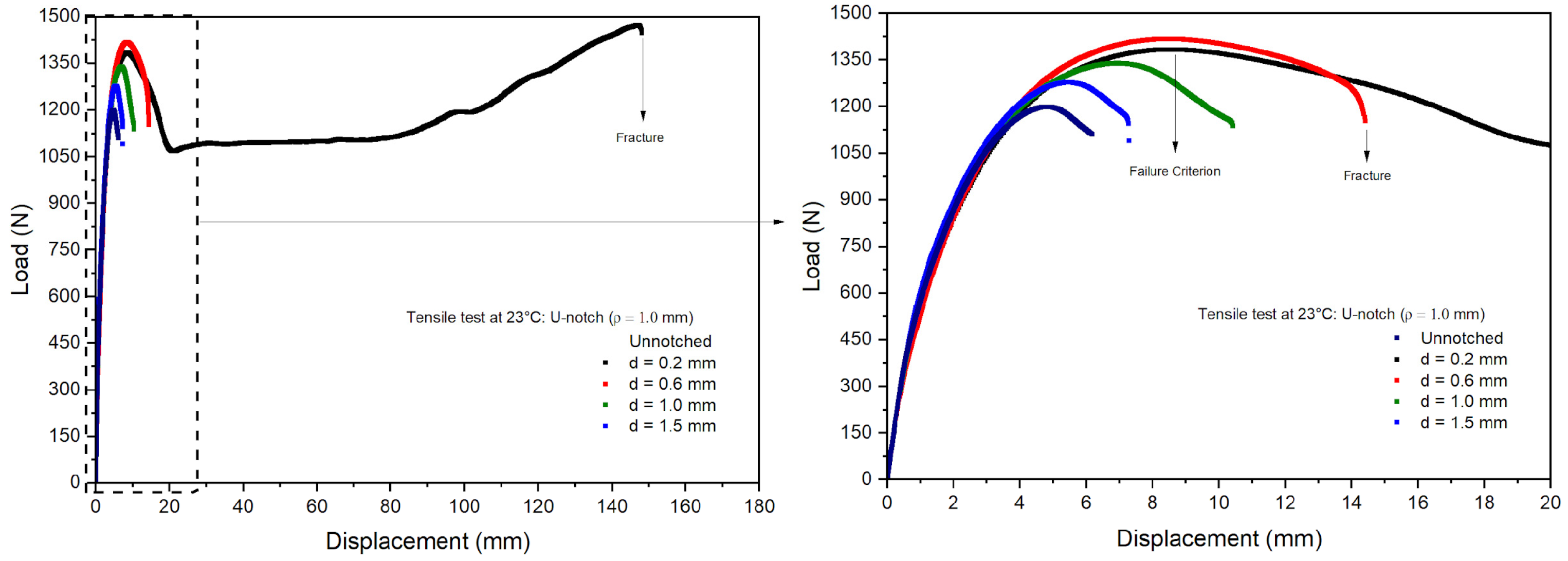
3.3. Tensile Behavior of Notched Specimens
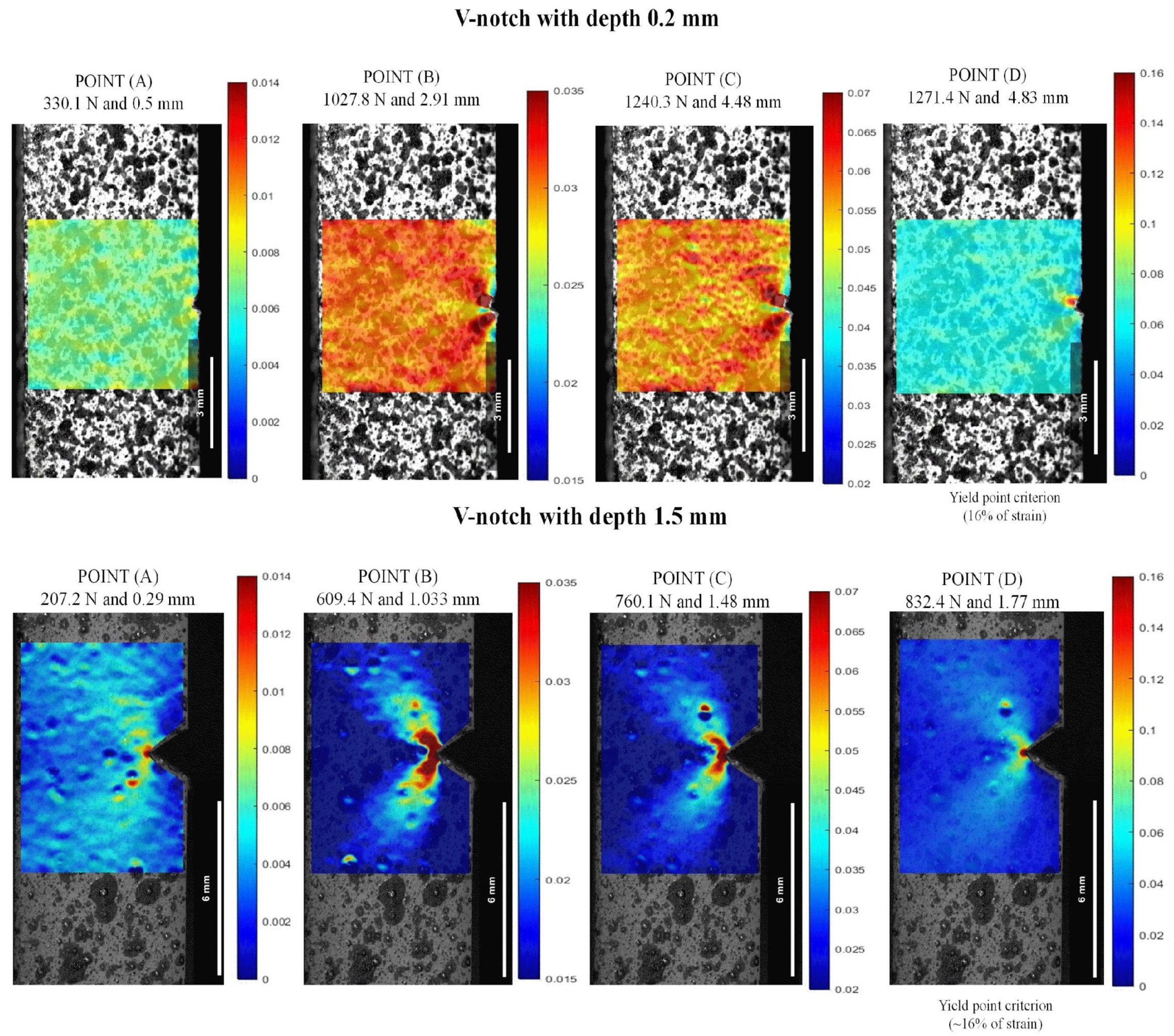
3.4. Strain Maps for Notched Specimens Using the DIC Technique
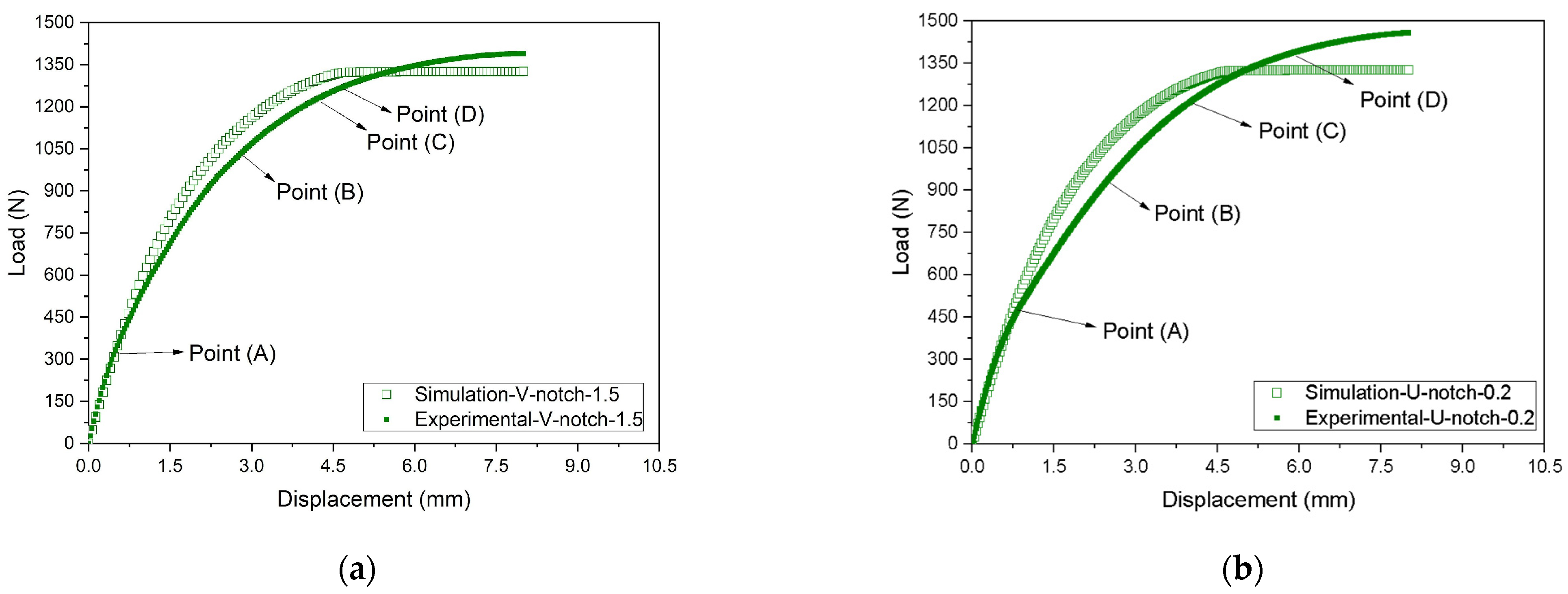
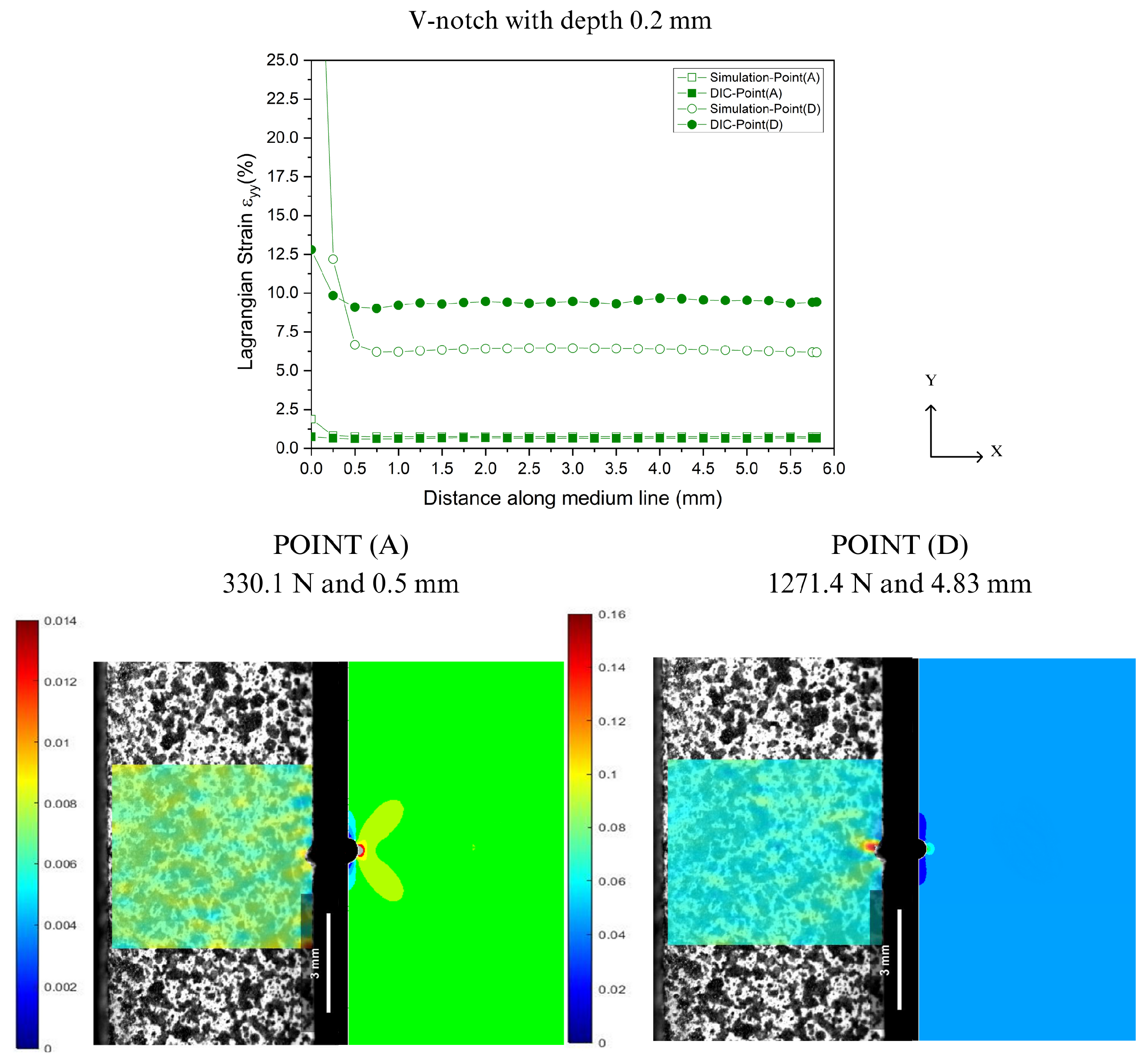
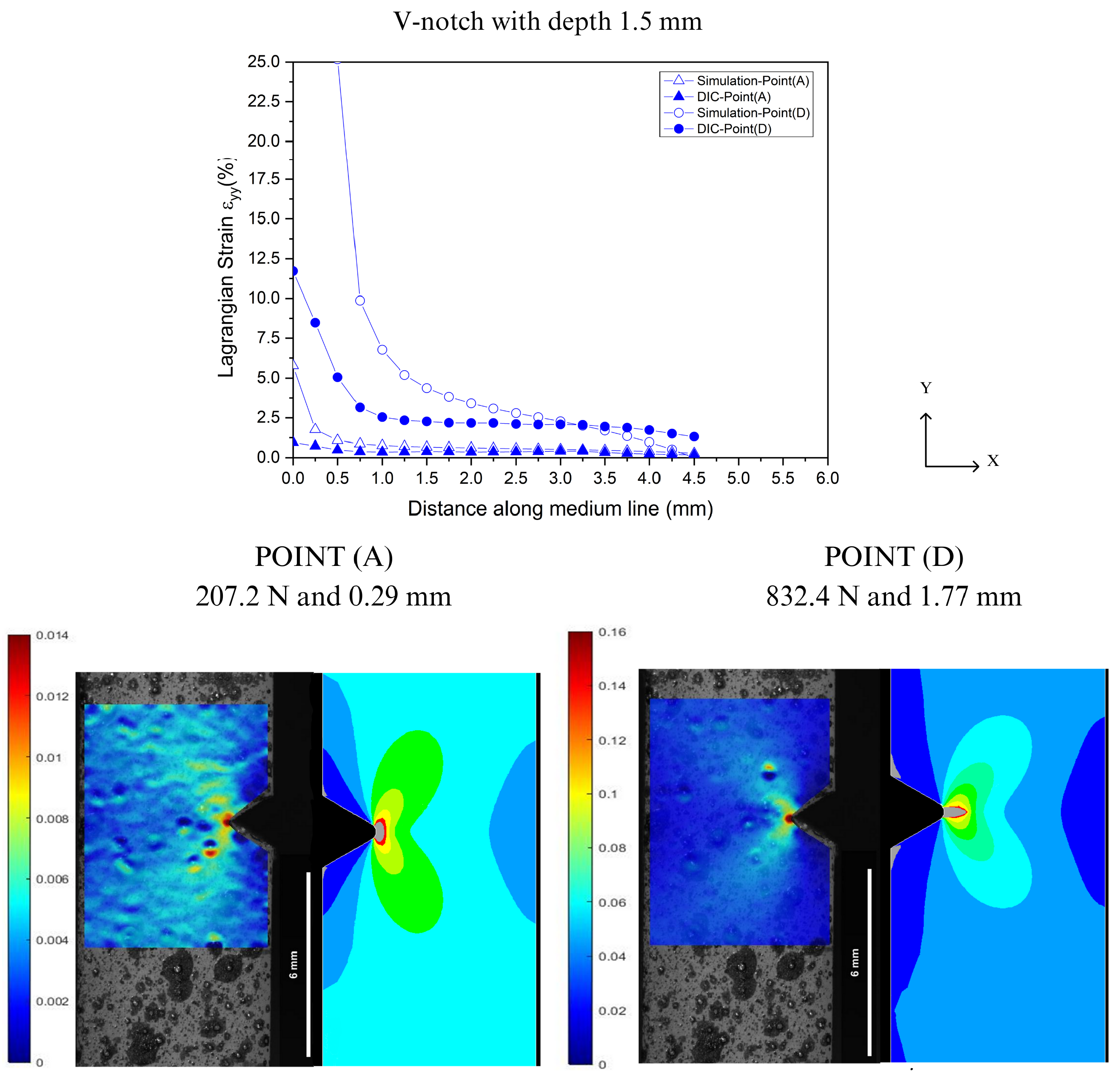
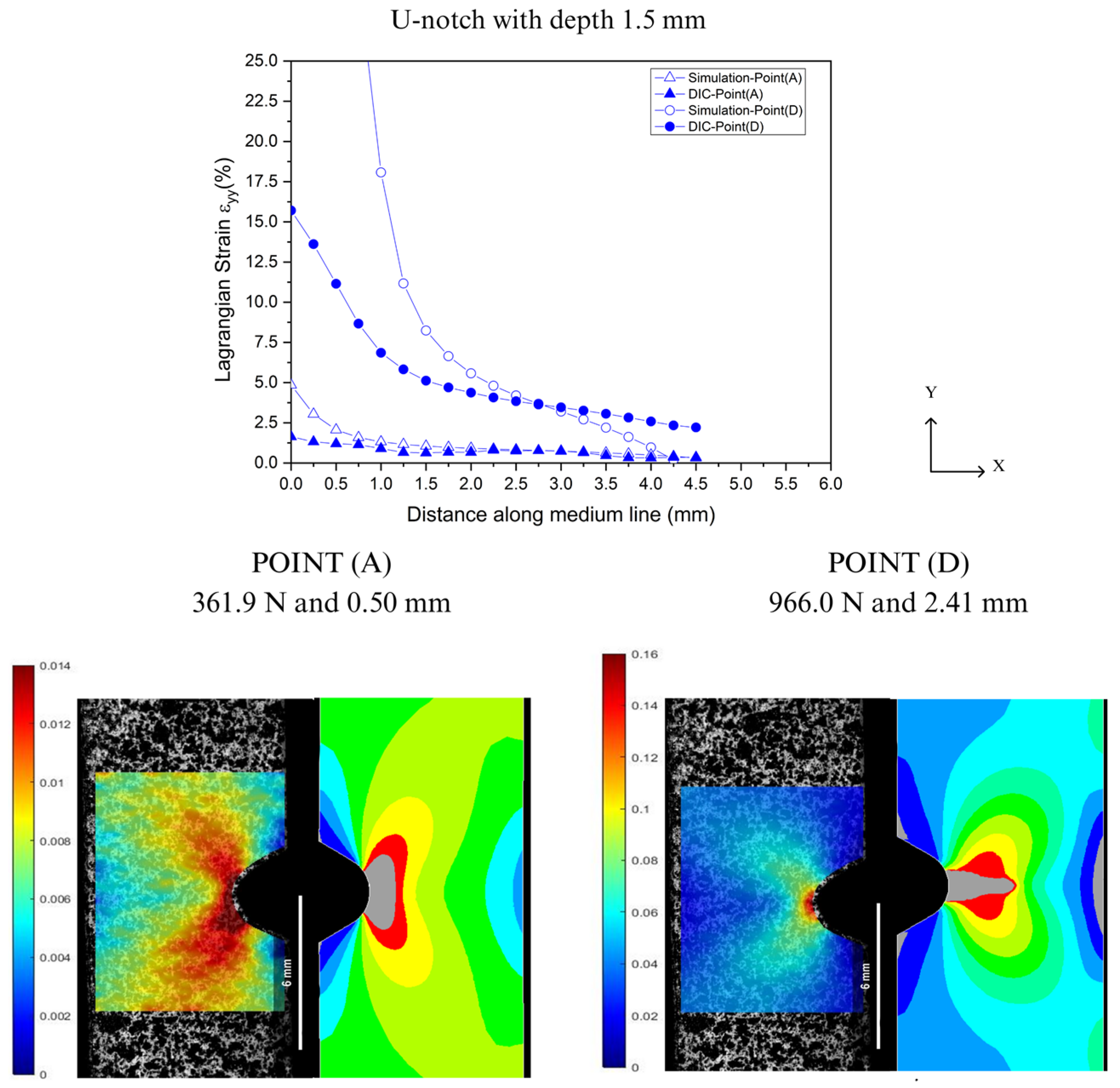
3.5. FEA of Notched Specimens
4. Conclusions
- In the experimental tests, the decay of the failure loads was observed with an increase in notch depths and a reduction in the notch radius in both U and V geometries. The decay is associated with an increased three-dimensional stress state at the notch tip.
- The DIC method showed the notch effect on the samples’ deformation by generating strain maps. Severe constraints were detected, and the deformation around the notch tip under tensile loading was quantified.
- The FEA showed similar load vs. displacement curves compared to those obtained experimentally. Hence, the FE model well captured the global behavior of the unnotched and notched samples.
- When comparing the DIC technique and FEA, it was observed that numerical simulation can lead to deviations, possibly due to the continuous approach adopted in the FE models, which may affect the stress field close to the notches. This aspect may be studied in future work. Additionally, the difference in resolution levels between the DIC technique and FEA can influence the results measured for strain around the notch. However, a reasonable convergence between numerical and experimental strain results was observed at points distant 0.5 mm or higher from the defect.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Hertzberg, R.W. Deformation and Fracture Mechanics of Engineering Materials, 4th ed.; John Wiley & Sons: New York, NY, USA, 1992; Volume 9. [Google Scholar]
- Schijve, J. Stress Concentration at Notches. In Fatigue of structure and Materials; Springer: Berlin/Heidelberg, Germany, 2008; pp. 59–88. [Google Scholar]
- Qu, R.; Zhang, P.; Zhang, Z. Notch Effect of Materials: Strengthening or Weakening? J. Mater. Sci. Technol. 2014, 30, 599–608. [Google Scholar] [CrossRef]
- Peterson, T.L.; Ast, D.G.; Kramer, E.J. Holographic Interferometry of Crazes in Polycarbonate. J. Appl. Phys. 1974, 45, 4220–4228. [Google Scholar] [CrossRef]
- Ayatollahi, M.R.; Mirsayar, M.M.; Dehghany, M. Experimental Determination of Stress Field Parameters in Bi-Material Notches Using Photoelasticity. Mater. Des. 2011, 32, 4901–4908. [Google Scholar] [CrossRef]
- Abshirini, M.; Dehnavi, M.Y.; Beni, M.A.; Soltani, N. Interaction of Two Parallel U-Notches with Tip Cracks in PMMA Plates under Tension Using Digital Image Correlation. Theor. Appl. Fract. Mech. 2014, 70, 75–82. [Google Scholar] [CrossRef]
- Dehnavi, M.Y.R.; Eshraghi, I.; Soltani, N. Investigation of Fracture Parameters of Edge V-Notches in a Polymer Material Using Digital Image Correlation. Polym. Test. 2013, 32, 778–784. [Google Scholar] [CrossRef]
- Ayatollahi, M.R.; Torabi, A.R.; Rahimi, A.S. Brittle Fracture Assessment of Engineering Components in the Presence of Notches: A Review. Fatigue Fract. Eng. Mater. Struct. 2015, 39, 267–291. [Google Scholar] [CrossRef]
- Pereira, I.C.S. Crack and Notch Sensitivity of Polyvinylidene Fluoride (PVDF) Experimentally Evaluated by DIC and Compared to FEM; UFRJ: Rio de Janeiro, Brazil, 2021. [Google Scholar]
- Bhadori, R.; Ayatollahi, M.R.; Shahmohammadi, M.; Berto, F. Geometry Effects on Mode I Brittle Fracture in U-Notched Specimens. Fatigue Fract. Eng. Mater. Struct. 2021, 44, 901–915. [Google Scholar] [CrossRef]
- Gómez, F.J.; Elices, M. Fracture of Components with V-Shaped Notches. Eng. Fract. Mech. 2003, 70, 1913–1927. [Google Scholar] [CrossRef]
- Brugo, T.M.; Campione, I.; Minak, G. Investigation by Digital Image Correlation of Mixed-Mode I and II Fracture Behavior of Polymeric IASCB Specimens with Additive Manufactured Crack-like Notch. Materials 2021, 14, 1084. [Google Scholar] [CrossRef]
- Spagnoli, A.; Terzano, M.; Brighenti, R.; Artoni, F.; Carpinteri, A. How Soft Polymers Cope with Cracks and Notches. Appl. Sci. 2019, 9, 1086. [Google Scholar] [CrossRef]
- Liu, M.; Guo, J.; Hui, C.Y.; Zehnder, A.T. Application of Digital Image Correlation (DIC) to the Measurement of Strain Concentration of a PVA Dual-Crosslink Hydrogel Under Large Deformation. Exp. Mech. 2019, 59, 1021–1032. [Google Scholar] [CrossRef]
- Torabi, A.R.; Bahrami, B.; Ayatollahi, M.R. On the Use of Digital Image Correlation Method for Determining the Stress Fi Eld at Blunt V-Notch Neighborhood. Eng. Fract. Mech. 2020, 223, 106768. [Google Scholar] [CrossRef]
- Bahrami, B.; Ayatollahi, M.R.; Torabi, A.R. In-Situ Brittle Fracture Analysis of Sharp V-Notched Components Using Digital Image Correlation. Theor. Appl. Fract. Mech. 2020, 106, 102484. [Google Scholar] [CrossRef]
- Esaklul, K.A.; Mason, J. Nonmetallics Applications in Oil and Gas Production (Pipes, Liners, Rehabilitations). In Trends in Oil and Gas Corrosion Research and Technologies: Production and Transmission; Elsevier Ltd.: Amsterdam, The Netherlands, 2017; pp. 627–660. ISBN 9780081012192. [Google Scholar]
- API RP 17B; Recommended Practice for Flexible Pipe. American Petroleum Institute: Washington, DC, USA, 2014.
- Razavi, S.M.J.; Ayatollahi, M.R.; Shahmohammadi, M.; Berto, F. Geometry Effect on Fracture Behavior of V-Notched Specimens. Procedia Struct. Integr. 2020, 26, 234–239. [Google Scholar] [CrossRef]
- Silva, A.J.d.J. Avaliação Do Evelhecimento Do Poli (Fluoreto de Vinilideno) (PVDF) Visando Aplicações Em Estruturas Para Contato Com Etanol Combustível; Universidade Federal do Rio de Janeiro: Rio de Janeiro, Brazil, 2017. [Google Scholar]
- Hund, J.; Møgster, H.; Nordmark, S.; Henrik, P.; Johnsen, J.; Holm, A. Impact of Stress Triaxiality, Strain Rate, and Temperature on the Mechanical Response and Morphology of PVDF. Polym. Test. 2022, 114, 107717. [Google Scholar] [CrossRef]
- Ychisawa, L.K. Uso Do Método Spb Com Uso de Digital Image Correlation (DIC) Para Avaliação Da Tenacidade à Fratura de PVDF; Universidade Federal do Rio de Janeiro: Rio de Janeiro, Brazil, 2019. [Google Scholar]
- ISO-527-2; Plastics—Determination of Tensile Properties—Part 2: Test Conditions for Moulding and Extrusion Plastics 2012. International Organization for Standardization: Geneva, Switzerland, 2012.
- Schneider, C.A.; Rasband, W.S.; Eliceiri, K.W. NIH Image to Image J: 25 Years of Image Analysis. Nat. Methods 2012, 9, 671–675. [Google Scholar] [CrossRef]
- Fagerholt, E. ECorrLab. Available online: https://folk.ntnu.no/egilf/ecorr/doc/acquisition/ecorrlabv4/ecorrlabv4.html (accessed on 3 July 2024).
- Blaber, J.; Adair, B.; Antoniou, A. Ncorr: Open-Source 2D Digital Image Correlation Matlab Software. Exp. Mech. 2015, 55, 1105–1122. [Google Scholar] [CrossRef]
- ANSYS® Academic Research Mechanical, ANSYS 2023, Release 19.2; ANSYS Inc.: Canonsburg, PA, USA, 2018.
- Thirumump, M.; Kalita, K.; Ramachandran, M.; Ghadai, R. A Numerical Study of SCF Convergence Using ANSYS. J. Eng. Appl. Sci. 2015, 10, 2233–2238. [Google Scholar]
- Zheng, Q.; Mashiwa, N.; Furushima, T. Evaluation of Large Plastic Deformation for Metals by a Non-Contacting Technique Using Digital Image Correlation with Laser Speckles. Mater. Des. 2020, 191, 108626. [Google Scholar] [CrossRef]
- Bartczak, Z.; Galeski, A. Plasticity of Semicrystalline Polymers. Macromol. Symp. 2010, 294, 67–90. [Google Scholar] [CrossRef]
- API 17J 2014; American Petroleum Institute Specification for Unbonded Flexible Pipe. American Petroleum Institute: Washington, DC, USA, 2014.
- Defebvin, J.; Barrau, S.; Stoclet, G.; Rochas, C.; Lefebvre, J.M. In Situ SAXS/WAXS Investigation of the Structural Evolution of Poly(Vinylidene Fluoride) upon Uniaxial Stretching. Polymer 2016, 84, 148–157. [Google Scholar] [CrossRef]
- Castagnet, S.; Gacougnolle, J.L.; Dang, P. Correlation between Macroscopical Viscoelastic Behaviour and Micromechanisms in Strained α Polyvinylidene Fluoride (PVDF). Mater. Sci. Eng. A 2000, 276, 152–159. [Google Scholar] [CrossRef]
- Castagnet, S.; Girault, S.; Gacougnolle, J.L.; Dang, P. Cavitation in Strained Polyvinylidene Fluoride: Mechanical and X-Ray Experimental Studies. Polymer 2000, 41, 7523–7530. [Google Scholar] [CrossRef]
- ASTM D638-14; Standard Test Method for Tensile Properties of Plastics. ASTM: West Conshohocken, PA, USA, 2014; pp. 1–9.
- Lakes, R.S.; Wineman, A. On Poisson’s Ratio in Linearly Viscoelastic Solids. J. Elast. 2006, 85, 45–63. [Google Scholar] [CrossRef]
- Goolsby, R.D.; Chatterjee, A.M. Notch Sensitivity and Fractography of Polyolefins. Polym. Eng. Sci. 1983, 23, 117–124. [Google Scholar] [CrossRef]
- Pilkey, W.D.; Pilkey, D.F. Peterson’s Stress Concentration Factors, 3rd ed.; John Wiley & Sons: New York, NY, USA, 2009; ISBN 9780471032212. [Google Scholar]
- Challier, M.; Besson, J.; Laiarinandrasana, L.; Piques, R.; Hochstetter, G.; Pignoc, S. Damage and Fracture of Polyvinylidene Fluoride (PVDF) at 20 °C: Experiments and Modelling. Eng. Fract. Mech. 2006, 73, 79–90. [Google Scholar] [CrossRef]
- Maier, G.A.; Wallner, G.; Lang, R.W.; Fratzl, P. Structural Changes during Plastic Deformation at Crack Tips in PVDF Films: A Scanning X-Ray Scattering Study. Macromolecules 2005, 38, 6099–6105. [Google Scholar] [CrossRef]
- Liu, M. Experimental Studies of the Mechanical Behavior of a PVA Dual-Crosslink Hydrogel; Faculty of the Graduate School of Cornell University: Ithaca, NY, USA, 2019. [Google Scholar]
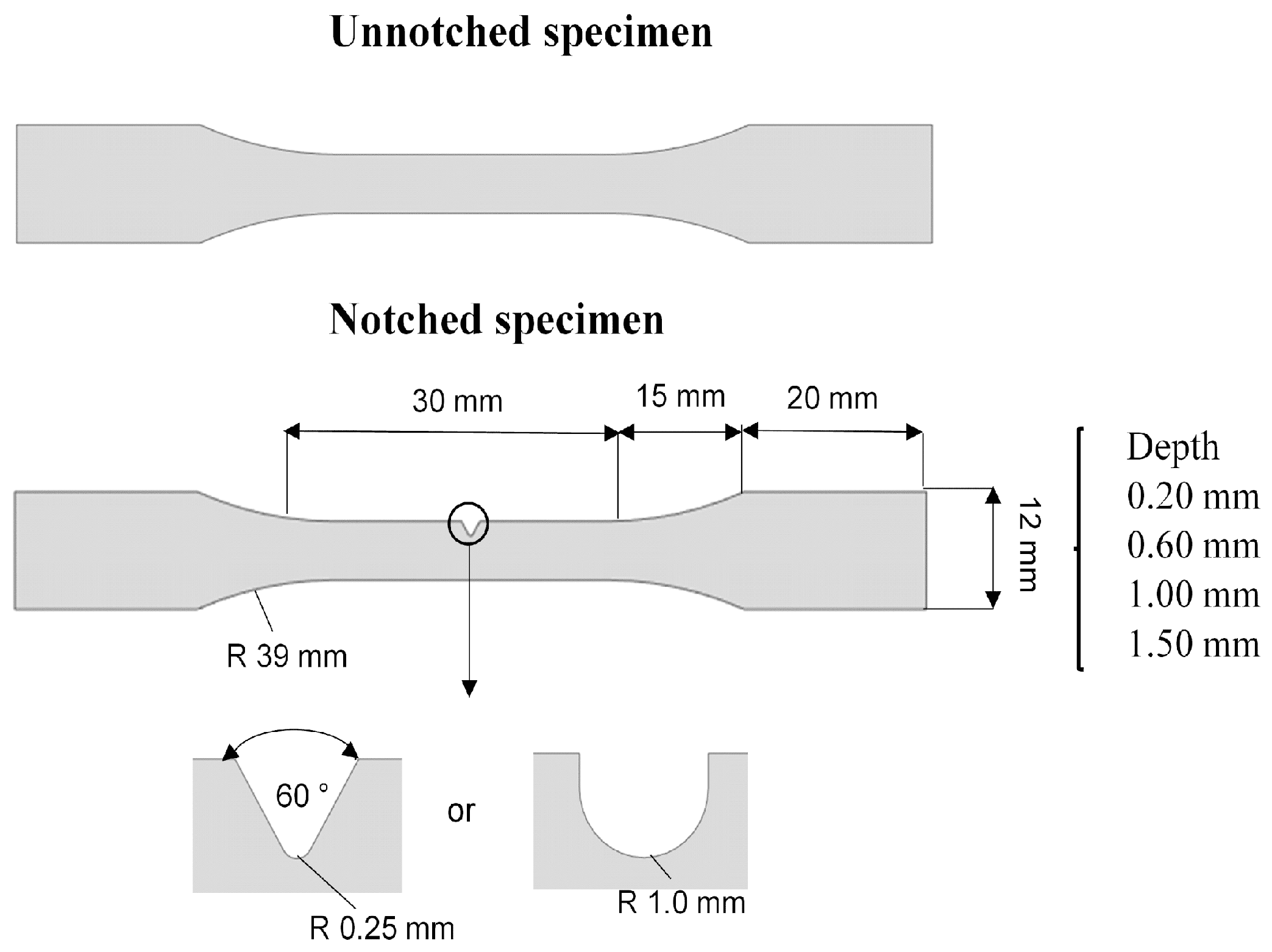
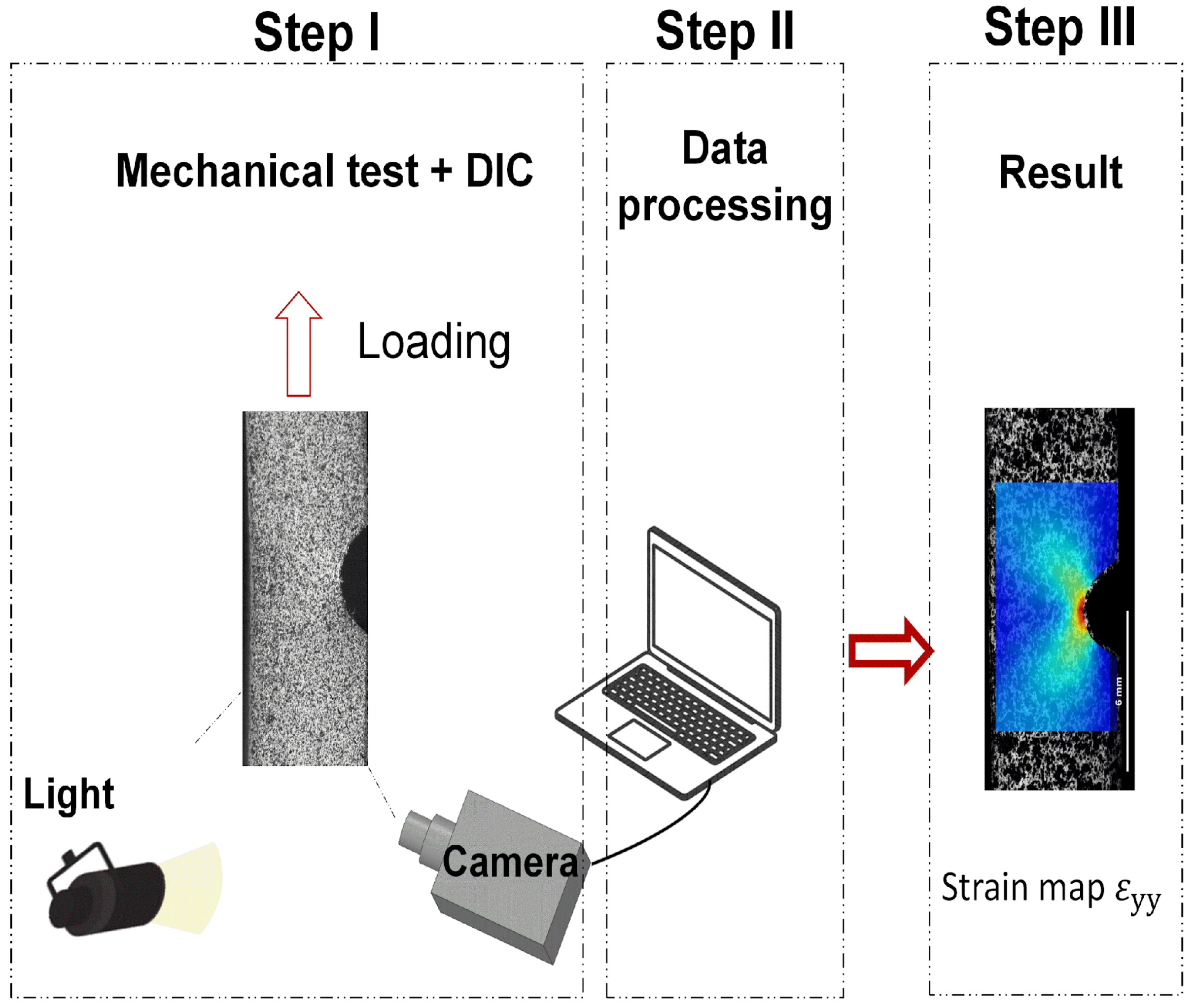
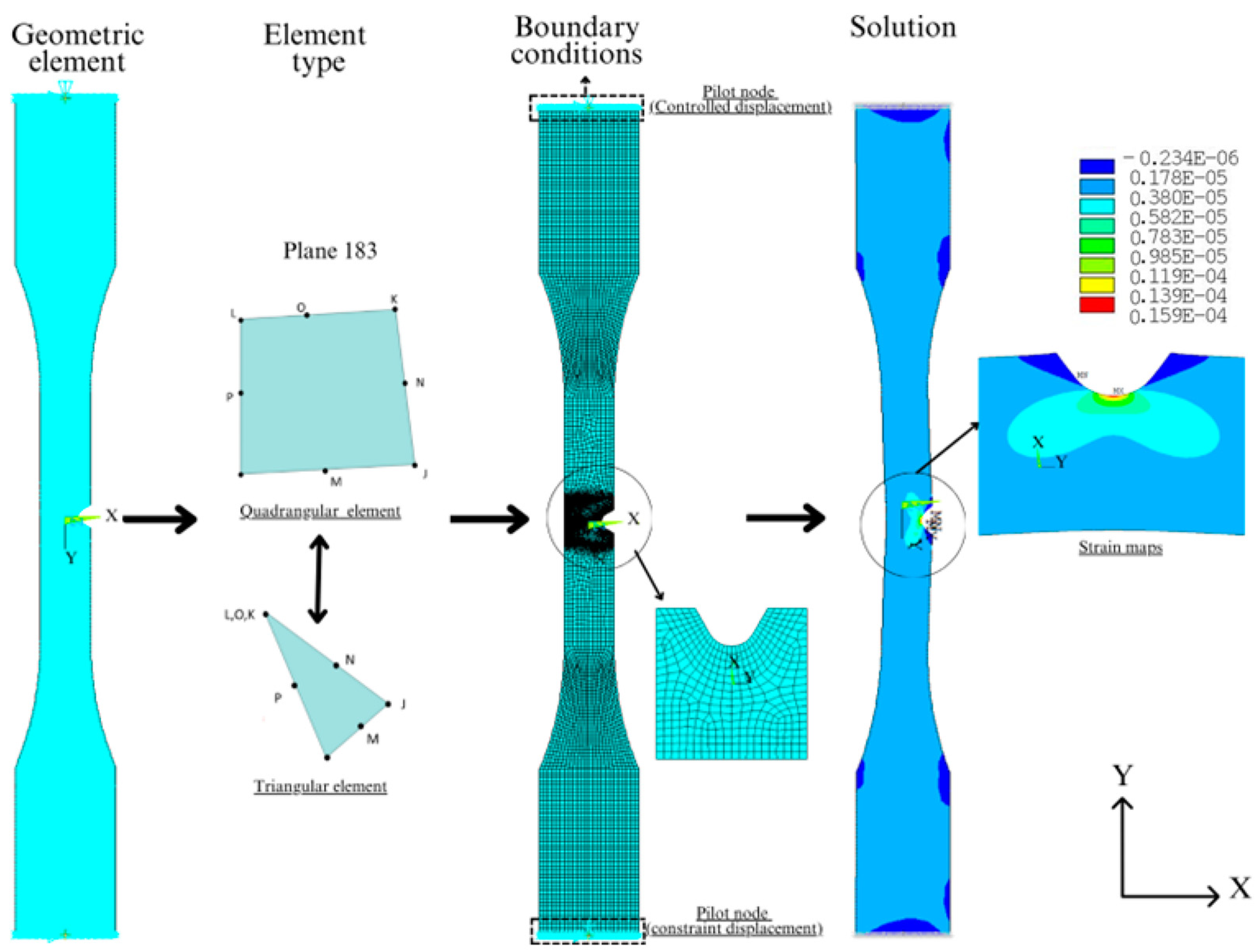
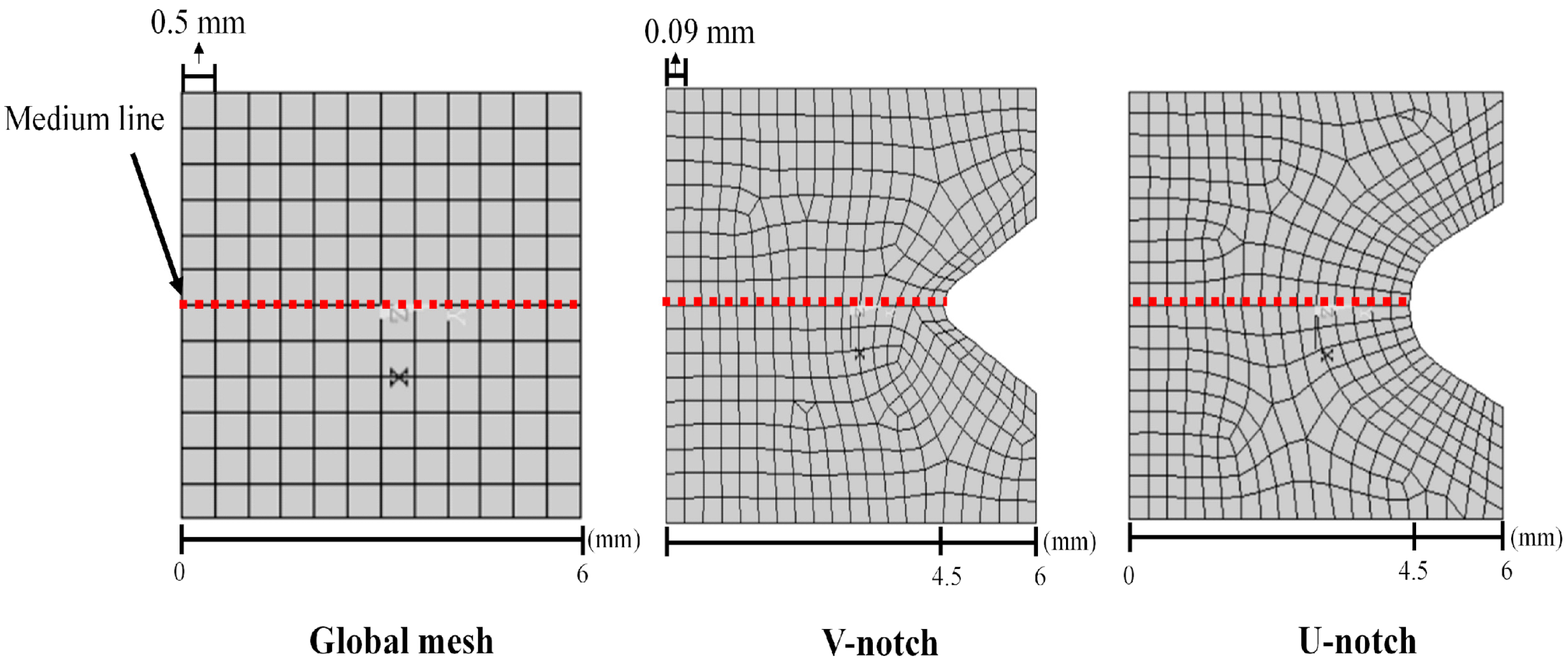





| Specimens’ Group | Depth (mm) |
|---|---|
| Unnotched | --- |
| V-notch | 0.2 |
| 0.6 | |
| 1.0 | |
| 1.5 | |
| U-notch | 0.2 |
| 0.6 | |
| 1.0 | |
| 1.5 |
| Properties | Values (Unity) |
|---|---|
| Density | 1.78 × 105 kg/m3 |
| Poisson’s ratio | 0.43 |
| Elastic modulus | 1280.15 MPa |
| Specimens’ Group | Depth (mm) | Number of Elements | Number of Nodes |
|---|---|---|---|
| V-notch | 0.2 | 8622 | 29,161 |
| 0.6 | 9087 | 27,550 | |
| 1.0 | 9047 | 27,510 | |
| 1.5 | 9003 | 27,288 | |
| U-notch | 0.2 | 9450 | 28,794 |
| 0.6 | 9017 | 23,760 | |
| 1.0 | 8847 | 26,860 | |
| 1.5 | 8414 | 25,547 |
| Specimens’ Group | Depth (mm) | Maximum Load (MPa) | Maximum Displacement (mm) |
|---|---|---|---|
| Unnotched | 1393.0 ± 31.4 | 8.0 ± 0.2 | |
| V-notch | 0.2 | 1391.4 ± 5.7 | 8.4 ± 0.2 |
| 0.6 | 1305.4 ± 30.6 | 6.1 ± 0.4 | |
| 1.0 | 1197.3 ± 6.9 | 4.5 ± 0.2 | |
| 1.5 | 1042.1 ± 17.2 | 3.3 ± 0.1 | |
| U-notch | 0.2 | 1447.0 ± 19.5 | 8.3 ± 0.3 |
| 0.6 | 1354.0 ± 19.4 | 7.2 ± 0.3 | |
| 1.0 | 1263.4 ± 23.4 | 5.6 ± 0.1 | |
| 1.5 | 1184.1 ± 21.5 | 4.7 ± 0.2 |
| Specimens’ Group | Depth (mm) | Load (N) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Point (A) | Point (B) | Point (C) | Point (D) | ||||||
| Exp. | FEA | Exp. | FEA | Exp. | FEA | Exp. | FEA | ||
| V-notch | 0.2 | 330.1 | 279.2 | 1027.8 | 1124.5 | 1240.3 | 1305.4 | 1271.4 | 1322.1 |
| 0.6 | 380.9 | 395.8 | 760.6 | 881.3 | 986.4 | 1104.2 | 1187.2 | 1222.1 | |
| 1.0 | 231.7 | 221.7 | 500.2 | 551.5 | 708.7 | 800.5 | 1042.6 | 1087.8 | |
| 1.5 | 207.2 | 169.8 | 609.4 | 556.2 | 760.1 | 732.4 | 832.4 | 818.5 | |
| U-notch | 0.2 | 493.6 | 540.9 | 923.0 | 1064.6 | 1218.3 | 1276.5 | 1377.2 | 1325.5 |
| 0.6 | 427.7 | 433.3 | 817.3 | 937.2 | 1069.4 | 1170.2 | 1248.3 | 1225.4 | |
| 1.0 | 399.6 | 396.2 | 799.9 | 794.5 | 1023.7 | 1022.9 | 1173.8 | 1123.6 | |
| 1.5 | 361.9 | 340.1 | 667.9 | 675.0 | 888.3 | 891.0 | 966.0 | 940.7 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pereira, I.C.S.; de Sousa, J.R.M.; Costa, C.A. Evaluation of U-Notch and V-Notch Geometries on the Mechanical Behavior of PVDF: The DIC Technique and FEA Approach. Polymers 2024, 16, 2906. https://doi.org/10.3390/polym16202906
Pereira ICS, de Sousa JRM, Costa CA. Evaluation of U-Notch and V-Notch Geometries on the Mechanical Behavior of PVDF: The DIC Technique and FEA Approach. Polymers. 2024; 16(20):2906. https://doi.org/10.3390/polym16202906
Chicago/Turabian StylePereira, Ingrid C. S., José Renato M. de Sousa, and Celio A. Costa. 2024. "Evaluation of U-Notch and V-Notch Geometries on the Mechanical Behavior of PVDF: The DIC Technique and FEA Approach" Polymers 16, no. 20: 2906. https://doi.org/10.3390/polym16202906
APA StylePereira, I. C. S., de Sousa, J. R. M., & Costa, C. A. (2024). Evaluation of U-Notch and V-Notch Geometries on the Mechanical Behavior of PVDF: The DIC Technique and FEA Approach. Polymers, 16(20), 2906. https://doi.org/10.3390/polym16202906





