Quantitative Study on Reinforcing Mechanism of Nanofiller Network in Silicone Elastomer Based on Fluorescence Labeling Technology
Abstract
1. Introduction
2. Experimental Section
2.1. Sample Preparation
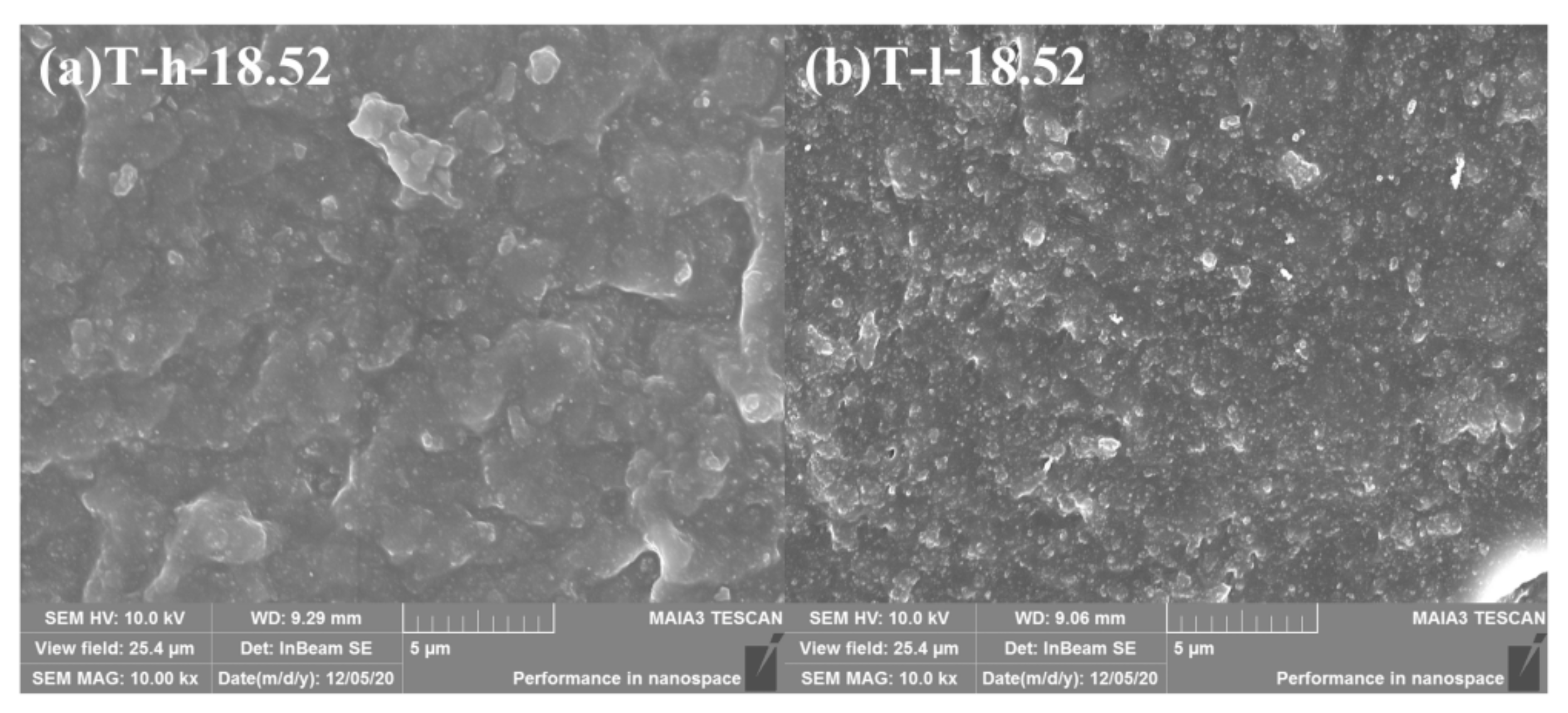
2.2. Field Emission Scanning Electron Microscopy
2.3. Laser Particle Analysis
2.4. Laser Scanning Confocal Microscope
2.5. Measurement of Dynamic Mechanical Properties
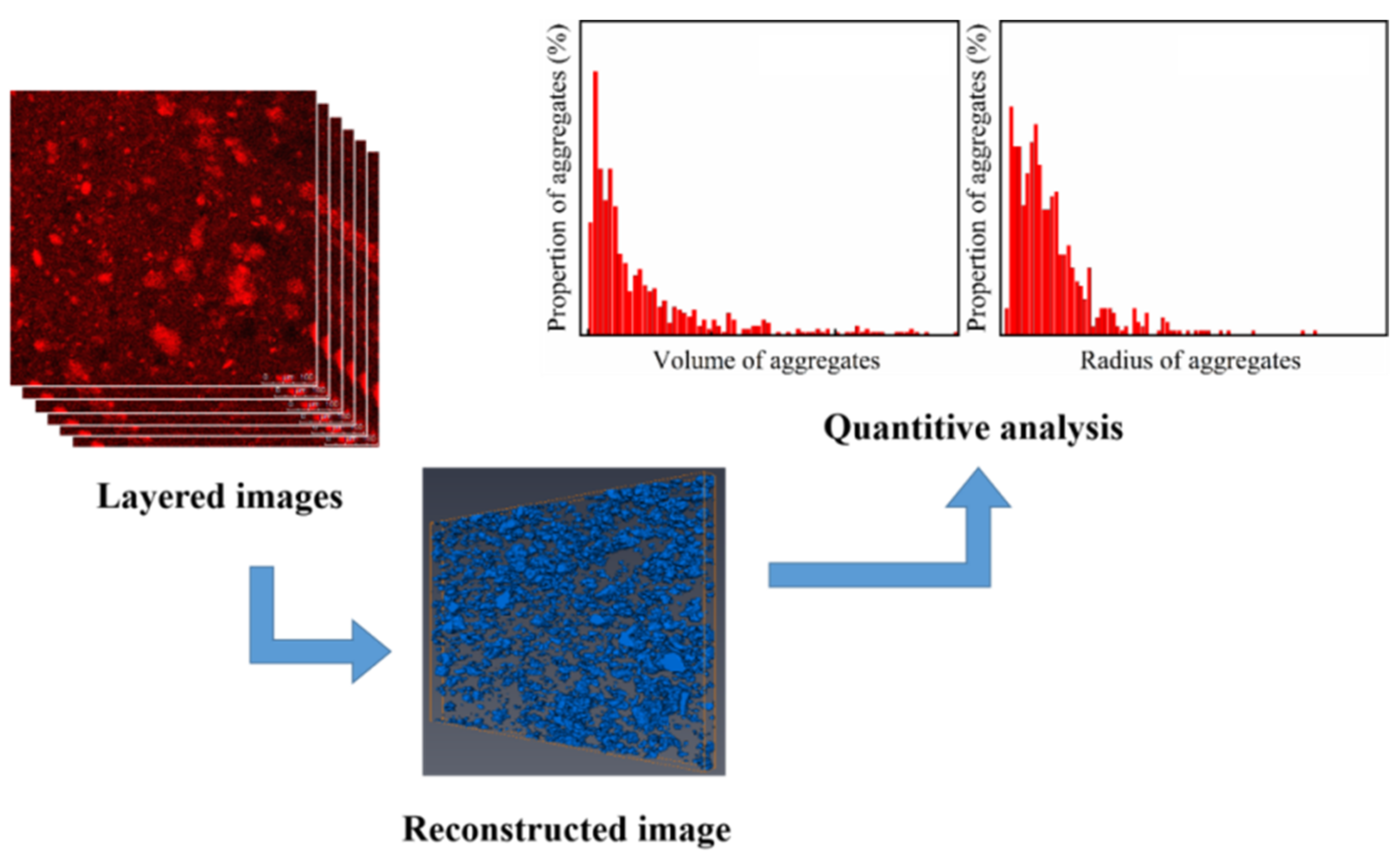
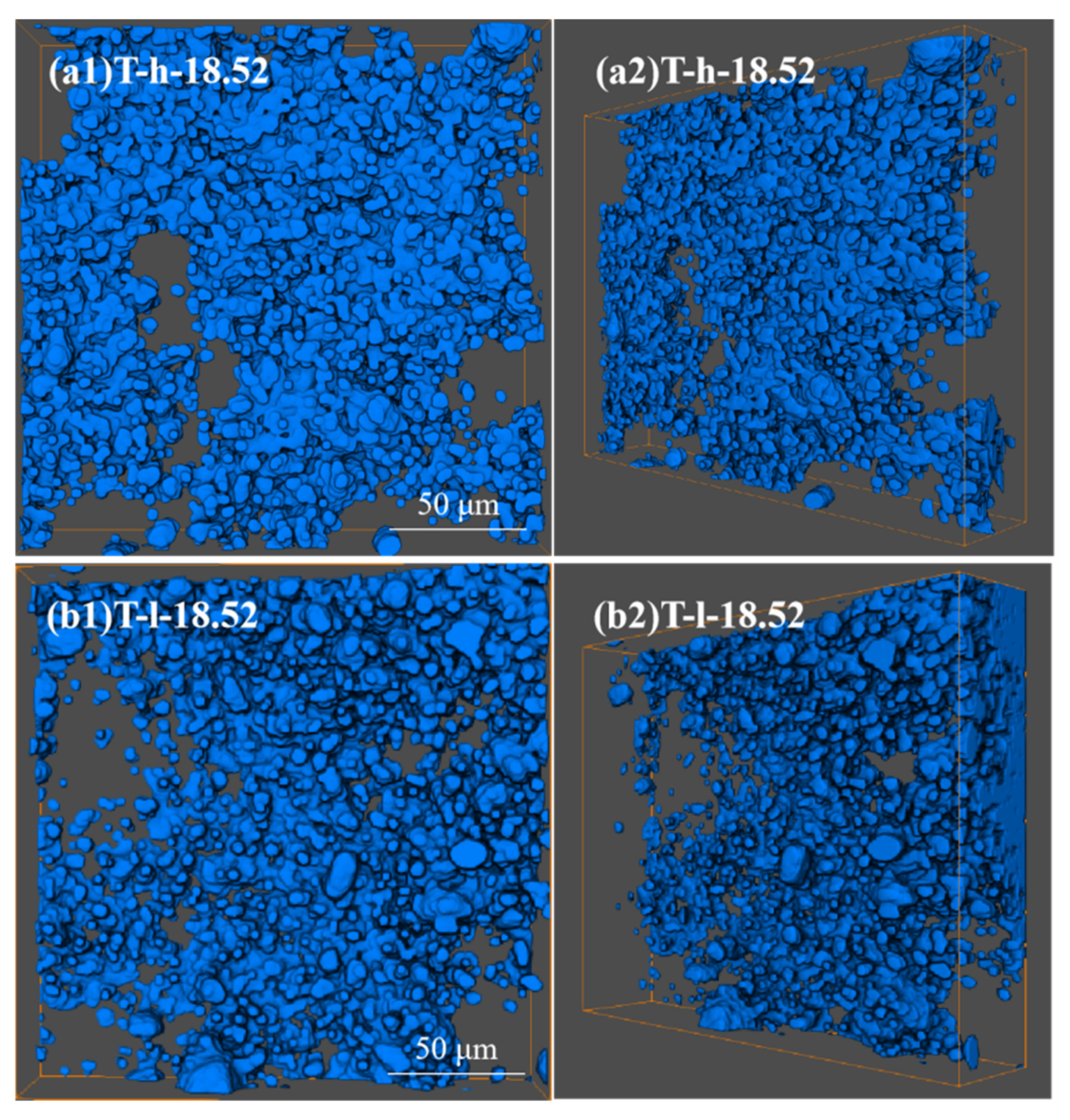
2.6. 3D Visualization and Quantitative Analysis
3. Results
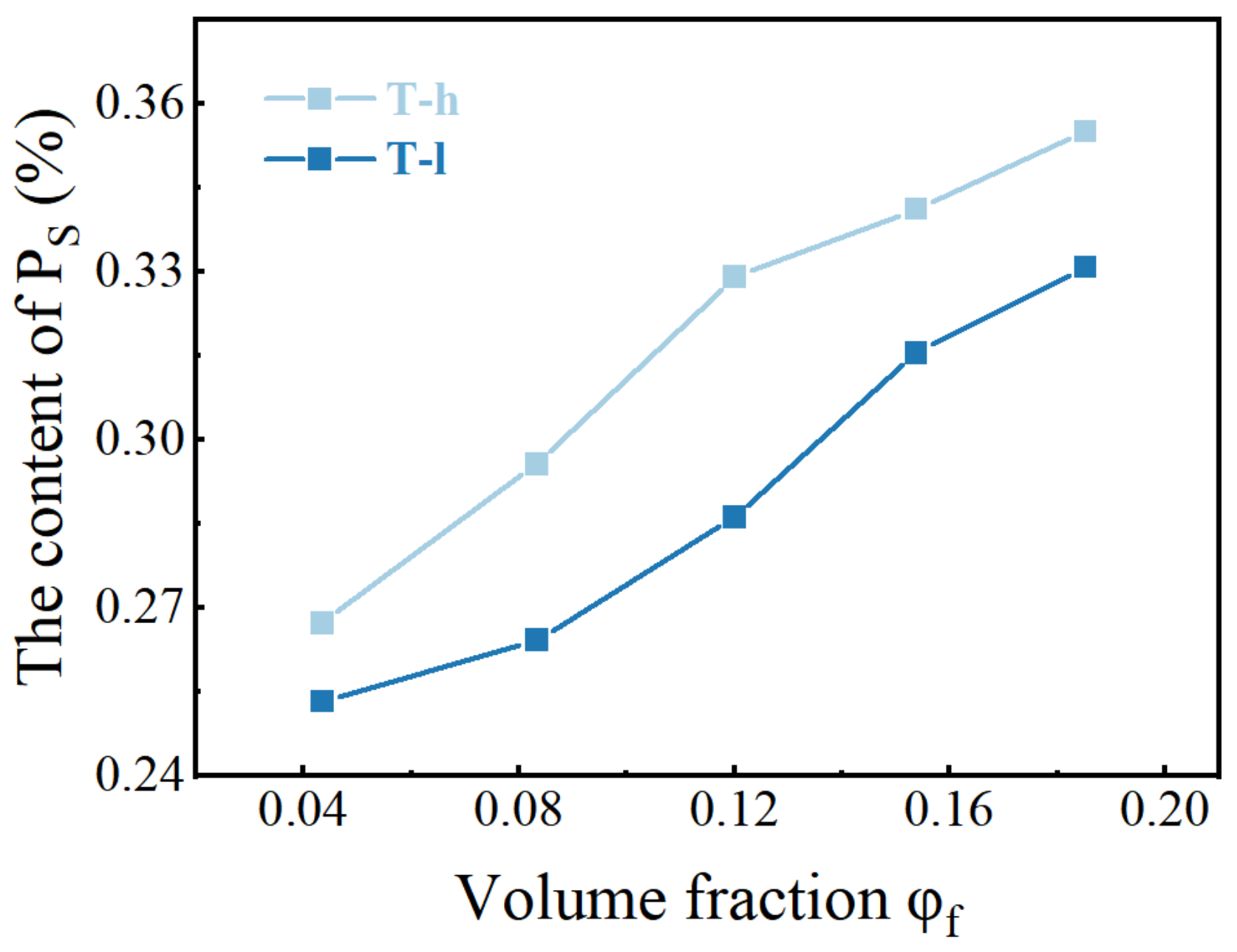
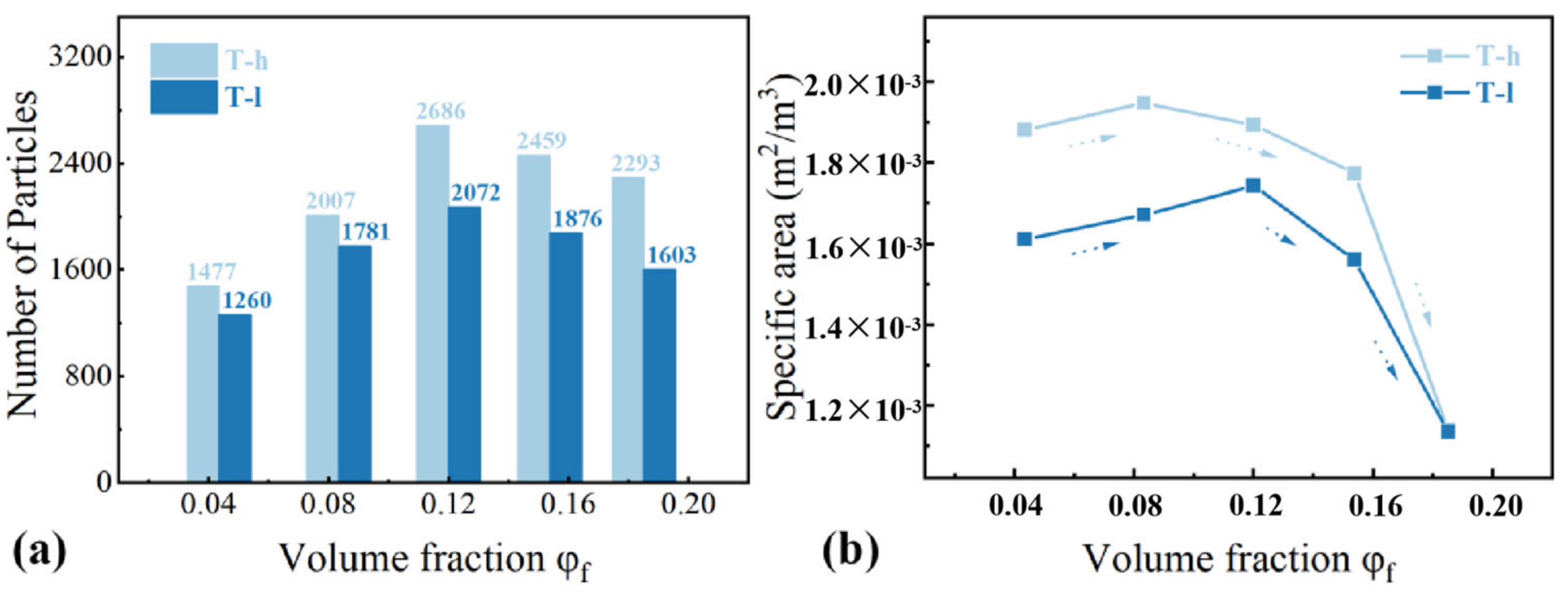
3.1. Visualization Study on the Three-Dimensional Dispersion Structure of Silica Network
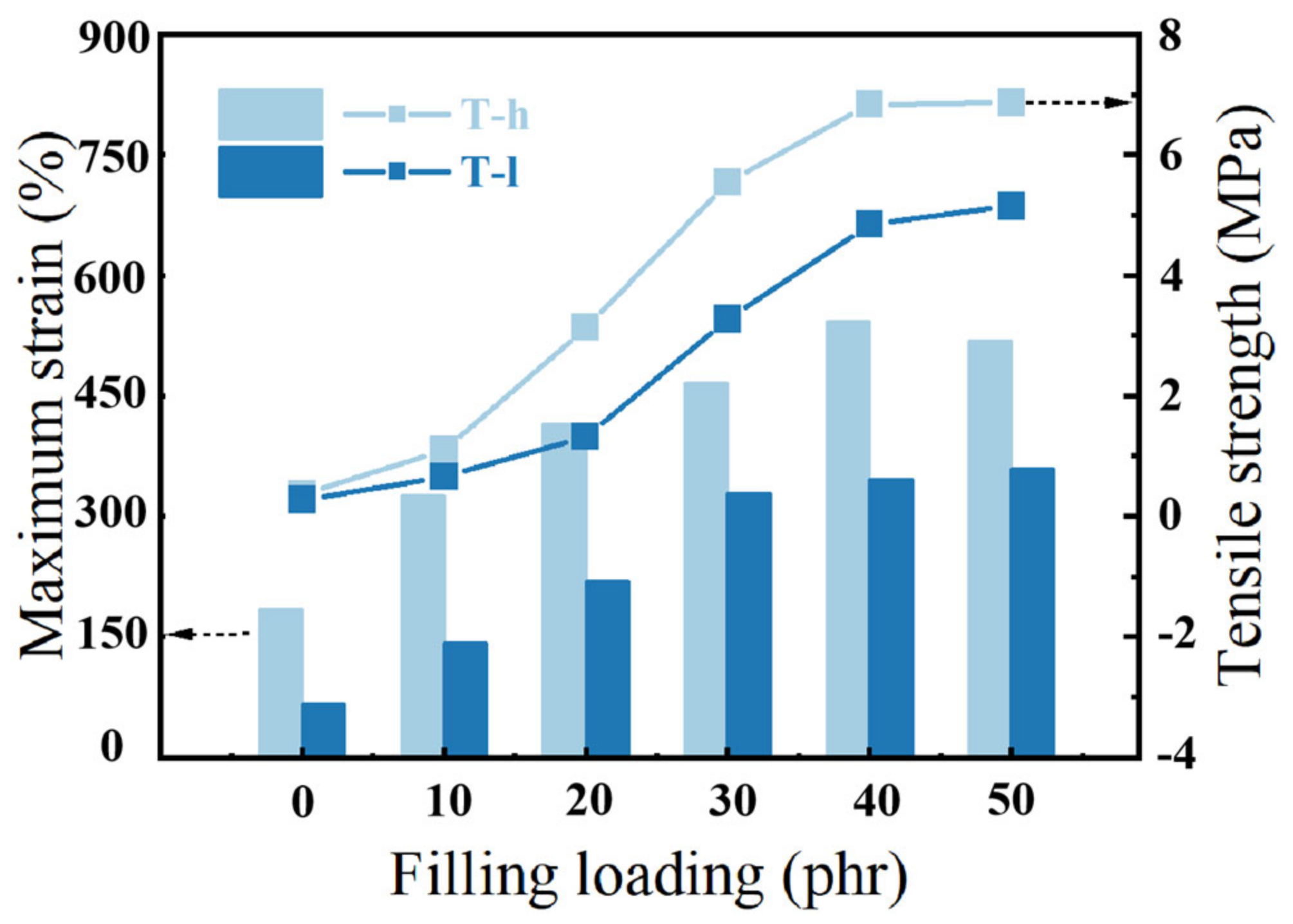
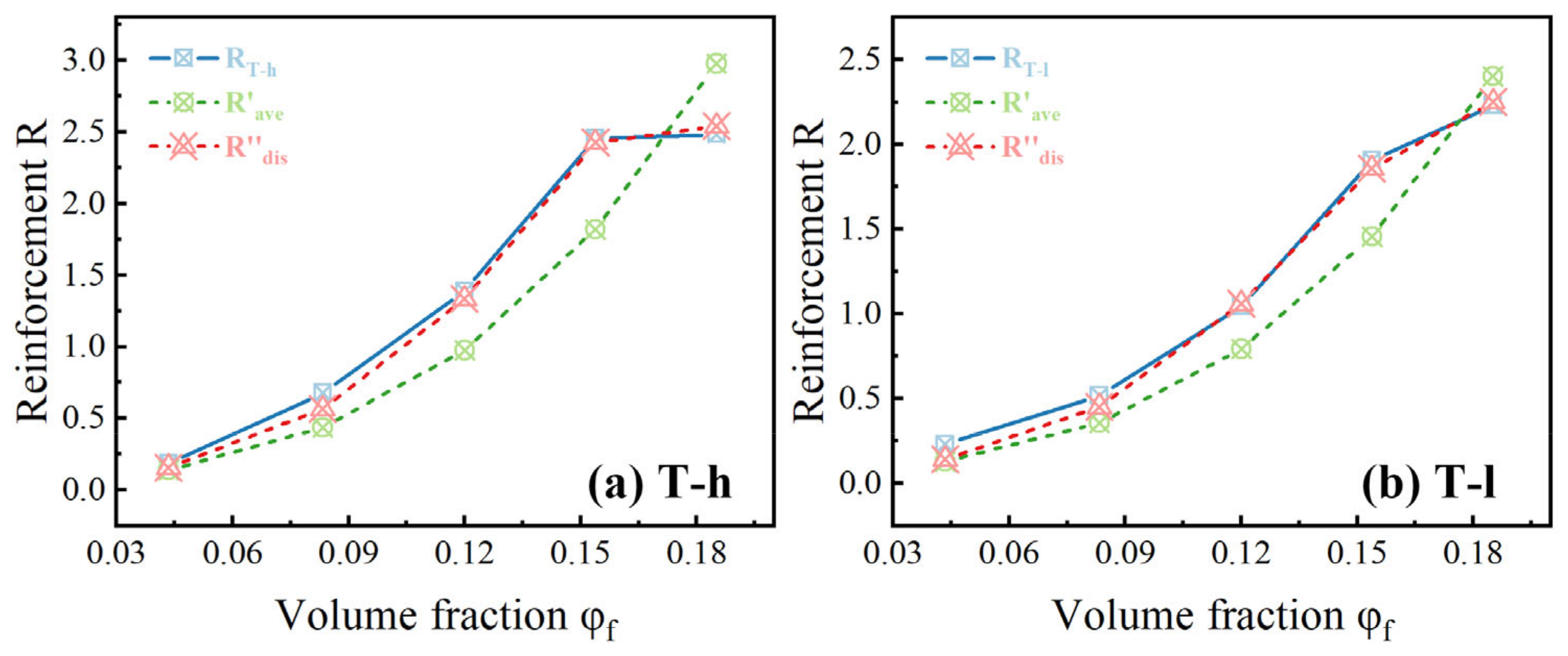
3.2. Discussion of Reinforcement Models
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Suzuki, N.; Zakaria, M.B.; Chiang, Y.D.; Wu, C.W.; Yamauchi, Y. Thermally stable polymer composites with improved transparency by using colloidal mesoporous silica nanoparticles as inorganic fillers. Phys. Chem. Chem. Phys. 2012, 14, 7427–7432. [Google Scholar] [CrossRef] [PubMed]
- Shang, S.; Gan, L.; Yuen, M.C.; Jiang, S.X.; Luo, N.M. Carbon nanotubes based high temperature vulcanized silicone rubber nanocomposite with excellent elasticity and electrical properties. Compos. Part A 2014, 66, 135–141. [Google Scholar] [CrossRef]
- Bouty, A.; Petitjean, L.; Degrandcourt, C.; Gummel, J.; Kwaśniewski, P.; Meneau, F.; Boué, F.; Couty, M.; Jestin, J. Nanofiller Structure and Reinforcement in Model Silica/Rubber Composites: A Quantitative Correlation Driven by Interfacial Agents. Macromolecules 2017, 47, 5365–5378. [Google Scholar] [CrossRef]
- Mao, L.; Han, J.; Zhao, D.; Song, N.; Shi, L.; Wang, J. Particle Packing Theory Guided Thermal Conductive Polymer Preparation and Related Properties. ACS Appl. Mater. Interfaces 2018, 10, 33556–33563. [Google Scholar] [CrossRef]
- Park, J.J.; Lee, J.Y.; Hong, Y.G. Effects of vinylsilane-modified nanosilica particles on electrical and mechanical properties of silicone rubber nanocomposites. Polymer 2020, 197, 122493. [Google Scholar] [CrossRef]
- Jean, L.L. Rubber-filler interactions and rheological properties in filled compounds. Prog. Polym. Sci. 2002, 27, 627–687. [Google Scholar] [CrossRef]
- Meier, J.G.; Klüppel, M. Carbon Black Networking in Elastomers Monitored by Dynamic Mechanical and Dielectric Spectroscopy. Macromol. Mater. Eng. 2008, 293, 12–38. [Google Scholar] [CrossRef]
- Diaz, R.; Diani, J.; Gilormini, P. Physical interpretation of the Mullins softening in a carbon-black filled SBR. Polymer 2014, 55, 4942–4947. [Google Scholar] [CrossRef]
- Goudarzi, T.; Spring, D.W.; Paulino, G.H.; Lopez-Pamies, O. Filled elastomers: A theory of filler reinforcement based on hydrodynamic and interphasial effects. J. Mech. Phys. Solids 2015, 80, 37–67. [Google Scholar] [CrossRef]
- Rishi, K.; Beaucage, G.; Kuppa, V.K.; Mcglasson, A.; Ilavsky, J. Impact of an Emergent Hierarchical Filler Network on Nanocomposite Dynamics. Macromolecules 2018, 51, 7893–7904. [Google Scholar] [CrossRef]
- Sarath, P.S.; Samson, S.V.; Reghunath, R.; Pandey, M.K.; George, S.C. Fabrication of exfoliated graphite reinforced silicone rubber composites—Mechanical, tribological and dielectric properties. Polym. Test 2020, 89, 106601. [Google Scholar] [CrossRef]
- Song, B.; He, W.; Wang, X.; Zeng, X.; Cheng, M.; Wu, F.; Moon, K.-s.; Wong, C.P. Fabrication of stretchable and conductive polymer nanocomposites based on interconnected graphene aeroge. Compos. Sci. Technol. 2020, 200, 108430. [Google Scholar] [CrossRef]
- Wei, Q.; Yang, D.; Yu, L.; Ni, Y.; Zhang, L. Fabrication of carboxyl nitrile butadiene rubber composites with high dielectric constant and thermal conductivity using Al2O3@PCPA@GO hybrids. Compos. Sci. Technol. 2020, 199, 108344. [Google Scholar] [CrossRef]
- Zare, Y.; Rhee, K.Y. Significances of interphase conductivity and tunneling resistance on the conductivity of carbon nanotubes nanocomposites. Polym. Compos. 2020, 41, 748–756. [Google Scholar] [CrossRef]
- Li, X.; Yang, Q.; Ye, Y.; Zhang, L.; Hong, S.; Ning, N.; Tian, M. Quantifying 3D-nanosized dispersion of SiO2 in elastomer nanocomposites by 3D-scanning transmission electron microscope (STEM). Compos. Part A Appl. Sci. Manuf. 2020, 131, 105778. [Google Scholar] [CrossRef]
- Smallwood, H.M. Limiting Law of the Reinforcement of Rubber. J. Appl. Phys. 1944, 15, 758–766. [Google Scholar] [CrossRef]
- Flory, P.; Rehner, J. Statistical mechanics of cross-linked polymer networks I: Rubberlike elasticity. J. Chem. Phys. 1943, 11, 512–520. [Google Scholar] [CrossRef]
- Christensen, R.M.; Lo, K.H. Solutions for effective shear properties in three phase sphere and cylinder models. J. Mech. Phys. Solids 1979, 27, 315–330. [Google Scholar] [CrossRef]
- Guth, E. Theory of Filler Reinforcement. Rubber Chem. Technol. 1945, 18, 596–604. [Google Scholar] [CrossRef]
- Mittal, V. Modeling of tensile modulus of polyolefin-layered silicate nanocomposites: Modified micro-mechanical and statistical methods. J. Polym. Eng. 2012, 32, 519–529. [Google Scholar] [CrossRef]
- Ouyang, G.B. Network junction model for mechanical properties of filled vulcanizates-modulus, hysteresis and the Payne effect. In Constitutive Models for Rubber III; Routledge: London, UK, 2003; pp. 325–332. [Google Scholar]
- Liu, Z.F.; Li, L.F. Viscoelastic behaviors of porous silicone rubbers under finite deformation. Acta Mech. Solida Sin. 2002, 23, 347–353. [Google Scholar]
- Mermet-Guyennet, M.R.B.; de Castro, J.G.; Varol, H.S.; Habibi, M.; Hosseinkhani, B.; Martzel, N.; Sprik, R.; Denn, M.; Zaccone, A.; Parekh, S.; et al. Size-dependent reinforcement of composite rubbers. Polymer 2015, 73, 170–173. [Google Scholar] [CrossRef]
- Pires, N.M.T.; Ferreira, A.A.; Lira, C.H.d.; Coutinho, P.L.A.; Nicolini, L.F.; Soares, B.G.; Coutinho, F.M.B. Performance evaluation of high-cis 1,4-polybutadienes. J. Appl. Polym. Sci. 2005, 99, 88–99. [Google Scholar] [CrossRef]
- Herd, C.R.; McDonald, G.C.; Hess, W.M. Morphology of Carbon-Black Aggregates: Fractal Versus Euclidean Geometry. Rubber Chem. Technol. 1992, 65, 107–129. [Google Scholar] [CrossRef]
- Jiang, N.; Endoh, M.K.; Koga, T.; Masui, T.; Kishimoto, H.; Nagao, M.; Satija, S.K.; Taniguchi, T. Nanostructures and Dynamics of Macromolecules Bound to Attractive Filler Surfaces. ACS Macro Lett. 2015, 8, 838–842. [Google Scholar] [CrossRef]
- Mujtaba, A.; Keller, M.; Ilisch, S.; Radusch, H.-J.; Thurn-Albrecht, T.; Saalwächter, K.; Beiner, M. Mechanical Properties and Cross-Link Density of Styrene–Butadiene Model Composites Containing Fillers with Bimodal Particle Size Distribution. Macromoleculars 2012, 45, 6504–6515. [Google Scholar] [CrossRef]
- Zhou, W.; Chen, L.; Lu, J.; Qi, Z.; Huang, N.; Li, L.; Huang, W. Imaging the strain induced carbon black filler network structure breakage with nano X-ray tomography. RSC Adv. 2014, 4, 54500–54505. [Google Scholar] [CrossRef]
- Song, L.; Wang, Z.; Tang, X.; Chen, L.; Chen, P.; Yuan, Q.; Li, L. Visualizing the toughening mechanism of nanofiller with 3D X-ray Nano-CT: Stress-induced phase separation of silica nanofiller and silicone polymer double networks. Macromolecules 2017, 50, 7249–7257. [Google Scholar] [CrossRef]
- Xiong, Y.; Shen, S.; Kang, M.; Wang, Z.; Lu, A. Effect of fluorescence labeling on mechanical properties of silica filled silicone rubber. Polymer 2020, 208, 122904. [Google Scholar] [CrossRef]
- Zeng, S.; Kang, M.; Chen, K.; Sun, R.; Lu, A.; Chang, G. Visual research filler network structure in polymer composites and its structure-activity relationship by fluorescent labeling and LSCM. Polym. Test 2020, 90, 106749. [Google Scholar] [CrossRef]
- Zaccardi, F.; Santonicola, M.G.; Laurenzi, S. Quantitative assessment of nanofiller dispersion based on grayscale image analysis: A case study on epoxy/ carbon nanocomposites. Compos. Part A Appl. Sci. Manuf. 2018, 115, 302–310. [Google Scholar] [CrossRef]
- Khare, H.S.; Burris, D.L. A quantitative method for measuring nanocomposite dispersion. Polymer 2010, 51, 719–729. [Google Scholar] [CrossRef]
- Zhao, D.; Ge, S.; Senses, E.; Akcora, P.; Jestin, J.; Kumar, S.K. Role of Filler Shape and Connectivity on the Viscoelastic Behavior in Polymer Nanocomposites. Macromoleculars 2015, 48, 5433–5438. [Google Scholar] [CrossRef]
- Davris, T.; Mermet-Guyennet, M.R.B.; Bonn, D.; Lyulin, A.V. Filler Size Effects on Reinforcement in Elastomer-Based Nanocomposites: Experimental and Simulational Insights into Physical Mechanisms. Macromolecules 2016, 49, 7077–7087. [Google Scholar] [CrossRef]



| Label | Sulfur (phr) | Fluorescent Silica T36-5 (phr) | (Vol %) | |
|---|---|---|---|---|
| Unfilled high-phenyl PVMQ ([2ph] = 25%) | 0-high | 1.5 | 0 | 0 |
| Unfilled low-phenyl PVMQ ([2ph] = 9.51%) | 0-low | 1.5 | 0 | 0 |
| High-phenyl PVMQ filled with T36-5 | T-h-4.35 | 1.5 | 10 | 4.35 |
| T-h-8.33 | 1.5 | 20 | 8.33 | |
| T-h-12.00 | 1.5 | 30 | 12.00 | |
| T-h-15.38 | 1.5 | 40 | 15.38 | |
| T-h-18.52 | 1.5 | 50 | 18.52 | |
| Low-phenyl PVMQ filled with T36-5 | T-l-4.35 | 1.5 | 10 | 4.35 |
| T-l-8.33 | 1.5 | 20 | 8.33 | |
| T-l-12.00 | 1.5 | 30 | 12.00 | |
| T-l-15.38 | 1.5 | 40 | 15.38 | |
| T-l-18.52 | 1.5 | 50 | 18.52 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Xiong, Y.; Kang, M.; Yu, F.; Lu, A. Quantitative Study on Reinforcing Mechanism of Nanofiller Network in Silicone Elastomer Based on Fluorescence Labeling Technology. Polymers 2024, 16, 2829. https://doi.org/10.3390/polym16192829
Li Y, Xiong Y, Kang M, Yu F, Lu A. Quantitative Study on Reinforcing Mechanism of Nanofiller Network in Silicone Elastomer Based on Fluorescence Labeling Technology. Polymers. 2024; 16(19):2829. https://doi.org/10.3390/polym16192829
Chicago/Turabian StyleLi, Yuquan, Yuqi Xiong, Ming Kang, Fengmei Yu, and Ai Lu. 2024. "Quantitative Study on Reinforcing Mechanism of Nanofiller Network in Silicone Elastomer Based on Fluorescence Labeling Technology" Polymers 16, no. 19: 2829. https://doi.org/10.3390/polym16192829
APA StyleLi, Y., Xiong, Y., Kang, M., Yu, F., & Lu, A. (2024). Quantitative Study on Reinforcing Mechanism of Nanofiller Network in Silicone Elastomer Based on Fluorescence Labeling Technology. Polymers, 16(19), 2829. https://doi.org/10.3390/polym16192829






