Research on the Mechanical and Thermal Properties of Carbon-Fiber-Reinforced Rubber Based on a Finite Element Simulation
Abstract
1. Introduction
2. Simplified Micromechanical Model of Carbon-Fiber-Reinforced Rubber
3. Theoretical ROM Models for Carbon-Fiber-Reinforced Rubber
3.1. Young’s Modulus
3.2. Shear Modulus
3.3. Thermal Expansion Coefficient
3.4. Poisson’s Ratio
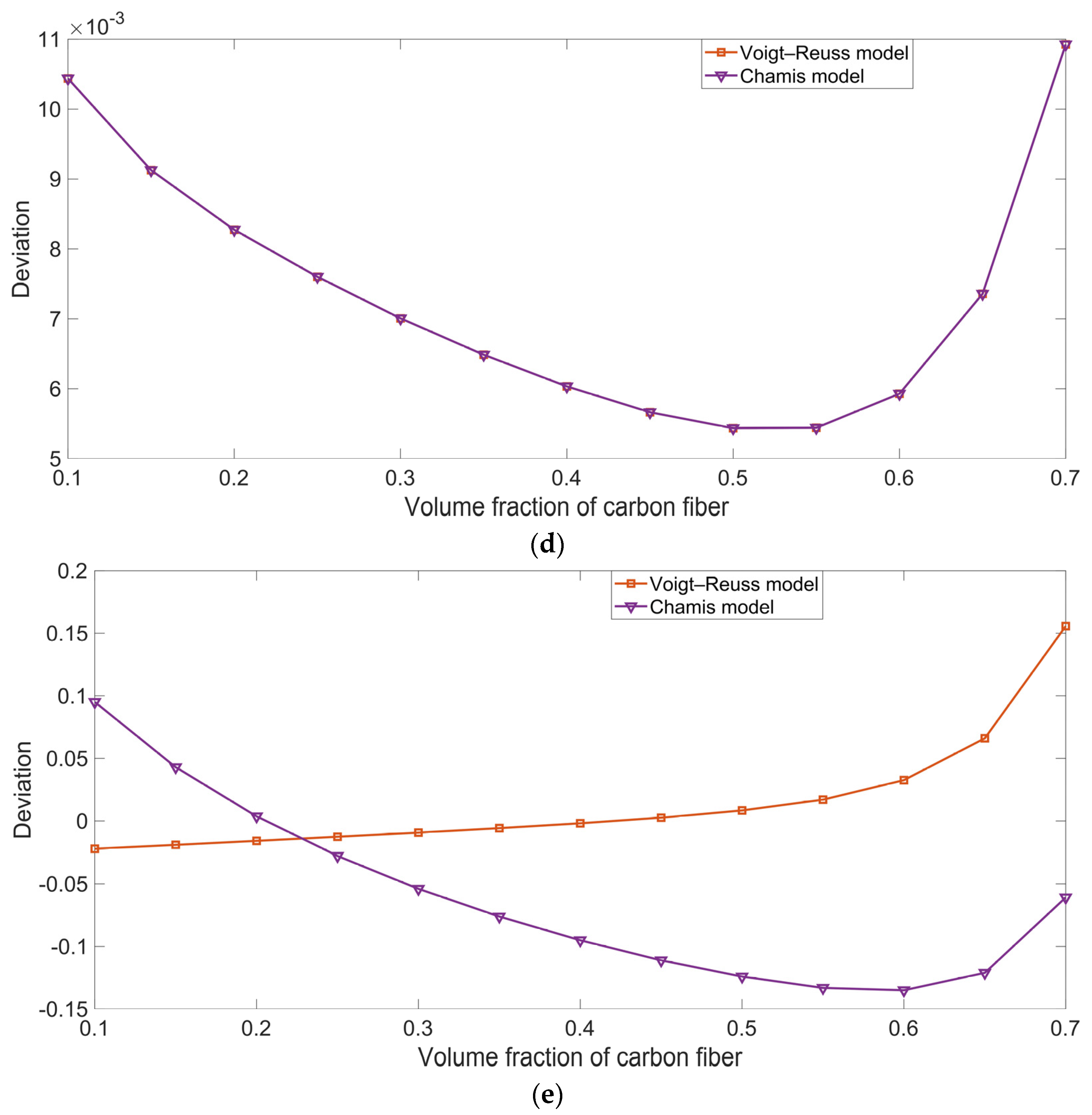
4. Results and Discussion
4.1. Longitudinal Young’s Modulus Exx
4.2. Transversal Young’s Modulus Eyy
4.3. In-Plane Shear Modulus Gxy
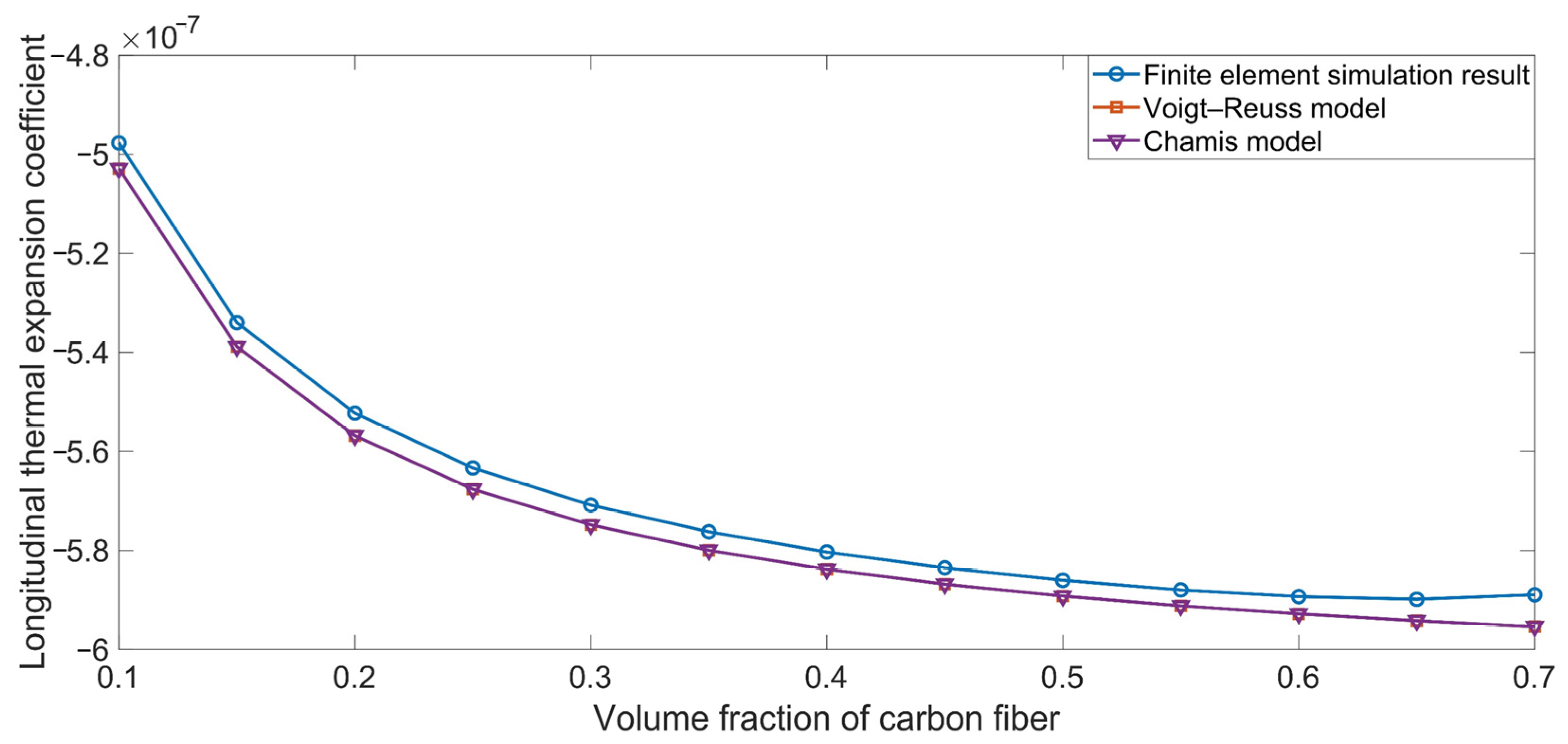
4.4. Longitudinal Thermal Expansion Coefficient αxx
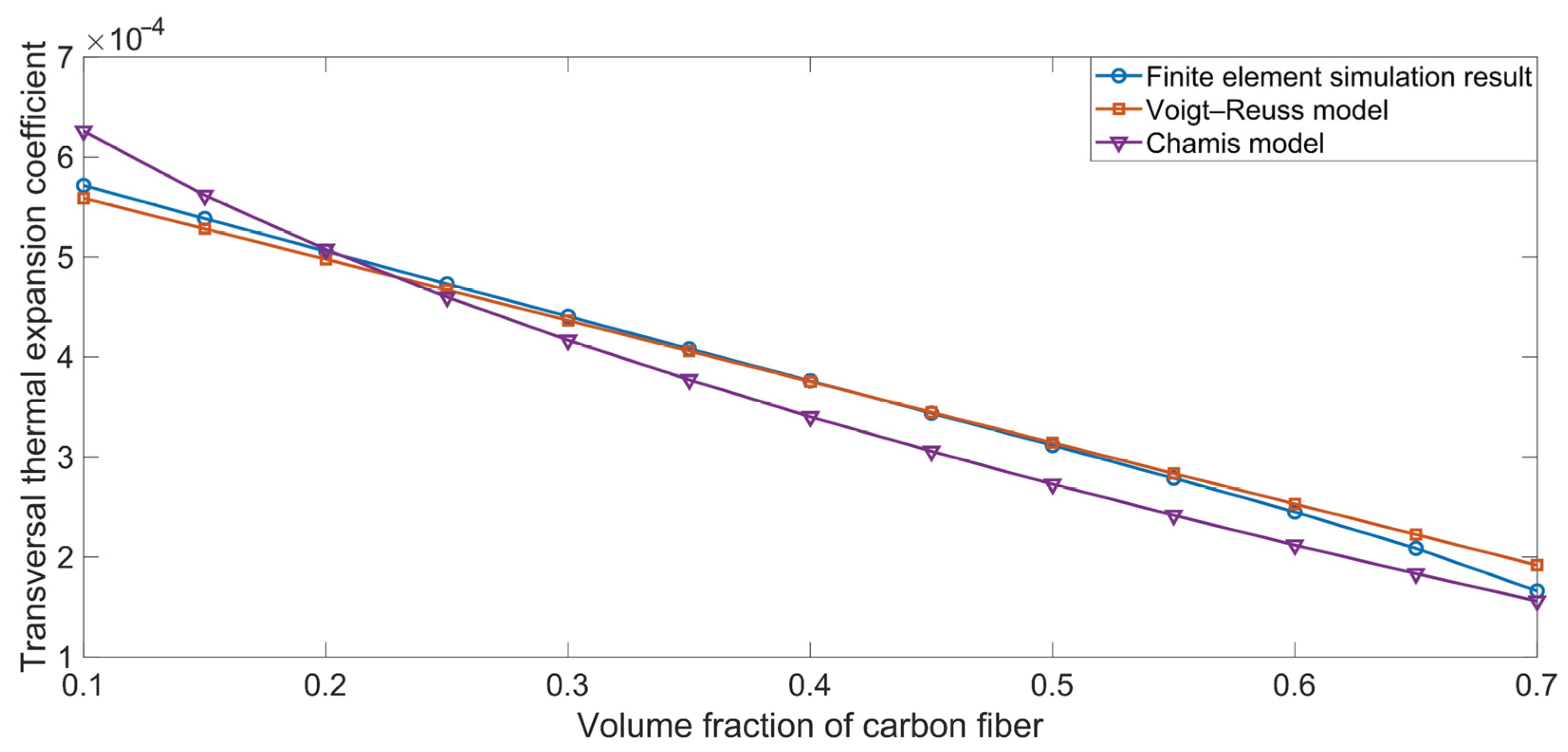
4.5. Transversal Thermal Expansion Coefficient αyy
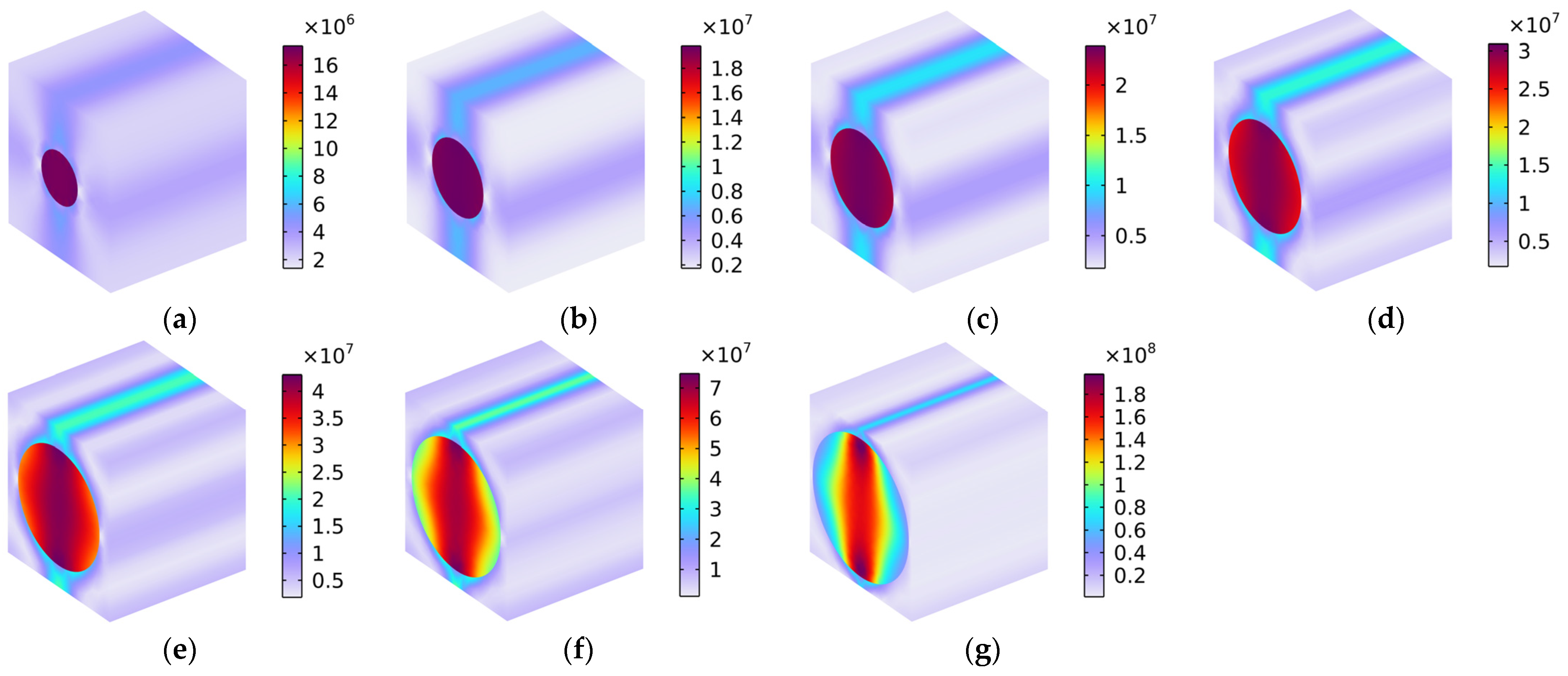
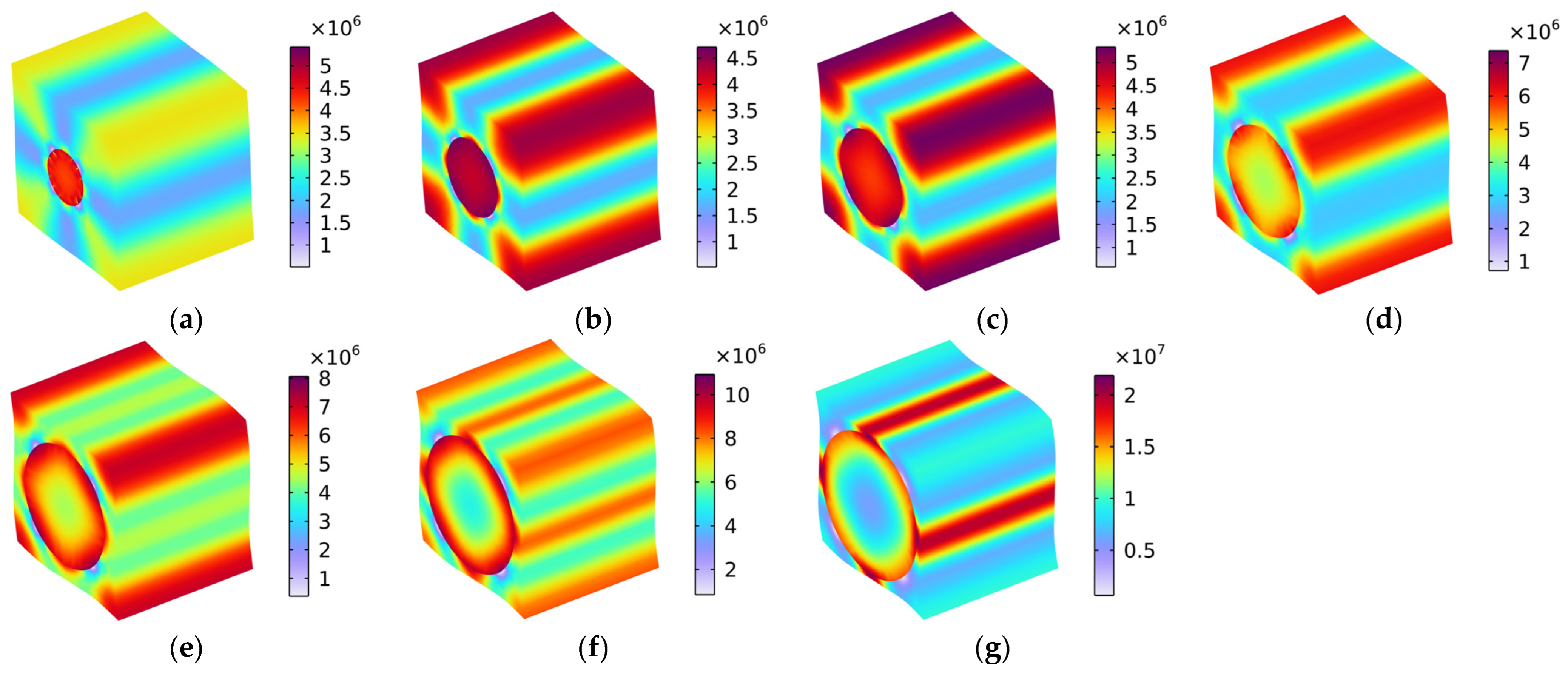
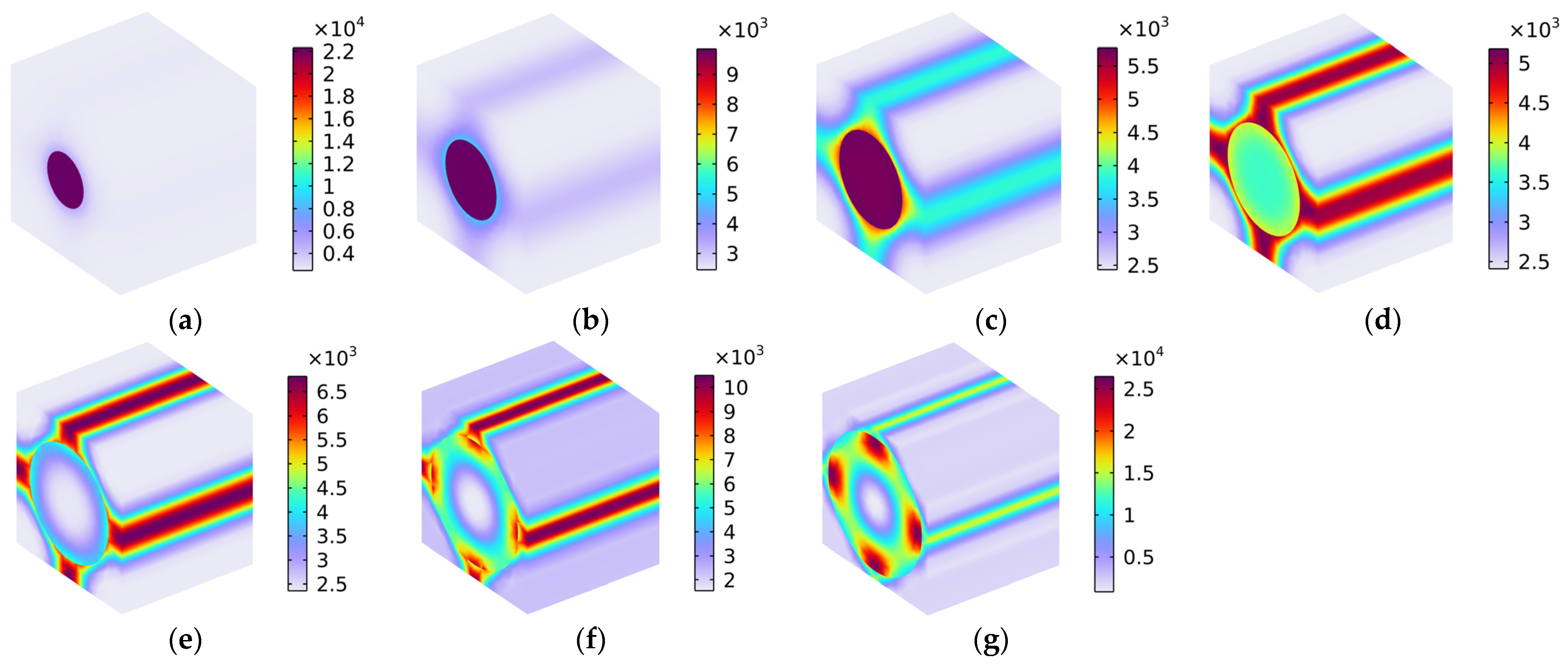
5. Mechanism Analysis
5.1. Mechanical Properties
5.1.1. Load 11
5.1.2. Load 22
5.1.3. Load 33
5.1.4. Load 12
5.1.5. Load 23
5.1.6. Load 13
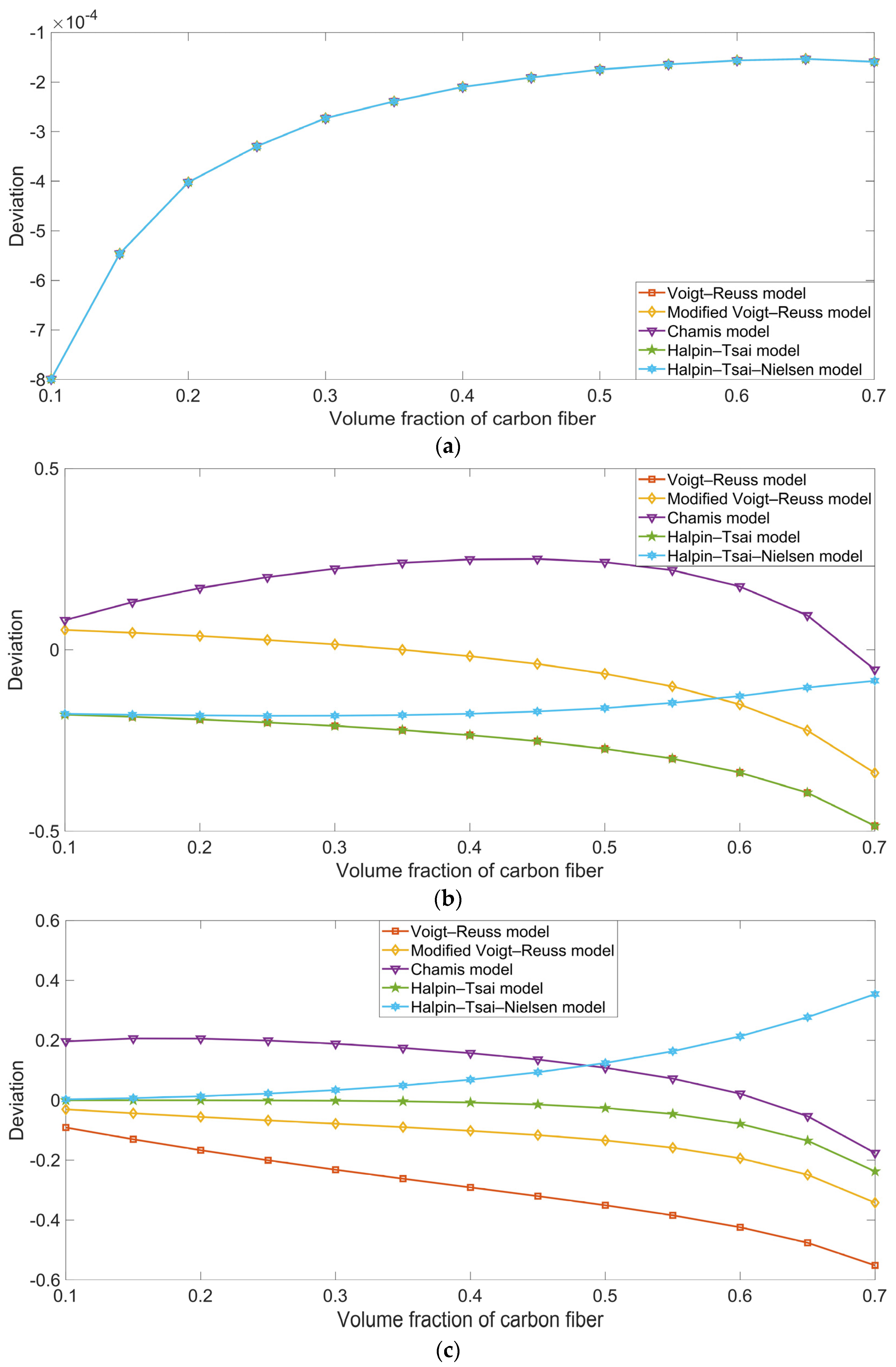
5.2. Thermal Properties
6. Conclusions
- (1)
- According to the mutual verification of the finite element simulation results obtained using a simplified micromechanical model and the theoretical data presented by the various ROM models, we provided a simple and effective method for investigating the properties of carbon-fiber-reinforced rubber. Compared to complex and time-consuming experimental tests, the finite element simulation method not only reduced the experimental cost but could also provide a visual representation of the influencing mechanism.
- (2)
- The finite element simulation results indicate that, as the fiber volume fraction Vfc increased from 10% to 70%, the mechanical properties of the longitudinal Young’s modulus Exx, the transversal Young’s modulus Eyy, and the in-plane shear modulus Gxy changed from 2.31 × 1010 Pa to 16.09 × 1010 Pa, from 0.54 × 107 Pa to 2.59 × 107 Pa, and from 1.66 × 106 Pa to 10.11 × 106 Pa, respectively. The reinforcing effect of the carbon fiber mainly worked in the x-axis direction because the fiber was installed in this direction. Meanwhile, the reinforcing effect gradually improved as the fiber volume fraction Vfc increased. Therefore, the carbon fiber can be installed along the desired direction, and the multidirectional reinforcement of the rubber matrix can be obtained using two-dimensional or three-dimensional braided carbon fibers.
- (3)
- Similarly, the finite element simulation results suggest that, as the fiber volume fraction Vfc increases from 10% to 70%, the longitudinal thermal expansion coefficient αxx and transversal thermal expansion coefficient αyy changed from −4.98 × 10−7 K−1 to −5.89 × 10−7 K−1 and from 5.72 × 10−4 K−1 to 1.66 × 10−4 K−1, respectively. Moreover, αxx had a negative value, while αyy had a positive value, and both of them decreased as the fiber volume fraction Vfc increased. Thus, the desired thermal properties for the composite material could be obtained by selecting the carbon fiber with the appropriate dimensions and installing it in the right direction.
- (4)
- Using finite element simulation, we determined the influencing mechanism of the volume fraction of carbon fiber Vfc to the distribution of Von Mises stress for the carbon-fiber-reinforced rubber; the resultant picture of stress concentration may provide guidance for subsequent research. The interface between the carbon fiber and the rubber matrix was the weak spot, and the binding force should be improved to further enhance the overall performance of the composite material.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Serial Number | Abbreviations | Full Title | Location of First Appearance |
|---|---|---|---|
| 1 | mDE | Acid-modified diatomaceous earth | Paragraph 1, Section “1. Introduction” |
| 2 | CNTs | Carbon nanotubes | Paragraph 1, Section “1. Introduction” |
| 3 | HTT | Hydrothermally treated | Paragraph 1, Section “1. Introduction” |
| 4 | RUC | Repeatable unit cell | Paragraph 5, Section “1. Introduction” |
| 5 | ROM | Rule of mixture | Paragraph 5, Section “1. Introduction” |
References
- Kazmi, S.M.S.; Munir, M.J.; Wu, Y.-F. Application of waste tire rubber and recycled aggregates in concrete products: A new compression casting approach. Resour. Conserv. Recycl. 2021, 167, 105353. [Google Scholar] [CrossRef]
- Alam, M.N.; Kumar, V.; Jung, H.-S.; Park, S.-S. Fabrication of High-Performance Natural Rubber Composites with Enhanced Filler–Rubber Interactions by Stearic Acid-Modified Diatomaceous Earth and Carbon Nanotubes for Mechanical and Energy Harvesting Applications. Polymers 2023, 15, 3612. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Li, S.; Yang, L.; Liu, B.; Xie, S.; Qi, R.; Zhan, Y.; Xia, H. Graphene-Based Hybrid Fillers for Rubber Composites. Molecules 2024, 29, 1009. [Google Scholar] [CrossRef] [PubMed]
- Shahamatifard, F.; Rodrigue, D.; Mighri, F. Thermal and mechanical properties of carbon-based rubber nanocomposites: A review. Plast. Rubber Compos. 2023, 52, 483–505. [Google Scholar] [CrossRef]
- Álvarez-Cortez, G.; Molina, F.; Urbano, B.F.; Dahrouch, M.; Hernández Santana, M.; Manchado, M.A.L.; Verdejo, R.; Aguilar Bolados, H. Design and Study of Novel Composites Based on EPDM Rubber Containing Bismuth (III) Oxide and Graphene Nanoplatelets for Gamma Radiation Shielding. Polymers 2024, 16, 633. [Google Scholar] [CrossRef]
- Sekar, P.; Noordermeer, J.W.; Anyszka, R.; Gojzewski, H.; Podschun, J.; Blume, A. Hydrothermally Treated Lignin as a Sustainable Biobased Filler for Rubber Compounds. ACS Appl. Polym. Mater. 2023, 5, 2501–2512. [Google Scholar] [CrossRef]
- Wang, H.; Meng, F.; Yi, M.; Fang, L.; Wang, Z.; Wang, S. Highly Strong and Damage-Resistant Natural Rubber Membrane via Self-Assembly and Construction of Double Network. Membranes 2022, 12, 933. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.; Hu, J.; Liu, K.; Zhang, L.; Jiang, H.; Li, C. Reinforcement mechanism of silica surface hydroxyl, the opposite effect. Appl. Surf. Sci. 2023, 623, 157000. [Google Scholar] [CrossRef]
- Bouhamed, A.; Jöhrmann, N.; Naifar, S.; Böhm, B.; Hellwig, O.; Wunderle, B.; Kanoun, O. Collaborative Filler Network for Enhancing the Performance of BaTiO3/PDMS Flexible Piezoelectric Polymer Composite Nanogenerators. Sensors 2022, 22, 4181. [Google Scholar] [CrossRef]
- Tagliaro, I.; Cobani, E.; Carignani, E.; Conzatti, L.; D’Arienzo, M.; Giannini, L.; Martini, F.; Nardelli, F.; Scotti, R.; Stagnaro, P. The self-assembly of sepiolite and silica fillers for advanced rubber materials: The role of collaborative filler network. Appl. Clay Sci. 2022, 218, 106383. [Google Scholar] [CrossRef]
- Zhang, B.; Shao, X.; Tian, M.; Ning, N.; Zhang, L.; Wang, W. Mussel-inspired environmentally friendly dipping system for aramid fiber and its interfacial adhesive mechanism with rubber. Polymer 2022, 238, 124414. [Google Scholar] [CrossRef]
- Zhang, B.; Liang, T.; Shao, X.; Tian, M.; Ning, N.; Zhang, L.; Wang, W. Nondestructive Grafting of ZnO on the Surface of Aramid Fibers Followed by Silane Grafting to Improve its Interfacial Adhesion Property with Rubber. ACS Appl. Polym. Mater. 2021, 3, 4587–4594. [Google Scholar] [CrossRef]
- Yin, L.; Luo, Z.; Zhong, J.; Yang, B.; Ji, Y. Behaviour and mechanism of fatigue crack growth in aramid-fibre-reinforced styrene–butadiene rubber composites. Int. J. Fatigue 2020, 134, 105502. [Google Scholar] [CrossRef]
- Yin, L.; Zhou, Z.; Luo, Z.; Zhong, J.; Li, P.; Yang, B.; Yang, L. Reinforcing effect of aramid fibers on fatigue behavior of SBR/aramid fiber composites. Polym. Test. 2019, 80, 106092. [Google Scholar] [CrossRef]
- Mei, S.; Wang, J.; Wan, J.; Wu, X. Preparation Methods and Properties of CNT/CF/G Carbon-Based Nano-Conductive Silicone Rubber. Appl. Sci. 2023, 13, 6726. [Google Scholar] [CrossRef]
- Lozada, E.R.; Gutiérrez Aguilar, C.M.; Jaramillo Carvalho, J.A.; Sánchez, J.C.; Barrera Torres, G. Vegetable Cellulose Fibers in Natural Rubber Composites. Polymers 2023, 15, 2914. [Google Scholar] [CrossRef] [PubMed]
- Akbolat, M.C.; Katnam, K.B.; Soutis, C.; Potluri, P.; Sprenger, S.; Taylor, J. On mode-I and mode-II interlaminar crack migration and R-curves in carbon/epoxy laminates with hybrid toughening via core-shell rubber particles and thermoplastic micro-fibre veils. Compos. Part B Eng. 2022, 238, 109900. [Google Scholar] [CrossRef]
- Nag, A.; Simorangkir, R.B.; Sapra, S.; Buckley, J.L.; O’Flynn, B.; Liu, Z.; Mukhopadhyay, S.C. Reduced graphene oxide for the development of wearable mechanical energy-harvesters: A review. IEEE Sens. J. 2021, 21, 26415–26425. [Google Scholar] [CrossRef]
- Fang, Z.H.; Tu, Q.Z.; Shen, X.M.; Yang, X.; Liang, K.; Pan, M.; Chen, Z.Y. Biomimetic surface modification of UHMWPE fibers to enhance interfacial adhesion with rubber matrix via constructing polydopamine functionalization platform and then depositing zinc oxide nanoparticles. Surf. Interfaces 2022, 29, 101728. [Google Scholar] [CrossRef]
- Fang, Z.H.; Tu, Q.Z.; Chen, Z.Y.; Shen, X.M.; Pan, M.; Liang, K.; Yang, X. Study on catechol/tetraethylenepentamine and nano zinc oxide co-modifying ultrahigh molecular weight polyethylene fiber surface to improve interfacial adhesion. Polym. Adv. Technol. 2022, 33, 4072–4083. [Google Scholar] [CrossRef]
- He, J.; Huang, B.; Wang, L.; Cai, Z.; Zhang, J.; Feng, J. Enhancing Natural Rubber Tearing Strength by Mixing Ultra-High Molecular Weight Polyethylene Short Fibers. Polymers 2023, 15, 1768. [Google Scholar] [CrossRef] [PubMed]
- Victory, W. A Review on the Utilization of Waste Material in Asphalt Pavements. Environ. Sci. Pollut. Res. 2022, 29, 27279–27282. [Google Scholar] [CrossRef] [PubMed]
- Statnik, E.S.; Cvjetinovic, J.; Ignatyev, S.D.; Wassouf, L.; Salimon, A.I.; Korsunsky, A.M. Hair-Reinforced Elastomer Matrix Composites: Formulation, Mechanical Testing, and Advanced Microstructural Characterization. Polymers 2023, 15, 4448. [Google Scholar] [CrossRef] [PubMed]
- Chao, Q.; Maneengam, A.; Margabandu, S.; Jindal, P.; Patil, P.Y.; Zheng, G.-W.; Selvaraj, R. Mechanical and damping property evaluation of jute fiber reinforced composite periodic core sandwich panels. J. Nat. Fibers 2022, 19, 11424–11434. [Google Scholar] [CrossRef]
- Aslani, F.; Dale, R.; Hamidi, F.; Valizadeh, A. Mechanical and shrinkage performance of 3D-printed rubberised engineered cementitious composites. Constr. Build. Mater. 2022, 339, 127665. [Google Scholar] [CrossRef]
- Sharma, A.; Joshi, S.C. Enhancement in fatigue performance of FRP composites with various fillers: A review. Compos. Struct. 2023, 309, 116724. [Google Scholar] [CrossRef]
- Velmurugan, N.; Manimaran, G.; Ravi, S.; Jayabalakrishnan, D. Effect of silanised reinforcements on thermal, wear, visco-elastic and fatigue behaviour of stitched E-glass fibre-reinforced epoxy hybrid composite. J. Rubber Res. 2021, 24, 41–50. [Google Scholar] [CrossRef]
- Lamy-Mendes, A.; Pontinha, A.D.R.; Santos, P.; Durães, L. Aerogel Composites Produced from Silica and Recycled Rubber Sols for Thermal Insulation. Materials 2022, 15, 7897. [Google Scholar] [CrossRef] [PubMed]
- Mahendra, I.P.; Wirjosentono, B.; Tamrin, T.; Ismail, H.; Mendez, J.A.; Causin, V. The effect of nanocrystalline cellulose and TEMPO-oxidized nanocellulose on the compatibility of polypropylene/cyclic natural rubber blends. J. Thermoplast. Compos. Mater. 2022, 35, 2146–2161. [Google Scholar] [CrossRef]
- El-Wakil, A.E.A.; Abd-Elbasseer, M.; El-Basheer, T.M. Mechanical and Acoustical Properties of Eichhornia crassipes (Water Hyacinth) Fiber-Reinforced Styrene Butadiene Rubber. Polym. Compos. 2021, 42, 3732–3745. [Google Scholar] [CrossRef]
- Yang, X.; Tang, S.; Shen, X.; Peng, W. Research on the Sound Insulation Performance of Composite Rubber Reinforced with Hollow Glass Microsphere Based on Acoustic Finite Element Simulation. Polymers 2023, 15, 611. [Google Scholar] [CrossRef] [PubMed]
- Miedzianowska, J.; Masłowski, M.; Strzelec, K. Thermoplastic Elastomeric Composites Filled with Lignocellulose Bioadditives, Part 2: Flammability, Thermo-Oxidative Aging Resistance, Mechanical and Barrier Properties. Materials 2020, 13, 1608. [Google Scholar] [CrossRef] [PubMed]
- Enieb, M.; Diab, A.; Yang, X. Short- and long-term properties of glass fiber reinforced asphalt mixtures. Int. J. Pavement Eng. 2021, 22, 64–76. [Google Scholar] [CrossRef]
- Xu, X.; Yao, X.; Dong, Y.; Yang, H.; Yan, H. Mechanical behaviors of non-orthogonal fabric rubber seal. Compos. Struct. 2021, 259, 113453. [Google Scholar] [CrossRef]
- He, H.; Wu, P.; Yang, Z.; Shi, Z.; Yu, W.; Liu, F.; Zhu, F.; Zheng, Q.; Zhang, D.; Li, S. A Facile Way to Modify Polyester Fabric to Enhance the Adhesion Behavior to Rubber. Coatings 2022, 12, 1344. [Google Scholar] [CrossRef]
- ASTM D3039; Standard Test Method for Tensile Properties of Polymer Matrix Composite Materials. ASTM International: West Conshehoken, PA, USA, 2000.
- ASTM D7264; Standard Test Method for Flexural Properties of Polymer Matrix Composite Materials. ASTM International: West Conshehoken, PA, USA, 2000.
- Láštic, D.; Řehák, K.; Prokop, A.; Krpalek, D.; Šplíchal, J.; Žlábek, P. An Initial Case Study of the Computational Modeling of Cord–Rubber Segments. Appl. Sci. 2024, 14, 1117. [Google Scholar] [CrossRef]
- Yang, J.; Lou, W. Molecule Dynamics Simulation of the Effect of Oxidative Aging on Properties of Nitrile Rubber. Polymers 2022, 14, 226. [Google Scholar] [CrossRef] [PubMed]
- Heydari, A.; Esmizadeh, E.; Vahidifar, A.; Naderi, G.; Rodrigue, D. Real 3D Structure-Based Finite Element Simulation of Elastomer Foams: Effect of the Foaming Agent Content. Ind. Eng. Chem. Res. 2022, 61, 7881–7890. [Google Scholar] [CrossRef]
- Singaravel, D.K.; Sharma, S.; Kumar, P. Recent progress in experimental and molecular dynamics study of carbon nanotube reinforced rubber composites: A review. Polym.-Plast. Technol. Mater. 2022, 61, 1792–1825. [Google Scholar] [CrossRef]
- Fathi, H.; Khosravi, M.; El-Sayegh, Z.; El-Gindy, M. An Advancement in Truck-Tire–Road Interaction Using the Finite Element Analysis. Mathematics 2023, 11, 2462. [Google Scholar] [CrossRef]
- Ma, Y.; Yuan, X.; Jiang, R.; Liao, J.; Yu, R.; Chen, Y.; Liao, L. Molecular Dynamic and Dissipative Particle Dynamic Simulation on the Miscibility of NR/CR Blends. Polymers 2023, 15, 856. [Google Scholar] [CrossRef] [PubMed]
- Sodeifian, G.; Nikooamal, H.R.; Yousefi, A.A. Molecular dynamics study of epoxy/clay nanocomposites: Rheology and molecular confinement. J. Polym. Res. 2012, 19, 9897. [Google Scholar] [CrossRef]
- Zhang, D.; Dong, H.; Zhao, S.; Yan, W.; Wang, Z. Effect of Impact Angle on the Impact Mechanical Properties of Bionic Foamed Silicone Rubber Sandwich Structure. Polymers 2023, 15, 688. [Google Scholar] [CrossRef] [PubMed]
- Jagadeesh, G.; Gangi Setti, S. A review on micromechanical methods for evaluation of mechanical behavior of particulate reinforced metal matrix composites. J. Mater. Sci. 2020, 55, 9848–9882. [Google Scholar] [CrossRef]
- Kundalwal, S.I. Review on micromechanics of nano-and micro-fiber reinforced composites. Polym. Compos. 2018, 39, 4243–4274. [Google Scholar] [CrossRef]
- Fedotov, A.F. Hybrid model of homogenization of engineering elastic moduli of composites reinforced with ellipsoid particles. Compos. Part B Eng. 2020, 182, 107585. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Dabbagh, A. Vibration analysis of multi-scale hybrid nanocomposite plates based on a Halpin-Tsai homogenization model. Compos. Part B Eng. 2019, 173, 106955. [Google Scholar] [CrossRef]
- Fredi, G.; Dorigato, A.; Fambri, L.; Pegoretti, A. Detailed experimental and theoretical investigation of the thermomechanical properties of epoxy composites containing paraffin microcapsules for thermal management. Polym. Eng. Sci. 2020, 60, 1202–1220. [Google Scholar] [CrossRef]
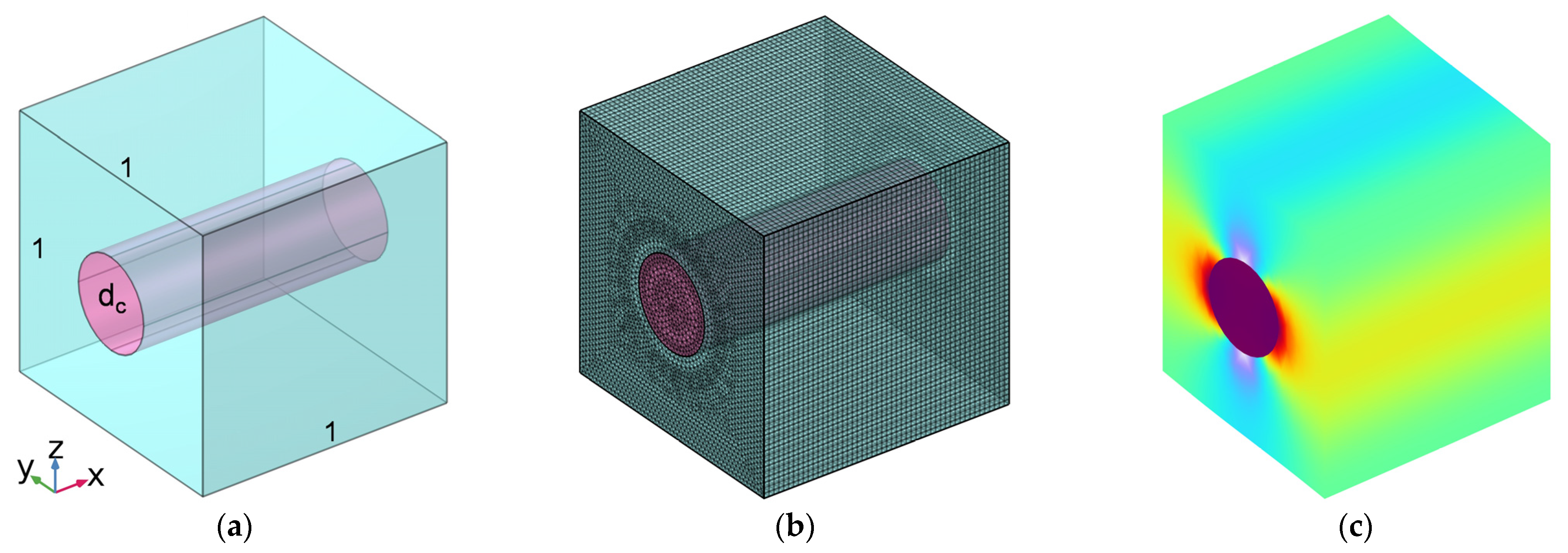







| Parameters | Symbol | Unit | Values |
|---|---|---|---|
| Young’s modulus | {Exc, Eyc, Ezc} | GPa | {230, 15, 15} |
| Shear modulus | {Gxyc, Gyzc, Gxzc} | GPa | {15, 7, 15} |
| Thermal expansion coefficient | {αxc, αyc, αzc} | 1/K | {−0.8 × 10−6, 25 × 10−6, 25 × 10−6} |
| Poisson’s ratio | {υxyc, υyzc, υxzc} | 1 | {0.3, 0.08, 0.3} |
| Density | ρc | Kg/m3 | 1800 |
| Parameters | Symbol | Unit | Values |
|---|---|---|---|
| Young’s modulus | Er | MPa | 4 |
| Shear modulus | Gr | MPa | 1.36 |
| Thermal expansion coefficient | αr | 1/K | 1 × 10−4 |
| Poisson’s ratio | υr | 1 | 0.47 |
| Density | ρr | Kg/m3 | 920 |
| Symbol | Load 11 | Load 22 | Load 33 | Load 12 | Load 23 | Load 13 |
| Loading direction | x-axis | y-axis | z-axis | x–y oblique plane | y–z oblique plane | x–z oblique plane |
| Vfc | 0% | 10% | 20% | 30% | 40% | 50% | 60% | 70% | 100% |
|---|---|---|---|---|---|---|---|---|---|
| Exx (GPa) | 0.0004 | 23.0220 | 46.0217 | 69.0216 | 92.0217 | 115.0221 | 138.0232 | 161.0268 | 230 |
| Eyy (MPa) | 4 | 5.4078 | 6.1820 | 7.2254 | 8.7080 | 10.9916 | 15.0935 | 25.8701 | 15,000 |
| Gxy (MPa) | 1.36 | 1.6630 | 2.0411 | 2.5306 | 3.1982 | 4.1898 | 5.9053 | 10.1056 | 15,000 |
| αxx (1/K) | 1 × 10−4 | −4.9768 × 10−7 | −5.5226 × 10−7 | −5.7082 × 10−7 | −5.8031 × 10−7 | −5.8602 × 10−7 | −5.8931 × 10−7 | −5.8894 × 10−7 | −8 × 10−7 |
| αyy (1/K) | 1 × 10−4 | 5.7151 × 10−4 | 5.0573 × 10−4 | 4.4064 × 10−4 | 3.7611 × 10−4 | 3.1162 × 10−4 | 2.4510 × 10−4 | 1.6609 × 10−4 | 0.25 × 10−4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, X.; Shen, X.; Peng, W.; Hu, D.; Wang, X.; Song, H.; Zhao, R.; Zhang, C. Research on the Mechanical and Thermal Properties of Carbon-Fiber-Reinforced Rubber Based on a Finite Element Simulation. Polymers 2024, 16, 2120. https://doi.org/10.3390/polym16152120
Yang X, Shen X, Peng W, Hu D, Wang X, Song H, Zhao R, Zhang C. Research on the Mechanical and Thermal Properties of Carbon-Fiber-Reinforced Rubber Based on a Finite Element Simulation. Polymers. 2024; 16(15):2120. https://doi.org/10.3390/polym16152120
Chicago/Turabian StyleYang, Xiaocui, Xinmin Shen, Wenqiang Peng, Daochun Hu, Xiaoyong Wang, Haichao Song, Rongxing Zhao, and Chunmei Zhang. 2024. "Research on the Mechanical and Thermal Properties of Carbon-Fiber-Reinforced Rubber Based on a Finite Element Simulation" Polymers 16, no. 15: 2120. https://doi.org/10.3390/polym16152120
APA StyleYang, X., Shen, X., Peng, W., Hu, D., Wang, X., Song, H., Zhao, R., & Zhang, C. (2024). Research on the Mechanical and Thermal Properties of Carbon-Fiber-Reinforced Rubber Based on a Finite Element Simulation. Polymers, 16(15), 2120. https://doi.org/10.3390/polym16152120






