Digital Image Correlation and Ultrasonic Lamb Waves for the Detection and Prediction of Crack-Type Damage in Fiber-Reinforced Polymer Composite Laminates
Abstract
1. Introduction
2. Materials and Methods
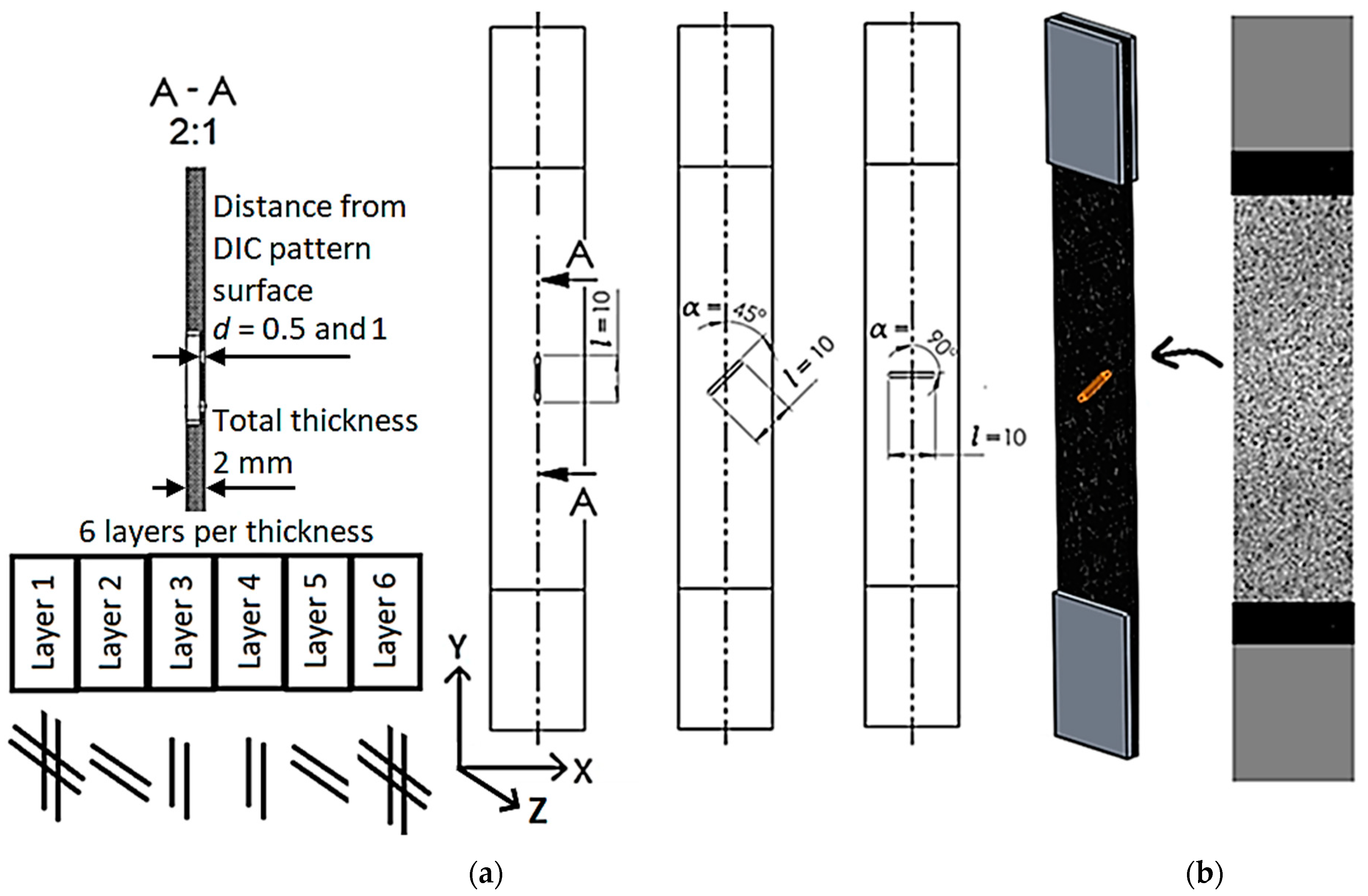
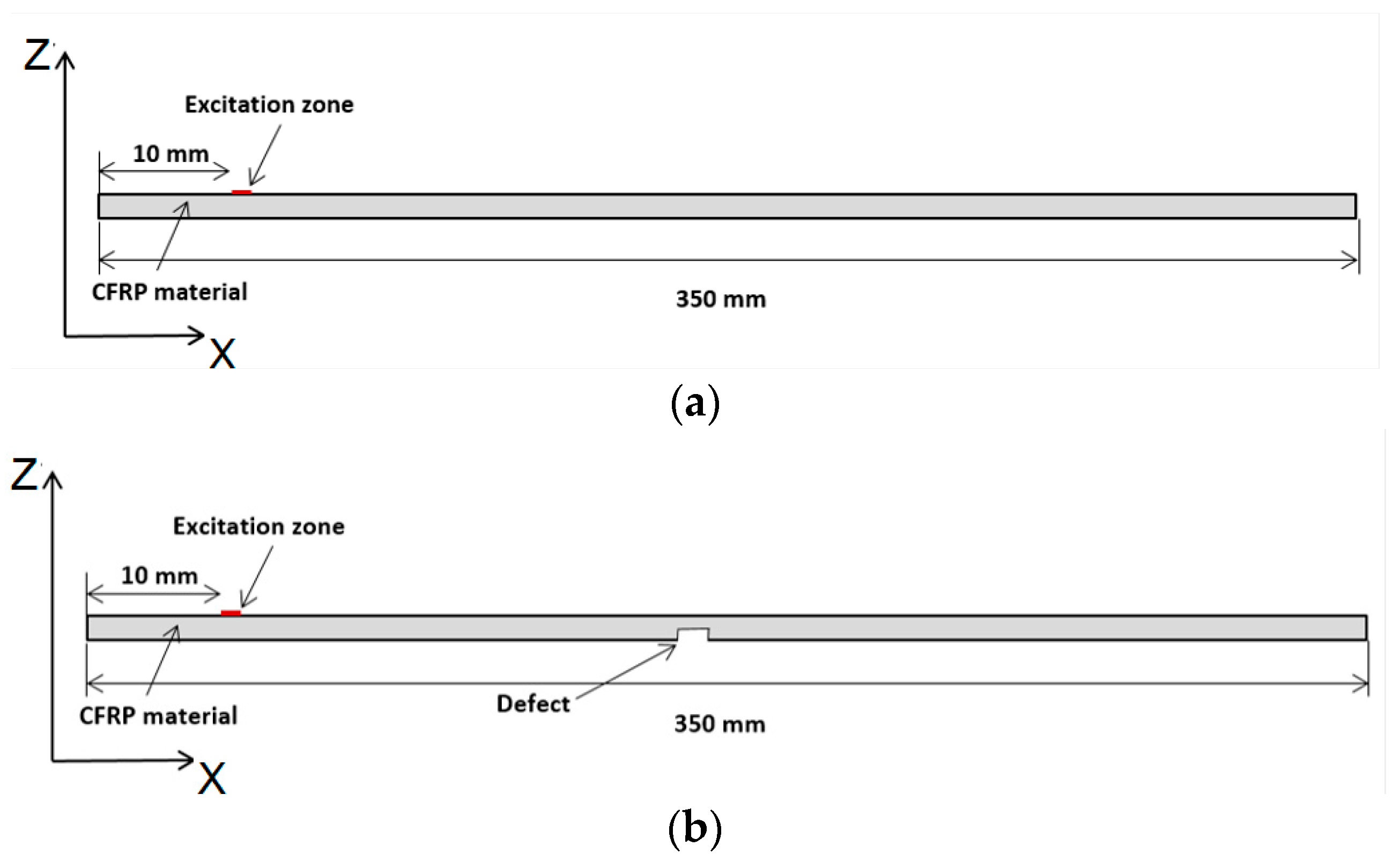
2.1. Test Specimens
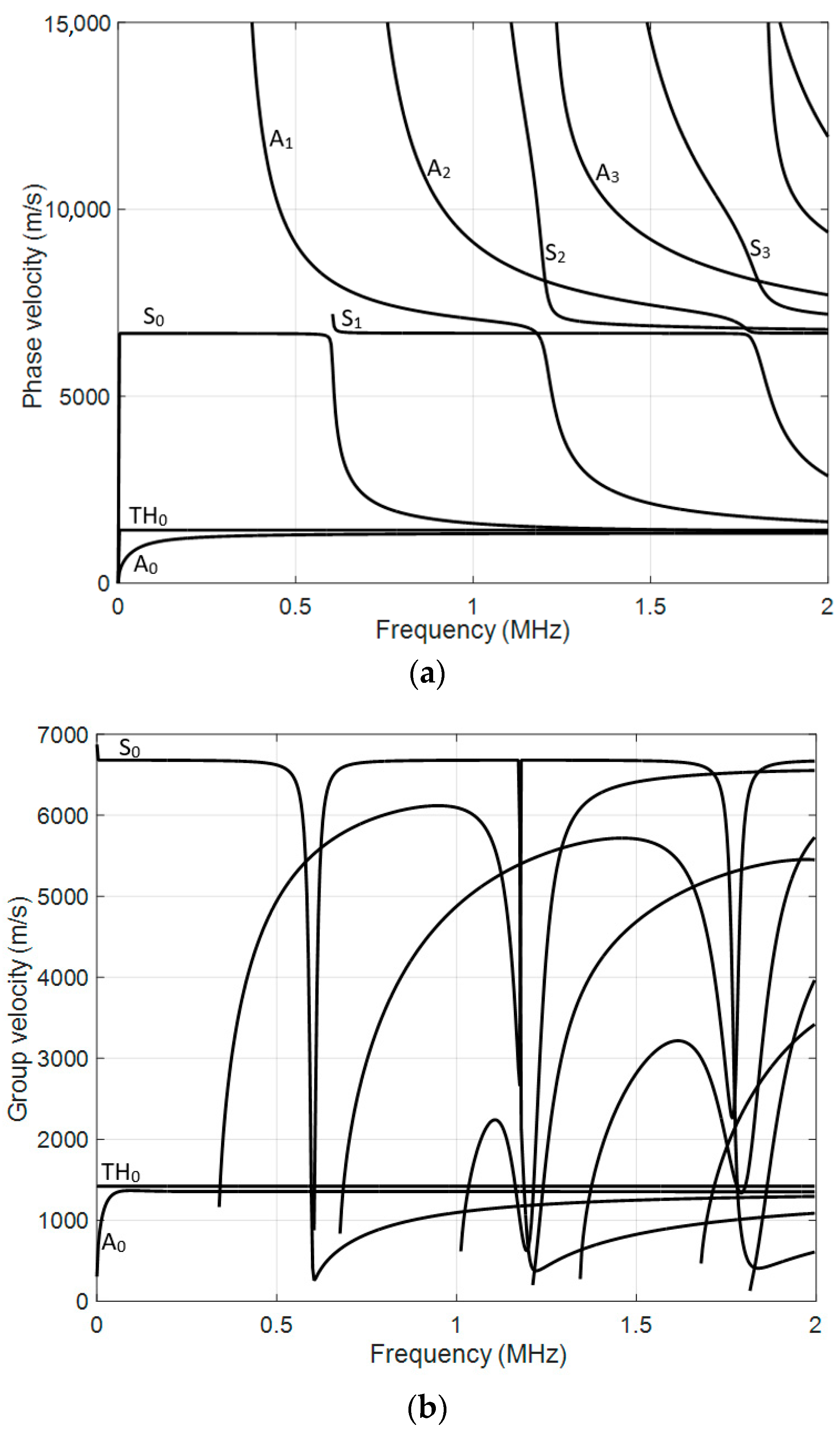
2.2. Calculation of Guided Wave Dispersion Curves Using the Semi-Analytical Finite Element (SAFE) Method
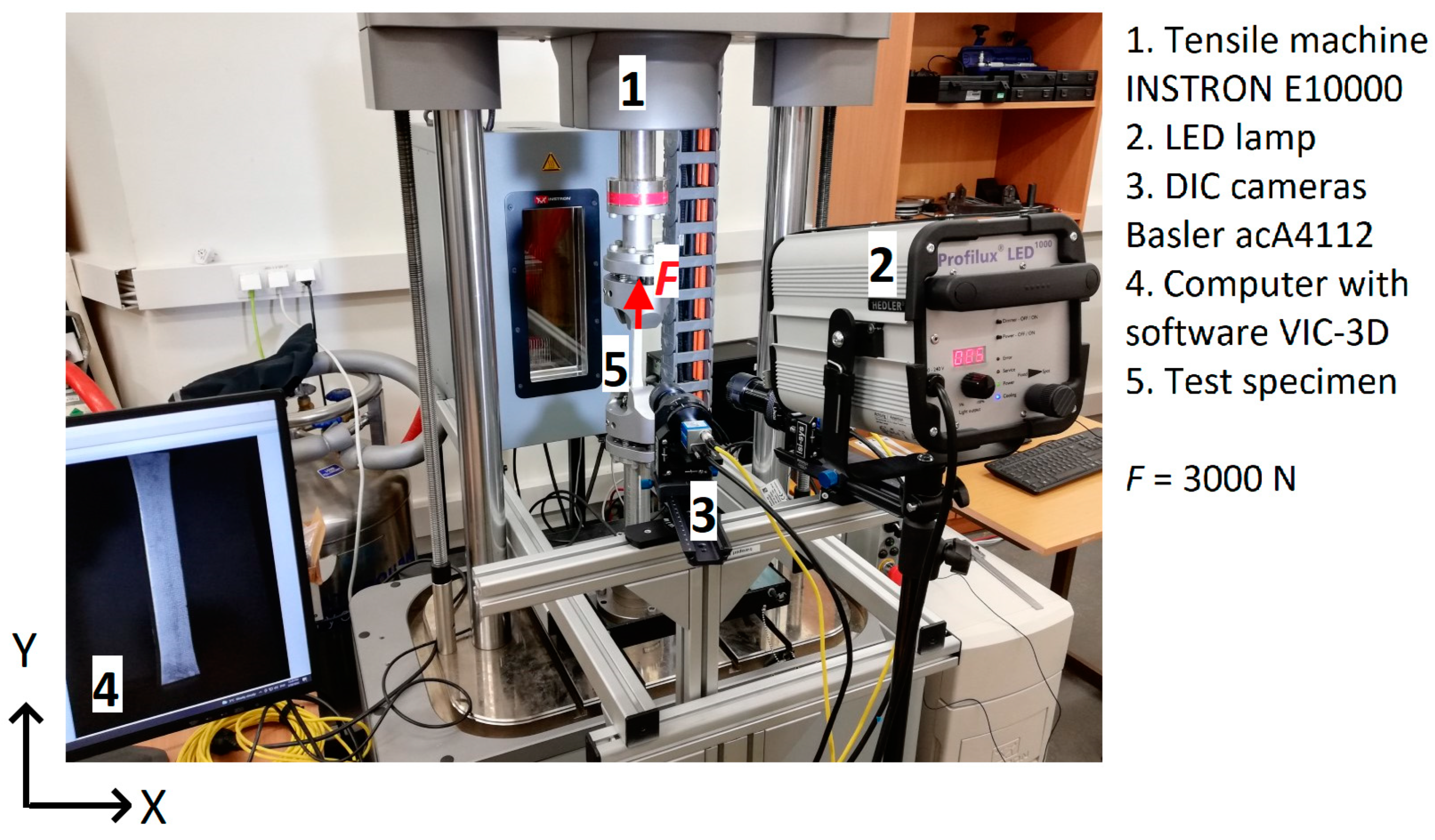
2.3. Digital Image Correlation
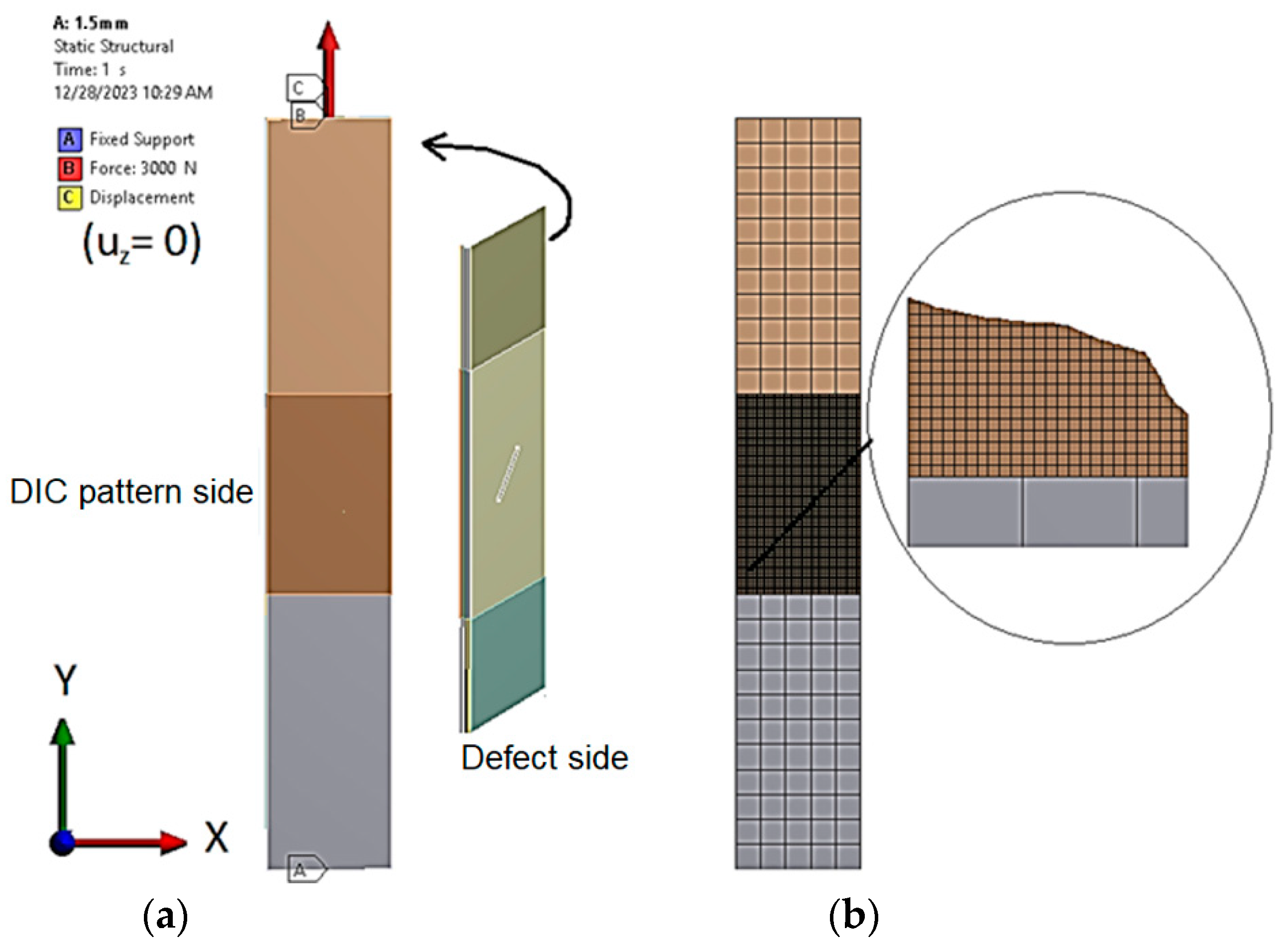
2.4. Numerical Modeling of the CFRP Specimen Surface Strains
3. Results and Discussion
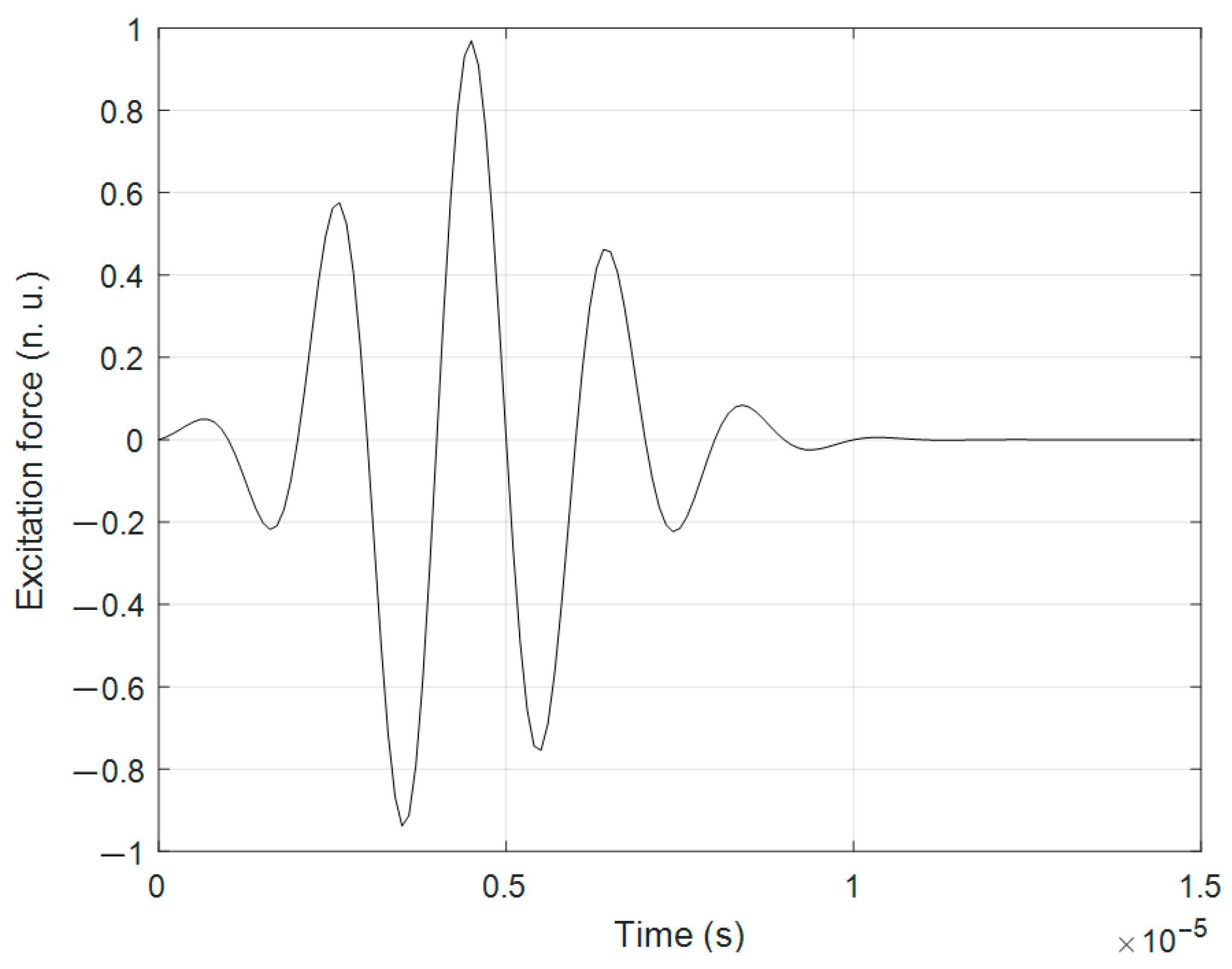
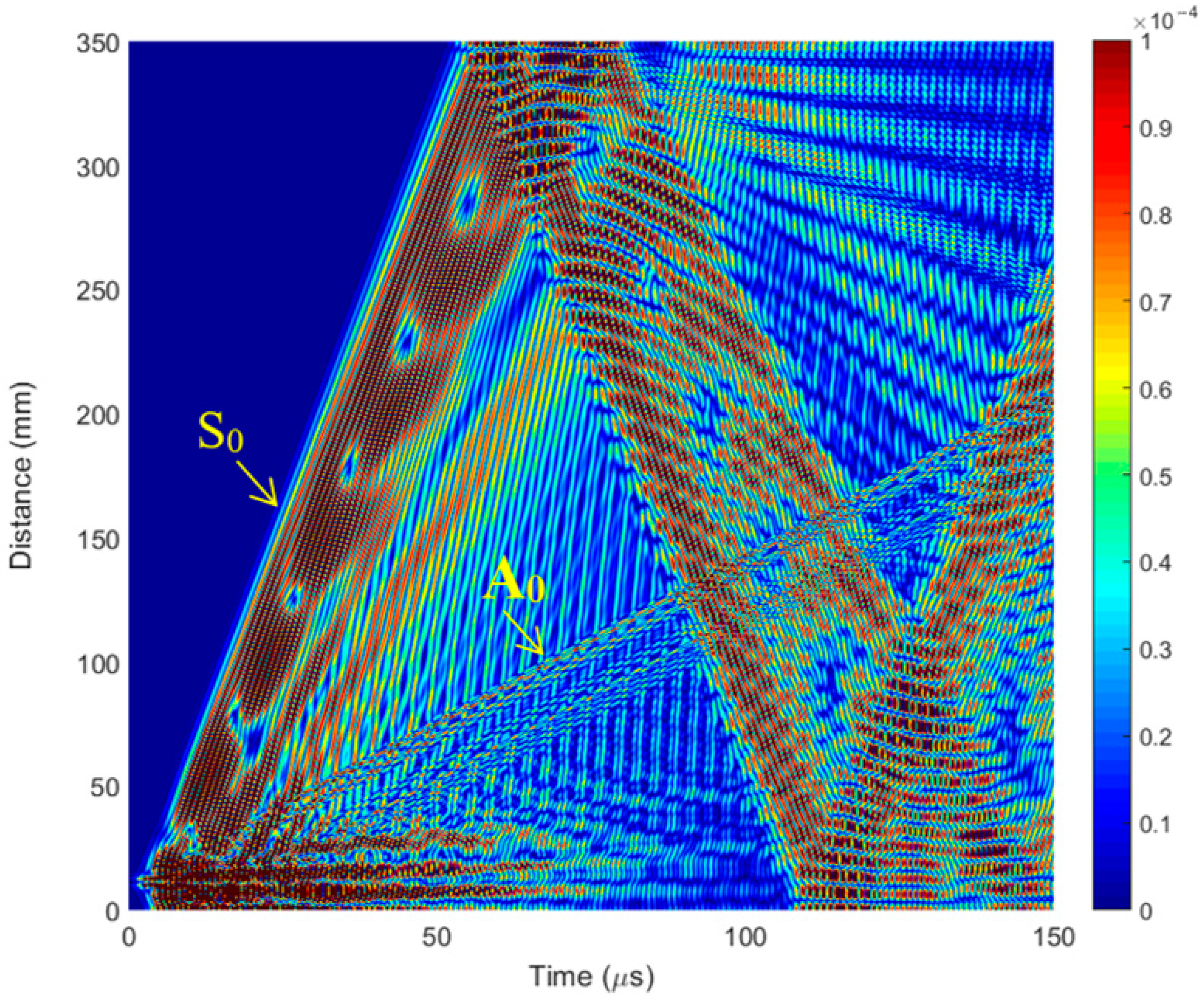
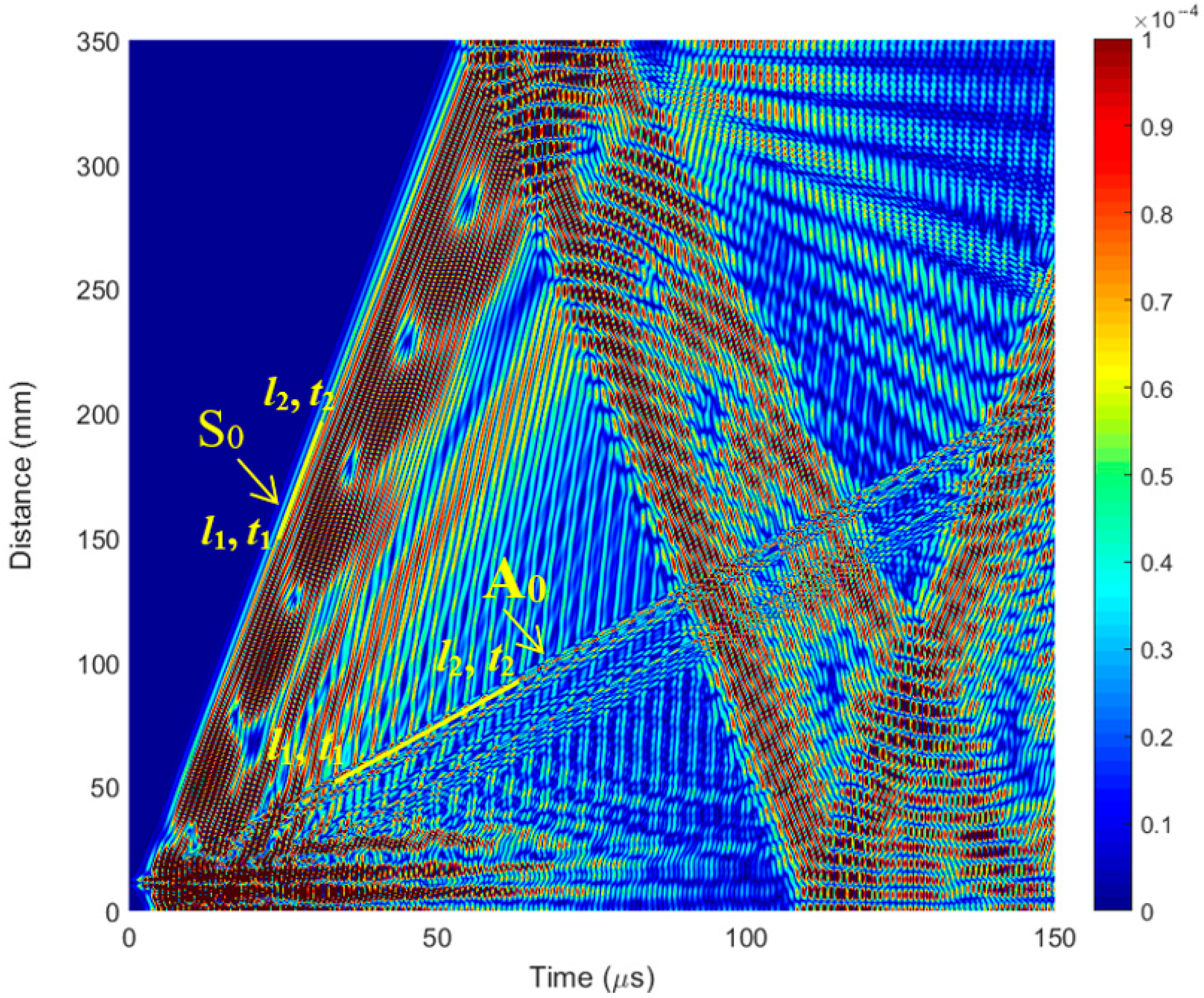
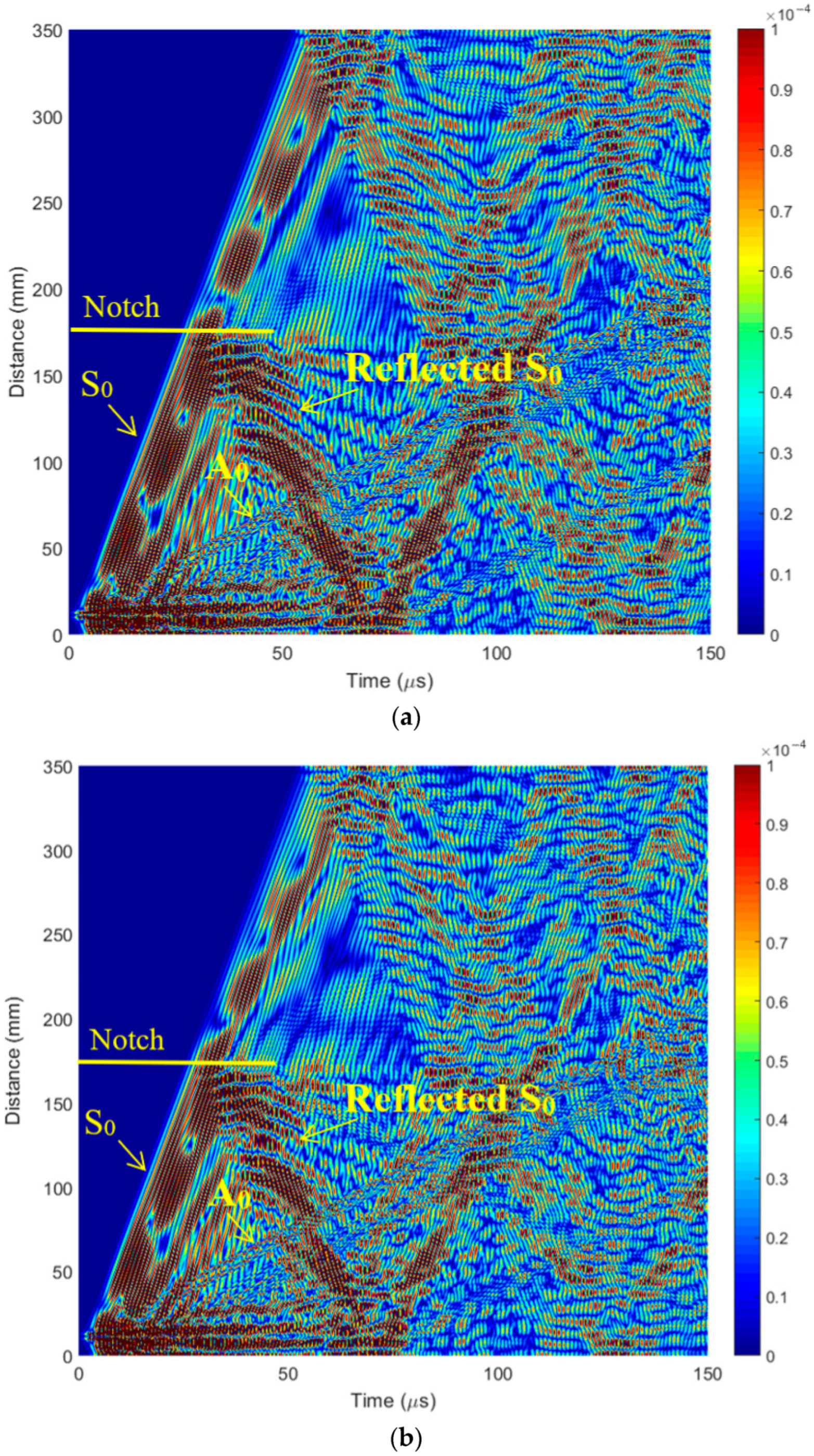
3.1. FEM Simulation of Lamb Wave Propagation
3.2. Digital Image Correlation Results
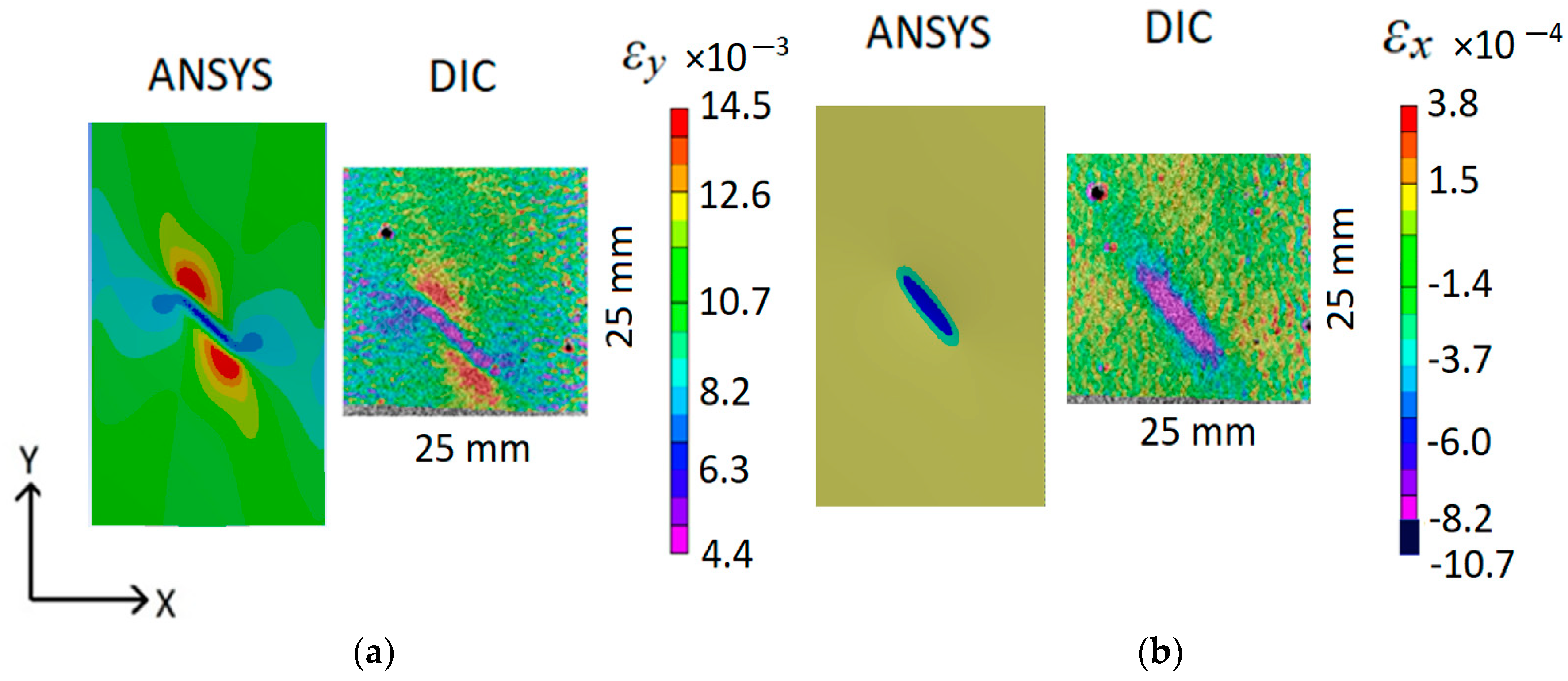
3.2.1. Finite Element Model Validation and Defect Detection
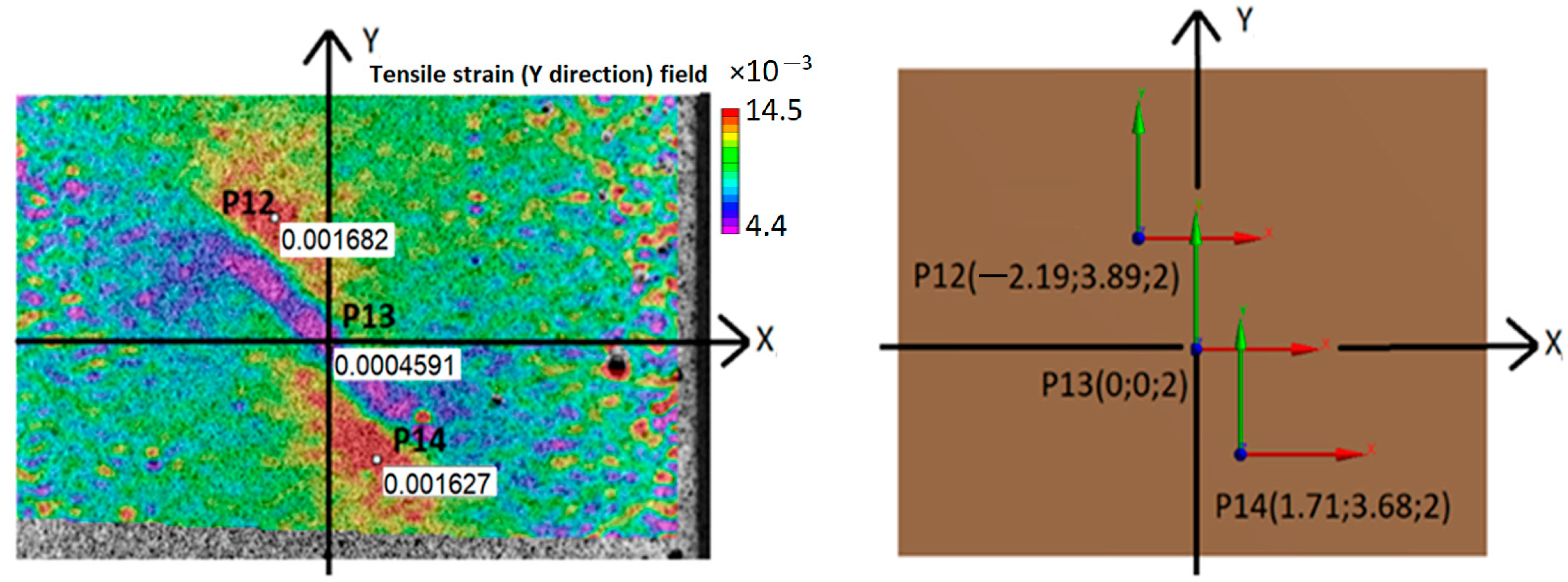
3.2.2. DIC Defect Detection Analysis for Tensile Loading
3.2.3. DIC Defect Detection for Bending Loading
4. Summary and Conclusions
- The Lamb wave analysis must first be applied to the tested structure to identify defects in the structure. This can help to distinguish defects from noise in the DIC surface strain measurement. Once defects have been identified with Lamb waves and are visible in the surface strain field measured by DIC, DIC can be used to characterize defects and monitor their growth. The maximum to average strain ratio r around the defect is used as a defect characterization criterion in this study. A defect is visible if r is greater than 1.2 and its generated strain field unevenness is greater than the tested composite surface roughness.
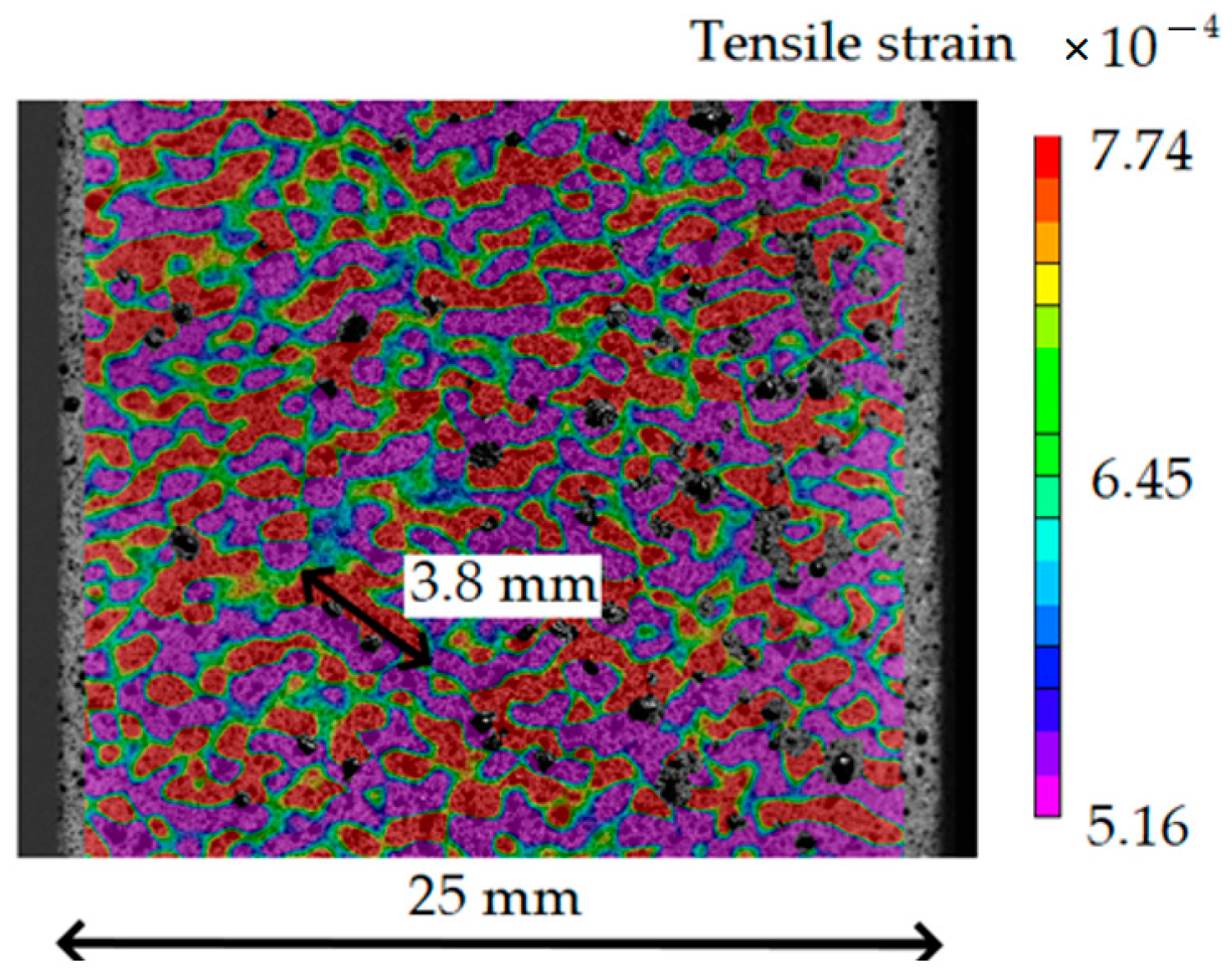
- The visibility of defects in the DIC strain field depends on the loading type of the structure, the defect location, the geometry, and the composite material. It has been demonstrated on the CFRP composite that tensile and cantilever beam bending loading have different defect visibility limits, e.g., tensile loading has an advantage over bending for defects that have a 1.5 times smaller angle to the loading direction, while bending has an advantage for the detection of deeper defects (75% of the structure thickness and more vs. a maximum limit of 65% of the structure thickness for tensile loading) from the structure surface. The minimum visible defect length is 10% of the structure width, but the unevenness of the composite surface limits the very minimum defect size to 3.8 mm (15% of the structure width) for the current CFRP.
- Due to the variety of different composite materials, their lay-ups, and the infinite number of structural boundary conditions, this study proposes a methodology for the analysis of structure defects with DIC. A validated FEM of the structure can be used to create a strain field database (similar to Table 1 or Table 2), analyze defects of different geometries in a particular composite structure, and determine if such defects can be detected, characterized, monitored by DIC, or require other testing methods.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Hliva, V.; Szebényi, G. Non-Destructive Evaluation and Damage Determination of Fiber-Reinforced Composites by Digital Image Correlation. J. Nondestr. Eval. 2023, 42, 43. [Google Scholar] [CrossRef]
- Data Bridge. Europe Structural Health Monitoring Market—Industry Trends and Forecast to 2030 n.d. Available online: https://www.databridgemarketresearch.com/reports/europe-structural-health-monitoring-market (accessed on 25 April 2024).
- Integrity Diagnostics. Integrity Diagnostics Diagnostic Acoustic Emission Solutions for Safety and Performance 2018. Available online: http://www.idinspections.com/acoustic-emission-phenomenon/ (accessed on 15 November 2023).
- Aerospeace Engineering. Defects and Non-Destructive Testing in Composites 2013. Available online: https://aerospaceengineeringblog.com/defects-and-non-destructive-testing-in-composites/ (accessed on 16 November 2023).
- Garcea, S.C.; Wang, Y.; Withers, P.J. X-ray computed tomography of polymer composites. Compos. Sci. Technol. 2018, 156, 305–319. [Google Scholar] [CrossRef]
- Kroeger, T. Thermographic inspection of composites. Reinf. Plast. 2014, 58, 42–43. [Google Scholar] [CrossRef]
- Correlated Solutions. Digital Image Correlation n.d. Available online: https://www.correlatedsolutions.com/about-us/ (accessed on 13 January 2022).
- Paper, C.; Hyderabad, T. Material Characterization of Carbon Fiber Reinforced Polymer Laminate Using Virtual Fields Method. In Proceedings of the ICTACEM 2014, International Conference on Theoretical, Applied, Computational and Experimental Mechanics, Kharagpur, India, 29–31 December 2014; pp. 1–10. [Google Scholar] [CrossRef]
- Quanjin, M.; Rejab, M.R.M.; Halim, Q.; Merzuki, M.N.M.; Darus, M.A.H. Experimental investigation of the tensile test using digital image correlation (DIC) method. Mater. Today Proc. 2020, 27, 757–763. [Google Scholar] [CrossRef]
- Guezo, Y.J.A.; Vipulanandan, C. Monitoring Crack Growth in Layered Polymer Composite Beams using Digital Image Correlation (DIC). System 2013, 2, 2–3. [Google Scholar]
- Schlothauer, A.; Pappas, G.A.; Ermanni, P. Material response and failure of highly deformable carbon fiber composite shells. Compos. Sci. Technol. 2020, 199, 108378. [Google Scholar] [CrossRef]
- Devivier, C.; Thompson, D.; Pierron, F.; Wisnom, M.R. Correlation between full-field measurements and numerical simulation results for multiple delamination composite specimens in bending. Appl. Mech. Mater. 2010, 24–25, 109–114. [Google Scholar] [CrossRef]
- Szebényi, G.; Hliva, V. Detection of delamination in polymer composites by digital image correlation-experimental test. Polymers 2019, 11, 523. [Google Scholar] [CrossRef] [PubMed]
- Bataxi Chen, X.; Yu, Z.; Wang, H.; Bil, C. Strain Monitoring on Damaged Composite Laminates Using Digital Image Correlation. Procedia Eng. 2015, 99, 353–360. [Google Scholar] [CrossRef]
- Griskevicius, P.; Spakauskas, K.; Mahato, S.; Grigaliunas, V.; Raisutis, R.; Eidukynas, D.; Perkowski, D.M.; Vilkauskas, A. Experimental and Numerical Study of Healing Effect on Delamination Defect in Infusible Thermoplastic Composite Laminates. Materials 2023, 16, 6764. [Google Scholar] [CrossRef]
- Ambu, R.; Aymerich, F.; Bertolino, F. Investigation of the effect of damage on the strength of notched composite laminates by digital image correlation. J. Strain. Anal. Eng. Des. 2005, 40, 451–461. [Google Scholar] [CrossRef]
- Gonabadi, H.; Oila, A.; Yadav, A.; Bull, S. Fatigue damage analysis of GFRP composites using digital image correlation. J. Ocean Eng. Mar. Energy 2021, 7, 25–40. [Google Scholar] [CrossRef]
- Swain, D.; Selvan, S.K.; Thomas, B.P.; Philip, J. Real-Time Detection of Defects on a Honeycomb Composite Sandwich Structure Using Digital Image Correlation (DIC). In Conference and Exhibition on Non Destructive Evaluation; Lecture Notes in Mechanical Engineering; Springer Nature: Singapore, 2022; pp. 51–61. [Google Scholar] [CrossRef]
- Vaitkunas, T.; Griskevicius, P.; Adumitroaie, A. Peridynamic Approach to Digital Image Correlation Strain Calculation Algorithm. Appl. Sci. 2022, 12, 6550. [Google Scholar] [CrossRef]
- Munian, R.K.; Mahapatra, D.R.; Gopalakrishnan, S. Lamb wave interaction with composite delamination. Compos. Struct. 2018, 206, 484–498. [Google Scholar] [CrossRef]
- Duflo, H.; Morvan, B.; Izbicki, J.L. Interaction of Lamb waves on bonded composite plates with defects. Compos. Struct. 2007, 79, 229–233. [Google Scholar] [CrossRef]
- Su, Z.; Ye, L.; Lu, Y. Guided Lamb waves for identification of damage in composite structures: A review. J. Sound Vib. 2006, 295, 753–780. [Google Scholar] [CrossRef]
- Ramadas, C.; Balasubramaniam, K.; Joshi, M.; Krishnamurthy, C.V. Interaction of guided Lamb waves with an asymmetrically located delamination in a laminated composite plate. Smart Mater. Struct. 2010, 19, 065009. [Google Scholar] [CrossRef]
- Samaitis, V.; Jasiūnienė, E.; Packo, P.; Smagulova, D. Ultrasonic Methods; Springer Aerospace Technology; Springer: Berlin/Heidelberg, Germany, 2021; pp. 87–131. [Google Scholar] [CrossRef]
- Staszewski, W.J.; Mahzan, S.; Traynor, R. Health monitoring of aerospace composite structures—Active and passive approach. Compos. Sci. Technol. 2009, 69, 1678–1685. [Google Scholar] [CrossRef]
- Ramadas, C.; Balasubramaniam, K.; Joshi, M.; Krishnamurthy, C.V. Characterisation of rectangular type delaminations in composite laminates through B- and D-scan images generated using Lamb waves. NDT E Int. 2011, 44, 281–289. [Google Scholar] [CrossRef]
- Papanaboina, M.R.; Jasiuniene, E.; Samaitis, V.; Mažeika, L.; Griškevičius, P. Delamination Localization in Multilayered CFRP Panel Based on Reconstruction of Guided Wave Modes. Appl. Sci. 2023, 13, 9687. [Google Scholar] [CrossRef]
- Papanaboina, M.R.; Jasiuniene, E.; Žukauskas, E.; Mažeika, L. Numerical Analysis of Guided Waves to Improve Damage Detection and Localization in Multilayered CFRP Panel. Materials 2022, 15, 3466. [Google Scholar] [CrossRef]
- Monson, P.; Junior, P.O.C.; Rodrigues, A.R.; Aguiar, P.; Junior, C.S. Method for Damage Detection of CFRP Plates Using Lamb Waves and Digital Signal Processing Techniques †. Eng. Proc. 2022, 27, 42. [Google Scholar] [CrossRef]
- Ihn, J.B.; Chang, F.K. Pitch-catch active sensing methods in structural health monitoring for aircraft structures. Struct. Health Monit. 2008, 7, 5–19. [Google Scholar] [CrossRef]
- ASTM D3039/D3039M-00; Standard Test Method for Tensile Properties of Polymer Matrix Composite Materials. ASTM International: West Conshohocken, PA, USA, 2000.
- Stress Engineering Services. Using Digital Image Correlation to Measure Large Areas of Strain and Displacement n.d. Available online: https://www.stress.com/dic-to-measure-large-areas-of-strain-displacement/ (accessed on 2 July 2024).


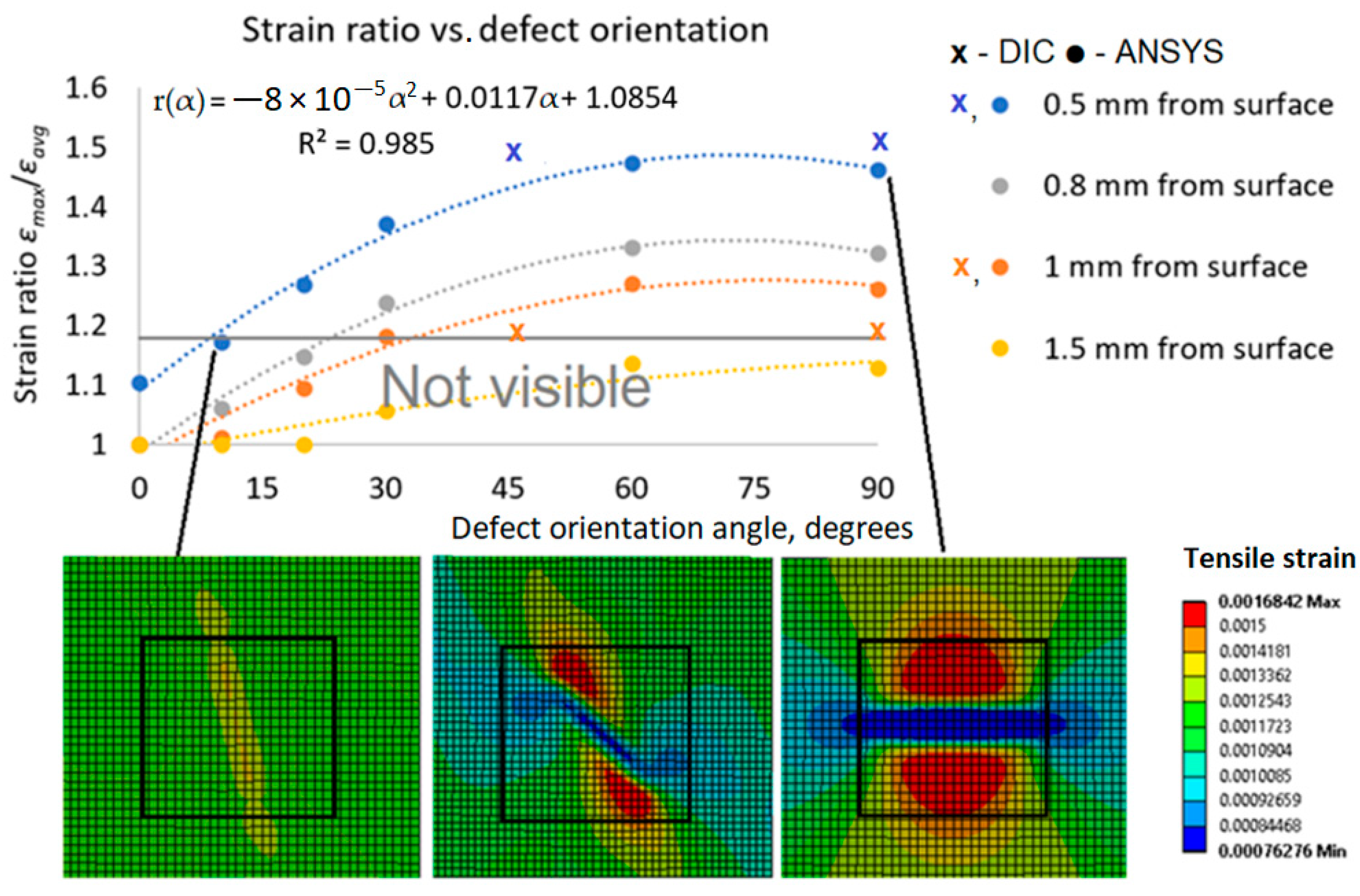
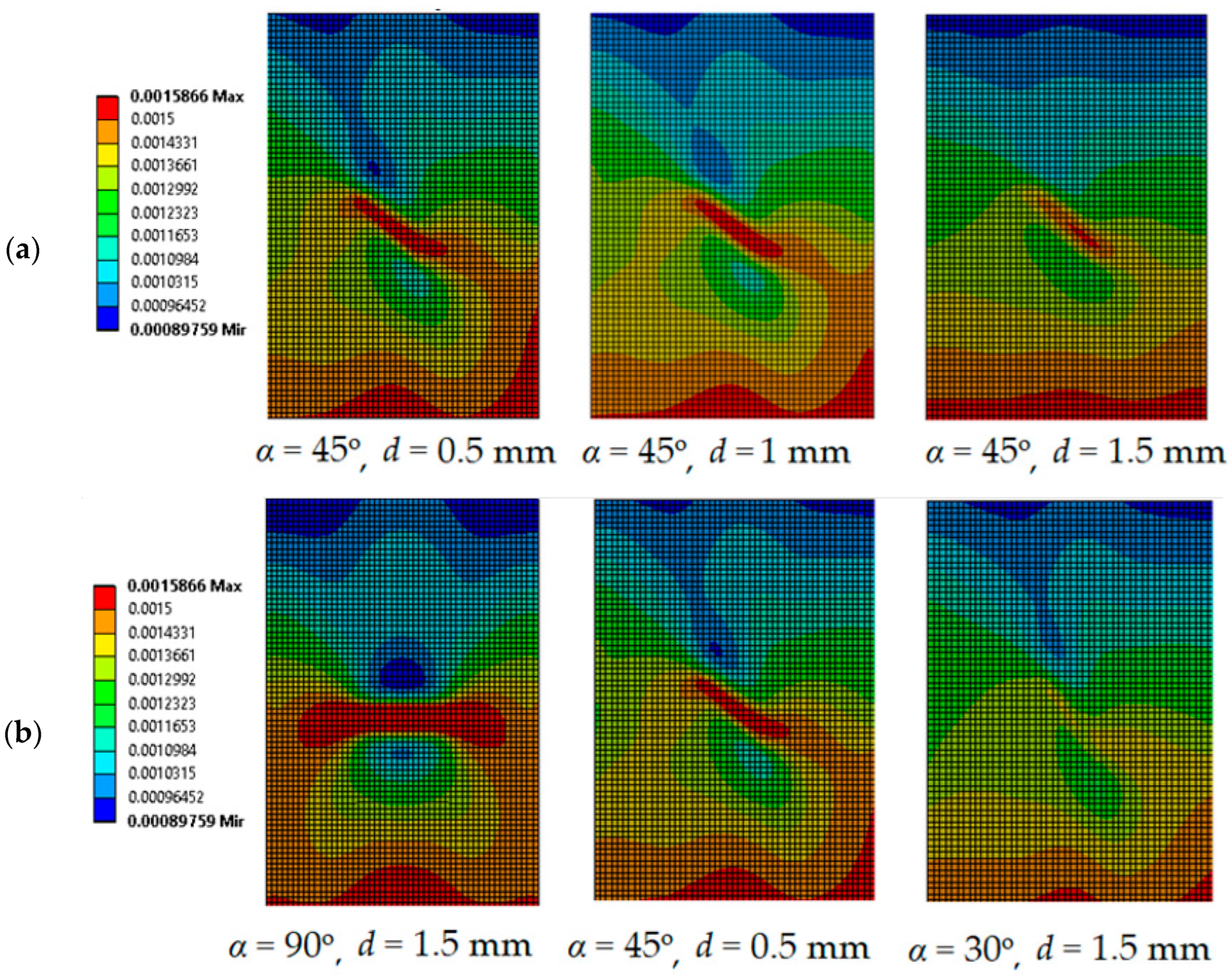
| Angle | 0 | 30 | 45 | 60 | 90 | ||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Length | 4 | 5 | 6 | 10 | 4 | 5 | 6 | 10 | 4 | 5 | 6 | 10 | 4 | 5 | 6 | 10 | 4 | 5 | 6 | 10 | |
| Distance from surface | 0.4 | 1.09 | 1.13 | 1.17 | 1.28 | 1.45 | 1.50 | 1.55 | 1.70 | 1.45 | 1.50 | 1.55 | 1.70 | 1.46 | 1.50 | 1.56 | 1.71 | 1.45 | 1.49 | 1.55 | 1.69 |
| 0.5 | 1.00 | 1.00 | 1.01 | 1.10 | 1.26 | 1.29 | 1.34 | 1.47 | 1.26 | 1.29 | 1.34 | 1.47 | 1.26 | 1.30 | 1.35 | 1.47 | 1.25 | 1.29 | 1.34 | 1.46 | |
| 0.6 | 1.00 | 1.00 | 1.00 | 1.00 | 1.14 | 1.17 | 1.21 | 1.33 | 1.14 | 1.17 | 1.21 | 1.33 | 1.14 | 1.17 | 1.22 | 1.33 | 1.13 | 1.17 | 1.21 | 1.32 | |
| 0.8 | 1.00 | 1.00 | 1.00 | 1.00 | 1.10 | 1.13 | 1.17 | 1.28 | 1.10 | 1.13 | 1.17 | 1.28 | 1.10 | 1.13 | 1.18 | 1.29 | 1.09 | 1.13 | 1.17 | 1.28 | |
| 1 | 1.00 | 1.00 | 1.00 | 1.00 | 1.08 | 1.12 | 1.16 | 1.27 | 1.08 | 1.12 | 1.16 | 1.27 | 1.09 | 1.12 | 1.16 | 1.27 | 1.08 | 1.11 | 1.15 | 1.26 | |
| 1.2 | 1.00 | 1.00 | 1.00 | 1.00 | 1.07 | 1.10 | 1.14 | 1.25 | 1.07 | 1.10 | 1.14 | 1.25 | 1.07 | 1.10 | 1.14 | 1.25 | 1.06 | 1.09 | 1.13 | 1.24 | |
| 1.4 | 1.00 | 1.00 | 1.00 | 1.00 | 1.00 | 1.03 | 1.07 | 1.17 | 1.00 | 1.03 | 1.07 | 1.17 | 1.01 | 1.04 | 1.08 | 1.18 | 1.00 | 1.03 | 1.07 | 1.17 | |
| 1.5 | 1.00 | 1.00 | 1.00 | 1.00 | 1.00 | 1.00 | 1.03 | 1.13 | 1.00 | 1.00 | 1.03 | 1.13 | 1.00 | 1.00 | 1.04 | 1.14 | 0.97 | 1.00 | 1.03 | 1.13 | |
| Not visible | Visible | ||||||||||||||||||||
| Angle | 0 | 30 | 45 | 60 | 90 | ||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Length | 4 | 5 | 6 | 10 | 4 | 5 | 6 | 10 | 4 | 5 | 6 | 10 | 4 | 5 | 6 | 10 | 4 | 5 | 6 | 10 | |
| Distance from surface | 0.4 | 1.02 | 1.02 | 1.04 | 1.11 | 1.02 | 1.04 | 1.06 | 1.13 | 1.16 | 1.18 | 1.20 | 1.28 | 1.35 | 1.37 | 1.40 | 1.49 | 1.55 | 1.58 | 1.61 | 1.72 |
| 0.5 | 1.00 | 1.00 | 1.02 | 1.08 | 1.00 | 1.01 | 1.03 | 1.10 | 1.13 | 1.15 | 1.17 | 1.25 | 1.31 | 1.34 | 1.36 | 1.46 | 1.51 | 1.54 | 1.57 | 1.68 | |
| 0.6 | 1.00 | 1.00 | 1.00 | 1.07 | 1.00 | 1.00 | 1.02 | 1.09 | 1.12 | 1.14 | 1.16 | 1.24 | 1.30 | 1.32 | 1.35 | 1.44 | 1.49 | 1.52 | 1.55 | 1.66 | |
| 0.8 | 1.00 | 1.00 | 1.00 | 1.07 | 1.00 | 1.00 | 1.02 | 1.09 | 1.11 | 1.13 | 1.16 | 1.23 | 1.29 | 1.32 | 1.34 | 1.44 | 1.49 | 1.51 | 1.55 | 1.65 | |
| 1 | 1.00 | 1.00 | 1.01 | 1.08 | 1.00 | 1.00 | 1.02 | 1.09 | 1.12 | 1.14 | 1.16 | 1.24 | 1.30 | 1.32 | 1.35 | 1.44 | 1.50 | 1.52 | 1.56 | 1.66 | |
| 1.2 | 1.00 | 1.00 | 1.00 | 1.07 | 1.00 | 1.00 | 1.02 | 1.09 | 1.11 | 1.13 | 1.16 | 1.24 | 1.30 | 1.32 | 1.35 | 1.44 | 1.49 | 1.52 | 1.55 | 1.66 | |
| 1.4 | 1.00 | 1.00 | 1.00 | 1.05 | 1.00 | 1.00 | 1.00 | 1.07 | 1.09 | 1.11 | 1.13 | 1.21 | 1.27 | 1.29 | 1.32 | 1.41 | 1.46 | 1.49 | 1.52 | 1.62 | |
| 1.5 | 1.00 | 1.00 | 1.00 | 1.03 | 1.00 | 1.00 | 1.00 | 1.04 | 1.07 | 1.09 | 1.11 | 1.19 | 1.24 | 1.26 | 1.29 | 1.38 | 1.43 | 1.45 | 1.48 | 1.59 | |
| Not visible | Visible | ||||||||||||||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jasiūnienė, E.; Vaitkūnas, T.; Šeštokė, J.; Griškevičius, P. Digital Image Correlation and Ultrasonic Lamb Waves for the Detection and Prediction of Crack-Type Damage in Fiber-Reinforced Polymer Composite Laminates. Polymers 2024, 16, 1980. https://doi.org/10.3390/polym16141980
Jasiūnienė E, Vaitkūnas T, Šeštokė J, Griškevičius P. Digital Image Correlation and Ultrasonic Lamb Waves for the Detection and Prediction of Crack-Type Damage in Fiber-Reinforced Polymer Composite Laminates. Polymers. 2024; 16(14):1980. https://doi.org/10.3390/polym16141980
Chicago/Turabian StyleJasiūnienė, Elena, Tomas Vaitkūnas, Justina Šeštokė, and Paulius Griškevičius. 2024. "Digital Image Correlation and Ultrasonic Lamb Waves for the Detection and Prediction of Crack-Type Damage in Fiber-Reinforced Polymer Composite Laminates" Polymers 16, no. 14: 1980. https://doi.org/10.3390/polym16141980
APA StyleJasiūnienė, E., Vaitkūnas, T., Šeštokė, J., & Griškevičius, P. (2024). Digital Image Correlation and Ultrasonic Lamb Waves for the Detection and Prediction of Crack-Type Damage in Fiber-Reinforced Polymer Composite Laminates. Polymers, 16(14), 1980. https://doi.org/10.3390/polym16141980







