Comparative Performance of Kevlar, Glass and Basalt Epoxy- and Elium-Based Composites under Static-, Low- and High-Velocity Loading Scenarios—Introduction to an Effective Recyclable and Eco-Friendly Composite
Abstract
1. Introduction
2. Literature Review
- -
- Delamination mode, which would occur when there existed a strong difference gradient in the bending stiffness between fiber layups [33]. Such delaminations would have an oblong appearance, with the longer axis being parallel to the fiber direction [34]. Dorey [34] stated that the development of delamination would be more likely in composites with a shorter length than longer. This observation, combined with that of Liu [33], is believed to be the most critical combination causing a delamination.
- -
- Matrix cracking mode mainly occurs when the absorbed energy leads to the formation of micro- and macrocracks. Unlike in delamination, matrix cracking occurs across fibers. This effect usually occurs when the impact energy is within a smaller range of values, usually below 5 J. It should be noted that damage to the matrix is usually the first form of damage during an impact event and is usually located in planes parallel to the fiber direction, caused by the existence of a differential gradient between the properties of the fiber and matrix [12]. These cracks are commonly categorized into two categories: (a) bending cracks and (b) shear cracks [35]. Bending cracks are usually formed perpendicular to the fibers and originate between fiber layers at the boundary of the fiber–matrix interface, whereas shear cracks, which are usually oriented at a 45-degree angle to the fibers, are formed as a result of large traverse shear stress often resulting from an impact [35].
- -
- Penetration mode occurs under concentrated high-impact energy levels, resulting in significant damage to both the matrix and the fibers. The significant damage to the matrix forces the fibers to sustain the load. Cantwell and Morton [36] found that the mode of penetration that has the highest energy absorption is the shear-out failure. This failure mode is recognized by a plug of material sheared out of the composite panel, absorbing between 50 and 60% of the impact energy, depending on the thickness of the composite.
3. Materials and Methods
3.1. Reinforcing Fibers
3.2. Resins
3.2.1. Elium© 150
3.2.2. West Systems Epoxy
4. Fabrication of the Composites
5. Experimental Investigations
5.1. Evaluation of the Basic Mechanical Properties
5.2. Impact Tests
5.2.1. Low-Velocity Impact Test
5.2.2. High-Velocity Impact Test
6. Results and Discussion
6.1. Basic Material and Mechanical Properties
6.1.1. Density and Void Content Results
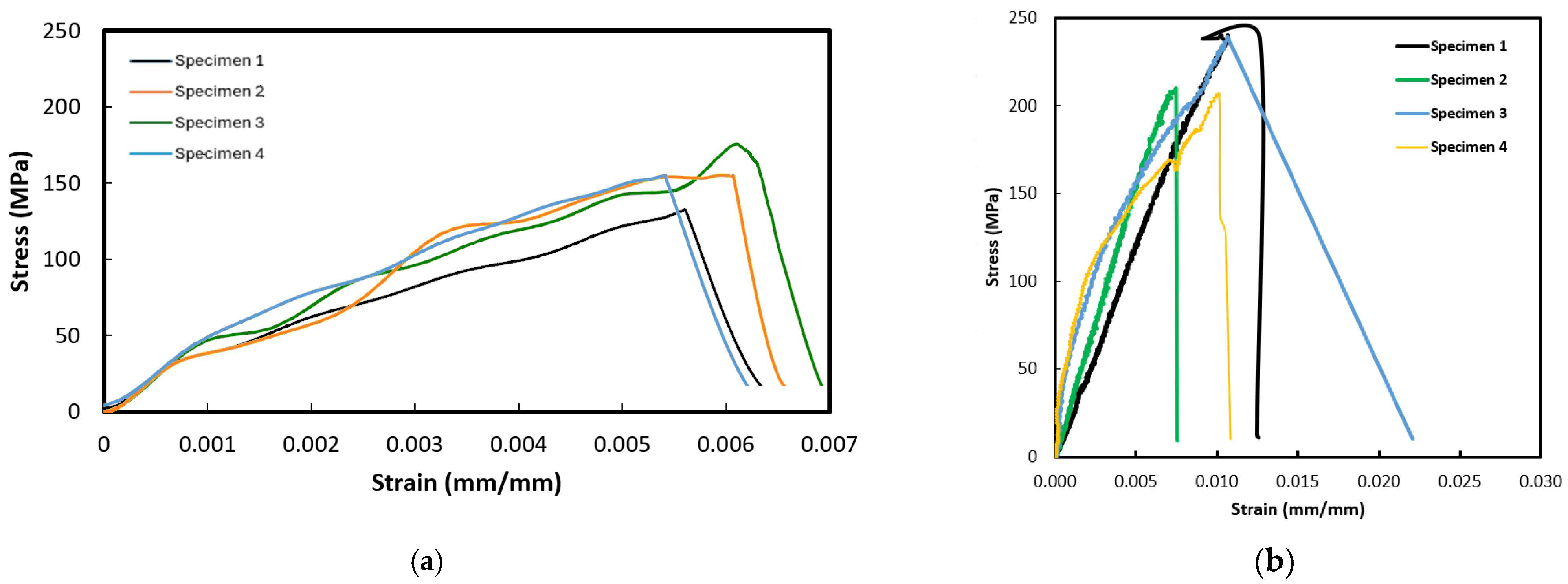
6.1.2. Tensile Test Results
6.1.3. Compression Test Results
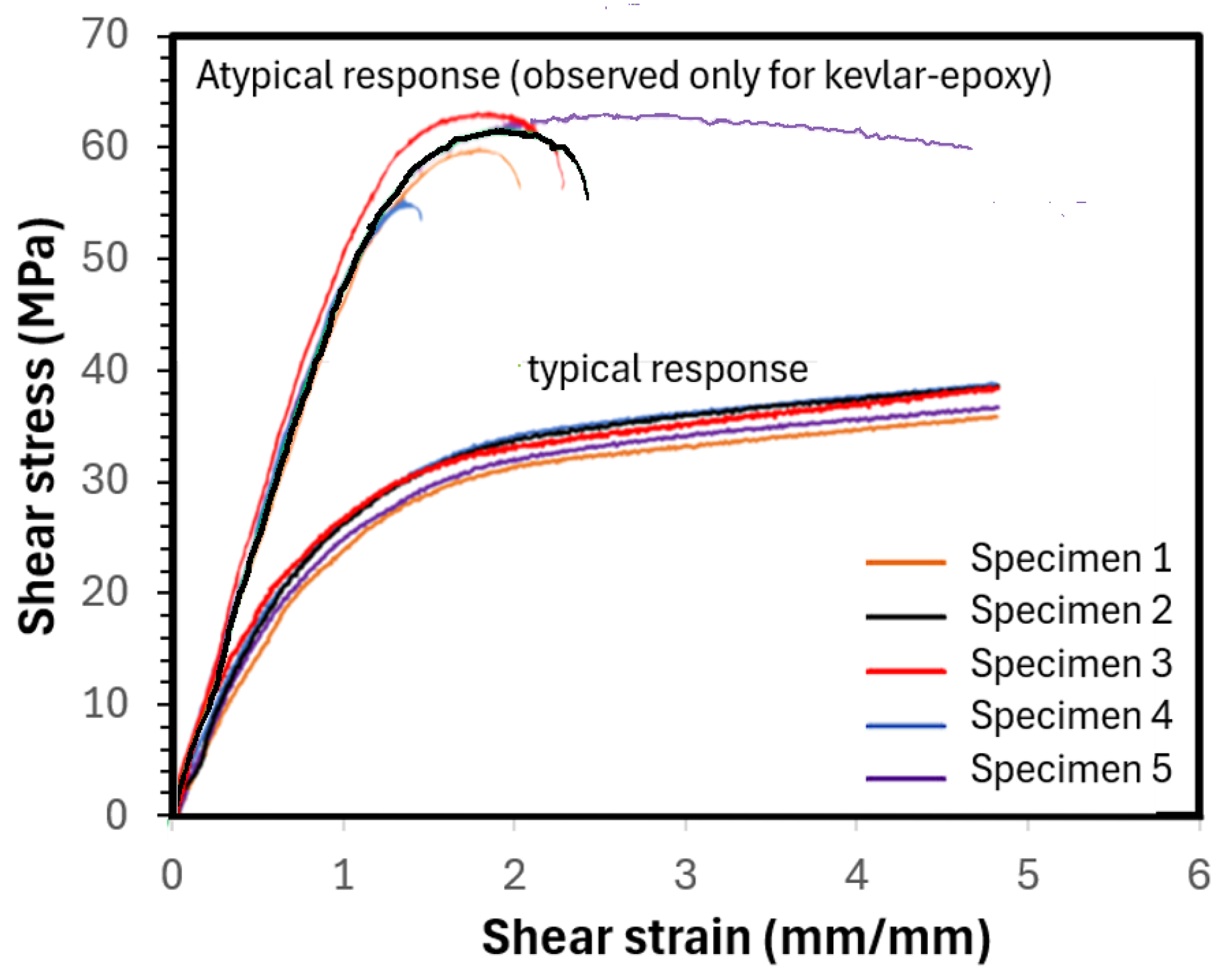
6.1.4. Shear Test Results
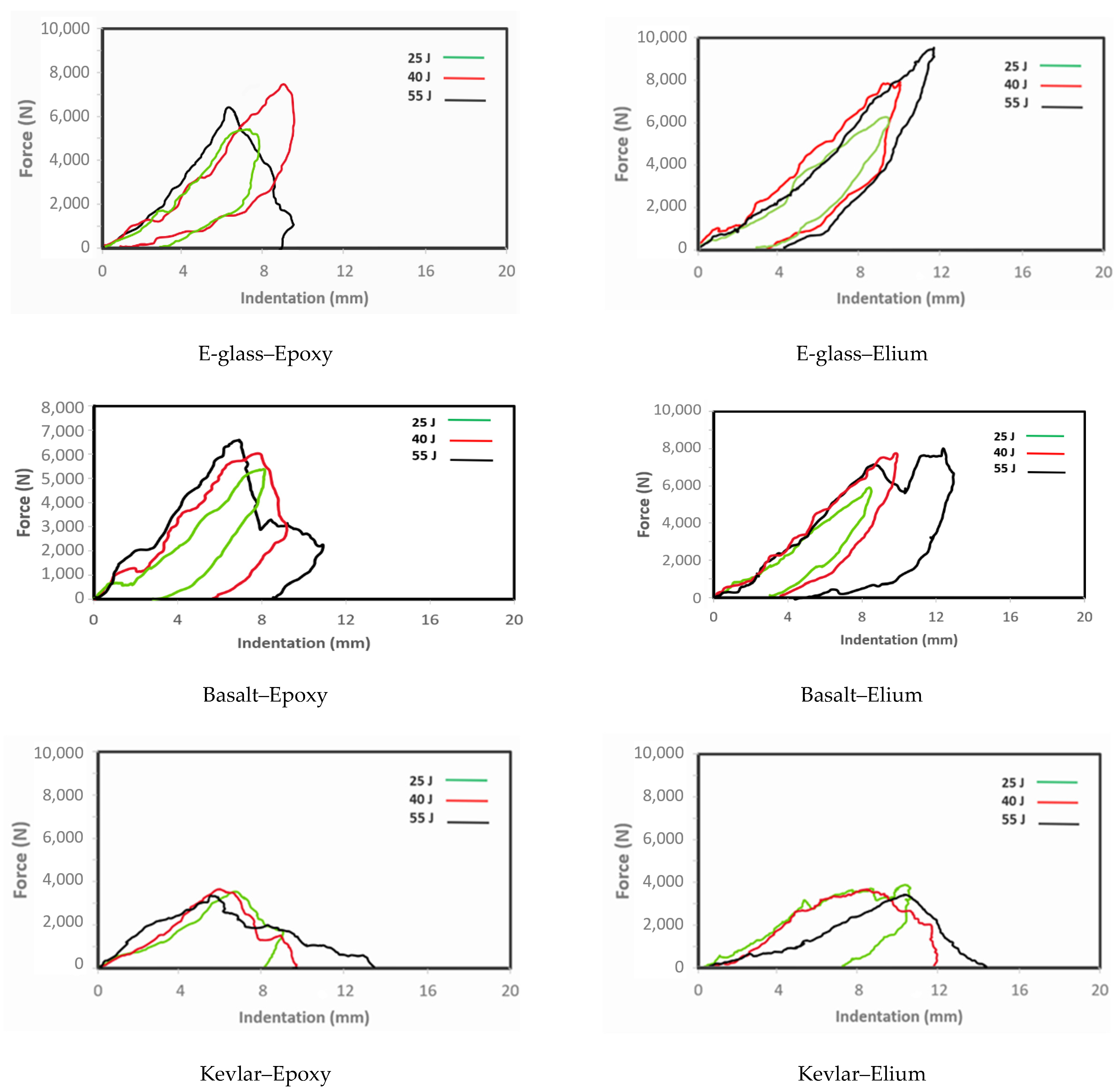
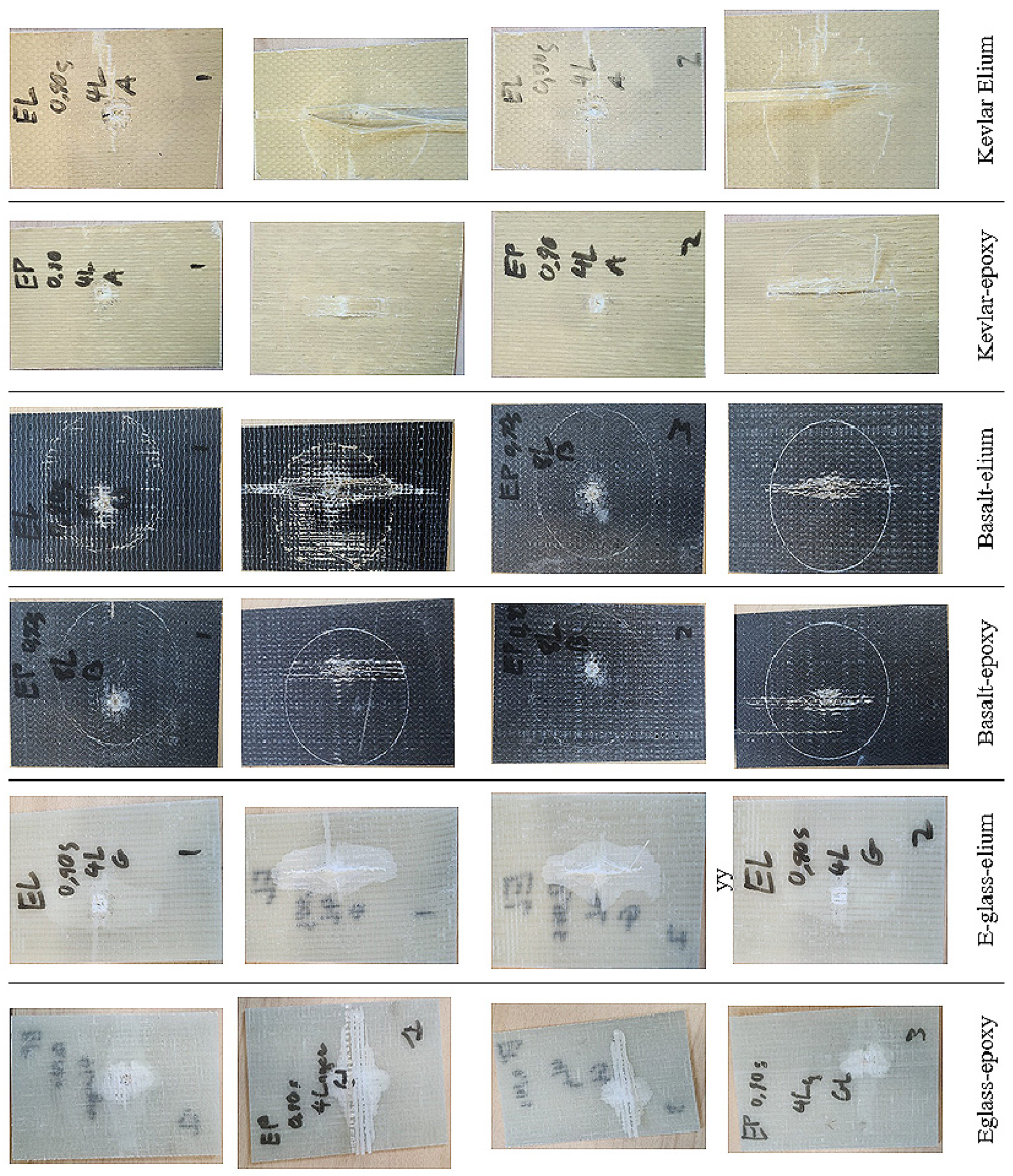
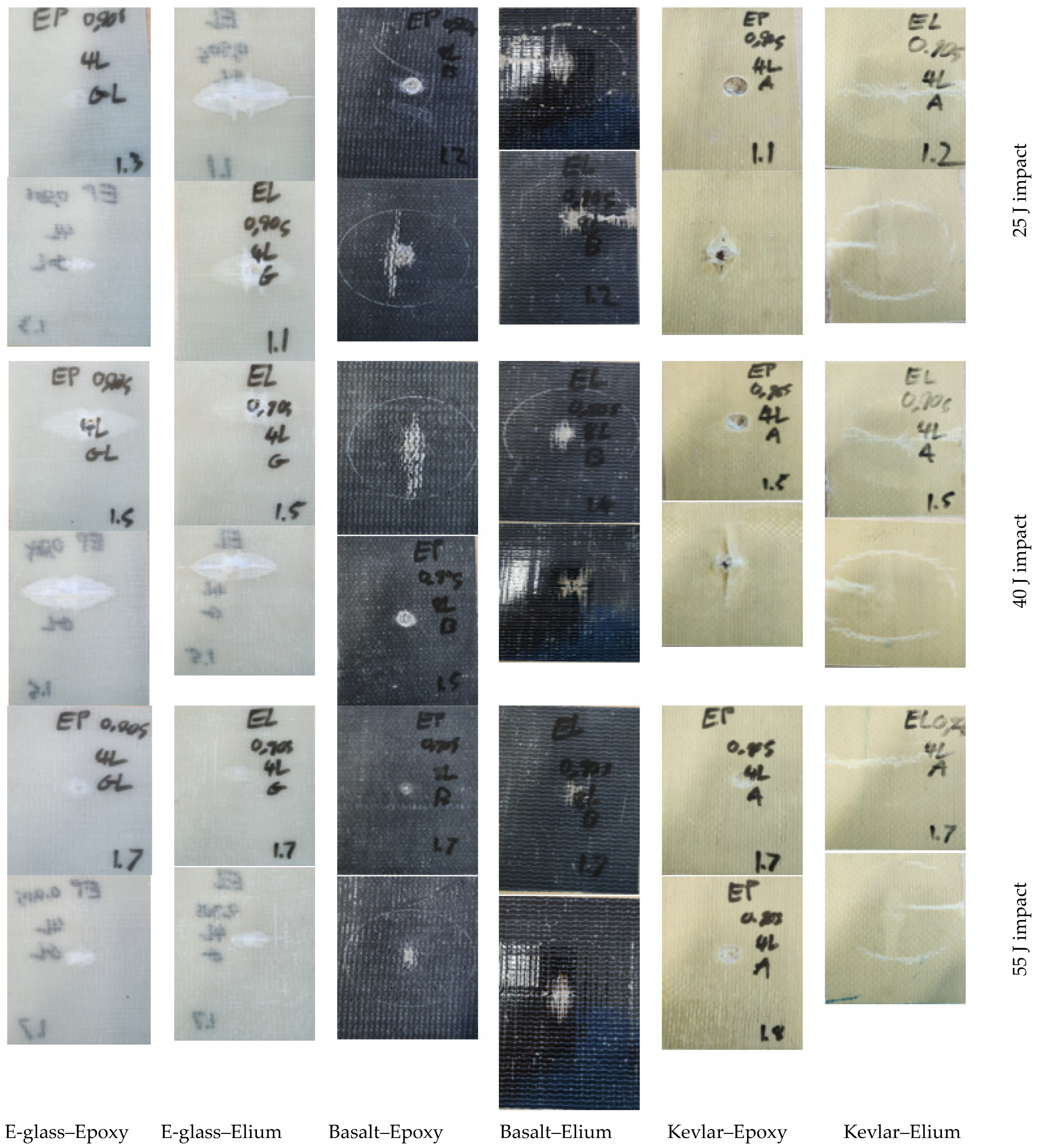
6.2. Low-Velocity Impact Test Results
6.3. High-Velocity Impact Test Results
7. Summary and Conclusions
- -
- All composites exhibited an average void content of approximately 7% and similar average fiber weight percentages, except for Kevlar–Elium, which had slightly higher fiber weight content.
- -
- Elium composites demonstrated superior tensile responses compared to epoxy composites.
- -
- Shear property evaluations revealed that both E-glass and basalt–Elium-based composites displayed higher ultimate shear strength and modulus values compared to their epoxy-based counterparts, with E-glass–Elium exhibiting the highest values. However, this trend was not observed in Kevlar composites.
- -
- Compressive property evaluations yielded mixed results: E-glass–Elium outperformed its epoxy counterpart, whereas basalt–epoxy composites outperformed basalt–Elium composites.
- -
- Elium-based composites exhibited significantly better low-velocity impact responses compared to epoxy-based composites. Basalt–Elium demonstrated the highest energy absorption capacity, with epoxy composites displaying more brittle failure modes and higher degrees of penetration during impact events. Kevlar-29–Elium experienced significant nonlinear and irreversible deformation under the highest impact energy, remaining unpenetrated. Some basalt–Elium specimens also remained unpenetrated, with smaller surface indentations compared to Kevlar–Elium.
- -
- High-velocity impact tests yielded results consistent with low-velocity impacts. Elium-based composites outperformed epoxy-based composites in both tests, with basalt–Elium achieving the highest ballistic limit. Similar failure modes were observed between low- and high-velocity tests, with Elium-based specimens exhibiting higher elastic-plastic deformation, while epoxy composites displayed brittle failure modes such as matrix cracking and projectile punch-through.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Singh, R.; Slipher, G. (Eds.) Mechanics of Composite and Multi-functional Materials, Proceedings of the 2019 Annual Conference on Experimental and Applied Mechanics, Volume 5; Springer: Cham, Switzerland, 2020. [Google Scholar] [CrossRef]
- Cheewaket, T.; Jaturapitakkul, C.; Chalee, W. Initial corrosion presented by chloride threshold penetration of concrete up to 10year-results under marine site. Constr. Build. Mater. 2012, 37, 693–698. [Google Scholar] [CrossRef]
- Basalt Continuous Fibers, n.d. Available online: http://web.archive.org/web/20091103234348/www.albarrie.com/techfabrics/continuousfiber.aspx (accessed on 9 May 2024).
- Kazemi, M.E.; Shanmugam, L.; Lu, D.; Wang, X.; Wang, B.; Yang, J. Mechanical properties and failure modes of hybrid fiber reinforced polymer composites with a novel liquid thermoplastic resin, Elium®. Compos. Part A Appl. Sci. Manuf. 2019, 125, 105523. [Google Scholar] [CrossRef]
- Raponi, O.D.A.; Barbosa, L.C.M.; de Souza, B.R.; Ancelotti Junior, A.C. Study of the influence of initiator content in the polymerization reaction of a thermoplastic liquid for advanced composite manufacturing. Adv. Polym. Technol. 2018, 37, 3579–3587. [Google Scholar] [CrossRef]
- Nash, N.H.; Portela, A.; Bachour-Sirerol, C.I.; Manolakis, I.; Comer, A.J. Effect of environmental conditioning on the properties of thermosetting- and thermoplastic-matrix composite materials by resin infusion for marine applications. Compos. B Eng. 2019, 177, 107271. [Google Scholar] [CrossRef]
- Murray, R.E.; Penumadu, D.; Cousins, D.; Beach, R.; Snowberg, D.; Berry, D.; Suzuki, Y.; Stebner, A. Manufacturing and Flexural Characterization of Infusion-Reacted Thermoplastic Wind Turbine Blade Subcomponents. Appl. Compos. Mater. 2019, 26, 945–961. [Google Scholar] [CrossRef]
- Murray, R.E.; Swan, D.; Snowberg, D.; Berry, D.; Beach, R.; Rooney, S. Manufacturing a 9-meter thermoplastic composite wind turbine blade. In Proceedings of the 32nd Technical Conference of the American Society for Composites, West Lafayette, Indiana, 23–25 October 2017; Volume 1, pp. 29–43. [Google Scholar] [CrossRef]
- Chilali, A.; Assarar, M.; Zouari, W.; Kebir, H.; Ayad, R. Mechanical characterization and damage events of flax fabric-reinforced biopolymer composites. Polym. Polym. Compos. 2020, 28, 631–644. [Google Scholar] [CrossRef]
- Bhudolia, S.K.; Joshi, S.C. Low-velocity impact response of carbon fibre composites with novel liquid Methylmethacrylate thermoplastic matrix. Compos. Struct. 2018, 203, 696–708. [Google Scholar] [CrossRef]
- Barbosa, L.C.M.; Bortoluzzi, D.B.; Ancelotti, A.C., Jr. Analysis of fracture toughness mode II and fractographic study of composites based on Elium® 150 thermoplastic matrix. Compos. B Eng. 2019, 175, 2. [Google Scholar] [CrossRef]
- Bhudolia, S.K.; Gohel, G.; Vasudevan, D.; Leong, K.F.; Gerard, P. On the mode II fracture toughness, failure, and toughening mechanisms of wholly thermoplastic composites with ultra-lightweight thermoplastic fabrics and innovative Elium® resin. Compos. Part A Appl. Sci. Manuf. 2022, 161, 107115. [Google Scholar] [CrossRef]
- Bhudolia, S.K.; Joshi, S.C.; Bert, A.; Di Boon, Y.D.; Makam, R.; Gohel, G. Flexural characteristics of novel carbon methylmethacrylate Composites. Compos. Commun. 2019, 13, 129–133. [Google Scholar] [CrossRef]
- Yaghoobi, H.; Taheri, F. Mechanical performance of a novel environmentally friendly basalt-elium® thermoplastic composite and its stainless steel-based fiber metal laminate. Polym. Compos. 2021, 42, 4660–4672. [Google Scholar] [CrossRef]
- Alshahrani, H.; Ahmed, A.; Kabrein, H.; Prakash, V.R.A. Mechanical Properties Study on Sandwich Composites of Glass Fiber Reinforced Plastics (GFRP) Using Liquid Thermoplastic Resin, Elium®: Preliminary Experiments. Coatings 2022, 12, 1423. [Google Scholar] [CrossRef]
- Allagui, S.; El Mahi, A.; Rebiere, J.L.; Beyaoui, M.; Bouguecha, A.; Haddar, M. Effect of recycling cycles on the mechanical and damping properties of flax fibre reinforced elium composite: Experimental and numerical studies. J. Renew. Mater. 2021, 9, 695. [Google Scholar] [CrossRef]
- Sahki, A. Development of Thermoplastic Composites Reinforced with Basalt and Glass Fabrics: Study of Their Durability and Recyclability; IMT-MINES ALES-IMT-Mines Alès Ecole Mines-Télécom: Alès, France, 2022. [Google Scholar]
- Bhudolia, S.K.; Gohel, G.; Fai, L.K.; Barsotti, R.J. Fatigue response of ultrasonically welded carbon/Elium® thermoplastic composites. Mater. Lett. 2020, 264, 2. [Google Scholar] [CrossRef]
- Bhudolia, S.K.; Perrotey, P.; Joshi, S.C. Enhanced vibration damping and dynamic mechanical characteristics of composites with novel pseudo-thermoset matrix system. Compos. Struct. 2017, 179, 502–513. [Google Scholar] [CrossRef]
- Bhudolia, S.K.; Gohel, G.; Joshi, S.C.; Leong, K.F. Quasi-static indentation response of core-shell particle reinforced novel NCCF/Elium® composites at different feed rates. Compos. Commun. 2020, 21, 100383. [Google Scholar] [CrossRef]
- Pinnoji, P.K.; Mahajan, P. Analysis of impact-induced damage and delamination in the composite shell of a helmet. Mater. Des. 2010, 31, 3716–3723. [Google Scholar] [CrossRef]
- Gohel, G.; Bhudolia, S.K.; Elisetty, S.B.S.; Leong, K.F.; Gerard, P. Development and impact characterization of acrylic thermoplastic composite bicycle helmet shell with improved safety and performance. Compos. B Eng. 2021, 221, 8. [Google Scholar] [CrossRef]
- Kazemi, M.E.; Shanmugam, L.; Li, Z.; Ma, R.; Yang, L.; Yang, J. Low-velocity impact behaviors of a fully thermoplastic composite laminate fabricated with an innovative acrylic resin. Compos. Struct. 2020, 250, 4. [Google Scholar] [CrossRef]
- Kazemi, M.E.; Shanmugam, L.; Dadashi, A.; Shakouri, M.; Lu, D.; Du, Z.; Hu, Y.; Wang, J.; Zhang, W.; Yank, L.; et al. Investigating the roles of fiber, resin, and stacking sequence on the low-velocity impact response of novel hybrid thermoplastic composites. Compos. Part. B 2021, 207, 1359–8368. [Google Scholar] [CrossRef]
- Bhudolia, S.K.; Gohel, G.; Kantipudi, J.; Leong, K.F.; Gerard, P. Manufacturing and investigating the load, energy and failure attributes of thin ply carbon/elium® thermoplastic hollow composites under low-velocity impact. Mater. Des. 2021, 206, 4. [Google Scholar] [CrossRef]
- Gohel, G.; Bhudolia, S.K.; Leong, K.F.; Gerard, P. Understanding the impact properties and damage phenomenon of ultra-lightweight all-thermoplastic compositructures. Int. J. Impact Eng. 2023, 172, 5. [Google Scholar] [CrossRef]
- Kinvi-Dossou, G.; Boumbimba, R.M.; Bonfoh, N.; Garzon-Hernandez, S.; Garcia-Gonzalez, D.; Gerard, P.; Arias, A. Innovative acrylic thermoplastic composites versus conventional composites: Improving the impact performances. Compos. Struct. 2019, 217, 1–13. [Google Scholar] [CrossRef]
- Libura, T.; Rusinek, A.; Rodrigue, M.B.; Kowalewski, Z.; Gerard, P. Influence of fatigue aging and fiber orientation on the high velocity impact resistance of glass woven reinforced Elium acrylic laminates. J. Compos. Adhes. 2022, 185–186. [Google Scholar]
- Cantwell, W.J.; Morton, J. The impact resistance of composite materials—A review. Composites 1991, 22, 347–362. [Google Scholar] [CrossRef]
- Richardson, M.O.W.; Wisheart, M.J. Review of low-velocity impact properties of composite materials. Compos. Part. A Appl. Sci. Manuf. 1996, 27, 1123–1131. [Google Scholar] [CrossRef]
- Robinson, P.; Davies, G.A.O. Impactor mass and specimen geometry effects in low velocity impact of laminated composites. Int. J. Impact Eng. 1992, 12, 189–207. [Google Scholar] [CrossRef]
- Yang, L.; Liao, Z.; Qiu, C.; Hong, Z.; Yang, J. Experimental study on the impact resistance and damage tolerance of thermoplastic FMLs. Thin-Walled Struct. 2024, 196, 111435. [Google Scholar] [CrossRef]
- Liu, D. Impact-induced delamination—A view of bending stiffness mismatching. Compos. Mater. 1988, 22, 674–692. [Google Scholar] [CrossRef]
- Dorey, G. Impact damage tolerance and assessment in advanced composite materials. In Seminar on Advanced Composites; UK Cranfield Institute of Technology: Cranfield, UK, 1986. [Google Scholar]
- Jih, C.J.; Sun, C.T. Prediction of Delamination in Composite Laminates Subjected to Low Velocity Impact. J. Compos. Mater. 1993, 27, 684–701. [Google Scholar] [CrossRef]
- Cantwell, W.J.; Morton, J. Geometrical effects in the low velocity impact response of CFRP. Compos. Struct. 1989, 12, 39–59. [Google Scholar] [CrossRef]
- Sela, N.; Ishai, O. Interlaminar fracture toughness and toughening of laminated composite materials: A review. Composites 1989, 20, 423–435. [Google Scholar] [CrossRef]
- Olsson, R. Mass criterion for wave controlled impact response of composite plates. Compos. Part. A Appl. Sci. Manuf. 2000, 31, 879–887. [Google Scholar] [CrossRef]
- Olsson, R. Closed form prediction of peak load and delamination onset under small mass impact. Compos. Struct. 2003, 59, 341–349. [Google Scholar] [CrossRef]
- Siva Kumar, K.; Balakrishna Bhat, T. Response of composite laminates on impact of high velocity projectiles. Key Eng. Mater. 1997, 141, 337–348. [Google Scholar] [CrossRef]
- Safri, S.N.A.; Sultan, M.T.H.; Yidris, N.; Mustapha, F. Low velocity and high velocity impact test on composite materials—A review. Int. J. Eng. Sci. 2014, 3, 50–60. [Google Scholar]
- Backman, M.E.; Goldsmith, W. The mechanics of penetration of projectiles into targets. Int. J. Eng. Sci. 1978, 16, 1–99. [Google Scholar] [CrossRef]
- Ferriter, E.A.; McCulloh, I.A.; deRosset, W. Techniques Used to Estimate Limit Velocity in Ballistics Testing with Small Sample Size. In Proceedings of the 13th Annual US Army Research Laboratory/United States Military Academy Technical Symposium, New York, NY, USA, 13–16 November 2005; pp. 72–95, ISBN 9781855734234. [Google Scholar]
- Reid, S.R.; Zhou, G. (Eds.) Impact Behaviour of Fibre-Reinforced Composite Materials and Structures; Elsevier: Amsterdam, The Netherlands, 2000. [Google Scholar]
- Mines, R.A.W.; Roach, A.M.; Jones, N. High velocity perforation behaviour of polymer composite laminates. Int. J. Impact Eng. 1999, 22, 561–588. [Google Scholar] [CrossRef]
- Aramid, K. Fiber Technical Guide-Dupont. Available online: https://www.dupont.com/content/dam/dupont/amer/us/en/safety/public/documents/en/Kevlar_Technical_Guide_0319.pdf (accessed on 18 July 2023).
- JPS Composite Materials. E-Glass & S-Glass Fabrics. Available online: https://jpscm.com/products/e-glass-s-glass/ (accessed on 18 July 2023).
- ELIUM® 150 Technical Datasheet. Liquid Thermoplastic Resin For Glass-Reinforced Composite; Arkema: Colombes, France, 2017. [Google Scholar]
- West System Epoxy System. Available online: https://www.westsystem.com (accessed on 12 November 2022).
- Llanos, J.G., Jr. Characterization of the Low- and High-Velocity Impact Performances of Fiber Reinforced Composites with Epoxy and Elium© Based Matrices. Master’s Thesis, Department of Mechanical Engineering, Dalhousie University, Halifax, NS, Canada, 2023. [Google Scholar]
- MIL-HDBK-17-1F Composite Materials Polymer Matrix, 1F-Polymer Matrix Composites Guidelines for Characterization of Structural Materials; U.S. Department of Defense: Washington, DC, USA, 2022.
- Adams, D.F. Test Methods for Composite Materials: Seminar Notes; Technomic Publishing Co.: Lancaster, PA, USA, 1996. [Google Scholar]
- ASTM D3039; Standard Test Method for Tensile Properties of Polymer Matrix Composite Materials. ASTM International: West Conshohocken, PA, USA; Philadelphia, PA, USA, 2017.
- ASTM-D3518; Standard Test Method for In-Plane Shear Response of Polymer Matrix Composite Materials by Tensile Test of a 45 Laminate. ASTM International: West Conshohocken, PA, USA; Philadelphia, PA, USA, 2007.
- ASTMD D3410; Standard Test Method for Compressive Properties of Polymer Matrix Composite Materials with Unsupported Gage Section by Shear Loading. ASTM International: West Conshohocken, PA, USA; Philadelphia, PA, USA, 2008.
- Wang, K.; Taheri, F. Comparison of the Low-Velocity Impact Responses and Compressive Residual Strengths of GLARE and a 3DFML. Polymers 2023, 15, 1723. [Google Scholar] [CrossRef]
- Sports, L. Competition Electronics Prochrono Ltd Ballistic Chronograph? Londero Sports n.d. Available online: https://www.londerosports.com/bows/competition-electronics-prochrono-ltd-ballistic-chronograph (accessed on 12 November 2022).
- Caldwell. Ballistic Precision Chronograph: Shooting Chronograph n.d. Available online: https://www.caldwellshooting.com/range-gear/chronographs-and-wind-meters/ballistic-precision-%0Achronograph/720001.html (accessed on 12 November 2022).
- ASTM D7136; Standard Test Method for Measuring the Damage Resistance of a Fiber-Reinforced Polymer Matrix Composite to a Drop-Weight Impact Event. ASTM International: West Conshohocken, PA, USA, 2005.
- ASTM D2734-16; Standard Test Methods for Void Content of Reinforced Plastics. ASTM International: West Conshohocken, PA, USA, 2016.


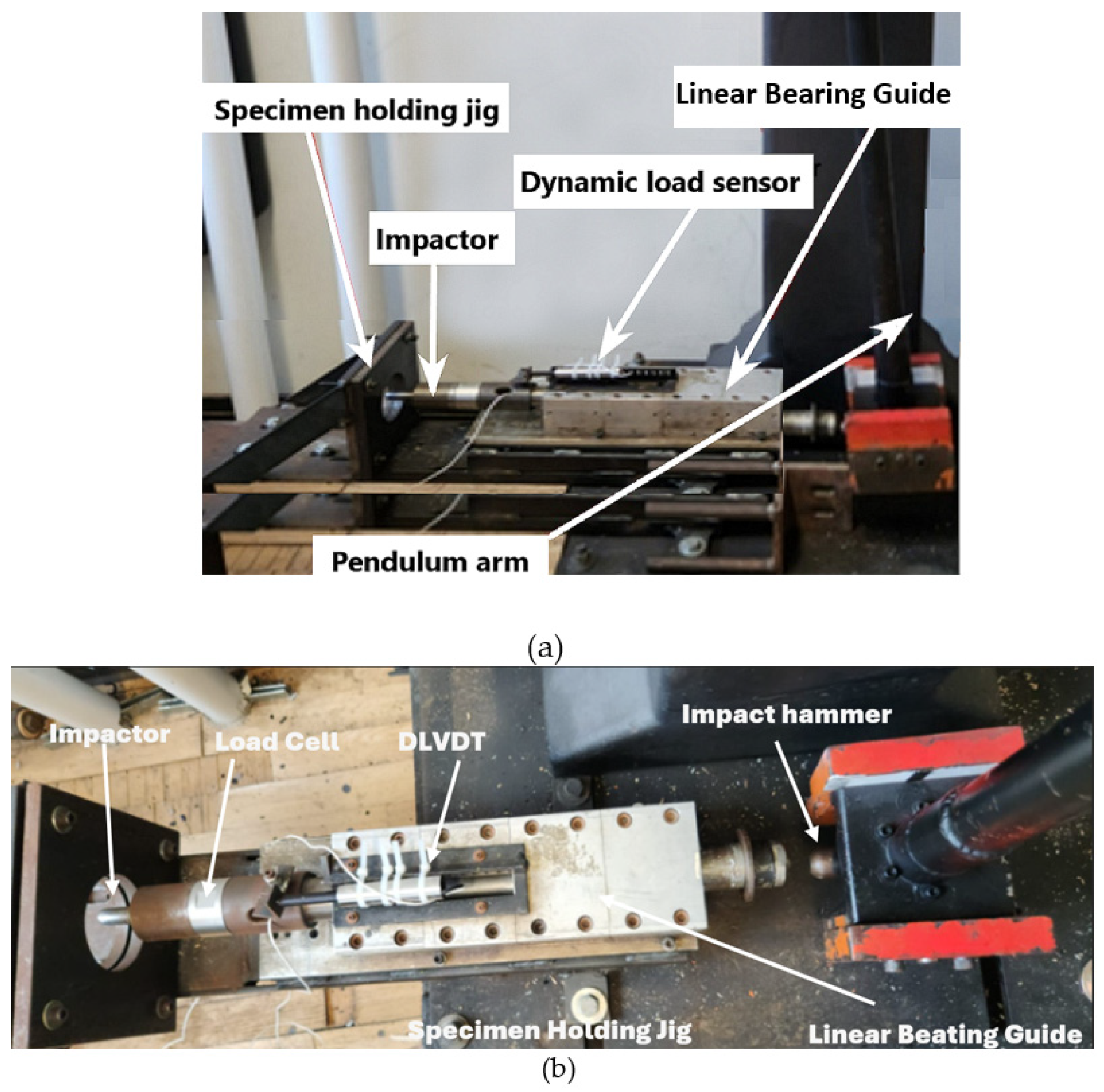
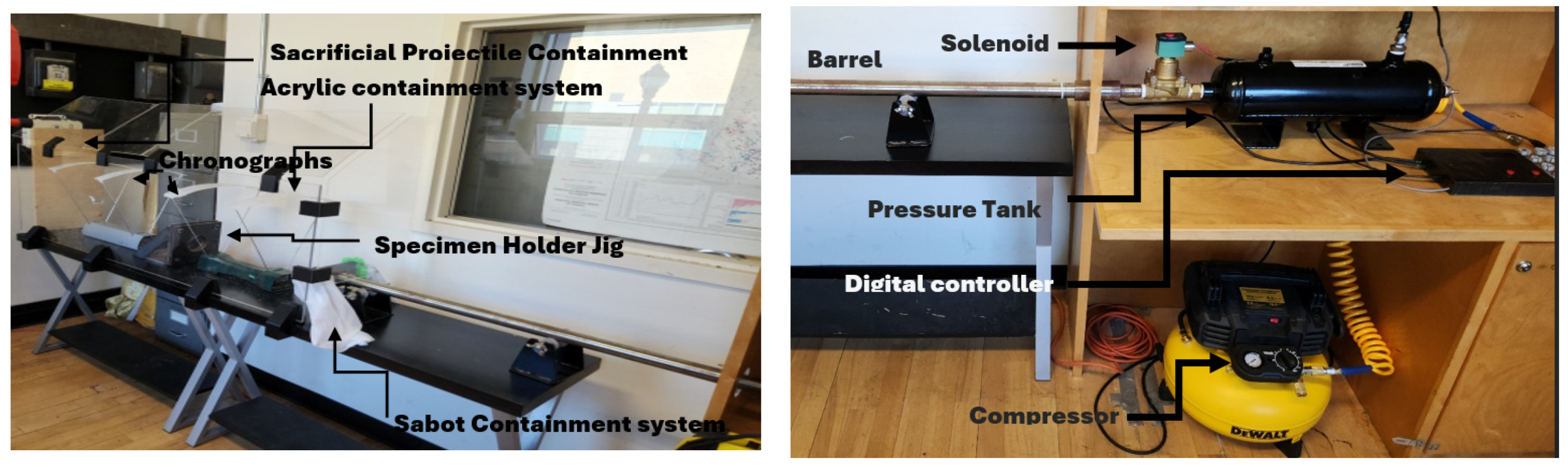
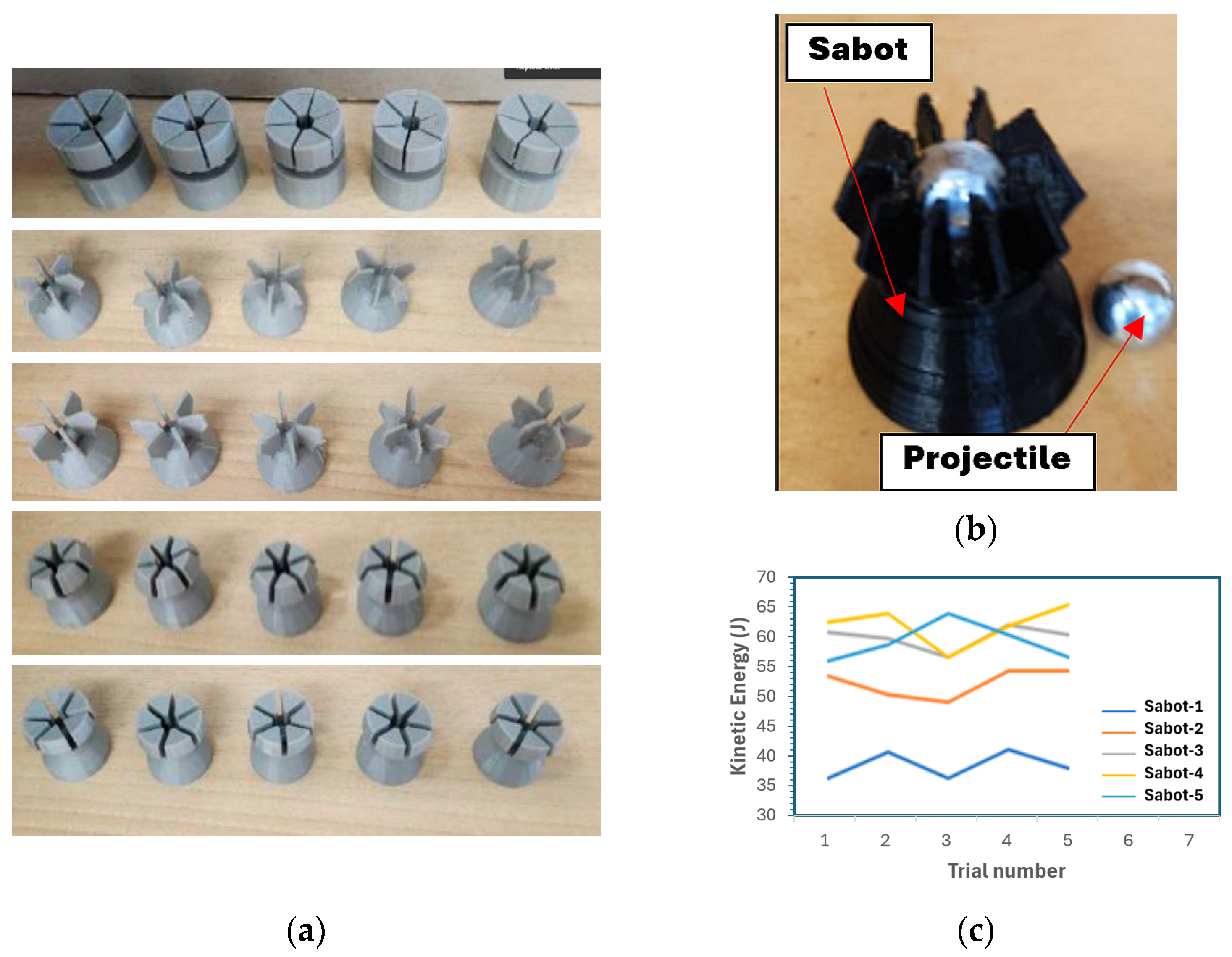
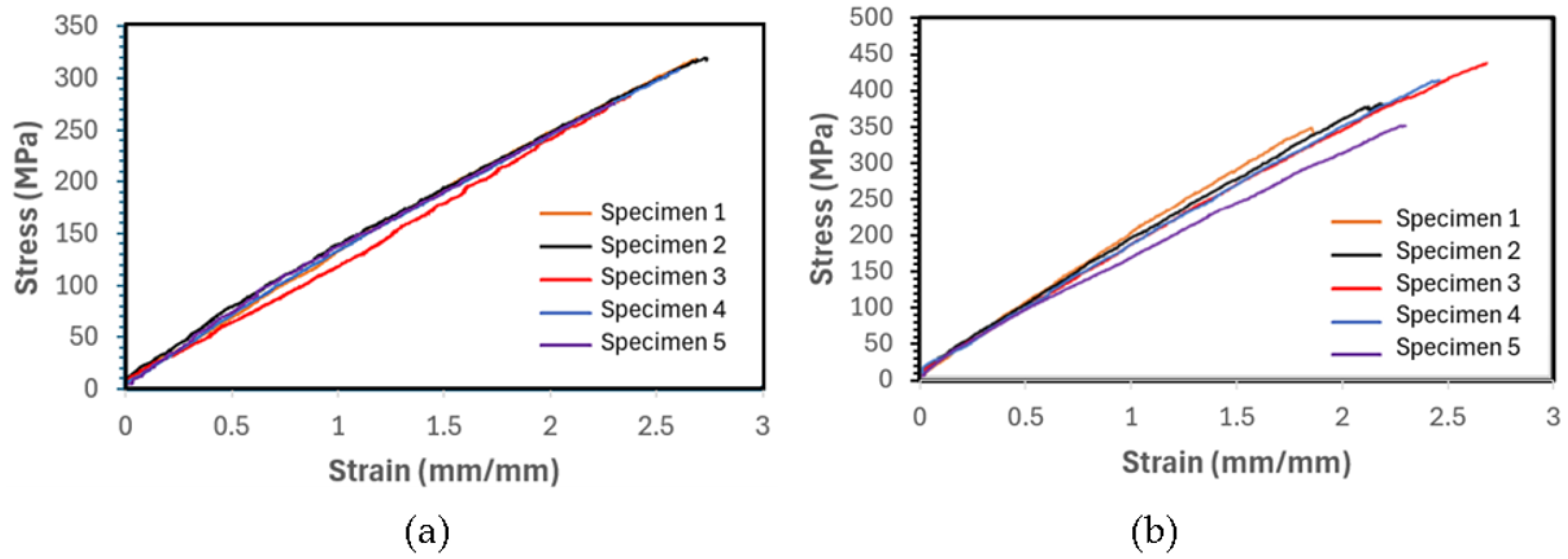

| Fiber | Tensile Strength (MPa) | Tensile Modulus (MPa) | Density g/(cm3) | Elongation at Break (%) | Reference |
|---|---|---|---|---|---|
| Kevlar-29 | 3600 | 83,000 | 1.44 | 3.60% | [46] |
| E-glass | 3400 | 72,000 | 2.54 | 4.70% | [47] |
| Basalt | 4840 | 89,000 | 2.70 | 3.15% | [3] |
| Property | Elium 150 | West Systems Epoxy |
|---|---|---|
| Shore D Hardness | 85–90 | 83 |
| Tensile Strength | 76 MPa | 50 MPa |
| Tensile Modulus | 3300 MPa | 3172 MPa |
| Tensile Deformation | 6% | 4.5% |
| Flexural Strength | 130 MPa | 81 MPa |
| Flexural Modulus | 3250 MPa | 3103 MPa |
| Compression Strength | 130 MPa | 79 MPa |
| Cured Specific Gravity | 1.19 | 1.18 |
| Liquid Viscosity | 100 mPa·s | 725 mPa·s |
| Material | Average Density (g/mL) | Void Content % | Average Fiber Volume Fraction | Average Resin Volume Fraction |
|---|---|---|---|---|
| E-glass–Epoxy | 1.49 | 4.68 | 0.29 | 0.66 |
| E-glass–Elium | 1.35 | 7.04 | 0.29 | 0.64 |
| Basalt–Epoxy | 1.59 | 6.54 | 0.32 | 0.61 |
| Basalt–Elium | 1.49 | 5.51 | 0.32 | 0.63 |
| Kevlar–Epoxy | 1.24 | 4.19 | 0.43 | 0.53 |
| Kevlar–Elium | 1.15 | 6.42 | 0.49 | 0.45 |
| Material | E-Glass–Epoxy | E-Glass–Elium | Basalt–Epoxy | Basalt–Elium | Kevlar-29–Epoxy | Kevlar-29–Elium |
|---|---|---|---|---|---|---|
| Tensile Strength (MPa) | 301.5 (23.4) * | 304.9 (2.33) | 298.5 (18.2) | 386.8 (27.4) | 463.2 (11.4) | 641.6 (9.8) |
| Tensile Modulus (GPa) | 11.073 (0.647) | 12.424 (0.475) | 13.854 (0.725) | 16.566 (0.996) | 27.828 (0.775) | 35.044 (0.894) |
| Material | E-Glass–Epoxy | E-Glass–Elium | Basalt–Epoxy | Basalt–Elium |
|---|---|---|---|---|
| Compressive Strength (MPa) | 218.25 (30.4) * | 419.96 (15.18) | 223.67 (16.07) | 146.51 (11.8) |
| Compressive Modulus (GPa) | 16.720 (0.533) | 27.232 (2.91) | 23.930 (3.515) | 27.779 (9.09) |
| Material | E-Glass–Epoxy | E-Glass–Elium | Basalt–Epoxy | Basalt–Elium | Kevlar-29–Epoxy | Kevlar-29–Elium |
|---|---|---|---|---|---|---|
| Shear Strength (MPa) | 47.9 (1.78) * | 69.8 (1.82) | 36.8 (1.16) | 39.9 (2.67) | 61.3 (2.82) | 11.2 (0.66) |
| Shear Modulus (GPa) | 3.145 (0.198) | 4.475 (0.273) | 2.677 (0.130) | 3.365 (0.097) | 4.221 (0.197) | 1.269 (0.063) |
| Material | Impact Energy (J) | Average Force (N) | Standard Deviation |
|---|---|---|---|
| E-glass–Epoxy | 55 | 7114.85 | 527.07 |
| 40 | 7511.58 | 44.96 | |
| 25 | 5982.90 | 735.46 | |
| E-glass–Elium | 55 | 8373.15 | 466.14 |
| 40 | 7892.95 | 260.80 | |
| 25 | 6631.65 | 443.14 | |
| Basalt–Epoxy | 55 | 7165.22 | 617.11 |
| 40 | 6886.80 | 244.49 | |
| 25 | 6029.54 | 88.67 | |
| Basalt–Elium | 55 | 8831.26 | 518.70 |
| 40 | 7942.06 | 718.62 | |
| 25 | 6484.40 | 511.77 | |
| Kevlar–Epoxy | 55 | 3051.58 | 670.51 |
| 40 | 4115.38 | 222.93 | |
| 25 | 3648.67 | 196.26 | |
| Kevlar–Elium | 55 | 3497.20 | 41.13 |
| 40 | 3885.79 | 52.61 | |
| 25 | 4634.42 | 517.83 |
| Material | Ballistic Limit Velocity—BLV (m/s) | Comparative * Energy Absorption Capacity (J) | % Increase in * Energy Absorption Capacity | Normalized BLV with Respect to Glass–Epoxy (m/s) |
|---|---|---|---|---|
| E-glass–Epoxy | 128.5 | - | - | 1 |
| E-glass–Elium | 131 | 1.14 | 1.95 | 1.02 |
| Basalt–Epoxy | 142.5 | - | - | 1.11 |
| Basalt–Elium | 148 | 2.80 | 3.86 | 1.15 |
| Kevlar–Epoxy | 116 | - | - | 0.90 |
| Kevlar–Elium | 122 | 2.50 | 5.17 | 0.95 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taheri, F.; Llanos, J.R.J.G. Comparative Performance of Kevlar, Glass and Basalt Epoxy- and Elium-Based Composites under Static-, Low- and High-Velocity Loading Scenarios—Introduction to an Effective Recyclable and Eco-Friendly Composite. Polymers 2024, 16, 1494. https://doi.org/10.3390/polym16111494
Taheri F, Llanos JRJG. Comparative Performance of Kevlar, Glass and Basalt Epoxy- and Elium-Based Composites under Static-, Low- and High-Velocity Loading Scenarios—Introduction to an Effective Recyclable and Eco-Friendly Composite. Polymers. 2024; 16(11):1494. https://doi.org/10.3390/polym16111494
Chicago/Turabian StyleTaheri, Farid, and Jesse R. J. G. Llanos. 2024. "Comparative Performance of Kevlar, Glass and Basalt Epoxy- and Elium-Based Composites under Static-, Low- and High-Velocity Loading Scenarios—Introduction to an Effective Recyclable and Eco-Friendly Composite" Polymers 16, no. 11: 1494. https://doi.org/10.3390/polym16111494
APA StyleTaheri, F., & Llanos, J. R. J. G. (2024). Comparative Performance of Kevlar, Glass and Basalt Epoxy- and Elium-Based Composites under Static-, Low- and High-Velocity Loading Scenarios—Introduction to an Effective Recyclable and Eco-Friendly Composite. Polymers, 16(11), 1494. https://doi.org/10.3390/polym16111494







