Acrylonitrile Butadiene Styrene-Based Composites with Permalloy with Tailored Magnetic Response
Abstract
1. Introduction
2. Experimental
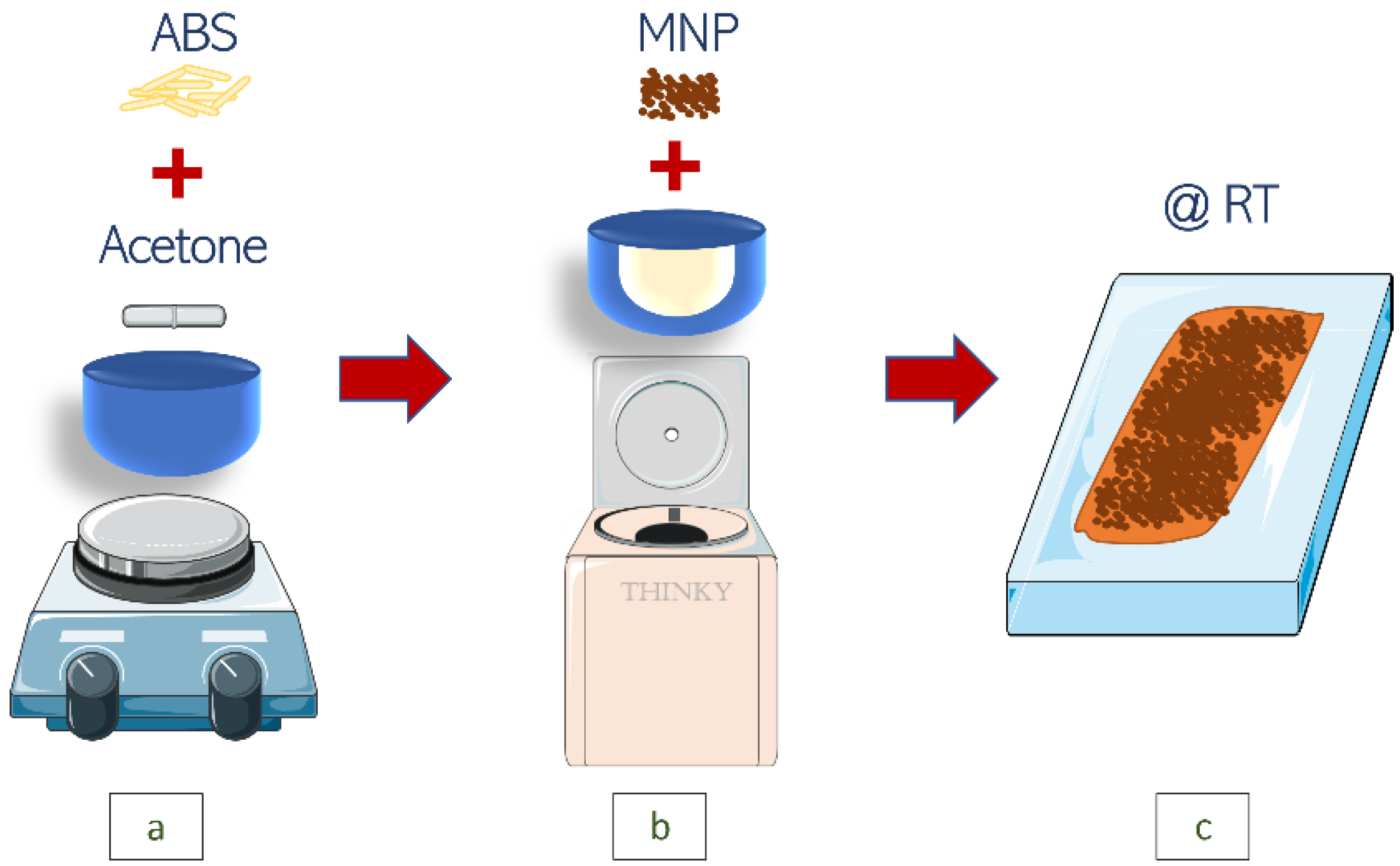
2.1. Materials and Sample Preparation
2.2. Sample Characterization
3. Results and Discussion
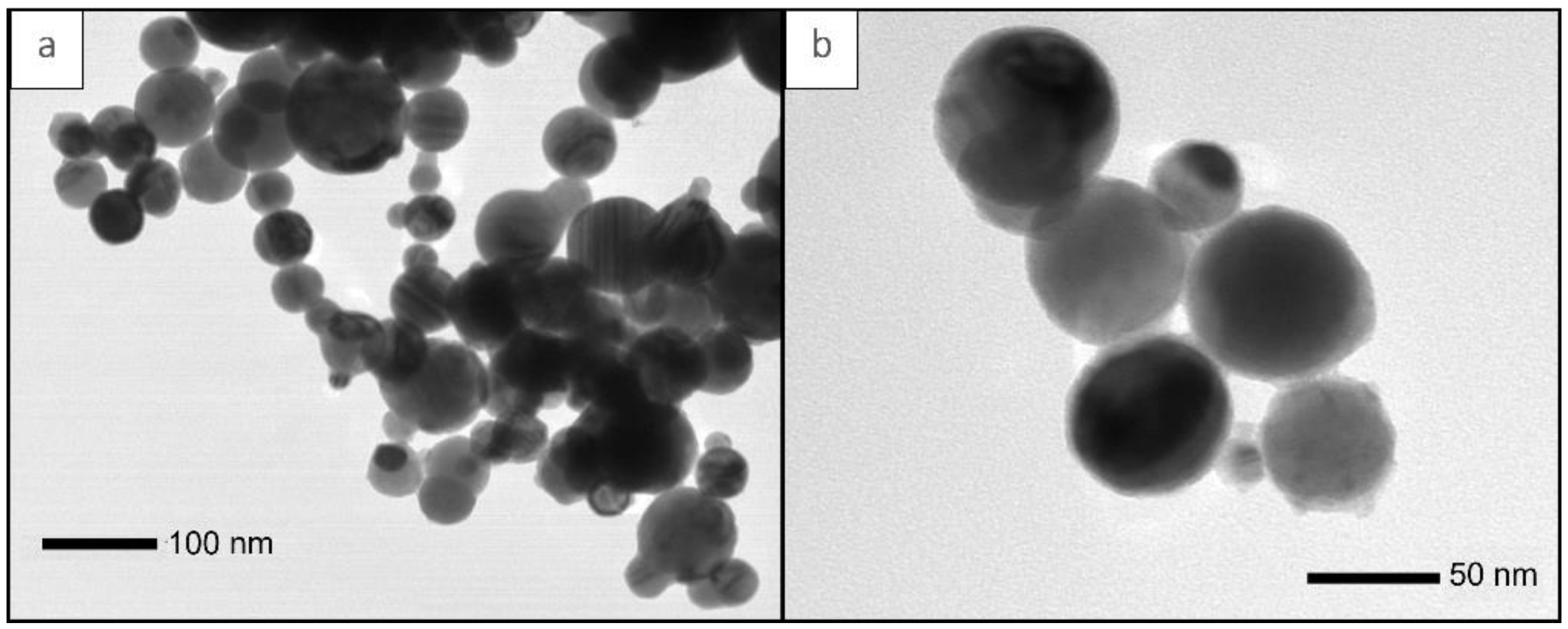
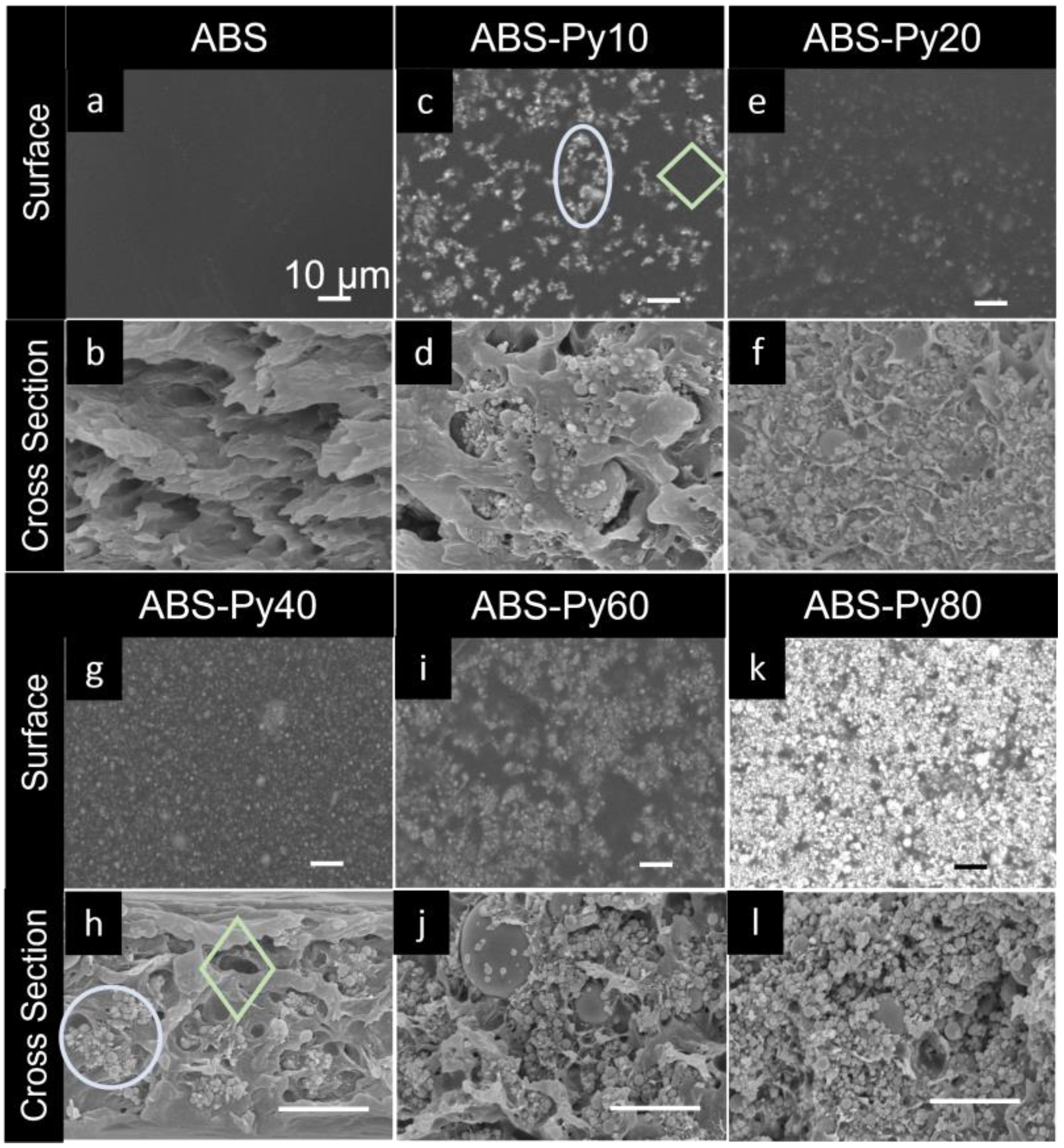
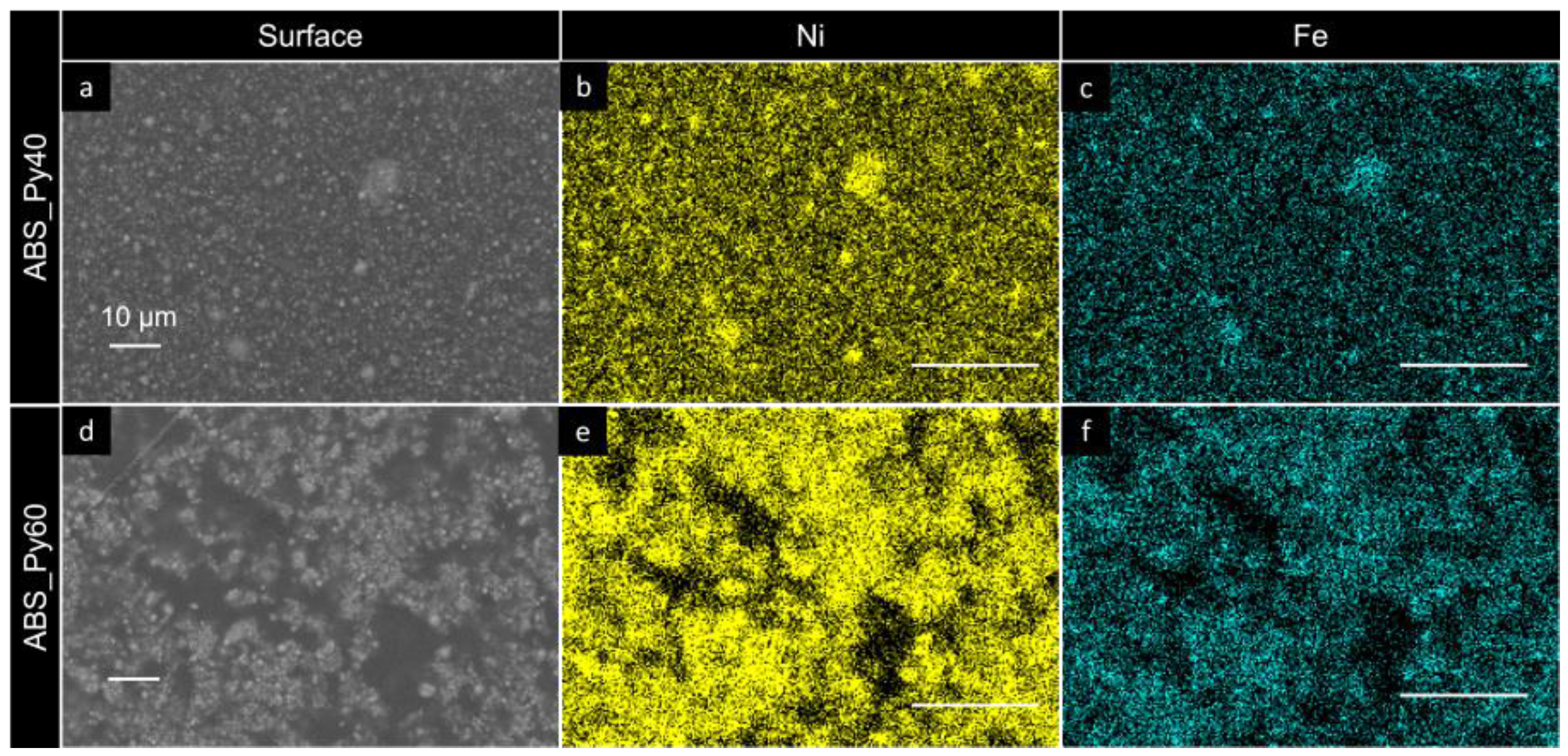
3.1. Morphological Features
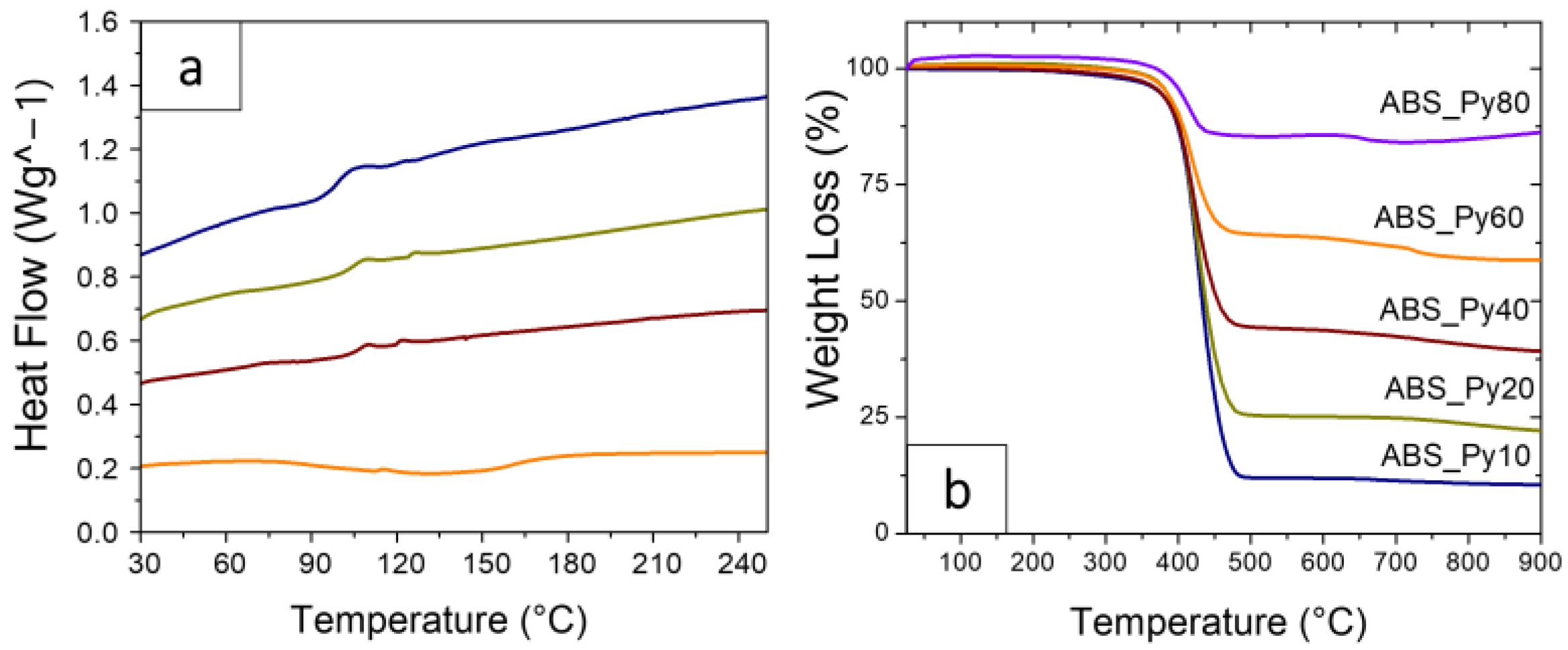
3.2. Thermal Properties
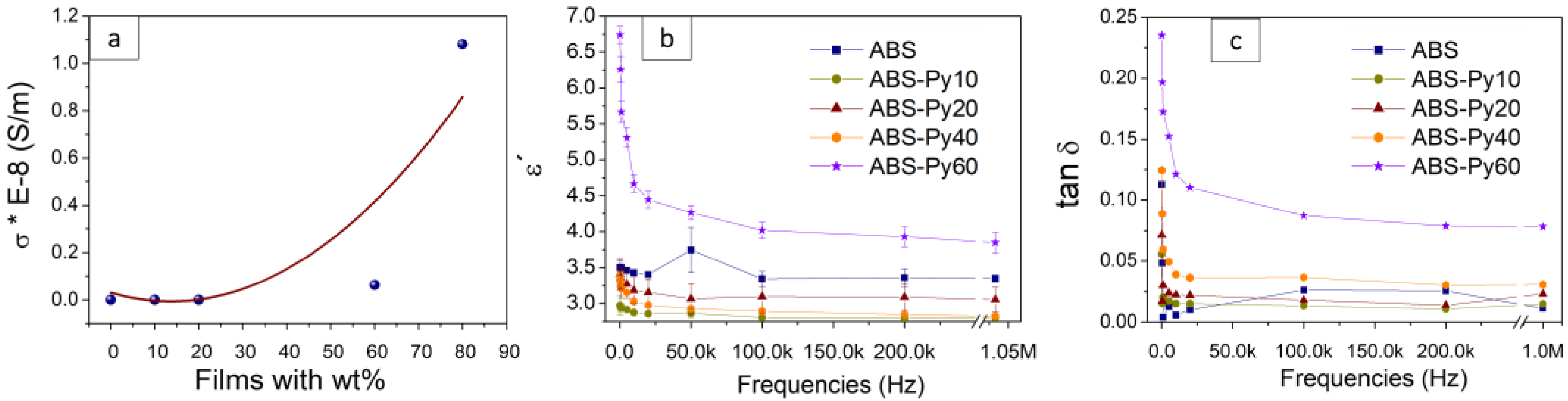
3.3. Electrical Conductivity
3.4. Dielectric Response
3.5. Mechanical Properties
3.6. Magnetic Response
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Hanemann, T.; Syperek, D.; Nötzel, D. 3D Printing of ABS Barium Ferrite Composites. Materials 2020, 13, 1481. [Google Scholar] [CrossRef]
- Khatri, B.; Lappe, K.; Noetzel, D.; Pursche, K.; Hanemann, T. A 3D-Printable Polymer-Metal Soft-Magnetic Functional Composite—Development and Characterization. Materials 2018, 11, 189. [Google Scholar] [CrossRef]
- Gass, J.; Poddar, P.; Almand, J.; Srinath, S.; Srikanth, H. Superparamagnetic Polymer Nanocomposites with Uniform Fe3O4 Nanoparticle Dispersions. Adv. Funct. Mater. 2005, 16, 71–75. [Google Scholar] [CrossRef]
- Jacimovic, J.; Binda, F.; Herrmann, L.G.; Greuter, F.; Genta, J.; Calvo, M.; Tomse, T.; Simon, R.A. Net Shape 3D Printed NdFeB Permanent Magnet. Adv. Eng. Mater. 2017, 19, 1700098. [Google Scholar] [CrossRef]
- Brito-Pereira, R.; Ribeiro, C.; Perinka, N.; Lanceros-Mendez, S.; Martins, P. Reconfigurable 3D-printable magnets with improved maximum energy producto. J. Mater. Chem. C 2020, 8, 952–958. [Google Scholar] [CrossRef]
- Wu, S.; Hu, W.; Ze, Q.; Sitti, M.; Zhao, R. Multifunctional magnetic soft composites: A review. Multifunct. Mater. 2020, 3, 042003. [Google Scholar] [CrossRef]
- Merazzo, K.J.; Lima, A.C.; Rincón-Iglesias, M.; Fernandes, L.C.; Pereira, N.; Lanceros-Mendez, S.; Martins, P. Magnetic materials: A journey from finding north to an exciting printed future. Mater. Horiz. 2021, 8, 2654–2684. [Google Scholar] [CrossRef]
- Haixia, L.; Zhiyong, H.; Geng, L.; Jican, L. Review of soft magnetic composite permanent magnet motor. In Proceedings of the International Symposium on Big Data and Artificial Intelligence, Hong Kong, China, 29–30 December 2018; pp. 294–298. [Google Scholar] [CrossRef]
- Rincón-Iglesias, M.; Salado, M.; Lanceros-Mendez, S.; Lizundia, E. Magnetically active nanocomposites based on biodegradable polylactide, polycaprolactone, polybutylene succinate and polybutylene adipate terephthalate. Polymer 2022, 249, 124804. [Google Scholar] [CrossRef]
- Xu, Y.; Gao, L.; Zhou, Y. Synthesis of trifunctional inorganic/organic hybrid nanocomposites and their applications for recognition and elimination of heavy metal ions. Appl. Surf. Sci. 2022, 605, 154659. [Google Scholar] [CrossRef]
- Stefan, M.; Leostean, C.; Popa, A.; Toloman, D.; Perhaita, I.; Cadis, A.; Macavei, S.; Pana, O. Highly stable MWCNT-CoFe2O4 photocatalyst. EGA-FTIR coupling as efficient tool to illustrate the formation mechanism. J. Alloys Compd. 2022, 928, 167188. [Google Scholar] [CrossRef]
- Du, X.; Zhang, L.; Guo, C.; Liu, G.; Yuan, H.; Li, Y.; Hu, W. FeCo/Graphene Nanocomposites for Applications as Electromagnetic Wave-Absorbing Materials. ACS Appl. Nano Mater. 2022, 5, 18730–18741. [Google Scholar] [CrossRef]
- Mendes-Felipe, C.; Garcia, A.; Salazar, D.; Vilas-Vilela, J.; Lanceros-Mendez, S. Photocurable magnetic materials with tailored functional properties. Compos. Part C Open Access 2021, 5, 100143. [Google Scholar] [CrossRef]
- Marques-Almeida, T.; Correia, V.; Martín, E.F.; Díez, A.G.; Ribeiro, C.; Lanceros-Mendez, S. Piezoelectric and Magnetically Responsive Biodegradable Composites with Tailored Porous Morphology for Biotechnological Applications. ACS Appl. Polym. Mater. 2022, 4, 8750–8763. [Google Scholar] [CrossRef]
- Hermenegildo, B.; Meira, R.; Correia, D.; Díez, A.; Ribeiro, S.; Serra, J.; Pérez-Álvarez, L.; Vilas-Vilela, J.; Lanceros-Méndez, S. Poly(lactic-co-glycolide) based biodegradable electrically and magnetically active microenvironments for tissue regeneration applications. Eur. Polym. J. 2022, 171, 111197. [Google Scholar] [CrossRef]
- Wan, X.; He, Y.; Liu, Y.; Leng, J. 4D printing of multiple shape memory polymer and nanocomposites with biocompatible, programmable and selectively actuated properties. Addit. Manuf. 2022, 53, 102689. [Google Scholar] [CrossRef]
- Bulgakova, A.; Chubarov, A.; Dmitrienko, E. Magnetic Nylon 6 Nanocomposites for the Microextraction of Nucleic Acids from Biological Samples. Magnetochemistry 2022, 8, 85. [Google Scholar] [CrossRef]
- Lum, G.Z.; Ye, Z.; Dong, X.; Marvi, H.; Erin, O.; Hu, W.; Sitti, M. Shape-programmable magnetic soft matter. Proc. Natl. Acad. Sci. USA 2016, 113, E6007–E6015. [Google Scholar] [CrossRef] [PubMed]
- Díez, A.G.; Tubio, C.R.; Gómez, A.; Berastegi, J.; Bou-Ali, M.M.; Etxebarria, J.G.; Lanceros-Mendez, S. Tuning magnetorheological functional response of thermoplastic elastomers by varying soft-magnetic nanofillers. Polym. Adv. Technol. 2022, 33, 2610–2619. [Google Scholar] [CrossRef]
- Biron, M. Thermoplastics and Thermoplastic Composites; Elsevier Science: Amsterdam, The Netherlands, 2007; ISBN 978-1-85617-478-7. [Google Scholar]
- Ching, Y.C.; Chuah, C.H.; Ching, K.Y.; Abdullah, L.C.; Rahman, A. Applications of thermoplastic-based blends. In Preparation and Characterisation; Elsevier: Amsterdam, The Netherlands, 2017; pp. 111–129. [Google Scholar] [CrossRef]
- Yadav, R.; Tirumali, M.; Wang, X.; Naebe, M.; Kandasubramanian, B. Polymer composite for antistatic application in aerospace. Def. Technol. 2019, 16, 107–118. [Google Scholar] [CrossRef]
- Banjanin, B.; Vladic, G.; Pál, M.; Balos, S.; Dramicanin, M.; Rackov, M.; Knezevic, I. Consistency analysis of mechanical properties of elements produced by FDM additive manufacturing technology. Matéria 2018, 23. [Google Scholar] [CrossRef]
- Sachdev, V.K.; Sharma, S.K.; Tomar, M.; Gupta, V.; Tandon, R.P. EMI shielding of MWCNT/ABS nanocomposites in contrast to graphite/ABS composites and MWCNT/PS nanocomposites. RSC Adv. 2016, 6, 45049–45058. [Google Scholar] [CrossRef]
- Jyoti, J.; Basu, S.; Singh, B.P.; Dhakate, S. Superior mechanical and electrical properties of multiwall carbon nanotube reinforced acrylonitrile butadiene styrene high performance composites. Compos. Part B Eng. 2015, 83, 58–65. [Google Scholar] [CrossRef]
- Ecco, L.G.; Dul, S.; Schmitz, D.P.; Barra, G.M.D.O.; Soares, B.G.; Fambri, L.; Pegoretti, A. Rapid Prototyping of Efficient Electromagnetic Interference Shielding Polymer Composites via Fused Deposition Modeling. Appl. Sci. 2018, 9, 37. [Google Scholar] [CrossRef]
- Gao, A.; Zhao, F.; Wang, F.; Zhang, G.; Zhao, S.; Cui, J.; Yan, Y. Highly conductive and light-weight acrylonitrile-butadiene-styrene copolymer/reduced graphene nanocomposites with segregated conductive structure. Compos. Part A Appl. Sci. Manuf. 2019, 122, 1–7. [Google Scholar] [CrossRef]
- Singh, R.; Sandhu, G.S.; Penna, R.; Farina, I. Investigations for Thermal and Electrical Conductivity of ABS-Graphene Blended Prototypes. Materials 2017, 10, 881. [Google Scholar] [CrossRef] [PubMed]
- Rabbi, M.F.; Chalivendra, V. Strain and damage sensing in additively manufactured CB/ABS polymer composites. Polym. Test. 2020, 90, 106688. [Google Scholar] [CrossRef]
- Wang, X. Investigation of Electromagnetic Shielding Effectiveness of Nanostructural Carbon Black/ABS Composites. J. Electromagn. Anal. Appl. 2011, 3, 160–164. [Google Scholar] [CrossRef]
- Schmitz, D.; Ecco, L.; Dul, S.; Pereira, E.; Soares, B.; Barra, G.; Pegoretti, A. Electromagnetic interference shielding effectiveness of ABS carbon-based composites manufactured via fused deposition modelling. Mater. Today Commun. 2018, 15, 70–80. [Google Scholar] [CrossRef]
- Jeong, S.; Song, Y.S.; Lim, E. Fabrication and Characterization of Aluminum Nanoparticle-Reinforced Composites. Polymers 2020, 12, 2772. [Google Scholar] [CrossRef]
- Hsu, Y.G.; Lin, F.J. Organic-inorganic composite materials from acrylonitrile-butadiene-styrene copolymers (ABS) and silica through anin situ sol-gel process. J. Appl. Polym. Sci. 2000, 75, 275–283. [Google Scholar] [CrossRef]
- Jiang, L.; Lam, Y.C.; Tam, M.K.; Chua, T.; Sim, G.; Ang, L. Strengthening acrylonitrile-butadiene-styrene (ABS) with nano-sized and micron-sized calcium carbonate. Polymer 2005, 46, 243–252. [Google Scholar] [CrossRef]
- Suetsuna, T.; Kinouchi, H.; Kawamoto, T.; Sanada, N. Soft magnetic composite containing magnetic flakes with in-plane uniaxial magnetic anisotropy. J. Magn. Magn. Mater. 2018, 473, 416–421. [Google Scholar] [CrossRef]
- Wang, Y.; Castles, F.; Grant, P.S. 3D Printing of NiZn ferrite/ABS Magnetic Composites for Electromagnetic Devices. MRS Proc. 2015, 1788, 29–35. [Google Scholar] [CrossRef]
- Ikeda, S.; Yamada, S. Magnetic particle composite materials for magnetic sensor made by fused deposition method. In Proceedings of the 2019 13th International Conference on Sensing Technology (ICST), Sydney, Australia, 2–4 December 2019; pp. 1–4. [Google Scholar] [CrossRef]
- Nabiyouni, G.; Ghanbari, D. Thermal, magnetic, and optical characteristics of ABS-Fe2O3 nanocomposites. J. Appl. Polym. Sci. 2012, 125, 3268–3274. [Google Scholar] [CrossRef]
- Thuyet-Nguyen, M.; Hai-Nguyen, H.; Kim, W.J.; Kim, H.Y.; Kim, J.-C. Synthesis and characterization of magnetic of Ni/ABS nanocomposites by electrical explosion of wire in liquid and solution blending methods. Met. Mater. Int. 2017, 23, 391–396. [Google Scholar] [CrossRef]
- Olekšáková, D.; Kollár, P.; Füzer, J.; Kusý, M.; Roth, S.; Polanski, K. The influence of mechanical milling on structure and soft magnetic properties of NiFe and NiFeMo alloys. J. Magn. Magn. Mater. 2007, 316, e838–e841. [Google Scholar] [CrossRef]
- Slovenský, P.; Kollár, P.; Jakubčin, M.; Füzer, J.; Olekšáková, D.; Fáberová, M.; Bureš, R. Characterization of Structure and Magnetic Properties of Warm Compacted Ni-Fe-Mo Soft Magnetic Alloy. Acta Phys. Pol. A 2020, 137, 876–878. [Google Scholar] [CrossRef]
- Jiles, D. Introduction to Magnetism and Magnetic Materials; CRC Press: Boca Raton, FL, USA, 1998; p. 354. ISBN 978-0-412-79860-3. [Google Scholar]
- Wienecke, A.; Müller-Weihrich, K.; Rissing, L. Investigation of Electrodeposited Nifemo for Application as Flux Concentrator in Magnetic Field Sensors. In ECS Meeting Abstracts; IOP Publishing: Chiyoda, Tokyo, 2014; p. 904. [Google Scholar] [CrossRef]
- ESPI Metals. Permalloy 80. Available online: https://www.espimetals.com/index.php/technical-data/175-permalloy-80 (accessed on 26 December 2022).
- Lee, S.-Y.; Lim, Y.-S.; Choi, I.-H.; Lee, D.-I.; Kim, S.-B. Effective Combination of Soft Magnetic Materials for Magnetic Shielding. IEEE Trans. Magn. 2012, 48, 4550–4553. [Google Scholar] [CrossRef]
- Sun, Z.; Reisner, M.; Fierlinger, P.; Schnabel, A.; Stuiber, S.; Li, L. Dynamic modeling of the behavior of permalloy for magnetic shielding. J. Appl. Phys. 2016, 119, 193902. [Google Scholar] [CrossRef]
- Park, J.Y.; Allen, M.G. Development of magnetic materials and processing techniques applicable to integrated micromagnetic devices. J. Micromech. Microeng. 1998, 8, 307–316. [Google Scholar] [CrossRef]
- Kwiatkowski, W.; Tumanski, S. The perma lloy mag netoresistive sensors-properties and applications. J. Phys. E Sci. Instrum. 1986, 19, 502. [Google Scholar] [CrossRef]
- Taylor, W.P.; Schneider, M.; Baltes, H.; Allen, M.G. A NiFeMo Electroplating Bath for Micromachined Structures. Electrochem. Solid-State Lett. 1999, 2, 624–626. [Google Scholar] [CrossRef]
- Sun, N.X.; Crawford, A.M.; Wang, S.X. Advanced Soft Magnetic Materials for Magnetic Recording Heads and Integrated Inductors. MRS Online Proc. Libr. (OPL) 2002, 721, 63. [Google Scholar] [CrossRef]
- Maat, S.; Marley, A.C. Physics and Design of Hard Disk Drive Magnetic Recording Read Heads. In Handbook of Spintronics; Springer: Dordrecht, The Netherlands, 2016; pp. 977–1028. [Google Scholar]
- Mendes-Felipe, C.; Rodrigues-Marinho, T.; Vilas, J.L.; Lanceros-Mendez, S. UV curable nanocomposites with tailored dielectric response. Polymer 2020, 196, 122498. [Google Scholar] [CrossRef]
- Castro, N.; Reis, S.; Silva, M.P.; Correia, V.; Lanceros-Mendez, S.; Martins, P. Development of a contactless DC current sensor with high linearity and sensitivity based on the magnetoelectric effect. Smart Mater. Struct. 2018, 27, 065012. [Google Scholar] [CrossRef]
- Pereira, N.; Lima, A.C.; Correia, V.; Peřinka, N.; Lanceros-Mendez, S.; Martins, P. Magnetic Proximity Sensor Based on Magnetoelectric Composites and Printed Coils. Materials 2020, 13, 1729. [Google Scholar] [CrossRef] [PubMed]
- Reizabal, A.; Costa, C.M.; Pereira, N.; Pérez-Álvarez, L.; Vilas-Vilela, J.-L.; Lanceros-Méndez, S. Silk Fibroin Based Magnetic Nanocomposites for Actuator Applications. Adv. Eng. Mater. 2020, 22, 2000111. [Google Scholar] [CrossRef]
- Šupová, M.; Martynková, G.S.; Barabaszová, K. Effect of Nanofillers Dispersion in Polymer Matrices: A Review. Sci. Adv. Mater. 2011, 3, 1–25. [Google Scholar] [CrossRef]
- Phenrat, T.; Saleh, N.; Sirk, K.; Tilton, R.D.; Lowry, G.V. Aggregation and Sedimentation of Aqueous Nanoscale Zerovalent Iron Dispersions. Environ. Sci. Technol. 2006, 41, 284–290. [Google Scholar] [CrossRef]
- Golas, P.L.; Louie, S.; Lowry, G.V.; Matyjaszewski, K.; Tilton, R.D. Comparative Study of Polymeric Stabilizers for Magnetite Nanoparticles Using ATRP. Langmuir 2010, 26, 16890–16900. [Google Scholar] [CrossRef]
- Sohel, A.; Mandal, A.; Mondal, A.; Pan, S.; Sengupta, A. Thermal analysis of ABS/PA6 polymer blend using differential scanning calorimetry. J. Therm. Anal. Calorim. 2017, 129, 1689–1695. [Google Scholar] [CrossRef]
- Chen, F.; Clough, A.; Reinhard, B.M.; Grinstaff, M.W.; Jiang, N.; Koga, T.; Tsui, O.K.C. Glass Transition Temperature of Polymer–Nanoparticle Composites: Effect of Polymer–Particle Interfacial Energy. Macromolecules 2013, 46, 4663–4669. [Google Scholar] [CrossRef]
- Suzuki, M.; Wilkie, C. The termal degradation of acrylonitrile-butadiene-styrene terpolymer as studied by TGA/FTIR. Polym. Degrad. Stab. 1995, 47, 217–221. [Google Scholar] [CrossRef]
- Guittoum, A.; Bourzami, A.; Layadi, A.; Schmerber, G. Structural, electrical and magnetic properties of evaporated permalloy thin films: Effect of substrate and thickness. Eur. Phys. J. Appl. Phys. 2012, 58, 20301. [Google Scholar] [CrossRef]
- Deng, H.; Lin, L.; Ji, M.; Zhang, S.; Yang, M.; Fu, Q. Progress on the morphological control of conductive network in conductive polymer composites and the use as electroactive multifunctional materials. Prog. Polym. Sci. 2014, 39, 627–655. [Google Scholar] [CrossRef]
- Costa, P.; Carvalho, M.F.; Correia, V.; Viana, J.C.; Lanceros-Mendez, S. Polymer Nanocomposite-Based Strain Sensors with Tailored Processability and Improved Device Integration. ACS Appl. Nano Mater. 2018, 1, 3015–3025. [Google Scholar] [CrossRef]
- Barde, W.S.; Pakade, S.V.; Yawale, S.P. Ionic conductivity in polypyrrole–poly (vinyl acetate) films synthesized by chemical oxidative polymerization method. J. Non-Cryst. Solids 2007, 353, 1460–1465. [Google Scholar] [CrossRef]
- Goswami, L.; Sarma, N.S.; Chowdhury, D. Determining the Ionic and Electronic Contribution in Conductivity of Polypyrrole/Au Nanocomposites. J. Phys. Chem. C 2011, 115, 19668–19675. [Google Scholar] [CrossRef]
- Markarian, J. New developments in antistatic and conductive additives. Plast. Addit. Compd. 2008, 10, 22–25. [Google Scholar] [CrossRef]
- Qi, B.; Yao, T.-P.; Zhang, Y.-D.; Shang, H.-K. Endowing the sustainable antistatic properties to epoxy-based composites through adding graphene nanoplatelets. J. Clean. Prod. 2020, 281, 124594. [Google Scholar] [CrossRef]
- da Silva, T.F.; Menezes, F.; Montagna, L.S.; Lemes, A.P.; Passador, F.R. Preparation and characterization of antistatic packaging for electronic components based on poly(lactic acid)/carbon black composites. J. Appl. Polym. Sci. 2019, 136, 47273. [Google Scholar] [CrossRef]
- Costa, P.; Nunes-Pereira, J.; Pereira, N.; Castro, N.; Gonçalves, S.; Lanceros-Mendez, S. Recent Progress on Piezoelectric, Pyroelectric, and Magnetoelectric Polymer-Based Energy-Harvesting Devices. Energy Technol. 2019, 7, 1800852. [Google Scholar] [CrossRef]
- Brito-Pereira, R.; Rodrigues-Marinho, T.; Tubio, C.R.; Costa, P.; Lanceros-Mendez, S. High-dielectric mouldable and printable wax reinforced with ceramic nanofillers and its suitability for capacitive sensing. Flex. Print. Electron. 2021, 6, 035005. [Google Scholar] [CrossRef]
- Costa, P.; Silva, J.; Lanceros Mendez, S. Strong increase of the dielectric response of carbon nanotube/poly(vinylidene fluoride) composites induced by carbon nanotube type and pre-treatment. Compos. Part B Eng. 2016, 93, 310–316. [Google Scholar] [CrossRef]
- Kim, Y.D.; Song, I.C. Electrorheological and dielectric properties of polypyrrole dispersions. J. Mater. Sci. 2002, 37, 5051–5055. [Google Scholar] [CrossRef]
- Balani, K.; Verma, V.; Agarwal, A.; Narayan, R. (Eds.) Physical, thermal, and mechanical properties of polymers. In Biosurfaces: A Materials Science and Engineering Perspective, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2015. [Google Scholar]
- Papageorgiou, D.G.; Kinloch, I.A.; Young, R.J. Mechanical properties of graphene and graphene-based nanocomposites. Prog. Mater. Sci. 2017, 90, 75–127. [Google Scholar] [CrossRef]
- Fu, S.; Feng, X.-Q.; Lauke, B.; Mai, Y. Effects of particle size, particle/matrix interface adhesion and particle loading on mechanical properties of particulate–polymer composites. Mater. Sci. Compos. Part B-Eng. 2008, 39, 933–961. [Google Scholar] [CrossRef]
- Young, R.J.; Beaumont, P.W.R. Effect of composition upon fracture of silica particle-filled epoxy–resin composites. J. Mater. Sci. 1977, 12, 684–692. [Google Scholar] [CrossRef]
- Pukanszky, B.; Vörös, G. Mechanism of interfacial interactions in particulate filled composites. Compos. Interfaces 1993, 1, 411–427. [Google Scholar] [CrossRef]
- Xu, W.; Jambhulkar, S.; Zhu, Y.; Ravichandran, D.; Kakarla, M.; Vernon, B.; Lott, D.G.; Cornella, J.L.; Shefi, O.; Miquelard-Garnier, G.; et al. 3D printing for polymer/particle-based processing: A review. Compos. Part B Eng. 2021, 223, 109102. [Google Scholar] [CrossRef]
- Caizer, C. Nanoparticle size effect on some magnetic properties. In Handbook of Nanoparticles; Aliofkhazraei, M., Ed.; Springer International Publishing: Cham, Switzerland, 2016; p. 475. [Google Scholar]
- O’Handley, R.C. Modern Magnetic Materials, Principles and Applications; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2000. [Google Scholar]
- Spaldin, N. Magnetic Materials, Fundamentals and Device Applications; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Vansteenkiste, A.; Leliaert, J.; Dvornik, M.; Helsen, M.; Garcia-Sanchez, F.; Van Waeyenberge, B. The design and verification of MuMax3. AIP Adv. 2014, 4, 107133. [Google Scholar] [CrossRef]
- Kákay, A.; Varga, L.K. Monodomain critical radius for soft-magnetic fine particles. J. Appl. Phys. 2005, 97, 083901. [Google Scholar] [CrossRef]
- Qin, G.; Pei, W.; Ren, Y.; Shimada, Y.; Endo, Y.; Yamaguchi, M.; Okamoto, S.; Kitakami, O. Ni80Fe20 permalloy nanoparticles: Wet chemical preparation, size control and their dynamic permeability characteristics when composited with Fe micron particles. J. Magn. Magn. Mater. 2009, 321, 4057–4062. [Google Scholar] [CrossRef]
- Ehrmann, A.; Blachowicz, T. Interaction between magnetic nanoparticles in clusters. AIMS Mater. Sci. 2017, 4, 383–390. [Google Scholar] [CrossRef]
- Gilbert, T. Classics in Magnetics A Phenomenological Theory of Damping in Ferromagnetic Materials. IEEE Trans. Magn. 2004, 40, 3443–3449. [Google Scholar] [CrossRef]


| Sample | Name |
|---|---|
| Pure ABS | ABS |
| ABS + Py 10 wt% | ABS-Py10 |
| ABS + Py 20 wt% | ABS-Py20 |
| ABS + Py 40 wt% | ABS-Py40 |
| ABS + Py 60 wt% | ABS-Py60 |
| ABS + Py 80 wt% | ABS-Py80 |
| Pure Py-NP (100%) | Py-NP |
| Sample | Tg (°C) ± 2 °C (ABS = 105 °C) | To (°C) ± 2 °C | T10 (°C) ± 2 °C | Residual Weight (%) at 900 °C ± 0.01% |
|---|---|---|---|---|
| ABS-Py10 | 106 | 350 | 394 | 10 |
| ABS-Py20 | 103 | 372 | 396 | 21 |
| ABS-Py40 | 106 | 371 | 399 | 38 |
| ABS-Py60 | 106 | 382 | 400 | 59 |
| ABS-Py80 | 112 | 390 | 420 | 85 |
| Sample | E (MPa) | Elongation at Break εb (%) | Breaking Stress σb (MPa) |
|---|---|---|---|
| ABS | 1155.22 ± 118.03 | 3.29 ± 0.55 | 19.86 ± 6.73 |
| ABS-Py10 | 781.47 ± 94.29 | 2.07 ± 0.39 | 14.43 ± 1.13 |
| ABS-Py20 | 759.03 ± 308.90 | 1.41 ± 0.14 | 8.45 ± 2.53 |
| ABS-Py40 | 1103.75 ± 280.90 | 2.18 ± 0.54 | 19.54 ± 3.49 |
| ABS-Py60 | 1980.27 ± 631.20 | 1.07 ± 0.31 | 19.26 ± 9.18 |
| Sample | Ms (emu/g) | Mr (emu/g) | Hc (Oe) |
|---|---|---|---|
| ABS-Py10 | 5.59 ± 1.05 | 0.53 ± 0.08 | 86.74 ± 8.31 |
| ABS-Py20 | 10.86 ± 3.93 | 0.91 ± 0.46 | 94.20 ± 6.27 |
| ABS-Py40 | 26.62 ± 2.77 | 2.35 ± 0.26 | 89.90 ± 7.31 |
| ABS-Py60 | 38.04 ± 0.74 | 4.62 ± 0.10 | 87.36 ± 3.38 |
| ABS-Py80 | 50.88 ± 4.71 | 8.01 ± 0.87 | 82.52 ± 1.99 |
| Py-NP | 65.78 | 5.34 | 72.45 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Merazzo, K.J.; Díez, A.G.; Tubio, C.R.; Manchado, J.C.; Malet, R.; Pérez, M.; Costa, P.; Lanceros-Mendez, S. Acrylonitrile Butadiene Styrene-Based Composites with Permalloy with Tailored Magnetic Response. Polymers 2023, 15, 626. https://doi.org/10.3390/polym15030626
Merazzo KJ, Díez AG, Tubio CR, Manchado JC, Malet R, Pérez M, Costa P, Lanceros-Mendez S. Acrylonitrile Butadiene Styrene-Based Composites with Permalloy with Tailored Magnetic Response. Polymers. 2023; 15(3):626. https://doi.org/10.3390/polym15030626
Chicago/Turabian StyleMerazzo, Karla J., Ander García Díez, Carmen R. Tubio, Juan Carlos Manchado, Ramón Malet, Marc Pérez, Pedro Costa, and Senentxu Lanceros-Mendez. 2023. "Acrylonitrile Butadiene Styrene-Based Composites with Permalloy with Tailored Magnetic Response" Polymers 15, no. 3: 626. https://doi.org/10.3390/polym15030626
APA StyleMerazzo, K. J., Díez, A. G., Tubio, C. R., Manchado, J. C., Malet, R., Pérez, M., Costa, P., & Lanceros-Mendez, S. (2023). Acrylonitrile Butadiene Styrene-Based Composites with Permalloy with Tailored Magnetic Response. Polymers, 15(3), 626. https://doi.org/10.3390/polym15030626










