Analysis of Deformation and Properties of Composite Melon Petals via Vibration Pretreatment—Microwave Compound Curing
Abstract
:1. Introduction
2. Theories in Vibration Pretreatment–Microwave Curing Compound Process
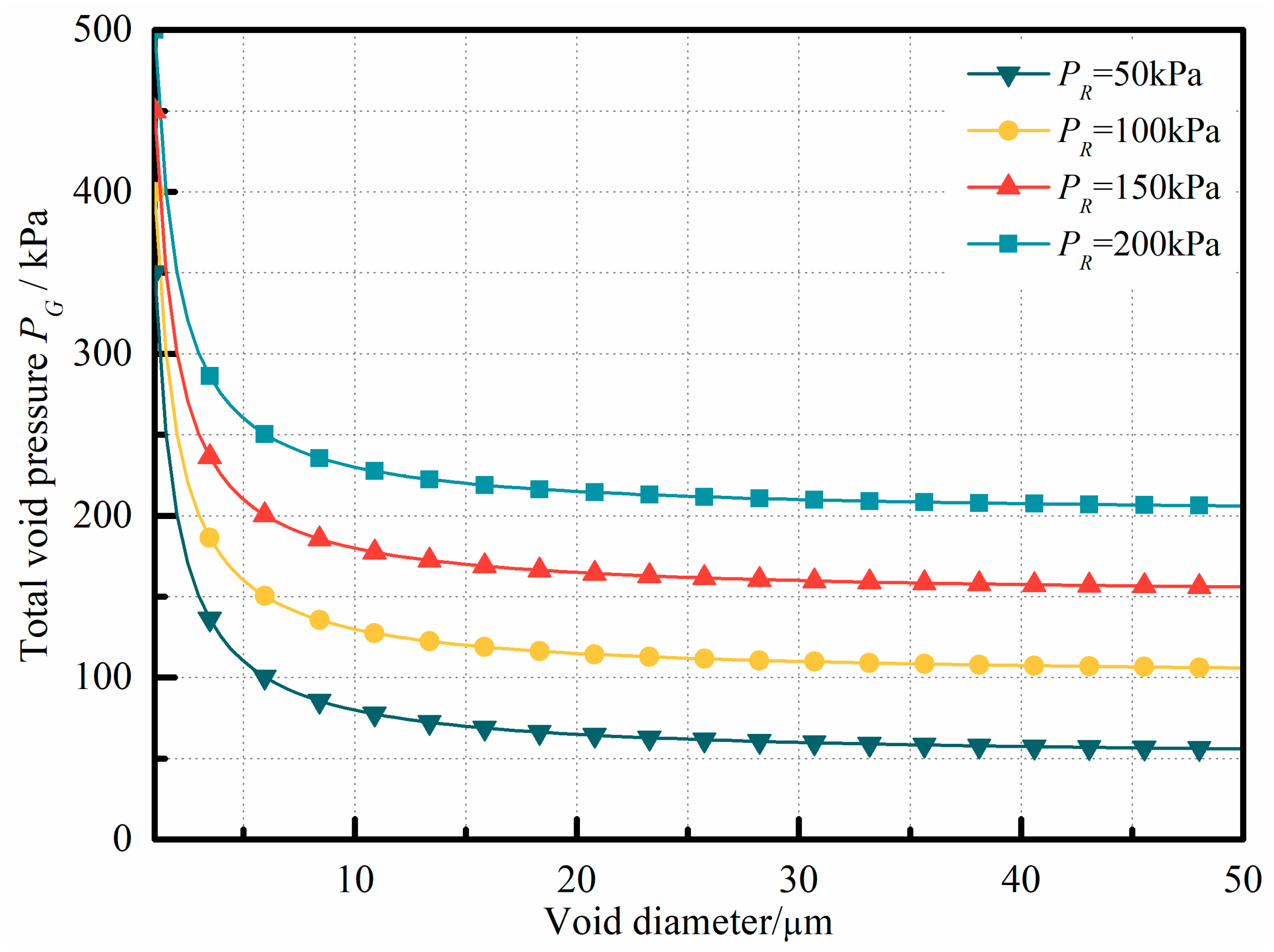
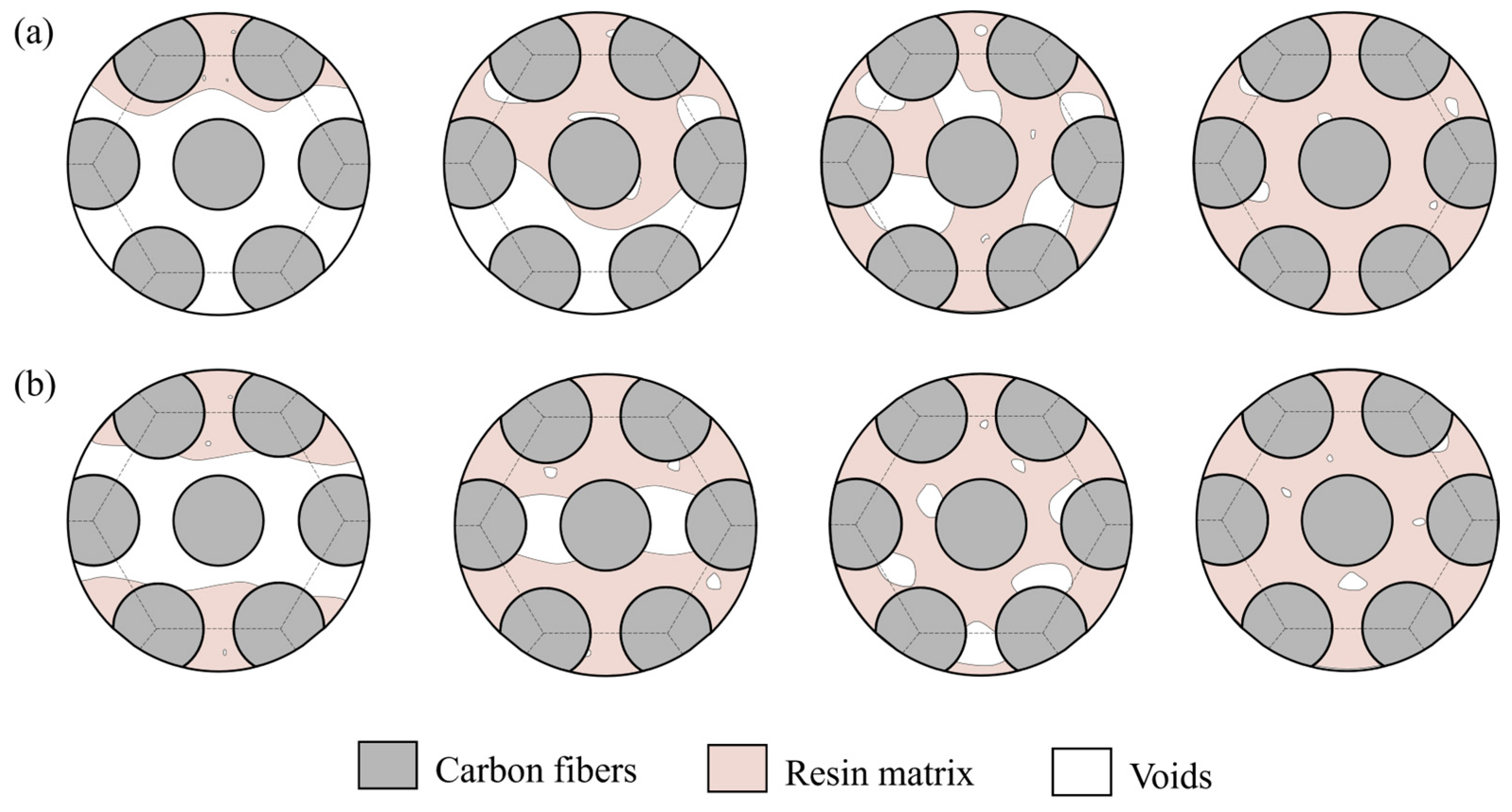
2.1. Mechanism of Void Reduction by Vibration Treatment
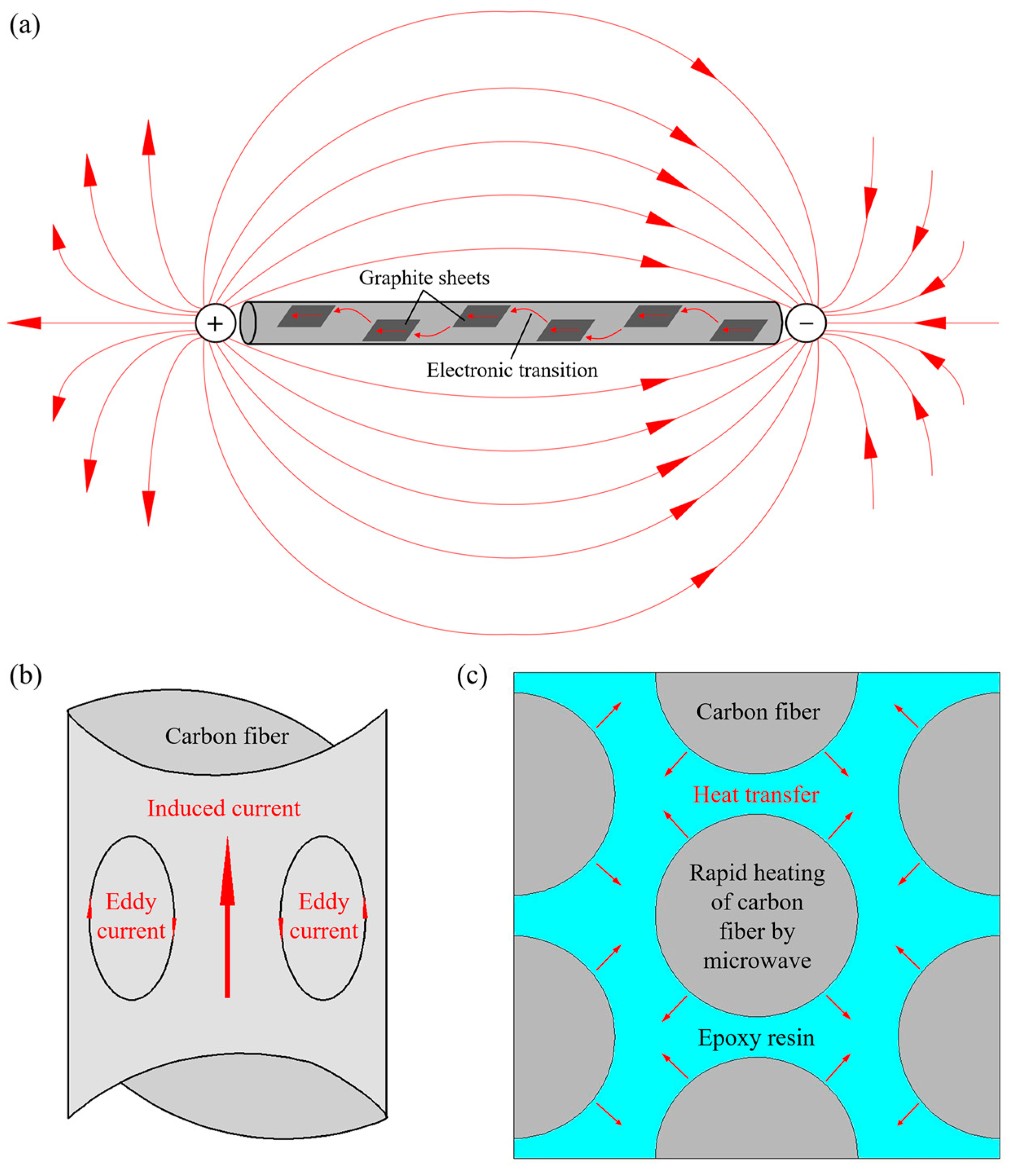
2.2. Mechanism of Uniform Heating by Microwave
2.2.1. Correlation Model of Composite Materials during Microwave Heating
2.2.2. Micro-Heating Mechanism of Composite Materials
3. Materials and Methods
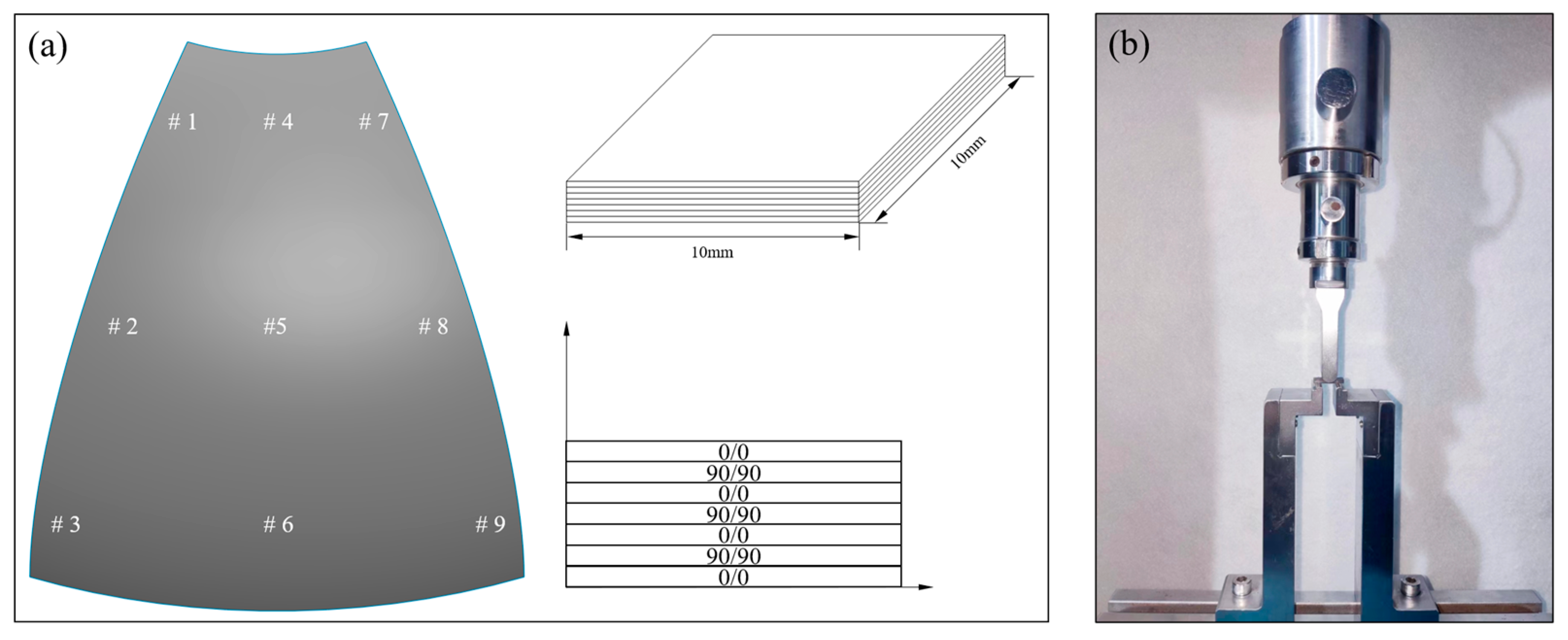
3.1. Materials and Equipment
3.2. Detection of Surface Profile Accuracy
3.3. Microscopic Characterization
3.4. Mechanical Property Tests
3.5. Cryogenic Permeability Tests
3.5.1. Testing Principle and Equipment Construction
3.5.2. Experimental Conditions
4. Results and Discussion
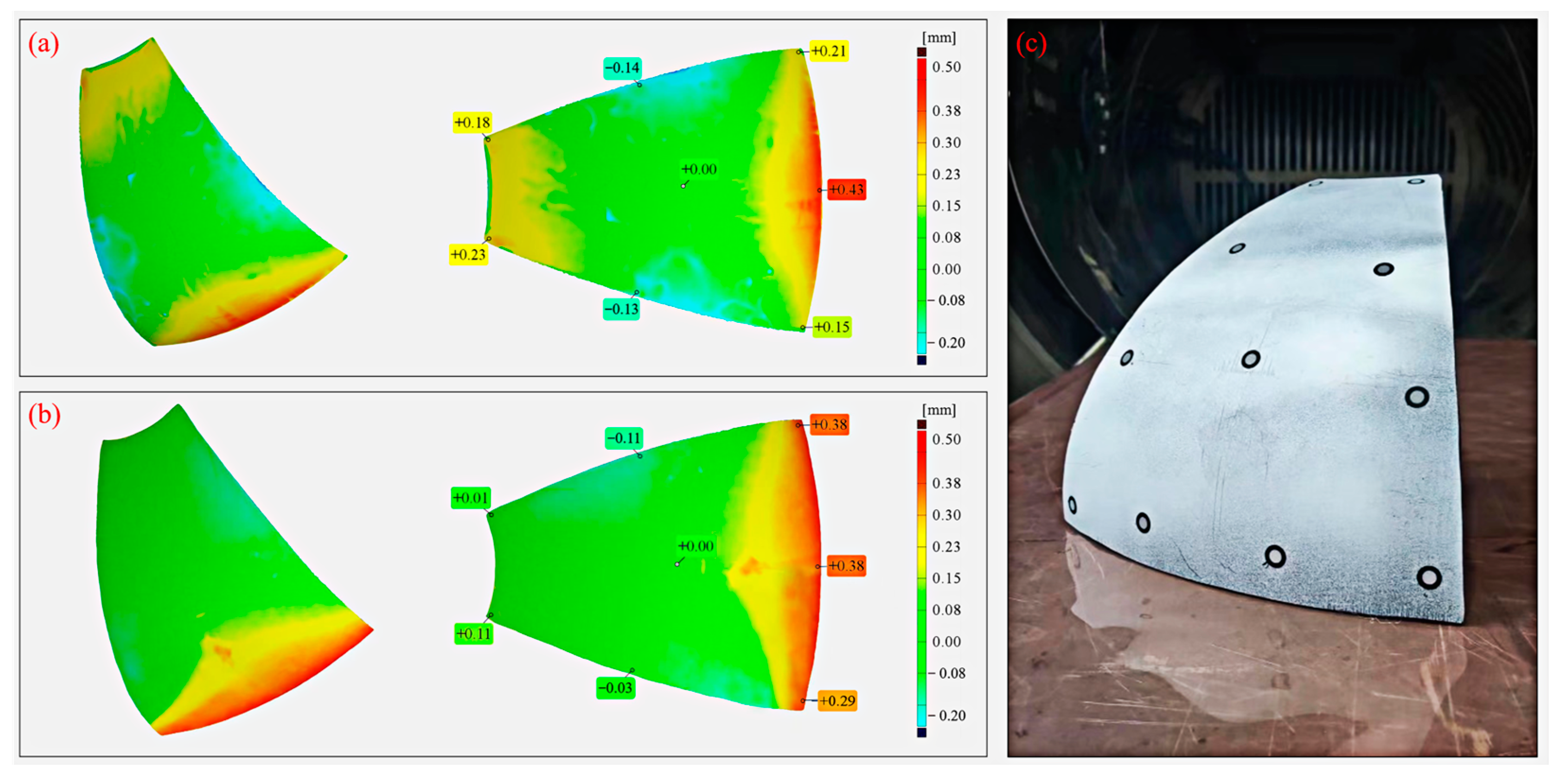
4.1. Analysis of Surface Accuracy
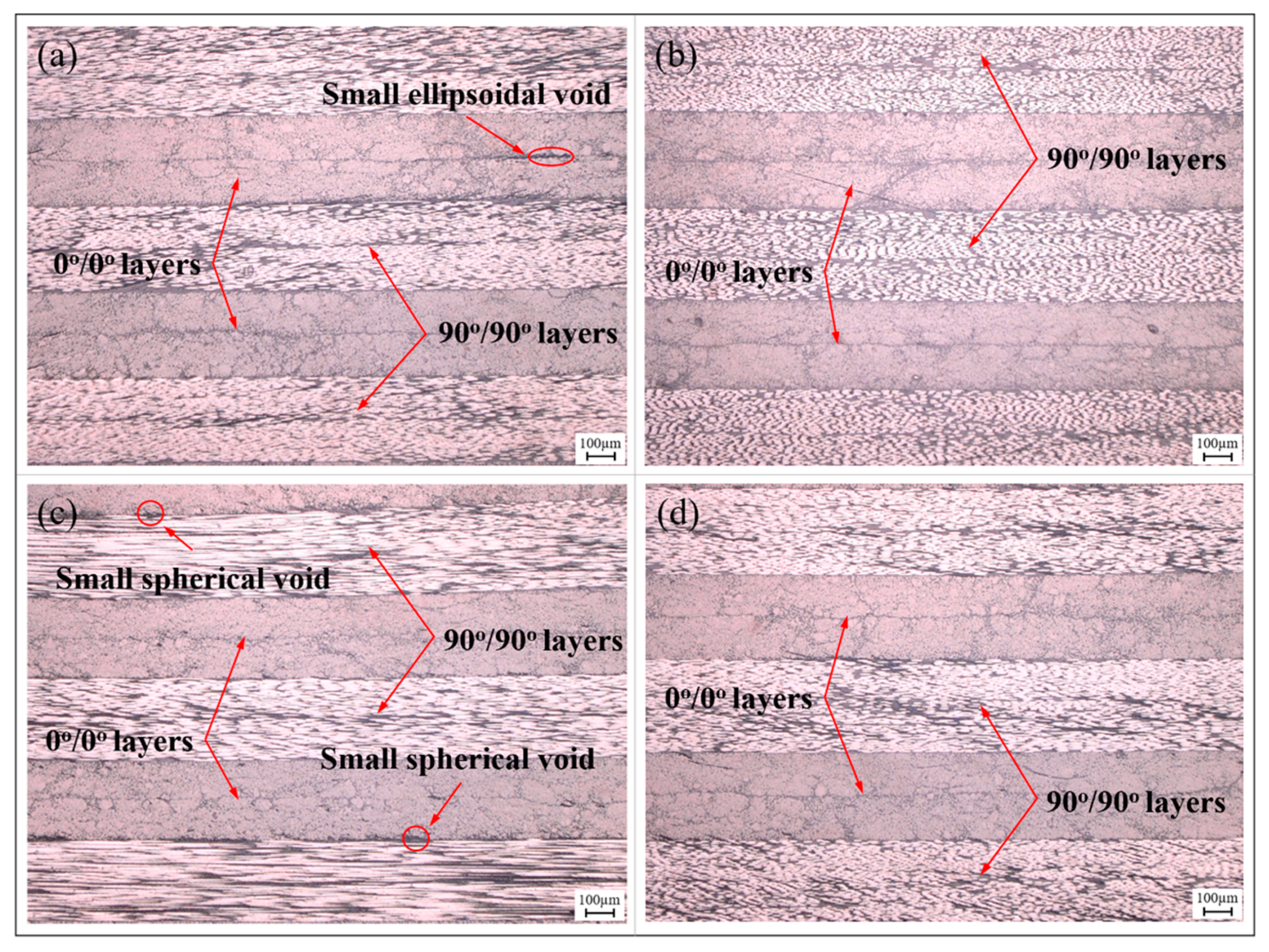
4.2. Microstructure and Porosity Analysis
4.3. Analysis of Interlaminar Bonding Property
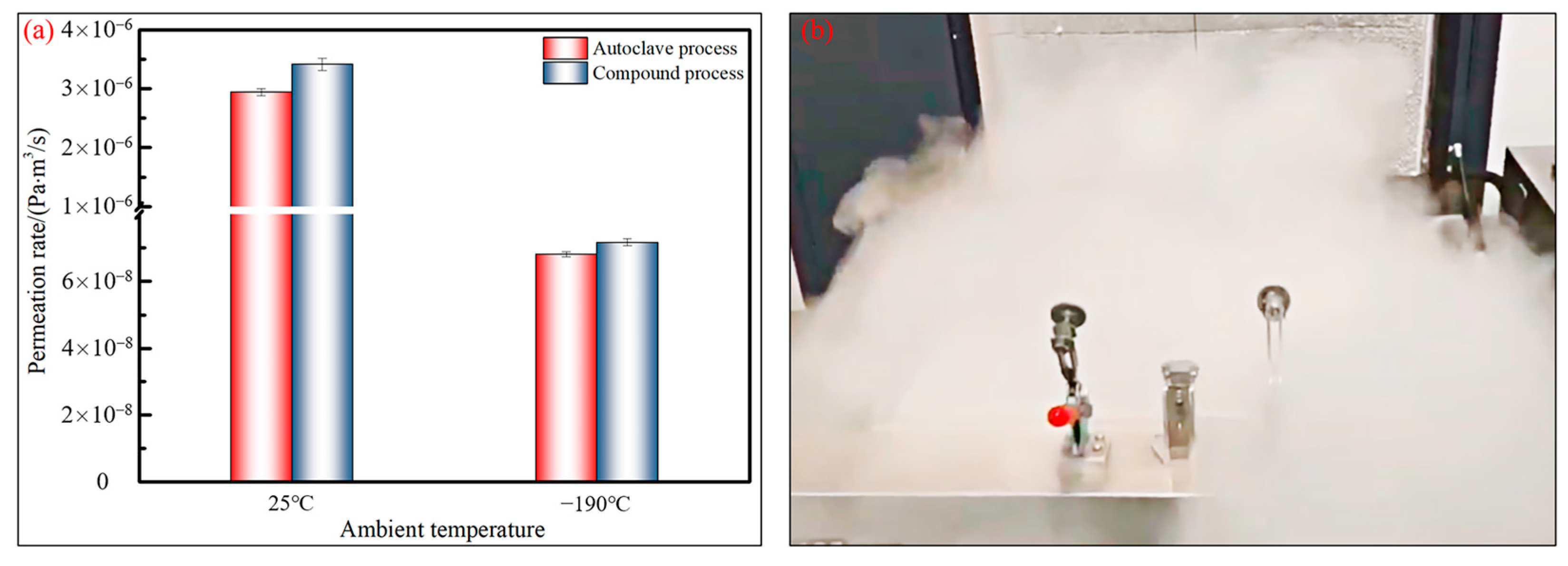
4.4. Analysis of Ambient/Low Temperature Permeability
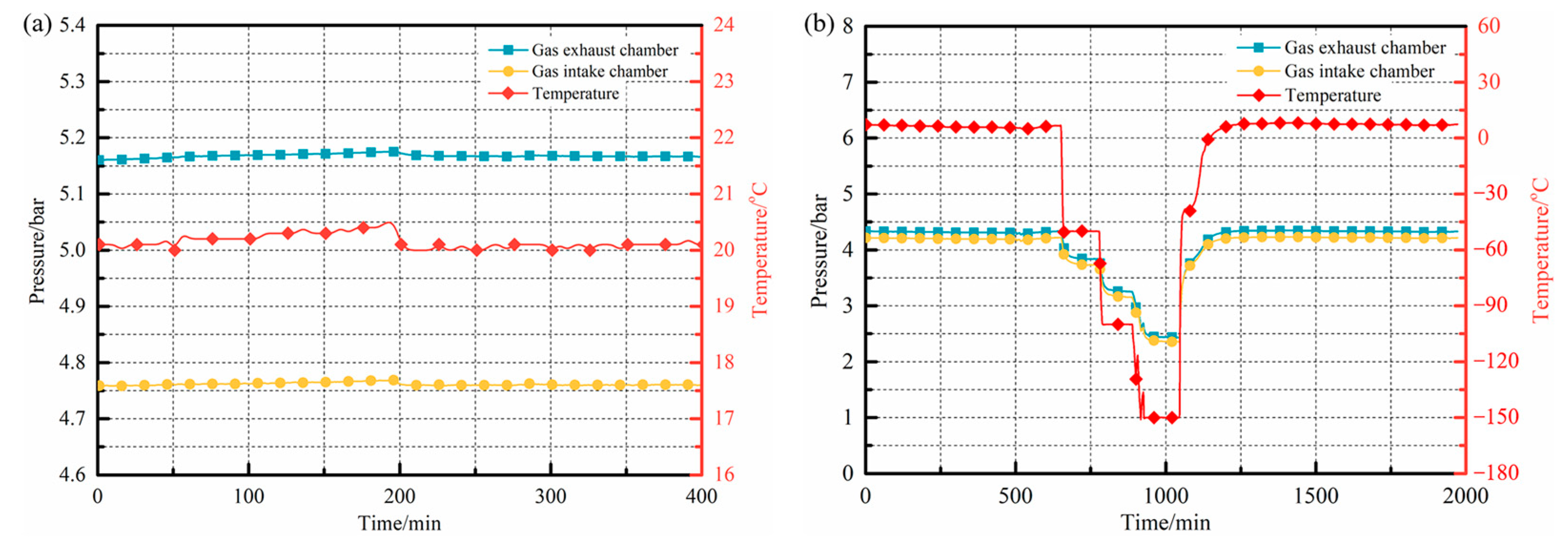
4.4.1. Validation of Equipment Sealing Performance
4.4.2. Permeation Rates of Components
5. Conclusions
- (1)
- The composite melon petals formed via the vibration pretreatment–microwave curing compound process exhibited excellent shaping precision, with the maximum deformation occurring at the larger end of the components, being approximately 0.40 mm. This deformation was less than the ±0.6 mm surface precision requirements for engineering applications;
- (2)
- There were no large-sized voids or delamination defects at various positions within the components, and the interior average porosity was only 0.39%, which demonstrated that the compound process was equally effective in reducing and inhibiting voids in the manufacturing of composite components with complex structural features;
- (3)
- The compound process fully leveraged the advantages of vibration pretreatment in inhibiting the generation of defects and microwave curing in enhancing the interfacial bonding performance, and the average ILSS of the cured melon petals reached 71.51 MPa. A sufficient fiber impregnation, together with a strong interfacial adhesion between the fibers and the matrix during the curing process, was obtained, thus increasing the capacity for load transfer from the matrix to the fibers through the interfaces.
- (4)
- At a pressure differential of 0.5 MPa, the melon petals cured via the compound process exhibited permeation rates of and at room temperature and low temperature, respectively. These results were within the same order of magnitude as those of the components manufactured using the standard autoclave process, thereby meeting the requirements for aerospace cryogenic composite tanks in resisting the permeation of small-molecule media.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, C.; Zhang, B. Application and key technology of composites tank in space cryogenic propulsion system. Acta Aeronaut. Astronaut. Sin. 2014, 35, 2747–2755. [Google Scholar]
- Belvin, W.K.; Watson, J.J.; Singhal, S.N. Structural concepts and materials for lunar exploration habitats. Space 2006, 12, 19–27. [Google Scholar]
- Chen, X. The development and applications of advanced polymer matrix composites. J. Aeronaut. Mater. 2003, 23, 198–204. [Google Scholar]
- Epaarachchi, J.A. Effects of static-fatigue (tension) on the tension-tension fatigue life of glass fiber reinforced plastic composites. Compos. Struct. 2006, 74, 19–40. [Google Scholar] [CrossRef]
- Chen, S. Development trends of advanced composite in near term. J. Mater. Eng. 2004, 9, 9–13, 18. [Google Scholar]
- Vickers, J.H. Composite Australia Conference Composite Cryotank Project Structures for Launch Vehicles. 2013. Available online: https://ntrs.nasa.gov/-search.jsp?R=20130013045 (accessed on 1 October 2023).
- Zeng, H. The developments in polymeric composites-The fiber-reinforced resin composites. J. Mater. Eng. 1989, 5, 6–13. [Google Scholar]
- Mccarville, D.A.; Guzman, J.C.; Dillon, A.K.; Jackson, J.R.; Birkland, J.O. 3.5 Design, Manufacture and test of cryotank components. Compr. Compos. Mater. II 2018, 3, 153–179. [Google Scholar]
- Huang, C.; Liu, D.; Wu, H.; Chang, Z. Application prospects of composite propellant tanks in domestic launch vehicles. J. Shenyang Aerosp. Univ. 2016, 33, 27–35. [Google Scholar]
- Knapscha, J. NASA/Boeing composite launch vehicle fuel tank scores firsts. Compos. World 2016, 2, 60–63. [Google Scholar]
- Guan, C. Basic Research on Vibration Pretreatment-Microwave Curing Compound Process of Polymer Matrix Composite Components. Ph.D. Thesis, Central South University, Changsha, China, 2022. [Google Scholar]
- Guan, C.; Zhan, L.; Shi, H.; Dai, G.; Xiao, Y. Simulation and experiment analysis of relationship between voids and permeability of composites. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2022, 236, 23–36. [Google Scholar] [CrossRef]
- Lin, J. Curing Temperature Field Analysis and Regulation of Composite Components in Autoclave Process. Master’s Thesis, Dalian University of Technology, Dalian, China, 2015. [Google Scholar]
- Wang, Y.; Liang, X.; Xue, X. Analysis of heat transfer and temperature field distribution on frame tooling in autoclave process. Aeronaut. Manuf. Technol. 2008, 22, 80–83. [Google Scholar]
- Wang, Y.; Liang, X.; Cao, Z. Review of the temperature field research of autoclave moulding for advanced composite components. Compos. Sci. Eng. 2009, 3, 81–85. [Google Scholar]
- Yue, G.; Zhang, B.; Du, S.; Dai, F.; Zhang, C.; Liang, X.; Wang, Y. Geometrical deformations of the framed-mould in autoclave processing for composite structures. Acta Mater. Compos. Sin. 2009, 26, 148–152. [Google Scholar]
- Li, Y.; Li, N.; Gao, J. Tooling design and microwave curing technologies for the manufacturing of fiber-reinforced polymer composites in aerospace applications. Int. J. Adv. Manuf. Technol. 2014, 70, 591–606. [Google Scholar] [CrossRef]
- Wen, Y.; Wen, Q.; Li, F.; Li, Y.; Li, N. Microwave curing of carbon fiber reinforced composites. Aeronaut. Manuf. Technol. 2015, 484, 61–64. [Google Scholar]
- Jackson, J.R.; Vickers, J.; Fikes, J. Composite Cryotank Technologies and Development 2.4 and 5.5m Out of Autoclave Tank Test Results. 2015. Available online: http://ntrs.nasa.gov/search.-jsp?R=20150021410 (accessed on 1 October 2023).
- Wang, T.; Liu, J. A review of microwave curing of polymeric materials. J. Electron. Manuf. 2000, 10, 181–189. [Google Scholar] [CrossRef]
- Mijovic, J.; Wijaya, J. Review of cure of polymers and composites by microwave energy. Polym. Compos. 1990, 11, 184–191. [Google Scholar] [CrossRef]
- Derradji, M.; Wang, J.; Liu, W. Phthalonitrile Resins and Composites: Properties and Applications, 1st ed.; William Andrew: Oxford, UK, 2018; pp. 243–247. [Google Scholar]
- Lee, W.I.; Springer, G.S. Microwave curing of composites. J. Compos. Mater. 1984, 18, 387–409. [Google Scholar] [CrossRef]
- Li, N.; Li, Y.; Jelonnek, J.; Link, G.; Gao, J. A new process control method for microwave curing of carbon fiber reinforced composites in aerospace applications. Compos. Part B Eng. 2017, 122, 61–70. [Google Scholar] [CrossRef]
- Chen, Z.; Jiao, W.; Yan, M.; Yang, F. Research progress of anti-leakage of carbon fiber reinforced resin composites at cryogenic tanks. Compos. Sci. Eng. 2018, 11, 109–116. [Google Scholar]
- Nightingale, C.; Day, R.J. Flexural and interlaminar shear strength properties of carbon fiber/epoxy composites cured thermally and with microwave radiation. Compos. Part A Appl. Sci. Manuf. 2002, 33, 1021–1030. [Google Scholar] [CrossRef]
- Boey, F.Y.C.; Lye, S.W. Void reduction in autoclave processing of thermoset composites: Part 2: Void reduction in a microwave curing process. Composites 1992, 23, 266–270. [Google Scholar] [CrossRef]
- Lye, S.W.; Boey, F.Y.C. PC-based monitoring and control system for microwave curing of polymer composites. Mater. Manuf. Process. 1994, 9, 851–868. [Google Scholar] [CrossRef]
- Chen, X.; Zhan, L.; Huang, M.; Chang, T.; Li, S.; Peng, W. A novel method for curing carbon fiber reinforced plastics by high-pressure microwave. Fiber. Polym. 2016, 17, 2143–2152. [Google Scholar] [CrossRef]
- Guan, C.; Zhan, L.; Dai, G.; Xiao, Y. Effect of vibration treatment on interfacial strength of microwave curing process for advanced composites. Compos. Interfaces 2021, 28, 237–253. [Google Scholar] [CrossRef]
- Guan, C.; Zhan, L.; Yang, X.; Dai, G.; Xiao, Y. Significant effect of vibration treatment on microwave curing carbon fiber reinforced plastic. J. Reinf. Plast. Compos. 2020, 39, 373–383. [Google Scholar] [CrossRef]
- Guan, C.; Zhan, L.; Dai, G.; Wu, X.; Xiao, Y. A unique method for curing composite materials by introducing vibration treatment into the hybrid heating process. J. Cent. South Univ. 2021, 28, 2961–2972. [Google Scholar] [CrossRef]
- Muric-Nesic, J.; Compston, P.; Noble, N.; Stachurski, Z.H. Effect of low frequency vibrations on void content in composite materials. Compos. Part A Appl. Sci. Manuf. 2009, 40, 548–551. [Google Scholar] [CrossRef]
- GB/T 3365-2008; Carbon Fiber Reinforced Plastics—Determination of Void Content and Fiber Volume Content. Standardization Administration of the Peopleʹs Republic of China: Beijing, China, 2008.
- ASTM D2344/D2344M-16; Standard Test Method for Short-Beam Strength of Polymer Matrix Composite Materials and Their Laminates. ASTM International: West Conshohocken, PA, USA, 2022.
- GB/T 1038-2000; Plastics—Film and Sheeting—Determination of Gas Transmission—Differential-Pressure Method. Standardization Administration of the Peopleʹs Republic of China: Beijing, China, 2000.





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guan, C.; Chi, T.; Zhan, L.; Chen, J.; Wang, B.; Xie, L.; Zhong, S. Analysis of Deformation and Properties of Composite Melon Petals via Vibration Pretreatment—Microwave Compound Curing. Polymers 2023, 15, 4541. https://doi.org/10.3390/polym15234541
Guan C, Chi T, Zhan L, Chen J, Wang B, Xie L, Zhong S. Analysis of Deformation and Properties of Composite Melon Petals via Vibration Pretreatment—Microwave Compound Curing. Polymers. 2023; 15(23):4541. https://doi.org/10.3390/polym15234541
Chicago/Turabian StyleGuan, Chenglong, Tongming Chi, Lihua Zhan, Junhao Chen, Bing Wang, Liping Xie, and Shuncong Zhong. 2023. "Analysis of Deformation and Properties of Composite Melon Petals via Vibration Pretreatment—Microwave Compound Curing" Polymers 15, no. 23: 4541. https://doi.org/10.3390/polym15234541
APA StyleGuan, C., Chi, T., Zhan, L., Chen, J., Wang, B., Xie, L., & Zhong, S. (2023). Analysis of Deformation and Properties of Composite Melon Petals via Vibration Pretreatment—Microwave Compound Curing. Polymers, 15(23), 4541. https://doi.org/10.3390/polym15234541









