Knot Formation on DNA Pushed Inside Chiral Nanochannels
Abstract
1. Introduction
2. Materials and Methods
2.1. Model of DNA
2.2. Model of Nano-Channels
2.3. Push by External Force and MD Simulation
3. Results and Discussion
3.1. General Polymer Metrics
3.2. The Monomer Distributions upon Pushing
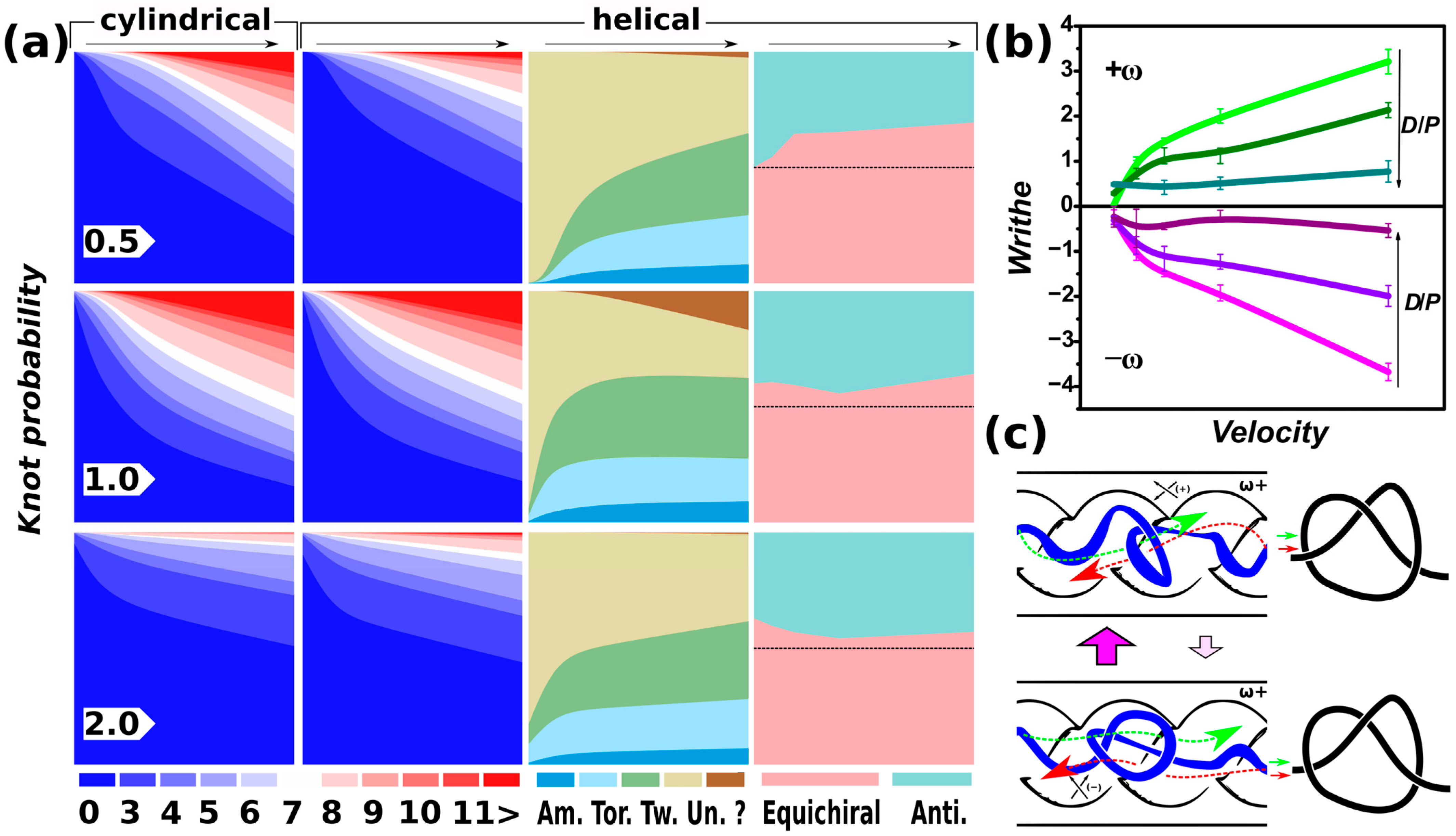
3.3. Knotting Probabilities and Topology
3.4. Handedness of the Channels and Knots
3.5. On the Mechanism of How Geometry of the Channels Induces Handedness of Knots
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hiemenz, P.C.; Lodge, T.P. Polymer Chemistry; CRC Press: Boca Raton, FL, USA, 2007; ISBN 1-4200-1827-2. [Google Scholar]
- Dietrich-Buchecker, C.; Rapenne, G.; Sauvage, J.-P. Molecular Knots—From Early Attempts to High-Yield Template Syntheses. In Molecular Catenanes, Rotaxanes and Knots; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 1999; pp. 107–142. ISBN 978-3-527-61372-4. [Google Scholar]
- Dietrich-Buchecker, C.O.; Sauvage, J.-P. A synthetic molecular trefoil knot. Angew. Chem. Int. Ed. Engl. 1989, 28, 189–192. [Google Scholar] [CrossRef]
- Deibler, R.W.; Mann, J.K.; Sumners, D.W.L.; Zechiedrich, L. Hin-mediated DNA knotting and recombining promote replicon dysfunction and mutation. BMC Mol. Biol. 2007, 8, 44. [Google Scholar] [CrossRef] [PubMed]
- Portugal, J.; Rodríguez-Campos, A. T7 RNA polymerase cannot transcribe through a highly knotted DNA template. Nucleic Acids Res. 1996, 24, 4890–4894. [Google Scholar] [CrossRef] [PubMed]
- Racko, D.; Benedetti, F.; Goundaroulis, D.; Stasiak, A. Chromatin Loop Extrusion and Chromatin Unknotting. Polymers 2018, 10, 1126. [Google Scholar] [CrossRef]
- Rawdon, E.J.; Dorier, J.; Racko, D.; Millett, K.C.; Stasiak, A. How topoisomerase IV can efficiently unknot and decatenate negatively supercoiled DNA molecules without causing their torsional relaxation. Nucleic Acids Res. 2016, 44, 4528–4538. [Google Scholar] [CrossRef]
- Racko, D.; Benedetti, F.; Dorier, J.; Burnier, Y.; Stasiak, A. Generation of supercoils in nicked and gapped DNA drives DNA unknotting and postreplicative decatenation. Nucleic Acids Res. 2015, 43, 7229–7236. [Google Scholar] [CrossRef]
- Dabrowski-Tumanski, P.; Sulkowska, J.I. Topological knots and links in proteins. Proc. Natl. Acad. Sci. USA 2017, 114, 3415–3420. [Google Scholar] [CrossRef]
- Virnau, P.; Mirny, L.A.; Kardar, M. Intricate knots in proteins: Function and evolution. PLoS Comput. Biol. 2006, 2, e122. [Google Scholar] [CrossRef]
- Jackson, S.E.; Suma, A.; Micheletti, C. How to fold intricately: Using theory and experiments to unravel the properties of knotted proteins. Curr. Opin. Struct. Biol. 2017, 42, 6–14. [Google Scholar] [CrossRef]
- Danon, J.J.; Krüger, A.; Leigh, D.A.; Lemonnier, J.-F.; Stephens, A.J.; Vitorica-Yrezabal, I.J.; Woltering, S.L. Braiding a molecular knot with eight crossings. Science 2017, 355, 159–162. [Google Scholar] [CrossRef]
- Sogo, J.; Stasiak, A.; Martínez-Robles, M.; Krimer, D.; Hernd_ndez, P.; Schvartzman, J. Formation of knots in partially replicated DNA molecules. J. Mol. Biol. 1999, 286, 637–643. [Google Scholar] [CrossRef] [PubMed]
- Lim, N.; Jackson, S. Molecular Knots in Biology and Chemistry. J. Physics. Condens. Matter Inst. Phys. J. 2015, 27, 354101. [Google Scholar] [CrossRef]
- Frisch, H.L.; Wasserman, E. Chemical Topology1. J. Am. Chem. Soc. 1961, 83, 3789–3795. [Google Scholar] [CrossRef]
- Dobay, A.; Sottas, P.-E.; Dubochet, J.; Stasiak, A. Predicting optimal lengths of random knots. Lett. Math. Phys. 2001, 55, 239–247. [Google Scholar] [CrossRef]
- Micheletti, C.; Marenduzzo, D.; Orlandini, E.; Summers, D.W. Knotting of random ring polymers in confined spaces. J. Chem. Phys. 2006, 124, 064903. [Google Scholar] [CrossRef]
- Wettermann, S.; Datta, R.; Virnau, P. Influence of ionic conditions on knotting in a coarse-grained model for DNA. Front. Chem. 2023, 10, 1627. [Google Scholar] [CrossRef]
- Binder, K.; de Gennes, P.-G.; Giannelis, E.P.; Grest, G.S.; Hervet, H.; Krishnamoorti, R.; Leger, L.; Manias, E.; Raphael, E.; Wang, S.-Q. Polymers in Confined Environments; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1998; Volume 138, ISBN 3-540-64266-8. [Google Scholar]
- Kasianowicz, J.J.; Kellermayer, M.; Deamer, D.W. Structure and Dynamics of Confined Polymers: Proceedings of the NATO Advanced Research Workshop on Biological, Biophysical & Theoretical Aspects of Polymer Structure and Transport Bikal, Hungary 20–25 June 1999; Springer Science & Business Media: Dordrecht, The Netherlands, 2012; Volume 87, ISBN 94-010-0401-3. [Google Scholar]
- Dai, L.; Doyle, P.S. Universal knot spectra for confined polymers. Macromolecules 2018, 51, 6327–6333. [Google Scholar] [CrossRef]
- Frykholm, K.; Müller, V.; KK, S.; Dorfman, K.D.; Westerlund, F. DNA in nanochannels: Theory and applications. Q. Rev. Biophys. 2022, 55, e12. [Google Scholar] [CrossRef]
- Bleha, T.; Cifra, P. Stretching and compression of DNA by external forces under nanochannel confinement. Soft Matter 2018, 14, 1247–1259. [Google Scholar] [CrossRef]
- Bleha, T.; Cifra, P. Compression and stretching of single DNA molecules under channel confinement. J. Phys. Chem. B 2020, 124, 1691–1702. [Google Scholar] [CrossRef] [PubMed]
- Cifra, P.; Bleha, T. Piston Compression of Semiflexible Ring Polymers in Channels. Macromol. Theory Simul. 2021, 30, 2100027. [Google Scholar] [CrossRef]
- Chakrabarti, B.; Liu, Y.; LaGrone, J.; Cortez, R.; Fauci, L.; Du Roure, O.; Saintillan, D.; Lindner, A. Flexible filaments buckle into helicoidal shapes in strong compressional flows. Nat. Phys. 2020, 16, 689–694. [Google Scholar] [CrossRef]
- Chen, W.; Kong, X.; Wei, Q.; Chen, H.; Liu, J.; Jiang, D. Compression and Stretching of Confined Linear and Ring Polymers by Applying Force. Polymers 2021, 13, 4193. [Google Scholar] [CrossRef]
- Michieletto, D.; Orlandini, E.; Turner, M.S.; Micheletti, C. Separation of geometrical and topological entanglement in confined polymers driven out of equilibrium. ACS Macro Lett. 2020, 9, 1081–1085. [Google Scholar] [CrossRef] [PubMed]
- Jung, Y.; Ha, B.-Y. Confinement induces helical organization of chromosome-like polymers. Sci. Rep. 2019, 9, 869. [Google Scholar] [CrossRef]
- Jun, S.; Thirumalai, D.; Ha, B.-Y. Compression and stretching of a self-avoiding chain in cylindrical nanopores. Phys. Rev. Lett. 2008, 101, 138101. [Google Scholar] [CrossRef]
- Hayase, Y.; Sakaue, T.; Nakanishi, H. Compressive response and helix formation of a semiflexible polymer confined in a nanochannel. Phys. Rev. E 2017, 95, 052502. [Google Scholar] [CrossRef] [PubMed]
- Huang, A.; Reisner, W.; Bhattacharya, A. Dynamics of DNA squeezed inside a nanochannel via a sliding gasket. Polymers 2016, 8, 352. [Google Scholar] [CrossRef]
- Bernier, S.; Huang, A.; Reisner, W.; Bhattacharya, A. Evolution of nested folding states in compression of a strongly confined semiflexible chain. Macromolecules 2018, 51, 4012–4022. [Google Scholar] [CrossRef]
- Rothörl, J.; Wettermann, S.; Virnau, P.; Bhattacharya, A. Knot formation of dsDNA pushed inside a nanochannel. Sci. Rep. 2022, 12, 5342. [Google Scholar] [CrossRef]
- Zeng, L.; Reisner, W.W. Organized states arising from compression of single semiflexible polymer chains in nanochannels. Phys. Rev. E 2022, 105, 064501. [Google Scholar] [CrossRef]
- Rusková, R.; Račko, D. Knot Factories with Helical Geometry Enhance Knotting and Induce Handedness to Knots. Polymers 2022, 14, 4201. [Google Scholar] [CrossRef] [PubMed]
- Chelakkot, R.; Winkler, R.G.; Gompper, G. Flow-induced helical coiling of semiflexible polymers in structured microchannels. Phys. Rev. Lett. 2012, 109, 178101. [Google Scholar] [CrossRef] [PubMed]
- Zhou, J.; Wang, Y.; Menard, L.D.; Panyukov, S.; Rubinstein, M.; Ramsey, J.M. Enhanced nanochannel translocation and localization of genomic DNA molecules using three-dimensional nanofunnels. Nat. Commun. 2017, 8, 807. [Google Scholar] [CrossRef] [PubMed]
- Cifra, P.; Bleha, T. Pressure of Linear and Ring Polymers Confined in a Cavity. J. Phys. Chem. B 2023, 127, 4646–4657. [Google Scholar] [CrossRef]
- Micheletti, C.; Marenduzzo, D.; Orlandini, E.; Sumners, D.W. Simulations of knotting in confined circular DNA. Biophys. J. 2008, 95, 3591–3599. [Google Scholar] [CrossRef]
- Khorshid, A.; Zimny, P.; Tétreault-La Roche, D.; Massarelli, G.; Sakaue, T.; Reisner, W. Dynamic compression of single nanochannel confined DNA via a nanodozer assay. Phys. Rev. Lett. 2014, 113, 268104. [Google Scholar] [CrossRef]
- Khorshid, A.; Amin, S.; Zhang, Z.; Sakaue, T.; Reisner, W.W. Nonequilibrium dynamics of nanochannel confined DNA. Macromolecules 2016, 49, 1933–1940. [Google Scholar] [CrossRef]
- Amin, S.; Khorshid, A.; Zeng, L.; Zimny, P.; Reisner, W. A nanofluidic knot factory based on compression of single DNA in nanochannels. Nat. Commun. 2018, 9, 1506. [Google Scholar] [CrossRef]
- Fielden, S.D.P.; Leigh, D.A.; Woltering, S.L. Molecular Knots. Angew. Chem. Int. Ed. 2017, 56, 11166–11194. [Google Scholar] [CrossRef]
- Valdés, A.; Martinez-Garcia, B.; Segura, J.; Dyson, S.; Díaz-Ingelmo, O.; Roca, J. Quantitative disclosure of DNA knot chirality by high-resolution 2D-gel electrophoresis. Nucleic Acids Res. 2019, 47, e29. [Google Scholar] [CrossRef]
- Katsonis, N.; Lancia, F.; Leigh, D.A.; Pirvu, L.; Ryabchun, A.; Schaufelberger, F. Knotting a molecular strand can invert macroscopic effects of chirality. Nat. Chem. 2020, 12, 939–944. [Google Scholar] [CrossRef]
- Shi, X.; Pumm, A.-K.; Isensee, J.; Zhao, W.; Verschueren, D.; Martin-Gonzalez, A.; Golestanian, R.; Dietz, H.; Dekker, C. Sustained unidirectional rotation of a self-organized DNA rotor on a nanopore. Nat. Phys. 2022, 18, 1105–1111. [Google Scholar] [CrossRef]
- Pairault, N.; Niemeyer, J. Chiral mechanically interlocked molecules–applications of rotaxanes, catenanes and molecular knots in stereoselective chemosensing and catalysis. Synlett 2018, 29, 689–698. [Google Scholar]
- Ashbridge, Z.; Fielden, S.D.; Leigh, D.A.; Pirvu, L.; Schaufelberger, F.; Zhang, L. Knotting matters: Orderly molecular entanglements. Chem. Soc. Rev. 2022, 51, 7779–7809. [Google Scholar] [CrossRef] [PubMed]
- Wang, T.; Jiang, L.; Zhang, Y.; Wu, L.; Chen, H.; Li, C. Fabrication of polyimide mixed matrix membranes with asymmetric confined mass transfer channels for improved CO2 separation. J. Membr. Sci. 2021, 637, 119653. [Google Scholar] [CrossRef]
- Cheng, Q.; Ma, Q.; Pei, H.; Mo, Z. Chiral membranes for enantiomer separation: A comprehensive review. Sep. Purif. Technol. 2022, 292, 121034. [Google Scholar] [CrossRef]
- Zhang, J.; Albelda, M.T.; Liu, Y.; Canary, J.W. Chiral nanotechnology. Chirality 2005, 17, 404–420. [Google Scholar] [CrossRef]
- Dorier, J.; Goundaroulis, D.; Benedetti, F.; Stasiak, A. Knoto-ID: A tool to study the entanglement of open protein chains using the concept of knotoids. Bioinformatics 2018, 34, 3402–3404. [Google Scholar] [CrossRef]
- Rolfsen, D. Knots and Links; AMS Chelsea Publishing: Providence, RI, USA, 2003; Volume 346.H, ISBN 978-0-8218-3436-7. [Google Scholar]
- Brasher, R.; Scharein, R.; Vázquez, M. New biologically motivated knot table. Biochem. Soc. Trans. 2013, 41, 606–611. [Google Scholar] [CrossRef]
- Di Stefano, M.; Tubiana, L.; Di Ventra, M.; Micheletti, C. Driving knots on DNA with AC/DC electric fields: Topological friction and memory effects. Soft Matter 2014, 10, 6491–6498. [Google Scholar] [CrossRef]
- Lu, Y.; Weers, B.; Stellwagen, N.C. DNA persistence length revisited. Biopolymers 2002, 61, 261–275. [Google Scholar] [CrossRef]
- Chávez, Y.; Chacón-Acosta, G.; Dagdug, L. Unbiased diffusion of Brownian particles in a helical tube. J. Chem. Phys. 2018, 148, 214106. [Google Scholar] [CrossRef] [PubMed]
- Limbach, H.J.; Arnold, A.; Mann, B.A.; Holm, C. ESPResSo—An extensible simulation package for research on soft matter systems. Comput. Phys. Commun. 2006, 174, 704–727. [Google Scholar] [CrossRef]
- Arnold, A.; Lenz, O.; Kesselheim, S.; Weeber, R.; Fahrenberger, F.; Roehm, D.; Košovan, P.; Holm, C. ESPResSo 3.1: Molecular Dynamics Software for Coarse-Grained Models. In Meshfree Methods for Partial Differential Equations VI; Griebel, M., Schweitzer, M.A., Eds.; Springer: Berlin/Heidelberg, Germany, 2013; pp. 1–23. [Google Scholar]
- Nievergelt, Y. Computing the distance from a point to a helix and solving Kepler’s equation. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2009, 598, 788–794. [Google Scholar] [CrossRef]
- Lam, E.T.; Hastie, A.; Lin, C.; Ehrlich, D.; Das, S.K.; Austin, M.D.; Deshpande, P.; Cao, H.; Nagarajan, N.; Xiao, M. Genome mapping on nanochannel arrays for structural variation analysis and sequence assembly. Nat. Biotechnol. 2012, 30, 771–776. [Google Scholar] [CrossRef]
- Rusková, R.; Račko, D. Channels with Helical Modulation Display Stereospecific Sensitivity for Chiral Superstructures. Polymers 2021, 13, 3726. [Google Scholar] [CrossRef] [PubMed]
- Pastorino, C.; Kreer, T.; Müller, M.; Binder, K. Comparison of dissipative particle dynamics and Langevin thermostats for out-of-equilibrium simulations of polymeric systems. Phys. Rev. E 2007, 76, 026706. [Google Scholar] [CrossRef]
- Ripoll, M.; Ernst, M.H.; Español, P. Large scale and mesoscopic hydrodynamics for dissipative particle dynamics. J. Chem. Phys. 2001, 115, 7271–7284. [Google Scholar] [CrossRef]
- Dorfman, K.D.; Gupta, D.; Jain, A.; Muralidhar, A.; Tree, D.R. Hydrodynamics of DNA confined in nanoslits and nanochannels. Eur. Phys. J. Spec. Top. 2014, 223, 3179–3200. [Google Scholar] [CrossRef]
- Hsieh, S.-S.; Wu, F.-H.; Tsai, M.-J. DNA stretching on the wall surfaces in curved microchannels with different radii. Nanoscale Res. Lett. 2014, 9, 382. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Cannavacciuolo, L.; Winkler, R.G.; Gompper, G. Mesoscale simulations of polymer dynamics in microchannel flows. Europhys. Lett. 2008, 83, 34007. [Google Scholar] [CrossRef][Green Version]
- Cifra, P.; Teraoka, I. Partitioning of polymer chains in solution with a square channel: Lattice Monte Carlo simulations. Polymer 2002, 43, 2409–2415. [Google Scholar] [CrossRef]
- Fritsche, M.; Heermann, D.W. Confinement driven spatial organization of semiflexible ring polymers: Implications for biopolymer packaging. Soft Matter 2011, 7, 6906–6913. [Google Scholar] [CrossRef]
- Odijk, T. The statistics and dynamics of confined or entangled stiff polymers. Macromolecules 1983, 16, 1340–1344. [Google Scholar] [CrossRef]
- Horie, K.; Kitano, T.; Matsumoto, M.; Suzuki, M. A Partial Order On The Set Of Prime Knots With Up To 11 Crossings. J. Knot Theory Ramif. 2011, 20, 275–303. [Google Scholar] [CrossRef]
- Tubiana, L.; Polles, G.; Orlandini, E.; Micheletti, C. KymoKnot: A web server and software package to identify and locate knots in trajectories of linear or circular polymers. Eur. Phys. J. E 2018, 41, 72. [Google Scholar] [CrossRef]
- Tubiana, L.; Orlandini, E.; Micheletti, C. Probing the entanglement and locating knots in ring polymers: A comparative study of different arc closure schemes. Prog. Theor. Phys. Suppl. 2011, 191, 192–204. [Google Scholar] [CrossRef]
- Dabrowski-Tumanski, P.; Piejko, M.; Niewieczerzal, S.; Stasiak, A.; Sulkowska, J.I. Protein Knotting by Active Threading of Nascent Polypeptide Chain Exiting from the Ribosome Exit Channel. J. Phys. Chem. B 2018, 122, 11616–11625. [Google Scholar] [CrossRef]
- Dabrowski-Tumanski, P.; Stasiak, A. AlphaFold Blindness to Topological Barriers Affects Its Ability to Correctly Predict Proteins’ Topology. Preprints 2023, 2023081698. [Google Scholar] [CrossRef]
- Katritch, V.; Bednar, J.; Michoud, D.; Scharein, R.G.; Dubochet, J.; Stasiak, A. Geometry and physics of knots. Nature 1996, 384, 142–145. [Google Scholar] [CrossRef]
- Metzler, R.; Reisner, W.; Riehn, R.; Austin, R.; Tegenfeldt, J.O.; Sokolov, I.M. Diffusion mechanisms of localised knots along a polymer. Europhys. Lett. (EPL) 2006, 76, 696–702. [Google Scholar] [CrossRef]
- El-Sagheer, A.H.; Brown, T. Click Nucleic Acid Ligation: Applications in Biology and Nanotechnology. Acc. Chem. Res. 2012, 45, 1258–1267. [Google Scholar] [CrossRef] [PubMed]
- Devaraj, N.K.; Finn, M.G. Introduction: Click Chemistry. Chem. Rev. 2021, 121, 6697–6698. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rusková, R.; Račko, D. Knot Formation on DNA Pushed Inside Chiral Nanochannels. Polymers 2023, 15, 4185. https://doi.org/10.3390/polym15204185
Rusková R, Račko D. Knot Formation on DNA Pushed Inside Chiral Nanochannels. Polymers. 2023; 15(20):4185. https://doi.org/10.3390/polym15204185
Chicago/Turabian StyleRusková, Renáta, and Dušan Račko. 2023. "Knot Formation on DNA Pushed Inside Chiral Nanochannels" Polymers 15, no. 20: 4185. https://doi.org/10.3390/polym15204185
APA StyleRusková, R., & Račko, D. (2023). Knot Formation on DNA Pushed Inside Chiral Nanochannels. Polymers, 15(20), 4185. https://doi.org/10.3390/polym15204185









