Problems with Applying the Ozawa–Avrami Crystallization Model to Non-Isothermal Crosslinking Polymerization
Abstract
:1. Introduction
2. Avrami and Ozawa–Avrami Models
3. Simulations
4. Analysis of Simulated A3 Data
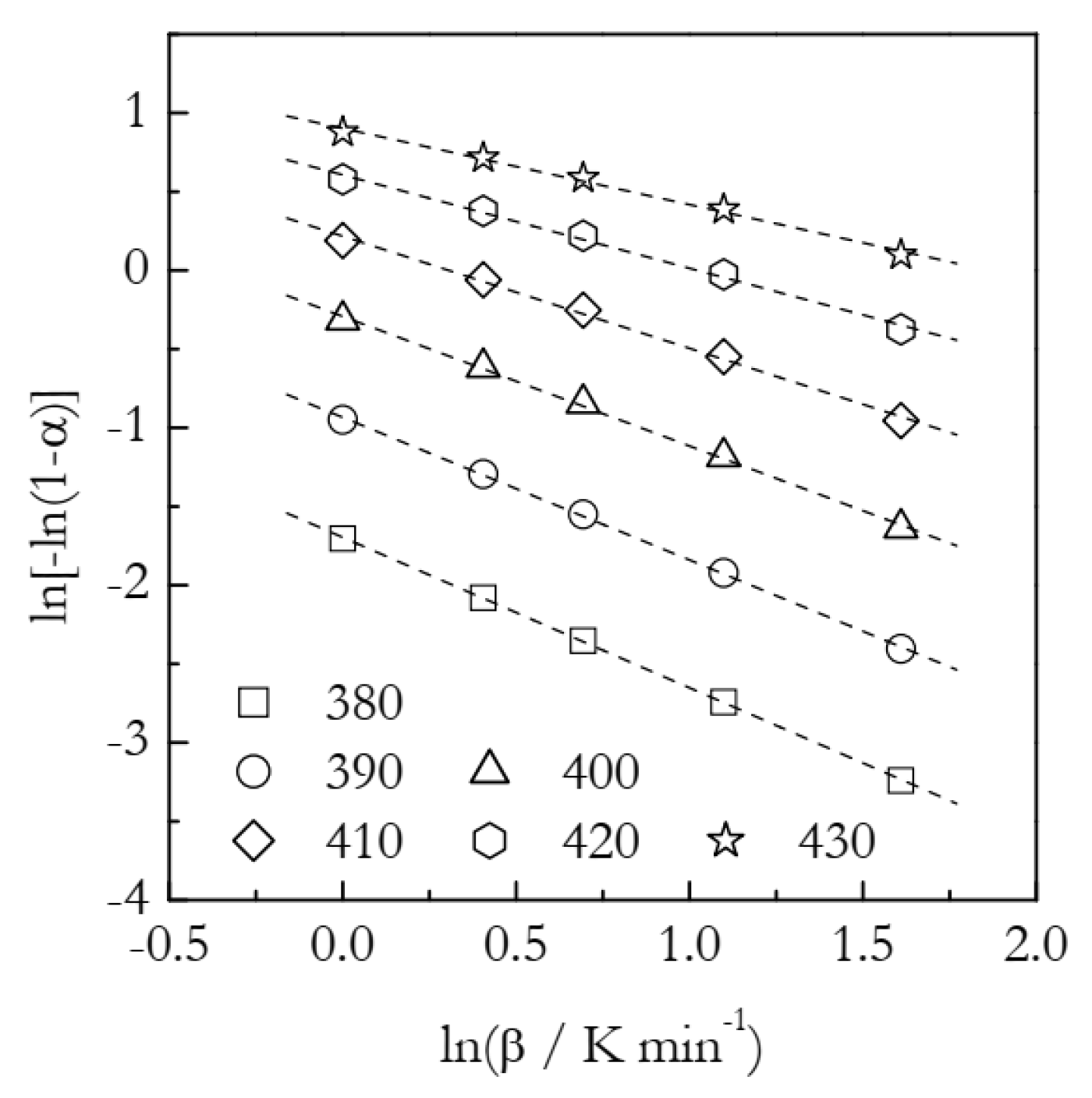
5. Analysis of Simulated F2 Data
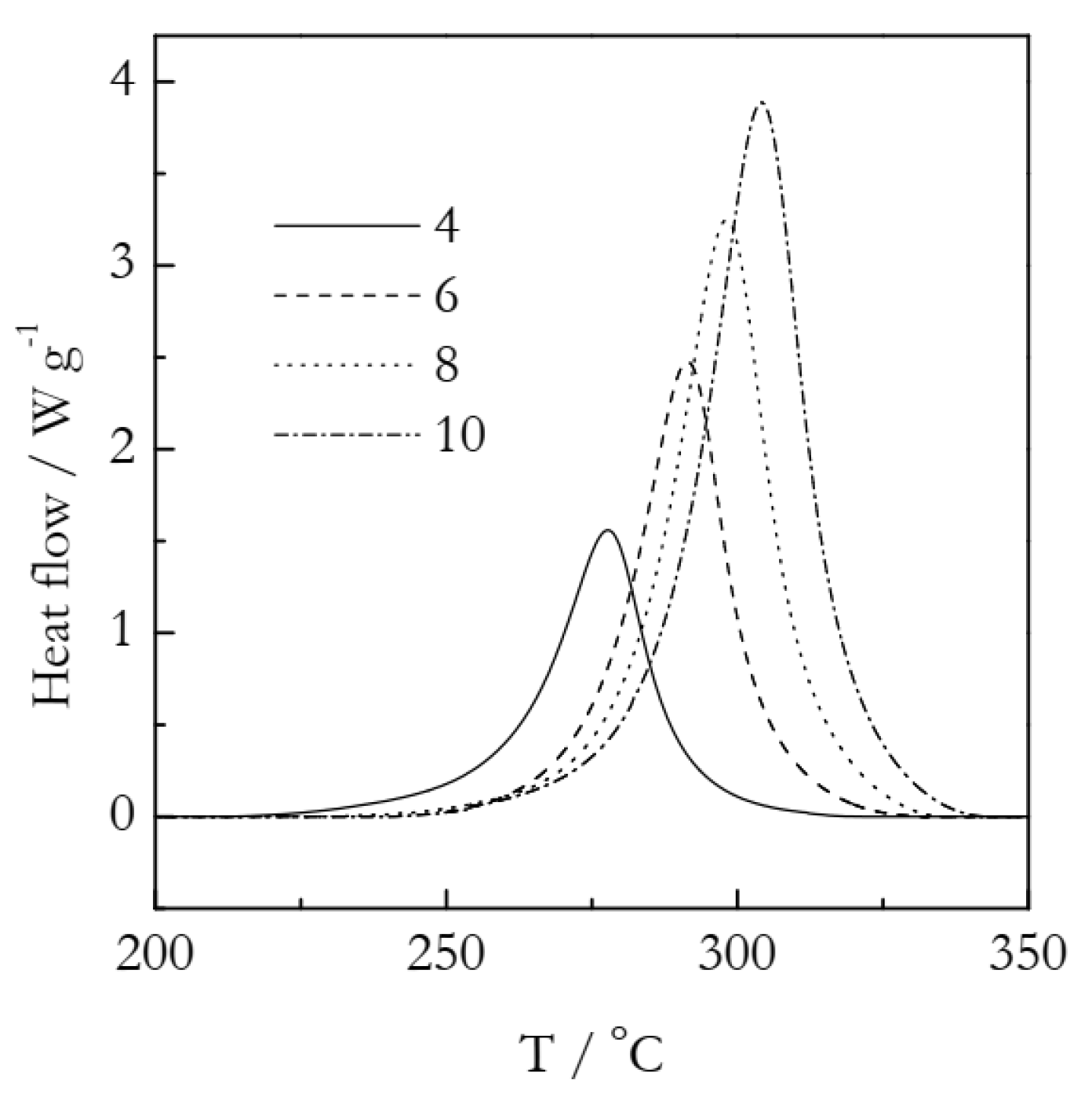
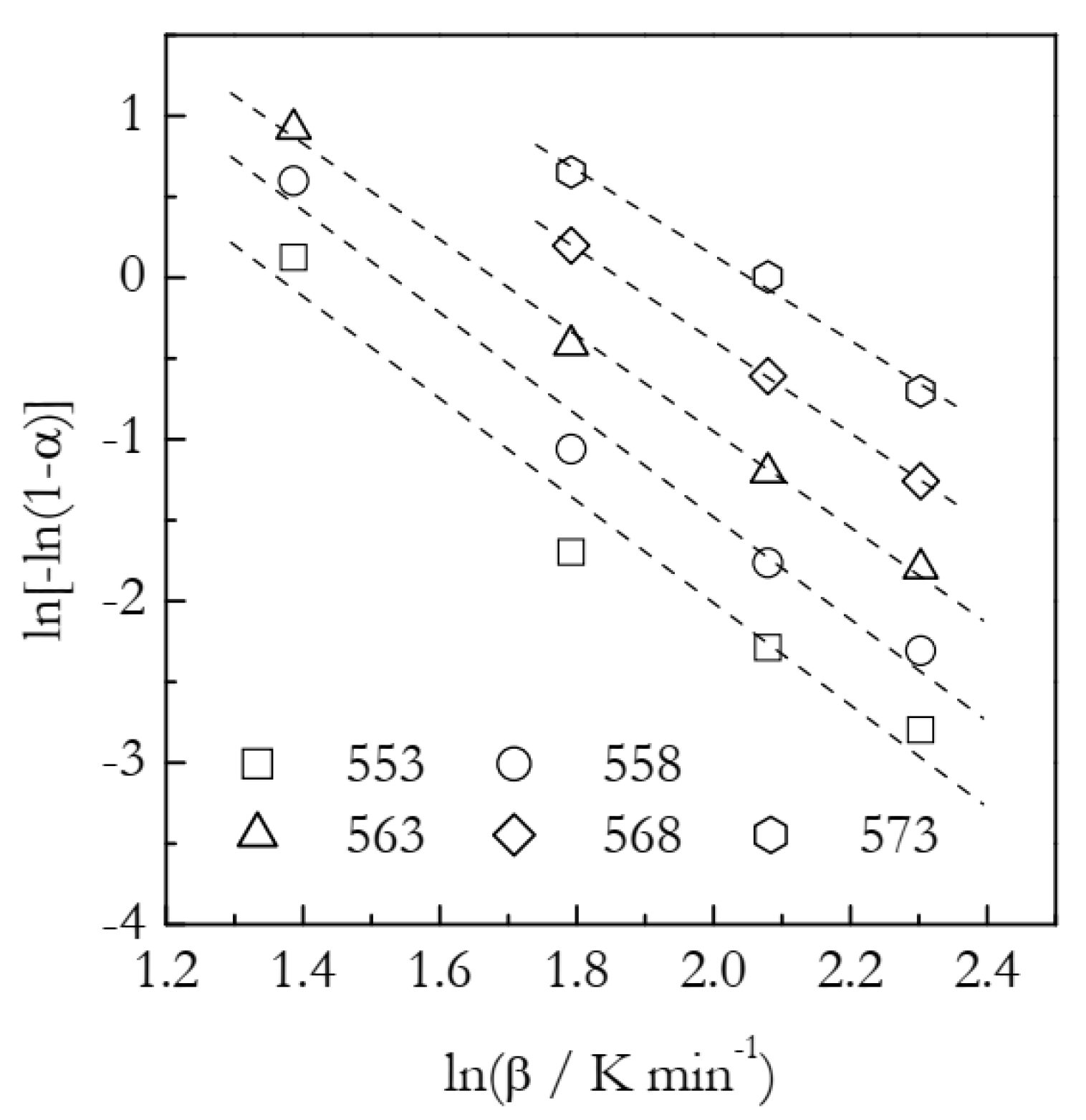
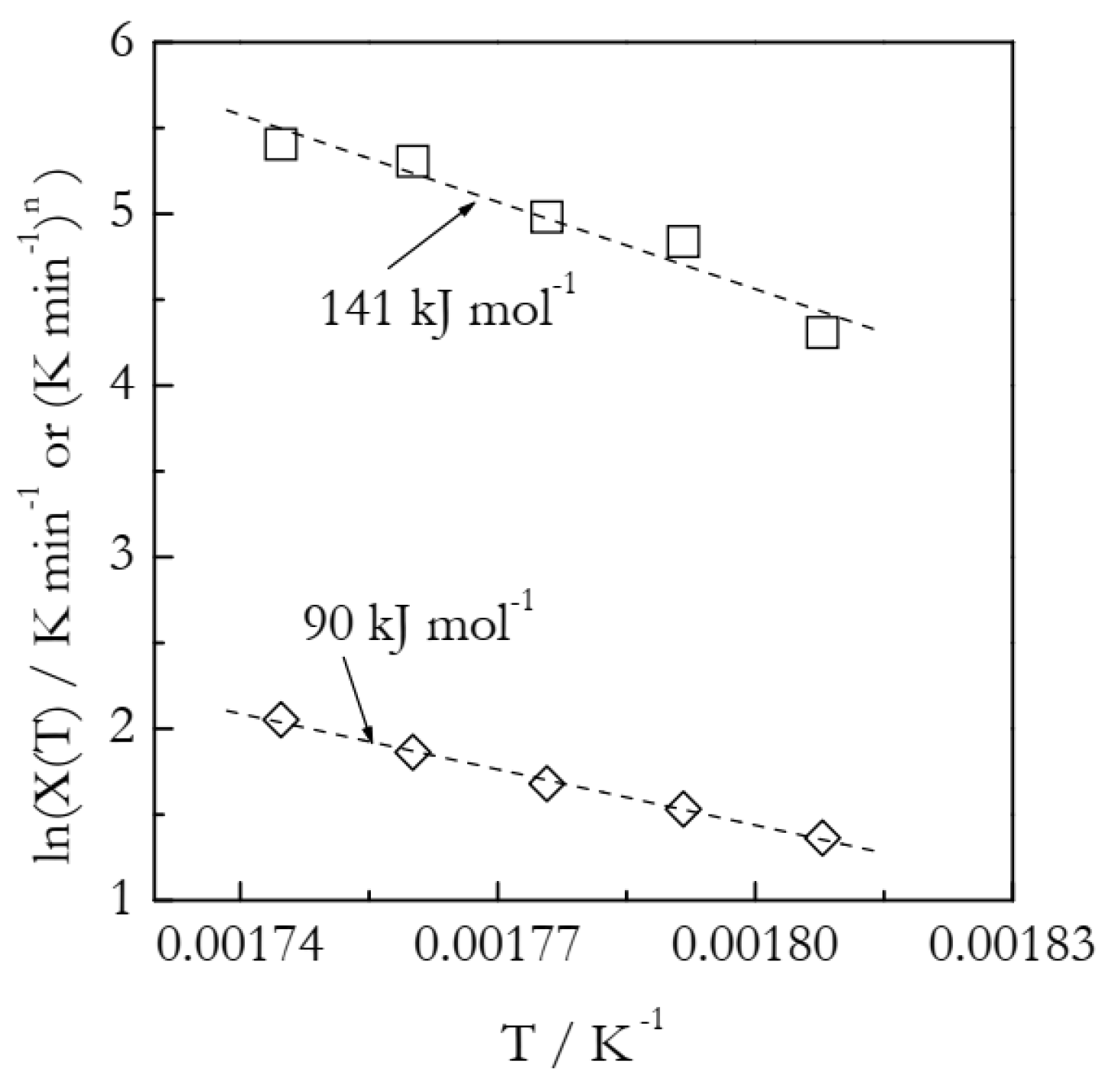
6. Analysis of Experimental Data
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Schultz, J. Polymer Crystallization; ACS & Oxford University Press: New York, NY, USA, 2001. [Google Scholar]
- Mandelkern, L. Crystallization of Polymers: Kinetics and Mechanisms, 2nd ed.; Cambridge University Press: Cambridge, UK, 2004; Volume 2. [Google Scholar]
- Djabourov, M.; Papon, P. Influence of thermal treatments on the structure and stability of gelatin gels. Polymer 1983, 24, 537–542. [Google Scholar] [CrossRef]
- Huang, X.; Terech, P.; Raghavan, S.R.; Weiss, R.G. Kinetics of 5α-Cholestan-3β-yl N-(2-Naphthyl)carbamate/n-Alkane Organogel Formation and Its Influence on the Fibrillar Networks. J. Am. Chem. Soc. 2005, 127, 4336–4344. [Google Scholar] [CrossRef] [PubMed]
- Nasr, P.; Leung, H.; Auzanneau, F.I.; Rogers, M.A. Supramolecular Fractal Growth of Self-Assembled Fibrillar Networks. Gels 2021, 7, 46. [Google Scholar] [CrossRef]
- Raposo, M.; Oliveira, O.N. Adsorption of Poly(o-methoxyaniline) in Layer-by-Layer Films. Langmuir 2002, 18, 6866–6874. [Google Scholar] [CrossRef]
- Cestari, A.R.; Vieira, E.F.S.; Vieira, G.S.; Almeida, L.E. The removal of anionic dyes from aqueous solutions in the presence of anionic surfactant using aminopropylsilica—A kinetic study. J. Hazard. Mater. 2006, 138, 133–141. [Google Scholar] [CrossRef]
- Vargas, A.M.M.; Cazetta, A.L.; Kunita, M.H.; Silva, T.L.; Almeida, V.C. Adsorption of methylene blue on activated carbon produced from flamboyant pods (Delonix regia): Study of adsorption isotherms and kinetic models. Chem. Eng. J. 2011, 168, 722–730. [Google Scholar] [CrossRef]
- Serna-Guerrero, R.; Sayari, A. Modeling adsorption of CO2 on amine-functionalized mesoporous silica. 2: Kinetics and breakthrough curves. Chem. Eng. J. 2010, 161, 182–190. [Google Scholar] [CrossRef]
- Kole, K.; Das, S.; Samanta, A.; Jana, S. Parametric Study and Detailed Kinetic Understanding of CO2 Adsorption over High-Surface-Area Flowery Silica Nanomaterials. Ind. Eng. Chem. Res. 2020, 59, 21393–21402. [Google Scholar] [CrossRef]
- Zhang, W.Z.; Chen, X.D.; Luo, W.-a.; Yang, J.; Zhang, M.Q.; Zhu, F.M. Study of Phase Separation of Poly(vinyl methyl ether) Aqueous Solutions with Rayleigh Scattering Technique. Macromolecules 2009, 42, 1720–1725. [Google Scholar] [CrossRef]
- Lo Nostro, P.; Giustini, L.; Fratini, E.; Ninham, B.W.; Ridi, F.; Baglioni, P. Threading, Growth, and Aggregation of Pseudopolyrotaxanes. J. Phys. Chem. B 2008, 112, 1071–1081. [Google Scholar] [CrossRef]
- Fibich, G. Bass-SIR model for diffusion of new products in social networks. Phys. Rev. E 2016, 94, 032305. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Irzhak, T.F.; Mezhikovskii, S.M.; Irzhak, V.I. The physical meaning of the Avrami equation in oligomer curing reactions. Polym. Sci. Ser. B 2008, 50, 201–203. [Google Scholar] [CrossRef]
- Pollard, M.; Kardos, J.L. Analysis of epoxy resin curing kinetics using the Avrami theory of phase change. Polym. Eng. Sci. 1987, 27, 829–836. [Google Scholar] [CrossRef]
- Lu, M.G.; Shim, M.J.; Kim, S.W. The macrokinetic model of thermosetting polymers by phase-change theory. Mater. Chem. Phys. 1998, 56, 193–197. [Google Scholar] [CrossRef]
- Lu, M.G.; Shim, M.J.; Kim, S.W. Curing behavior of an unsaturated polyester system analyzed by Avrami equation. Thermochim. Acta 1998, 323, 37–42. [Google Scholar] [CrossRef]
- Kim, S.-W.; Lu, M.-G.; Shim, M.-J. The Isothermal Cure Kinetic of Epoxy/Amine System Analyzed by Phase Change Theory. Polym. J. 1998, 30, 90–94. [Google Scholar] [CrossRef]
- Lu, M.; Shim, M.; Kim, S. Effect of filler on cure behavior of an epoxy system: Cure modeling. Polym. Eng. Sci. 1999, 39, 274–285. [Google Scholar] [CrossRef]
- Ozawa, T. Kinetics of non-isothermal crystallization. Polymer 1971, 12, 150–158. [Google Scholar] [CrossRef]
- Lu, M.G.; Shim, M.J.; Kim, S.W. Dynamic DSC Characterization of Epoxy Resin by Means of the Avrami Equation. J. Therm. Anal. Calorim. 1999, 58, 701–709. [Google Scholar] [CrossRef]
- Xin, C.; Yang, X.; Yu, D. Non-isothermal Cure Kinetics of Polybenzoxazine/Carbon Fiber Composites by Phase Change Theory. Polym. Polym. Compos. 2005, 13, 599–605. [Google Scholar] [CrossRef]
- Hong Zhang, X.; Qin Min, Y.; Zhao, H.; Mei Wan, H.; Rong Qi, G. Novel nitrogen-containing epoxy resin. II. Cure kinetics by differential scanning calorimetry. J. Appl. Polym. Sci. 2006, 100, 3483–3489. [Google Scholar] [CrossRef]
- Janeczek, H.; Siwy, M.; Schab-Balcerzak, E. Polymers based on N,N-diglycidylaniline. I. Investigations of the curing kinetics by dynamic differential scanning calorimetry measurements. J. Appl. Polym. Sci. 2009, 113, 3596–3604. [Google Scholar] [CrossRef]
- Liu, Q.Y.; Chen, J.B.; Liu, S.M.; Zhao, J.Q. Dynamic cure kinetics of epoxy resins using an amine-containing borate as a latent hardener. Polym. Int. 2012, 61, 959–965. [Google Scholar] [CrossRef]
- Wang, W.; Di, N.Y.; Cao, W.R.; Liu, X.D.; Yao, J.M. Cure kinetics of epoxy resin using 1,2,4,5-benzenetetracarboxylic acid/2-ethyl-4-methylimidazole salt as a latent hardener. Mater. Res. Innov. 2015, 19, 502–507. [Google Scholar] [CrossRef]
- Cao, H.; Liu, B.; Ye, Y.; Liu, Y.; Li, P. Reconstruction of the Microstructure of Cyanate Ester Resin by Using Prepared Cyanate Ester Resin Nanoparticles and Analysis of the Curing Kinetics Using the Avrami Equation of Phase Change. Appl. Sci. 2019, 9, 2365. [Google Scholar] [CrossRef] [Green Version]
- Prime, R.B. Thermosets. In Thermal Characterization of Polymeric Materials; Turi, E.A., Ed.; Academic Press: Cambridge, MA, USA, 1997; pp. 1380–1766. [Google Scholar] [CrossRef]
- Yousefi, A.; Lafleur, P.G.; Gauvin, R. Kinetic studies of thermoset cure reactions: A review. Polym. Compos. 1997, 18, 157–168. [Google Scholar] [CrossRef]
- Vyazovkin, S.; Sbirrazzuoli, N. Kinetic methods to study isothermal and nonisothermal epoxy-anhydride cure. Macromol. Chem. Phys. 1999, 200, 2294–2303. [Google Scholar] [CrossRef]
- Vyazovkin, S.; Burnham, A.K.; Criado, J.M.; Perez-Maqueda, L.A.; Popescu, C.; Sbirrazzuoli, N. ICTAC Kinetics Committee recommendations for performing kinetic computations on thermal analysis data. Thermochim. Acta 2011, 520, 1–19. [Google Scholar] [CrossRef]
- De Keer, L.; Kilic, K.I.; Van Steenberge, P.H.M.; Daelemans, L.; Kodura, D.; Frisch, H.; De Clerck, K.; Reyniers, M.-F.; Barner-Kowollik, C.; Dauskardt, R.H.; et al. Computational prediction of the molecular configuration of three-dimensional network polymers. Nat. Mater. 2021, 20, 1422–1430. [Google Scholar] [CrossRef]
- Borchardt, H.J.; Daniels, F. The Application of Differential Thermal Analysis to the Study of Reaction Kinetics1. J. Am. Chem. Soc. 1957, 79, 41–46. [Google Scholar] [CrossRef]
- Šesták, J. Ignoring heat inertia impairs accuracy of determination of activation energy in thermal analysis. Int. J. Chem. Kinet. 2019, 51, 74–80. [Google Scholar] [CrossRef] [Green Version]
- Vyazovkin, S. How much is the accuracy of activation energy affected by ignoring thermal inertia? Int. J. Chem. Kinet. 2020, 52, 23–28. [Google Scholar] [CrossRef]
- Vyazovkin, S. Activation Energies and Temperature Dependencies of the Rates of Crystallization and Melting of Polymers. Polymers 2020, 12, 1070. [Google Scholar] [CrossRef] [PubMed]
- Bruijn, T.J.W.D.; Jong, W.A.d.; Berg, P.J. Kinetic parameters in Avrami-Erofeev type reactions from isothermal and non-isothermal experiments. Thermochim. Acta 1981, 45, 315–325. [Google Scholar] [CrossRef]
- Yinnon, H.; Uhlmann, D.R. Applications of thermoanalytical techniques to the study of crystallization kinetics in glass-forming liquids, part I: Theory. J. Non-Cryst. Solids 1983, 54, 253–275. [Google Scholar] [CrossRef]
- Fatemi, N.; Whitehead, R.; Price, D.; Dollimore, D. Some comments on the use of Avrami-Erofeev expressions and solid state decomposition rate constants. Thermochim. Acta 1986, 104, 93–100. [Google Scholar] [CrossRef]
- Khanna, Y.P.; Taylor, T.J. Comments and recommendations on the use of the Avrami equation for physico-chemical kinetics. Polym. Eng. Sci. 1988, 28, 1042–1045. [Google Scholar] [CrossRef]
- Brown, M.E.; Galwey, A.K. Arrhenius parameters for solid-state reactions from isothermal rate-time curves. Anal. Chem. 1989, 61, 1136–1139. [Google Scholar] [CrossRef]
- Jackson, K.A. Kinetic Processes. Crystal Growth, Diffusion, and Phase Transitions in Materials; Wiley-VCH: Weinheim, Germany, 2004; p. 453. [Google Scholar]
- Vyazovkin, S. Nonisothermal crystallization of polymers: Getting more out of kinetic analysis of differential scanning calorimetry data. Polym. Cryst. 2018, 1, e10003. [Google Scholar] [CrossRef]
- Haudin, J.M.; Billon, N. Solidification of semi-crystalline polymers during melt processing. Progr. Colloid Polym. Sci. 1992, 87, 132–137. [Google Scholar]
- Hieber, C.A. Correlations for the quiescent crystallization kinetics of isotactic polypropylene and poly(ethylene terephthalate). Polymer 1995, 36, 1455–1467. [Google Scholar] [CrossRef]
- Sajkiewicz, P.; Carpaneto, L.; Wasiak, A. Application of the Ozawa model to non-isothermal crystallization of poly(ethylene terephthalate). Polymer 2001, 42, 5365–5370. [Google Scholar] [CrossRef]
- Zhang, Z.; Xiao, C.; Dong, Z. Comparison of the Ozawa and modified Avrami models of polymer crystallization under nonisothermal conditions using a computer simulation method. Thermochim. Acta 2007, 466, 22–28. [Google Scholar] [CrossRef]
- Vyazovkin, S. Isoconversional Kinetics of Thermally Stimulated Processes; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Senum, G.I.; Yang, R.T. Rational approximations of integral of Arrhenius function. J. Therm. Anal. 1977, 11, 445–449. [Google Scholar] [CrossRef]
- Flynn, J.H. The ‘Temperature Integral’—Its use and abuse. Thermochim. Acta 1997, 300, 83–92. [Google Scholar] [CrossRef]
- Doyle, C.D. Kinetic analysis of thermogravimetric data. J. Appl. Polym. Sci. 1961, 5, 285–292. [Google Scholar] [CrossRef]
- Vyazovkin, S. Modification of the integral isoconversional method to account for variation in the activation energy. J. Comput. Chem. 2001, 22, 178–183. [Google Scholar] [CrossRef]
- Vyazovkin, S. Determining Preexponential Factor in Model-Free Kinetic Methods: How and Why? Molecules 2021, 26, 3077. [Google Scholar] [CrossRef]
- Galukhin, A.; Nosov, R.; Taimova, G.; Islamov, D.; Vyazovkin, S. Synthesis and Polymerization Kinetics of Novel Dicyanate Ester Based on Dimer of 4-tert-butylphenol. Macromol. Chem. Phys. 2021, 222, 2000410. [Google Scholar] [CrossRef]
- Gao, X.; Chen, D.; Dollimore, D. The correlation between the value of α at the maximum reaction rate and the reaction mechanisms: A theoretical study. Thermochim. Acta 1993, 223, 75–82. [Google Scholar] [CrossRef]
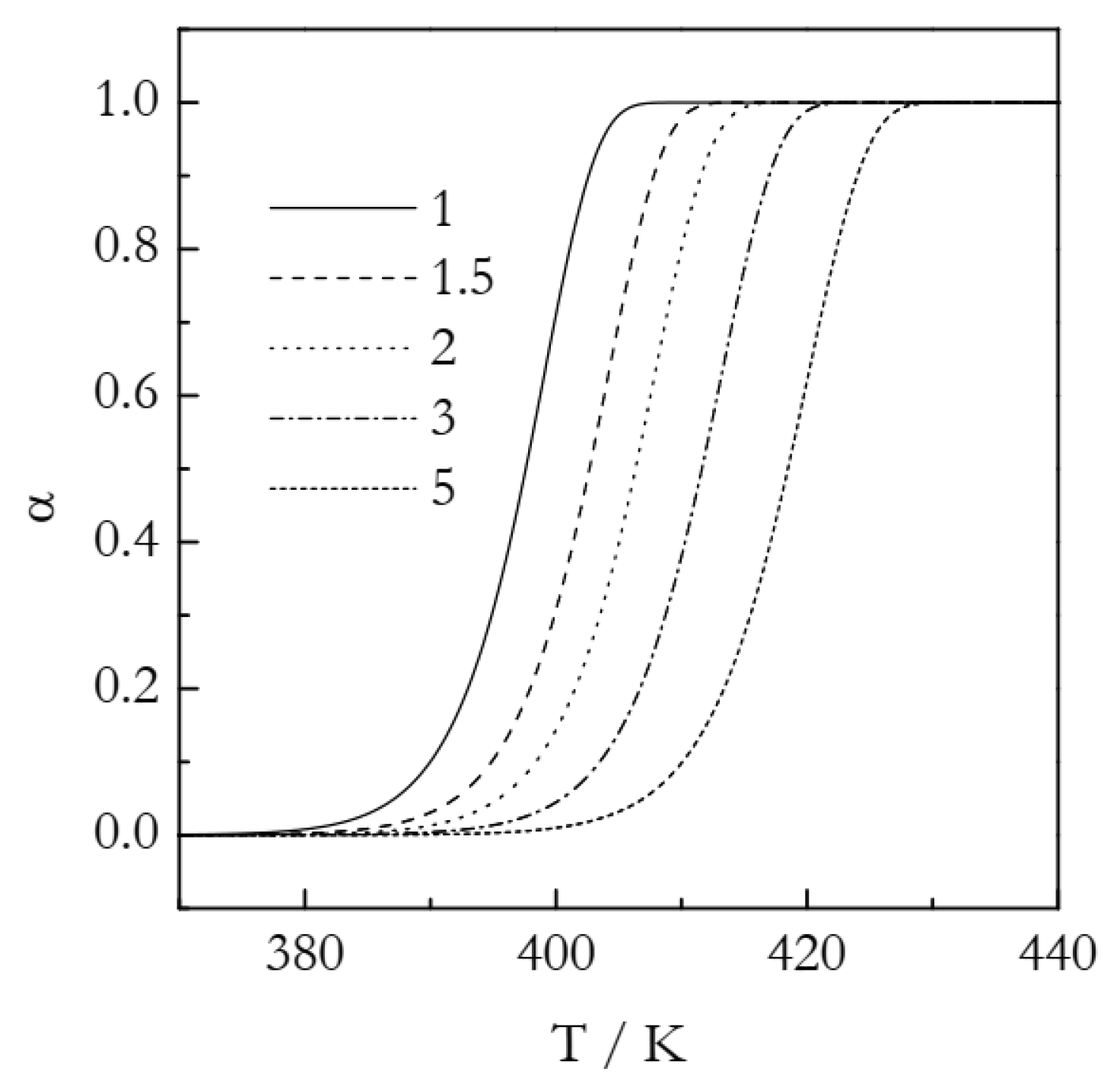
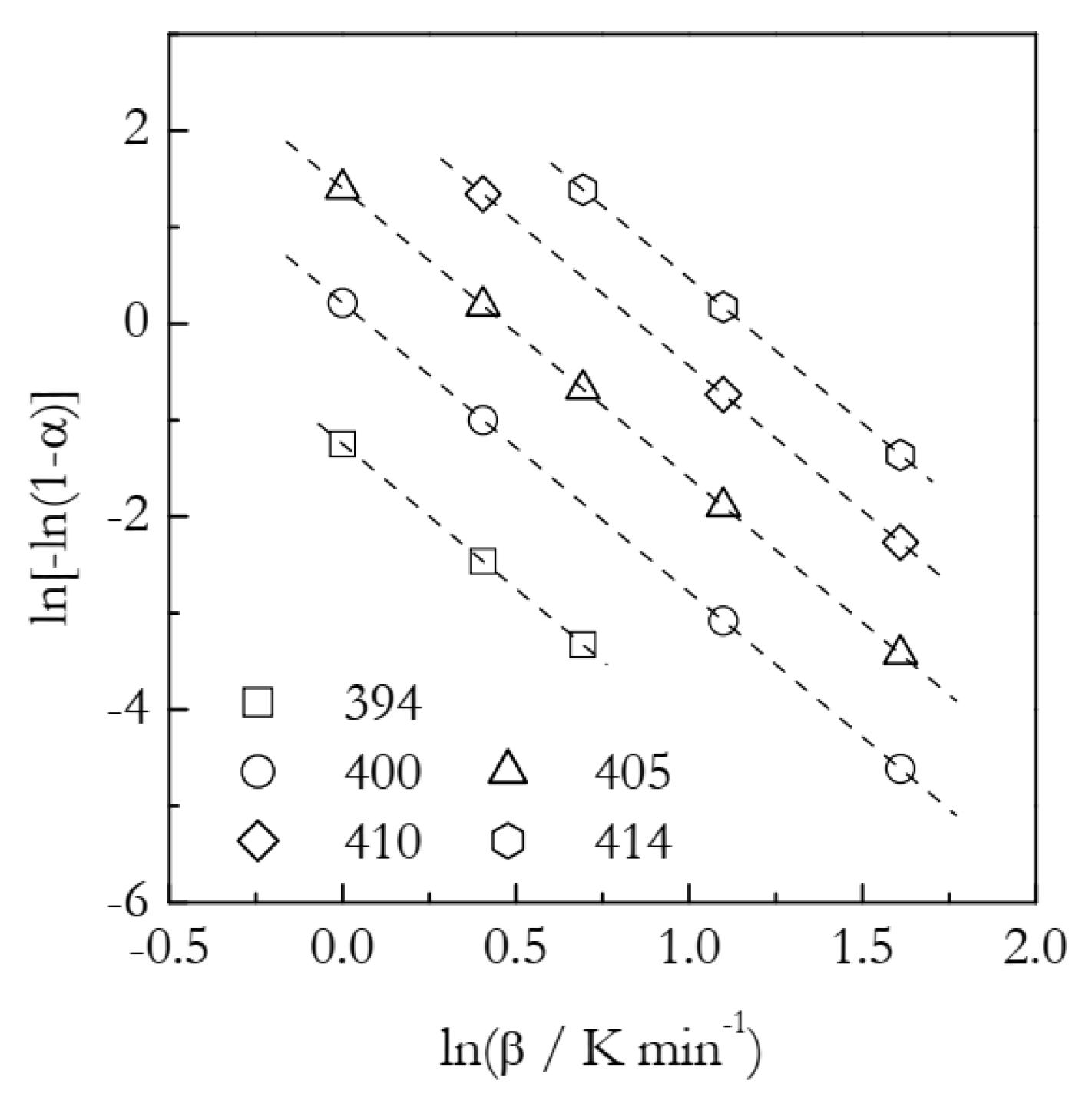
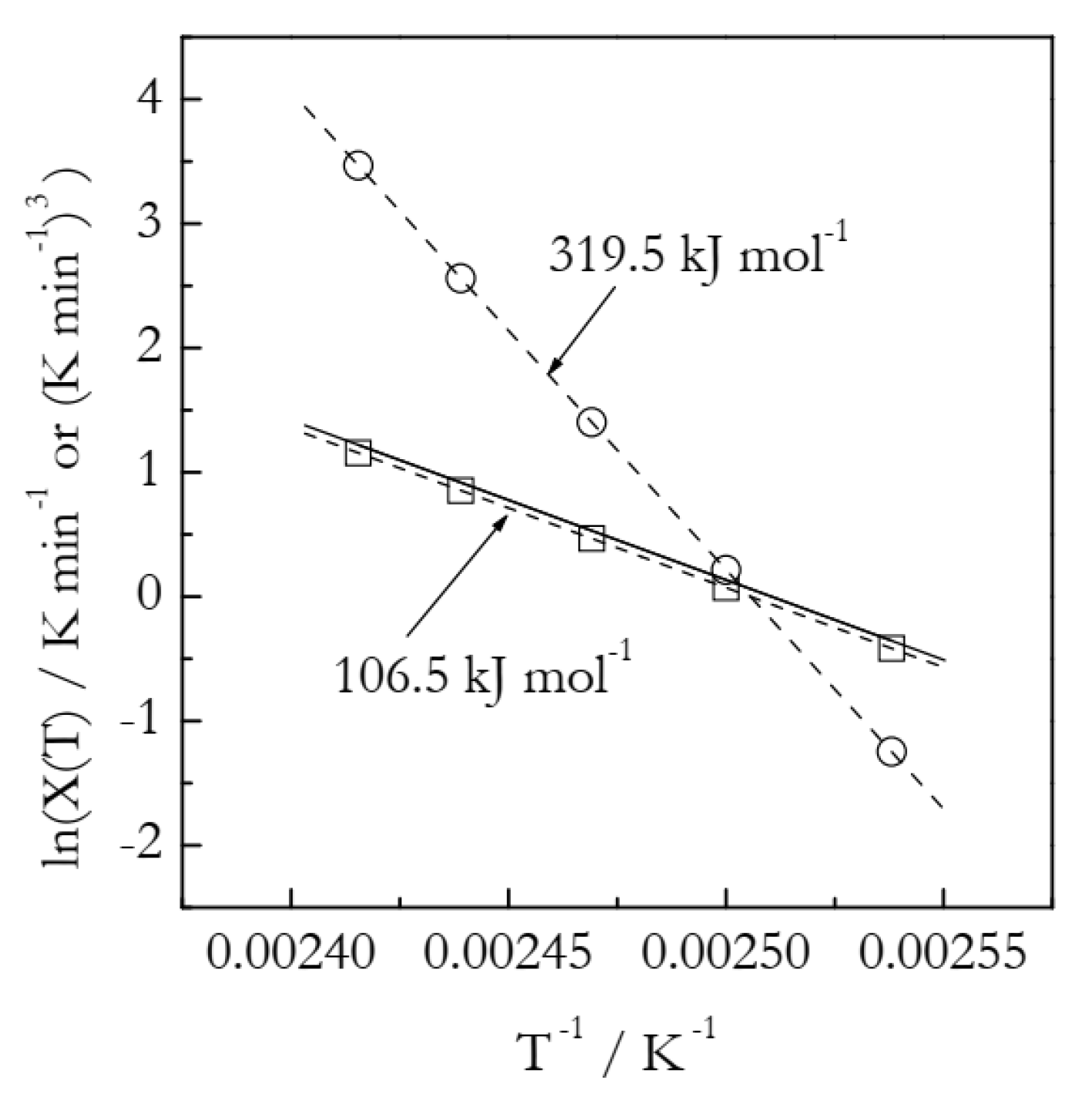


| T/K | n | ln [χ(T)/(K min−1)n] |
|---|---|---|
| 394 | 3.00019 | −1.24723 |
| 400 | 3.00009 | 0.21521 |
| 405 | 2.99992 | 1.4017 |
| 410 | 3.00002 | 2.56022 |
| 414 | 3.00005 | 3.46748 |
| T/K | n | ln [χ(T)/(K min−1)n] |
|---|---|---|
| 380 | 0.95570 | −1.69611 |
| 390 | 0.90559 | −0.93423 |
| 400 | 0.82293 | −0.2926 |
| 410 | 0.71248 | 0.21716 |
| 420 | 0.59298 | 0.60641 |
| 430 | 0.48454 | 0.90207 |
| T/K | n | ln [χ(T)/(K min−1)n] |
|---|---|---|
| 553 | 3.1599 | 4.308 |
| 558 | 3.1596 | 4.8384 |
| 563 | 2.9663 | 4.9814 |
| 568 | 2.8487 | 5.3061 |
| 573 | 2.6340 | 5.4057 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vyazovkin, S.; Galukhin, A. Problems with Applying the Ozawa–Avrami Crystallization Model to Non-Isothermal Crosslinking Polymerization. Polymers 2022, 14, 693. https://doi.org/10.3390/polym14040693
Vyazovkin S, Galukhin A. Problems with Applying the Ozawa–Avrami Crystallization Model to Non-Isothermal Crosslinking Polymerization. Polymers. 2022; 14(4):693. https://doi.org/10.3390/polym14040693
Chicago/Turabian StyleVyazovkin, Sergey, and Andrey Galukhin. 2022. "Problems with Applying the Ozawa–Avrami Crystallization Model to Non-Isothermal Crosslinking Polymerization" Polymers 14, no. 4: 693. https://doi.org/10.3390/polym14040693
APA StyleVyazovkin, S., & Galukhin, A. (2022). Problems with Applying the Ozawa–Avrami Crystallization Model to Non-Isothermal Crosslinking Polymerization. Polymers, 14(4), 693. https://doi.org/10.3390/polym14040693







