The Role of Eucalyptus Species on the Structural and Thermal Performance of Cellulose Nanocrystals (CNCs) Isolated by Acid Hydrolysis
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.1.1. Eucalyptus Wood Samples
2.1.2. Chemicals and Reagents
2.1.3. Kraft Pulping and Bleaching
2.2. Characterization of Pulp
2.3. Production of Cellulose Nanocrystals (CNCs)
2.4. Characterization of Cellulose Nanocrystals
2.4.1. Chemical Oxygen Demand (COD)
2.4.2. Field-Emission Scanning Electron Microscopy (FE-SEM)
2.4.3. Fourier-Transform Infrared Spectroscopy (FTIR)
2.4.4. X-ray Diffraction (XRD)
2.4.5. Differential Scanning Calorimetry (DSC)
2.4.6. Thermogravimetric Analysis (TGA)
2.4.7. Kinetic Analysis for the Thermal Decomposition
3. Results and Discussion
3.1. From Eucalyptus Pulp to Cellulose Nanocrystals (CNCs)
3.2. Cellulose Nanocrystals (CNCs)
3.2.1. Morphology
3.2.2. Chemical Structure
3.2.3. Crystalline Structure
3.2.4. Thermal Properties and Thermal Stability
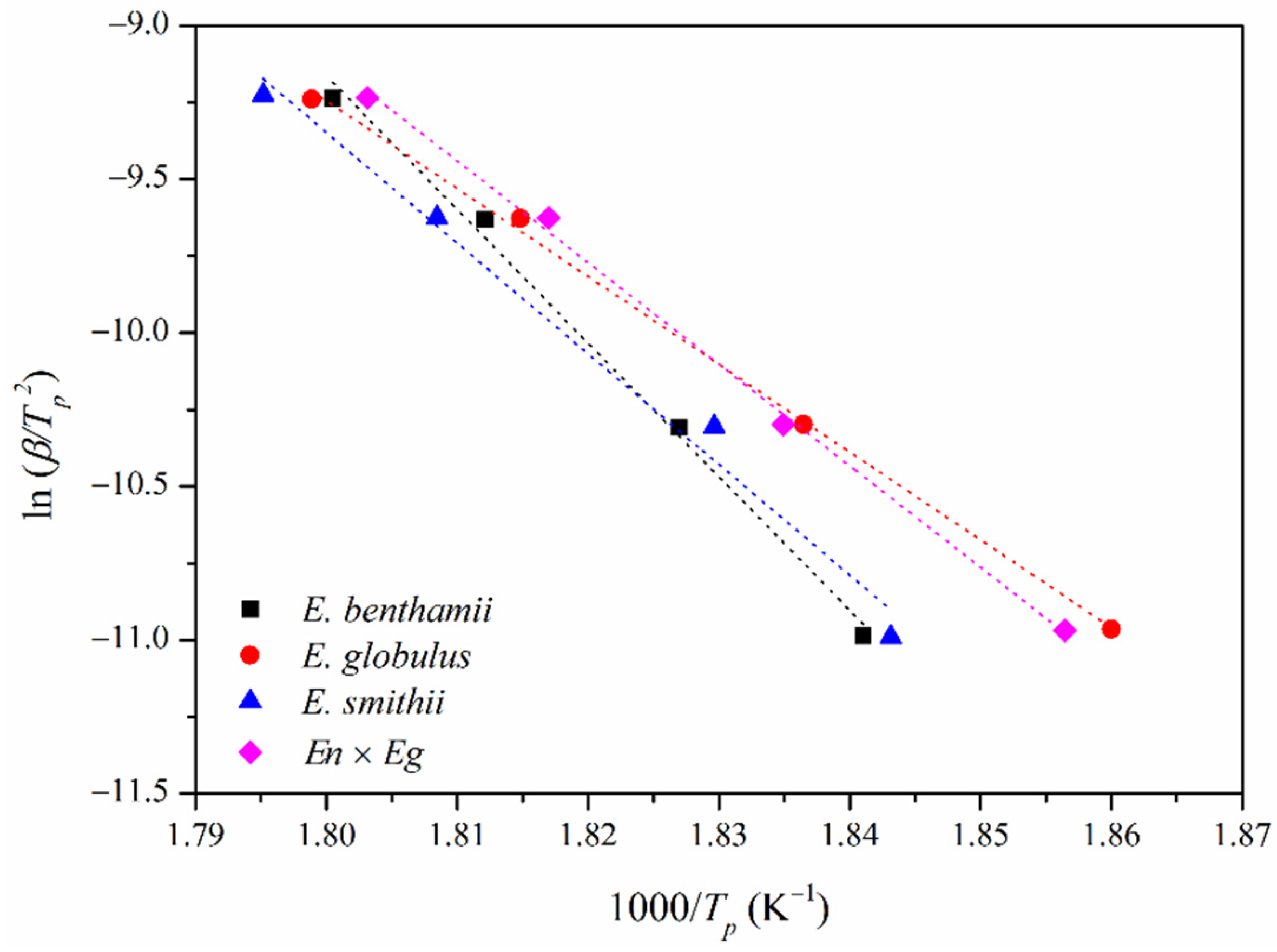
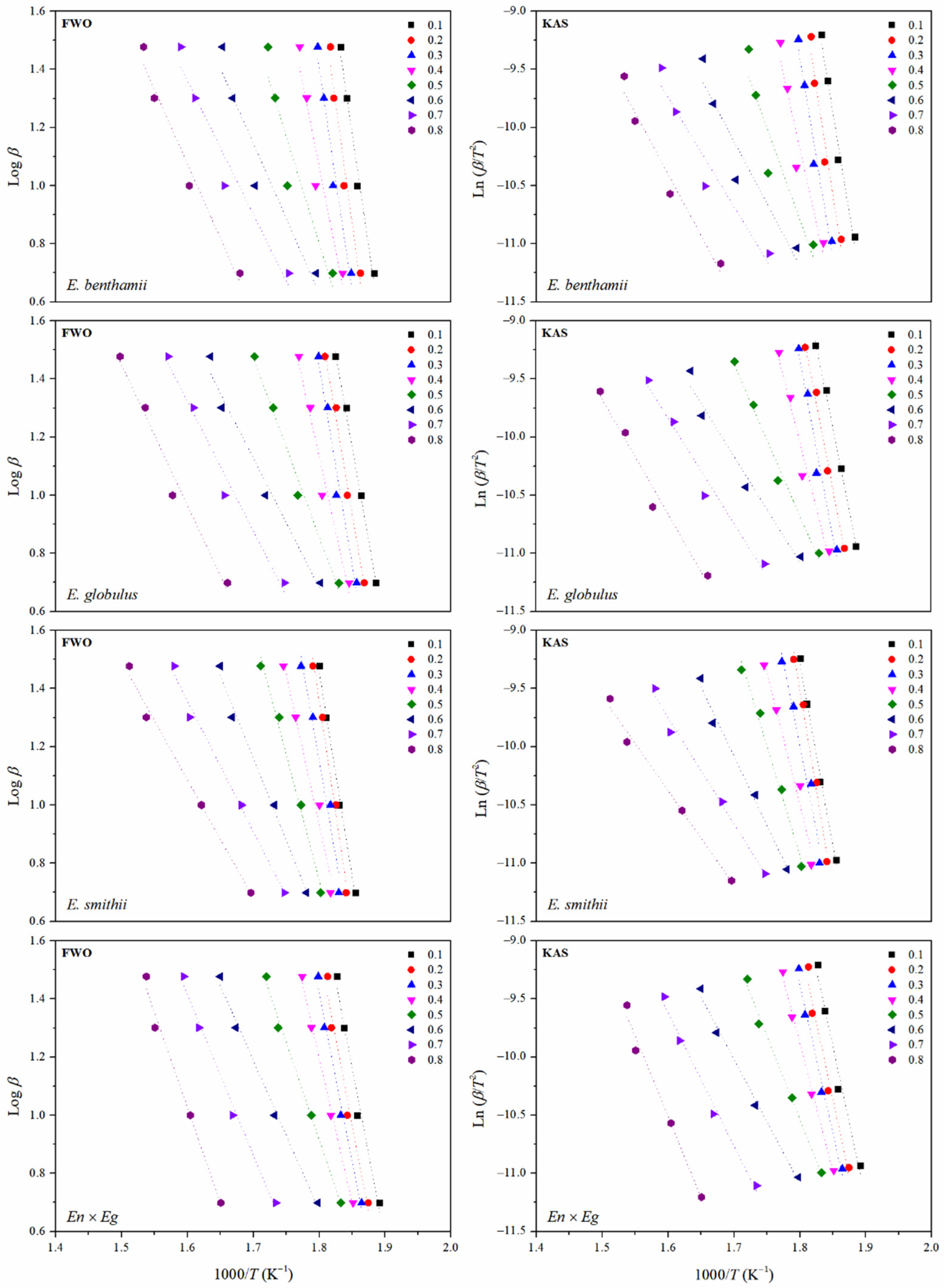
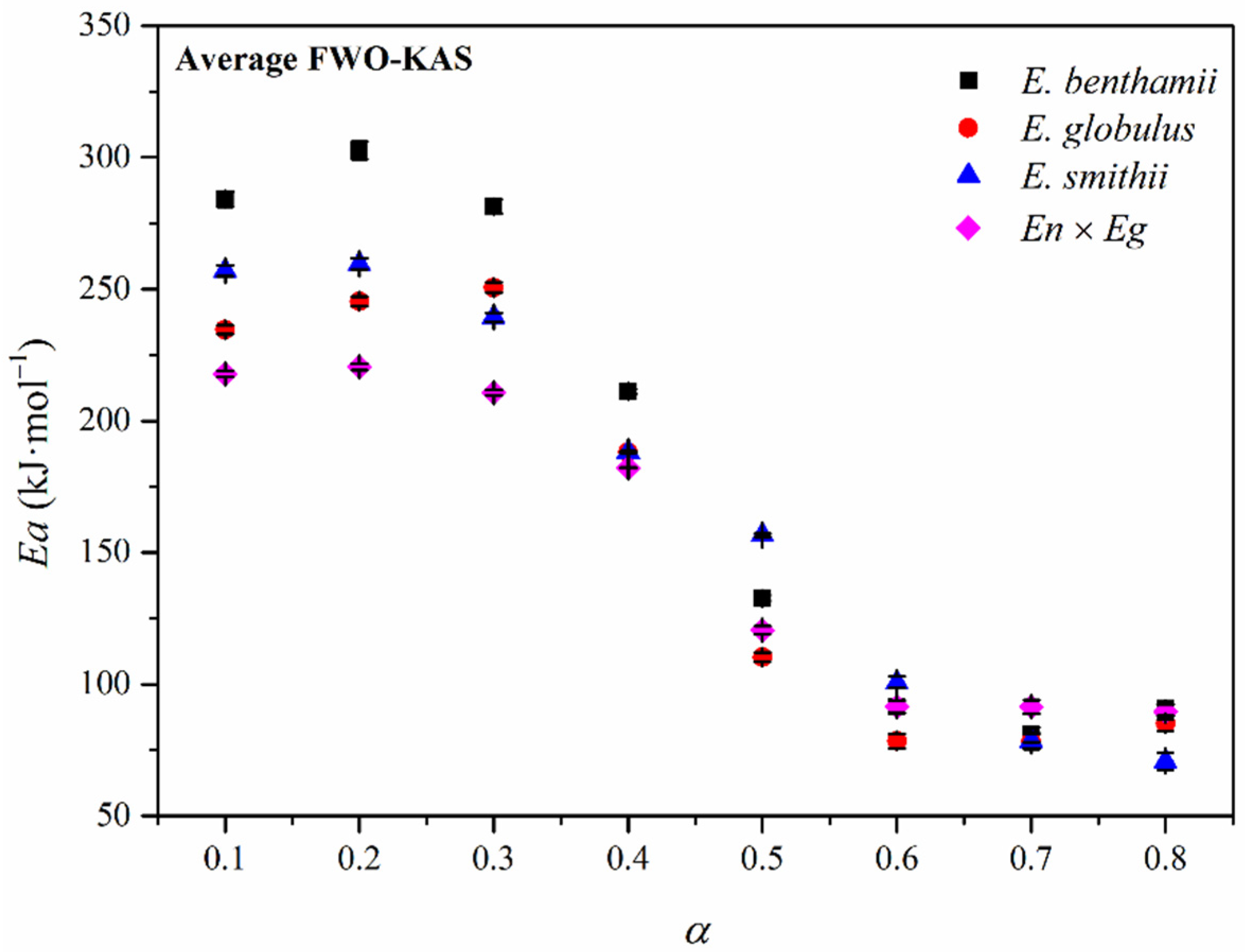
3.3. Kinetic Analysis and Apparent Activation Energy during Pyrolysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Carrillo, I.; Valenzuela, S.; Elissetche, J.P. Comparative evaluation of Eucalyptus globulus and E. nitens wood and fibre quality. IAWA J. 2017, 38, 105–116. [Google Scholar] [CrossRef]
- Carrillo, I.; Vidal, C.; Elissetche, J.P.; Mendonça, R.T. Wood anatomical and chemical properties related to the pulpability of Eucalyptus globulus: A review. South. For. 2018, 80, 1–8. [Google Scholar] [CrossRef]
- Sixta, H. Handbook of Pulp; Sixta, H., Ed.; Wiley-VCH Verlag GmbH & Co. KGaA: Wenheim, Germany, 2008; Volume 1–2, ISBN 3527309993. [Google Scholar]
- Clarke, C.R.E.; Palmer, B.; Gounden, D. Understanding and adding value to Eucalyptus fibre. South. For. 2008, 70, 169–174. [Google Scholar] [CrossRef]
- Gellerstedt, G.; Ek, M.; Henriksson, G. Wood Chemistry and Wood Biotechnology; Ek, M., Gellerstedt, G., Henriksson, G., Eds.; Walter de Gruyter: Berlin, Germany, 2009; ISBN 9783110213409. [Google Scholar]
- González, C.K.; Caselli, A.J.G.; González, V.A.; Pardo, V.E. Mercado forestal Junio 2021; INFOR: New York, NY, USA, 2021. [Google Scholar]
- Carrillo, I.; Mendonça, R.T.; Ago, M.; Rojas, O.J. Comparative study of cellulosic components isolated from different Eucalyptus species. Cellulose 2018, 25, 1011–1029. [Google Scholar] [CrossRef]
- Rencoret, J.; Suárez, A.G.; Río Andrade, J.C. Chemical composition of different eucalyptus wood species used for paper pulp manufacturing. In Proceedings of the 9th European Workshop on Lignocellulosics and Pulp, Viena, Austria, 27–30 August 2006; pp. 447–450. [Google Scholar]
- Mahmud, M.M.; Perveen, A.; Jahan, R.A.; Matin, M.A.; Wong, S.Y.; Li, X.; Arafat, M.T. Preparation of different polymorphs of cellulose from different acid hydrolysis medium. Int. J. Biol. Macromol. 2019, 130, 969–976. [Google Scholar] [CrossRef] [PubMed]
- Katiyar, V.; Dhar, P. Cellulose Nanocrystals: Emerging Bio-Precursors for Chemical Prcesses, Berlin, Boston; Walter de Gruyter: Berlin, Germany, 2020. [Google Scholar]
- Klemm, D.; Heublein, B.; Fink, H.-P.; Bohn, A. Cellulose: Fascinating Biopolymer and Sustainable Raw Material. Angew. Chemie Int. Ed. 2005, 44, 3358–3393. [Google Scholar] [CrossRef]
- Peng, B.L.; Dhar, N.; Liu, H.L.; Tam, K.C. Chemistry and applications of nanocrystalline cellulose and its derivatives: A nanotechnology perspective. Can. J. Chem. Eng. 2011, 89, 1191–1206. [Google Scholar] [CrossRef]
- Gars, M.L.; Douard, L.; Belgacem, N.; Bras, J. Cellulose Nanocrystals: From Classical Hydrolysis to the Use of Deep Eutectic Solvents. Smart Nanosyst. Biomed. Optoelectron. Catal. 2019, 1, 1–18. [Google Scholar]
- Rovera, C.; Ghaani, M.; Santo, N.; Trabattoni, S.; Olsson, R.T.; Romano, D.; Farris, S. Enzymatic Hydrolysis in the Green Production of Bacterial Cellulose Nanocrystals. ACS Sustain. Chem. Eng. 2018, 6, 7725–7734. [Google Scholar] [CrossRef]
- Habibi, Y.; Lucia, L.A.; Rojas, O.J. Cellulose Nanocrystals: Chemistry, Self-Assembly, and Applications. Chem. Rev. 2010, 110, 3479–3500. [Google Scholar] [CrossRef] [PubMed]
- Khairnar, Y.; Hansora, D.; Hazra, C.; Kundu, D.; Tayde, S.; Tonde, S.; Naik, J.; Chatterjee, A. Cellulose bionanocomposites for sustainable planet and people: A global snapshot of preparation, properties, and applications. Carbohydr. Polym. Technol. Appl. 2021, 2, 100065. [Google Scholar] [CrossRef]
- Frank, B.P.; Smith, C.; Caudill, E.R.; Lankone, R.S.; Carlin, K.; Benware, S.; Pedersen, J.A.; Fairbrother, D.H. Biodegradation of Functionalized Nanocellulose. Environ. Sci. Technol. 2021, 55, 10744–10757. [Google Scholar] [CrossRef]
- Fortunati, E.; Rinaldi, S.; Peltzer, M.; Bloise, N.; Visai, L.; Armentano, I.; Jiménez, A.; Latterini, L.; Kenny, J.M. Nano-biocomposite films with modified cellulose nanocrystals and synthesized silver nanoparticles. Carbohydr. Polym. 2014, 101, 1122–1133. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- George, J.; Sabapathi, S.N. Cellulose nanocrystals: Synthesis, functional properties, and applications. Nanotechnol. Sci. Appl. 2015, 8, 45–54. [Google Scholar] [CrossRef] [Green Version]
- Fatona, A.; Berry, R.M.; Brook, M.A.; Moran-Mirabal, J.M. Versatile Surface Modification of Cellulose Fibers and Cellulose Nanocrystals through Modular Triazinyl Chemistry. Chem. Mater. 2018, 30, 2424–2435. [Google Scholar] [CrossRef]
- Abraham, E.; Weber, D.E.; Sharon, S.; Lapidot, S.; Shoseyov, O. Multifunctional cellulosic scaffolds from modified cellulose nanocrystals. ACS Appl. Mater. Interfaces 2017, 9, 2010–2015. [Google Scholar] [CrossRef] [PubMed]
- Abraham, E.; Kam, D.; Nevo, Y.; Slattegard, R.; Rivkin, A.; Lapidot, S.; Shoseyov, O. Highly Modified Cellulose Nanocrystals and Formation of Epoxy-Nanocrystalline Cellulose (CNC) Nanocomposites. ACS Appl. Mater. Interfaces 2016, 8, 28086–28095. [Google Scholar] [CrossRef] [PubMed]
- Yue, L.; Maiorana, A.; Khelifa, F.; Patel, A.; Raquez, J.M.; Bonnaud, L.; Gross, R.; Dubois, P.; Manas-Zloczower, I. Surface-modified cellulose nanocrystals for biobased epoxy nanocomposites. Polymer 2018, 134, 155–162. [Google Scholar] [CrossRef]
- Carrillo-Varela, I.; Retamal, R.; Pereira, M.; Mendonça, R.T. Structure and reactivity of cellulose from bleached kraft pulps of different Eucalyptus species upgraded to dissolving pulp. Cellulose 2019, 26, 5731–5744. [Google Scholar] [CrossRef]
- Li, H.; Legere, S.; He, Z.; Zhang, H.; Li, J.; Yang, B.; Zhang, S.; Zhang, L.; Zheng, L.; Ni, Y. Methods to increase the reactivity of dissolving pulp in the viscose rayon production process: A review. Cellulose 2018, 25, 3733–3753. [Google Scholar] [CrossRef]
- Dou, X.; Tang, Y. The Influence of Cold Caustic Extraction on the Purity, Accessibility and Reactivity of Dissolving-Grade Pulp. ChemistrySelect 2017, 2, 11462–11468. [Google Scholar] [CrossRef]
- Li, J.; Zhang, S.; Li, H.; Ouyang, X.; Huang, L.; Ni, Y.; Chen, L. Cellulase pretreatment for enhancing cold caustic extraction-based separation of hemicelluloses and cellulose from cellulosic fibers. Bioresour. Technol. 2018, 251, 1–6. [Google Scholar] [CrossRef]
- Gan, P.G.; Sam, S.T.; Abdullah, M.F.; Omar, M.F. Thermal properties of nanocellulose-reinforced composites: A review. J. Appl. Polym. Sci. 2020, 137, 48544. [Google Scholar] [CrossRef] [Green Version]
- Roman, M.; Winter, W.T. Effect of sulfate groups from sulfuric acid hydrolysis on the thermal degradation behavior of bacterial cellulose. Biomacromolecules 2004, 5, 1671–1677. [Google Scholar] [CrossRef]
- Corradini, E.; Pineda, E.A.G.; Corrêa, A.C.; Teixeira, E.M.; Mattoso, L.H.C. Thermal stability of cellulose nanocrystals from curaua fiber isolated by acid hydrolysis. Cell. Chem. Technol 2016, 50, 737–743. [Google Scholar]
- Merlini, A.; Claumann, C.; Zibetti, A.W.; Coirolo, A.; Rieg, T.; Machado, R.A.F. Kinetic Study of the Thermal Decomposition of Cellulose Nanocrystals with Different Crystal Structures and Morphologies. Ind. Eng. Chem. Res. 2020, 59, 13428–13439. [Google Scholar] [CrossRef]
- Henrique, M.A.; Neto, W.P.F.; Silvério, H.A.; Martins, D.F.; Gurgel, L.V.A.; da Silva Barud, H.; de Morais, L.C.; Pasquini, D. Kinetic study of the thermal decomposition of cellulose nanocrystals with different polymorphs, cellulose I and II, extracted from different sources and using different types of acids. Ind. Crops Prod. 2015, 76, 128–140. [Google Scholar] [CrossRef] [Green Version]
- Huang, S.; Zhou, L.; Li, M.-C.C.; Wu, Q.; Zhou, D. Cellulose nanocrystals (CNCs) from corn stalk: Activation energy analysis. Materials 2017, 10, 80. [Google Scholar] [CrossRef] [Green Version]
- Mendonça, R.T.; Jara, J.F.; González, V.; Elissetche, J.P.; Freer, J. Evaluation of the white-rot fungi Ganoderma australe and Ceriporiopsis subvermispora in biotechnological applications. J. Ind. Microbiol. Biotechnol. 2008, 35, 1323–1330. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.; Wang, Q.; Hirth, K.; Baez, C.; Agarwal, U.P.; Zhu, J.Y. Tailoring the yield and characteristics of wood cellulose nanocrystals (CNC) using concentrated acid hydrolysis. Cellulose 2015, 22, 1753–1762. [Google Scholar] [CrossRef]
- Bondeson, D.; Mathew, A.; Oksman, K. Optimization of the isolation of nanocrystals from microcrystalline cellulose by acid hydrolysis. Cellulose 2006, 13, 171–180. [Google Scholar] [CrossRef]
- Wang, Q.Q.; Zhu, J.Y.; Reiner, R.S.; Verrill, S.P.; Baxa, U.; McNeil, S.E. Approaching zero cellulose loss in cellulose nanocrystal (CNC) production: Recovery and characterization of cellulosic solid residues (CSR) and CNC. Cellulose 2012, 19, 2033–2047. [Google Scholar] [CrossRef]
- O’connor, R.T.; Dupré, E.F.; Mitcham, D. Applications of Infrared Absorption Spectroscopy to Investigations of Cotton and Modified Cottons:Part I: Physical and Crystalline Modifications and Oxidation. Text. Res. J. 1958, 28, 382–392. [Google Scholar] [CrossRef]
- Nelson, M.L.; O’Connor, R.T. Relation of certain infrared bands to cellulose crystallinity and crystal lattice type. Part II. A new infrared ratio for estimation of crystallinity in celluloses I and II. J. Appl. Polym. Sci. 1964, 8, 1325–1341. [Google Scholar] [CrossRef]
- Gil-Castell, O.; Badia, J.D.; Ribes-Greus, A. Suitability of blends from virgin and reprocessed polylactide: Performance and energy valorization kinetics. J. Renew. Mater. 2018, 6, 370–382. [Google Scholar] [CrossRef]
- Badia, J.D.; Santonja-Blasco, L.; Martínez-Felipe, A.; Ribes-Greus, A. A methodology to assess the energetic valorization of bio-based polymers from the packaging industry: Pyrolysis of reprocessed polylactide. Bioresour. Technol. 2012, 111, 468–475. [Google Scholar] [CrossRef]
- Badia, J.D.; Vilaplana, F.; Karlsson, S.; Ribes-Greus, A. Thermal analysis as a quality tool for assessing the influence of thermo-mechanical degradation on recycled poly(ethylene terephthalate). Polym. Test. 2009, 28, 169–175. [Google Scholar] [CrossRef] [Green Version]
- Gil-Castell, O.; Mascia, N.; Primaz, C.; Vásqez-Garay, F.; Baschetti, M.G.G.; Ribes-Greus, A. Brewer’s spent grains as biofuels in combustion-based energy recovery processes: Evaluation of thermo-oxidative decomposition. Fuel 2022, 312, 122955. [Google Scholar] [CrossRef]
- Kissinger, H.E. Reaction kinetics in differential thermal analysis. Anal. Chem. 1957, 29, 1702–1706. [Google Scholar] [CrossRef]
- Flynn, J.H.; Wall, L.A. A quick, direct method for the determination of activation energy from thermogravimetric data. J. Polym. Sci. Part B Polym. Lett. 1966, 4, 323–328. [Google Scholar] [CrossRef]
- Ozawa, T. A New Method of Analyzing Thermogravimetric Data. Bull. Chem. Soc. Jpn. 1965, 38, 1881–1886. [Google Scholar] [CrossRef] [Green Version]
- Akahira, T.; Sunose, T. Joint convention of four electrical institutes. Rep. Res. Chiba Inst. Technol. 1971, 16, 22–31. [Google Scholar]
- Monrroy, M.; García, J.R.; Mendonça, R.T.; Baeza, J.; Freer, J. Kraft pulping of eucalyptus globulus as a pretreatment for bioethanol production by simultaneous saccharification and fermentation. J. Chil. Chem. Soc. 2012, 57, 1113–1117. [Google Scholar] [CrossRef] [Green Version]
- Seta, F.T.; An, X.; Liu, L.; Zhang, H.; Yang, J.; Zhang, W.; Nie, S.; Yao, S.; Cao, H.; Xu, Q.; et al. Preparation and characterization of high yield cellulose nanocrystals (CNC) derived from ball mill pretreatment and maleic acid hydrolysis. Carbohydr. Polym. 2020, 234, 115942. [Google Scholar] [CrossRef]
- Pirich, C.L.; Picheth, G.F.; Machado, J.P.E.; Sakakibara, C.N.; Martin, A.A.; de Freitas, R.A.; Sierakowski, M.R. Influence of mechanical pretreatment to isolate cellulose nanocrystals by sulfuric acid hydrolysis. Int. J. Biol. Macromol. 2019, 130, 622–626. [Google Scholar] [CrossRef]
- ISO/TS 20477:2017. Nanotechnologies—Standard Terms and Their Definition for Cellulose; International Organization for Standardization: Geneva, Switzerland, 2017; Volume 2017. [Google Scholar]
- Marchessault, R.H.; Morehead, F.F.; Walter, N.M.; Glaudemans, C.P.J.; Timell, T.E. Morphology of xylan single crystals. J. Polym. Sci. 1961, 51, S66–S68. [Google Scholar] [CrossRef]
- Spiliopoulos, P.; Spirk, S.; Pääkkönen, T.; Viljanen, M.; Svedströ, K.; Pitkä, L.; Awais, M.; Kontturi, E. Visualizing Degradation of Cellulose Nanofibers by Acid Hydrolysis. Biomacromolecules 2021, 22, 1399–1405. [Google Scholar] [CrossRef] [PubMed]
- Rosa, M.F.; Medeiros, E.S.; Malmonge, J.A.; Gregorski, K.S.; Wood, D.F.; Mattoso, L.H.C.; Glenn, G.; Orts, W.J.; Imam, S.H. Cellulose nanowhiskers from coconut husk fibers: Effect of preparation conditions on their thermal and morphological behavior. Carbohydr. Polym. 2010, 81, 83–92. [Google Scholar] [CrossRef]
- Agwuncha, S.C.; Anusionwu, C.G.; Owonubi, S.J.; Sadiku, E.R.; Busuguma, U.A.; Ibrahim, I.D. Extraction of Cellulose Nanofibers and Their Eco/Friendly Polymer Composites. In Sustainable Polymer Composites and Nanocomposites; Springer: Berlin/Heidelberg, Germany, 2019; pp. 37–64. ISBN 9783030053994. [Google Scholar]
- Aguayo, M.; Fernández Pérez, A.; Reyes, G.; Oviedo, C.; Gacitúa, W.; Gonzalez, R.; Uyarte, O. Isolation and Characterization of Cellulose Nanocrystals from Rejected Fibers Originated in the Kraft Pulping Process. Polymers 2018, 10, 1145. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Duarte Urueña, G.; Ribeiro, K.C.; Prestes, E.; Pinheiro, L.A.; Carvalho, B.M. Extraction of Cellulose Nanocrystal from Multilayer Packaging Residues Composed of a Mixture of Eucalyptus and Pine Fibers. Waste Biomass Valorization 2021, 12, 5763–5777. [Google Scholar] [CrossRef]
- Fortunati, E.; Peltzer, M.; Armentano, I.; Torre, L.; Jiménez, A.; Kenny, J.M. Effects of modified cellulose nanocrystals on the barrier and migration properties of PLA nano-biocomposites. Carbohydr. Polym. 2012, 90, 948–956. [Google Scholar] [CrossRef]
- Wulandari, W.T.; Rochliadi, A.; Arcana, I.M. Nanocellulose prepared by acid hydrolysis of isolated cellulose from sugarcane bagasse. In Proceedings of the 10th Joint Conference on Chemistry, Surakart, Indonesia, 8–9 September 2016; Volume 107, p. 012045. [Google Scholar]
- Listyanda, R.F.; Wildan, M.W.; Ilman, M.N. Preparation and characterization of cellulose nanocrystal extracted from ramie fibers by sulfuric acid hydrolysis. Heliyon 2020, 6, e05486. [Google Scholar] [CrossRef] [PubMed]
- Esteves, B.; Marques, A.V.; Domingos, I.; Pereira, H. Chemical changes of heat treated pine and eucalypt wood monitored by FTIR. Maderas. Cienc. Tecnol. 2013, 15, 245–258. [Google Scholar] [CrossRef] [Green Version]
- Cheng, X.Y.; Li, X.J.; Xu, K.; Huang, Q.T.; Sun, H.N.; Wu, Y.Q. Effect of Thermal Treatment on Functional Groups and Degree of Cellulose Crystallinity of Eucalyptus Wood (Eucalyptus grandis × Eucalyptus urophylla). For. Prod. J. 2017, 67, 135–140. [Google Scholar] [CrossRef]
- Kargarzadeh, H.; Ahmad, I.; Abdullah, I.; Dufresne, A.; Zainudin, S.Y.; Sheltami, R.M. Effects of hydrolysis conditions on the morphology, crystallinity, and thermal stability of cellulose nanocrystals extracted from kenaf bast fibers. Cellulose 2012, 19, 855–866. [Google Scholar] [CrossRef]
- Hamad, W.Y.; Hu, T.Q. Structure–Process–Yield interrelations in nanocrystalline cellulose extraction. Can. J. Chem. Eng. 2010, 88, 392–402. [Google Scholar] [CrossRef]
- Besbes, I.; Alila, S.; Boufi, S. Nanofibrillated cellulose from TEMPO-oxidized eucalyptus fibres: Effect of the carboxyl content. Carbohydr. Polym. 2011, 84, 975–983. [Google Scholar] [CrossRef]
- Zhang, R.; Liu, Y. High energy oxidation and organosolv solubilization for high yield isolation of cellulose nanocrystals (CNC) from Eucalyptus hardwood. Sci. Rep. 2018, 8, 16505. [Google Scholar] [CrossRef] [PubMed]
- Carrillo, F.; Colom, X.; Suñol, J.; Saurina, J.; Adapa, P.K.; Karunakaran, C.; Tabil, L.G.; Schoenau, G.J. Qualitative and quantitative analysis of lignocellulosic biomass using infrared spectroscopy. Eur. Polym. J. 2004, 40, 1–20. [Google Scholar]
- Beltramino, F.; Roncero, M.B.; Torres, A.L.; Vidal, T.; Valls, C. Optimization of sulfuric acid hydrolysis conditions for preparation of nanocrystalline cellulose from enzymatically pretreated fibers. Cellulose 2016, 23, 1777–1789. [Google Scholar] [CrossRef] [Green Version]
- Jiang, Y.; Zhou, J.; Zhang, Q.; Zhao, G.; Heng, L.; Chen, D.; Liu, D. Preparation of cellulose nanocrystals from Humulus japonicus stem and the influence of high temperature pretreatment. Carbohydr. Polym. 2017, 164, 284–293. [Google Scholar] [CrossRef] [PubMed]
- Rashid, E.S.A.; Gul, A.; Yehya, W.A.H.; Julkapli, N.M. Physico-chemical characteristics of nanocellulose at the variation of catalytic hydrolysis process. Heliyon 2021, 7, e07267. [Google Scholar] [CrossRef] [PubMed]
- Wang, N.; Ding, E.; Cheng, R. Thermal degradation behaviors of spherical cellulose nanocrystals with sulfate groups. Polymer 2007, 48, 3486–3493. [Google Scholar] [CrossRef]
- De Souza, L.M.; Assis, R.Q.; Pinilla, C.M.B.; Pinilla, M.B.; Stange, R.; Vieira, H.C.; Vieira, C.; Brandelli, A.; Costa, M.H.; de Oliveira Rios, A.; et al. Eucalyptus spp. cellulose nanocrystals obtained by acid hydrolysis and ultrasound processing for structural strengthening in paper packaging. Wood Sci. Technol. 2021, 55, 639–657. [Google Scholar] [CrossRef]
- Wang, Y.; Zheng, Q.; Li, W.; Ma, Y.; Zhao, X.; Zhang, C. Measurement of free water in foods by secondary derivative thermogravimetry. CyTA-J. Food 2018, 16, 438–443. [Google Scholar] [CrossRef] [Green Version]
- Silvério, H.A.; Neto, W.P.F.; Dantas, N.O.; Pasquini, D. Extraction and characterization of cellulose nanocrystals from corncob for application as reinforcing agent in nanocomposites. Ind. Crops Prod. 2013, 44, 427–436. [Google Scholar] [CrossRef]
- Lu, H.; Gui, Y.; Zheng, L.; Liu, X. Morphological, crystalline, thermal and physicochemical properties of cellulose nanocrystals obtained from sweet potato residue. Food Res. Int. 2013, 50, 121–128. [Google Scholar] [CrossRef]
- Kim, U.J.; Eom, S.H.; Wada, M. Thermal decomposition of native cellulose: Influence on crystallite size. Polym. Degrad. Stab. 2010, 95, 778–781. [Google Scholar] [CrossRef]
- Julien, S.; Chornet, E.; Overend, R.P. Influence of acid pretreatment (H2SO4, HCl, HNO3) on reaction selectivity in the vacuum pyrolysis of cellulose. J. Anal. Appl. Pyrolysis 1993, 27, 25–43. [Google Scholar] [CrossRef]
- Nevell, T.P.; Zeronian, S.H. Cellulose Chemistry Fundamentals, 1st ed.; Nevell, T.P., Zeronian, S.H., Eds.; Ellis Horwood Limited: Chichester, UK; Wiley: Hoboken, NJ, USA, 1985; ISBN 0-85312-463-9. [Google Scholar]
- Haafiz, M.K.M.; Hassan, A.; Zakaria, Z.; Inuwa, I.M. Isolation and characterization of cellulose nanowhiskers from oil palm biomass microcrystalline cellulose. Carbohydr. Polym. 2014, 103, 119–125. [Google Scholar] [CrossRef]
- Badia, J.D.; Santonja-Blasco, L.; Martí-nez-Felipe, A.; Ribes-Greus, A.; Martínez-Felipe, A.; Ribes-Greus, A. Reprocessed polylactide: Studies of thermo-oxidative decomposition. Bioresour. Technol. 2012, 114, 622–628. [Google Scholar] [CrossRef]
- Badia, J.D.; Martinez-Felipe, A.; Santonja-Blasco, L.; Ribes-Greus, A. Thermal and thermo-oxidative stability of reprocessed poly(ethylene terephthalate). J. Anal. Appl. Pyrolysis 2013, 99, 191–202. [Google Scholar] [CrossRef] [Green Version]
- Scheirs, J.; Camino, G.; Tumiatti, W. Overview of water evolution during the thermal degradation of cellulose. Eur. Polym. J. 2001, 37, 933–942. [Google Scholar] [CrossRef]
- Mothé, C.G.; de Miranda, I.C. Study of kinetic parameters of thermal decomposition of bagasse and sugarcane straw using Friedman and Ozawa–Flynn–Wall isoconversional methods. J. Therm. Anal. Calorim. 2013, 113, 497–505. [Google Scholar] [CrossRef]
- Neves, R.M.; Ornaghi, H.L.; Ornaghi, F.G.; Amico, S.C.; Zattera, A.J. Degradation kinetics and lifetime prediction for polystyrene/nanocellulose nanocomposites. J. Therm. Anal. Calorim. 2020, 147, 879–890. [Google Scholar] [CrossRef]
- Dhar, P.; Katiyar, V. Thermal degradation kinetics of polylactic acid/acid fabricated cellulose nanocrystal based bionanocomposites. Int. J. Biol. Macromol. 2017, 104, 827–836. [Google Scholar]
- de Carvalho Benini, K.C.C.; Ornaghi, H.L.; de Medeiros, N.M.; Pereira, P.H.F.; Cioffi, M.O.H. Thermal characterization and lifetime prediction of the PHBV/nanocellulose biocomposites using different kinetic approaches. Cellulose 2020, 27, 7503–7522. [Google Scholar] [CrossRef]
- Wang, Q.; Ji, C.; Sun, J.; Yao, Q.; Liu, J.; Saeed, R.M.Y.; Zhu, Q. Kinetic thermal behavior of nanocellulose filled polylactic acid filament for fused filament fabrication 3D printing. J. Appl. Polym. Sci. 2020, 137, 48374. [Google Scholar] [CrossRef]
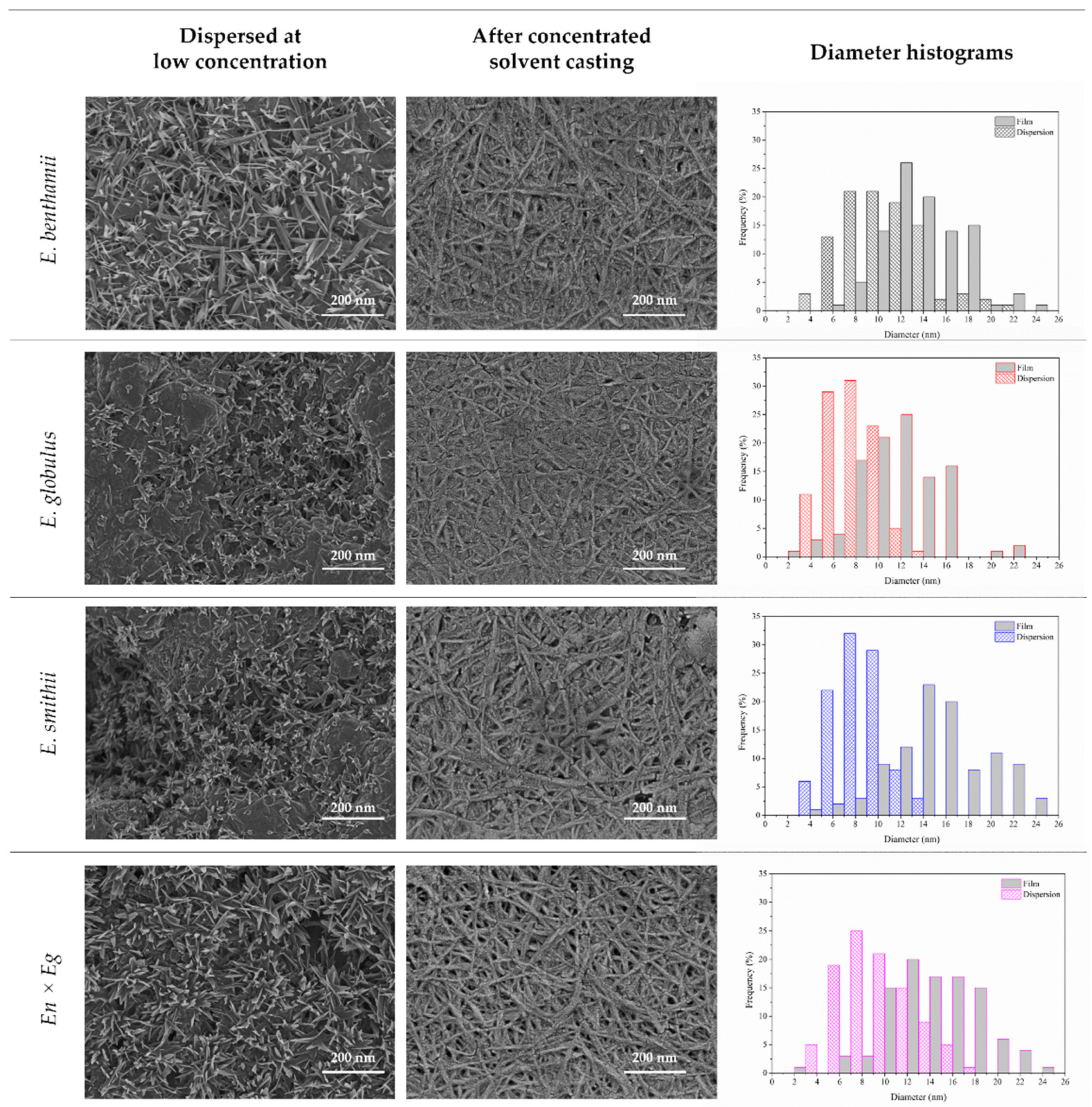
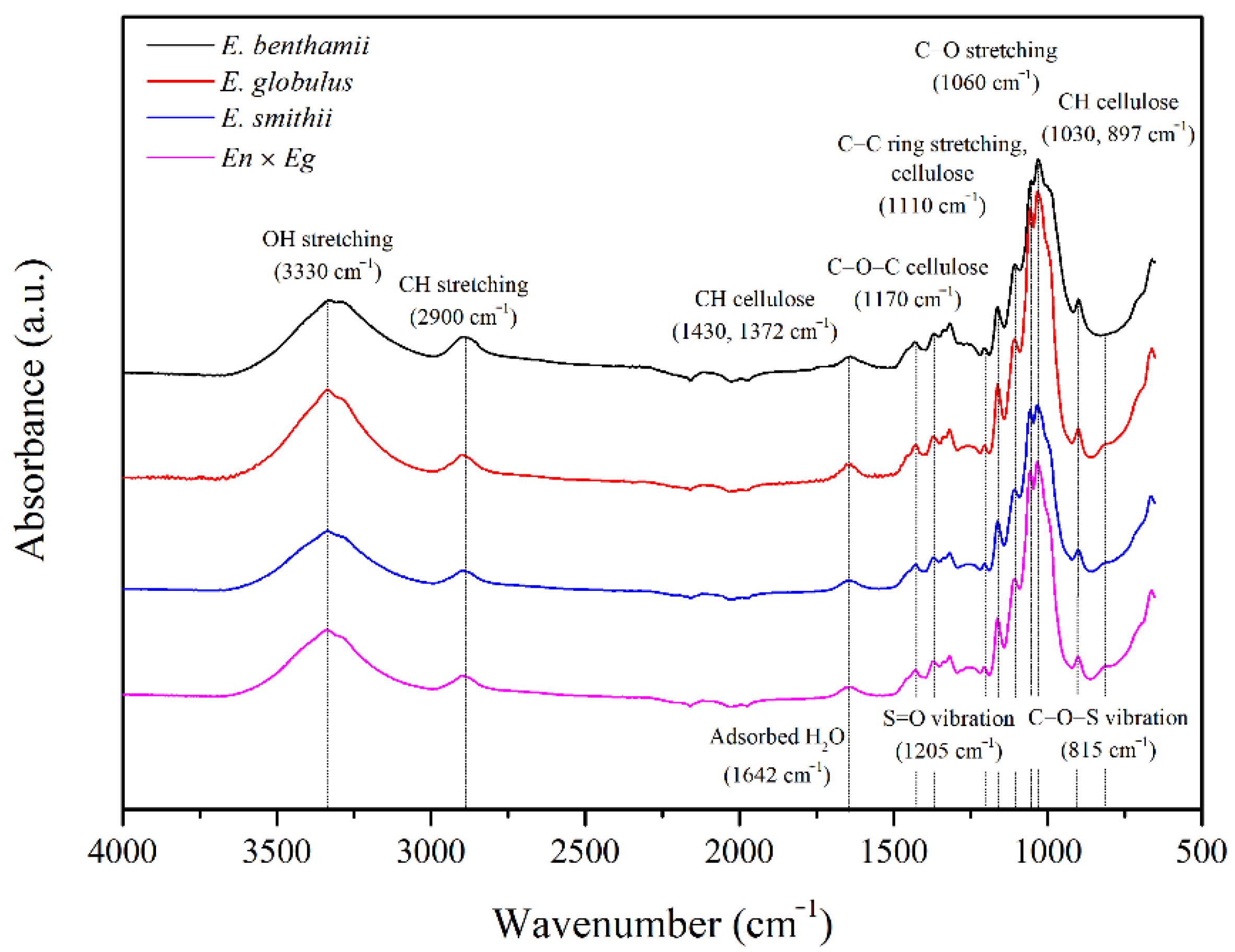
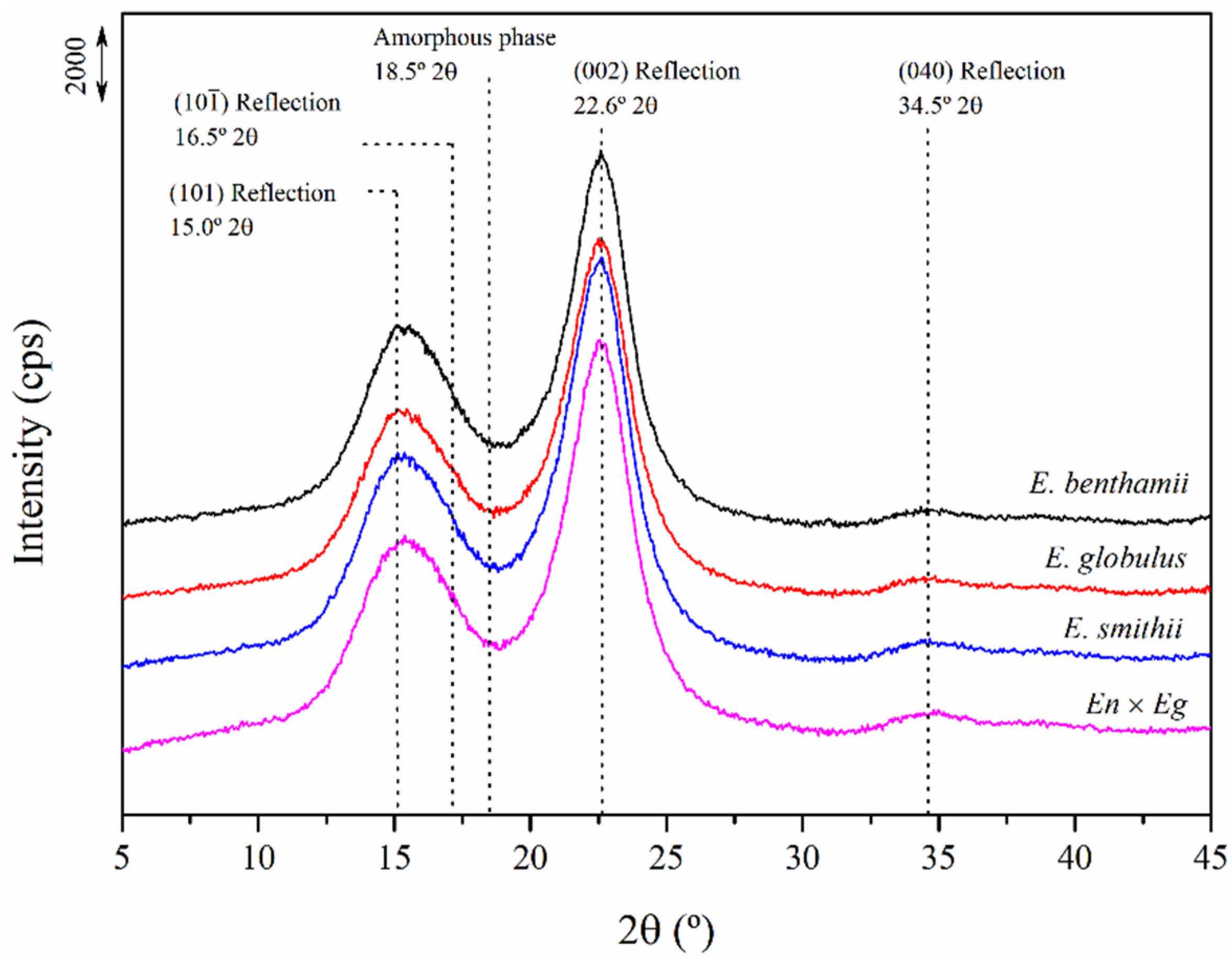

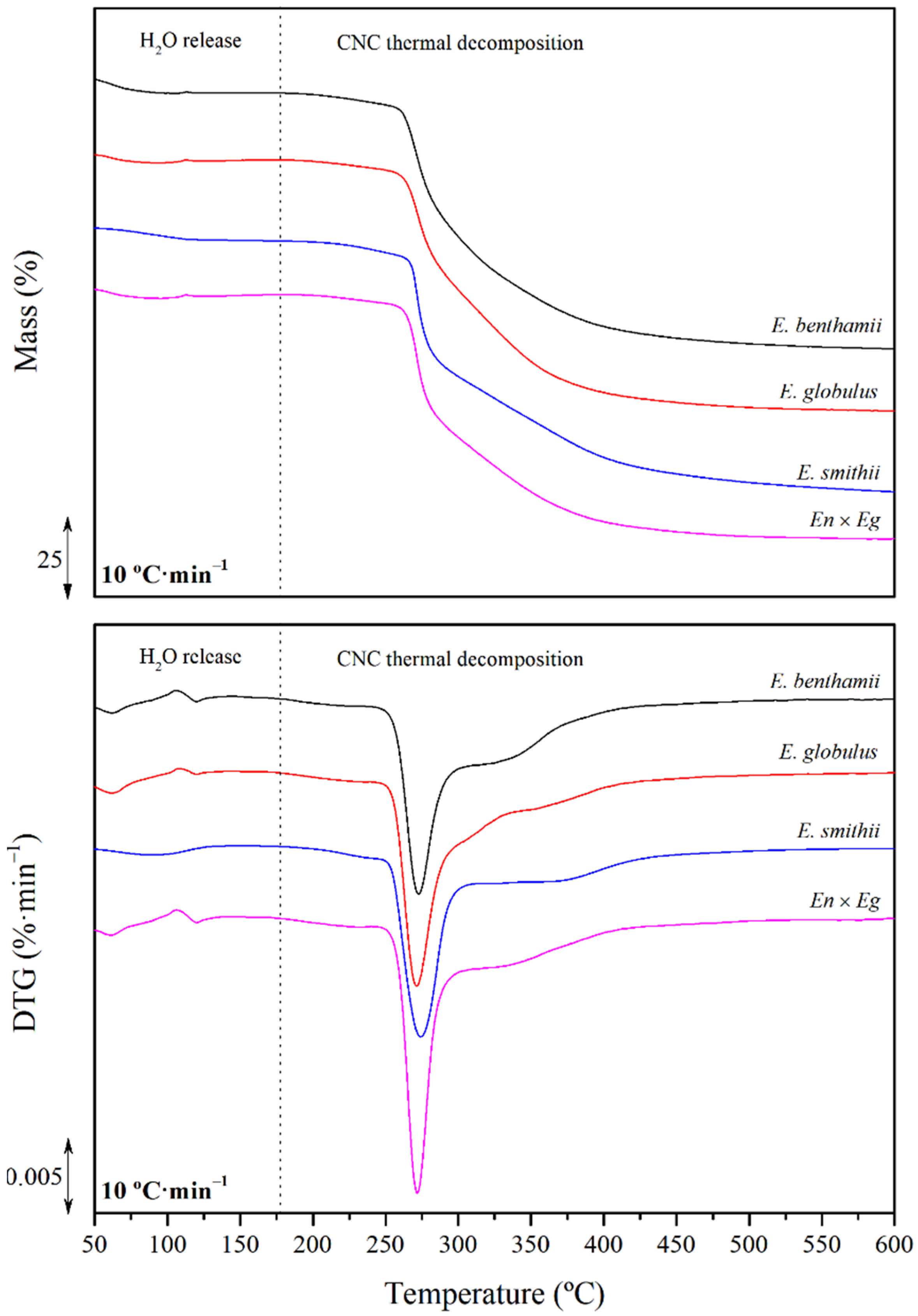

| Unbleached Kraft Pulps | Bleached Kraft Pulps | CNC | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Yield | Glucans | Xylans | Lignin | Yield | Glucans | Xylans | Lignin | Yield | |
| (%) | (% wt) | (% wt) | (% wt) | (%) | (% wt) | (% wt) | (% wt) | (% wt) | |
| E. benthamii | 49 ± 2 | 73 ± 1 | 15 ± 1 | 4.3 ± 0.9 | 45 ± 2 | 73 ± 1 | 17 ± 1 | 1.3 ± 0.6 | 21.7 ± 1.0 |
| E. globulus | 57 ± 1 | 71 ± 1 | 18 ± 1 | 3.8 ± 0.6 | 53 ± 1 | 65 ± 1 | 19 ± 1 | 1.3 ± 0.4 | 14.6 ± 0.4 |
| E. smithii | 56 ± 2 | 73 ± 2 | 18 ± 1 | 3.7 ± 1.1 | 53 ± 2 | 74 ± 1 | 22 ± 1 | <1.0 | 22.4 ± 1.5 |
| En × Eg | 57 ± 2 | 75 ± 2 | 19 ± 1 | 4.6 ± 1.3 | 52 ± 2 | 71 ± 2 | 22 ± 1 | 1.7 ± 0.7 | 24.9 ± 1.2 |
| Sulphate Index (%) | |
|---|---|
| E. benthamii | 0.21 |
| E. globulus | 0.97 |
| E. smithii | 0.73 |
| En × Eg | 0.85 |
| Crystallinity Evaluation | ||||
|---|---|---|---|---|
| CrI (%) | L002 (nm) | ICI (A1430/A897) | ICR (A1372/A2900) | |
| E. benthamii | 65.8 ± 1.3 | 10.9 ± 1.3 | 1.041 | 0.997 |
| E. globulus | 65.0 ± 1.7 | 10.1 ± 1.1 | 1.014 | 0.983 |
| E. smithii | 64.4 ± 1.6 | 10.1 ± 0.8 | 1.014 | 0.988 |
| En × Eg | 65.2 ± 1.9 | 10.0 ± 1.4 | 1.011 | 0.987 |
| H2O Release | CNC Thermal Decomposition | |||
|---|---|---|---|---|
| Tfree | ∆hH2O | Tcel | ∆hcel | |
| (°C) | (J·g−1) | (°C) | (J·g−1) | |
| E. benthamii | 69.2 ± 0.7 | 57.1 ± 4.3 | 279.7 ± 0.2 | 114.0 ± 0.8 |
| E. globulus | 82.5 ± 2.3 | 84.5 ± 4.3 | 269.7 ± 1.8 | 109.2 ± 0.8 |
| E. smithii | 69.8 ± 0.7 | 49.8 ± 2.2 | 278.6 ± 0.2 | 115.2 ± 0.6 |
| En × Eg | 78.2 ± 1.9 | 76.1 ± 6.6 | 273.6 ± 0.6 | 120.8 ± 1.0 |
| β | H2O Release | CNC Thermal Decomposition | ||||||
|---|---|---|---|---|---|---|---|---|
| (°C·min−1) | Tfree (°C) | ∆mH2O (%) | To (°C) | Tp (°C) | Te (°C) | ∆mcel (%) | R (%) | |
| 5 | E. benthamii | 69.5 ± 1.6 | 2.5 ± 0.4 | 260.0 ± 0.5 | 270.0 ± 1.4 | 295.1 ± 2.9 | 69.3 ± 0.1 | 28.3 ± 0.8 |
| E. globulus | 69.8 ± 1.6 | 4.4 ± 0.7 | 253.6 ± 2.6 | 264.5 ± 1.7 | 287.1 ± 0.8 | 74.3 ± 0.2 | 22.3 ± 0.4 | |
| E. smithii | 69.1 ± 1.7 | 3.4 ± 0.5 | 255.0 ± 0.4 | 269.4 ± 1.3 | 292.9 ± 2.4 | 69.4 ± 2.4 | 26.5 ± 0.9 | |
| En × Eg | 70.6 ± 1.7 | 4.1 ± 0.3 | 253.8 ± 0.9 | 265.5 ± 2.7 | 284.9 ± 0.9 | 71.5 ± 0.9 | 24.1 ± 1.1 | |
| 10 | E. benthamii | 61.8 ± 0.5 | 2.2 ± 1.0 | 261.2 ± 0.2 | 274.2 ± 0.6 | 301.2 ± 2.6 | 75.3 ± 0.1 | 22.5 ± 0.9 |
| E. globulus | 87.9 ± 0.2 | 4.8 ± 1.0 | 260.1 ± 1.9 | 271.4 ± 1.4 | 295.0 ± 2.2 | 78.4 ± 1.7 | 16.8 ± 1.2 | |
| E. smithii | 61.2 ± 1.6 | 2.5 ± 0.4 | 260.5 ± 1.5 | 273.4 ± 1.3 | 297.9 ± 3.1 | 72.5 ± 0.7 | 23.1 ± 0.7 | |
| En × Eg | 61.1 ± 1.7 | 4.4 ± 0.9 | 261.4 ± 0.4 | 271.8 ± 0.2 | 293.5 ± 1.1 | 73.4 ± 1.4 | 24.1 ± 0.4 | |
| 20 | E. benthamii | 80.9 ± 0.8 | 3.6 ± 0.6 | 265.5 ± 0.2 | 278.7 ± 0.9 | 309.6 ± 1.5 | 78.2 ± 0.5 | 18.2 ± 0.8 |
| E. globulus | 83.7 ± 1.9 | 4.8 ± 0.9 | 273.2 ± 0.7 | 277.9 ± 0.1 | 304.3 ± 2.1 | 74.8 ± 0.2 | 19.4 ± 0.6 | |
| E. smithii | 79.0 ± 1.5 | 4.1 ± 1.6 | 265.3 ± 2.5 | 279.8 ± 0.2 | 308.1 ± 2.9 | 78.7 ± 2.3 | 14.8 ± 1.2 | |
| En × Eg | 89.6 ± 0.4 | 4.5 ± 0.1 | 267.1 ± 1.8 | 277.2 ± 2.8 | 298.5 ± 5.6 | 80.0 ± 0.4 | 15.9 ± 0.9 | |
| 30 | E. benthamii | 83.7 ± 1.9 | 3.4 ± 0.2 | 267.3 ± 1.0 | 282.3 ± 1.3 | 316.9 ± 0.9 | 76.8 ± 0.1 | 18.8 ± 0.7 |
| E. globulus | 87.9 ± 0.1 | 4.9 ± 0.8 | 272.9 ± 1.7 | 282.8 ± 1.5 | 310.3 ± 3.8 | 74.2 ± 0.9 | 20.9 ± 0.4 | |
| E. smithii | 81.5 ± 3.0 | 3.3 ± 0.9 | 267.7 ± 1.0 | 283.9 ± 0.1 | 310.4 ± 0.3 | 81.4 ± 0.7 | 14.8 ± 0.9 | |
| En × Eg | 89.7 ± 1.7 | 3.8 ± 0.2 | 269.5 ± 1.9 | 281.4 ± 2.5 | 304.2 ± 3.7 | 76.9 ± 0.1 | 19.8 ± 1.1 | |
| ZDT | a | b | k | R2 | ||
|---|---|---|---|---|---|---|
| (°C) | (°C) | |||||
| E. benthamii | To | 253.0 | 271.5 | 0.04 | 0.06 | 0.998 |
| Tp | 265.5 | 286.9 | 0.04 | 0.05 | 0.991 | |
| Te | 289.0 | 338.7 | 0.09 | 0.03 | 0.998 | |
| E. globulus | To | 246.7 | 280.8 | 0.07 | 0.05 | 0.990 |
| Tp | 256.5 | 286.8 | 0.06 | 0.07 | 0.987 | |
| Te | 277.3 | 316.9 | 0.07 | 0.06 | 0.998 | |
| E. smithii | To | 246.8 | 268.8 | 0.04 | 0.10 | 0.996 |
| Tp | 270.1 | 292.4 | 0.02 | 0.07 | 0.972 | |
| Te | 283.3 | 314.8 | 0.06 | 0.07 | 0.957 | |
| En × Eg | To | 243.9 | 270.4 | 0.05 | 0.11 | 0.998 |
| Tp | 258.1 | 284.3 | 0.05 | 0.07 | 0.981 | |
| Te | 275.6 | 306.4 | 0.06 | 0.08 | 0.928 |
| Kissinger | FWO | KAS | |||||
|---|---|---|---|---|---|---|---|
| Ea | R2 | Ea | R2 | Ea | R2 | ||
| (kJ·mol−1) | (kJ·mol−1) | (kJ·mol−1) | |||||
| E. benthamii | 0.1 | 361.5 | 1.00 | 281.3 | 0.98 | 287.1 | 0.98 |
| 0.2 | 299.4 | 0.97 | 306.0 | 0.96 | |||
| 0.3 | 278.6 | 0.97 | 284.0 | 0.97 | |||
| 0.4 | 210.2 | 0.93 | 212.0 | 0.93 | |||
| 0.5 | 133.9 | 0.89 | 131.5 | 0.88 | |||
| 0.6 | 93.8 | 0.92 | 89.1 | 0.91 | |||
| 0.7 | 83.6 | 0.95 | 78.0 | 0.94 | |||
| 0.8 | 93.3 | 0.97 | 87.8 | 0.97 | |||
| E. globulus | 0.1 | 237.6 | 0.95 | 233.1 | 1.00 | 236.3 | 1.00 |
| 0.2 | 243.6 | 0.99 | 247.3 | 0.99 | |||
| 0.3 | 248.7 | 0.97 | 252.7 | 0.97 | |||
| 0.4 | 187.8 | 0.97 | 188.4 | 0.97 | |||
| 0.5 | 112.0 | 0.99 | 108.5 | 0.99 | |||
| 0.6 | 81.2 | 0.98 | 75.7 | 0.97 | |||
| 0.7 | 81.1 | 0.98 | 75.3 | 0.97 | |||
| 0.8 | 88.1 | 0.98 | 236.3 | 0.97 | |||
| E. smithii | 0.1 | 295.2 | 1.00 | 254.9 | 0.99 | 259.2 | 0.99 |
| 0.2 | 257.6 | 1.00 | 261.9 | 1.00 | |||
| 0.3 | 237.8 | 0.97 | 241.0 | 0.97 | |||
| 0.4 | 188.0 | 0.98 | 188.5 | 0.97 | |||
| 0.5 | 157.2 | 0.99 | 156.0 | 0.99 | |||
| 0.6 | 102.9 | 0.99 | 98.5 | 0.99 | |||
| 0.7 | 81.4 | 0.99 | 75.6 | 0.99 | |||
| 0.8 | 74,0 | 0.99 | 67.5 | 0.99 | |||
| En × Eg | 0.1 | 274.9 | 1.00 | 216.6 | 0.98 | 219.0 | 0.98 |
| 0.2 | 219.3 | 0.97 | 221.8 | 0.97 | |||
| 0.3 | 209.8 | 0.99 | 211.7 | 0.99 | |||
| 0.4 | 182.0 | 1.00 | 182.4 | 1.00 | |||
| 0.5 | 122.0 | 1.00 | 119.0 | 0.99 | |||
| 0.6 | 93.9 | 0.99 | 89.1 | 0.99 | |||
| 0.7 | 94.0 | 1.00 | 88.9 | 1.00 | |||
| 0.8 | 92.4 | 0.99 | 86.7 | 0.99 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gil-Castell, O.; Reyes-Contreras, P.; Barra, P.A.; Teixeira Mendonça, R.; Carrillo-Varela, I.; Badia, J.D.; Serra, A.; Ribes-Greus, A. The Role of Eucalyptus Species on the Structural and Thermal Performance of Cellulose Nanocrystals (CNCs) Isolated by Acid Hydrolysis. Polymers 2022, 14, 423. https://doi.org/10.3390/polym14030423
Gil-Castell O, Reyes-Contreras P, Barra PA, Teixeira Mendonça R, Carrillo-Varela I, Badia JD, Serra A, Ribes-Greus A. The Role of Eucalyptus Species on the Structural and Thermal Performance of Cellulose Nanocrystals (CNCs) Isolated by Acid Hydrolysis. Polymers. 2022; 14(3):423. https://doi.org/10.3390/polym14030423
Chicago/Turabian StyleGil-Castell, Oscar, Pablo Reyes-Contreras, Pabla Andrea Barra, Regis Teixeira Mendonça, Isabel Carrillo-Varela, José David Badia, Angels Serra, and Amparo Ribes-Greus. 2022. "The Role of Eucalyptus Species on the Structural and Thermal Performance of Cellulose Nanocrystals (CNCs) Isolated by Acid Hydrolysis" Polymers 14, no. 3: 423. https://doi.org/10.3390/polym14030423
APA StyleGil-Castell, O., Reyes-Contreras, P., Barra, P. A., Teixeira Mendonça, R., Carrillo-Varela, I., Badia, J. D., Serra, A., & Ribes-Greus, A. (2022). The Role of Eucalyptus Species on the Structural and Thermal Performance of Cellulose Nanocrystals (CNCs) Isolated by Acid Hydrolysis. Polymers, 14(3), 423. https://doi.org/10.3390/polym14030423










