Study on Curing Deformation of Composite Thin Shells Prepared by M-CRTM with Adjustable Injection Gap
Abstract
1. Introduction
2. Preparation of a Composite Helmet Shell
2.1. Materials and Techniques
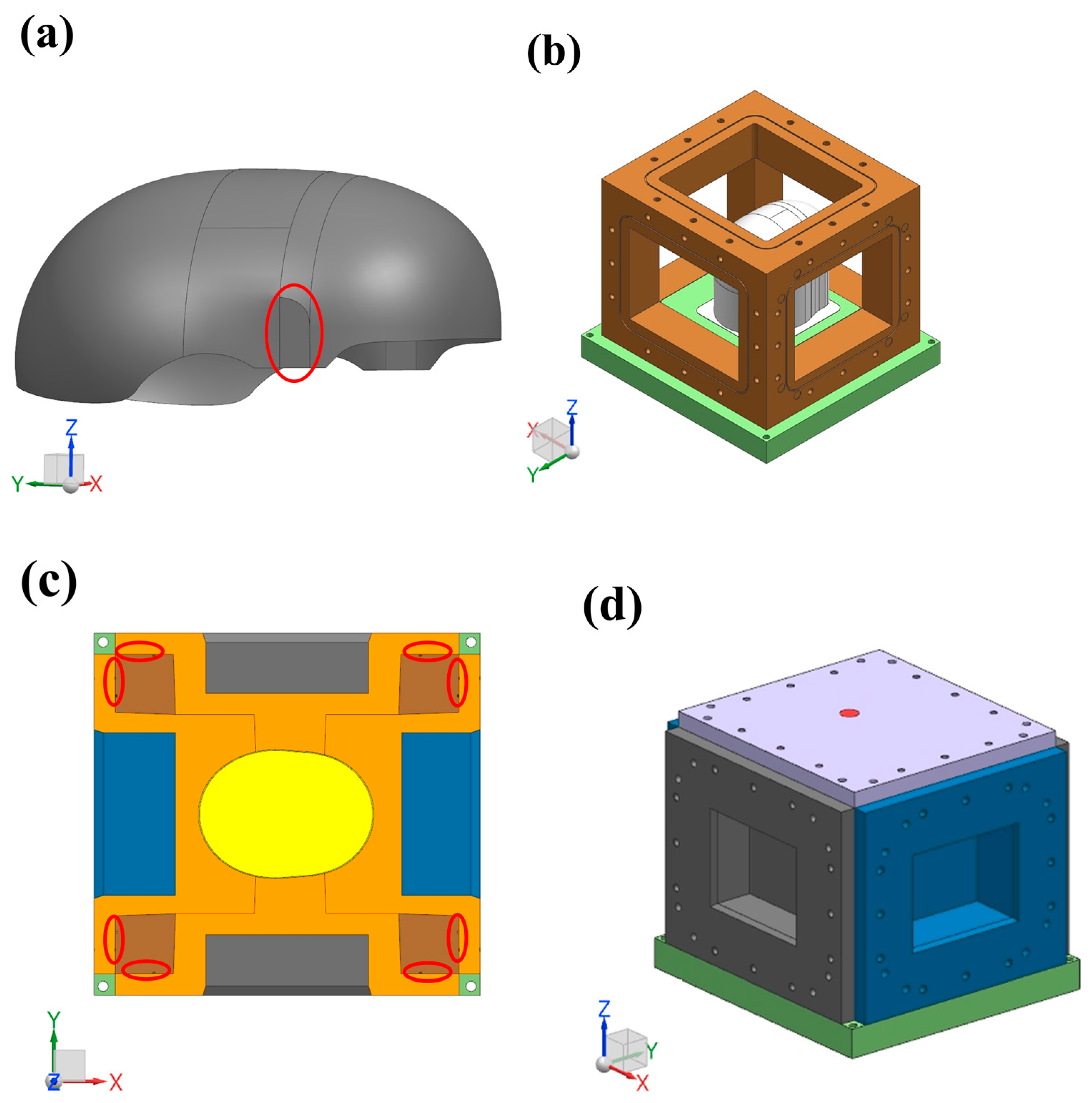
2.2. Helmet Specimen Manufacturing Process
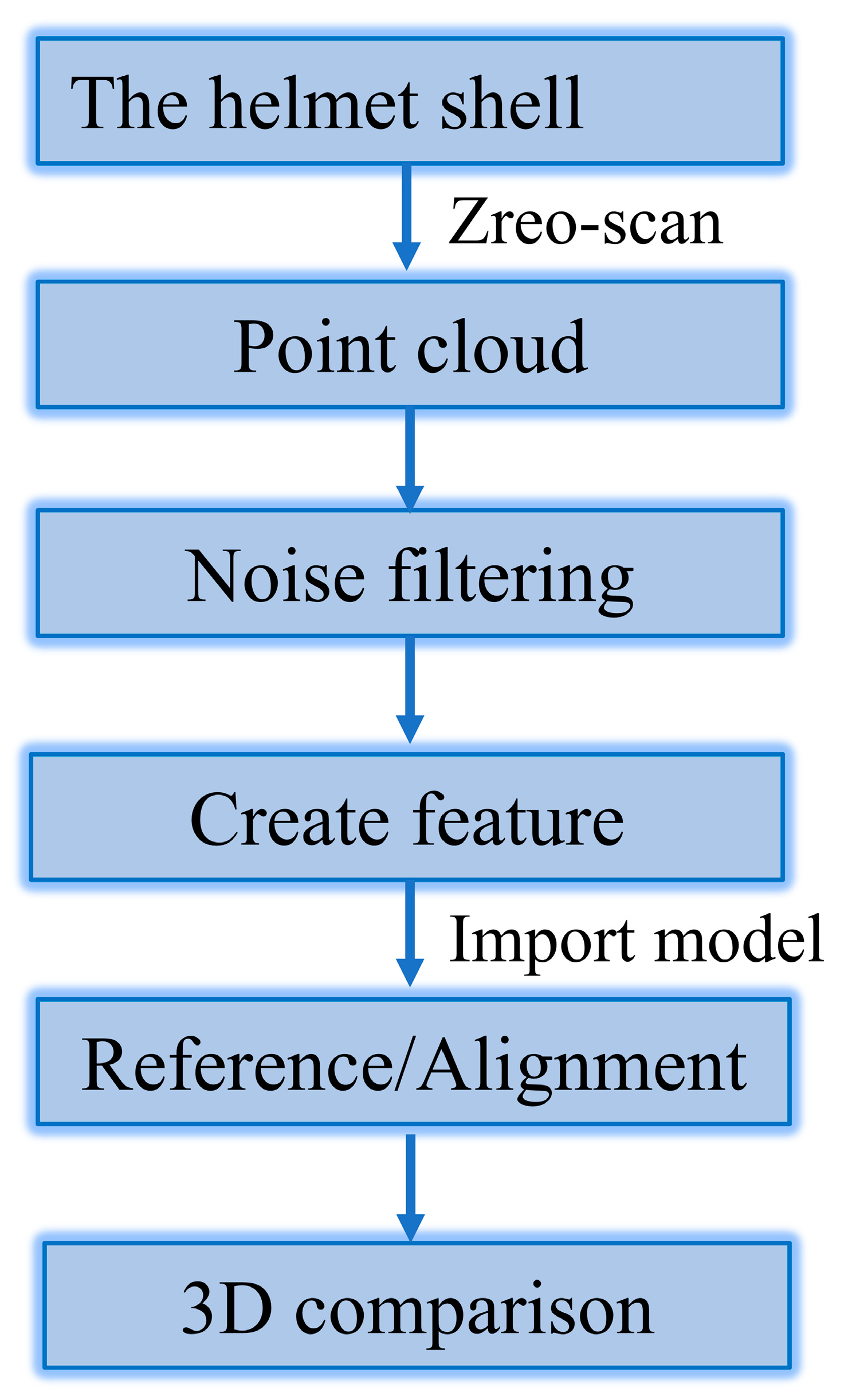
2.3. Micro-CT Detection
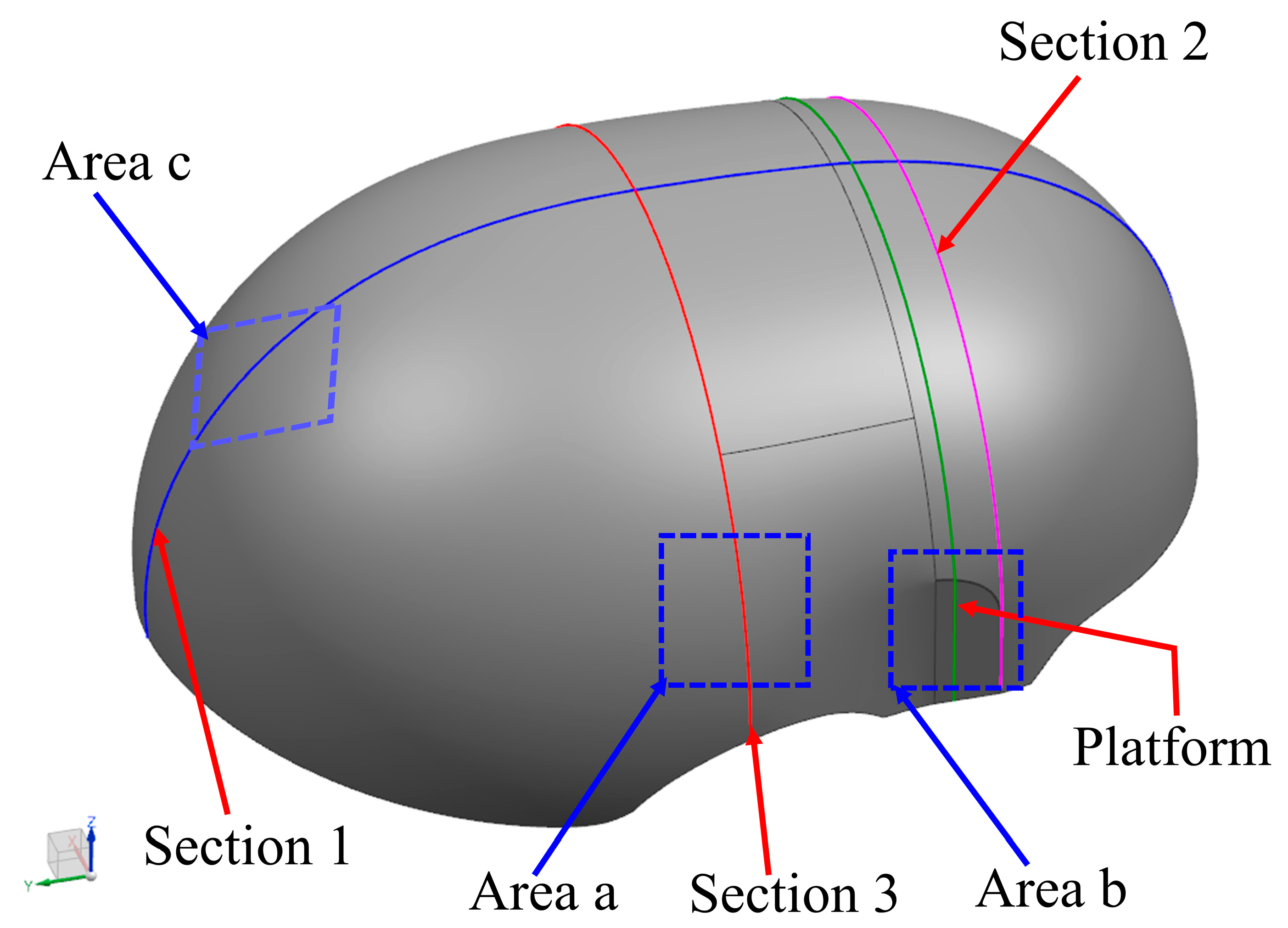
2.4. Data Extraction and Curing Deformation Evaluation
3. Results and Discussion
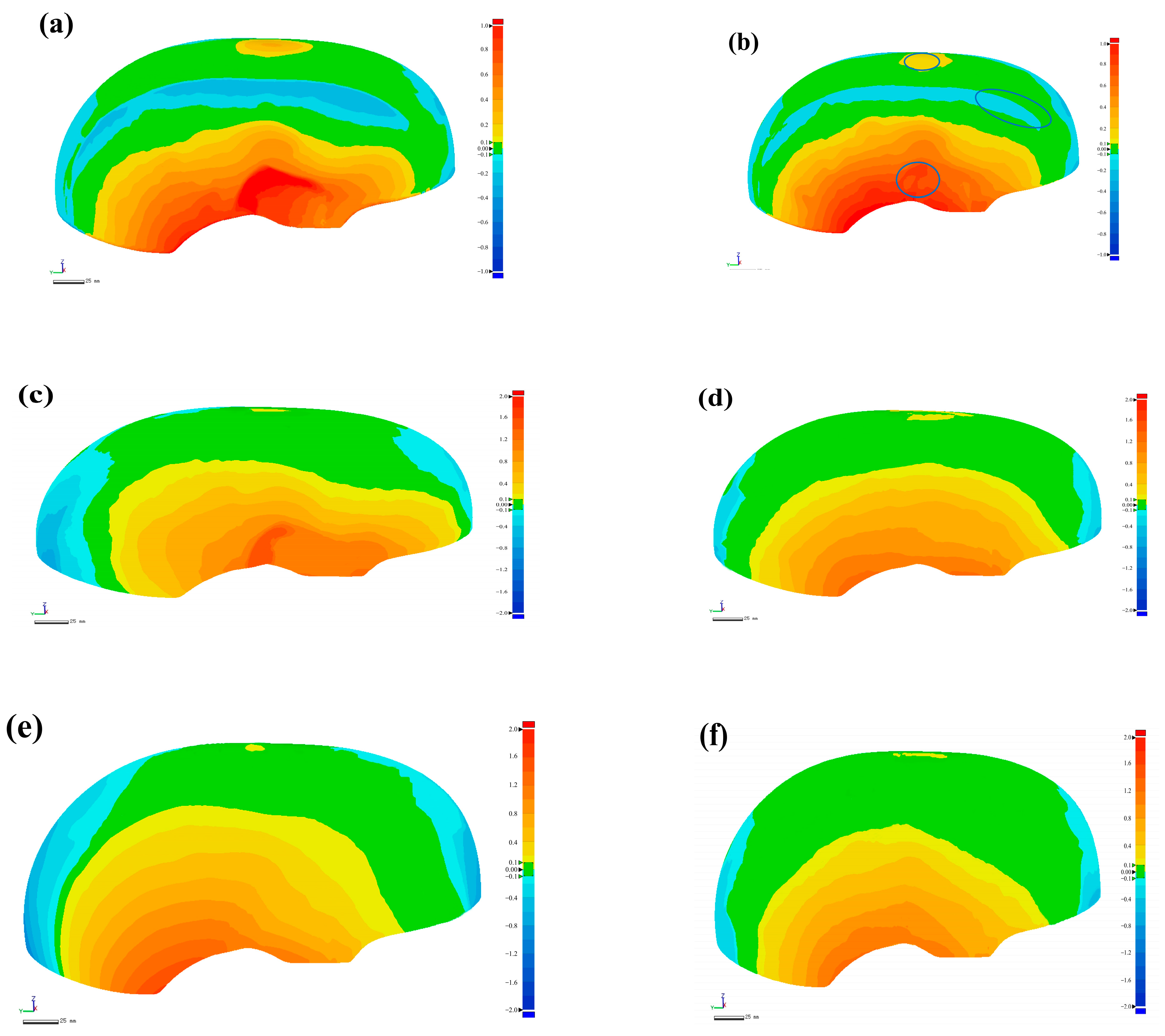
3.1. The Overall Deformation Analysis of Helmet Shell
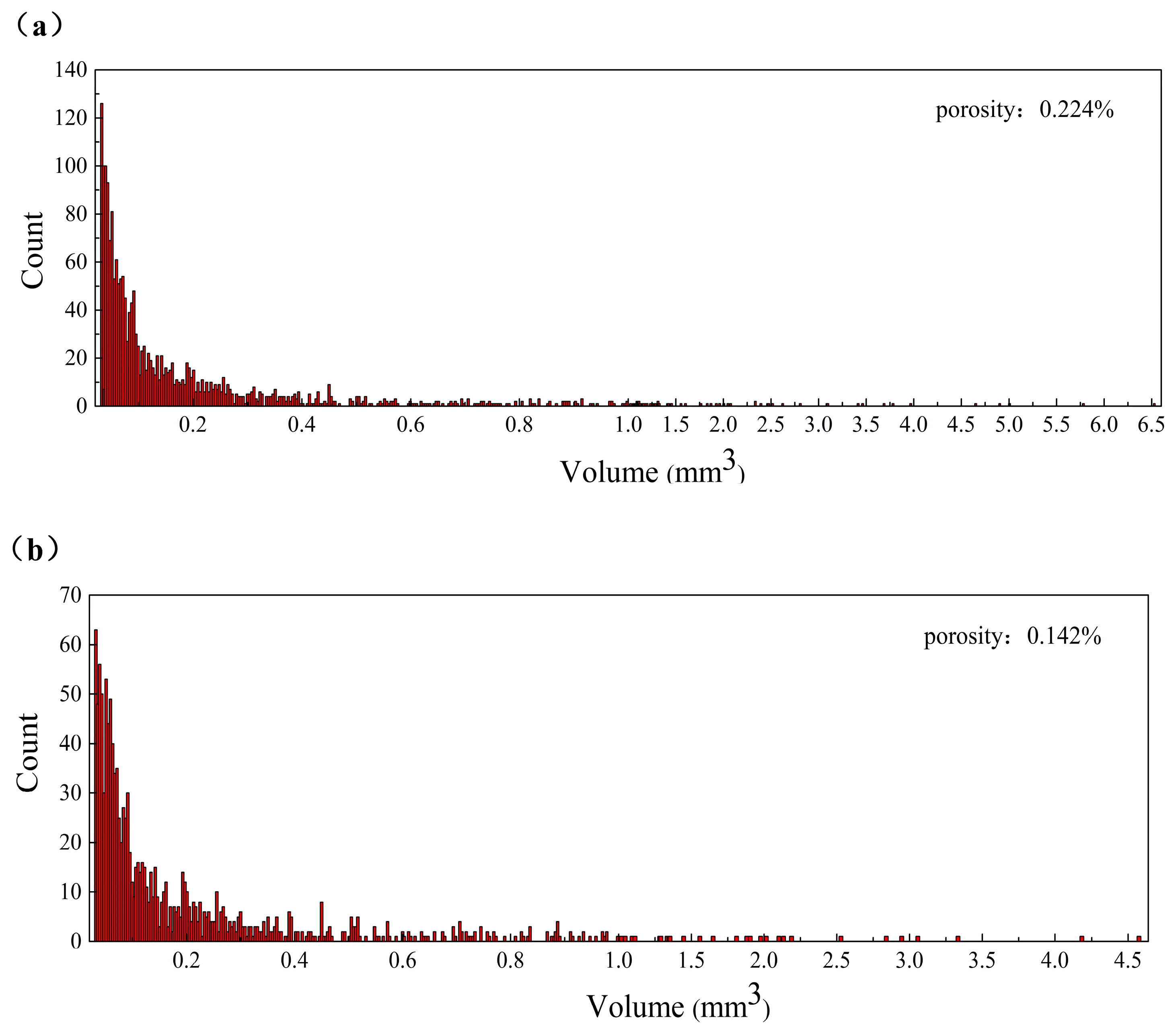
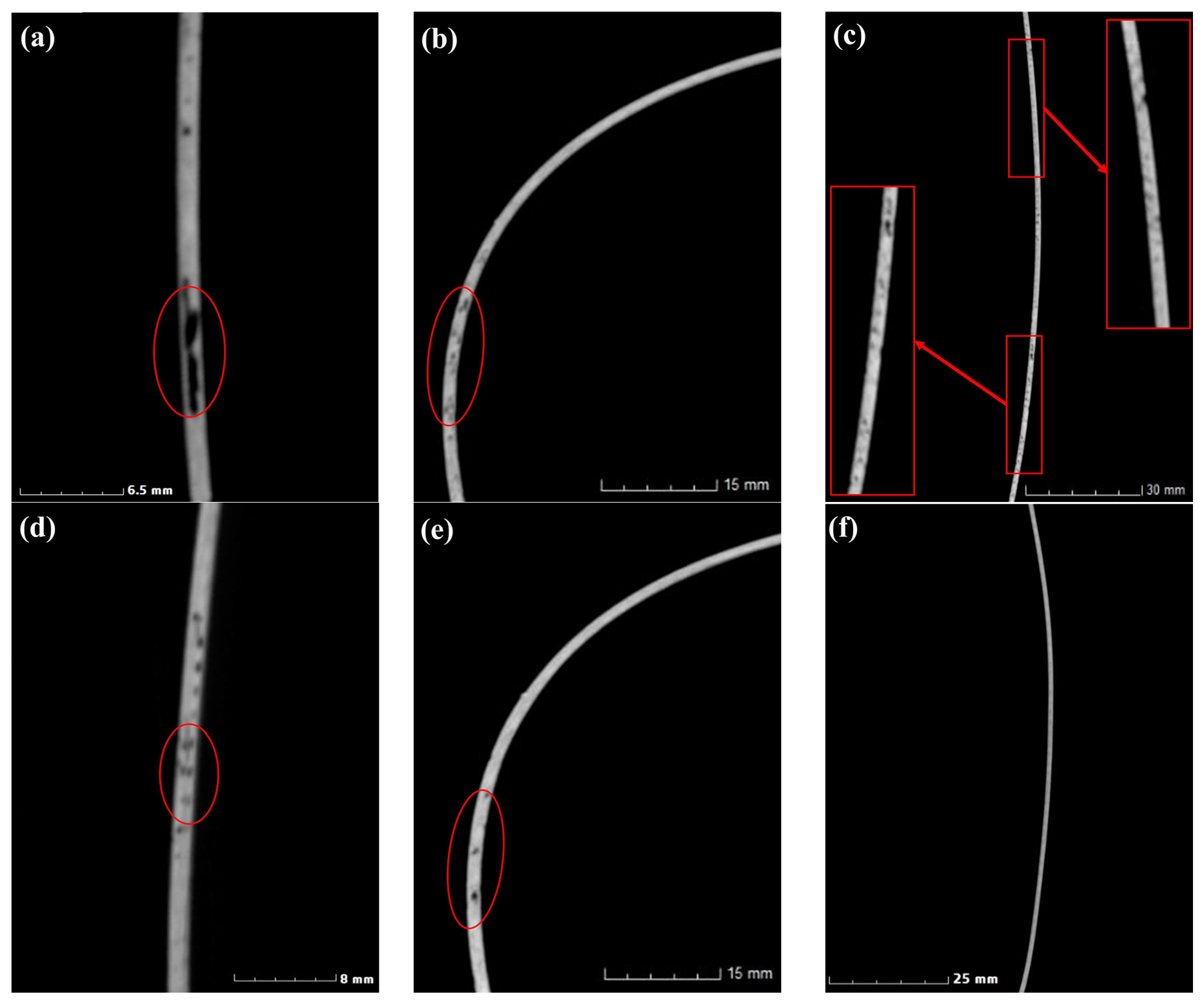
3.2. Void Analysis Using Micro-CT
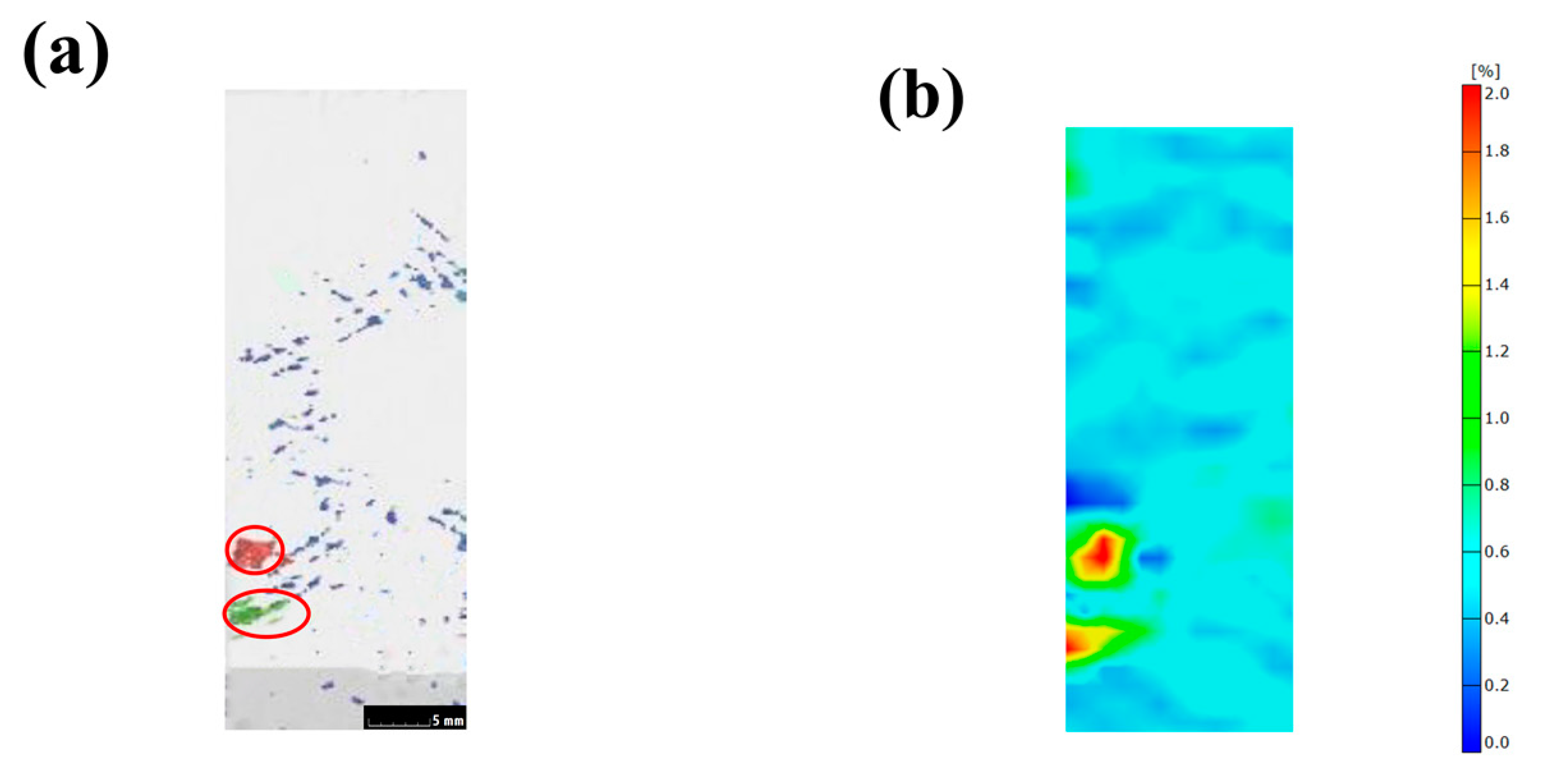
3.3. Void Analysis of Helmet Shell Section
3.4. Flexural Modulus Analysis
3.5. Local Stress Distribution of Sample with Voids
3.6. Curing Deformation Analysis of Helmet Section
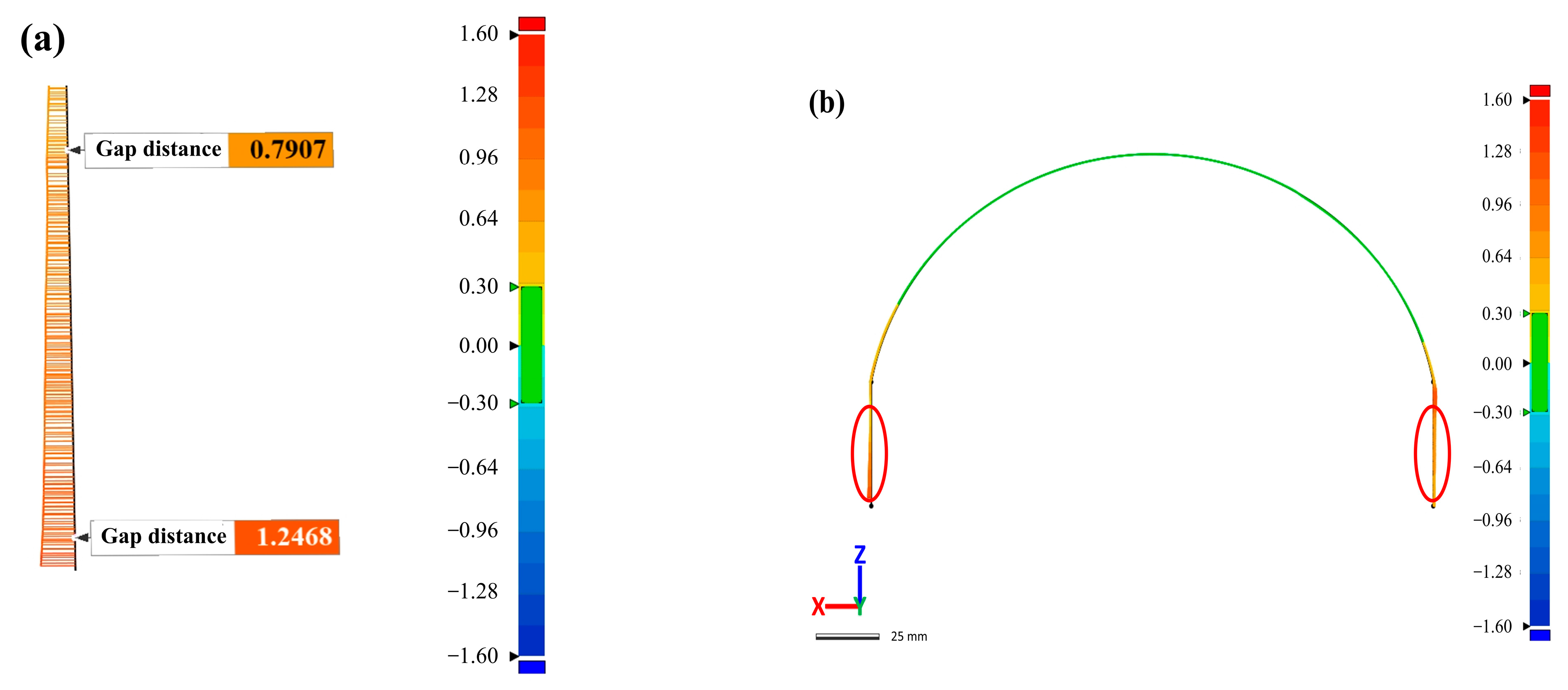
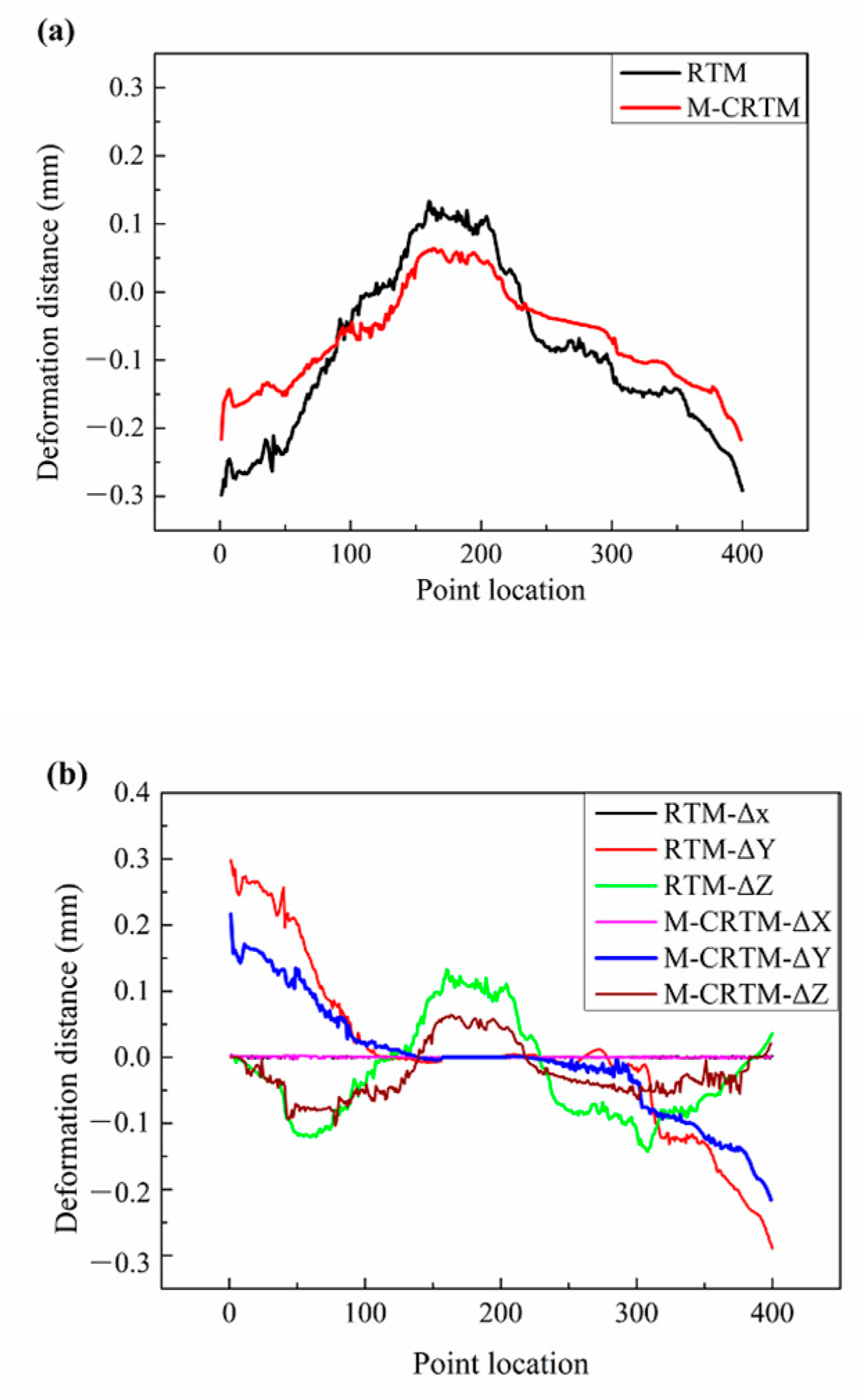
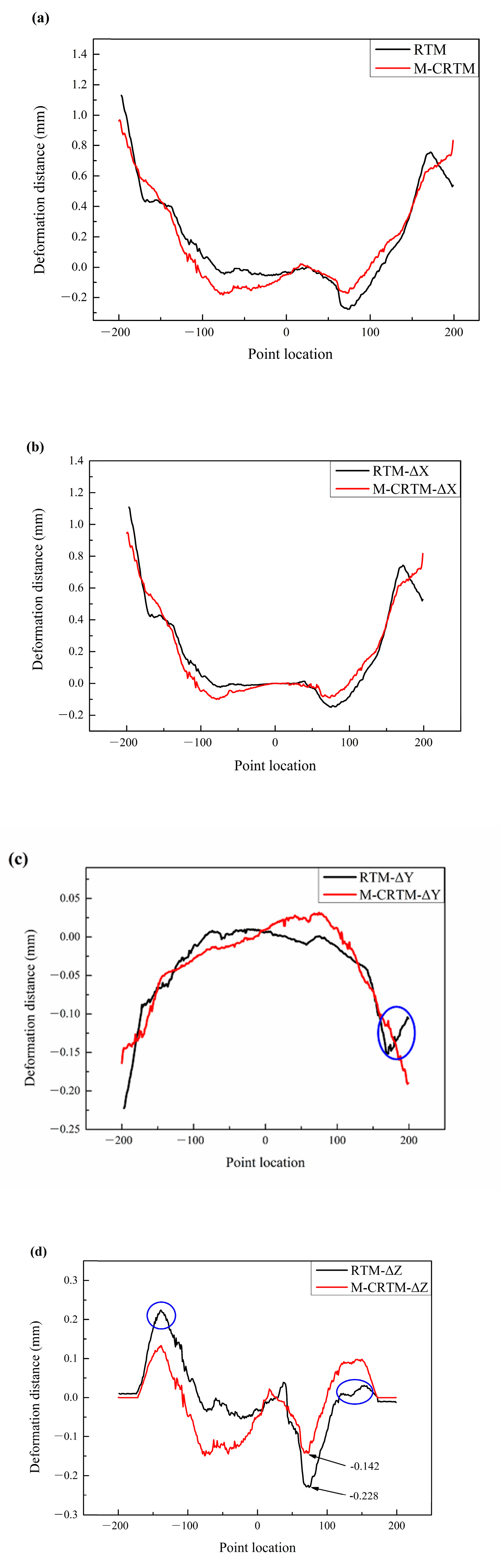
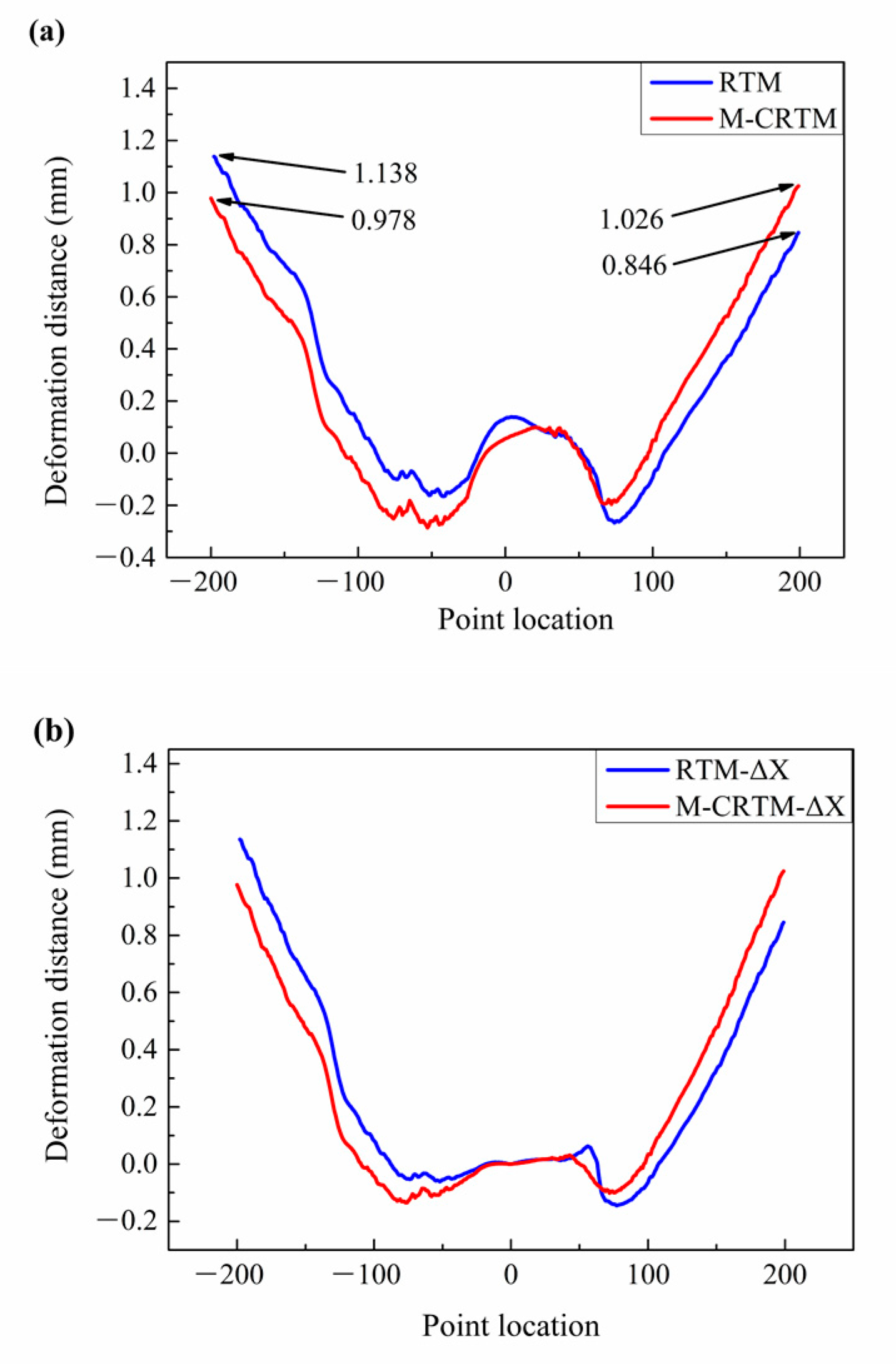
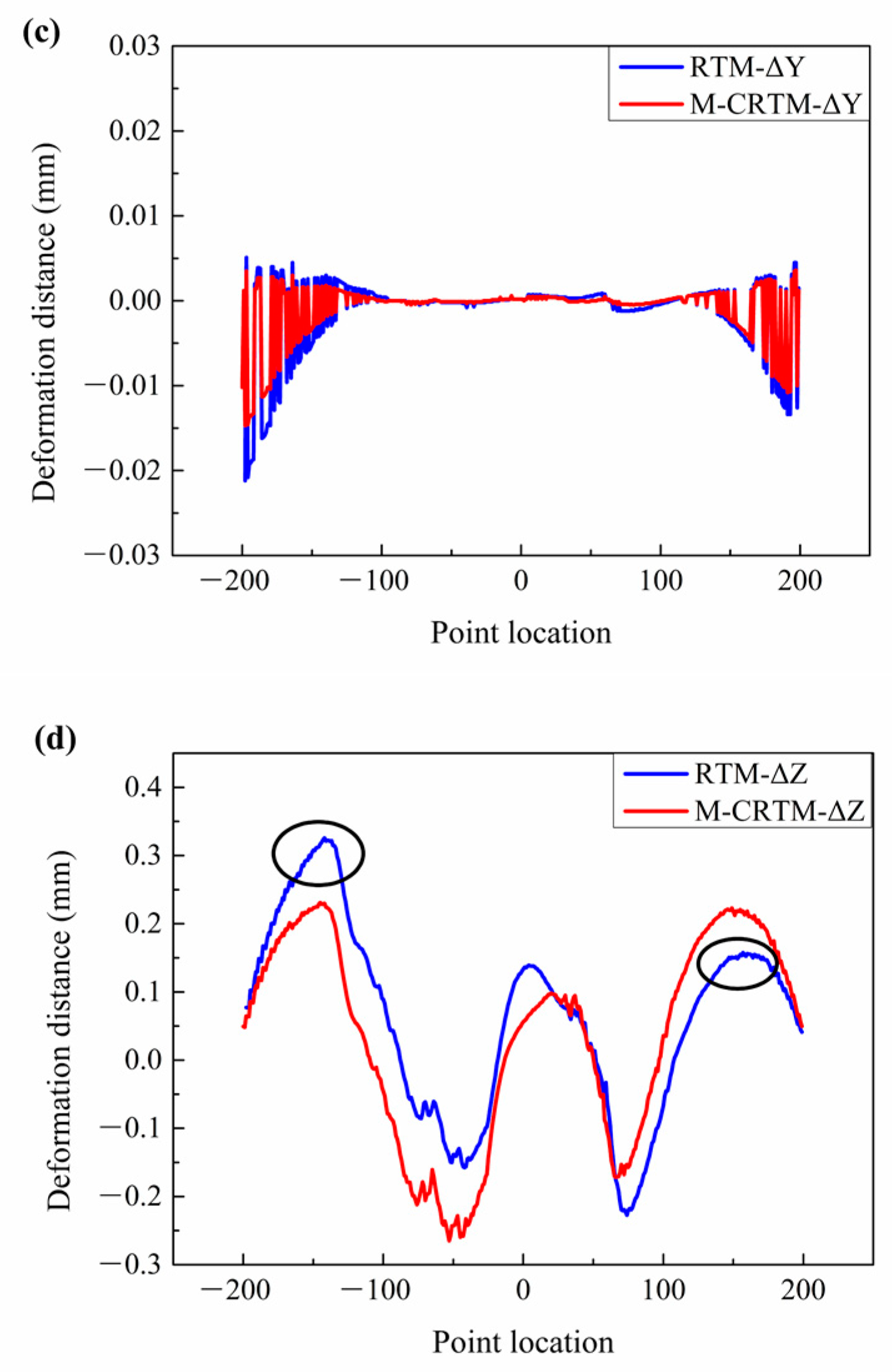
3.6.1. Deformation Analysis of Helmet Mounting Platform
3.6.2. Deformation Analysis of Helmet Shell Section
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Luo, G.M.; Liou, G.Y.; Xiao, H.Z. Using a Fiber Bragg Grating Sensor to Measure Residual Strain in the Vacuum-Assisted Resin Transfer Molding Process. Polymers 2022, 14, 1446. [Google Scholar] [CrossRef] [PubMed]
- Kondratiev, A.; Pistek, V.; Vambol, O.; Kucera, P. Effect of Heating Conditions during Moulding on Residual Stress-Strain Behaviour of a Composite Panel. Polymers 2022, 14, 1660. [Google Scholar] [CrossRef] [PubMed]
- Patel, A.; Kravchenko, O.; Manas-Zloczower, I. Effect of Curing Rate on the Microstructure and Macroscopic Properties of Epoxy Fiberglass Composites. Polymers 2018, 10, 125. [Google Scholar] [CrossRef] [PubMed]
- Zhang, C.; Zhang, G.L.; Xu, J.; Shi, X.P.; Wang, X. Review of curing deformation control methods for carbon fiber reinforced resin composites. Polym. Compos. 2022, 43, 3350–3370. [Google Scholar] [CrossRef]
- Liu, K.; Zhang, B.M.; Xu, X.Y.; Ye, J.R.; Liu, C.X. Simulation and Analysis of Process-Induced Distortions in Hemispherical Thermostamping for Unidirectional Thermoplastic Composites. Polym. Compos. 2019, 40, 1786–1800. [Google Scholar] [CrossRef]
- Fernlund, G.; Rahman, N.; Courdji, R.; Bresslauer, M.; Poursartip, A.; Willden, K.; Nelson, K. Experimental and numerical study of the effect of cure cycle, tool surface, geometry, and lay-up on the dimensional fidelity of autoclave-processed composite parts. Compos. Part A-Appl. Sci. Manuf. 2002, 33, 341–351. [Google Scholar] [CrossRef]
- Wang, X.X.; Zhao, Y.R.; Su, H.; Jia, Y.X. Curing Process-induced Internal Stress and Deformation of Fiber Reinforced Resin Matrix Composites: Numerical Comparison Between Elastic and Viscoelastic Models. Polym. Polym. Compos. 2016, 24, 155–160. [Google Scholar] [CrossRef]
- Exner, W.; Hein, R.; Mahrholz, T.; Wierach, P.; Monner, H.P.; Sinapius, M. Impact of nanoparticles on the process-induced distortions of carbon fiber reinforced plastics: An experimental and simulative approach. J. Appl. Polym. Sci. 2019, 136, 47031. [Google Scholar] [CrossRef]
- Albert, C.; Fernlund, G. Spring-in and warpage of angled composite laminates. Compos. Sci. Technol. 2002, 62, 1895–1912. [Google Scholar] [CrossRef]
- Liu, Z.D.; Zheng, X.T.; Fan, W.J.; Wang, F.; Ahmed, S.; Yan, L.L. An alternative method to reduce process-induced deformation of CFRP by introducing prestresses. Chin. J. Aeronaut. 2022, 35, 314–323. [Google Scholar] [CrossRef]
- Mesogitis, T.S.; Skordos, A.A.; Long, A.C. Uncertainty in the manufacturing of fibrous thermosetting composites: A review. Compos. Part A-Appl. Sci. Manuf. 2014, 57, 67–75. [Google Scholar] [CrossRef]
- He, C.; Ge, J.; Cao, X.; Chen, Y.; Chen, H.; Fang, D. The effects of fiber radius and fiber shape deviations and of matrix void content on the strengths and failure mechanisms of UD composites by computational micromechanics. Compos. Sci. Technol. 2022, 218, 109139. [Google Scholar] [CrossRef]
- de Almeida, S.F.M.; Neto, Z.d.S.N. Effect of void content on the strength of composite laminates. Compos. Struct. 1994, 28, 139–148. [Google Scholar] [CrossRef]
- Zhang, A.; Li, D.; Lu, H.; Zhang, D. Qualitative separation of the effect of voids on the bending fatigue performance of hygrothermal conditioned carbon/epoxy composites. Mater. Des. 2011, 32, 4803–4809. [Google Scholar] [CrossRef]
- Liu, L.; Zhang, B.M.; Wang, D.F.; Wu, Z.J. Effects of cure cycles on void content and mechanical properties of composite laminates. Compos. Struct. 2006, 73, 303–309. [Google Scholar] [CrossRef]
- Cuong-Le, T.; Nguyen, K.D.; Nguyen-Trong, N.; Khatir, S.; Nguyen-Xuan, H.; Abdel-Wahab, M. A three-dimensional solution for free vibration and buckling of annular plate, conical, cylinder and cylindrical shell of FG porous-cellular materials using IGA. Compos. Struct. 2021, 259, 113216. [Google Scholar] [CrossRef]
- Thanh, C.L.; Nguyen, K.D.; Hoang, L.M.; Phuong, P.V.; Phuoc, N.T.; Tounsi, A. Nonlinear bending analysis of porous sigmoid FGM nanoplate via IGA and nonlocal strain gradient theory. Adv. Nano Res. 2022, 12, 441–455. [Google Scholar] [CrossRef]
- Wang, P.D.; Lei, H.S.; Zhu, X.L.; Chen, H.S.; Fang, D.N. Investigation on the mechanical properties of epoxy resin with void defects using digital image correlation and image-based finite element method. Polym. Test. 2018, 72, 223–231. [Google Scholar] [CrossRef]
- Bilyeu, B.; Brostow, W.; Menard, K.P. Determination of volume changes during cure via void elimination and shrinkage of an epoxy prepreg using a quartz dilatometry cell. Polimery 2001, 46, 799–802. [Google Scholar] [CrossRef]
- Bhat, P.; Merotte, J.; Simacek, P.; Advani, S.G. Process analysis of compression resin transfer molding. Compos. Part A-Appl. Sci. Manuf. 2009, 40, 431–441. [Google Scholar] [CrossRef]
- Simacek, P.; Advani, S.G.; Iobst, S.A. Modeling Flow in Compression Resin Transfer Molding for Manufacturing of Complex Lightweight High-Performance Automotive Parts. J. Compos. Mater. 2008, 42, 2523–2545. [Google Scholar] [CrossRef]
- Chang, C.Y. Simulation of Compression Effect on Filling Process in Compression Resin Transfer Molding. Adv. Compos. Mater. 2011, 20, 197–211. [Google Scholar] [CrossRef]
- Young, W.-B.; Chiu, C.-W. Study on Compression Transfer Molding. J. Compos. Mater. 1995, 29, 2180–2191. [Google Scholar] [CrossRef]
- Chang, C.-Y.; Lin, H.-J. Unsaturated polyester/E-glass fiber composites made by vacuum assisted compression resin transfer molding. J. Polym. Eng. 2012, 32, 539–546. [Google Scholar] [CrossRef]
- Chang, C.Y.; Hourng, L.W.; Chou, T.Y. Effect of process variables on the quality of compression resin transfer molding. J. Reinf. Plast. Compos. 2006, 25, 1027–1037. [Google Scholar] [CrossRef]
- Xiang, H.; Jiang, Y.M.; Qi, Y.X.; Li, J.L. Process-Induced Distortions Characterization of MBWK Fabric Reinforced Composite Helmet Shell. Materials 2020, 13, 2983. [Google Scholar] [CrossRef] [PubMed]


| Sample ID | RTM | M-CRTM | ||
|---|---|---|---|---|
| MPD (mm) | MND (mm) | MPD (mm) | MND (mm) | |
| First group | 1.6006 | −1.6001 | 1.3817 | −1.0621 |
| Second group | 1.8050 | −1.4163 | 1.2981 | −0.9283 |
| Third group | 1.8551 | −1.4523 | 1.3051 | −1.0351 |
| Average | 1.7536 | −1.4895 | 1.3283 | −1.0085 |
| Samples | Flexural Modulus of RTM (GPa) | Flexural Modulus of M-CRTM (GPa) |
|---|---|---|
| 1 | 50.88 | 51.95 |
| 2 | 48.23 | 50.86 |
| 3 | 50.31 | 50.30 |
| 4 | 47.46 | 51.41 |
| 49.86 | 51.13 | |
| CV (%) | 2.89 | 1.20 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, C.; Sun, Y.; Xu, J.; Shi, X.; Zhang, G. Study on Curing Deformation of Composite Thin Shells Prepared by M-CRTM with Adjustable Injection Gap. Polymers 2022, 14, 5564. https://doi.org/10.3390/polym14245564
Zhang C, Sun Y, Xu J, Shi X, Zhang G. Study on Curing Deformation of Composite Thin Shells Prepared by M-CRTM with Adjustable Injection Gap. Polymers. 2022; 14(24):5564. https://doi.org/10.3390/polym14245564
Chicago/Turabian StyleZhang, Ce, Ying Sun, Jing Xu, Xiaoping Shi, and Guoli Zhang. 2022. "Study on Curing Deformation of Composite Thin Shells Prepared by M-CRTM with Adjustable Injection Gap" Polymers 14, no. 24: 5564. https://doi.org/10.3390/polym14245564
APA StyleZhang, C., Sun, Y., Xu, J., Shi, X., & Zhang, G. (2022). Study on Curing Deformation of Composite Thin Shells Prepared by M-CRTM with Adjustable Injection Gap. Polymers, 14(24), 5564. https://doi.org/10.3390/polym14245564





