Nonlinear Thermopower Behaviour of N-Type Carbon Nanofibres and Their Melt Mixed Polypropylene Composites
Abstract
1. Introduction
2. Materials and Methods
2.1. Material Processing
2.2. TEM, SEM, Raman and XPS Analysis
2.3. Thermoelectric Analysis
3. Results and Discussion
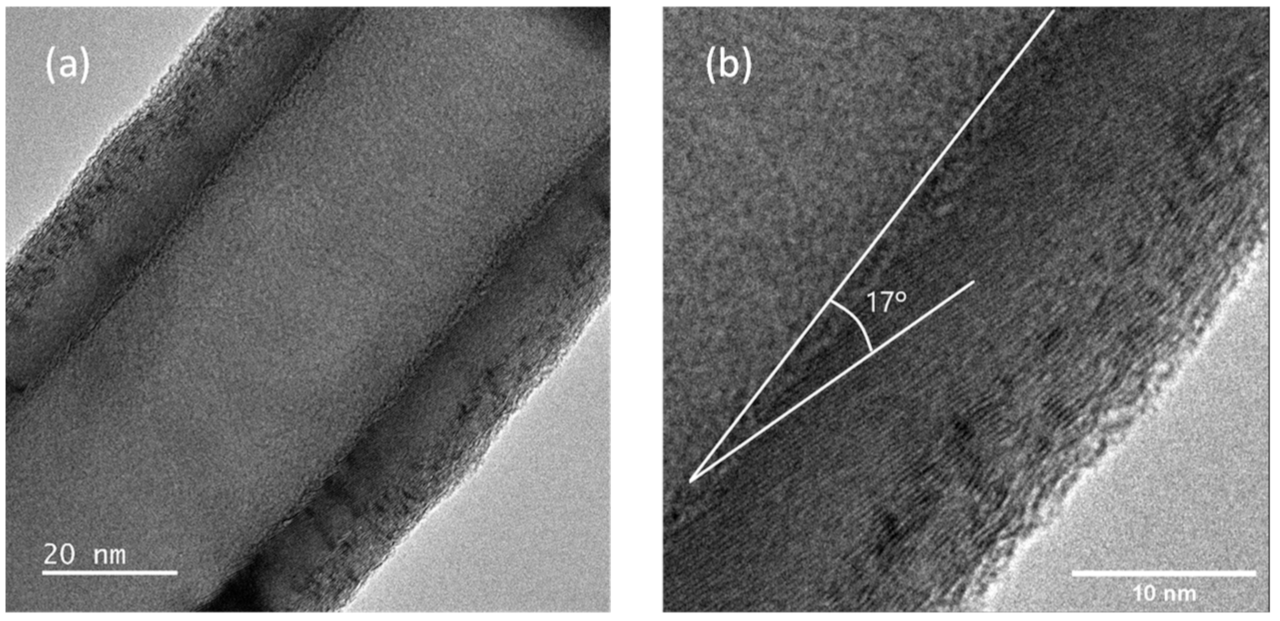
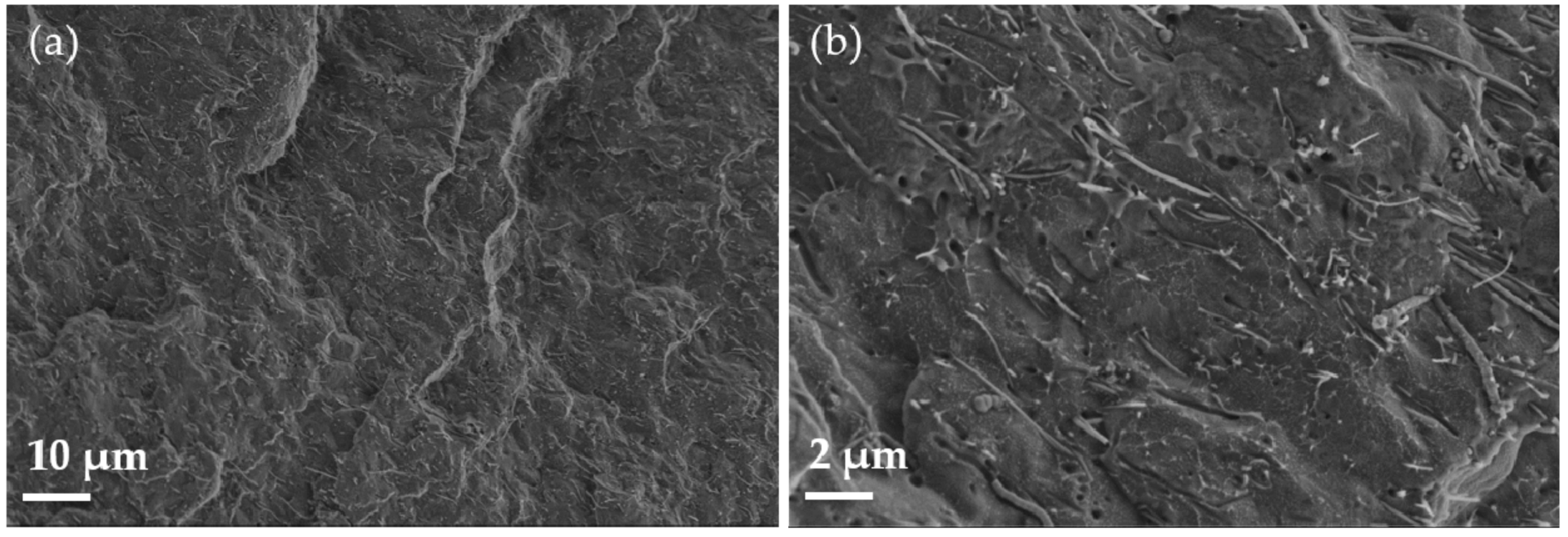
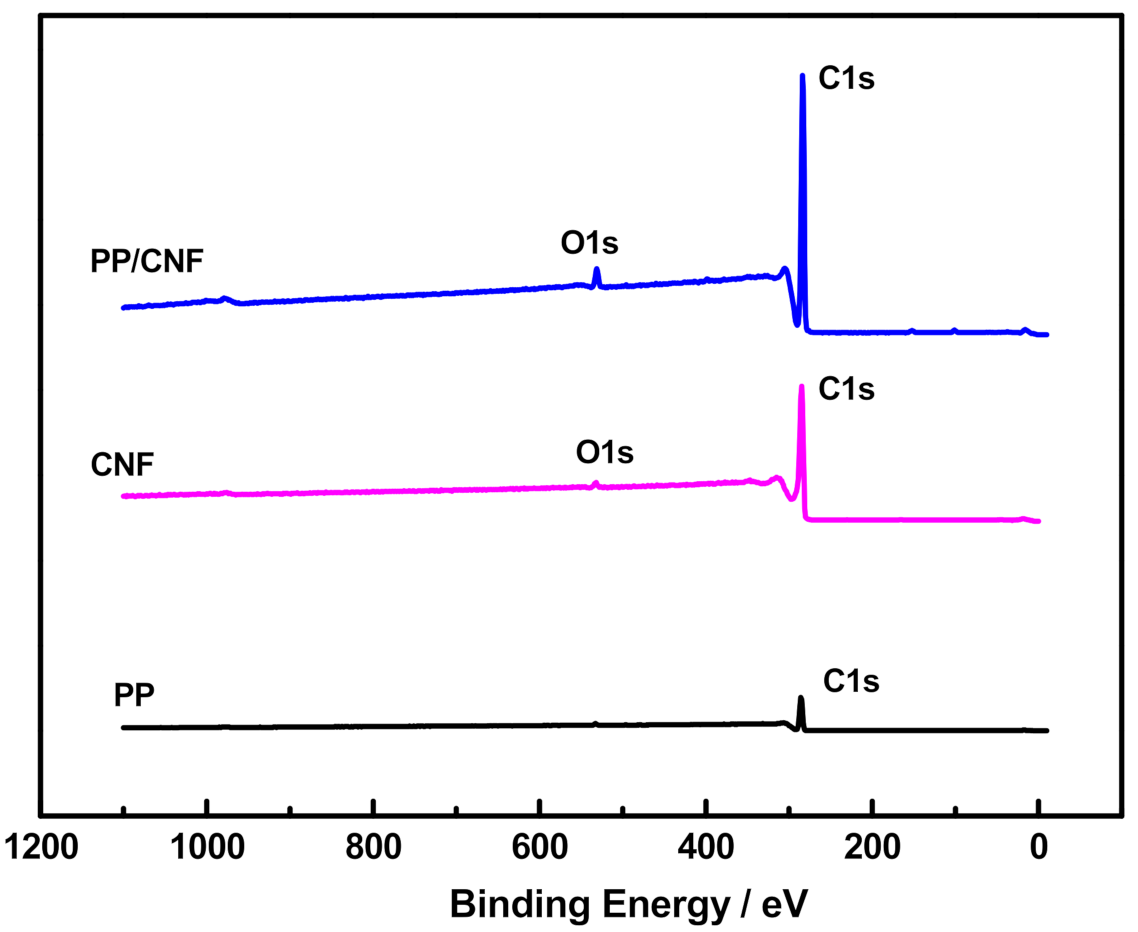
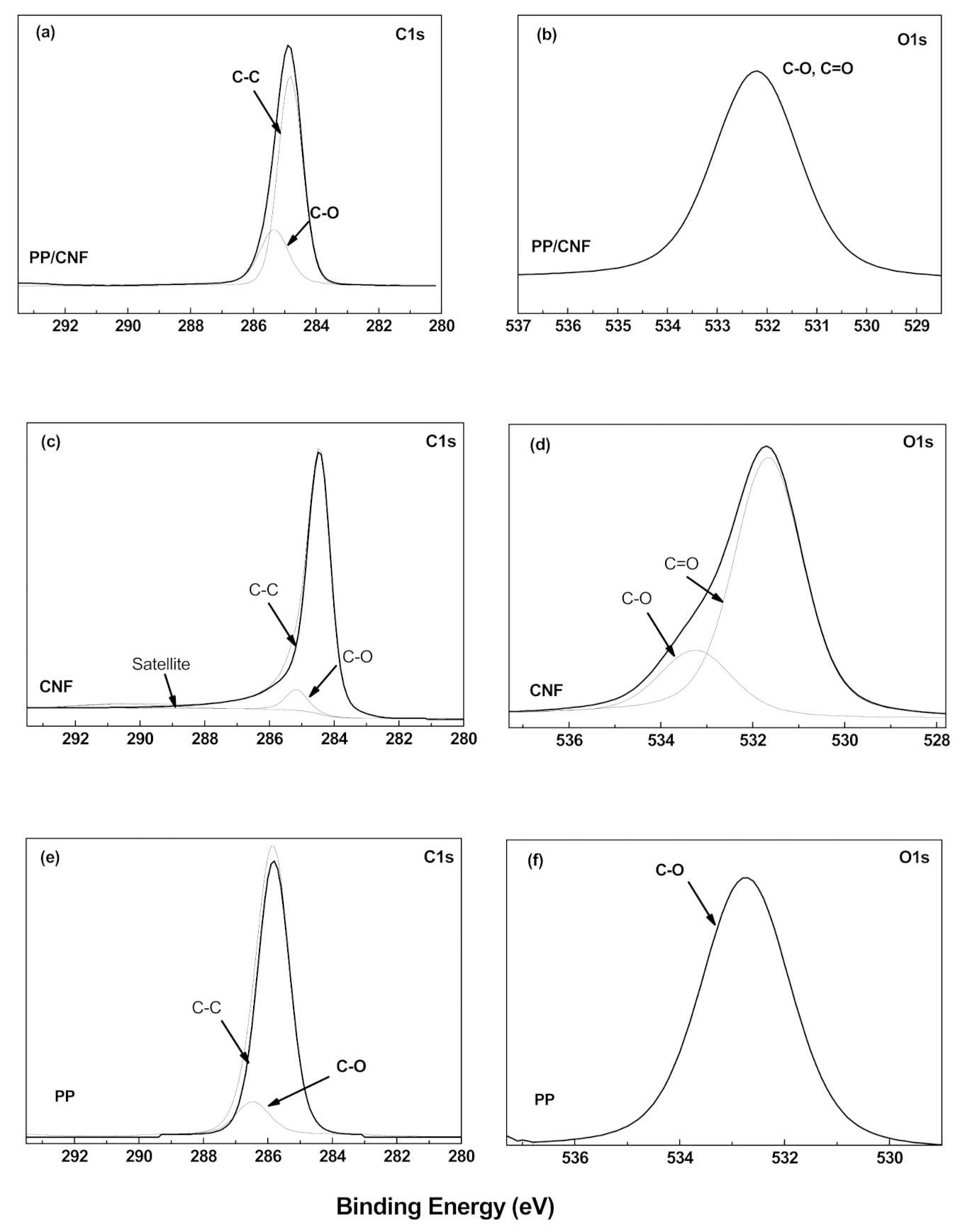
3.1. TEM, SEM, Raman and XPS Analysis
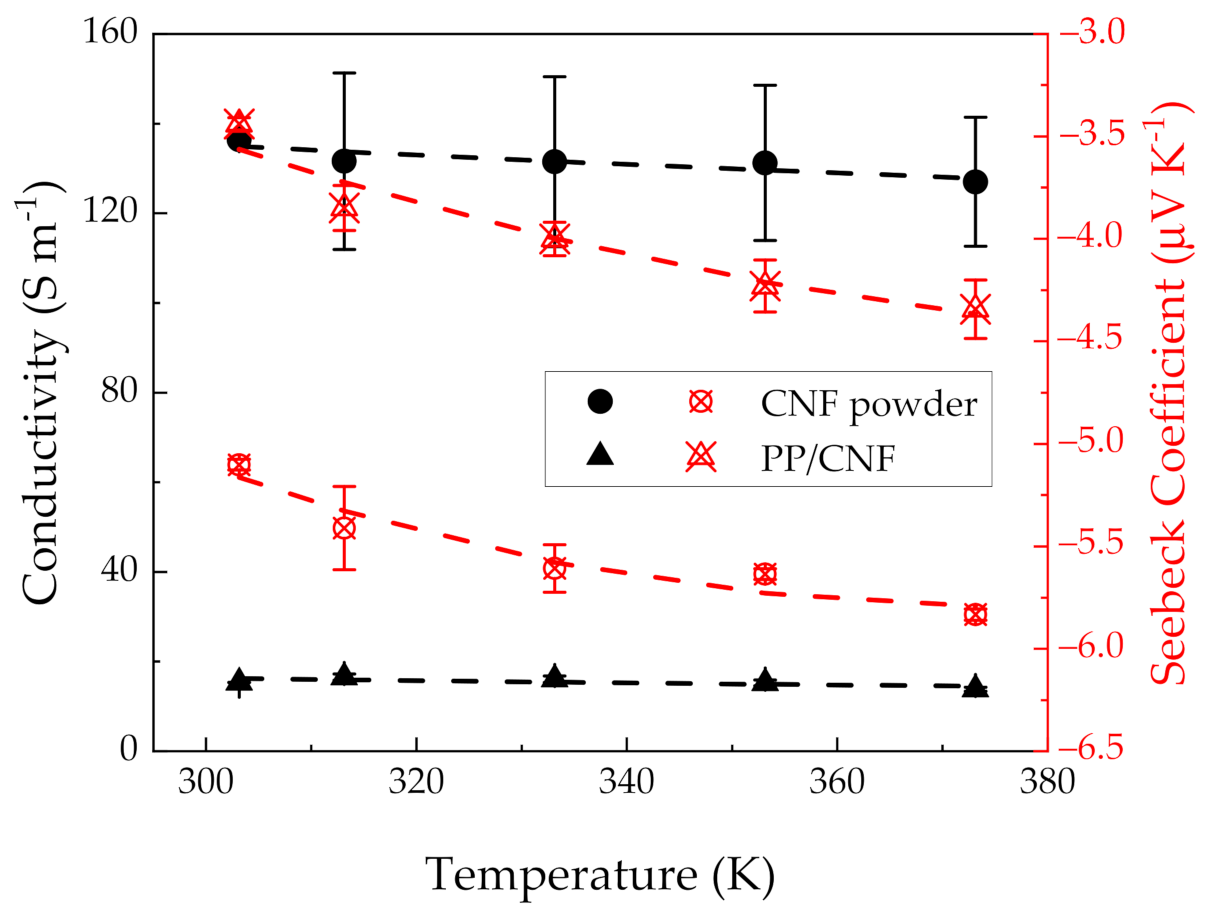
3.2. Thermoelectric Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Nandihalli, N.; Liu, C.-J.; Mori, T. Polymer based thermoelectric nanocomposite materials and devices: Fabrication and characteristics. Nano Energy 2020, 78, 105186. [Google Scholar] [CrossRef]
- Rowe, D.M. CRC Handbook of Thermoelectrics; CRC Press: Boca Raton, FL, USA, 1995. [Google Scholar]
- Blackburn, J.L.; Ferguson, A.J.; Cho, C.; Grunlan, J.C. Carbon-Nanotube-Based Thermoelectric Materials and Devices. Adv. Mater. 2018, 30, 1704386. [Google Scholar] [CrossRef] [PubMed]
- Vineis, C.J.; Shakouri, A.; Majumdar, A.; Kanatzidis, M.G. Nanostructured Thermoelectrics: Big Efficiency Gains from Small Features. Adv. Mater. 2010, 22, 3970–3980. [Google Scholar] [CrossRef] [PubMed]
- Tan, G.; Zhao, L.-D.; Kanatzidis, M.G. Rationally Designing High-Performance Bulk Thermoelectric Materials. Chem. Rev. 2016, 116, 12123–12149. [Google Scholar] [CrossRef]
- Han, S.; Chen, S.; Jiao, F. Insulating polymers for flexible thermoelectric composites: A multi-perspective review. Compos. Commun. 2021, 28, 100914. [Google Scholar] [CrossRef]
- Baghdadi, N.; Zoromba, M.S.; Abdel-Aziz, M.H.; Al-Hossainy, A.F.; Bassyouni, M.; Salah, N. One-Dimensional Nanocomposites Based on Polypyrrole-Carbon Nanotubes and Their Thermoelectric Performance. Polymers 2021, 13, 278. [Google Scholar] [CrossRef]
- Du, Y.; Cai, K.F.; Chen, S.; Cizek, P.; Lin, T. Facile Preparation and Thermoelectric Properties of Bi2Te3 Based Alloy Nanosheet/PEDOT:PSS Composite Films. ACS Appl. Mater. Interfaces 2014, 6, 5735–5743. [Google Scholar] [CrossRef]
- He, M.; Ge, J.; Lin, Z.; Feng, X.; Wang, X.; Lu, H.; Yang, Y.; Qiu, F. Thermopower enhancement in conducting polymer nanocomposites via carrier energy scattering at the organic–inorganic semiconductor interface. Energy Environ. Sci. 2012, 5, 8351–8358. [Google Scholar] [CrossRef]
- Bitenieks, J.; Buks, K.; Merijs-Meri, R.; Andzane, J.; Ivanova, T.; Bugovecka, L.; Voikiva, V.; Zicans, J.; Erts, D. Flexible N-Type Thermoelectric Composites Based on Non-Conductive Polymer with Innovative Bi2Se3-CNT Hybrid Nanostructured Filler. Polymers 2021, 13, 4264. [Google Scholar] [CrossRef]
- Luo, J.; Cerretti, G.; Krause, B.; Zhang, L.; Otto, T.; Jenschke, W.; Ullrich, M.; Tremel, W.; Voit, B.; Pötschke, P. Polypropylene-based melt mixed composites with singlewalled carbon nanotubes for thermoelectric applications: Switching from p-type to n-type by the addition of polyethylene glycol. Polymer 2017, 108, 513–520. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, Q.; Chen, G. Carbon and carbon composites for thermoelectric applications. Carbon Energy 2020, 2, 408–436. [Google Scholar] [CrossRef]
- Massetti, M.; Jiao, F.; Ferguson, A.J.; Zhao, D.; Wijeratne, K.; Würger, A.; Blackburn, J.L.; Crispin, X.; Fabiano, S. Unconventional Thermoelectric Materials for Energy Harvesting and Sensing Applications. Chem. Rev. 2021, 20, 12465–12547. [Google Scholar] [CrossRef]
- Krause, B.; Barbier, C.; Levente, J.; Klaus, M.; Pötschke, P. Screening of Different Carbon Nanotubes in Melt-Mixed Polymer Composites with Different Polymer Matrices for Their Thermoelectrical Properties. J. Compos. Sci. 2019, 3, 106. [Google Scholar] [CrossRef]
- Krause, B.; Konidakis, I.; Arjmand, M.; Sundararaj, U.; Fuge, R.; Liebscher, M.; Hampel, S.; Klaus, M.; Serpetzoglou, E.; Stratakis, E.; et al. Nitrogen-Doped Carbon Nanotube/Polypropylene Composites with Negative Seebeck Coefficient. J. Compos. Sci. 2020, 4, 14. [Google Scholar] [CrossRef]
- Kang, I.; Heung, Y.Y.; Kim, J.H.; Lee, J.W.; Gollapudi, R.; Subramaniam, S.; Narasimhadevara, S.; Hurd, D.; Kirikera, G.R.; Shanov, V.; et al. Introduction to carbon nanotube and nanofiber smart materials. Compos. Part B Eng. 2006, 37, 382–394. [Google Scholar] [CrossRef]
- Endo, M.; Tamagawa, I.; Koyama, T. Thermoelectric Power of Carbon Fibers Prepared from Benzene. Jpn. J. Appl. Phys. 1977, 16, 1771–1774. [Google Scholar] [CrossRef]
- Heremans, J.; Beetz, C.P. Thermal conductivity and thermopower of vapor-grown graphite fibers. Phys. Rev. B 1985, 32, 1981–1986. [Google Scholar] [CrossRef] [PubMed]
- Stokes, K.L.; Tritt, T.M.; Fuller-Mora, W.W.; Ehrlich, A.C.; Jacobsen, R.L. Electronic transport properties of highly conducting vapor-grown carbon fiber composites. In Proceedings of the Fifteenth International Conference on Thermoelectrics. Proceedings ICT ‘96, Pasadena, CA, USA, 26–29 March 1996; pp. 164–167. [Google Scholar] [CrossRef]
- Brownlie, L.; Shapter, J. Advances in carbon nanotube n-type doping: Methods, analysis and applications. Carbon 2018, 126, 257–270. [Google Scholar] [CrossRef]
- Paleo, A.J.; Krause, B.; Cerqueira, M.F.; Melle-Franco, M.; Pötschke, P.; Rocha, A.M. Thermoelectric properties of polypropylene carbon nanofiber melt-mixed composites: Exploring the role of polymer on their Seebeck coefficient. Polym. J. 2021, 53, 1145–1152. [Google Scholar] [CrossRef]
- Paleo, A.J.; Vieira, E.M.F.; Wan, K.; Bondarchuk, O.; Cerqueira, M.F.; Goncalves, L.M.; Bilotti, E.; Alpuim, P.; Rocha, A.M. Negative thermoelectric power of melt mixed vapor grown carbon nanofiber polypropylene composites. Carbon 2019, 150, 408–416. [Google Scholar] [CrossRef]
- Paleo, A.J.; Sencadas, V.; Van Hattum, F.W.J.; Lanceros-Méndez, S.; Ares, A. Carbon nanofiber type and content dependence of the physical properties of carbon nanofiber reinforced polypropylene composites. Polym. Eng. Sci. 2014, 54, 117–128. [Google Scholar] [CrossRef]
- Jenschke, W.; Ullrich, M.; Krause, B.; Pötschke, P. Messanlage zur Untersuchung des Seebeck-Effektes in Polymermaterialien Measuring apparatus for study of Seebeck effect in polymer materials. Tech. Mess. 2020, 87, 495–503. [Google Scholar] [CrossRef]
- Gnanaseelan, M.; Chen, Y.; Luo, J.; Krause, B.; Pionteck, J.; Pötschke, P.; Qi, H. Cellulose-carbon nanotube composite aerogels as novel thermoelectric materials. Compos. Sci. Technol. 2018, 163, 133–140. [Google Scholar] [CrossRef]
- Tessonnier, J.P.; Rosenthal, D.; Hansen, T.W.; Hess, C.; Schuster, M.E.; Blume, R.; Girgsdies, F.; Pfänder, N.; Timpe, O.; Su, D.S.; et al. Analysis of the structure and chemical properties of some commercial carbon nanostructures. Carbon 2009, 47, 1779–1798. [Google Scholar] [CrossRef]
- Nielsen, A.S.; Pyrz, R. A Raman study into the effect of transcrystallisation on thermal stresses in embedded single fibres. J. Mater. Sci. 2003, 38, 597–601. [Google Scholar] [CrossRef]
- Snyder, R.G.; Schachtschneider, J.H. Valence force calculation of the vibrational spectra of crystalline isotactic polypropylene and some deuterated polypropylenes. Spectrochim. Acta 1964, 20, 853–869. [Google Scholar] [CrossRef]
- Lehman, J.H.; Terrones, M.; Mansfield, E.; Hurst, K.E.; Meunier, V. Evaluating the characteristics of multiwall carbon nanotubes. Carbon 2011, 49, 2581–2602. [Google Scholar] [CrossRef]
- Wang, Y.; Alsmeyer, D.C.; McCreery, R.L. Raman Spectroscopy of Carbon Materials: Structural Basis of Observed Spectra. Chem. Mater. 1990, 2, 557–563. [Google Scholar] [CrossRef]
- Chipara, M.; Villarreal, J.R.; Chipara, M.D.; Lozano, K.; Chipara, A.C.; Sellmyer, D.J. Spectroscopic investigations on polypropylene-carbon nanofiber composites. I. Raman and electron spin resonance spectroscopy. J. Polym. Sci. Part B Polym. Phys. 2009, 47, 1644–1652. [Google Scholar] [CrossRef]
- Knight, D.S.; White, W.B. Characterization of diamond films by Raman spectroscopy. J. Mater. Res. 1989, 4, 385–393. [Google Scholar] [CrossRef]
- Ferrari, A.; Robertson, J. Interpretation of Raman spectra of disordered and amorphous carbon. Phys. Rev. B-Condens. Matter Mater. Phys. 2000, 61, 14095–14107. [Google Scholar] [CrossRef]
- Chiang, M.H.; Liao, K.C.; Lin, I.M.; Lu, C.C.; Huang, H.Y.; Kuo, C.L.; Wu, J.S. Modification of hydrophilic property of polypropylene films by a parallel-plate nitrogen-based dielectric barrier discharge jet. IEEE Trans. Plasma Sci. 2010, 38, 1489–1498. [Google Scholar] [CrossRef]
- Klein, K.L.; Melechko, A.V.; McKnight, T.E.; Retterer, S.T.; Rack, P.D.; Fowlkes, J.D.; Joy, D.C.; Simpson, M.L. Surface characterization and functionalization of carbon nanofibers. J. Appl. Phys. 2008, 103, 3. [Google Scholar] [CrossRef]
- Zhou, J.H.; Sui, Z.J.; Zhu, J.; Li, P.; Chen, D.; Dai, Y.C.; Yuan, W.K. Characterization of surface oxygen complexes on carbon nanofibers by TPD, XPS and FT-IR. Carbon 2007, 45, 785–796. [Google Scholar] [CrossRef]
- Kim, H.I.; Kim, S.S. Plasma treatment of polypropylene and polysulfone supports for thin film composite reverse osmosis membrane. J. Membr. Sci. 2006, 286, 193–201. [Google Scholar] [CrossRef]
- Cuervo, M.R.; Asedegbega-Nieto, E.; Díaz, E.; Vega, A.; Ordóñez, S.; Castillejos-López, E.; Rodríguez-Ramos, I. Effect of carbon nanofiber functionalization on the adsorption properties of volatile organic compounds. J. Chromatogr. A 2008, 1188, 264–273. [Google Scholar] [CrossRef]
- Zhong, R.S.; Qin, Y.H.; Niu, D.F.; Zhang, X.S.; Zhou, X.G.; Sun, S.G.; Yuan, W.K. Effect of carbon nanofiber surface groups on oxygen reduction reaction of supported Pt electrocatalyst. Electrochim. Acta 2013, 89, 157–162. [Google Scholar] [CrossRef]
- Al-Saleh, M.H.; Sundararaj, U. A review of vapor grown carbon nanofiber/polymer conductive composites. Carbon 2009, 47, 2–22. [Google Scholar] [CrossRef]
- Hewitt, C.A.; Kaiser, A.B.; Roth, S.; Craps, M.; Czerw, R.; Carroll, D.L. Varying the concentration of single walled carbon nanotubes in thin film polymer composites, and its effect on thermoelectric power. Appl. Phys. Lett. 2011, 98. [Google Scholar] [CrossRef]
- Kymakis, E.; Amaratunga, G.A.J. Electrical properties of single-wall carbon nanotube-polymer composite films. J. Appl. Phys. 2006, 99, 084302. [Google Scholar] [CrossRef]
- Fuhrer, M.S.; Holmes, W.; Richards, P.L.; Delaney, P.; Louie, S.G.; Zettl, A. Nonlinear transport and localization in single-walled carbon nanotubes. Synth. Met. 1999, 103, 2529–2532. [Google Scholar] [CrossRef][Green Version]
- Choi, Y.M.; Lee, D.S.; Czerw, R.; Chiu, P.W.; Grobert, N.; Terrones, M.; Reyes-Reyes, M.; Terrones, H.; Charlier, J.C.; Ajayan, P.M.; et al. Nonlinear behavior in the thermopower of doped carbon nanotubes due to strong, localized states. Nano Lett. 2003, 3, 839–842. [Google Scholar] [CrossRef]
- Mott, N.F. Conduction in glasses containing transition metal ions. J. Non-Cryst. Solids 1968, 1, 1–17. [Google Scholar] [CrossRef]
- Mahan, G.D. Impurity resonances in carbon nanotubes. Phys. Rev. B-Condens. Matter Mater. Phys. 2004, 69, 125407. [Google Scholar] [CrossRef]
- Ivanov, D.K.; Ivanov, K.G.; Uryupin, O.N. Resistance and thermoelectric power of carbon fibers upon changing the conductivity type. Semiconductors 2017, 51, 834–835. [Google Scholar] [CrossRef]
- Paleo, A.J.; Van Hattum, F.W.J.; Pereira, J.; Rocha, J.G.; Silva, J.; Sencadas, V.; Lanceros-Méndez, S. The piezoresistive effect in polypropylene-carbon nanofibre composites obtained by shear extrusion. Smart Mater. Struct. 2010, 19, 065013. [Google Scholar] [CrossRef]
- Li, C.; Thostenson, E.T.; Chou, T.W. Dominant role of tunneling resistance in the electrical conductivity of carbon nanotube-based composites. Appl. Phys. Lett. 2007, 91, 223114. [Google Scholar] [CrossRef]
- Stavropoulos, S.G.; Sanida, A.; Psarras, G.C. A comparative study on the electrical properties of different forms of carbon allotropes-Epoxy nanocomposites. Express Polym. Lett. 2020, 14, 477–490. [Google Scholar] [CrossRef]
- Psarras, G.C. Charge transport properties in carbon black/polymer composites. J. Polym. Sci. Part B Polym. Phys. 2007, 45, 2535–2545. [Google Scholar] [CrossRef]
- Cardoso, P.; Silva, J.; Agostinho Moreira, J.; Klosterman, D.; Van Hattum, F.W.J.; Simoes, R.; Lanceros-Mendez, S. Temperature dependence of the electrical conductivity of vapor grown carbon nanofiber/epoxy composites with different filler dispersion levels. Phys. Lett. Sect. A Gen. At. Solid State Phys. 2012, 376, 3290–3294. [Google Scholar] [CrossRef]
- Collins, P.G.; Bradley, K.; Ishigami, M.; Zettl, A. Extreme Oxygen Sensitivity of Electronic Properties of Carbon Nanotubes. Science 2000, 287, 1801–1804. [Google Scholar] [CrossRef] [PubMed]
- Kumanek, B.; Stando, G.; Wróbel, P.S.; Janas, D. Impact of synthesis parameters of multi-walled carbon nanotubes on their thermoelectric properties. Materials 2019, 12, 3567. [Google Scholar] [CrossRef]
- Hewitt, C.A.; Kaiser, A.B.; Craps, M.; Czerw, R.; Carroll, D.L. Negative thermoelectric power from large diameter multiwalled carbon nanotubes grown at high chemical vapor deposition temperatures. J. Appl. Phys. 2013, 114, 083701. [Google Scholar] [CrossRef]
- Krause, B.; Bezugly, V.; Khavrus, V.; Ye, L.; Cuniberti, G.; Pötschke, P. Boron Doping of SWCNTs as a Way to Enhance the Thermoelectric Properties of Melt-Mixed Polypropylene/SWCNT Composites. Energies 2020, 13, 394. [Google Scholar] [CrossRef]
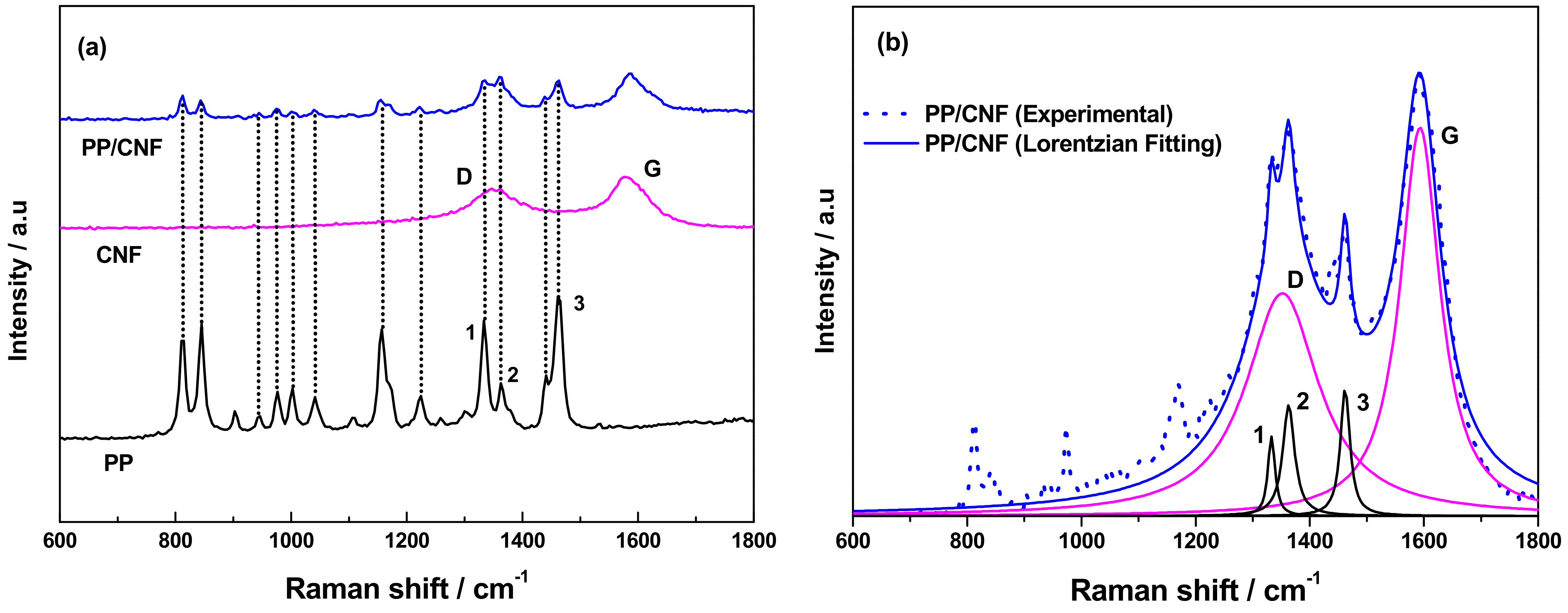
| Sample | ωG (cm−1) | FWHMG (cm−1) | ωD (cm−1) | FWHMD (cm−1) | ID/IG | La(nm) |
|---|---|---|---|---|---|---|
| CNF | 1580 | 90 | 1352 | 115 | 0.76 | 5.8 |
| PP/CNF | 1587 | 50 | 1353 | 75 | 0.7 | 6.3 |
| Sample | O1s | C1s | S2p | Si2p |
|---|---|---|---|---|
| PP | 2.0 | 97.5 | - | 0.5 |
| CNF | 1.6 | 98.2 | 0.1 | - |
| PP/CNF | 2.6 | 96.9 | - | 0.5 |
| CNF Powder | PP/CNF | |||||||
|---|---|---|---|---|---|---|---|---|
| Temperature (K) | σ (S m−1) | S (µV K−1) | PF (μW m−1K−2) | σ (S m−1) | S (µV K−1) | PF (μW m−1K−2) | k (W m−1K−1) | zT |
| 303.15 | 136.39 ± 0.22 | −5.10 ± 0.03 | 3.55 × 10−3 | 15.36 ± 0.01 | −3.44 ± 0.03 | 1.82 × 10−4 | 0.25 | 2.20 × 10−7 |
| 313.15 | 131.51 ± 19.70 | −5.41 ± 0.20 | 3.85 × 10−3 | 16.54 ± 0.70 | −3.85 ± 0.11 | 2.45 × 10−4 | 0.25 | 3.07 × 10−7 |
| 333.15 | 131.48 ± 18.95 | −5.61 ± 0.12 | 4.13 × 10−3 | 16.07 ± 0.72 | −4.00 ± 0.08 | 2.57 × 10−4 | 0.24 | 3.57 × 10−7 |
| 353.15 | 131.21 ± 17.33 | −5.64 ± 0.03 | 4.17 × 10−3 | 15.26 ± 0.64 | −4.23 ± 0.13 | 2.73 × 10−4 | 0.24 | 4.09 × 10−7 |
| 373.15 | 127.01 ± 14.36 | −5.83 ± 0.03 | 4.32 × 10−3 | 13.86 ± 0.45 | −4.34 ± 0.14 | 2.61 × 10−4 | 0.22 | 4.39 × 10−7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paleo, A.J.; Krause, B.; Cerqueira, M.F.; Muñoz, E.; Pötschke, P.; Rocha, A.M. Nonlinear Thermopower Behaviour of N-Type Carbon Nanofibres and Their Melt Mixed Polypropylene Composites. Polymers 2022, 14, 269. https://doi.org/10.3390/polym14020269
Paleo AJ, Krause B, Cerqueira MF, Muñoz E, Pötschke P, Rocha AM. Nonlinear Thermopower Behaviour of N-Type Carbon Nanofibres and Their Melt Mixed Polypropylene Composites. Polymers. 2022; 14(2):269. https://doi.org/10.3390/polym14020269
Chicago/Turabian StylePaleo, Antonio J., Beate Krause, Maria F. Cerqueira, Enrique Muñoz, Petra Pötschke, and Ana M. Rocha. 2022. "Nonlinear Thermopower Behaviour of N-Type Carbon Nanofibres and Their Melt Mixed Polypropylene Composites" Polymers 14, no. 2: 269. https://doi.org/10.3390/polym14020269
APA StylePaleo, A. J., Krause, B., Cerqueira, M. F., Muñoz, E., Pötschke, P., & Rocha, A. M. (2022). Nonlinear Thermopower Behaviour of N-Type Carbon Nanofibres and Their Melt Mixed Polypropylene Composites. Polymers, 14(2), 269. https://doi.org/10.3390/polym14020269








