Piezoresistive Properties of 3D-Printed Polylactic Acid (PLA) Nanocomposites
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Nanocomposites Preparation
2.3. 3D Printing
2.4. Electrical Resistance Measurement
2.5. Piezoresistive Pressure Measurement
3. Results and Discussion
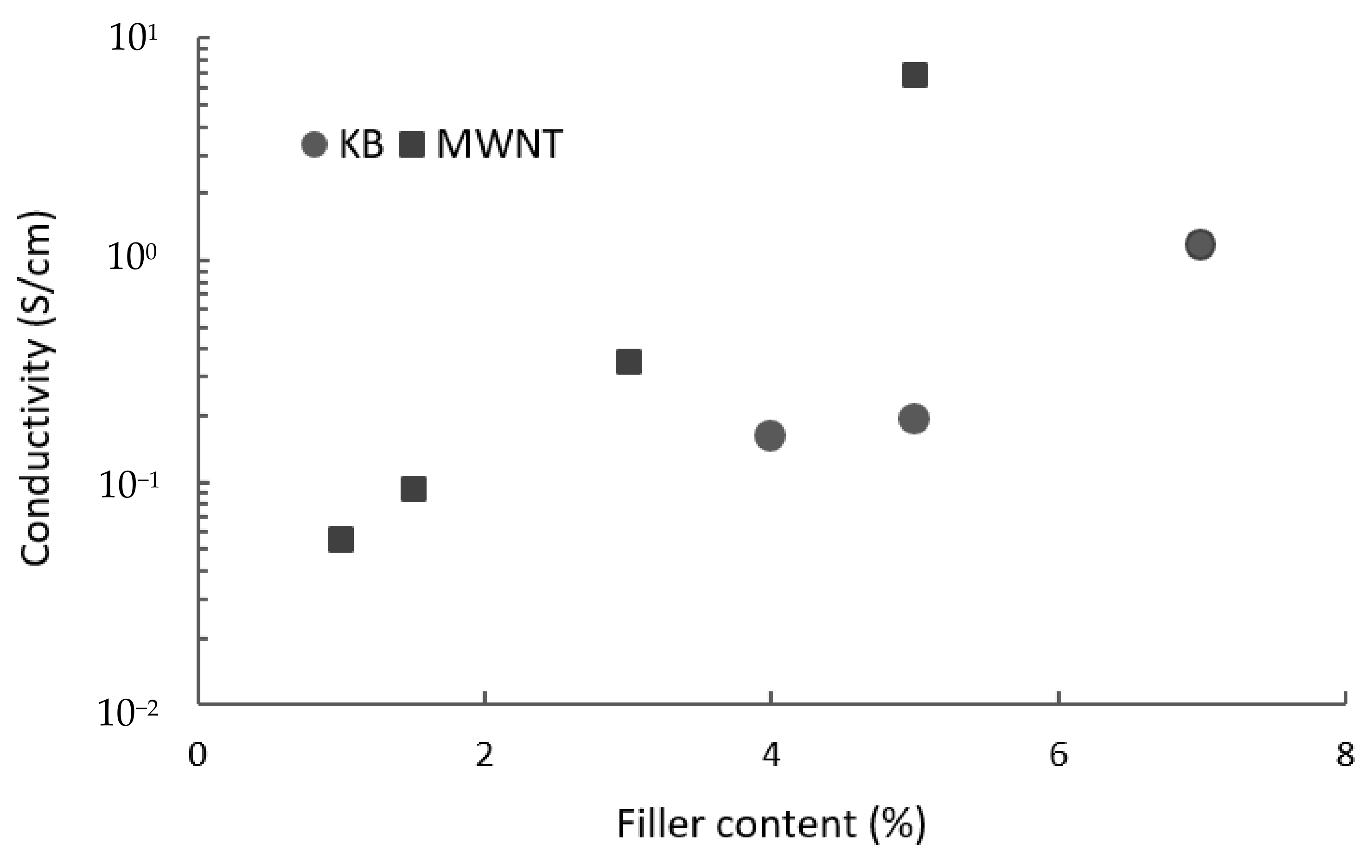
3.1. Electrical Characterization
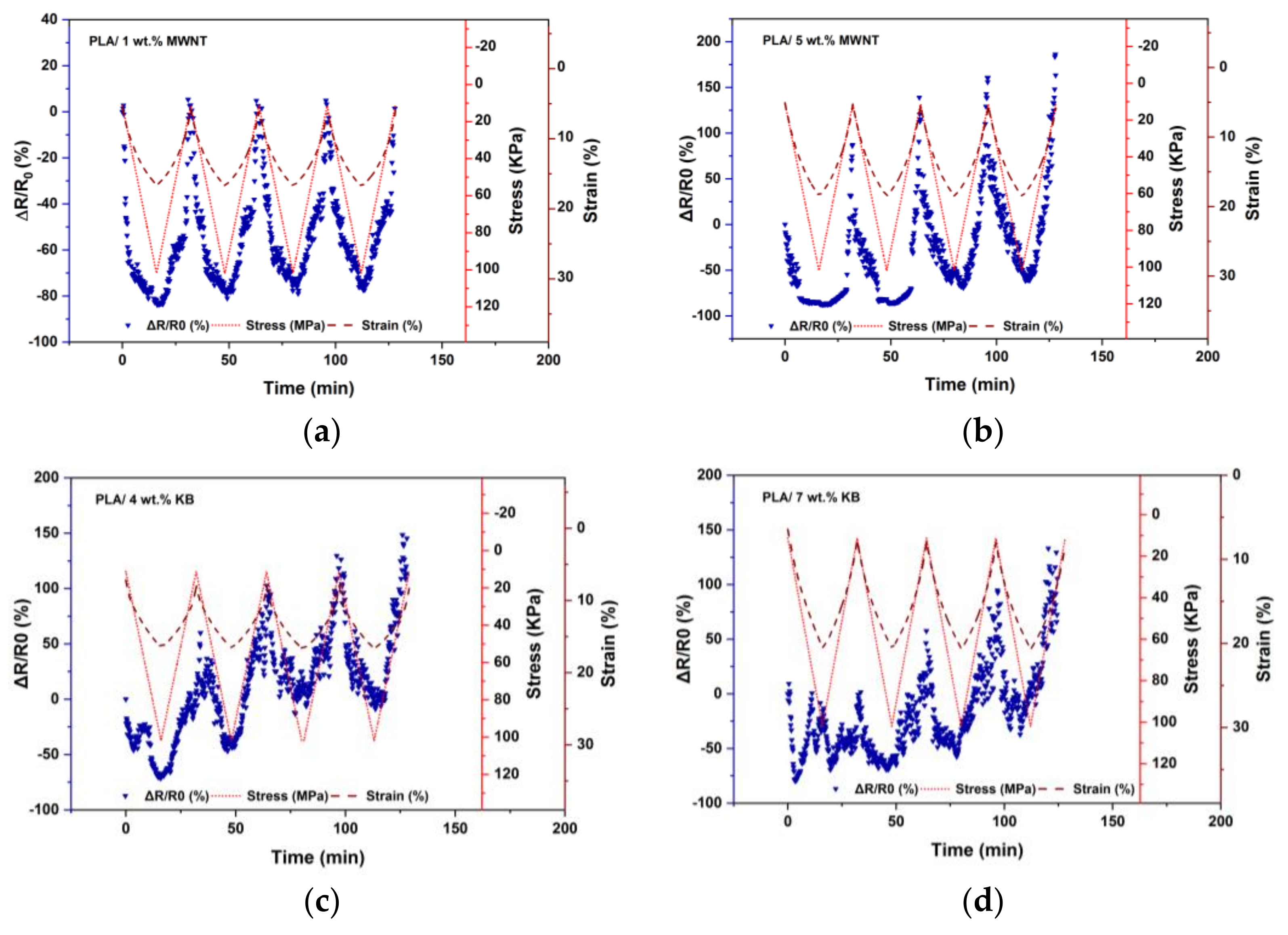
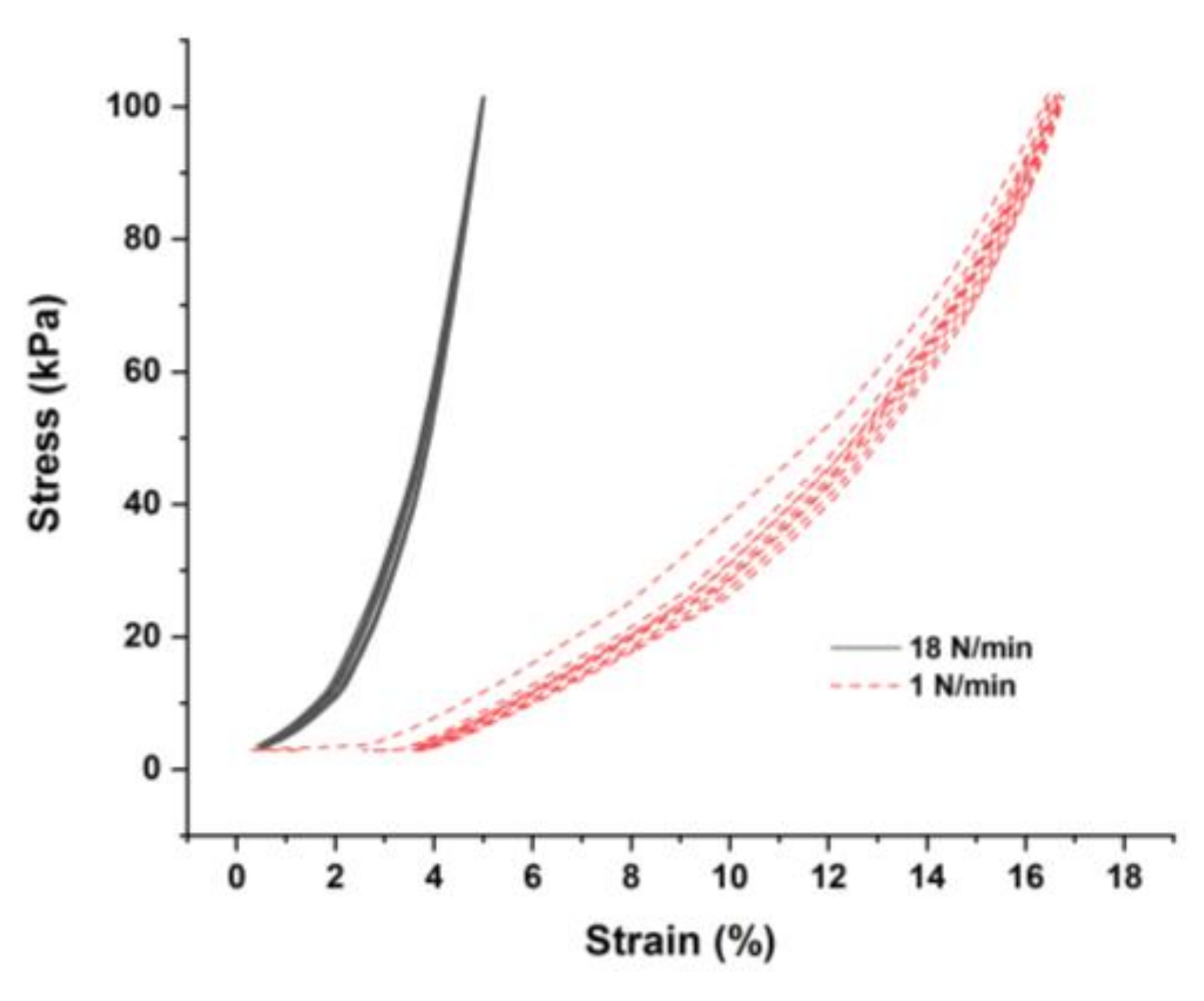
3.2. Compression Piezoresistive Properties
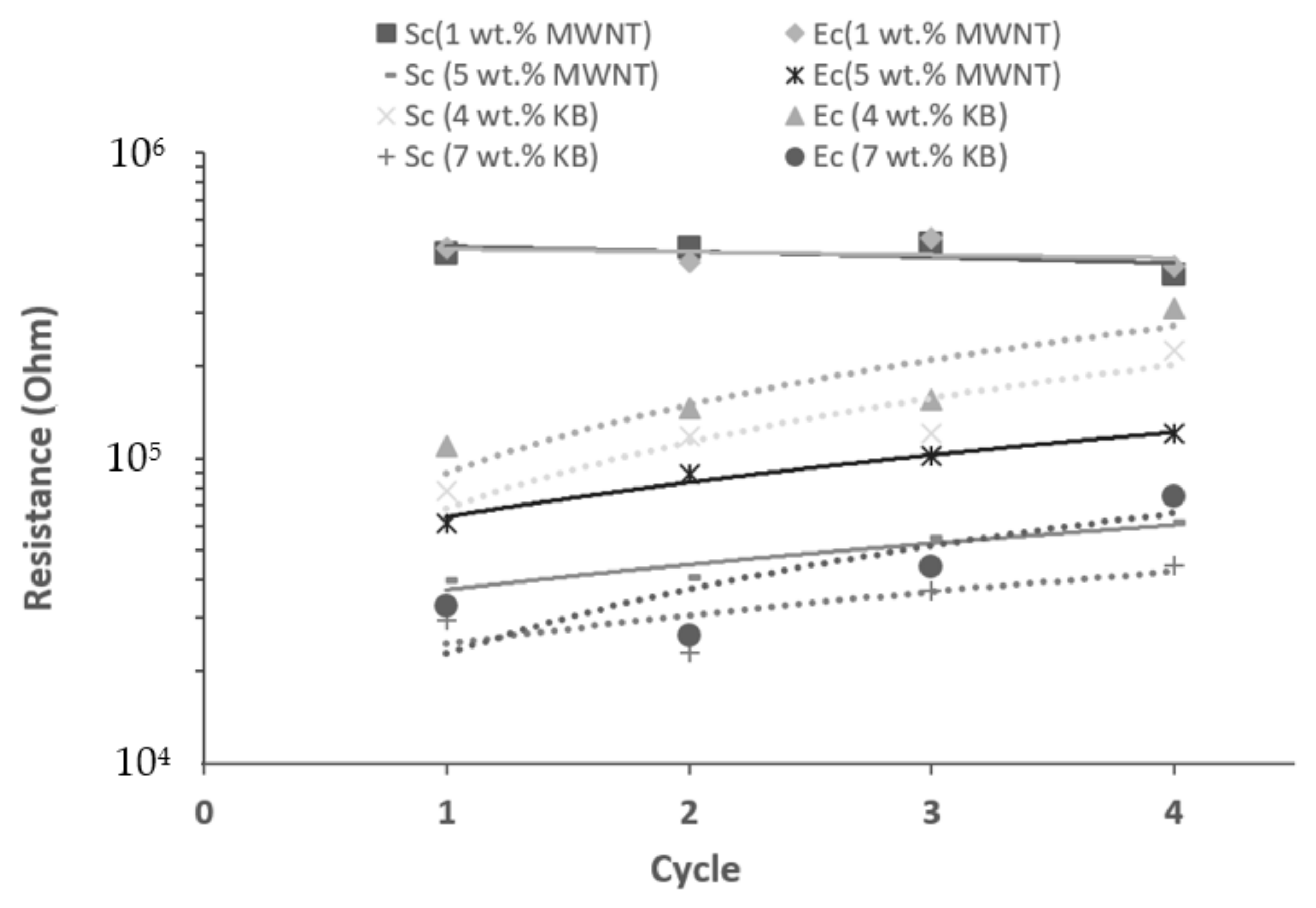
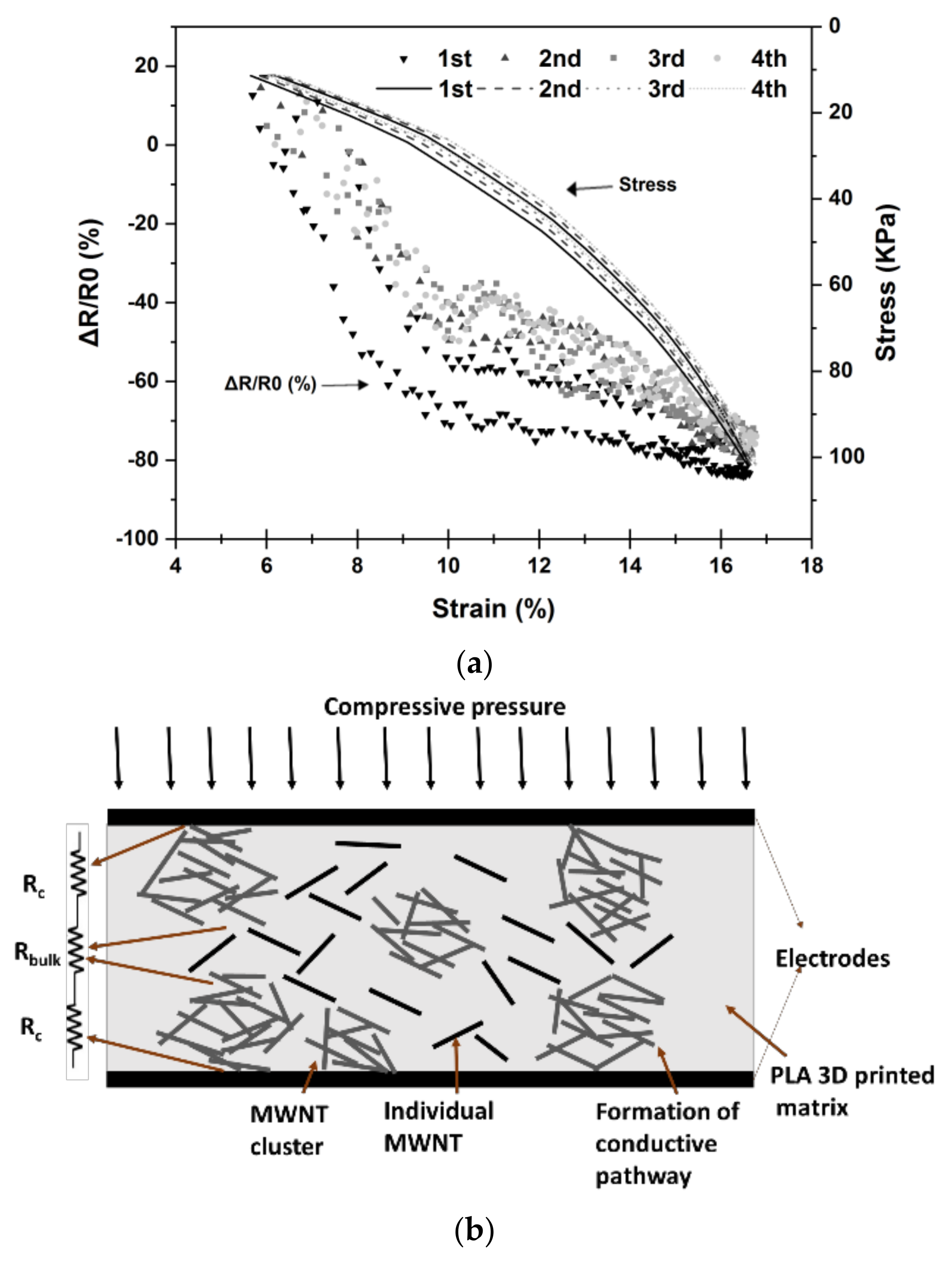
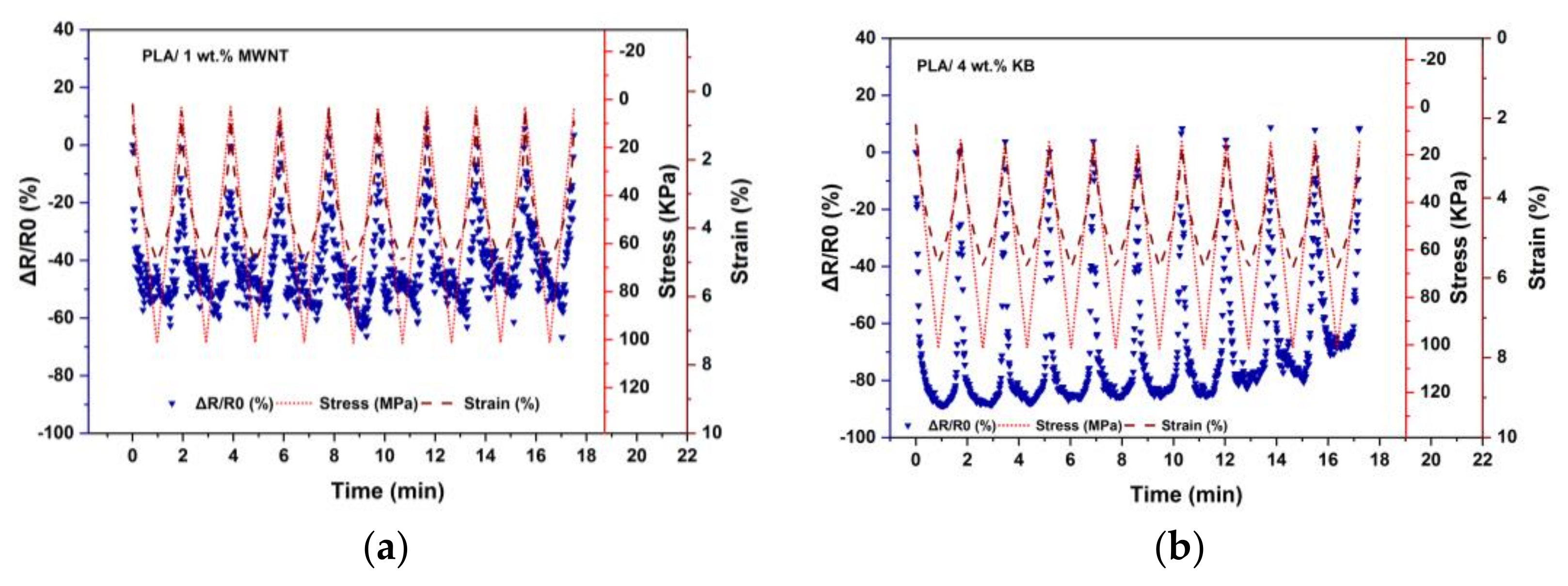
3.3. Compression Piezoresistive Behavior in a Cyclic Mode
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tee, Y.L.; Tran, P.; Leary, M.; Pille, P.; Brandt, M. 3D Printing of polymer composites with material jetting: Mechanical and fractographic analysis. Addit. Manuf. 2020, 36, 101558. [Google Scholar] [CrossRef]
- Mehrpouya, M.; Tuma, D.; Vaneker, T.; Afrasiabi, M.; Advanced, M.B.; Lab, M.; Zurich, E.; Gibson, I. Multimaterial powder bed fusion techniques. Rapid Prototyp. J. 2022, 28, 1–19. [Google Scholar] [CrossRef]
- Boyle, B.M.; Xiong, P.T.; Mensch, T.E.; Werder, T.J.; Miyake, G.M. 3D printing using powder melt extrusion. Addit. Manuf. 2019, 29, 100811. [Google Scholar] [CrossRef] [PubMed]
- Pohanka, M. Three-dimensional printing in analytical chemistry: Principles and applications. Anal. Lett. 2016, 49, 2865–2882. [Google Scholar] [CrossRef]
- Ahn, D.-G. Directed energy deposition (DED) process: State of the art. Int. J. Precis. Eng. Manuf. Technol. 2021, 8, 703–742. [Google Scholar] [CrossRef]
- Bagheri, A.; Jin, J. Photopolymerization in 3D Printing. ACS Appl. Polym. Mater. 2019, 1, 593–611. [Google Scholar] [CrossRef] [Green Version]
- Prolongo, S.G.; Moriche, R.; Jiménez-Suárez, A.; Delgado, A.; Ureña, A. Printable self-heating coatings based on the use of carbon nanoreinforcements. Polym. Compos. 2020, 41, 271–278. [Google Scholar] [CrossRef]
- Mostafaei, A.; Elliott, A.M.; Barnes, J.E.; Li, F.; Tan, W.; Cramer, C.L.; Nandwana, P.; Chmielus, M. Binder jet 3D printing—Process parameters, materials, properties, modeling, and challenges. Prog. Mater. Sci. 2021, 119, 100707. [Google Scholar] [CrossRef]
- Xu, Y.; Wu, X.; Guo, X.; Kong, B.; Zhang, M.; Qian, X.; Mi, S.; Sun, W.; Lindquist, N.; Wittenberg, N.; et al. The boom in 3D-printed sensor technology. Sensors 2017, 17, 1166. [Google Scholar] [CrossRef]
- Dudek, P. FDM 3D printing technology in manufacturing composite elements. Arch. Metall. Mater. 2013, 58, 10–13. [Google Scholar] [CrossRef]
- Melnikova, R.; Ehrmann, A.; Finsterbusch, K. 3D printing of textile-based structures by Fused Deposition Modelling (FDM) with different polymer materials. IOP Conf. Ser. Mater. Sci. Eng. 2014, 62, 12018. [Google Scholar] [CrossRef] [Green Version]
- Espalin, D.; Muse, D.W.; MacDonald, E.; Wicker, R.B. 3D Printing multifunctionality: Structures with electronics. Int. J. Adv. Manuf. Technol. 2014, 72, 963–978. [Google Scholar] [CrossRef]
- MacDonald, E.; Wicker, R. Multiprocess 3D printing for increasing component functionality. Science 2016, 353, aaf2093. [Google Scholar] [CrossRef]
- Kesner, S.B.; Howe, R.D. Design principles for rapid prototyping forces sensors using 3-D printing. IEEE/ASME Trans. Mechatron. 2011, 16, 866–870. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Muth, J.T.; Vogt, D.M.; Truby, R.L.; Mengüç, Y.; Kolesky, D.B.; Wood, R.J.; Lewis, J.A. Embedded 3D printing of strain sensors within highly stretchable elastomers. Adv. Mater. 2014, 26, 6307–6312. [Google Scholar] [CrossRef]
- Igrec, B.; Bosiljevac, M.; Sipus, Z.; Babic, D.; Rudan, S. Fiber optic vibration sensor for high-power electric machines realized using 3D printing technology. In Proceedings of the Photonic Instrumentation Engineering III, San Francisco, CA, USA, 17–18 February 2016; Volume 9754. [Google Scholar] [CrossRef]
- Haque, R.I.; Ogam, E.; Loussert, C.; Benaben, P.; Boddaert, X. Fabrication of capacitive acoustic resonators combining 3D printing and 2D inkjet printing techniques. Sensors 2015, 15, 26018–26038. [Google Scholar] [CrossRef] [Green Version]
- Saari, M.; Xia, B.; Cox, B.; Krueger, P.S.; Cohen, A.L.; Richer, E. Fabrication and analysis of a composite 3D Printed capacitive force sensor. 3D Print. Addit. Manuf. 2016, 3, 137–141. [Google Scholar] [CrossRef]
- Suaste-Gómez, E.; Rodríguez-Roldán, G.; Reyes-Cruz, H.; Terán-Jiménez, O. Developing an ear prosthesis fabricated in polyvinylidene fluoride by a 3D printer with sensory intrinsic properties of pressure and temperature. Sensors 2016, 16, 332. [Google Scholar] [CrossRef]
- Krachunov, S.; Casson, A.J. 3D Printed Dry EEG Electrodes. Sensors 2016, 16, 1635. [Google Scholar] [CrossRef]
- Hamzah, H.H.; Keattch, O.; Yeoman, M.S.; Covill, D.; Patel, B.A. Three-dimensional-printed electrochemical sensor for simultaneous dual monitoring of serotonin overflow and circular muscle contraction. Anal. Chem. 2019, 91, 12014–12020. [Google Scholar] [CrossRef]
- Barbosa, J.R.; Amorim, P.H.O.; Mariana, M.C.; Dornellas, R.M.; Pereira, R.P.; Semaan, F.S. Evaluation of 3D printing parameters on the electrochemical performance of conductive polymeric components for chemical warfare agent sensing. In Smart Innovation, Systems and Technologies; Rocha, Á., Pereira, R., Eds.; Springer: Singapore, 2020. [Google Scholar] [CrossRef]
- Kennedy, Z.C.; Christ, J.F.; Evans, K.A.; Arey, B.W.; Sweet, L.E.; Warner, M.G.; Erikson, R.L.; Barrett, C.A. 3D-printed poly(vinylidene fluoride)/carbon nanotube composites as a tunable, low-cost chemical vapour sensing platform. Nanoscale 2017, 9, 5458–5466. [Google Scholar] [CrossRef]
- Leigh, S.J.; Bradley, R.J.; Purssell, C.P.; Billson, D.R.; Hutchins, D.A. A simple, low-cost conductive composite material for 3D printing of electronic sensors. PLoS ONE 2012, 7, e49365. [Google Scholar] [CrossRef] [PubMed]
- Kim, K.; Park, J.; Suh, J.H.; Kim, M.; Jeong, Y.; Park, I. 3D printing of multiaxial force sensors using carbon nanotube (CNT)/thermoplastic polyurethane (TPU) filaments. Sens. Actuators A Phys. 2017, 263, 493–500. [Google Scholar] [CrossRef]
- Christ, J.F.; Aliheidari, N.; Ameli, A.; Pötschke, P. 3D printed highly elastic strain sensors of multiwalled carbon nanotube/thermoplastic polyurethane nanocomposites. Mater. Des. 2017, 131, 394–401. [Google Scholar] [CrossRef]
- Gul, J.Z.; Sajid, M.; Choi, K.H. 3D printed highly flexible strain sensor based on TPU-graphene composite for feedback from high speed robotic applications. J. Mater. Chem. C 2019, 8, 2597. [Google Scholar] [CrossRef]
- Azimi, P.; Zhao, D.; Pouzet, C.; Crain, N.E.; Stephens, B. Emissions of ultrafine particles and volatile organic compounds from commercially available desktop three-dimensional printers with multiple filaments. Environ. Sci. Technol. 2016, 50, 1260–1268. [Google Scholar] [CrossRef]
- Sathies, T.; Senthil, P.; Prakash, C. Application of 3D printed PLA-carbon black conductive polymer composite in solvent sensing. Mater. Res. Express 2019, 6, 115349. [Google Scholar] [CrossRef]
- Thangavel, S.; Ponnusamy, S. Application of 3D printed polymer composite as capacitive sensor. Sens. Rev. 2019, 40, 54–61. [Google Scholar] [CrossRef]
- Cardoso, R.M.; Silva, P.R.L.; Lima, A.P.; Rocha, D.P.; Oliveira, T.C.; do Prado, T.M.; Fava, E.L.; Fatibello-Filho, O.; Richter, E.M.; Muñoz, R.A.A. 3D-Printed graphene/polylactic acid electrode for bioanalysis: Biosensing of glucose and simultaneous determination of uric acid and nitrite in biological fluids. Sens. Actuators B Chem. 2020, 307, 127621. [Google Scholar] [CrossRef]
- Kalinke, C.; Neumsteir, N.V.; de Oliveira Aparecido, G.; de Barros Ferraz, T.V.; Dos Santos, P.L.; Janegitz, B.C.; Bonacin, J.A. Comparison of activation processes for 3D printed PLA-graphene electrodes: Electrochemical properties and application for sensing of dopamine. Analyst 2020, 145, 1207–1218. [Google Scholar] [CrossRef]
- Foster, C.W.; Elbardisy, H.M.; Down, M.P.; Keefe, E.M.; Smith, G.C.; Banks, C.E. Additively manufactured graphitic electrochemical sensing platforms. Chem. Eng. J. 2020, 381, 122343. [Google Scholar] [CrossRef]
- Hashemi Sanatgar, R.; Campagne, C.; Nierstrasz, V. Investigation of the adhesion properties of direct 3D printing of polymers and nanocomposites on textiles: Effect of FDM printing process parameters. Appl. Surf. Sci. 2017, 403, 551–563. [Google Scholar] [CrossRef]
- Chen, S.; Zhuo, B.; Guo, X. Large area one-step facile processing of microstructured elastomeric dielectric film for high sensitivity and durable sensing over wide pressure range. ACS Appl. Mater. Interfaces 2016, 8, 20364–20370. [Google Scholar] [CrossRef] [PubMed]
- Trung, T.Q.; Lee, N.E. Flexible and stretchable physical sensor integrated platforms for wearable human-activity monitoringand personal healthcare. Adv. Mater. 2016, 28, 4338–4372. [Google Scholar] [CrossRef]
- Tessarolo, M.; Possanzini, L.; Campari, E.G.; Bonfiglioli, R.; Violante, F.S.; Bonfiglio, A.; Fraboni, B. Adaptable pressure textile sensors based on a conductive polymer. Flex. Print. Electron. 2018, 3, 034001. [Google Scholar] [CrossRef]
- Mannsfeld, S.C.B.; Tee, B.C.-K.; Stoltenberg, R.M.; Chen, C.V.H.-H.; Barman, S.; Muir, B.V.O.; Sokolov, A.N.; Reese, C.; Bao, Z. Highly sensitive flexible pressure sensors with microstructured rubber dielectric layers. Nat. Mater. 2010, 9, 859–864. [Google Scholar] [CrossRef]
- Dios, J.R.; Garcia-Astrain, C.; Gonçalves, S.; Costa, P.; Lanceros-Méndez, S. Piezoresistive performance of polymer-based materials as a function of the matrix and nanofiller content to walking detection application. Compos. Sci. Technol. 2019, 181, 107678. [Google Scholar] [CrossRef]
- Tung, T.T.; Robert, C.; Castro, M.; Feller, J.F.; Kim, T.Y.; Suh, K.S. Enhancing the sensitivity of graphene/polyurethane nanocomposite flexible piezo-resistive pressure sensors with magnetite nano-spacers. Carbon 2016, 108, 450–460. [Google Scholar] [CrossRef]
- Toprakci, H.A.K. Piezoresistive Properties of Polyvinyl Chloride Composites. Ph.D. Thesis, North Carolina State University, Raleigh, NC, USA, 2012. [Google Scholar]
- So, H.; Woo, J.; Kwon, J.; Yun, J.; Baik, S.; Seok, W. Carbon nanotube based pressure sensor for flexible electronics. Mater. Res. Bull. 2013, 48, 5036–5039. [Google Scholar] [CrossRef]
- Slobodian, P.; Riha, P.; Lengalova, A.; Saha, P. Compressive stress-electrical conductivity characteristics of multiwall carbon nanotube networks. J. Mater. Sci 2011, 46, 3186–3190. [Google Scholar] [CrossRef] [Green Version]
- Sanatgar, R.H.; Cayla, A.; Campagne, C.; Nierstrasz, V. Morphological and electrical characterization of conductive polylactic acid based nanocomposite before and after FDM 3D printing. J. Appl. Polym. Sci. 2019, 136, 47040. [Google Scholar] [CrossRef]
- Gnanasekaran, K.; Heijmans, T.; van Bennekom, S.; Woldhuis, H.; Wijnia, S.; de With, G.; Friedrich, H. 3D printing of CNT- and graphene-based conductive polymer nanocomposites by fused deposition modeling. Appl. Mater. Today 2017, 9, 21–28. [Google Scholar] [CrossRef]
- Paredes-Madrid, L.; Matute, A.; Bareño, J.O.; Vargas, C.A.P.; Velásquez, E.I.G. Underlying physics of conductive polymer composites and Force Sensing Resistors (FSRs). A study on creep response and dynamic loading. Materials 2017, 10, 1334. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Slobodian, P.; Saha, P. Stress-strain hysteresis of a carbon nanotube network as polymer nanocomposite filler under cyclic deformation. AIP Conf. Proc. 2011, 231, 224–231. [Google Scholar] [CrossRef] [Green Version]
- Hussein, M. Effects of strain rate and temperature on the mechanical behavior of carbon black reinforced elastomers based on butyl rubber and high molecular weight polyethylene. Results Phys. 2018, 9, 511–517. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hashemi Sanatgar, R.; Cayla, A.; Guan, J.; Chen, G.; Nierstrasz, V.; Campagne, C. Piezoresistive Properties of 3D-Printed Polylactic Acid (PLA) Nanocomposites. Polymers 2022, 14, 2981. https://doi.org/10.3390/polym14152981
Hashemi Sanatgar R, Cayla A, Guan J, Chen G, Nierstrasz V, Campagne C. Piezoresistive Properties of 3D-Printed Polylactic Acid (PLA) Nanocomposites. Polymers. 2022; 14(15):2981. https://doi.org/10.3390/polym14152981
Chicago/Turabian StyleHashemi Sanatgar, Razieh, Aurélie Cayla, Jinping Guan, Guoqiang Chen, Vincent Nierstrasz, and Christine Campagne. 2022. "Piezoresistive Properties of 3D-Printed Polylactic Acid (PLA) Nanocomposites" Polymers 14, no. 15: 2981. https://doi.org/10.3390/polym14152981
APA StyleHashemi Sanatgar, R., Cayla, A., Guan, J., Chen, G., Nierstrasz, V., & Campagne, C. (2022). Piezoresistive Properties of 3D-Printed Polylactic Acid (PLA) Nanocomposites. Polymers, 14(15), 2981. https://doi.org/10.3390/polym14152981






