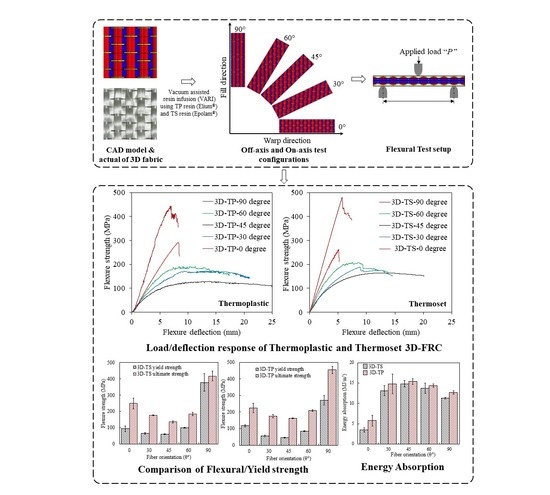
Off-Axis and On-Axis Performance of Novel Acrylic Thermoplastic (Elium®) 3D Fibre-Reinforced Composites under Flexure Load
Abstract
:1. Introduction
1.1. Flexure Response of FRC
2. Materials and Methods
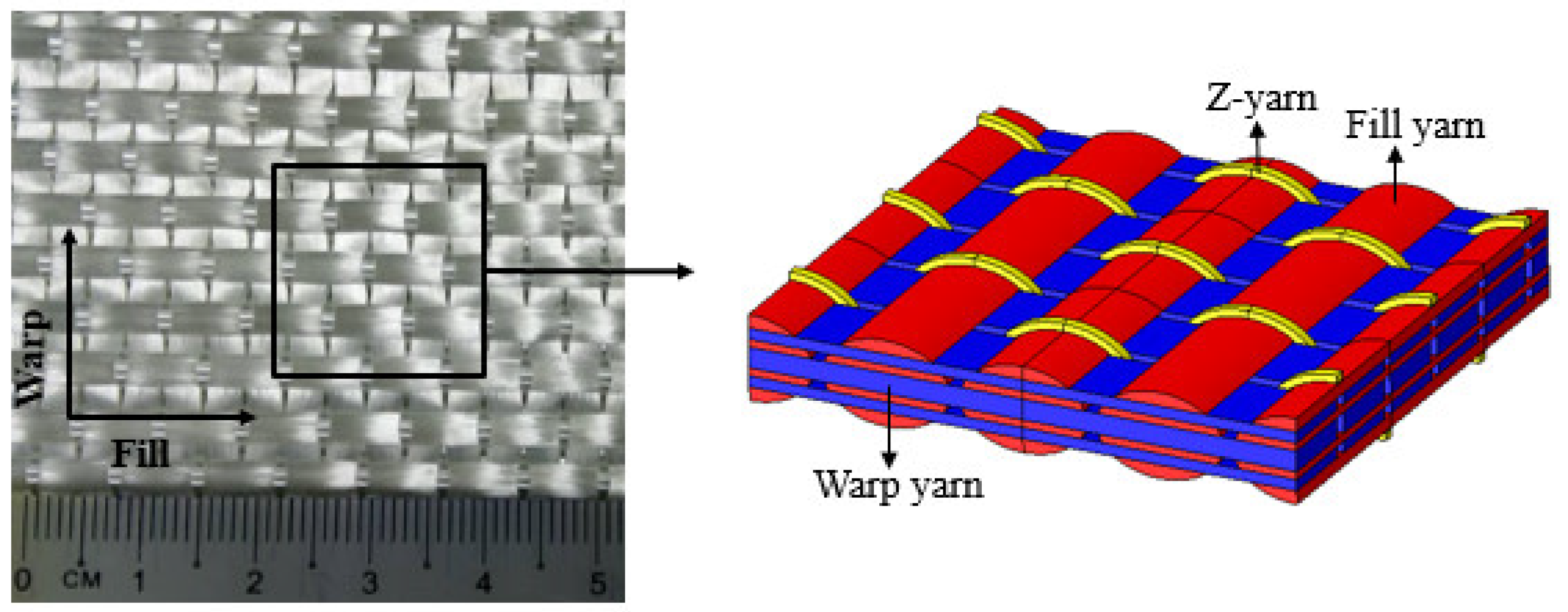
2.1. Material Used
2.2. Fabrication Process
2.3. Physical Parameters of the Cured Panels and Samples
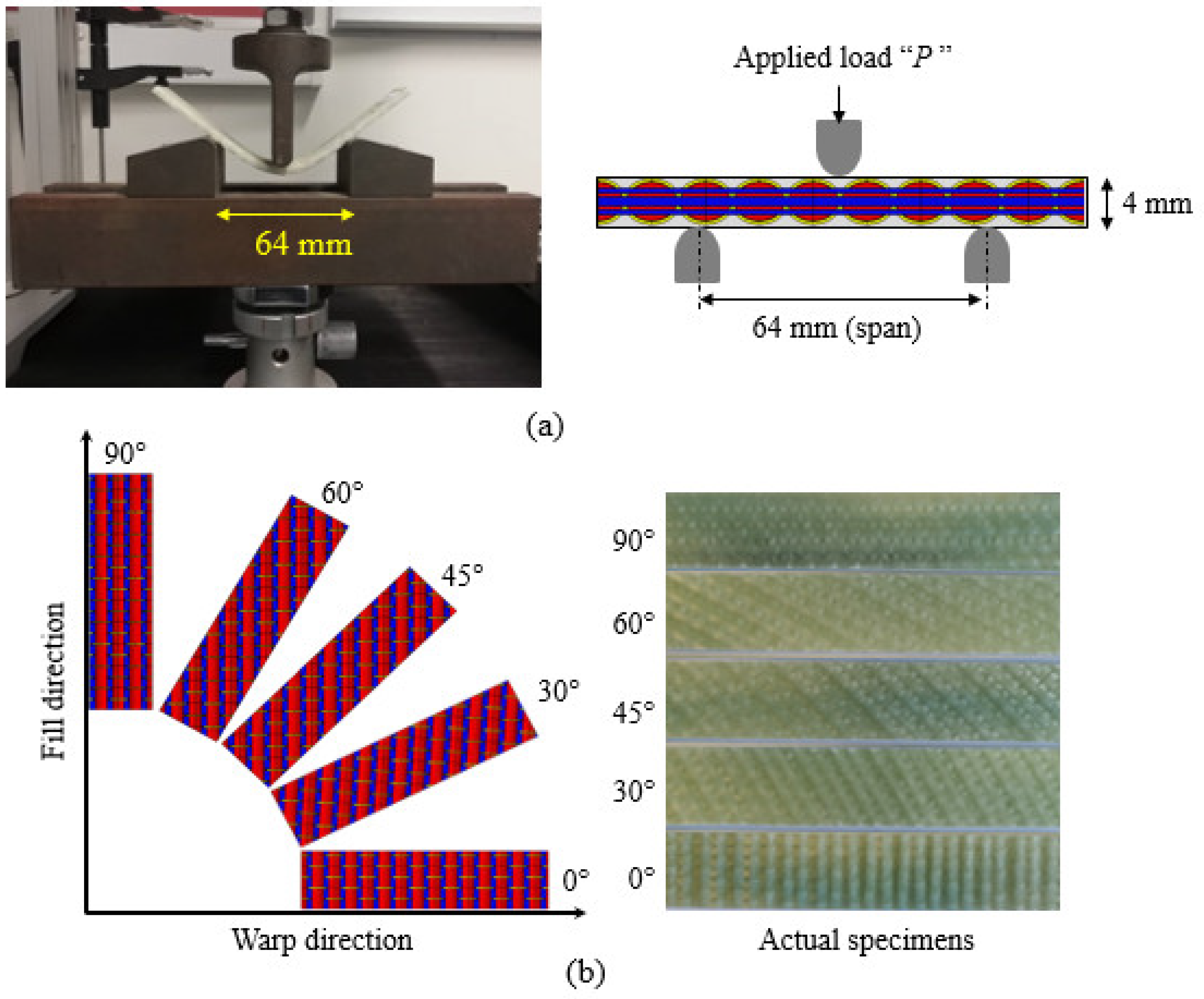
2.4. Flexural Testing
2.5. Damage Evaluation Method
3. Results and Discussion
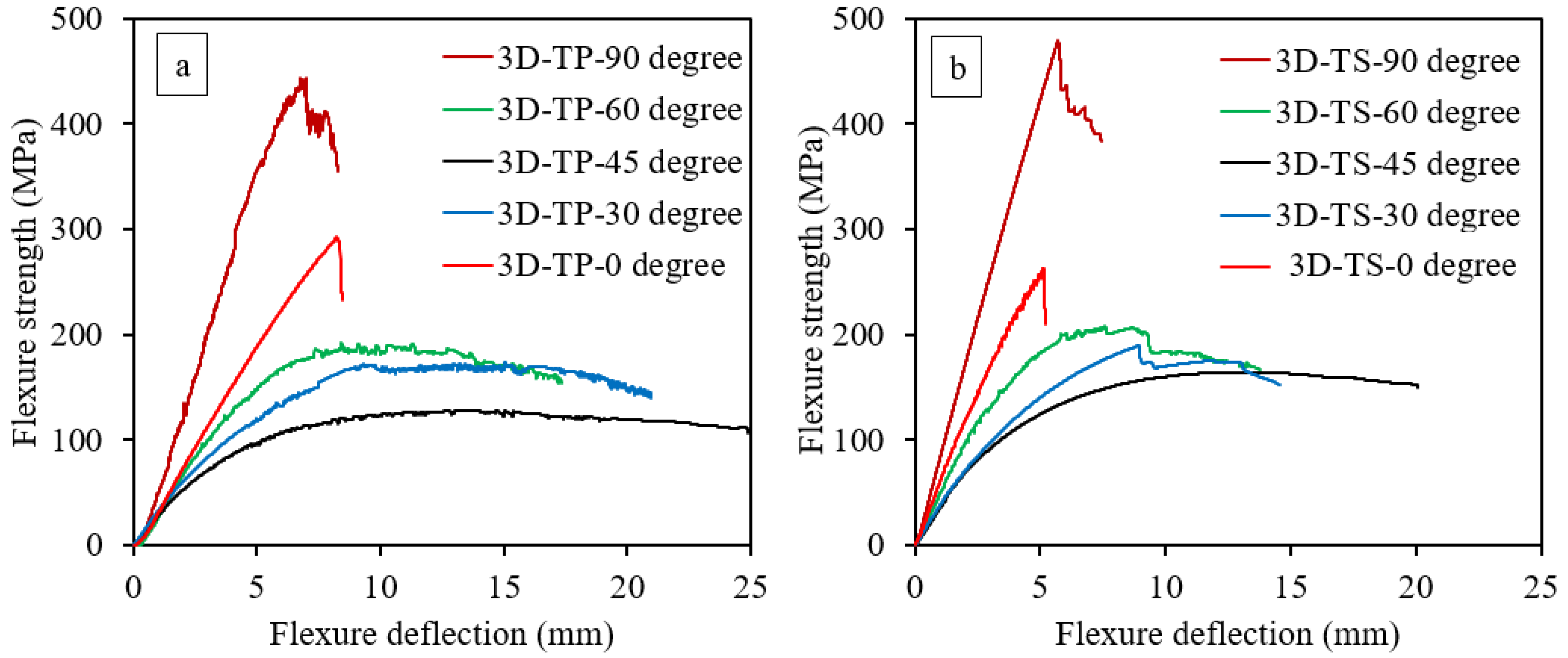
3.1. Comparison of the Stress-Displacement Curve
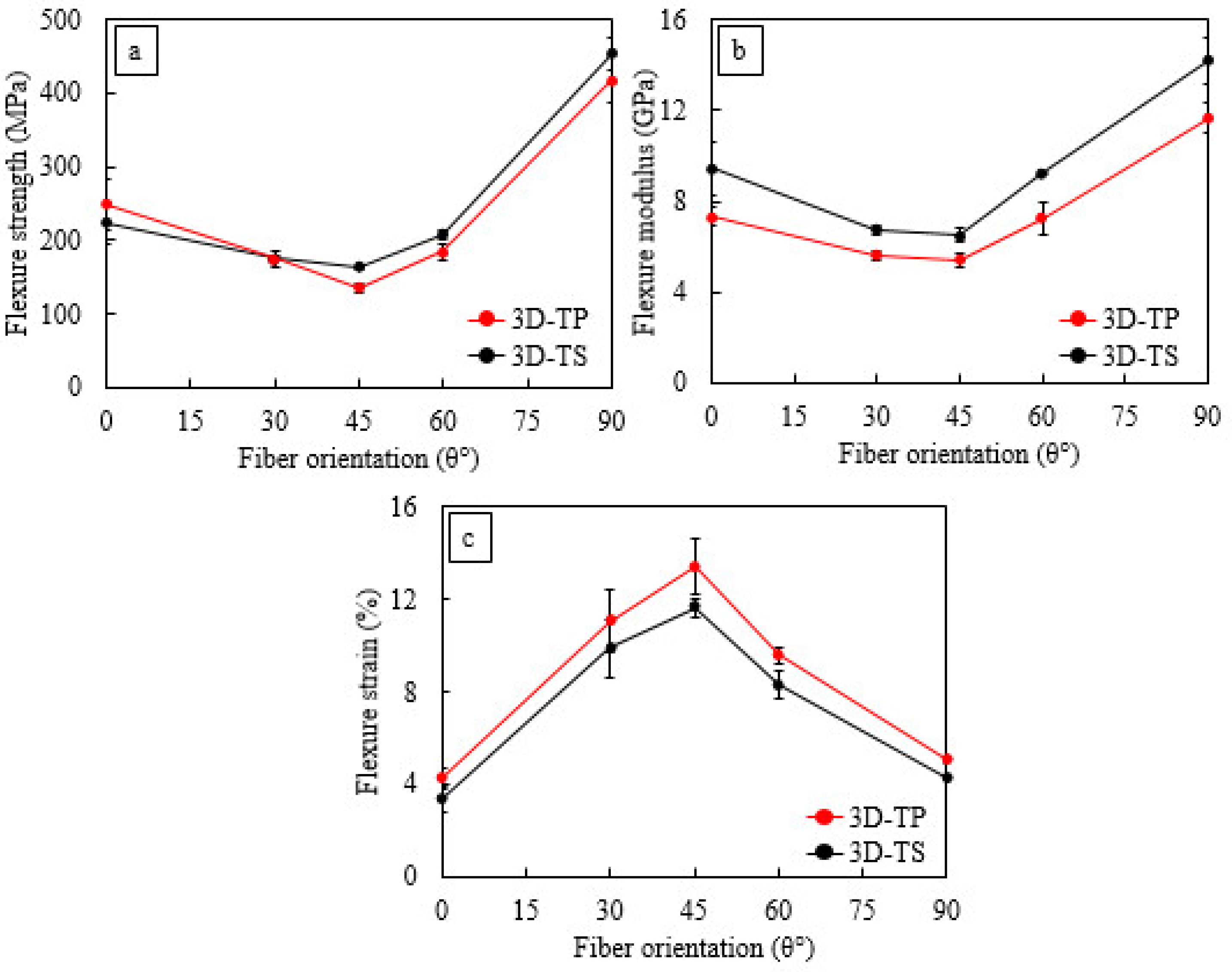
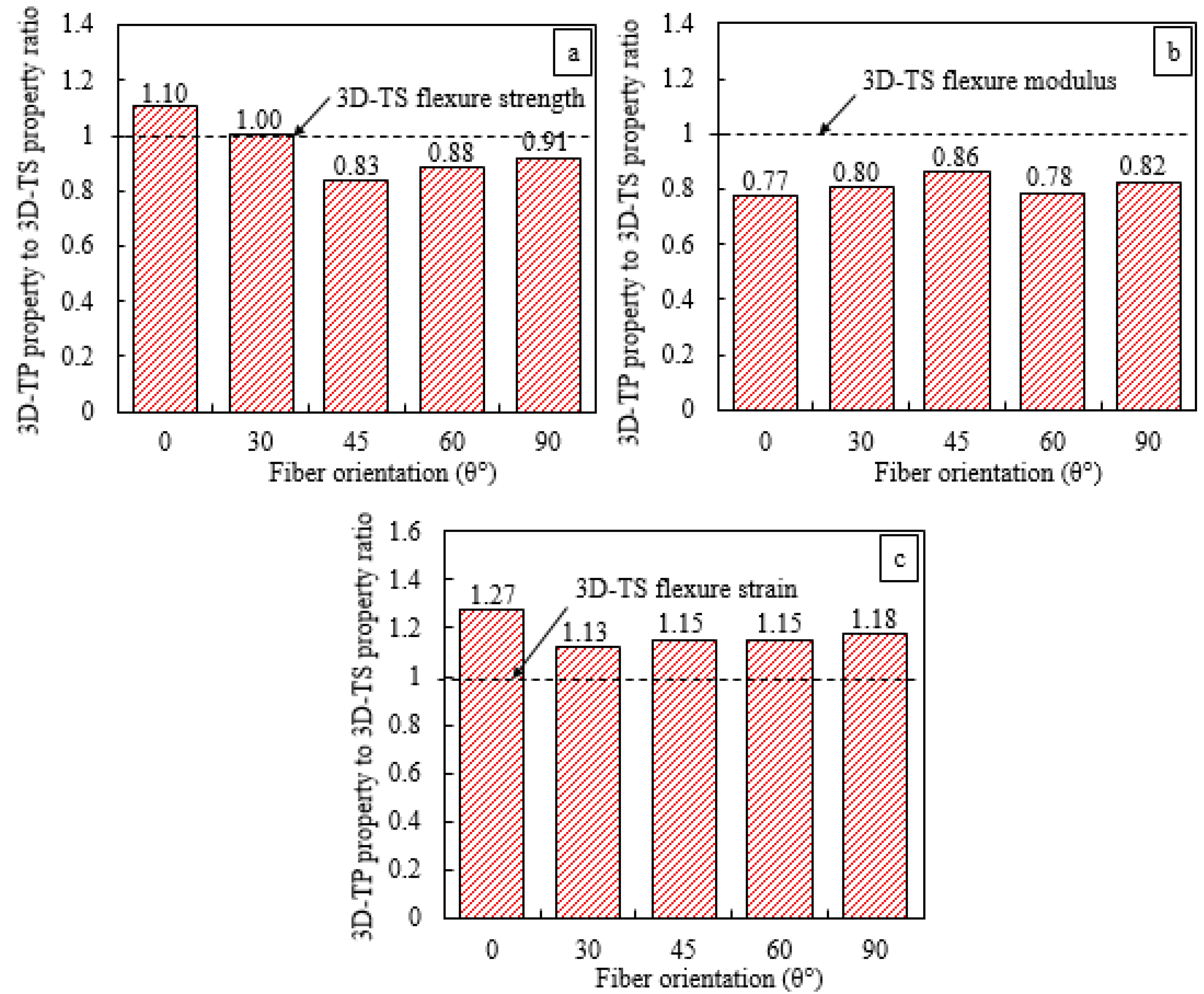
3.2. Comparison of Flexure Strength, Modulus and Failure Strain
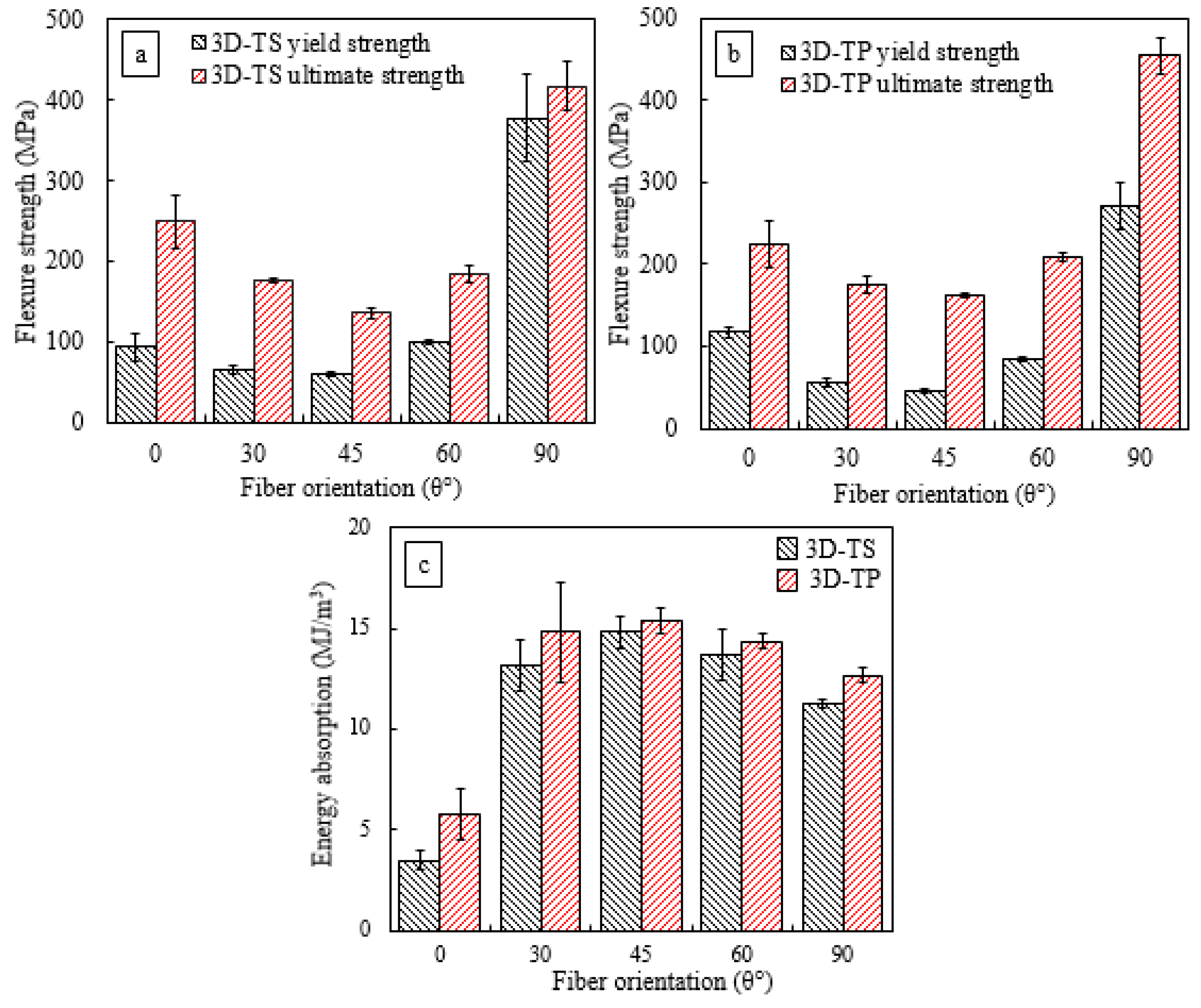
3.3. Flexural vs. Yield Strength of 3D-FRC
3.4. Effect of Resin Toughness on Energy Absorption
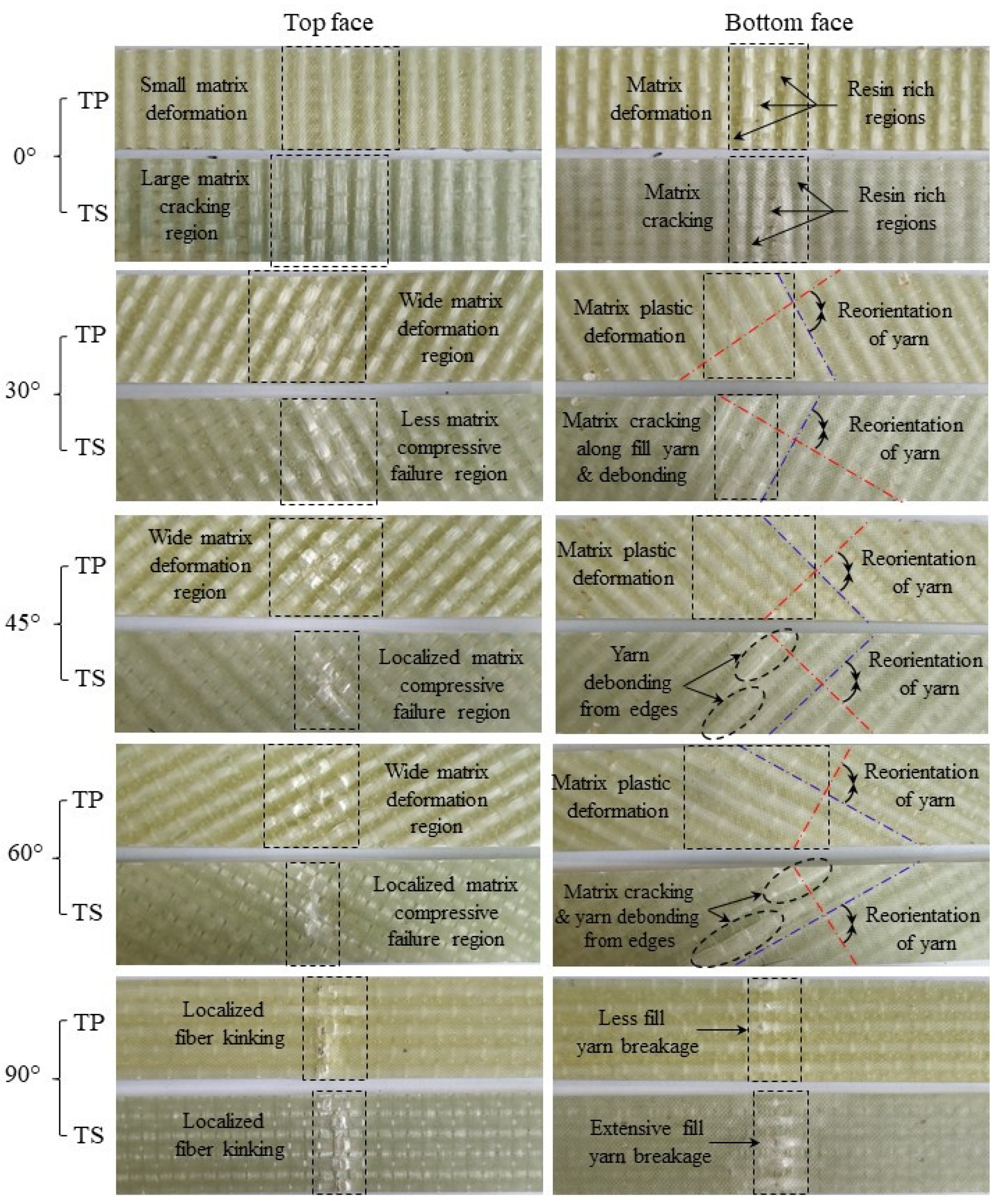
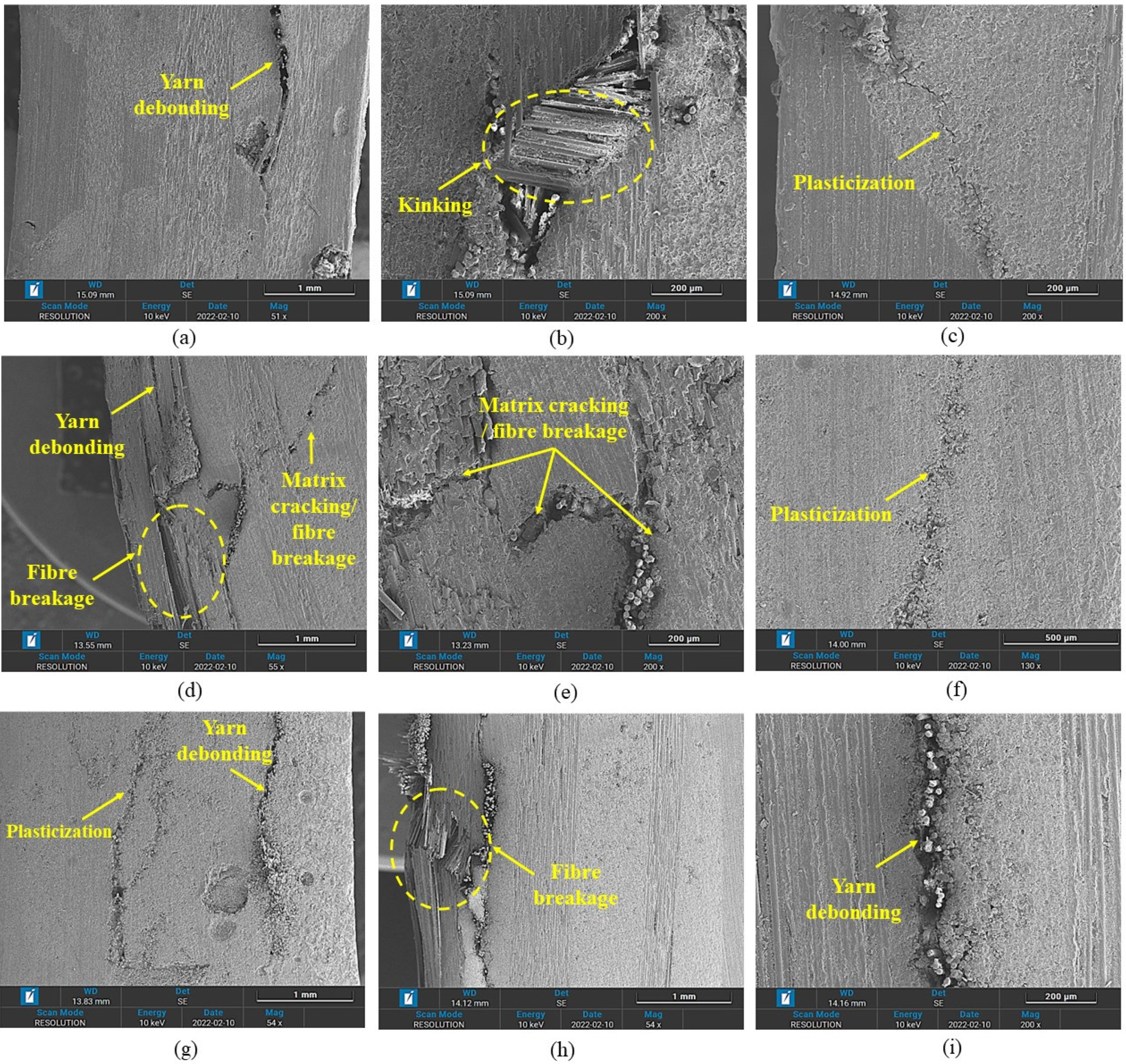
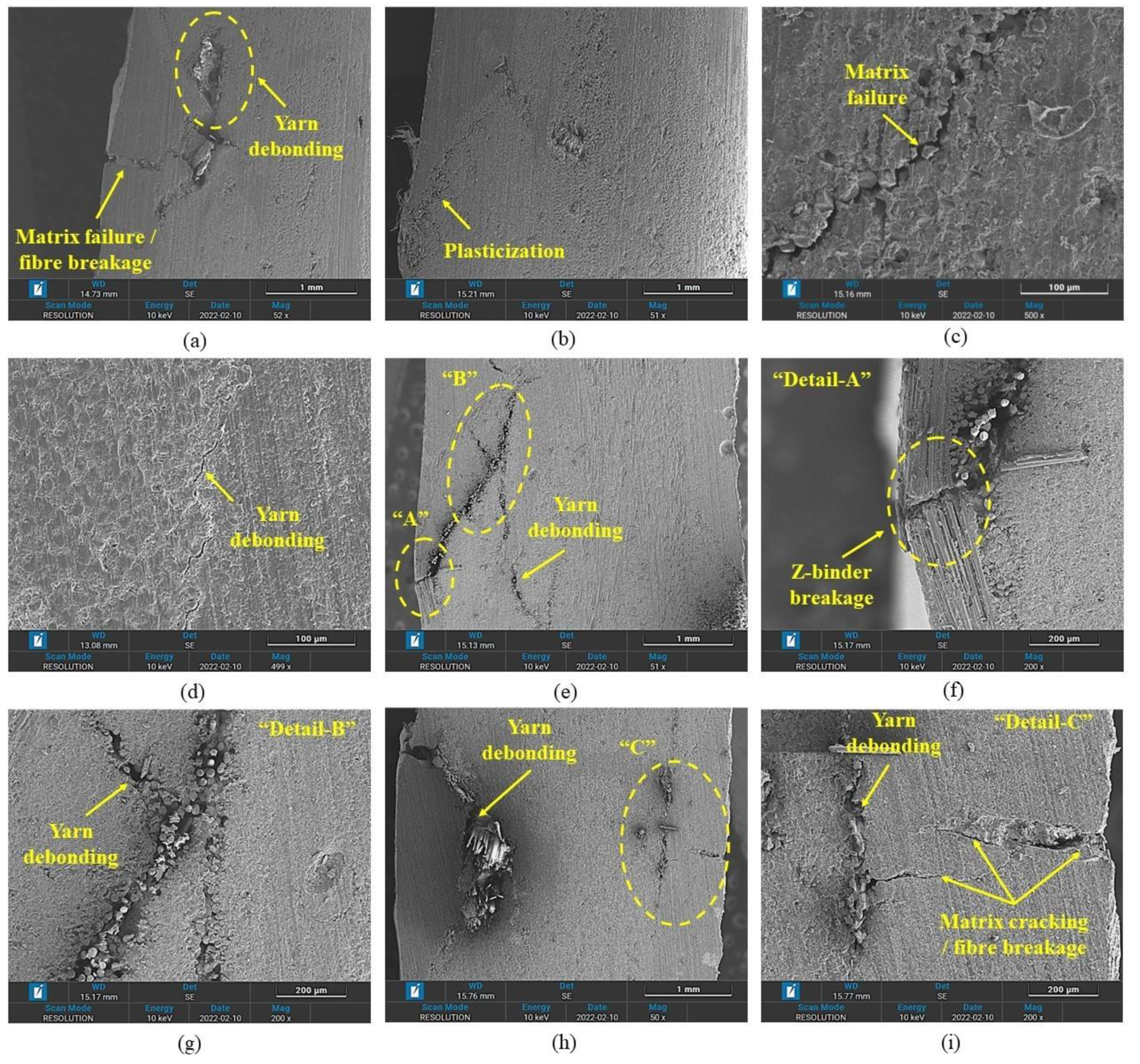
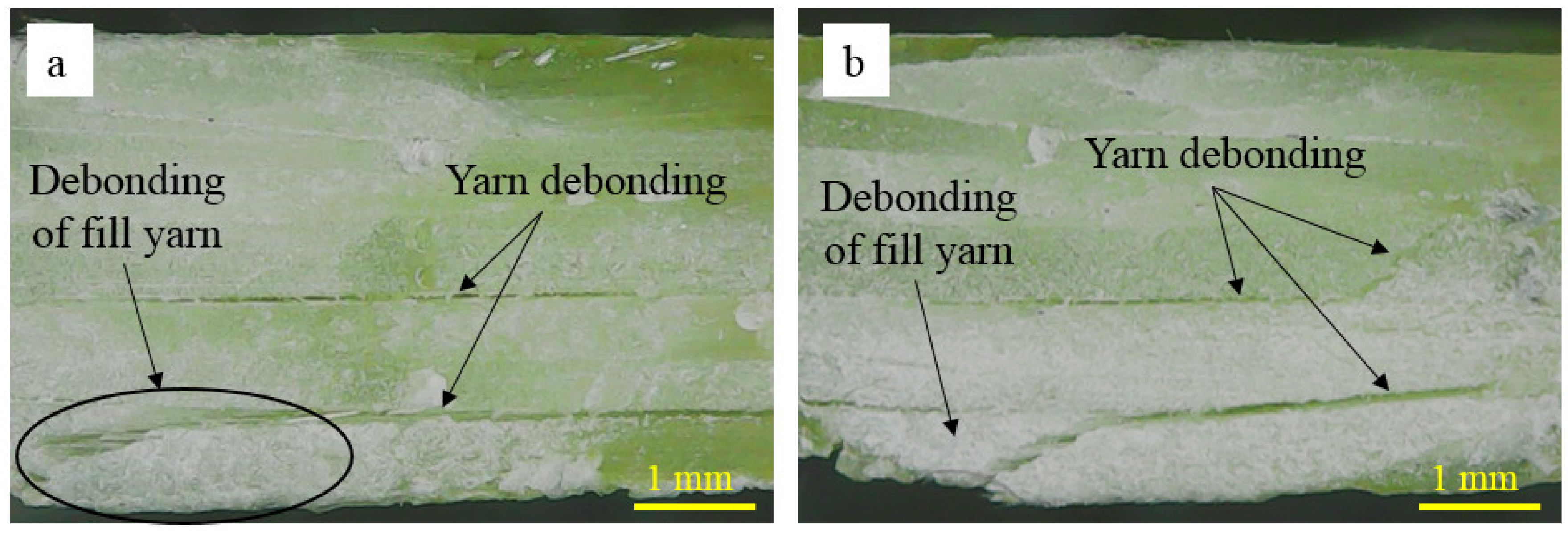
3.5. Failure Mechanisms in TP and TS 3D Composites under Flexure Load
3.5.1. Intralaminar Failure Mechanisms
3.5.2. Interlaminar Failure Mechanisms
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shah, S.Z.H.; Choudhry, R.S.; Khan, L.A. Challenges in compression testing of 3D angle-interlocked woven-glass fabric-reinforced polymeric composites. ASTM J. Test. Eval. 2017, 5, 1502–1523. [Google Scholar] [CrossRef] [Green Version]
- Shah, S.Z.H.; Karuppanan, S.; Megat-Yusoff, P.; Sajid, Z. Impact resistance and damage tolerance of fiber reinforced composites: A review. Composite 2019, 217, 100–121. [Google Scholar] [CrossRef]
- Sajid, Z.; Karuppanan, S.; Sallih, N.; Kee, K.E.; Shah, S.Z.H. Role of washer size in mitigating adverse effects of bolt-hole clearance in a single-lap, single-bolt basalt composite joint. Compos. Struct. 2021, 266, 113802. [Google Scholar] [CrossRef]
- Ud Din, I.; Tu, S.; Hao, P.; Panier, S.; Khan, K.A.; Umer, R.; Shah, S.Z.H.; Franz, G.; Aamir, M. Sequential damage study induced in fiber reinforced composites by shear and tensile stress using a newly developed Arcan fixture. J. Mater. Res. Technol. 2020, 9, 13352–13364. [Google Scholar] [CrossRef]
- Sajid, Z.; Karuppanan, S.; Shah, S.Z.H. Open-Hole and Filled-Hole Failure Envelopes of BFRP and GFRP: A Comparative Study. In Advances in Manufacturing Engineering. Lecture Notes in Mechanical Engineering; Emamian, S., Awang, M., Yusof, F., Eds.; Springer: Singapore, 2020. [Google Scholar] [CrossRef]
- Shah, S.Z.H.; Megat-Yusoff, P.S.M.; Karuppanan, S.; Choudhry, R.S.; Ahmad, F.; Sajid, Z. Mechanical Properties and Failure Mechanisms of Novel Resin-infused Thermoplastic and Conventional Thermoset 3D Fabric Composites. Appl. Compos. Mater. 2021, 29, 515–545. [Google Scholar] [CrossRef]
- Cox, B.N.; Dadkhah, M.S.; Morris, W. On the tensile failure of 3D woven composites. Compos. Part A Appl. Sci. Manuf. 1996, 27, 447–458. [Google Scholar] [CrossRef]
- Kuo, W.S.; Tse-Hao, K. Compressive damage in 3-axis orthogonal fabric composites. Compos. Part A Appl. Sci. Manuf. 2000, 31, 1091–1105. [Google Scholar] [CrossRef]
- Shah, S.; Lee, J. Stochastic lightning damage prediction of carbon/epoxy composites with material uncertainties. Compos. Struct. 2022, 282, 115014. [Google Scholar] [CrossRef]
- Stig, F.; Hallström, S. Assessment of the mechanical properties of a new 3D woven fibre composite material. Compos. Sci. Technol. 2009, 69, 1686–1692. [Google Scholar] [CrossRef] [Green Version]
- Ladani, R.B.; Pingkarawat, K.; Nguyen, A.T.; Wang, C.H.; Mouritz, A.P. Delamination toughening and healing performance of woven composites with hybrid z-fibre reinforcement. Compos. Part A Appl. Sci. Manuf. 2018, 110, 258–267. [Google Scholar] [CrossRef]
- Shah, S.Z.H.; Megat Yusoff, P.S.M.; Karuppanan, S.; Sajid, Z. Elastic Constants Prediction of 3D Fiber-Reinforced Composites Using Multiscale Homogenization. Processes 2020, 8, 722. [Google Scholar] [CrossRef]
- Zhang, D.; Sun, M.; Liu, X.; Xiao, X.; Qian, K. Off-axis bending behaviors and failure characterization of 3D woven composites. Compos. Struct. 2019, 208, 45–55. [Google Scholar] [CrossRef]
- Zaghloul, M.M.Y.; Zaghloul, M.M.Y. Influence of flame retardant magnesium hydroxide on the mechanical properties of high density polyethylene composites. J. Reinf. Plast. Compos. 2017, 36, 1802–1816. [Google Scholar] [CrossRef]
- Shah, S.Z.H.; Choudhry, R.S.; Mahadzir, S. A new approach for strength and stiffness prediction of discontinuous fibre reinforced composites (DFC). Compos. Part B Eng. 2020, 183, 107676. [Google Scholar] [CrossRef]
- Obande, W.; Mamalis, D.; Ray, D.; Yang, L.; Bradaigh, C.M.O. Mechanical and thermomechanical characterisation of vacuum-infused thermoplastic and thermoset-based composites. Mater. Des. 2019, 175, 107828. [Google Scholar] [CrossRef]
- Luo, H.; Xiong, G.; Yang, Z.; Raman, S.R.; Li, Q.; Ma, C.; Li, D.; Wang, Z.; Wan, Y. Preparation of three-dimensional braided carbon fiber-reinforced PEEK composites for potential load-bearing bone fixations. Part I. Mechanical properties and cytocompatibility. J. Mech. Behav. Biomed. Mater. 2014, 29, 103–113. [Google Scholar] [CrossRef]
- Kuo, W.S.; Jiunn, F. Processing and characterization of 3D woven and braided thermoplastic composites. Compos. Sci. Technol. 2000, 60, 643–656. [Google Scholar] [CrossRef]
- Sajid, Z.; Karuppanan, S.; Kee, K.E.; Sallih, N.; Shah, S.Z.H. Carbon/basalt hybrid composite bolted joint for improved bearing performance and cost efficiency. Compos. Struct. 2021, 275, 114427. [Google Scholar] [CrossRef]
- Sajid, Z.; Karuppanan, S.; Shah, S.Z.H. Effect of Washer Size and Tightening Torque on Bearing Performance of Basalt Fiber Composite Bolted Joints. J. Nat. Fibers 2021, 1–18. [Google Scholar] [CrossRef]
- Zaghloul, M.Y.M.; Zaghloul, M.M.Y.; Zaghloul, M.M.Y. Developments in polyester composite materials–An in-depth review on natural fibres and nano fillers. Compos. Struct. 2021, 278, 114698. [Google Scholar] [CrossRef]
- Kuo, W.-S.; Ko, T.-H.; Cheng, K.-B.; Hsieh, K.-Y. Flexural behavior of three-axis woven carbon/carbon composites. J. Mater. Sci. 2001, 36, 2743–2752. [Google Scholar] [CrossRef]
- Umer, R.; Alhussein, H.; Zhou, J.; Cantwell, W.J. The mechanical properties of 3D woven composites. J. Compos. Mater. 2016, 51, 1703–1716. [Google Scholar] [CrossRef] [Green Version]
- Dai, S.; Cunningham, P.; Marshall, S.; Silva, C. Influence of fibre architecture on the tensile, compressive and flexural behaviour of 3D woven composites. Compos. Part A Appl. Sci. Manuf. 2015, 69, 195–207. [Google Scholar] [CrossRef] [Green Version]
- Jin, L.; Hu, H.; Sun, B.; Gu, B. Three point bending fatigue behavior of 3D angle interlock woven composite. J. Compos. Mater. 2012, 46, 883–894. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, D. Effect of Fabric Structures on the Mechanical Properties of 3-D Textile Composites. J. Ind. Text. 2006, 35, 239–256. [Google Scholar] [CrossRef]
- Adanur, S.; Tam, C.A. On-machine interlocking of 3D laminate structures for composites. Compos. Part B Eng. 1997, 28, 497–506. [Google Scholar] [CrossRef]
- Zhang, Q.; Fang, X.; Sun, X.; Sun, B.; Qiu, Y. Comparison of the Mechanical Properties between 2D and 3D Orthogonal Woven Ramie Fiber Reinforced Polypropylene Composites. Polym. Polym. Compos. 2014, 22, 187–192. [Google Scholar] [CrossRef]
- Archer, E.; Mulligan, R.; Dixon, D.; Buchanan, S.; Stewart, G.; McIlhagger, A.T. An investigation into thermoplastic matrix 3D woven carbon fibre composites. J. Reinf. Plast. Compos. 2012, 31, 863–873. [Google Scholar] [CrossRef]
- Bhudolia, S.K.; Gohel, G.; Kah Fai, L.; Barsotti, R.J. Fatigue response of ultrasonically welded carbon/Elium® thermoplastic composites. Mater. Lett. 2020, 264, 127362. [Google Scholar] [CrossRef]
- Shah, S.Z.H.; Megat-Yusoff, P.; Karuppanan, S.; Choudhry, R.; Ahmad, F.; Sajid, Z.; Gerard, P.; Sharp, K. Performance comparison of resin-infused thermoplastic and thermoset 3D fabric composites under impact loading. Int. J. Mech. Sci. 2020, 189, 105984. [Google Scholar] [CrossRef]
- Shah, S.Z.H.; Megat-Yusoff, P.; Karuppanan, S.; Choudhry, R.; Ud Din, I.; Othman, A.; Gerard, P.; Sharp, K. Compression and buckling after impact response of resin-infused thermoplastic and thermoset 3D woven composites. Compos. Part B Eng. 2021, 207, 108592. [Google Scholar] [CrossRef]
- Shah, S.Z.H.; Megat-Yusoff, P.S.M.; Choudhry, R.S.; Sajid, Z.; Din, I.U. Experimental investigation on the quasi-static crush performance of resin-infused thermoplastic 3D fibre-reinforced composites. Compos. Commun. 2021, 28, 100916. [Google Scholar] [CrossRef]
- Murray, R.E.; Penumadu, D.; Cousins, D.; Beach, R.; Snowberg, D.; Berry, D.; Suzuki, Y.; Stebner, A. Manufacturing and Flexural Characterization of Infusion-Reacted Thermoplastic Wind Turbine Blade Subcomponents. Appl. Compos. Mater. 2019, 26, 945–961. [Google Scholar] [CrossRef]
- Zhang, D.; Waas, A.M.; Yen, C.-F. Progressive damage and failure response of hybrid 3D textile composites subjected to flexural loading, part I: Experimental studies. Int. J. Solids Struct. 2015, 75–76, 309–320. [Google Scholar] [CrossRef]
- Bhudolia, S.K.; Perrotey, P.; Joshi, S.C. Optimizing polymer infusion process for thin ply textile composites with novel matrix system. Materials 2017, 10, 293. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kazemi, M.E.; Shanmugam, L.; Lu, D.; Wang, X.; Wang, B.; Yang, J. Mechanical properties and failure modes of hybrid fiber reinforced polymer composites with a novel liquid thermoplastic resin, Elium®. Compos. Part A Appl. Sci. Manuf. 2019, 125, 105523. [Google Scholar] [CrossRef]
- Zhang, H.; Yang, J. Development of self-healing polymers via amine–epoxy chemistry: II. Systematic evaluation of self-healing performance. Smart Mater. Struct. 2014, 23, 065004. [Google Scholar] [CrossRef]
- Shah, S.Z.H.; Megat-Yusoff, P.; Karuppanan, S.; Choudhry, R.; Sajid, Z. Multiscale damage modelling of 3D woven composites under static and impact loads. Compos. Part A Appl. Sci. Manuf. 2021, 151, 106659. [Google Scholar] [CrossRef]
- ASTM D7264/D7264M-07; Standard Test Method for Flexural Properties of Polymer Matrix Composite Materials. ASTM International: West Conshohocken, PA, USA, 2007. Available online: www.astm.org (accessed on 20 April 2022).
- Turner, P.; Liu, T.; Zeng, X. Collapse of 3D orthogonal woven carbon fibre composites under in-plane tension/compression and out-of-plane bending. Compos. Struct. 2016, 142, 286–297. [Google Scholar] [CrossRef]
- Hufner, D.R.; Accorsi, M.L. A progressive failure theory for woven polymer-based composites subjected to dynamic loading. Compos. Struct. 2009, 89, 177–185. [Google Scholar] [CrossRef]
- Bhudolia, S.K.; Perrotey, P.; Joshi, S.C. Mode I fracture toughness and fractographic investigation of carbon fibre composites with liquid Methylmethacrylate thermoplastic matrix. Compos. Part B Eng. 2018, 134, 246–253. [Google Scholar] [CrossRef]

| Property | Elium® 188x0 | Epolam® 5015/5015 |
|---|---|---|
| Tensile strength (MPa) a | 76 | 80 |
| Tensile modulus (GPa) a | 3.3 | 3.1 |
| Elongation at failure (%) c | 6 | 3.1 |
| Flexural strength (MPa) a | 130 | 100 |
| Flexural modulus (GPa) a | 3.25 | 2.6 |
| Fracture toughness (kJ/m2) b | 0.5 | 0.12 |
| Rockwell Hardness d | 99 | 119 |
| Density (g/cc) d | 1.17 | 1.15 |
| Material | Fibre Orientation (Degree) | Flexure Modulus (GPa) | Flexure Yield Strength (MPa) | Flexure Strength (MPa) | Flexure Strain (%) |
|---|---|---|---|---|---|
| 3D-TP-FRC | 0° | 7.3 (6.1%) | 117 (14.5%) | 249 (15%) | 4.3 (12%) |
| 30° | 5.6 (4.7%) | 55 (9.0%) | 176 (1.9%) | 11 (12%) | |
| 45° | 5.4 (6.6%) | 45 (4.5%) | 136 (6.0%) | 13.4 (9.2%) | |
| 60° | 7.2 (11%) | 86 (2.5%) | 184 (6.5%) | 9.6 (3.7%) | |
| 90° | 11.6 (5.7%) | 272 (20%) | 418 (8.0%) | 5.0 (3.0%) | |
| 3D-TS-FRC | 0° | 9.4 (14%) | 94 (6.5%) | 225 (14%) | 3.4 (17%) |
| 30° | 6.7 (3.0%) | 65 (8.0%) | 176 (6.4%) | 9.9 (14%) | |
| 45° | 6.5 (5.0%) | 61 (5.0%) | 162 (1.3%) | 11.7 (3.5%) | |
| 60° | 9.2 (1.0%) | 101 (2.5%) | 208 (2.8%) | 8.3 (7.3%) | |
| 90° | 14.2 (8.2%) | 378 (8.0%) | 455 (4.9%) | 4.3 (2.3%) |
| Mat. | Damage Type | Damage Mechanisms | Case-1 (0º) | Case-2 (30º) | Case-3 (45º) | Case-4 (60º) | Case-5 (90º) |
|---|---|---|---|---|---|---|---|
| 3D-TP-FRC | Intralaminar | Fibre breakage | Moderate | None | None | None | Moderate |
| Plasticization | Some | Moderate | Significant | Moderate | Some | ||
| Matrix cracking | None | None | Some | None | None | ||
| Kinking | Some | None | None | None | Some | ||
| Interlaminar | Yarn debonding | Some | None | None | Moderate | Moderate | |
| Yarn re-orientation | None | Moderate | Significant | Moderate | None | ||
| Matrix deformation | Some | Moderate | Significant | Moderate | Some | ||
| 3D-TS-FRC | Intralaminar | Fibre breakage | Moderate | None | None | None | Significant |
| Plasticization | None | None | None | None | None | ||
| Matrix cracking | Moderate | Significant | Significant | Significant | Moderate | ||
| Kinking | Some | None | None | None | Some | ||
| Interlaminar | Yarn debonding | Moderate | Moderate | Moderate | Significant | Some | |
| Yarn reorientation | None | Moderate | Significant | Moderate | None | ||
| Matrix comp. failure | Some | Moderate | Significant | Moderate | Some |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shah, S.Z.H.; Megat-Yusoff, P.S.M.; Karuppanan, S.; Choudhry, R.S.; Sajid, Z. Off-Axis and On-Axis Performance of Novel Acrylic Thermoplastic (Elium®) 3D Fibre-Reinforced Composites under Flexure Load. Polymers 2022, 14, 2225. https://doi.org/10.3390/polym14112225
Shah SZH, Megat-Yusoff PSM, Karuppanan S, Choudhry RS, Sajid Z. Off-Axis and On-Axis Performance of Novel Acrylic Thermoplastic (Elium®) 3D Fibre-Reinforced Composites under Flexure Load. Polymers. 2022; 14(11):2225. https://doi.org/10.3390/polym14112225
Chicago/Turabian StyleShah, Syed Zulfiqar Hussain, Puteri S. M. Megat-Yusoff, Saravanan Karuppanan, Rizwan Saeed Choudhry, and Zubair Sajid. 2022. "Off-Axis and On-Axis Performance of Novel Acrylic Thermoplastic (Elium®) 3D Fibre-Reinforced Composites under Flexure Load" Polymers 14, no. 11: 2225. https://doi.org/10.3390/polym14112225
APA StyleShah, S. Z. H., Megat-Yusoff, P. S. M., Karuppanan, S., Choudhry, R. S., & Sajid, Z. (2022). Off-Axis and On-Axis Performance of Novel Acrylic Thermoplastic (Elium®) 3D Fibre-Reinforced Composites under Flexure Load. Polymers, 14(11), 2225. https://doi.org/10.3390/polym14112225