Fabrication and Characterization of Polypyrrole/Multi-Walled Carbon Nanotubes Thin Films Using Thermal Evaporation
Abstract
:1. Introduction
2. Experimental
2.1. Raw Materials
2.2. Synthesis of Polypyrrole/Multi-Walled Carbon Nanotube (MWCNT) Composites
2.3. Fabrication of the Thin Films
2.4. Computational Study
2.5. Characterization
3. Results and Discussion
3.1. Fourier Transform Infrared (FT-IR) Spectroscopy
3.2. Surface Morphology
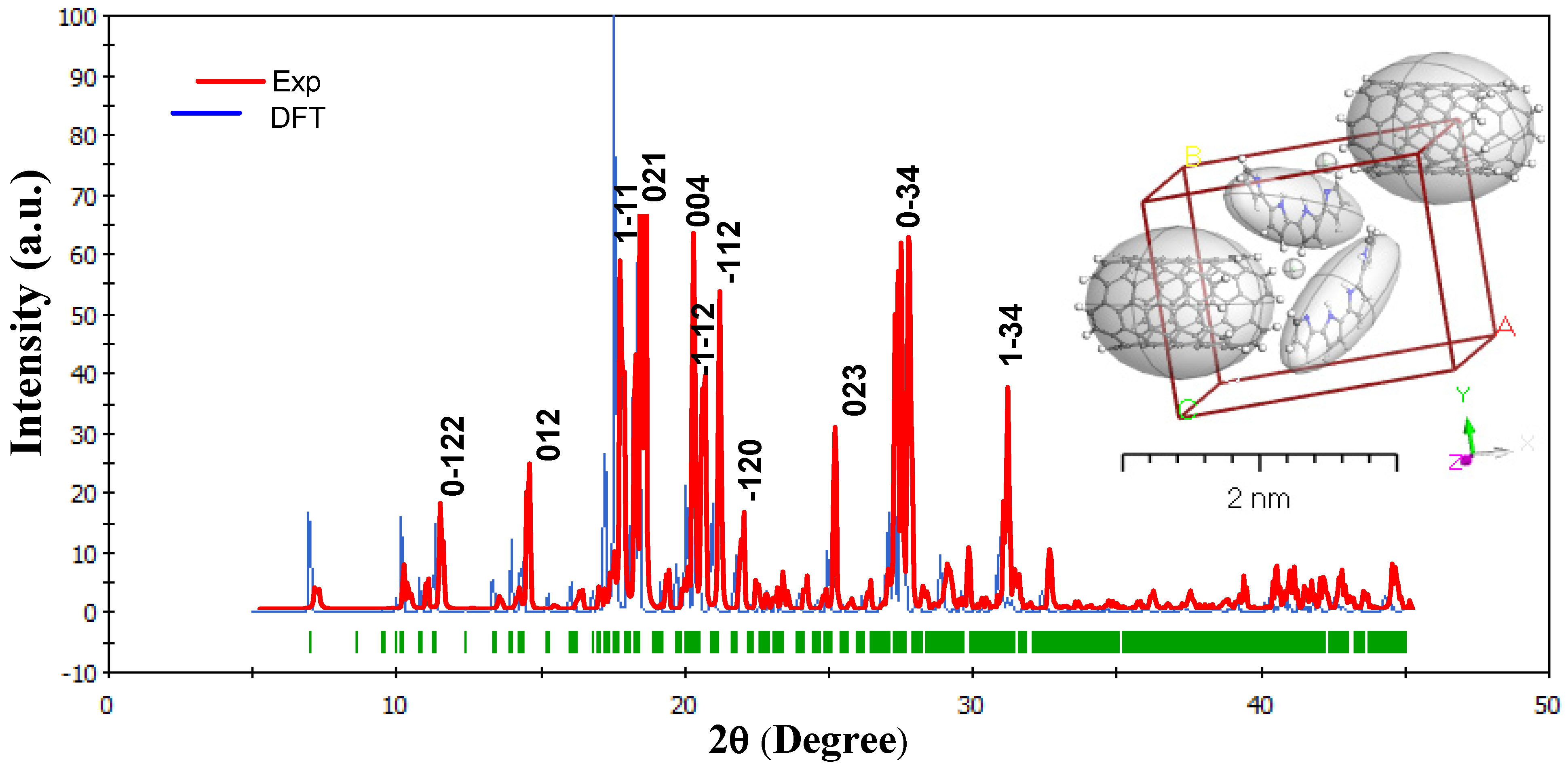
3.3. X-ray Diffraction (XRD) Analysis
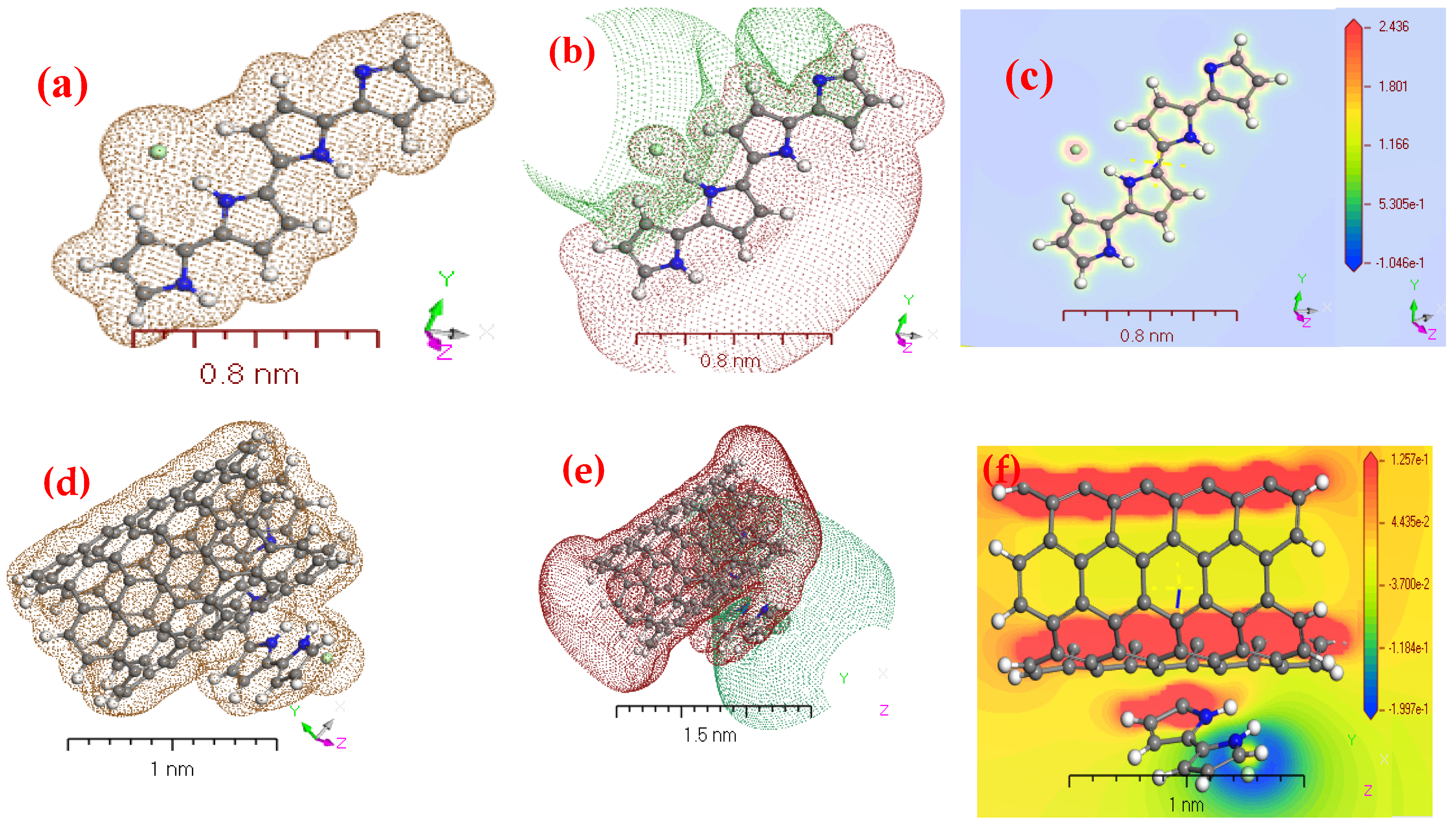
3.4. Geometric Study
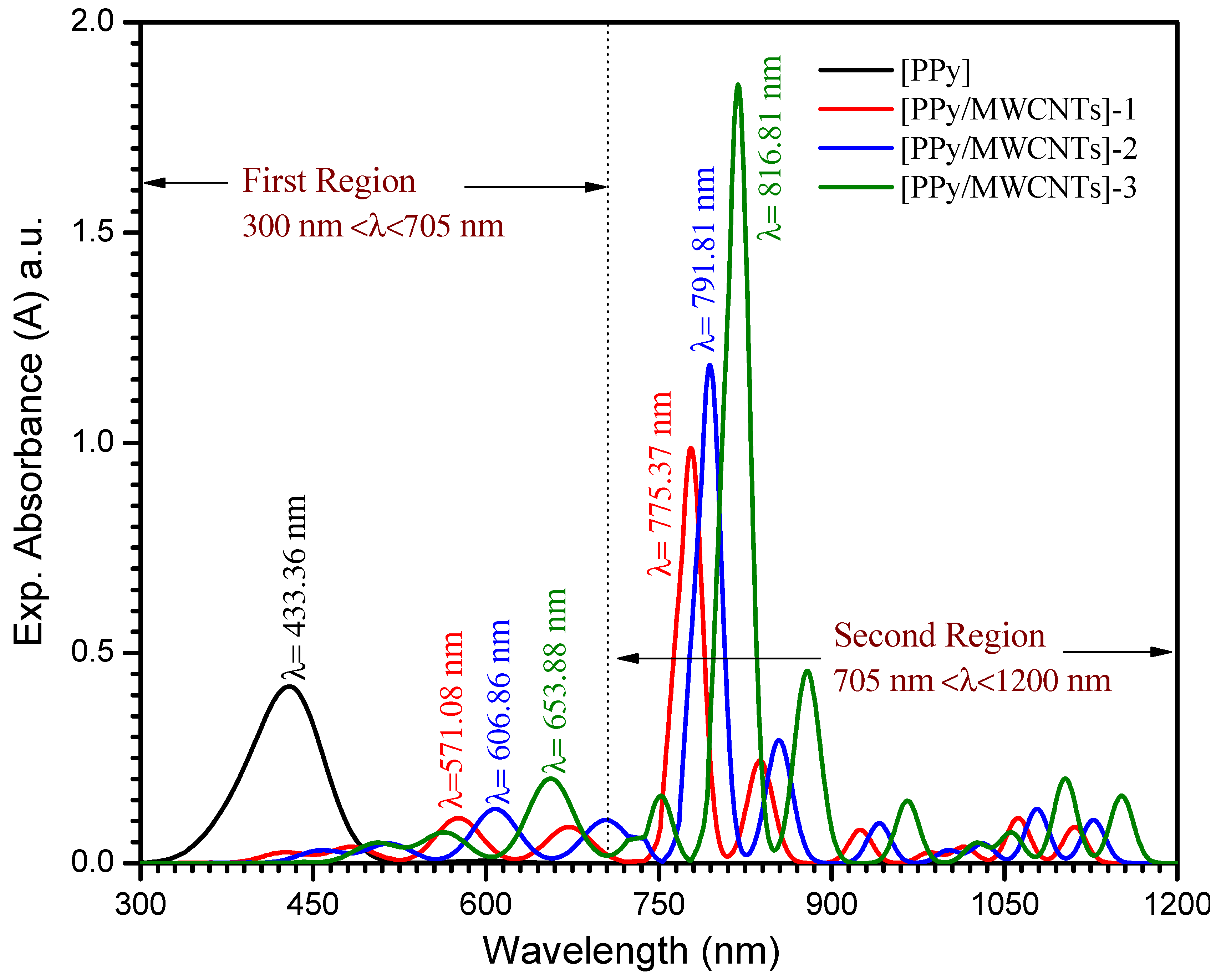
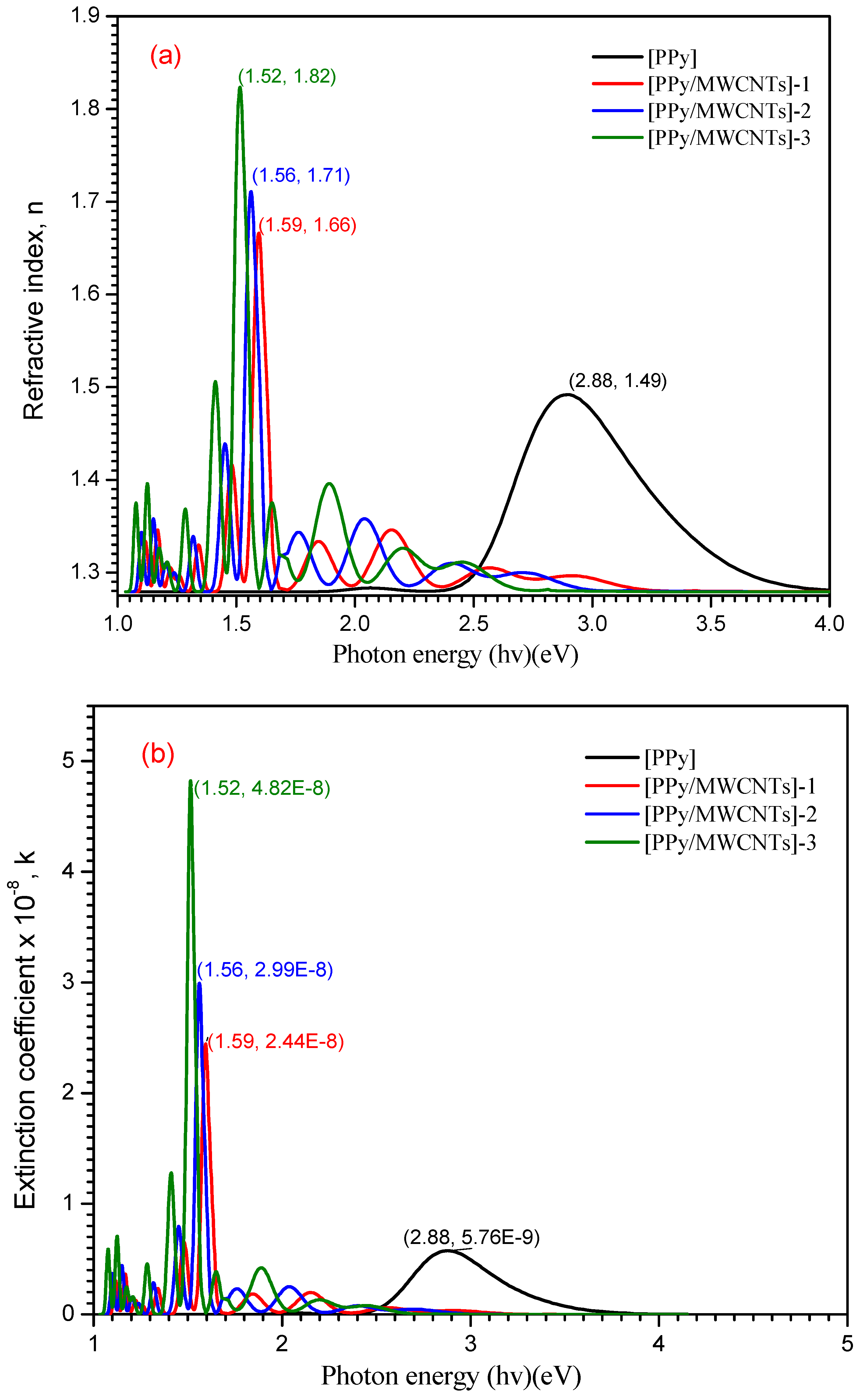
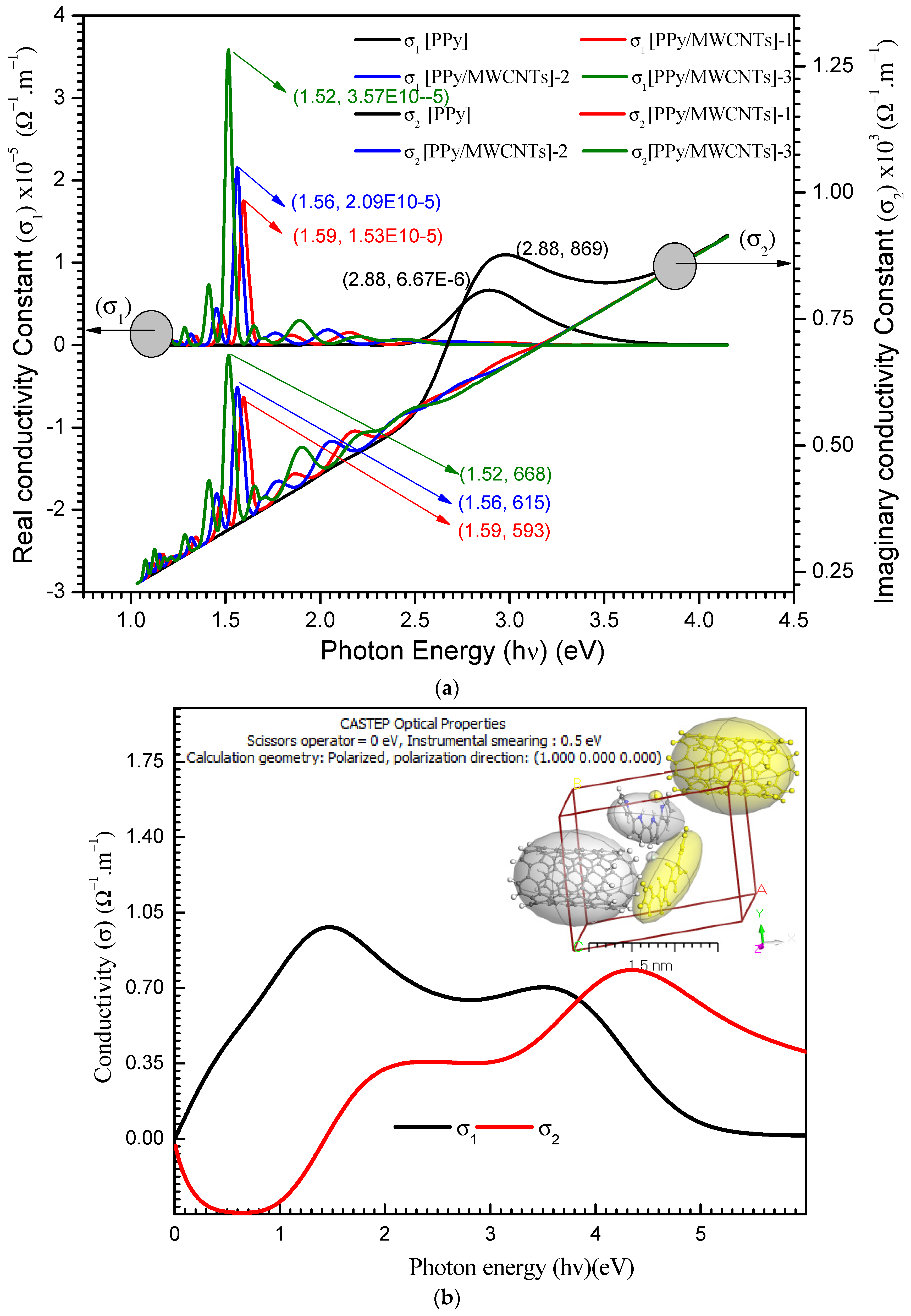
3.5. Optical Properties
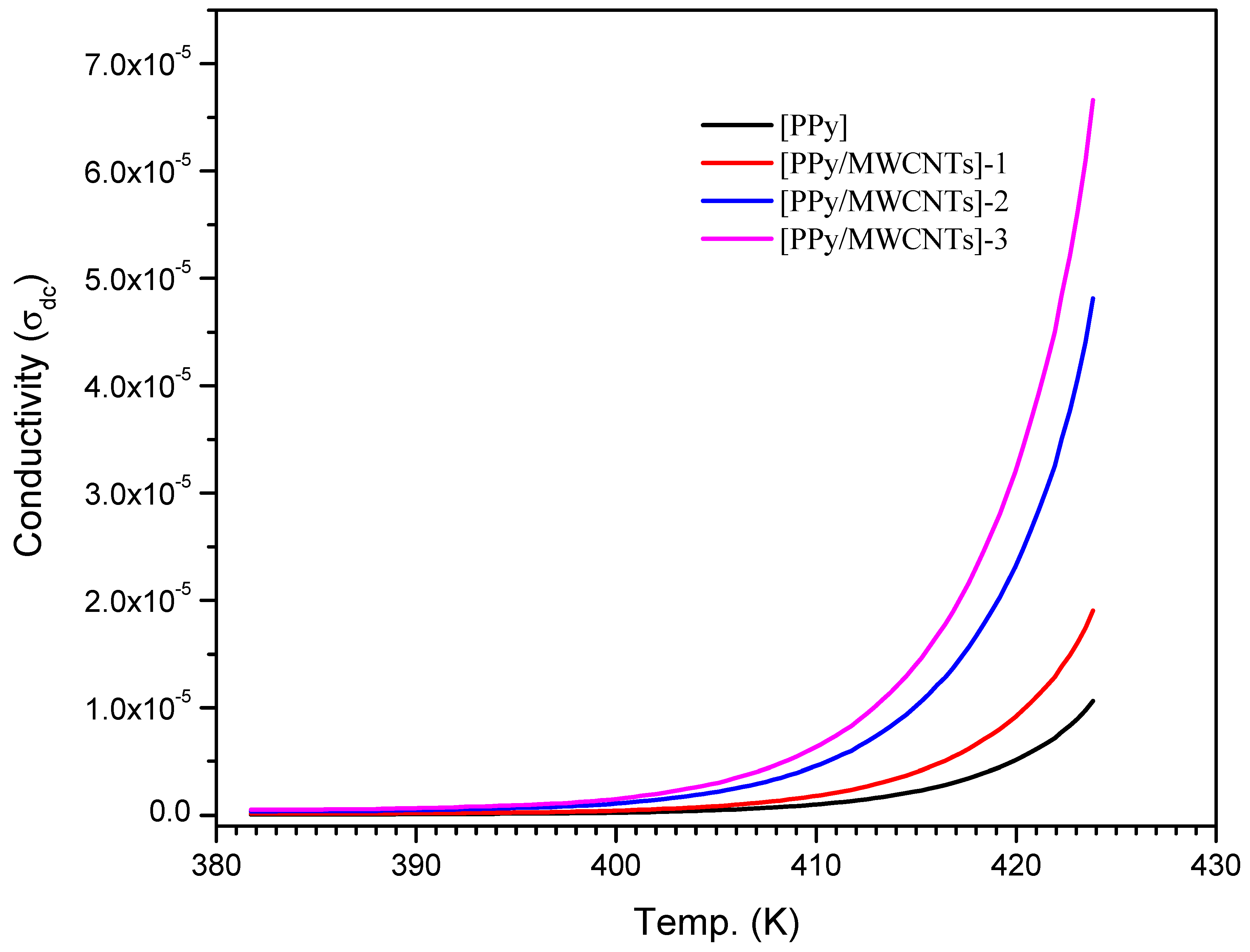
3.6. Electrical Properties
3.6.1. The Influence of Applied Potential Difference (V) on the Current (I)
3.6.2. The Effect of Multiwalled Carbon Nanotubes Composites (MWCNTs) on Direct Current (DC) Conductivity
3.6.3. Photovoltaic Properties of PPy/MWCNTs Composites Films
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Krishnaswamy, S.; Ragupathi, V.; Raman, S.; Panigrahi, P.; Nagarajan, G.S. Optical properties of P-type polypyrrole thin film synthesized by pulse laser deposition technique: Hole transport layer in electroluminescence devices. Optik 2019, 194, 163034. [Google Scholar] [CrossRef]
- Yadav, A.A.; Kulkarni, S.B.; Lokhande, C.D. Synthesis and characterization of polypyrrole thin film by MW-CBD method for NH 3 gas sensor. Polym. Bull. 2018, 75, 4547–4553. [Google Scholar] [CrossRef]
- Cho, J.H.; Yu, J.B.; Kim, J.S.; Sohn, S.O.; Lee, D.D.; Huh, J.S. Sensing behaviors of polypyrrole sensor under humidity condition. Sens. Actuators B Chem. 2005, 108, 389–392. [Google Scholar] [CrossRef]
- Jiang, L.; Jun, H.K.; Hoh, Y.S.; Lim, J.O.; Lee, D.D.; Huh, J.S. Sensing characteristics of polypyrrole–poly (vinyl alcohol) methanol sensors prepared by in situ vapor state polymerization. Sens. Actuators B Chem. 2005, 105, 132–137. [Google Scholar] [CrossRef]
- Vidal, J.C.; García, E.; Castillo, J.R. In situ preparation of a cholesterol biosensor: Entrapment of cholesterol oxidase in an overoxidized polypyrrole film electrodeposited in a flow system: Determination of total cholesterol in serum. Anal. Chim. Acta 1999, 385, 213–222. [Google Scholar] [CrossRef]
- Campbell, T.E.; Hodgson, A.J.; Wallace, G.G. Incorporation of erythrocytes into polypyrrole to form the basis of a biosensor to screen for rhesus (D) blood groups and rhesus (D) antibodies. Electroanal. Int. J. Devoted Fundam. Pract. Asp. Electroanal. 1999, 11, 215–222. [Google Scholar] [CrossRef]
- Kincal, D.; Kumar, A.; Child, A.D.; Reynolds, J.R. Conductivity switching in polypyrrole-coated textile fabrics as gas sensors. Synth. Met. 1998, 92, 53–56. [Google Scholar] [CrossRef]
- Kemp, N.T.; Fianagan, G.U.; Kaiser, A.B.; Trodahl, H.J.; Chapman, B.; Partridge, A.C.; Buckley, R.G. Temperature-dependent conductivity of conducting polymers exposed to gases. Synth. Met. 1999, 101, 434–435. [Google Scholar] [CrossRef]
- Smela, E. Microfabrication of PPy microactuators and other conjugated polymer devices. J. Micromech. Microeng. 1999, 9, 1. [Google Scholar] [CrossRef]
- Sandid, A.; Marni, M.; Bassyouni, D.N.; Elhenawy, Y. Experimental and simulation study of multichannel air gap membrane distillation process with two types of solar collectors. Energy Convers. Manag. 2021, 243, 114431. [Google Scholar] [CrossRef]
- MA, C.; SG, P.; PR, G.; Shashwati, S. Synthesis and characterization of polypyrrole (PPy) thin films. Soft Nanosci. Lett. 2011, 1, 3660. [Google Scholar]
- Baghdadi, N.; Zoromba, M.S.; Abdel-Aziz, M.H.; Al-Hossainy, A.F.; Bassyouni, M.; Salah, N. One-Dimensional Nanocomposites Based on Polypyrrole-Carbon Nanotubes and Their Thermoelectric Performance. Polymers 2021, 13, 278. [Google Scholar] [CrossRef] [PubMed]
- Iroh, J.O.; Williams, C. Formation of thermally stable polypyrrole-naphthalene/benzene sulfonate-carbon fiber composites by an electrochemical process. Synth. Met. 1999, 99, 1–8. [Google Scholar] [CrossRef]
- Jacobs, I.E.; Moulé, A.J. Controlling molecular doping in organic semiconductors. Adv. Mater. 2017, 29, 1703063. [Google Scholar] [CrossRef]
- Pulickel, M.A.; Tour, J.M. Materials science: Nanotube composites. Nature 2007, 447, 1066–1068. [Google Scholar]
- Endo, M.; Kim, Y.A.; Ezaka, M.; Osada, K.; Yanagisawa, T.; Hayashi, T.; Terrones, M.; Dresselhaus, M.S. Selective and efficient impregnation of metal nanoparticles on cup-stacked-type carbon nanofibers. Nano Lett. 2003, 3, 723–726. [Google Scholar] [CrossRef]
- De Heer, W.A.; Poncharal, P.; Berger, C.; Gezo, J.; Song, Z.; Bettini, J.; Ugarte, D. Liquid carbon, carbon-glass beads, and the crystallization of carbon nanotubes. Science 2005, 307, 907–910. [Google Scholar] [CrossRef]
- Algarni, M. The Influence of Raster Angle and Moisture Content on the Mechanical Properties of PLA Parts Produced by Fused Deposition Modeling. Polymers 2021, 13, 237. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Dai, J.; Yarlagadda, T. Carbon nanotube-conducting-polymer composite nanowires. Langmuir 2005, 21, 9–12. [Google Scholar] [CrossRef]
- Sanghvi, A.B.; Miller, K.P.H.; Belcher, A.M.; Schmidt, C.E. Biomaterials functionalization using a novel peptide that selectively binds to a conducting polymer. Nat. Mater. 2005, 4, 496–502. [Google Scholar] [CrossRef]
- Algarni, M.; Ghazali, S. Comparative Study of the Sensitivity of PLA, ABS, PEEK, and PETG’s Mechanical Properties to FDM Printing Process Parameters. Crystals 2021, 11, 995. [Google Scholar] [CrossRef]
- Ma, Y.; Cheung, W.; Wei, D.; Bogozi, A.; Chiu, P.L.; Wang, L.; Pontoriero, F.; Mendelsohn, R.; He, H. Improved conductivity of carbon nanotube networks by in situ polymerization of a thin skin of conducting polymer. ACS Nano 2008, 2, 1197–1204. [Google Scholar] [CrossRef] [PubMed]
- Hatton, R.A.; Miller, A.J.; Silva, S.R.P. Carbon nanotubes: A multi-functional material for organic optoelectronics. J. Mater. Chem. 2008, 18, 1183–1192. [Google Scholar] [CrossRef]
- Ren, S.; Bernardi, M.; Lunt, R.R.; Bulovic, V.; Grossman, J.C.; Gradecak, S. Toward efficient carbon nanotube/P3HT solar cells: Active layer morphology, electrical, and optical properties. Nano Lett. 2011, 11, 5316–5321. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bell, J.M.; Goh, R.G.S.; Waclawik, E.R.; Giulianini, M.; Motta, N. Polymer-carbon nanotube composites: Basic science and applications. Mater. Forum 2008, 32, 144–152. [Google Scholar]
- Capasso, A.; Salamandra, L.; Di Carlo, A.; Bell, J.M.; Motta, N. Low-temperature synthesis of carbon nanotubes on indium tin oxide electrodes for organic solar cells. Beilstein J. Nanotechnol. 2012, 3, 524–532. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schuettfort, T.; Snaith, H.J.; Nish, A.; Nicholas, R.J. Synthesis and spectroscopic characterization of solution processable highly ordered polythiophene–carbon nanotube nanohybrid structures. Nanotechnology 2009, 21, 25201. [Google Scholar] [CrossRef] [PubMed]
- Yan, J.; Uddin, M.J.; Dickens, T.J.; Okoli, O.I. Carbon nanotubes (CNTs) enrich the solar cells. Sol. Energy 2013, 96, 239–252. [Google Scholar] [CrossRef]
- Alturaif, H.A.; ALOthman, Z.A.; Chapter, J.G.; Wabaidur, S.M. Use of carbon nanotubes (CNTs) with polymers in solar cells. Molecules 2014, 19, 17329–17344. [Google Scholar] [CrossRef]
- Zwawi, M.; Attar, A.; Al-Hossainy, A.F.; Abdel-Aziz, M.H.; Zoromba, M.S. Polypyrrole/functionalized multi-walled carbon nanotube composite for optoelectronic device application. Chem. Pap. 2021, 75, 6575–6589. [Google Scholar] [CrossRef]
- Čvančara, P.; Boretius, T.; López-Álvarez, V.M.; Maciejasz, P.; Andreu, D.; Raspopovic, S.; Petrini, F.; Micera, S.; Granata, G.; Fernandez, E.; et al. Stability of flexible thin-film metallization stimulation electrodes: Analysis of explants after first-in-human study and improvement of in vivo performance. J. Neural Eng. 2020, 17, 046006. [Google Scholar] [CrossRef] [PubMed]
- Zoromba, M.S.; Tashkandi, M.A.; Alshehri, A.A.; Abdel-Aziz, M.H.; Bassyouni, M.; Mahmoud, S.A.; Slimane, A.B.; Al-Hossainy, A.F. Polymer solar cell based on doped o-anthranilic acid and o-aminophenol copolymer. Opt. Mater. 2020, 104, 109947. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional thermochemistry. I. The effect of the exchange-only gradient correction. J. Chem. Phys. 1992, 96, 2155–2160. [Google Scholar] [CrossRef]
- Delley, B. DMol3 DFT studies: From molecules and molecular environments to surfaces and solids. Comput. Mater. Sci. 2000, 17, 122–126. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 03, Revision E.; Gaussian, Inc.: Wallingford, CT, USA, 2009; Volume 1. [Google Scholar]
- Gangopadhyay, R. Peering into polypyrrole–SDS nanodispersions: Rheological view. J. Appl. Polym. Sci. 2013, 128, 1398–1408. [Google Scholar] [CrossRef]
- Kampf, A.R.; Plášil, J.; Kasatkin, A.V.; Marty, J. Na7(UO2)(SO4)4(SO3OH)(H2O)3, a new uranyl sulfate mineral from the Blue Lizard mine, San Juan County, Utah, USA. Mineral. Mag. 2014, 78, 639–649. [Google Scholar] [CrossRef]
- Reis, D.T.; Ribeiro, I.H.S.; & Pereira, D.H. DFT study of the application of polymers cellulose and cellulose acetate for adsorption of metal ions (Cd2+, Cu 2+ and Cr3+) potentially toxic. Polym. Bull. 2020, 77, 3443–3456. [Google Scholar] [CrossRef]
- Mori-Sanchez, P.; Wu, Q.; Yang, W. Accurate polymer polarizabilities with exact exchange density-functional theory. J. Chem. Phys. 2003, 119, 11001–11004. [Google Scholar] [CrossRef]
- Demir, P.; Akman, F. Molecular structure, spectroscopic characterization, HOMO and LUMO analysis of PU and PCL grafted onto PEMA-co-PHEMA with DFT quantum chemical calculations. J. Mol. Struct. 2017, 1134, 404–415. [Google Scholar] [CrossRef]
- Taha, T.A.; Hendawy, N.; El-Rabaie, S.; Esmat, A.; El-Mansy, M.K. Effect of NiO NPs doping on the structure and optical properties of PVC polymer films. Polym. Bull. 2019, 76, 4769–4784. [Google Scholar] [CrossRef]
- Mohamad, A.H.; Saeed, S.R.; Abdullah, O.G. Synthesis of very-fine PbS nanoparticles dispersed homogeneously in MC matrix: Effect of concentration on the structural and optical properties of host polymer. Mater. Res. Express 2019, 6, 115332. [Google Scholar] [CrossRef]
- Kaya, S.; Tüzün, B.; Kaya, C.; Obot, I.B. Determination of corrosion inhibition effects of amino acids: Quantum chemical and molecular dynamic simulation study. J. Taiwan Inst. Chem. Eng. 2016, 58, 528–535. [Google Scholar] [CrossRef]
- Kaya, S.; Guo, L.; Kaya, C.; Tüzün, B.; Obot, I.B.; Touir, R.; Islam, N. Quantum chemical and molecular dynamic simulation studies for the prediction of inhibition efficiencies of some piperidine derivatives on the corrosion of iron. J. Taiwan Inst. Chem. Eng. 2016, 65, 522–529. [Google Scholar] [CrossRef]
- Vidya, Y.S.; Anantharaju, K.S.; Nagabhushana, H.; Sharma, S.C.; Nagaswarupa, H.P.; Prashantha, S.C.; Shivakumara, C. Combustion synthesized tetragonal ZrO2: Eu3+ nanophosphors: Structural and photoluminescence studies. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2015, 135, 241–251. [Google Scholar] [CrossRef] [Green Version]
- Gao, F.; Yang, C.L.; Wang, M.S.; Ma, X.G.; Liu, W.W. Theoretical insight on hybrid nanocomposites of graphene quantum dot and carbazole–carbazole dyes as an efficient sensitizer of DSSC. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2019, 216, 69–75. [Google Scholar] [CrossRef] [PubMed]
- Aflaki, M.; Davar, F. Synthesis, luminescence and photocatalyst properties of zirconia nanosheets by modified Pechini method. J. Mol. Liq. 2016, 221, 1071–1079. [Google Scholar] [CrossRef] [Green Version]
- Alotaibi, S.H.; Radwan, A.S.; Abdel-Monem, Y.K.; Makhlouf, M.M. Synthesis, thermal behavior and optical characterizations of thin films of a novel thiazole azo dye and its copper complexes. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2018, 205, 364–375. [Google Scholar] [CrossRef] [PubMed]
- Kasap, S. Springer Handbook of Electronic and Photonic Materials; Springer Science & Business Media: Cham, Switzerland, 2006. [Google Scholar]
- Caglar, M.; Zor, M.; Ilican, S.; Caglar, Y. Effect of indium incorporation on the optical properties of spray pyrolyzed Cd 0.22 Zn 0.78 S thin films. Czechoslov. J. Phys. 2006, 56, 277–287. [Google Scholar] [CrossRef]
- Ali, N.A. Optical Properties of Biodegradable Polylactic Acid/Silver Nanocomposites: A Study. J. Chem. Pharm. Res. 2017, 9, 238–243. [Google Scholar]
- Ibrahim, S.M.; Bourezgui, A.; Abd-Elmageed, A.A.I.; Kacem, I.; Al-Hossainy, A.F. Structural and optical characterization of novel [ZnKCMC] TF for optoelectronic device applications. J. Mater. Sci. Mater. Electron. 2020, 31, 8690–8704. [Google Scholar] [CrossRef]
- Abkowitz, M.; Facci, J.S.; Rehm, J. Direct evaluation of contact injection efficiency into small molecule based transport layers: Influence of extrinsic factors. J. Appl. Phys. 1998, 83, 2670–2676. [Google Scholar] [CrossRef]
- Bunakov, A.; Lachinov, A.; Salikhov, R. Current-voltage characteristics of thin poly (biphenyl-4-ylphthalide) films. Macromol. Symp. 2004, 212, 387–392. [Google Scholar] [CrossRef]
- Naz, A.; Kausar, A.; Siddiq, M.; Choudhary, M.A. Comparative review on structure, properties, fabrication techniques, and relevance of polymer nanocomposites reinforced with carbon nanotube and graphite fillers. Polym.-Plast. Technol. Eng. 2016, 55, 171–198. [Google Scholar] [CrossRef]
- Khan, S.U.; Pothnis, J.R.; Kim, J.K. Effects of carbon nanotube alignment on electrical and mechanical properties of epoxy nanocomposites. Compos. Part A Appl. Sci. Manuf. 2013, 49, 26–34. [Google Scholar] [CrossRef]
- Sudha; Mishra, B.M.; Kumar, D. Effect of multiwalled carbon nanotubes on the conductivity and swelling properties of porous polyacrylamide hydrogels. Part. Sci. Technol. 2014, 32, 624–631. [Google Scholar] [CrossRef]
- Park, S.B.; Lee, M.S.; Park, M. Study on lowering the percolation threshold of carbon nanotube-filled conductive polypropylene composites. Carbon Lett. 2014, 15, 117–124. [Google Scholar] [CrossRef] [Green Version]
- Ma, P.C.; Siddiqui, N.A.; Marom, G.; Kim, J.K. Dispersion and functionalization of carbon nanotubes for polymer-based nanocomposites: A review. Compos. Part A Appl. Sci. Manuf. 2010, 41, 1345–1367. [Google Scholar] [CrossRef]
- Gupta, P.; Rajput, M.; Singla, N.; Kumar, V.; Lahiri, D. Electric field and current assisted alignment of CNT inside polymer matrix and its effects on electrical and mechanical properties. Polymer 2016, 89, 119–127. [Google Scholar] [CrossRef]
- Bachhav, S.G.; Patil, D.R. Study of Polypyrrole-Coated MWCNT Nanocomposites for Ammonia Sensing at Room Temperature. J. Mater. Sci. Chem. Eng. 2015, 3, 30. [Google Scholar] [CrossRef] [Green Version]
- Imani, A.; Farzi, G.; Ltaief, A. Facile synthesis and characterization of polypyrrole-multiwalled carbon nanotubes by in situ oxidative polymerization. Int. Nano Lett. 2013, 3, 52. [Google Scholar] [CrossRef] [Green Version]
- Chakraborty, G.; Gupta, K.; Meikap, A.K.; Babu, R.; Blau, W.J. Synthesis, electrical and magnetotransport properties of polypyrrole-MWCNT nanocomposite. Solid State Commun. 2012, 152, 13–18. [Google Scholar] [CrossRef]
- Wu, T.-M.; Chang, H.-L.; Lin, Y.-W. Synthesis and characterization of conductive polypyrrole/multi-walled carbon nanotubes composites with improved solubility and conductivity. Compos. Sci. Technol. 2009, 69, 639–644. [Google Scholar] [CrossRef]
- Monti, M.; Natali, M.; Torre, L.; Kenny, J.M. The alignment of single walled carbon nanotubes in an epoxy resin by applying a DC electric field. Carbon 2012, 50, 2453–2464. [Google Scholar] [CrossRef]



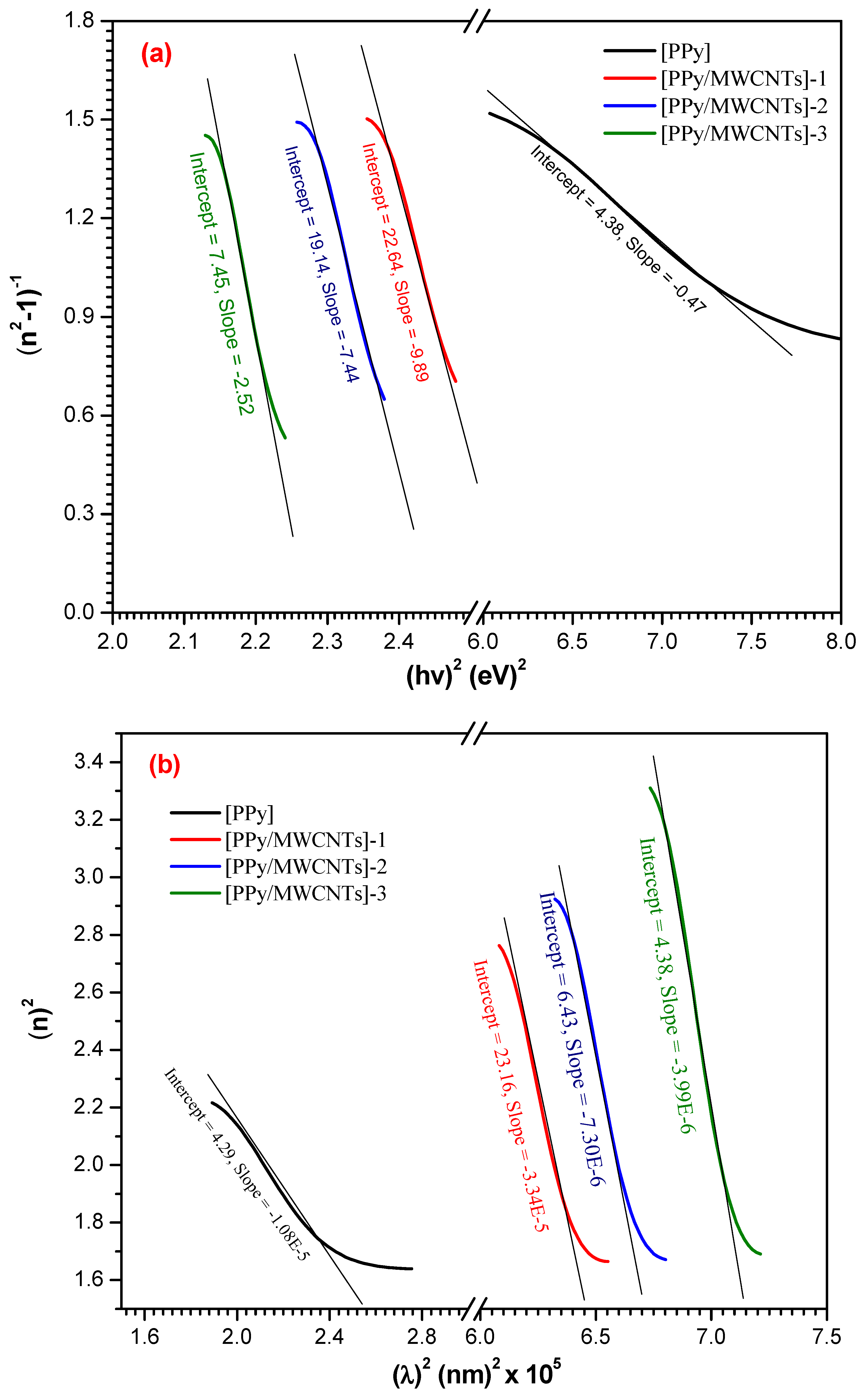
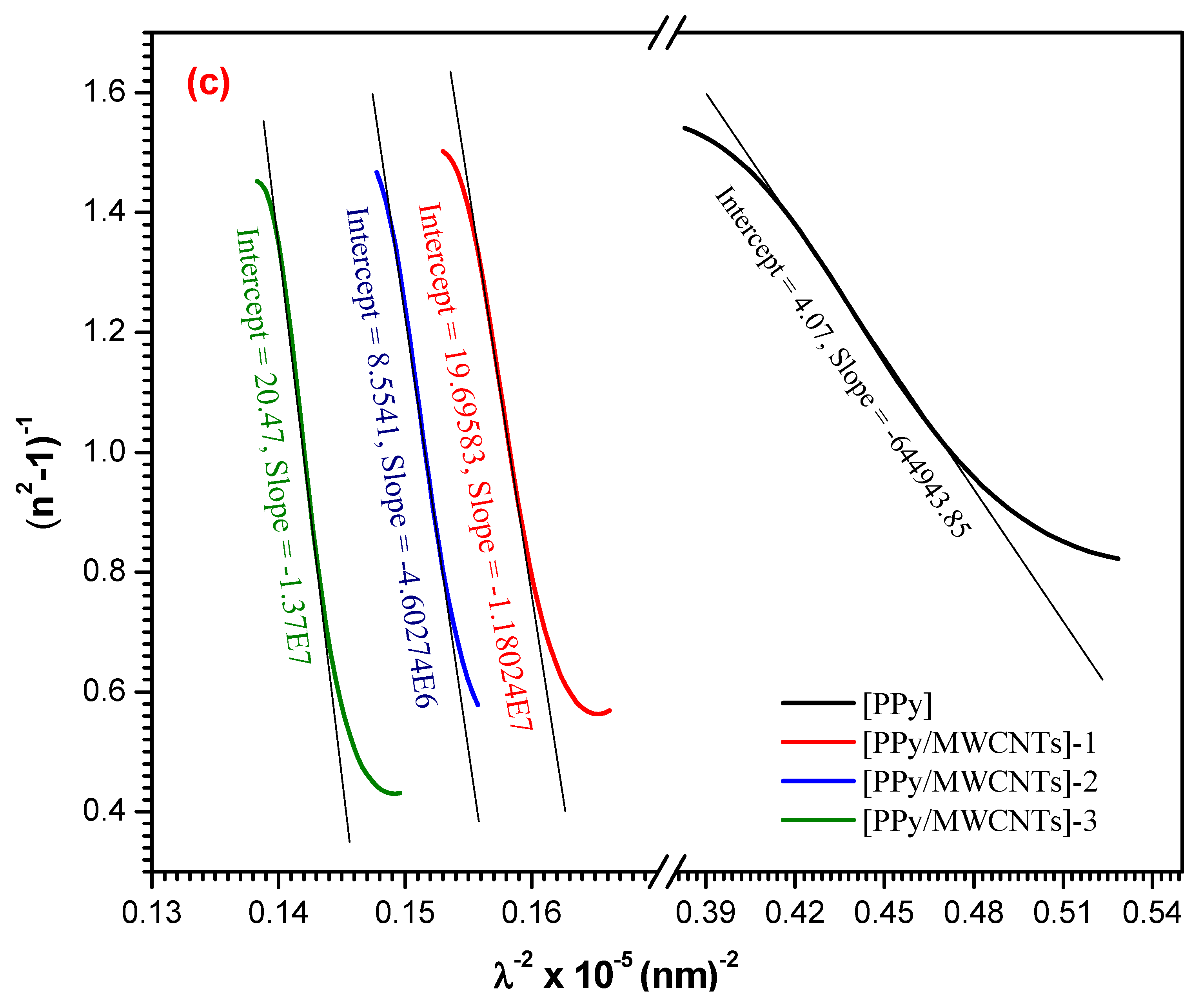
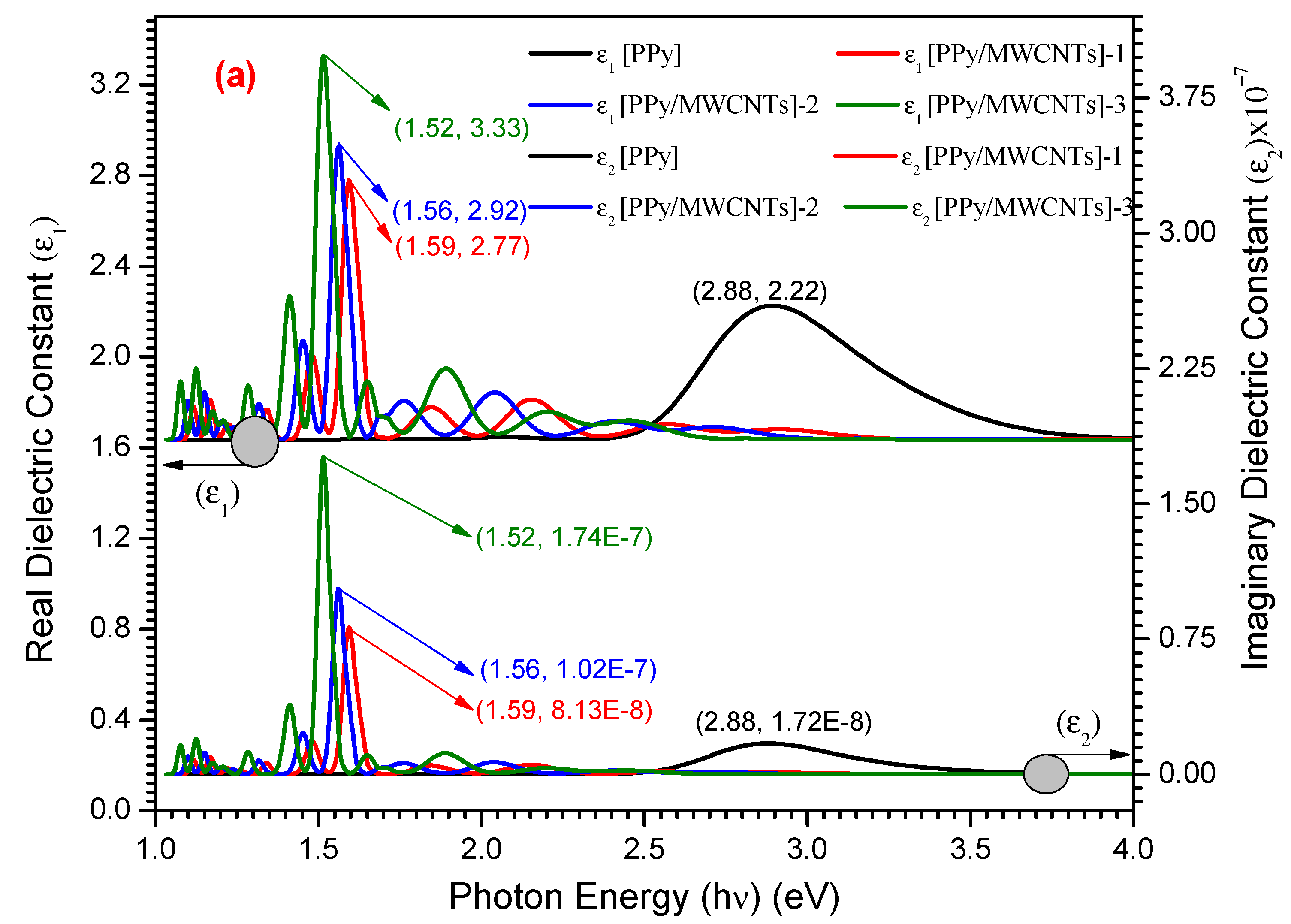
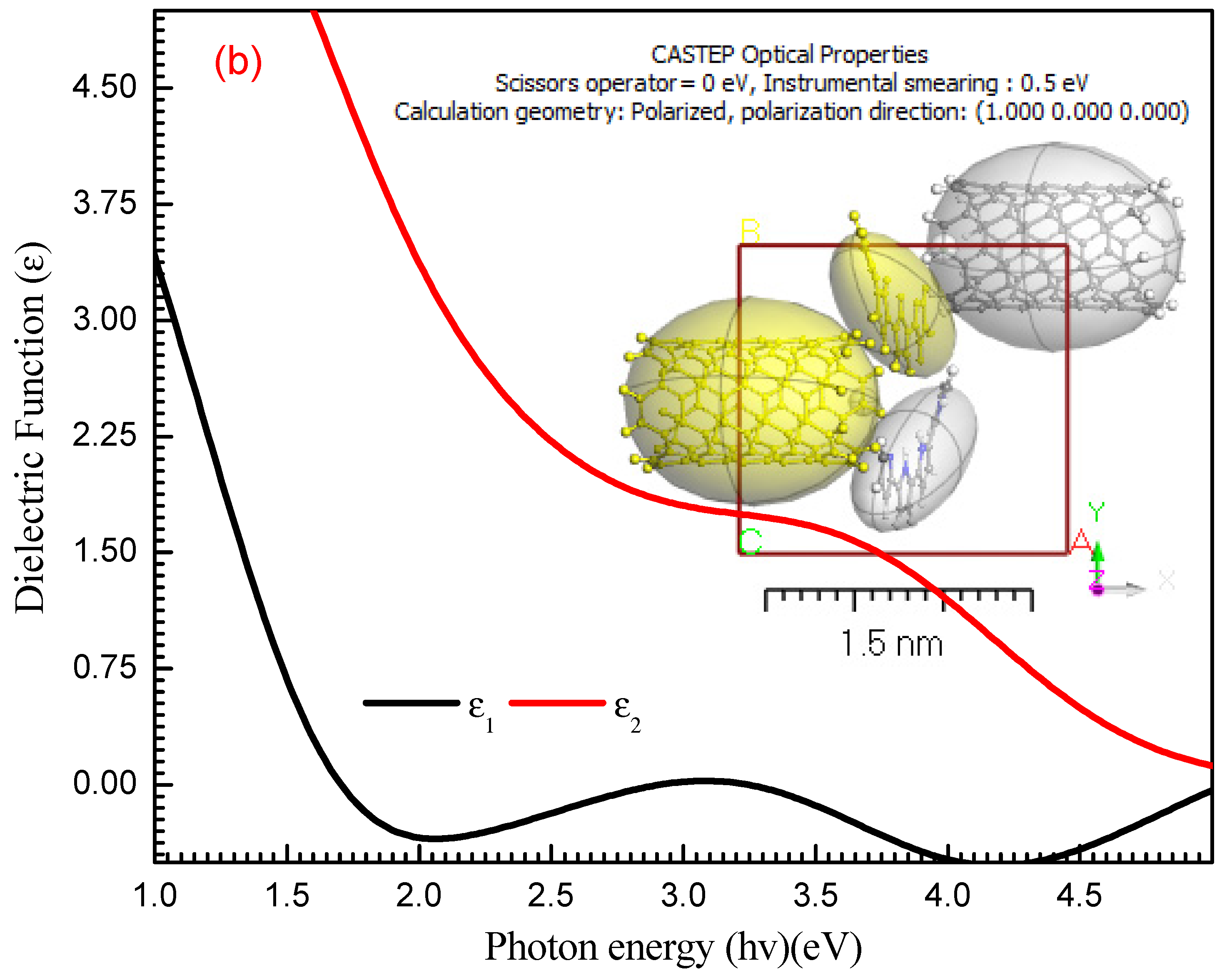

| Analysis | Instrumentation |
|---|---|
| SEM | SEM; (Inspect S, FEI, Holland), operated at an accelerating voltage of 3.0 kV. |
| Film thickness | Digital micrometer with accuracy ± 10−3 nm |
| XRD | Philips X-ray diffractometer (model X’pert) with monochromatic Cu Kα radiation operated at 40 kV. |
| UV | SHIMADZU UV-3101 UV–vis–NIR pc spectrophotometer. |
| ρ & σ | The electrical resistivity and conductivity values of the thin film were calculated by a Keithley 6517B electrometer. |
| Symmetry | Observed | Calculated | Difference | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Compound | 2tdeta | d | hkl | 2tdeta | d | 2tdeta | d | FWHM | Dav (b) |
| [PPy/MWCNTs], Triclinic | 11.27 | 7.83 | 02 | 11.15 | 7.92 | −0.12 | −0.08 | 0.19 | 43.23 |
| a = 5.47(2); | 14.31 | 6.18 | 012 | 14.41 | 6.14 | 0.10 | 0.04 | 0.26 | 32.49 |
| b = 11.30(3) | 17.54 | 5.05 | 11 | 17.59 | 5.03 | 0.05 | 0.01 | 0.21 | 39.84 |
| and c = 18.30(5) nm | 18.30 | 4.84 | 021 | 18.25 | 4.85 | −0.06 | −0.01 | 0.18 | 46.29 |
| α =104.7(1)°, | 20.04 | 4.42 | 004 | 20.04 | 4.42 | −0.003 | −0.001 | 0.11 | 76.52 |
| γ =96.4(2)°, | 20.38 | 4.35 | 2 | 20.36 | 4.35 | −0.02 | −0.004 | 0.20 | 43.20 |
| β = 89(3)° | 20.56 | 4.24 | 12 | 20.92 | 4.24 | −0.02 | −0.004 | 0.11 | 72.58 |
| V= 1080 (4) | 20.93 | 4.08 | 20 | 21.75 | 4.08 | 0.02 | 0.004 | 0.16 | 52.12 |
| rmse (a) = 0.000305 | 24.93 | 3.57 | 023 | 24.93 | 3.57 | 0.00 | 0.00 | 0.06 | 144.87 |
| λ = 1.541838 Å | 27.51 | 3.24 | 04 | 27.54 | 3.23 | 0.03 | 0.003 | 0.10 | 81.57 |
| machine error = 0.016 | 30.92 | 2.89 | 14 | 30.90 | 2.89 | −0.02 | −0.002 | 0.17 | 52.12 |
| Average | 0.16 | 62.26 | |||||||
| Compound | χ (eV) | µ (eV) | η (eV) | S (eV) | ω (eV) | (eV−1) | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| PPy | −5.05 | −2.16 | −2.89 | 3.60 | −3.60 | 1.44 | 0.35 | 4.50 | 2.50 | 0.69 |
| [PPy/MWCNTs] | −4.20 | −2.62 | −1.58 | 3.41 | −3.41 | 0.79 | 0.63 | 7.38 | 4.32 | 1.27 |
| Composition | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Polymer PPy | 2.65 | 2.41 | 2.89 | 0.70 | 3.07 | 4.29 | 1.33 × 1040 | 1.23 | 1.25 | 197 | 6.31 × 1012 |
| [PPy/MWCNTs]-1 | 1.55 | 1.55 | 1.58 | 0.07 | 1.51 | 4.33 | 4.10 × 1040 | 1.04 | 1.05 | 215 | 1.09 × 1012 |
| [PPy/MWCNTs]-2 | 1.50 | 1.50 | 0.08 | 1.60 | 4.38 | 4.89 × 1039 | 1.05 | 1.12 | 251 | 1.86 × 1012 | |
| [PPy/MWCNTs]-3 | 1.48 | 1.48 | 0.23 | 1.72 | 6.43 | 8.95 × 1039 | 1.13 | 1.05 | 181 | 1.5 × 1012 |
| Temp. (K) | Pure PPy | PPy/MWCNTs-1 | PPy/MWCNTs-2 | PPy/MWCNTs-3 | Activation Energy | ||||
|---|---|---|---|---|---|---|---|---|---|
| 290 | 1.16 | 1.93 | 1.25 | 2.15 | 1.31 | 2.31 | 1.83 | 2.37 | 2.78 |
| 307 | 1.19 | 1.72 | 1.36 | 1.73 | 1.45 | 2.02 | 1.66 | 2.18 | |
| 324 | 1.22 | 1.53 | 1.48 | 1.82 | 1.37 | 1.93 | 1.90 | 2.02 | |
| 341 | 1.17 | 1.45 | 1.40 | 1.48 | 1.60 | 1.74 | 1.71 | 2.22 | |
| 358 | 1.18 | 1.40 | 1.42 | 1.34 | 1.57 | 1.45 | 1.59 | 2.20 | |
| Int. a | Vmb | Jmc | Vocb | Jscd | Power | FF | η (PCE) |
|---|---|---|---|---|---|---|---|
| 30 | 0.17 | 1.48 × 10−5 | 0.56 | 1.60 × 10−4 | 2.46 × 10−6 | 0.03 | 1.31 |
| 60 | 0.23 | 5.08 × 10−5 | 0.92 | 3.36 × 10−4 | 1.15 × 10−5 | 0.04 | 3.06 |
| 90 | 0.27 | 1.08 × 10−4 | 1.04 | 4.78 × 10−4 | 2.89 × 10−5 | 0.06 | 5.15 |
| 120 | 0.27 | 1.65 × 10−4 | 1.24 | 6.53 × 10−4 | 4.51 × 10−5 | 0.06 | 6.01 |
| 150 | 0.29 | 2.22 × 10−4 | 1.4 | 9.35 × 10−4 | 6.43 × 10−5 | 0.05 | 6.86 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Attar, A.; Alharthy, R.D.; Zwawi, M.; Algarni, M.; Albatati, F.; Bassyouni, M.; Abdel-Aziz, M.H.; Zoromba, M.S.; Al-Hossainy, A.F. Fabrication and Characterization of Polypyrrole/Multi-Walled Carbon Nanotubes Thin Films Using Thermal Evaporation. Polymers 2021, 13, 4045. https://doi.org/10.3390/polym13224045
Attar A, Alharthy RD, Zwawi M, Algarni M, Albatati F, Bassyouni M, Abdel-Aziz MH, Zoromba MS, Al-Hossainy AF. Fabrication and Characterization of Polypyrrole/Multi-Walled Carbon Nanotubes Thin Films Using Thermal Evaporation. Polymers. 2021; 13(22):4045. https://doi.org/10.3390/polym13224045
Chicago/Turabian StyleAttar, Alaa, Rima D. Alharthy, Mohammed Zwawi, Mohammed Algarni, Faisal Albatati, Mohamed Bassyouni, Mohamed Helmy Abdel-Aziz, Mohamed Shafick Zoromba, and Ahmed F. Al-Hossainy. 2021. "Fabrication and Characterization of Polypyrrole/Multi-Walled Carbon Nanotubes Thin Films Using Thermal Evaporation" Polymers 13, no. 22: 4045. https://doi.org/10.3390/polym13224045
APA StyleAttar, A., Alharthy, R. D., Zwawi, M., Algarni, M., Albatati, F., Bassyouni, M., Abdel-Aziz, M. H., Zoromba, M. S., & Al-Hossainy, A. F. (2021). Fabrication and Characterization of Polypyrrole/Multi-Walled Carbon Nanotubes Thin Films Using Thermal Evaporation. Polymers, 13(22), 4045. https://doi.org/10.3390/polym13224045









