Numerical and Experimental Investigations of Polymer Viscoelastic Materials Obtained by 3D Printing
Abstract
1. Introduction
2. Materials and Methods
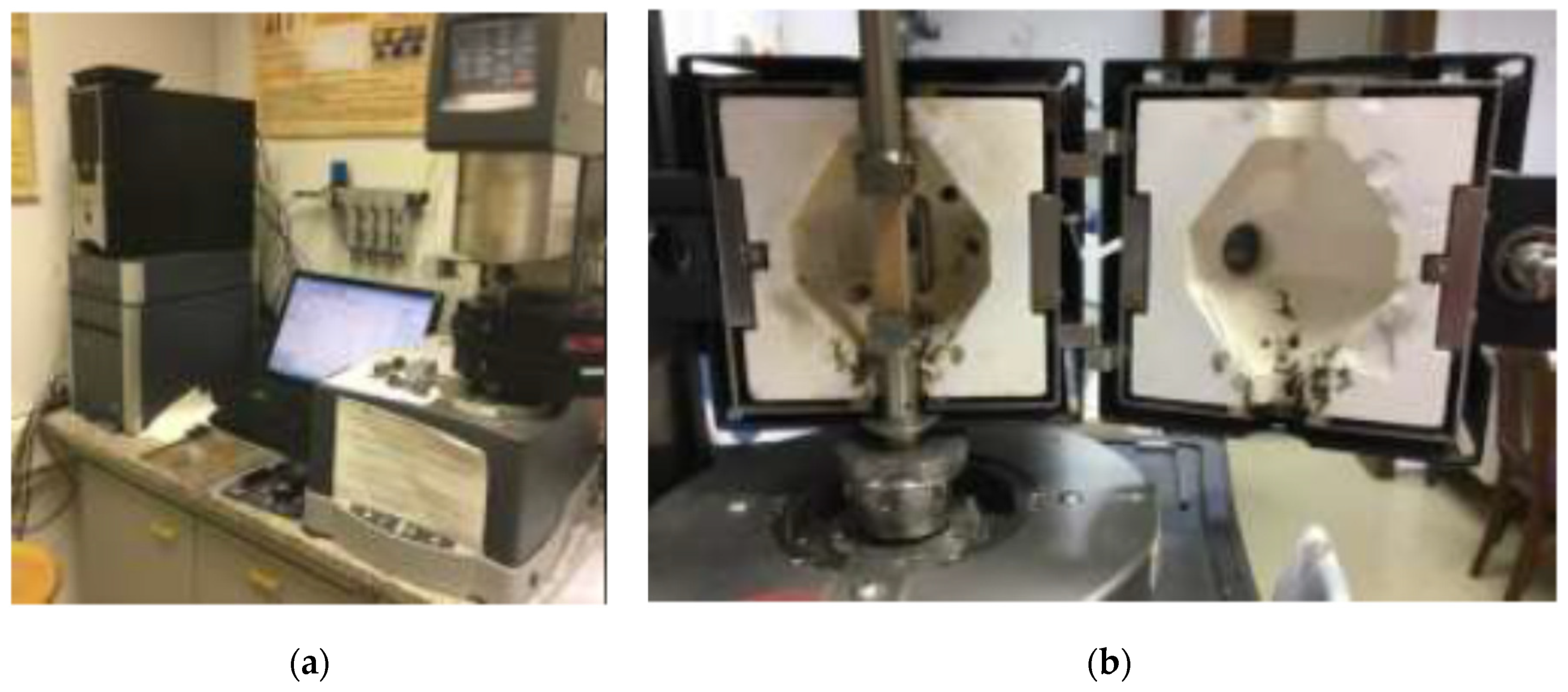
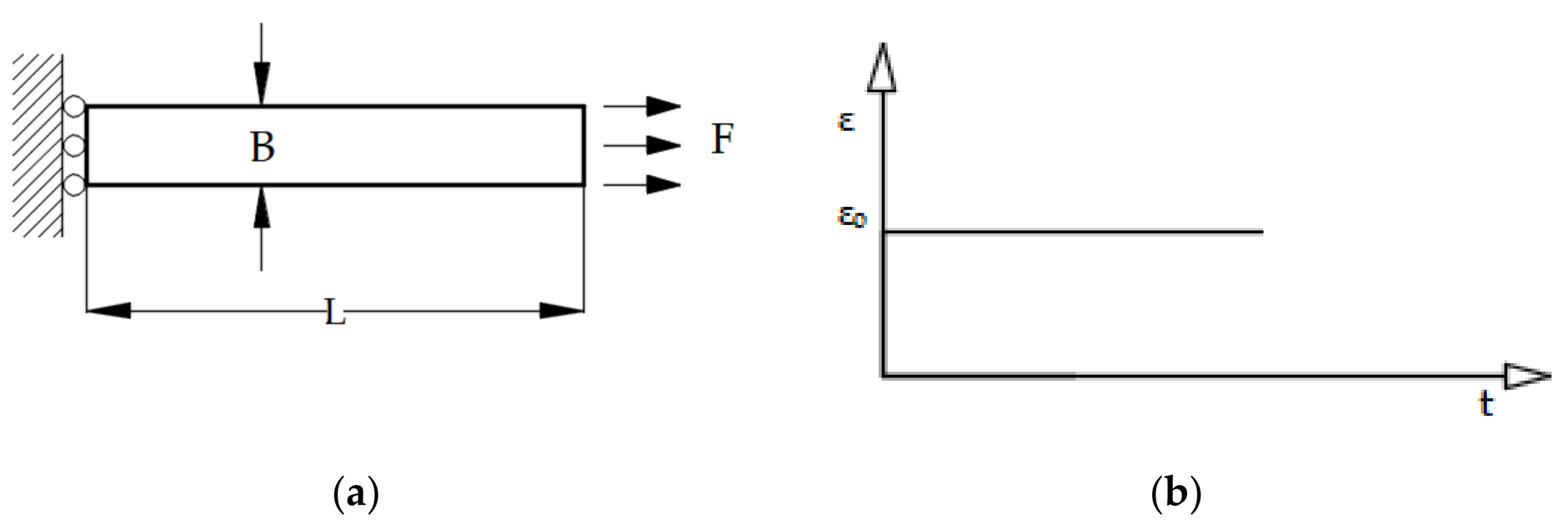
2.1. Experimental Analysis
2.2. Numerical Method
2.2.1. Governing Equations
2.2.2. Constitutive Relations
2.2.3. Initial and Boundary Conditions
2.2.4. Correction of Boundary Conditions
2.2.5. Calculation of Total Stress
3. Results
3.1. Experimental Results
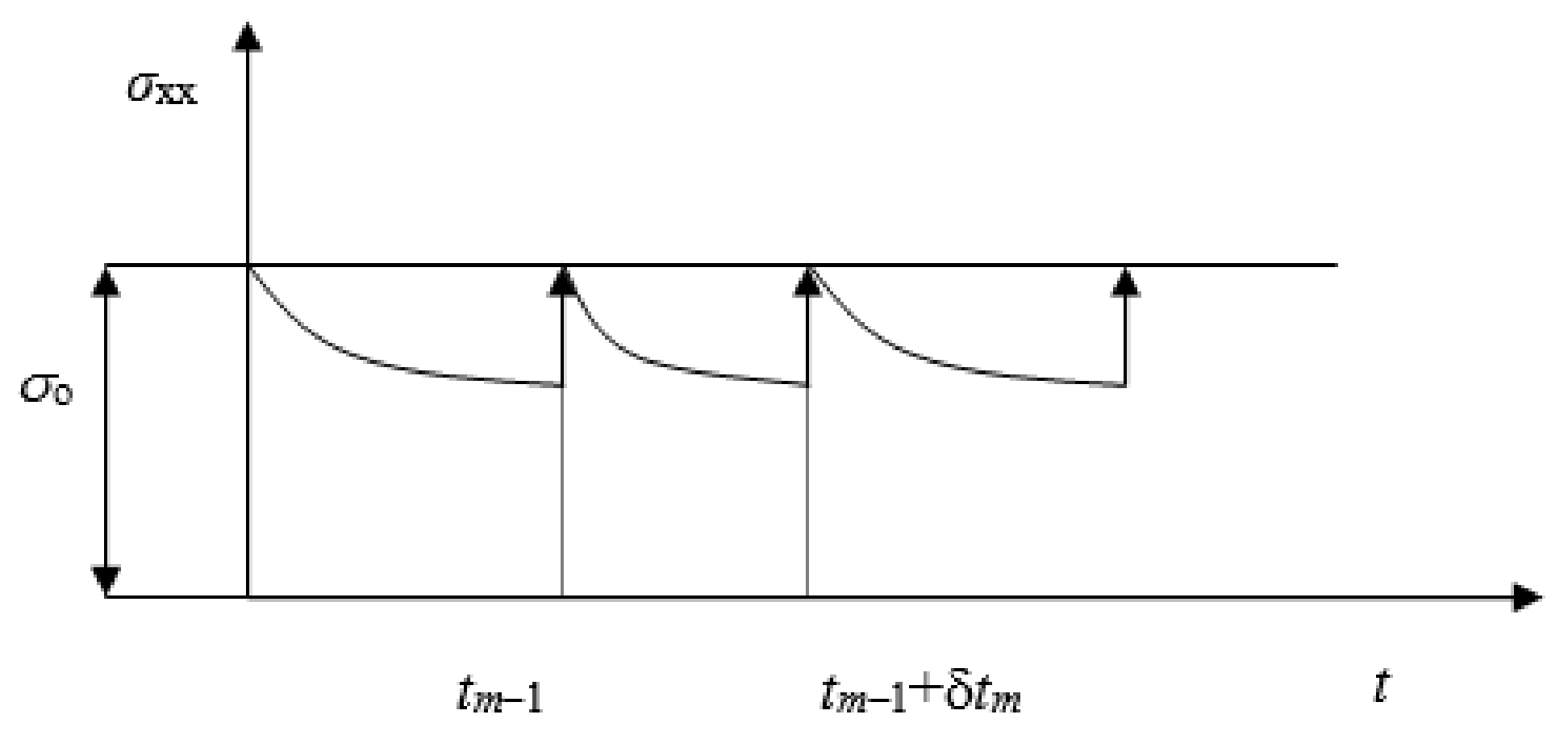
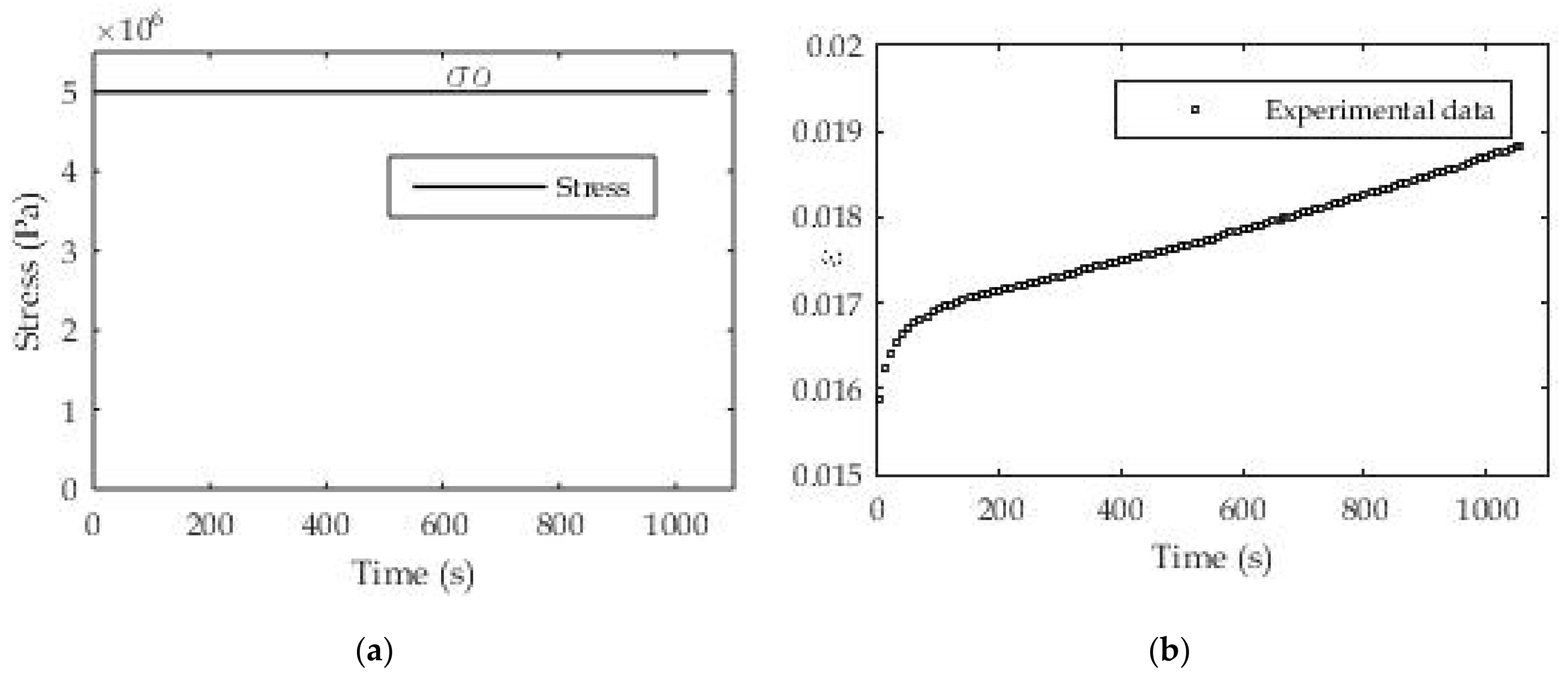
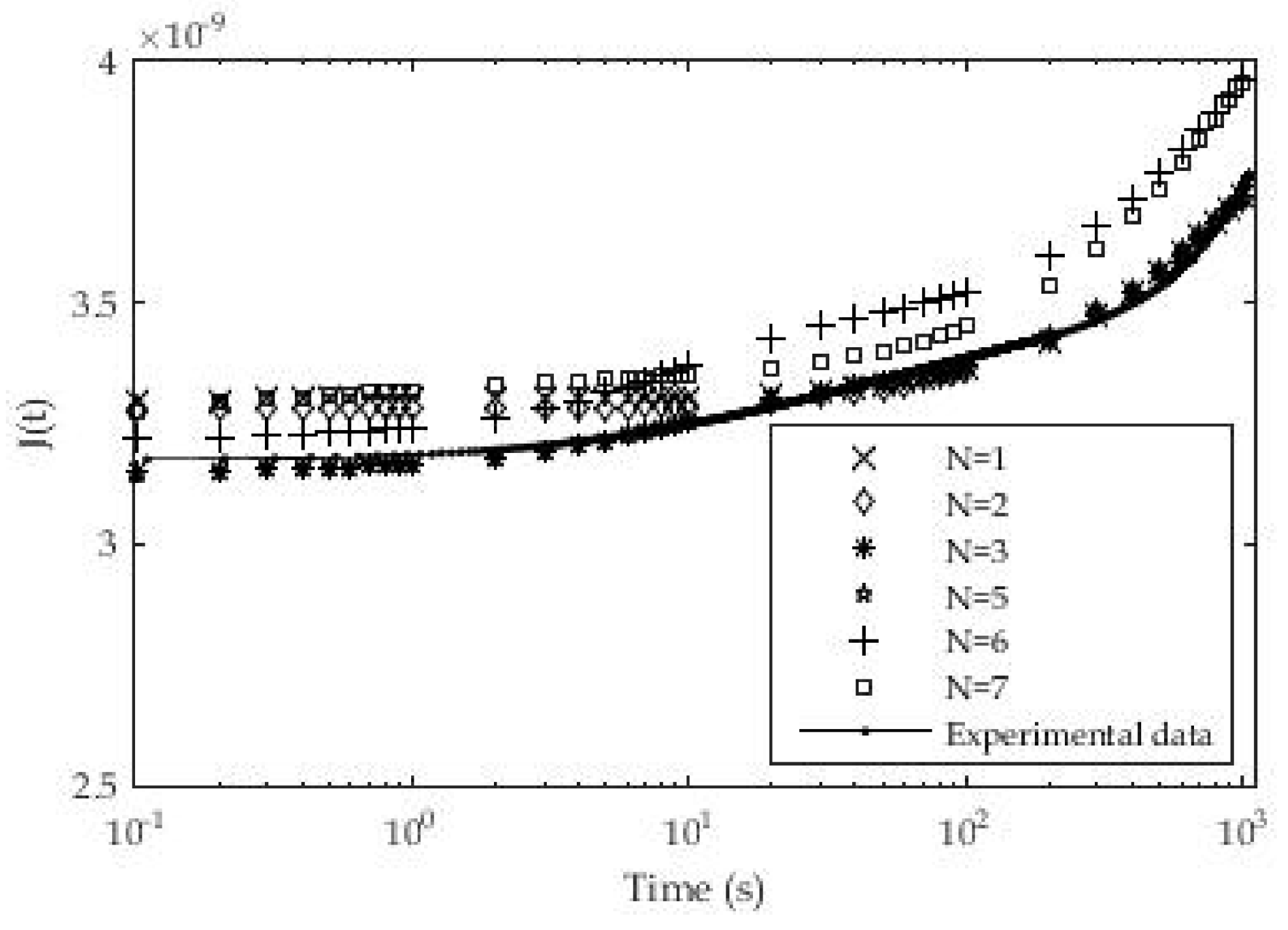
3.1.1. Determination of Creep Modulus from Creep Test
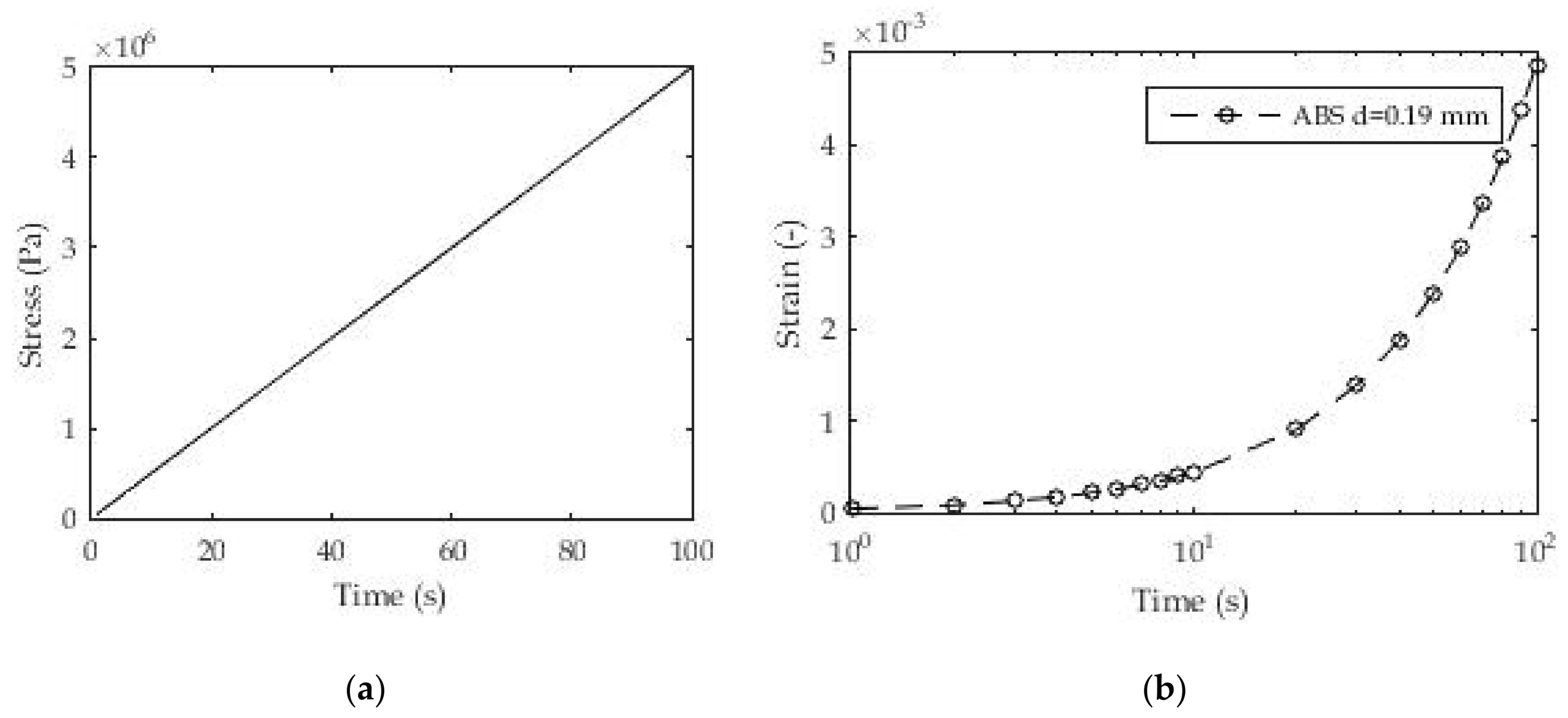
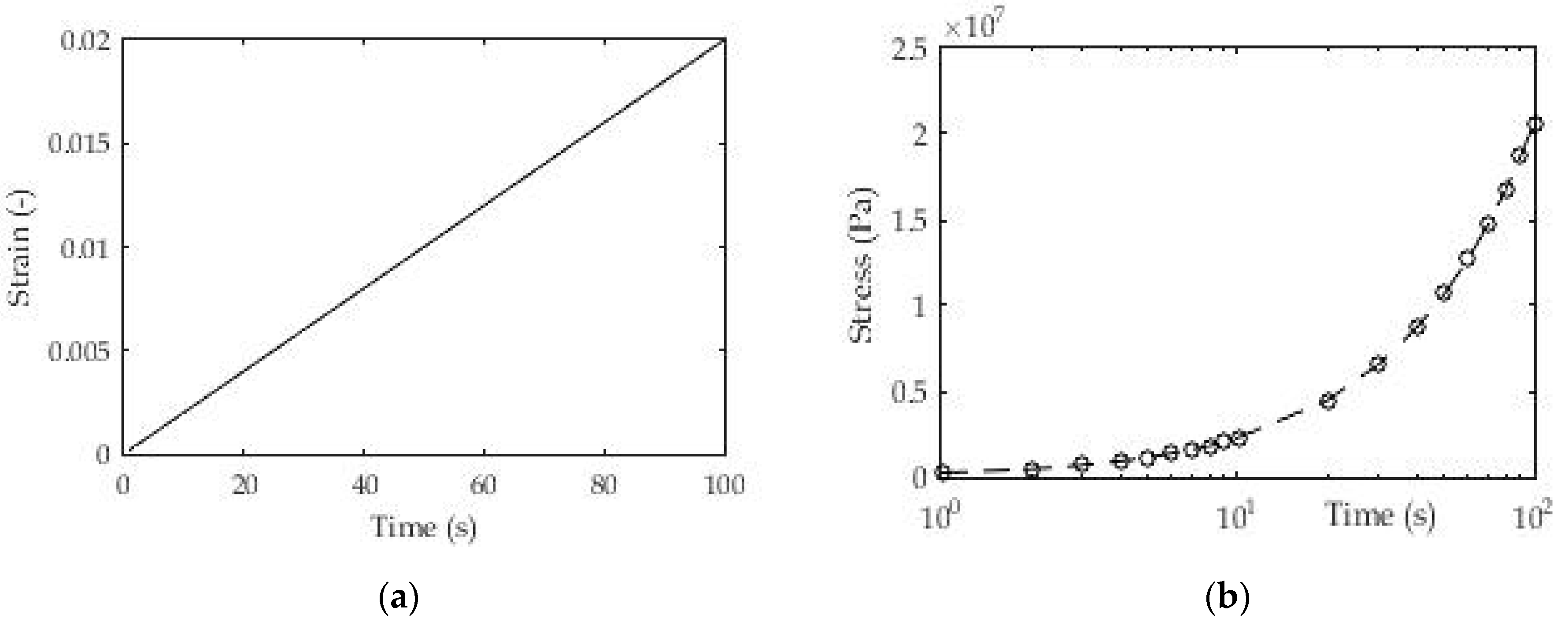
3.1.2. Determination of Creep Modulus from Constant Stress-Rate Test
3.1.3. Determination of Relaxation Modulus from the Constant Strain-Rate Test
3.1.4. Determination of Relaxation Module from Dynamic Test “Frequency Sweep”
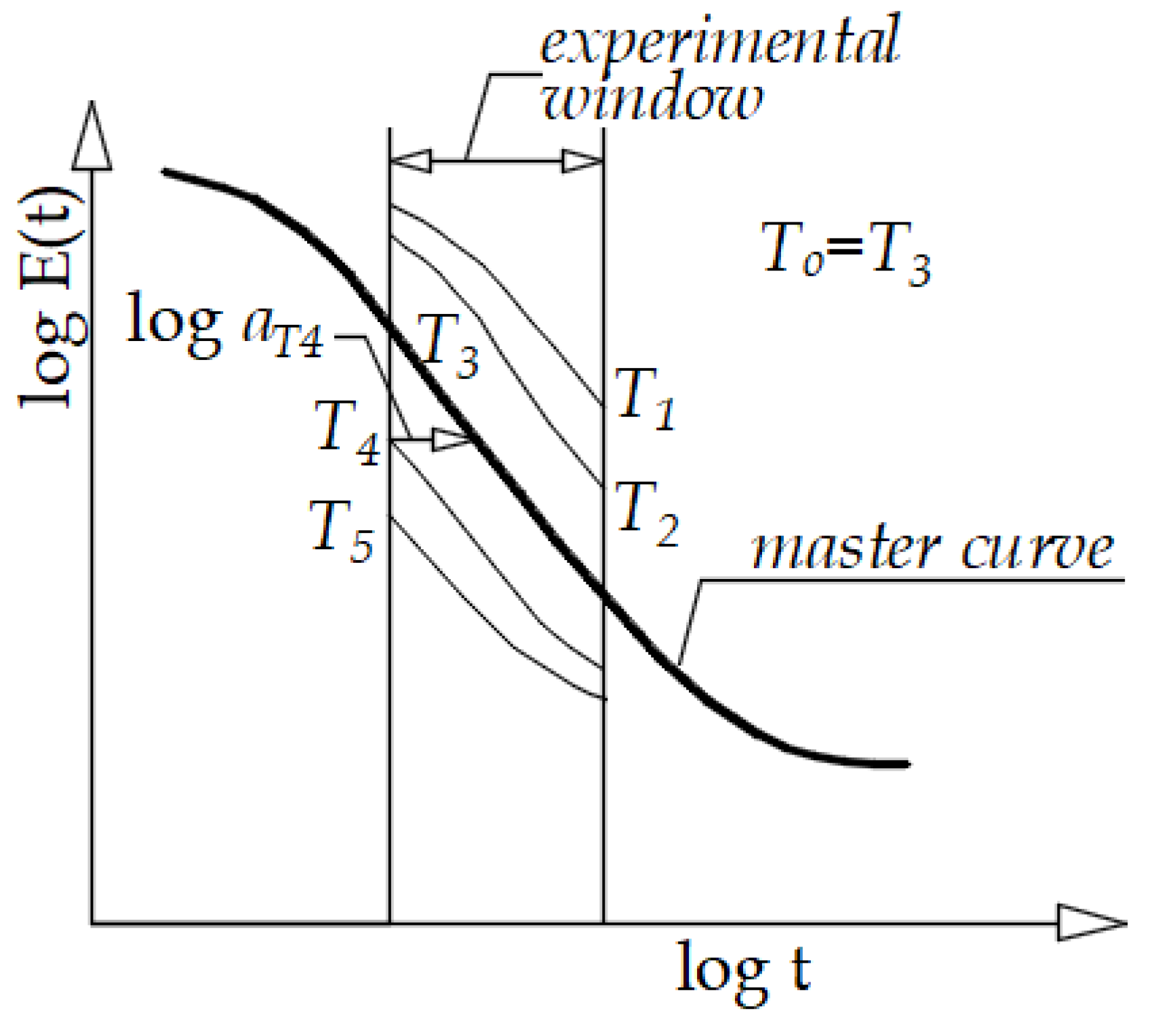
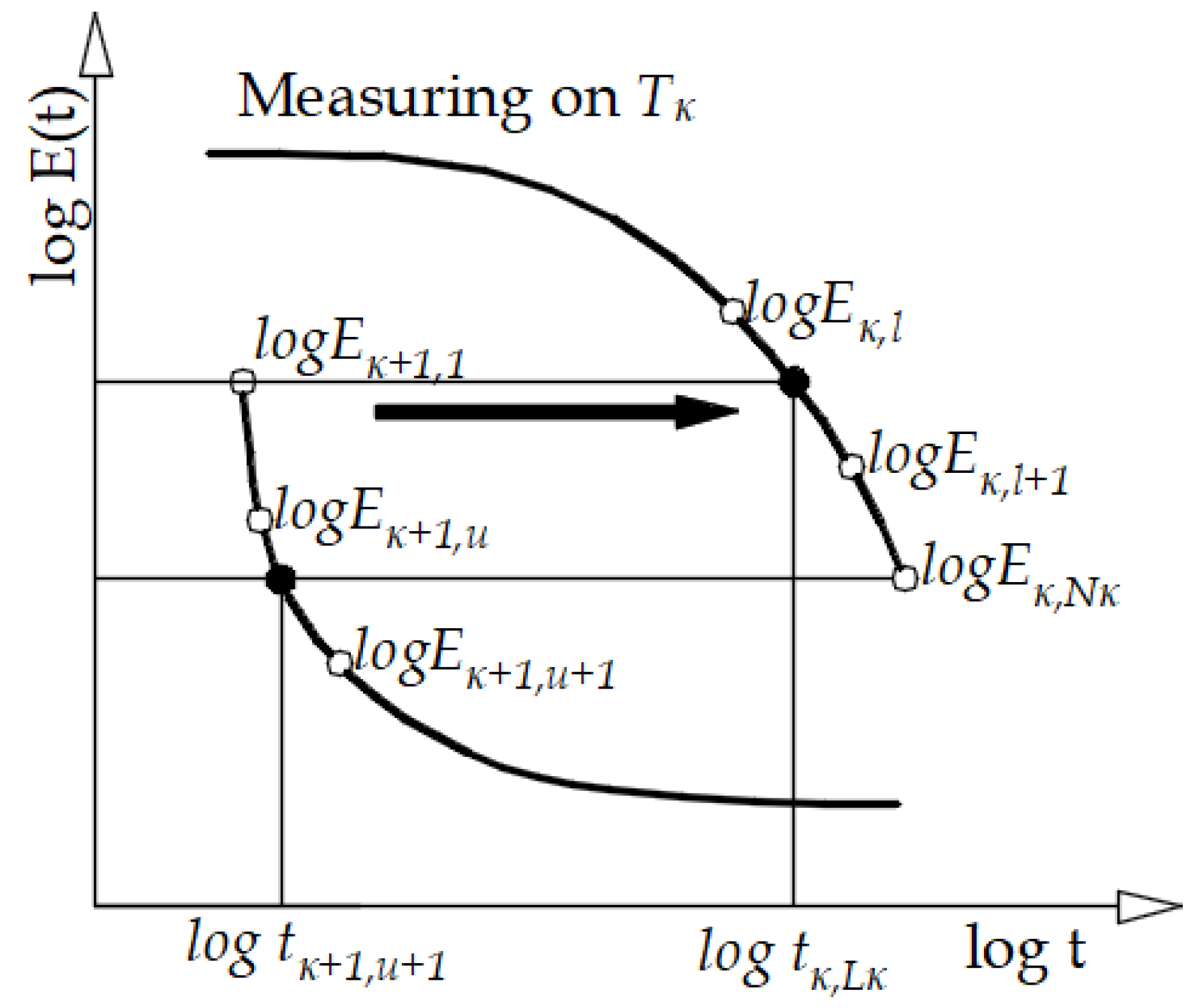
3.1.5. Determination of Time-Temperature Coefficient aT
3.2. Examples of Numerical Simulations
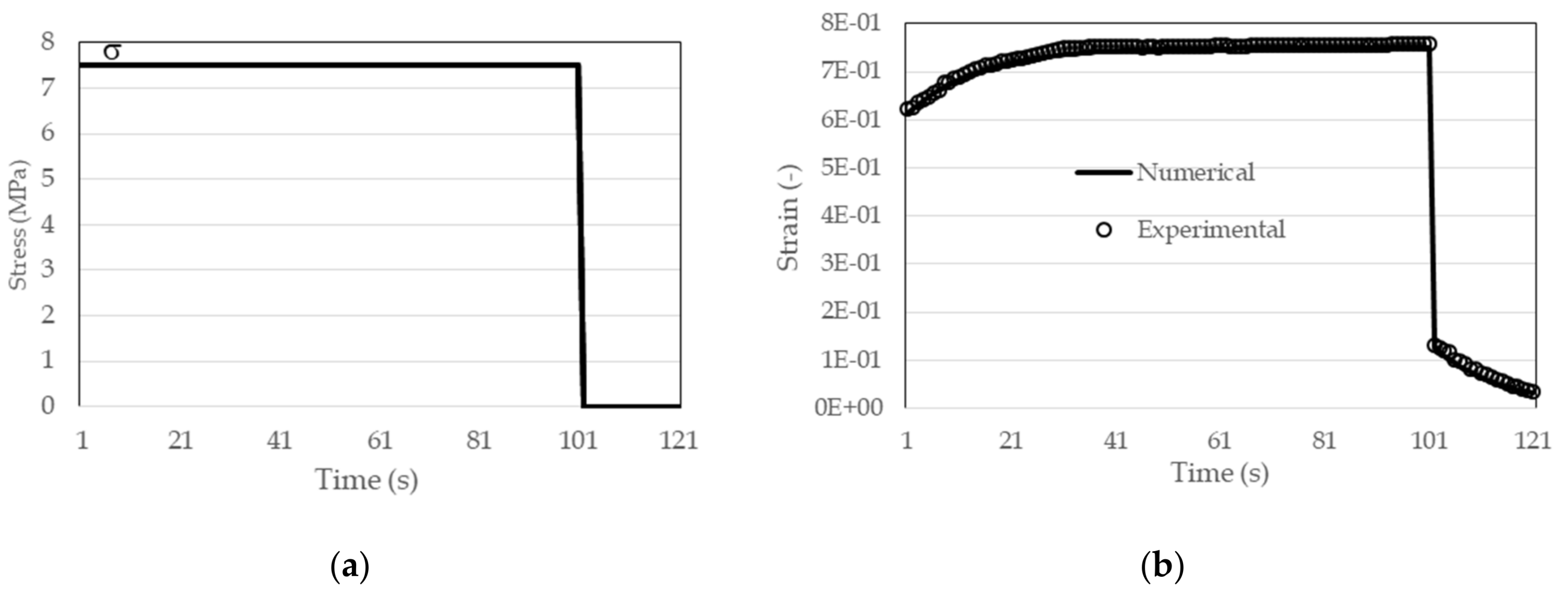
3.2.1. Relaxation Test
3.2.2. Creep Recovery Test
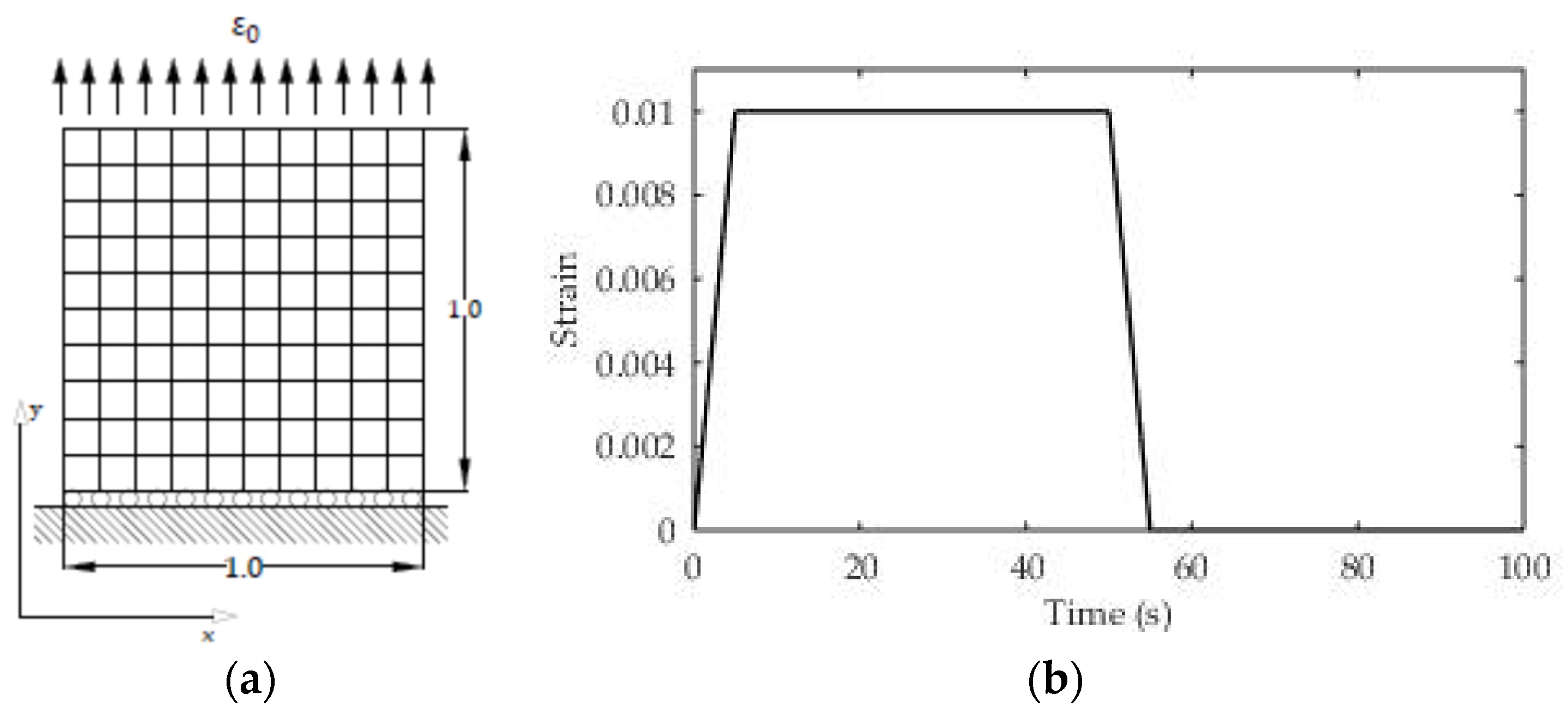
3.2.3. Plane-Strain Time-Varying Test
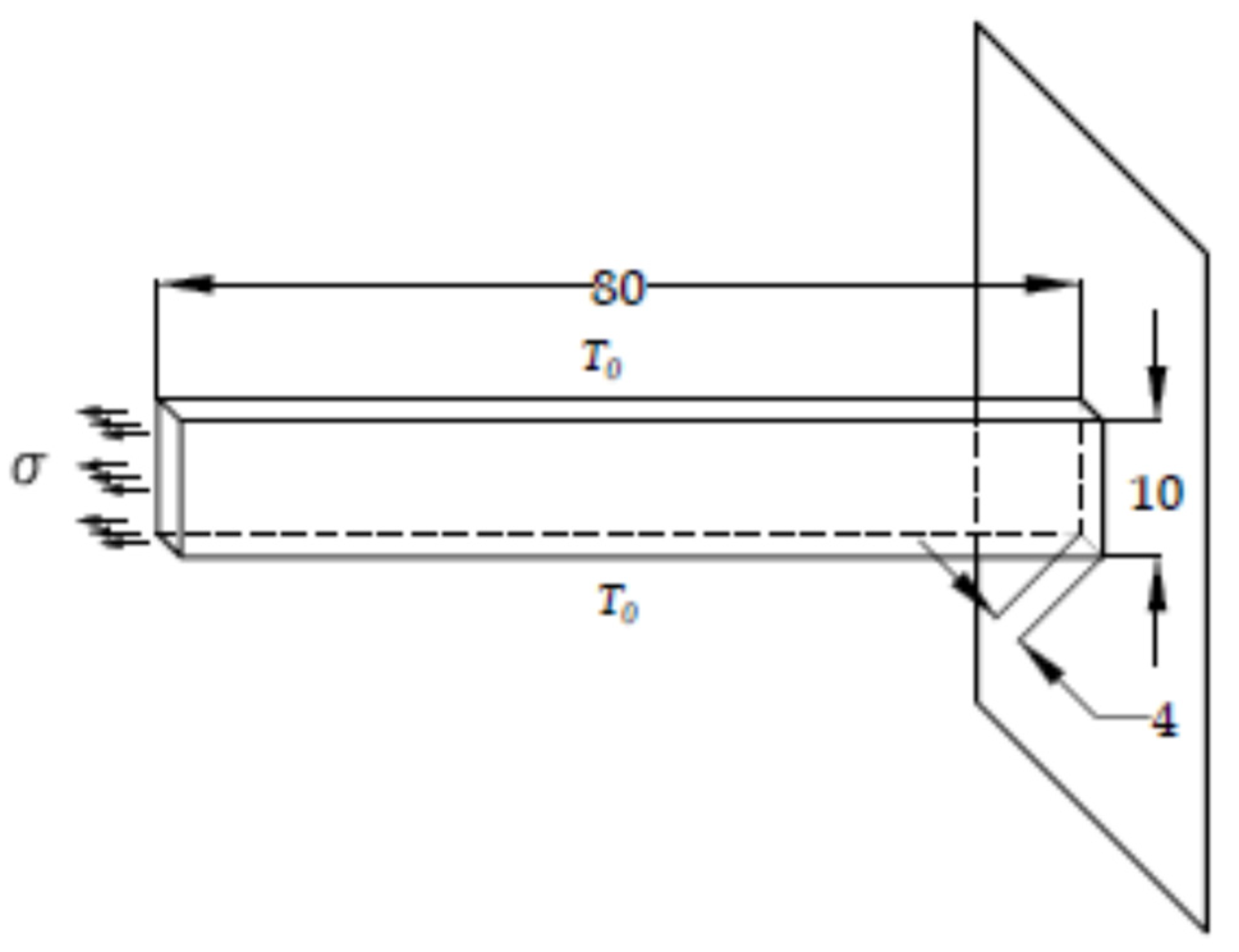
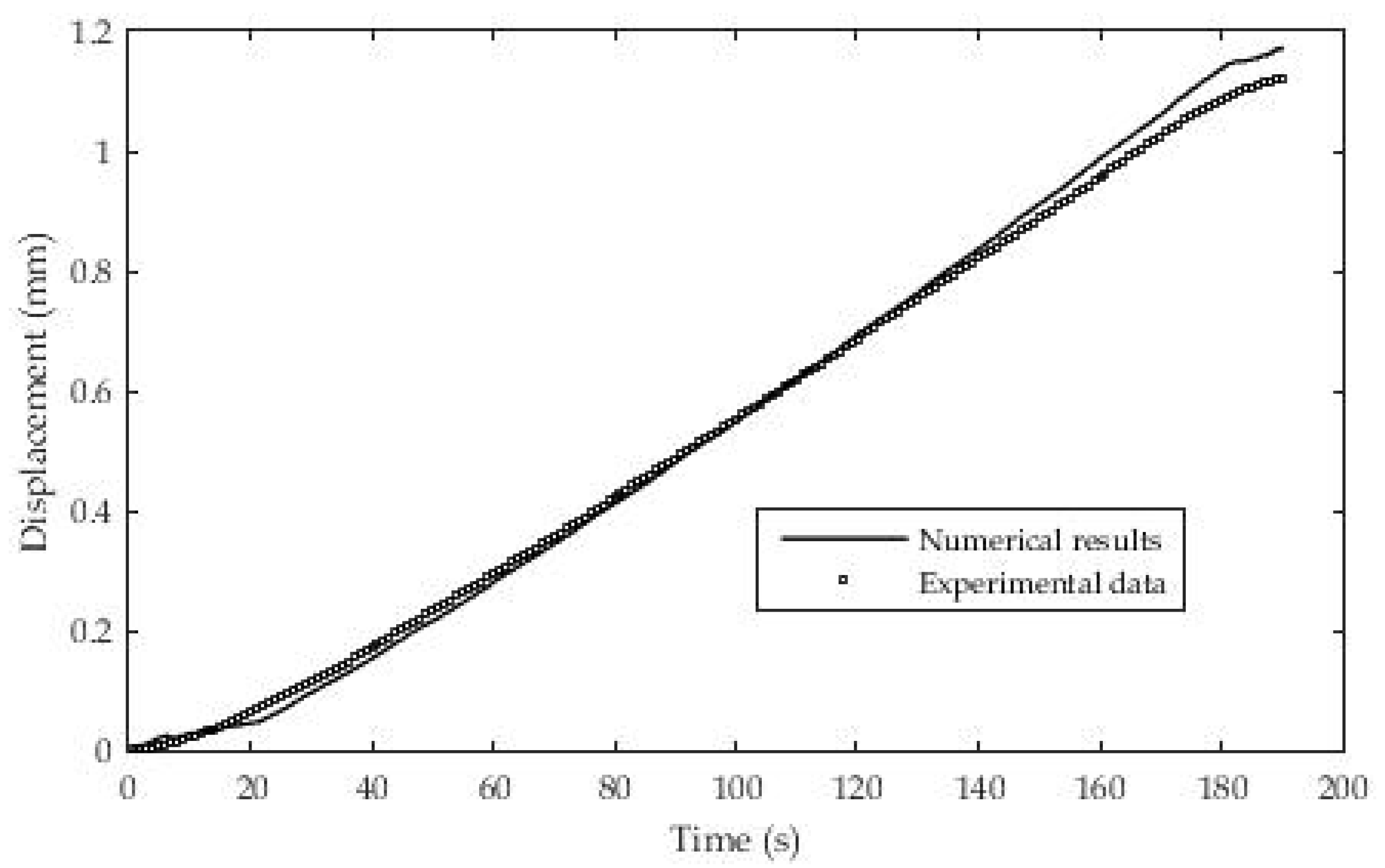
3.2.4. Analysis of Viscoelastic Material with Simultaneous Mechanical and Thermal Loading
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| a, b | coefficients in discretized equations |
| aT | shift factor |
| c | specific heat |
| d | location vector |
| e | internal energy |
| E | Young’s relaxation modulus |
| f | force |
| G | shear relaxation modulus |
| I | unit tensor |
| i | Cartesian unit vector |
| J | creep compliance |
| K | volume relaxation modulus |
| k | thermal conductivity |
| P0, Pj | centers of control volumes |
| q | heat flux vector |
| Q, q | source terms |
| r | distance vector |
| n | vector of outer normal |
| t | time |
| t’ | reduced time |
| T | temperature |
| U | total displacement vector |
| u | displacement vector |
| V | volume |
| α | thermal expansion coefficient |
| Ґ | diffused term |
| δt | time increment |
| δT | temperature increment |
| δε | strain increment |
| λ, μ | Lame’s coefficients |
| ρ | density |
| σ | stress tensor |
| τ | incremental time |
| ν | Poisson’s number |
| ω | angular velocity |
| φ | generic variable |
| γ | angular strain |
| Subscripts | |
| B | boundary |
| i | Cartesian components |
| j | cell index (volume) |
| k | index in the Prony series |
| r | radial direction |
| x,y,z | Cartesian coordinates |
| Superscripts | |
| i | time-step counter |
| j | iteration counter |
| T | transpose |
| Abbreviations | |
| DMA | dynamic-mechanic analysis |
| FDM | fused deposition modeling |
| PLA | poly-lactic acid |
| ABS | acrylonitrile butadiene styrene |
| PE | polietilen |
| CFS | closed-form shifting |
| TTSP | time temperature |
| superposition principle | |
| FE | finite element method |
| FVM | finite volume method |
References
- Emri, I.; Gergesova, M. Time-dependent behaviour of solid polymers. In Encyclopedia of Life Support Systems, Rheology, EOLLS; UNESCO: Oxford, UK, 2010; pp. 247–330. [Google Scholar]
- Saprunov, I.; Gergesova, M.; Emri, I. Prediction of viscoelastic material functions from constant stress-or strain-rate experiments. Mech. TimeDepend. Mater. 2013, 18, 349–372. [Google Scholar] [CrossRef]
- Schapery, R.A. On the characterization of non-linear viscoelastic materials. Polym. Eng. Sci. 1969, 9, 295–310. [Google Scholar] [CrossRef]
- Rajagopal, K.R.; Srinivasa, A.R.; Ponnalagu, A. Thermo-Inelastic Response of Polymeric Solids; Technical Report, Final Report; Texas Engineering Experiment Station: College Station, TX, USA, 2014. [Google Scholar]
- Wang, L.; Melnik, R. Finite volume analysis of nonlinear thermo-mechanical dynamics of shape memory alloys. Heat Mass Transf. 2007, 43, 535–546. [Google Scholar] [CrossRef][Green Version]
- Fryer, Y.D.; Bailey, C.; Cross, M.; Lai, C.-H. A control volume procedure for solving the elastic stress-strain equations on an unstructured mesh. Appl. Math. Model. 1991, 15, 639–645. [Google Scholar] [CrossRef]
- Bailey, C.; Cross, M. A finite volume procedure to solve elastic solid mechanics problems in three dimensions on an unstructured mesh. Int. J. Numer. Methods Eng. 1995, 38, 1757–1776. [Google Scholar] [CrossRef]
- Taylor, G.A.; Bailey, C.; Cross, M. Solution of the elastic/visco-plastic constitutive equations: A finite volume approach. Appl. Math. Model. 1995, 19, 746–760. [Google Scholar] [CrossRef]
- Tukovic, Z.; Cardiff, P.; Karac, A.; Jasak, H.; Ivankovic, A. OpenFOAM library for fluid structure interaction. In Proceedings of the 9th OpenFOAM Workshop, Zagreb, Croatia, 23–26 June 2014. [Google Scholar]
- Zarrabi, K.; Basu, A. An axisymmetric finite volume formulation for creep analysis. J. Mech. Behav. Mater. 1999, 10, 325–340. [Google Scholar] [CrossRef]
- Dzaferovic, E.; Ivankovic, A.; Demirdzic, I. Finite volume modelling of linear viscoelastic deformation. In Proceedings of the 3rd Congress of Croatian Society of Mechanics, Dubrovnik, Croatia, 17 December 2000. [Google Scholar]
- Zarrabi, K.; Basu, A. A finite volume element formulation for solution of elastic axisymmetric pressurized components. Int. J. Press. Vessel. Pip. 2000, 77, 479–484. [Google Scholar] [CrossRef]
- Demirdzic, I.; Dzaferovic, E.; Ivankovic, A. Finite-volume approach to thermoviscoelasticity. Numer. Heat Transf. Part B Fundam. 2005, 47, 213–237. [Google Scholar] [CrossRef]
- Safari, A.; Tuković, Z.; Cardiff, P.; Walter, M.; Casey, E.; Ivanković, A. Interfacial separation of a mature biofilm from a glass surface-a combined experimental and cohesive zone modelling approach. J. Mech. Behav. Biomed. Mater. 2016, 54, 205–218. [Google Scholar] [CrossRef] [PubMed]
- Cardiff, P.; Tukovic, Z.; Jasak, H.; Ivankovic, A. A block-coupled Finite Volume methodology for linear elasticity and unstructured meshes. Comput. Struct. 2016, 175, 100–122. [Google Scholar] [CrossRef]
- Das, S.; Mathur, S.R.; Murthy, J.Y. Finite-volume method for creep analysis of thin RF MEMS devices using the theory of plates. Numer. Heat Transf. Part B Fundam. 2012, 61, 71–90. [Google Scholar] [CrossRef]
- Tropsa, V.; Ivankovic, A.; Williams, J.G. Predicting residual stresses due to solidification in cast plastic plates. Plast. Rubber Compos. 2000, 29, 468–474. [Google Scholar] [CrossRef]
- Dzaferovic, E. Interaction Between Viscoplastic Fluid and Viscoelastic Solid Numerical Modelling. Ph.D. Thesis, University of Sarajevo, Sarajevo, Bosnia and Herzegovina, 2002. (In Bosnian). [Google Scholar]
- Obućina, M.; Džaferovic, E.; Resnik, J. Viskoelastična svojstva drveta pri promjeni vlage. Drv. Ind. 2004, 55, 69–76. [Google Scholar]
- Bajramović, R.; Obućina, M.; Džaferović, E.; Resnik, J. Analiza izbora parametara mehaničkih modela pri određivanju viskoelastičnih svojstava materijala. In Proceedings of the 5th International Scientific Conference on Production Engineering RIM, Bihać, Bosnia and Herzegovina, 24 September 2005; pp. 503–508. [Google Scholar]
- Obućina, M.; Dzaferovic, E.; Bajramovic, R.; Resnik, J. Influence of gluing technology on viscoelasticity properties of LVL. Wood Res. 2006, 51, 11–22. [Google Scholar]
- Obucina, M.; Bajramovic, R.; Dzaferovic, E. Estimation of unknown parameters in mechanical models for determination viscoelasticity properties of materials. In Annals of DAAAM for 2008, Proceedings of the 19th International DAAAM Symposium, Trnava, Slovakia, 22–25 October 2008; DAAAM International Vienna: Vienna, Austria, 2008; p. 492. ISBN 978-3-901509-68-1. ISSN 1726-9679. [Google Scholar]
- Obucina, M.; Dzaferović, E.; Ibrulj, J.; Cekic, A.; Kuzman, K.M.; Kariz, M.; Sernek, M. Analysis of the viscoelastic properties of polymeric materials used for 3d printing. In DAAM International Scientific Book; DAAAM International Vienna: Vienna, Austria, 2018; Chapter 8; pp. 83–96. [Google Scholar]
- Emri, I.; Tschoegl, N.W. Determination of mechanical spectra from experimental data. Int. J. Solids Struct. 1995, 32, 817–826. [Google Scholar] [CrossRef]
- Knauss, W.G.; Zhao, J. Improved relaxation time coverage in ramp-strain histories. Mech. Time Depend. Mater. 2007, 11, 199–216. [Google Scholar] [CrossRef]
- Kariz, M.; Sernek, M.; Obućina, M.; Kuzman, M.K. Effect of wood content in FDM filament on properties of 3D printed parts. Mater. Today Commun. 2017, 14, 135–140. [Google Scholar] [CrossRef]
- Ayrilmis, N.; Kariz, M.; Kwon, J.H.; Kuzman, M.K. Effect of printing layer thickness on water absorption and mechanical properties of 3D-printed wood/PLA composite materials. Int. J. Adv. Manuf. Technol. 2019, 102, 2195–2200. [Google Scholar] [CrossRef]
- Kuzman, M.K.; Ayrilmis, N.; Sernek, M.; Kariz, M. Effect of selected printing settings on viscoelastic behaviour of 3D printed polymers with and without wood. Mater. Res. Express 2019, 6, 105362. [Google Scholar] [CrossRef]



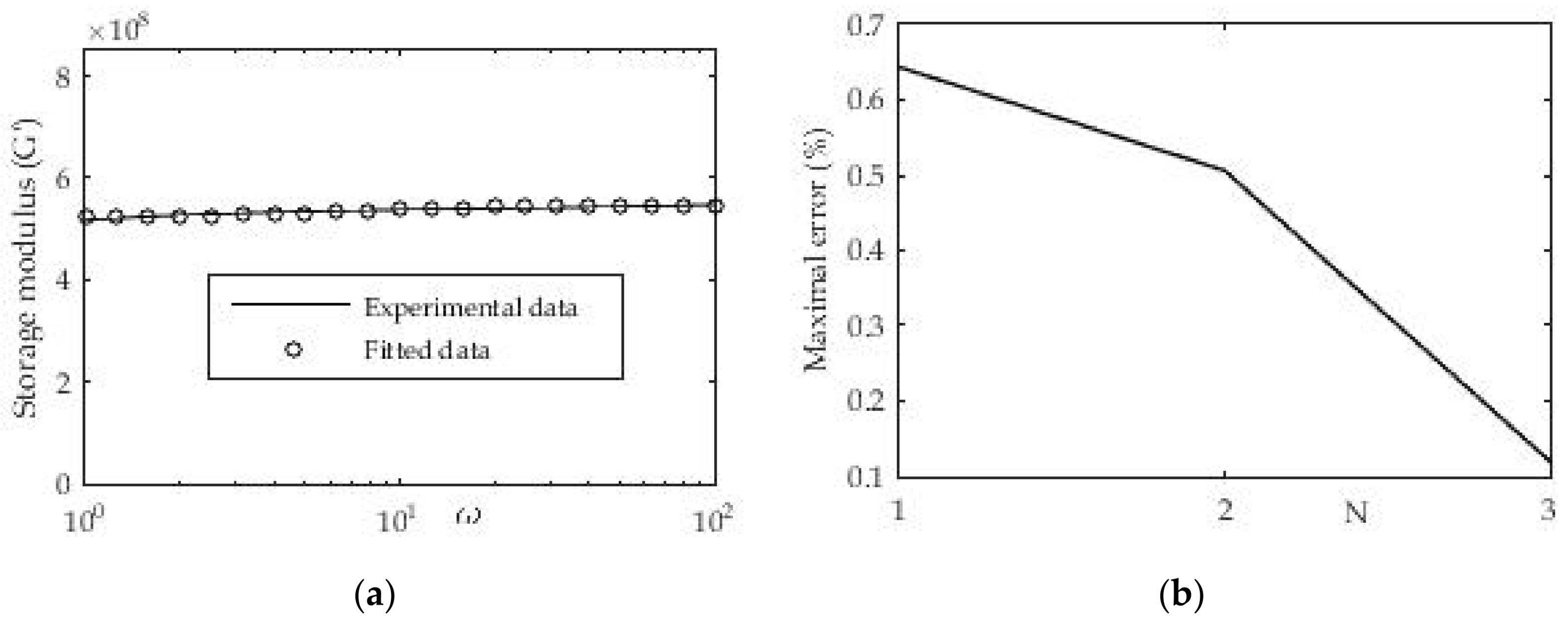
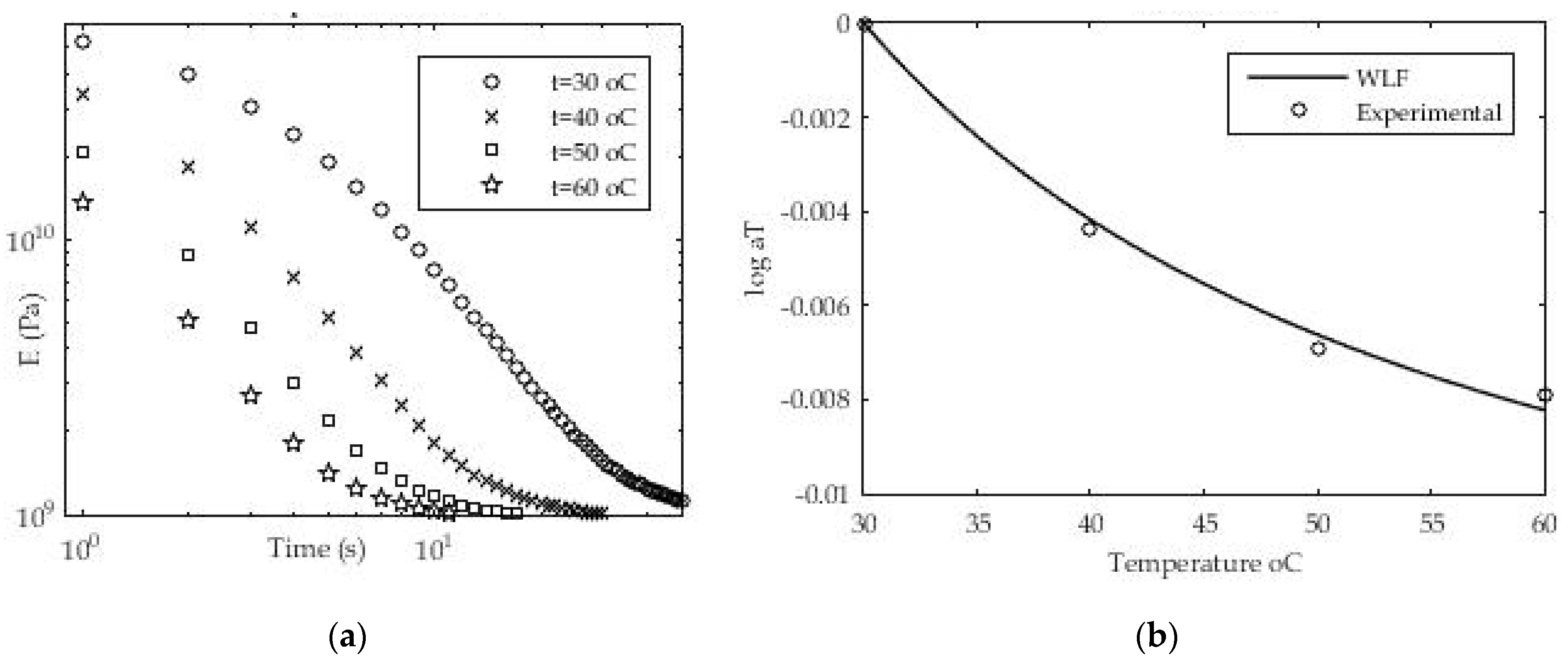


| Number of Terms | Number of Terms | Number of Terms | Number of Terms | |||||
|---|---|---|---|---|---|---|---|---|
| 1 | 3 | 5 | 7 | |||||
| i | Ji | τi | Ji | τi | Ji | τi | Ji | τi |
| 0 | 3.3 × 10−9 | 3.2 × 10−9 | 3.1 × 10−9 | 2.9 × 10−9 | ||||
| 1 | 6.7 × 10−10 | 1.0 × 103 | 1.6 × 10−10 | 1.0 × 101 | 2.3 × 10−11 | 1.0 × 10−1 | 3.8 × 10−11 | 1.0 × 10−3 |
| 2 | 1.3 × 10−12 | 1.0 × 102 | 3.3 × 10−14 | 1.0 × 10−0 | 3.0 × 10−10 | 1.0 × 10−2 | ||
| 3 | 6.5 × 10−10 | 1.0 × 103 | 1.5 × 10−10 | 1.0 × 101 | 5.0 × 10−11 | 1.0 × 10−1 | ||
| 4 | 8.7 × 10−12 | 1.0 × 102 | 4.0 × 10−11 | 1.0 × 10−0 | ||||
| 5 | 6.4 × 10−10 | 1.0 × 103 | 1.3 × 10−11 | 1.0 × 101 | ||||
| 6 | 3.2 × 10−11 | 1.0 × 102 | ||||||
| 7 | 9.2 × 10−10 | 1.0 × 103 | ||||||
| Number of Terms | Number of Terms | Number of Terms | Number of Terms | |
|---|---|---|---|---|
| 1 | 3 | 5 | 7 | |
| Average error (%) | 1.8 | 1.6 | 0.9 | 0.8 |
| Number of Terms | Number of Terms | Number of Terms | Number of Terms | |||||
|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 5 | 10 | |||||
| τi (s) | Ji | τi (s) | Ji | τi (s) | Ji | τi (s) | Ji | |
| 0 | 1.00 × 10+9 | 9.85 × 10+8 | 9.46 × 10+8 | 9.25× 10+8 | ||||
| 1 | 10.95 | 2.25 × 10+8 | 4.93 | 3.98 × 10−1 | 2.22 | 7.82 × 10+7 | 1.55 | 1.28 × 10+7 |
| 2 | 24.33 | 1.94 × 10+8 | 4.93 | 2.53 × 10−1 | 2.39 | 1.21 × 10+7 | ||
| 3 | 10.95 | 4.92 × 10−1 | 3.69 | 1.89 × 10+7 | ||||
| 4 | 24.33 | 1.96 × 10−1 | 5.70 | 2.57 × 10+7 | ||||
| 5 | 54.03 | 1.89 × 10+8 | 8.81 | 2.92 × 10+4 | ||||
| 6 | 13.62 | 4.90 × 10+6 | ||||||
| 7 | 21.04 | 1.20 × 10+7 | ||||||
| 8 | 32.52 | 2.00 × 10+6 | ||||||
| 9 | 50.25 | 1.44 × 10+6 | ||||||
| 10 | 77.65 | 1.82 × 10+8 | ||||||
| Number of Terms | Number of Terms | Number of Terms | Number of Terms | |||||
|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 5 | 10 | |||||
| τi (s) | Ei (Pa) | τi (s) | Ei (Pa) | τi (s) | Ei (Pa) | τi (s) | Ei (Pa) | |
| 0 | 1.00 × 10+9 | 9.85 × 10+8 | 9.46 × 10+8 | 9.25× 10+8 | ||||
| 1 | 10.95 | 2.25 × 10+8 | 4.93 | 3.98 × 10−1 | 2.22 | 7.82 × 10+7 | 1.55 | 1.28 × 10+7 |
| 2 | 24.33 | 1.94 × 10+8 | 4.93 | 2.53 × 10−1 | 2.39 | 1.21 × 10+7 | ||
| 3 | 10.95 | 4.92 × 10−1 | 3.69 | 1.89 × 10+7 | ||||
| 4 | 24.33 | 1.96 × 10−1 | 5.70 | 2.57 × 10+7 | ||||
| 5 | 54.03 | 1.89 × 10+8 | 8.81 | 2.92 × 10+4 | ||||
| 6 | 13.62 | 4.90 × 10+6 | ||||||
| 7 | 21.04 | 1.20 × 10+7 | ||||||
| 8 | 32.52 | 2.00 × 10+6 | ||||||
| 9 | 50.25 | 1.44 × 10+6 | ||||||
| 10 | 77.65 | 1.82 × 10+8 | ||||||
| Coefficients of Prony Series | ||||||
|---|---|---|---|---|---|---|
| 1 Term | 2 Terms | 3 Terms | ||||
| i | Gi | τi | Gi | τi | Gi | τi |
| 0 | 5.6 × 10+8 | 5.6 × 10+8 | 5.7 × 10+8 | |||
| 1 | 1.5 × 10+7 | 1.0 × 10−1 | 1.1 × 10+7 | 3.3 × 10−1 | 1.5 × 10+9 | 2.4 × 10−5 |
| 2 | 1.0 × 10+7 | 3.1 × 10−2 | 1.5 × 10+7 | 6.4 × 10−2 | ||
| 3 | 5.7 × 10+5 | 3.2 × 10+2 | ||||
| Density ρ (kg/m3) | Heat Conductivity λt (W/m2K) | Linear Thermal Expansion α (K−1) |
|---|---|---|
| 900 | 0.1 | 2.2× 10−4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ibrulj, J.; Dzaferovic, E.; Obucina, M.; Kuzman, M.K. Numerical and Experimental Investigations of Polymer Viscoelastic Materials Obtained by 3D Printing. Polymers 2021, 13, 3276. https://doi.org/10.3390/polym13193276
Ibrulj J, Dzaferovic E, Obucina M, Kuzman MK. Numerical and Experimental Investigations of Polymer Viscoelastic Materials Obtained by 3D Printing. Polymers. 2021; 13(19):3276. https://doi.org/10.3390/polym13193276
Chicago/Turabian StyleIbrulj, Jusuf, Ejub Dzaferovic, Murco Obucina, and Manja Kitek Kuzman. 2021. "Numerical and Experimental Investigations of Polymer Viscoelastic Materials Obtained by 3D Printing" Polymers 13, no. 19: 3276. https://doi.org/10.3390/polym13193276
APA StyleIbrulj, J., Dzaferovic, E., Obucina, M., & Kuzman, M. K. (2021). Numerical and Experimental Investigations of Polymer Viscoelastic Materials Obtained by 3D Printing. Polymers, 13(19), 3276. https://doi.org/10.3390/polym13193276








