A Phenomenological Primary–Secondary–Tertiary Creep Model for Polymer-Bonded Composite Materials
Abstract
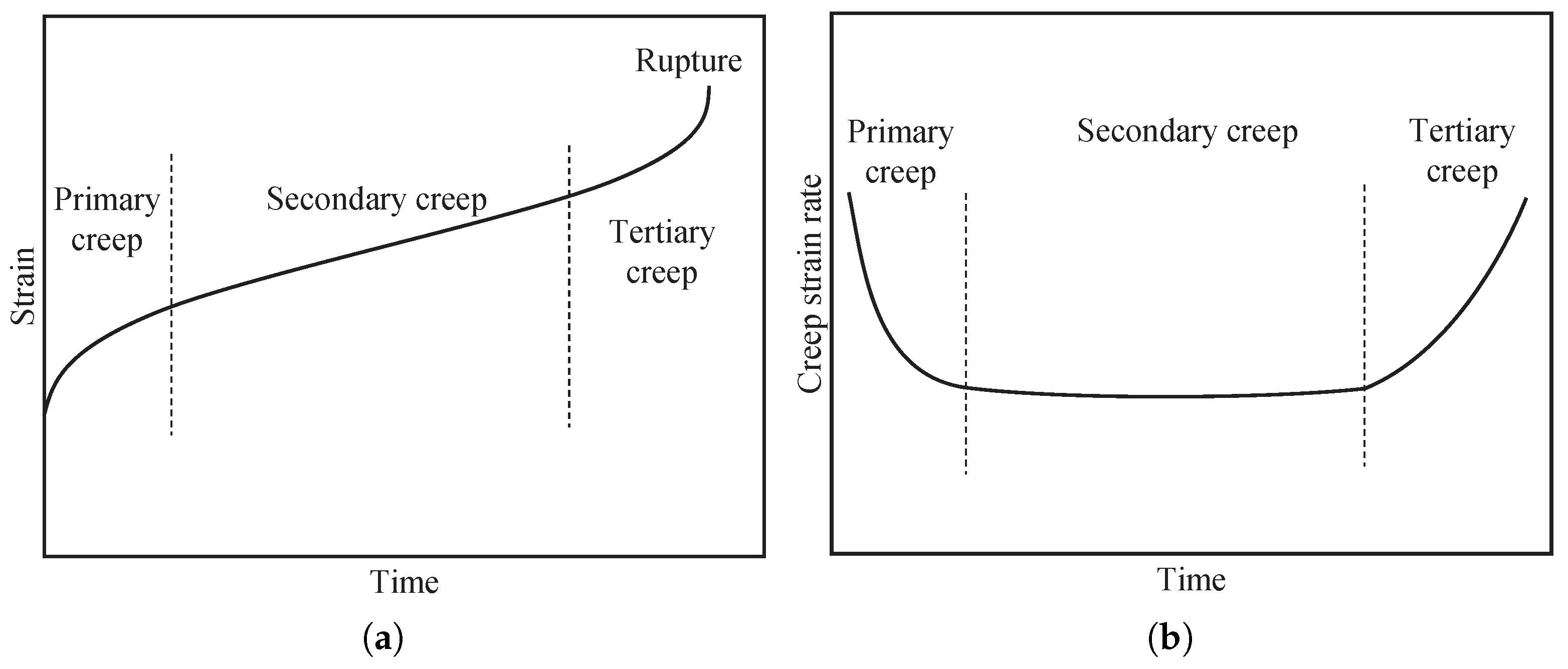
:1. Introduction
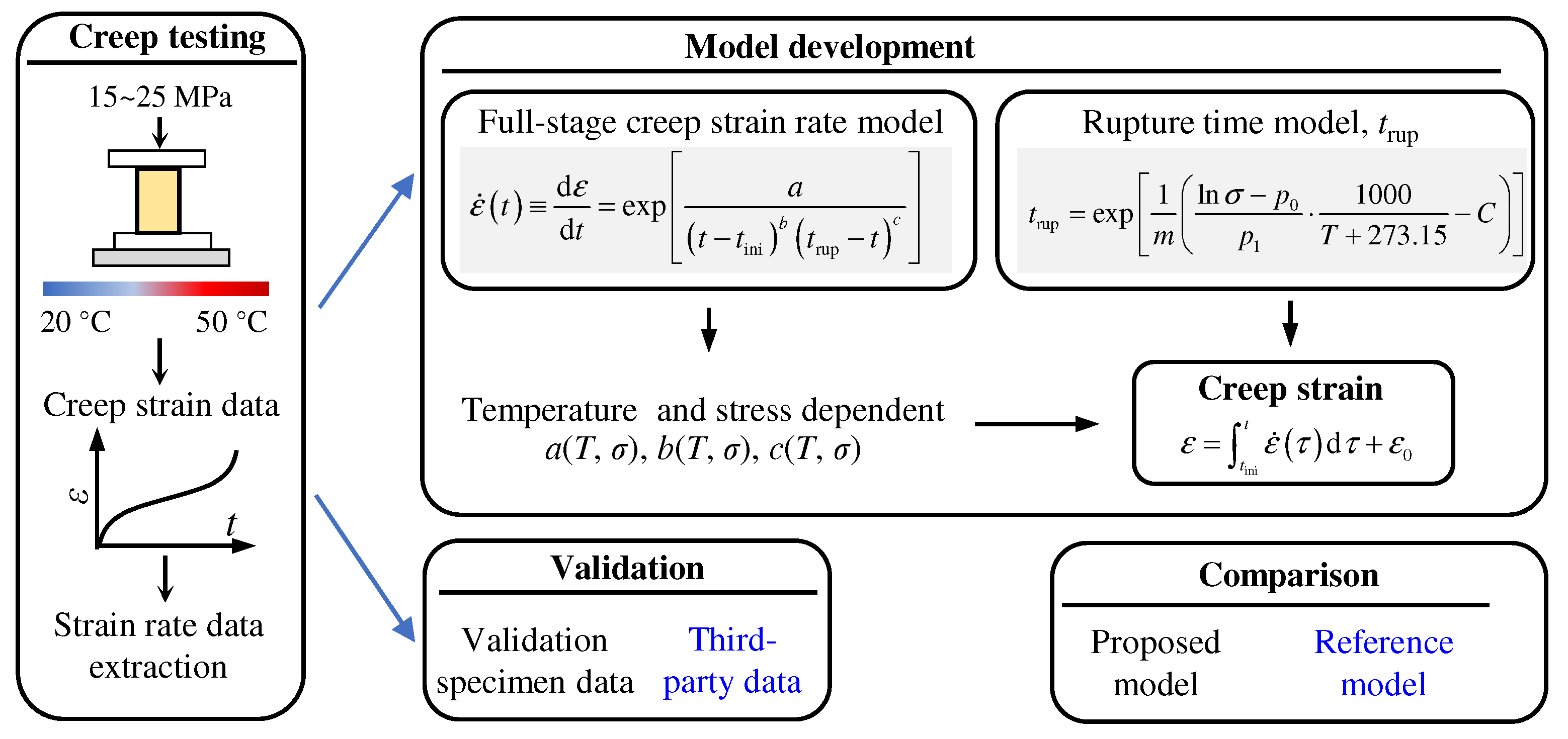
2. Experimental Testing
2.1. Specimens and Experimental Setup
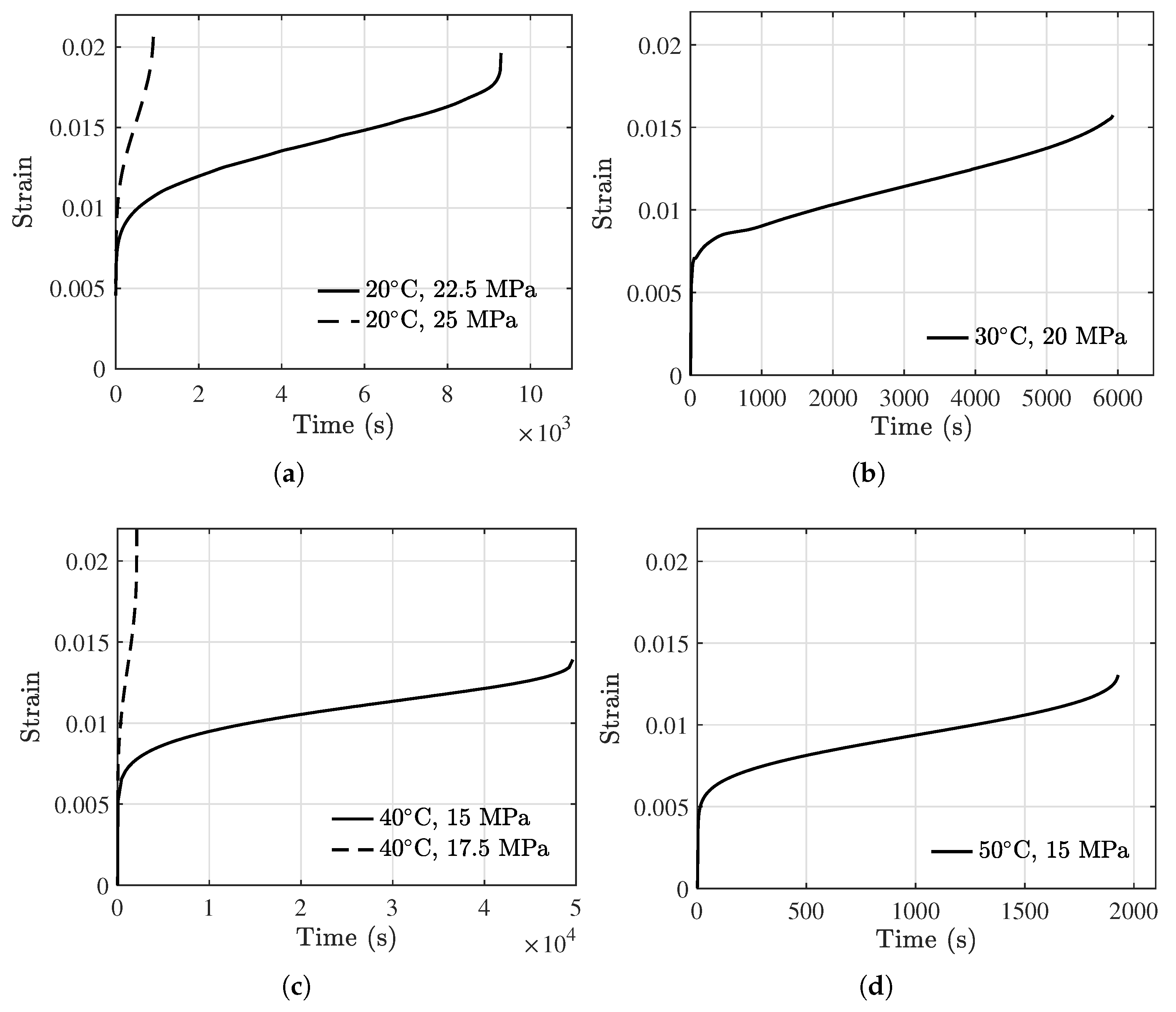
2.2. Creep Testing Results
2.3. Strain Rate Extraction
3. Creep Model Development
3.1. Creep Strain Rate Model
3.2. Temperature and Stress Dependence Model
3.3. Creep Rupture Time Model
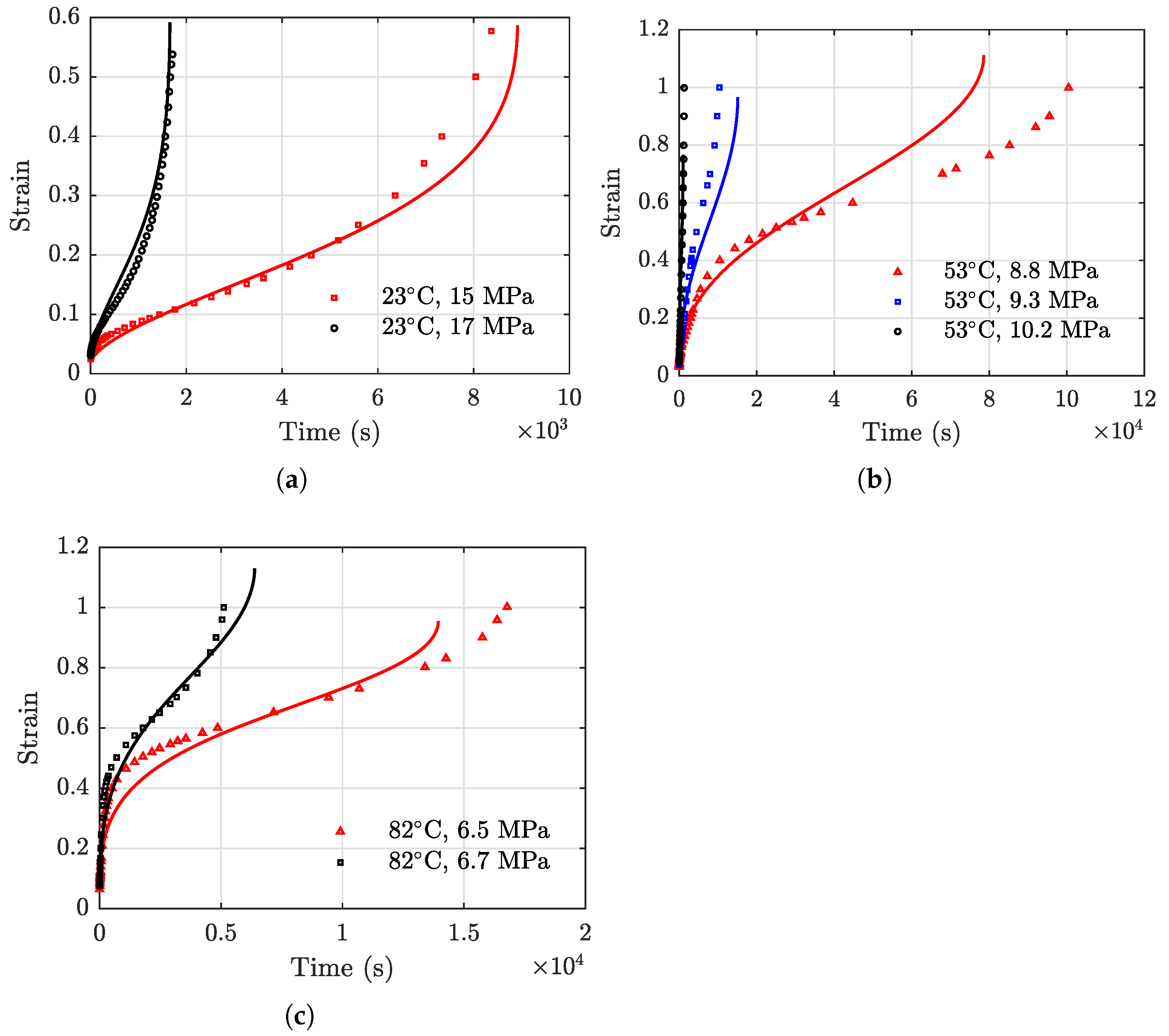
4. Model Validations and Comparisons
4.1. Model Validation
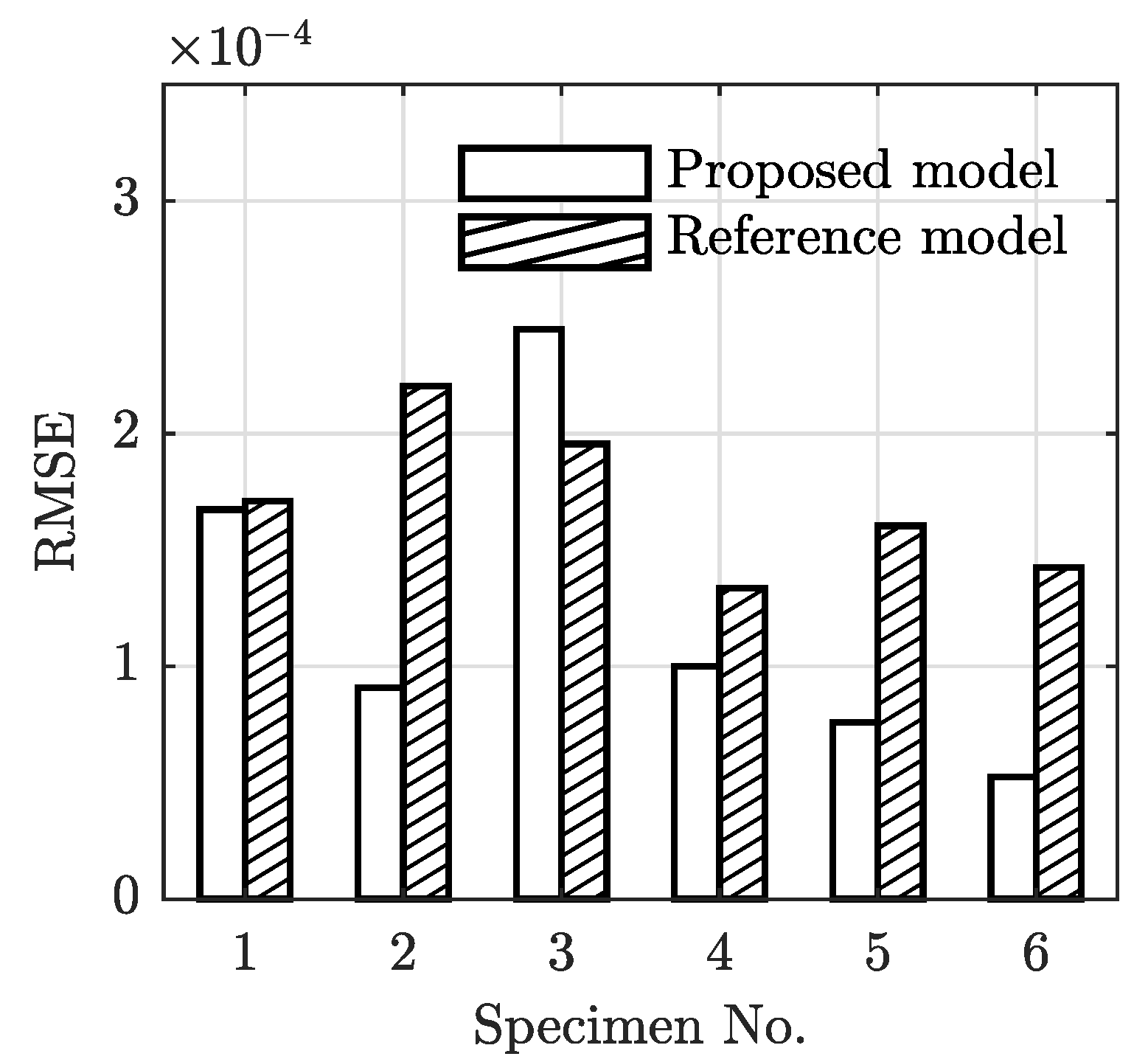
4.2. Model Comparisons
5. Conclusions
- The developed unified phenomenological creep model can describe the full primary–secondary–tertiary creep process under more general conditions of temperature and stress. The effectiveness of the model was validated using both independent and third-party testing data.
- The Larson–Miller parameter can be used for predicting the rupture time of PBMs. Combined with the proposed strain rate model, it can be used to predict the creep behavior under more general conditions without testing data on rupture life.
- The developed model was compared with an existing reference model. Results show that the developed model is more accurate in terms of root mean squared error. For the testing data used in this study, the proposed model reduces the overall errors by 28.5%. In addition, the proposed model is more reliable for the tertiary creep prediction due to the monotonic strain rate equation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| PBMs | Polymer-bonded composites materials |
| CT | Computed tomography |
| RMSE | Root mean square error |
References
- Odqvist, F. Historical survey of the development of creep mechanics from its beginnings in the last century to 1970. In Creep in Structures; Springer: Berlin/Heidelberg, Germany, 1981; pp. 1–12. [Google Scholar]
- Bochnia, J.; Blasiak, S. Stress Relaxation and Creep of a Polymer-Aluminum Composite Produced through Selective Laser Sintering. Polymers 2020, 12, 830. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rebeiz, K. Time-temperature properties of polymer concrete using recycled PET. Cem. Concr. Compos. 1995, 17, 119–124. [Google Scholar] [CrossRef]
- Scheerbaum, N.; Hinz, D.; Gutfleisch, O.; Müller, K.H.; Schultz, L. Textured polymer bonded composites with Ni–Mn–Ga magnetic shape memory particles. Acta Mater. 2007, 55, 2707–2713. [Google Scholar] [CrossRef]
- Lin, C.; Liu, J.; Huang, Z.; Gong, F.; Li, Y.; Pan, L.; Zhang, J.; Liu, S. Enhancement of Creep Properties of TATB-Based Polymer-Bonded Explosive Using Styrene Copolymer. Propellants Explos. Pyrotech. 2015, 40, 189–196. [Google Scholar] [CrossRef]
- Fulmali, A.O.; Sen, B.; Ray, B.C.; Prusty, R.K. Effects of carbon nanotube/polymer interfacial bonding on the long-term creep performance of nanophased glass fiber/epoxy composites. Polym. Compos. 2020, 41, 478–493. [Google Scholar] [CrossRef]
- Shi, X.B.; Wu, C.L.; Rong, M.Z.; Czigany, T.; Ruan, W.H.; Zhang, M.Q. Improvement of creep resistance of polytetrafluoroethylene films by nano-inclusions. Chin. J. Polym. Sci. 2013, 31, 377–387. [Google Scholar] [CrossRef]
- Rehman, M.M.; Zeeshan, M.; Shaker, K.; Nawab, Y. Effect of micro-crystalline cellulose particles on mechanical properties of alkaline treated jute fabric reinforced green epoxy composite. Cellulose 2019, 26, 9057–9069. [Google Scholar] [CrossRef]
- Nuñez, A.J.; Marcovich, N.E.; Aranguren, M.I. Analysis of the creep behavior of polypropylene-woodflour composites. Polym. Eng. Sci. 2004, 44, 1594–1603. [Google Scholar] [CrossRef]
- Starkova, O.; Yang, J.; Zhang, Z. Application of time–stress superposition to nonlinear creep of polyamide 66 filled with nanoparticles of various sizes. Compos. Sci. Technol. 2007, 67, 2691–2698. [Google Scholar] [CrossRef] [Green Version]
- Fu, S.Y.; Feng, X.Q.; Lauke, B.; Mai, Y.W. Effects of particle size, particle/matrix interface adhesion and particle loading on mechanical properties of particulate–polymer composites. Compos. Part B Eng. 2008, 39, 933–961. [Google Scholar] [CrossRef]
- Lin, C.; Liu, J.; He, G.; Yang, Z.; Pan, L.; Liu, S.; Li, J.; Guo, S. Effect of crystal quality and particle size of HMX on the creep resistance for TATB/HMX composites. Propellants Explos. Pyrotech. 2017, 42, 1410–1417. [Google Scholar] [CrossRef]
- Gong, L.X.; Pei, Y.B.; Han, Q.Y.; Zhao, L.; Wu, L.B.; Jiang, J.X.; Tang, L.C. Polymer grafted reduced graphene oxide sheets for improving stress transfer in polymer composites. Compos. Sci. Technol. 2016, 134, 144–152. [Google Scholar] [CrossRef]
- Shi, J.; Wang, X.; Wu, Z.; Zhu, Z. Creep behavior enhancement of a basalt fiber-reinforced polymer tendon. Constr. Build. Mater. 2015, 94, 750–757. [Google Scholar] [CrossRef]
- Jo, B.W.; Tae, G.H.; Kim, C.H. Uniaxial creep behavior and prediction of recycled-PET polymer concrete. Constr. Build. Mater. 2007, 21, 1552–1559. [Google Scholar] [CrossRef]
- Eftekhari, M.; Fatemi, A. Tensile, creep and fatigue behaviours of short fibre reinforced polymer composites at elevated temperatures: A literature survey. Fatigue Fract. Eng. Mater. Struct. 2015, 38, 1395–1418. [Google Scholar] [CrossRef]
- Yang, T.C.; Wu, T.L.; Hung, K.C.; Chen, Y.L.; Wu, J.H. Mechanical properties and extended creep behavior of bamboo fiber reinforced recycled poly (lactic acid) composites using the time–temperature superposition principle. Constr. Build. Mater. 2015, 93, 558–563. [Google Scholar] [CrossRef]
- Sun, T.; Yu, C.; Yang, W.; Zhong, J.; Xu, Q. Experimental and numerical research on the nonlinear creep response of polymeric composites under humid environments. Compos. Struct. 2020, 251, 112673. [Google Scholar] [CrossRef]
- Eftekhari, M.; Fatemi, A. Tensile behavior of thermoplastic composites including temperature, moisture, and hygrothermal effects. Polym. Test. 2016, 51, 151–164. [Google Scholar] [CrossRef]
- Khotbehsara, M.M.; Manalo, A.; Aravinthan, T.; Ferdous, W.; Nguyen, K.T.; Hota, G. Ageing of particulate-filled epoxy resin under hygrothermal conditions. Constr. Build. Mater. 2020, 249, 118846. [Google Scholar] [CrossRef]
- Khotbehsara, M.M.; Manalo, A.; Aravinthan, T.; Turner, J.; Ferdous, W.; Hota, G. Effects of ultraviolet solar radiation on the properties of particulate-filled epoxy based polymer coating. Polym. Degrad. Stab. 2020, 181, 109352. [Google Scholar] [CrossRef]
- Hung, K.C.; Wu, T.L.; Chen, Y.L.; Wu, J.H. Assessing the effect of wood acetylation on mechanical properties and extended creep behavior of wood/recycled-polypropylene composites. Constr. Build. Mater. 2016, 108, 139–145. [Google Scholar] [CrossRef]
- Park, B.D.; Balatinecz, J.J. Short term flexural creep behavior of wood-fiber/polypropylene composites. Polym. Compos. 1998, 19, 377–382. [Google Scholar] [CrossRef]
- Zhang, W.; Joshi, A.; Wang, Z.; Kane, R.; Koratkar, N. Creep mitigation in composites using carbon nanotube additives. Nanotechnology 2007, 18, 185703. [Google Scholar] [CrossRef]
- Bihari, B.K.; Rao, N.P.; Gupta, M.; Murthy, K. A study on creep behavior of composite solid propellants using the kelvin-Voigt model. Cent. Eur. J. Energ. Mater. 2017, 14, 742–756. [Google Scholar] [CrossRef]
- Wang, W.H.; Huang, H.B.; Du, H.H.; Wang, H. Effects of fiber size on short-term creep behavior of wood fiber/HDPE composites. Polym. Eng. Sci. 2015, 55, 693–700. [Google Scholar] [CrossRef]
- Wu, C.; Wu, R.; Xia, W.; Tam, L. Understanding Creep Behavior of Semicrystalline Polymer via Coarse-Grained Modeling. J. Polym. Sci. Part B Polym. Phys. 2019, 57, 1779–1791. [Google Scholar] [CrossRef]
- Houshyar, S.; Shanks, R.; Hodzic, A. Tensile creep behaviour of polypropylene fibre reinforced polypropylene composites. Polym. Test. 2005, 24, 257–264. [Google Scholar] [CrossRef]
- Naumenko, K.; Altenbach, H. Modeling of Creep for Structural Analysis; Springer Science & Business Media: Cham, Switzerland, 2007. [Google Scholar]
- Norton, F.H. The Creep of Steel at High Temperatures; McGraw-Hill Book Company, Incorporated: New York, NY, USA, 1929. [Google Scholar]
- Bailey, R. Creep of steel under simple and compound stress. Engineering 1930, 121, 265. [Google Scholar]
- Soderberg, C. The interpretation of creep tests for machine design. Trans. ASME 1936, 58, 733–743. [Google Scholar]
- Prandtl, L. A conceptual model to the kinetic theory of solid bodies. Z. Angew. Math. Mech 1928, 8, 85–106. [Google Scholar] [CrossRef]
- Nadai, A. On the creep of solids at elevated temperature. J. Appl. Phys. 1937, 8, 418–432. [Google Scholar] [CrossRef]
- Johnson, A.E.; Khan, B. Creep of metallic thick-walled spherical vessels subject to pressure and radial thermal gradient at elevated temperatures. Int. J. Mech. Sci. 1963, 5, 507–532. [Google Scholar] [CrossRef]
- Garofalo, F. An empirical relation defining the stress dependence of minimum creep rate in metals. Trans. AIME 1963, 227, 351–356. [Google Scholar]
- Sivasithamparam, N.; Karstunen, M.; Bonnier, P. Modelling creep behaviour of anisotropic soft soils. Comput. Geotech. 2015, 69, 46–57. [Google Scholar] [CrossRef] [Green Version]
- Shokuhfar, A.; Zare-Shahabadi, A.; Atai, A.A.; Ebrahimi-Nejad, S.; Termeh, M. Predictive modeling of creep in polymer/layered silicate nanocomposites. Polym. Test. 2012, 31, 345–354. [Google Scholar] [CrossRef]
- Xu, F.; Jones, I.A.; Li, S. A continuum damage model for transverse cracking in UD composites of linear viscoelastic behaviour. Compos. Struct. 2019, 225, 110812. [Google Scholar] [CrossRef]
- Li, K.; Gao, X.L.; Roy, A. Micromechanical modeling of viscoelastic properties of carbon nanotube-reinforced polymer composites. Mech. Adv. Mater. Struct. 2006, 13, 317–328. [Google Scholar] [CrossRef]
- He, G.; Liu, Y.; Hammi, Y.; Bammann, D.; Horstemeyer, M. A combined viscoelasticity-viscoplasticity-anisotropic damage model with evolving internal state variables applied to fiber reinforced polymer composites. Mech. Adv. Mater. Struct. 2020, 28, 1–22. [Google Scholar] [CrossRef]
- Li, Y.; Kessler, M.R. Creep-resistant behavior of self-reinforcing liquid crystalline epoxy resins. Polymer 2014, 55, 2021–2027. [Google Scholar] [CrossRef] [Green Version]
- Fang, C.; Sun, H.Y.; Gu, J.P. Application of fractional calculus methods to viscoelastic response of amorphous shape memory polymers. J. Mech. 2015, 31, 427–432. [Google Scholar] [CrossRef]
- Rubio-López, A.; Hoang, T.; Santiuste, C. Constitutive model to predict the viscoplastic behaviour of natural fibres based composites. Compos. Struct. 2016, 155, 8–18. [Google Scholar] [CrossRef]
- He, G.; Gong, F.; Liu, J.; Pan, L.; Zhang, J.; Liu, S. Improved mechanical properties of highly explosive-filled polymer composites through graphene nanoplatelets. Polym. Compos. 2018, 39, 3924–3934. [Google Scholar] [CrossRef]
- Khan, A.; Zhang, H. Finite deformation of a polymer: Experiments and modeling. Int. J. Plast. 2001, 17, 1167–1188. [Google Scholar] [CrossRef]
- Sadigh, M.S.; Paygozar, B.; da Silva, L.; Tahami, F.V. Creep deformation simulation of adhesively bonded joints at different temperature levels using a modified power-law model. Polym. Test. 2019, 79, 106087. [Google Scholar] [CrossRef]
- Chevali, V.S.; Dean, D.R.; Janowski, G.M. Flexural creep behavior of discontinuous thermoplastic composites: Non-linear viscoelastic modeling and time–temperature–stress superposition. Compos. Part A Appl. Sci. Manuf. 2009, 40, 870–877. [Google Scholar] [CrossRef]
- Osman, A.S.; Birchall, T.J.; Rouainia, M. A simple model for tertiary creep in geomaterials. Geotech. Res. 2019, 7, 26–39. [Google Scholar] [CrossRef] [Green Version]
- Kränkel, T.; Lowke, D.; Gehlen, C. Prediction of the creep behaviour of bonded anchors until failure–A rheological approach. Constr. Build. Mater. 2015, 75, 458–464. [Google Scholar] [CrossRef]
- Sudduth, R.D. Indications that the yield point at constant strain rate and the inception of tertiary creep are manifestations of the same failure criterion using the universal viscoelastic model. J. Mater. Sci. 2003, 38, 1123–1134. [Google Scholar] [CrossRef]
- GJB 772A-1997. Explosive Test Method; China Ordnance Industrial Standardization Research Institute: Beijing, China, 1997. [Google Scholar]
- Duan, X.; Yuan, H.; Tang, W.; He, J.; Guan, X. A General Temperature-Dependent Stress–Strain Constitutive Model for Polymer-Bonded Composite Materials. Polymers 2021, 13, 1393. [Google Scholar] [CrossRef]
- Larson, F.R. A time-temperature relationship for rupture and creep stresses. Trans. ASME 1952, 74, 765–775. [Google Scholar]
- Amjadi, M.; Fatemi, A. Creep behavior and modeling of high-density polyethylene (HDPE). Polym. Test. 2021, 94, 107031. [Google Scholar] [CrossRef]

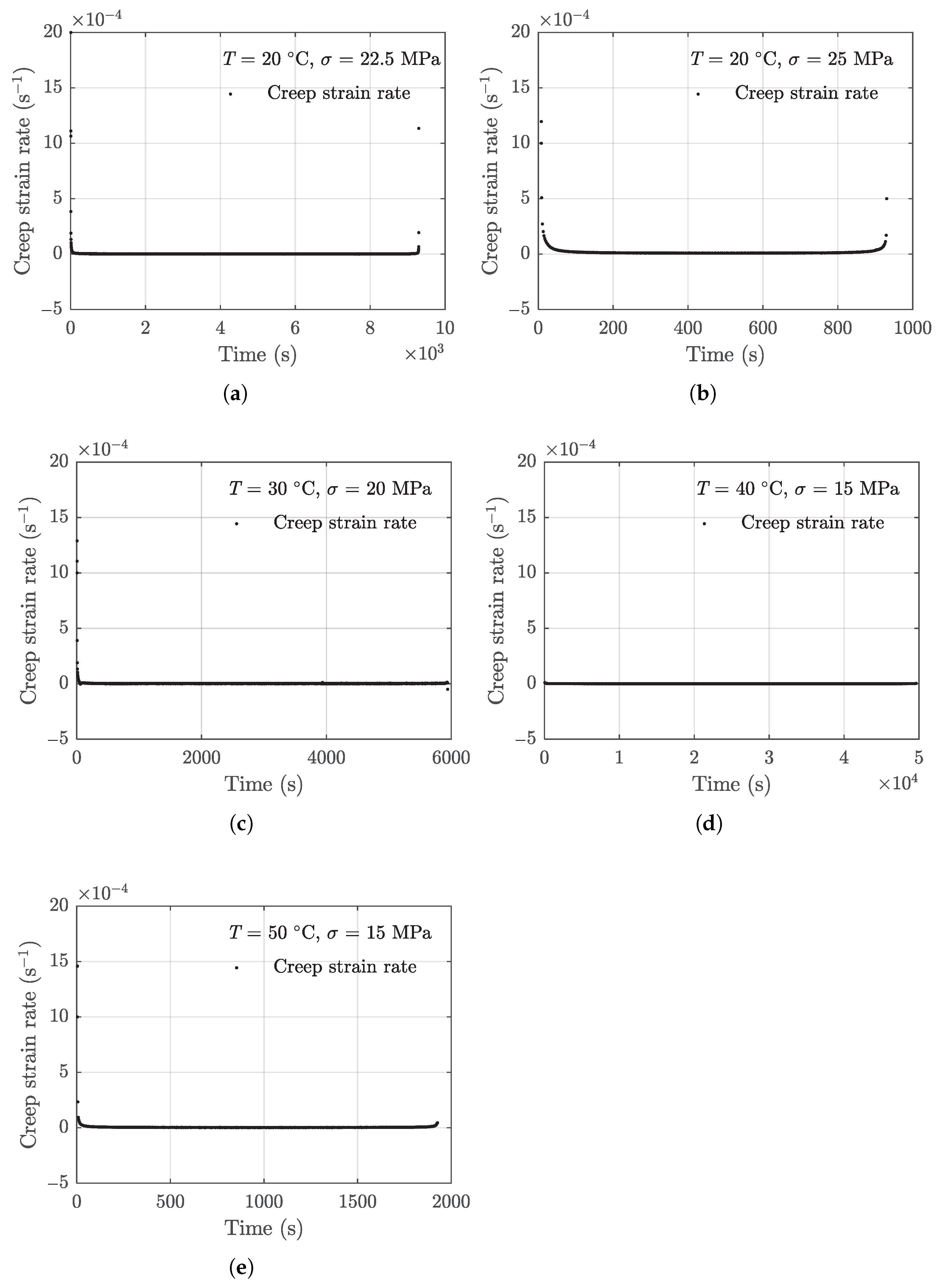




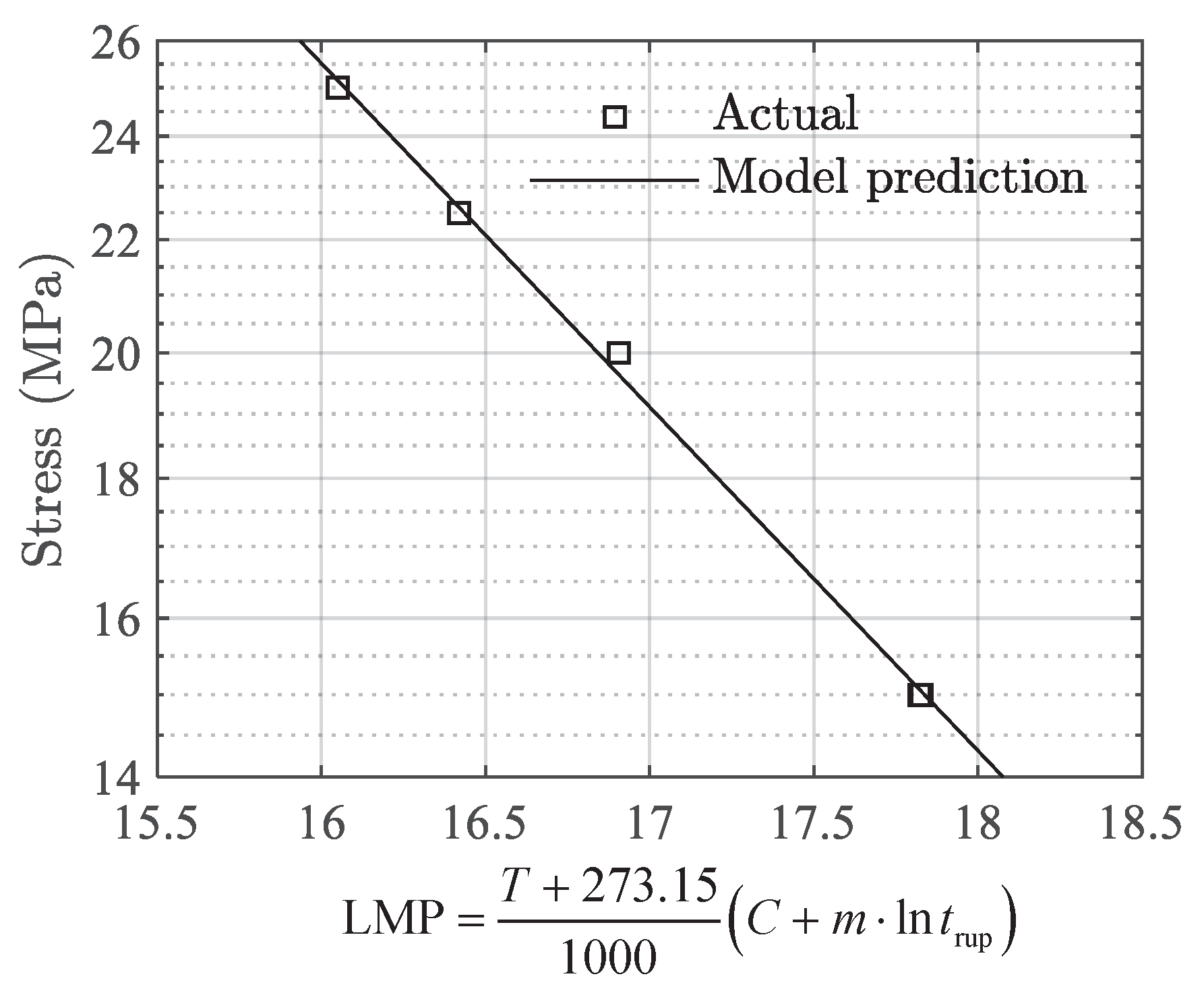
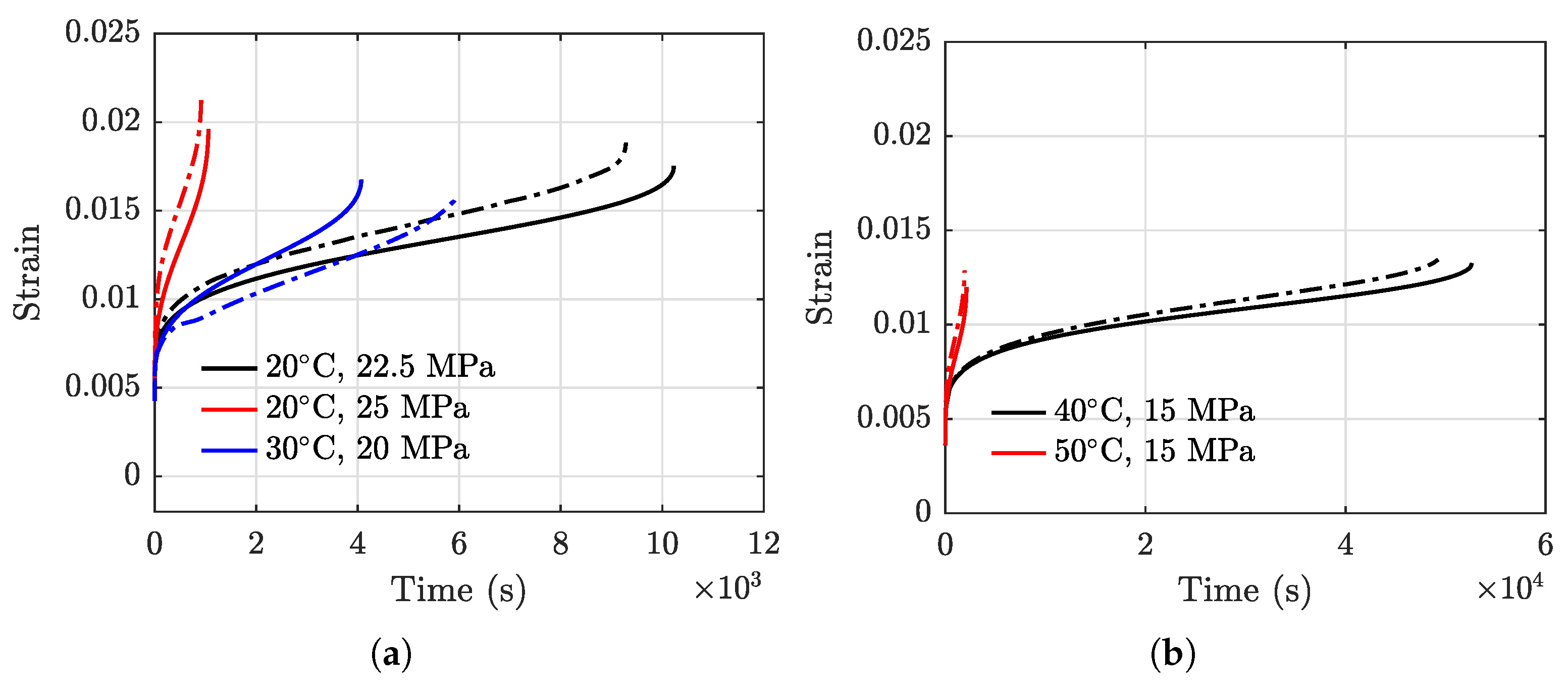
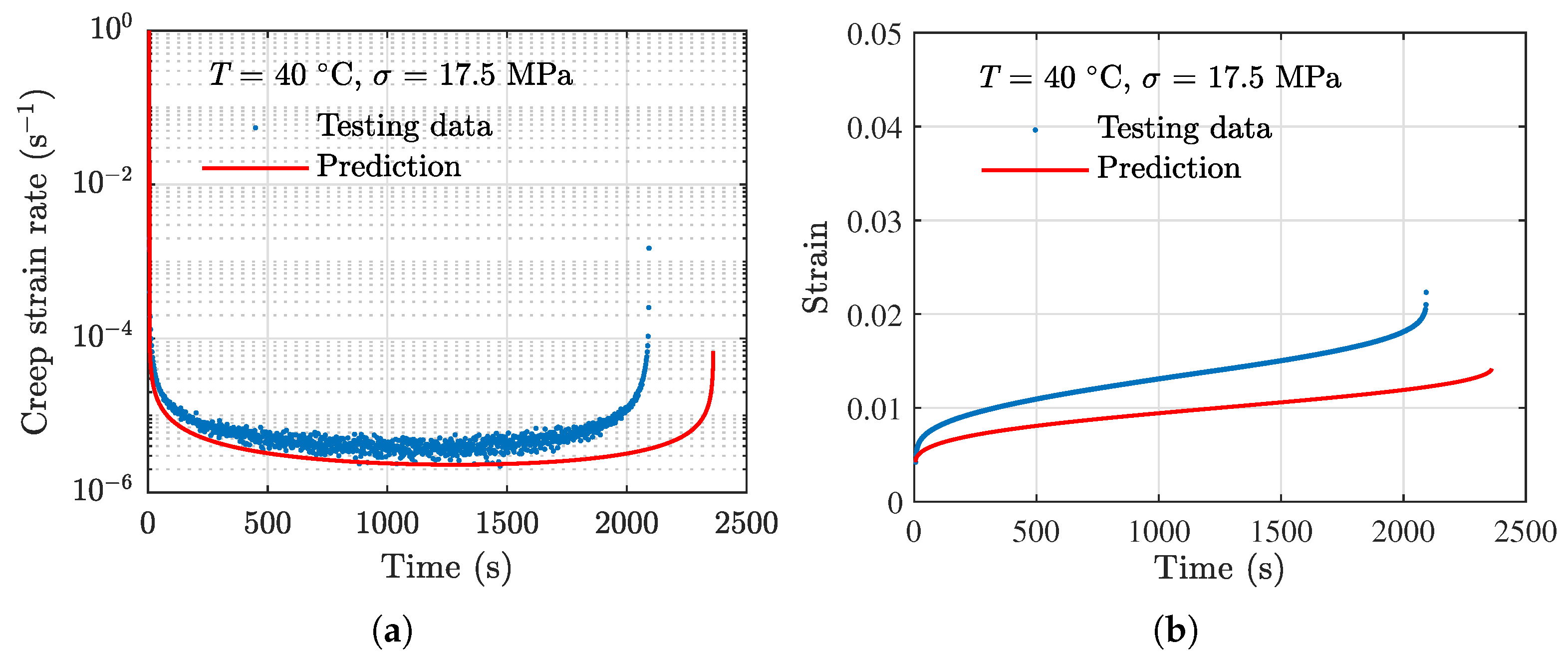

| No. | T (°C) | (MPa) | Usage |
|---|---|---|---|
| 1 | 20 | 22.5 | Modeling |
| 2 | 20 | 25 | Modeling |
| 3 | 30 | 20 | Modeling |
| 4 | 40 | 15 | Modeling |
| 5 | 40 | 17.5 | Validation |
| 6 | 50 | 15 | Modeling |
| No. | T (°C) | (MPa) | a | b | c | (s) | (s) |
|---|---|---|---|---|---|---|---|
| 1 | 20 | 22.5 | −5.763 | −0.6108 | −0.04702 | 7.491 | 9299 |
| 2 | 20 | 25 | −5.052 | −0.07327 | −0.06429 | 8.127 | 930.9 |
| 3 | 30 | 20 | −7.200 | −0.04438 | −0.03786 | 6.406 | 5944 |
| 4 | 40 | 15 | −6.334 | −0.05687 | −0.03744 | 37.00 | 49637 |
| 6 | 50 | 15 | −5.671 | −0.06509 | −0.05838 | 4.945 | 1516 |
| T (°C) | (MPa) | RMSE |
|---|---|---|
| 20 | 22.5 | 0.001073 |
| 20 | 25 | 0.002222 |
| 30 | 20 | 0.002700 |
| 40 | 15 | |
| 50 | 15 |
| T (°C) | (MPa) | RMSE |
|---|---|---|
| 23 | 15 | 0.03264 |
| 23 | 17 | 0.03817 |
| 53 | 8.8 | 0.1196 |
| 53 | 9.3 | 0.09552 |
| 53 | 10.2 | 0.009159 |
| 82 | 6.5 | 0.07257 |
| 82 | 6.7 | 0.05441 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duan, X.; Yuan, H.; Tang, W.; He, J.; Guan, X. A Phenomenological Primary–Secondary–Tertiary Creep Model for Polymer-Bonded Composite Materials. Polymers 2021, 13, 2353. https://doi.org/10.3390/polym13142353
Duan X, Yuan H, Tang W, He J, Guan X. A Phenomenological Primary–Secondary–Tertiary Creep Model for Polymer-Bonded Composite Materials. Polymers. 2021; 13(14):2353. https://doi.org/10.3390/polym13142353
Chicago/Turabian StyleDuan, Xiaochang, Hongwei Yuan, Wei Tang, Jingjing He, and Xuefei Guan. 2021. "A Phenomenological Primary–Secondary–Tertiary Creep Model for Polymer-Bonded Composite Materials" Polymers 13, no. 14: 2353. https://doi.org/10.3390/polym13142353
APA StyleDuan, X., Yuan, H., Tang, W., He, J., & Guan, X. (2021). A Phenomenological Primary–Secondary–Tertiary Creep Model for Polymer-Bonded Composite Materials. Polymers, 13(14), 2353. https://doi.org/10.3390/polym13142353






