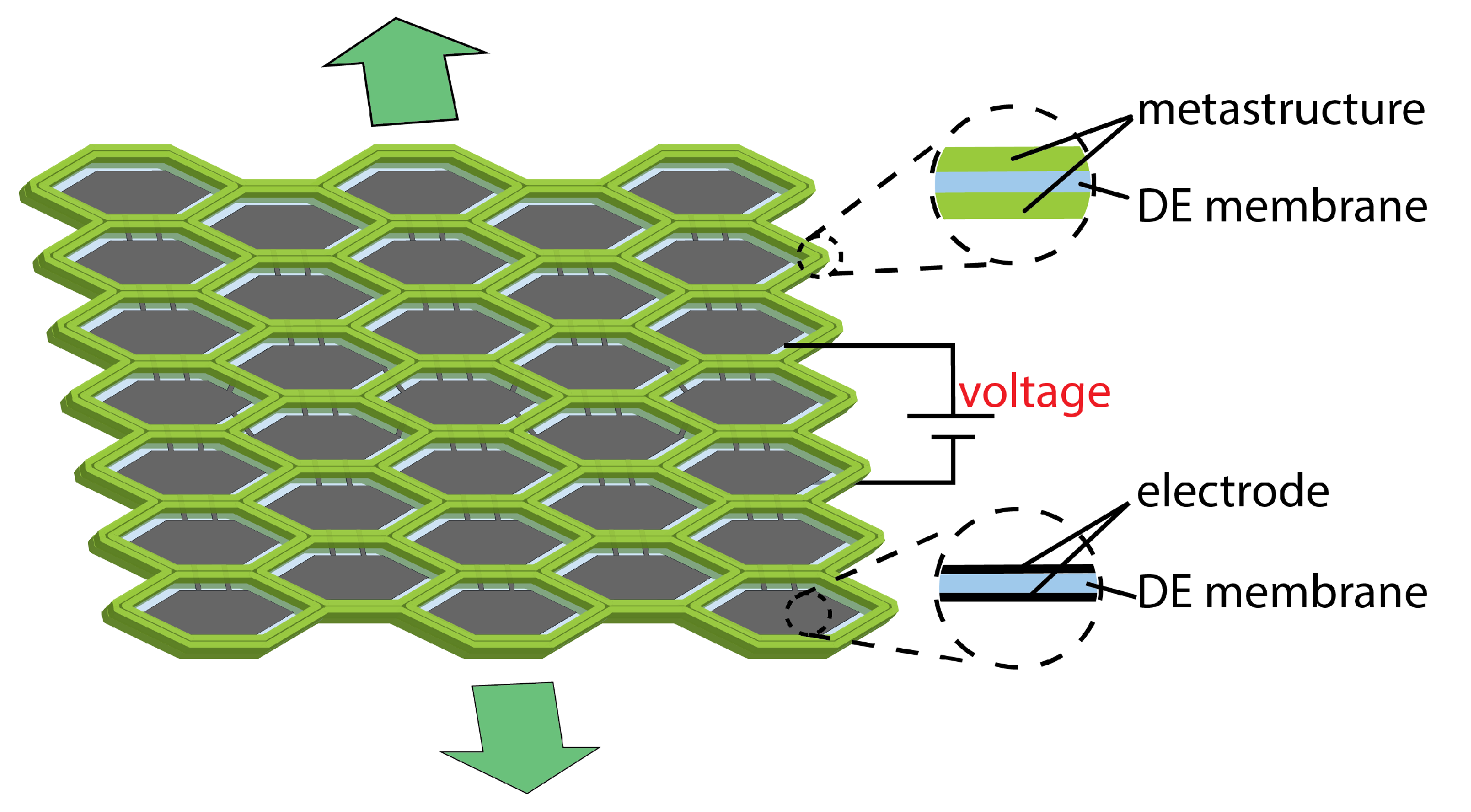
A Unidirectional Soft Dielectric Elastomer Actuator Enabled by Built-In Honeycomb Metastructures
Abstract
1. Introduction
2. Materials and Fabrication
2.1. Materials Selection
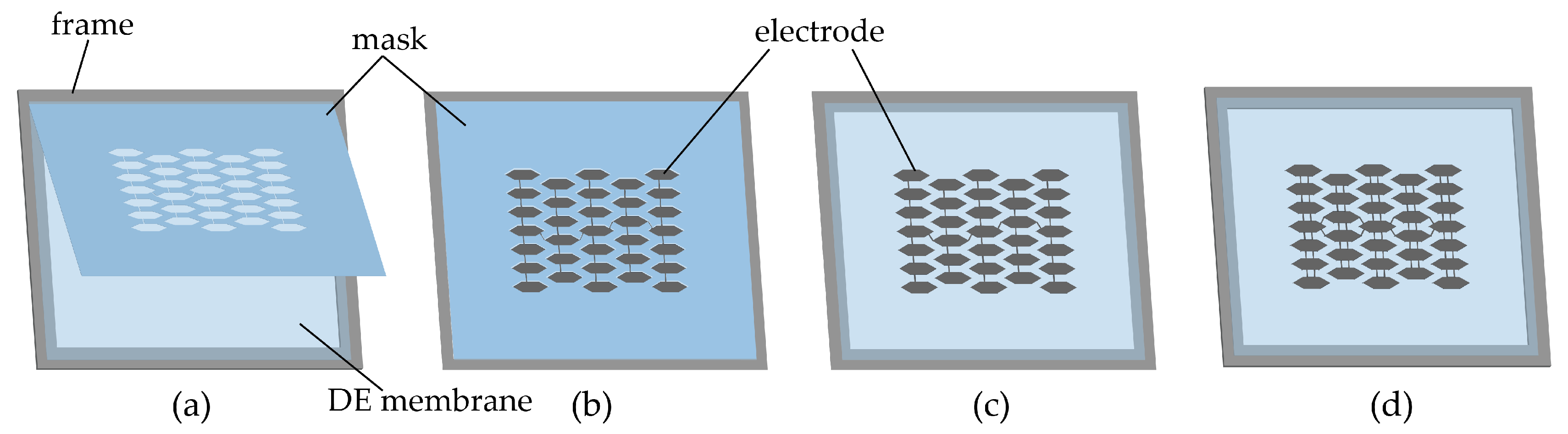
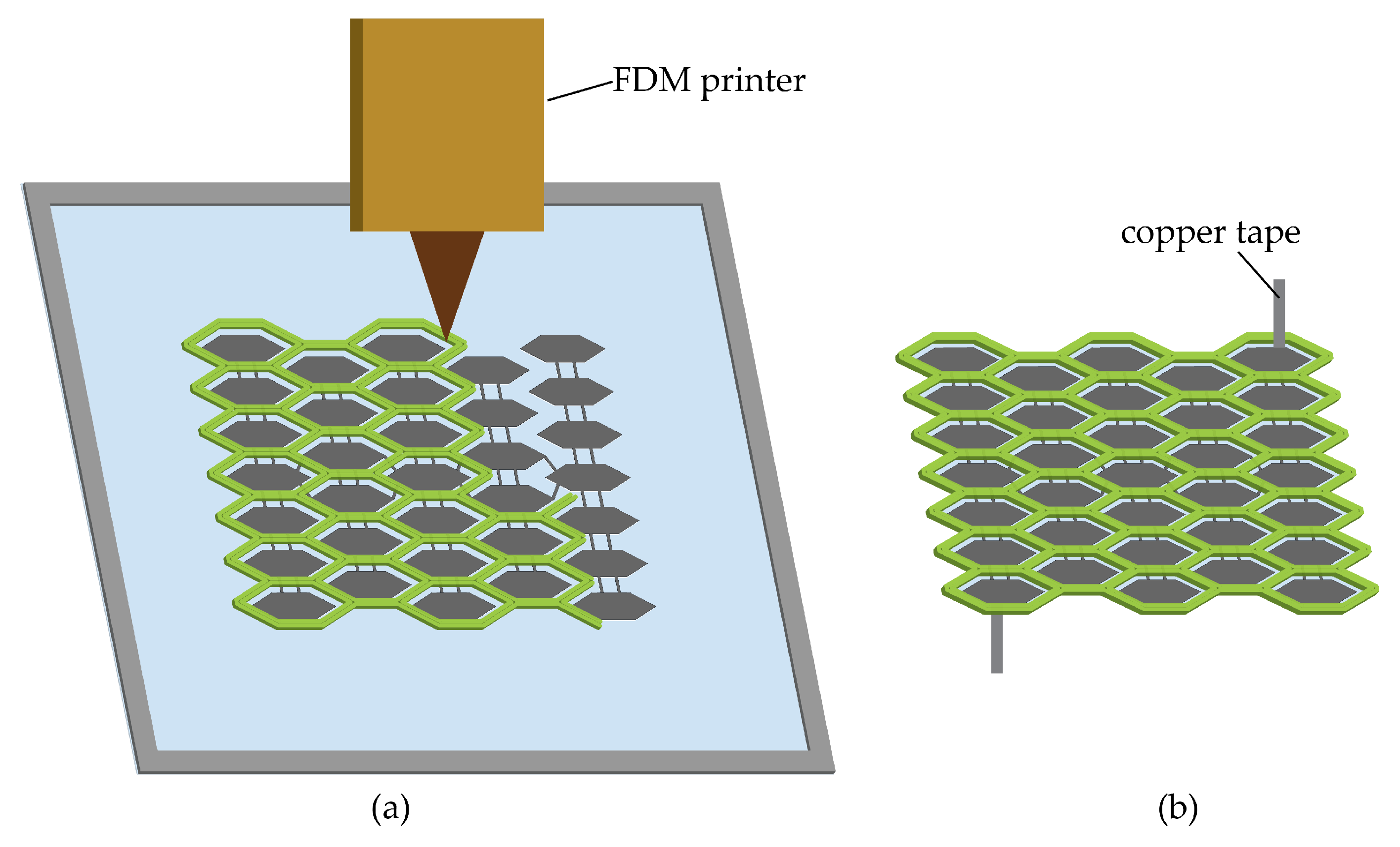
2.2. Fabrication
3. Model and Simulation
3.1. Modeling of DEs
3.2. Finite Element Analysis
3.2.1. Setting
3.2.2. Effect of Prestretches: and
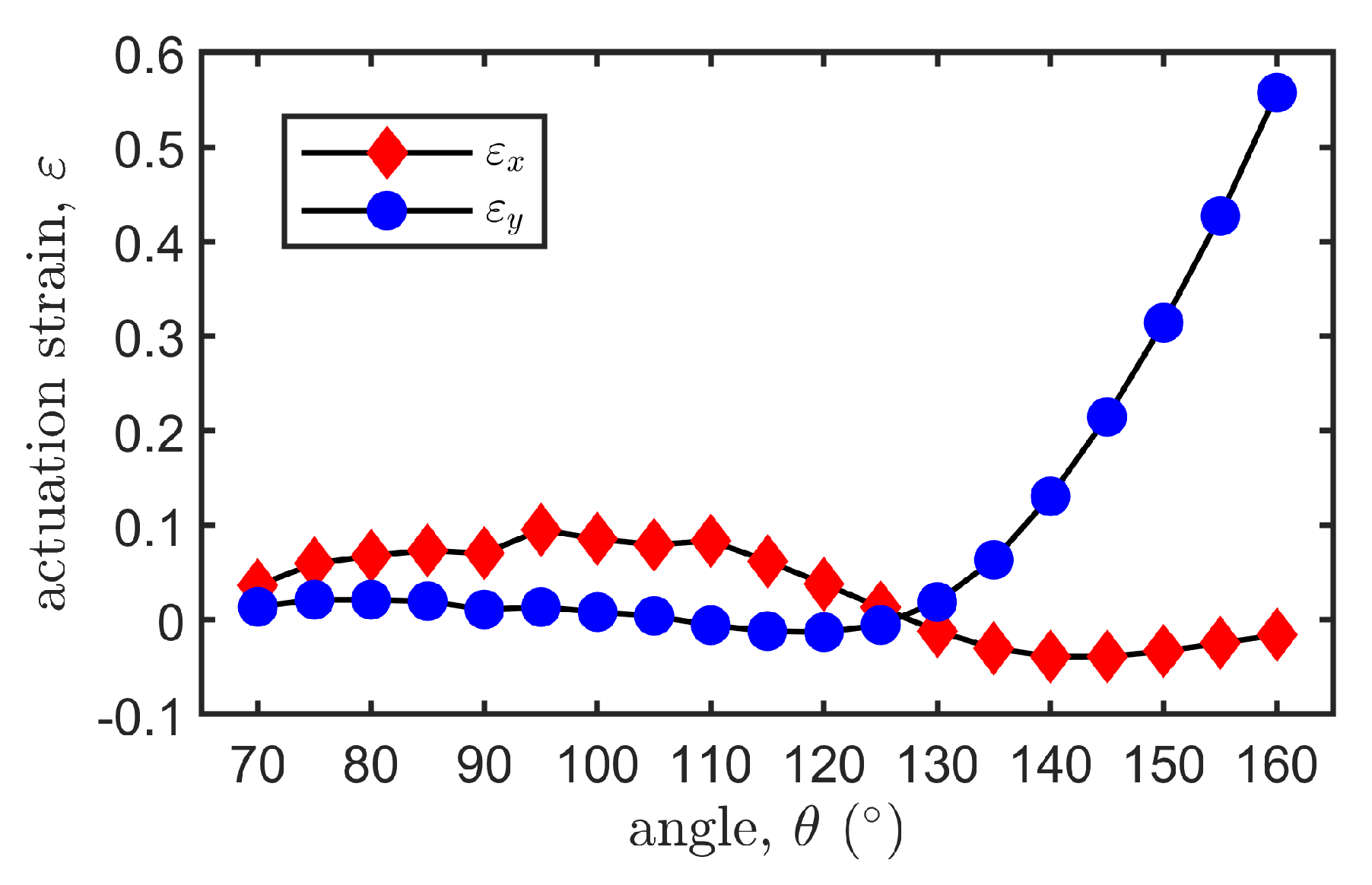
3.2.3. Effect of Honeycomb Angle
3.3. Final Design for Prototyping
4. Experimental Section
4.1. Setup
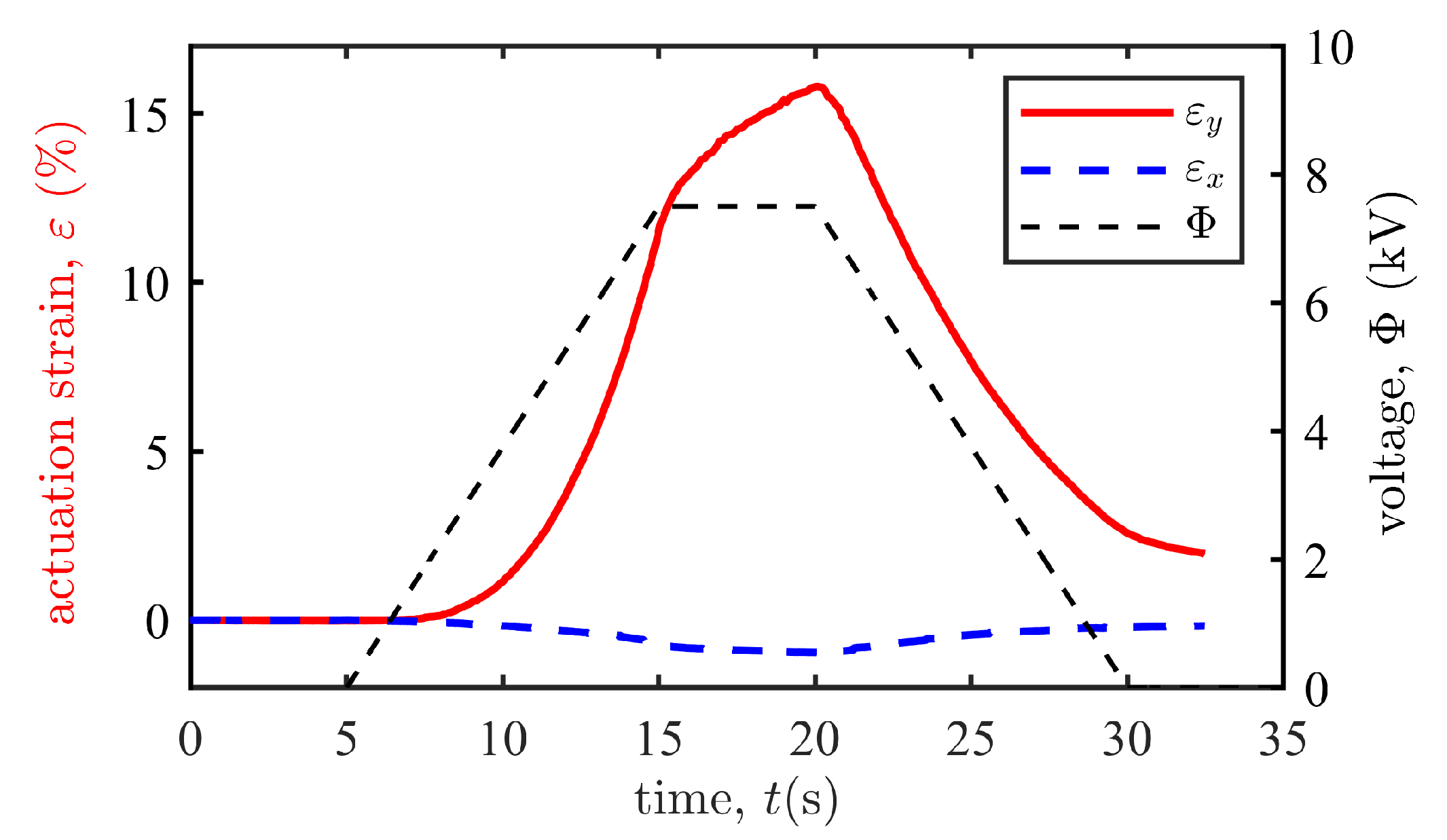
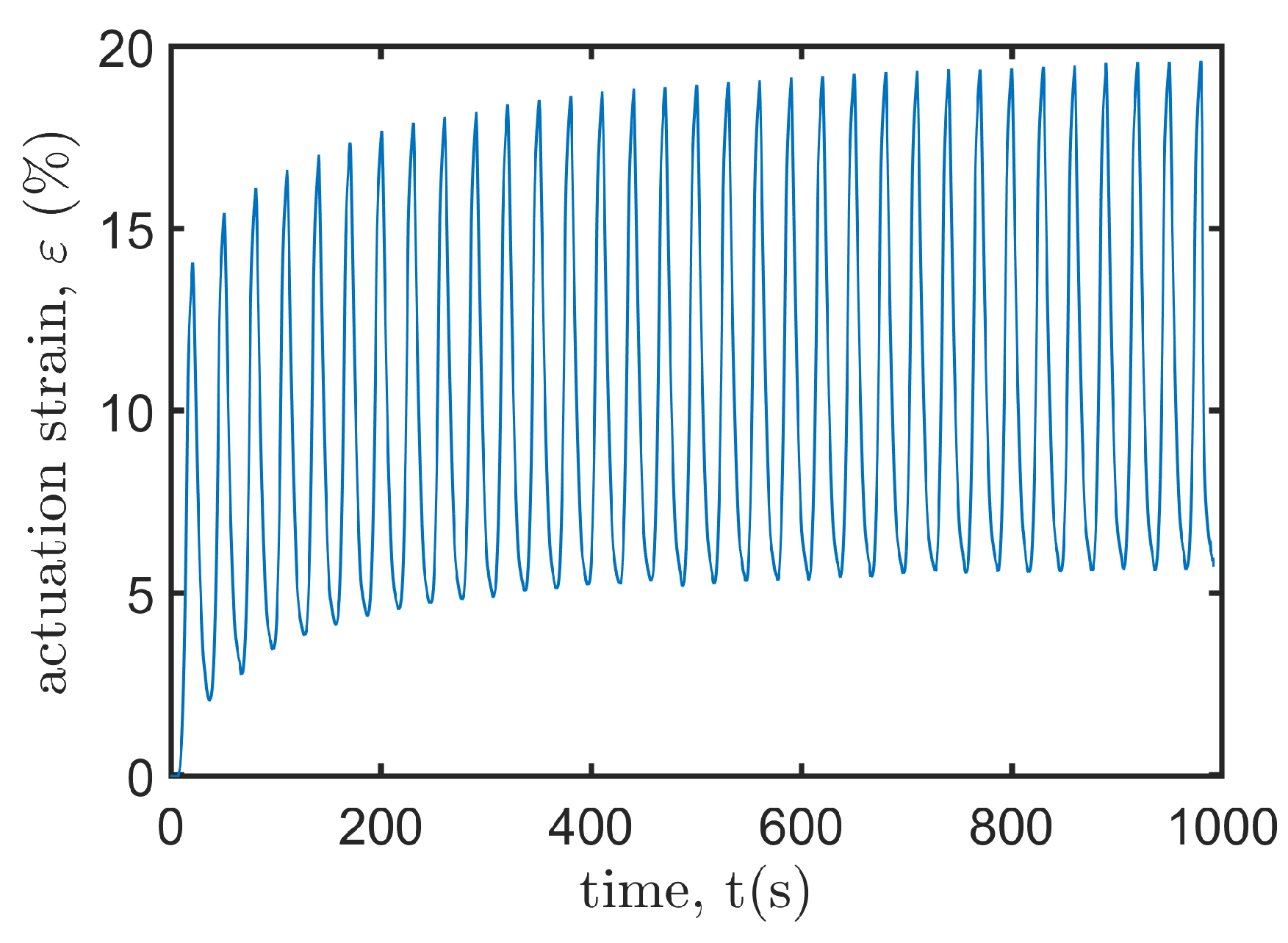
4.2. Results
4.3. Discussion
5. Conclusions and Future Outlook
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Rus, D.; Tolley, M.T. Design, fabrication and control of soft robots. Nature 2015, 521, 467–475. [Google Scholar] [CrossRef] [PubMed]
- Gu, G.; Zou, J.; Zhao, R.; Zhao, X.; Zhu, X. Soft wall-climbing robots. Sci. Robot. 2018, 3, eaat2874. [Google Scholar] [CrossRef]
- Kim, Y.; Yuk, H.; Zhao, R.; Chester, S.A.; Zhao, X. Printing ferromagnetic domains for untethered fast-transforming soft materials. Nature 2018, 558, 274. [Google Scholar] [CrossRef] [PubMed]
- Chen, F.; Xu, W.; Zhang, H.; Wang, Y.; Cao, J.; Wang, M.Y.; Ren, H.; Zhu, J.; Zhang, Y. Topology Optimized Design, Fabrication, and Characterization of a Soft Cable-Driven Gripper. IEEE Robot. Autom. Lett. 2018, 3, 2463–2470. [Google Scholar] [CrossRef]
- Kim, Y.; Parada, G.A.; Liu, S.; Zhao, X. Ferromagnetic soft continuum robots. Sci. Robot. 2019, 4, eaax7329. [Google Scholar] [CrossRef]
- Ge, L.; Chen, F.; Wang, D.; Zhang, Y.; Han, D.; Wang, T.; Gu, G. Design, Modeling, and Evaluation of Fabric-Based Pneumatic Actuators for Soft Wearable Assistive Gloves. Soft Robot. 2020. [Google Scholar] [CrossRef]
- Mosadegh, B.; Polygerinos, P.; Keplinger, C.; Wennstedt, S.; Shepherd, R.F.; Gupta, U.; Shim, J.; Bertoldi, K.; Walsh, C.J.; Whitesides, G.M. Pneumatic networks for soft robotics that actuate rapidly. Adv. Funct. Mater. 2014, 24, 2163–2170. [Google Scholar] [CrossRef]
- Bishop-Moser, J.; Kota, S. Design and modeling of generalized fiber-reinforced pneumatic soft actuators. IEEE Trans. Robot. 2015, 31, 536–545. [Google Scholar] [CrossRef]
- Polygerinos, P.; Wang, Z.; Overvelde, J.T.; Galloway, K.C.; Wood, R.J.; Bertoldi, K.; Walsh, C.J. Modeling of soft fiber-reinforced bending actuators. IEEE Trans. Robot. 2015, 31, 778–789. [Google Scholar] [CrossRef]
- Ge, Q.; Sakhaei, A.H.; Lee, H.; Dunn, C.K.; Fang, N.X.; Dunn, M.L. Multimaterial 4D printing with tailorable shape memory polymers. Sci. Rep. 2016, 6, 31110. [Google Scholar] [CrossRef]
- Ge, Q.; Yu, K.; Ding, Y.; Qi, H.J. Prediction of temperature-dependent free recovery behaviors of amorphous shape memory polymers. Soft Matter 2012, 8, 11098–11105. [Google Scholar] [CrossRef]
- Zhang, Y.F.; Zhang, N.; Hingorani, H.; Ding, N.; Wang, D.; Yuan, C.; Zhang, B.; Gu, G.; Ge, Q. Fast-Response, Stiffness-Tunable Soft Actuator by Hybrid Multimaterial 3D Printing. Adv. Funct. Mater. 2019, 29, 1806698. [Google Scholar] [CrossRef]
- Shahinpoor, M.; Kim, K.J. Ionic polymer-metal composites: I. Fundamentals. Smart Mater. Struct. 2001, 10, 819. [Google Scholar] [CrossRef]
- Pelrine, R.; Kornbluh, R.; Pei, Q.; Joseph, J. High-speed electrically actuated elastomers with strain greater than 100%. Science 2000, 287, 836–839. [Google Scholar] [CrossRef] [PubMed]
- Carpi, F.; Bauer, S.; De Rossi, D. Stretching dielectric elastomer performance. Science 2010, 330, 1759–1761. [Google Scholar] [CrossRef]
- Chen, F.; Wang, M.Y.; Zhu, J.; Zhang, Y.F. Interactions between dielectric elastomer actuators and soft bodies. Soft Robot. 2016, 3, 161–169. [Google Scholar] [CrossRef]
- Gu, G.Y.; Zhu, J.; Zhu, L.M.; Zhu, X. A survey on dielectric elastomer actuators for soft robots. Bioinspir. Biomim. 2017, 12, 011003. [Google Scholar] [CrossRef]
- Gu, G.Y.; Gupta, U.; Zhu, J.; Zhu, L.M.; Zhu, X. Modeling of viscoelastic electromechanical behavior in a soft dielectric elastomer actuator. IEEE Trans. Robot. 2017, 33, 1263–1271. [Google Scholar] [CrossRef]
- Koo, I.M.; Jung, K.; Koo, J.C.; Nam, J.D.; Lee, Y.K.; Choi, H.R. Development of soft-actuator-based wearable tactile display. IEEE Trans. Robot. 2008, 24, 549–558. [Google Scholar] [CrossRef]
- Lotz, P.; Matysek, M.; Schlaak, H.F. Fabrication and application of miniaturized dielectric elastomer stack actuators. IEEE/ASME Trans. Mechatron. 2011, 16, 58–66. [Google Scholar] [CrossRef]
- Chen, F.; Cao, J.; Zhang, H.; Wang, M.Y.; Zhu, J.; Zhang, Y. Programmable Deformations of Networked Inflated Dielectric Elastomer Actuators. IEEE/ASME Trans. Mechatron. 2019, 24, 45–55. [Google Scholar] [CrossRef]
- Shintake, J.; Rosset, S.; Schubert, B.; Floreano, D.; Shea, H. Versatile soft grippers with intrinsic electroadhesion based on multifunctional polymer actuators. Adv. Mater. 2016, 28, 231–238. [Google Scholar] [CrossRef] [PubMed]
- Lau, G.K.; Heng, K.R.; Ahmed, A.S.; Shrestha, M. Dielectric elastomer fingers for versatile grasping and nimble pinching. Appl. Phys. Lett. 2017, 110, 182906. [Google Scholar] [CrossRef]
- Pourazadi, S.; Bui, H.T.; Menon, C. Investigation on a soft grasping gripper based on dielectric elastomer actuators. Smart Mater. Struct. 2019, 28. [Google Scholar] [CrossRef]
- Li, T.; Li, G.; Liang, Y.; Cheng, T.; Dai, J.; Yang, X.; Liu, B.; Zeng, Z.; Huang, Z.; Luo, Y.; et al. Fast-moving soft electronic fish. Sci. Adv. 2017, 3, e1602045. [Google Scholar] [CrossRef]
- Cao, J.; Qin, L.; Liu, J.; Ren, Q.; Foo, C.C.; Wang, H.; Lee, H.P.; Zhu, J. Untethered soft robot capable of stable locomotion using soft electrostatic actuators. Extrem. Mech. Lett. 2018, 21, 9–16. [Google Scholar] [CrossRef]
- Chen, Y.; Zhao, H.; Mao, J.; Chirarattananon, P.; Helbling, E.F.; Hyun, N.s.P.; Clarke, D.R.; Wood, R.J. Controlled flight of a microrobot powered by soft artificial muscles. Nature 2019, 575, 324–329. [Google Scholar] [CrossRef]
- Huang, J.; Lu, T.; Zhu, J.; Clarke, D.R.; Suo, Z. Large, uni-directional actuation in dielectric elastomers achieved by fiber stiffening. Appl. Phys. Lett. 2012, 100, 211901. [Google Scholar] [CrossRef]
- Lu, T.; Huang, J.; Jordi, C.; Kovacs, G.; Huang, R.; Clarke, D.R.; Suo, Z. Dielectric elastomer actuators under equal-biaxial forces, uniaxial forces, and uniaxial constraint of stiff fibers. Soft Matter 2012, 8, 6167–6173. [Google Scholar] [CrossRef]
- Chen, F.; Liu, K.; Wang, Y.; Zou, J.; Gu, G.; Zhu, X. Automatic Design of Soft Dielectric Elastomer Actuators With Optimal Spatial Electric Fields. IEEE Trans. Robot. 2019, 35, 1150–1165. [Google Scholar] [CrossRef]
- Wang, N.; Guo, H.; Chen, B.; Cui, C.; Zhang, X. Design of a rotary dielectric elastomer actuator using a topology optimization method based on pairs of curves. Smart Mater. Struct. 2018, 27, 055011. [Google Scholar] [CrossRef]
- Chen, F.; Liu, K.; Zhu, X. Buckling-induced Shape Morphing using Dielectric Elastomer Actuators Patterned with Spatially-varying Electrodes. In Proceedings of the 2019 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Macau, China, 4–8 November 2019; pp. 8306–8311. [Google Scholar]
- Hajiesmaili, E.; Clarke, D.R. Reconfigurable shape-morphing dielectric elastomers using spatially varying electric fields. Nat. Commun. 2019, 10, 183. [Google Scholar] [CrossRef] [PubMed]
- Estakhri, N.M.; Edwards, B.; Engheta, N. Inverse-designed metastructures that solve equations. Science 2019, 363, 1333–1338. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Guo, X.; Wu, J.; Fang, D.; Zhang, Y. Soft mechanical metamaterials with unusual swelling behavior and tunable stress-strain curves. Sci. Adv. 2018, 4, eaar8535. [Google Scholar] [CrossRef] [PubMed]
- Babaee, S.; Shim, J.; Weaver, J.C.; Chen, E.R.; Patel, N.; Bertoldi, K. 3D soft metamaterials with negative Poisson’s ratio. Adv. Mater. 2013, 25, 5044–5049. [Google Scholar] [CrossRef] [PubMed]
- Yasuda, H.; Yang, J. Reentrant origami-based metamaterials with negative Poisson’s ratio and bistability. Phys. Rev. Lett. 2015, 114, 185502. [Google Scholar] [CrossRef] [PubMed]
- Clausen, A.; Wang, F.; Jensen, J.S.; Sigmund, O.; Lewis, J.A. Topology optimized architectures with programmable Poisson’s ratio over large deformations. Adv. Mater. 2015, 27, 5523–5527. [Google Scholar] [CrossRef]
- Zhou, F.; Zhang, M.; Cao, X.; Zhang, Z.; Chen, X.; Xiao, Y.; Liang, Y.; Wong, T.W.; Li, T.; Xu, Z. Fabrication and modeling of dielectric elastomer soft actuator with 3D printed thermoplastic frame. Sens. Actuators Phys. 2019, 292, 112–120. [Google Scholar] [CrossRef]
- Carpi, F.; Sommer-Larsen, P.; De Rossi, D.; Gaudenzi, P.; Lampani, L.; Campanile, F.; Pfeiffer, E.; Neri, G.; Baldacci, S. Electroactive polymers: new materials for spacecraft structures. In Spacecraft Structures, Materials and Mechanical Testing 2005; SUB Gottingen: Göttingen, Germany, 2005; Volume 581. [Google Scholar]
- Technical Data Sheet TPU 95A. Available online: https://ultimaker.com/en/resources/49917-tpu-95a (accessed on 30 November 2019).
- Suo, Z. Theory of dielectric elastomers. Acta Mech. Solida Sin. 2010, 23, 549–578. [Google Scholar] [CrossRef]
- Zhao, X.; Wang, Q. Harnessing large deformation and instabilities of soft dielectrics: Theory, experiment, and application. Appl. Phys. Rev. 2014, 1, 021304. [Google Scholar] [CrossRef]
- Zou, J.; Gu, G. High-precision tracking control of a soft dielectric elastomer actuator with inverse viscoelastic hysteresis compensation. IEEE/ASME Trans. Mechatron. 2019, 24, 36–44. [Google Scholar] [CrossRef]
- Rosset, S.; Araromi, O.A.; Schlatter, S.; Shea, H.R. Fabrication process of silicone-based dielectric elastomer actuators. JoVE J. Vis. Exp. 2016, 108, e53423. [Google Scholar] [CrossRef] [PubMed]
- Ji, X.; Liu, X.; Cacucciolo, V.; Imboden, M.; Civet, Y.; El Haitami, A.; Cantin, S.; Perriard, Y.; Shea, H. An autonomous untethered fast soft robotic insect driven by low-voltage dielectric elastomer actuators. Sci. Robot. 2019, 4, eaaz6451. [Google Scholar] [CrossRef]




| Original Thickness | Shear Modulus | Dielectric Constant | Breakdown Strength |
|---|---|---|---|
| 1 mm | 45 kPa | F/m | ∼100 MV/m |
| Flexural Modulus | Poisson’s Ratio | Beam Length L | Beam Width w | Beam Height h |
|---|---|---|---|---|
| 78 MPa | 0.36 | 8 mm | 0.8 mm | 2 mm |
| Case | (%) | (%) | ||
|---|---|---|---|---|
| 1 | 4.0 | 2.5 | 7.02 | 1.09 |
| 2 | 4.0 | 2.0 | 5.57 | 1.13 |
| 3 | 3.2 | 3.2 | 5.71 | 0.66 |
| 4 | 3.0 | 3.0 | 4.87 | 0.58 |
| 5 | 2.5 | 4.0 | 8.40 | 0.69 |
| 6 | 2.0 | 4.0 | 7.56 | 0.58 |
| Case | (%) | (%) | ||
|---|---|---|---|---|
| 1 | 4.0 | 2.5 | −3.32 | 31.41 |
| 2 | 4.0 | 2.0 | −3.28 | 23.77 |
| 3 | 3.2 | 3.2 | −1.71 | 30.97 |
| 4 | 3.0 | 3.0 | −1.44 | 26.59 |
| 5 | 2.5 | 4.0 | −0.62 | 23.27 |
| 6 | 2.0 | 4.0 | −0.27 | 14.45 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, K.; Chen, S.; Chen, F.; Zhu, X. A Unidirectional Soft Dielectric Elastomer Actuator Enabled by Built-In Honeycomb Metastructures. Polymers 2020, 12, 619. https://doi.org/10.3390/polym12030619
Liu K, Chen S, Chen F, Zhu X. A Unidirectional Soft Dielectric Elastomer Actuator Enabled by Built-In Honeycomb Metastructures. Polymers. 2020; 12(3):619. https://doi.org/10.3390/polym12030619
Chicago/Turabian StyleLiu, Kun, Shitong Chen, Feifei Chen, and Xiangyang Zhu. 2020. "A Unidirectional Soft Dielectric Elastomer Actuator Enabled by Built-In Honeycomb Metastructures" Polymers 12, no. 3: 619. https://doi.org/10.3390/polym12030619
APA StyleLiu, K., Chen, S., Chen, F., & Zhu, X. (2020). A Unidirectional Soft Dielectric Elastomer Actuator Enabled by Built-In Honeycomb Metastructures. Polymers, 12(3), 619. https://doi.org/10.3390/polym12030619





