Effect of Weaving Structures on the Water Wicking–Evaporating Behavior of Woven Fabrics
Abstract
1. Introduction
2. Materials and Methods
2.1. Samples and Materials
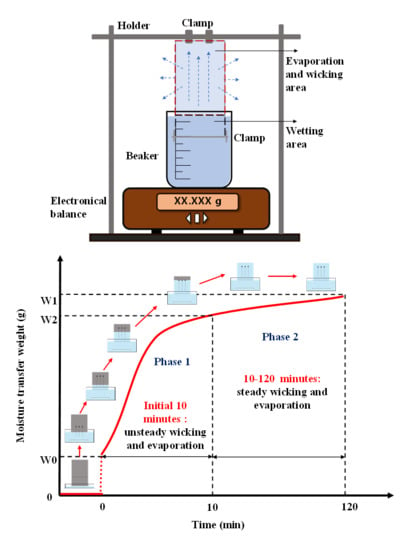
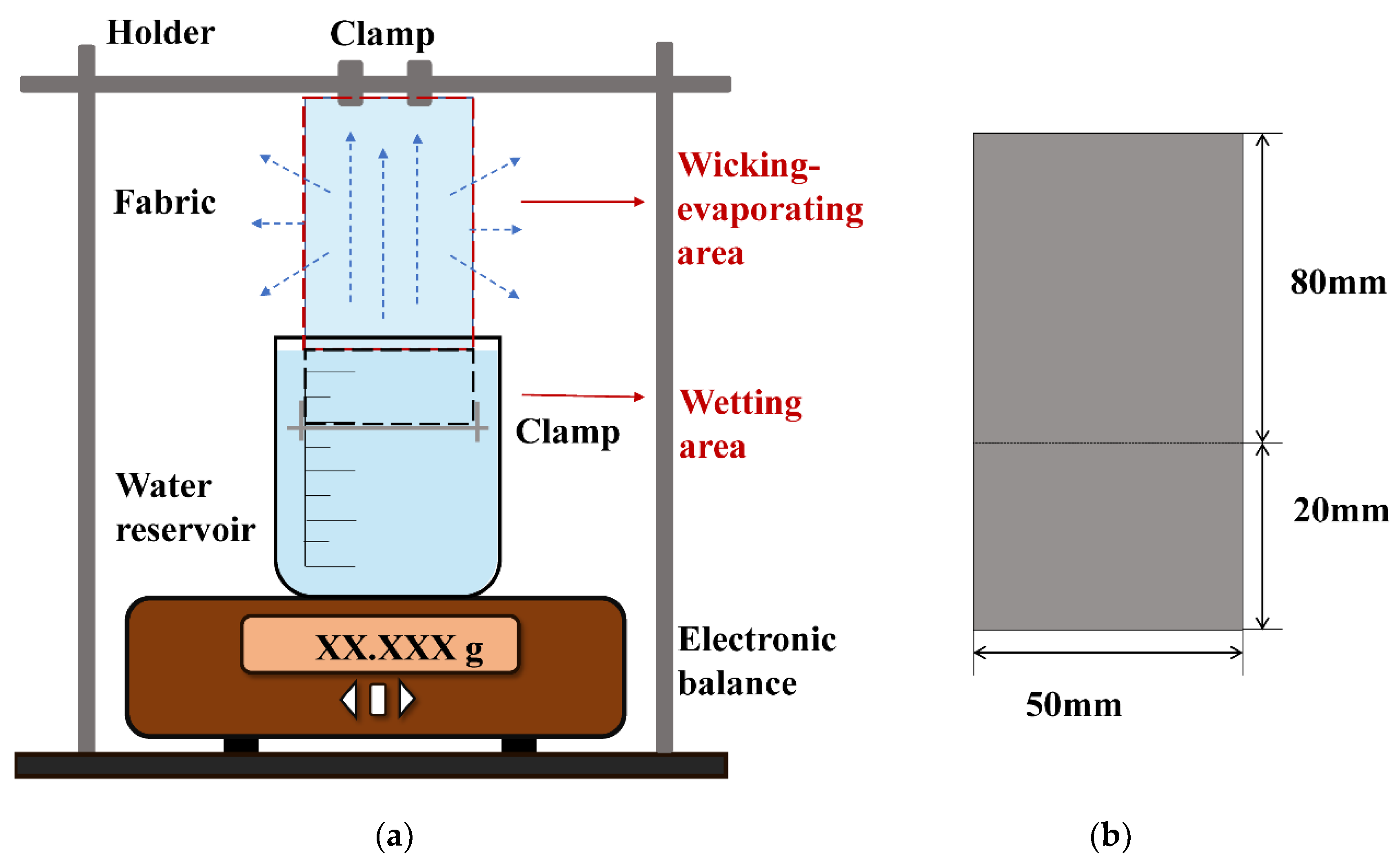
2.2. Testing Methods
- ●
- An electronic balance with an accuracy of 0.001 mg to record the wicking–evaporating-induced weight loss versus time;
- ●
- A liquid reservoir with a capacity of 250 mL distilled water to supply water to the fabric;
- ●
- An iron holder to mount the test samples; and
- ●
- A clamp to add pre-tension to the lower end of the sample and to keep samples free of wrinkles.
3. Results and Discussion
3.1. The Wicking–Evaporating Behavior
3.2. The Effect of Wicking–Evaporating Behavior
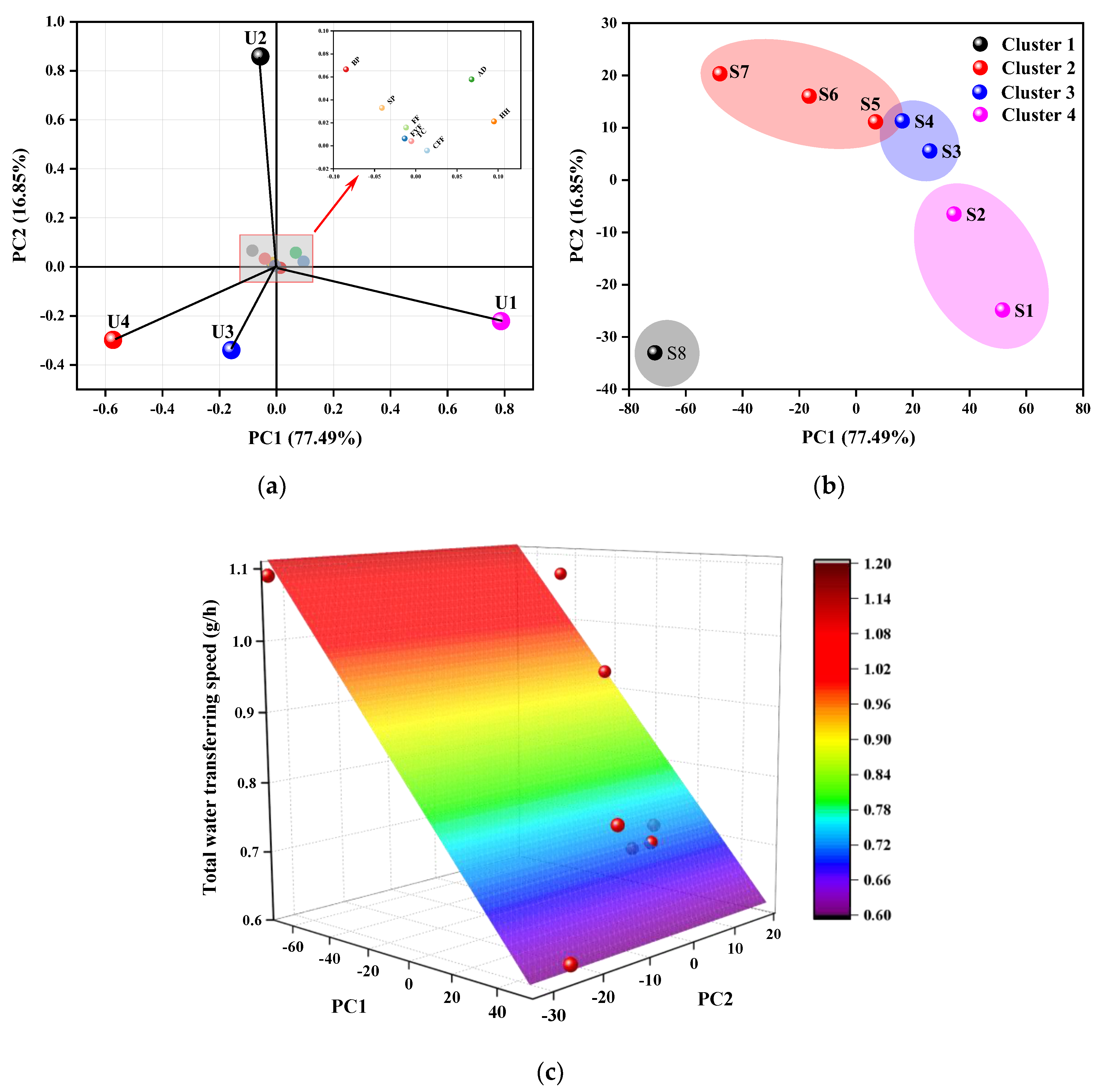
3.3. Prediction of the Total Water Transfer Speed
4. Conclusions
- (1)
- There are two phases during the wicking–evaporating process: Phase I, with unsteady wicking and evaporating; and Phase II, with steady wicking–evaporating due to equilibrium between the capillary and gravity forces. Because of the uneven degree of capillary channels induced by different structures, the water transfer speed in Phase I varied with samples. However, it was constant regardless of weave structure in Phase II. Because of the screening phenomenon induced by continuous saturated conditions, the water transfer rate from a fully wet fabric surface is basically identical to that from a free liquid surface.
- (2)
- Woven fabric with more float has more macropores, possessing nonuniform capillary channels and more air space for evaporating. Float can increase the total water transfer in the wicking–evaporating process more than interlacing points.
Author Contributions
Funding
Conflicts of Interest
References
- Song, G. Improving Comfort in Clothing; Elsevier: Cambridge, UK, 2011. [Google Scholar]
- Chinta, S.; Gujar, P.D. Significance of moisture management for high performance textile fabrics. Int. J. Innov. Res. Sci. Eng. Technol. 2013, 2, 814–819. [Google Scholar]
- Guan, M.; Annaheim, S.; Camenzind, M.; Li, J.; Mandal, S.; Psikuta, A.; Rossi, R.M. Moisture transfer of the clothing–human body system during continuous sweating under radiant heat. Text. Res. J. 2019, 89, 4537–4553. [Google Scholar] [CrossRef]
- Wang, F. Moisture Absorption and Transport through Textiles. In Engineering of High-Performance Textiles; Elsevier: Duxford, UK, 2018; pp. 247–275. [Google Scholar]
- Fukazawa, T.; Havenith, G. Differences in comfort perception in relation to local and whole body skin wettedness. Eur. J. Appl. Physiol. 2009, 106, 15–24. [Google Scholar] [CrossRef] [PubMed]
- Ghali, K.; Jones, B.; Tracy, J. Modeling moisture transfer in fabrics. Exp. Therm. Fluid Sci. 1994, 9, 330–336. [Google Scholar] [CrossRef]
- Parada, M.; Vontobel, P.; Rossi, R.M.; Derome, D.; Carmeliet, J. Dynamic wicking process in textiles. Transp. Porous Media 2017, 119, 611–632. [Google Scholar] [CrossRef]
- Parada, M.; Zhou, X.; Derome, D.; Rossi, R.M.; Carmeliet, J. Modeling wicking in textiles using the dual porosity approach. Text. Res. J. 2019, 89, 3519–3528. [Google Scholar] [CrossRef]
- Parada, M.; Derome, D.; Rossi, R.M.; Carmeliet, J. A review on advanced imaging technologies for the quantification of wicking in textiles. Text. Res. J. 2017, 87, 110–132. [Google Scholar] [CrossRef]
- Tang, K.-P.M.; Kan, C.-W.; Fan, J.-T. Comparison of test methods for measuring water absorption and transport test methods of fabrics. Measurement 2017, 97, 126–137. [Google Scholar] [CrossRef]
- Liu, T.; Choi, K.-F.; Li, Y. Wicking in twisted yarns. J. Colloid Interface Sci. 2008, 318, 134–139. [Google Scholar] [CrossRef]
- Fangueiro, R.; Filgueiras, A.; Soutinho, F.; Xie, M. Wicking behavior and drying capability of functional knitted fabrics. Text. Res. J. 2010, 80, 1522–1530. [Google Scholar] [CrossRef]
- Babu, V.R.; Koushik, C.V.; Lakshmikantha, C.B.; Subramaniam, V. Influence of the weave factor on the character of fabric wicking measured by a multiple probe vertical wicking tester. Fibres Text. East. Eur. 2011, 19, 60–63. [Google Scholar]
- Yanilmaz, M.; Kalaoglu, F. Investigation of wicking, wetting and drying properties of acrylic knitted fabrics. Text. Res. J. 2012, 82, 820–831. [Google Scholar] [CrossRef]
- Duru, S.C.; Candan, C. Effect of repeated laundering on wicking and drying properties of fabrics of seamless garments. Text. Res. J. 2013, 83, 591–605. [Google Scholar] [CrossRef]
- Saricam, C.; Kalaoglu, F. Investigation of the wicking and drying behaviour of polyester woven fabrics. Fibres Text. East. Eur. 2014, 22, 73–78. [Google Scholar]
- Wang, X.; Huang, Z.; Miao, D.; Zhao, J.; Yu, J.; Ding, B. Biomimetic fibrous murray membranes with ultrafast water transport and evaporation for smart moisture-wicking fabrics. ACS Nano 2019, 13, 1060–1070. [Google Scholar] [CrossRef] [PubMed]
- Zhu, C.; Tada, H.; Shi, J.; Yan, J.; Morikawa, H. Water transport on interlaced yarns. Text. Res. J. 2019, 89, 5198–5208. [Google Scholar] [CrossRef]
- Masoodi, R.; Pillai, K.M. Evaporation and Wicking. In Wicking in Porous Materials: Traditional and Modern Modeling Approaches; CRC Press: Boca Raton, FL, USA, 2012; pp. 201–236. [Google Scholar] [CrossRef]
- Beyhaghi, S.; Geoffroy, S.; Prat, M.; Pillai, K.M. Wicking and evaporation of liquids in porous wicks: A simple analytical approach to optimization of wick design. AIChE J. 2014, 60, 1930–1940. [Google Scholar] [CrossRef]
- Fries, N.; Odic, K.; Conrath, M.; Dreyer, M. The effect of evaporation on the wicking of liquids into a metallic weave. J. Colloid Interface Sci. 2008, 321, 118–129. [Google Scholar] [CrossRef]
- Chen, C.; Duru, P.; Joseph, P.; Geoffroy, S.; Prat, M. Control of evaporation by geometry in capillary structures. From confined pillar arrays in a gap radial gradient to phyllotaxy-inspired geometry. Sci. Rep. 2017, 7, 15110. [Google Scholar] [CrossRef]
- Bishop, P.; Balilonis, G.; Davis, J.; Zhang, Y. Ergonomics and comfort in protective and sport clothing: A brief review. J. Ergon. 2013, S2, 5. [Google Scholar] [CrossRef]
- Pan, N.; Gibson, P. Textile Structure and Moisture Transport. In Thermal and Moisture Transport in Fibrous Materials; Woodhead Publishing: Cambridge, UK, 2006; pp. 3–41. [Google Scholar]
- Dubrovski, P.D.; Ziberna-Sujica, M. The connection between woven fabric construction parameters and air permeability. Fibres Text. East. Eur. 1995, 3, 37–41. [Google Scholar]
- Thanikai Vimal, J.; Prakash, C.; Jebastin Rajwin, A. Effect of weave parameters on the tear strength of woven fabrics. J. Nat. Fibers 2018. [Google Scholar] [CrossRef]
- Asayesh, A.; Talaei, M.; Maroufi, M. The effect of weave pattern on the thermal properties of woven fabrics. Int. J. Cloth. Sci. Technol. 2018, 30, 525–535. [Google Scholar] [CrossRef]
- Perwuelz, A.; Mondon, P.; Caze, C. Experimental study ofcapillary flow in yarns. Text. Res. J. 2000, 70, 333–339. [Google Scholar] [CrossRef]
- Raja, D.; Babu, V.R.; Senthilkumar, M.; Ramakrishnan, G.; Kannan, N. A dynamic sweat transfer tester for analyzing transverse sweat transfer properties of multi-weave structure fabrics. J. Ind. Text. 2014, 44, 211–231. [Google Scholar] [CrossRef]
- Hasan, M.M.B.; Calvimontes, A.; Synytska, A.; Dutschk, V. Effects of topographic structure on wettability of differently woven fabrics. Text. Res. J. 2008, 78, 996–1003. [Google Scholar] [CrossRef]
- Calvimontes, A.; Dutschk, V.; Stamm, M. Advances in topographic characterization of textile materials. Text. Res. J. 2010, 80, 1004–1015. [Google Scholar] [CrossRef]
- Lolaki, A.; Shanbeh, M.; Borhani, S. Effect of structural parameters of porous yarns and fabric on air permeability and moisture transfer of double-face woven fabrics. J. Text. Inst. 2017, 108, 992–1000. [Google Scholar] [CrossRef]
- Umair, M.; Hussain, T.; Shaker, K.; Nawab, Y.; Maqsood, M.; Jabbar, M. Effect of woven fabric structure on the air permeability and moisture management properties. J. Text. Inst. 2016, 107, 596–605. [Google Scholar] [CrossRef]
- Zupin, Z.; Hladnik, A.; Dimitrovski, K. Prediction of one-layer woven fabrics air permeability using porosity parameters. Text. Res. J. 2012, 82, 117–128. [Google Scholar] [CrossRef]
- Turan, R.B.; Okur, A. Investigation of pore parameters of woven fabrics by theoretical and image analysis methods. J. Text. Inst. 2012, 103, 875–884. [Google Scholar] [CrossRef]
- Morino, H.; Matsudaira, M.; Furutani, M. Predicting mechanical properties and hand values from the parameters of weave structures. Text. Res. J. 2005, 75, 252–257. [Google Scholar] [CrossRef]
- Masoodi, R.; Pillai, K.M. Pore-Network Modeling of Wicking: A Two-Phase Flow Approach. In Wicking in Porous Materials: Traditional and Modern Modeling Approaches; CRC Press: Boca Raton, FL, USA, 2012; pp. 237–262. [Google Scholar] [CrossRef]
- Fan, J.; Li, C.-X.; Qi, Y.-Y.; Wang, L.-L.; Shou, W.; Liu, Y. Liquid transport in non-uniform capillary fibrous media. Text. Res. J. 2019, 89, 1684–1698. [Google Scholar] [CrossRef]
- Shou, D.; Ye, L.; Fan, J.; Fu, K. Optimal design of porous structures for the fastest liquid absorption. Langmuir 2013, 30, 149–155. [Google Scholar] [CrossRef]
- Benltoufa, S.; Fayala, F.; BenNasrallah, S. Capillary rise in macro and micro pores of jersey knitting structure. J. Eng. Fibers Fabr. 2008, 3, 47–54. [Google Scholar] [CrossRef]
- Chraibi, H.; Prat, M.; Chapuis, O. Influence of contact angle on slow evaporation in two-dimensional porous media. Phys. Rev. E 2009, 79, 026313. [Google Scholar] [CrossRef]

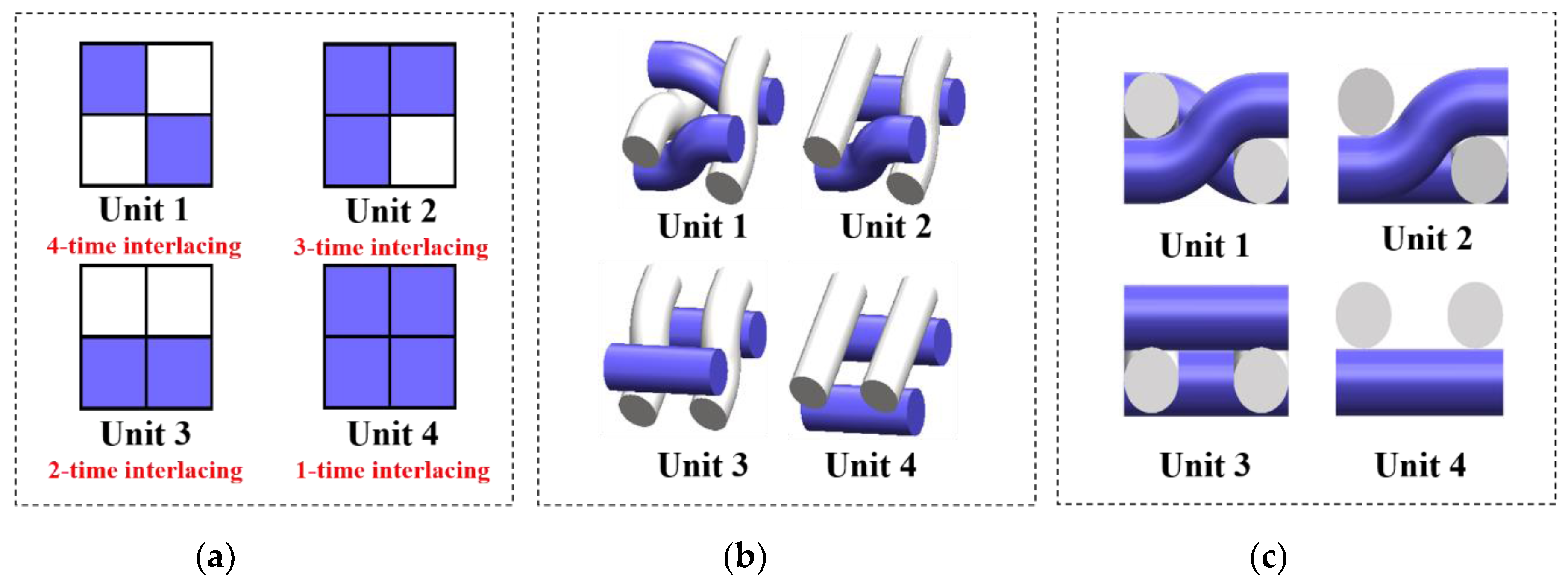
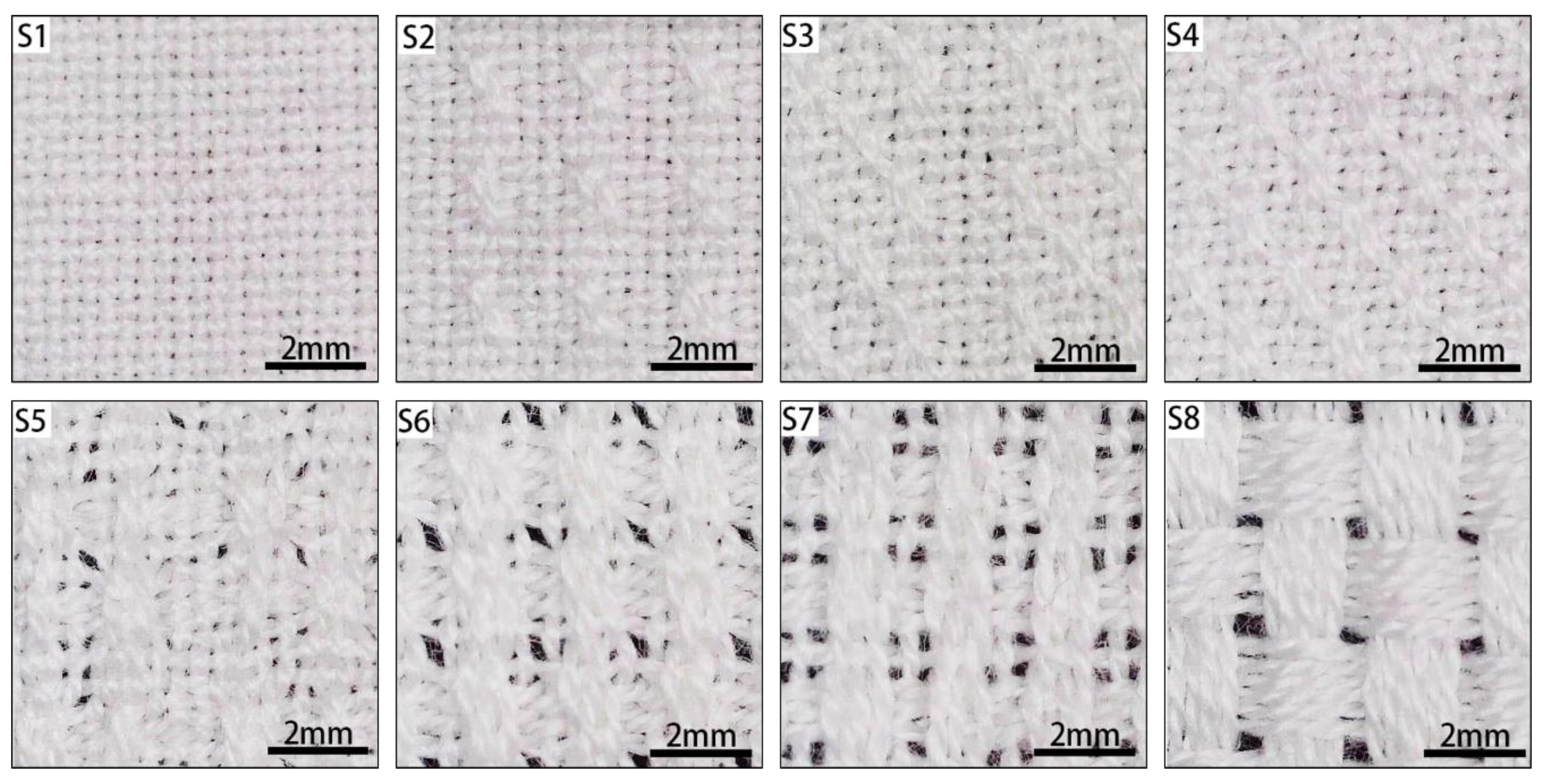


| Sample Code | Weave | CFF | FYF | Proportion of Different Units (%) | |||
|---|---|---|---|---|---|---|---|
| U1 | U2 | U3 | U4 | ||||
| S1 |  | 2.000 | 0.000 | 100.00 | 0.00 | 0.00 | 00.00 |
| S2 |  | 1.647 | 0.471 | 80.56 | 16.67 | 00.00 | 2.78 |
| S3 |  | 1.429 | 0.571 | 69.44 | 27.78 | 00.00 | 2.78 |
| S4 |  | 1.273 | 0.727 | 61.11 | 33.33 | 00.00 | 5.56 |
| S5 |  | 1.286 | 0.857 | 55.57 | 33.33 | 00.00 | 11.11 |
| S6 |  | 0.909 | 1.454 | 36.11 | 38.89 | 00.00 | 25.00 |
| S7 |  | 0.400 | 1.533 | 11.11 | 44.44 | 00.00 | 44.44 |
| S8 |  | 0.303 | 1.667 | 2.78 | 0.00 | 27.78 | 69.44 |
| Sample Code | Areal Density (g/m2) | Thickness (mm) | Firmness Factor (%) | Bulk Porosity (%) | Surface Porosity (%) | Hydraulic Head (μm) |
|---|---|---|---|---|---|---|
| S1 | 168.73 | 0.55 | 84.56 | 0.8058 | 0.98 | 146.90 |
| S2 | 170.50 | 0.68 | 83.26 | 0.8413 | 1.65 | 157.16 |
| S3 | 166.75 | 0.67 | 81.95 | 0.8425 | 2.21 | 167.58 |
| S4 | 172.58 | 0.69 | 84.11 | 0.8417 | 1.33 | 150.35 |
| S5 | 176.67 | 1.01 | 86.26 | 0.8893 | 2.49 | 133.70 |
| S6 | 171.58 | 1.04 | 85.45 | 0.8956 | 4.24 | 139.90 |
| S7 | 160.42 | 1.08 | 85.30 | 0.9041 | 4.31 | 141.37 |
| S8 | 163.58 | 1.25 | 84.38 | 0.9188 | 7.07 | 148.26 |
| r | CFF | FYF | U1 | U2 | U3 | U4 | FF | AD | TC | BP | SP | HH | S |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| CFF | 1 | −0.972 ** | 0.980 ** | −0.236 | −0.586 | −0.920 ** | −0.325 | 0.552 | −0.893 ** | −0.912 ** | −0.871 ** | 0.323 | −0.869 ** |
| FYF | - | 1 | −0.996 ** | 0.364 | 0.519 | 0.874 ** | 0.405 | −0.409 | 0.917 ** | 0.945 ** | 0.856 ** | −0.404 | 0.897 * |
| U1 | - | - | 1 | −0.324 | −0.539 | −0.900 ** | −0.378 | 0.485 | −0.912 ** | −0.933 ** | −0.881 ** | 0.376 | −0.869 ** |
| U2 | - | - | - | 1 | −0.559 | −0.117 | 0.252 | 0.209 | 0.388 | 0.415 | 0.381 | −0.248 | 0.514 |
| U3 | - | - | - | - | 1 | 0.798 * | −0.009 | −0.410 | 0.333 | 0.374 | 0.251 | 0.004 | 0.280 |
| U4 | - | - | - | - | - | 1 | 0.302 | −0.633 | 0.795 * | 0.796 * | 0.778 * | −0.302 | 0.708 |
| FF | - | - | - | - | - | - | 1 | 0.246 | 0.620 | 0.544 | 0.393 | −1.000 ** | 0.520 |
| AD | - | - | - | - | - | - | - | 1 | −0.357 | −0.318 | −0.650 | −0.25 | −0.301 |
| TC | - | - | - | - | - | - | - | - | 1 | 0.987 ** | −0.916 ** | −0.616 | 0.824 ** |
| BP | - | - | - | - | - | - | - | - | - | 1 | 0.885 ** | −0.540 | 0.821 * |
| SP | - | - | - | - | - | - | - | - | - | - | 1 | −0.388 | 0.784 * |
| HH | - | - | - | - | - | - | - | - | - | - | - | 1 | −0.520 |
| PC | Eigenvalue | Contribution Rate | Accumulated Contribution Rate |
|---|---|---|---|
| 1 | 1766.11 | 77.49 | 77.49 |
| 2 | 384.16 | 16.85 | 94.34 |
| 3 | 109.72 | 4.81 | 99.15 |
| 4 | 18.55 | 0.81 | 99.97 |
| 5 | 0.60 | 0.03 | 99.99 |
| 6 | 0.14 | 0.01 | 100.00 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lei, M.; Li, Y.; Liu, Y.; Ma, Y.; Cheng, L.; Hu, Y. Effect of Weaving Structures on the Water Wicking–Evaporating Behavior of Woven Fabrics. Polymers 2020, 12, 422. https://doi.org/10.3390/polym12020422
Lei M, Li Y, Liu Y, Ma Y, Cheng L, Hu Y. Effect of Weaving Structures on the Water Wicking–Evaporating Behavior of Woven Fabrics. Polymers. 2020; 12(2):422. https://doi.org/10.3390/polym12020422
Chicago/Turabian StyleLei, Min, Yuling Li, Yanping Liu, Yanxue Ma, Longdi Cheng, and Yue Hu. 2020. "Effect of Weaving Structures on the Water Wicking–Evaporating Behavior of Woven Fabrics" Polymers 12, no. 2: 422. https://doi.org/10.3390/polym12020422
APA StyleLei, M., Li, Y., Liu, Y., Ma, Y., Cheng, L., & Hu, Y. (2020). Effect of Weaving Structures on the Water Wicking–Evaporating Behavior of Woven Fabrics. Polymers, 12(2), 422. https://doi.org/10.3390/polym12020422