Pyrolysis Kinetic Study and Reaction Mechanism of Epoxy Glass Fiber Reinforced Plastic by Thermogravimetric Analyzer (TG) and TG–FTIR (Fourier-Transform Infrared) Techniques
Abstract
1. Introduction
2. Experimental
3. Kinetic Method
3.1. Model-Free Method
3.2. Model Fitting Methods
4. Result and Discussion
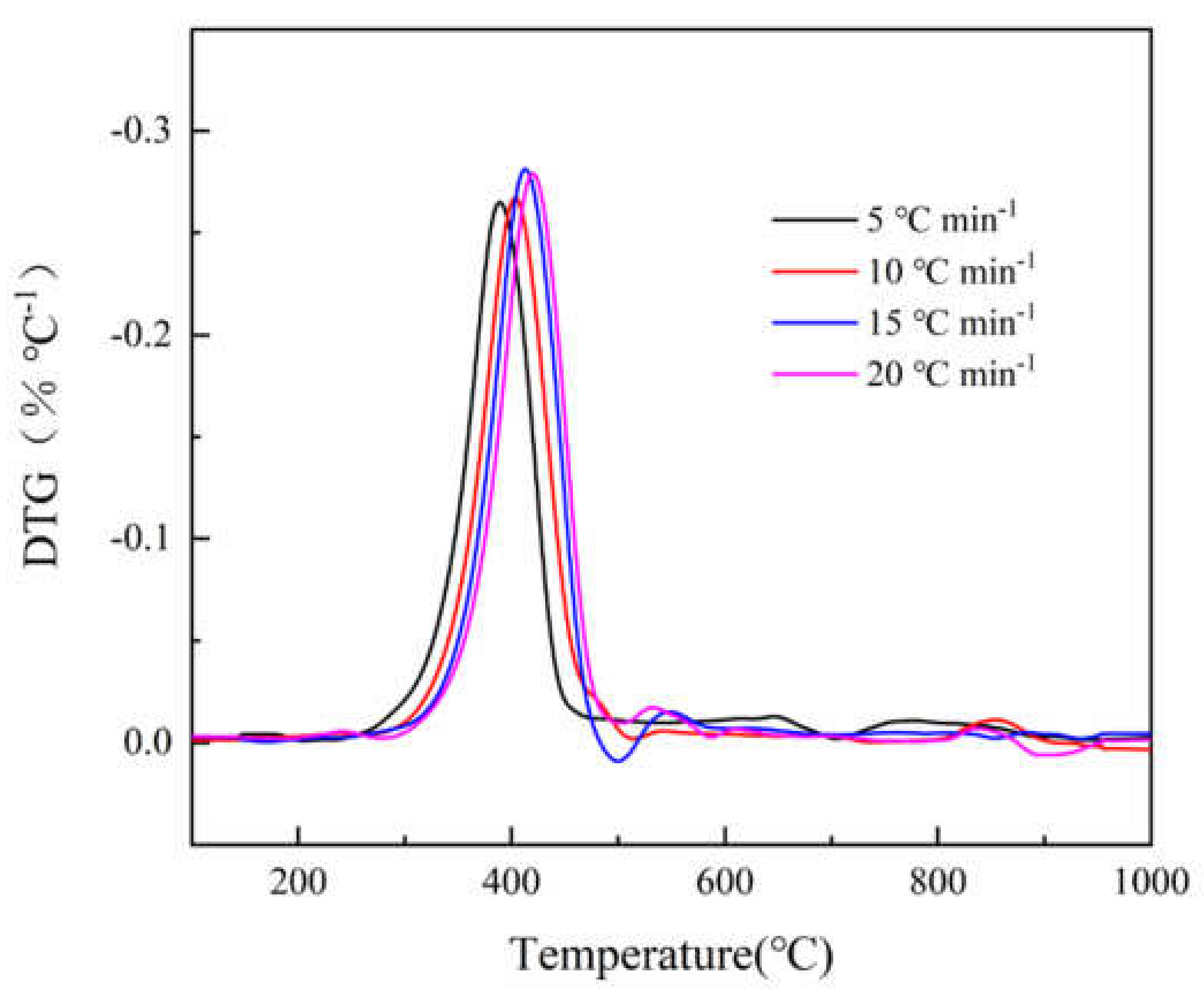
4.1. TGA Analysis
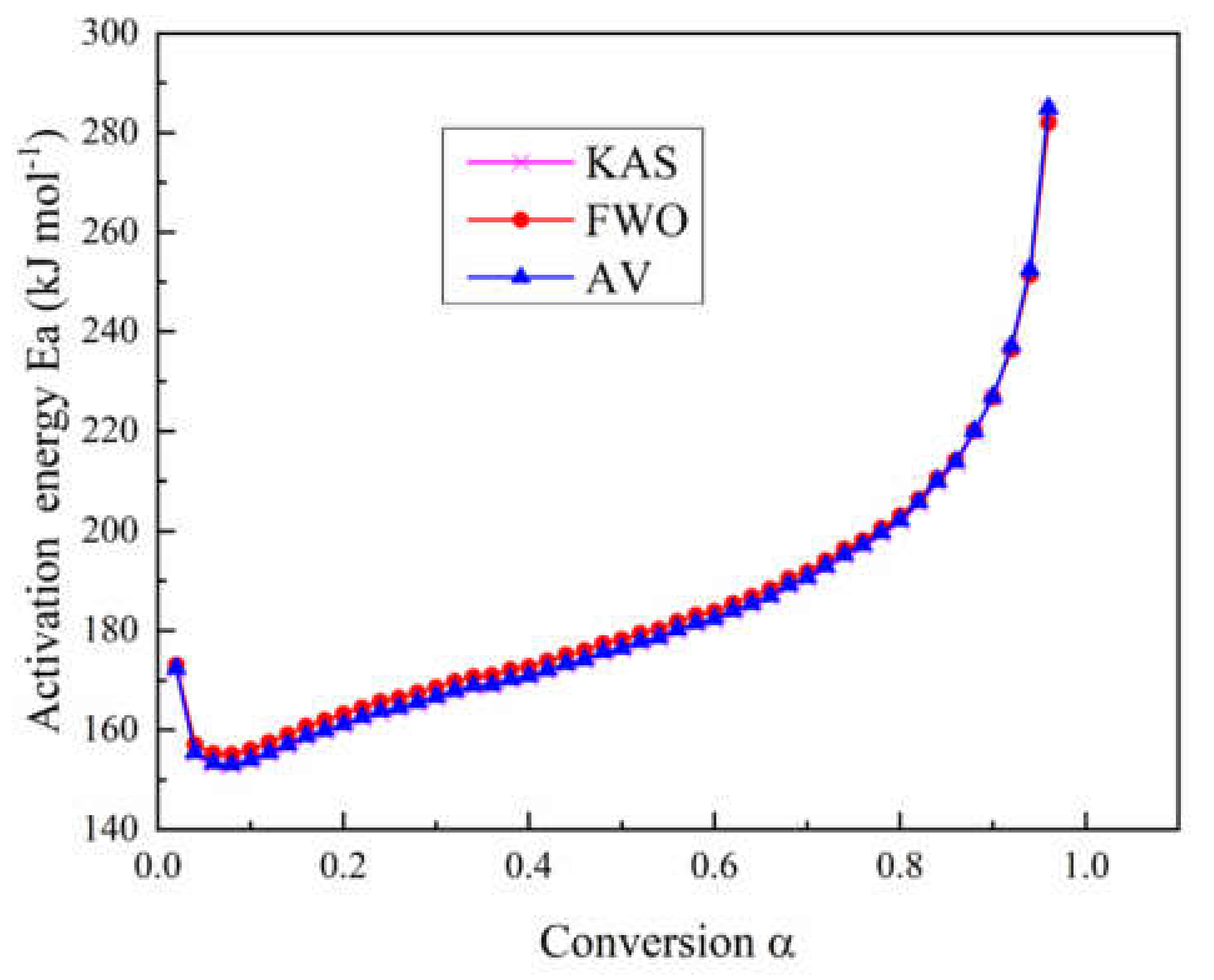
4.2. Model-Free Analysis
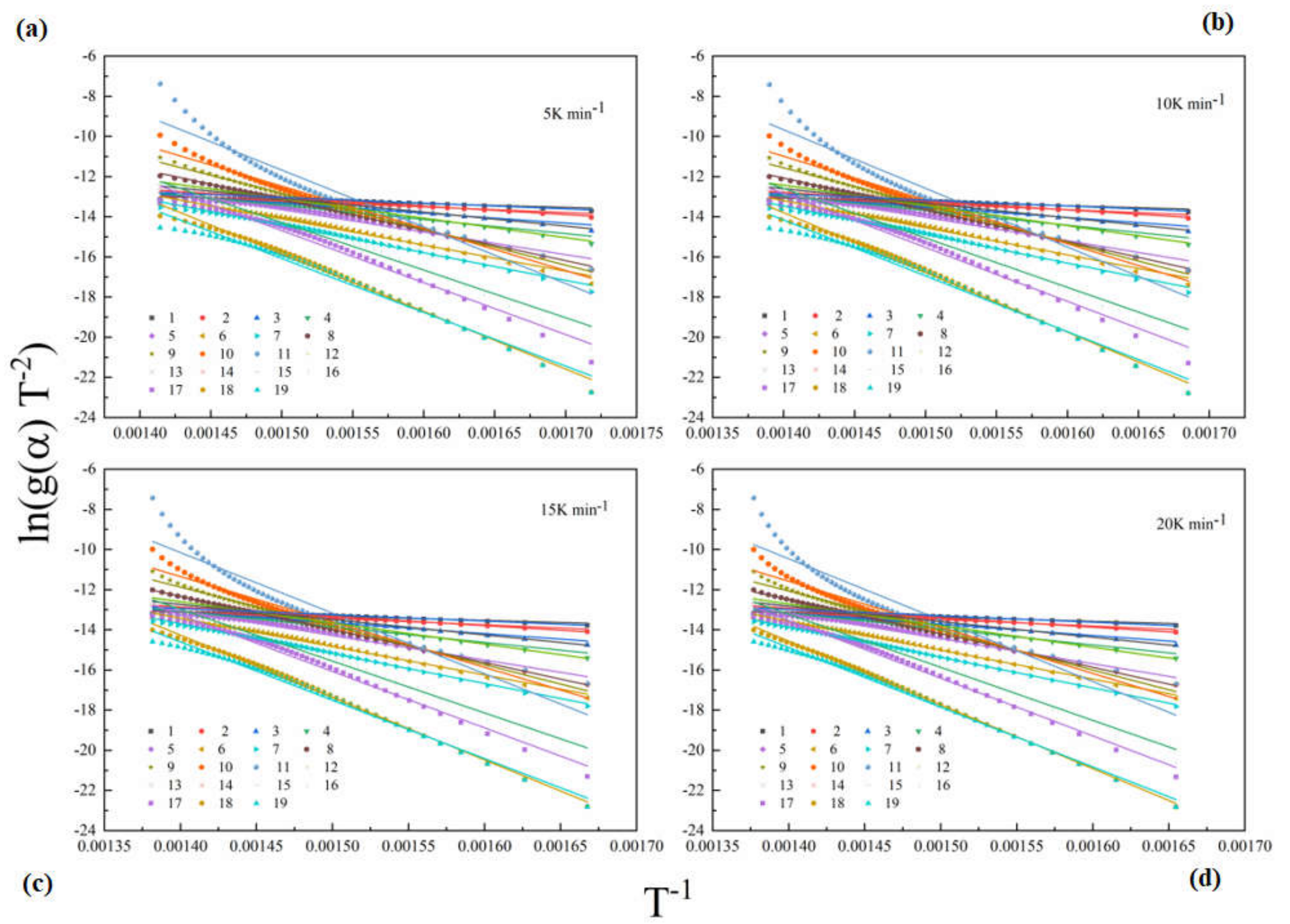
4.3. Model Fitting Analysis
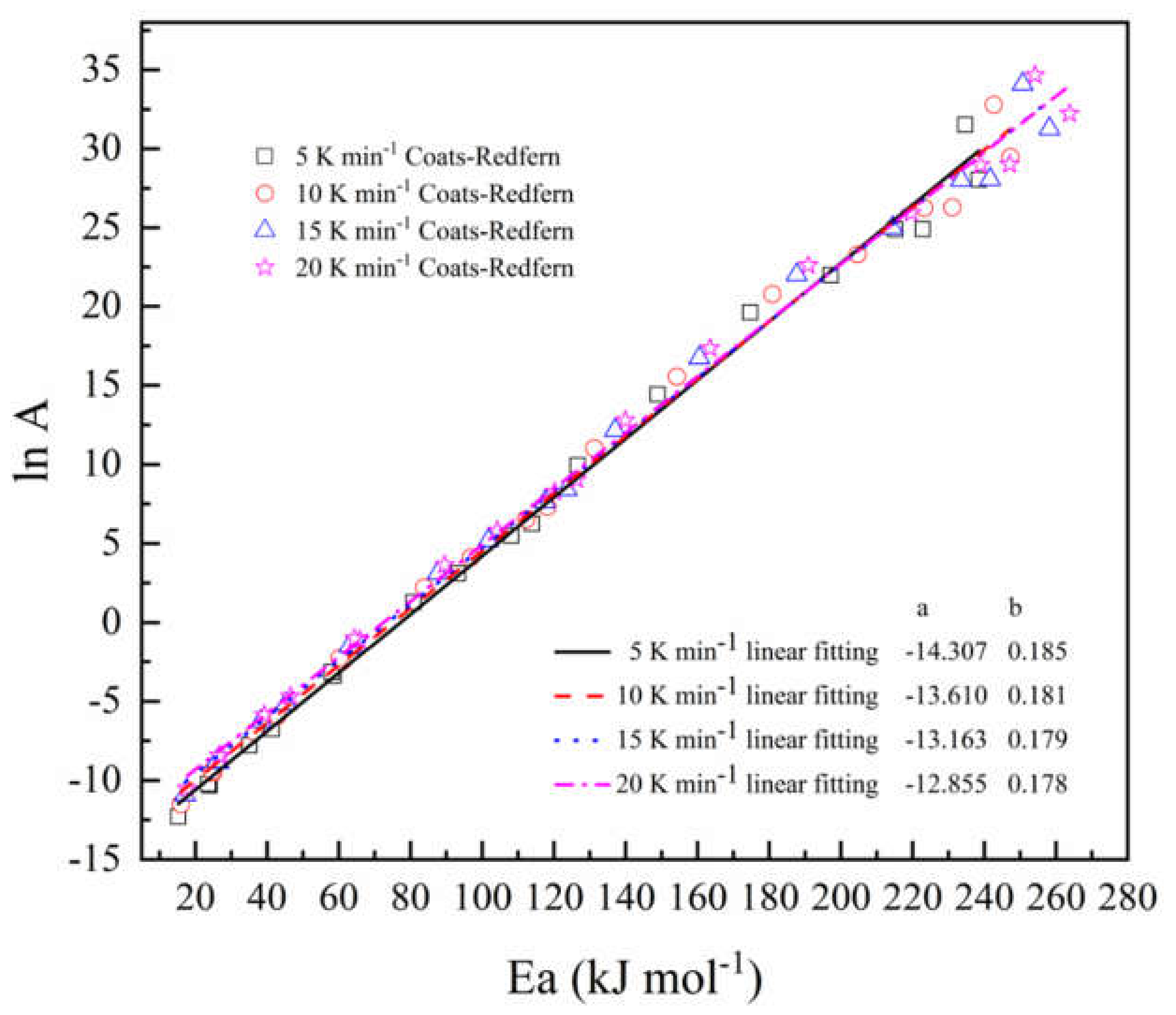
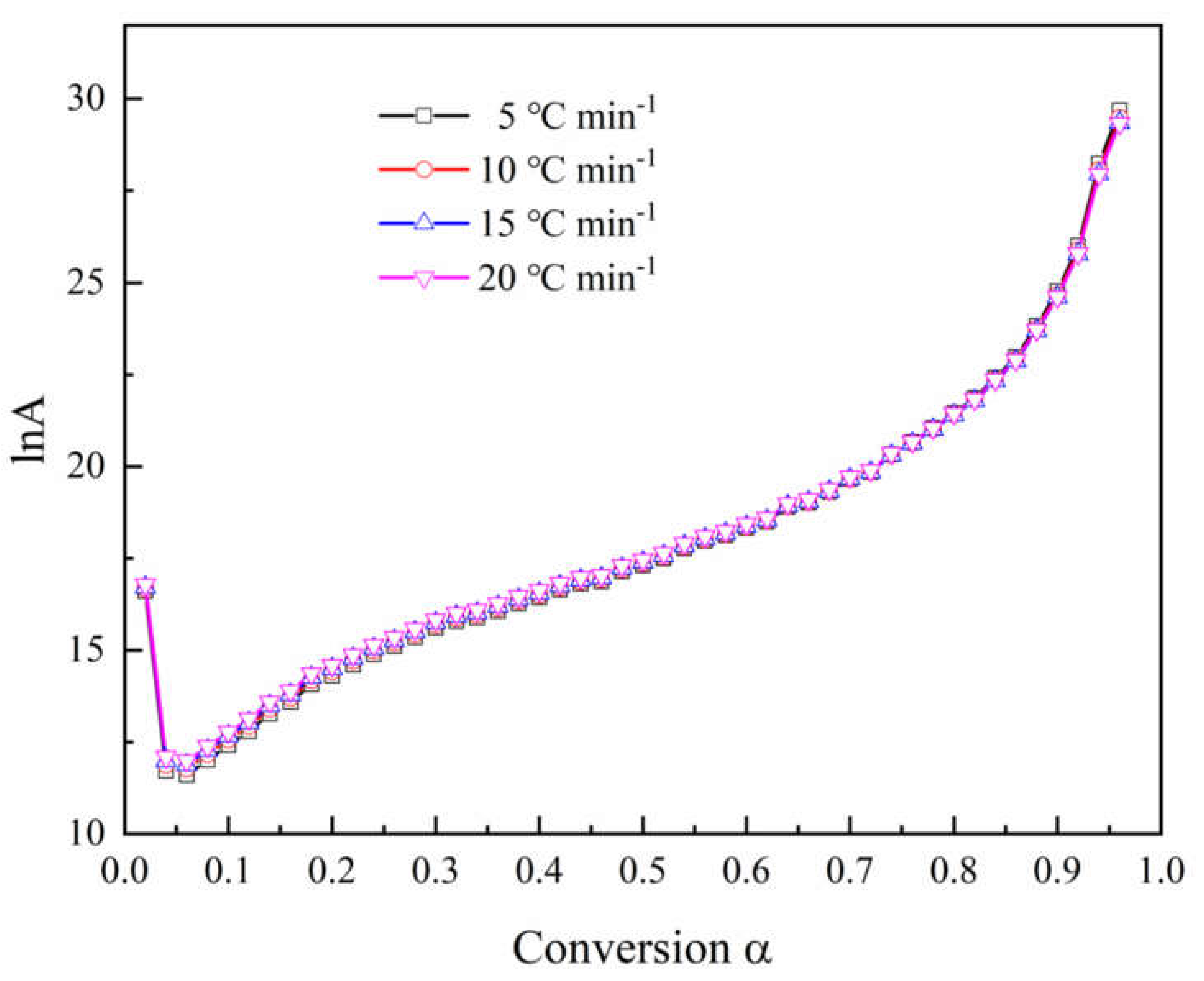
4.4. Kinetic Compensation Effect
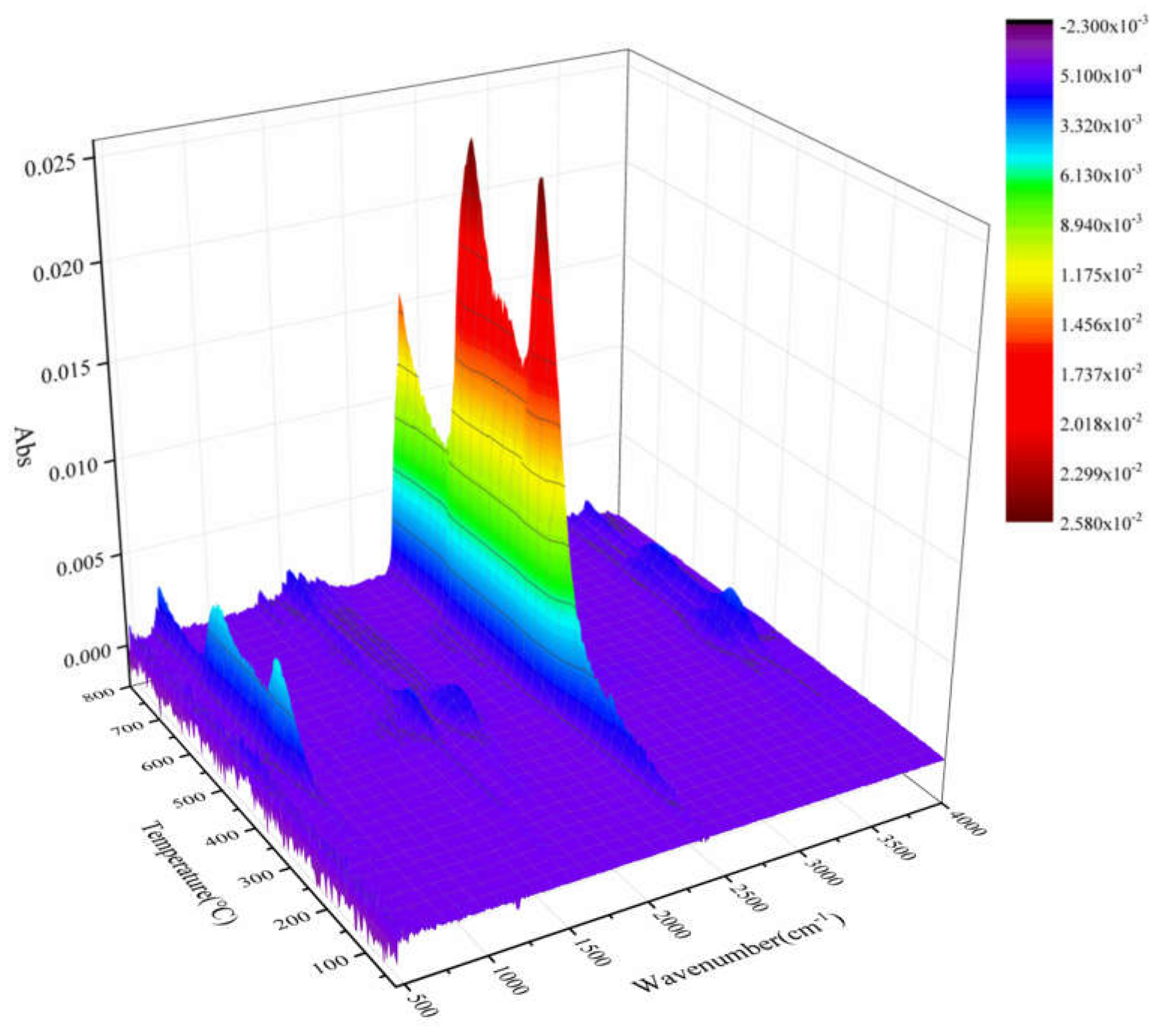
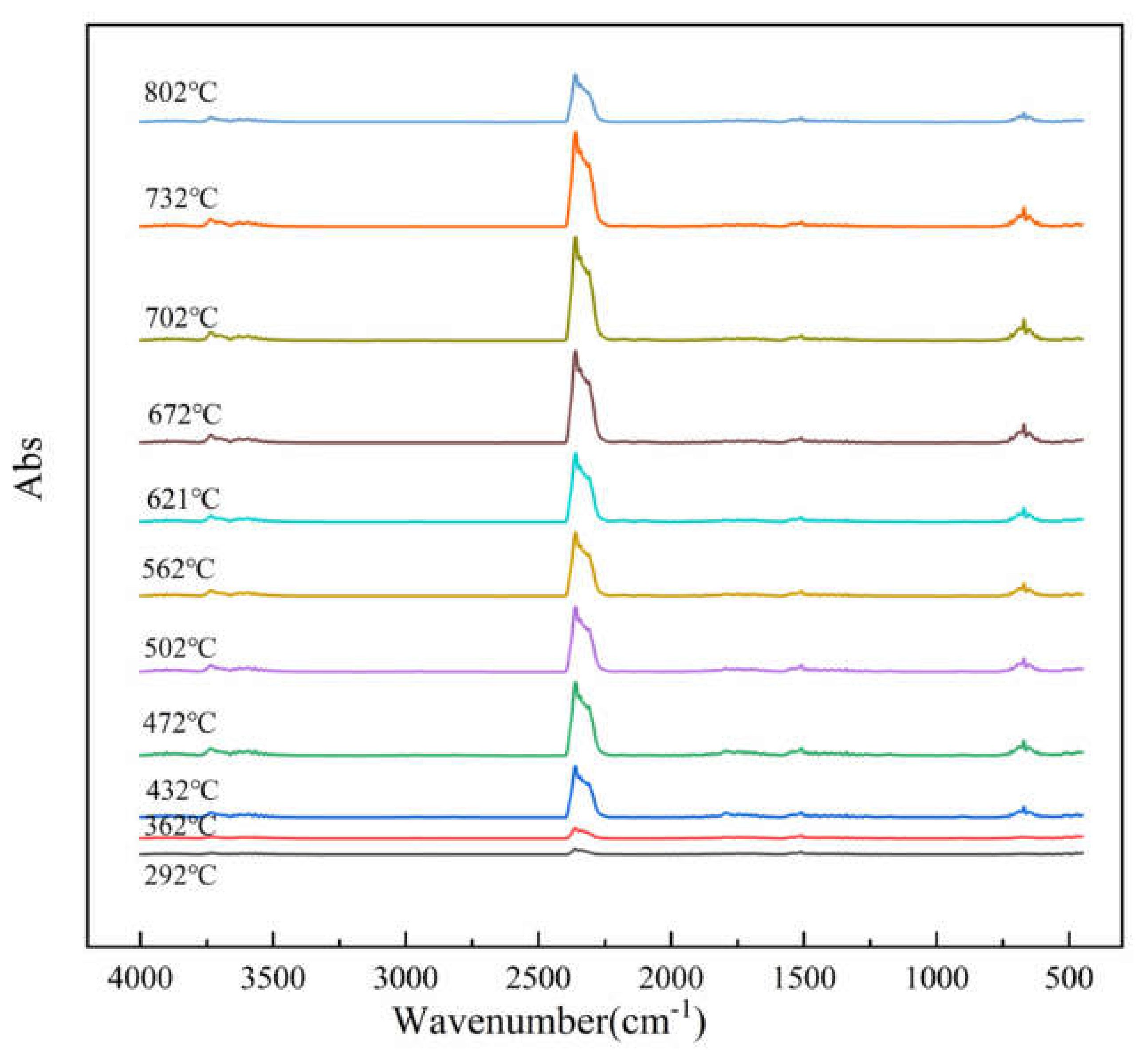
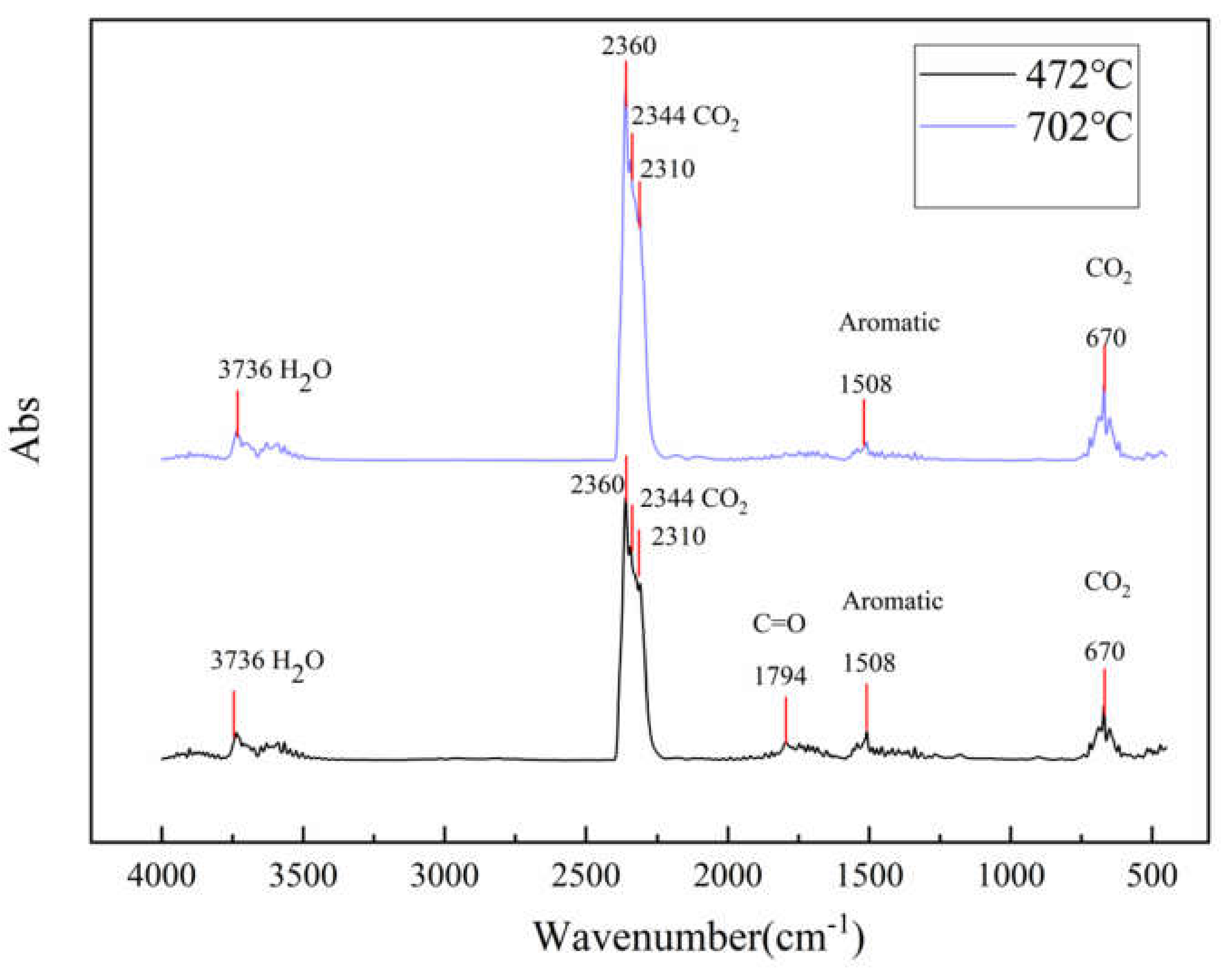
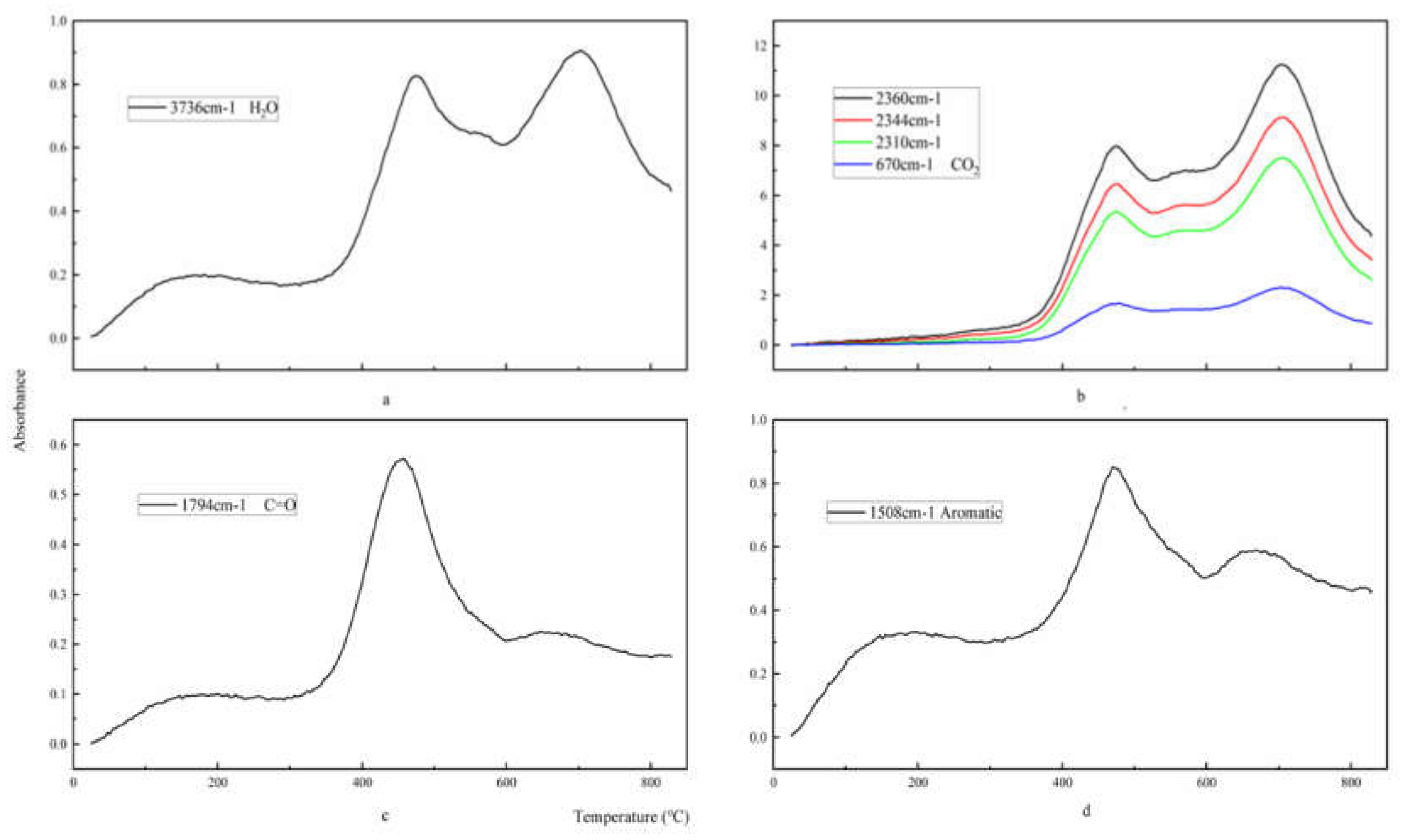
4.5. FTIR Analysis
5. Conclusions
- (1)
- The pyrolysis process of epoxy glass fiber reinforced plastic in a nitrogen atmosphere can be roughly divided into two stages. During the pyrolysis stage, the main volatile products are H2O (3736 cm−1), CO2 (2360, 2344, 2310 and 670 cm−1), carbonyl components (1794 cm−1), and aromatic components (1510 cm−1). The first stage corresponds to the temperature range of 290–460 °C, and the weight loss is about 20%. In the first stage, the main chain of the additives and epoxy resin matrix in the glass fiber reinforced plastic sample were broken and decomposed into small molecules such as H2O and CO2 gas and a large amount of volatile macromolecular benzene. The second stage corresponds to 460–1000 °C and the weight loss is about 5%. In the second stage, the volatile macromolecular benzene is further decomposed to generate a large amount of H2O and CO2.
- (2)
- Model-free and model fitting methods were employed to calculate kinetic parameters of epoxy glass fiber reinforced plastic. Through the model-free method, we found that the activation energy Ea of epoxy glass fiber reinforced plastic decreased firstly and then increased with the conversion rate α. When 0.02 < α < 0.08, Ea decreases from 172.4 to 153.1 kJ mol−1. When 0.08 < α < 0.96, Ea increases from 153.1 to 285 kJ mol−1. Through the model fitting method, we found that the three models with the best fitting under four different heating rates are first-order (F1), A3/2 (Avrami–Eroféev (n = 1.5)), and A2 (Avrami–Eroféev (n = 2)), respectively.
Author Contributions
Funding
Conflicts of Interest
References
- Shamsuddoha, M.; Djukic, L.P.; Islam, M.M.; Aravinthan, T.; Manalo, A. Mechanical and thermal properties of glass fiber-vinyl ester resin composite for pipeline repair exposed to hot-wet conditioning. J. Compos. Mater. 2016, 51, 1605–1617. [Google Scholar] [CrossRef]
- Naresh, K.; Shankar, K.; Rao, B.S.; Shankar Velmurugan, R. Effect of high strain rate on glass/carbon/hybrid fiber reinforced epoxy laminated composites. Compos. Part B 2016, 100, 125–135. [Google Scholar] [CrossRef]
- Xu, D.S.; Liu, H.B.; Luo, W.L. Evaluation of interface shear behavior of glass fiber reinforced plastic soil nails with a strain-transfer model and distributed fiber-optic sensors. Comput. Geotech. 2017, 95, 180–190. [Google Scholar] [CrossRef]
- Xue, W.C.; Wang, W.; Fu, K. Experimental study on tensile properties of glass fiber reinforced plastic rebars under different stress levels as exposed to alkaline solution. Acta Mater. Compos. Sin. 2013, 30, 67–75. [Google Scholar]
- Kashi, A.; Ramezanianpour, A.A.; Moodi, F. Effect of cement based coatings on durability enhancement of glass fiber reinforced plastic-wrapped columns in marine environments. Constr. Build. Mater. 2017, 137, 307–316. [Google Scholar] [CrossRef]
- Garcia-Espinel, J.D.; Castro-Fresno, D.; Gayo, P.P.; Ballester-Munoz, F. Effects of sea water environment on glass fiber reinforced plastic materials used for marine civil engineering constructions. Mater. Des. 2015, 66, 46–50. [Google Scholar] [CrossRef]
- Li, Y.C. Application situation of FRP and composite for automobile and its development trend at home and abroad. Thermoseting Resin 2001, 16, 14–17. [Google Scholar]
- Yan, S.C.; Liang, K.R. Development, application and prospect of FRP/composite. Guangdong Chem. Ind. 2014, 41, 72–73. [Google Scholar]
- Yun, Y.M.; Seo, M.W.; Koo, G.H.; Sang, J.Y.; Yong, K.K.; Jae, G.L.; Jae, H.K. Pyrolysis characteristics of glass fiber reinforced plastic (Glass Fiber Reinforced Plastic) under non-isothermal conditions. Fuel 2014, 137, 321–327. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, Z.; Xu, Y.Y. Study on pyrolysis kinetics of high-strength glass fiber/epoxy resin composites. Fire Tech. 2017, 36, 149–152. [Google Scholar]
- Zheng, L.F.; Wang, Z.Z.; Xie, Y.J.; Yue, L.N.; Wang, L. Study on the thermal decomposition kinetics of glass fiber reinforced plastic under γ irradiation. Chin. J. Chem. Eng. 2018, 69, 161–169. [Google Scholar]
- Yang, X.T.; Tang, L.; Guo, Y.Q. Improvement of thermal conductivities for PPS dielectric nanocomposites via incorporating NH2-POSS functionalized nBN fillers. Compos. Part A Appl. Sci. 2017, 101, 237–242. [Google Scholar] [CrossRef]
- Shi, J.; Bao, L. Optimum decomposition conditions for glass fiber reinforced plastic recycling by superheated steam. Jpn. J. Appl. Phys. 2011, 50. [Google Scholar] [CrossRef]
- Chen, K.S.; Yeh, R.Z. Pyrolysis kinetics of epoxy resin in a nitrogen atmosphere. J. Hazard. Mater. 1996, 49, 105–113. [Google Scholar] [CrossRef]
- Nakagawa, H.; Tsuge, S.; Koyama, T. Studies on thermal degradation of epoxy resins by high-resolution pyrolysis-gas chromatography. J. Anal. Appl. Pyrol. 1987, 12, 97–113. [Google Scholar] [CrossRef]
- Kissinger, H.E. Reaction Kinetics in Differential Thermal Analysis. Anal. Chem. 1957, 29, 1702–1706. [Google Scholar] [CrossRef]
- Akahira, T.; Sunose, T. Method of determining activation deterioration constant of electrical insulating materials. Res. Rep. Chiba Inst. Technol. 1971, 16, 22–31. [Google Scholar]
- Flynn, J.H.; Wall, L.A. A quick direct method for the determination of activation energy from thermogravimetric data. Polym. Sci. Pol. Lett. 1966, 4, 323–328. [Google Scholar] [CrossRef]
- Ozawa, T. A new method of analyzing thermogravimetric data. Bull. Chem. Soc. Jpn. 1965, 38, 1881–1886. [Google Scholar] [CrossRef]
- Meng, X.L.; Wang, D.D.; Wang, P. Thermal degradation kinetics of poly (benzobisoxazole imide). Acta Polym. Sin. 2013, 2, 154–159. [Google Scholar]
- Tian, X.J.; Wang, Z.W.; Yu, Q.; Gao, J. Kinetics of thermal decomposition of epoxy resins modified with phosphorus-containing flame retardant. CIESC J. 2014, 65, 5082–5089. [Google Scholar]
- Cao, H.L.; Li, G.Q.; Huang, S.H. Research on kinetic characteristics of cattle manure pyrolysis using isoconversional methods. Acta Eng. Solar. Sin. 2015, 36, 1773–1778. [Google Scholar]
- Gao, X.; Jiang, L.; Xu, Q.; Wu, W.-Q.; Mensah, R.A. Thermal kinetics and reactive mechanism of cellulose nitrate decomposition by traditional multi kinetics and modeling calculation under isothermal and non-isothermal conditions. Ind. Crop. Prod. 2020, 145, 112085. [Google Scholar] [CrossRef]
- Wu, B.; Wang, Y.Z.; Wang, X.L. Kinetics of thermal oxidative degradation of phosphorus-containing flame retardant copolyesters. Polym. Degrad. Stabil. 2002, 76, 401–409. [Google Scholar] [CrossRef]
- Wang, Q.F.; Shi, W.F. Kinetics study of thermal decomposition of epoxy resins containing flame retardant components. Polym. Degrad. Stabil. 2006, 91, 1747–1754. [Google Scholar] [CrossRef]
- Vyazovkin, S.; Dollimore, D. Linear and nonlinear procedures in isoconversional computations of the activation energy of nonisothermal reactions in solids. Chem. Inf. Model. 1996, 36, 42–45. [Google Scholar] [CrossRef]
- Vyazovkin, S. Advanced isoconversional method. J. Therm. Anal. Calorim. 1997, 49, 1493–1499. [Google Scholar] [CrossRef]
- Vyazovkin, S. Evaluation of activation energy of thermally stimulated solid-state reactions under arbitrary variation of temperature. Comput. Chem. 1997, 18, 393–402. [Google Scholar] [CrossRef]
- Farjas, J.; Roura, P. Isoconversional analysis of solid state transformations. J. Therm. Anal. Calorim. 2011, 105, 757–766. [Google Scholar] [CrossRef]
- Jiang, L.; Zhang, D.; Li, M. Pyrolytic behavior of waste extruded polystyrene and rigid polyurethane by multi kinetics methods and Py-GC/M. Fuel 2018, 222, 11–20. [Google Scholar] [CrossRef]
- Vyazovkin, S.; Wight, C.A. Isothermal and Nonisothermal Reaction Kinetics in Solids: In Search of Ways toward Consensus. J. Phys. Chem. A 1997, 101, 8279–8284. [Google Scholar] [CrossRef]
- Coats, A.W.; Redfern, J.P. Kinetic parameters from thermogravimetric data. Nature 1964, 201, 68–69. [Google Scholar] [CrossRef]
- Coats, A.W.; Redfern, J.P. Kinetic parameters from thermogravimetric data. II. J. Polym. Sci. Part B Polym. Lett. 1965, 3, 917–920. [Google Scholar] [CrossRef]
- Hornsby, P.R.; Rothon, R.; Jackson, G. Thermal decomposition behaviour of polyamide fire-retardant compositions containing magnesium hydroxide filler. Polym. Degrad. Stabil. 1996, 51, 235–249. [Google Scholar] [CrossRef]


| Reaction Model | Model Code | f(α) | g(α) |
|---|---|---|---|
| P1 | Power law | 4α3/4 | α1/4 |
| P2 | Power law | 3α2/3 | α1/3 |
| P3 | Power law | 2α1/2 | α1/2 |
| P4 | Power law | 2/3α−1/2 | α3/2 |
| R1 | Zero-order (Polany-Winger Equation) | 1 | α |
| R2 | Phase-boundary controlled reaction | 2(1 − α)1/2 | [1 − (1 − α)1/2] |
| R3 | Phase-boundary controlled reaction | 3(1 − α)2/3 | [1 − (1 − α)1/3] |
| F1 | First-order | (1 − α) | −ln(1 − α) |
| F3/2 | Three-halves order | (1 − α)3/2 | 2[(1 − α)−1/2 − 1] |
| F2 | Second-order | (1 − α)2 | (1 − α)−1 − 1 |
| F3 | Third-order | (1 − α)3 | (1/2)[(1 − α)−2 − 1] |
| A3/2 | Avrami–Eroféev (n = 1.5) | (3/2)(1−α)[−ln(1 − α)]1/3 | [−ln(1 − α)]2/3 |
| A2 | Avrami–Eroféev (n = 2) | 2(1 − α)[−ln(1 − α)]1/2 | [−ln(1 − α)]1/2 |
| A3 | Avrami–Eroféev (n = 3) | 3(1 − α)[−ln(1 − α)]2/3 | [−ln(1 − α)]1/3 |
| A4 | Avrami–Eroféev (n = 4) | 4(1 − α)[−ln(1 − α)]3/4 | [−ln(1 − α)]1/4 |
| D1 | One-dimensional diffusion | 1/2α | α2 |
| D2 | Two-dimensional diffusion | 1/[−ln(1 − α)] | (1 − α)ln(1 − α) + α |
| D3 | Three-dimensional diffusion | 3(1 − α)1/3/2[(1 − α)−1/3 − 1] | [1 − (1 − αα)1/3]2 |
| D4 | Three-dimensional diffusion | 3/2[(1 − α)−1/3 − 1] | (1 − 2α/3) − (1 − α)2/3 |
| β (°C min−1) | T0 (°C) | Tmax (°C) | Tf (°C) |
|---|---|---|---|
| 5 | 234.9 | 388.6 | 479.6 |
| 10 | 253.5 | 403.9 | 520.9 |
| 15 | 257.2 | 412.2 | 502.1 |
| 20 | 273.9 | 421.5 | 513.7 |
| 5 °C min−1 | 10 °C min−1 | 15 °C min−1 | 20 °C min−1 | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Model | lnA | Ea | R2 | Model | lnA | Ea | R2 | Model | lnA | Ea | R2 | Model | lnA | Ea | R2 | |
| glass fiber reinforced plastic | F1 | 9.94 | 126.61 | 0.9985 | F1 | 11.01 | 131.31 | 0.9991 | F1 | 12.16 | 137.04 | 0.9998 | F1 | 12.78 | 139.90 | 0.9993 |
| A3/2 | 1.28 | 80.83 | 0.9983 | A3/2 | 2.22 | 83.89 | 0.9990 | A3/2 | 3.13 | 87.67 | 0.9998 | A3/2 | 3.65 | 89.56 | 0.9992 | |
| A2 | −3.16 | 57.94 | 0.9980 | A2 | −2.28 | 60.18 | 0.9988 | A2 | −1.49 | 62.98 | 0.9998 | A2 | −1.03 | 64.38 | 0.9992 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiao, Y.; Das, O.; Zhao, S.-N.; Sun, T.-S.; Xu, Q.; Jiang, L. Pyrolysis Kinetic Study and Reaction Mechanism of Epoxy Glass Fiber Reinforced Plastic by Thermogravimetric Analyzer (TG) and TG–FTIR (Fourier-Transform Infrared) Techniques. Polymers 2020, 12, 2739. https://doi.org/10.3390/polym12112739
Qiao Y, Das O, Zhao S-N, Sun T-S, Xu Q, Jiang L. Pyrolysis Kinetic Study and Reaction Mechanism of Epoxy Glass Fiber Reinforced Plastic by Thermogravimetric Analyzer (TG) and TG–FTIR (Fourier-Transform Infrared) Techniques. Polymers. 2020; 12(11):2739. https://doi.org/10.3390/polym12112739
Chicago/Turabian StyleQiao, Yuanhua, Oisik Das, Shu-Na Zhao, Tong-Sheng Sun, Qiang Xu, and Lin Jiang. 2020. "Pyrolysis Kinetic Study and Reaction Mechanism of Epoxy Glass Fiber Reinforced Plastic by Thermogravimetric Analyzer (TG) and TG–FTIR (Fourier-Transform Infrared) Techniques" Polymers 12, no. 11: 2739. https://doi.org/10.3390/polym12112739
APA StyleQiao, Y., Das, O., Zhao, S.-N., Sun, T.-S., Xu, Q., & Jiang, L. (2020). Pyrolysis Kinetic Study and Reaction Mechanism of Epoxy Glass Fiber Reinforced Plastic by Thermogravimetric Analyzer (TG) and TG–FTIR (Fourier-Transform Infrared) Techniques. Polymers, 12(11), 2739. https://doi.org/10.3390/polym12112739