Thermomechanical Modeling of Microstructure Evolution Caused by Strain-Induced Crystallization
Abstract
1. Introduction
2. Thermodynamically Consistent Framework
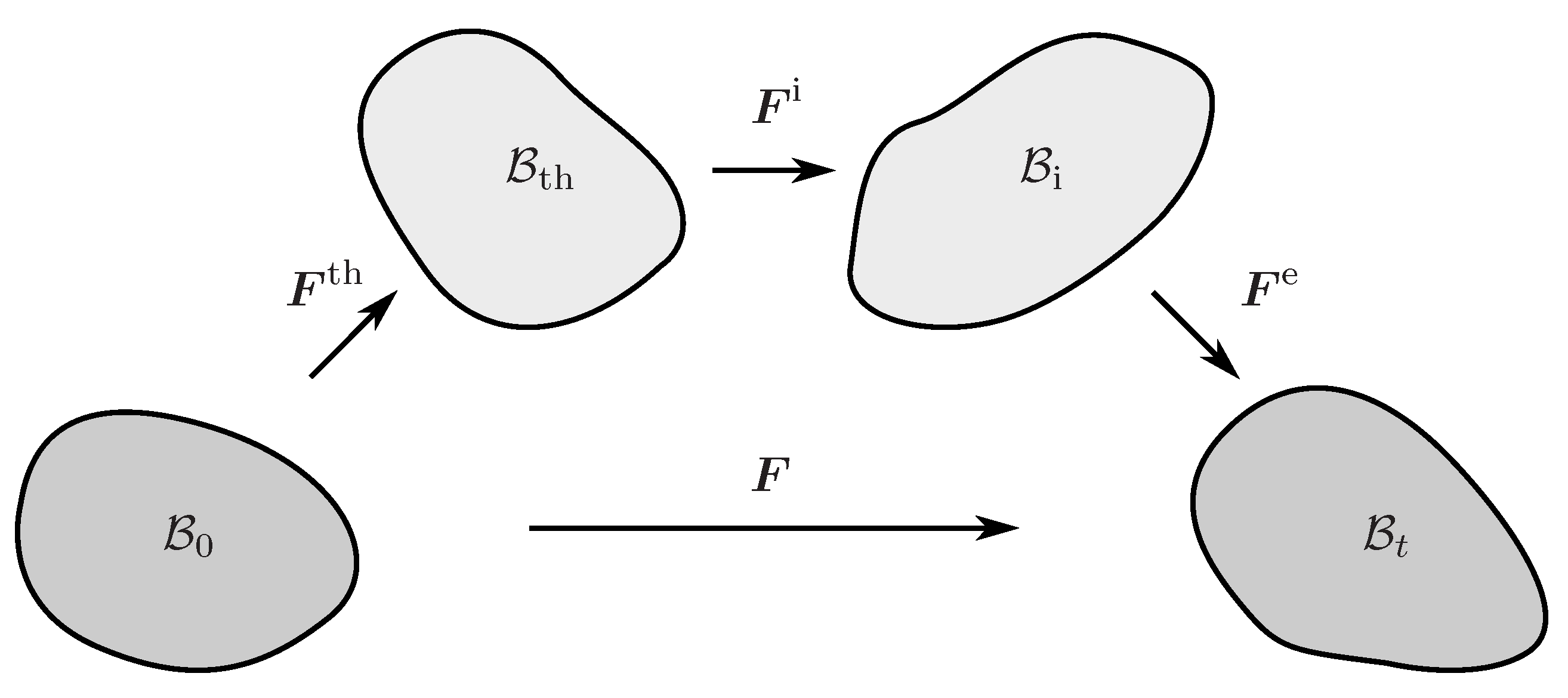
2.1. Kinematics within the Finite Thermomechanical Framework
2.2. The Arruda–Boyce Elastic Energy
2.3. Thermodynamic Consistency
2.4. Derivation of Evolution Equations
2.5. Balance Equations
3. Thermomechanical Modeling of the SIC
3.1. Definition of Internal Variables
3.2. Assumption for the Helmholtz Free Energy Density
3.3. Coupling Conditions
3.4. Derivation of Driving Forces
3.5. Assumption for the Dissipation Potential and Derivation of Evolution Equations
4. Numerical Implementation of the SIC Model
4.1. Implementation of the Thermomechanical Coupled Problem into the FEM
4.2. Time Discretization and Simulation of the Unloading Phase
4.3. Algorithmic Aspects of the SIC Model Implementation
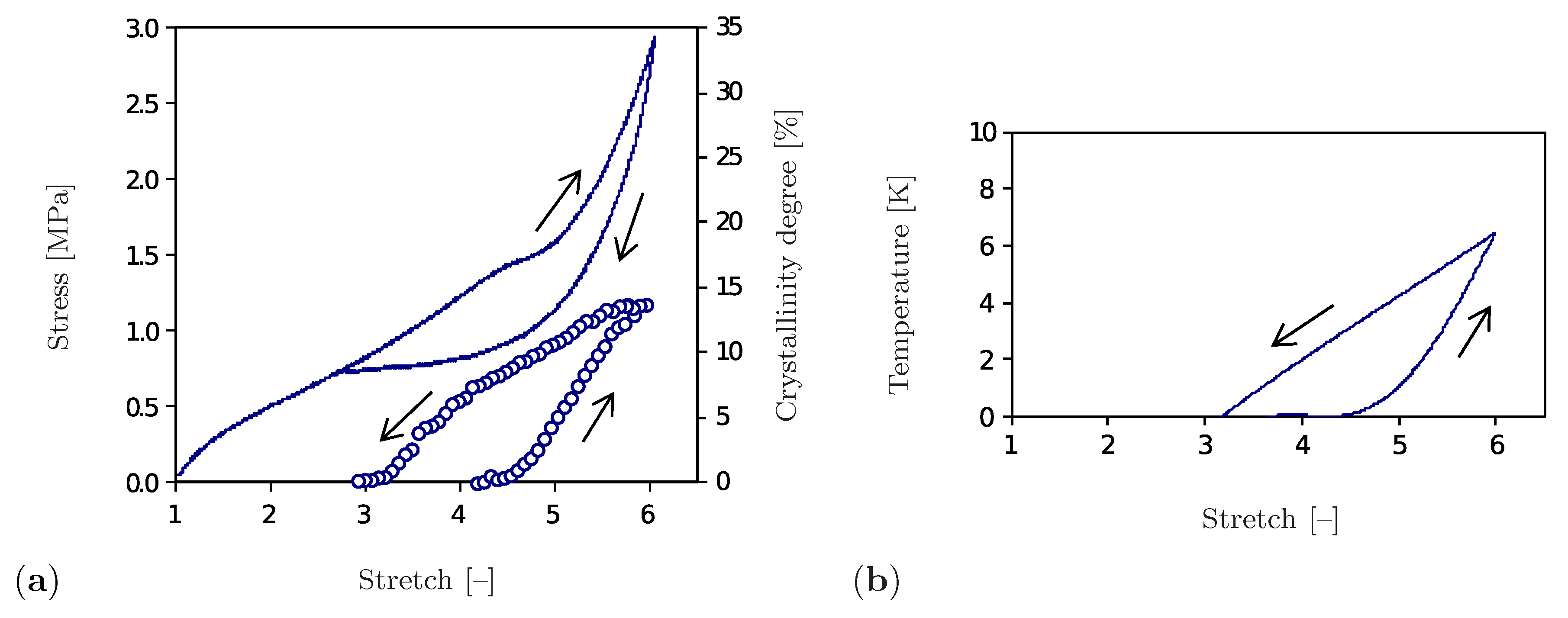
5. Numerical Examples
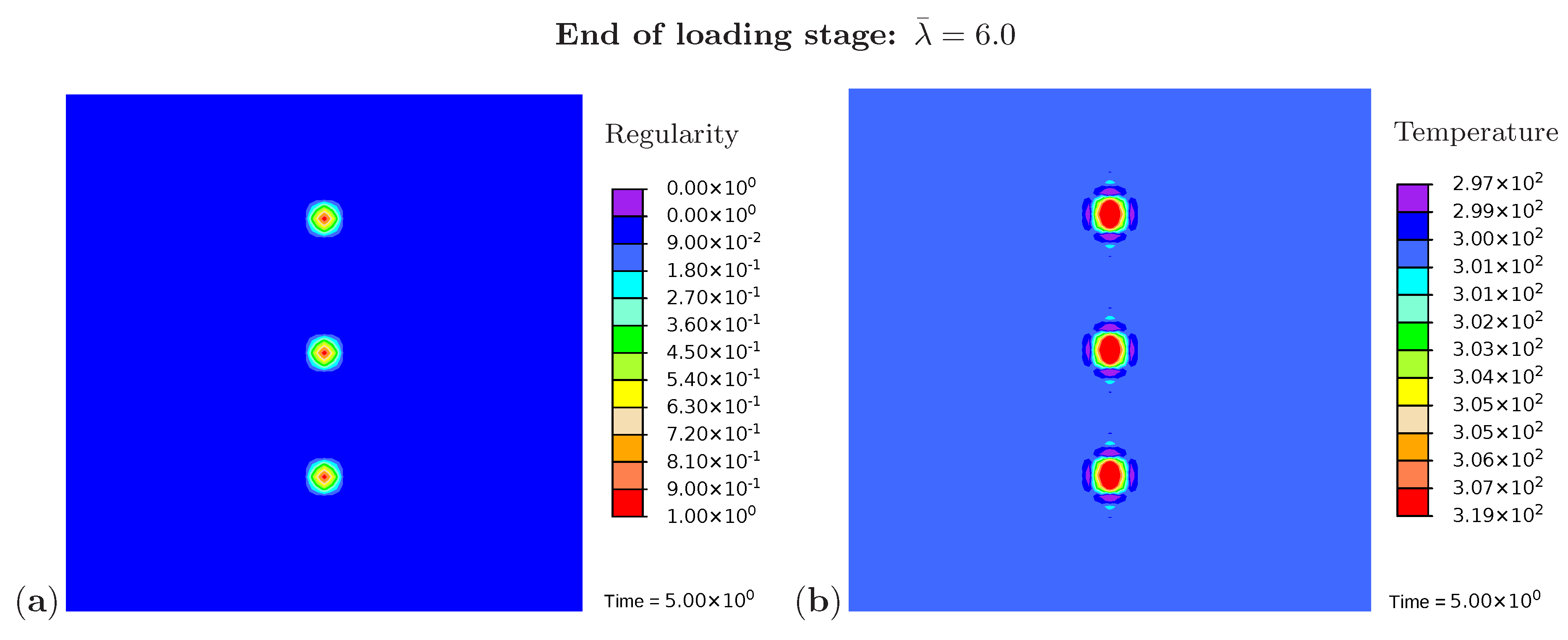
5.1. Simulation of Single Crystalline Regions Embedded in the Matrix Material
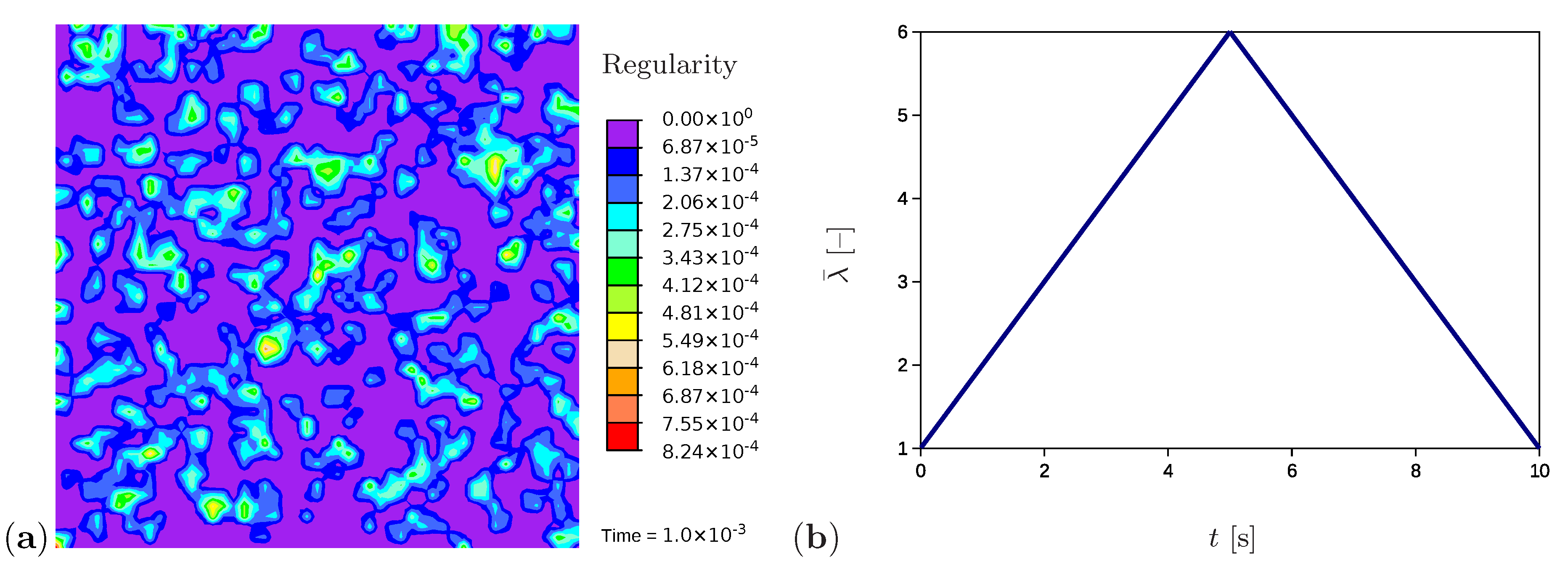
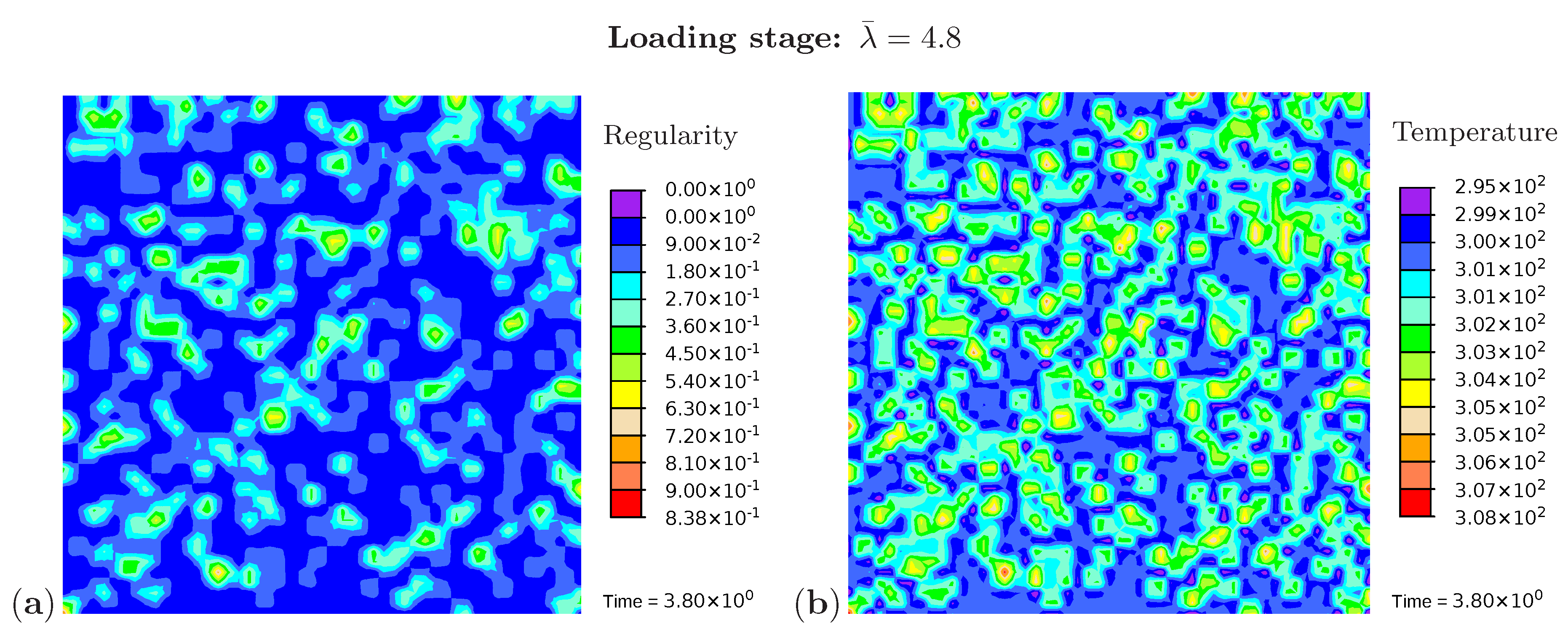
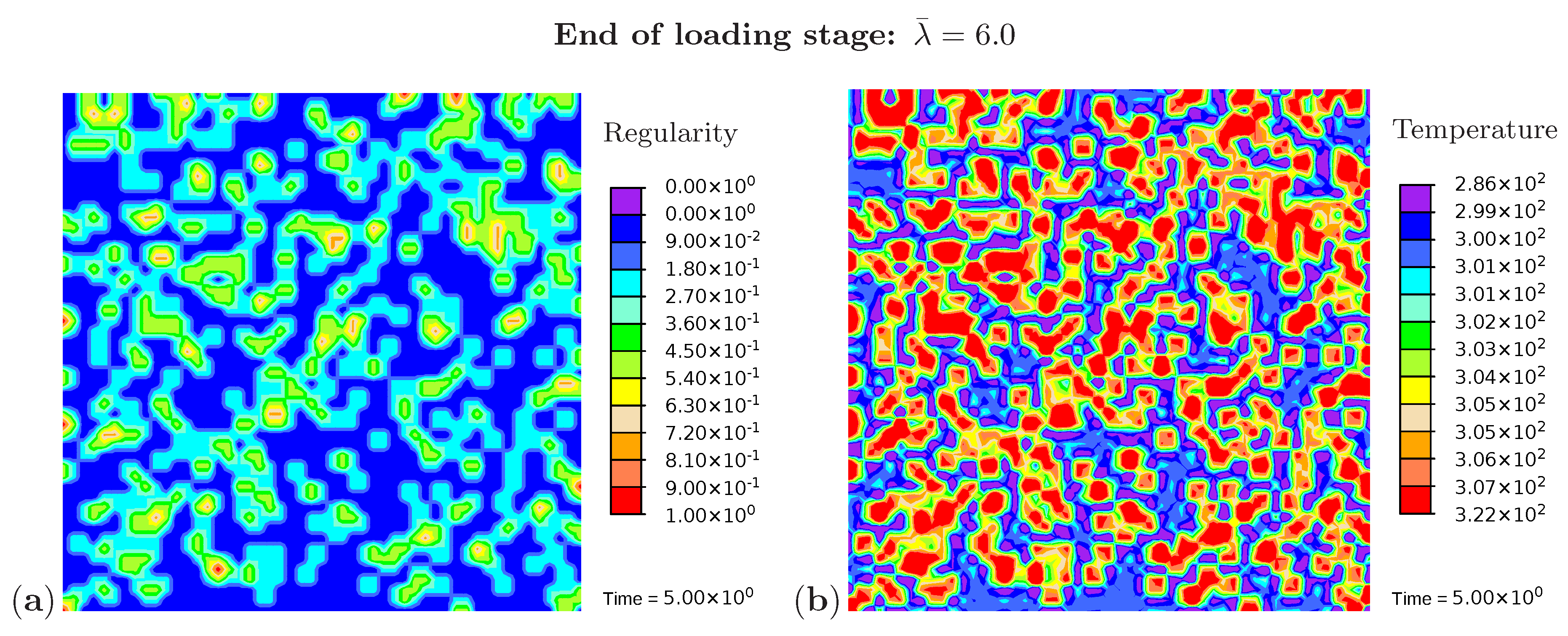
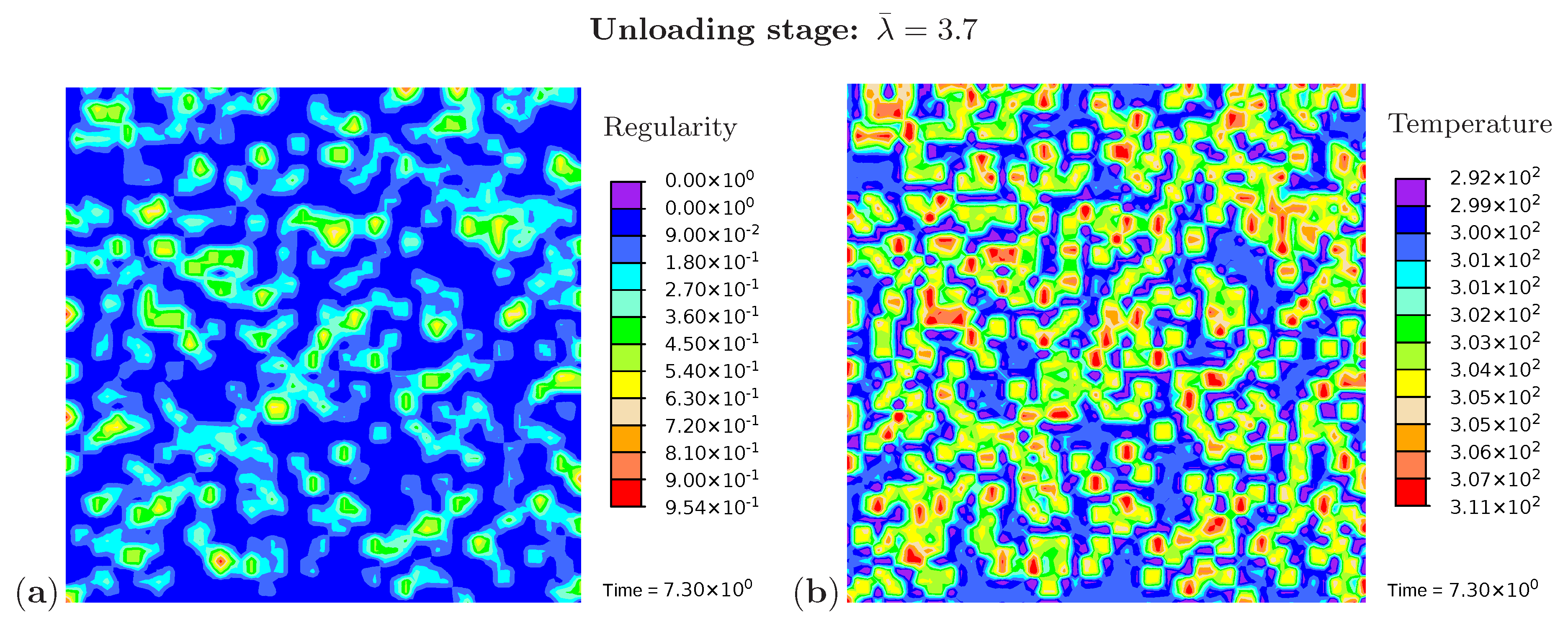
5.2. Microstructure Evolution for a Sample with the Complex Initial Microconfiguration
6. Conclusions and Outlook
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Le Cam, J.B. A review of volume changes in rubbers: The effect of stretching. Rubber Chem. Technol. 2010, 83, 247–269. [Google Scholar] [CrossRef]
- Qu, L.; Huang, G.; Liu, Z.; Zhang, P.; Weng, G.; Nie, Y. Remarkable reinforcement of natural rubber by deformation-induced crystallization in the presence of organophilic montmorillonite. Acta Mater. 2009, 57, 5053–5060. [Google Scholar] [CrossRef]
- Kojio, K.; Matsuo, K.; Motokucho, S.; Yoshinaga, K.; Shimodaira, Y.; Kimura, K. Simultaneous small-angle X-ray scattering/wide-angle X-ray diffraction study of the microdomain structure of polyurethane elastomers during mechanical deformation. Polym. J. 2011, 43, 692–699. [Google Scholar] [CrossRef]
- Sommer, S.; Raidt, T.; Fischer, B.; Katzenberg, F.; Tiller, J.; Koch, M. THz-Spectroscopy on High Density Polyethylene with Different Crystallinity. J. Infrared Millim. Terahertz Waves 2016, 37, 189–197. [Google Scholar] [CrossRef]
- Beurrot-Borgarino, S.; Huneau, B.; Verron, E.; Thiaudière, D.; Mocuta, C.; Zozulya, A. Characteristics of strain-induced crystallization in natural rubber during fatigue testing: In situ wide-angle x-ray diffraction measurements using synchrotron radiation. Rubber Chem. Technol. 2014, 87, 184–196. [Google Scholar] [CrossRef]
- Tosaka, M.; Senoo, K.; Sato, K.; Noda, M.; Ohta, N. Detection of fast and slow crystallization processes in instantaneously-strained samples of cis-1,4-polyisoprene. Polymer 2012, 53, 864–872. [Google Scholar] [CrossRef]
- Brüning, K.; Schneider, K.; Roth, S.; Heinrich, G. Kinetics of Strain-Induced Crystallization in Natural Rubber Studied by WAXD: Dynamic and Impact Tensile Experiments. Macromolecules 2012, 45, 7914–7919. [Google Scholar] [CrossRef]
- Candau, N.; Laghmach, R.; Chazeau, L.; Chenal, J.M.; Gauthier, C.; Biben, T.; Munch, E. Strain-Induced Crystallization of Natural Rubber and Cross-Link Densities Heterogeneities. Macromolecules 2014, 47, 5815–5824. [Google Scholar] [CrossRef]
- Spratte, T.; Plagge, J.; Wunde, M.; Klüppel, M. Investigation of strain-induced crystallization of carbon black and silica filled natural rubber composites based on mechanical and temperature measurements. Polymer 2017, 115, 12–20. [Google Scholar] [CrossRef]
- Le Cam, J.B. Strain-induced crystallization in rubber: A new measurement technique. Strain 2018, 54, e12256. [Google Scholar] [CrossRef]
- Le Cam, J.B. Measuring Strain-Induced Crystallinity in Rubbers from IR Thermography. In Residual Stress, Thermomechanics & Infrared Imaging, Hybrid Techniques and Inverse Problems; Baldi, A., Quinn, S., Balandraud, X., Dulieu-Barton, J.M., Bossuyt, S., Eds.; Springer International Publishing: Cham, Switzerland, 2019; Volume 7, pp. 57–62. [Google Scholar]
- Candau, N.; Laghmach, R.; Chazeau, L.; Chenal, J.M.; Gauthier, C.; Biben, T.; Munch, E. Influence of strain rate and temperature on the onset of strain induced crystallization in natural rubber. Eur. Polym. J. 2015, 64, 244–252. [Google Scholar] [CrossRef]
- Alfrey, T.; Mark, H. A Statistical Treatment of Crystallization Phenomena in High Polymers. J. Phys. Chem. 1942, 46, 112–118. [Google Scholar] [CrossRef]
- Flory, P.J. Thermodynamics of Crystallization in High Polymers. I. Crystallization Induced by Stretching. J. Phys. Chem. 1947, 15, 397–408. [Google Scholar] [CrossRef]
- Flory, P.J. Thermodynamics of Crystallization in High Polymers. IV. A Theory of Crystalline States and Fusion in Polymers, Copolymers, and Their Mixtures with Diluents. J. Phys. Chem. 1949, 17, 223–240. [Google Scholar] [CrossRef]
- Doufas, A.K.; Dairanieh, I.S.; McHugh, A.J. A continuum model for flow-induced crystallization of polymer melts. J. Rheol. 1999, 43, 85–109. [Google Scholar] [CrossRef]
- Ahzi, S.; Makradi, A.; Gregory, R.; Edie, D. Modeling of deformation behavior and strain-induced crystallization in poly(ethylene terephthalate) above the glass transition temperature. Mech. Mater. 2003, 35, 1139–1148. [Google Scholar] [CrossRef]
- Arruda, E.M.; Boyce, M.C. A three-dimensional constitutive model for the large stretch behavior of rubber elastic materials. J. Mech. Phys. Solids 1993, 41, 389–412. [Google Scholar] [CrossRef]
- Negahban, M. Modeling the thermomechanical effects of crystallization in natural rubber: III. Mechanical properties. Int. J. Solids Struct. 2000, 37, 2811–2824. [Google Scholar] [CrossRef]
- Rao, I.; Rajagopal, K. A study of strain-induced crystallization of polymers. Int. J. Solids Struct. 2001, 38, 1149–1167. [Google Scholar] [CrossRef]
- Tosaka, M.; Murakami, S.; Poompradub, S.; Kohjiya, S.; Ikeda, Y.; Toki, S.; Sics, I.; Hsiao, B.S. Orientation and Crystallization of Natural Rubber Network As Revealed by WAXD Using Synchrotron Radiation. Macromolecules 2004, 37, 3299–3309. [Google Scholar] [CrossRef]
- Kroon, M. A constitutive model for strain-crystallising Rubber-like materials. Mech. Mater. 2010, 42, 873–885. [Google Scholar] [CrossRef]
- Mistry, S.J.; Govindjee, S. A micro-mechanically based continuum model for strain-induced crystallization in natural rubber. Int. J. Solids Struct. 2014, 51, 530–539. [Google Scholar] [CrossRef]
- Nateghi, A.; Dal, H.; Keip, M.A.; Miehe, C. An affine microsphere approach to modeling strain-induced crystallization in rubbery polymers. Contin. Mech. Thermodyn. 2018, 30, 485–507. [Google Scholar] [CrossRef]
- Dargazany, R.; Khiêm, V.N.; Poshtan, E.A.; Itskov, M. Constitutive modeling of strain-induced crystallization in filled rubbers. Phys. Rev. E 2014, 89, 022604. [Google Scholar] [CrossRef]
- Behnke, R.; Berger, T.; Kaliske, M. Numerical modeling of time- and temperature-dependent strain-induced crystallization in rubber. Int. J. Solids Struct. 2018, 141–142, 15–34. [Google Scholar] [CrossRef]
- Khiêm, V.N.; Itskov, M. Analytical network-averaging of the tube model: Strain-induced crystallization in natural rubber. J. Mech. Phys. Solids 2018, 116, 350–369. [Google Scholar] [CrossRef]
- Khiêm, V.N.; Balandraud, X.; Itskov, M. Thermo-micromechanics of strain-induced crystallization. In Constitutive Models for Rubber XI; Huneau, B., Le Cam, J.B., Marco, Y., Verron, E., Eds.; Taylor & Francis Ltd.: London, UK, 2019; pp. 30–35. [Google Scholar]
- Felder, S.; Holthusen, H.; Hesseler, S.; Pohlkemper, F.; Simon, J.W.; Gries, T.; Reese, S. A finite strain thermo-mechanically coupled material model for semi-crystalline polymers. In XV International Conference on Computational Plasticity: Fundamentals and Applications; Onatea, E., Owen, D.R.J., Peric, D., Chiumenti, M., de Souza Neto, E., Eds.; CIMNE: Barcelona, Spain, 2019; pp. 249–260. [Google Scholar]
- Nie, Y. Strain-induced crystallization of natural rubber/zinc dimethacrylate composites studied using synchrotron X-ray diffraction and molecular simulation. J. Polym. Res. 2015, 22, 1. [Google Scholar] [CrossRef]
- Yamamoto, T. Molecular Dynamics Simulation of Stretch-Induced Crystallization in Polyethylene: Emergence of Fiber Structure and Molecular Network. Macromolecules 2019, 52, 1695–1706. [Google Scholar] [CrossRef]
- Aygün, S.; Klinge, S. Continuum mechanical modeling of strain-induced crystallization in polymers. Int. J. Solids Struct. 2020, 196–197, 129–139. [Google Scholar] [CrossRef]
- Klinge, S.; Bartels, A.; Steinmann, P. The multiscale approach to the curing of polymers incorporating viscous and shrinkage effects. Int. J. Solids Struct. 2012, 49, 3883–3900. [Google Scholar] [CrossRef]
- Liao, Z.; Hossain, M.; Yao, X.; Mehnert, M.; Steinmann, P. On thermo-viscoelastic experimental characterization and numerical modelling of VHB polymer. Int. J. Nonlin. Mech. 2020, 118, 103263. [Google Scholar] [CrossRef]
- Hartmann, S. Comparison of the Multiplicative Decompositions F = FΘFM and F = FMFΘ in Finite Strain Thermo-Elasticity; Technical report; Faculty of Mathematics/Computer Science and Mechanical Engineering, Clausthal University of Technology: Clausthal-Zellerfeld, Germany, 2012. [Google Scholar]
- Flory, P.J. Thermodynamic relations for high elastic materials. Trans. Faraday Soc. 1961, 57, 829–838. [Google Scholar] [CrossRef]
- Doll, S.; Hauptmann, R.; Schweizerhof, K.; Freischläger, C. On volumetric locking of low order solid and solid-shell elements for finite elastoviscoplastic deformations and selective reduced integration. Eng. Comput. 2000, 17, 874–902. [Google Scholar] [CrossRef]
- Steinmann, P.; Hossain, M.; Possart, G. Hyperelastic models for rubber-like materials: Consistent tangent operators and suitability for Treloar’s data. Arch. Appl. Mech. 2012, 82. [Google Scholar] [CrossRef]
- Hoss, L.; Marczak, R. A new constitutive model for rubber-like materials. AMCA 2010, 29, 2759–2773. [Google Scholar]
- Mansouri, M.; Darijani, H. Constitutive modeling of isotropic hyperelastic materials in an exponential framework using a self-contained approach. Int. J. Solids Struct. 2014, 51, 4316–4326. [Google Scholar] [CrossRef]
- Treloar, L.R.G. The photoelastic properties of short-chain molecular networks. Trans. Faraday Soc. 1954, 50, 881–896. [Google Scholar] [CrossRef]
- Itskov, M.; Dargazany, R.; Hörnes, K. Taylor expansion of the inverse function with application to the Langevin function. Math. Mech. Solids 2012, 17, 693–701. [Google Scholar] [CrossRef]
- Horgan, C.; Murphyj, J. Simple Shearing of Incompressible and Slightly Compressible Isotropic Nonlinearly Elastic Materials. J. Elast. 2010, 98, 205–221. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, X.; Yang, Q. Extracting the isotropic uniaxial stress-strain relationship of hyperelastic soft materials based on new nonlinear indentation strain and stress measure. AIP Adv. 2018, 8, 115013. [Google Scholar] [CrossRef]
- Jedynak, R. A comprehensive study of the mathematical methods used to approximate the inverse Langevin function. Math. Mech. Solids 2018, 24, 1992–2016. [Google Scholar] [CrossRef]
- Carroll, M.M. Molecular chain networks and strain energy functions in rubber elasticity. Philos. Trans. R. Soc. A 2019, 377, 20180067. [Google Scholar] [CrossRef]
- Simo, J.C.; Taylor, R.L. Quasi-incompressible finite elasticity in principal stretches. Continuum basis and numerical algorithms. Comput. Methods Appl. Mech. Eng. 1991, 85, 273–310. [Google Scholar] [CrossRef]
- Ogden, R.W.; Hill, R. Large deformation isotropic elasticity: On the correlation of theory and experiment for compressible rubberlike solids. Proc. R. Soc. A 1972, 328, 567–583. [Google Scholar] [CrossRef]
- Hartmann, S.; Neff, P. Polyconvexity of generalized polynomial-type hyperelastic strain energy functions for near-incompressibility. Int. J. Solids Struct. 2003, 40, 2767–2791. [Google Scholar] [CrossRef]
- Kadapa, C.; Hossain, M. A linearized consistent mixed displacement-pressure formulation for hyperelasticity. Mech. Adv. Mater. Struct. 2020, 1–18. [Google Scholar] [CrossRef]
- Kurth, J.A.; Haupt, P. Continuum Mechanics and Theory of Materials; Advanced Texts in Physics; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Mahnken, R. Thermodynamic consistent modeling of polymer curing coupled to visco–elasticity at large strains. Int. J. Solids Struct. 2013, 50, 2003–2021. [Google Scholar] [CrossRef]
- Coleman, B.D.; Noll, W. The thermodynamics of elastic materials with heat conduction and viscosity. Arch. Ration. Mech. Anal. 1963, 13, 167–178. [Google Scholar] [CrossRef]
- Hackl, K.; Fischer, F.D. On the relation between the principle of maximum dissipation and inelastic evolution given by dissipation potentials. Philos. Trans. R. Soc. A 2008, 464, 117–132. [Google Scholar] [CrossRef]
- Klinge, S.; Hackl, K.; Renner, J. A mechanical model for dissolution–precipitation creep based on the minimum principle of the dissipation potential. Philos. Trans. R. Soc. A 2015, 471, 20140994. [Google Scholar] [CrossRef]
- Reese, S.; Govindjee, S. Theoretical and Numerical Aspects in the Thermo-Viscoelastic Material Behaviour of Rubber-Like Polymers. Mech. Time Depend. Mater. 1997, 1, 357–396. [Google Scholar] [CrossRef]
- Reese, S. A micromechanically motivated material model for the thermo-viscoelastic material behaviour of rubber-like polymers. Int. J. Plast. 2003, 19, 909–940. [Google Scholar] [CrossRef]
- McBride, A.; Javili, A.; Steinmann, P.; Bargmann, S. Geometrically nonlinear continuum thermomechanics with surface energies coupled to diffusion. J. Mech. Phys. Solids 2011, 59, 2116–2133. [Google Scholar] [CrossRef]
- Brady, J.; Dürig, T.; Lee, P.; Li, J.X. Chapter 7 - Polymer Properties and Characterization. In Developing Solid Oral Dosage Forms, 2nd ed.; Qiu, Y., Chen, Y., Zhang, G.G., Yu, L., Mantri, R.V., Eds.; Academic Press: Boston, MA, USA, 2017; pp. 181–223. [Google Scholar]
- Gasser, T.; Holzapfel, G. A rate-independent elastoplastic constitutive model for biological fiber-reinforced composites at finite strains: Continuum basis, algorithmic formulation and finite element implementation. Comput. Mech. 2002, 29, 340–360. [Google Scholar] [CrossRef]
- Elguedj, T.; Hughes, T. Isogeometric analysis of nearly incompressible large strain plasticity. Comput. Methods Appl. Mech. Eng. 2014, 268, 388–416. [Google Scholar] [CrossRef]
- Raniecki, B.; Bruhns, O. Thermodynamic reference model for elastic-plastic solids undergoing phase transformations. Arch. Mech. 1991, 43, 343–376. [Google Scholar]
- Hajidehi, M.R.; Stupkiewicz, S. Gradient-enhanced model and its micromorphic regularization for simulation of Lüders-like bands in shape memory alloys. Int. J. Solids Struct. 2017, 135, 208–218. [Google Scholar] [CrossRef]
- Holzapfel, G.A.; Simo, J.C. Entropy elasticity of isotropic rubber-like solids at finite strains. Comput. Methods Appl. Mech. Eng. 1996, 132, 17–44. [Google Scholar] [CrossRef]
- de Souza Neto, E.; Peric, D.; Owen, D. Computational Methods for Plasticity: Theory and Applications; Wiley: Hoboken, NJ, USA, 2011. [Google Scholar]
- Moler, C.; Van Loan, C. Nineteen Dubious Ways to Compute the Exponential of a Matrix, Twenty-Five Years Later. SIAM Rev. 2003, 45, 3–49. [Google Scholar] [CrossRef]
- Shahzad, M.; Kamran, A.; Siddiqui, M.Z.; Farhan, M. Mechanical Characterization and FE Modelling of a Hyperelastic Material. Mater. Res. 2015, 18, 918–924. [Google Scholar] [CrossRef]
- Maeda, N.; Fujikawa, M.; Makabe, C.; Yamabe, J.; Kodama, Y.; Koishi, M. Performance evaluation of various hyperelastic constitutive models of rubbers. In Constitutive Models for Rubbers IX; CRC Press: Boca Raton, FL, USA, 2015; pp. 271–277. [Google Scholar]
- Toki, S.; Sics, I.; Ran, S.; Liu, L.; Hsiao, B.S. Molecular orientation and structural development in vulcanized polyisoprene rubbers during uniaxial deformation by in situ synchrotron X-ray diffraction. Polymer 2003, 44, 6003–6011. [Google Scholar] [CrossRef]
- Gschwandl, M.; Kerschbaumer, R.C.; Schrittesser, B.; Fuchs, P.F.; Stieger, S.; Meinhart, L. Thermal conductivity measurement of industrial rubber compounds using laser flash analysis: Applicability, comparison and evaluation. AIP Conf. Proc. 2019, 2065, 030041. [Google Scholar]
- Le Gac, P.Y.; Albouy, P.A.; Petermann, D. Strain-induced crystallization in an unfilled polychloroprene rubber: Kinetics and mechanical cycling. Polymer 2018, 142, 209–217. [Google Scholar]
- Huneau, B. Strain-Induced Crystallization of Natural Rubber: A Review of X-ray Diffraction Investigations. Rubber Chem. Technol. 2011, 84, 425–452. [Google Scholar]
- Juhre, D.; Raghunath, R.; Klueppel, M.; Lorenz, H. A microstructure-based model for filled elastomers including time-dependent effects. Const. Models Rubber VIII 2013, 8, 293–298. [Google Scholar]
- Voges, J.; Makvandi, R.; Juhre, D. Numerical investigation of the phase evolution in polymer blends under external mechanical loadings. Tech. Mech. 2017, 37, 37–47. [Google Scholar]





| Elastic Constants | |||
| Bulk modulus | K | Pa | |
| Shear modulus | Pa | ||
| Limiting network stretch | 2 | - | |
| Crystalline Constants | |||
| Coupling constant | - | ||
| Crystallization limit | A | Pa | |
| Hardening constant | Pa | ||
| Softening constant | Pa | ||
| Constant in function f | - | ||
| Constant in function f | - | ||
| Exponent in function f | 2 | - | |
| Thermal Constants | |||
| Reference temperature | 300 | K | |
| Thermal flexibility modulus | Pa/K | ||
| Coupling constant | - | ||
| Thermal flexibility coefficient | Pa | ||
| Exponent | 6 | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aygün, S.; Klinge, S. Thermomechanical Modeling of Microstructure Evolution Caused by Strain-Induced Crystallization. Polymers 2020, 12, 2575. https://doi.org/10.3390/polym12112575
Aygün S, Klinge S. Thermomechanical Modeling of Microstructure Evolution Caused by Strain-Induced Crystallization. Polymers. 2020; 12(11):2575. https://doi.org/10.3390/polym12112575
Chicago/Turabian StyleAygün, Serhat, and Sandra Klinge. 2020. "Thermomechanical Modeling of Microstructure Evolution Caused by Strain-Induced Crystallization" Polymers 12, no. 11: 2575. https://doi.org/10.3390/polym12112575
APA StyleAygün, S., & Klinge, S. (2020). Thermomechanical Modeling of Microstructure Evolution Caused by Strain-Induced Crystallization. Polymers, 12(11), 2575. https://doi.org/10.3390/polym12112575





