Designing Cellular Structures for Additive Manufacturing Using Voronoi–Monte Carlo Approach
Abstract
1. Introduction
2. Mathematical Background
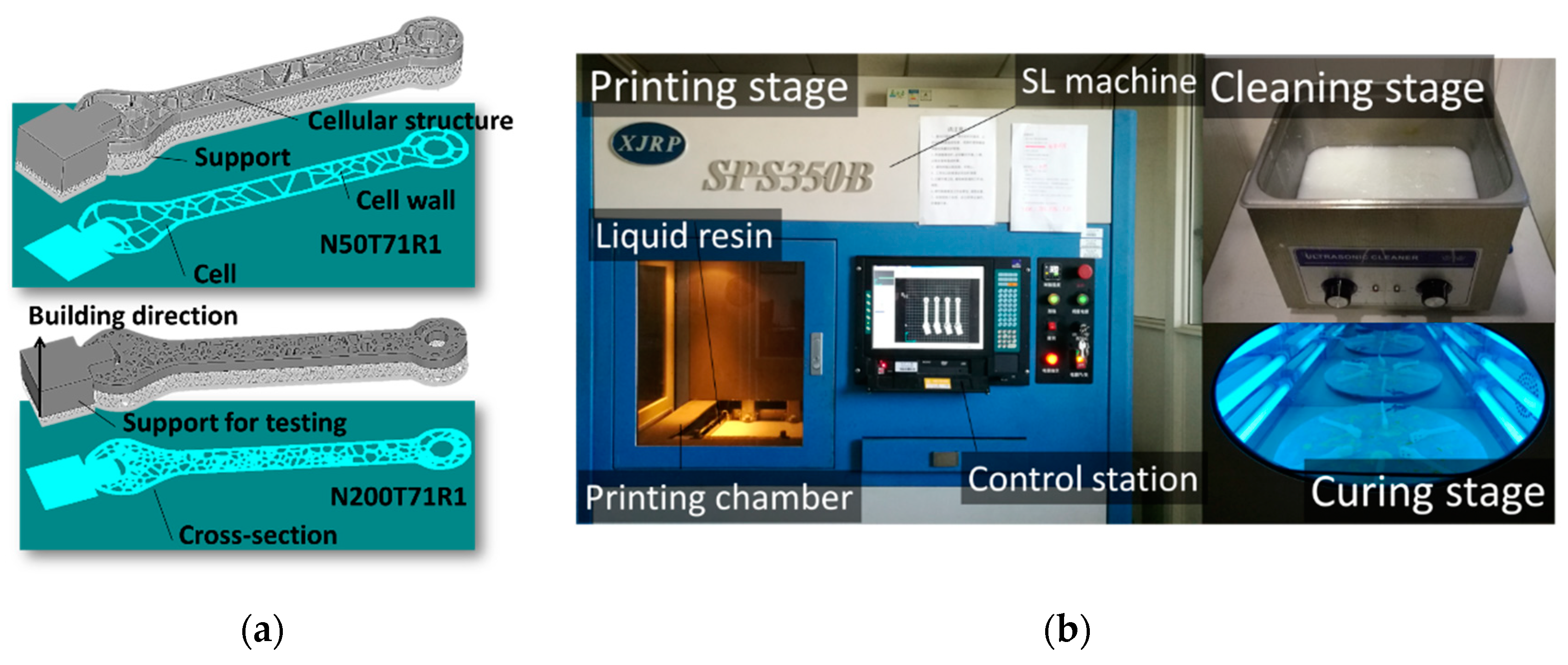
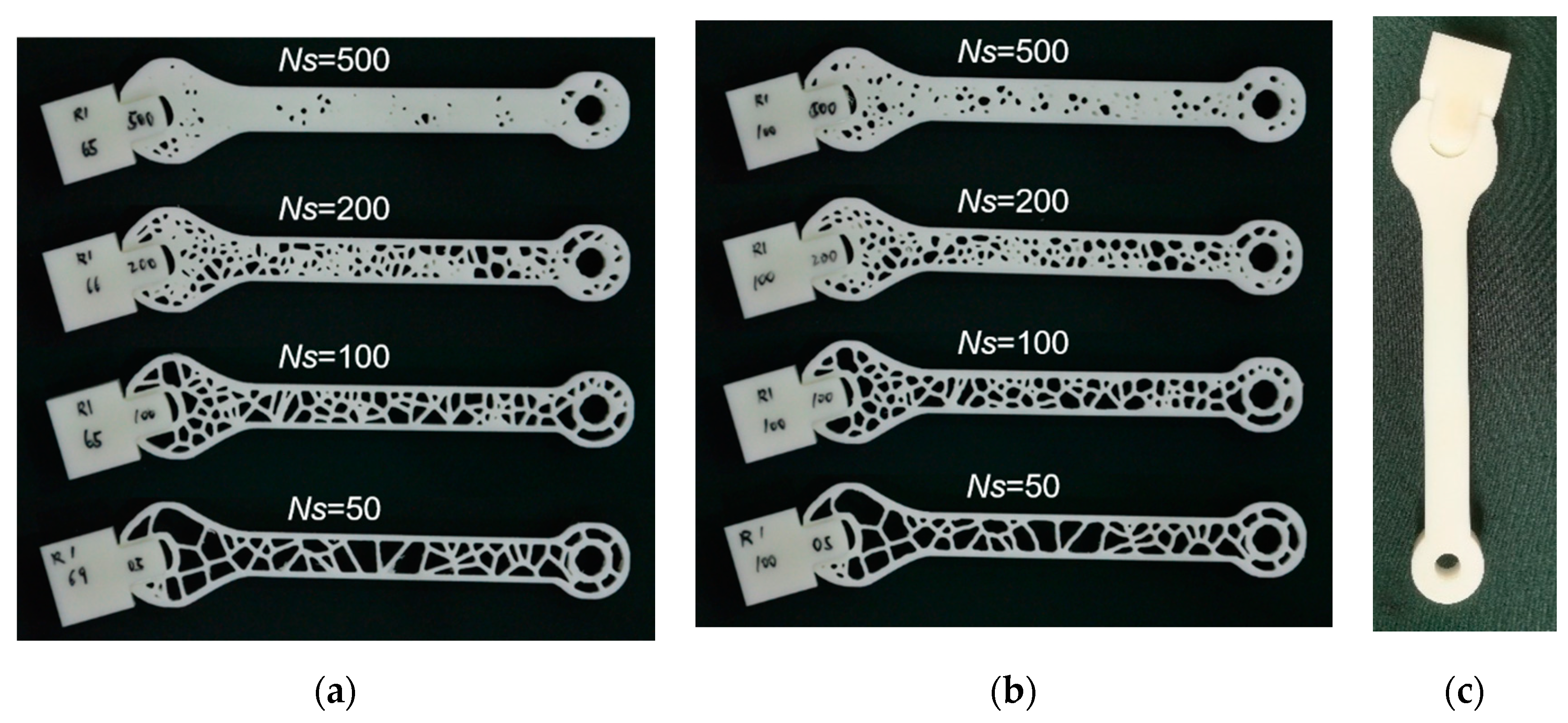
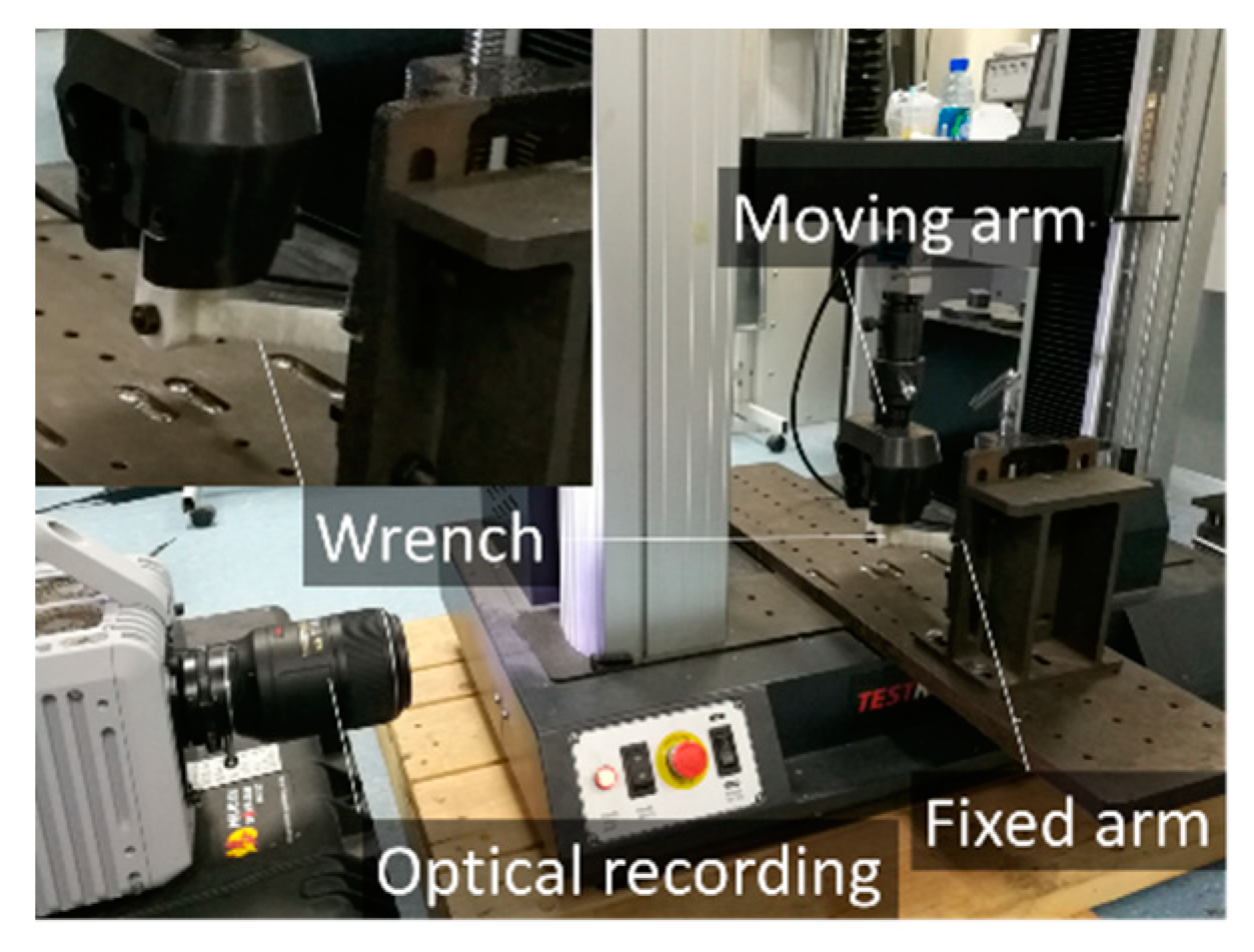
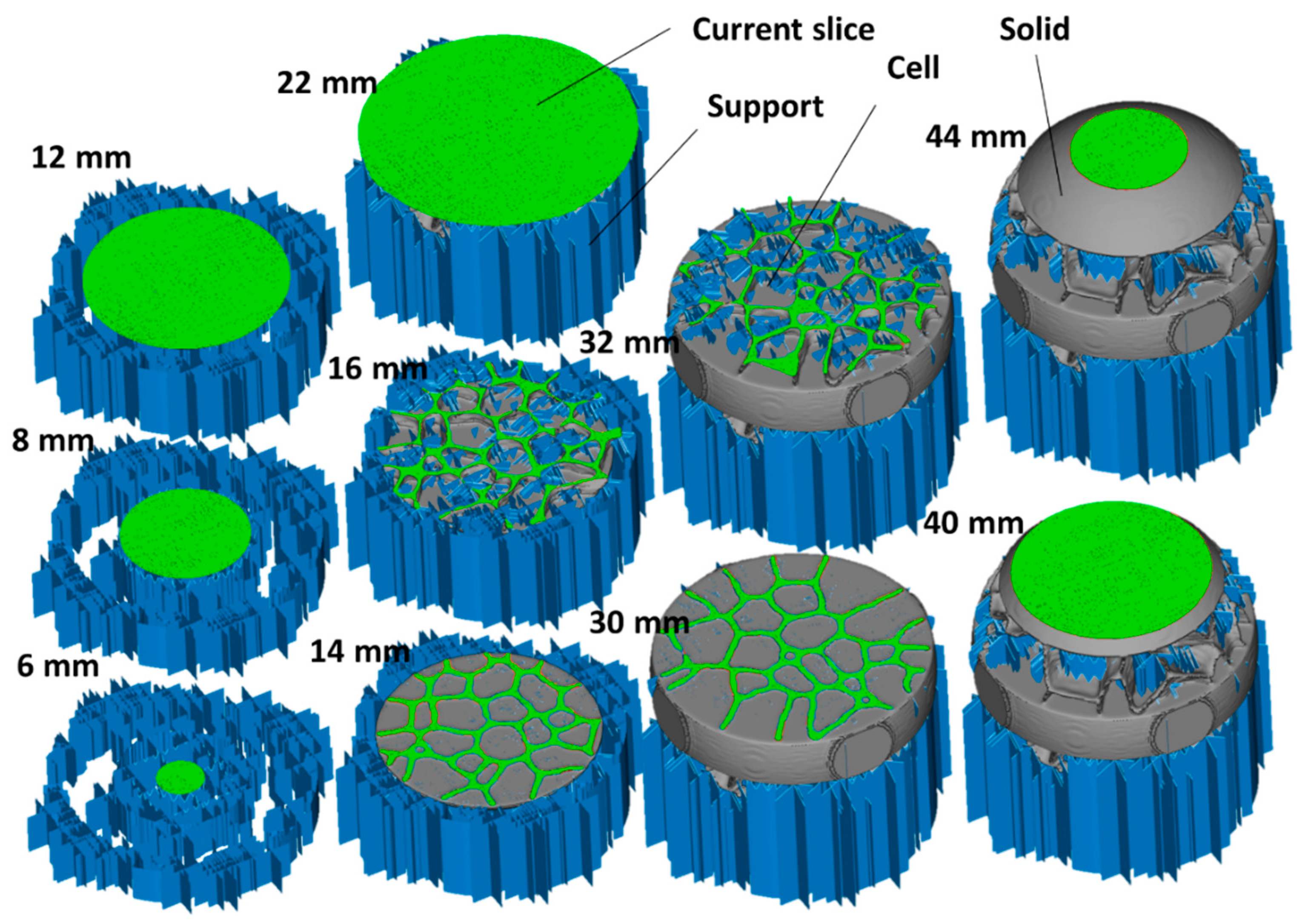
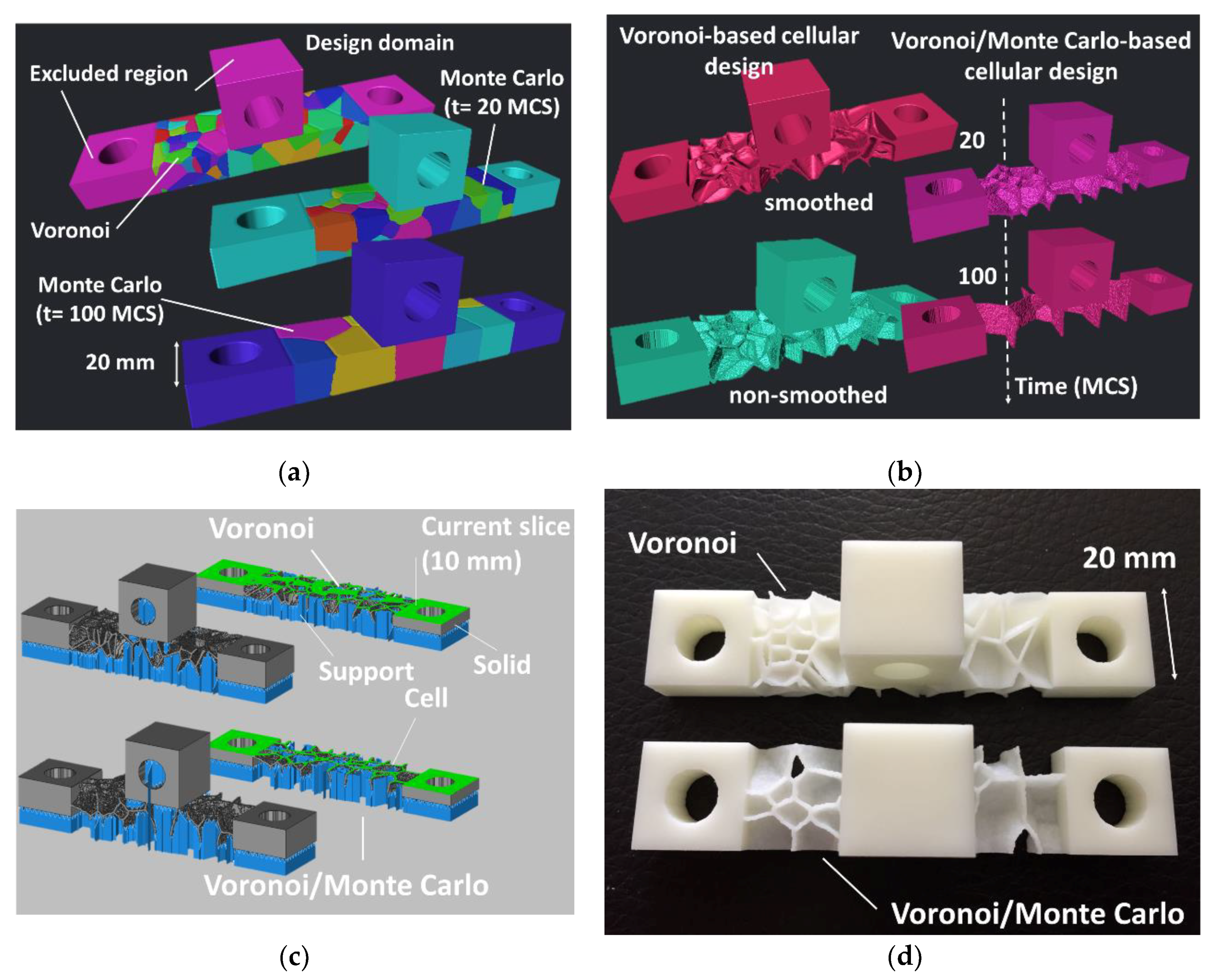
3. Experimental Layout
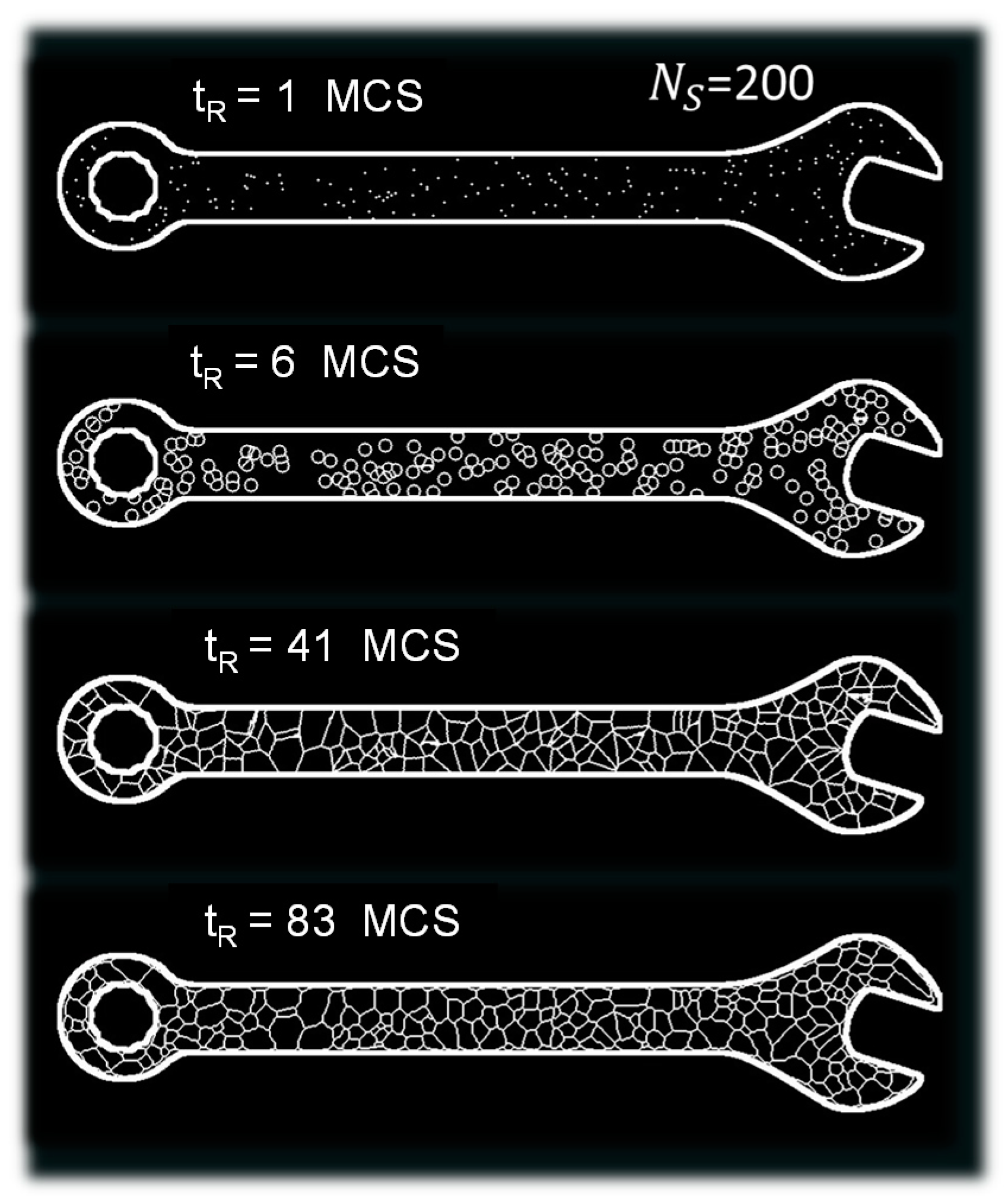
4. Results and Discussion
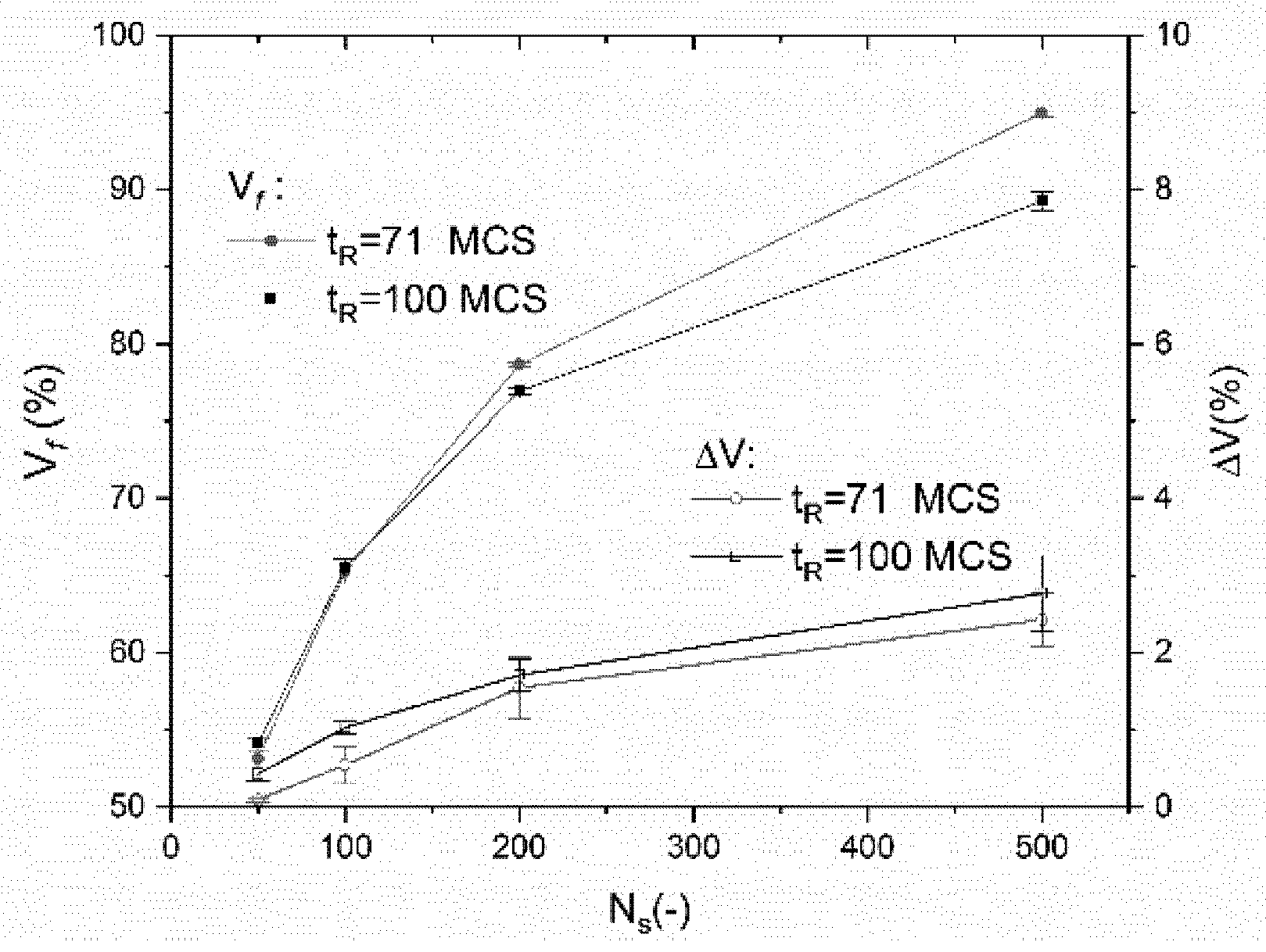
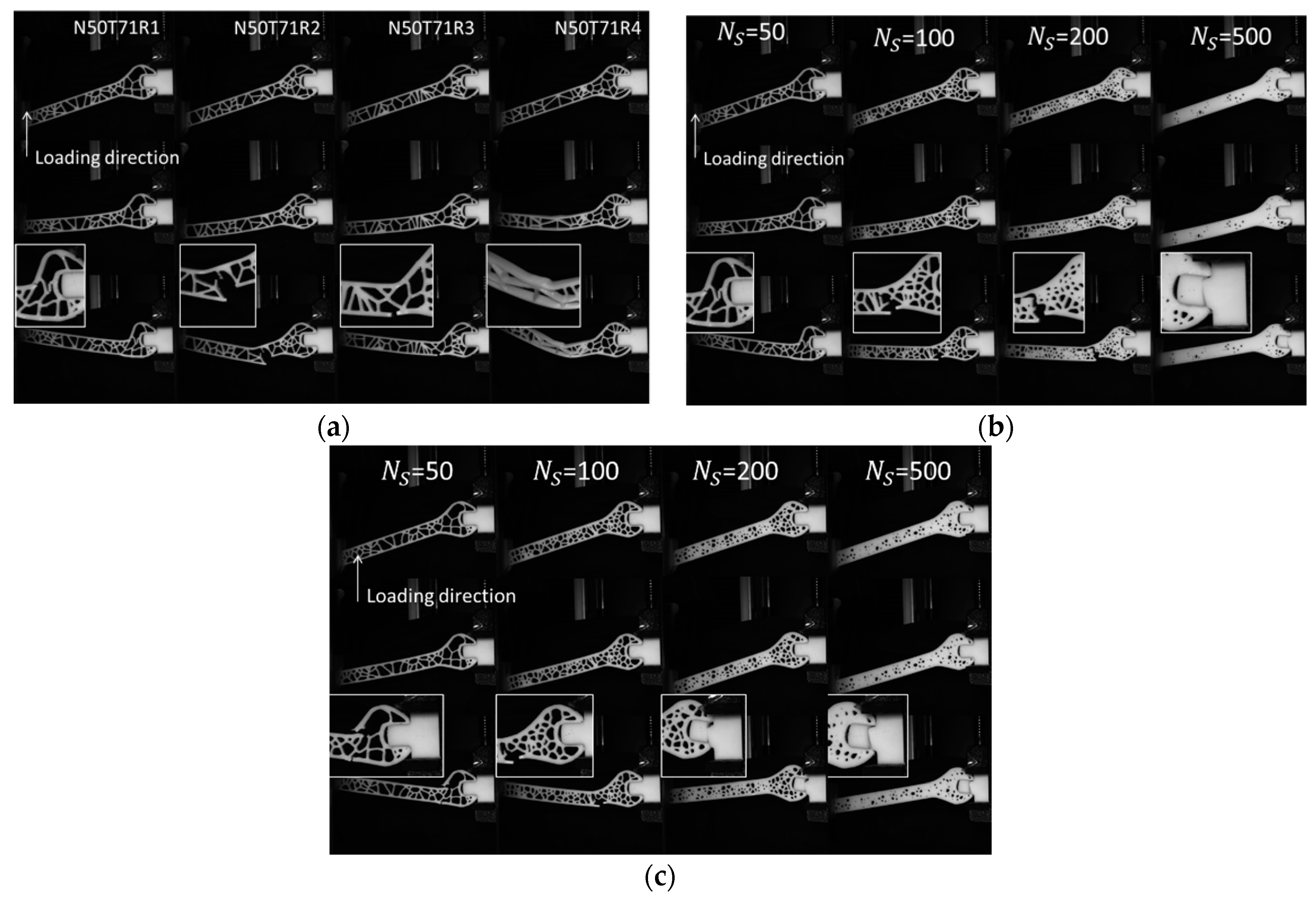
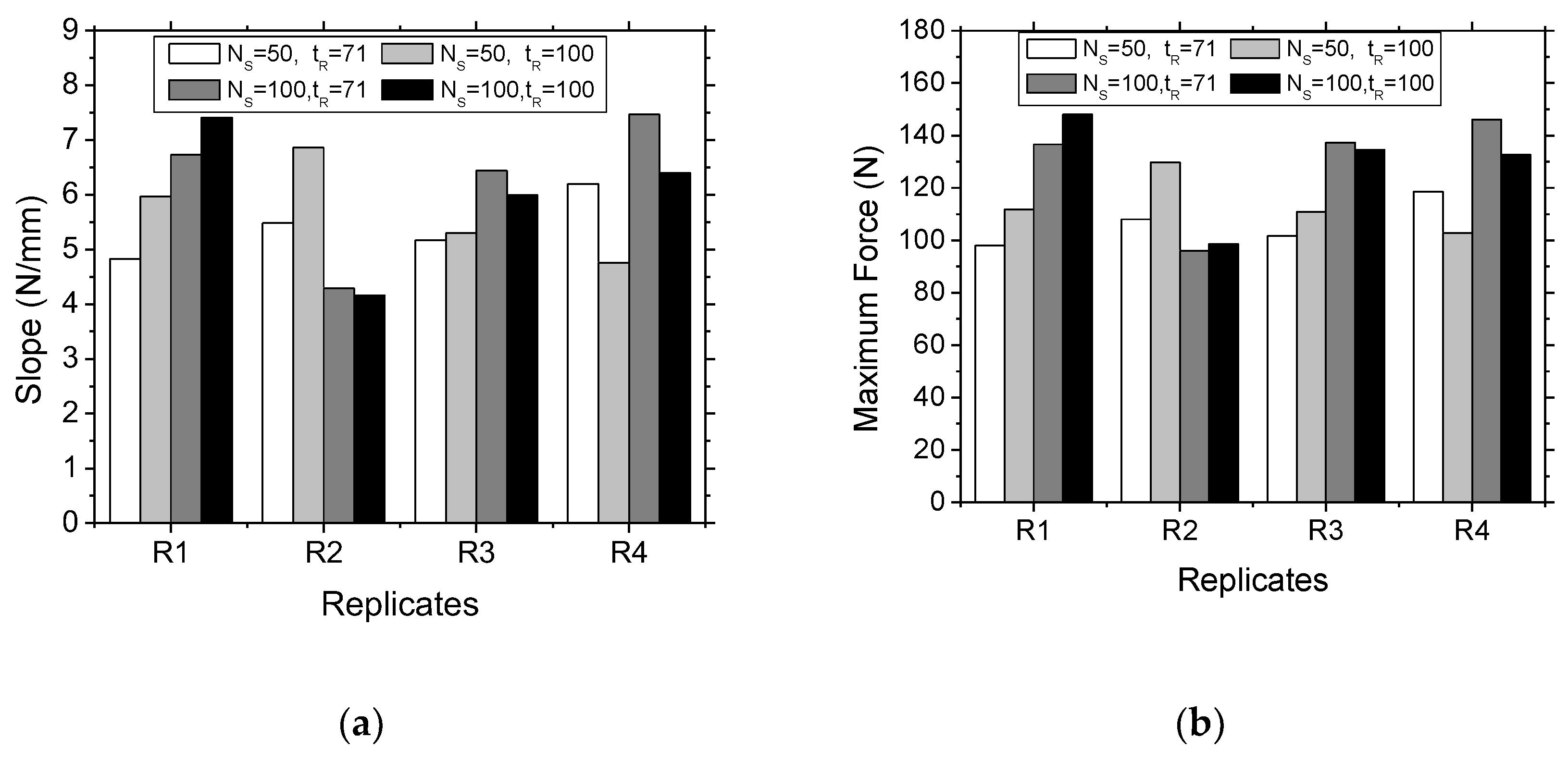
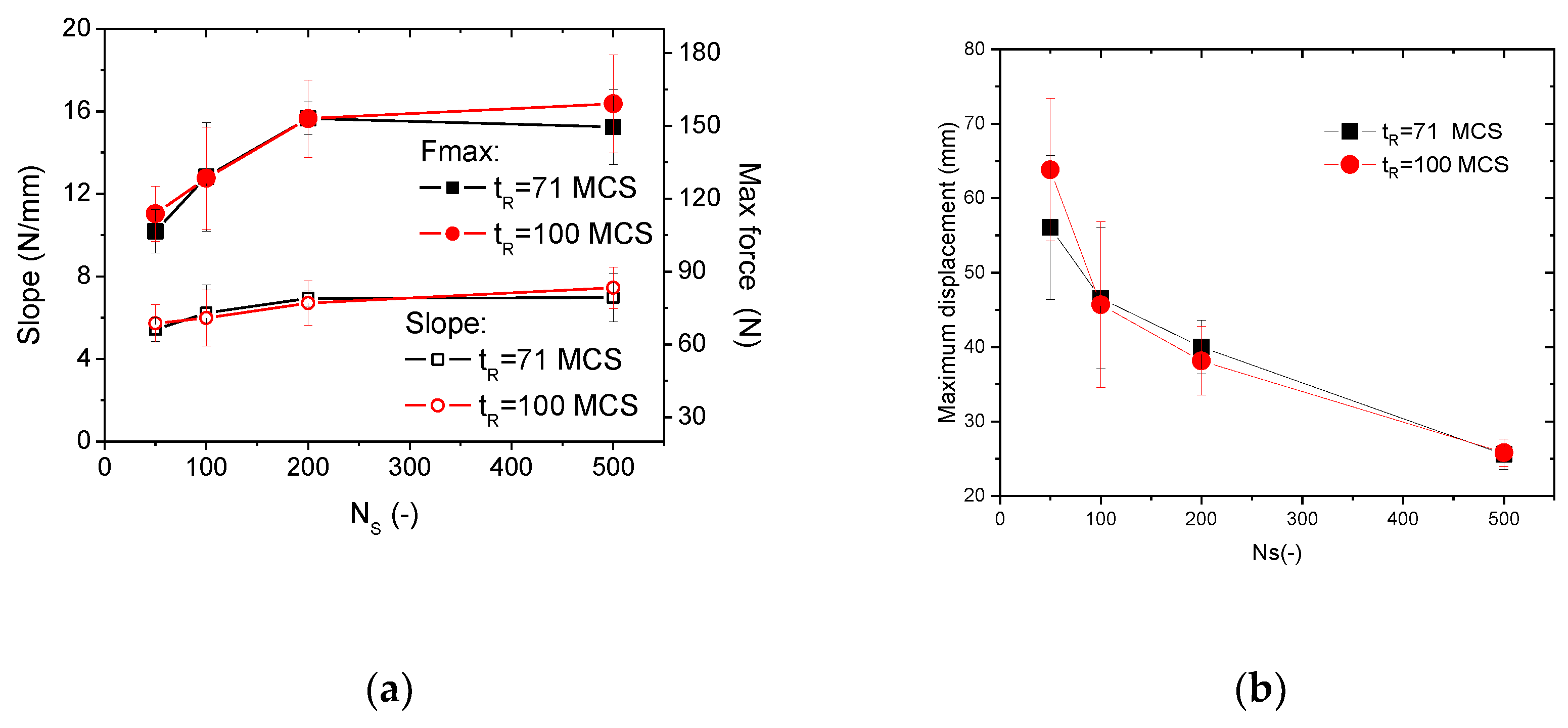
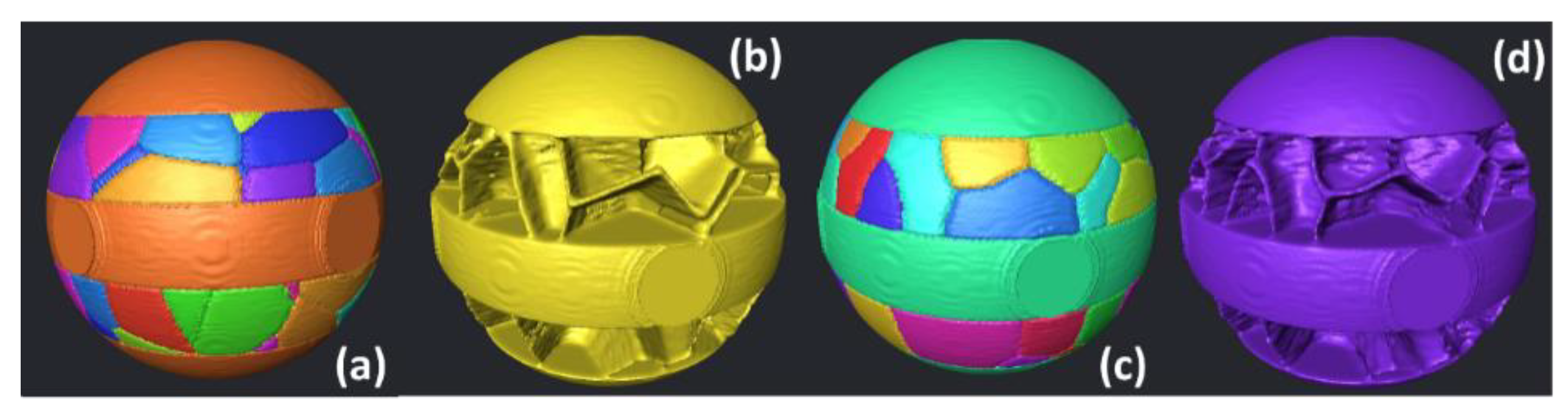
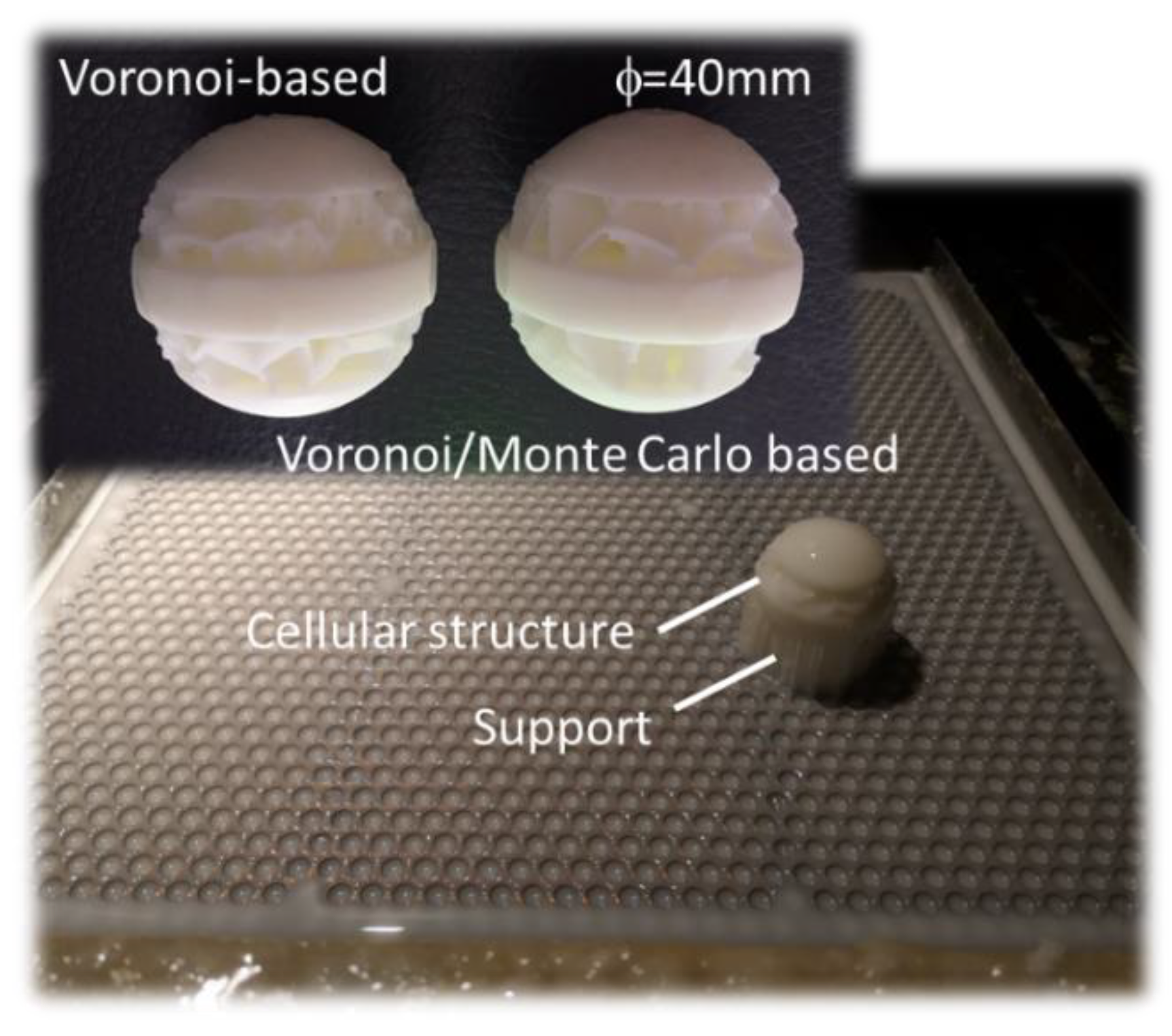
4.1. Voronoi/Monte Carlo Based Cellular Wrench
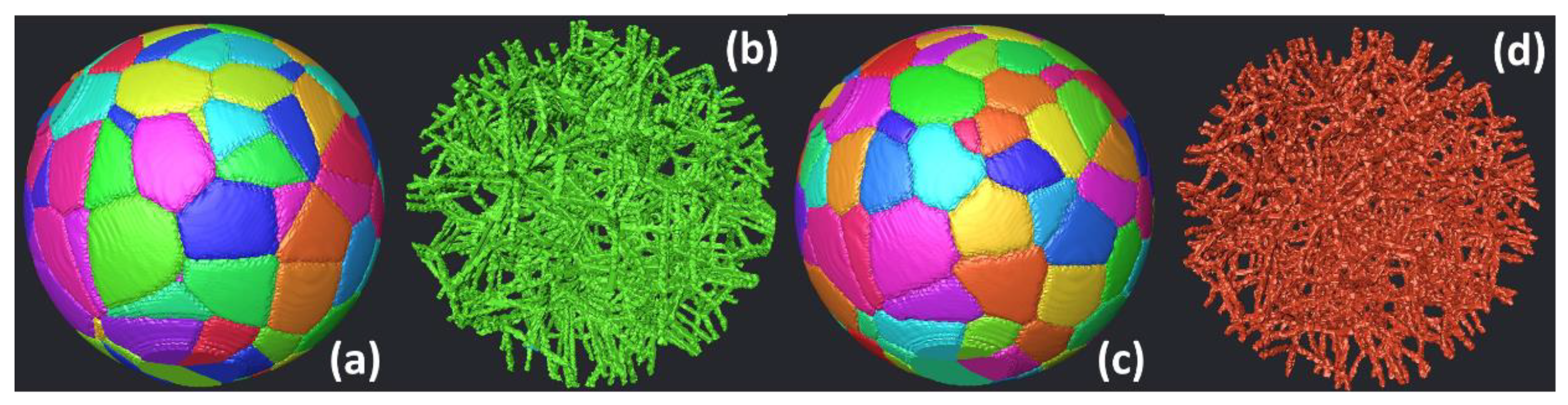
4.2. Extension to 3D Voronoi–Monte Carlo Scheme
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Gibson, L.; Ashby, M. Cellular Solids: Structure and Properties; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Tang, Y.; Zhao, Y.F. A survey of the design methods for additive manufacturing to improve functional performance. Rapid Prototyp. J. 2016, 22, 569–590. [Google Scholar] [CrossRef]
- Altenbach, H. Mechanics of advanced materials for lightweight structures. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2011, 225, 2481–2496. [Google Scholar] [CrossRef]
- Kim, T.; Zhao, C.Y.; Lu, T.J.; Hodson, H.P. Convective heat dissipation with lattice-frame materials. Mech. Mater. 2004, 36, 767–780. [Google Scholar] [CrossRef]
- Xu, F.; Zhang, X.; Zhang, H. A review on functionally graded structures and materials for energy absorption. Eng. Struct. 2018, 171, 309–325. [Google Scholar] [CrossRef]
- Lu, T.J.; Chen, F.; He, D. Sound absorption of cellular metals with semiopen cells. J. Acoust. Soc. Am. 2000, 108, 1697–1709. [Google Scholar] [CrossRef] [PubMed]
- Ozdemir, Z.; Tyas, A.; Goodall, R.; Askes, H. Energy absorption in lattice structures in dynamics: Nonlinear fe simulations. Int. J. Impact Eng. 2017, 102, 1–15. [Google Scholar] [CrossRef]
- Arenas, J.; Crocker, M. Recent trends in porous sound-absorbing materials. Sound Vib. 2010, 44, 12–18. [Google Scholar]
- Lehman, J.; Lakes, R.S. Stiff, strong, zero thermal expansion lattices via material hierarchy. Compos. Struct. 2014, 107, 654–663. [Google Scholar] [CrossRef]
- Wang, Y.H.; Lin, J.P.; He, Y.H.; Zu, C.K.; Chen, G.L. Pore structures and thermal insulating properties of high nb containing tial porous alloys. J. Alloys Compd. 2010, 492, 213–218. [Google Scholar] [CrossRef]
- Brooks, H.; Brigden, K. Design of conformal cooling layers with self-supporting lattices for additively manufactured tooling. Addit. Manuf. 2016, 11, 16–22. [Google Scholar] [CrossRef]
- Hollister, S.J. Porous scaffold design for tissue engineering. Nat. Mater. 2005, 4, 518–524. [Google Scholar] [CrossRef] [PubMed]
- Wadley, H.N.G. Cellular metals manufacturing. Adv. Eng. Mater. 2002, 4, 726–733. [Google Scholar] [CrossRef]
- Helou, M.; Kara, S. Design, analysis and manufacturing of lattice structures: An overview. Int. J. Comput. Integr. Manuf. 2017, 31, 243–261. [Google Scholar] [CrossRef]
- Gibson, I.; Rosen, D.; Stucker, B. Additive Manufacturing Technologies; Springer: Berlin, Germany, 2010. [Google Scholar]
- Melchels, F.P.W.; Feijen, J.; Grijpma, D.W. A review on stereolithography and its applications in biomedical engineering. Biomaterials 2010, 31, 6121–6130. [Google Scholar] [CrossRef] [PubMed]
- Bártolo, P. Stereolithography: Materials, Processes and Applications; Springer Science & Business Media: Berlin, Germany, 2011. [Google Scholar]
- Zhao, Z.; Luc, Z. Adaptive direct slicing of the solid model for rapid prototyping. Int. J. Prod. Res. 2010, 38, 69–83. [Google Scholar] [CrossRef]
- Zhou, J.G.; Herscovici, D.; Chen, C.C. Parametric process optimization to improve the accuracy of rapid prototyped stereolithography parts. Int. J. Mach. Tools Manuf. 2000, 40, 363–379. [Google Scholar] [CrossRef]
- Canellidis, V.; Giannatsis, J.; Dedoussis, V. Genetic-algorithm-based multi-objective optimization of the build orientation in stereolithography. Int. J. Adv. Manuf. Technol. 2009, 45, 714–730. [Google Scholar] [CrossRef]
- Tapia, G.; Elwany, A. A review on process monitoring and control in metal-based additive manufacturing. J. Manuf. Sci. Eng. 2014, 136, 060801. [Google Scholar] [CrossRef]
- Guo, X.; Cheng, G.-D. Recent development in structural design and optimization. Acta Mech. Sin. 2010, 26, 807–823. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, W.; Xia, L. Topology optimization in aircraft and aerospace structures design. Arch. Comput. Methods Eng. 2016, 23, 595–622. [Google Scholar] [CrossRef]
- Sigmund, O. Tailoring materials with prescribed elastic properties. Mech. Mater. 1995, 20, 351–368. [Google Scholar] [CrossRef]
- Neves, M.M.; Rodrigues, H.; Guedes, J.M. Optimal design of periodic linear elastic microstructures. Comput. Struct. 2000, 76, 421–429. [Google Scholar] [CrossRef]
- Li, H.; Luo, Z.; Zhang, N.; Gao, L.; Brown, T. Integrated design of cellular composites using a level-set topology optimization method. Comput. Methods Appl. Mech. Eng. 2016, 309, 453–475. [Google Scholar] [CrossRef]
- Huang, X.; Radman, A.; Xie, Y.M. Topological design of microstructures of cellular materials for maximum bulk or shear modulus. Comp. Mater. Sci. 2011, 50, 1861–1870. [Google Scholar] [CrossRef]
- Zhou, S.; Li, Q. Computational design of multi-phase microstructural materials for extremal conductivity. Comp. Mater. Sci. 2008, 43, 549–564. [Google Scholar] [CrossRef]
- Zhou, S.; Li, W.; Sun, G.; Li, Q. A level-set procedure for the design of electromagnetic metamaterials. Opt. Express 2010, 18, 6693–6702. [Google Scholar] [CrossRef] [PubMed]
- Torquato, S.; Hyun, S.; Donev, A. Optimal design of manufacturable three-dimensional composites with multifunctional characteristics. J. Appl. Phys. 2003, 94, 5748–5755. [Google Scholar] [CrossRef]
- Guest, J.K.; Prévost, J.H. Optimizing multifunctional materials: Design of microstructures for maximized stiffness and fluid permeability. Int. J. Solids Struct. 2006, 43, 7028–7047. [Google Scholar] [CrossRef]
- Giannitelli, S.M.; Accoto, D.; Trombetta, M.; Rainer, A. Current trends in the design of scaffolds for computer-aided tissue engineering. Acta Biomater. 2014, 10, 580–594. [Google Scholar] [CrossRef]
- Bargmann, S.; Klusemann, B.; Markmann, J.; Schnabel, J.E.; Schneider, K.; Soyarslan, C.; Wilmers, J. Generation of 3d representative volume elements for heterogeneous materials: A review. Prog. Mater. Sci. 2018, 96, 322–384. [Google Scholar] [CrossRef]
- Lee, M.; Fang, Q.; Cho, Y.; Ryu, J.; Liu, L.; Kim, D.-S. Support-free hollowing for 3d printing via voronoi diagram of ellipses. Comput. Aided Des. 2018, 101, 23–36. [Google Scholar] [CrossRef]
- Rodgers, T.M.; Madison, J.D.; Tikare, V. Simulation of metal additive manufacturing microstructures using kinetic monte carlo. Comput. Mater. Sci. 2017, 135, 78–89. [Google Scholar] [CrossRef]
- Guessasma, S.; Suzon, E.; Grosdidier, T.; Wagner, F. Static recrystallisation in an ods feal alloy: The effect of particles on texture and anisotropic grain growth developments. Intermetallics 2008, 16, 1013–1025. [Google Scholar] [CrossRef]
- Guessasma, S.; Rouag, N. Grain boundary character and pinning effect during grain growth of two-phase alloys. Solid State Phenom. 2005, 105, 163–168. [Google Scholar] [CrossRef]
- Hague, R.; Mansour, S.; Saleh, N.; Harris, R. Materials analysis of stereolithography resins for use in rapid manufacturing. J. Mater. Sci. 2004, 39, 2457–2464. [Google Scholar] [CrossRef]
- Guessasma, S. Young’s modulus of 2d cellular structures under periodic boundary conditions and subject to structural effects. Comput. Mater. Sci. 2008, 44, 552–565. [Google Scholar] [CrossRef]
- Dulieu-Barton, J.M.; Fulton, M.C. Mechanical properties of a typical stereolithography resin. Strain 2000, 36, 81–87. [Google Scholar] [CrossRef]
- Quintana, R.; Choi, J.-W.; Puebla, K.; Wicker, R. Effects of build orientation on tensile strength for stereolithography-manufactured astm d-638 type i specimens. Int. J. Adv. Manuf. Technol. 2009, 46, 201–215. [Google Scholar] [CrossRef]

| Liquid State | Magnitude | Solid State | Magnitude |
|---|---|---|---|
| Appearance | White | Flexural modulus | 2692–2775 MPa |
| Density | 1.13 g/cm3 | Flexural strength | 69–74 MPa |
| Viscosity | 355 cps | Tensile modulus | 2189–2395 MPa |
| Penetration distance | 0.145 mm | Tensile strength | 27–31 MPa |
| Critical exposure energy | 9.3 mJ/cm2 | Elongation at break | 12–20% |
| Building layer thickness | 0.1 mm | Density | 1.16 g/cm3 |
| - | - | Glass transition | 62 °C |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, T.; Guessasma, S.; Zhu, J.; Zhang, W. Designing Cellular Structures for Additive Manufacturing Using Voronoi–Monte Carlo Approach. Polymers 2019, 11, 1158. https://doi.org/10.3390/polym11071158
Liu T, Guessasma S, Zhu J, Zhang W. Designing Cellular Structures for Additive Manufacturing Using Voronoi–Monte Carlo Approach. Polymers. 2019; 11(7):1158. https://doi.org/10.3390/polym11071158
Chicago/Turabian StyleLiu, Tao, Sofiane Guessasma, Jihong Zhu, and Weihong Zhang. 2019. "Designing Cellular Structures for Additive Manufacturing Using Voronoi–Monte Carlo Approach" Polymers 11, no. 7: 1158. https://doi.org/10.3390/polym11071158
APA StyleLiu, T., Guessasma, S., Zhu, J., & Zhang, W. (2019). Designing Cellular Structures for Additive Manufacturing Using Voronoi–Monte Carlo Approach. Polymers, 11(7), 1158. https://doi.org/10.3390/polym11071158








