A Hyper-Elastic Creep Approach and Characterization Analysis for Rubber Vibration Systems
Abstract
1. Introduction
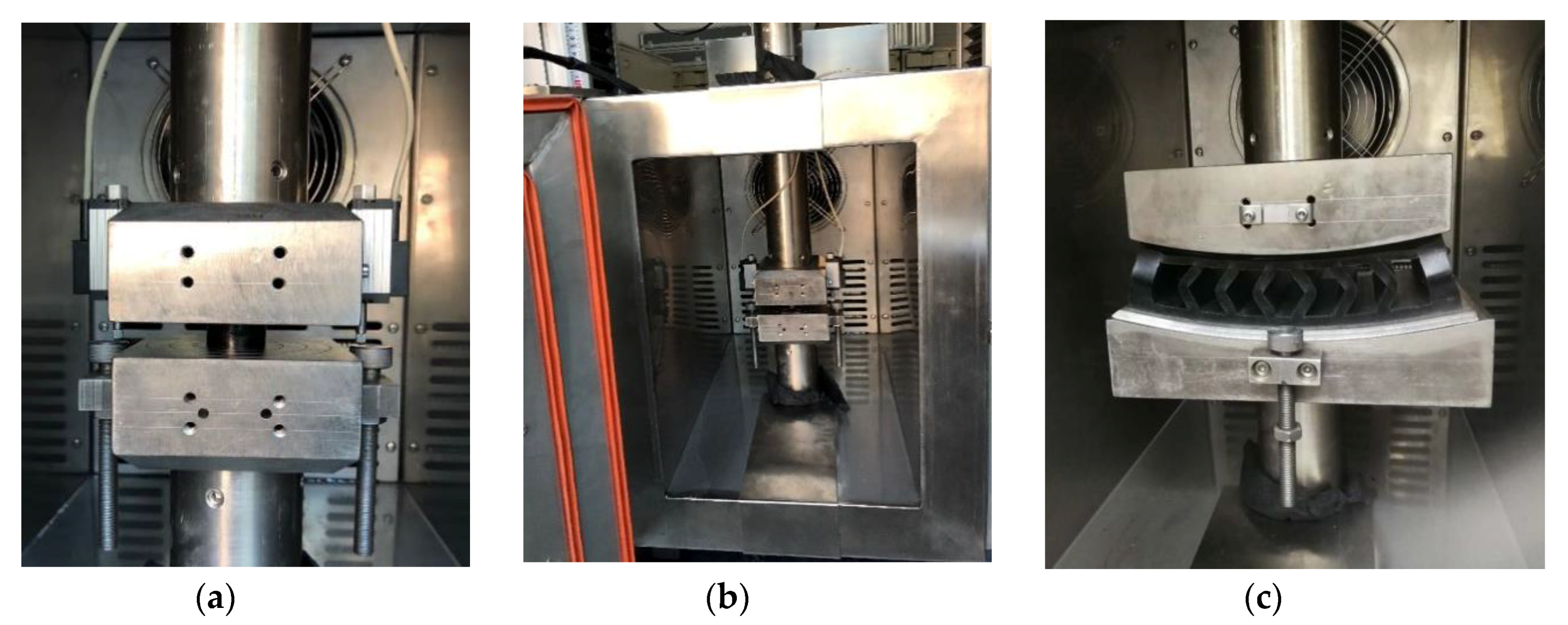
2. Experimental Testing
2.1. Quasi-Static Compression Tests
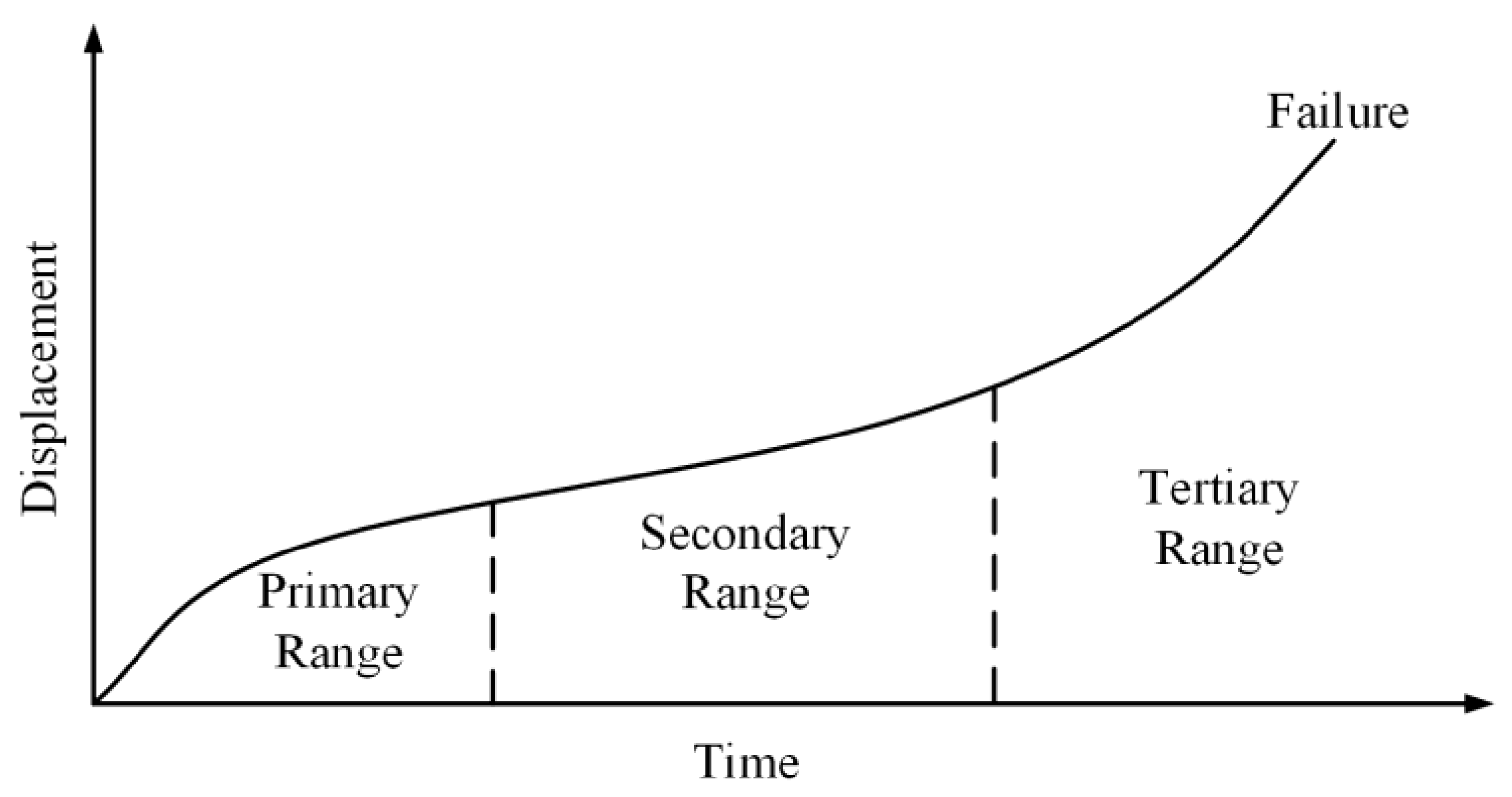
2.2. Creep Compression Tests
3. Numerical Simulation
3.1. Constitutive Model
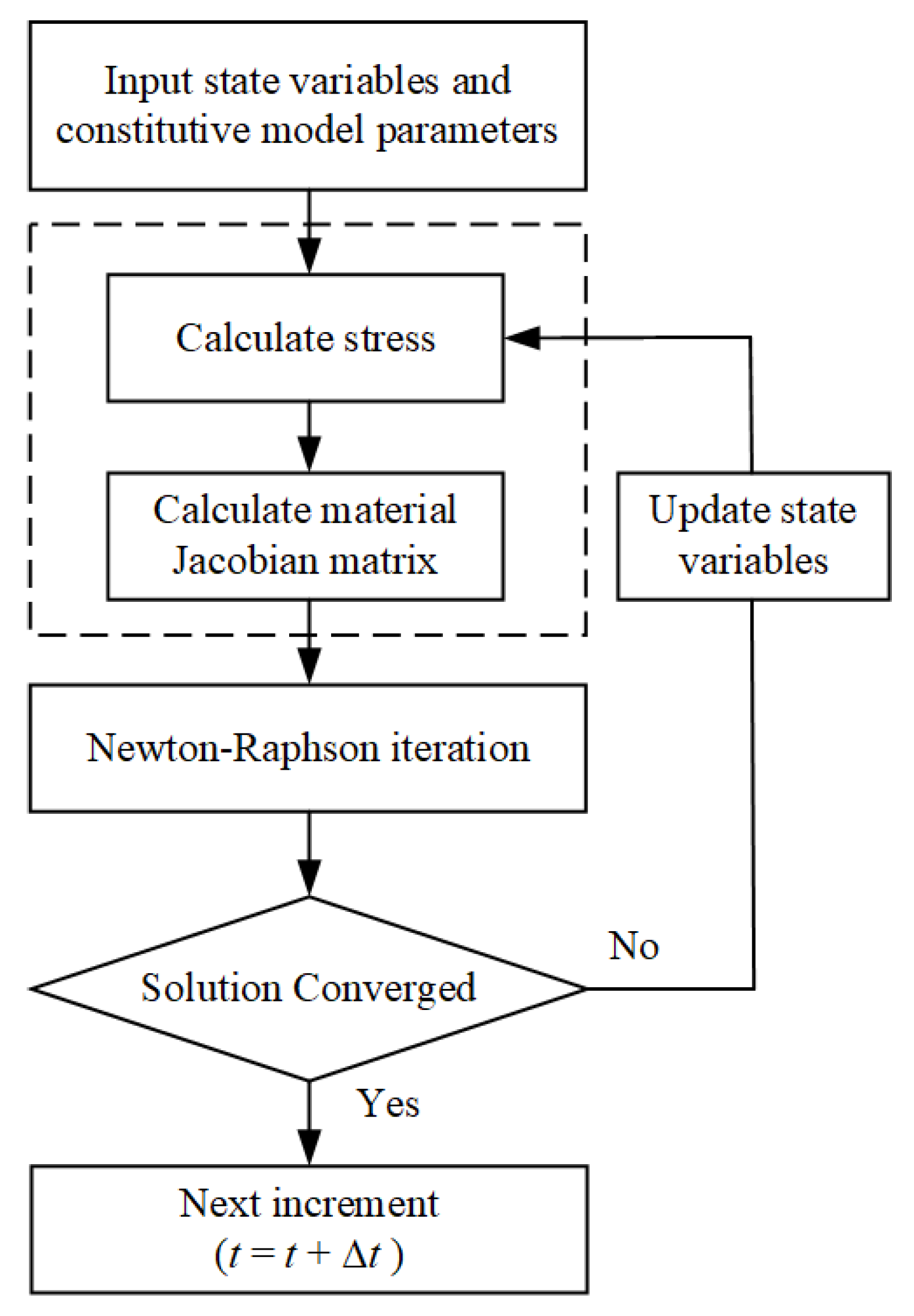
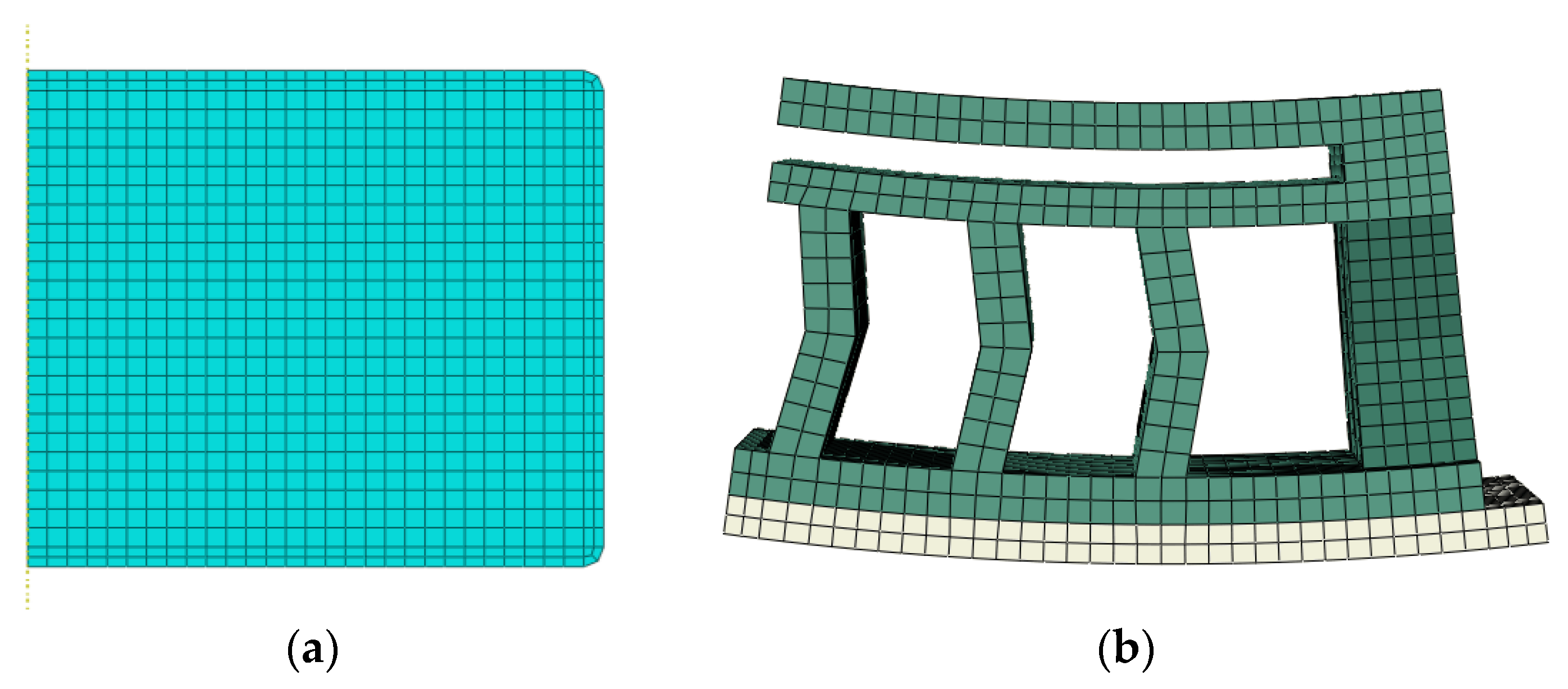
3.2. Implementation of Finite Element Method
4. Results and Discussions
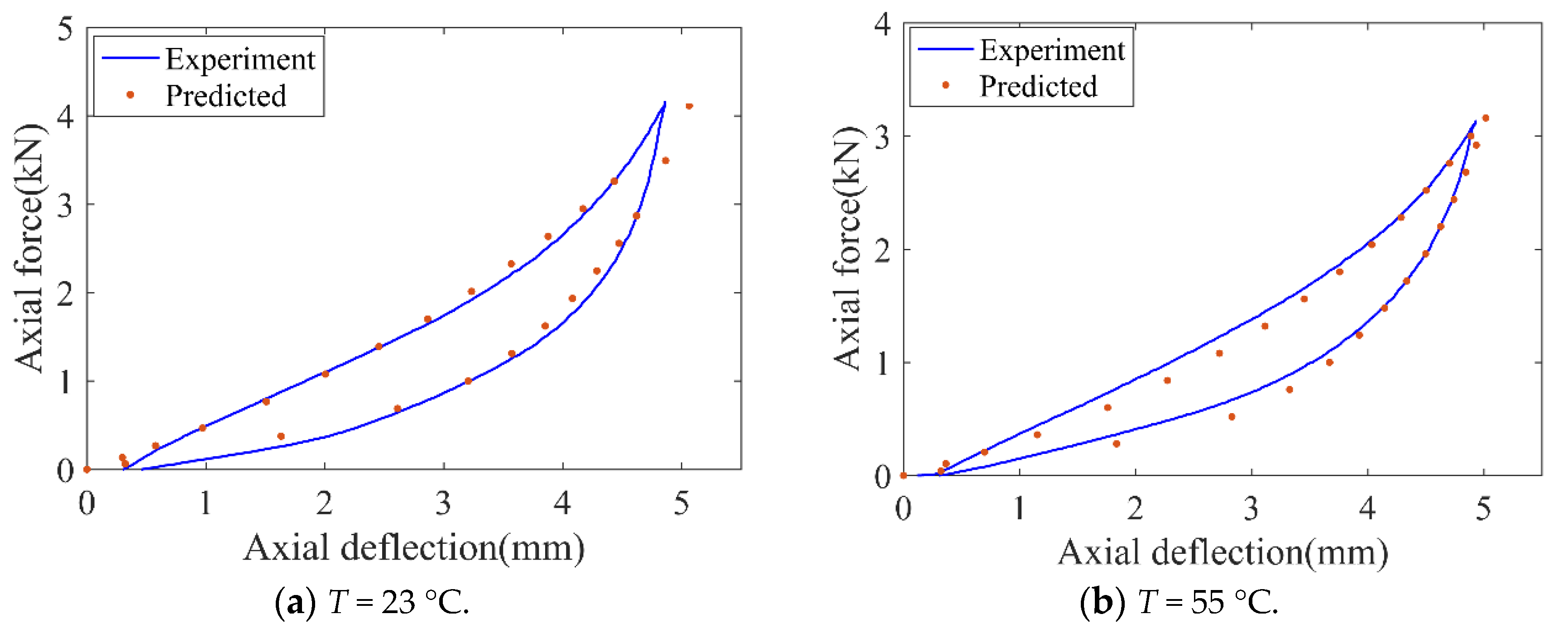
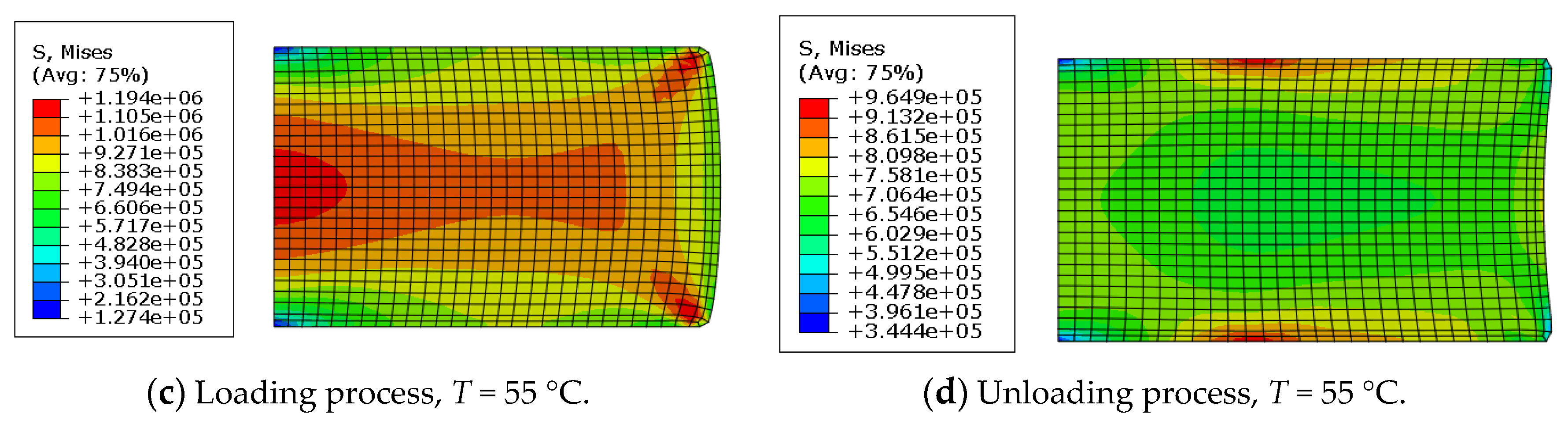
4.1. Quasi-Static Analysis
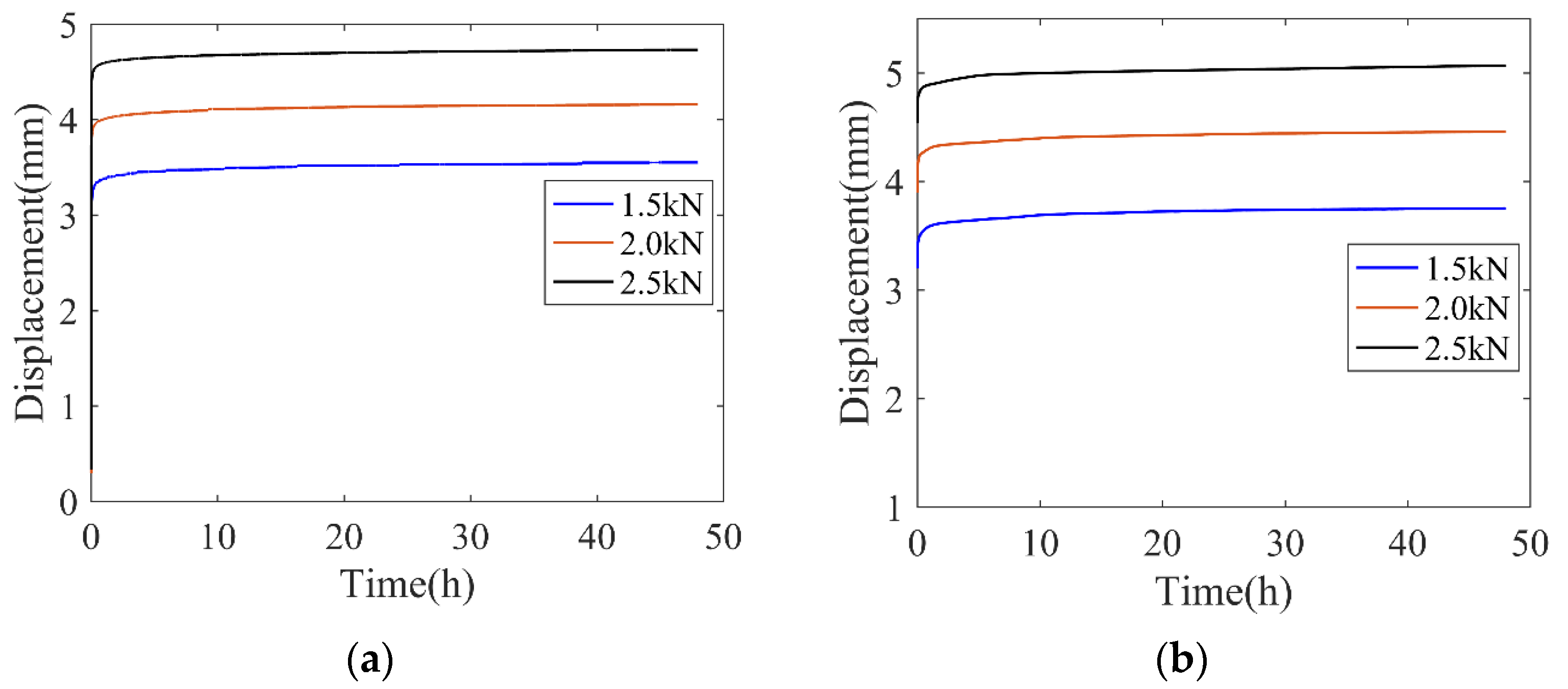
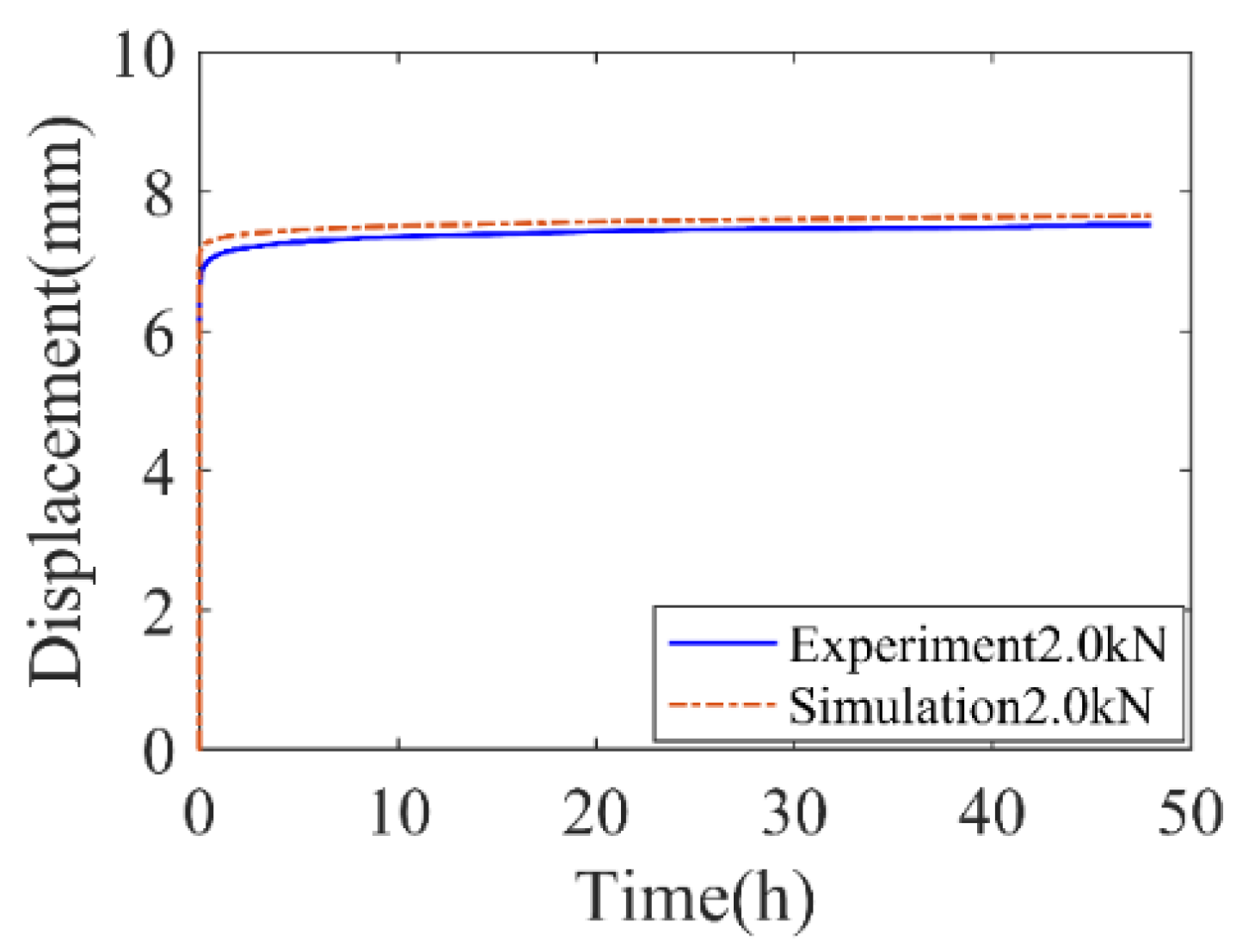
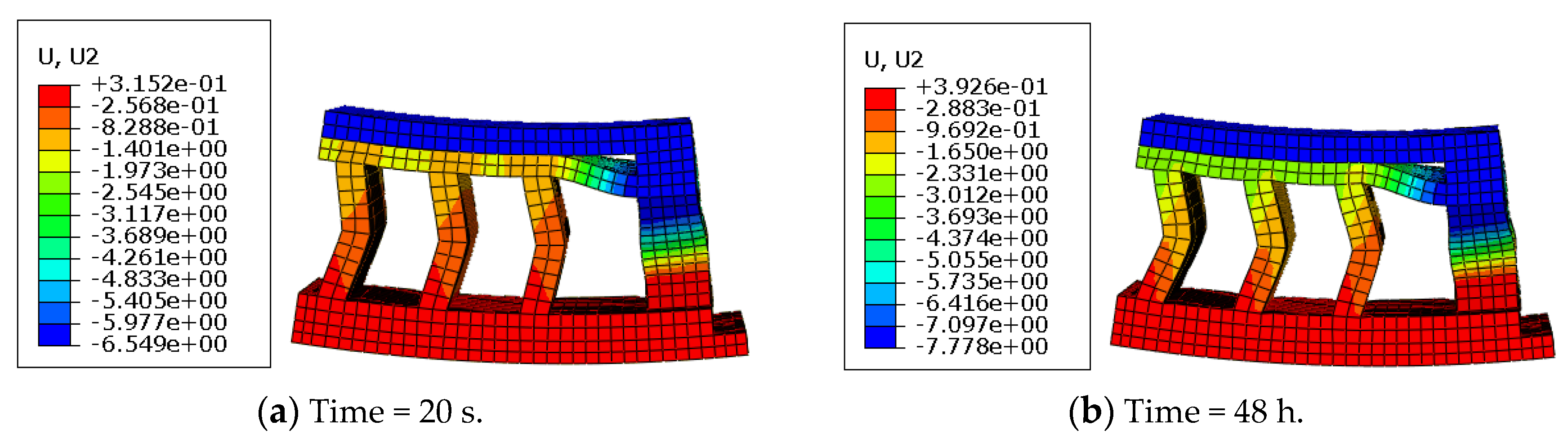
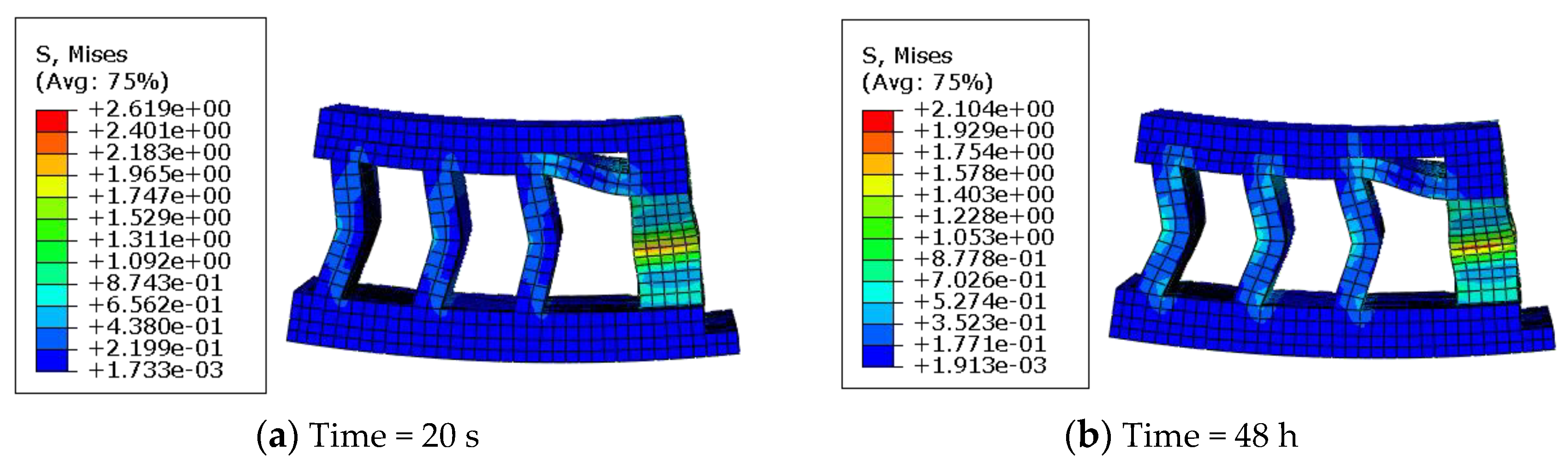
4.2. Creep Analysis
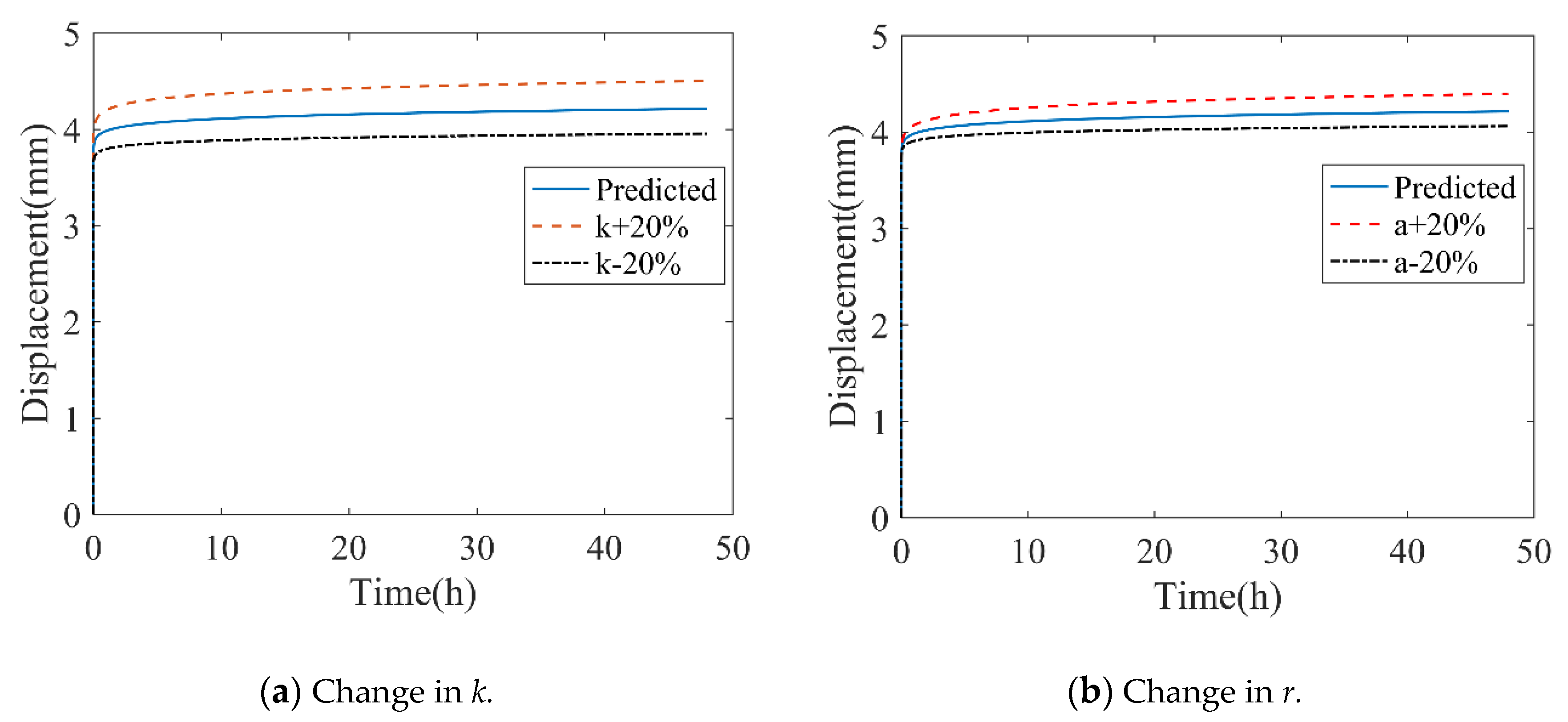
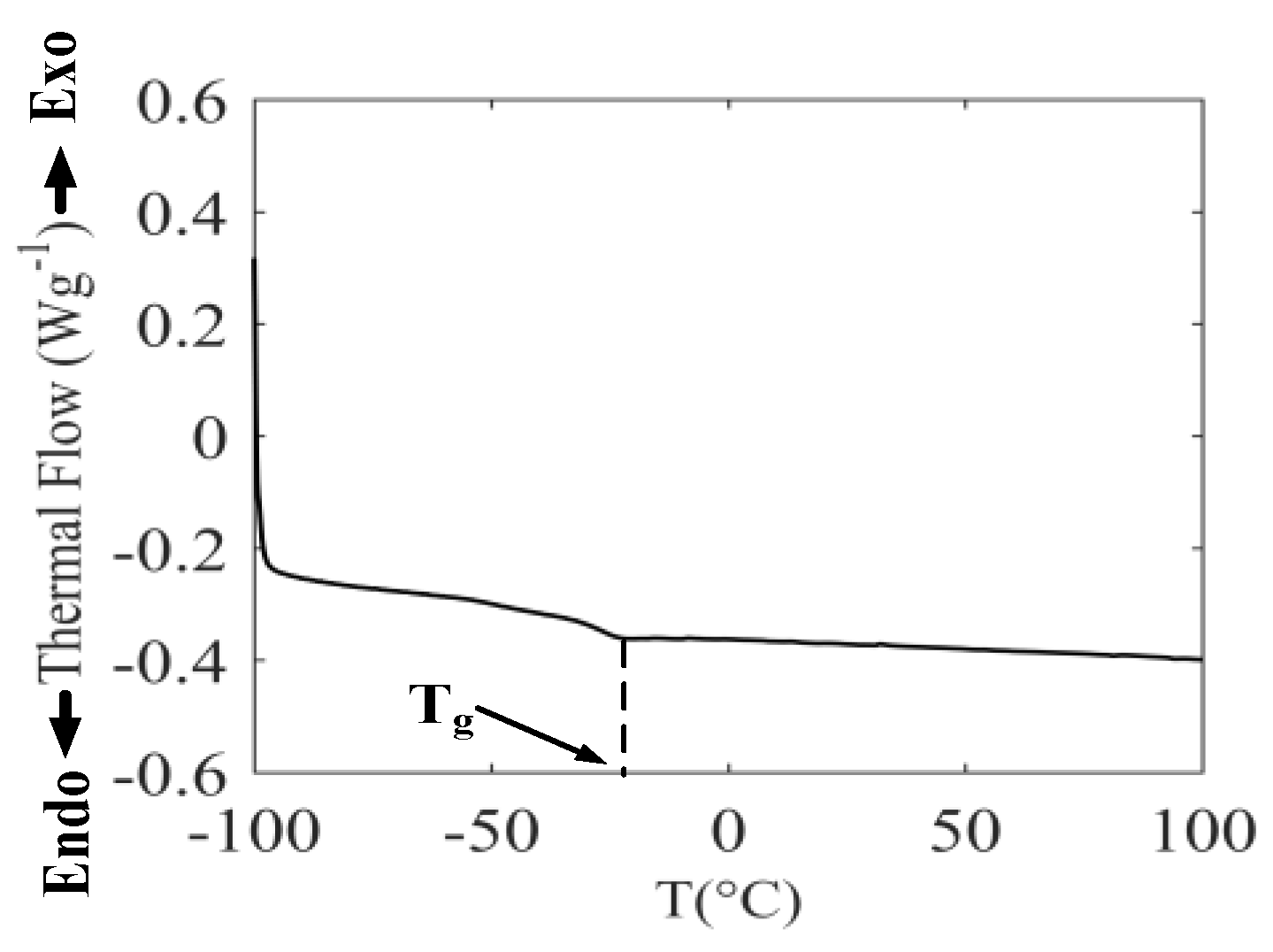
5. Time–Temperature Equivalent Analysis
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Daver, F.; Kajtaz, M.; Brandt, M.; Shanks, R. Creep and recovery behavior of polyolefin-rubber nanocomposites developed for additive manufacturing. Polymers 2016, 8, 437. [Google Scholar] [CrossRef] [PubMed]
- Francesca, C.; Ettore, B.; Roly, W. Limitations of Viscoelastic Constitutive Models for Carbon-Black Reinforced Rubber in Medium Dynamic Strains and Medium Strain Rates. Polymers 2018, 10, 988. [Google Scholar]
- Pawel, M.; Skrodzewicz, J. A modified creep model of epoxy adhesive at ambient temperature. Int. J. Adhes. Adhes. 2009, 29, 396–404. [Google Scholar]
- Wang, Y.; Liu, Y.; Zhang, Z.; Wang, C.; Shi, S.; Chen, X. Mechanical properties of cerium oxide-modified vulcanised natural rubber at elevated temperature. Plast. Rubber Compos. 2017, 46, 306–313. [Google Scholar] [CrossRef]
- Boyce, M.C.; Arruda, E.M. Constitutive Models of Rubber Elasticity: A Review. Rubber Chem. Technol. 2000, 73, 504–523. [Google Scholar] [CrossRef]
- Rivlin, R.S. Large Elastic Deformations of Isotropic Materials; Springer: New York, NY, USA, 1997. [Google Scholar]
- Mooney, M.A. Theory of Large Elastic Deformation. J. Appl. Phys. 1940, 11, 582. [Google Scholar] [CrossRef]
- Mansouri, M.R.; Darijani, H. Constitutive modeling of isotropic hyperelastic materials in an exponential framework using a self-contained approach. Int. J. Solids Struct. 2014, 51, 4316–4326. [Google Scholar] [CrossRef]
- Ogden, R.W. Large Deformation Isotropic Elasticity—On the Correlation of Theory and Experiment for Incompressible Rubberlike Solids. Proc. R. Soc. Lond. 1972, 326, 565–584. [Google Scholar] [CrossRef]
- Yeoh, O.H. Some Forms of the Strain Energy Function for Rubber. Rubber Chem. Technol. 1993, 66, 754–771. [Google Scholar] [CrossRef]
- Li, X.; Wei, Y. Classic strain energy functions and constitutive tests of rubber-like materials. Rubber Chem. Technol. 2015, 88, 604–627. [Google Scholar] [CrossRef]
- Arruda, E.M.; Boyce, M.C. A three-dimensional constitutive model for the large stretch behavior of rubber elastic materials. J. Mech. Phys. Solids 1993, 41, 389–412. [Google Scholar] [CrossRef]
- Erman, B.; Flory, P.J. Relationships between stress, strain, and molecular constitution of polymer networks. Comparison of theory with experiments. Macromolecules 1982, 15, 806–811. [Google Scholar] [CrossRef]
- Luo, R.K.; Zhou, X.L.; Tang, J.F. Numerical prediction and experiment on rubber creep and stress relaxation using time-dependent hyper elastic approach. Polym. Test. 2016, 52, 246–253. [Google Scholar] [CrossRef]
- Skrzypek, J.J.; Hetnarski, R.B.; Mróz, Z. Plasticity and creep. Encycl. Tribol. 1994, 61, 749–750. [Google Scholar] [CrossRef]
- Lee, B.S.; Rivin, E.I. Finite element analysis of load-deflection and creep characteristics of compressed rubber components for vibration control devices. J. Mech. Des. 1996, 118, 328–336. [Google Scholar] [CrossRef]
- Luo, R.K.; Wu, X.; Peng, L. Creep loading response and complete loading–unloading investigation of industrial anti-vibration systems. Polym. Test. 2015, 46, 134–143. [Google Scholar] [CrossRef]
- Rivin, E.I.; Lee, B.S. Experimental study of load-deflection and creep characteristics of compressed rubber components for vibration control devices. J. Mech. Des. 1994, 116, 539–549. [Google Scholar] [CrossRef]
- Oman, S.; Nagode, M. Observation of the relation between uniaxial creep and stress relaxation of filled rubber. Mater. Des. 2014, 60, 451–457. [Google Scholar] [CrossRef]
- Wang, H.; You, Z.; Mills-Beale, J.; Hao, P. Laboratory evaluation on high temperature viscosity and low temperature stiffness of asphalt binder with high percent scrap tire rubber. Constr. Build. Mater. 2012, 26, 583–590. [Google Scholar] [CrossRef]
- ASTM D575-91(2018) Standard Test Method for Rubber Properties in Compression, American Society for Testing Materials Standard; ASTM International: West Conshohocken, PA, USA, 2018.
- Mullins, L.; Harwood, J.A.C.; Payne, A.R. Stress Softening in Natural Rubber Vulcanizates. J. Appl. Polym. Sci. 1965, 9, 3011–3021. [Google Scholar]
- ABAQUS User’s Manual; Version 5.6; Hibbitt, Karlsson & Sorensen, Inc.: Pawtucket, RI, USA, 1996.
- Luo, R.K. Creep modelling and unloading evaluation of the rubber suspensions of rail vehicles. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2015. [Google Scholar] [CrossRef]
- Suchocki, C. Finite element implementation of slightly compressible and incompressible first invariant-based hyperelasticity: Theory, coding, exemplary problems. J. Theor. Appl. Mech. 2017, 55, 787–800. [Google Scholar] [CrossRef]
- Derham, C.J. Creep and stress relaxation of rubbers—The effects of stress history and temperature changes. J. Mater. Sci. 1973, 8, 1023–1029. [Google Scholar] [CrossRef]
- Luo, W.; Wang, C.; Hu, X.; Yang, T. Long-term creep assessment of viscoelastic polymer by time-temperature-stress superposition. Acta Mech. Solida Sin. 2012, 25, 571–578. [Google Scholar] [CrossRef]
- Jazouli, S.; Luo, W.; Bremand, F.; Vu-Khanh, T. Application of time–stress equivalence to nonlinear creep of polycarbonate. Polym. Test. 2005, 24, 463–467. [Google Scholar] [CrossRef]
- Williams, M.L.; Landel, R.F.; Ferry, J.D. The Temperature Dependence of Relaxation Mechanisms in Amorphous Polymers and Other Glass-forming Liquids. J. Am. Chem. Soc. 1955, 77, 3701–3707. [Google Scholar] [CrossRef]
- Shivakumar, E.; Das, C.K.; Segal, E.; Narkis, M. Viscoelastic properties of ternary in situ elastomer composites based on fluorocarbon, acrylic elastomers and thermotropic liquid crystalline polymer blends. Polymer 2005, 46, 3363–3371. [Google Scholar] [CrossRef]
- Wei, K.; Wang, F.; Wang, P.; Liu, Z.X.; Zhang, P. Effect of temperature- and frequency-dependent dynamic properties of rail pads on high-speed vehicle–track coupled vibrations. Veh. Syst. Dyn. 2017, 55, 351–370. [Google Scholar] [CrossRef]






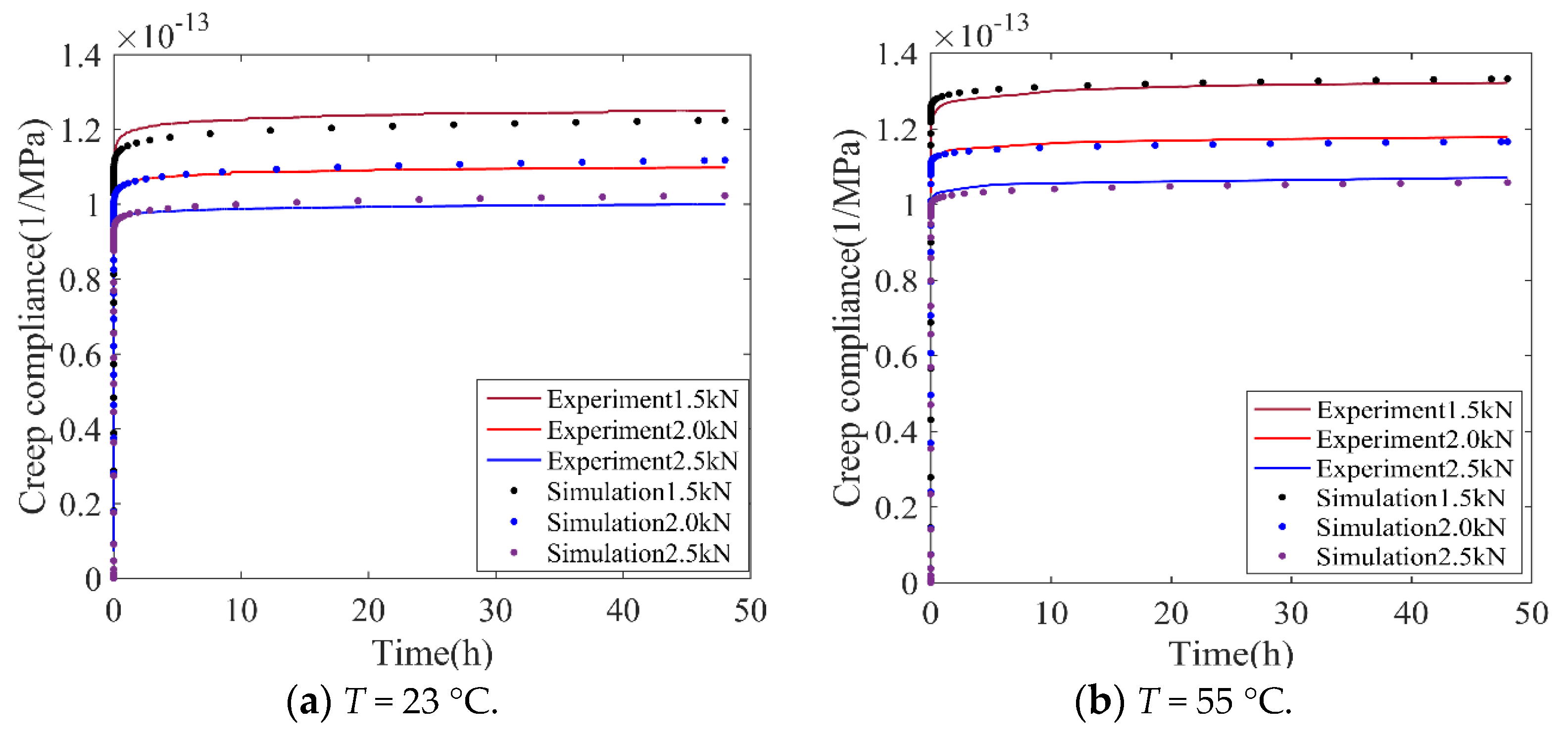
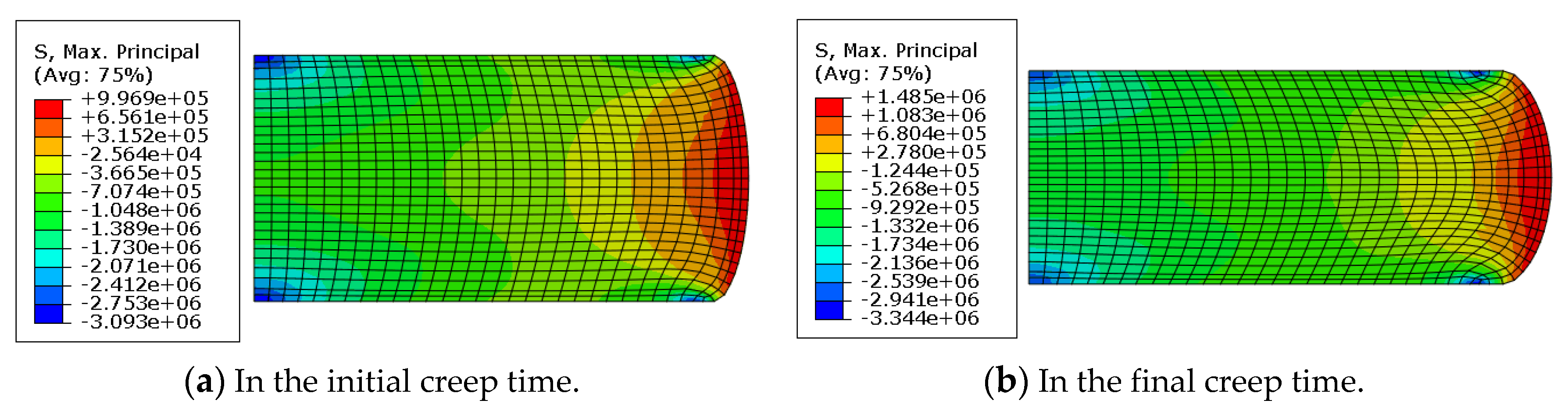
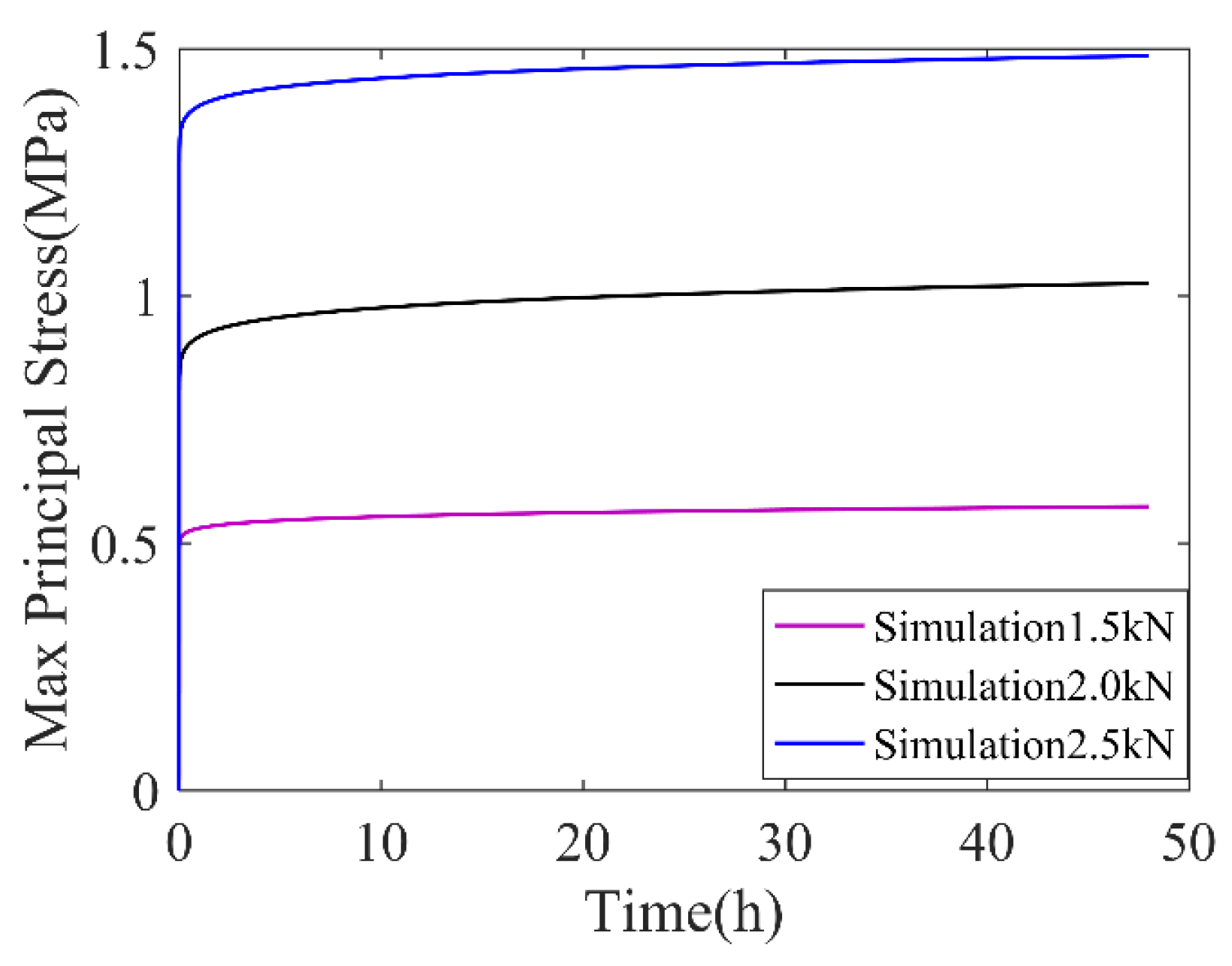

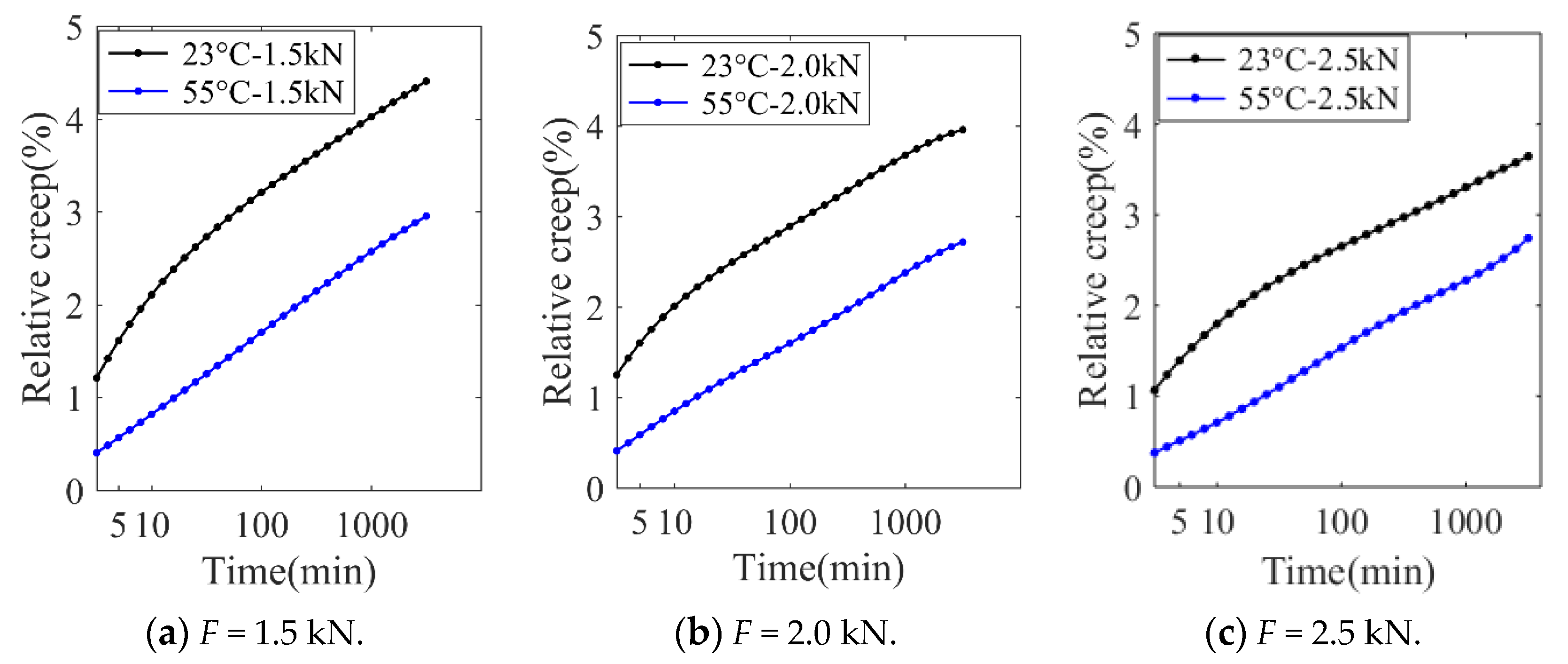

| Creep Models | Loading | Error Indexes | ||
|---|---|---|---|---|
| SCC | MAPE | MSE | ||
| Power-low function | 1.5 kN | 0.9991 | 2.9821 | 0.0113 |
| 2.0 kN | 0.9996 | 1.7582 | 0.0093 | |
| 2.5 kN | 0.9998 | 1.6917 | 0.0087 | |
| Logarithmic function | 1.5 kN | 0.9995 | 4.9595 | 0.0303 |
| 2.0 kN | 0.9990 | 3.4315 | 0.0224 | |
| 2.5 kN | 0.9988 | 3.7001 | 0.0337 | |
| Exponential function | 1.5 kN | 0.9959 | 5.3389 | 0.0497 |
| 2.0 kN | 0.9976 | 4.7459 | 0.0661 | |
| 2.5 kN | 0.9986 | 4.3447 | 0.0633 | |
| Error Index | Formula | Analysis |
|---|---|---|
| SCC | Larger is better | |
| MAPE | Smaller is better | |
| MSE | Smaller is better |
| Temperature | Loading | λ (%) | ||
|---|---|---|---|---|
| 23 °C | 1.5 kN | 0.0413 | 0.0598 | 44.95% |
| 2.0 kN | 0.0669 | 1.0025 | 53.21% | |
| 2.5 kN | 0.0997 | 1.0485 | 48.95% | |
| 55 °C | 1.5 kN | 0.0403 | 0.0439 | 8.93% |
| 2.0 kN | 0.0645 | 0.0691 | 7.13% | |
| 2.5 kN | 0.0913 | 0.0923 | 1.10% |
| Loading | 1.5 kN | 2.0 kN | 2.5 kN | ||||
|---|---|---|---|---|---|---|---|
| Temperature | 23 °C | 55 °C | 23 °C | 55 °C | 23 °C | 55 °C | |
| Time | 1 min | 27.17% | 26.78% | 31.90% | 29.50% | 30.71% | 25.81% |
| 30 min | 72.50% | 58.88% | 74.31% | 60.50% | 74.28% | 57.00% | |
| 1 h | 77.50% | 66.70% | 78.62% | 68.14% | 78.50% | 61.55% | |
| 6 h | 89.33% | 83.27% | 88.97% | 80.65% | 88.48% | 81.86% | |
| 12 h | 92.67% | 89.07% | 93.97% | 89.23% | 92.32% | 85.94% | |
| 24 h | 96.00% | 95.42% | 97.24% | 94.69% | 96.16% | 91.37% | |
| 48 h | 100.00% | 100.00% | 100.00% | 100.00% | 100.00% | 100.00% | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leng, D.; Xu, K.; Qin, L.; Ma, Y.; Liu, G. A Hyper-Elastic Creep Approach and Characterization Analysis for Rubber Vibration Systems. Polymers 2019, 11, 988. https://doi.org/10.3390/polym11060988
Leng D, Xu K, Qin L, Ma Y, Liu G. A Hyper-Elastic Creep Approach and Characterization Analysis for Rubber Vibration Systems. Polymers. 2019; 11(6):988. https://doi.org/10.3390/polym11060988
Chicago/Turabian StyleLeng, Dingxin, Kai Xu, Liping Qin, Yong Ma, and Guijie Liu. 2019. "A Hyper-Elastic Creep Approach and Characterization Analysis for Rubber Vibration Systems" Polymers 11, no. 6: 988. https://doi.org/10.3390/polym11060988
APA StyleLeng, D., Xu, K., Qin, L., Ma, Y., & Liu, G. (2019). A Hyper-Elastic Creep Approach and Characterization Analysis for Rubber Vibration Systems. Polymers, 11(6), 988. https://doi.org/10.3390/polym11060988





