3D Printing with the Commercial UV-Curable Standard Blend Resin: Optimized Process Parameters towards the Fabrication of Tiny Functional Parts
Abstract
:1. Introduction
2. Materials and Methods
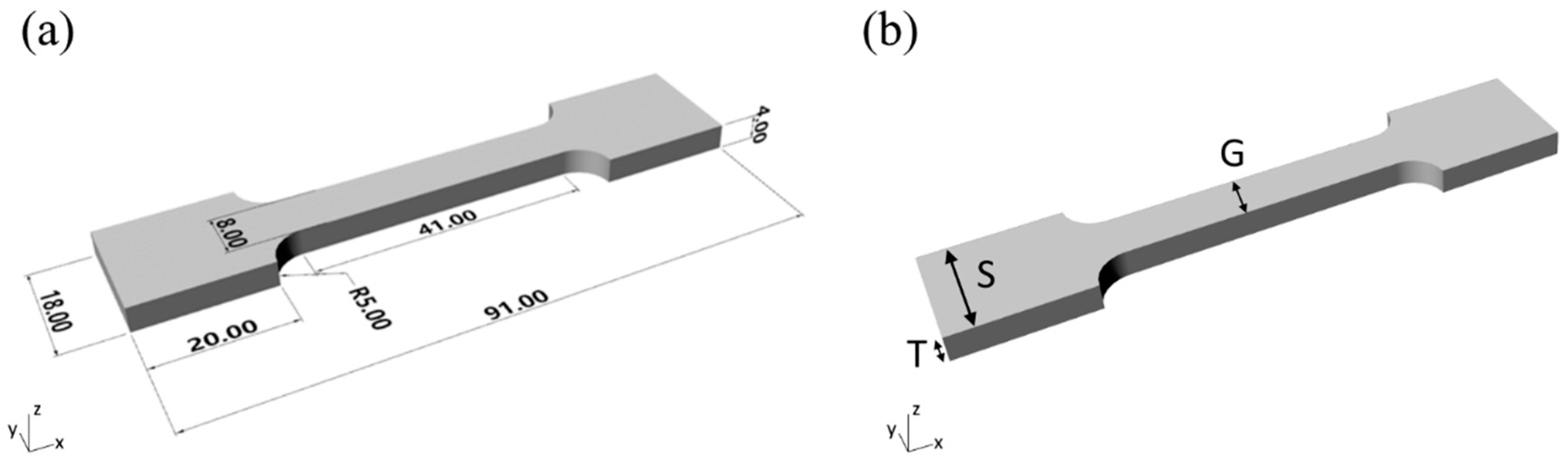
2.1. CAD Modeling
2.2. Printing Equipment and Material
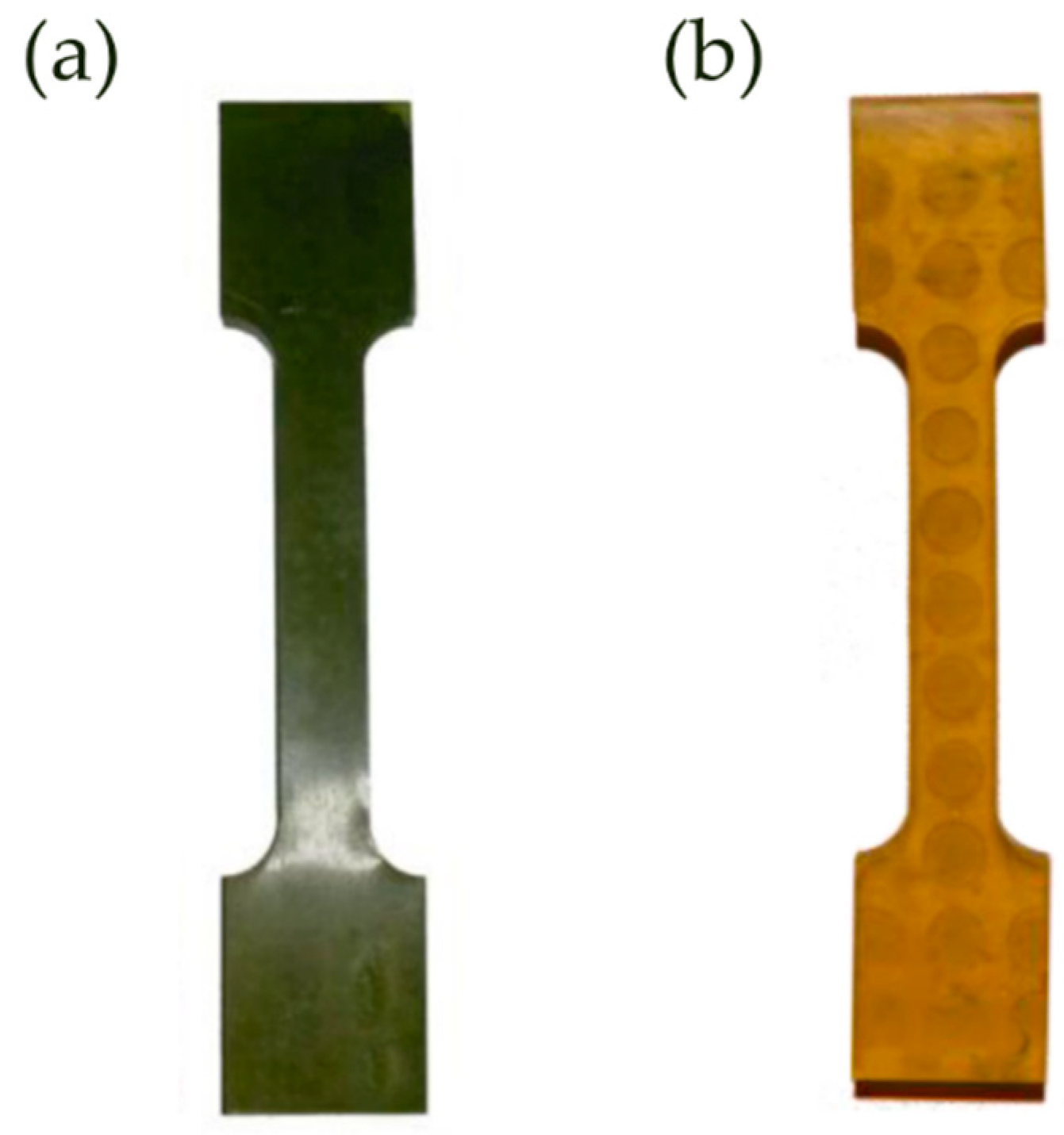
2.3. Printing of the Samples
2.4. Post-Curing Treatment
2.5. Dimensional Characterization
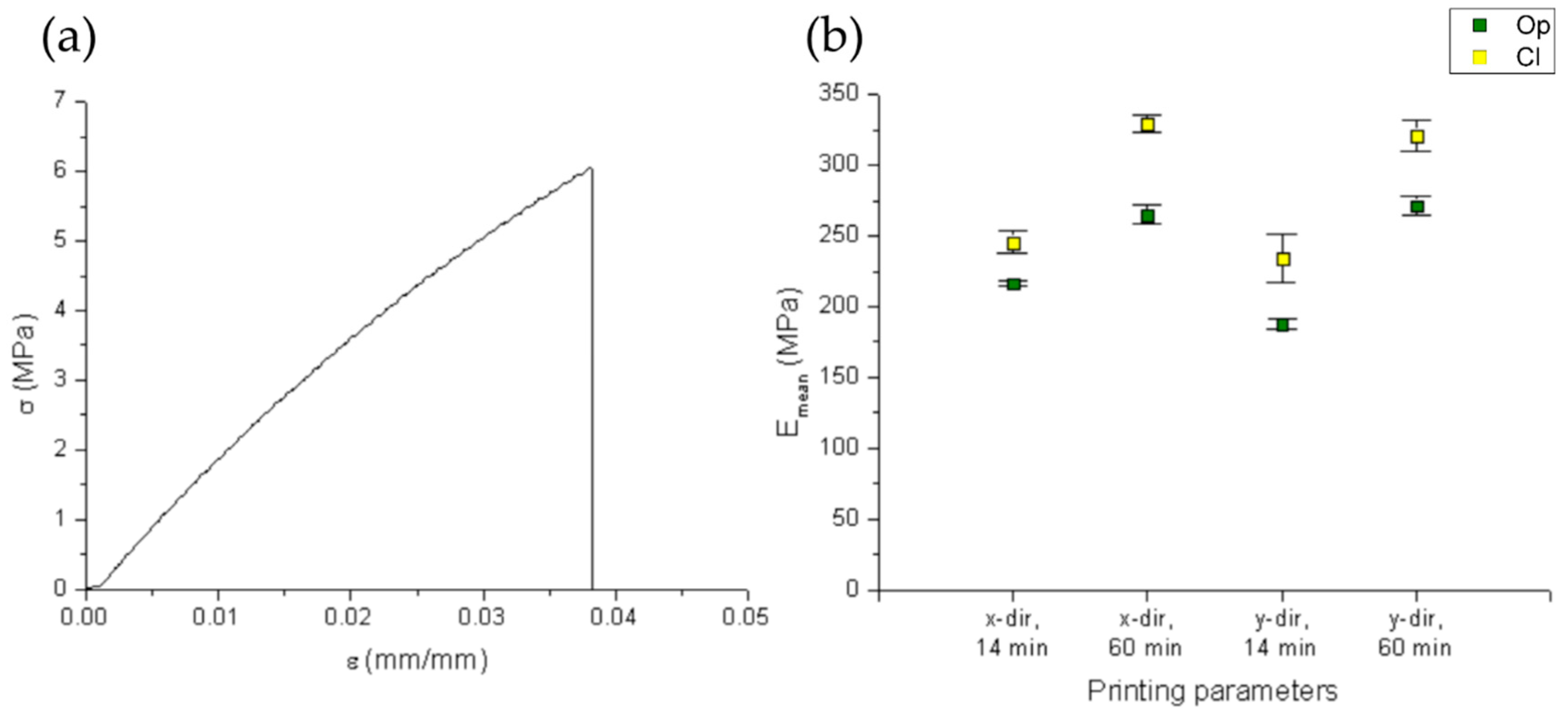
2.6. Mechanical Characterization
2.7. Statistical Analysis of the Results
2.8. Micrometric Spring Printing
3. Results
3.1. Dimensional Characterization
3.2. Mechanical Characterization
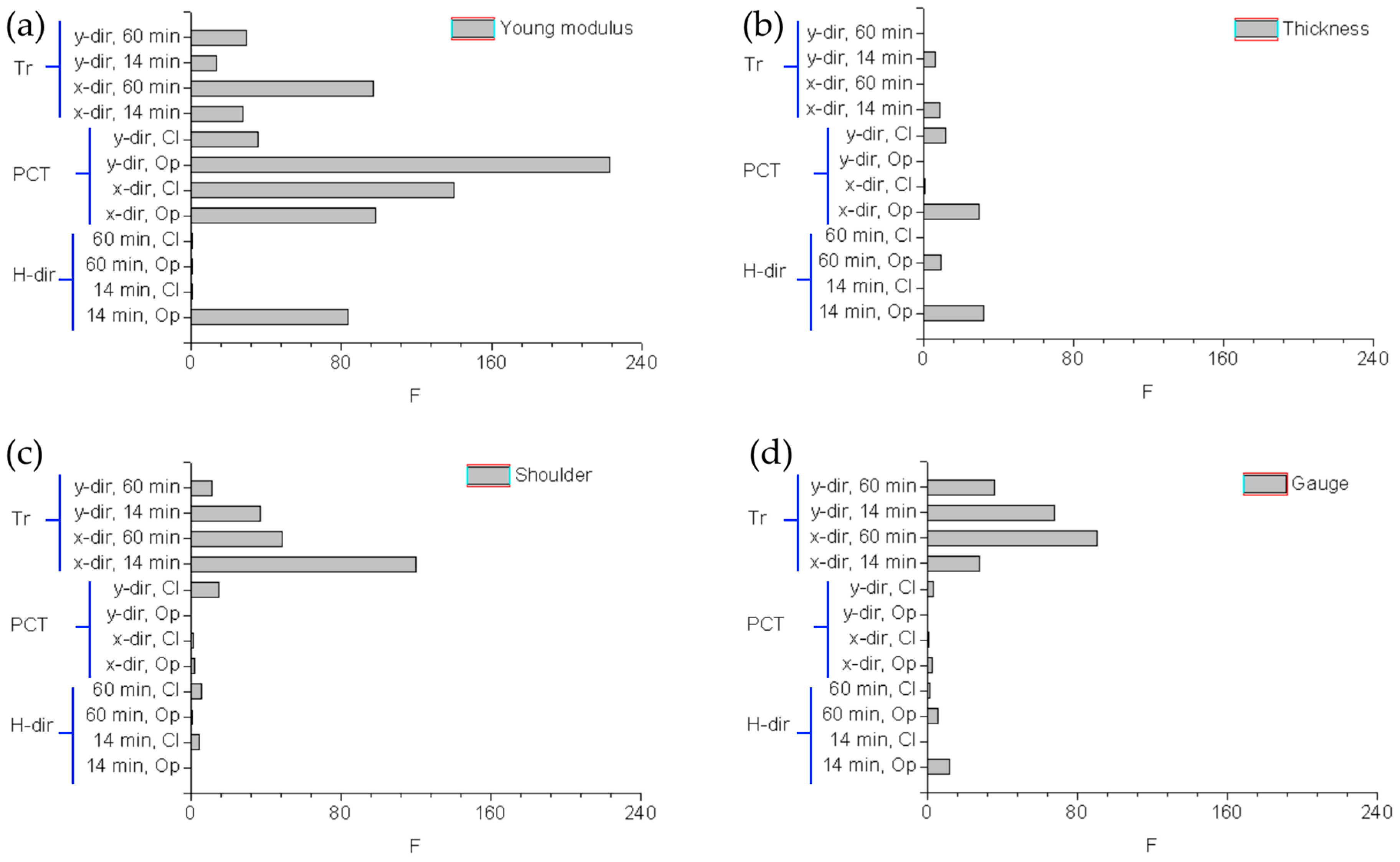
3.3. Statistical Analysis of the Results
3.4. Micrometric Spring Printing
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
| Literature | Investigated Parameters * | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Influence on: | Year | Author | LT | HS | SV | O | HO | LP | PC | Resin Used |
| Mechanical properties | 1992 | Jacobs [33] | x | x | x | x | NR* | |||
| 1997 | Schaub et al. [34] | x | x | x | NR | |||||
| 2004 | Hague et al. [27] | x | x | Epoxy-based | ||||||
| 2006 | Chockalingam et al. [35] | x | x | x | Epoxy-based | |||||
| 2008 | Chockalingam et al. [22] | x | x | x | Epoxy-based | |||||
| 2009 | Salmoria et al. [25] | x | x | Epoxy-based | ||||||
| 2014 | Raju et al. [36] | x | x | x | Epoxy-based | |||||
| 2014 | Bangalore et al. [37] | x | x | x | Epoxy-based | |||||
| Dimensional accuracy | 1997 | Rahmati et al. [38] | x | x | x | Epoxy-based | ||||
| 1998 | Onuh et al. [39] | x | x | x | Acrylic-based | |||||
| 2000 | Zhou et al. [28] | x | x | x | NR | |||||
| 2000 | Cho et al. [23] | x | x | x | Epoxy-based | |||||
| 2001 | Lee et al. [40] | x | x | x | NR | |||||
| 2016 | Chockalingam et al. [41] | x | x | x | Epoxy-based | |||||
References
- Hull, C.W. Apparatus for Production of Three-Dimensional Objects by Stereolithography. U.S. Patent 4,575,330A, 8 August 1986. [Google Scholar]
- De Pasquale, G. Artificial human joint for the characterization of piezoelectric transducers in self-powered telemedicine applications. Meccanica 2016, 51, 2259–2275. [Google Scholar] [CrossRef]
- Vecchio, M.D.; Fea, A.M.; Spinetta, R.; Marasso, S.L.; Cocuzza, M.; Scaltrito, L.; Canavese, G. Behaviour of the intraocular pressure during manual and vented gas forced infusion in a simulated pars plana vitrectomy. Int. J. Appl. Eng. Res. 2017, 12, 6751–6757. [Google Scholar]
- De Pasquale, G.; Kim, S.-G.; De Pasquale, D. GoldFinger: Wireless human-machine interface with dedicated software and biomechanical energy harvesting system. IEEE/ASME Trans. Mechatron. 2015, 21, 565–575. [Google Scholar] [CrossRef]
- Vaezi, M.; Seitz, H.; Yang, S. A review on 3D micro-additive manufacturing technologies. Int. J. Adv. Manuf. Technol. 2013, 67, 1721–1754. [Google Scholar] [CrossRef]
- Bertana, V.; Potrich, C.; Pirri, C.F.; Pederzolli, C. 3D-printed microfluidics on thin poly(methyl methacrylate) substrates for genetic applications. J. Vac. Sci. Technol. B Nanotechnol. Microelectron. Mater. Process. Meas. Phenom. 2018, 36, 01A106. [Google Scholar] [CrossRef]
- Perrucci, F.; Bertana, V.; Marasso, S.L.; Scordo, G.; Ferrero, S.; Pirri, C.F.; Cocuzza, M.; El-Tamer, A.; Hinze, U.; Chichkov, B.N.; et al. Optimization of a suspended two photon polymerized microfluidic filtration system. Microelectron. Eng. 2018, 195, 95–100. [Google Scholar] [CrossRef]
- Mao, M.; He, J.; Li, X.; Zhang, B.; Lei, Q.; Liu, Y.; Li, D. The emerging frontiers and applications of high-resolution 3D printing. Micromachines 2017, 8, 113. [Google Scholar] [CrossRef]
- Gonzalez, G.; Chiappone, A.; Roppolo, I.; Fantino, E.; Bertana, V.; Perrucci, F.; Scaltrito, L.; Pirri, F.; Sangermano, M. Development of 3D printable formulations containing CNT with enhanced electrical properties. Polymer 2017, 109, 246–253. [Google Scholar] [CrossRef]
- Scordo, G.; Bertana, V.; Scaltrito, L.; Ferrero, S.; Cocuzza, M.; Marasso, S.L.; Romano, S.; Sesana, R.; Catania, F.; Pirri, C.F. A novel highly electrically conductive composite resin for stereolithography. Mater. Today Commun. 2019, 19, 12–17. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, Z.; Song, X.; Zhu, B.; Hsiai, T.; Wu, P.; Xiong, R.; Shi, J.; Chen, Y.; Zhou, Q.; et al. Three dimensional printing of high dielectric capacitor using projection based stereolithography method. Nano Energy 2016, 22, 414–421. [Google Scholar] [CrossRef]
- Tanwilaisiri, A.; Zhang, R.; Xu, Y.; Harrison, D.; Fyson, J. A manufacturing process for an energy storage device using 3D printing. In Proceedings of the 2016 IEEE International Conference on Industrial Technology (ICIT), Taipei, Taiwan, 14–17 March 2016. [Google Scholar]
- Nassar, I.T.; Weller, T.M. An electrically-small, 3-D cube antenna fabricated with additive manufacturing. In Proceedings of the 2013 IEEE Topical Conference on Wireless Sensors and Sensor Networks (WiSNet), Austin, TX, USA, 20–23 January 2013; pp. 85–87. [Google Scholar]
- Massaccesi, A.; Pirinoli, P.; Bertana, V.; Scordo, G.; Marasso, S.L.; Cocuzza, M.; Dassano, G. 3D-Printable Dielectric Transmitarray With Enhanced Bandwidth at Millimeter-Waves. IEEE Access. 2018, 6, 46407–46418. [Google Scholar] [CrossRef]
- De Pasquale, G. Energy harvesters for powering wireless systems. In Handbook of MEMS for Wireless and Mobile Applications; Uttamchandani, D., Ed.; Woodhead Publishing Ltd.: Cambridge, UK, 2013; pp. 345–400. [Google Scholar]
- Hofstätter, T.; Pedersen, D.B.; Tosello, G.; Hansen, H.N. Applications of Fiber-Reinforced Polymers in Additive Manufacturing. Procedia CIRP 2017, 66, 312–316. [Google Scholar] [CrossRef]
- Hofstaetter, T.; Pedersen, D.B.; Nielsen, J.S.; Mischkot, M.; Hansen, H.N. Investigation of digital light processing using fibre-reinforced polymers. In Proceedings of the Euspen’s 16th International Conference & Exhibition, Nottingham, UK, 30 May–3 June 2016. [Google Scholar]
- Füzesi, K.; Gyöngy, M. Comparison of Two Inexpensive Rapid Prototyping Methods for Manufacturing Filament Target Ultrasound Phantoms. Ultrasound Med. Biol. 2017, 43, 712–720. [Google Scholar] [CrossRef] [PubMed]
- Macarie, L.; Ilia, G. Influence of pigment properties on UV-curing efficiency. J. Appl. Polym. Sci. 2007, 104, 247–252. [Google Scholar] [CrossRef]
- Im, Y.G.; Chung, S.I.; Son, J.H.; Jung, Y.D.; Jo, J.G.; Jeong, H.D. Functional prototype development: Inner visible multi-color prototype fabrication process using stereo lithography. J. Mater. Process. Technol. 2002, 130–131, 372–377. [Google Scholar] [CrossRef]
- Gong, H.-J.; Mao-Lu, W.; Yang, W. Surface Hardness Evaluation of Components Made by Color Stereolithography. J. Shanghai Jiaotong Univ. 2013, 18, 38–741. [Google Scholar] [CrossRef]
- Chockalingam, K.; Jawahar, N.; Chandrasekar, U.; Ramanathan, K.N. Establishment of process model for part strength in stereolithography. J. Mater. Process. Technol. 2008, 208, 348–365. [Google Scholar] [CrossRef]
- Cho, H.S.; Park, W.S.; Choi, B.W.; Leu, M.C. Determining optimal parameters for stereolithography processes via genetic algorithm. J. Manuf. Syst. 2000, 19, 18–27. [Google Scholar] [CrossRef]
- Salmoria, G.V.; Ahrens, C.H.; Fredel, M.; Soldi, V.; Pires, A.T.N. Stereolithography somos 7110 resin: Mechanical behavior and fractography of parts post-cured by different methods. Polym. Test. 2005, 24, 157–162. [Google Scholar] [CrossRef]
- Salmoria, G.V.; Ahrens, C.H.; Beal, V.E.; Pires, A.T.N.; Soldi, V. Evaluation of post-curing and laser manufacturing parameters on the properties of SOMOS 7110 photosensitive resin used in stereolithography. Mater. Des. 2009, 30, 758–763. [Google Scholar] [CrossRef]
- Karalekas, D.; Aggelopoulos, A. Study of shrinkage strains in a stereolithography cured acrylic photopolymer resin. J. Mater. Process. Technol. 2003, 136, 146–150. [Google Scholar] [CrossRef]
- Hague, R.; Mansour, S.; Saleh, N.; Harris, R. Materials analysis of stereolithography resins for use in Rapid Manufacturing. J. Mater. Sci. 2004, 39, 2457–2464. [Google Scholar] [CrossRef]
- Zhou, J.G.; Herscovici, D.; Chen, C.C. Parametric process optimization to improve the accuracy of rapid prototyped stereolithography parts. Int. J. Mach. Tools Manuf. 2000, 40, 363–379. [Google Scholar] [CrossRef]
- Lynn-Charney, C.; Rosen, D.W. Usage of accuracy models in stereolithography process planning. Rapid Prototyp. J. 2000, 6, 77–87. [Google Scholar] [CrossRef]
- Horton, L.; Gargiulo, E.; Keefe, M. An experimental study of the parameters affecting curl in parts created using stereolithography. In Solid Freeform Fabrication Symposium; Texas Academic Press: Austin, TX, USA, 1993; pp. 178–185. [Google Scholar]
- Wang, W.L.; Cheah, C.M.; Fuh, J.Y.H.; Lu, L. Influence of process parameters on stereolithography part shrinkage. Mater. Des. 1996, 17, 205–213. [Google Scholar] [CrossRef]
- Quintana, R.; Choi, J.W.; Puebla, K.; Wicker, R. Effects of build orientation on tensile strength for stereolithography- manufactured ASTM D-638 type i specimens. Int. J. Adv. Manuf. Technol. 2010, 46, 201–215. [Google Scholar] [CrossRef]
- Jacobs, P.F. Rapid Prototyping & Manufacturing: Fundamentals of Stereolithography, 1st ed.; McGraw-Hill: New York, NY, USA, 1992. [Google Scholar]
- Schaub, D.A.; Chu, K.-R.; Montgomery, D.C. Optimizing stereolithography throughput. J. Manuf. Syst. 1997, 16, 290–303. [Google Scholar] [CrossRef]
- Chockalingam, K.; Jawahar, N.; Ramanathan, K.N.; Banerjee, P.S. Optimization of stereolithography process parameters for part strength using design of experiments. Int. J. Adv. Manuf. Technol. 2006, 29, 79–88. [Google Scholar] [CrossRef]
- Raju, B.S.; Shekar, U.C.; Venkateswarlu, K.; Drakashayani, D.N. Establishment of Process Model for Rapid Prototyping Technique (Stereolithography) to Enhance the Part Quality by Taguchi Method. Procedia Technol. 2014, 14, 380–389. [Google Scholar] [CrossRef]
- Bangalore, R.; Gowda, S.; Udayagiri, C.S.; Narendra, D.D. Studies on the Process Parameters of Rapid Prototyping Technique (Stereolithography) for the Betterment of Part Quality. Int. J. Manuf. Eng. 2014, 2014, 11. [Google Scholar]
- Rahmati, S.; Dickens, P. Stereolithography for injection mould tooling. Rapid Prototyp. J. 1997, 3, 53–60. [Google Scholar] [CrossRef]
- Onuh, S.O.; Hon, K.K.B. Application of the Taguchi method and new hatch styles for quality improvement in stereolithography. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 1998, 212, 461–472. [Google Scholar] [CrossRef]
- Lee, S.H.; Park, W.S.; Cho, H.S.; Zhang, W.; Leu, M.C. A neural network approach to the modelling and analysis of stereolithography processes. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2001, 215, 1719–1733. [Google Scholar] [CrossRef]
- Chockalingam, K.; Jawahar, N.; Chandrasekhar, U.; Praveen, J.; Karthic, M. Development of Process Model for Optimal Selection of Process Parameters for Geometric Tolerances and Surface Roughness in Stereolithography. Int. J. Adv. Des. Manuf. Technol. 2016, 9, 103–113. [Google Scholar]



| Parameter * | Level 1 | Level 2 |
|---|---|---|
| PCT | 14 min (7 min per side) | 60 min (30 min per side) |
| Tr | Op | Cl |
| H-dir | x-dir | y-dir |
| Comb. # | H-dir | PCT | Tr | Samples # |
|---|---|---|---|---|
| 1 | x | 14 min | Op | 1 to 3 |
| 2 | x | 60 min | Op | 4 to 6 |
| 3 | y | 14 min | Op | 7 to 9 |
| 4 | y | 60 min | Op | 10 to 12 |
| 5 | x | 14 min | Cl | 13 to 15 |
| 6 | x | 60 min | Cl | 16 to 18 |
| 7 | y | 14 min | Cl | 19 to 21 |
| 8 | y | 60 min | Cl | 22 to 24 |
| Parameters Combination | Fixed Values | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| H-dir | PCT | Tr | |||||||
| x | y | 14 | 60 | Op | Cl | Group | Comb. # | ||
| Factor of influence (F) | H-dir | - | - | ✓ | ✓ | a | 1-3 | ||
| - | - | ✓ | ✓ | b | 5-7 | ||||
| - | - | ✓ | ✓ | c | 2-4 | ||||
| - | - | ✓ | ✓ | d | 6-8 | ||||
| PCT | ✓ | - | - | ✓ | e | 1-2 | |||
| ✓ | - | - | ✓ | f | 5-6 | ||||
| ✓ | - | - | ✓ | g | 3-4 | ||||
| ✓ | - | - | ✓ | h | 7-8 | ||||
| Tr | ✓ | ✓ | - | - | i | 1-5 | |||
| ✓ | ✓ | - | - | l | 2-6 | ||||
| ✓ | ✓ | - | - | m | 3-7 | ||||
| ✓ | ✓ | - | - | n | 4-8 | ||||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bertana, V.; De Pasquale, G.; Ferrero, S.; Scaltrito, L.; Catania, F.; Nicosia, C.; Marasso, S.L.; Cocuzza, M.; Perrucci, F. 3D Printing with the Commercial UV-Curable Standard Blend Resin: Optimized Process Parameters towards the Fabrication of Tiny Functional Parts. Polymers 2019, 11, 292. https://doi.org/10.3390/polym11020292
Bertana V, De Pasquale G, Ferrero S, Scaltrito L, Catania F, Nicosia C, Marasso SL, Cocuzza M, Perrucci F. 3D Printing with the Commercial UV-Curable Standard Blend Resin: Optimized Process Parameters towards the Fabrication of Tiny Functional Parts. Polymers. 2019; 11(2):292. https://doi.org/10.3390/polym11020292
Chicago/Turabian StyleBertana, Valentina, Giorgio De Pasquale, Sergio Ferrero, Luciano Scaltrito, Felice Catania, Carmelo Nicosia, Simone L. Marasso, Matteo Cocuzza, and Francesco Perrucci. 2019. "3D Printing with the Commercial UV-Curable Standard Blend Resin: Optimized Process Parameters towards the Fabrication of Tiny Functional Parts" Polymers 11, no. 2: 292. https://doi.org/10.3390/polym11020292
APA StyleBertana, V., De Pasquale, G., Ferrero, S., Scaltrito, L., Catania, F., Nicosia, C., Marasso, S. L., Cocuzza, M., & Perrucci, F. (2019). 3D Printing with the Commercial UV-Curable Standard Blend Resin: Optimized Process Parameters towards the Fabrication of Tiny Functional Parts. Polymers, 11(2), 292. https://doi.org/10.3390/polym11020292







