Kinetic Prediction of Fast Curing Polyurethane Resins by Model-Free Isoconversional Methods
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
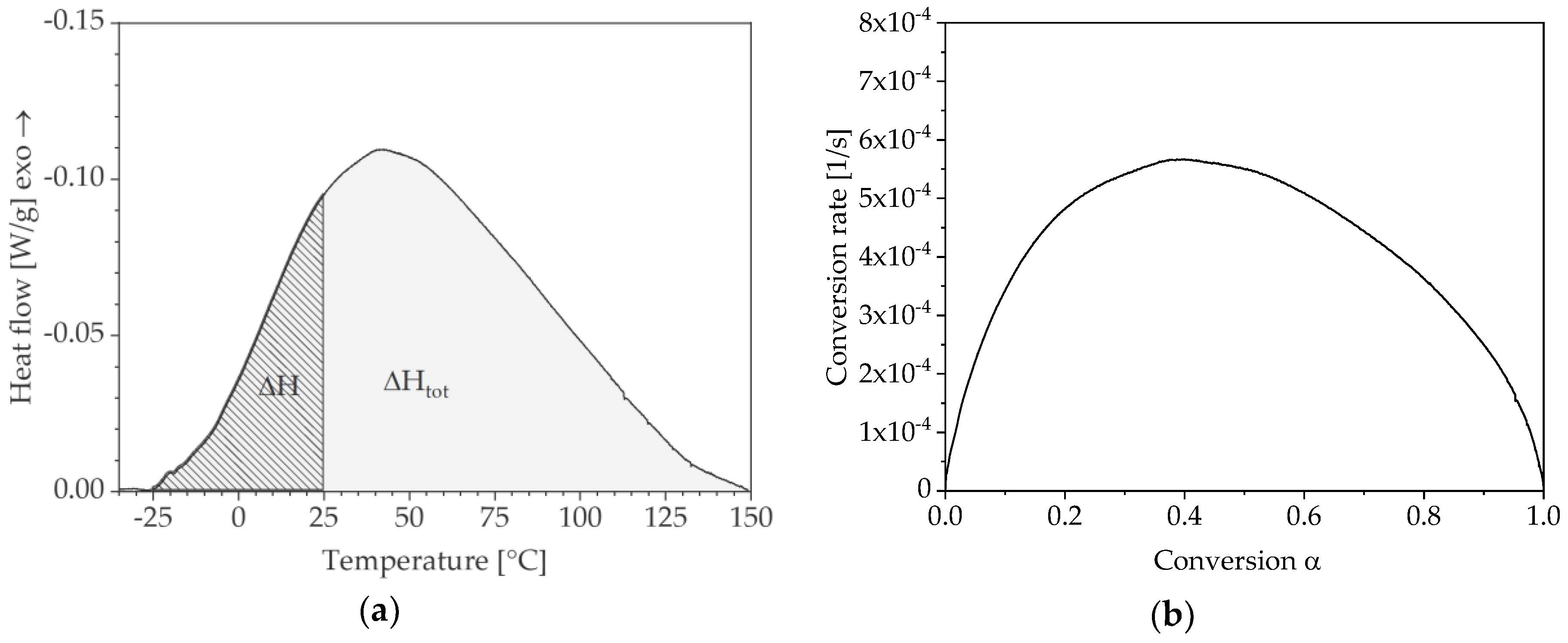
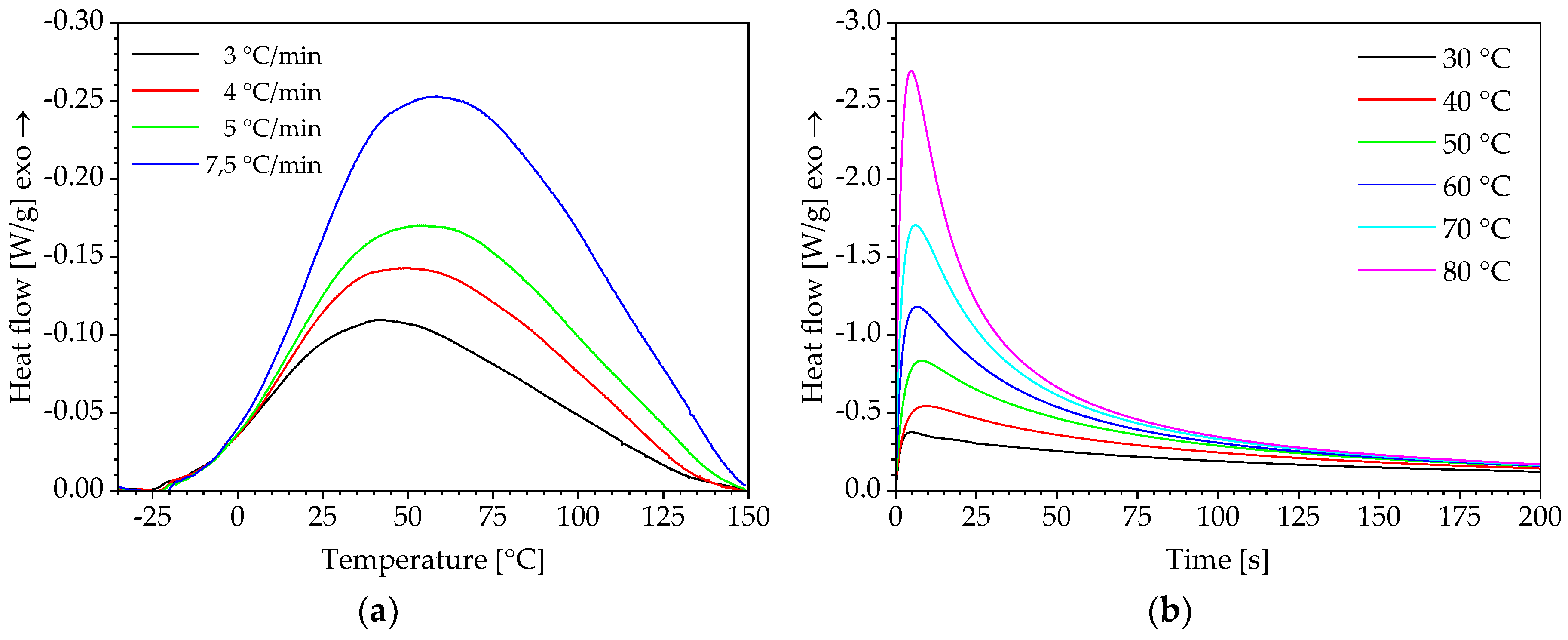
2.2. Differential Scanning Calorimetry
2.3. Reaction Kinetic Modelling
3. Results and Discussion
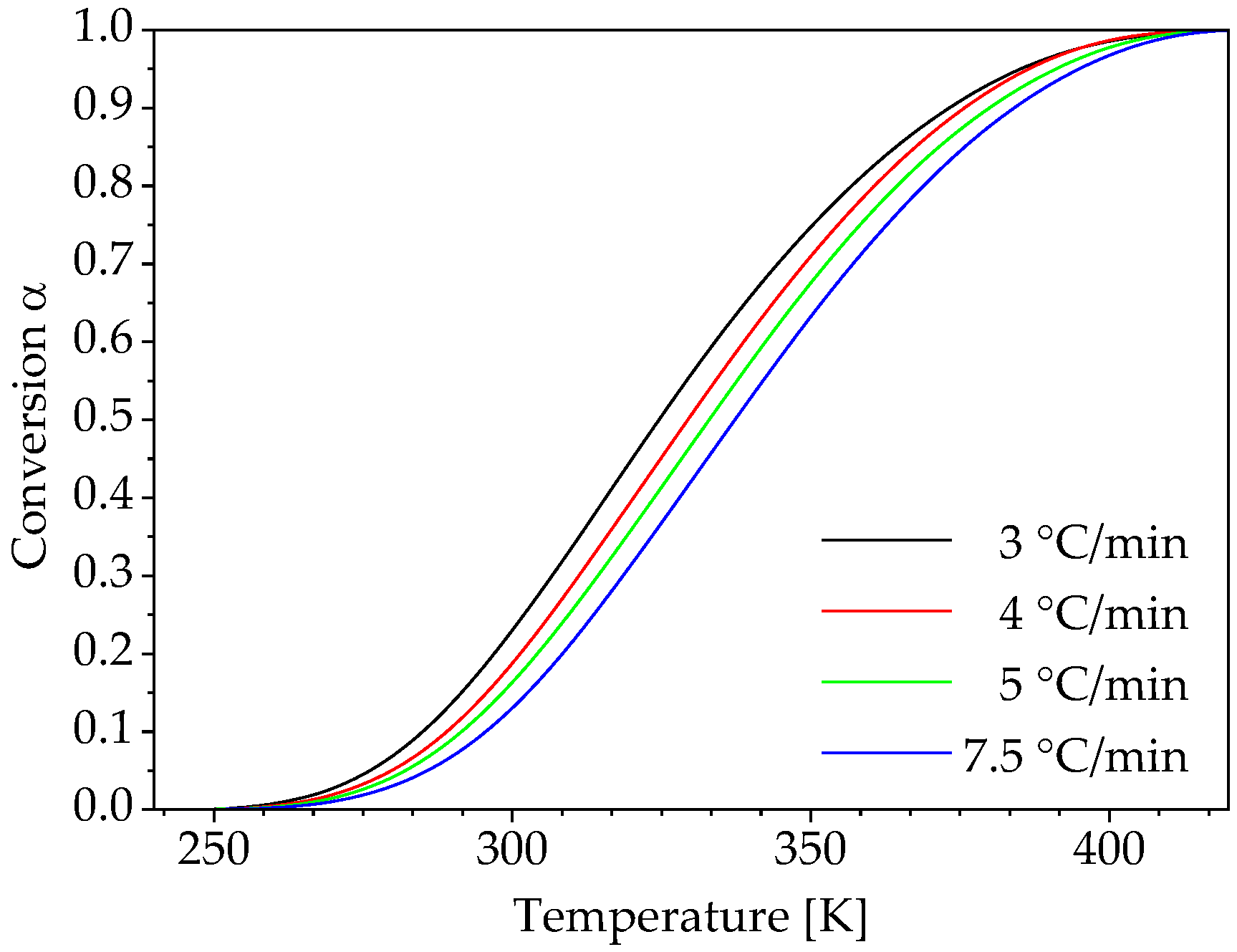
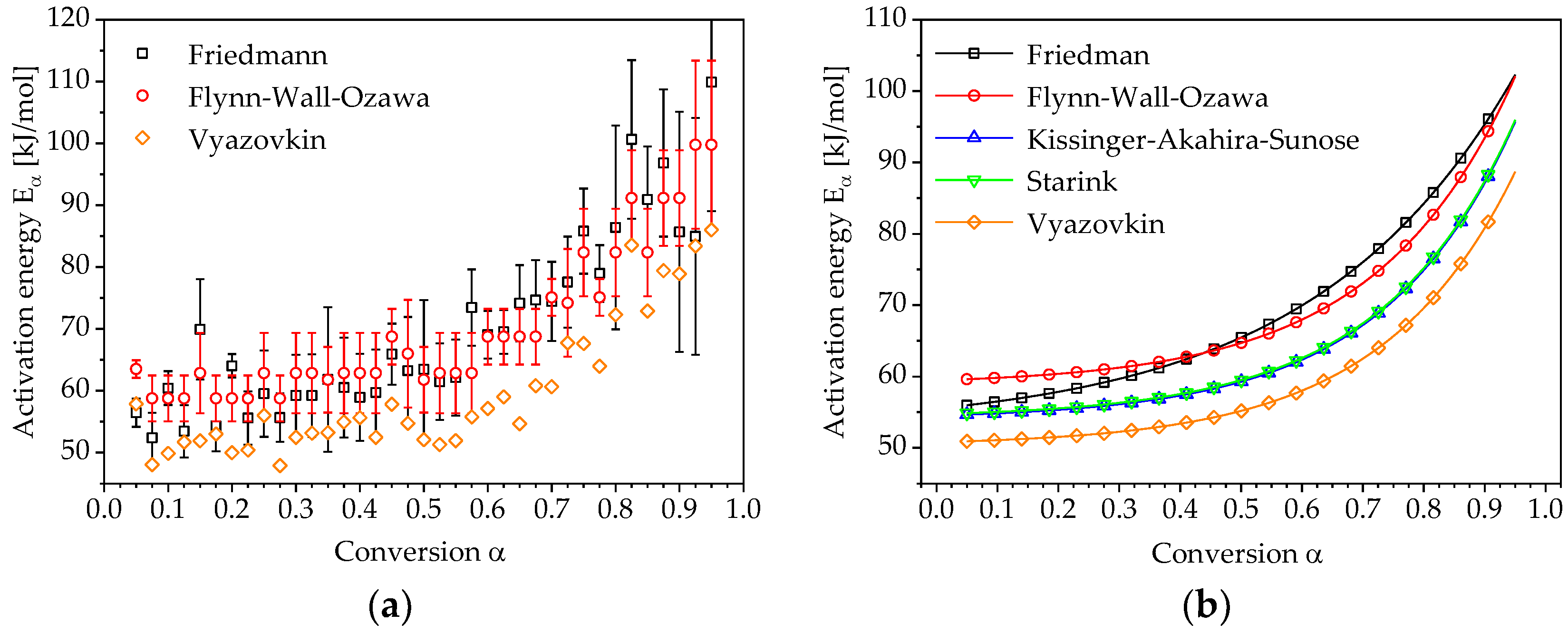
3.1. Determination of Conversion-Dependent Activation Energy
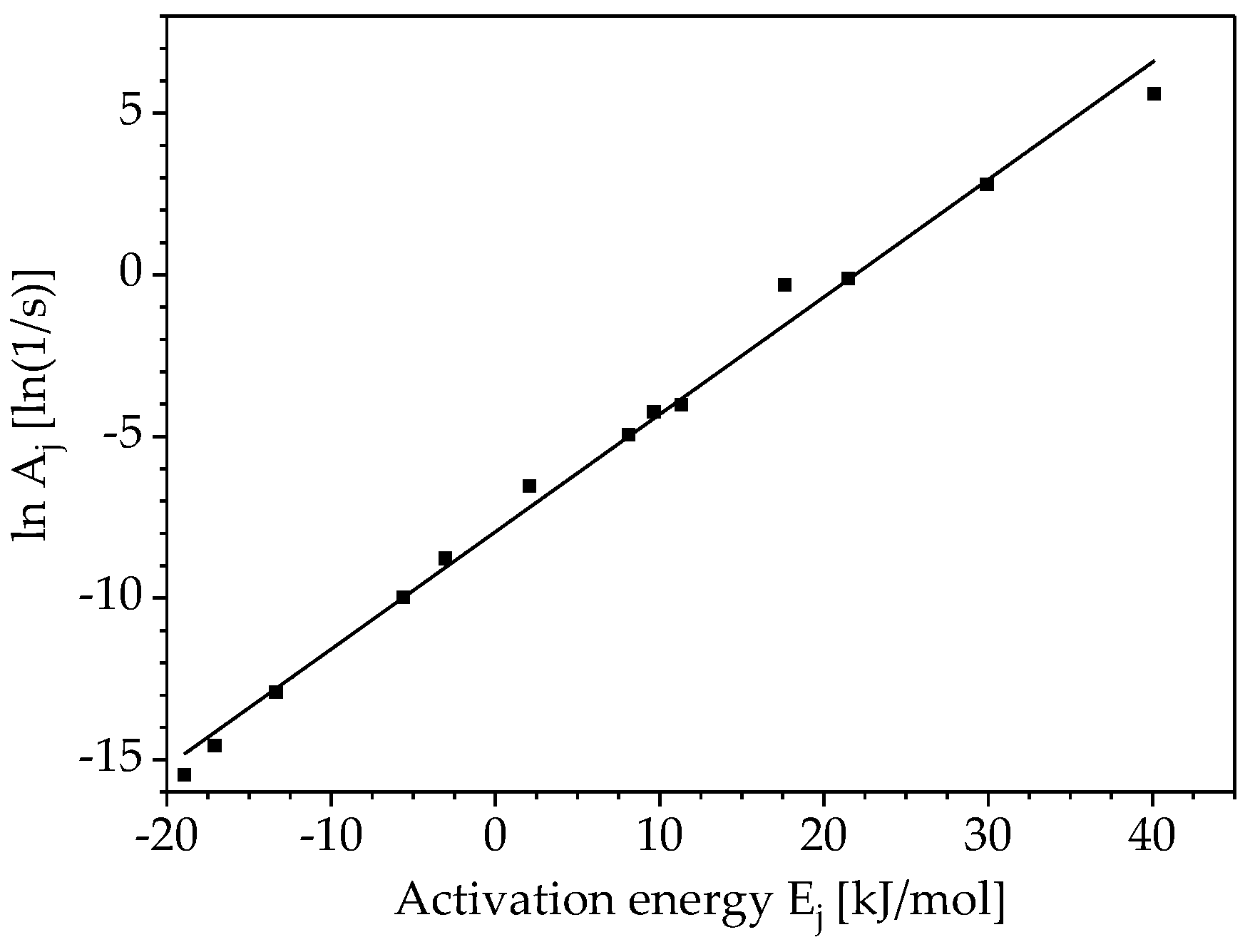
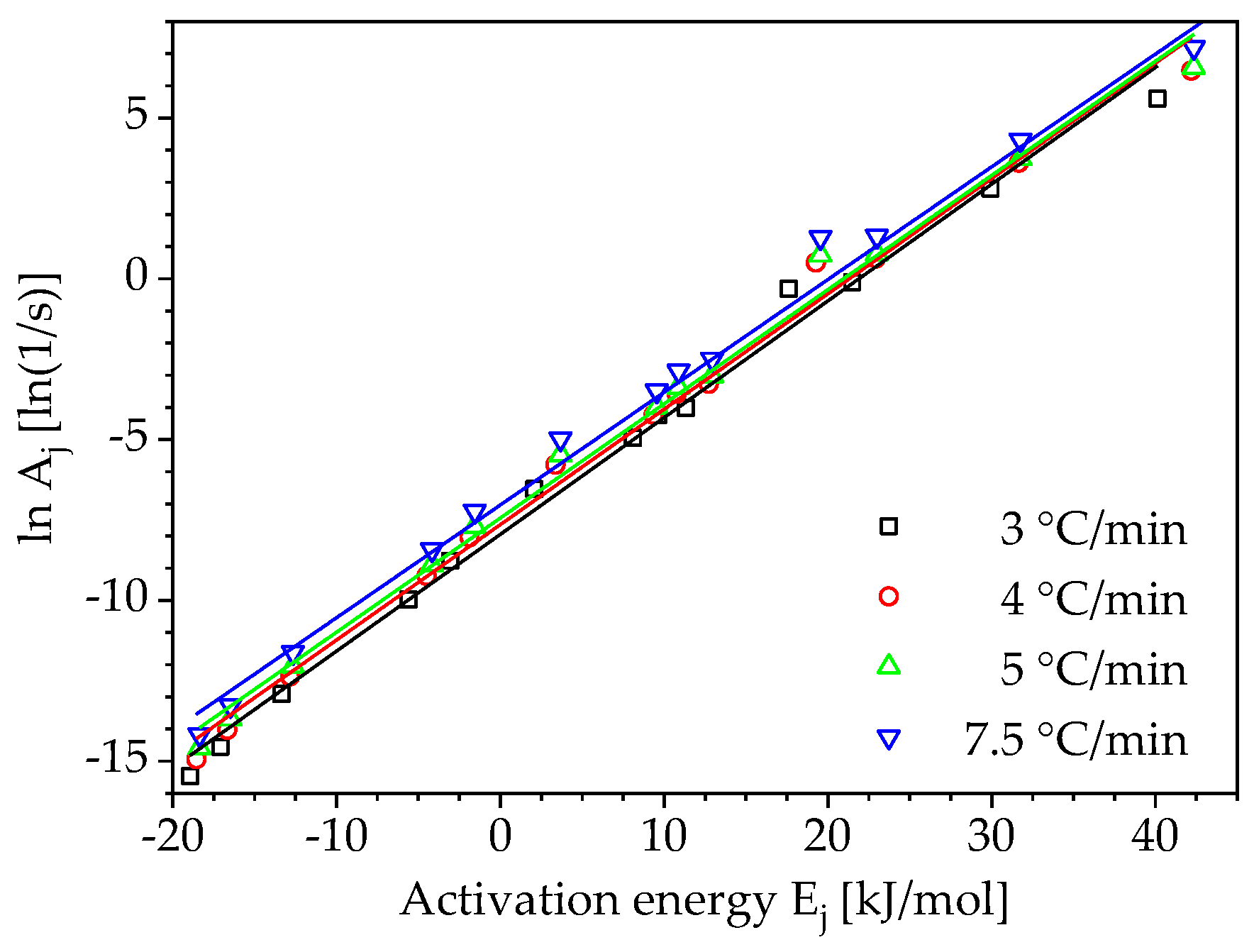
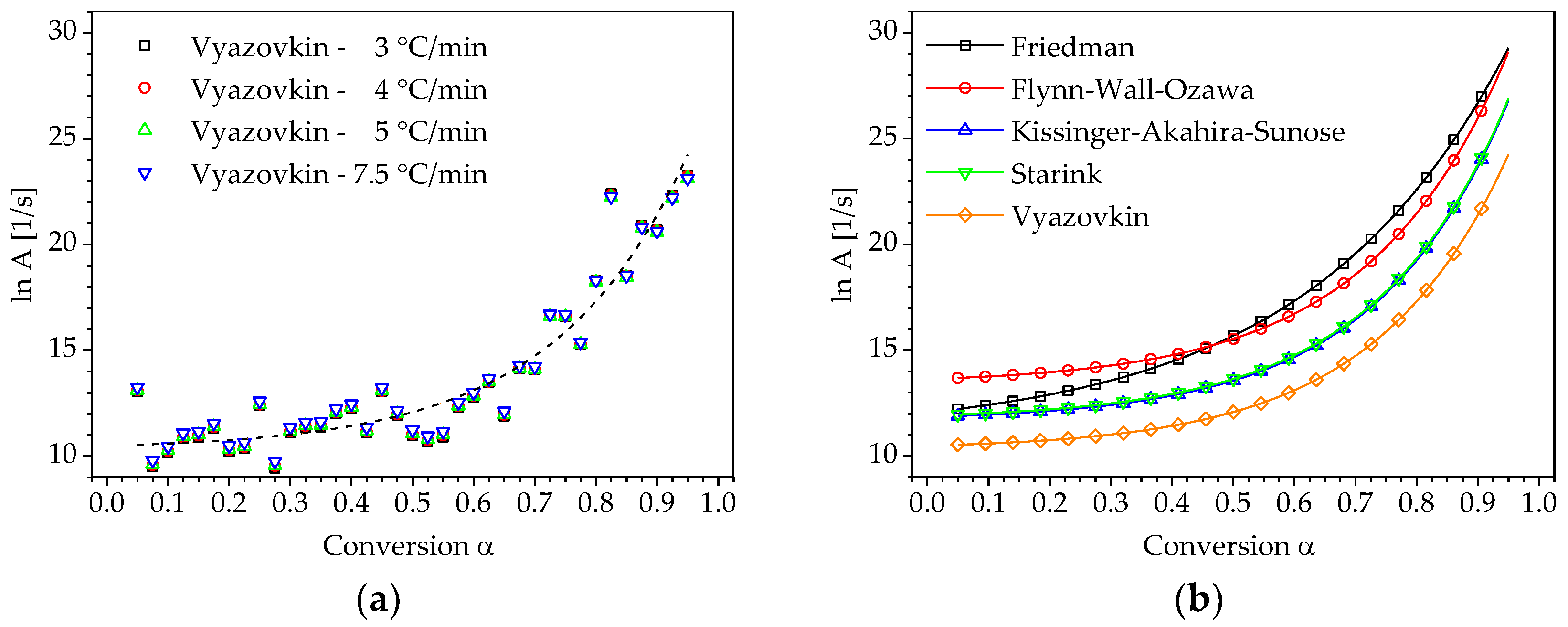
3.2. Determination of Pre-Exponential Factor and Reaction Model
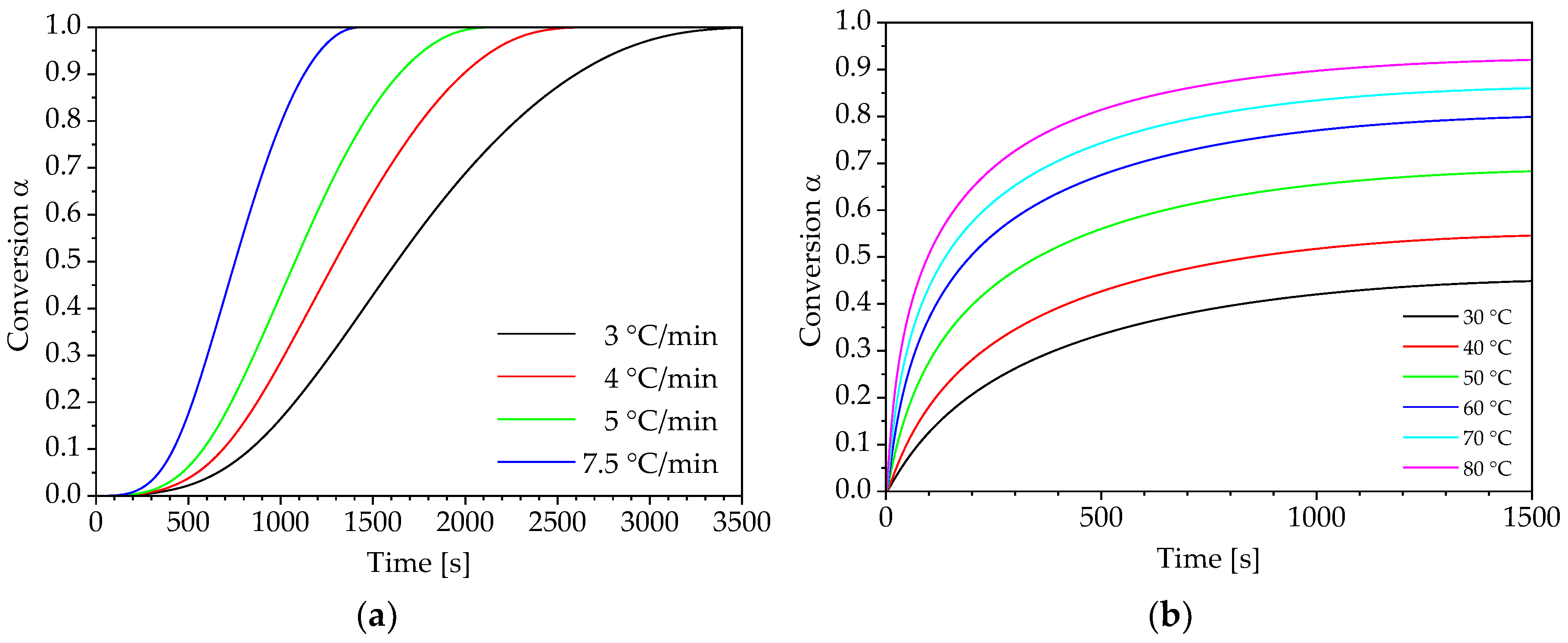
3.3. Prediction of Reaction Kinetics
4. Conclusions and Outlook
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lee, W.H.; Lee, S.W.; Kang, T.J.; Chung, K.; Youn, J.R. Processing of polyurethane/polystyrene hybrid foam and numerical simulation. Fibers Polym. 2002, 3, 159–168. [Google Scholar] [CrossRef]
- Samkhaniani, N.; Gharehbaghi, A.; Ahmadi, Z. Numerical simulation of reaction injection molding with polyurethane foam. J. Cell. Plast. 2013, 49, 405–421. [Google Scholar] [CrossRef]
- Canal, L.P.; Benavente, M.; Hausmann, M.; Michaud, V. Process-induced strains in RTM processing of polyurethane/carbon composites. Compos. Part A 2015, 78, 264–273. [Google Scholar] [CrossRef]
- Puaux, J.-P.; Cassagnau, P.; Bozga, G.; Nagy, I. Modeling of polyurethane synthesis by reactive extrusion. Chem. Eng. Process. Process Intensif. 2006, 45, 481–487. [Google Scholar] [CrossRef]
- Seo, D.; Ryoun Youn, J.; Tucker, C.L. Numerical simulation of mold filling in foam reaction injection molding. Int. J. Numer. Methods Fluids 2003, 42, 1105–1134. [Google Scholar] [CrossRef]
- Seo, D.; Youn, J.R. Numerical analysis on reaction injection molding of polyurethane foam by using a finite volume method. Polymer 2005, 46, 6482–6493. [Google Scholar] [CrossRef]
- Tröltzsch, J.; Schäfer, K.; Niedziela, D.; Ireka, I.; Steiner, K.; Kroll, L. Simulation of RIM-process for Polyurethane Foam Expansion in Fiber Reinforced Sandwich Structures. Procedia CIRP 2017, 66, 62–67. [Google Scholar] [CrossRef]
- Geier, S.; Winkler, C.; Piesche, M. Numerical Simulation of Mold Filling Processes with Polyurethane Foams. Chem. Eng. Technol. 2009, 32, 1438–1447. [Google Scholar] [CrossRef]
- Abdessalam, H.; Abbès, B.; Guo, Y.Q.; Kwassi, E.; Romain, J.L. Numerical Simulation of Polyurethane Foaming Process Using Finite Point Method. AMR 2013, 856, 179–183. [Google Scholar] [CrossRef]
- Abdessalam, H.; Abbès, B.; Li, Y.; Guo, Y.-Q.; Kwassi, E.; Romain, J.-L.; Saanouni, K. Prediction of acoustic foam properties by numerical simulation of polyurethane foaming process. MATEC Web Conf. 2016, 80, 16005. [Google Scholar] [CrossRef] [Green Version]
- Bikard, J.; Bruchon, J.; Coupez, T.; Silva, L. Numerical simulation of 3D polyurethane expansion during manufacturing process. Colloids Surf. A Physicochem. Eng. Asp. 2007, 309, 49–63. [Google Scholar] [CrossRef]
- Riviere, S.; Khelladi, S.; Farzaneh, S.; Bakir, F.; Tcharkhtchi, A. Simulation of polymer flow using smoothed particle hydrodynamics method. Polym. Eng. Sci. 2013, 53, 2509–2518. [Google Scholar] [CrossRef] [Green Version]
- Chiacchiarelli, L.M.; Puri, I.; Puglia, D.; Kenny, J.M.; Torre, L. Cure kinetics of a highly reactive silica–polyurethane nanocomposite. Thermochim. Acta 2012, 549, 172–178. [Google Scholar] [CrossRef]
- Kim, D.S.; Kim, J.-T.; Woo, W.B. Reaction kinetics and characteristics of polyurethane/clay nanocomposites. J. Appl. Polym. Sci. 2005, 96, 1641–1647. [Google Scholar] [CrossRef]
- Chiacchiarelli, L.M.; Kenny, J.M.; Torre, L. Kinetic and chemorheological modeling of the vitrification effect of highly reactive poly(urethane-isocyanurate) thermosets. Thermochim. Acta 2013, 574, 88–97. [Google Scholar] [CrossRef]
- Dimier, F.; Sbirrazzuoli, N.; Vergnes, B.; Vincent, M. Curing kinetics and chemorheological analysis of polyurethane formation. Polym. Eng. Sci. 2004, 44, 518–527. [Google Scholar] [CrossRef]
- Sun, X.; Toth, J.; Lee, L.J. Chemorheology of poly(urethane/isocyanurate) formation. Polym. Eng. Sci. 1997, 37, 143–152. [Google Scholar] [CrossRef]
- Fernandez d’Arlas, B.; Rueda, L.; Stefani, P.M.; de la Caba, K.; Mondragon, I.; Eceiza, A. Kinetic and thermodynamic studies of the formation of a polyurethane based on 1,6-hexamethylene diisocyanate and poly(carbonate-co-ester)diol. Thermochim. Acta 2007, 459, 94–103. [Google Scholar] [CrossRef]
- Friedman, H.L. Kinetics of thermal degradation of char-forming plastics from thermogravimetry. Application to a phenolic plastic. J. Polym. Sci. C Polym. Symp. 1964, 6, 183–195. [Google Scholar] [CrossRef]
- Ozawa, T. A New Method of Analyzing Thermogravimetric Data. Bull. Chem. Soc. Jpn. 1965, 38, 1881–1886. [Google Scholar] [CrossRef] [Green Version]
- Kissinger, H.E. Reaction Kinetics in Differential Thermal Analysis. Anal. Chem. 1957, 29, 1702–1706. [Google Scholar] [CrossRef]
- Kamal, M.R.; Sourour, S. Kinetics and thermal characterization of thermoset cure. Polym. Eng. Sci. 1973, 13, 59–64. [Google Scholar] [CrossRef]
- Vyazovkin, S.; Dollimore, D. Linear and Nonlinear Procedures in Isoconversional Computations of the Activation Energy of Nonisothermal Reactions in Solids. J. Chem. Inf. Comput. Sci. 1996, 36, 42–45. [Google Scholar] [CrossRef]
- Vyazovkin, S. Isoconversional Kinetics of Thermally Stimulated Processes; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Vyazovkin, S.; Wight, C.A. Model-free and model-fitting approaches to kinetic analysis of isothermal and nonisothermal data. Thermochim. Acta 1999, 340–341, 53–68. [Google Scholar] [CrossRef]
- Ramis, X.; Cadenato, A.; Morancho, J.M.; Salla, J.M. Curing of a thermosetting powder coating by means of DMTA, TMA and DSC. Polymer 2003, 44, 2067–2079. [Google Scholar] [CrossRef]
- Sbirrazzuoli, N. Determination of pre-exponential factors and of the mathematical functions f(α) or G(α) that describe the reaction mechanism in a model-free way. Thermochim. Acta 2013, 564, 59–69. [Google Scholar] [CrossRef]
- Ramis, X.; Salla, J.M.; Cadenato, A.; Morancho, J.M. Simulation of isothermal cure of A powder coating. J. Therm. Anal. Calorim. 2003, 72, 707–718. [Google Scholar] [CrossRef]
- Fernandez, X.; Ramis, X.; Salla, J.M. Cationic copolymerization of cycloaliphatic epoxy resin with an spirobislactone with lanthanum triflate as initiator. Thermochim. Acta 2005, 438, 144–154. [Google Scholar] [CrossRef]
- Doyle, C.D. Estimating isothermal life from thermogravimetric data. J. Appl. Polym. Sci. 1962, 6, 639–642. [Google Scholar] [CrossRef]
- Flynn, J.H.; Wall, L.A. A quick, direct method for the determination of activation energy from thermogravimetric data. J. Polym. Sci. B Polym. Lett. 1966, 4, 323–328. [Google Scholar] [CrossRef]
- Starink, M.J. The determination of activation energy from linear heating rate experiments: A comparison of the accuracy of isoconversion methods. Thermochim. Acta 2003, 404, 163–176. [Google Scholar] [CrossRef]
- Gotor, F.J.; Criado, J.M.; Malek, J.; Koga, N. Kinetic Analysis of Solid-State Reactions: The Universality of Master Plots for Analyzing Isothermal and Nonisothermal Experiments. J. Phys. Chem. A 2000, 104, 10777–10782. [Google Scholar] [CrossRef]
- Roşu, D.; Mititelu, A.; Caşcaval, C.N. Cure kinetics of a liquid-crystalline epoxy resin studied by non-isothermal data. Polym. Test. 2004, 23, 209–215. [Google Scholar] [CrossRef]
- Criado, J.M.; Pérez-Maqueda, L.A.; Gotor, F.J.; Málek, J.; Koga, N. A unified theory for the kinetic analysis of solid state reactions under any thermal pathway. J. Therm. Anal. Calorim. 2003, 72, 901–906. [Google Scholar] [CrossRef]
- Cadenato, A.; Morancho, J.M.; Fernández-Francos, X.; Salla, J.M.; Ramis, X. Comparative kinetic study of the non-isothermal thermal curing of bis-GMA/TEGDMA systems. J. Therm. Anal. Calorim. 2007, 89, 233–244. [Google Scholar] [CrossRef]
- Vyazovkin, S. Evaluation of activation energy of thermally stimulated solid-state reactions under arbitrary variation of temperature. J. Comput. Chem. 1997, 18, 393–402. [Google Scholar] [CrossRef]
- Adeli, M.; Zarnegar, Z.; Dadkhah, A.; Hossieni, R.; Salimi, F.; Kanani, A. Linear-dendritic ABA triblock copolymers as nanocarriers. J. Appl. Polym. Sci. 2007, 104, 267–272. [Google Scholar] [CrossRef]
- Vergnaud, J.-W.; Bouzon, J. Differential Scanning Calorimetry. In Cure of Thermosetting Resins; Vergnaud, J.-W., Bouzon, J., Eds.; Springer: London, UK, 1992; pp. 213–268. [Google Scholar]



| Code | Reaction model | |||
|---|---|---|---|---|
| 1 | P4 | Power law | ||
| 2 | P3 | Power law | ||
| 3 | P2 | Power law | ||
| 4 | P2/3 | Power law | ||
| 5 | D1 | One-dimensional diffusion | ||
| 6 | F1 | Mampel (first-order) | ||
| 7 | A4 | Avrami-Erofeev | ||
| 8 | A3 | Avrami-Erofeev | ||
| 9 | A2 | Avrami-Erofeev | ||
| 10 | D3 | Three-dimensional diffusion | ||
| 11 | R3 | Contracting sphere | ||
| 12 | R2 | Contracting cylinder | ||
| 13 | D2 | Second-order |
| DSC isotherm | DSC non-isotherm | ||||
|---|---|---|---|---|---|
| [°C] | [J/g] | [J/g] | [J/g] | [°C/min] | [J/g] |
| 30 | 75.3 | 46.2 | 121.5 | 3 | 193.3 |
| 40 | 91.5 | 32.2 | 123.7 | 4 | 194.0 |
| 50 | 114.4 | 24.4 | 138.8 | 5 | 188.8 |
| 60 | 133.7 | 19.6 | 153.3 | 7.5 | 187.4 |
| 70 | 143.3 | 14.7 | 158.0 | ||
| 80 | 155.4 | 12.9 | 168.3 | ||
| 90 | 158.9 | 5.9 | 164.8 | ||
| 100 | 161.5 | 1.5 | 163.0 | ||
| 110 | 170.2 | 0 | 170.2 | ||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stanko, M.; Stommel, M. Kinetic Prediction of Fast Curing Polyurethane Resins by Model-Free Isoconversional Methods. Polymers 2018, 10, 698. https://doi.org/10.3390/polym10070698
Stanko M, Stommel M. Kinetic Prediction of Fast Curing Polyurethane Resins by Model-Free Isoconversional Methods. Polymers. 2018; 10(7):698. https://doi.org/10.3390/polym10070698
Chicago/Turabian StyleStanko, Michael, and Markus Stommel. 2018. "Kinetic Prediction of Fast Curing Polyurethane Resins by Model-Free Isoconversional Methods" Polymers 10, no. 7: 698. https://doi.org/10.3390/polym10070698
APA StyleStanko, M., & Stommel, M. (2018). Kinetic Prediction of Fast Curing Polyurethane Resins by Model-Free Isoconversional Methods. Polymers, 10(7), 698. https://doi.org/10.3390/polym10070698






