Supramolecular Assemblies in Pb(II) Complexes with Hydrazido-Based Ligands
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
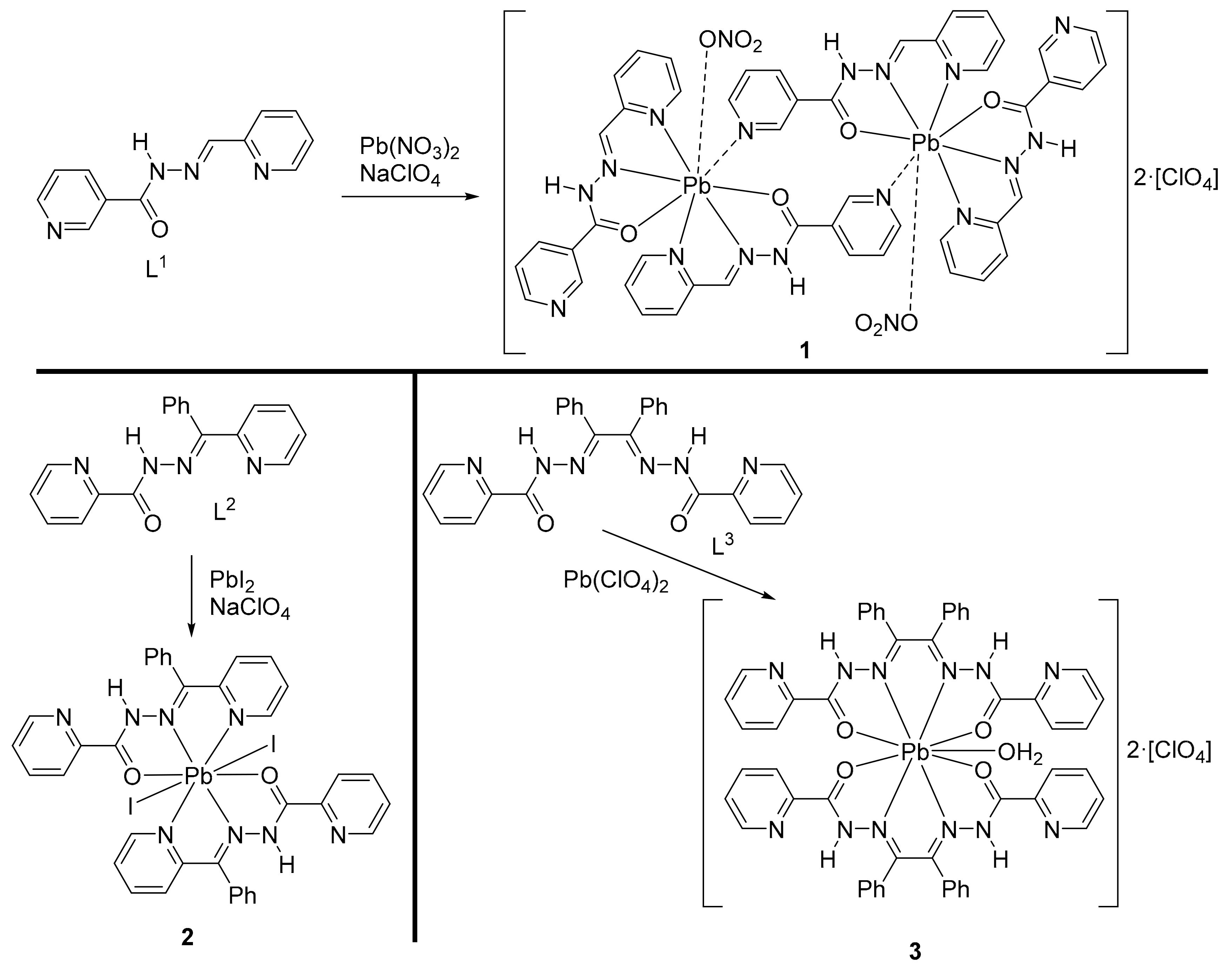
2.2. Synthesis of the Crystals 1–3
2.3. Characterization
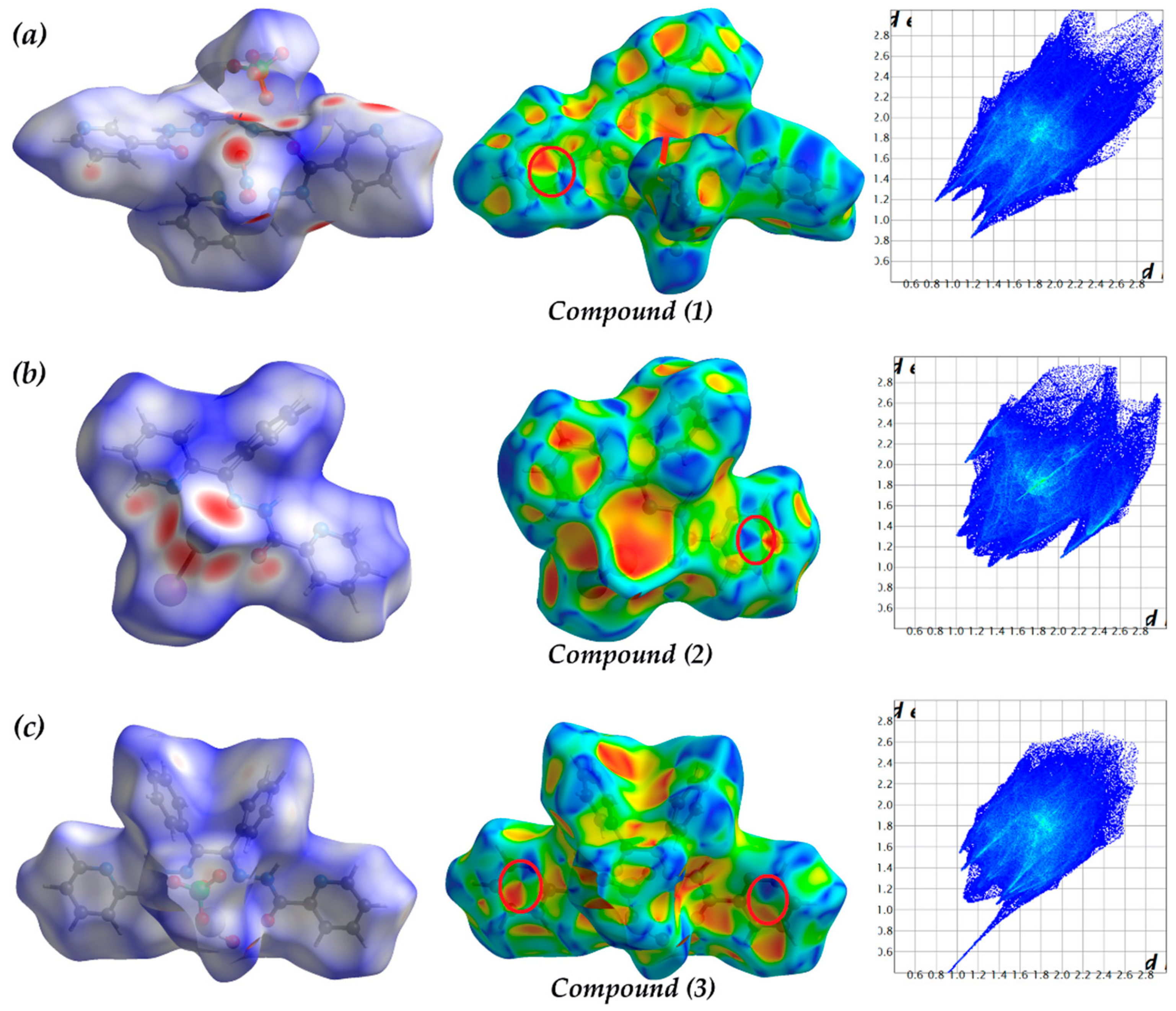
2.4. Hirshfeld Surface Analysis
2.5. Computational Details
3. Results and Discussion
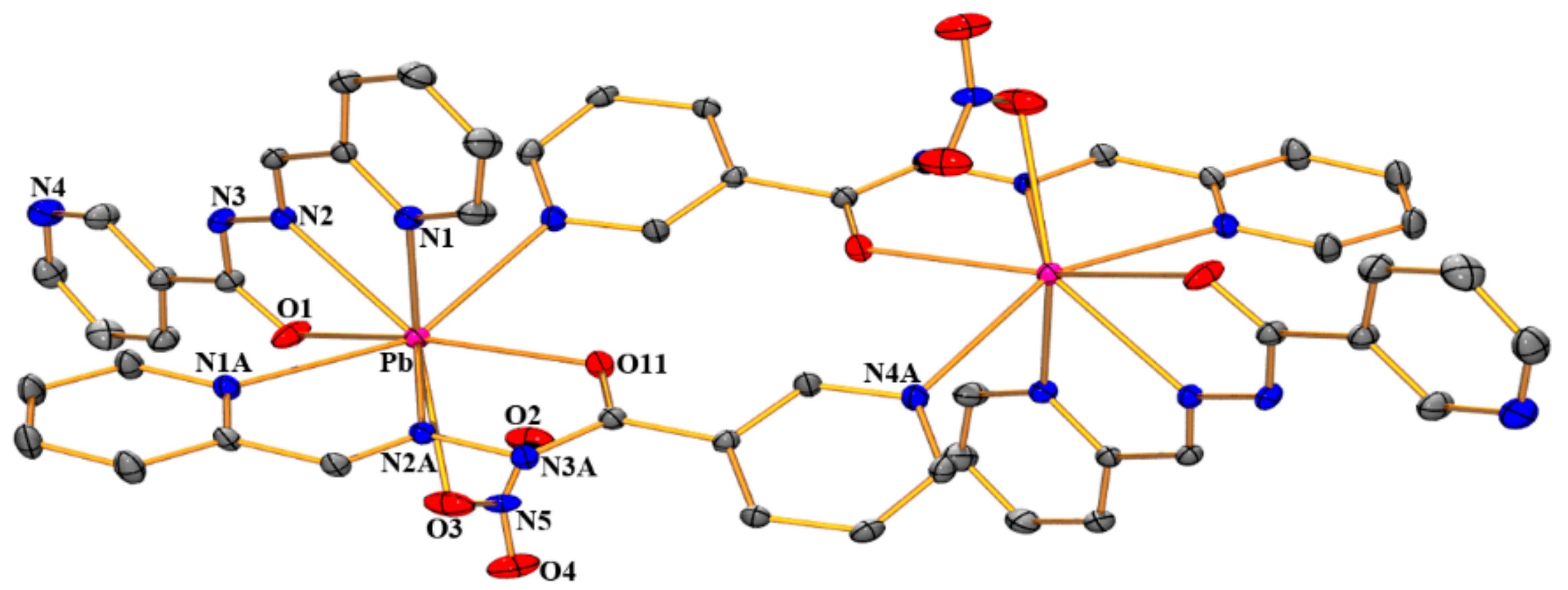
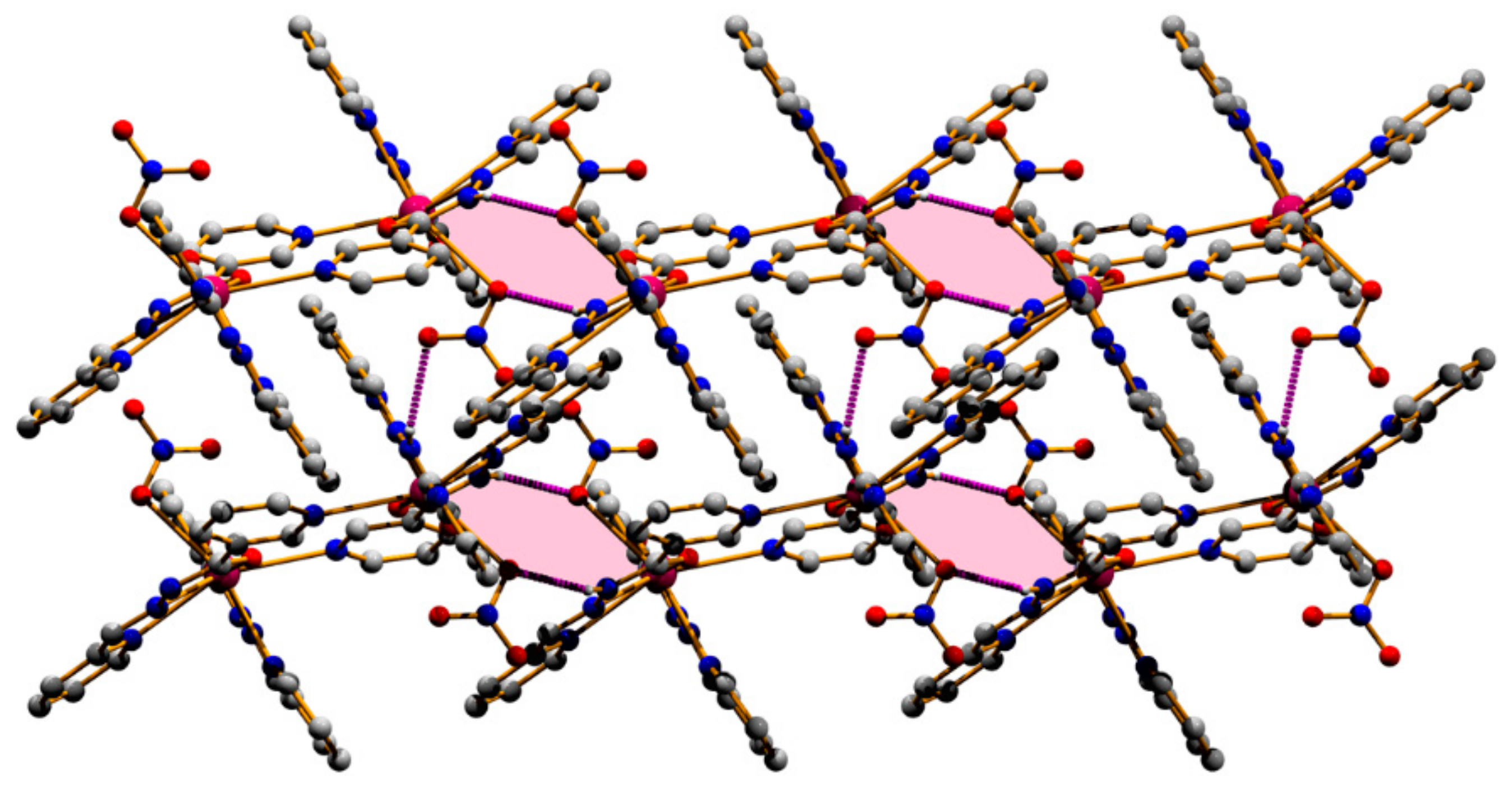
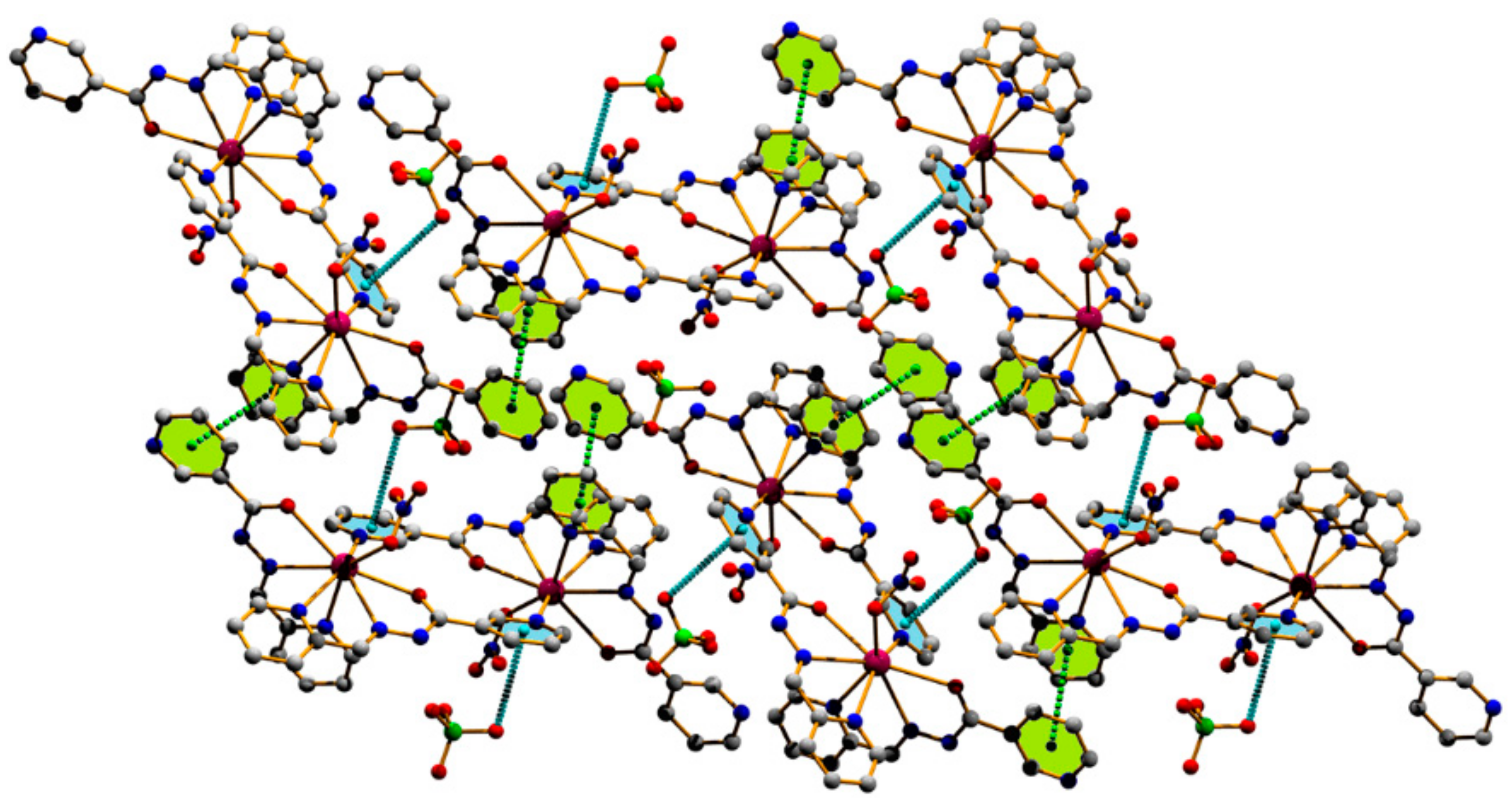
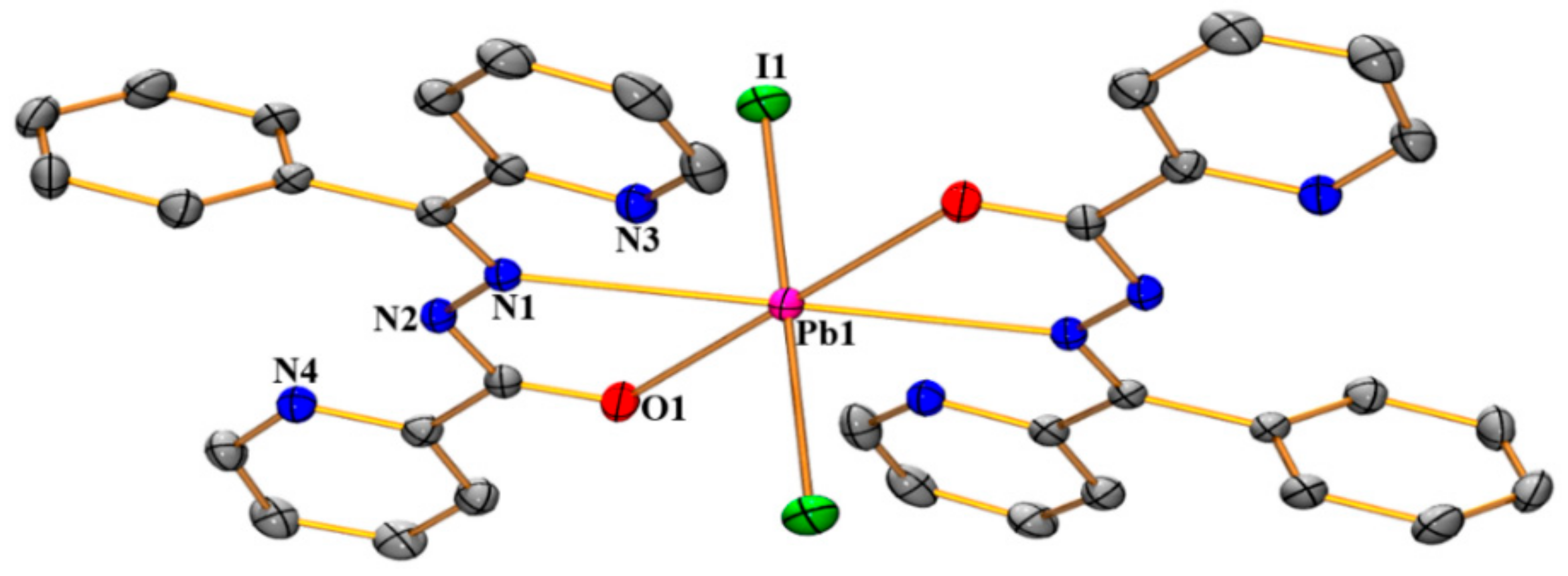
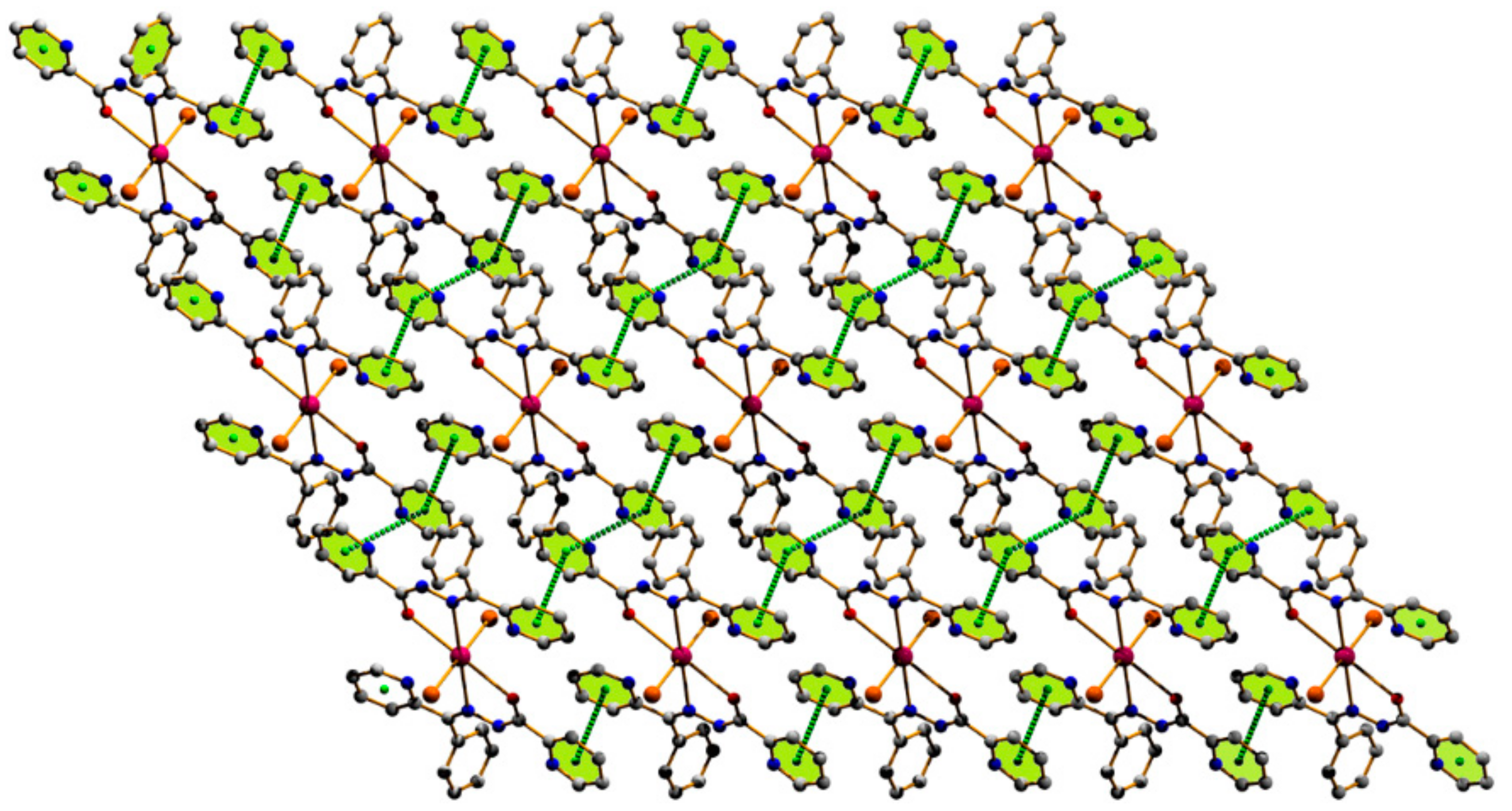
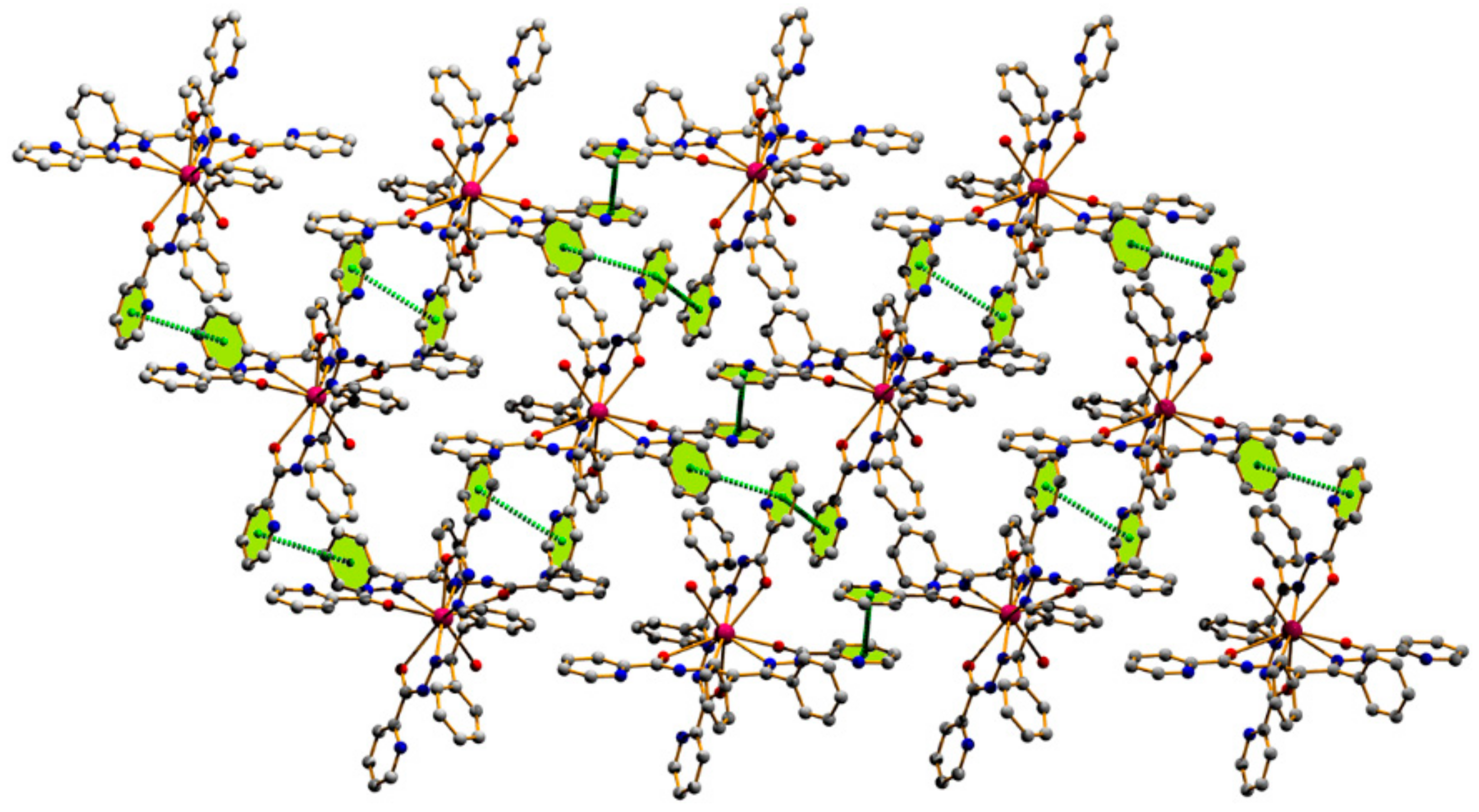
3.1. Structural Analysis
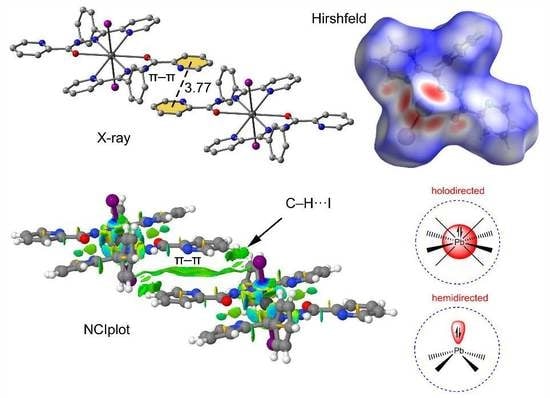
3.2. Hirshfeld Surface Analysis
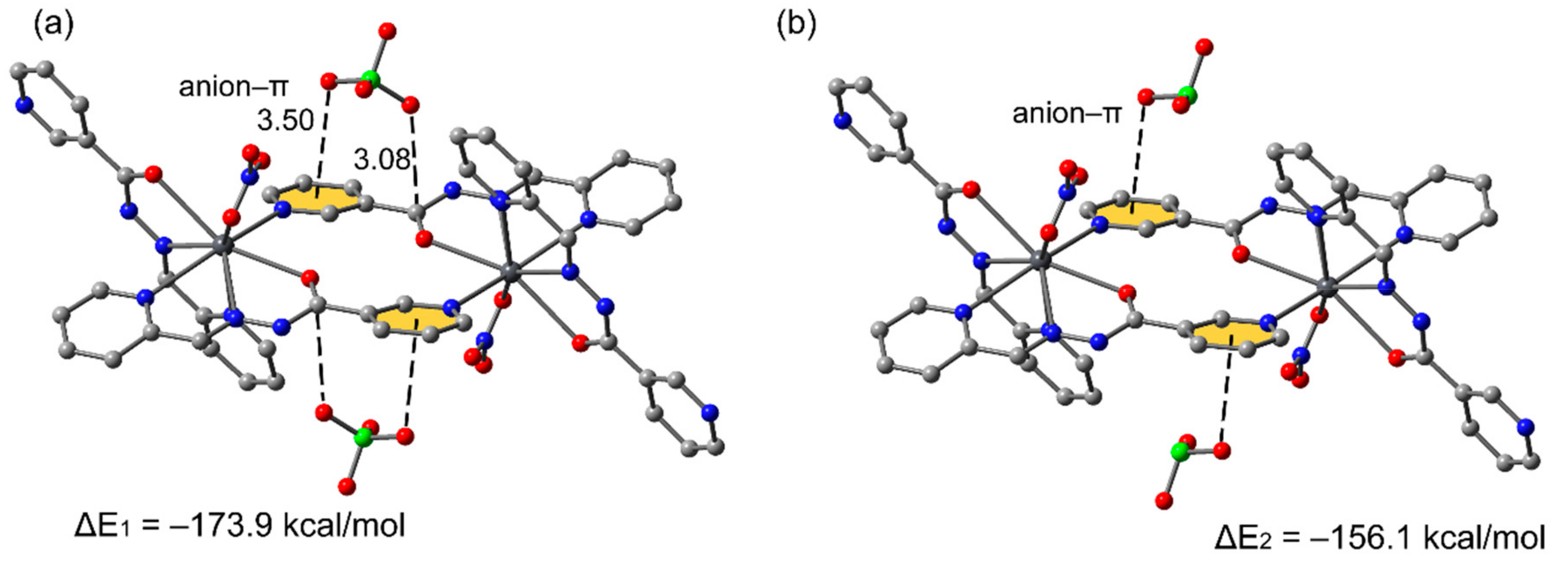
3.3. Theoretical Study
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hancock, R.D.; Reibenspies, J.H.; Maumela, H. Structural Effects of the Lone Pair on Lead(II), and Parallels with the Coordination Geometry of Mercury(II). Does the Lone Pair on Lead(II) Form H-Bonds? Structures of the Lead(II) and Mercury(II) Complexes of the Pendant-Donor Macrocycle DOTAM (1,4,7,10-Tetrakis(carbamoylmethyl)-1,4,7,10-tetraazacyclododecane). Inorg. Chem. 2004, 43, 2981–2987. [Google Scholar]
- Platas-Iglesias, C.; Esteban-Gomez, D.; Enriquez-Perez, T.; Avecilla, F.; de Blas, A.; Rodriguez-Blas, T. Lead(II) Thiocyanate Complexes with Bibracchial Lariat Ethers: An X-ray and DFT Study. Inorg. Chem. 2005, 44, 2224–2233. [Google Scholar] [CrossRef]
- Reger, D.L.; Wright, T.D.; Little, C.A.; Lamba, J.J.S.; Smith, M.D. Control of the Stereochemical Impact of the Lone Pair in Lead(II) Tris(pyrazolyl)methane Complexes. Improved Preparation of Na{B[3,5-(CF3)2C6H3]4}. Inorg. Chem. 2001, 40, 3810–3814. [Google Scholar]
- Fleischer, H.; Schollmeyer, D. Synthesis of and Structural Studies on Lead(II) Cysteamin Complexes. Inorg. Chem. 2004, 43, 5529–5536. [Google Scholar] [CrossRef]
- Mahmudov, K.T.; Gurbanov, A.V.; Guseinov, F.I.; Guedes da Silva, M.F.C. Noncovalent interactions in metal complex catalysis. Coord. Chem. Rev. 2019, 387, 32–46. [Google Scholar] [CrossRef]
- Zhang, L.; Qin, Y.-Y.; Li, Z.-J.; Lin, Q.-P.; Cheng, J.-K.; Zhang, J.; Yao, Y.-G. Topology Analysis and Nonlinear-Optical-Active Properties of Luminescent Metal−Organic Framework Materials Based on Zinc/Lead Isophthalates. Inorg. Chem. 2008, 47, 8286–8293. [Google Scholar] [CrossRef]
- Olvera, A.; Shi, G.; Djieutedjeu, H.; Page, A.; Uher, C.; Kioupakis, E.; Poudeu, P.F.P. Pb7Bi4Se13: A Lillianite Homologue with Promising Thermoelectric Properties. Inorg. Chem. 2015, 54, 746–755. [Google Scholar] [CrossRef]
- Cheng, F.; Liang, J.; Tao, Z.; Chen, J. Functional materials for rechargeable batteries. Adv. Mater. 2011, 23, 1695–1715. [Google Scholar] [CrossRef]
- Casas, J.S.; Sordo, J. Lead: Chemistry, Analytical Aspects, Enviromental Impact and Health Effects; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Seaton, C.L.; Lasman, J.; Smith, D.R. The Effects of CaNa2EDTA on Brain Lead Mobilization in Rodents Determined Using a Stable Lead Isotope Tracer. Toxicol. Appl. Pharmacol. 1999, 159, 153–160. [Google Scholar] [CrossRef]
- Mohammadzadeh, M.; Basu, O.D.; Herrera, J.E. Impact of Water Chemistry on Lead Carbonate Dissolution in Drinking Water Distribution Systems. J. Water Resour. Prot. 2015, 7, 389–397. [Google Scholar] [CrossRef][Green Version]
- Markowitz, M.E.; Rosen, J.F. Need for the lead mobilization test in children with lead poisoning. J. Pediatr 1991, 119, 305–310. [Google Scholar] [CrossRef]
- Fan, S.-R.; Zhu, L.-G. Syntheses, Structures, and Characterizations of Four New Lead(II) 5-Sulfosalicylate Complexes with Both Chelating and Bridging Neutral Ligands. Inorg. Chem. 2007, 46, 6785–6793. [Google Scholar] [CrossRef]
- Ferreirs-Martínez, R.; Esteban-Gomez, D.; Toth, E.; de Blas, A.; Platas-Iglesias, C.; Rodríguez-Blas, T. Macrocyclic Receptor Showing Extremely High Sr(II)/Ca(II) and Pb(II)/Ca(II) Selectivities with Potential Application in Chelation Treatment of Metal Intoxication. Inorg. Chem. 2011, 50, 3772–3784. [Google Scholar] [CrossRef]
- Ratcliffe, J.M. Lead in Man and Environment; John Wiley & Sons: New York, NY, USA, 1981. [Google Scholar]
- Shimoni-Livny, L.; Glusker, J.P.; Bock, C.W. Lone Pair Functionality in Divalent Lead Compounds. Inorg. Chem. 1998, 37, 1853. [Google Scholar]
- Li, D.-Q.; Liu, X.; Zhou, J. Two New Hemidirected Lead(II) Complexes: [Pb(pcih)(bha)] and [Pb(pcih)(NO3)]. Z. Naturforsch. 2010, 65b, 1084–1088. [Google Scholar] [CrossRef]
- Gourlaouen, C.; Gérard, H.; Piquemal, J.P.; Parisel, O. Understanding Lead Chemistry from Topological Insights: The Transition between Holo- and Hemidirected Structures within the [Pb(CO)n]2+ Model Series. Chem. Eur. J. 2008, 14, 2730–2743. [Google Scholar] [CrossRef]
- Gourlaouen, C.; van Severen, M.-C.; Parisel, O. Application of the topological analysis of the electronic localization function to archetypical [Pb(II)Ln]p complexes: The bonding of Pb2+ revisited. J. Comput. Chem. 2010, 31, 185–194. [Google Scholar]
- Gourlaouen, C.; Gérard, H.; Parisel, O. Exploring the Hydration of Pb2+: Ab Initio Studies and First-Principles Molecular Dynamics. Chem. Eur. J. 2006, 12, 5024–5032. [Google Scholar] [CrossRef]
- Gourlaouen, C.; Parisela, O.; Gérard, H. Revisiting the holo- and hemidirected structural transition within the [Pb(CO)n]2+ model series using first-principles Molecular Dynamics. Dalton Trans. 2011, 40, 11282–11288. [Google Scholar] [CrossRef]
- Bauzá, A.; Seth, S.K.; Frontera, A. Tetrel bonding interactions at work: Impact on tin and lead coordination compounds. Coord. Chem. Rev. 2019, 384, 107–125. [Google Scholar] [CrossRef]
- Davidovich, R.L.; Stavila, V.; Marinin, D.V.; Voit, E.I.; Whitmire, K.H. Stereochemistry of lead(II) complexes with oxygen donor ligands. Coord. Chem. Rev. 2009, 253, 1316–1352. [Google Scholar] [CrossRef]
- Mahmoudi, G.; Bauzá, A.; Frontera, A. Concurrent agostic and tetrel bonding interactions in lead(ii) complexes with an isonicotinohydrazide based ligand and several anions. Dalton Trans. 2016, 45, 4965–4969. [Google Scholar] [CrossRef] [PubMed]
- Mahmoudi, G.; Dey, L.; Chowdhury, H.; Bauzá, A.; Ghosh, B.K.; Kirillov, A.M.; Seth, S.K.; Gurbanov, A.V. Synthesis and crystal structures of three new lead(II) isonicotinoylhydrazone derivatives: Anion controlled nuclearity and dimensionality. Inorg. Chim. Acta 2017, 461, 192–205. [Google Scholar] [CrossRef]
- Mahmoudi, G.; Bauzá, A.; Frontera, A.; Garczarek, P.; Stilinović, V.; Kirillov, A.M.; Kennedy, A.; Ruiz-Pérez, C. Metal-organic and supramolecular lead(ii) networks assembled from isomeric nicotinoylhydrazone blocks: the effects of ligand geometry and counter-ion on topology and supramolecular assembly. CrystEngComm 2016, 18, 5375–5385. [Google Scholar] [CrossRef]
- Mahmoudi, G.; Gurbanov, A.V.; Rodríguez-Hermida, S.; Carballo, R.; Amini, M.; Bacchi, A.; Mitoraj, M.P.; Sagan, F.; Kukułka, M.; Safin, D.A. Ligand-Driven Coordination Sphere-Induced Engineering of Hybride Materials Constructed from PbCl2 and Bis-Pyridyl Organic Linkers for Single-Component Light-Emitting Phosphors. Inorg. Chem. 2017, 56, 9698–9709. [Google Scholar]
- Mahmoudi, G.; Zangrando, E.; Mitoraj, M.P.; Gurbanov, A.V.; Zubkov, F.I.; Moosavifar, M.; Konyaeva, I.A.; Kirillov, A.M.; Safin, D.A. Extended lead(ii) architectures engineered via tetrel bonding interactions. New J. Chem. 2018, 42, 4959–4971. [Google Scholar] [CrossRef]
- Roy, S.; Drew, M.G.B.; Bauzá, A.; Frontera, A.; Chattopadhyay, S. Non-covalent tetrel bonding interactions in hemidirectional lead(II) complexes with nickel(II)-salen type metalloligands. New J. Chem. 2018, 42, 6062–6076. [Google Scholar] [CrossRef]
- Servati, G.M.; Stilinović, V.; Bauzá, A.; Frontera, A.; McArdle, P.; Van Derveer, D.; Ng, S.W.; Mahmoudi, G. Design of Lead(II) Metal-Organic Frameworks Based on Covalent and Tetrel Bonding. Chem. Eur. J. 2015, 21, 17951–17958. [Google Scholar] [CrossRef]
- Khandar, A.A.; Ghosh, B.K.; Lampropoulos, C.; Servati Gargari, M.; Yilmaz, V.T.; Bhar, K.; Hosseini-Yazdi, S.A.; Cain, J.M.; Mahmoudi, G. Coordination complexes and polymers from the initial application of phenyl-2-pyridyl ketone azine in mercury chemistry. Polyhedron 2015, 85, 467. [Google Scholar] [CrossRef]
- Bruker. SAINT, version 6.36a. Bruker-AXS Inc.: Madison, WI, USA, 2002. [Google Scholar]
- Bruker. SMART, Version 5.625 and SADABS, Version 2.03a. Bruker AXS Inc.: Madison, WI, USA, 2001. [Google Scholar]
- Sheldrick, G.M. A short history of SHELX. Acta Cryst. 2008, A64, 112–122. [Google Scholar] [CrossRef]
- Sheldrick, G.M. Crystal structure refinement with SHELXL. Acta Cryst. 2015, C71, 3–8. [Google Scholar]
- Farrugia, L.J. WinGX suit for small-molecule single-crystal crystallography. J. Appl. Crystallogr. 1999, 32, 837–838. [Google Scholar] [CrossRef]
- Spek, A.L. Single-crystal structure validation with the program PLATON. J. Appl. Crystallogr. 2003, 36, 7–13. [Google Scholar] [CrossRef]
- Spackman, M.A.; McKinnon, J.J. Fingerprinting intermolecular interactions in molecular crystals. CrystEngComm 2002, 4, 378–392. [Google Scholar] [CrossRef]
- Seth, S.K.; Saha, I.; Estarellas, C.; Frontera, A.; Kar, T.; Mukhopadhyay, S. Supramolecular Self-Assembly of M-IDA Complexes Involving Lone-Pair⋯π Interactions: Crystal Structures, Hirshfeld Surface Analysis, and DFT Calculations [H2IDA = iminodiacetic acid, M = Cu(II), Ni(II)]. Cryst. Growth Des. 2011, 11, 3250–3265. [Google Scholar] [CrossRef]
- Mitra, M.; Manna, P.; Bauzá, A.; Ballester, P.; Seth, S.K.; Choudhury, S.R.; Frontera, A.; Mukhopadhyay, S. 3-Picoline Mediated Self-Assembly of M(II)‒Malonate Complexes (M = Ni/Co/Mn/Mg/Zn/Cu) Assisted by VariousWeak Forces Involving Lone Pair⋯π, π‒π, and Anion⋯π‒Hole Interactions. J. Phys. Chem. B 2014, 118, 14713–14726. [Google Scholar] [CrossRef] [PubMed]
- McKinnon, J.J.; Spackman, M.A.; Mitchell, A.S. Novel tools for visualizing and exploring intermolecular interactions in molecular crystals. Acta Crystallogr. B 2004, 60, 627–668. [Google Scholar] [CrossRef]
- Rohl, A.L.; Moret, M.; Kaminsky, W.; Claborn, K.; Mckinnon, J.J.; Kahr, B. Hirshfeld Surfaces Identify Inadequacies in Computations of Intermolecular Interactions in Crystals: Pentamorphic 1,8-Dihydroxyanthraquinone. Cryst. Growth Des. 2008, 8, 4517–4525. [Google Scholar] [CrossRef]
- Seth, S.K. Tuning the formation of MOFs by pH influence: X-ray structural variations and Hirshfeld surface analyses of 2-amino-5-nitropyridine with cadmium chloride. Acta Cryst. 2013, 15, 1772–1781. [Google Scholar] [CrossRef]
- Seth, S.K.; Bauzá, A.; Frontera, A. Screening polymorphism in a Ni(II) metal–organic framework: experimental observations, Hirshfeld surface analyses and DFT studies. CrystEngComm 2018, 20, 746–754. [Google Scholar] [CrossRef]
- Seth, S.K. Structural characterization and Hirshfeld surface analysis of a CoII complex with imidazo[1,2-a]-pyridine. Acta Cryst. 2018, E74, 600–606. [Google Scholar] [CrossRef]
- Maity, T.; Mandal, H.; Bauza, A.; Samanta, B.C.; Frontera, A.; Seth, S.K. Quantifying conventional C–H⋯π(aryl) and unconventional C–H⋯π(chelate) interactions in dinuclear Cu(II) complexes: experimental observations, Hirshfeld surface and theoretical DFT study. New J. Chem. 2018, 42, 10202–10213. [Google Scholar] [CrossRef]
- Wolff, S.K.; Grimwood, D.J.; McKinnon, J.J.; Jayatilaka, D.; Spackman, M.A. CrystalExplorer 3.1; University of Western Australia: Perth, Australia, 2007. [Google Scholar]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Grimme, S. Semiempirical GGA-type density functional constructed with a long-range dispersion correction. J. Comp. Chem. 2006, 27, 1787–1799. [Google Scholar] [CrossRef]
- McLean, A.D.; Chandler, G.S. Contracted Gaussian-basis sets for molecular calculations. I. Second row atoms, Z=11-18. J. Chem. Phys. 1980, 72, 5639–5648. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Revision C.01; Gaussian, Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
- Bergner, A.; Dolg, M.; Küchle, W.; Stoll, H.; Preuss, H. Ab initio energy adjusted pseudopotentials for elements of groups 13–17. Mol. Phys. 1993, 80, 1431. [Google Scholar]
- Das, A.; Choudhury, S.R.; Estarellas, C.; Dey, B.; Frontera, A.; Hemming, J.; Helliwell, M.; Gamez, P.; Mukhopadhyay, S. Supramolecular assemblies involving anion–π and lone pair–π interactions: experimental observation and theoretical analysis. CrystEngComm 2011, 13, 4519–4527. [Google Scholar] [CrossRef]
- Manna, P.; Seth, S.K.; Bauzá, A.; Mitra, M.; Choudhury, S.R.; Frontera, A.; Mukhopadhyay, S. pH Dependent Formation of Unprecedented Water‒Bromide Cluster in the Bromide Salts of PTP Assisted by Anion⋯π Interactions: Synthesis, Structure, and DFT Study. Cryst. Growth Des. 2014, 14, 747–755. [Google Scholar] [CrossRef]
- Manna, P.; Seth, S.K.; Mitra, M.; Choudhury, S.R.; Bauzá, A.; Frontera, A.; Mukhopadhyay, S. Experimental and Computational Study of Counterintuitive ClO4‒⋯ClO4‒ Interactions and the Interplay between π+⋯π and Anion⋯π+ Interactions. Cryst. Growth Des. 2014, 14, 5812–5821. [Google Scholar] [CrossRef]
- Seth, S.K.; Sarkar, D.; Kar, T. Use of π–π forces to steer the assembly of chromone derivatives into hydrogen bonded supramolecular layers: crystal structures and Hirshfeld surface analyses. CrystEngComm 2011, 13, 4528–4535. [Google Scholar] [CrossRef]
- Manna, P.; Seth, S.K.; Mitra, M.; Das, A.; Singh, N.J.; Choudhury, S.R.; Kar, T.; Mukhopadhyay, S. A successive layer-by-layer assembly of supramolecular frameworks driven by a novel type of face-to-face π+–π+ interactions. CrystEngComm 2013, 15, 7879–7886. [Google Scholar] [CrossRef]
- Saha, U.; Dutta, D.; Nath, H.; Franconetti, A.; Frontera, A.; Bhattacharyya, M.K. Supramolecular association in Cu (II) coordination complexes involving energetically significant NO⋯ NO π–hole interaction and cooperative π-stacked ternary assembly. Inorg. Chim. Acta 2019, 488, 159–169. [Google Scholar] [CrossRef]
- Galmes, B.; Martinez, D.; Infante-Carrió, M.F.; Franconetti, A.; Frontera, A. Theoretical Ab Initio Study on Cooperativity Effects between Nitro π-hole and Halogen Bonding Interactions. ChemPhysChem 2019, 20, 1135–1144. [Google Scholar] [CrossRef] [PubMed]
- Dutta, D.; Nath, H.; Frontera, A.; Bhattacharyya, M.K. A novel oxalato bridged supramolecular ternary complex of Cu (II) involving energetically significant π-hole interaction: Experimental and theoretical studies. Inorg. Chim. Acta 2019, 487, 354–361. [Google Scholar] [CrossRef]
- Dutta, B.; Pratik, S.M.; Jana, S.; Sinha, C.; Datta, A.; Mir, M.M. Novel Br⋅⋅⋅π(Chelate) Interaction in a 1D Coordination Polymer Revealing Aromaticity. ChemistrySelect 2018, 3, 4289–4291. [Google Scholar] [CrossRef]



| Structure | (1) | (2) | (3) |
|---|---|---|---|
| Empirical formula | C48H40Cl2N18O18Pb2 | C36H28I2N8O2Pb | C52H41Cl2N12O13Pb |
| Formula Weight | 1642.26 | 1065.65 | 1320.06 |
| Temperature (K) | 296(2) | 296(2) | 293(2) |
| Wavelength (Å) | 0.71073 | 0.71073 | 0.71073 |
| Crystal system | Monoclinic | Triclinic | Monoclinic |
| space group | P 21/c | P‒1 | C2/c |
| a, b, c (Å) | 10.5189(8), 20.6580(2), 13.455(1) | 8.8711(1), 10.4600(2), 10.5452(2) | 16.2884(9), 15.4978(9) 21.409(2) |
| α, β, γ (°) | 90, 92.218(1), 90 | 79.134(1), 77.219(1), 74.3900(10) | 90, 100.016(6), 90 |
| Volume (Å3) | 2921.6(3) | 910.36(3) | 5321.9(6) |
| Z/Density (calc.) (Mg/m3) | 2/1.867 | 1/1.944 | 4/1.648 |
| Absorption coefficient (mm−1) | 5.933 | 6.373 | 3.347 |
| F(000) | 1592 | 504 | 2628 |
| Crystal size (mm3) | 0.26 × 0.20 × 0.17 | 0.20 × 0.14 × 0.02 | 0.23 × 0.11 × 0.07 |
| θ range for data collection | 1.807 to 26.998 | 2.00 to 26.33 | 3.866 to 26.999 |
| Limiting indices | −13 ≤ h ≤ 13 −26 ≤ k ≤ 26 17 ≤ l ≤ 17 | −11 ≤ h ≤ 11, −13 ≤ k ≤ 13, −13 ≤ l ≤ 13 | −20 ≤ h ≤ 20 −19 ≤ k ≤ 19 −27 ≤ l ≤ 27 |
| Reflections collected/unique | 36943/6377 | 13426/3639 | 19102/4472 |
| Completeness to θ (%) | 100.0 | 98.3 | 99.5 |
| Absorption correction | Semi-empirical from equivalents | Semi-empirical from equivalents | Semi-empirical from equivalents |
| Max. and min. transmission | 0.382 and 0.265 | 0.890 and 0.352 | 1.000 and 0.785 |
| Refinement method | Full-matrix least-squares on F2 | Full-matrix least-squares on F2 | Full-matrix least-squares on F2 |
| Data/parameters | 6377/393 | 3639/223 | 5776/363 |
| Goodness-of - fit on F2 | 1.034 | 1.021 | 0.968 |
| Final R indices [I > 2σ(I)] | R1 = 0.0343, wR2 = 0.0763 | R1 = 0.0288, wR2 = 0.0506 | R1 = 0.0360, wR2 = 0.0821 |
| R indices (all data) | R1 = 0.0542, wR2 = 0.0836 | R1 = 0.0403, wR2 = 0.0540 | R1 = 0.0518, wR2 = 0.0847 |
| Largest diff. peak and hole (e.Å−3) | 0.986 and −0.702 | 0.570 and −0.779 | 1.188 and −0.685 |
| Compound (1) | |||
| Pb-N(1) | 2.614(4) | Pb-N(2A) | 2.723(3) |
| Pb-N(2) | 2.646(4) | Pb-N(1A) | 2.744(4) |
| Pb-O(1) | 2.775(2) | Pb-O(3) | 2.844(2) |
| Pb-O(11) | 2.648(3) | Pb-N(4A) | 2.816(2) |
| N(1)-Pb-N(2) | 62.08(13) | O(11)-Pb-N(2A) | 57.92(11) |
| N(1)-Pb-O(11) | 79.64(13) | N(1)-Pb-N(1A) | 84.10(13) |
| N(2)-Pb-O(11) | 138.55(13) | N(2)-Pb-N(1A) | 76.60(12) |
| N(1)-Pb-N(2A) | 82.70(12) | O(11)-Pb-N(1A) | 116.63(12) |
| N(2)-Pb-N(2A) | 126.17(12) | N(2A)-Pb-N(1A) | 59.47(12) |
| Compound (2) | |||
| Pb(1)-O(1) | 2.710(3) | Pb(1)-N(1) | 2.833(2) |
| Pb(1)-I(1) | 3.188(3) | Pb(1)-N(3) | 2.917(2) |
| O(1)-Pb(1)-O(1) * | 180.00(8) | O(1)-Pb(1)-N(1) | 57.84(7) |
| O(1)-Pb(1)-I(1) | 86.61(7) | N(1)-Pb(1)-I(1) | 99.97(8) |
| O(1)-Pb(1)-I(1) * | 93.39(7) | N(I)-Pb(1)-I(1) * | 80.03(11) |
| I(1)-Pb(1)-I(1) * | 180.00(7) | N(1)-Pb(1)-N(1) * | 180.00(12) |
| Compound (3) | |||
| Pb(1)-O(1) | 2.581(3) | Pb(1)-N(4) | 2.701(3) |
| Pb(1)-N(3) | 2.675(3) | Pb(1)-O(7) | 2.737(7) |
| O(1)-Pb(1)-N(3) | 60.39(9) | O(1)-Pb(1)-O(7) | 142.78(7) |
| O(1)-Pb(1)-N(4) | 115.35(9) | N(3)-Pb(1)-O(7) | 117.30(7) |
| N(3)-Pb(1)-N(4) | 57.86(9) | N(4)-Pb(1)-O(7) | 84.17(6) |
| D–H⋯A | D–H | H⋯A | D⋯A | D–H⋯A | Symmetry Operation |
|---|---|---|---|---|---|
| Compound (1) | |||||
| N3A–H3B⋯O3 | 0.90 | 2.10 | 2.975(6) | 164 | −x, −y, 1 − z |
| N3A–H3B⋯O4 | 0.90 | 2.58 | 3.309(7) | 139 | −x, −y, 1 − z |
| N3–H3N⋯O2 | 0.90 | 2.15 | 2.970(6) | 151 | x, 1/2 − y, −1/2 + z |
| C3–H3⋯O8 | 0.93 | 2.29 | 3.068(10) | 141 | x, y, −1 + z |
| C4–H4⋯O6 | 0.93 | 2.50 | 3.299(12) | 144 | 1 − x, −y, 1 − z |
| C5–H5⋯O11 | 0.93 | 2.58 | 3.239(8) | 128 | - |
| C6–H6⋯O2 | 0.93 | 2.53 | 3.178(6) | 127 | x, 1/2 − y, −1/2 + z |
| C9–H9⋯O6 | 0.93 | 2.51 | 3.247(10) | 136 | x, 1/2 − y, −1/2 + z |
| C9A–H9A⋯O3 | 0.93 | 2.41 | 3.317(7) | 165 | −x, −y, 1 − z |
| C11A–H11A⋯O8 | 0.93 | 2.51 | 3.300(11) | 143 | 1 − x, −1/2 + y, 3/2 − z |
| C12A–H12A⋯O11 | 0.93 | 2.33 | 3.072(6) | 136 | 1 − x, −y, 1 − z |
| Compound (2) | |||||
| N2–H2⋯N4 | 0.90 | 2.24 | 2.655(5) | 108 | - |
| C13–H13⋯O1 | 0.93 | 2.37 | 2.982(6) | 123 | −x, 2 − y, 1 − z |
| Compound (3) | |||||
| N2–H1N⋯N1 | 0.86 | 2.33 | 2.673(4) | 104 | - |
| O7–H1W⋯O5 | 0.82 | 2.41 | 3.214(6) | 169 | - |
| N5–H2N⋯N6 | 0.86 | 2.25 | 2.625(5) | 107 | - |
| C25–H25⋯O6 | 0.93 | 2.57 | 3.424(6) | 153 | x, 1 − y, 1/2 + z |
| Y–X⋯Cg(I) | X⋯Cg | Y⋯Cg | Y–X⋯Cg | X-Perp | Symmetry Operation |
|---|---|---|---|---|---|
| C (11)–O(5)⋯Cg(4) | 3.498(6) | 4.148(3) | 108 | 3.485 | 1 − x, −y, 1 − z |
| rings (i)⋯(j) | Rc | R1v | R2v | α | β | γ | Symmetry |
|---|---|---|---|---|---|---|---|
| Compound (1) | |||||||
| Cg(1)⋯Cg(3) | 3.734(3) | 3.445(2) | 3.530(3) | 3.80 | 19.00 | 22.69 | x, 1/2 − y, −1/2+z |
| Compound (2) | |||||||
| Cg(1)⋯Cg(2) | 3.783(3) | 3.666(2) | 3.773(2) | 15.80 | 4.09 | 14.27 | −1 + x, y, z |
| Cg(2)⋯Cg(2) | 3.771(3) | 3.391(2) | 3.391(2) | 0.00 | 25.95 | 25.95 | 1 − x, 1 − y, 1 − z |
| Compound (3) | |||||||
| Cg(1)⋯Cg(1) | 4.113(2) | 3.279(2) | 3.279(2) | 0.0 | 37.12 | 37.12 | 2 − x, −y, −z |
| Cg(2)⋯Cg(2) | 4.218(2) | 3.407(2) | 3.407(2) | 0.0 | 36.14 | 36.14 | 5/2 − x, 1/2 − y, 1 − z |
| Cg(2)⋯Cg(3) | 4.105(3) | 2.846(2) | 3.949(2) | 31.8 | 15.86 | 46.11 | 5/2 − x, 1/2 + y, 1/2 − z |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mahmoudi, G.; Seth, S.K.; Zubkov, F.I.; López-Torres, E.; Bacchi, A.; Stilinović, V.; Frontera, A. Supramolecular Assemblies in Pb(II) Complexes with Hydrazido-Based Ligands. Crystals 2019, 9, 323. https://doi.org/10.3390/cryst9060323
Mahmoudi G, Seth SK, Zubkov FI, López-Torres E, Bacchi A, Stilinović V, Frontera A. Supramolecular Assemblies in Pb(II) Complexes with Hydrazido-Based Ligands. Crystals. 2019; 9(6):323. https://doi.org/10.3390/cryst9060323
Chicago/Turabian StyleMahmoudi, Ghodrat, Saikat Kumar Seth, Fedor I. Zubkov, Elena López-Torres, Alessia Bacchi, Vladimir Stilinović, and Antonio Frontera. 2019. "Supramolecular Assemblies in Pb(II) Complexes with Hydrazido-Based Ligands" Crystals 9, no. 6: 323. https://doi.org/10.3390/cryst9060323
APA StyleMahmoudi, G., Seth, S. K., Zubkov, F. I., López-Torres, E., Bacchi, A., Stilinović, V., & Frontera, A. (2019). Supramolecular Assemblies in Pb(II) Complexes with Hydrazido-Based Ligands. Crystals, 9(6), 323. https://doi.org/10.3390/cryst9060323