Growth of Calcite in Confinement
Abstract
:1. Introduction
2. Experimental Section
2.1. Microfluidic Growth Control
- nucleate calcium carbonate crystals in a limited area that permits imaging access;
- remove other polymorphs than calcite (polymorphs are determined by the crystal shape);
- control stable saturation conditions at the growing crystal surface;
- permit slow growth of rhombohedral crystals from the nuclei;
- avoid clogging of the microfluidic device due to crystal growth elsewhere in the device.
2.2. Topography Measurements
3. Results
3.1. Interpretation and Quantification of In Situ Image Data
3.2. Summary of Growth Rates
4. Discussion
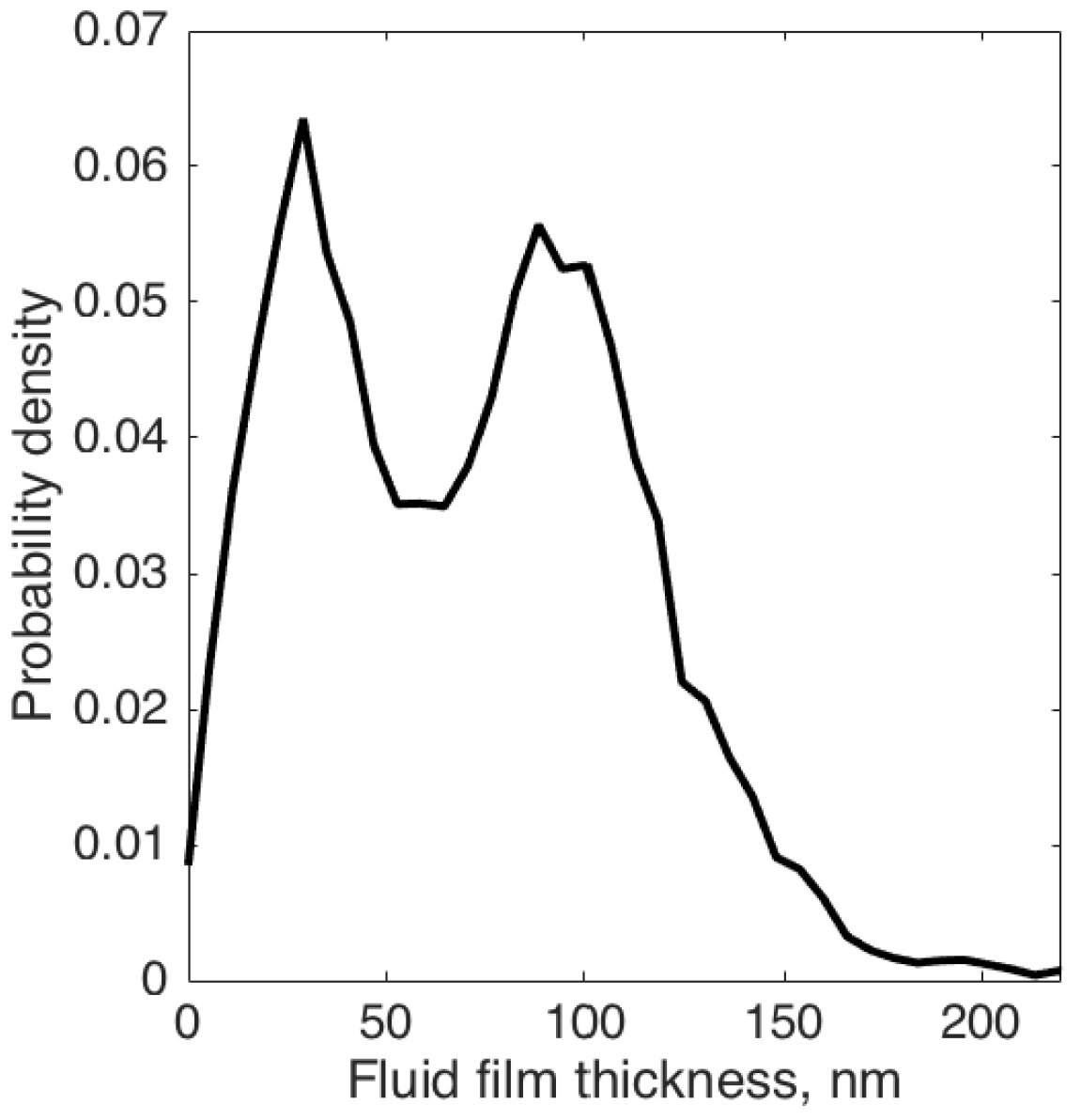
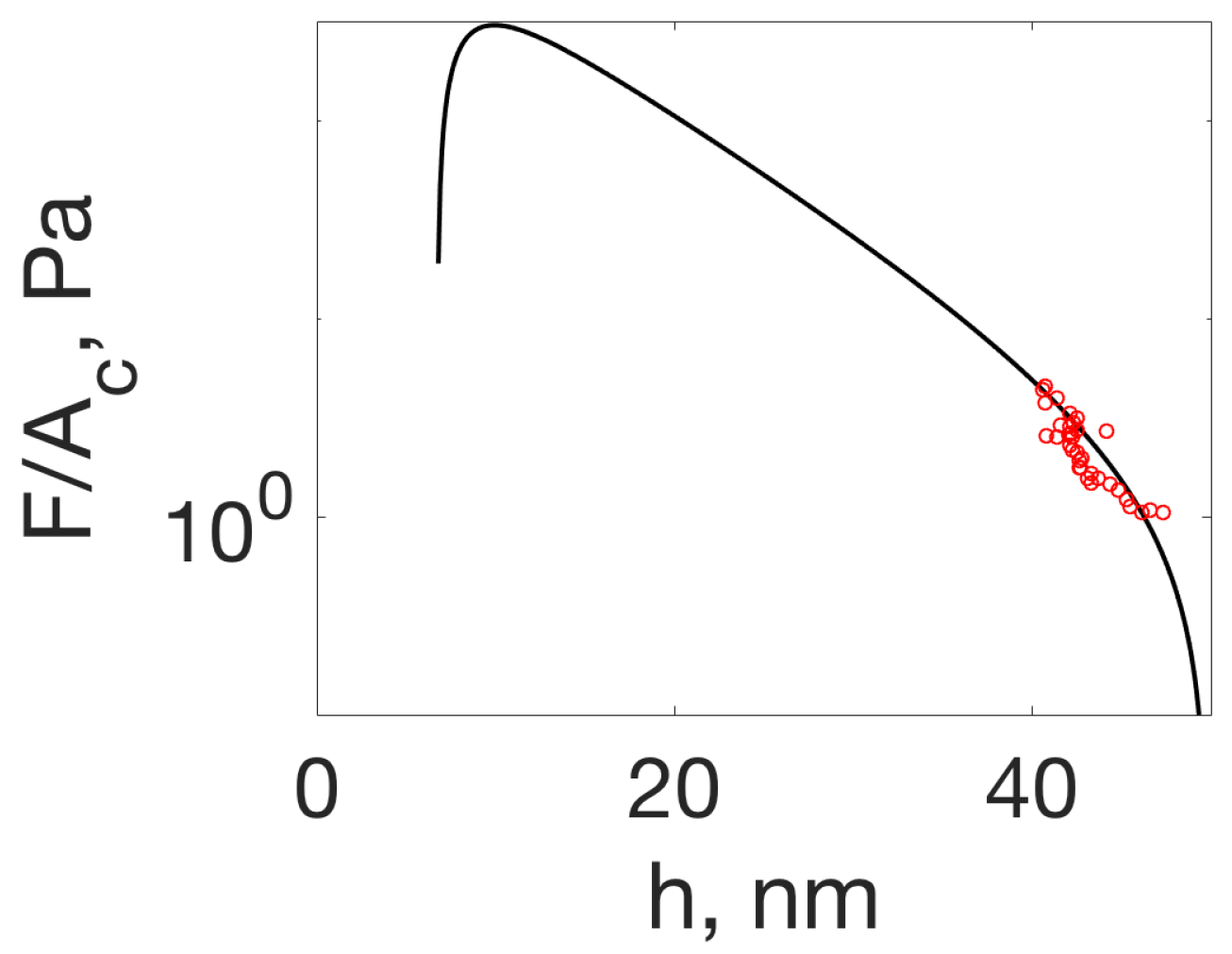
4.1. Disjoining Pressure: The Hovering Crystal
4.2. Smooth and Rough Contacts
- Dislocations? In some cases, there seems to be growth resembling (atomic) step spirals emanating from larger steps on the surface. However, the fact that the macroscopic steps on the growth rim move around does not match with a screw dislocation normal to the surface.
- Local poisoning of growth by “dirt”, asperities on the glass surface or organic molecules from the PDMS? We have no evidence that the solutions or the PDMS are different in the experiments of smooth vs. rough growth rims. Sometimes, we have observed smooth and rough growth rims on crystals adjacent to each other in the same experiment like Crystals E and F (see the images in the Supplementary Materials). If a surface active contamination adheres to the growing surface, it is likely to be incorporated in the crystal when it continues growing. Then, there would have to be a continuous addition of contamination for rough crystals to keep them rough and no contamination for smooth crystals. If the “contamination” adheres to the glass surface, it would be a constant source of “disturbance” at a fix point in space.
- An inherent transport–growth instability induced by nanoconfinement? The fact that similar roughness is found on growth rims of otherwise perfectly faceted crystals of sodium chlorate [11], potassium alum [16] and calcite (this study) suggests that this is a general nonlinearity/transport–growth feedback mechanism in confinement that arises from random perturbations, be they local contamination, roughness of the support or something else. We have yet to pinpoint the nature of the feedback mechanism and formulate a mathematical model for it.
4.3. Rim Widths of Smooth Rims
4.4. Rim Widths of Rough Rims
5. Conclusions
5.1. Summary of Main Results
5.2. Outlook
Supplementary Materials
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| RICM | Reflection interference contrast microscopy |
| PDMS | Polydimethylsiloxane |
| PGMEA | Propylene glycol methyl ether acetate |
| LED | Light-emitting diode |
References and Notes
- Wilkinson, B.H. Biomineralization, paleoceanography, and the evolution of calcareous marine organisms. Geology 1979, 7, 524–527. [Google Scholar] [CrossRef]
- Stephens, C.J.; Ladden, S.F.; Meldrum, F.C.; Christenson, H.K. Amorphous Calcium Carbonate is Stabilized in Confinement. Adv. Funct. Mater. 2010, 20, 2108–2115. [Google Scholar] [CrossRef]
- Gratier, J.-P.; Dysthe, D.K.; Renard, F. The role of pressure solution creep in the ductility of the Earth’s upper crust. Adv. Geophys. 2013, 54, 47–179. [Google Scholar]
- Flatt, R.J.; Caruso, F.; Sanchez, A.M.A.; Scherer, G.W. Chemo-mechanics of salt damage in stone. Nat. Commun. 2014, 5, 4823. [Google Scholar] [CrossRef] [PubMed]
- Chang, C.F.; Chen, J.W. The experimental investigation of concrete carbonation depth. Cem. Concr. Res. 2006, 36, 1760–1767. [Google Scholar] [CrossRef]
- Rothrock, E.P. On the force of crystallization of calcite. J. Geol. 1925, 33, 80–83. [Google Scholar] [CrossRef]
- Gratier, J.P.; Frery, E.; Deschamps, P.; Røyne, A.; Renard, F.; Dysthe, D.; Ellouz-Zimmerman, N.; Hamelin, B. How travertine veins grow from top to bottom and lift the rocks above them: The effect of crystallization force. Geology 2012, 40, 1015–1018. [Google Scholar] [CrossRef]
- Gibbs, J.W. Scientific Papers of J. Willard Gibbs, Volume 1: Thermodynamics; Longmans, Green and co.: Harlow, UK, 1906. [Google Scholar]
- Correns, C.W. Growth and dissolution of crystals under linear pressure. Discuss. Faraday Soc. 1949, 5, 267–271. [Google Scholar] [CrossRef]
- Weyl, P.K. Pressure solution and the force of crystallization: A phenomenological theory. J. Geophys. Res. 1959, 64, 2001–2025. [Google Scholar] [CrossRef]
- Røyne, A.; Dysthe, D.K. Rim formation on crystal faces growing in confinement. J. Cryst. Growth 2012, 346, 89–100. [Google Scholar] [CrossRef]
- Espinosa-Marzal, R.M.; Scherer, G.W. Advances in understanding damage by salt crystallization. Acc. Chem. Res. 2010, 43, 897–905. [Google Scholar] [CrossRef] [PubMed]
- Noiriel, C.; Renard, F.; Doan, M.L.; Gratier, J.P. Intense fracturing and fracture sealing induced by mineral growth in porous rocks. Chem. Geol. 2010, 269, 197–209. [Google Scholar] [CrossRef]
- Wheeler, J. Dramatic effects of stress on metamorphic reactions. Geology 2014, 42, 647–650. [Google Scholar] [CrossRef]
- Schmalholz, S.M.; Podladchikov, Y. Metamorphism under stress: The problem of relating minerals to depth. Geology 2014, 42, 733–734. [Google Scholar] [CrossRef]
- Becker, G.F.; Day, A.L. The linerar force of growing crystals. Proc. Wash. Acad. Sci. 1905, 7, 283–288. [Google Scholar]
- Taber, S. The growth of crystals under external pressure. Am. J. Sci. 1916, 41, 532–556. [Google Scholar] [CrossRef]
- Flatt, R.J.; Steiger, M.; Scherer, G.W. A commented translation of the paper by C.W. Correns and W. Steinborn on crystallization pressure. Environ. Geol. 2007, 52, 221–237. [Google Scholar] [CrossRef]
- It should be noted that Correns stated that sodium chlorate did not crystallize in confinement to produce work, whereas Røyne and Dysthe [11] did measure such work, although the rates of growth were very small. It is also worth noting that all studies before Røyne and Dysthe [11] were performed using hydrated salts, while sodium chlorate, as calcite in this study, does not consist of hydrated salts.
- Israelachvili, J.N. Intermolecular and Surface Forces; Academic Press: Cambridge, MA, USA, 2011; p. 674. [Google Scholar]
- Dysthe, D.K.; Renard, F.; Porcheron, F.; Rousseau, B. Fluid in mineral interfaces—Molecular simulations of structure and diffusion. Geophys. Res. Lett. 2002, 29, 13–14. [Google Scholar] [CrossRef]
- Li, L.; Sanchez, J.R.; Kohler, F.; Røyne, A.; Dysthe, D.K. Microfluidic control of nucleation and growth of calcite. arXiv, 2017; arXiv:1708.06299. [Google Scholar]
- Ploem, J.S. Reflection-contrast microscopy as a tool for investigation of the attachment of living cells to a glass surface. In Mononuclear Phagocytes in Immunity, Infection and Pathology; Furth, R.V., Ed.; Blackwell Scientific Publications: Oxford, UK, 1975; pp. 405–421. [Google Scholar]
- The value of h depends on an estimate of the minimum intensity attainable in the contact and the maximum value recorded for the constructive interference, and we consider the accuracy to be about ±10 nm. The dependence of h on intensity is more accurate because the total intensity range has an accuracy better than 10%. This means that the height data in Figure 2 is accurate to ±10 nm, and the slope that these points form (dP/dh in Figure 2) is accurate to 10%.
- Kohler, F.; Dysthe, D.K.; Gagliardi, L.; Pierre-Louis, O. Cavity formation in confined growing crystals. Phys. Rev. Lett. 2017. under review. [Google Scholar]
- Diao, Y.; Espinosa-Marzal, R.M. Molecular insight into the nanoconfined calcite-solution interface. Proc. Natl. Acad. Sci. USA 2016, 113, 12047–12052. [Google Scholar] [CrossRef] [PubMed]
- Using the data in Dysthe et al. [21], the diffusion coefficient in a confined fluid film of thickness h is D(h) = D(h = ∞)(1 − e−h/h0), where h0 = 5.6 nm.


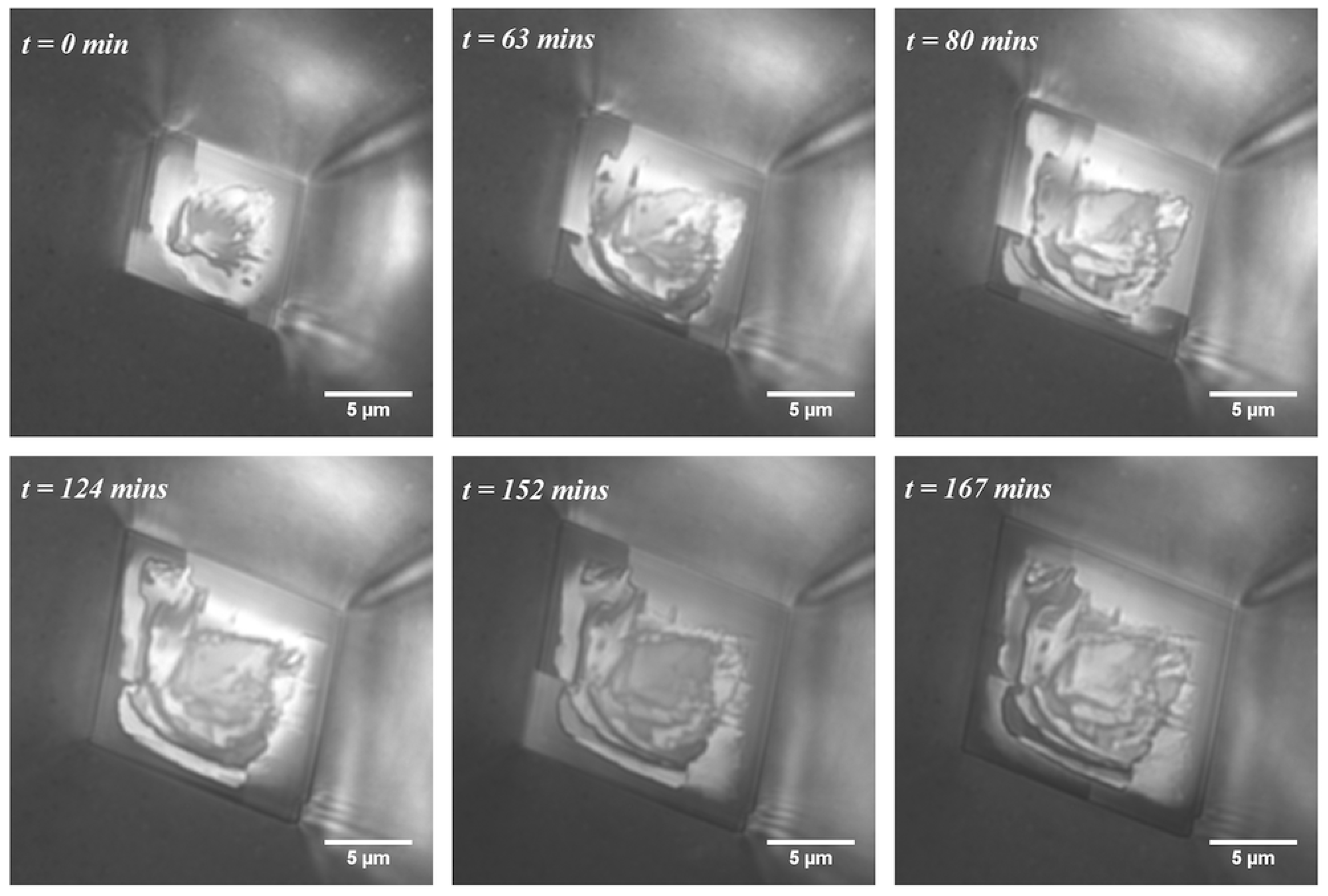
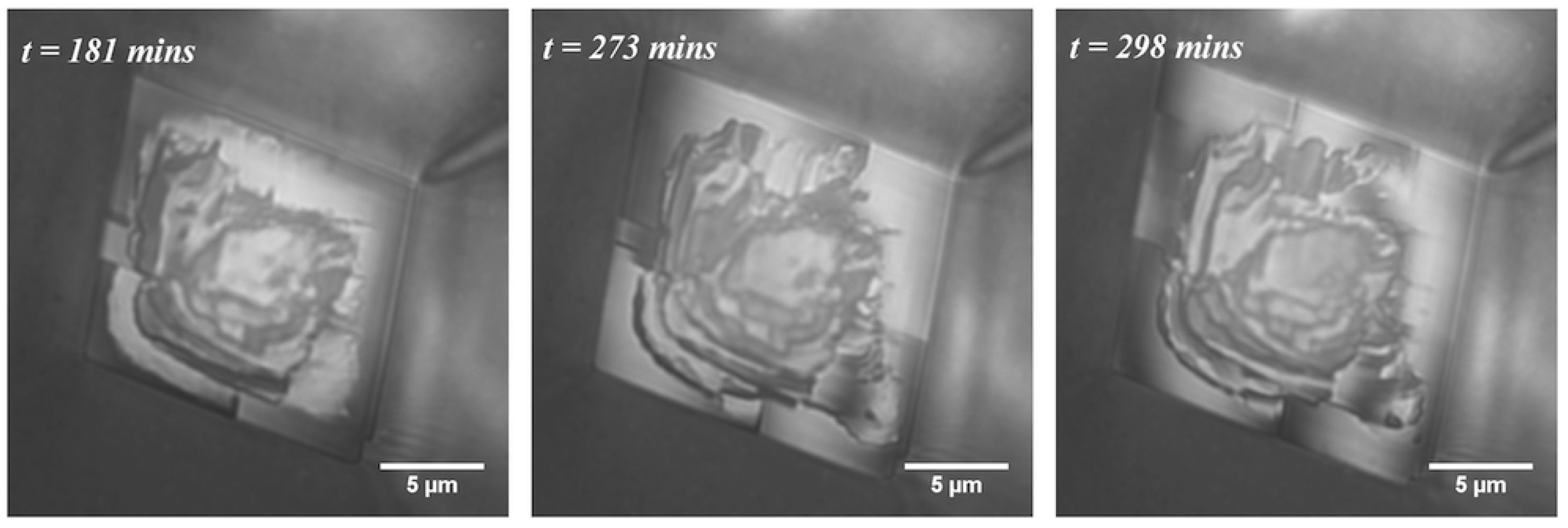
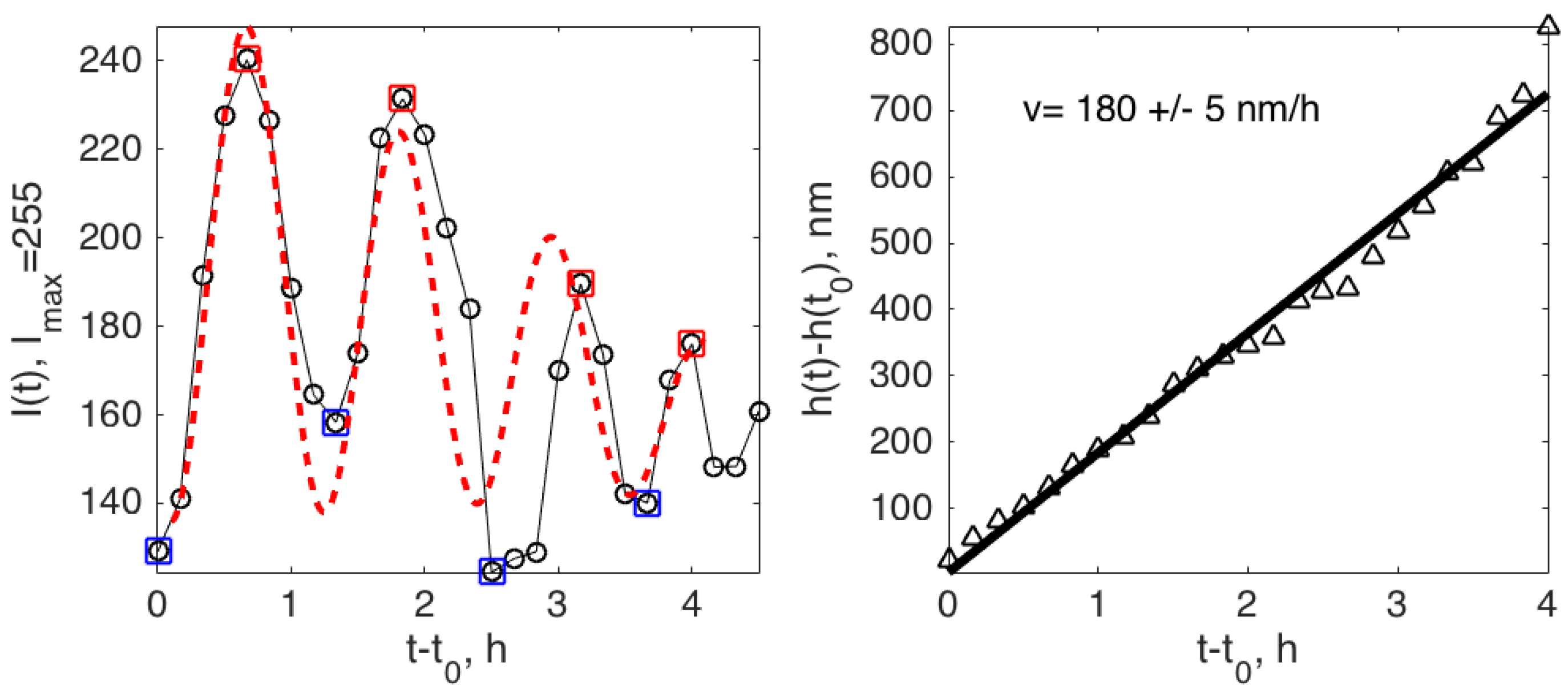
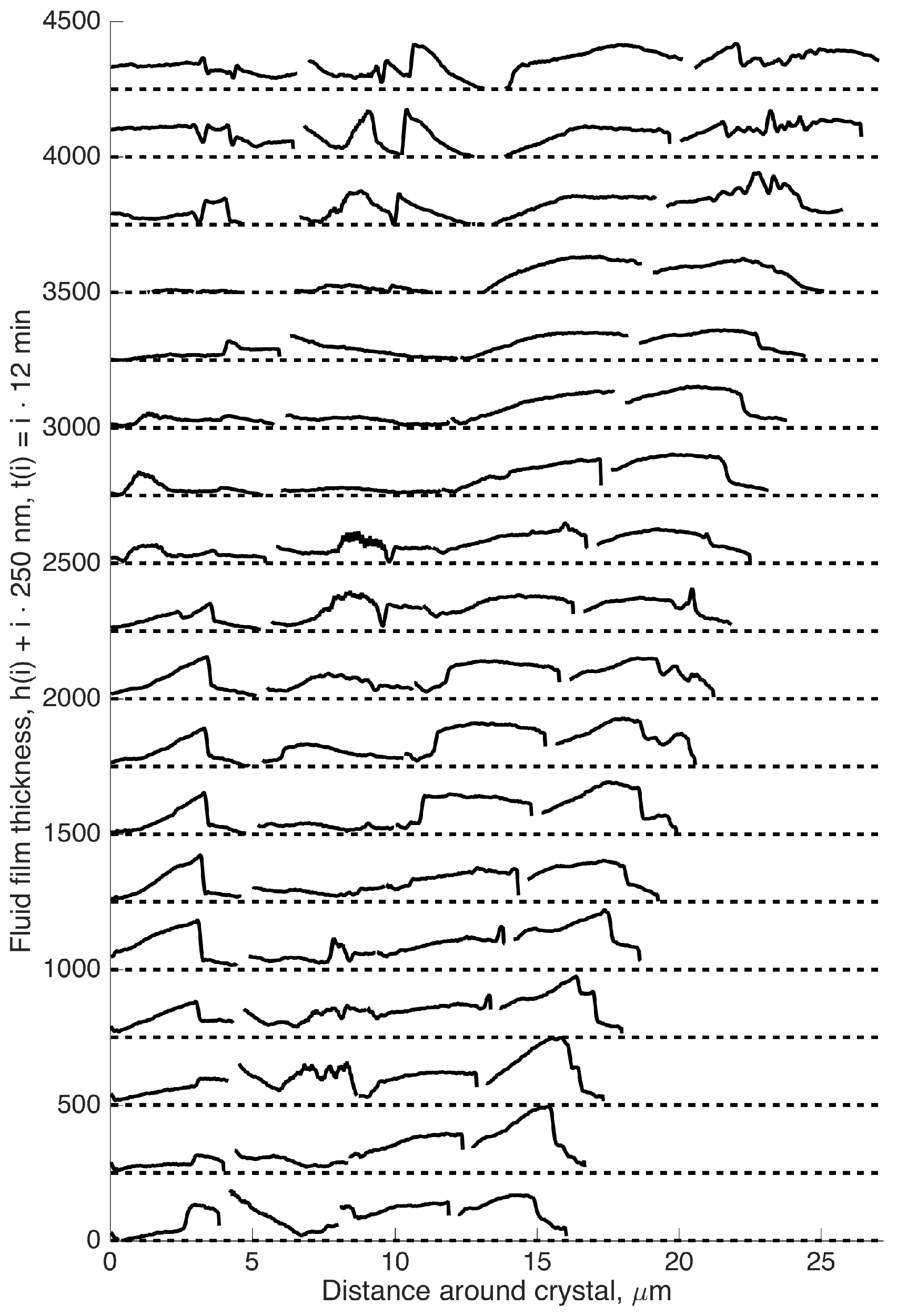

| Crystal | Concentration c mM | Supersaturation | Downward | Smooth/Intermittent | Outwards | Relative |
|---|---|---|---|---|---|---|
| Growth Rate | Growth Rate | Growth Rate | ||||
| nm/h | nm/h | % | ||||
| A | 0.8 | 0.6 | Smooth | (6→)0.4 | ||
| B | 0.7 | 0.4 | Rough | 21 | ||
| C | 0.8 | 0.6 | Smooth | 8(→)0 | ||
| D | 0.7 | 0.4 | Smooth | 5 | ||
| E | 0.8 | 0.6 | Rough | 12 | ||
| F | 0.8 | 0.6 | Smooth | (3→)0 | ||
| G | 0.7 | 0.4 | Smooth | (12→)3 | ||
| H | 0.8 | 0.6 | Rough | 6 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, L.; Kohler, F.; Røyne, A.; Dysthe, D.K. Growth of Calcite in Confinement. Crystals 2017, 7, 361. https://doi.org/10.3390/cryst7120361
Li L, Kohler F, Røyne A, Dysthe DK. Growth of Calcite in Confinement. Crystals. 2017; 7(12):361. https://doi.org/10.3390/cryst7120361
Chicago/Turabian StyleLi, Lei, Felix Kohler, Anja Røyne, and Dag Kristian Dysthe. 2017. "Growth of Calcite in Confinement" Crystals 7, no. 12: 361. https://doi.org/10.3390/cryst7120361
APA StyleLi, L., Kohler, F., Røyne, A., & Dysthe, D. K. (2017). Growth of Calcite in Confinement. Crystals, 7(12), 361. https://doi.org/10.3390/cryst7120361






