Broadband Transmission Loss Using the Overlap of Resonances in 3D Sonic Crystals
Abstract
:1. Introduction
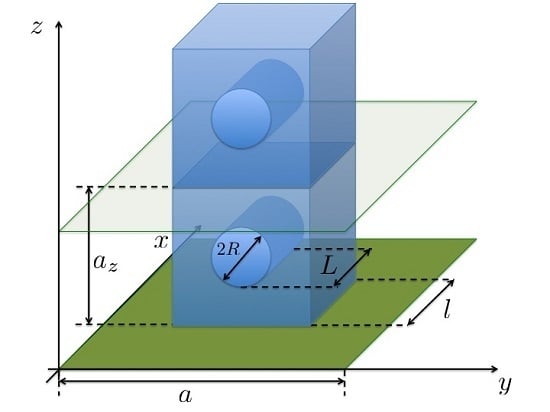
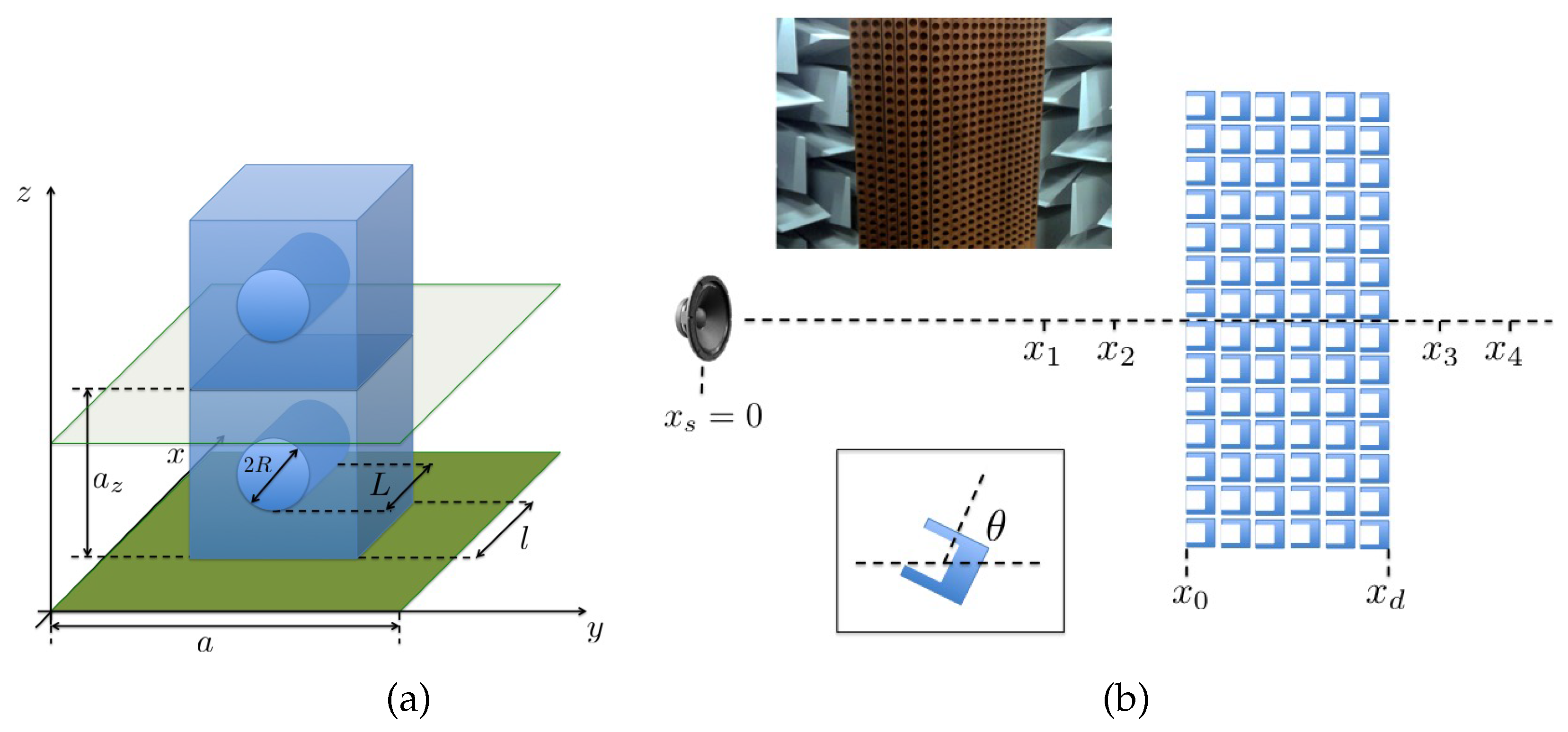
2. Experimental Set-Up
3. Numerical Characterization
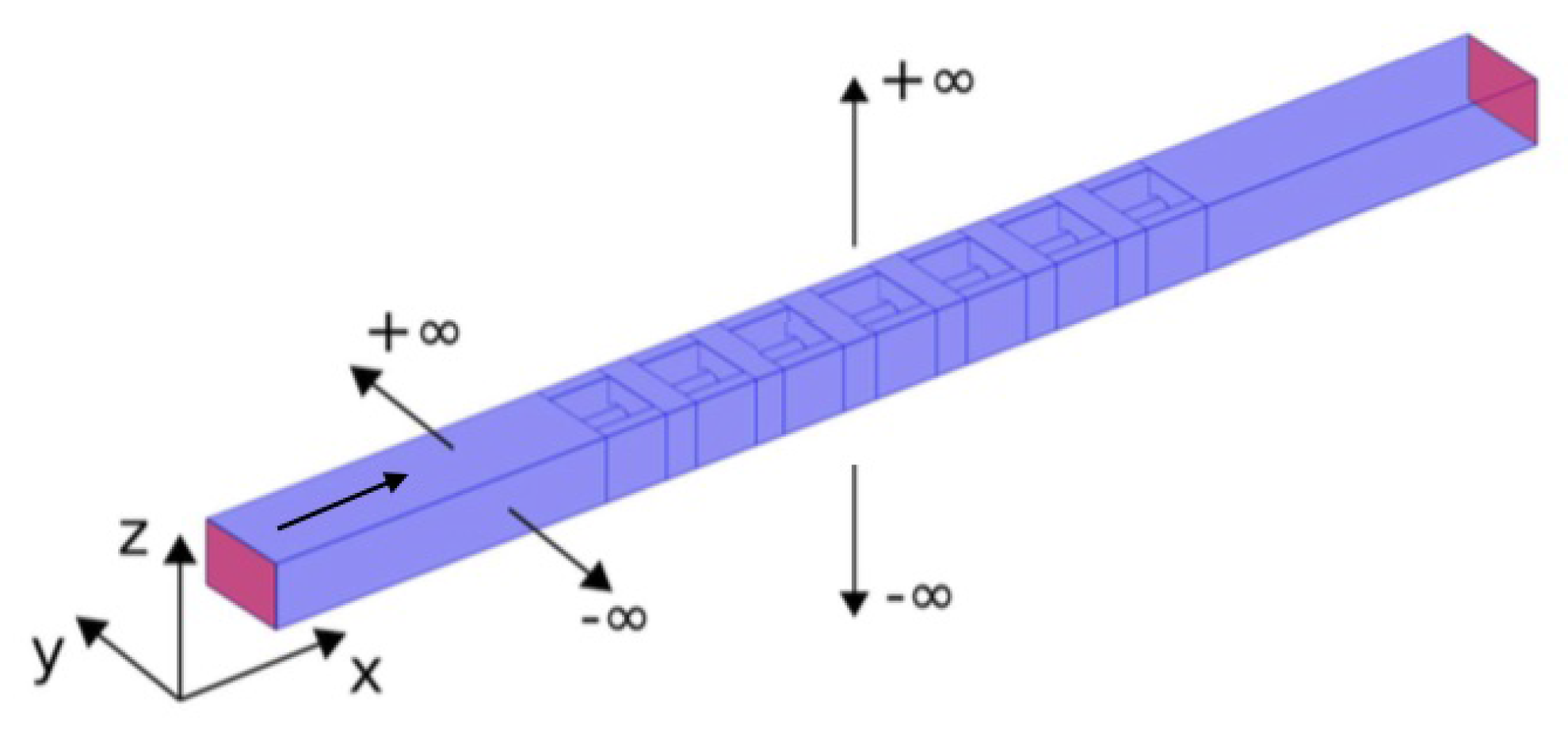
3.1. Eigenvalue Problem
3.2. Scattering Problem
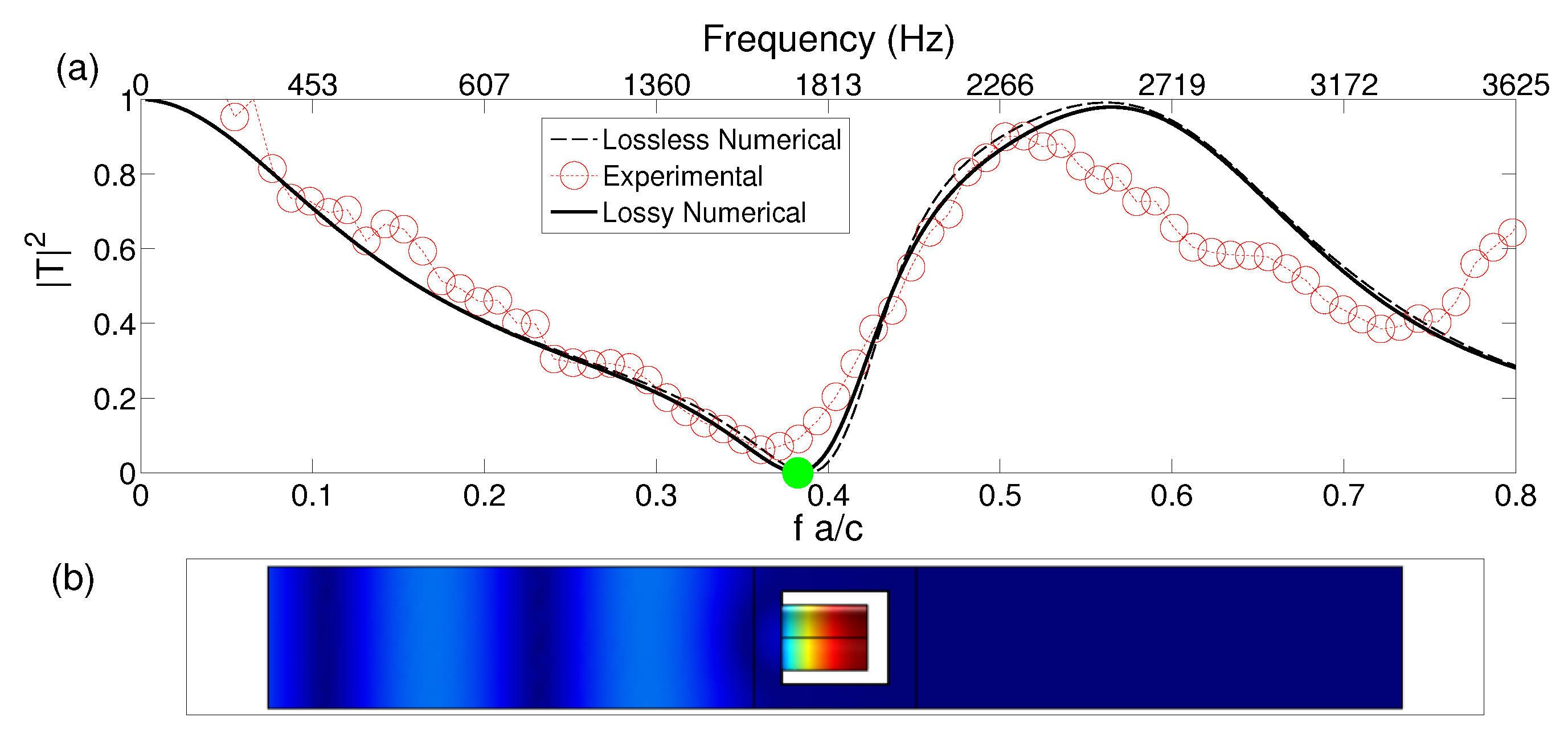
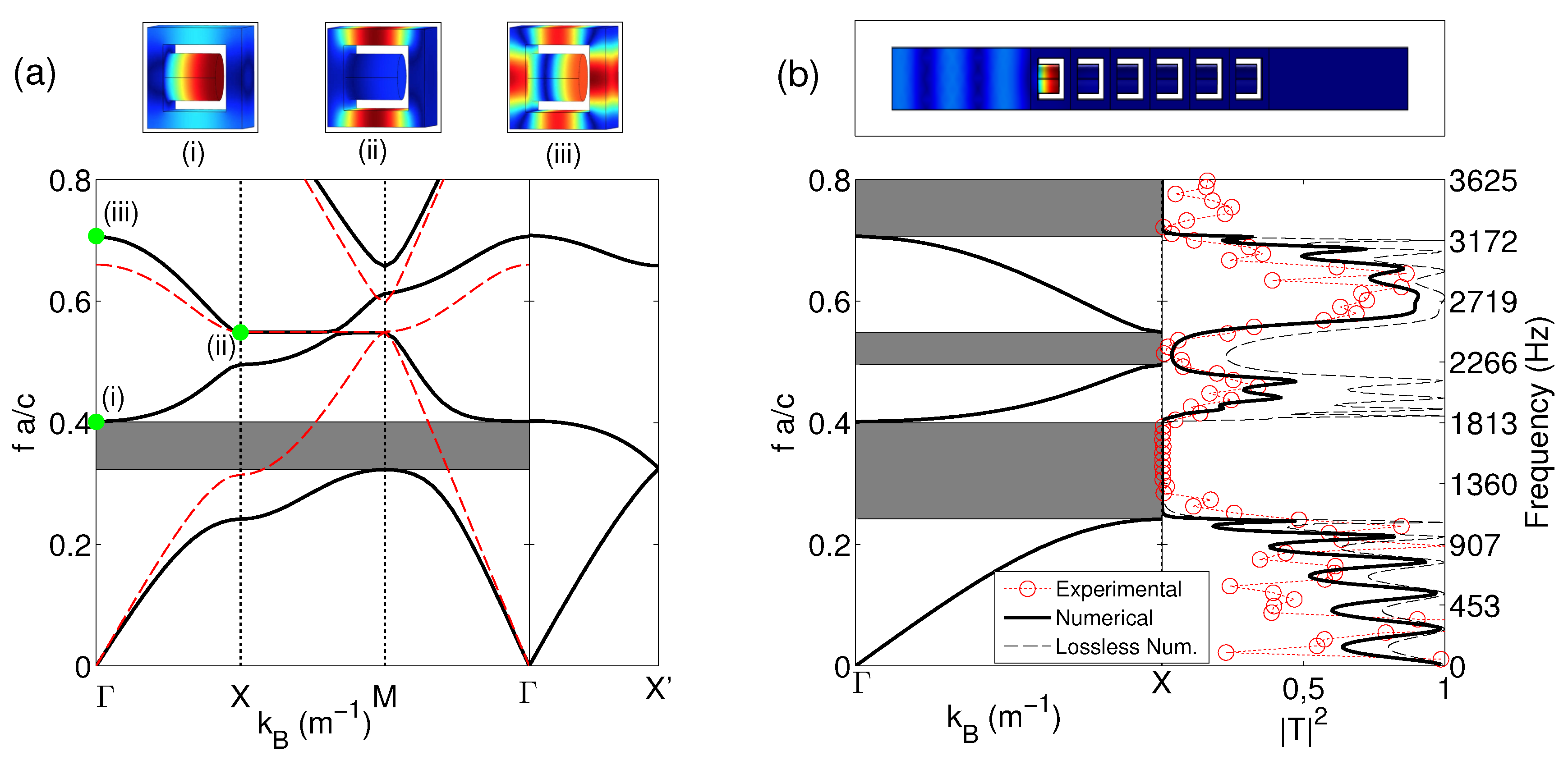
4. Results
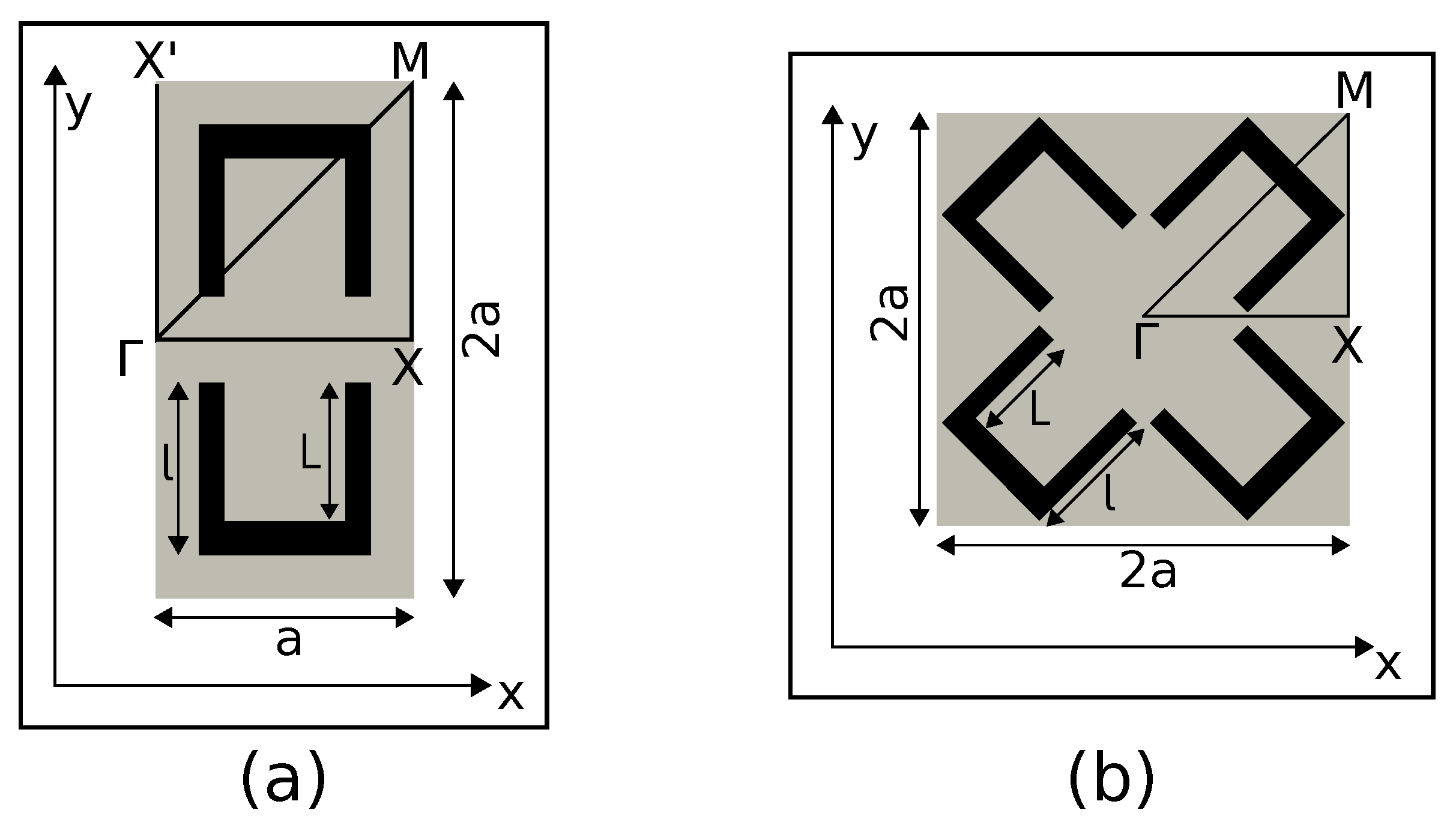
4.1. Single Resonant SC Made of Square-Rod Scatterers with Quarter Wavelengths Resonators
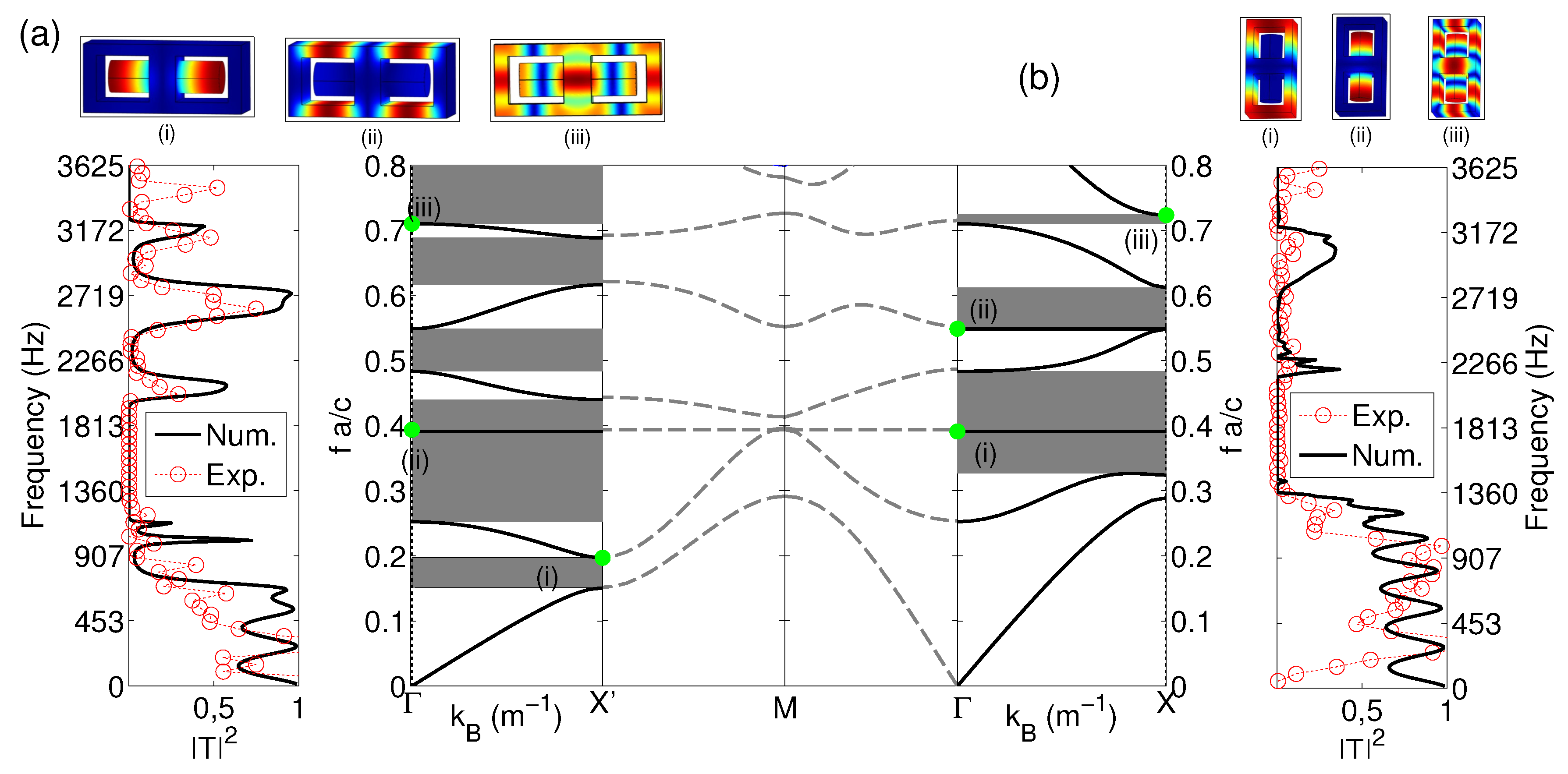
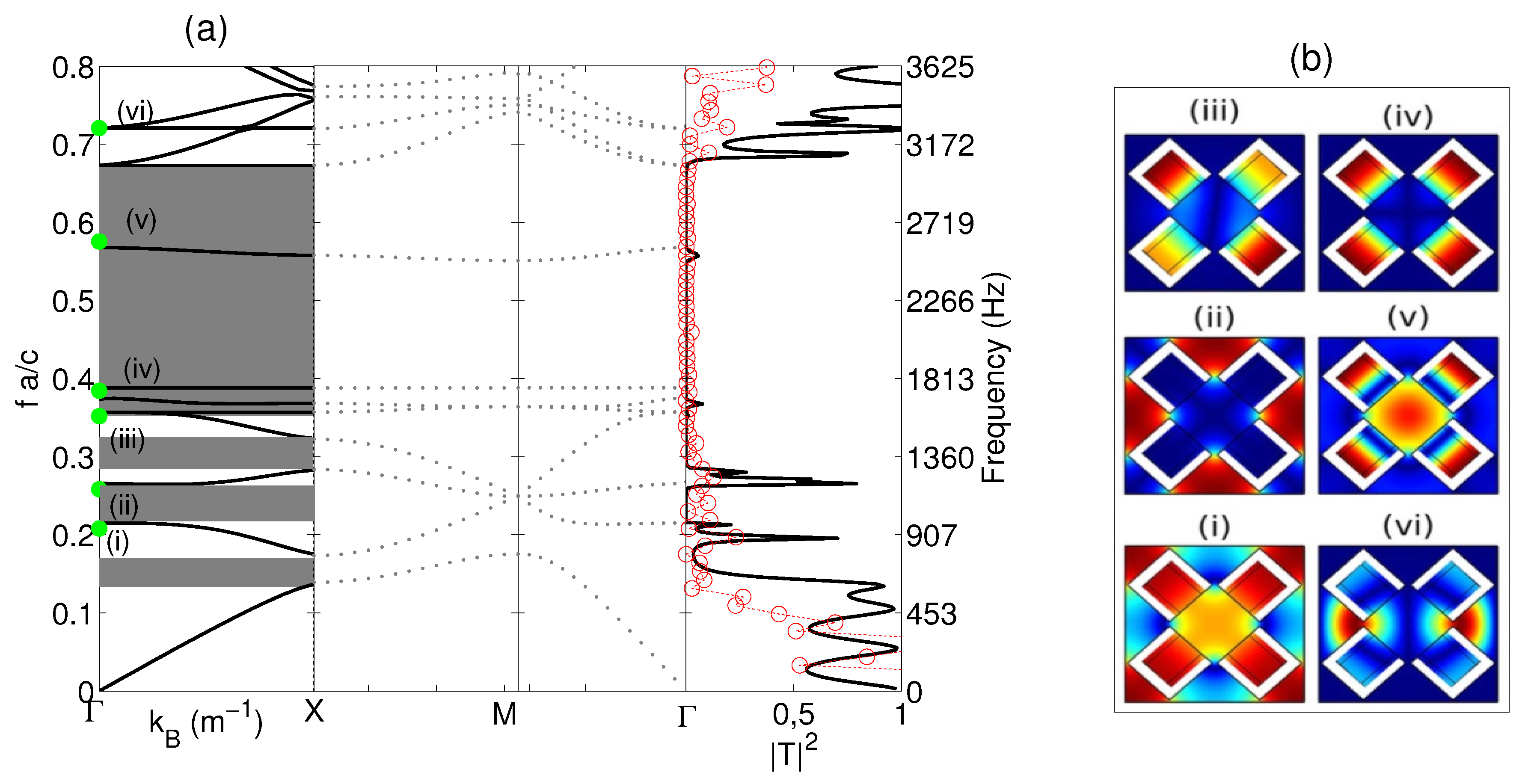
4.2. Multi-Resonant SC Made of Square-Rod Scatterers with Quarter Wavelengths Resonators
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Martínez-Sala, R.; Sancho, J.; Sánchez, J.V.; Gómez, V.; Llinares, J.; Meseguer, F. Sound Attenuation by sculpture. Nature 1995, 378, 241. [Google Scholar] [CrossRef]
- Sigalas, M. Elastic wave band gaps and defect states in two-dimensional composites. J. Acoust. Soc. Am. 1997, 101, 1256–1261. [Google Scholar] [CrossRef]
- Li, X.; Liu, Z. Coupling of cavity modes and guiding modes in two-dimensional phononic crystals. Solid State Commun. 2005, 133, 397–402. [Google Scholar] [CrossRef]
- Laude, V.; Achaoui, Y.; Benchabane, S.; Khelif, A. Evanescent Bloch waves and the complex band structure of phononic crystals. Phys. Rev. B 2009, 80, 092301. [Google Scholar] [CrossRef]
- Romero-García, V.; Sánchez-Pérez, J.V.; Castiñeira Ibáñez, S.C.; Garcia-Raffi, L.M. Evidences of evanescent Bloch waves in Phononic Crystals. Appl. Phys. Lett. 2010, 96, 124102. [Google Scholar] [CrossRef]
- Sánchez-Morcillo, V.; Staliunas, K.; Espinosa, V.; Pérez-Arjona, I.; Redondo, J.; Soliveres, E. Propagation of sound beams behind sonic crystals. Phys. Rev. B 2009, 80, 134303. [Google Scholar] [CrossRef]
- Khelif, A.; Choujaa, A.; Djafari-Rouhani, B.; Wilm, M.; Ballandras, S.; Laude, V. Trapping and guiding of acoustic waves by defect modes in a full-band-gap ultrasonic crystal. Phys. Rev. B 2003, 68, 214301. [Google Scholar] [CrossRef]
- Romero-García, V.; Vasseur, J.O.; Hladky-Hennion, A.C.; Garcia-Raffi, L.M.; Sánchez-Pérez, J.V. Level repulsion and evanescent waves in sonic crystals. Phys. Rev. B 2011, 84, 212302. [Google Scholar] [CrossRef]
- Romero-García, V.; Vasseur, J.; Garcia-Raffi, L.; Hladky-Hennion, A.C. Theoretical and experimental evidence of level repulsion states and evanescent modes in sonic crystal stubbed waveguides. New J. Phys. 2012, 14, 023049. [Google Scholar] [CrossRef]
- Caballero, D.; Sánchez-Dehesa, J.; Rubio, C.; Martínez-Sala, R.; Sánchez-Pérez, J.; Meseguer, F.; Llinares, J. Large two-dimensional sonic band gaps. Phys. Rev. E 1999, 60, R6316–R6319. [Google Scholar] [CrossRef]
- Anderson, C.; Giapis, K. Larger Two-Dimensional Photonic Band Gaps. Phys. Rev. Lett. 1996, 77, 2949–2952. [Google Scholar] [CrossRef] [PubMed]
- Anderson, C.; Giapis, K. Symmetry reduction in group 4mm photonic crystals. Phys. Rev. Lett. 1997, 56, 7313. [Google Scholar]
- Kushwaha, M.; Halevi, P.; Dobrzynski, L.; Djafari-Rouhani, B. Acoustic Band Structure of Periodic Elastic Composites. Phys. Rev. Lett. 1993, 71, 2022–2025. [Google Scholar] [CrossRef] [PubMed]
- Wang, R.; Wang, X.H.; Gu, B.Y.; Yang, G.Z. Effects of shapes and orientations of scatterers and lattice symmetries on the photonic band gap in two-dimensional photonic crystals. J. Appl. Phys. 2001, 90, 4307–4313. [Google Scholar] [CrossRef]
- Goffaux, C.; Vigneron, J.P. Theoretical study of a tunable phononic band gap system. Phys. Rev. B 2001, 64, 075118. [Google Scholar] [CrossRef]
- Norris, R.C.; Hamel, J.S.; Nadeaua, P. Phononic band gap crystals with periodic fractal inclusions: Theoretical study using numerical analysis. J. Appl. Phys. 2008, 103, 104908. [Google Scholar] [CrossRef]
- Bilal, O.R.; Hussein, M.I. Ultrawide phononic band gap for combined in-plane and out-of-plane waves. Phys. Rev. E 2011, 84, 065701. [Google Scholar] [CrossRef] [PubMed]
- Romero-García, V.; Lagarrigue, C.; Groby, J.P.; Richoux, O.; Tournat., V. Tunability of band gaps and waveguides in periodic arrays of square-rod scatterers: Theory and experimental realization. J. Phys. D Appl. Phys. 2013, 46, 305108. [Google Scholar] [CrossRef]
- Bradley, C.E. Acoustic Bloch Wave Propagation in a Periodic Waveguide; Technical Report of Applied Research Laboratories, ARL-TR-91-19 (July); The University of Texas Austin: Austin, TX, USA, 1991. [Google Scholar]
- Sugimoto, N.; Horioka, T. Dispersion characteristics of sound waves in a tunnel with an array of Helmholtz resonators. J. Acoust. Soc. Am. 1995, 97, 1446–1459. [Google Scholar] [CrossRef]
- Liu, Z.; Zhang, X.; Mao, Y.; Zhu, Y.; Yang, Z.; Chan, C.; Sheng, P. Locally Resonant Sonic Materials. Science 2000, 289, 1734–1736. [Google Scholar] [CrossRef] [PubMed]
- Lagarrigue, C.; Groby, J.-P.; Tournat, V. Sustainable sonic crystal made of resonating bamboo rods. J. Acous. Soc. Am. 2013, 133, 247–254. [Google Scholar] [CrossRef] [PubMed]
- Fang, N.; Xi, D.; Xu, J.; Ambati, M.; Srituravanich, W.; Sun, C.; Zhang, X. Ultrasonic metamaterials with negative modulus. Nat. Mater. 2006, 5, 452–456. [Google Scholar] [CrossRef] [PubMed]
- Groby, J.-P.; Nennig, B.; Lagarrigue, C.; Brouard, B.; Dazel, O.; Tournat, V. Enhancing the absorption properties of acoustic porous plates by periodically embedding Helmholtz resonators. J. Acous. Soc. Am. 2015, 137, 273–280. [Google Scholar] [CrossRef] [PubMed]
- Zwikker, C.; Kosten, C. Sound Absorbing Materials; Elsevier Publishing Company: New York, NY, USA, 1949. [Google Scholar]
- Duclos, A.; Lafarge, D.; Pagneux, V. Transmission of acoustic waves through 2D phononic crystal: Visco-thermal and multiple scattering effects. Eur. Phys. J. Appl. Phys. 2009, 45, 11302. [Google Scholar] [CrossRef]
- Moiseyenko, R.; Laude, V. Material loss influence on the complex band structure and group velocity in phononic crystals. Phys. Rev. B 2011, 83, 064301. [Google Scholar] [CrossRef]
- Theocharis, G.; Richoux, O.; Romero-García, V.; Tournat, V. Slow sound propagation in lossy locally resonant periodic structures. New J. Phys. 2014, 16, 093017. [Google Scholar] [CrossRef]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lardeau, A.; Groby, J.-P.; Romero-García, V. Broadband Transmission Loss Using the Overlap of Resonances in 3D Sonic Crystals. Crystals 2016, 6, 51. https://doi.org/10.3390/cryst6050051
Lardeau A, Groby J-P, Romero-García V. Broadband Transmission Loss Using the Overlap of Resonances in 3D Sonic Crystals. Crystals. 2016; 6(5):51. https://doi.org/10.3390/cryst6050051
Chicago/Turabian StyleLardeau, Alexandre, Jean-Philippe Groby, and Vicente Romero-García. 2016. "Broadband Transmission Loss Using the Overlap of Resonances in 3D Sonic Crystals" Crystals 6, no. 5: 51. https://doi.org/10.3390/cryst6050051
APA StyleLardeau, A., Groby, J.-P., & Romero-García, V. (2016). Broadband Transmission Loss Using the Overlap of Resonances in 3D Sonic Crystals. Crystals, 6(5), 51. https://doi.org/10.3390/cryst6050051