Effect of Deposits on Micron Particle Collision and Deposition in Cooling Duct of Turbine Blades
Abstract
1. Introduction
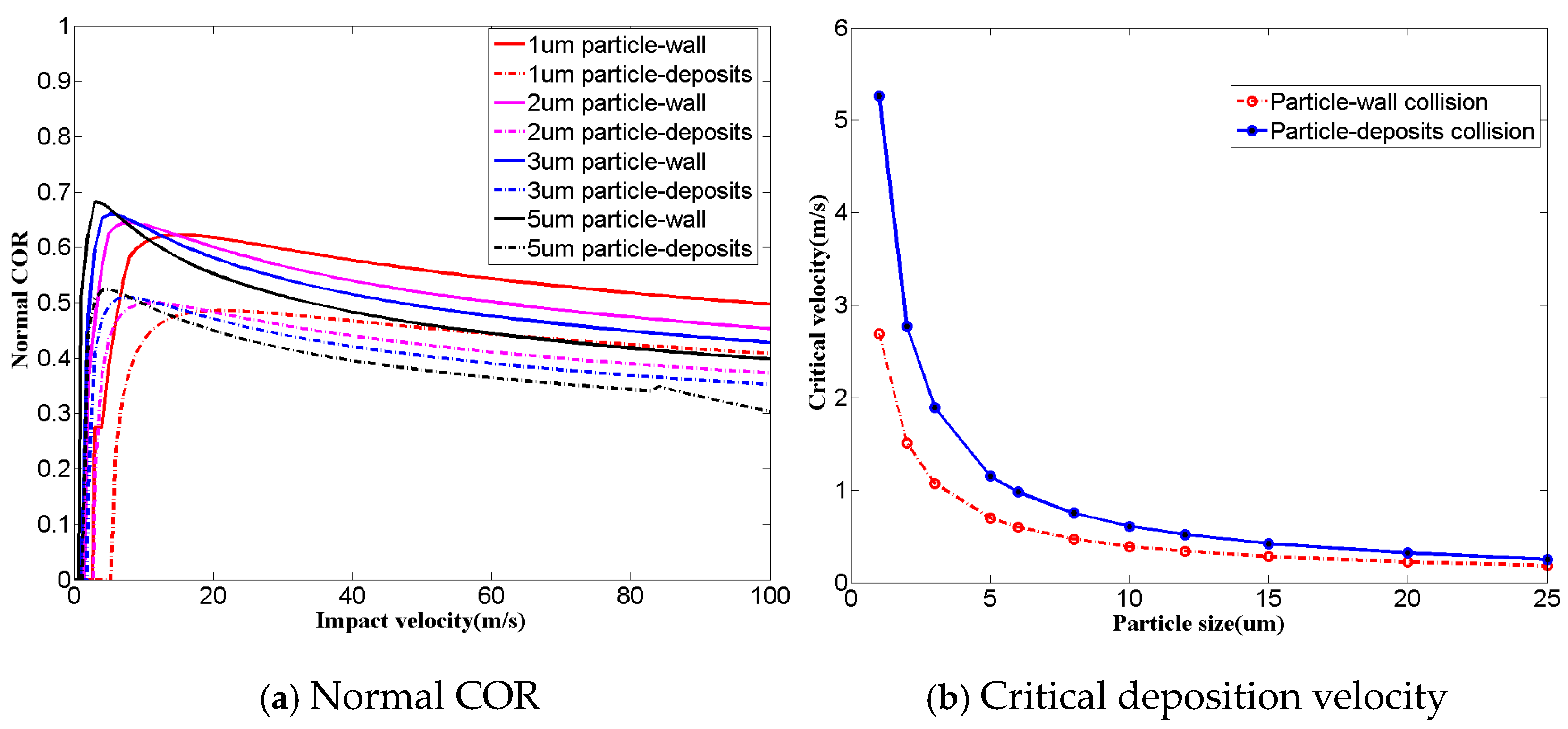
2. Analysis of Deposits’ Influence Based on Particle–Wall Collision Theory
3. Particle Collision with Surface Covered with Deposits
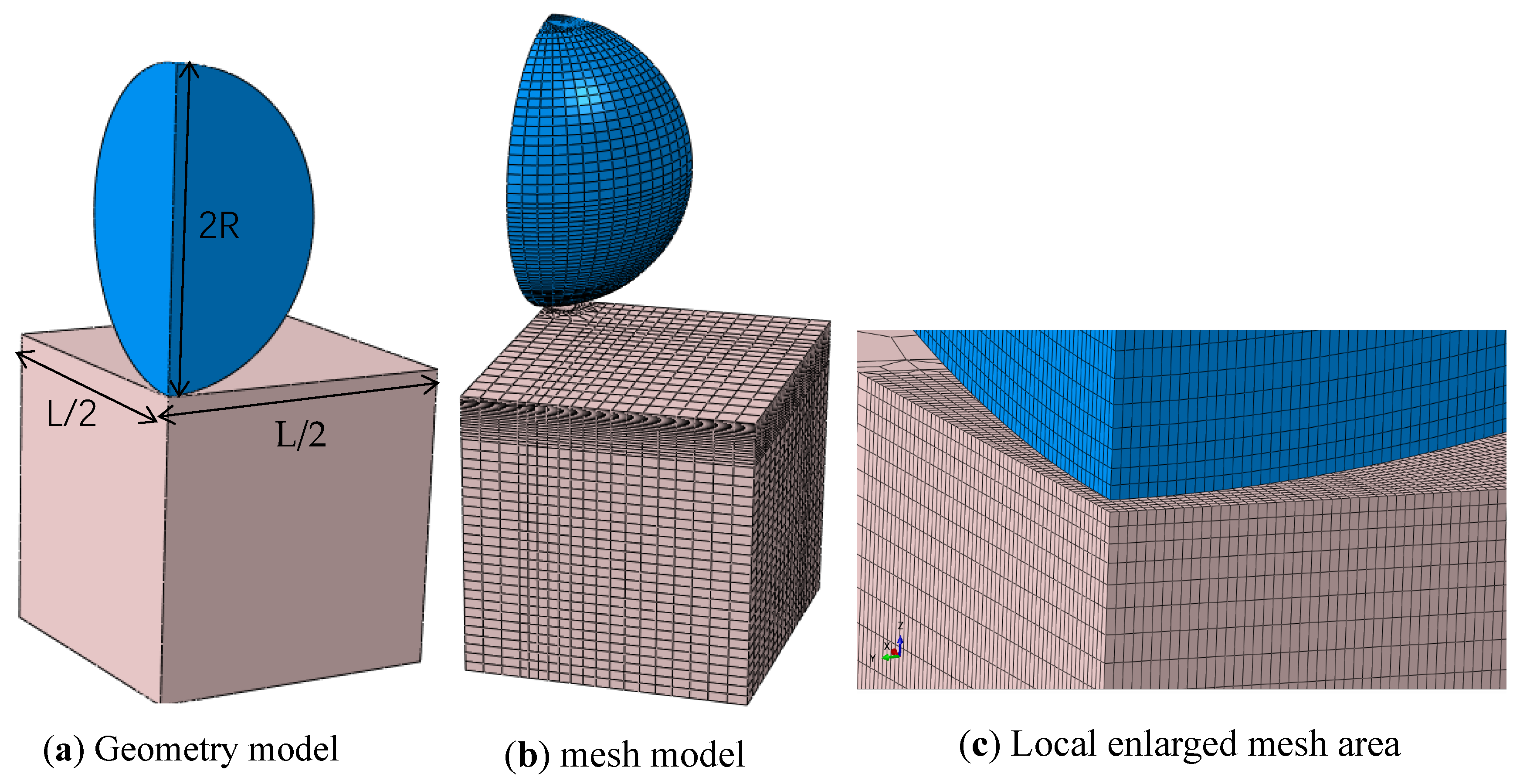
3.1. Model of Flat Surface for Particle Collision
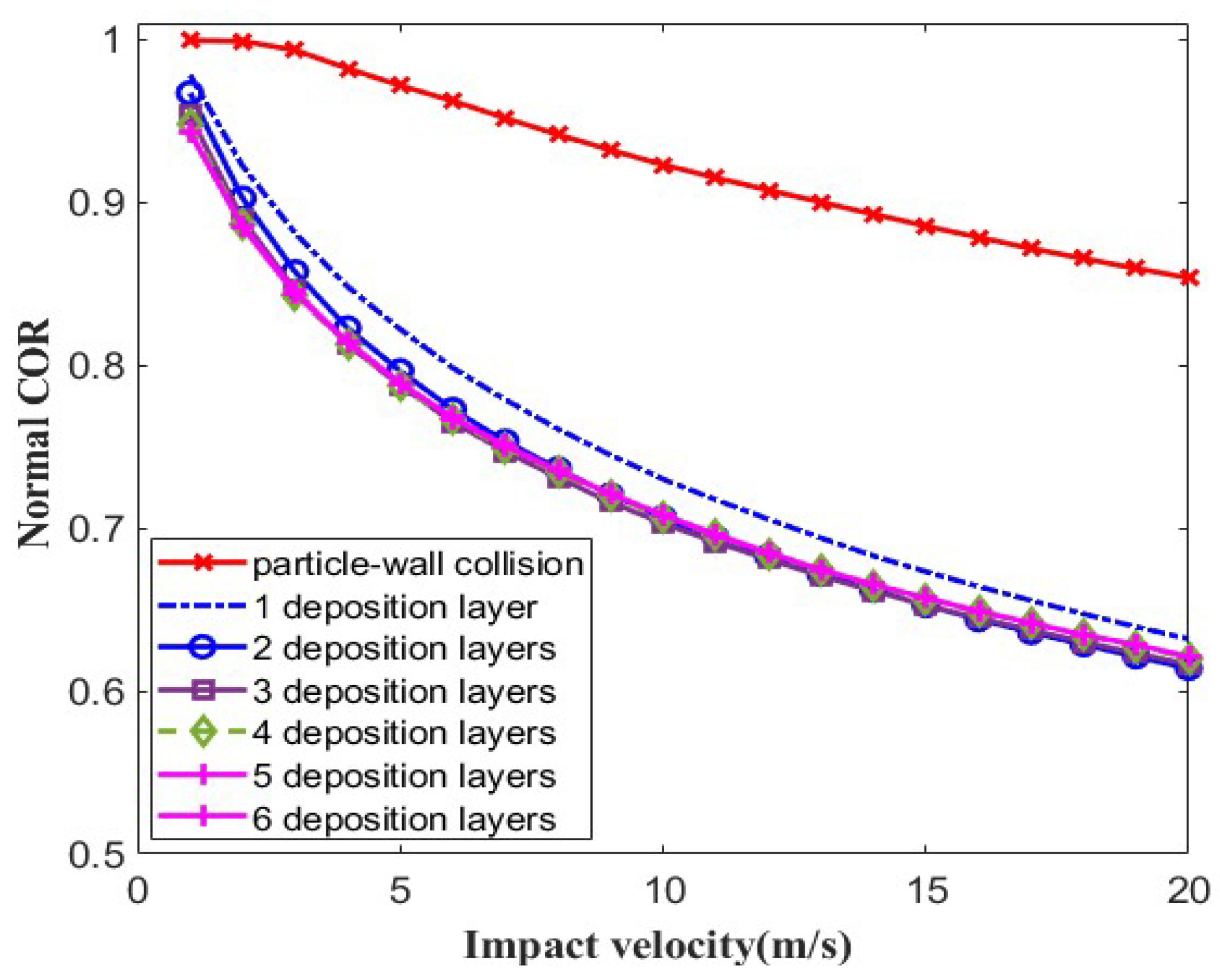
3.2. Deposit Thickness Analysis
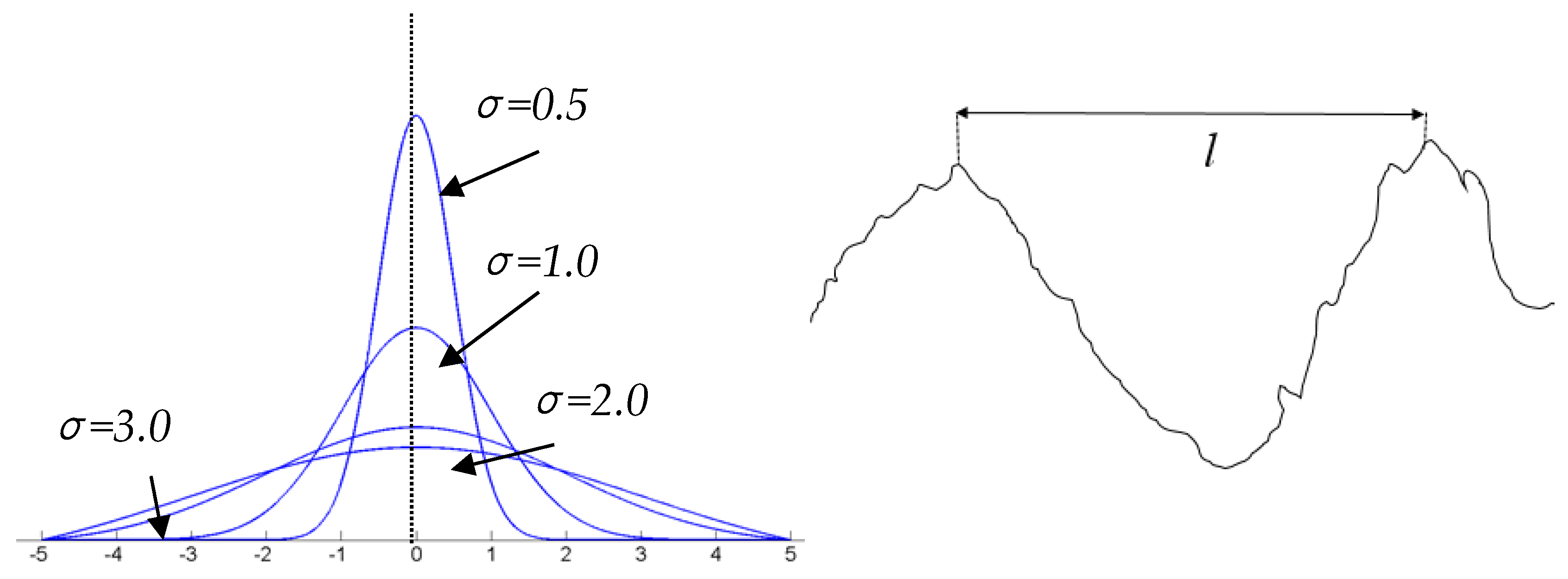
3.3. Generation of Rough Surface Model
3.4. Effect of Surface Roughness of Deposits
4. Conclusions
- The theoretical analysis shows that the normal restitution coefficient for particle–deposit collision is much lower than that of particle–DD3 nickel-based single-crystal substrate collision. The critical deposition velocity of the particles is higher for particle–deposit collisions and decreases with the increase in particle size.
- The numerical study of the effects of deposits with flat surfaces displayed that when the height of the deposits is greater than twice the particle diameter, the COR tends to be unchanged, which means particle–wall collision becomes particle–deposit collision.
- Random Gaussian rough surfaces with constant Rms and different correlation lengths l were constructed and analyzed under particle collision. The results demonstrate that surface roughness plays an important role in particle contact behavior. A rough surface with a smaller correlation length, representing a high density of asperities, and that is steeper has a longer contact time and smaller contact area. The restitution coefficient decreases with increasing surface roughness. The correlation length l has minimal effect on the rotational velocity.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, D.; Ding, Q.; Zhou, Q.; Zhou, D.; Wei, X.; Zhao, X.; Zhang, Z.; Bei, H. Microstructure, Mechanical Properties and Thermal Stability of Ni-Based Single Crystal Superalloys with Low Specific Weight. Crystals 2023, 13, 610. [Google Scholar] [CrossRef]
- Wylie, S.; Bucknell, A.; Forsyth, P.; McGilvray, M.; Gillespie, D.R. Reduction in flow parameter resulting from volcanic ash deposition in engine representative cooling passages. J. Turbomach. 2017, 139, 031008. [Google Scholar] [CrossRef]
- Boulanger, A.; Patel, H.; Hutchinson, J.; DeShong, W.; Xu, W.; Ng, W.; Ekkad, S. Preliminary experimental investigation of initial onset of sand deposition in the turbine section of gas turbines. In Turbo Expo: Power for Land, Sea, and Air; GT2016-56059; American Society of Mechanical Engineers: New York, NY, USA, 2016. [Google Scholar]
- Wammack, J.E.; Crosby, J.; Fletcher, D.; Bons, J.P.; Fletcher, T.H. Evolution of surface deposits on a high pressure turbine blade, part i: Physical characteristics. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 2006; Volume 4238, pp. 1055–1063. [Google Scholar]
- Yang, X.J.; Cui, M.H.; Liu, Z.G. Experimental study on deposition of particulate on plate surface with cooling film. Propuls. Technol. 2018, 39, 1323–1330. (In Chinese) [Google Scholar]
- Lundgreen, R.; Sacco, C.; Prenter, R.; Bons, J.P. Temperature effects on nozzle guide vane deposition in a new turbine cascade rig. In Turbo Expo: Power for Land, Sea, and Air; GT2016-57560; American Society of Mechanical Engineers: New York, NY, USA, 2016. [Google Scholar]
- Davidson, F.T.; Kistenmacher, D.A.; Bogard, D.G. A Study of Deposition on a Turbine Vane With a Thermal Barrier Coating and Various Film Cooling Geometries. J. Turbomach. 2014, 136, 041009. [Google Scholar] [CrossRef]
- Laycock, R.; Fletcher, T.H. Independent effects of surface and gas temperature on coal fly ash deposition in gas turbines at temperatures up to 1400 °C. J. Eng. Gas Turbines Power 2016, 138, 021402. [Google Scholar] [CrossRef]
- Lawson, S.A.; Lynch, S.P.; Thole, K.A. Simulations of multiphase particle deposition on a non-axisymmetric contoured end wall with film-cooling. J. Turbomach. 2013, 135, 031032. [Google Scholar] [CrossRef]
- Albert, J.E.; Bogard, D.G. Experimental simulation of contaminant deposition on a film cooled turbine air-foil leading edge. J. Turbomach. 2012, 134, 51011. [Google Scholar] [CrossRef]
- Albert, J.E.; Bogard, D.G. Experimental simulation of contaminant deposition on a film-cooled turbine vane pressure side with a trench. J. Turbomach. 2013, 135, 51001. [Google Scholar] [CrossRef]
- Xin, S.; Yu, K.; Xu, X.; Yao, S.; Wang, H. Simulation of surface deposits of micron sand particles in the internal cooling duct with a Gaussian probability density model. Comput. Part. Mech. 2024, 11, 2465–2481. [Google Scholar] [CrossRef]
- Brach, R.M.; Dunn, P.F. A mathematical model of the impact and adhesion of microspheres. Aerosol Sci. Technol. 1992, 16, 51–64. [Google Scholar] [CrossRef]
- Tafti, D.; Sreedharan, S. Composition dependent model for the prediction of syngas ash deposition with application to a leading edge turbine vane. In Proceedings of the ASME Turbo Expo, 2010, GT2010-23655, Glasgow, UK, 14–18 June 2010. [Google Scholar]
- Singh, S.; Tafti, D. Particle deposition model for particulate flows at high temperatures in gas turbine components. Int. J. Heat Fluid Flow 2015, 52, 72–83. [Google Scholar] [CrossRef]
- Yu, K.; Tafti, D. Impact model for micrometer-sized sand particles. Powder Technol. 2016, 294, 11–21. [Google Scholar] [CrossRef]
- Yu, K.; Elghannay, H.A.; Tafti, D. An impulse based model for spherical particle collisions with sliding and rolling. Powder Technol. 2017, 319, 102–116. [Google Scholar] [CrossRef]
- Yu, K.; Tafti, D. Size and temperature dependent collision and deposition model for micron-sized sand particles. J. Turbomach. -Trans. ASME 2019, 141, 031001. [Google Scholar] [CrossRef]
- Dowd, C.; Tafti, D.; Yu, K. Sand transport and deposition in rotating two-passed ribbed duct with Coriolis and centrifugal Buoyancy forces at RE=100,000. In Turbo Expo: Turbomachinery Technical Conference and Exposition; GT2017-63167; American Society of Mechanical Engineers: New York, NY, USA, 2017. [Google Scholar]
- Yu, K.; Liu, J.; Xu, X.; Yao, S.; Hou, N.X.; Yue, Z. Dust transport investigation in ribbed cooling duct integrating temperature-dependent elastic-plastic particle collision model. Part. Sci. Technol. 2022, 40, 42–52. [Google Scholar] [CrossRef]
- Forsyth, P.R.; Gillespie, D.R.; McGilvray, M. Development and applications of a couple deposition dynamic mesh morphing approach for the numerical simulation of gas turbine flows. J. Eng. Gas Turbines Power Trans. ASME 2018, 140, 022603. [Google Scholar] [CrossRef]
- Mu, L.; Wang, S.; Zhai, Z. Unsteady CFD simulation on ash particle deposition and removal characteristics in tube banks: Focusing on particle diameter, flow velocity, and temperature. J.-Energy Inst. 2020, 93, 1481–1494. [Google Scholar] [CrossRef]
- Feuillebois, F.; Gensdarmes, F.; Mana, Z.; Ricciardi, L.; Monier, C.; Le Meur, G.; Reynaud, C.; Rabaud, M. Three-dimensional motion of particles in a shear flow near a rough wall. J. Aerosol Sci. 2016, 96, 69–95. [Google Scholar] [CrossRef]
- Mizes, H.; Ott, M.; Eklund, E.; Hays, D. Small particle adhesion: Measurement and control. Colloids Surf. A 2000, 165, 11–23. [Google Scholar] [CrossRef]
- Greenwood, J.A.; Williamson, J.B.P. Contact of nominally flat surfaces. Proc. R. Soc. Lond. 1966, 295, 300–319. [Google Scholar]
- Patil, D.B.; Eriten, M. Effects of interfacial strength and roughness on the static friction coefficient. Tribol. Lett. 2014, 56, 355–374. [Google Scholar] [CrossRef]
- Krull, F.; Mathy, J.; Breuninger, P.; Antonyuk, S. Influence of the surface roughness on the collision behavior of fine particles in ambient fluids. Powder Technol. 2021, 392, 58–68. [Google Scholar] [CrossRef]
- Rajupet, S.; Riet, A.A.; Chen, Q.; Sow, M.; Lacks, D.J. Relative importance of electrostatic and van der Waals forces in particle adhesion to rough conducting surfaces. Phys. Rev. E 2021, 103, 042906. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Zhang, Y.; Fang, Z.; Wu, X. The effect of surface roughness on the viscoelastic energy dissipation in a particle-wall collision. J. Aerosol Sci. 2024, 175, 106280. [Google Scholar] [CrossRef]
- Gao, Y.F.; Bower, A. Elastic-plastic contact of a rough surface with weierstrass profile. Proc. R. Soc. A Math. Phys. Eng. Sci. 2006, 462, 319–348. [Google Scholar] [CrossRef]
- Jackson, R.L.; Green, I. A statistical model of elasto-plastic asperity contact between rough surfaces. Tribol. Int. 2006, 39, 906–914. [Google Scholar] [CrossRef]
- Yu, K.; Peng, C.; Shang, L.; Tafti, D. Elastic-plastic collision mechanism of micron-sized particle impacting rough surfaces. Powder Technol. 2024, 442, 119903. [Google Scholar] [CrossRef]
- Liu, J.; Yu, K.; Tafti, D.; Yang, X.; Xu, H. Dust adhesion and deposition behavior in internal cooling duct with pin fins. Int. J. Therm. Sci. 2023, 184, 107943. [Google Scholar] [CrossRef]
- Wu, C.Y.; Li, L.Y.; Thornton, C. Energy dissipation during normal impact of elastic and elastic–plastic spheres. Int. J. Impact Eng. 2005, 32, 593–604. [Google Scholar] [CrossRef]
- Qu, X.; Cai, X.; Zhang, M.; Lin, H.; Leihong, Z.; Liao, B.Q. A facile method for simulating randomly rough membrane surface associated with interface behaviors. Appl. Surf. Sci. 2017, 427, 915–921. [Google Scholar] [CrossRef]
- Li, X.; Dong, M.; Jiang, D.; Li, S.; Shang, Y. The effect of surface roughness on normal restitution coefficient, adhesion force and friction coefficient of the particle-wall collision. Powder Technol. 2020, 362, 17–25. [Google Scholar] [CrossRef]


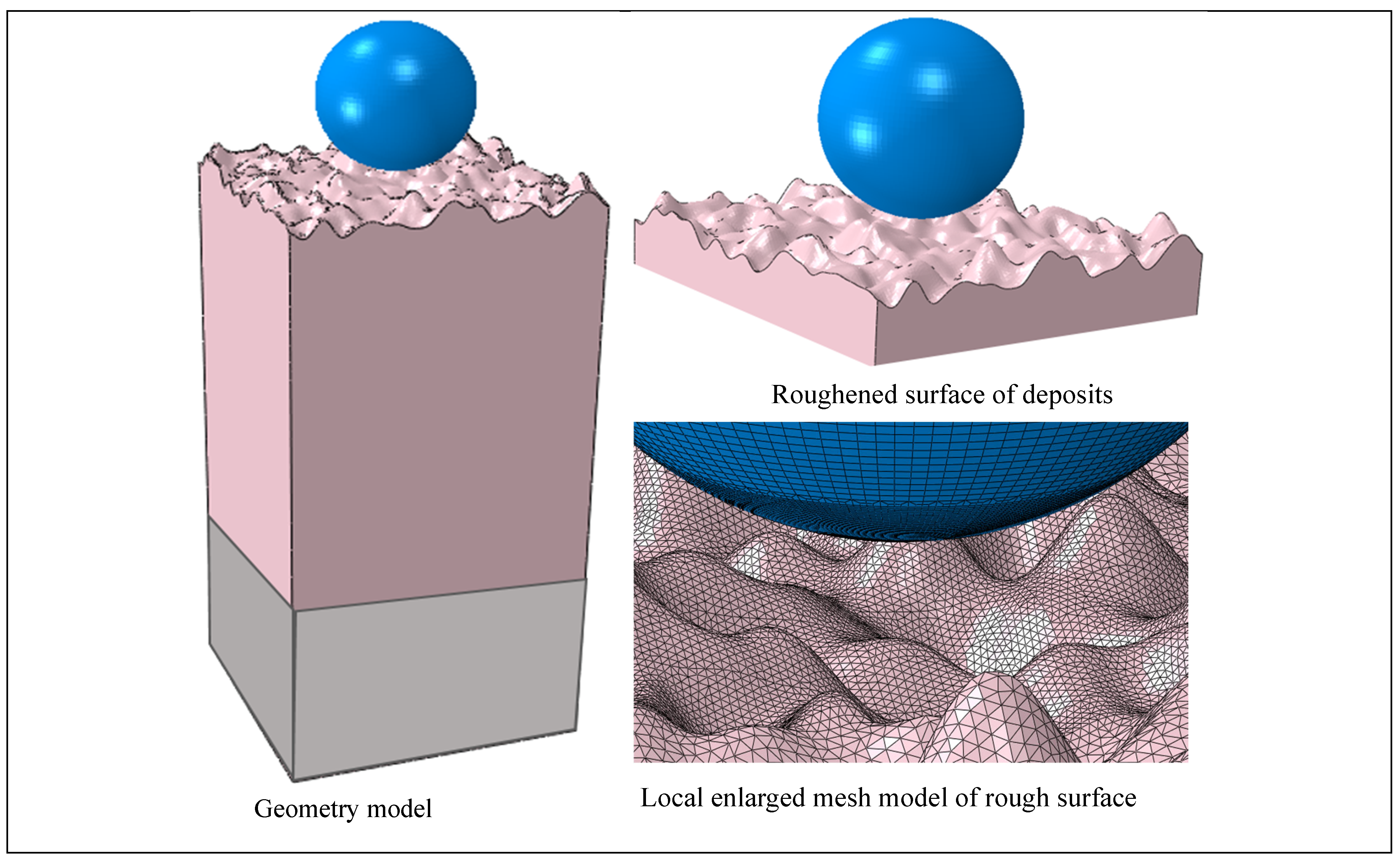
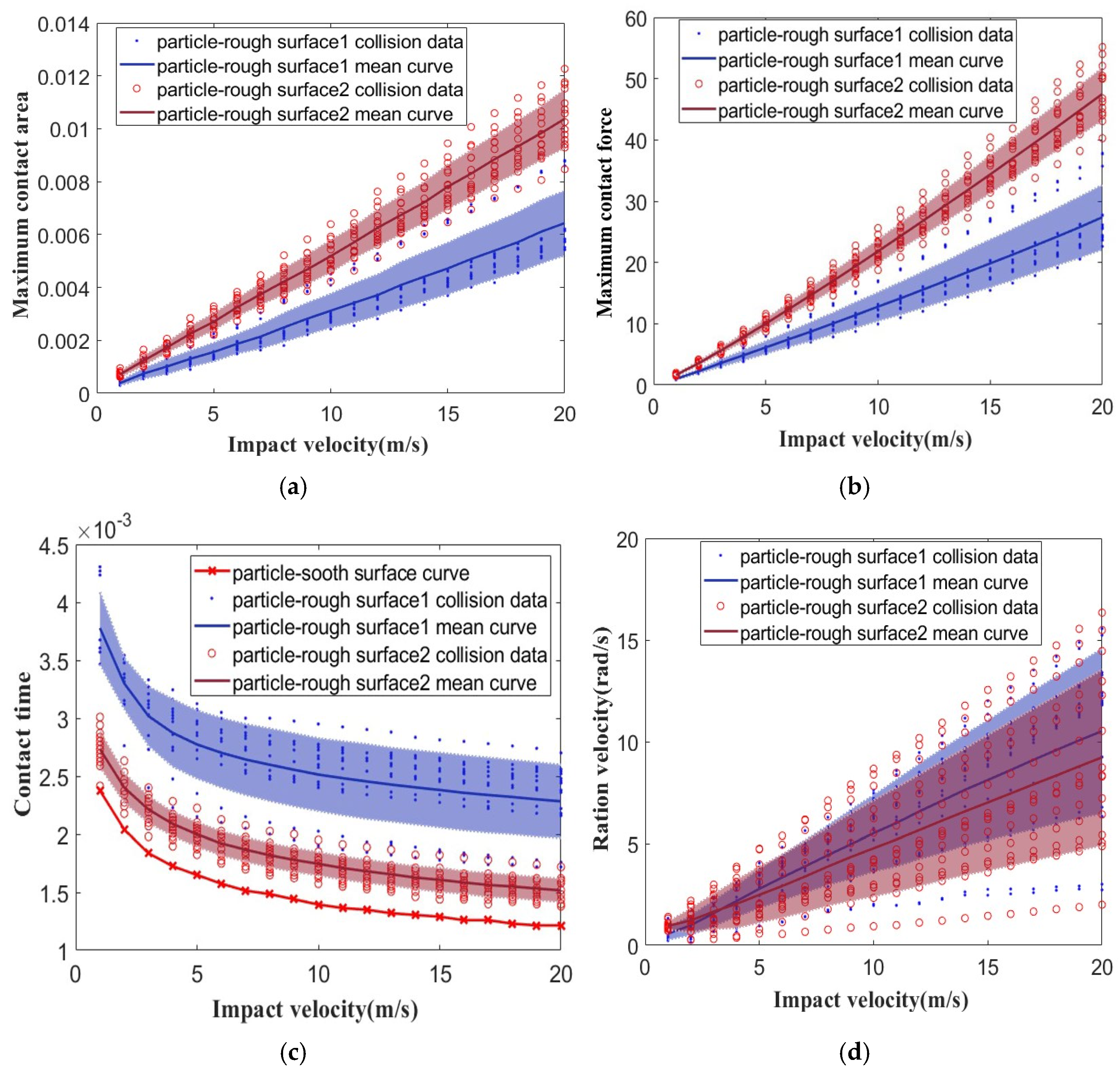
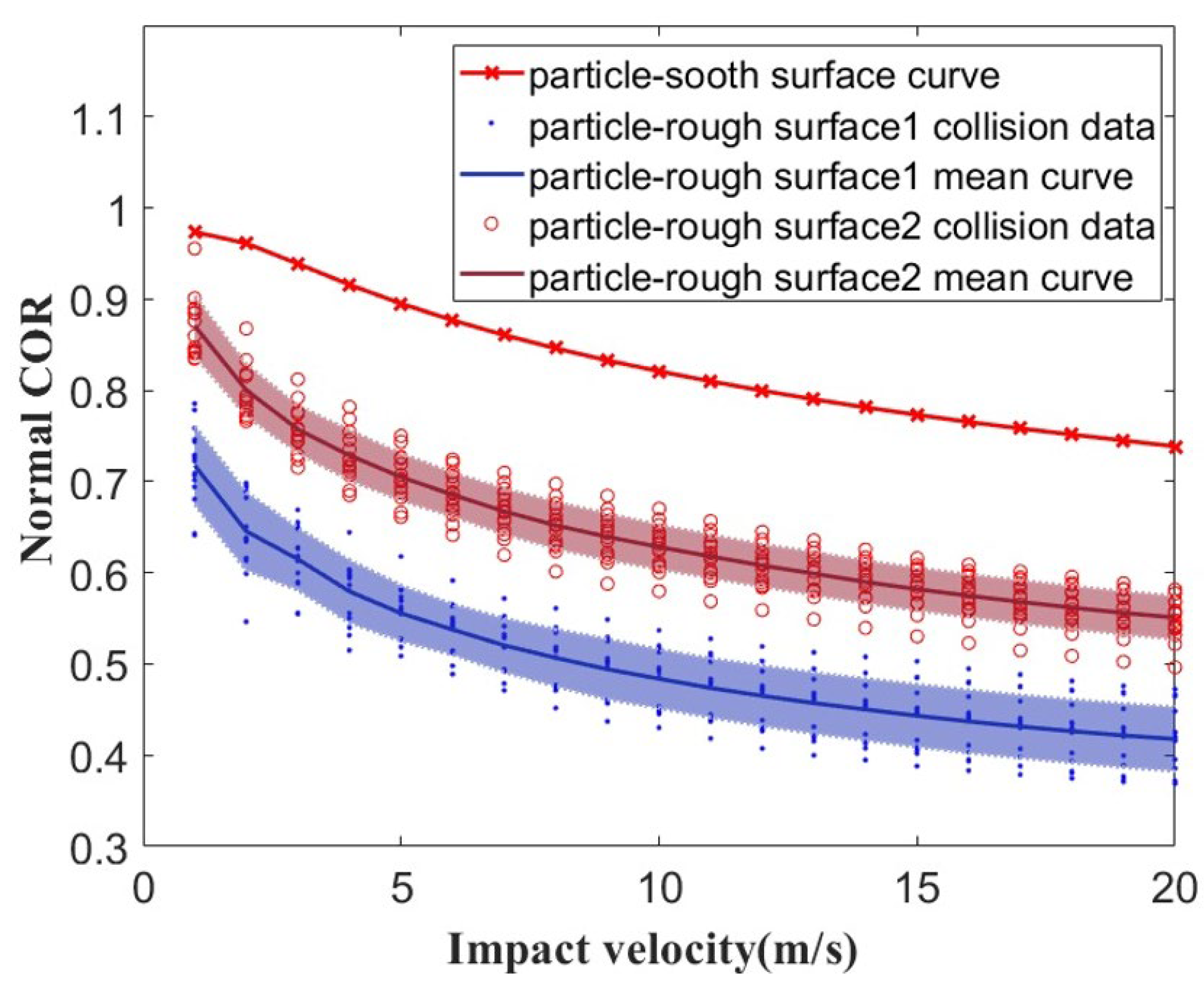
| Material | Elastic Modulus/GPa | Yield Stress/MPa/ | Density/kg/m3 | Poisson’s Ratio |
|---|---|---|---|---|
| Particle | 195 | 900 | 2630 | 0.23 |
| Wall | 61 | 440 | 8200 | 0.33 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xin, S.; Peng, C.; Qi, J.; Su, B.; Xiao, Y. Effect of Deposits on Micron Particle Collision and Deposition in Cooling Duct of Turbine Blades. Crystals 2025, 15, 510. https://doi.org/10.3390/cryst15060510
Xin S, Peng C, Qi J, Su B, Xiao Y. Effect of Deposits on Micron Particle Collision and Deposition in Cooling Duct of Turbine Blades. Crystals. 2025; 15(6):510. https://doi.org/10.3390/cryst15060510
Chicago/Turabian StyleXin, Shihong, Chuqi Peng, Junchao Qi, Baiwan Su, and Yan Xiao. 2025. "Effect of Deposits on Micron Particle Collision and Deposition in Cooling Duct of Turbine Blades" Crystals 15, no. 6: 510. https://doi.org/10.3390/cryst15060510
APA StyleXin, S., Peng, C., Qi, J., Su, B., & Xiao, Y. (2025). Effect of Deposits on Micron Particle Collision and Deposition in Cooling Duct of Turbine Blades. Crystals, 15(6), 510. https://doi.org/10.3390/cryst15060510






