Influence of Yttria Nanoclusters on the Void Nucleation in BCC Iron During Multi-Axial Tensile Deformation: A Molecular Dynamics Simulation
Abstract
:1. Introduction
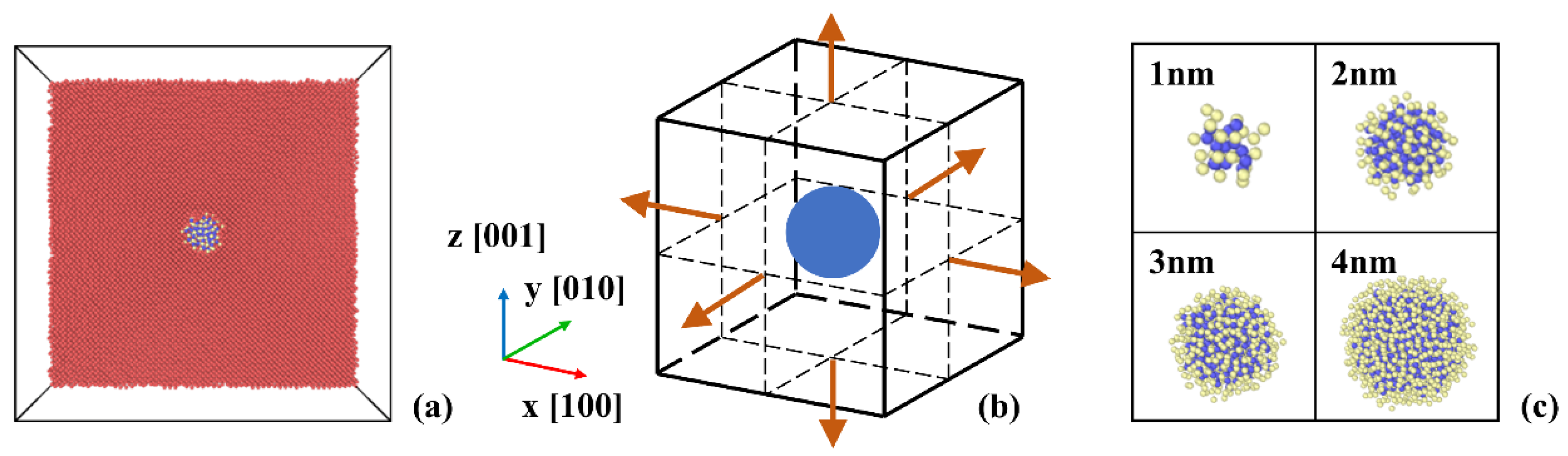
2. Computational Method
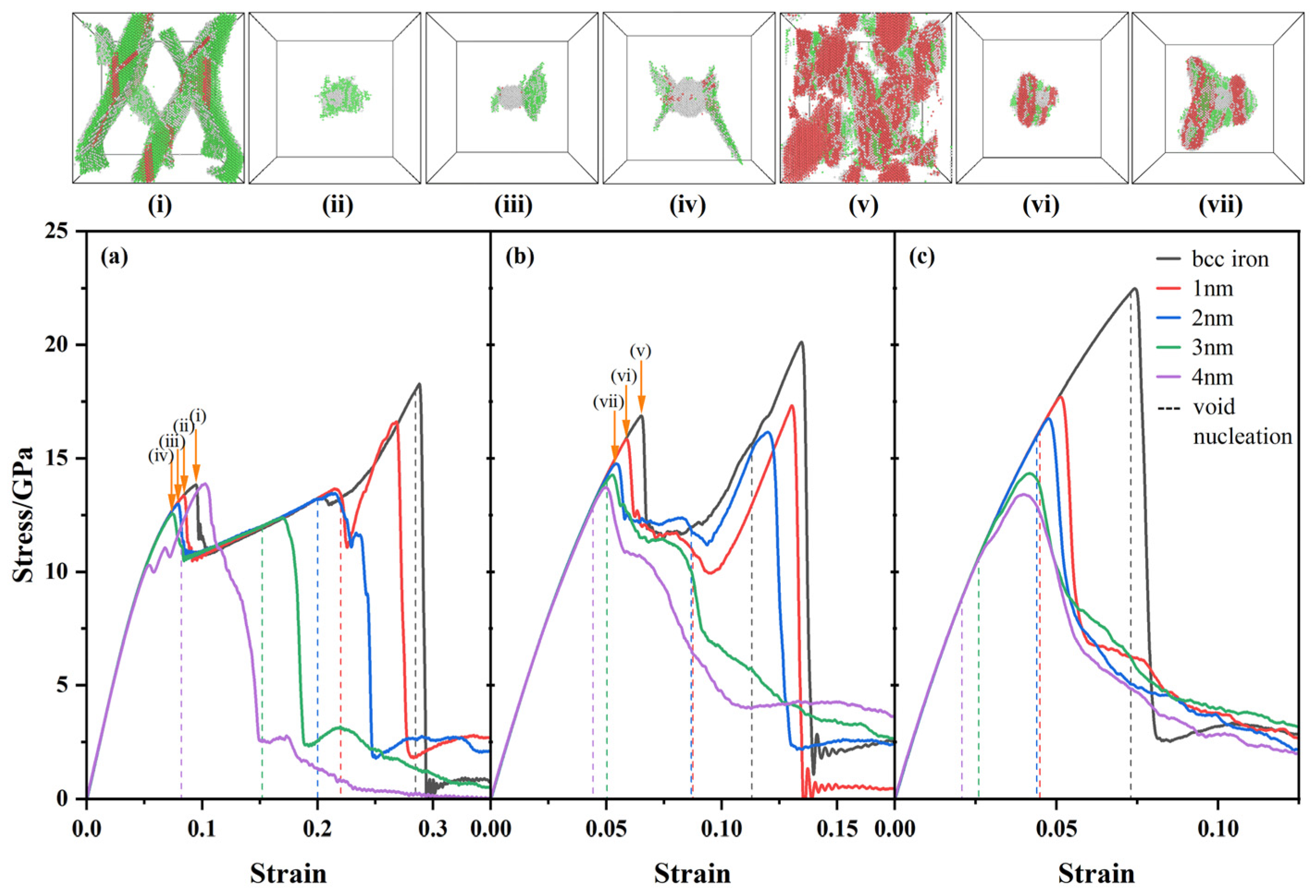
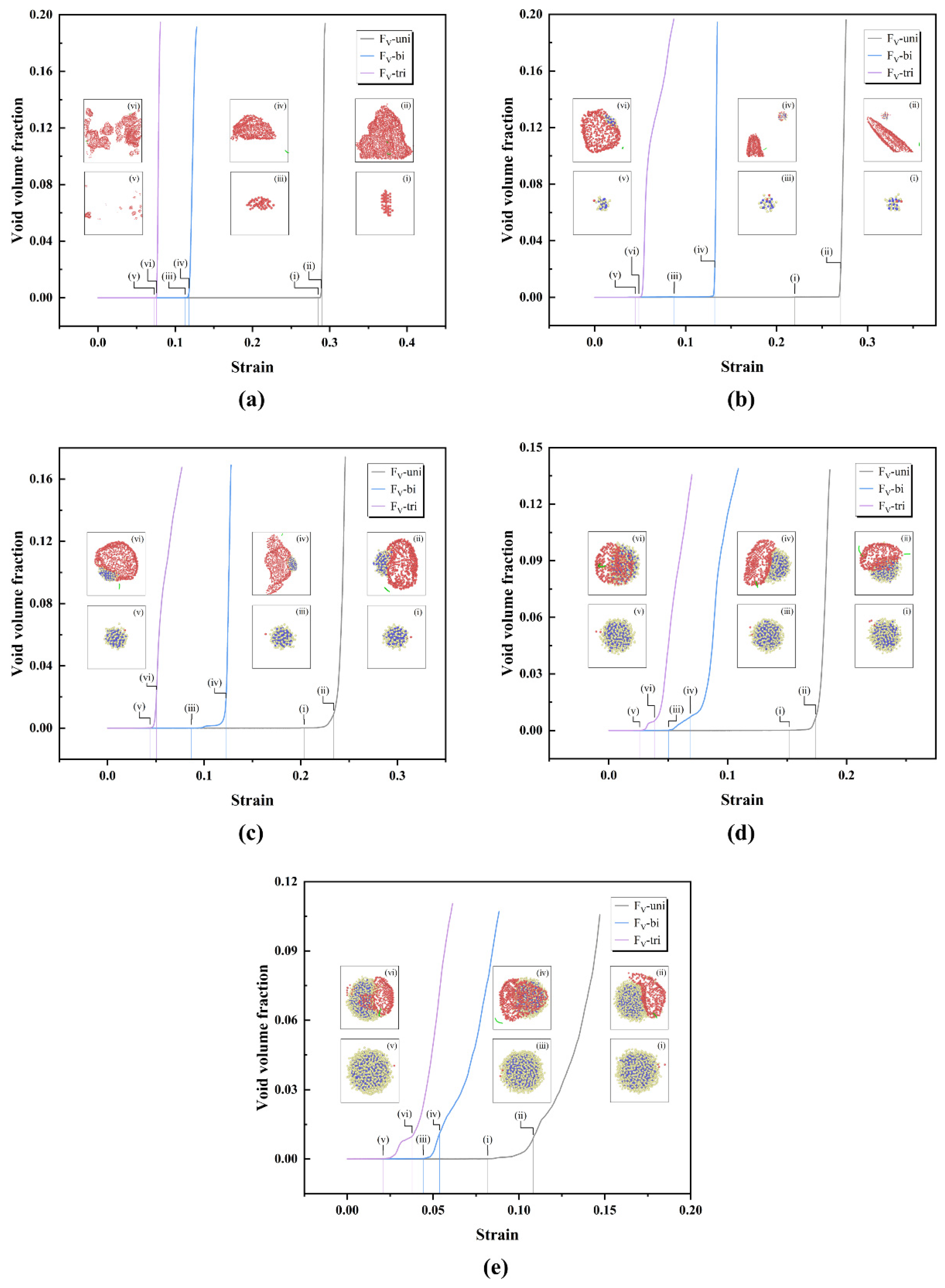
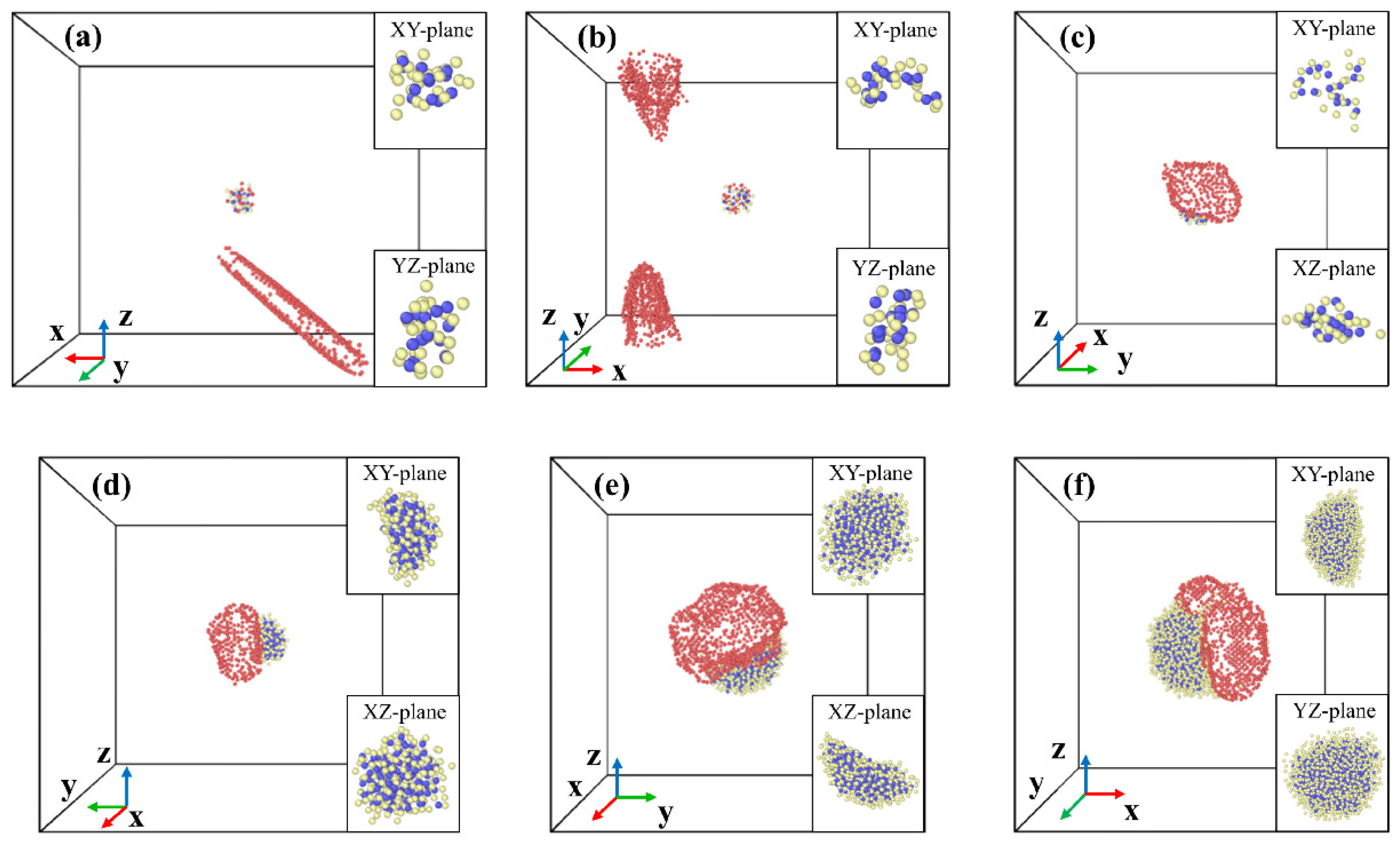
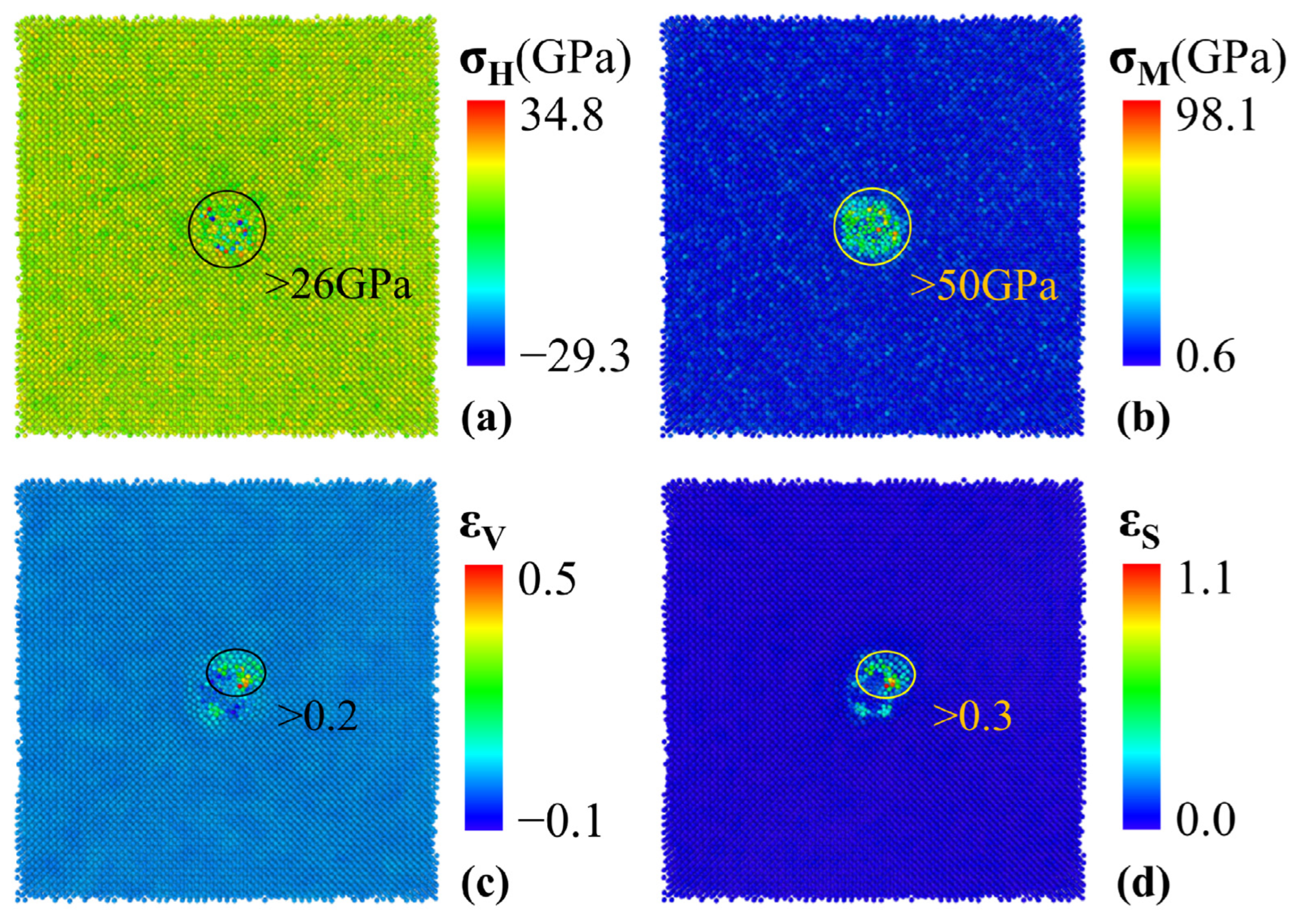
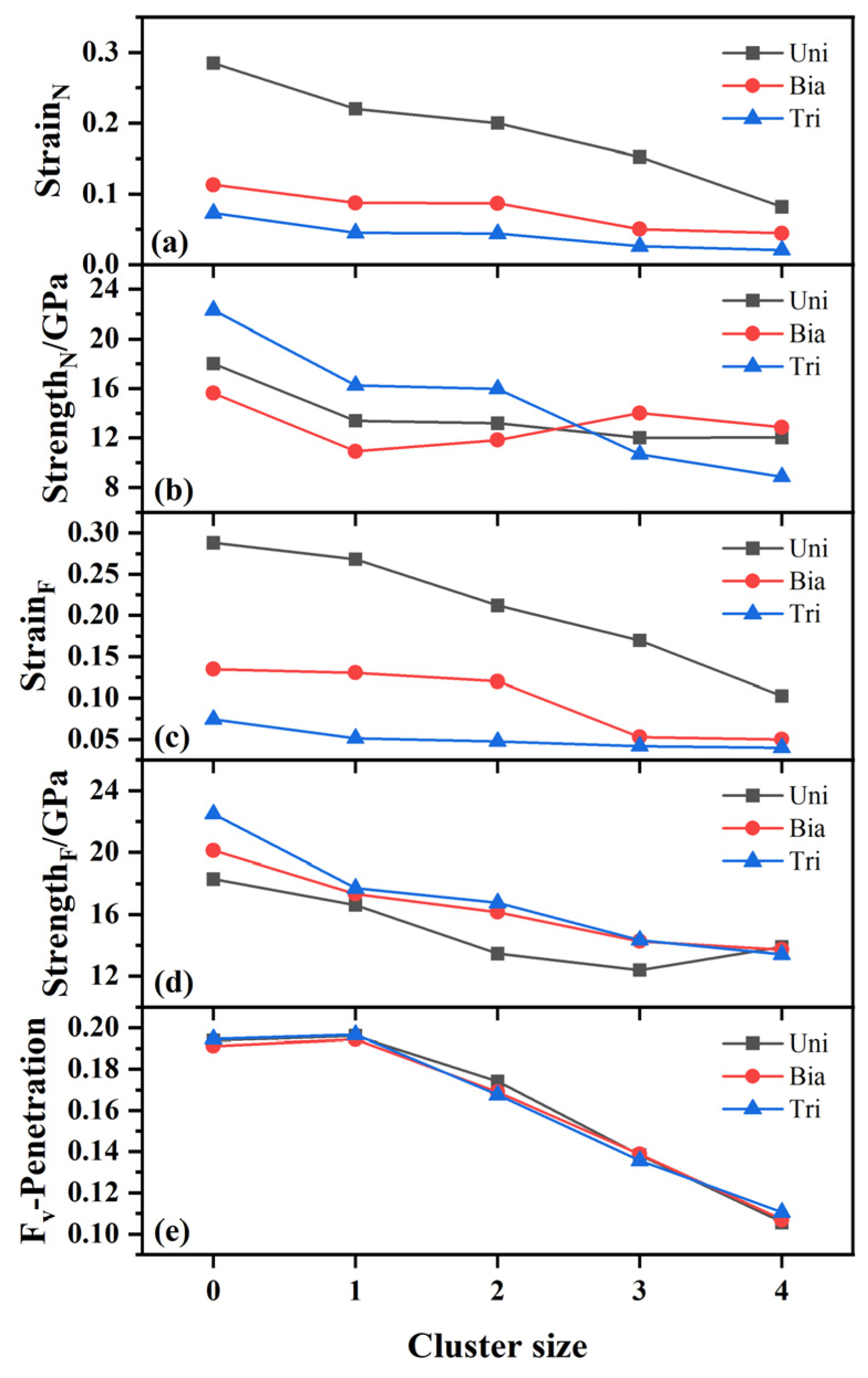
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- He, P.; Yao, W.; Lyu, J.; Gao, B.; Li, X. Radiation Resistance Design and Nanoscale Second-phase Particles Characterization for ODS Steels: A Review. Cailiao Daobao/Mater. Rep. 2018, 32, 34–40. [Google Scholar] [CrossRef]
- Yang, T.X.; Dou, P.; Zhang, P.; Yang, Y.C. STEM and HRTEM study on matrix microstructure and oxide particles in 11Cr ferritic/martensitic ODS steel. J. Nucl. Mater. 2023, 576, 154259. [Google Scholar] [CrossRef]
- Bhattacharya, A.; Zinkle, S.J.; Henry, J.; Levine, S.M.; Edmondson, P.D.; Gilbert, M.R.; Tanigawa, H.; Kessel, C.E. Irradiation damage concurrent challenges with RAFM and ODS steels for fusion reactor first-wall/blanket: A review. J. Phys. Energy 2022, 4, 034003. [Google Scholar] [CrossRef]
- Rieth, M.; Dürrschnabel, M.; Bonk, S.; Pintsuk, G.; Aiello, G.; Henry, J.; de Carlan, Y.; Ghidersa, B.E.; Neuberger, H.; Rey, J.; et al. Impact of materials technology on the breeding blanket design—Recent progress and case studies in materials technology. Fusion Eng. Des. 2021, 166, 112275. [Google Scholar] [CrossRef]
- Wharry, J.P.; Swenson, M.J.; Yano, K.H. A review of the irradiation evolution of dispersed oxide nanoparticles in the b.c.c. Fe-Cr system: Current understanding and future directions. J. Nucl. Mater. 2017, 486, 11–20. [Google Scholar] [CrossRef]
- Deng, L.; Luo, J.-R.; Tu, J.; Hu, R.; Guo, N.; Zeng, W.-Y.; Wang, C.-H.; He, P.; Zhang, Y. Achieving excellent mechanical properties of ODS steel by Y2O3 addition. Mater. Sci. Eng. A 2023, 872, 145008. [Google Scholar] [CrossRef]
- Ribis, J.; Mouton, I.; Baumier, C.; Gentils, A.; Loyer-Prost, M.; Lunéville, L.; Siméone, D. Nano-Structured Materials under Irradiation: Oxide Dispersion-Strengthened Steels. Nanomaterials 2021, 11, 2590. [Google Scholar] [CrossRef]
- Klimenkov, M.; Jäntsch, U.; Rieth, M.; Dürrschnabel, M.; Möslang, A.; Schneider, H.C. Post-irradiation microstructural examination of EUROFER-ODS steel irradiated at 300 °C and 400 °C. J. Nucl. Mater. 2021, 557, 153259. [Google Scholar] [CrossRef]
- McClintock, D.A.; Hoelzer, D.T.; Sokolov, M.A.; Nanstad, R.K. Mechanical properties of neutron irradiated nanostructured ferritic alloy 14YWT. J. Nucl. Mater. 2009, 386–388, 307–311. [Google Scholar] [CrossRef]
- Noell, P.J.; Sills, R.B.; Benzerga, A.A.; Boyce, B.L. Void nucleation during ductile rupture of metals: A review. Prog. Mater. Sci. 2023, 135, 101085. [Google Scholar] [CrossRef]
- Zhao, M.; Zhang, P.; Xu, J.; Ye, W.; Yin, S.; Zhao, J.; Qiao, Y.; Yan, Y. Optimization of microstructure and tensile properties for a 13Cr-1W ODS steel prepared by mechanical alloying and spark plasma sintering using pre-alloyed powder. Mater. Charact. 2024, 207, 113581. [Google Scholar] [CrossRef]
- Cui, Y.; Chen, Z. Void initiation from interfacial debonding of spherical silicon particles inside a silicon-copper nanocomposite: A molecular dynamics study. Model. Simul. Mater. Sci. Eng. 2017, 25, 025007. [Google Scholar] [CrossRef]
- Zhao, Q.Q.; Boyce, B.L.; Sills, R.B. Micromechanics of Void Nucleation and Early Growth at Incoherent Precipitates: Lattice-Trapped and Dislocation-Mediated Delamination Modes. Crystals 2021, 11, 45–65. [Google Scholar] [CrossRef]
- Pogorelko, V.V.; Mayer, A.E. Influence of copper inclusions on the strength of aluminum matrix at high-rate tension. Mater. Sci. Eng. A 2015, 642, 351–359. [Google Scholar] [CrossRef]
- Pogorelko, V.V.; Mayer, A.E. Influence of titanium and magnesium nanoinclusions on the strength of aluminum at high-rate tension: Molecular dynamics simulations. Mater. Sci. Eng. A 2016, 662, 227–240. [Google Scholar] [CrossRef]
- Pogorelko, V.V.; Mayer, A.E. Tensile strength of Al matrix with nanoscale Cu, Ti and Mg inclusions. J. Phys. Conf. Ser. 2016, 774, 012034. [Google Scholar] [CrossRef]
- Pogorelko, V.V.; Mayer, A.E. Tensile strength of Fe–Ni and Mg–Al nanocomposites: Molecular dynamic simulations. J. Phys. Conf. Ser. 2018, 946, 012043. [Google Scholar] [CrossRef]
- Qin, C.; Xu, Z.; Dou, W.; Du, Y.; Huang, F. Plastic flow properties and constitutive model of metallic materials under complex stress states. Explos. Shock. Waves 2022, 42, 091404. [Google Scholar] [CrossRef]
- Wu, Y.; Ciston, J.; Kräemer, S.; Bailey, N.; Odette, G.R.; Hosemann, P. The crystal structure, orientation relationships and interfaces of the nanoscale oxides in nanostructured ferritic alloys. Acta Mater. 2016, 111, 108–115. [Google Scholar] [CrossRef]
- Aydogan, E.; Weaver, J.S.; Carvajal-Nunez, U.; Schneider, M.M.; Gigax, J.G.; Krumwiede, D.L.; Hosemann, P.; Saleh, T.A.; Mara, N.A.; Hoelzer, D.T.; et al. Response of 14YWT alloys under neutron irradiation: A complementary study on microstructure and mechanical properties. Acta Mater. 2019, 167, 181–196. [Google Scholar] [CrossRef]
- Higgins, M.P.; Lu, C.Y.; Lu, Z.; Shao, L.; Wang, L.M.; Gao, F. Crossover from disordered to core-shell structures of nano-oxide Y2O3 dispersed particles in Fe. Appl. Phys. Lett. 2016, 109, 031911. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Hammond, K.D.; Voigt, H.-J.L.; Marus, L.A.; Juslin, N.; Wirth, B.D. Simple pair-wise interactions for hybrid Monte Carlo–molecular dynamics simulations of titania/yttria-doped iron. J. Phys. Condens. Matter 2013, 25, 055402. [Google Scholar] [CrossRef]
- Malerba, L.; Marinica, M.C.; Anento, N.; Björkas, C.; Nguyen, H.; Domain, C.; Djurabekova, F.; Olsson, P.; Nordlund, K.; Serra, A.; et al. Comparison of empirical interatomic potentials for iron applied to radiation damage studies. J. Nucl. Mater. 2010, 406, 19–38. [Google Scholar] [CrossRef]
- Higgins, M.P.; Peng, Q.; Shao, L.; Gao, F. Reduction of defect generation and development of sinks at nanocluster boundary in oxide dispersion-strengthened steel. J. Appl. Phys. 2019, 126, 084302. [Google Scholar] [CrossRef]
- Azeem, M.M.; Wang, Q.; Li, Z.; Zhang, Y. Dislocation-oxide interaction in Y2O3 embedded Fe: A molecular dynamics simulation study. Nucl. Eng. Technol. 2020, 52, 337–343. [Google Scholar] [CrossRef]
- Sun, Y.Q.; Lai, W.S. Molecular dynamics simulations of cascade damage near the Y2Ti2O7 nanocluster/ferrite interface in nanostructured ferritic alloys. Chin. Phys. B 2017, 26, 076106. [Google Scholar] [CrossRef]
- Emelyanova, O.V.; Gentils, A.; Borodin, V.A.; Dzhumaev, P.S.; Vladimirov, P.V.; Lindau, R.; Möslang, A. Microstructural evolution in ODS-EUROFER steel caused by high-dose He ion implantations with systematic variation of implantation parameters. Nucl. Mater. Energy 2023, 35, 101456. [Google Scholar] [CrossRef]
- Fu, J.; Davis, T.P.; Kumar, A.; Richardson, I.M.; Hermans, M.J.M. Characterisation of the influence of vanadium and tantalum on yttrium-based nano-oxides in ODS Eurofer steel. Mater. Charact. 2021, 175, 111072. [Google Scholar] [CrossRef]
- Bergner, F.; Hlawacek, G.; Heintze, C. Helium-ion microscopy, helium-ion irradiation and nanoindentation of Eurofer 97 and ODS Eurofer. J. Nucl. Mater. 2018, 505, 267–275. [Google Scholar] [CrossRef]
- Zilnyk, K.D.; Pradeep, K.G.; Choi, P.; Sandim, H.R.Z.; Raabe, D. Long-term thermal stability of nanoclusters in ODS-Eurofer steel: An atom probe tomography study. J. Nucl. Mater. 2017, 492, 142–147. [Google Scholar] [CrossRef]
- Klimenkov, M.; Lindau, R.; Jäntsch, U.; Möslang, A. Effect of irradiation temperature on microstructure of ferritic-martensitic ODS steel. J. Nucl. Mater. 2017, 493, 426–435. [Google Scholar] [CrossRef]
- Rogozhkin, S.; Bogachev, A.; Korchuganova, O.; Nikitin, A.; Orlov, N.; Aleev, A.; Zaluzhnyi, A.; Kozodaev, M.; Kulevoy, T.; Chalykh, B.; et al. Nanostructure evolution in ODS steels under ion irradiation. Nucl. Mater. Energy 2016, 9, 66–74. [Google Scholar] [CrossRef]
- Rogozhkin, S.V.; Aleev, A.A.; Zaluzhnyi, A.G.; Nikitin, A.A.; Iskandarov, N.A.; Vladimirov, P.; Lindau, R.; Möslang, A. Atom probe characterization of nano-scaled features in irradiated ODS Eurofer steel. J. Nucl. Mater. 2011, 409, 94–99. [Google Scholar] [CrossRef]
- Aleev, A.A.; Iskandarov, N.A.; Klimenkov, M.; Lindau, R.; Möslang, A.; Nikitin, A.A.; Rogozhkin, S.V.; Vladimirov, P.; Zaluzhnyi, A.G. Investigation of oxide particles in unirradiated ODS Eurofer by tomographic atom probe. J. Nucl. Mater. 2011, 409, 65–71. [Google Scholar] [CrossRef]
- Stukowski, A. Visualization and Analysis of Atomistic Simulation Data with OVITO—The Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2010, 18, 015012. [Google Scholar] [CrossRef]
- Wang, Y.-T.; Zeng, X.-G.; Yang, X. Molecular dynamics simulation of effect of temperature on void nucleation and growth of single crystal iron at a high strain rate. Acta Phys. Sin. 2019, 68, 246102. [Google Scholar] [CrossRef]
- Yeheskel, O.; Tevet, O. Elastic Moduli of Transparent Yttria. J. Am. Ceram. Soc. 1999, 82, 136–144. [Google Scholar] [CrossRef]
- Simmons, G.; Wang, H.F. Single Crystal Elastic Constants and Calculated Aggregate Properties: A Handbook; M.I.T. Press: Cambridge, MA, USA, 1971. [Google Scholar]
- Rayne, J.A.; Chandrasekhar, B.S. Elastic Constants of Iron from 4.2 to 300 K. Phys. Rev. 1961, 122, 1714–1716. [Google Scholar] [CrossRef]
- Tvergaard, V.; Needleman, A. Analysis of the cup-cone fracture in a round tensile bar. Acta Metall. 1984, 32, 157–169. [Google Scholar] [CrossRef]
- Dighe, M.D.; Gokhale, A.M.; Horstemeyer, M.F. Effect of loading condition and stress state on damage evolution of silicon particles in an Al-Si-Mg-base cast alloy. Metall. Mater. Trans. A-Phys. Metall. Mater. Sci. 2002, 33, 555–565. [Google Scholar] [CrossRef]
- Shabrov, M.N.; Needleman, A. An analysis of inclusion morphology effects on void nucleation. Model. Simul. Mater. Sci. Eng. 2002, 10, 163–183. [Google Scholar] [CrossRef]
- Marino, B.; Mudry, F.; Pineau, A. Experimental study of cavity growth in ductile rupture. Eng. Fract. Mech. 1985, 22, 989–996. [Google Scholar] [CrossRef]
- Bourcier, R.J.; Koss, D.A.; Smelser, R.E.; Richmond, O. The influence of porosity on the deformation and fracture of alloys. Acta Metall. 1986, 34, 2443–2453. [Google Scholar] [CrossRef]
- Kumar, S.; Das, S.K. Characterization of mechanical properties and nano-porous structure of Aluminium-Magnesium alloy during multi-axial tensile deformation: An atomistic investigation. J. Alloys Compd. 2018, 740, 626–638. [Google Scholar] [CrossRef]
- Mock, M.; Albe, K. Modelling of dislocation-solute interaction in ODS steels: Analytic bond-order potential for the iron-yttrium system. J. Nucl. Mater. 2018, 509, 102–113. [Google Scholar] [CrossRef]
- Yashiro, K.; Yamaguchi, A.; Tanaka, M.; Okuda, T.; Koga, K.; Segi, T. Molecular Dynamics Simulation on Interaction between Screw Dislocation and Pseudo Yttrium Oxide in Bcc-Fe. Mater. Trans. 2012, 53, 401–406. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, Z.; Sun, Y.; Hu, Y.; Peng, L.; Shi, J.; Shi, Y.; Chen, S.; Ma, Y. Influence of Yttria Nanoclusters on the Void Nucleation in BCC Iron During Multi-Axial Tensile Deformation: A Molecular Dynamics Simulation. Crystals 2025, 15, 476. https://doi.org/10.3390/cryst15050476
Wei Z, Sun Y, Hu Y, Peng L, Shi J, Shi Y, Chen S, Ma Y. Influence of Yttria Nanoclusters on the Void Nucleation in BCC Iron During Multi-Axial Tensile Deformation: A Molecular Dynamics Simulation. Crystals. 2025; 15(5):476. https://doi.org/10.3390/cryst15050476
Chicago/Turabian StyleWei, Zhenyu, Yongjie Sun, Yeshang Hu, Lei Peng, Jingyi Shi, Yifan Shi, Shangming Chen, and Yiyi Ma. 2025. "Influence of Yttria Nanoclusters on the Void Nucleation in BCC Iron During Multi-Axial Tensile Deformation: A Molecular Dynamics Simulation" Crystals 15, no. 5: 476. https://doi.org/10.3390/cryst15050476
APA StyleWei, Z., Sun, Y., Hu, Y., Peng, L., Shi, J., Shi, Y., Chen, S., & Ma, Y. (2025). Influence of Yttria Nanoclusters on the Void Nucleation in BCC Iron During Multi-Axial Tensile Deformation: A Molecular Dynamics Simulation. Crystals, 15(5), 476. https://doi.org/10.3390/cryst15050476





