During the process of creating dispersed structures, the surface endoenergetic effect associated with the creation of a free α phase surface ΔE
s = E
s− a
As,max/a
Ab is balanced by the sum of the exoenergetic effects ΔG
b + ΔG
2D (
Figure 1). The creation of two-dimensional structures on the resulting surface, as an exothermic process, can be represented as the vector ΔG
2D. Thus, the sum of energies associated with surface transformations can be expressed as ΔG
s = ΔE
s − ΔG
2D. Both the free enthalpy of the α solution formation ΔG
b and the free enthalpy of creating 2D structures ΔG
2D are functions of the chemical potential of the gas component. As a result of establishing equilibrium in the dispersion process, smaller crystallites are formed with a specific active surface area under constant formation conditions of temperature and chemical potential of component B.
2.1. Kinetics of the Reaction in Solid Solution
Based on the literature data [
31], the actual diffusion coefficients for carbon, nitrogen, and oxygen in metals in the temperature range of 200–500 °C are within the range of 10
−14–10
−12 m
2/s. A constant value of the diffusion coefficient in the volume of the sorbate nanocrystallite D = 10
−13 m
2/s was assumed for model calculations.
In order to determine the effect of the diffusion rate in the volume of nanocrystallite on the process rate in a solution with unlimited solubility of absorbate, model calculations of concentrations in the volume of a spherical nanocrystallite (with radius r
max in spheres with different radii r ∈ (0, r
max>) at constant gas-phase potential and surface concentration of adsorbent were performed using the equation proposed by Crank [
1] and here modified to the following form:
where t—time, x
b—molar concentration of absorbate, and n—number of spheres in nanocrystallite (assumed n = r
max/
a),
a—lattice constant.
Figure 2 shows the results for crystallites of various sizes at a constant molar concentration of adsorbate on the surface for a defined, constant maximum potential P
max, assuming that ΔG
seg = 0.
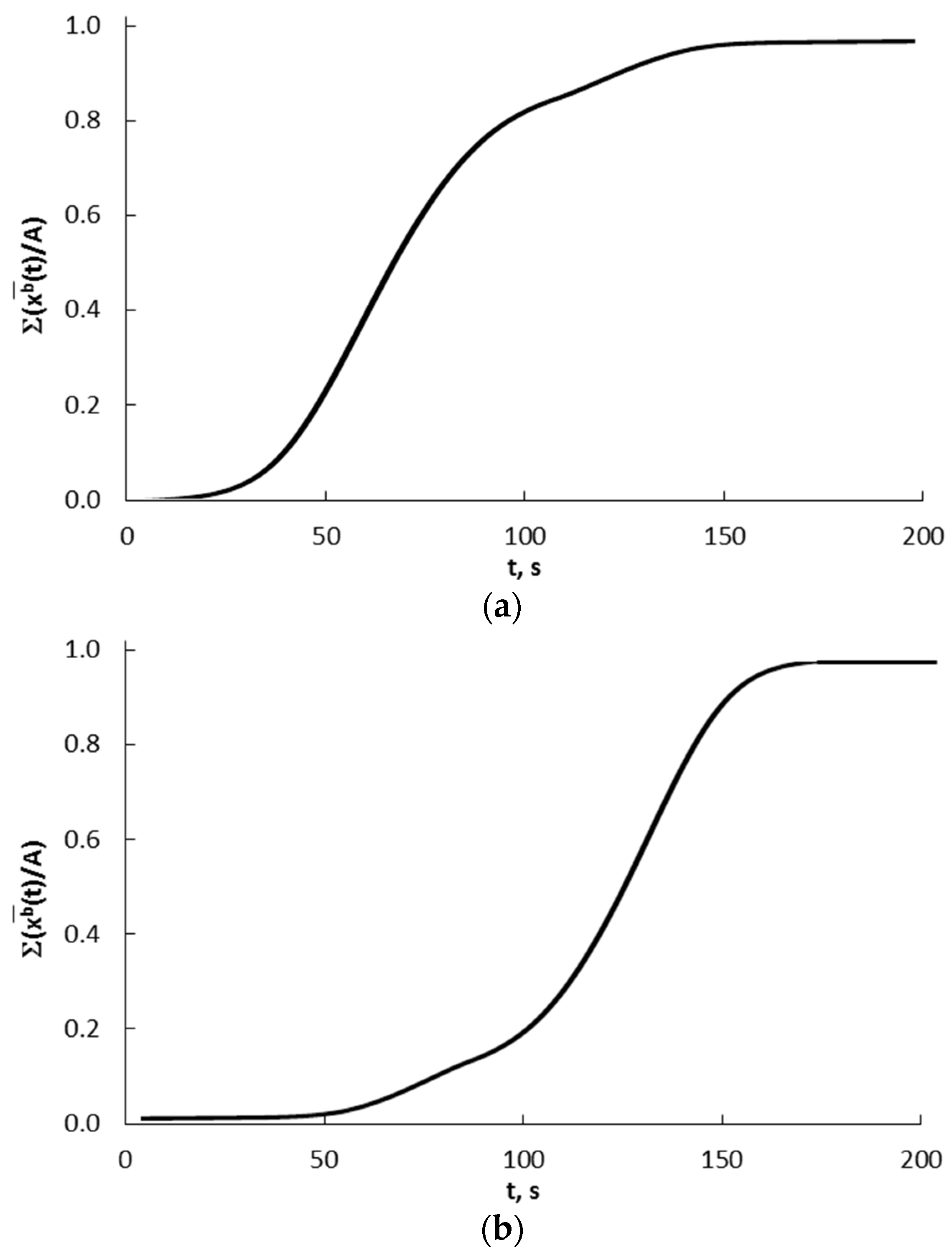
From
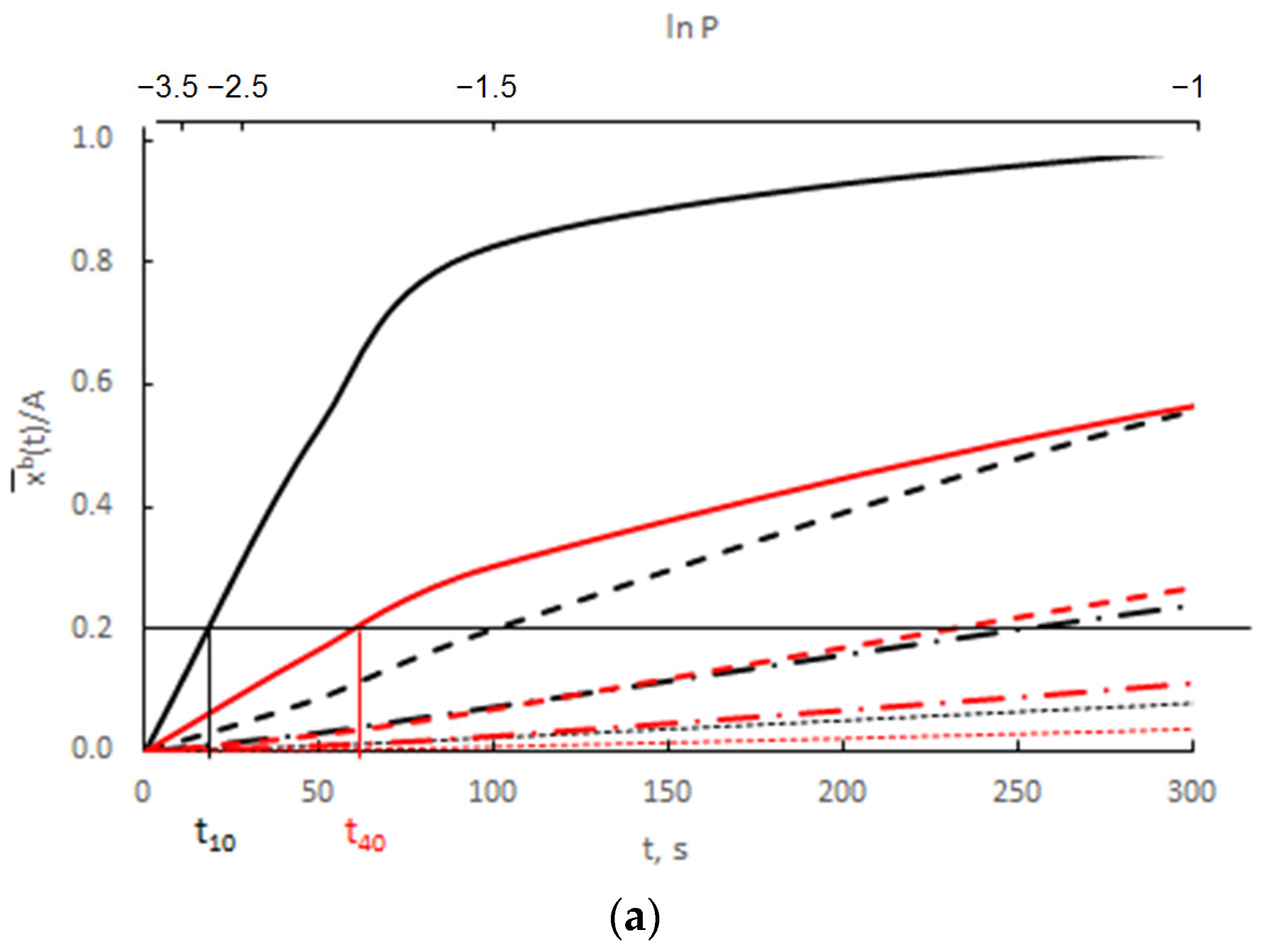
Figure 2a it can be concluded that for nanocrystallites of r = 40 nm, concentrations within their whole volume equilibrate and reach maximum values in less than 1 s (red, dashed line in
Figure 2a). If we more precisely consider the process of equilibration of those concentrations in nanocrystallites of r = 40 nm (
Figure 2b), we may state (based on model calculation results) that the time needed to equilibrate the concentration in the volume of such nanocrystallite is 0.01 s (red, dashed line in
Figure 2b).
That model value r = 40 nm is based on the results of our measurements using scanning electron microscopy. According to these results, the largest iron nanocrystallites in the tested samples had diameters of approximately 80 nm. Our intention is to determine the values of characteristic parameters primarily for the largest nanocrystallites as those representing the end of the chemical reaction—if these large nanocrystallites undergo a phase transformation over time, smaller ones will undergo this transformation faster.
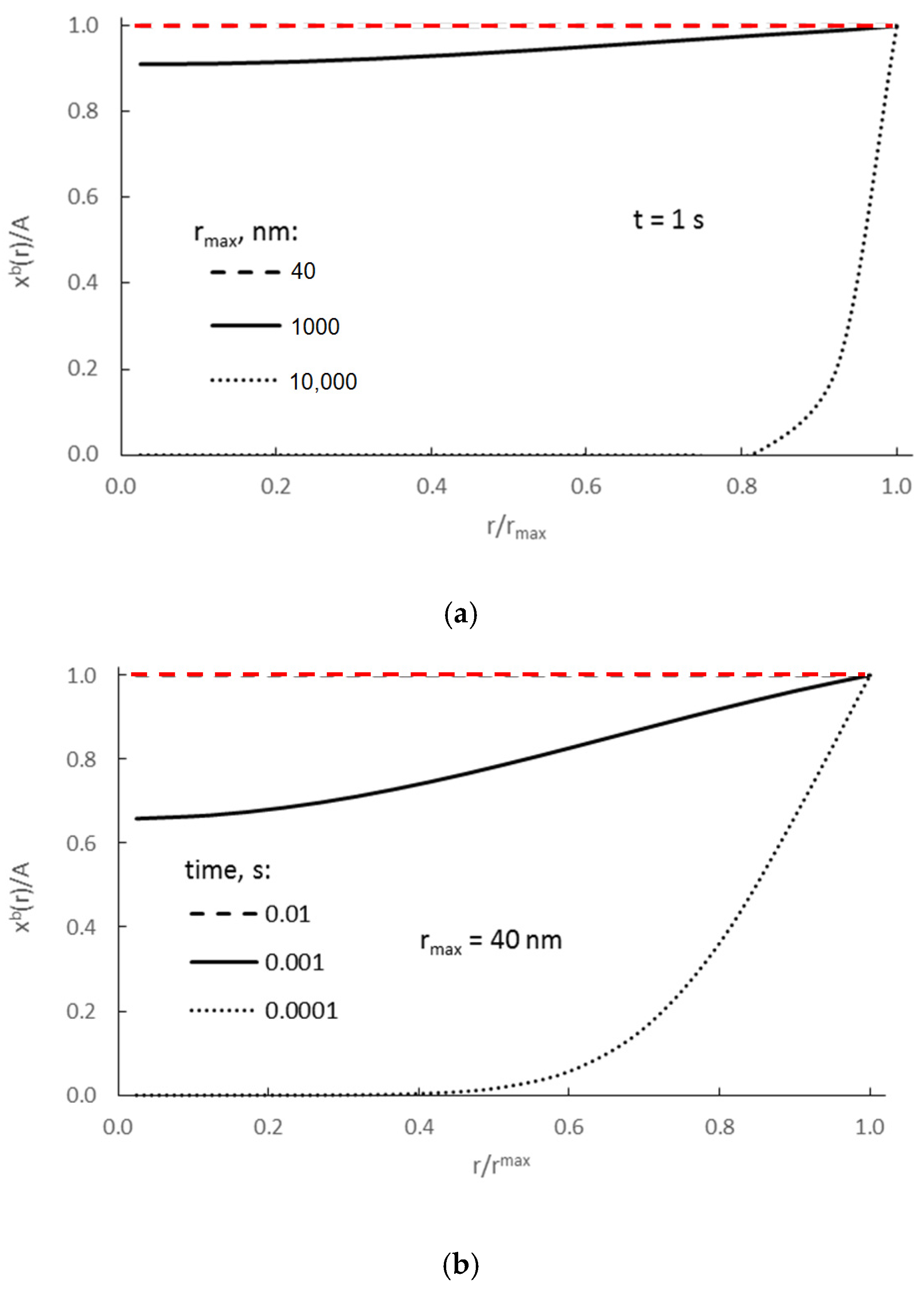
Based on the assumptions made so far, the schematic dependence of the absorbate concentration in nanocrystallite volume x
b on the process time and nanocrystallite radius was presented at a constant surface concentration of adsorbate x
s and with a diffusion coefficient of D = 10
−19 m
2/s at the interface composed of single layer of atoms of solid phase (
Figure 3).
The surface concentration will be greater than the equilibrium concentration determined by the equilibrium between the surface area and the volume of the nanocrystallite. The recrystallization of the substrate phase to the product phase will take place after reaching the average surface and volume concentration of sorbate in the nanocrystallite, corresponding to the critical concentration in its entire volume. The rate at which the nanocrystallite saturates depends on the difference in surface concentration and the concentration in volume of the nanocrystallite.
In the solid phase, a constant level of absorbate concentration is quickly established at a constant maximum during the experiment at the adsorbate potential in the gas phase Pmax.
Extensive research on diffusion and a thorough arrangement of various equations and models describing this process can be found in the work [
32]. This work extends the scope of application of the presented models to nonlinear multicomponent diffusion, taking into account the kinetics of the chemical reactions in various states of matter. In the current work, we focused on processes involving chemical reactions in the solid phase, which is an attempt to answer one of the questions posed in the conclusions by the authors of the work [
32] on diffusion and chemical reactions in such systems. Additionally, we assumed that the rate-limiting step of the process is the passage of atoms adsorbed on the surface into the volume of iron nanocrystallites through a single surface layer of iron atoms (the diffusion of the dissolved component in the volume of nanocrystallites is much faster and a product layer is not formed on the disappearing core as in the shrinking core model) [
33].
The average molar concentration of a substance dissolved in the entire volume of nanocrystallite
as a function of time was calculated using Equation proposed by Crank [
1]:
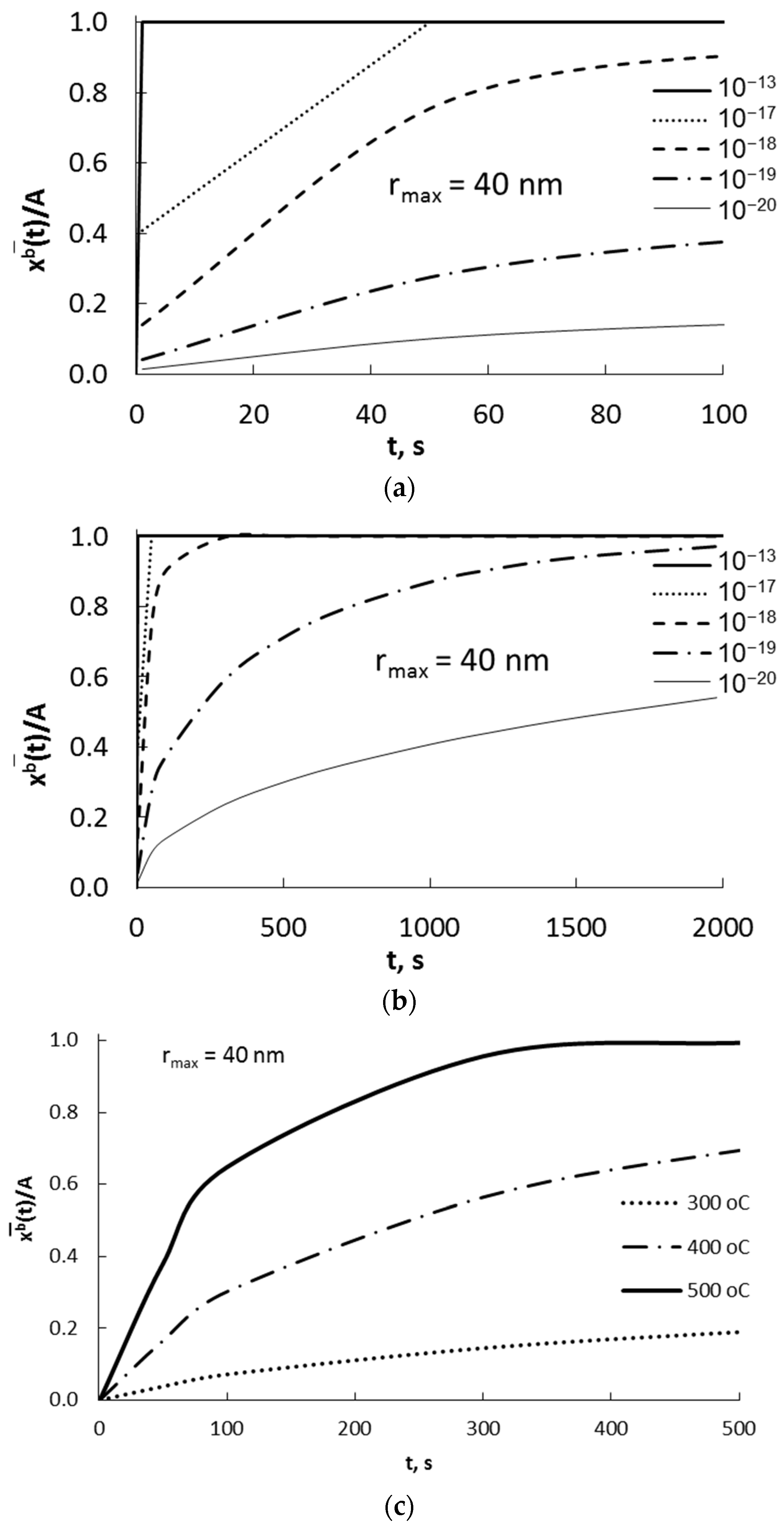
Using Equation (11), the dependence of the average molar concentration of absorbate in a nanocrystallite with a radius of 40 nm on the time with diffusion coefficients D = 10
−20–10
−13 m
2/s is presented in
Figure 4a,b and for different temperatures in
Figure 4c.
It was found that for a diffusion coefficient of 10
−13 m
2/s (typical for coarse-crystalline materials) in nanocrystalline materials, the concentration of absorbate and the concentration of adsorbate on the surface of the nanocrystallite were equalized in a very short period of time (
Figure 4a). For a diffusion coefficient of 10
−19 m
2/s, the steady state absorbate concentration was reached over a period of about 2000 s (
Figure 4b).
A significant difference in the values of diffusion coefficients leads to the conclusion that the stage limiting the rate of the process is the rate of diffusion of the adsorbate from the surface across the phase interface into the nanocrystallite volume. The adsorbate must overcome not only the energy barrier related to the vibrations of the lattice but also the barrier related to the dissolution of the absorbate in the crystallite volume. Equation (1) will change its form:
where E
a—activation energy of diffusion through the crystallite surface, and ΔG
dis = −ΔG
seg—enthalpy of dissolution of the adsorbate in the volume of nanocrystallite.
The effect of the change in E
a + ΔG
dis and the temperature on the change in concentration over time is shown in
Figure 4b.
The rate of the chemical reaction between the gaseous substance and one nanocrystalline solid of α phase can be described taking into account the influence of three parameters on the course of the chemical reaction: temperature, chemical potential of the gas phase, and nanocrystallite size distribution:
For a single nanocrystallite, the reaction rate can be described as:
From Equations (12) and (13) and taking into account Equation (14), we obtain:
The measured reaction rate is the sum of the process rates on individual i-th nanocrystallites in the sample:
The actual system consists of a set of crystallites described by the probability density distribution of their sizes characterized by the specific surface area of the crystallite A = S/V. It was assumed in this paper that this is a bimodal distribution consistent with experimental tests [
25,
26,
28,
29,
30] and model calculations [
29,
30] for the nanocrystalline iron-ammonia-hydrogen system.
The dependence of the total concentration of the absorbate in the volume of sample of nanocrystallites x
b on temperature, chemical potential of the gas phase, and crystallite size can be described by continuous functions as follows:
In the area of one α phase and at constant temperature as well as at constant potential of the gas phase, the averaged reaction rate is as follows:
where C
1, and C
2—constants taking into account the influence of temperature and gas-phase potential on the reaction rate.
2.3. Thermodynamics and Kinetics of the System with Phase Transformation (Two-Phase System)
With the chemical potential of the adsorbate in the gas phase P
0(A)
i and depending on the specific active surface area of the i-th nanocrystallite at the critical concentration, x
b,cri, the i-th nanocrystallite of the α phase undergoes a phase change α→β in its entire volume. At this potential, the phase β is formed with the concentration x
ib,β,min and the maximum concentration of defects x
Dmax (
Figure 6). The defect concentration is equal to the difference between the maximum absorbate concentration in the β phase and the minimum absorbate concentration in this phase after the transition from the α phase:
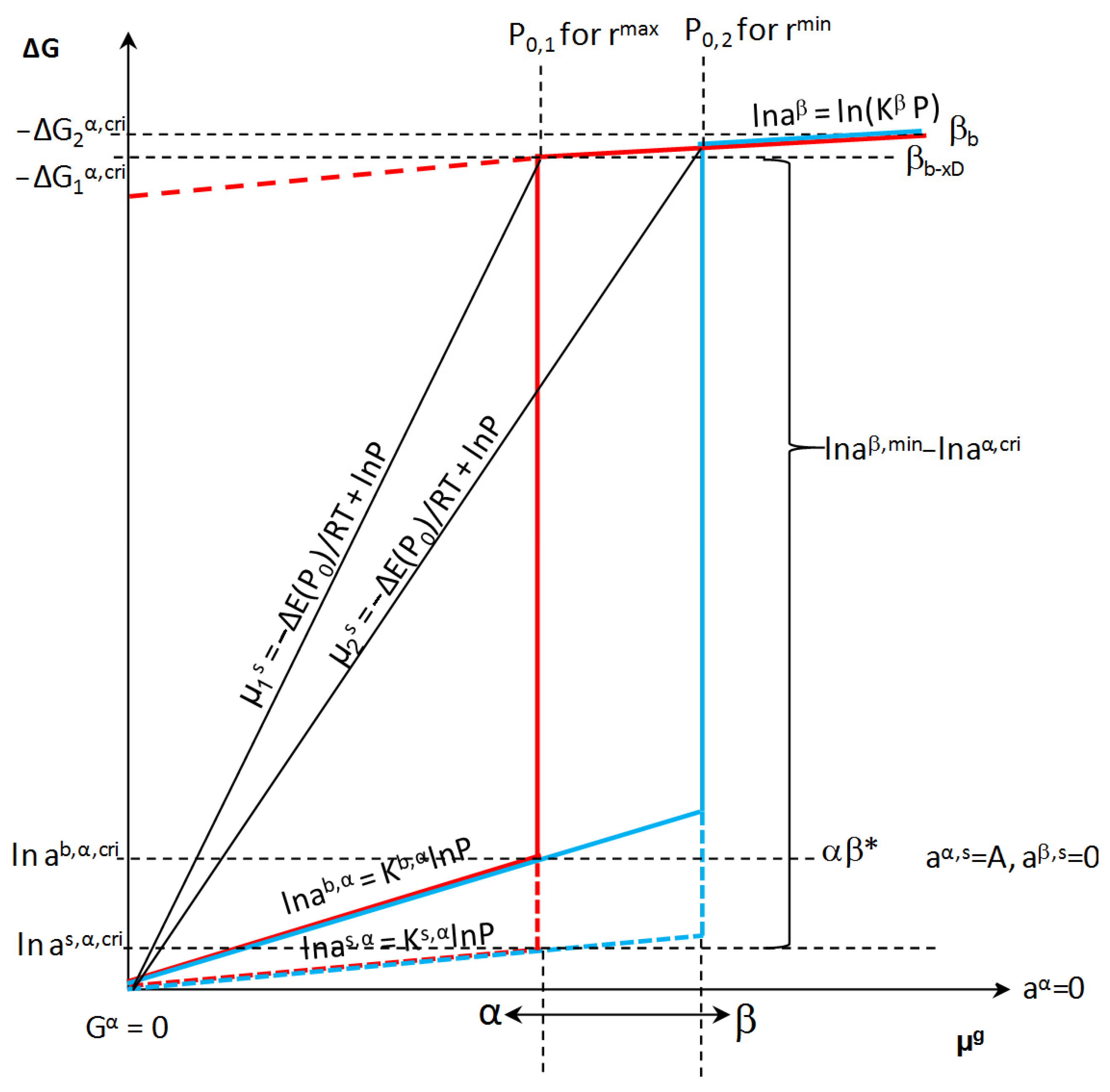
At constant chemical potentials and after an infinitely long time, the state of chemical equilibrium is established, characterized by a specific equilibrium potential of the gas phase, activities in the solid phase on the surface and in the volume, process temperature, and specific active surface (
Figure 6). The phase transformation of the α phase occurs when the critical values are taken into account in Equation (3):
where a
s,cri—critical activity at the surface, a
b,cri—critical activity in crystallite volume, and P
0—equilibrium potential of the gas phase.
Assuming that the reference conditions are the process conditions (constant temperature and zero chemical potential of the gas phase), the change in free enthalpies can be replaced by the values of free enthalpies of sorbate in the individual phases:
For small concentrations, a simplification can be used:
The ratio of the equilibrium constants under phase transition conditions is as follows:
According to the balance (26), the phase transformation takes place at a constant energy of the system, i.e., the sum of the free enthalpies: of deformation of the crystal lattice in the
α phase volume in the absorption process Δ
Gα→β, of a component dissolved in the nanocrystallite volume ΔG
b, of a chemisorbed component on the nanocrystallite surface ΔG
s; and the change in surface energy of nanocrystallite ΔE is equal to zero and Equations (26)–(28) are also satisfied [
29]:
For low gas-phase potentials:
Due to the interactions between the atoms adsorbed on the adsorbent surface, for high concentrations of the adsorbate, Equation (28) will take the form:
The change in surface energy at high adsorbate concentrations is expressed as:
wherein
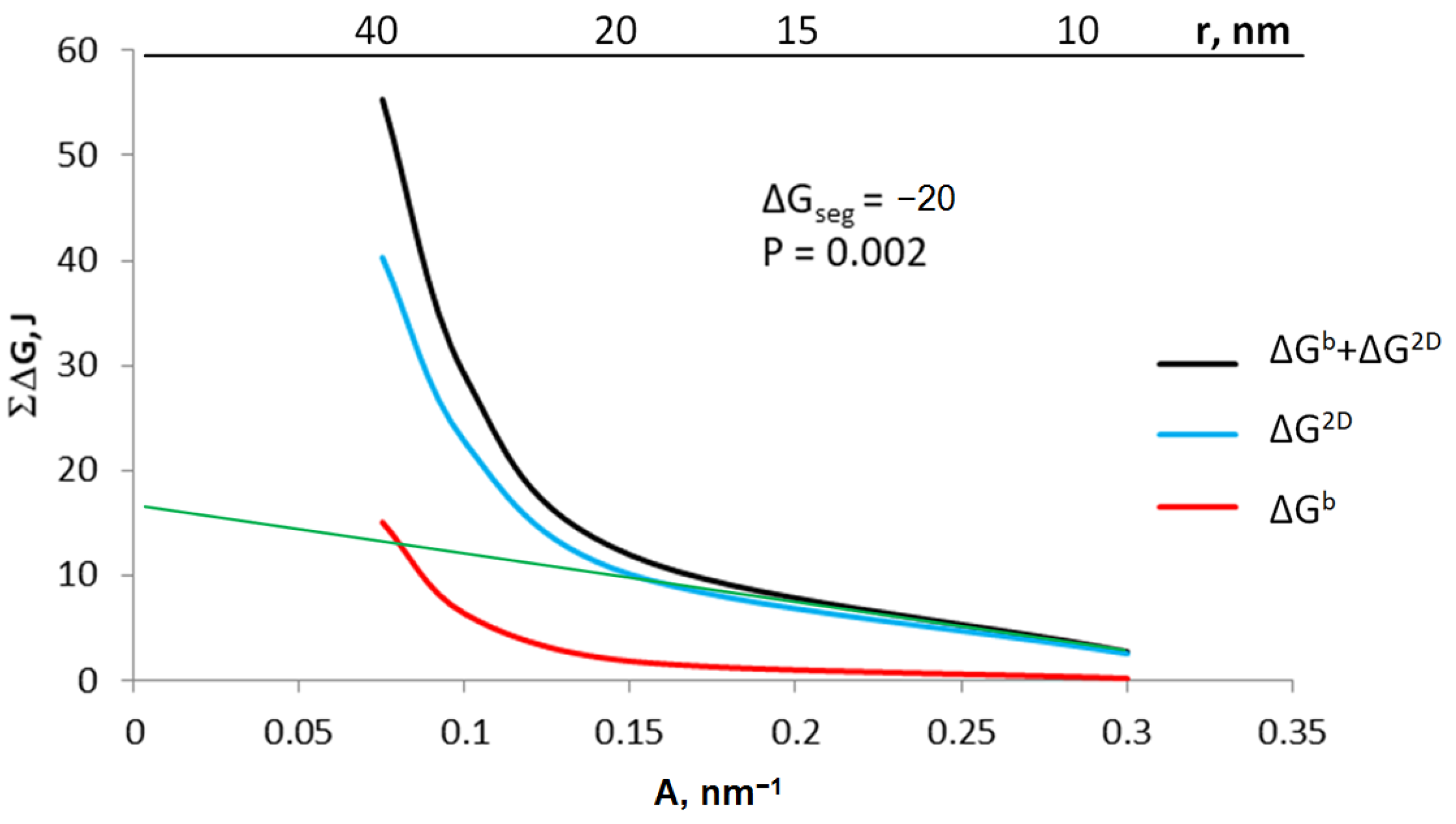
Based on the Equation (8), the sum of ΔG
b + ΔG
2D was calculated for crystallites with specific active surfaces in the range of 0.075–0.300 nm
−1 (
Figure 7). The graph also shows the values of ΔG
2D and ΔG
b for individual crystallites calculated on the basis of the Equations (4b) and (5), respectively, with a gas-phase potential of 0.002 and segregation free enthalpy ΔG
seg = −20 kJ.
The proportion of free enthalpy related to the crystallite surface of the total free enthalpy of the system is 97% for a crystallite with a radius of 20 nm. Thus, it can be assumed that smaller crystallites can be treated as nanocrystallites, and larger crystallites will have properties corresponding to coarse-crystalline substances. Based on Equation (6d), knowing the enthalpy balance for at least two crystallites and extrapolating the obtained values to the conditions of coarse-crystalline substances (green line in
Figure 7), it is possible to determine the value of the enthalpy ΔE
s related to the process of coarse-crystalline substance dispersion.
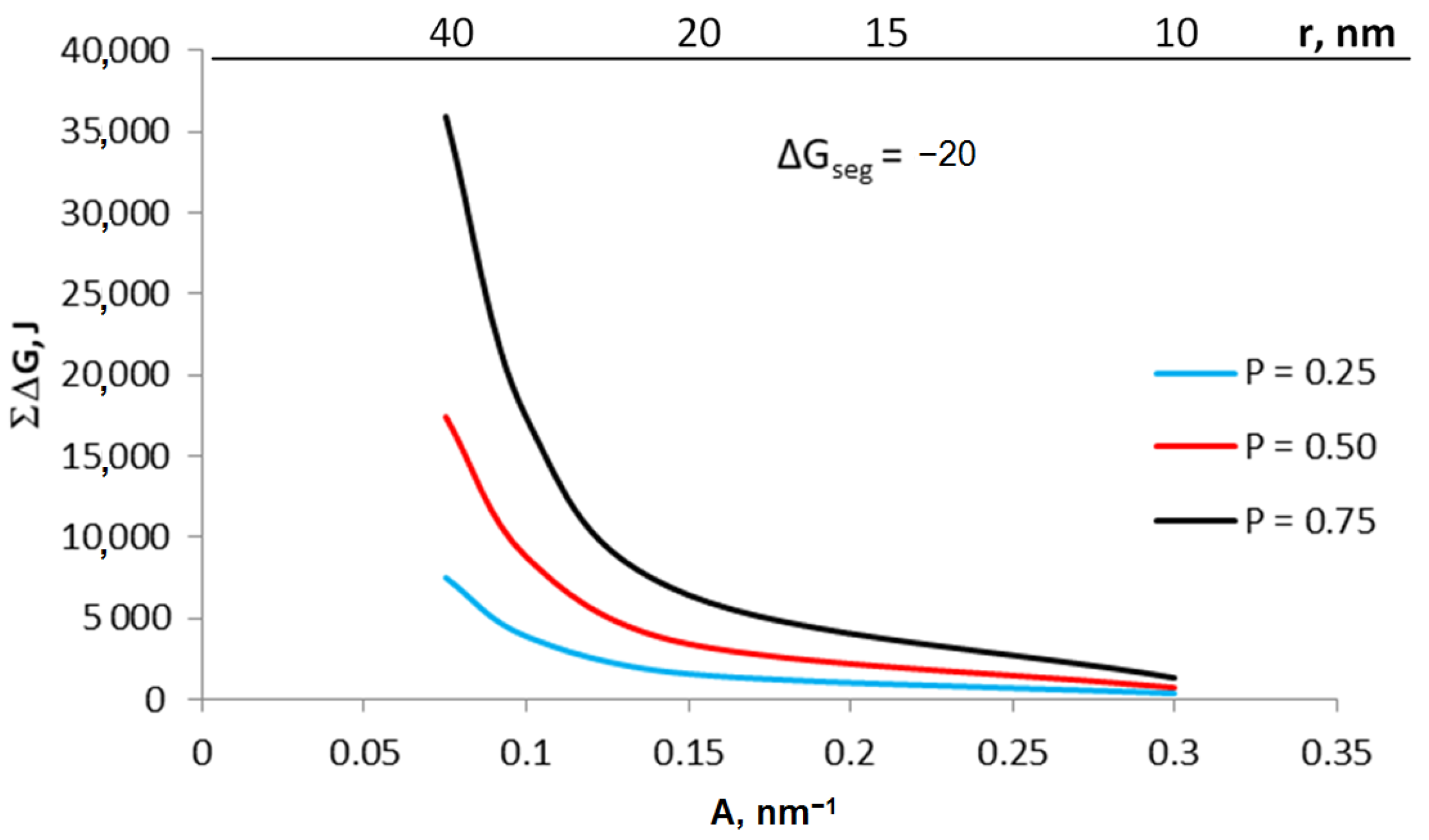
The values of the expression ΔG
b + ΔG
2D were determined on the basis of Equation (8) for crystallites with specific active surfaces in the range of 0.075–0.300 nm
−1 (
Figure 8) with a gas-phase potential in the range of 0.25–0.75 and segregation free enthalpy ΔG
seg = −20 kJ.
For crystallites with different A, the expression ΔG2D/ΔGb will have a different value: the smaller the value of A, the smaller the ratio of ΔG2D/ΔGb. As a result of establishing equilibrium, smaller crystallites are transformed under the higher chemical potential of the gas components rather than larger crystallites.
In terms of surface tension, usually the joules (J) per square meter of surface area (m
2) unit is used [
34,
35]. According to the definitions adopted in this work, unit J/mol of surface [
36] is used for the molar chemical potential of the surface where the surface area is expressed in terms of mole of surface atoms which corresponds to the usual unit for surface tension. The energy for creating a clean surface ΔE
s is then given by:
where E
s−—molar chemical potential of free surface, and f—activity coefficient.
The total surface energy ΣΔE
As, defined for a specific surface and volume of crystallite and taking into account the fraction of free and occupied crystallite surface, is a function of surface concentration adsorbate and is equal to [
27]:
where E
s−ad—molar chemical potential of occupied surface.
After substituting Equations (6d), (7) and (32):
This balance applies to a crystallite and only to the extent of restructuring its surface. When as,max is changed, the active specific surface area changes despite the constant xb. Equation (34) represents the minimum system energy at a given volume of crystallites and their specific surface.
The dependence of the energy change needed for the phase transformation for the S/V value of the i-th crystallite on the potential can be approximated by a linear function:
When ΔG
seg is less than or greater than zero, according to the McLean–Langmuir equation at equilibrium, x
smax will be different from x
bmax, and moreover, these concentrations will be a function of the crystallite radius and the potential P
0α,max(A
i):
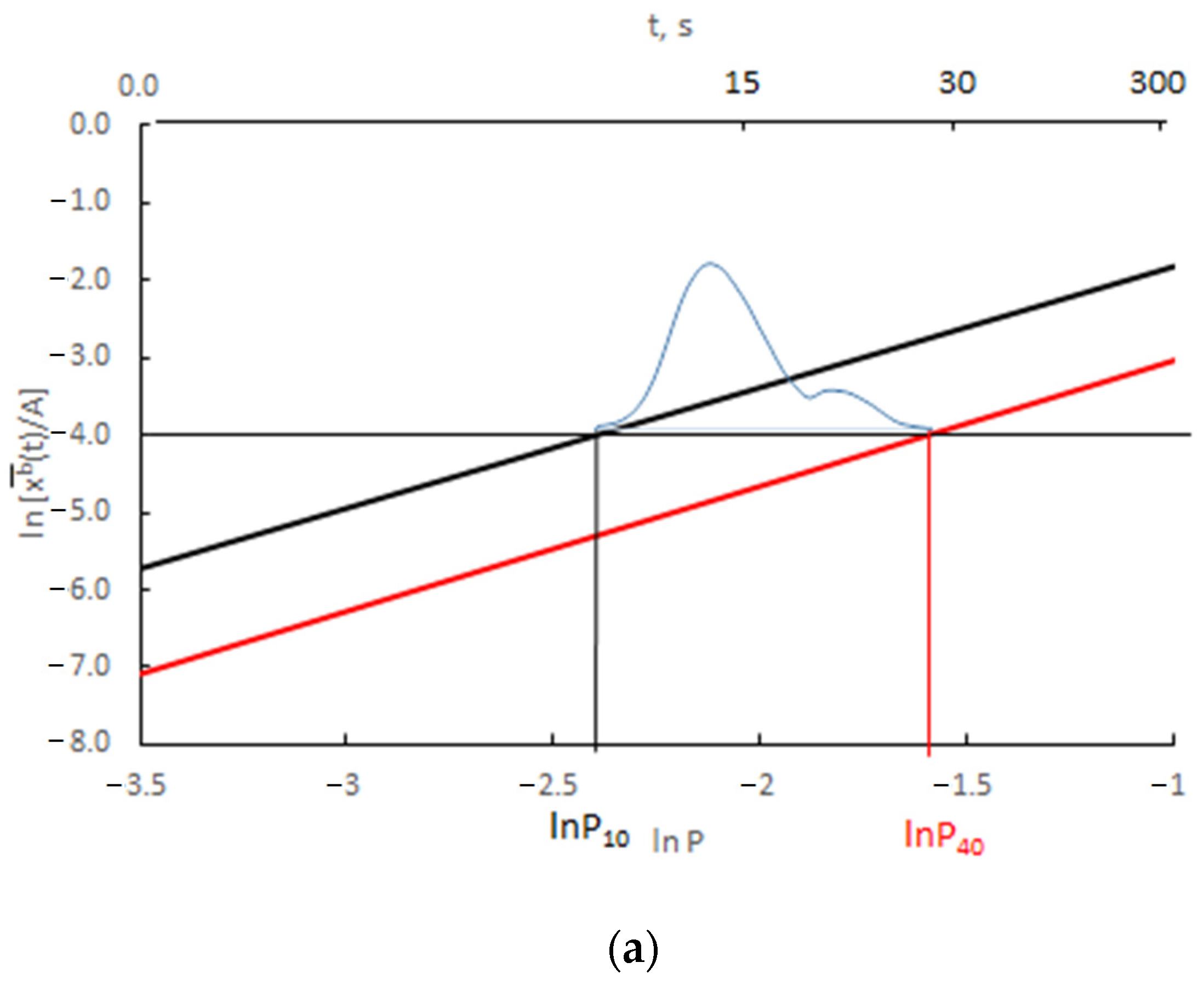
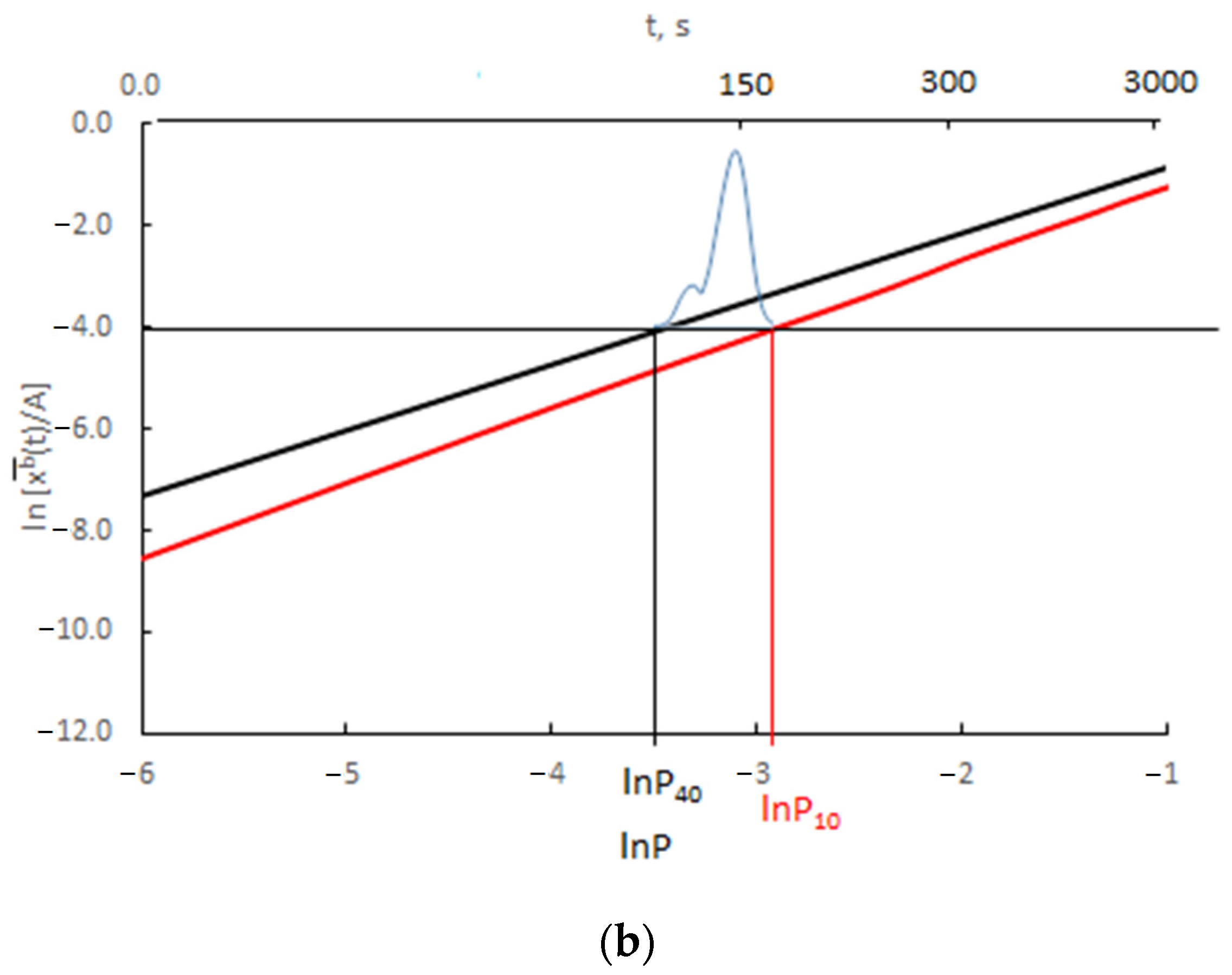
After calculating the logarithm of the maximal activities of the absorbate and the adsorbate in the α phase, the value of the free segregation enthalpy ΔGsegα can be determined for each crystallite in the system. In the coordinate system ln = f(ln) = f(lnP0max), the relation / is a line with slope Kα,ab.
If we consider a system containing nanocrystallites with specific active surfaces A
i ∈ <A
min–A
max>, nanocrystallites will undergo phase transformation in their entire volume at the potentials P
0(A)
i ∈ <P
0(A
min)–P
0(A
max)>. The value of ΔE is directly proportional to the specific active surface area, and therefore, the fractions of larger crystallites will undergo a phase change at lower P
0(A
i) potentials and smaller x
s and x
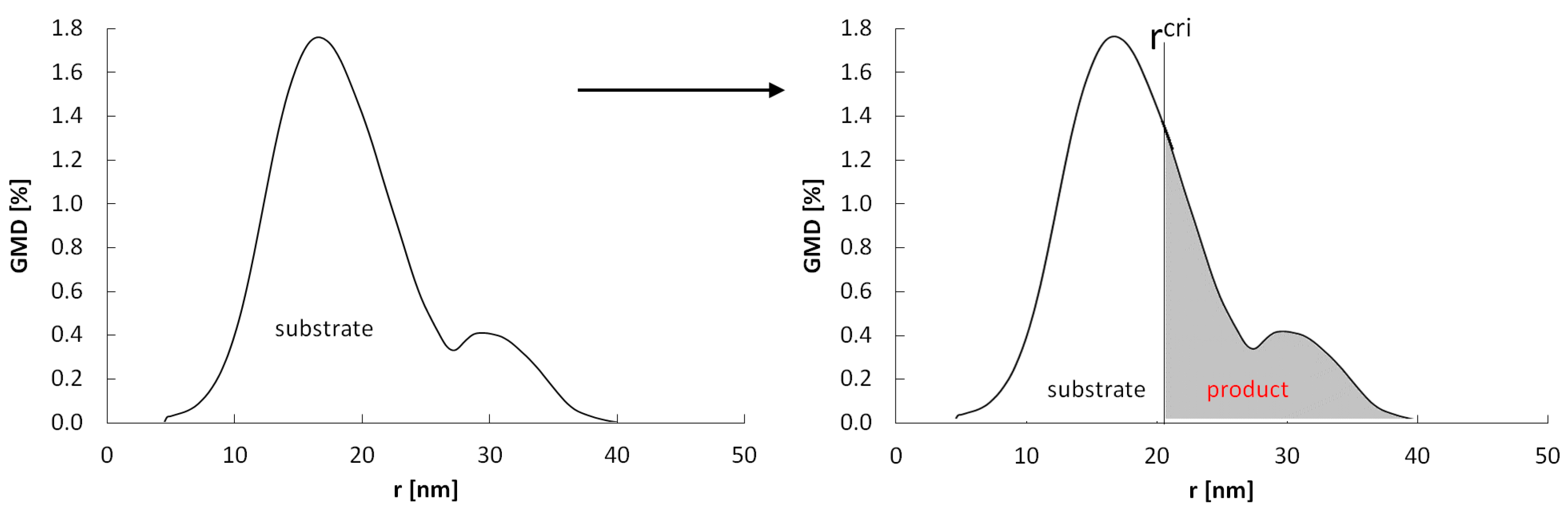
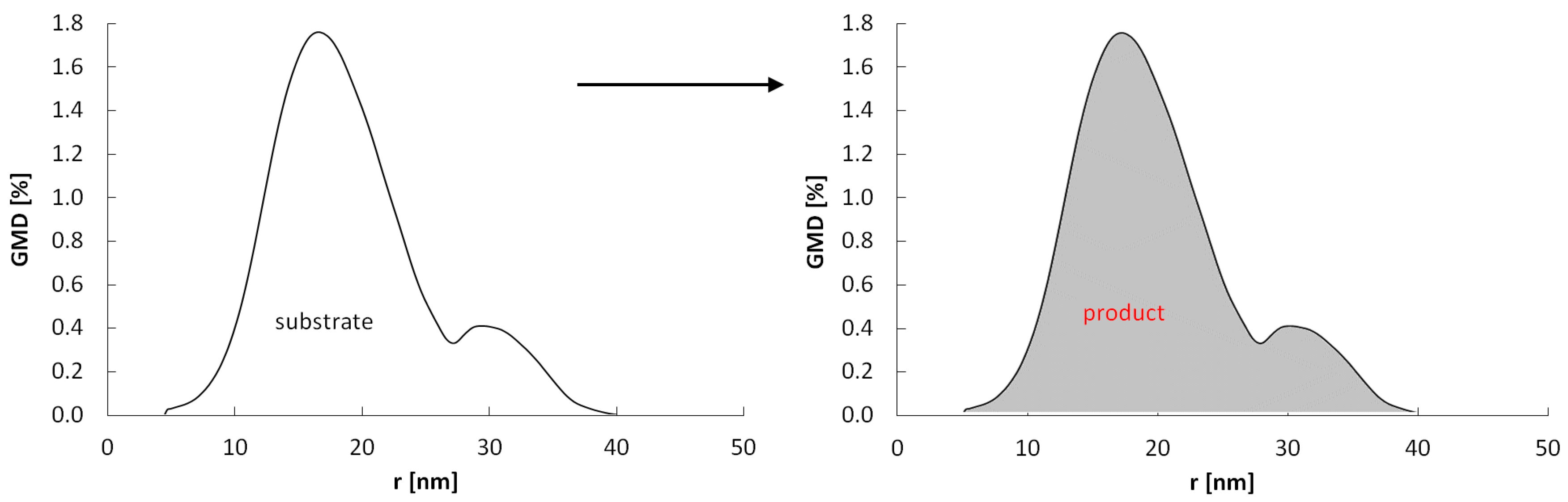
b concentrations than smaller crystallites (
Figure 9)—in equilibrium states established with increasing gas-phase potential, crystallites will reach maximum concentrations and undergo phase changes in the order of their radius from the largest to the smallest, and the α and β phase crystallites will be present in the sample next to each other at a specific potential P
0(A
i) ≈ P
0(A
min).
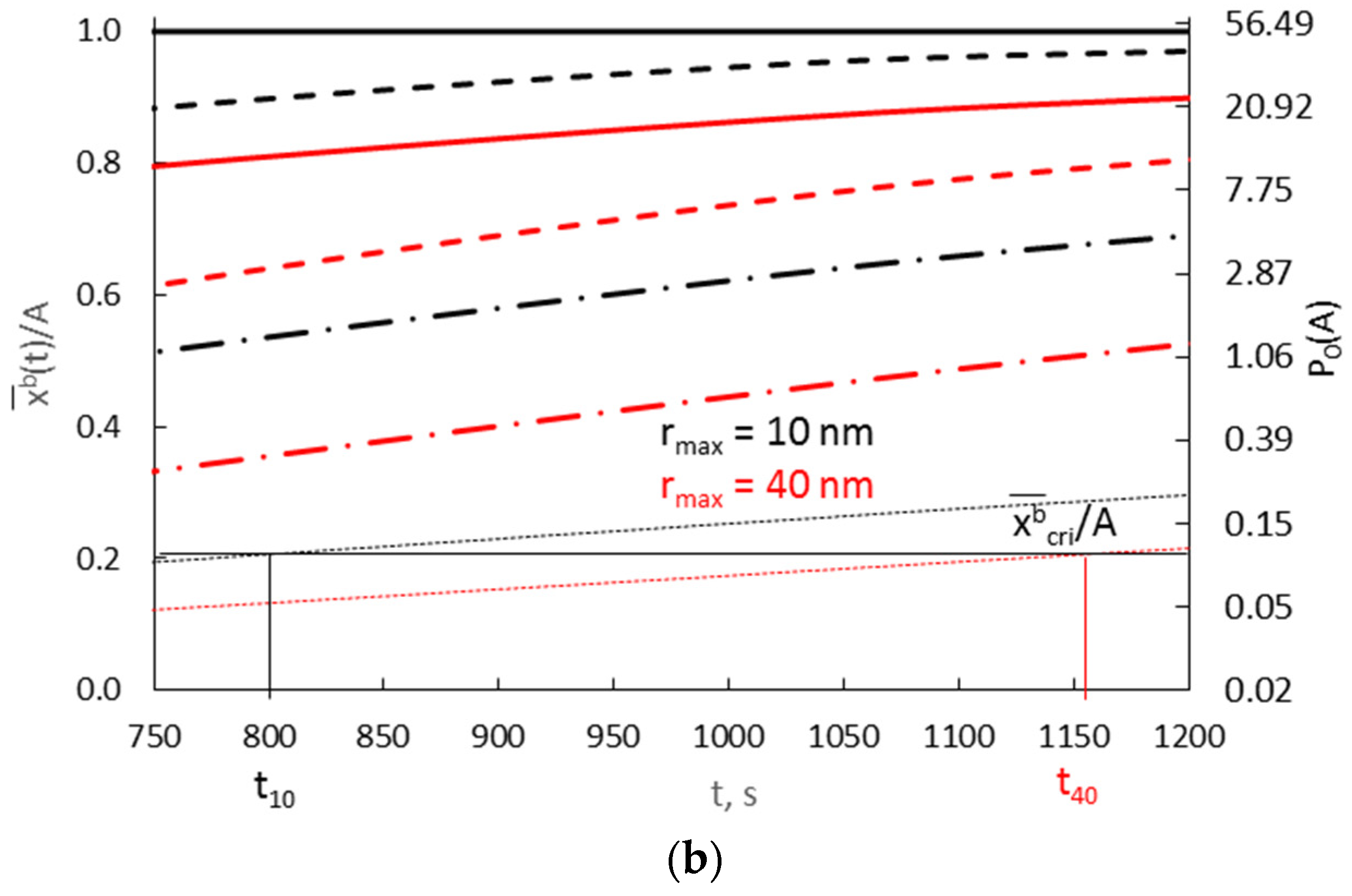
With (P(t) − P
0(A
min) → 0, the rate of crystallite conversion with r
max = 10 nm is lower and occurs at higher gas-phase potentials than the crystallite conversion rate with r
max = 40 nm. The chemical potential at which phase transformation of i-th crystallite occurs can be expressed by the relationship:
Under the conditions where the potential P(t) >> P
0(A
min), all crystallites can undergo a phase change (
Figure 10) and the rate of crystallite conversion with r
max = 10 nm is higher and occurs at lower gas-phase potentials than the crystallite conversion rate with r
max = 40 nm.
Taking into account the phase transformation and the formation of the second nanocrystalline phase β for a single nanocrystallite, the total average reaction rate can be expressed by the equation:
After the phase transition, the concentration difference is expressed only by the second component of the right side of the Equation (38).
2.5. Process in the Isothermal System and the Exponential Relationship P = f (t)
Assuming that the adsorbate concentration in the gas phase x
g(t) varies according to the leaching model with perfect mixing:
where v—gas flow rate, V
r—reactor volume, x
0g—maximum concentration in the gas phase, and the adsorbate surface concentration changes over time according to Equation (3a).
Any changes in absorbate concentration x
b as a function of radius, when the concentration changes in adsorbate are described by Equations (3a) and (43), can be calculated using Equation (44) (for the initial conditions, the concentration in the nanocrystallite volume is zero and the concentration on its surface is a function of time) [
1]:
The average concentration of the solute in the entire volume of nanocrystallite during the reaction in the gas phase can be determined using Equation (45) [
1]:
Calculation results derived from Equation (44) for 10 nm (A = 0.3000 nm
−1) and 40 nm nanocrystallites (A = 0.0750 nm
−1), with a diffusion coefficient of 10
−19 m
2/s and various values of the parameter v/V
r = <0.0003–0.0300>, are shown in
Figure 12.
The results presented in
Figure 12 are presented as the change in ln
depending on ln P(t) = ln x
g (
Figure 13).
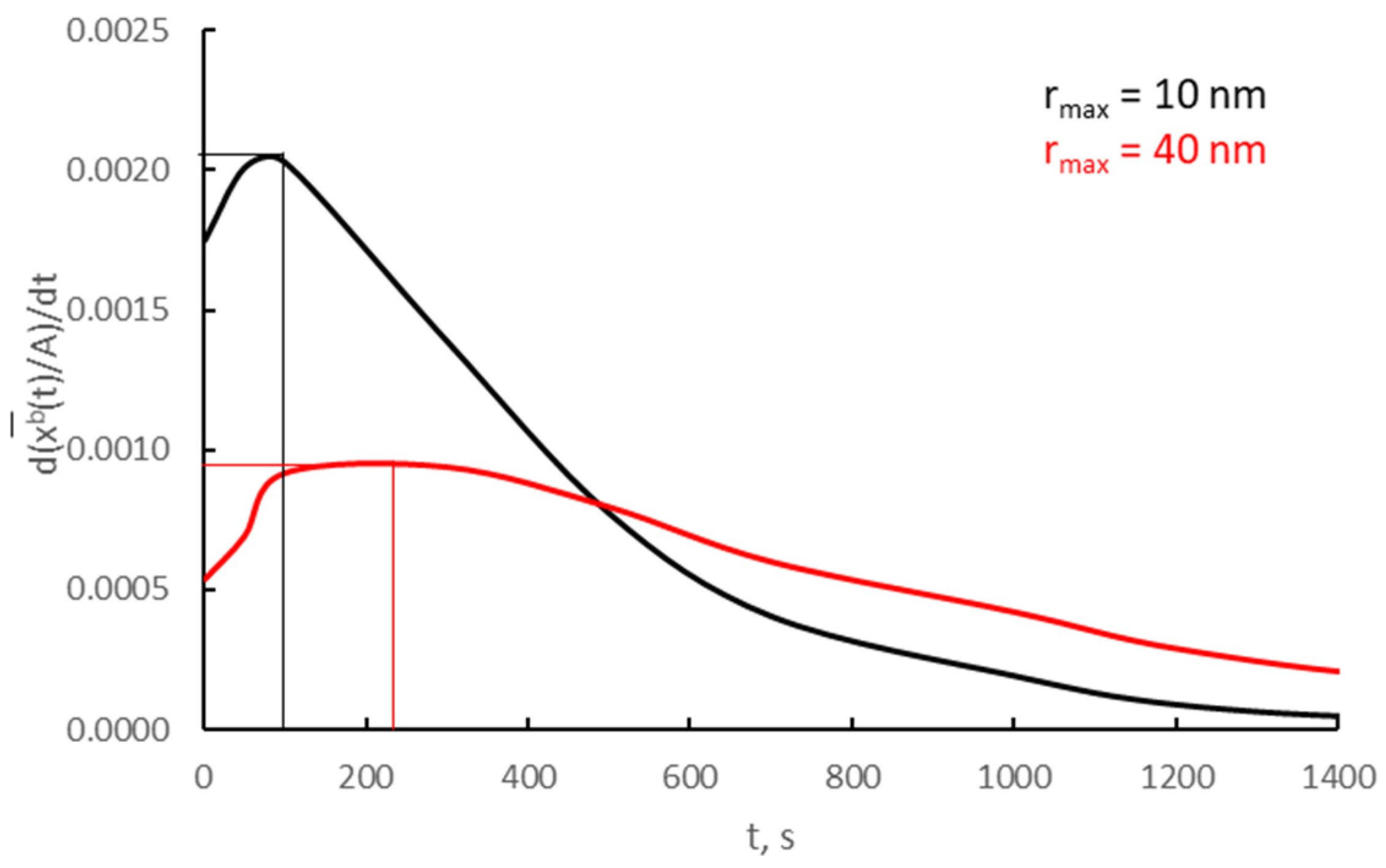
After differentiating over time, the relationships shown in
Figure 12a for v/V
r = 0.0030 and two crystallites; the average rate of the absorption process was determined according to Equation (19) and presented in
Figure 14.
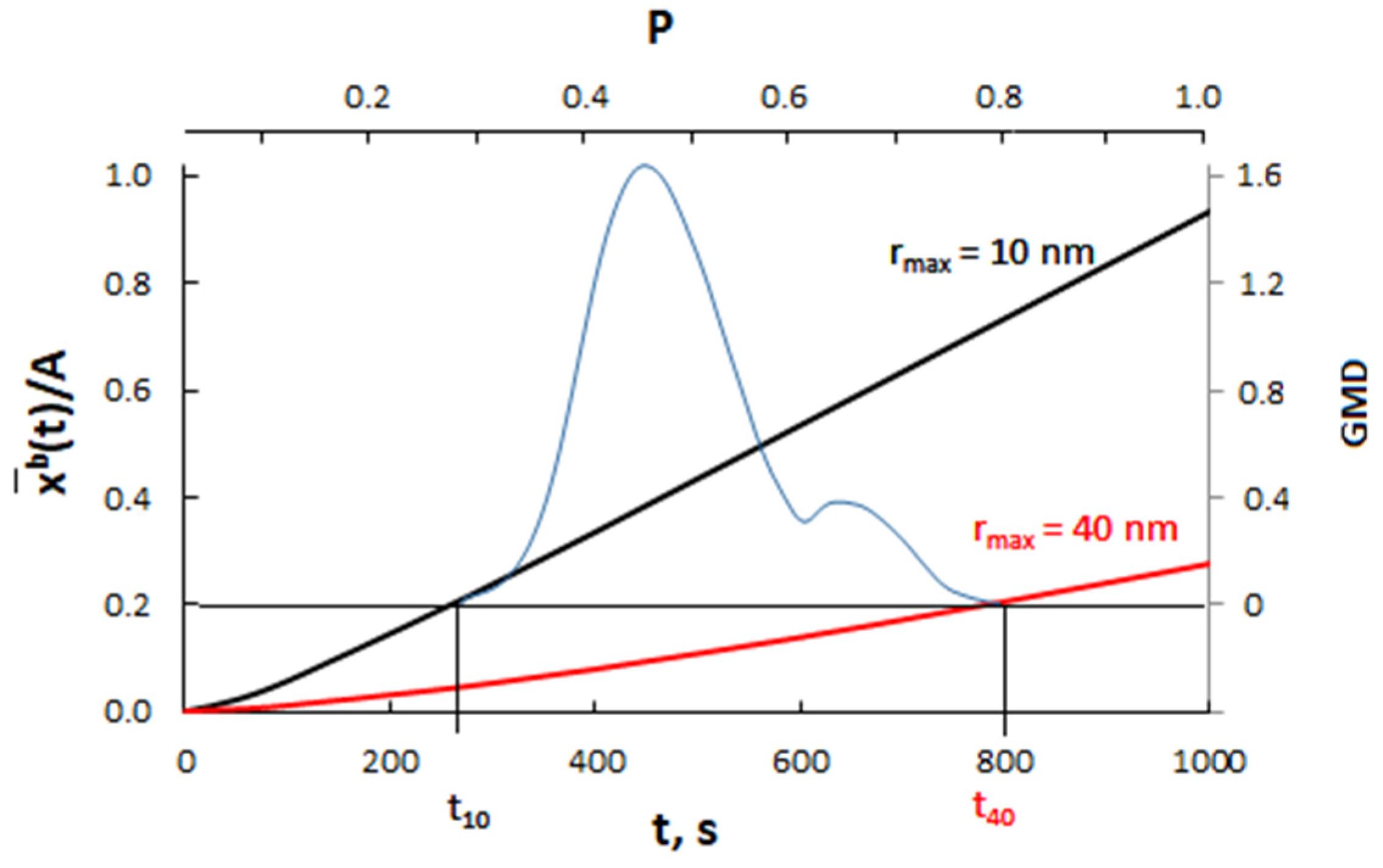
The rate of the absorption process at the time of the phase change for crystallites of 10 and 40 nm is, respectively, 0.0021 and 0.0009 t−1.
After summing the results presented in
Figure 12 for individual crystallites in the range of 10–40 nm (every 1 nm) and according to the crystallite shares given by the bimodal mass distribution, a total and integral diagram of concentration changes in the entire crystallite system is obtained for the kinetic (
Figure 15a) and equilibrium regions (
Figure 15b).
To sum up, in the current work, we deal with a chemical reaction in a solid phase—nitriding reaction of nanocrystallite iron, for which we have assumed a probable mechanism of reaction with the diffusion resistance of an extremely thin surface layer (literally surface layer—we consider the change in the thermodynamic states of nitrogen after passing through a single layer of iron atoms on the nanocrystallite surface)—model No. 1. Since this is the rate-limiting step of the entire process, the equalization of concentrations (i.e., eliminating concentration gradients in the volume of iron nanocrystallites) should occur faster, and we will not observe a growing layer of the product but rather an equal concentration in the volume of nanocrystallites. Then, after exceeding the critical concentration, the phase transformation of the substrate into the product will occur immediately in the entire volume of the nanocrystallite. If the product layer does not grow in the proposed model, the associated diffusion resistance does not change (increase) as the reaction progresses, as occurs in the shrinking core model (model No. 2) [
33]. The adsorption model of the reaction is also known (model No. 3) [
27], in which the rate of the process is limited by the rate of adsorption and dissociation of the gaseous substrate on the nanocrystallite surface. It was also assumed that there is no concentration gradient in the volume of nanocrystallites (an equilibrium is established between the adsorbate on the surface and the substrate dissolved in the volume of nanocrystallites), and the substrate–product phase transformation will occur in the entire volume of nanocrystallites. So, to sum up, we may compare those models and conclude that there are some similarities (in model No. 1 and in model No. 3 there is no concentration gradient in the volume of nanocrystallites; in model No. 1 and in model No. 2 the process rate is limited by the diffusion rate) but also differences (in model No. 1, the process rate is limited by the diffusion rate and in model No. 3 by the rate of gas adsorption on the surface of the nanocrystallites; in model No. 1, diffusion only concerns the passage of adsorbate atoms from the surface through an unchanging interface into the nanocrystallite volume, where the concentrations are equalized, and in model No. 2, the diffusion rate changes due to the surface layer of the product growing as the reaction progresses, limiting the possibility of contacting the gaseous substrates and those remaining in the solid phase).
The proposed model can be used to describe any reaction system in which a phase transformation may occur as a result of exceeding a certain critical concentration in the volume of nanoparticles. Examples include nitriding, carburizing, and oxidation reactions of transition metal nanoctystalites and reduction in the resulting products, e.g., with hydrogen. Experimental results for the reactions we obtained earlier inspired us to develop the current reaction model. Another example may be the growth of a solid clathrate hydrate phase upon exposure of ice to hydrate-forming gases, such as methane or carbon dioxide described in a scientific paper by Englezos et al. [
37].

 10,
10,  20,
20,  30 and
30 and  40, at four different gas-phase potentials (
40, at four different gas-phase potentials ( 0.25,
0.25,  0.50,
0.50,  0.75 and
0.75 and  1.00), diffusion coefficient D = 10−19 m2/s.
1.00), diffusion coefficient D = 10−19 m2/s.
 10,
10,  20,
20,  30 and
30 and  40, at four different gas-phase potentials (
40, at four different gas-phase potentials ( 0.25,
0.25,  0.50,
0.50,  0.75 and
0.75 and  1.00), diffusion coefficient D = 10−19 m2/s.
1.00), diffusion coefficient D = 10−19 m2/s.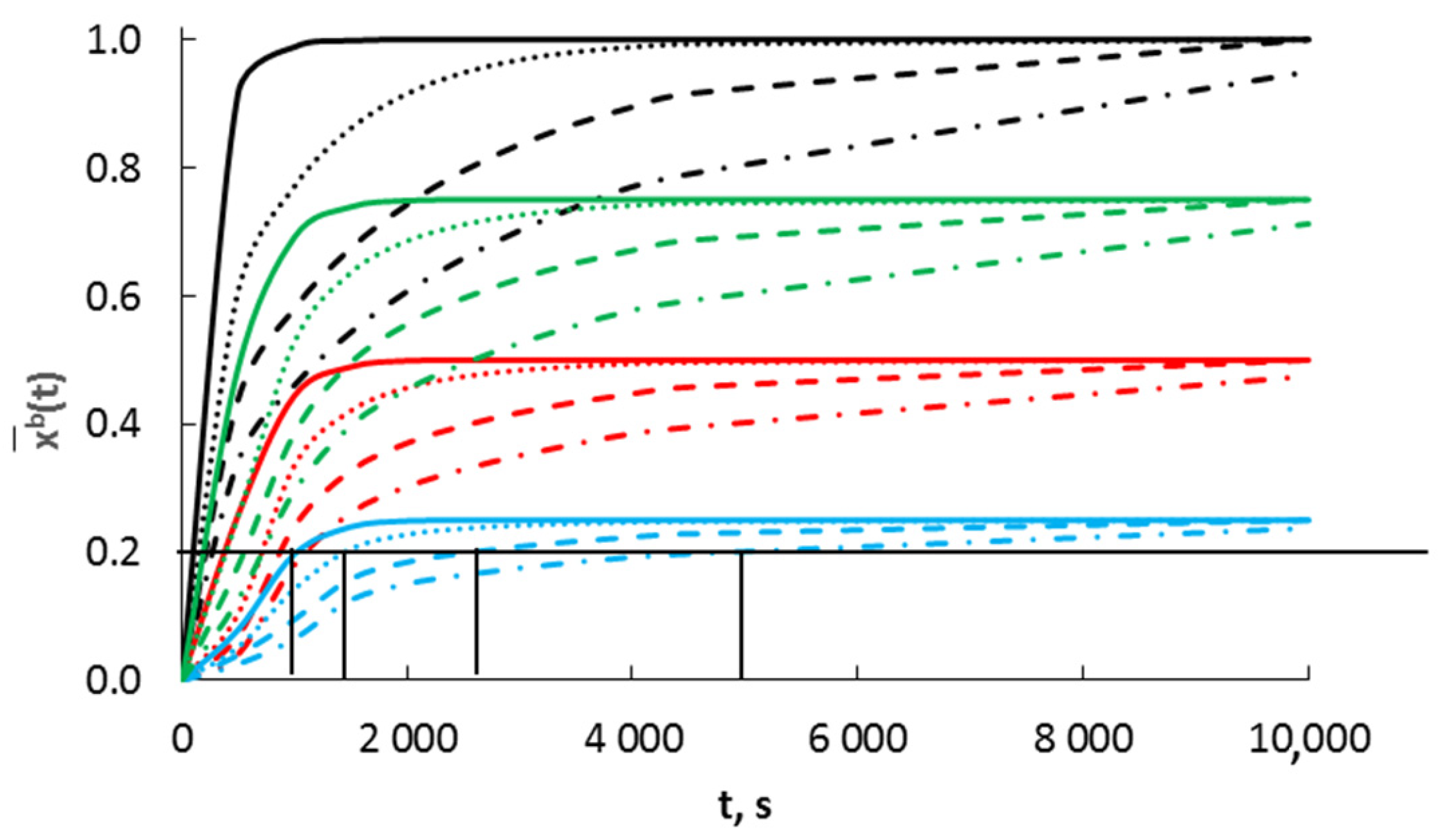
 0.0003,
0.0003,  0.0010,
0.0010,  0.0030
0.0030  0.0300).
0.0300).
 0.0003,
0.0003,  0.0010,
0.0010,  0.0030
0.0030  0.0300).
0.0300).