Optical Parameters of Deuterated Triglycine Sulfate in Terahertz Range
Abstract
1. Introduction
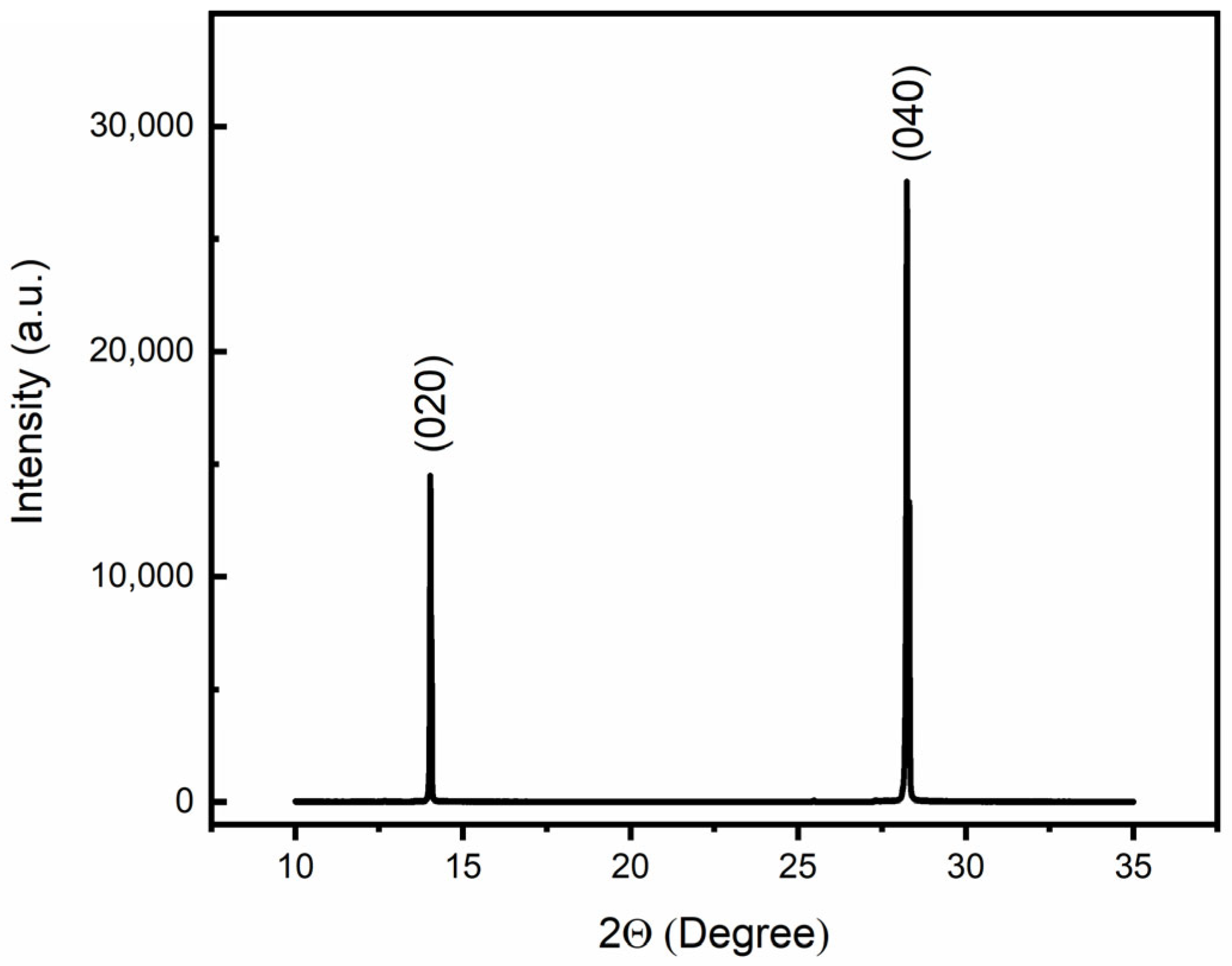
2. Materials and Methods
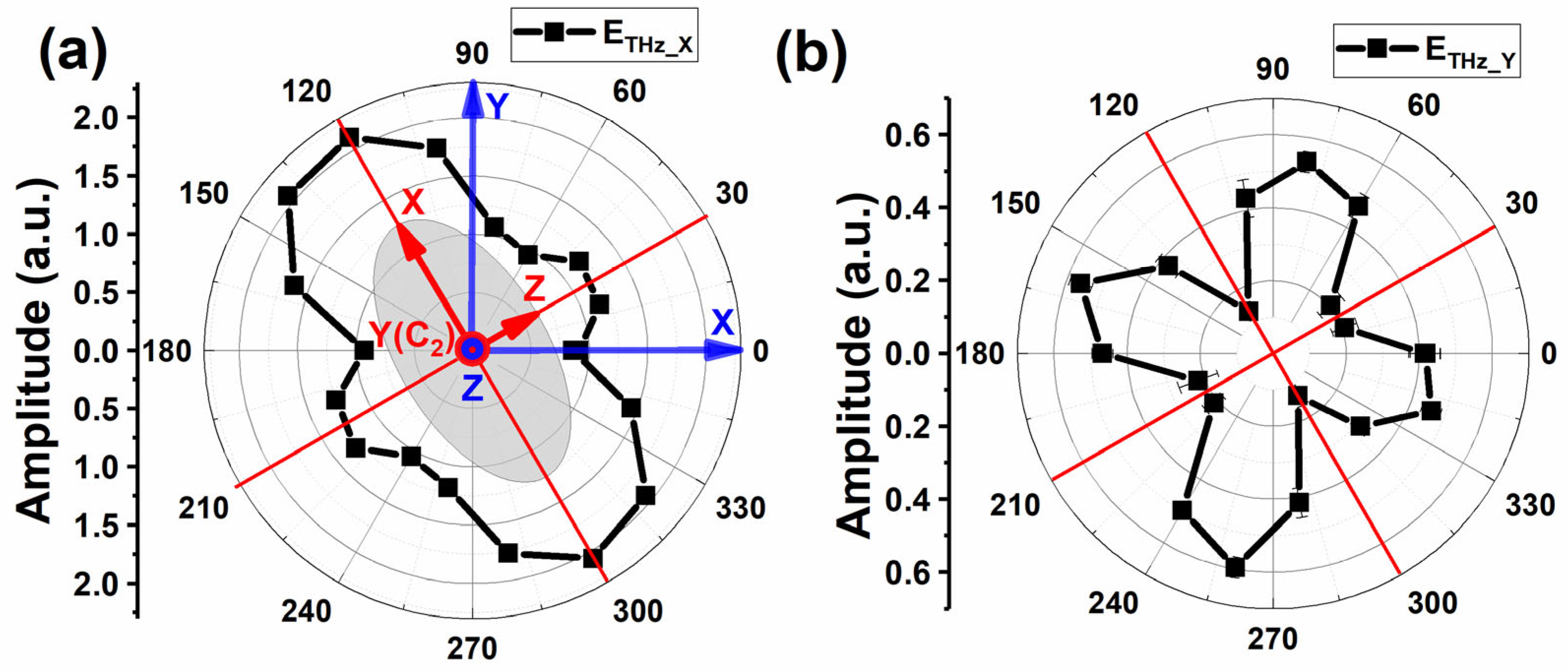
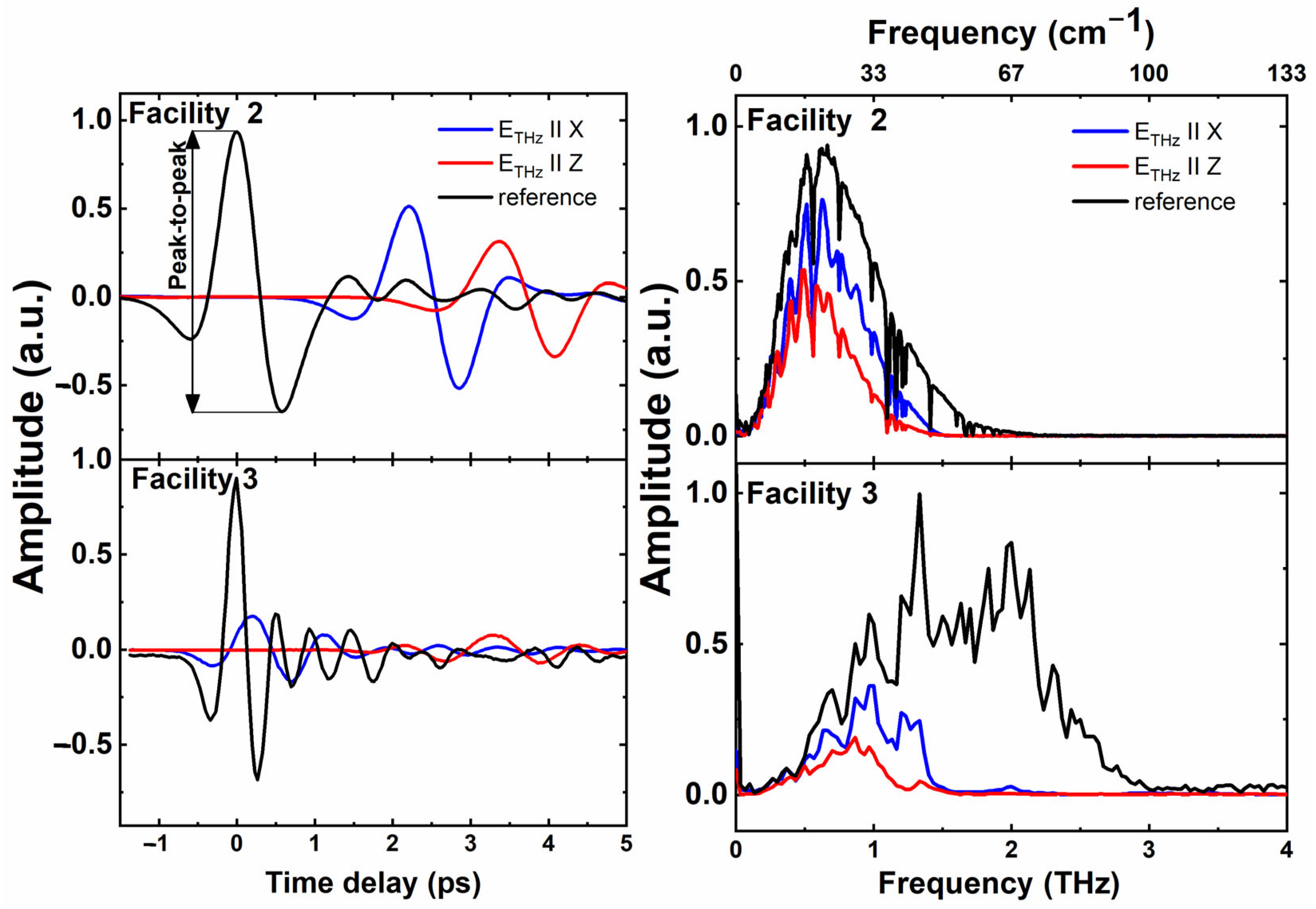
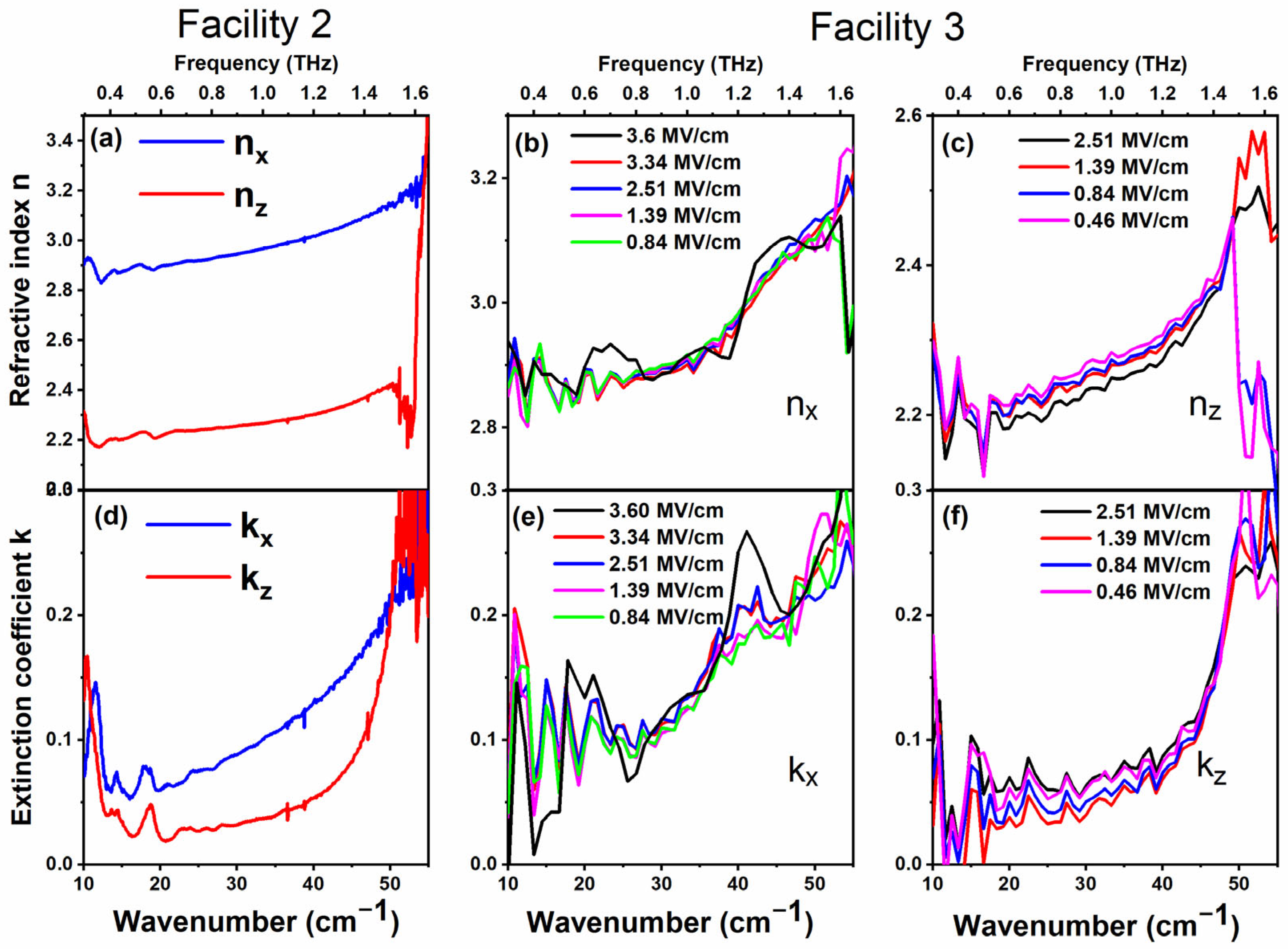
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shirokov, V.B.; Biryukov, S.V.; Mukhortov, V.M.; Yuzyuk, Y.I. Polarization of thin barium-strontium titanate films by an external electric field. Tech. Phys. 2011, 56, 1175–1180. [Google Scholar] [CrossRef]
- Turygin, A.P.; Shikhova, V.A.; Kosobokov, M.S.; Akhmatkhanov, A.R.; Sergeeva, O.N.; Shur, V.Y. Highly Anisotropic Tip-Induced Domain Growth in Polydomain Triglycine Sulfate. ACS Appl. Electron. Mater. 2022, 4, 5215–5220. [Google Scholar] [CrossRef]
- Asada, K.; Miyamoto, T.; Yamakawa, H.; Hirata, J.; Takamura, N.; Morimoto, T.; Suzuki, K.; Otaki, T.; Kida, N.; Nakamura, T.; et al. Ultrafast control of electronic states by a terahertz electric field pulse in the quasi-one-dimensional organic ferroelectric (TMTTF)2PF6. Phys. Rev. B 2021, 104, 195148. [Google Scholar] [CrossRef]
- Bilyk, V.; Brekhov, K.; Ovchinnikov, A.; Chefonov, O.; Mishina, E. Terahertz-induced polar state in quantum paraelectric SrTiO3. MRS Adv. 2023, 8, 943–946. [Google Scholar] [CrossRef]
- Mishina, E.; Bilyk, V.; Sherstyuk, N.; Sigov, A. Ferroelectric switching by (sub)-picosecond electromagnetic pulse. Ferroelectrics 2021, 577, 1–12. [Google Scholar] [CrossRef]
- Shin, D.; Latini, S.; Schäfer, C.; Sato, S.A.; Baldini, E.; De Giovannini, U.; Hübener, H.; Rubio, A. Simulating Terahertz Field-Induced Ferroelectricity in Quantum Paraelectric SrTiO3. Phys. Rev. Lett. 2022, 129, 167401. [Google Scholar] [CrossRef]
- Zhou, S.; Ji, J.; Tian, Y.; Ling, F.; Yu, W. Optically tuned dielectric property of barium titanate thin film by THz spectroscopy. Opt. Mater. 2017, 73, 623–627. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, Y.; Guo, X.; Chen, T.; Liang, H.; Hao, X.; Hou, X.; Kou, W.; Zhao, Y.; Zhou, T.; et al. A Review of THz Modulators with Dynamic Tunable Metasurfaces. Nanomaterials 2019, 9, 965. [Google Scholar] [CrossRef]
- Yue, J.; Ling, F.; Yao, J. All-optical tunable terahertz modulator based on a BiFeO3 /Si heterostructure. Opt. Mater. Express 2020, 10, 2919. [Google Scholar] [CrossRef]
- Sanjuan, F.; Tocho, J.O. Optical properties of silicon, sapphire, silica and glass in the Terahertz range. In Proceedings of the Latin America Optics and Photonics Conference, São Sebastião, Brazil, 11–13 November 2012; OSA: Washington, DC, USA, 2012; p. LT4C.1. [Google Scholar]
- Rogalin, V.E.; Kaplunov, I.A.; Kropotov, G.I. Optical Materials for the THz Range. Opt. Spectrosc. 2018, 125, 1053–1064. [Google Scholar] [CrossRef]
- Naftaly, M.; Gregory, A. Terahertz and Microwave Optical Properties of Single-Crystal Quartz and Vitreous Silica and the Behavior of the Boson Peak. Appl. Sci. 2021, 11, 6733. [Google Scholar] [CrossRef]
- Duvillaret, L.; Garet, F.; Coutaz, J.-L. Highly precise determination of optical constants and sample thickness in terahertz time-domain spectroscopy. Appl. Opt. 1999, 38, 409. [Google Scholar] [CrossRef]
- Bilyk, V.R.; Grishunin, K.A. Complex Refractive Index of Strontium Titanate in the Terahertz Frequency Range. Russ. Technol. J. 2019, 7, 71–80. [Google Scholar] [CrossRef]
- Białek, M.; Ito, T.; Rønnow, H.; Ansermet, J.-P. Terahertz-optical properties of a bismuth ferrite single crystal. Phys. Rev. B 2019, 99, 064429. [Google Scholar] [CrossRef]
- Lal, R.B.; Batra, A.K. Growth and properties of triglycine sulfate (TGS) crystals: Review. Ferroelectrics 1993, 142, 51–82. [Google Scholar] [CrossRef]
- Sabarish, V.C.B.; Kumar, G.R.; Raj, S.G.; Durairajan, A.; Rajashekar, B.N. Growth and Characterization of Triglycine Sulphate Single Crystal by Sankaranaryanan–Ramasamy Method. Mater. Today Proc. 2018, 5, 18815–18822. [Google Scholar] [CrossRef]
- Gowda, J.; Moolya, B.N.; Ravindraswami, K. Growth and characterization of barium doped triglycine sulphate (BaTGS) single crystals. In Proceedings of the AIP Conference, Mumbai, India, 10 April 2018; p. 100016. [Google Scholar]
- Alemu, D.; Firehun, S.; Abza, T.; Peter, M.E. The Study of Structural, Optical, and Dielectric Properties of Magnesium Chloride-Doped Triglycine Sulphate Ferroelectric Single Crystals. Adv. Mater. Sci. Eng. 2022, 2022, 1–6. [Google Scholar] [CrossRef]
- Iwasaki, K.; Fujii, S.; Kimura, T.; Yamamoto, M.; Tanabe, T. Crystal Growth of Triglycine Sulfate Crystals Under 40-GHz Irradiation and the Infrared Detection of their Device Characteristics. J. Electron. Mater. 2024, 53, 4841–4847. [Google Scholar] [CrossRef]
- Bilyk, V.; Grishunin, K.; Tinnemans, P.; Rasing, T.; Kirilyuk, A.; Sergeeva, O.; Sherstyuk, N.; Mishina, E.; Lavrov, S.; Sigov, A. Terahertz wave rectification in a ferroelectric triglycine sulfate single crystal. Opt. Lett. 2023, 48, 2889. [Google Scholar] [CrossRef]
- Sivakumar, A.; Dai, L.; Dhas, S.S.J.; Dhas, S.A.M.B.; Freire, P.T.C.; Kumar, R.S.; Almansour, A.I. Acoustic shock wave-induced ordered to disordered switchable phase transitions: A case study of ferroelectric triglycine sulphate single crystal for the application of molecular switches. J. Solid. State Chem. 2024, 332, 124552. [Google Scholar] [CrossRef]
- Ghane-Motlagh, R.; Woias, P. A pyroelectric thin film of oriented triglycine sulfate nano-crystals for thermal energy harvesting. Smart Mater. Struct. 2019, 28, 104002. [Google Scholar] [CrossRef]
- Sudhakaran, J.M.; Philip, J. Triglycine sulphate and its deuterated analog in polyurethane matrix for thermal/infrared detection: A comparison. J. Appl. Polym. Sci. 2015, 132, 42250. [Google Scholar] [CrossRef]
- Alomari, A.; Kropachev, A.; Baliga, S.; Chakraborty, R.; Batra, A.K.; Bowen, C.R. Synthesis, growth, and characterization of deuterated l-alanine triglycine sulfate (DLaTGS) single crystal for infra-red detector applications. Opt. Mater. 2024, 150, 115152. [Google Scholar] [CrossRef]
- Liu, C.; Yang, T.; Xie, M.; He, Z.; Xie, H.; Wei, Y.; Geng, D.; Guan, H.; Lu, H. Pyro-Phototronic Circularly Polarized Light Detection Based on Deuterated L-Alanine Doped Triglycine Sulfate’s Bulk Photovoltaic Effect. Laser Photon. Rev. 2024, 18, 2400527. [Google Scholar] [CrossRef]
- MenloSystems (Official Cite). Tera K15. Available online: https://www.menlosystems.com/products/thz-time-domain-solutions/terak15-terahertz-spectrometer/ (accessed on 4 December 2024).
- Zainullin, F.A.; Khusyainov, D.I.; Kozintseva, M.V.; Buryakov, A.M. Polarization analysis of THz radiation using a wire grid polarizer and ZnTe crystal. Russ. Technol. J. 2022, 10, 74–84. [Google Scholar] [CrossRef]
- Vicario, C.; Jazbinsek, M.; Ovchinnikov, A.V.; Chefonov, O.V.; Ashitkov, S.I.; Agranat, M.B.; Hauri, C.P. High efficiency THz generation in DSTMS, DAST and OH1 pumped by Cr:forsterite laser. Opt. Express 2015, 23, 4573. [Google Scholar] [CrossRef]
- Jepsen, P.U.; Cooke, D.G.; Koch, M. Terahertz spectroscopy and imaging—Modern techniques and applications. Laser Photon. Rev. 2011, 5, 124–166. [Google Scholar] [CrossRef]
- Jepsen, P.U.; Fischer, B.M. Dynamic range in terahertz time-domain transmission and reflection spectroscopy. Opt. Lett. 2005, 30, 29. [Google Scholar] [CrossRef]
- Santra, L.; Verma, A.L.; Bajpai, P.K.; Hilczer, B.; Huong, P.V. Raman spectroscopic study of alanine doped triglycine sulphate ferroelectric single crystals. J. Phys. Chem. Solids 1994, 55, 405–411. [Google Scholar] [CrossRef]
- Hadni, A.; Gerbaux, X. Far IR excitation of longitudinal optical phonons in triglycine sulphate. Ferroelectrics 2000, 248, 15–26. [Google Scholar] [CrossRef]
- Bajpai, P.K.; Verma, A.L. Molecular dynamics of glycine ions in alanine doped TGS single crystal as probed by polarized laser raman spectroscopy. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2012, 96, 906–915. [Google Scholar] [CrossRef]
- Tashiro, K.; Yagi, N.; Kobayashi, M.; Kawaguchi, T. Determination of the LO–TO Splittings in the Raman Spectra of Ferroelectric Triglycine Sulfate (TGS). Jpn. J. Appl. Phys. 1987, 26, 699. [Google Scholar] [CrossRef]
- Vicario, C.; Trisorio, A.; Allenspach, S.; Rüegg, C.; Giorgianni, F. Narrow-band and tunable intense terahertz pulses for mode-selective coherent phonon excitation. Appl. Phys. Lett. 2020, 117, 101101. [Google Scholar] [CrossRef]
- Brekhov, K.; Bilyk, V.; Ovchinnikov, A.; Chefonov, O.; Mukhortov, V.; Mishina, E. Resonant Excitation of the Ferroelectric Soft Mode by a Narrow-Band THz Pulse. Nanomaterials 2023, 13, 1961. [Google Scholar] [CrossRef]
- Basini, M.; Unikandanunni, V.; Gabriele, F.; Cross, M.; Derrico, A.M.; Gray, A.X.; Hoffmann, M.C.; Forte, F.; Cuoco, M.; Bonetti, S. Terahertz-driven parametric excitation of Raman-active phonons in LaAlO3. arXiv 2024, arXiv:2410.06748. [Google Scholar]
- Nishizawa, S.; Sakai, K.; Hangyo, M.; Nagashima, T.; Takeda, M.W.; Tominaga, K.; Oka, A.; Tanaka, K.; Morikawa, O. Terahertz Time-Domain Spectroscopy. In Terahertz Optoelectronics; Springer: Berlin/Heidelberg, Germany, 2005; pp. 203–270. [Google Scholar]
- Afanasiev, D.; Hortensius, J.R.; Ivanov, B.A.; Sasani, A.; Bousquet, E.; Blanter, Y.M.; Mikhaylovskiy, R.V.; Kimel, A.V.; Caviglia, A.D. Ultrafast control of magnetic interactions via light-driven phonons. Nat. Mater. 2021, 20, 607–611. [Google Scholar] [CrossRef]
- Lejman, M.; Vaudel, G.; Infante, I.C.; Chaban, I.; Pezeril, T.; Edely, M.; Nataf, G.F.; Guennou, M.; Kreisel, J.; Gusev, V.E.; et al. Ultrafast acousto-optic mode conversion in optically birefringent ferroelectrics. Nat. Commun. 2016, 7, 12345. [Google Scholar] [CrossRef]
- Handa, H.; Okamura, Y.; Yoshimi, R.; Tsukazaki, A.; Takahashi, K.S.; Tokura, Y.; Takahashi, Y. Terahertz field driven giant nonlinear phonon response in ferroelectric semiconductor In-doped (Sn,Pb)Te. Phys. Rev. B 2024, 109, L081102. [Google Scholar] [CrossRef]
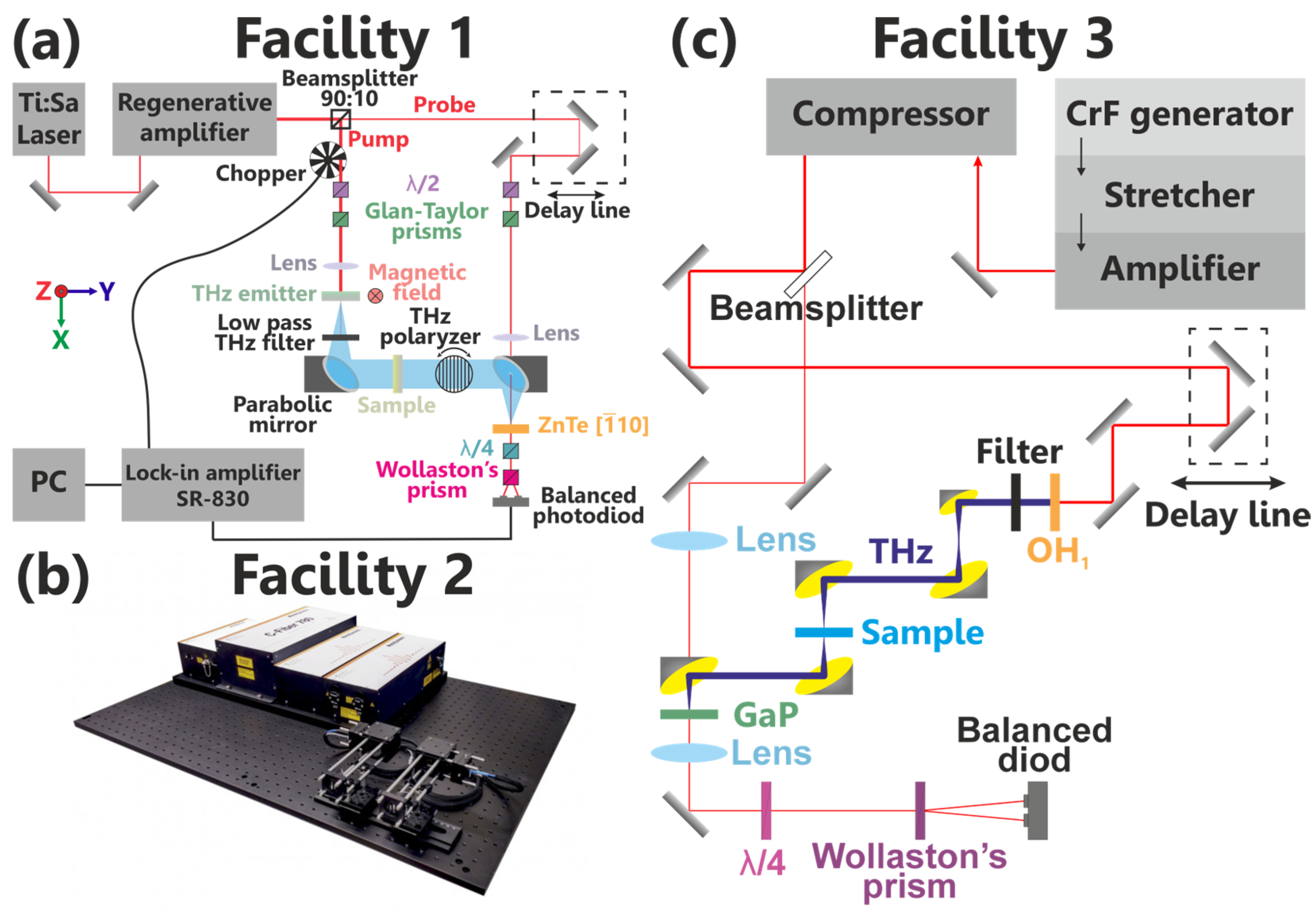
| Facility 1 | Facility 2 | Facility 3 | |
|---|---|---|---|
| THz source | SE CoFeB/Pt | Photoconductive antenna | OH1 organic crystal |
| Repetition rate | 3 kHz | 100 MHz | 10 Hz |
| Possibility of sample rotation | Yes | No | No |
| Possibility of X and Y THz component measurements | Yes | No | No |
| THz field | <50 kV/cm | <50 kV/cm | Up to 3.6 MV/cm |
| Possibility of THz field changing | No | No | Yes |
| Evaluate of the error | 4% | <1% | 12% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brekhov, K.; Gorbatova, A.; Lebedeva, E.; Avdeev, P.; Kudryavtsev, A.; Chefonov, O.; Zhukov, S.; Mishina, E. Optical Parameters of Deuterated Triglycine Sulfate in Terahertz Range. Crystals 2024, 14, 1093. https://doi.org/10.3390/cryst14121093
Brekhov K, Gorbatova A, Lebedeva E, Avdeev P, Kudryavtsev A, Chefonov O, Zhukov S, Mishina E. Optical Parameters of Deuterated Triglycine Sulfate in Terahertz Range. Crystals. 2024; 14(12):1093. https://doi.org/10.3390/cryst14121093
Chicago/Turabian StyleBrekhov, Kirill, Anastasia Gorbatova, Ekaterina Lebedeva, Pavel Avdeev, Andrey Kudryavtsev, Oleg Chefonov, Sergey Zhukov, and Elena Mishina. 2024. "Optical Parameters of Deuterated Triglycine Sulfate in Terahertz Range" Crystals 14, no. 12: 1093. https://doi.org/10.3390/cryst14121093
APA StyleBrekhov, K., Gorbatova, A., Lebedeva, E., Avdeev, P., Kudryavtsev, A., Chefonov, O., Zhukov, S., & Mishina, E. (2024). Optical Parameters of Deuterated Triglycine Sulfate in Terahertz Range. Crystals, 14(12), 1093. https://doi.org/10.3390/cryst14121093






