Novel High-Temperature Modification of Belomarinaite KNaSO4: Crystal Structure and Thermal Order–Disorder Phase Transitions
Abstract
:1. Introduction
2. Materials and Methods
2.1. Samples
2.2. Single Crystal X-ray Diffraction
2.3. BVS for Na-Ion Transport
2.4. Structural Complexity Calculation
3. Results and Discussion
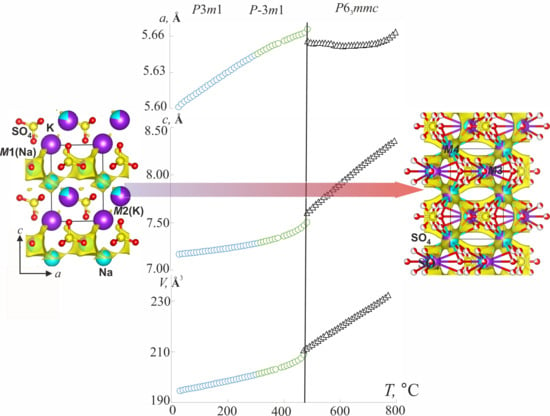
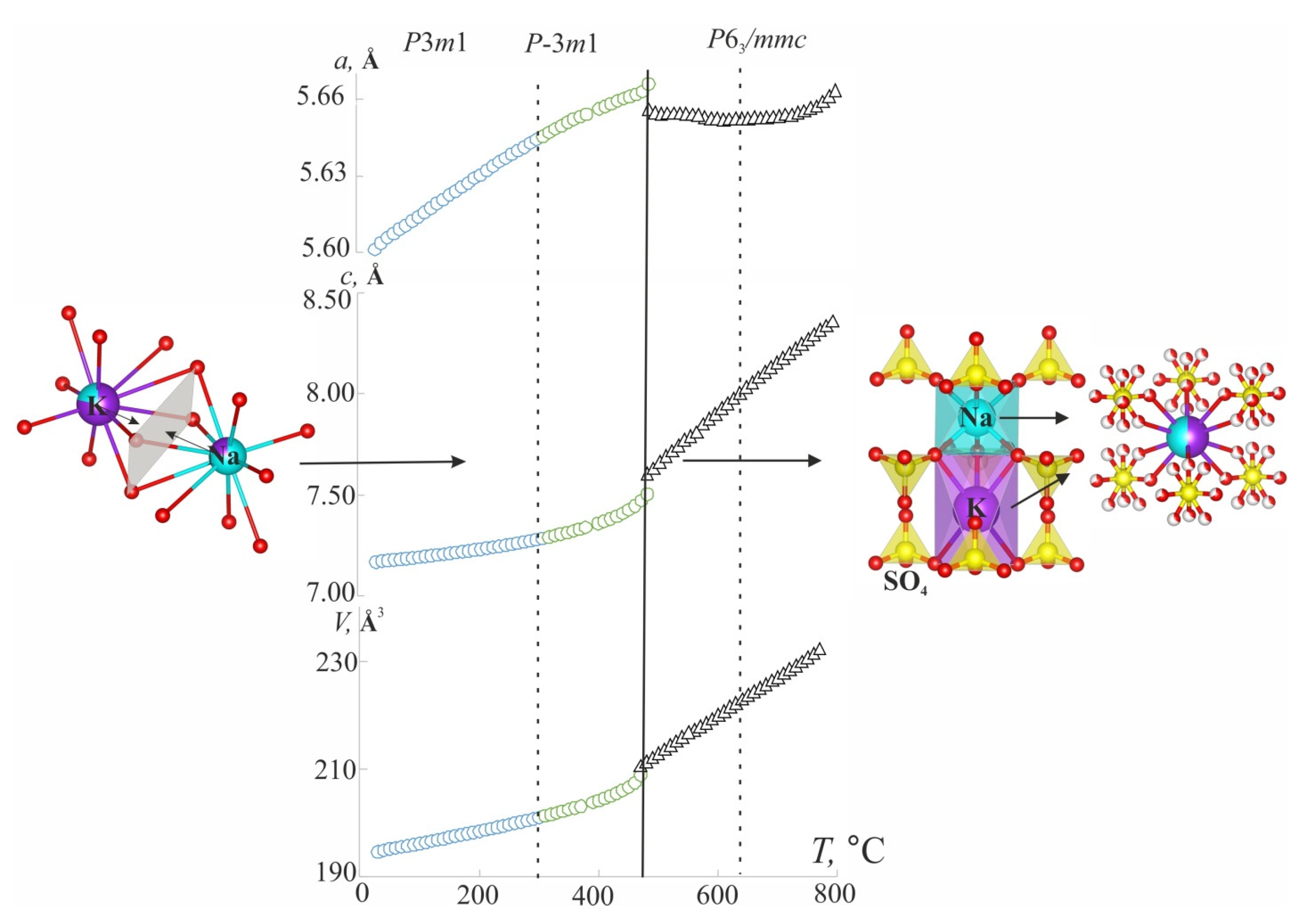
3.1. Thermal Order–Disorder Phase Transitions
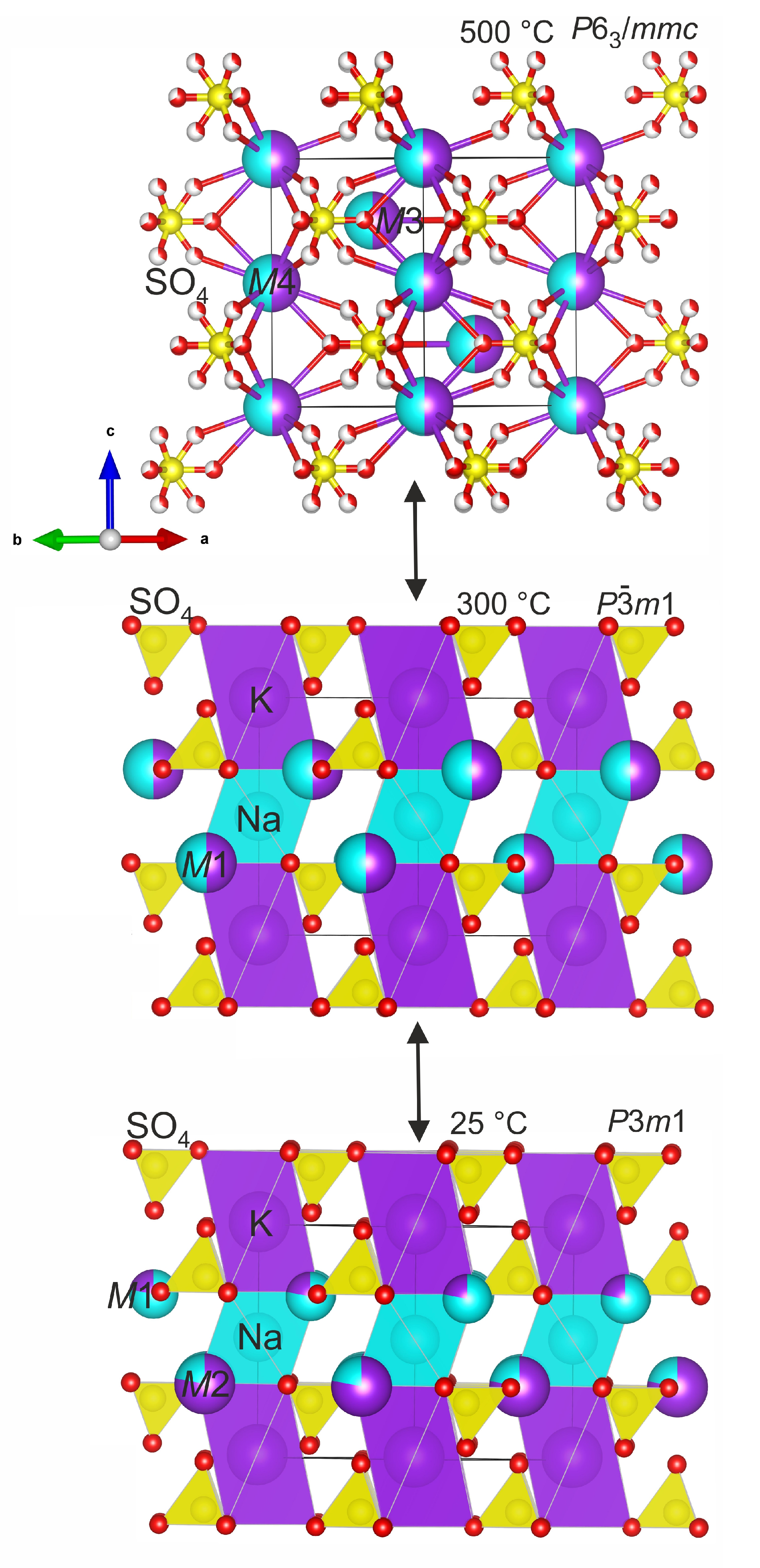
3.2. Temperature-Dependent Inheritance of KNaSO4 Structural Transformations
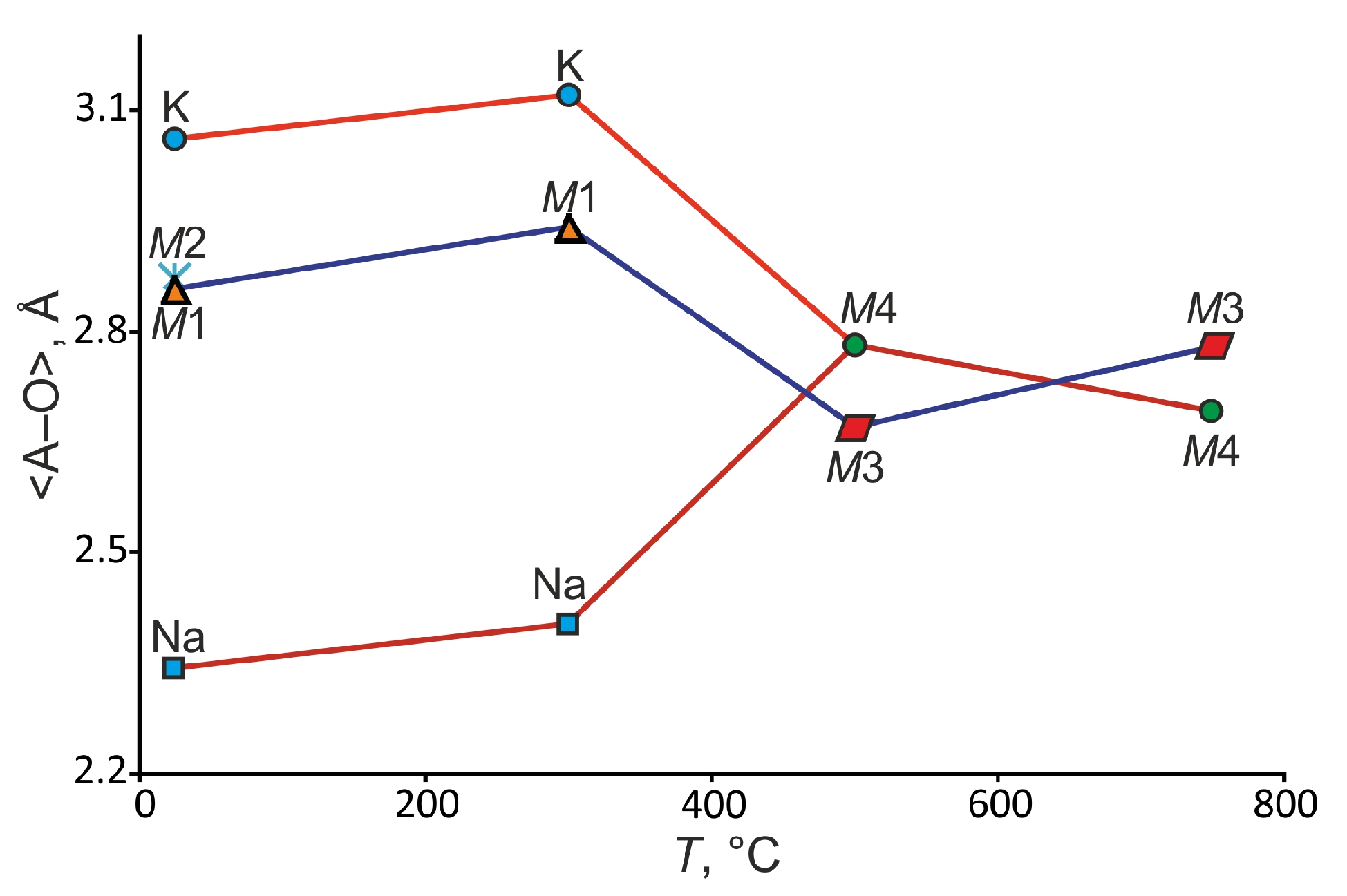
3.3. Sodium-Ion Migration in KNaSO4 at Different Temperatures
3.4. Structural Complexity
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Filatov, S.K.; Shablinskii, A.P.; Vergasova, L.P.; Saprykina, O.U.; Bubnova, R.S.; Moskaleva, S.V.; Belousov, A.B. Belomarinaite KNa(SO4): A new sulfate from 2012-2013 Tolbachik Fissure eruption, Kamchatka Peninsule, Russia. Mineral. Mag. 2019, 83, 569–575. [Google Scholar] [CrossRef]
- Belousov, A.B.; Belousova, M.G.; Edwards, B.; Volynets, A.O.; Melnikov, D.V. Overview of the precursors and dynamics of the 2012–13 basaltic fissure eruption of Tolbachik Volcano, Kamchatka, Russia. J. Volcanol. Geotherm. Res. 2015, 307, 22–37. [Google Scholar] [CrossRef]
- Shchipalkina, N.V.; Pekov, I.V.; Chukanov, N.V.; Zubkova, N.V.; Belakovskiy, D.I.; Koshlyakova, N.N.; Britvin, S.N.; Sidorov, E.G.; Vozchikova, S.A. Alkali sulfates with aphthitalite-like structures from fumaroles of the Tolbachik volcano, Kamchatka, Russia. II A new mineral, natroaphthitalite, and new data on belomarinaite. Can. Mineral. 2020, 58, 167–181. [Google Scholar] [CrossRef]
- Pekov, I.V.; Agakhanov, A.A.; Zubkova, N.V.; Koshlyakova, N.N.; Shchipalkina, N.V.; Sandalov, F.D.; Yapaskurt, V.O.; Turchkova, A.G.; Sidorov, E.G. Oxidizing-type fumarolic systems of the Tolbachik volcano—A mineralogical and geochemical unique. Russ. Geol. Geophys. 2020, 61, 675–688. [Google Scholar] [CrossRef]
- Belousova, M.G.; Saprykina, O.Y.; Bubnova, R.S.; Shablinskii, A.P.; Vergasova, L.P.; Belousov, A.B.; Filatov, S.K. A thermal study of the new mineral belomarinaite KNaSO4. J. Volcanol. Seismol. 2021, 15, 51–57. [Google Scholar] [CrossRef]
- Okada, K.; Osaka, J. Structures of potassium sodium sulfate and tripotassium sodium disulphate. Acta Crystallogr. 1980, B36, 919–921. [Google Scholar] [CrossRef]
- Moore, P.B. Bracelets and pinwheels: A topological-geometrical approach to the calcium orthosilicate and alkali sulphate structures. Am. Mineral. 1973, 58, 32–42. [Google Scholar]
- Moore, P.B. The glaserite, K3Na(SO4)2, structure type as a‘super’ densepacked oxide: Evidence for icosahedral geometry and cation-anion mixed layer packings. Neues Jahrb Fur Miner. 1976, 127, 187–196. [Google Scholar]
- Moore, P.B. Complex crystal structures related to glaserite, K3Na(SO4)2: Evidence for very dense packings among oxysalts. Bull Miner. 1981, 104, 536–547. [Google Scholar] [CrossRef]
- Eysel, W. Crystal chemistry of the system Na2SO4–K2SO4–K2CrO4–Na2CrO4 and of the glaserite phase. Am. Mineral. 1973, 58, 736–747. [Google Scholar]
- Egorov-Tismenko, Y.K.; Sokolova, E.V.; Smirnova, N.L.; Yamnova, N.A. Crystal chemical features of minerals related to the glaserite structure type. Mineral. Zhurnal 1984, 6, 3–9. (In Russian) [Google Scholar]
- Hawthorne, F.C.; Krivovichev, S.V.; Burns, P.C. The crystal chemistry of sulfate minerals. Rev. Miner. Geochem 2000, 40, 1–112. [Google Scholar] [CrossRef]
- Fischmeister, H.F. Roentgenkristallographische Ausdehnungsmessungen an einigen Alkalisulfaten. Monatshefte Fuer Chem. 1962, 93, 420–434. [Google Scholar] [CrossRef]
- van den Berg, A.J.; Tuinstra, F. The space group and structure of alpha-K2SO4. Acta Crystallogr. B 1978, 34, 3177–3181. [Google Scholar] [CrossRef]
- Miyake, M.; Morikawa, H.; Iwai, S.I. Structure reinvestigation of the high-temperature form of K2SO4. Acta Crystallogr. B 1980, 36, 532–536. [Google Scholar] [CrossRef]
- Arnold, H.; Kurtz, W.; Richter-Zinnius, A.; Bethke, J. The phase transition of K2SO4 at about 850 K. Acta Crystallogr. B 1981, 37, 1643–1651. [Google Scholar] [CrossRef]
- Eysel, W.; Hoefer, H.H.; Keester, K.L.; Hahn, T. Crystal chemistry and structure of Na2SO4(I) and its solid solutions. Acta Crystallogr. B 1985, 41, 5–11. [Google Scholar] [CrossRef]
- Naruse, H.; Tanaka, K.; Morikawa, H.; Marumo, F. Structure of Na2SO4 at 693 K. Acta Crystallogr. B 1987, 43, 143–146. [Google Scholar] [CrossRef]
- Rasmussen, S.E.; Jorgensen, J.E.; Lundtoft, B. Structures and Phase Transitions of Na2SO4. J. Appl. Crystallogr. 1996, 29, 42–47. [Google Scholar] [CrossRef]
- CRYSALISPRO Software System, version 1.171.39.44; Rigaku Oxford Diffraction: Oxford, UK, 2015.
- Sheldrick, G.M. Crystal structure refinement with SHELXL. Acta Crystallogr. B 2015, 71, 3–8. [Google Scholar] [CrossRef]
- Petricek, V.; Dusek, M.; Palatinus, L. JANA2006; The Crystallographic Computing System; Institute of Physics, Academy of Sciences of the Czech Republic: Staré Město, Czechia, 2006. [Google Scholar]
- Spek, A.L. Single-crystal structure validation with the program PLATON. J. Appl. Crystallogr. 2003, 36, 7–13. [Google Scholar] [CrossRef]
- Brown, I.D.; Altermatt, D. Bond-valence parameters obtained from a systematic analysis of the Inorganic Crystal Structure Database. Acta Crystallogr. 1985, 41, 244–247. [Google Scholar] [CrossRef]
- Rodríguez-Carvajal, J. The Program BondStr (or Bond_Str) and Its GUI GBond_Str Version December 2018. Available online: https://journals.iucr.org/j/issues/2019/01/00/po5136/po5136sup2.pdf (accessed on 4 December 2023).
- Momma, K.; Izumi, F. VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data. J. Appl. Crystallogr. 2011, 44, 1272–1276. [Google Scholar] [CrossRef]
- Krivovichev, S.V. Structural complexity of minerals: Information storage and processing in the mineral world. Mineral. Mag. 2013, 77, 275–326. [Google Scholar] [CrossRef]
- Krivovichev, S.V.; Krivovichev, V.G.; Hazen, R.M.; Aksenov, S.M.; Avdontceva, M.S.; Banaru, A.M.; Gorelova, L.A.; Ismagilova, R.M.; Kornyakov, I.V.; Kuporev, I.V.; et al. Structural and Chemical Complexity of Minerals: An Update. Mineral. Mag. 2022, 86, 183–204. [Google Scholar] [CrossRef]
- Blatov, V.A.; Shevchenko, A.P.; Proserpio, D.M. Applied topological analysis of crystal structures with the program package ToposPro. Cryst. Growth Des. 2014, 14, 3576–3586. [Google Scholar] [CrossRef]
- Kumari, M.S.; Secco, E.A., II. Electrical conductivity and phase transition studies on pure and doped (Cd2+, Gd3+)KNaSO4 and K3Na(SO4)2. Can. J. Chem. 1983, 61, 599–601. [Google Scholar] [CrossRef]
- Abboudy, S.; Hamed, A.E.; Kassem, M.E.; Abou-El-Nasr, L.I. Stoichiometric ratio and doping effects on the thermal properties of sodium potassium sulphate crystals. J. Therm. Anal. 1993, 39, 301–308. [Google Scholar] [CrossRef]
- O’Keeffe, M.; Hyde, B.G. An alternative approach to non-molecular crystal structures with emphasis on the arrangements of cations. Struct. Bond. 1985, 61, 77–144. [Google Scholar]
- Krivovichev, S.V.; Filatov, S.K. Metal arrays in structural units based on anion-centered metal tetrahedra. Acta Crystallogr. B 1999, 55, 664–676. [Google Scholar] [CrossRef]
- Vegas, A. Cations in Inorganic Solids. Crystallogr. Rev. 2000, 7, 189–283. [Google Scholar] [CrossRef]
- Gorelova, L.A.; Vergasova, L.P.; Krivovichev, S.V.; Avdontseva, E.Y.; Moskaleva, S.V.; Karpov, G.A.; Filatov, S.K. Bubnovaite, K2Na8Ca(SO4)6, a new mineral species with modular structure from the Tolbachik volcano, Kamchatka peninsula, Russia. Eur. J. Miner. 2016, 28, 677–686. [Google Scholar] [CrossRef]
- Krivovichev, S.V. Structure description, interpretation and classification in structural mineralogy. Crystallogr. Rev. 2017, 23, 2–71. [Google Scholar] [CrossRef]
- Shablinskii, A.P.; Filatov, S.K.; Krivovichev, S.V.; Vergasova, L.P.; Moskaleva, S.V.; Avdontseva, E.Y.; Knyazev, A.V.; Bubnova, R.S. Dobrovolskyite, Na4Ca(SO4)3, a new fumarolic sulfate from the Great Tolbachik fissure eruption, Kamchatka Peninsula. Russia. Mineral. Mag. 2021, 85, 233–241. [Google Scholar] [CrossRef]
- Filatov, S.K. High Temperature Crystal Chemistry; Nedra: Leningrad, Russia, 1990; 288p. (In Russian) [Google Scholar]
- Filatov, S.K.; Shablinskii, A.P.; Volkov, S.N.; Bubnova, R.S. Forms of solid solution ordering upon decreasing temperature. J. Struct. Chem. 2017, 58, 135–158. [Google Scholar] [CrossRef]
- Shablinskii, A.P.; Filatov, S.K.; Biryukov, Y.P. Crystal structures inherited from parent high-temperature disordered microblocks: Ca2SiO4, Na2SO4–K2SO4 sulfates, and related minerals (bubnovaite and dobrovolskyite). Phys. Chem. Miner. 2023, 50, 30. [Google Scholar] [CrossRef]
- Voronkov, A.A.; Ilyukhin, V.V.; Belov, N.V. Crystal chemistry of mixed frameworks. Principles of Their Formation. Kristallographia 1975, 20, 556–566. (In Russian) [Google Scholar]
- Pekov, I.V.; Shchipalkina, N.V.; Zubkova, N.V.; Gurzhiy, V.V.; Agakhanov, A.A.; Belakovskiy, D.I.; Chukanov, N.V.; Lykova, I.S.; Vigasina, M.F.; Koshlyakova, N.N.; et al. Alkali sulfates with aphthitalite-like structures from fumaroles of the Tolbachik volcano, Kamchatka, Russia. I. Metathenardite, a natural high-temperature modification of Na2SO4. Can. Mineral. 2019, 57, 885–901. [Google Scholar] [CrossRef]
- Filatov, S.K.; Shablinskii, A.P.; Krivovichev, S.V.; Vergasova, L.P.; Moskaleva, S.V. Petrovite, Na10CaCu2(SO4)8, a new fumarolic sulfate from the Great Tolbachik fissure eruption, Kamchatka Peninsula, Russia. Mineral. Mag. 2020, 84, 691–698. [Google Scholar] [CrossRef]
- Kovrugin, V.M.; Nekrasova, D.O.; Siidra, O.I.; Mentre, O.; Masquelier, C.; Stefanovich, S.Y.; Colmont, M. Mineral-inspired crystal growth and physical properties Na2Cu(SO4)2 and review of Na2M(SO4)2(H2O)x (x = 0–6) Compounds. Cryst. Growth Des. 2019, 19, 1233–1244. [Google Scholar] [CrossRef]
- Krivovichev, S.V. Topological complexity of crystal structures: Quantitative approach. Acta Crystallogr. 2012, A68, 393–398. [Google Scholar] [CrossRef] [PubMed]
- Landau, L.D.; Lifshitz, E.M. Statistical Physics. Course of Theoretical Physics; Pergamon Press Oxford: Oxford, UK, 1980; Volume 5, Part 1; p. 440. [Google Scholar]
- Tröster, A.; Schranz, W.; Ehsan, S.; Belbase, K.; Blaha, P. Symmetry-adapted finite Landau theory applied to KMnF3. Crystals 2020, 10, 124. [Google Scholar] [CrossRef]
- Errandonea, D. Landau theory applied to phase transitions in calcium orthotungstate and isostructural compounds. EPL 2007, 77, 56001. [Google Scholar] [CrossRef]
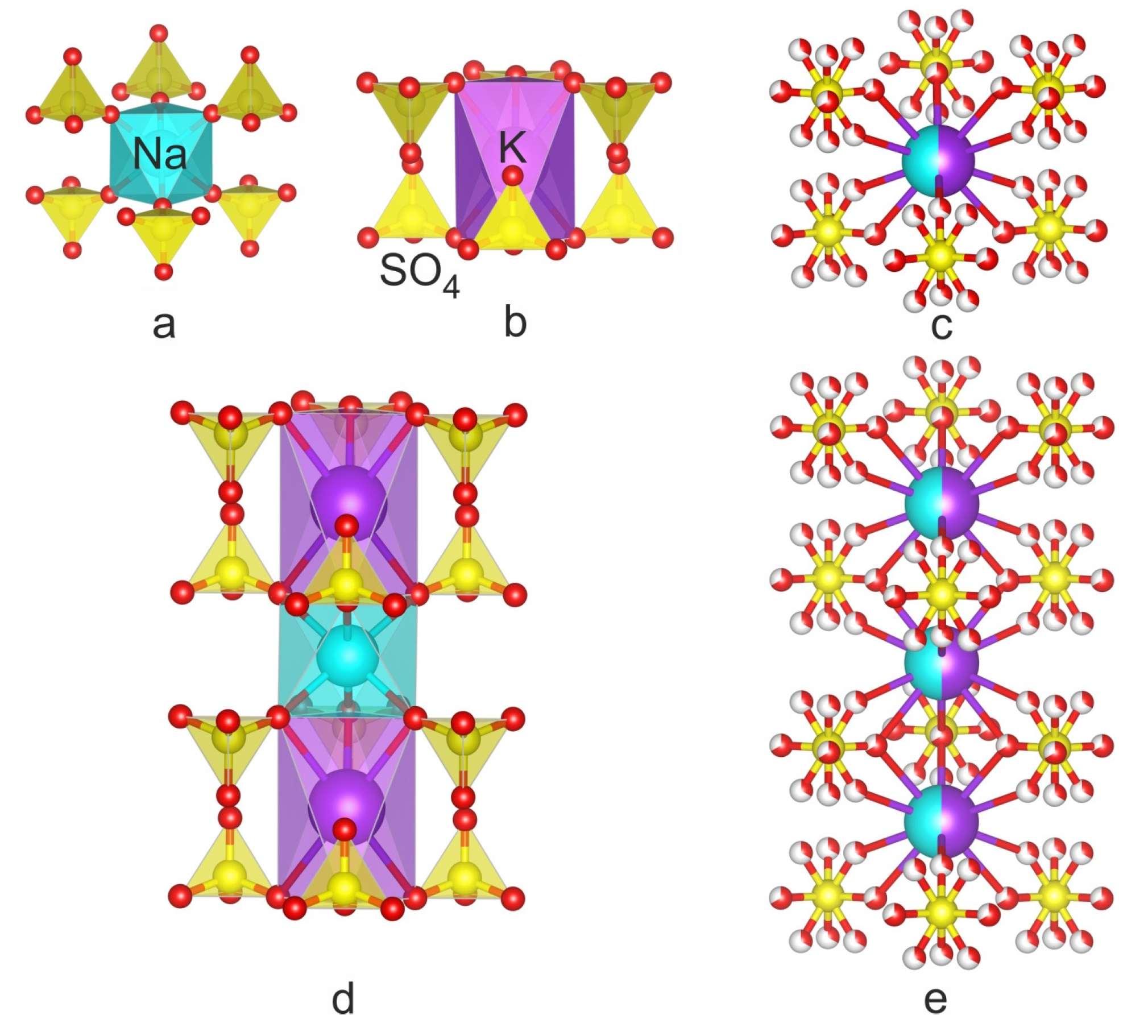

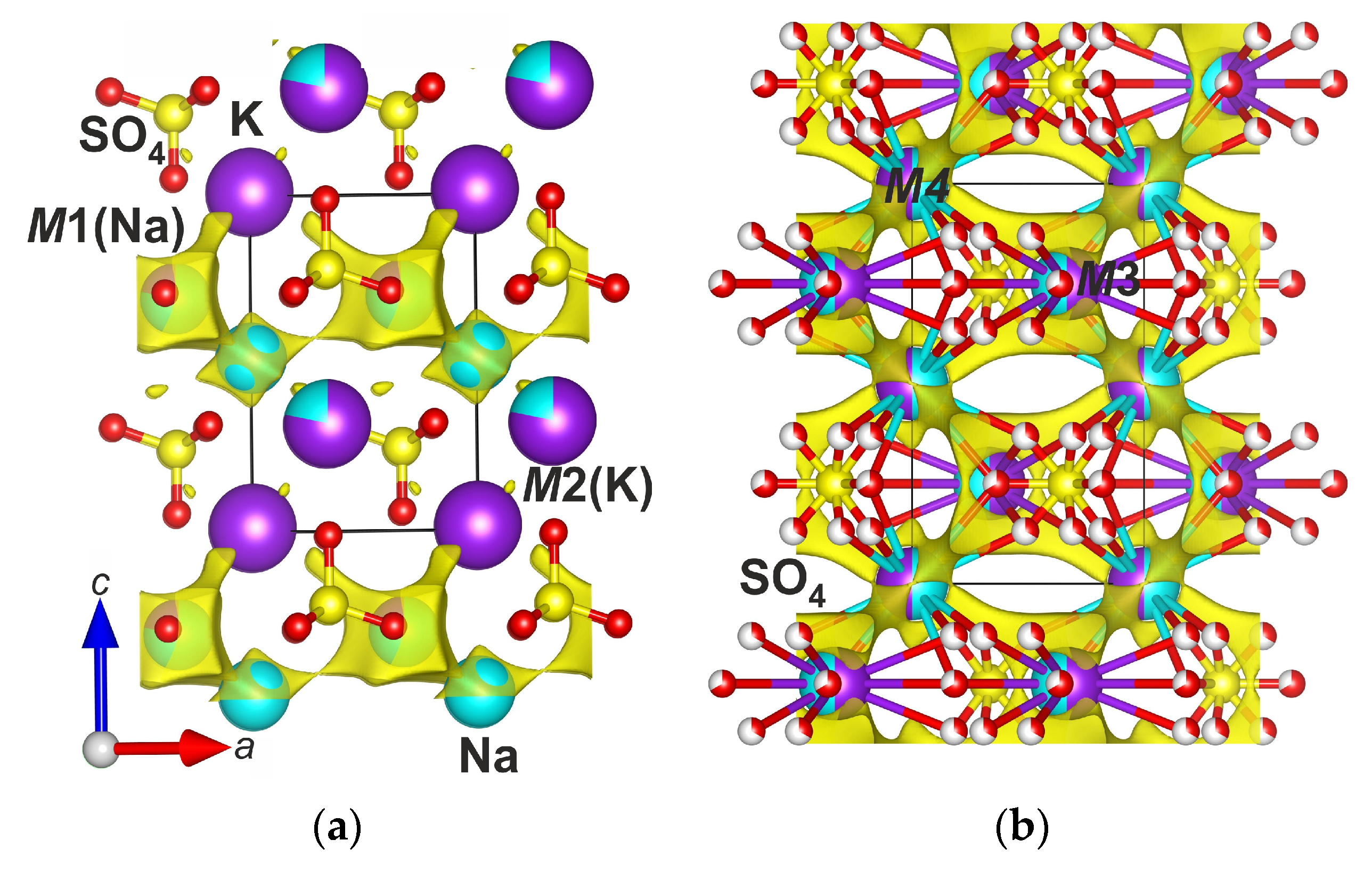
| Chemical Formula | KNaSO4 | |||
|---|---|---|---|---|
| Mr | 158.1 | |||
| Crystal system, | Trigonal | Hexagonal | ||
| Space group | P3m1 | P63/mmc | P63/mmc | |
| Temperature °C | 25 [1] | 300 | 500 | 750 |
| a, c (Å) | 5.6072(3), 7.1781(4) | 5.6914(6), 7.370(3) | 5.669(5), 7.750(3) | 5.654(4), 8.353(6) |
| V (Å3) | 195.45(2) | 206.73(9) | 215.68(8) | 231.25(16) |
| Z | 2 | |||
| Radiation type | Mo Kα | |||
| µ (мм−1) | 1.87 | 1.77 | 1.70 | 1.58 |
| Diffractometer | Rigaku XtaLAB Synergy-S | |||
| No. of measured, independent and observed [I > 3σ(I)] reflections | 2069, 713, 664 | 684, 221, 173 | 738, 116, 47 | 1452, 103, 28 |
| Rint | 0.012 | 0.035 | 0.075 | 0.082 |
| (sin θ/λ)max (Å−1) | 0.851 | 0.686 | 0.669 | 0.682 |
| R(obs), wR(obs), S | 0.027, 0.033, 1.95 | 0.057, 0.088, 3.73 | 0.056, 0.067, 1.74 | 0.048, 0.103, 1.16 |
| Parameters | 38 | 21 | 14 | 14 |
| 25 °C [1] | 300 °C | 500 °C | 750 °C | ||||
|---|---|---|---|---|---|---|---|
| Bond | Distance, Å | Bond | Distance, Å | Bond | Distance, Å | Bond | Distance, Å |
| K–O3 | 2.842(7) × 3 | K–O2 | 2.943(12) × 6 | M4–O1 | 2.724(7) × 6 | M4–O1 | 2.746(12) × 6 |
| K–O4 | 2.885(7) × 3 | ||||||
| K–O1 | 3.243(2) × 3 | K–O1 | 3.305(2) × 6 | M4–O2 | 2.835(12) × 6 | M4–O2 | 2.66(8) × 12 |
| K–O2 | 3.254(2) × 3 | <M4–O>12 | 2.78 | <M4–O>18 | 2.69 | ||
| <K–O>12 | 3.056 | <K–O>12 | 3.124 | ||||
| Na–O3 | 2.332(8) × 3 | Na–O2 | 2.405(10) × 6 | ||||
| Na–O4 | 2.355(8) × 3 | ||||||
| <Na–O>6 | 2.344 | <Na–O>6 | 2.405 | ||||
| M1–O1 | 2.588(10) | M1–O1 | 2.59(2) | M3–O2 | 2.50(3) × 6 | M3–O2 | 2.76(10) × 12 |
| M1–O3 | 2.816(4) × 6 | M1–O2 | 2.859(3) × 6 | M3–O1 | 2.848(6) × 6 | M3–O1 | 2.83(2) × 6 |
| M1–O4 | 3.050(8) × 3 | M1–O2 | 3.210(15) × 3 | <M3–O>12 | 2.67 | <M3–O>18 | 2.78 |
| <M1–O>10 | 2.863 | <M1–O>10 | 2.937 | ||||
| M2–O2 | 2.388(7) | ||||||
| M2–O2 | 2.815(2) × 6 | ||||||
| M2–O2 | 3.150(5) × 3 | ||||||
| <M2–O>10 | 2.873 | ||||||
| S1–O1 | 1.378(10) | S1–O1 | 1.42(2) | S1–O2 | 1.50(3) × 6 | S1–O2 | 1.37(3) × 12 |
| S1–O4 | 1.446(4) × 3 | S1–O2 | 1.441(6) × 3 | S1–O1 | 1.358(9) × 3 | S1–O1 | 1.48(2) × 3 |
| <S1–O>4 | 1.429 | <S1–O>4 | 1.435 | <S1–O>9 | 1.45 | <S1–O>15 | 1.39 |
| S2–O2 | 1.485(8) | ||||||
| S2–O3 | 1.458(5) × 3 | ||||||
| <S2–O>4 | 1.465 | ||||||
| Temperature, °C | Space Group | IG (bits/atom) | IG, total (bits/cell) |
|---|---|---|---|
| 25 | P3m1 | 3.128 | 43.793 |
| 300 | 2.271 | 31.793 | |
| 500 (edge model) 1 | P63/mmc | 2.156 | 34.490 |
| 750 (apex model) 1 | P63/mmc | 2.156 | 34.490 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shablinskii, A.; Bubnova, R.; Shorets, O.; Krzhizhanovskaya, M.; Volkov, S.; Filatov, S. Novel High-Temperature Modification of Belomarinaite KNaSO4: Crystal Structure and Thermal Order–Disorder Phase Transitions. Crystals 2024, 14, 27. https://doi.org/10.3390/cryst14010027
Shablinskii A, Bubnova R, Shorets O, Krzhizhanovskaya M, Volkov S, Filatov S. Novel High-Temperature Modification of Belomarinaite KNaSO4: Crystal Structure and Thermal Order–Disorder Phase Transitions. Crystals. 2024; 14(1):27. https://doi.org/10.3390/cryst14010027
Chicago/Turabian StyleShablinskii, Andrey, Rimma Bubnova, Olga Shorets, Maria Krzhizhanovskaya, Sergey Volkov, and Stanislav Filatov. 2024. "Novel High-Temperature Modification of Belomarinaite KNaSO4: Crystal Structure and Thermal Order–Disorder Phase Transitions" Crystals 14, no. 1: 27. https://doi.org/10.3390/cryst14010027
APA StyleShablinskii, A., Bubnova, R., Shorets, O., Krzhizhanovskaya, M., Volkov, S., & Filatov, S. (2024). Novel High-Temperature Modification of Belomarinaite KNaSO4: Crystal Structure and Thermal Order–Disorder Phase Transitions. Crystals, 14(1), 27. https://doi.org/10.3390/cryst14010027