Mode-I Plane Elasticity Problem of Two Asymmetrical Edge Cracks Emanating from an Elliptical Hole in Two-Dimensional Decagonal Quasicrystals
Abstract
1. Introduction
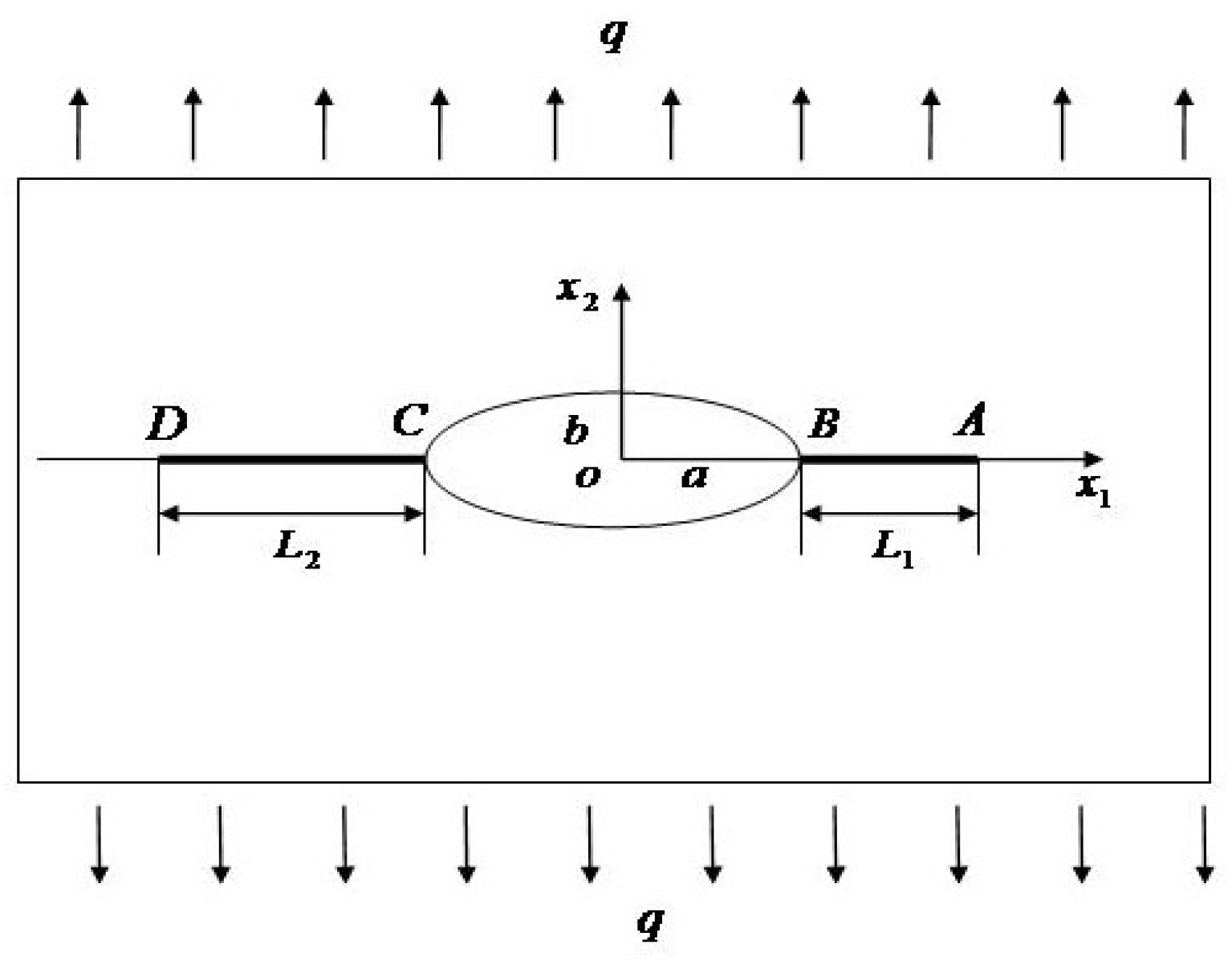
2. Basic Equation
3. Complex Expression of the Boundary Conditions
4. Mode-I Plane Elasticity Problem and Exact Solution
4.1. Stress Functions
- (1)
- Elliptical hole. When , the two asymmetrical edge cracks emanating from an elliptical hole degenerate into an elliptical hole, which can be obtained by Equations (31), (32), and (33):which are identical to the results of the reference [34].
- (2)
- Cross crack. When , the two asymmetrical edge cracks emanating from an elliptical hole degenerate into the asymmetrical cross crack, so we havewhere
- (3)
- T-shape crack. When , the two asymmetrical edge cracks emanating from an elliptical hole degenerate into a T-shaped crack, so we havewhere
- (4)
- Griffith crack. When , the two asymmetrical edge cracks emanating from an elliptical hole degenerate into a Griffith crack, so we havewhich are identical to the results of Ref. [35].
4.2. Stress Intensity Factors
- (1)
- When , Equation (56) becomeswhich is the case of a Griffith crack. Clearly, the stress intensity factor is the same as that of shown in Ref. [24].
- (2)
- When Equation (56) becomes
- (3)
- When Equation (56) becomeswherewhich is the stress intensity factor of a cross crack tip.
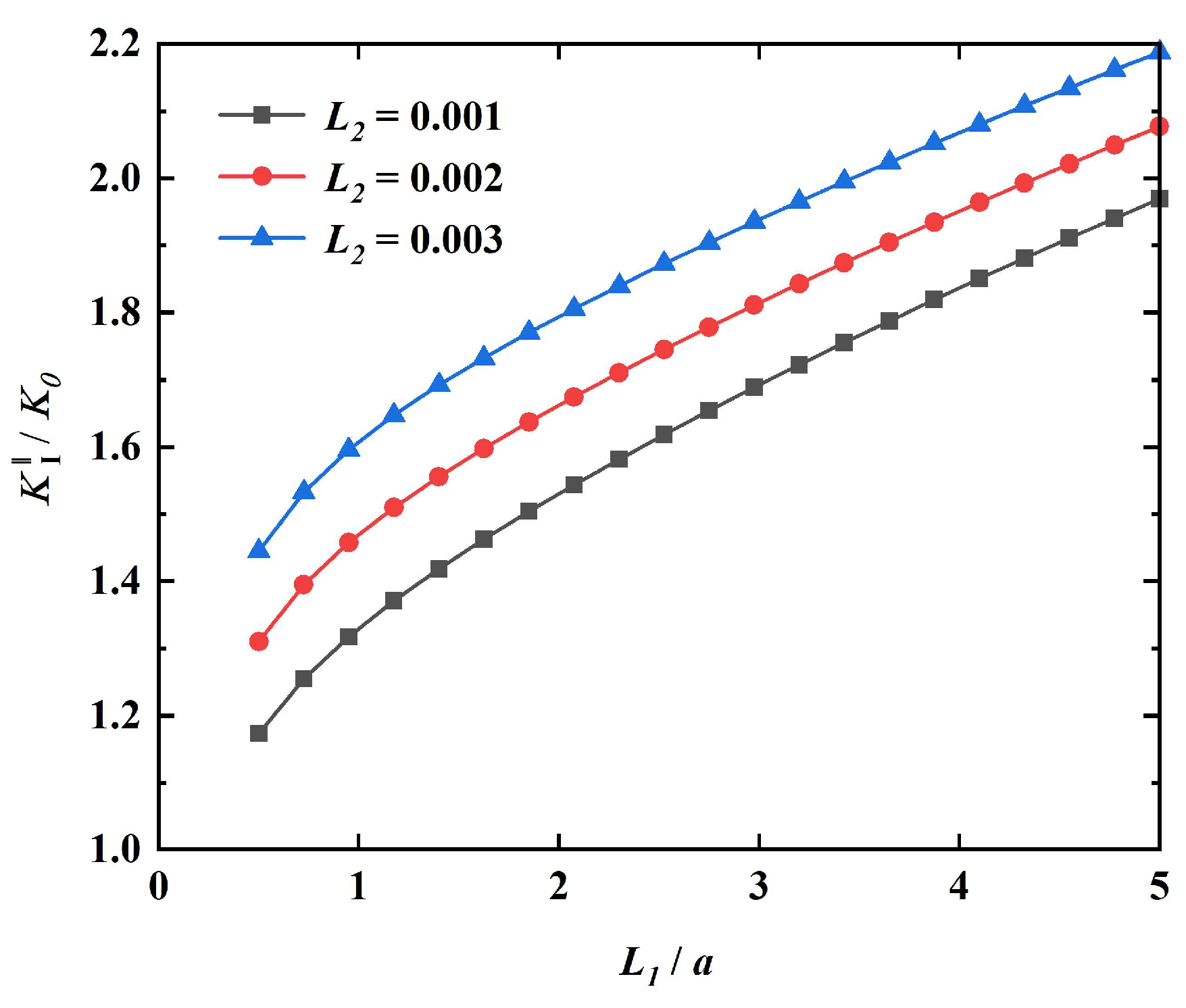
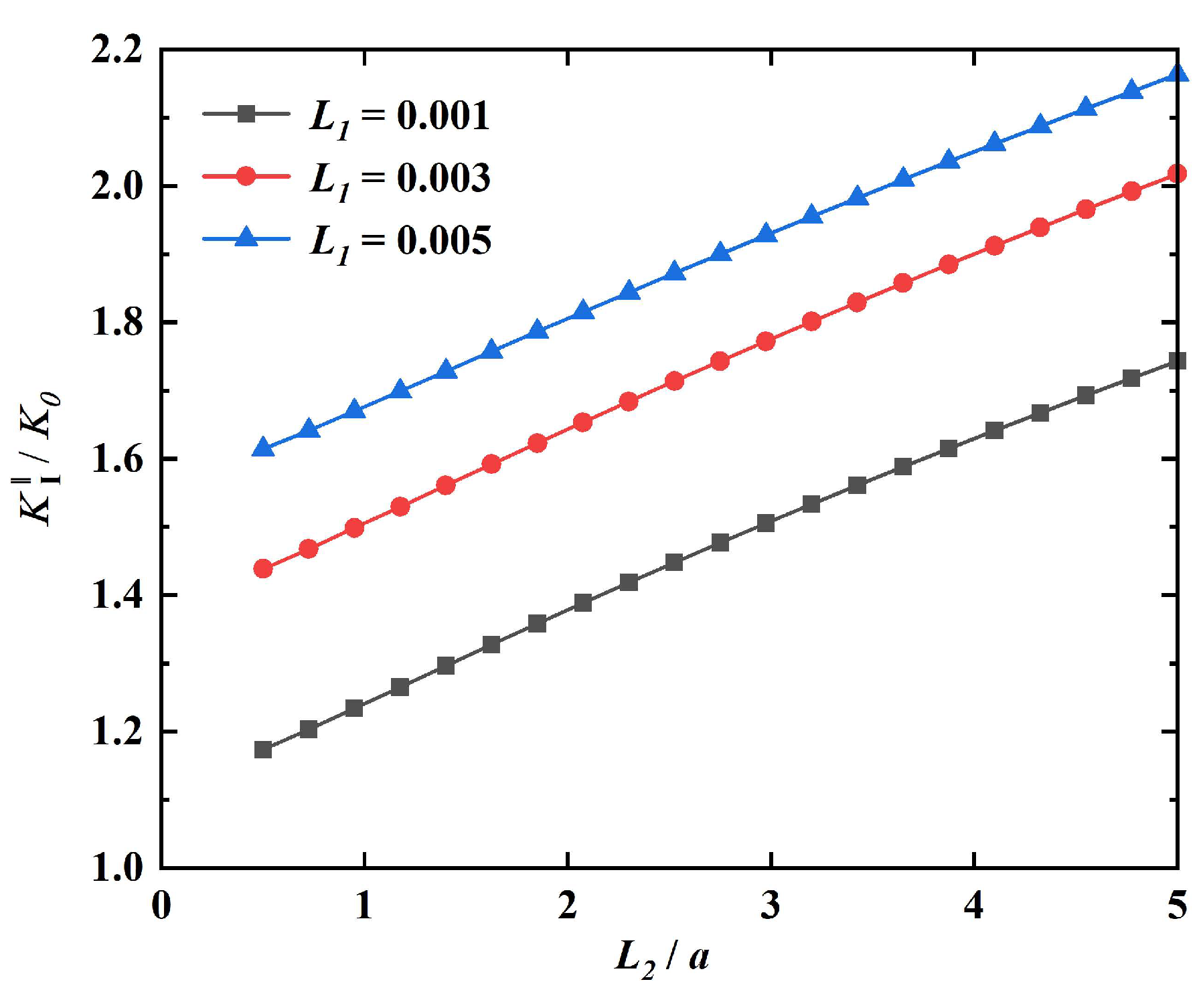
5. Numerical Results and Discussions
6. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shechtman, D.; Blech, I.; Gratias, D.; Cahn, J.W. Metallic phase with long-range orientational order and no translational symmetry. Phys. Rev. Lett. 1984, 53, 1951–1953. [Google Scholar] [CrossRef]
- Elser, V. Comment on quasicrystals: A new class of ordered structures. Phys. Rev. Lett. 1985, 54, 1730. [Google Scholar] [CrossRef] [PubMed]
- Kramer, P.; Neri, R. On periodic and non-periodic space fillings obtained by projection. Acta Crystallogr. Sect. A 1984, 40, 580–587. [Google Scholar] [CrossRef]
- Prekul, A.F.; Kuz’min, N.Y.; Shchegolikhina, N.I. Electronic structure of icosahedral quasicrystals. J. Alloys Compd. 2002, 342, 405–409. [Google Scholar] [CrossRef]
- Saito, H.; Fukamichi, K.; Goto, T.; Tsai, A.P.; Inoue, A.; Masumoto, T. Concentration dependence of the magnetic properties of melt-quenched P-type Mg30GdxZn70-x quasicrystals. J. Alloys Compd. 1997, 252, 6–11. [Google Scholar] [CrossRef]
- Mizutani, T.; Nakano, H.; Kashimoto, S.; Takatani, Y.; Mori, M.; Ishimasa, T.; Matsuo, S. Ten-fold-like magnetic anisotropy in electrical conductivity of AlPdMn icosahedral quasicrystal. J. Alloys Compd. 2002, 342, 360–364. [Google Scholar] [CrossRef]
- Meng, X.M.; Tong, B.Y.; Wu, Y.K. Mechanical properties of Al65 Cu20 Co15. Acta Metall. Sin. 1994, 30, 60–64. (In Chinese) [Google Scholar]
- Zhang, Z.; Urban, K. Transmission electron microscope observation of dislocation and stacking faults in a decagonal Al-Cu-Co alloy. Philos. Mag. Lett. 1989, 60, 97–102. [Google Scholar] [CrossRef]
- Dubois, J.M. New prospects from potential applications of quasicrystalline materials. Mater. Sci. Eng. A 2000, 294, 4–9. [Google Scholar] [CrossRef]
- Balbyshev, V.N.; King, D.J.; Khramov, A.N.; Kasten, L.S.; Donley, M.S. Investigation of quaternary Al-based quasicrystal thin films for corrosion protection. Thin Solid Film. 2004, 447–448, 558–563. [Google Scholar] [CrossRef]
- Dubois, J.M. So Useful, those quasicrystals. Isr. J. Chem. 2011, 11, 1168–1175. [Google Scholar] [CrossRef]
- Fikar, J. Al-Cu-Fe Quasicrystalline Coatings and Composites Studied by Mechanical Spectroscopy; Ecole Polytechnique Federale de Lausanne: Paris, France, 2002. [Google Scholar]
- Eisenhammer, T. Quasicrystal films: Numerical optimization as a solar selective absorber. Thin Solid Film. 1995, 270, 1–5. [Google Scholar] [CrossRef]
- Ding, D.H.; Yang, W.G.; Hu, C.Z.; Wang, R.H. Generalized elasticity theory of quasicrystals. Phys. Rev. B 1993, 48, 7003–7009. [Google Scholar] [CrossRef] [PubMed]
- Levine, D.; Lubensky, T.C.; Ostlund, S.; Ramaswamy, S.; Steinhardt, P.J.; Toner, J. Elasticity and dislocations in pentagonal and icosahedral quasicrystals. Phys. Rev. Lett. 1985, 54, 1520–1523. [Google Scholar] [CrossRef] [PubMed]
- Wang, R.H.; Yang, W.G.; Hu, C.Z.; Ding, D.H. Point and space groups and elastic behaviours of one-dimensional quasicrystals. J. Phys. Condens. Matter. 1997, 9, 2411–2422. [Google Scholar] [CrossRef]
- Fan, T.Y.; Mai, Y.W. Elasticity theory, fracture mechanics, and some relevant thermal properties of quasi-crystalline materials. Appl. Mech. Rev. 2004, 57, 325–343. [Google Scholar] [CrossRef]
- Ding, D.H.; Wang, R.; Yang, W.; Hu, C.; Qin, Y. Elasticity theory of straight dislocations in quasicrystals. Philos. Mag. Lett. 1995, 72, 352–359. [Google Scholar] [CrossRef]
- Peng, Y.Z.; Fan, T.Y. Elastic theory of 1D quasiperiodic stacking of 2D crystals. J. Phys. Condens. Matter 2000, 12, 9381–9387. [Google Scholar] [CrossRef]
- Liu, G.T.; Fan, T.Y.; Guo, R.P. Governing equations and general solutions of plane elasticity of one-dimensional quasicrystals. Int. J. Solids Struct. 2004, 41, 3949–3959. [Google Scholar] [CrossRef]
- Fan, T.Y.; Guo, L.H. The final governing equation and fundamental solution of plane elasticity of icosahedral quasicrystals. Phys. Lett. A 2005, 341, 235–239. [Google Scholar] [CrossRef]
- Peng, Y.Z.; Fan, T.Y. Crack and indentation problems for one-dimensional hexagonal Quasicrystals. Eur. Phys. J. B 2001, 21, 39–44. [Google Scholar] [CrossRef]
- Gao, Y.; Xu, S.P.; Zhao, B.S. General solutions of equilibrium equations for 1D hexagonal quasicrystals. Mech. Res. Commun. 2009, 36, 302–308. [Google Scholar] [CrossRef]
- Li, X.F.; Fan, T.Y.; Sun, Y.F. A decagonal quasicrystal with a Griffth crack. Philos. Mag. A 1999, 79, 1943–1952. [Google Scholar]
- Fan, T.Y. Mathematical Theory of Elasticity of Quasicrystals and Its Applications; Science Press: Beijing, China; Springer-Verlag: Heidelberg, Germany, 2010. [Google Scholar]
- Liu, G.T.; Guo, R.P.; Fan, T.Y. On the interaction between dislocations and cracks in one-dimensional hexagonal quasicrystals. Chin. Phys. 2003, 12, 1149–1155. [Google Scholar]
- Li, X.F.; Fan, T.Y. A straight dislocation in one dimensional hexagonal quasicrystals. Phys. Status Solidi 1999, 212, 19–26. [Google Scholar] [CrossRef]
- Guo, J.H.; Liu, G.T. Analytic solutions to problem of elliptic hole with two straight cracks in one-dimensional hexagonal quasicrystals. Appl. Math. Mech. 2008, 29, 485–493. [Google Scholar] [CrossRef]
- Guo, J.H.; Liu, G.T. Exact analytic solutions for an elliptic hole with asymmetric collinear cracks in a one-dimensional hexagonal quasi-crystal. Chin. Phys. B 2008, 17, 2610–2620. [Google Scholar]
- Guo, J.H.; Lu, Z.X. Exact solution of four cracks originating from an elliptical hole in one-dimensional hexagonal quasicrystals. Appl. Math. Comput. 2011, 217, 9397–9403. [Google Scholar] [CrossRef]
- Gao, Y.; Zhao, Y.T.; Zhao, B.S. Boundary value problems of holomorphic vector functions in 1D QCs. Phys. B Condens. Matter 2007, 394, 56–61. [Google Scholar] [CrossRef]
- Bendersky, L. Quasicrystal with one-dimensional translational symmetry and a tenfold rotation axis. Phys. Rev. Lett. 1985, 55, 1461–1463. [Google Scholar] [CrossRef]
- Fung, K.K.; Yang, C.Y.; Zhou, Y.Q.; Zhao, J.G.; Zhan, W.S.; Shen, B.G. Icosahedrally related decagonal quasicrystal in rapidly cooled Al-14-at.%-Fe alloy. Phys. Rev. Lett. 1986, 56, 2060–2063. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Li, D.X.; Kuo, K.H. New type of two-dimensional quasicrystal with twelve fold rotational symmetry. Phys. Rev. Lett. 1988, 60, 1645–1648. [Google Scholar] [CrossRef] [PubMed]
- Li, X.F.; Fan, T.Y. New method for solving elasticity problems of some planar quasicrystals and solutions. Chin. Phys. Lett. 1998, 15, 278–280. [Google Scholar] [CrossRef]
- Li, L.H.; Fan, T.Y. Complex variable function method for the plane elasticity and the dislocation problem of quasicrystals with point group 10 mm. Phys. Lett. A 2008, 372, 510–514. [Google Scholar] [CrossRef]
- Li, L.H.; Fan, T.Y. Exact solutions of two semi-infinite collinear cracks in a strip of one dimensional hexagonal quasicrystal. Appl. Math. Comput. 2008, 196, 1–5. [Google Scholar] [CrossRef]
- Wang, X.; Zhong, Z. Interaction between a semi-infinite crack and a straight dislocation in a decagonal quasicrystal. Int. J. Eng. Sci. 2004, 42, 521–538. [Google Scholar] [CrossRef]
- Li, L.H. Complex potential theory for the plane elasticity problem of decagonal quasicrystals and its application. Appl. Math. Comput. 2013, 219, 10105–10111. [Google Scholar] [CrossRef]
- Radi, E.; Mariano, P.M. Stationary straight cracks in quasicrystals. Int. J. Fract. 2010, 166, 102–120. [Google Scholar] [CrossRef]
- Li, L.H.; Liu, G.T. Stroh formalism for icosahedral quasicrystal and its application. Phys. Lett. A 2012, 376, 987–990. [Google Scholar] [CrossRef]
- Altay, G.; Cengiz, D.M. On the fundamental equations of piezoelasticity of quasicrystal media. Int. J. Solids Struct. 2012, 49, 3255–3262. [Google Scholar] [CrossRef]
- Tounsi, A.; Mostefa, A.H.; Attia, A.; Bousahla, A.A.; Bourada, F.; Tounsi, A.; Al-Osta, M.A. Free vibration investigation of functionally graded plates with temperature-dependent properties resting on a viscoelastic foundation. Struct. Eng. Mech. 2023, 86, 1–16. [Google Scholar]
- Bounouara, F.; Aldosari, S.M.; Chikh, A.; Kaci, A.; Bousahla, A.A.; Anis, A.; Bourada, F.; Tounsi, A.; Benrahou, K.H.; Albalawi, H.; et al. The effect of visco-Pasternak foundation on the free vibration behavior of exponentially graded sandwich plates with various boundary conditions. Steel Compos. Struct. 2023, 46, 367–383. [Google Scholar]
- Li, L.H.; Fan, T.Y. Stress potential function formulation and complex variable function method for solving the elasticity of quasicrystals of point group 10 and the exact solution for the notch problem. J. Phys. Condens. Matter 2006, 18, 10631–10641. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, J. Mode-I Plane Elasticity Problem of Two Asymmetrical Edge Cracks Emanating from an Elliptical Hole in Two-Dimensional Decagonal Quasicrystals. Crystals 2023, 13, 1038. https://doi.org/10.3390/cryst13071038
Yu J. Mode-I Plane Elasticity Problem of Two Asymmetrical Edge Cracks Emanating from an Elliptical Hole in Two-Dimensional Decagonal Quasicrystals. Crystals. 2023; 13(7):1038. https://doi.org/10.3390/cryst13071038
Chicago/Turabian StyleYu, Jing. 2023. "Mode-I Plane Elasticity Problem of Two Asymmetrical Edge Cracks Emanating from an Elliptical Hole in Two-Dimensional Decagonal Quasicrystals" Crystals 13, no. 7: 1038. https://doi.org/10.3390/cryst13071038
APA StyleYu, J. (2023). Mode-I Plane Elasticity Problem of Two Asymmetrical Edge Cracks Emanating from an Elliptical Hole in Two-Dimensional Decagonal Quasicrystals. Crystals, 13(7), 1038. https://doi.org/10.3390/cryst13071038




