Review on Eutectic-Type Alloys Solidified under Static Magnetic Field
Abstract
1. Introduction
2. Basic Physical Principles and General Experimental Methods of SMFs in Material Processing
2.1. Magnetic Force
2.2. Magnetic Energy
2.3. General Experimental Methods
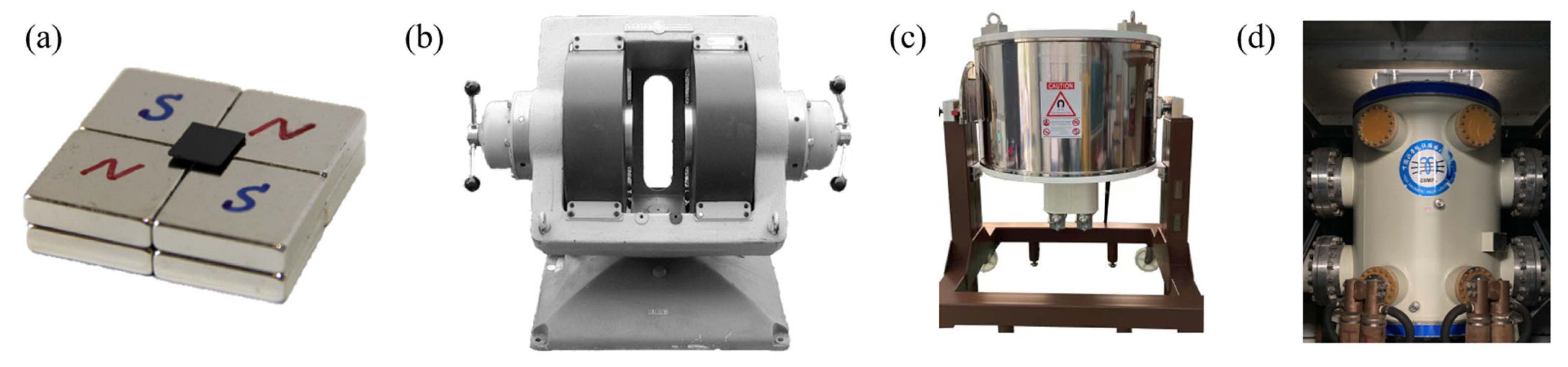
2.3.1. Magnetic Field Generator
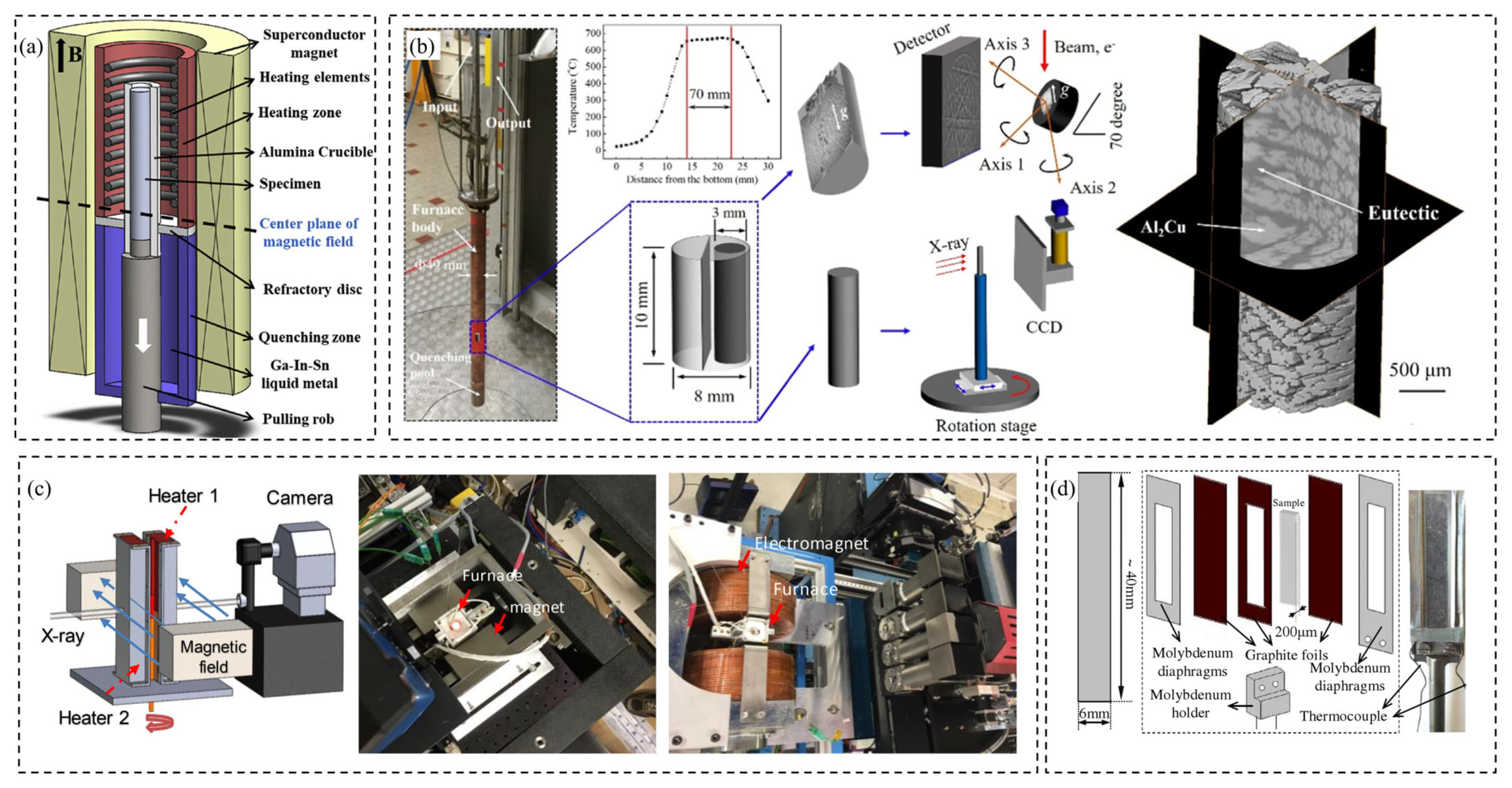
2.3.2. General Experimental Facilities
3. Magnetohydrodynamic Effect
3.1. Magnetic Braking Effect during Solidification
3.2. TEM Effect during Solidification
- (1)
- The thermoelectric powers of the liquid and the solid must be different, Sl − Ss ≠ 0.
- (2)
- A temperature gradient exists at the vicinity of the interface.
- (3)
- The temperature gradient must not be perpendicular to the interface.
4. Magnetic Force during Solidification
5. Magnetic Gibbs Energy during Phase Transition
5.1. Phase Transition Temperature and Nucleation Events
5.2. Magnetic Orientation of Crystals Assisted by High SMF
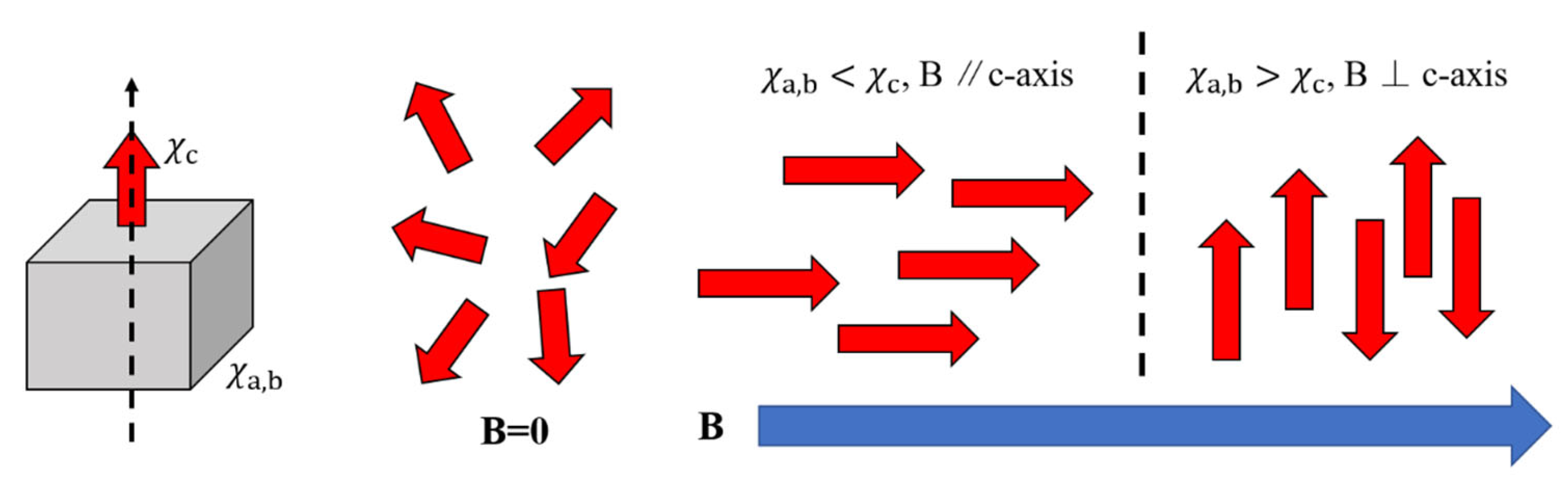
5.2.1. Magnetic Anisotropy of Crystal
5.2.2. Magnetic Torque
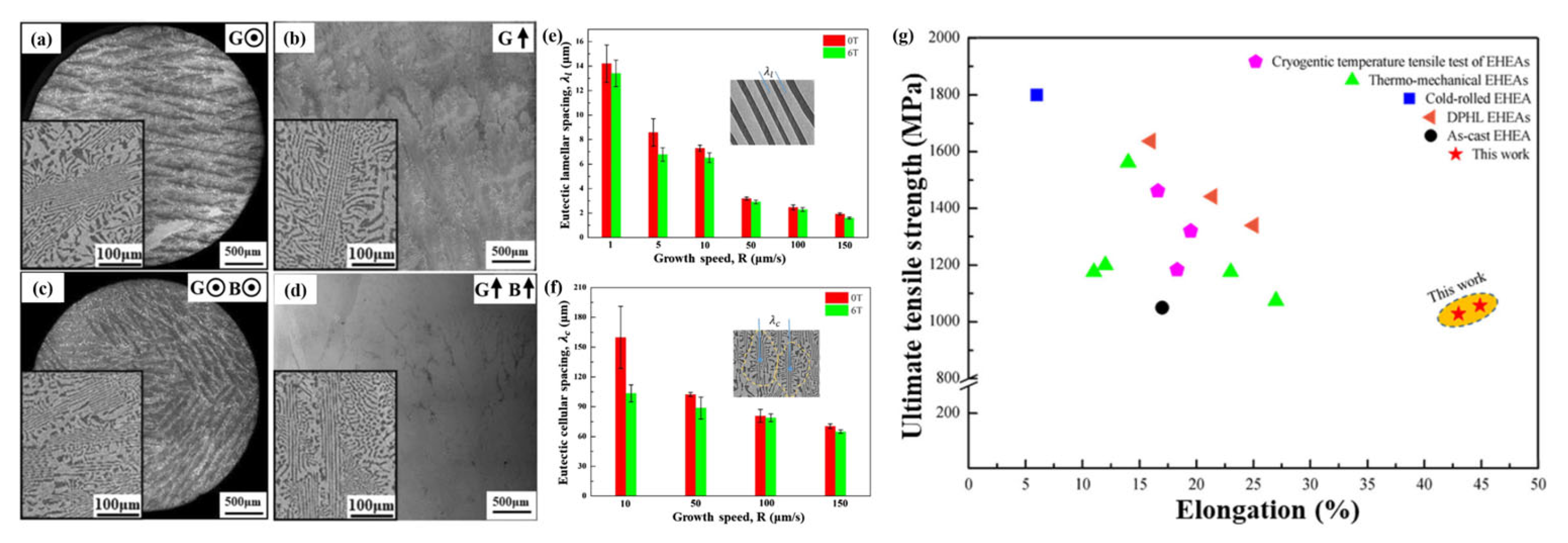
6. Eutectic High Entropy Alloy Solidified under the SMF
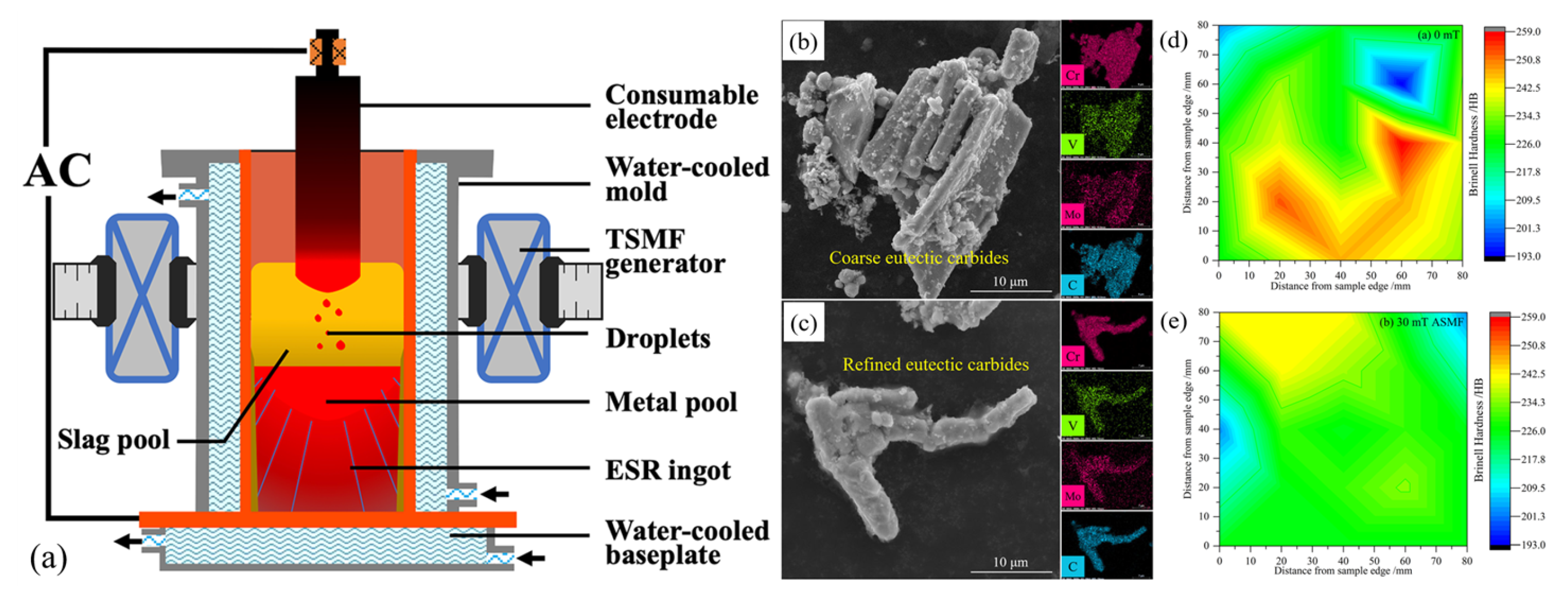
7. Industry Application
8. Summary and Perspective
- (1)
- Magnetic braking effect: The SMF can significantly reduce or even eliminate melt convections, thereby promoting diffusion-limited crystal growth. This can lead to the production of high-quality single crystals.
- (2)
- TEM effect: The interaction between the thermoelectric current near the solid–liquid interface and the applied SMF generates a TEM force, which can influence the atomic transport behavior at the interface. Once this force is greater than a critical value, dendrites can be broken and fragments can be migrated. As a result, a CET event happens. Thus, TEM convection can not only modify the morphology of the solid–liquid interface and change solute distribution, but also refine the microstructure. Ex situ and in situ observation of the solidification process have been conducted under the weak SMF to reveal the TEM effect on the evolution of microstructure.
- (3)
- Gradient high SMF effect: The gradient magnetic force induced by the SMF can effectively control the migration of solute and primary crystals, making it useful for processing graded metal materials, removing Fe-rich intermetallic compounds in recycled aluminum alloys, and levitating materials.
- (4)
- Magnetic Gibbs energy effect: The high SMF can influence the phase transition of during solidification, which may be attributed to the change of solid–liquid interfacial free energy.
- (5)
- Magnetic torque effect: Crystals with magnetic anisotropy in their unit cell can be oriented by high SMF. This is for the sake of the magnetic torque on the crystal and leads to the rotation of the crystal along the direction of the SMF. The orientation efficiency mainly depends on the crystal size and MFD.
- (6)
- Effect on EHEAs: The high SMF can effectively affect the microstructures of EHEAs, which provides a potential way to enhance their mechanical properties.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chang, I.; Cai, Q. From simple binary to complex multicomponent eutectic alloys. Prog. Mater. Sci. 2022, 123, 100779. [Google Scholar] [CrossRef]
- Khorasani, M.; Ghasemi, A.; Leary, M.; Sharabian, E.; Cordova, L.; Gibson, I.; Downing, D.; Bateman, S.; Brandt, M.; Rolfe, B. The effect of absorption ratio on meltpool features in laser-based powder bed fusion of IN718. Opt. Laser Technol. 2022, 153, 108263. [Google Scholar] [CrossRef]
- Kim, S.H.; Yeon, S.-M.; Lee, J.H.; Kim, Y.W.; Lee, H.; Park, J.; Lee, N.-K.; Choi, J.P.; Aranas, C.; Lee, Y.J.; et al. Additive manufacturing of a shift block via laser powder bed fusion: The simultaneous utilisation of optimised topology and a lattice structure. Virtual Phys. Prototyp. 2020, 15, 460–480. [Google Scholar] [CrossRef]
- Räbiger, D.; Zhang, Y.; Galindo, V.; Franke, S.; Willers, B.; Eckert, S. The relevance of melt convection to grain refinement in Al-Si alloys solidified under the impact of electric currents. Acta Mater. 2014, 79, 327–338. [Google Scholar] [CrossRef]
- Abou-Khalil, L.; Salloum-Abou-Jaoude, G.; Reinhart, G.; Pickmann, C.; Zimmermann, G.; Nguyen-Thi, H. Influence of gravity level on Columnar-to-Equiaxed Transition during directional solidification of Al-20wt.%Cu alloys. Acta Mater. 2016, 110, 44–52. [Google Scholar] [CrossRef]
- Wang, S.; Kang, J.; Guo, Z.; Lee, T.L.; Zhang, X.; Wang, Q.; Deng, C.; Mi, J. In situ high speed imaging study and modelling of the fatigue fragmentation of dendritic structures in ultrasonic fields. Acta Mater. 2019, 165, 388–397. [Google Scholar] [CrossRef]
- Sun, Z.H.I.; Guo, M.; Vleugels, J.; Van der Biest, O.; Blanpain, B. Processing of non-ferromagnetic materials in strong static magnetic field. Curr. Opin. Solid State Mater. Sci. 2013, 17, 193–201. [Google Scholar] [CrossRef]
- Liu, T.; Wang, Q.; Yuan, Y.; Wang, K.; Li, G. High-gradient magnetic field-controlled migration of solutes and particles and their effects on solidification microstructure: A review. Chin. Phys. B 2018, 27, 118103. [Google Scholar] [CrossRef]
- Vives, C.; Perry, C. Solidification of a pure metal in the presence of a stationary magnetic field. Int. Commun. Heat Mass Transf. 1986, 13, 253–263. [Google Scholar] [CrossRef]
- Vives, C.; Perry, C. Transfer-effects of magnetially damped convection during the controlled solidification of metals and alloys. Int. J. Heat Mass Transf. 1987, 30, 479–496. [Google Scholar] [CrossRef]
- Yasuda, H.; Ohnaka, I.; Ninomiya, Y.; Ishii, R.; Fujita, S.; Kishio, K. Levitation of metallic melt by using the simultaneous imposition of the alternating and the static magnetic fields. J. Cryst. Growth 2004, 260, 475–485. [Google Scholar] [CrossRef]
- Asai, S.; Sassa, K.-S.; Tahashi, M. Crystal orientation of non-magnetic materials by imposition of a high magnetic field. Sci. Technol. Adv. Mater. 2016, 4, 455–460. [Google Scholar] [CrossRef]
- Koyama, K.; Mitsui, Y.; Choi, E.S.; Ikehara, Y.; Palm, E.C.; Watanabe, K. Change of the equilibrium state of ferromagnetic MnBi by high magnetic fields. J. Alloys Compd. 2011, 509, L78–L80. [Google Scholar] [CrossRef]
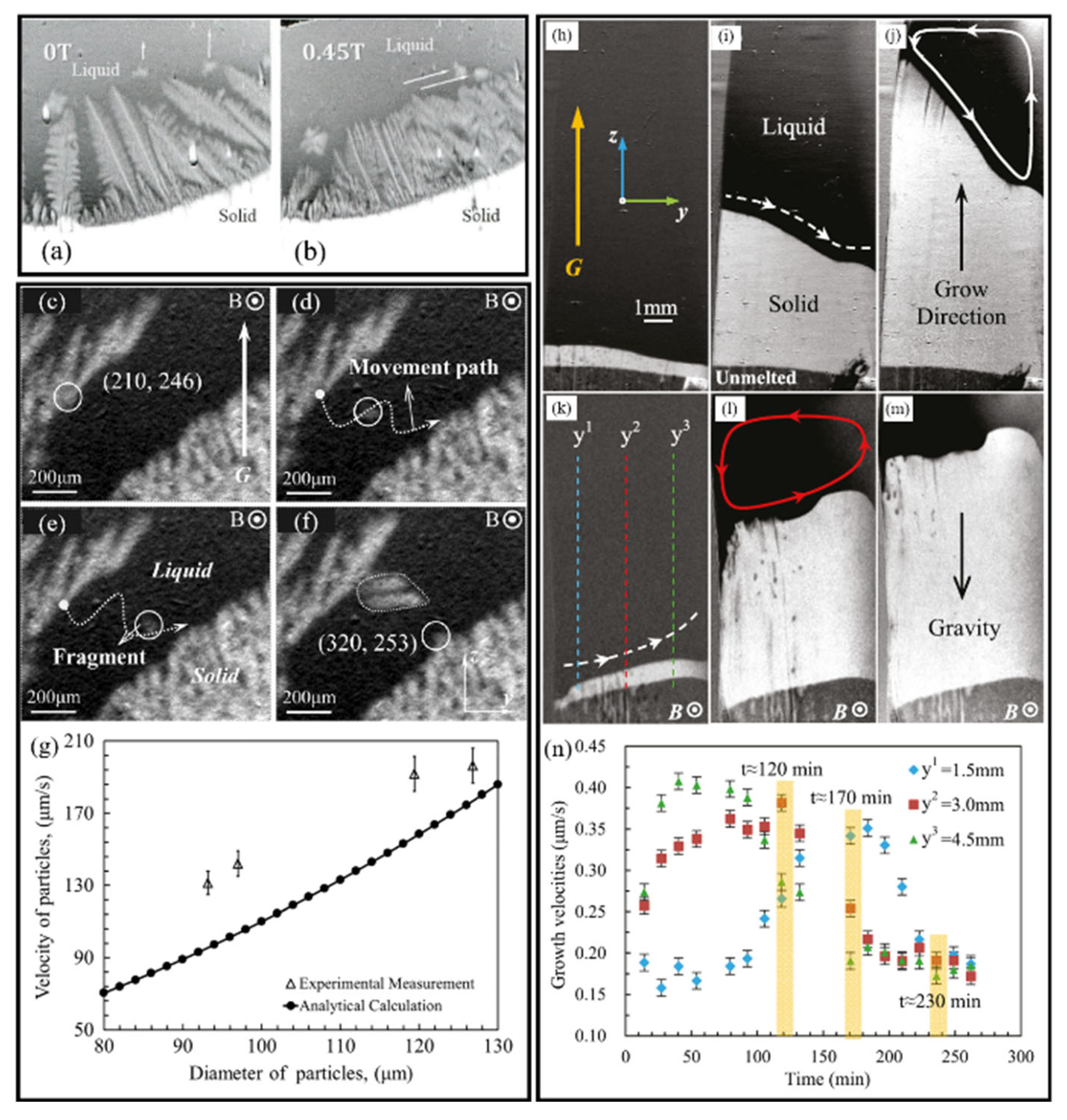
- Li, X.; Ren, Z.; Fautrelle, Y. Effect of a high axial magnetic field on the microstructure in a directionally solidified Al-Al2Cu eutectic alloy. Acta Mater. 2006, 54, 5349–5360. [Google Scholar] [CrossRef]
- Li, X.; Ren, Z.; Fautrelle, Y. The spiral growth of lamellar eutectics in a high magnetic field during the directional solidification process. Scr. Mater. 2007, 56, 505–508. [Google Scholar] [CrossRef]
- Li, X.; Fautrelle, Y.; Ren, Z. Influence of thermoelectric effects on the solid-liquid interface shape and cellular morphology in the mushy zone during the directional solidification of Al-Cu alloys under a magnetic field. Acta Mater. 2007, 55, 3803–3813. [Google Scholar] [CrossRef]
- Huang, C.; Shuai, S.; Wang, P.; Liu, X.; Wang, J.; Ren, Z. The effect of static magnetic field on solid-liquid interfacial free energy of Al-Cu alloy system. Scr. Mater. 2020, 187, 232–236. [Google Scholar] [CrossRef]
- Beaugnon, E.; Bourgault, D.; Braithwaite, D.; de Rango, P.; Perrier de la Bathie, R.; Sulpice, A.; Tournier, R. Material processing in high static magnetic field. A review of an experimental study on levitation, phase separation, convection and texturation. J. Phys. I 1993, 3, 399–421. [Google Scholar] [CrossRef]
- Lehmann, P.; Moreau, R.; Camel, D.; Bolcato, R. Modification of interdendritic convection in directional solidification by a uniform magnetic field. Acta Mater. 1998, 46, 4067–4079. [Google Scholar] [CrossRef]
- Zuo, X.; Zhao, C.; Niu, R.; Wang, E.; Han, K. Microstructural dependence of magnetoresistance in CuAg alloy solidified with high magnetic field. J. Mater. Process. Technol. 2015, 224, 208–212. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, C.J.; Liu, T.; Wang, K.; He, J.C. Control of solidified structures in aluminum-silicon alloys by high magnetic fields. J. Mater. Sci. 2007, 42, 10000–10006. [Google Scholar] [CrossRef]
- Liu, T.; Wang, Q.; Zhang, H.-W.; Lou, C.-S.; Nakajima, K.; He, J.-C. Effects of high magnetic fields on solidification microstructure of Al-Si alloys. J. Mater. Sci. 2010, 46, 1628–1634. [Google Scholar] [CrossRef]
- Chen, X.; Zhong, Y.; Zheng, T.; Shen, Z.; Wang, J.; Fan, L.; Zhai, Y.; Peng, M.; Zhou, B.; Ren, W.; et al. Refinement of primary Si in the bulk solidified Al-20wt.%Si alloy assisting by high static magnetic field and phosphorus addition. J. Alloys Compd. 2017, 714, 39–46. [Google Scholar] [CrossRef]
- Wang, Q.; Lou, C.; Liu, T.; Pang, X.; Nakajima, K.; He, J. Effects of Uniform and Gradient High Magnetic Fields on Gravity Segregation in Aluminum Alloys. ISIJ Int. 2009, 49, 1094–1097. [Google Scholar] [CrossRef]
- Li, L.; Zhang, Y.; Esling, C.; Jiang, H.; Zhao, Z.; Zuo, Y.; Cui, J. Influence of a high magnetic field on the precipitation behavior of the primary Al3Fe phase during the solidification of a hypereutectic Al-3.31wt.%Fe alloy. J. Cryst. Growth 2012, 339, 61–69. [Google Scholar] [CrossRef]
- Wu, M.; Liu, T.; Dong, M.; Sun, J.; Dong, S.; Wang, Q. Directional solidification of Al-8 wt. %Fe alloy under high magnetic field gradient. J. Appl. Phys. 2017, 121, 064901. [Google Scholar] [CrossRef]
- Tang, P.; Tian, Y.; Liu, S.; Lv, Y.; Xie, Y.; Yan, J.; Liu, T.; Wang, Q. Microstructure development in eutectic Al-Fe alloy during directional solidification under high magnetic fields at different growth velocities. J. Mater. Sci. 2021, 56, 16134–16144. [Google Scholar] [CrossRef]
- Yan, J.; Liu, T.; Wang, M.; Sun, J.; Dong, S.; Zhao, L.; Wang, Q. Constitutional supercooling and corresponding microstructure transition triggered by high magnetic field gradient during directional solidification of Al-Fe eutectic alloy. Mater. Charact. 2022, 188, 111920. [Google Scholar] [CrossRef]
- Xiao, Y.; Liu, T.; Tong, Y.; Dong, M.; Li, J.; Wang, J.; Wang, Q. Microstructure evolution of peritectic Al-18at.%Ni alloy directionally solidified in high magnetic fields. J. Mater. Sci. Technol. 2021, 76, 51–59. [Google Scholar] [CrossRef]
- Shuai, S.; Lin, X.; Dong, Y.; Hou, L.; Liao, H.; Wang, J.; Ren, Z. Three dimensional dendritic morphology and orientation transition induced by high static magnetic field in directionally solidified Al-10 wt.%Zn alloy. J. Mater. Sci. Technol. 2019, 35, 1587–1592. [Google Scholar] [CrossRef]
- Moreau, R.; Laskar, O.; Tanaka, M.; Camel, D. Thermoelectric magnetohydrodynamic effects on solidification of metallic alloys in the dendritic regime. Mater. Sci. Eng. A 1993, 173, 93–100. [Google Scholar] [CrossRef]
- Shen, Z.; Peng, M.; Zhu, D.; Zheng, T.; Zhong, Y.; Ren, W.; Li, C.; Xuan, W.; Ren, Z. Evolution of the microstructure and solute distribution of Sn-10wt.%Bi alloys during electromagnetic field-assisted directional solidification. J. Mater. Sci. Technol. 2019, 35, 568–577. [Google Scholar] [CrossRef]
- Shen, Z.; Zhou, B.; Zhong, Y.; Zheng, T.; Ren, W.; Lei, Z.; Ren, Z. Revealing influence mechanism of a transverse static magnetic field on the refinement of primary dendrite spacing during directional solidification. J. Cryst. Growth 2019, 517, 54–58. [Google Scholar] [CrossRef]
- Song, H.; Tewari, S.N.; de Groh, H.C., III. Convection during thermally unstable solidification of Pb-Sn in a magnetic field. Metall. Mater. Trans. A 1996, 27, 1095–1110. [Google Scholar] [CrossRef]
- Kaldre, I.; Fautrelle, Y.; Etay, J.; Bojarevics, A.; Buligins, L. Thermoelectric current and magnetic field interaction influence on the structure of directionally solidified Sn-10wt.%Pb alloy. J. Alloys Compd. 2013, 571, 50–55. [Google Scholar] [CrossRef]
- Du, D.; Hou, L.; Gagnoud, A.; Ren, Z.; Fautrelle, Y.; Cao, G.; Li, X. Effect of an axial high magnetic field on Sn dendrite morphology of Pb-Sn alloys during directional solidification. J. Alloys Compd. 2014, 588, 190–198. [Google Scholar] [CrossRef]
- Li, L.; Zhang, R.; Ban, C.; Zhang, H.; Liu, T.; Zhang, H.; Wang, X.; Esling, C.; Cui, J. Growth behavior of Zn-rich phase in Zn-Mg alloy under a high magnetic field. Mater. Charact. 2019, 151, 191–202. [Google Scholar] [CrossRef]
- Li, L.; Ban, C.; Bi, Y.; Zhang, R.; Zhang, H.; Liu, T.; Zhu, Q.; Zuo, Y.; Wang, X.; Cui, J. A crystallographic study on the growth of Laves phase MgZn2 during the solidification process of Zn-Mg alloy under a high magnetic field. J. Mater. Sci. 2018, 53, 15181–15195. [Google Scholar] [CrossRef]
- Zhai, C.; Luo, Q.; Cai, Q.; Guan, R.; Li, Q. Thermodynamically analyzing the formation of Mg12Nd and Mg41Nd5 in Mg-Nd system under a static magnetic field. J. Alloys Compd. 2019, 773, 202–209. [Google Scholar] [CrossRef]
- He, Y.; Li, J.; Li, L.; Wang, J.; Yildiz, E.; Beaugnon, E. Magnetic-field-induced chain-like assemblies of the primary phase during non-equilibrium solidification of a Co-B eutectic alloy: Experiments and modeling. J. Alloys Compd. 2020, 815, 152446. [Google Scholar] [CrossRef]
- Bu, F.; Zhang, Y.; Liu, H.; Wang, J.; Beaugnon, E.; Li, J.; He, Y. Magnetic field intensity dependent microstructure evolution and recrystallization behavior in a Co-B eutectic alloy. J. Mater. Sci. Technol. 2023, 138, 93–107. [Google Scholar] [CrossRef]
- Wang, J.; He, Y.; Li, J.; Kou, H.; Beaugnon, E. Strong magnetic field effect on the nucleation of a highly undercooled Co-Sn melt. Sci. Rep. 2017, 7, 4958. [Google Scholar] [CrossRef]
- Liu, T.; Wang, Q.; Gao, A.; Zhang, C.; Li, D.; He, J. Crystal orientation and grain alignment in a hypoeutectic Mn-Sb alloy under high magnetic field conditions. J. Alloys Compd. 2009, 481, 755–760. [Google Scholar] [CrossRef]
- Li, X.; Ren, Z.; Fautrelle, Y. Effect of high magnetic fields on the microstructure in directionally solidified Bi-Mn eutectic alloy. J. Cryst. Growth 2007, 299, 41–47. [Google Scholar] [CrossRef]
- Cao, J.; Shuai, S.; Huang, C.; Hu, T.; Chen, C.; Wang, J.; Ren, Z. 4D synchrotron X-ray tomographic study of the influence of transverse magnetic field on iron intermetallic compounds precipitation behavior during solidification of Al-Si-Fe alloy. Intermetallics 2022, 143, 107471. [Google Scholar] [CrossRef]
- Du, D.; Fautrelle, Y.; Ren, Z.; Moreau, R.; Li, X. Effect of a high magnetic field on the growth of ternary Al-Cu-Ag alloys during directional solidification. Acta Mater. 2016, 121, 240–256. [Google Scholar] [CrossRef]
- Du, D.; Dong, A.; Shu, D.; Zhu, G.; Sun, B.; Li, X. Microstructure and Crystallization Evolution of Directionally Solidified Al-Cu-Si Alloys with the Assistance of a Static Magnetic Field. Metall. Mater. Trans. A 2022, 143, 107471. [Google Scholar] [CrossRef]
- Liu, H.; Xuan, W.; Xie, X.; Yu, J.; Wang, J.; Li, X.; Zhong, Y.; Ren, Z.; Wang, H.; Dai, Y. Effect of a high magnetic field on solidification structure in directionally solidified NiAl-Cr(Mo)-Hf eutectic alloy. J. Alloys Compd. 2018, 737, 74–82. [Google Scholar] [CrossRef]
- Utech, H.P.; Flemings, M.C. Elimination of Solute Banding in Indium Antimonide Crystals by Growth in a Magnetic Field. J. Appl. Phys. 1966, 37, 2021–2024. [Google Scholar] [CrossRef]
- Shercliff, J.A. Thermoelectric magnetohydrodynamics. J. Fluid Mech. 2006, 91, 231–251. [Google Scholar] [CrossRef]
- Zheng, T.; Zhong, Y.; Dong, L.; Zhou, B.; Ren, Z.; Debray, F.; Beaugnon, E. Orientation of Magnetized MnBi in a Strong Static Magnetic Field. Metall. Mater. Trans. A 2018, 49, 1981–1985. [Google Scholar] [CrossRef]
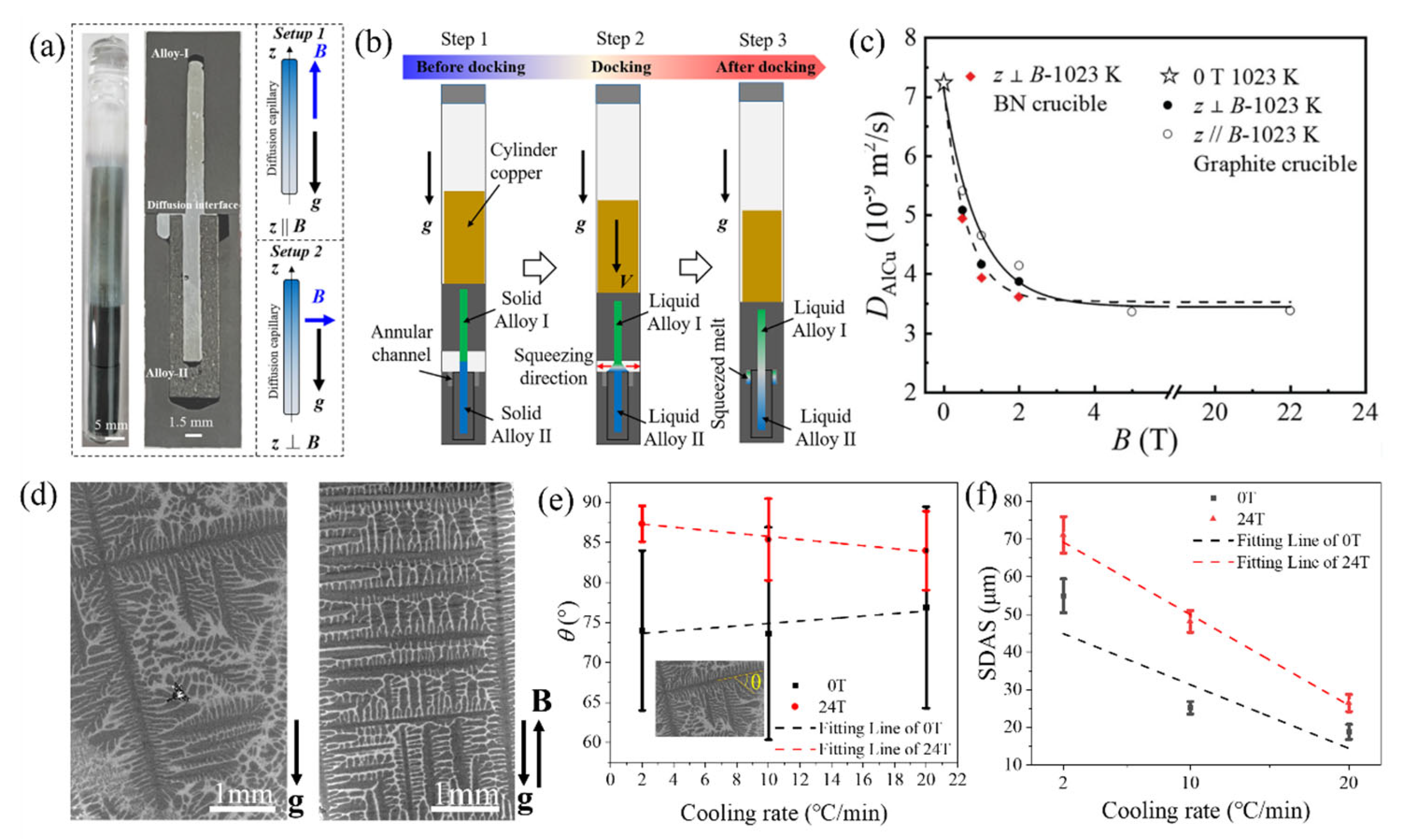
- Botton, V.; Lehmann, P.; Bolcato, R.; Moreau, R.; Haettel, R. Measurement of solute diffusivities. Part II. Experimental measurements in a convection-controlled shear cell. Interest of a uniform magnetic field. Int. J. Heat Mass Transf. 2001, 44, 3345–3357. [Google Scholar] [CrossRef]
- Miyake, T.; Inatomi, Y.; Kuribayashi, K. Measurement of Diffusion Coefficient in Liquid Metal under Static Magnetic Field. Jpn. J. Appl. Phys. 2002, 41, L811–L813. [Google Scholar] [CrossRef]
- Cahoon, J.R.; Youdelis, W.V. Application of a magnetic field to suppress convective velocities during liquid diffusion. Can. Metall. Q. 2013, 8, 39–40. [Google Scholar] [CrossRef]
- Liu, Y.; Lin, W.; Zhou, B.; Zheng, T.; Zhong, Y.; Zhang, L. A novel gravity-assisted automatic docking device for studying diffusion in liquid metal melts assisted by a strong static magnetic field. Rev. Sci. Instrum. 2021, 92, 094903. [Google Scholar] [CrossRef] [PubMed]
- Zhou, B.; Lin, W.; Liu, Y.; Cai, H.; Zheng, T.; Liu, C.; Zhong, Y. Atomic diffusion in liquid Al-Cu melt under static magnetic fields. J. Appl. Phys. 2022, 132, 165106. [Google Scholar] [CrossRef]
- Cheng, N.; Guo, R.; Shuai, S.; Wang, J.; Xia, M.; Li, J.; Ren, Z.; Li, J.; Wang, Q. Influence of static magnetic field on the heterogeneous nucleation behavior of Al on single crystal Al2O3 substrate. Materialia 2020, 13, 100847. [Google Scholar] [CrossRef]
- Wang, P.; Shuai, S.; Huang, C.; Liu, X.; Fu, Y.; Wang, J.; Ren, Z. Revealing the influence of high magnetic field on the solute distribution during directional solidification of Al-Cu alloy. J. Mater. Sci. Technol. 2021, 88, 226–232. [Google Scholar] [CrossRef]
- He, S.Y.; Li, C.J.; Guo, R.; Xuan, W.D.; Wang, J.; Ren, Z.M. Evolution of microsegregation in directionally solidified Al-Cu alloys under steady magnetic field. J. Alloys Compd. 2019, 800, 41–49. [Google Scholar] [CrossRef]
- Liu, T.; Wang, Q.; Gao, A.; Zhang, C.; Wang, C.; He, J. Fabrication of functionally graded materials by a semi-solid forming process under magnetic field gradients. Scr. Mater. 2007, 57, 992–995. [Google Scholar] [CrossRef]
- Lin, W.; Zhou, B.; Liu, Y.; Guo, X.; Zheng, T.; Zhong, Y.; Zhang, L.; Zhang, Q.; Wang, Q. Dendrite morphology in Al-20wt.%Cu hypoeutectic alloys in 24 T high magnetic field quantified by ex-situ X-ray tomography. J. Alloys Compd. 2022, 918, 165679. [Google Scholar] [CrossRef]
- Li, X.; Fautrelle, Y.; Ren, Z. Morphological instability of cell and dendrite during directional solidification under a high magnetic field. Acta Mater. 2008, 56, 3146–3161. [Google Scholar] [CrossRef]
- He, S.Y.; Zhan, T.J.; Li, C.J.; Xuan, W.D.; Wang, J.; Ren, Z.M. Enhanced Dendrite Coarsening and Microsegregation in Al-Cu Alloy under a Steady Magnetic Field. Mater. Trans. 2019, 60, 1921–1927. [Google Scholar] [CrossRef]
- Lou, C.; Wang, Q.; Wang, C.; Liu, T.; Nakajima, K.; He, J. Migration and rotation of TiAl3 particles in an Al-melt solidified under high magnetic field conditions. J. Alloys Compd. 2009, 472, 225–229. [Google Scholar] [CrossRef]
- Guo, R.; Li, C.; He, S.; Wang, J.; Xuan, W.; Li, X.; Zhong, Y.; Ren, Z. Effect of steady magnetic field on undercooling of Al-Cu alloy melts. Europhys. Lett. 2019, 126, 46001. [Google Scholar] [CrossRef]
- Zheng, T.; Shi, P.; Shen, Z.; Zhou, B.; Lin, W.; Liang, X.; Liu, W.; Chen, X.; Zhong, Y.; Wang, H.; et al. Diffusion-controlled mechanical property-enhancement of Al-20wt.%Si ribbon annealed under high static magnetic fields, from the microscale to the atomic scale. Mater. Des. 2020, 188, 108476. [Google Scholar] [CrossRef]
- Liu, Y.; Lin, W.; Zhou, B.; Guo, X.; Cai, H.; Zheng, T.; Ding, B.; Zhong, Y.; Zhang, L. Ex-situ study of diffusion in liquid Al-Cu melts under a transverse magnetic field using X-ray imaging. Philos. Mag. Lett. 2022, 102, 151–159. [Google Scholar] [CrossRef]
- Lin, W.; Liu, Y.; Zhou, B.; Cai, H.; Shen, Z.; Ren, W.; Lei, Z.; Liu, C.; Zheng, T.; Zhong, Y.; et al. Synthesis of single metallic crystal in microgravity simulated by static magnetic field. Scr. Mater. 2022, 221, 114969. [Google Scholar] [CrossRef]
- Zhou, B.; Lin, W.; Shen, Z.; Zheng, T.; Zhong, Y.; Beaugnon, E.; Debray, F.; Zhang, L.; Wang, H.; Wang, Q. Growth dynamics of the segregated phase in Zn-6wt.%Bi immiscible alloy superheated in super high static magnetic field. J. Alloys Compd. 2021, 879, 160410. [Google Scholar] [CrossRef]
- Zhou, B.; Guo, X.; Lin, W.; Liu, Y.; Guo, Y.; Zheng, T.; Zhong, Y.; Wang, H.; Wang, Q. Effect of Vertical High Magnetic Field on the Morphology of Solid-Liquid Interface during the Directional Solidification of Zn-2wt.%Bi Immiscible Alloy. Metals 2022, 12, 875. [Google Scholar] [CrossRef]
- Zhou, B.; Lin, W.; Guo, Y.; Zheng, T.; Zhong, Y.; Zhang, L.; Zhang, Q. Tailoring homogeneous immiscible alloy via magneto-Archimedes levitation. Scr. Mater. 2023, 226, 115268. [Google Scholar] [CrossRef]
- Guo, Y.; Qi, W.; Xia, Z.; Lin, W.; Liu, C.; Shi, P.; Li, Q.; Shen, Z.; Ding, B.; Zheng, T.; et al. Refinement of Eutectic Carbides in M2 High Speed Steel by Adjusting Magnetic Flux Density During Magnetic Controlled ESR Process. Metall. Mater. Trans. B 2022, 53, 3384–3395. [Google Scholar] [CrossRef]
- Guo, Y.; Qi, W.; Xia, Z.; Zhao, X.; Li, Q.; Liu, C.; Ding, B.; Shen, Z.; Zheng, T.; Zhong, Y. Morphology tailoring of metal pool and eutectic carbides in magnetic-controlled electroslag remelted M2 high-speed steel. J. Mater. Res. Technol. 2022, 16, 1122–1135. [Google Scholar] [CrossRef]
- Qi, W.; Guo, Y.; Ma, C.; Xia, Z.; Li, Q.; Liu, C.; Shi, P.; Ding, B.; Shen, Z.; Zheng, T.; et al. Multi-Stage Enhanced Removal of Inclusions During Electroslag Remelting Process by a Static Magnetic Field. Steel Res. Int. 2022, 93, 2200273. [Google Scholar] [CrossRef]
- Alboussiere, T.; Moreau, R.; Camel, D. Influence of a magnetic-field on the solidification of metallic alloys. C. R. Acad. Sci. 1991, 313, 749–755. Available online: https://gallica.bnf.fr/ark:/12148/bpt6k63186794/f761.item# (accessed on 22 April 2023).
- Sun, Z.; Vleugels, M.G.J.; Van Der Biest, O.; Blanpain, B. Numerical Calculations on Inclusion Removal From Liquid Metals Under Strong Magnetic Fields. Prog. Electromagn. Res. 2009, 98, 359–373. [Google Scholar] [CrossRef]
- Beaugnon, E.; Tournier, R. Levitation of organic materials. Nature 1991, 349, 470. [Google Scholar] [CrossRef]
- Joo, H.D.; Choi, J.K.; Kim, S.U.; Shin, N.S.; Koo, Y.M. An effect of a strong magnetic field on the phase transformation in plain carbon steels. Metall. Mater. Trans. A 2004, 35, 1663–1668. [Google Scholar] [CrossRef]
- Li, Z.; Chen, L.; Meng, S.; Guo, L.; Huang, J.; Liu, Y.; Wang, W.; Chen, X. Field and temperature dependence of intrinsic diamagnetism in graphene: Theory and experiment. Phys. Rev. B 2015, 91, 094429. [Google Scholar] [CrossRef]
- Zheng, T.; Zhou, B.; Wang, J.; Shuai, S.; Zhong, Y.; Ren, W.; Ren, Z.; Debray, F.; Beaugnon, E. Compression properties enhancement of Al-Cu alloy solidified under a 29 T high static magnetic field. Mater. Sci. Eng. A 2018, 733, 170–178. [Google Scholar] [CrossRef]
- Cai, B.; Kao, A.; Boller, E.; Magdysyuk, O.V.; Atwood, R.C.; Vo, N.T.; Pericleous, K.; Lee, P.D. Revealing the mechanisms by which magneto-hydrodynamics disrupts solidification microstructures. Acta Mater. 2020, 196, 200–209. [Google Scholar] [CrossRef]
- Wang, J.; Fautrelle, Y.; Nguyen-Thi, H.; Mangelinck-Noel, N.; Salloum Abou Jaoude, G.; Kaldre, I.; Bojarevics, A.; Buligins, L. Thermoelectric magnetic force acting on the solid during directional solidification under a static magnetic field. Appl. Phys. Lett. 2012, 101, 251904. [Google Scholar] [CrossRef]
- Fautrelle, Y.; Wang, J.; Du, D.; Li, X.; Ren, Z. High Magnetic Field Processing of Metal Alloys. In Solidification Processing of Metallic Alloys Under External Fields; Eskin, D., Mi, J., Eds.; Springer: Cham, Switzerland, 2018; Volume 273. [Google Scholar] [CrossRef]
- Gelfgat, A.Y.; Bar-Yoseph, P.Z.; Solan, A. Effect of axial magnetic field on three-dimensional instability of natural convection in a vertical Bridgman growth configuration. J. Cryst. Growth 2001, 230, 63–72. [Google Scholar] [CrossRef]
- Xu, B.; Li, B.Q.; Stock, D.E. An experimental study of thermally induced convection of molten gallium in magnetic fields. Int. J. Heat Mass Transf. 2006, 49, 2009–2019. [Google Scholar] [CrossRef]
- Chen, L.; Liu, B.Q.; Ni, M.J. Study of natural convection in a heated cavity with magnetic fields normal to the main circulation. Int. J. Heat Mass Transf. 2018, 127, 267–277. [Google Scholar] [CrossRef]
- Mao, S.; Wang, X.; Sun, D.; Wang, J. Numerical modeling of dendrite growth in a steady magnetic field using the two relaxation times lattice Boltzmann-phase field model. Comp. Mater. Sci. 2022, 204, 111149. [Google Scholar] [CrossRef]
- Yu, H.; Tandon, K.N.; Cahoon, J.R. Solidification of Hypereutectic Al-38 Wt Pct Cu Alloy in Microgravity and in Unit Gravity. Metall. Mater. Trans. A 1997, 28, 1245–1250. [Google Scholar] [CrossRef]
- Li, L.; Xu, B.; Tong, W.P.; He, L.Z.; Ban, C.Y.; Zhang, H.; Zuo, Y.B.; Zhu, Q.F.; Cui, J.Z. Directional growth behavior of α(Al) dendrites during concentration-gradient-controlled solidification process in static magnetic field. Trans. Nonferrous Met. Soc. China 2015, 25, 2438–2445. [Google Scholar] [CrossRef]
- Li, L.; Xu, B.; Tong, W.P.; Zhang, H.; Ban, C.Y.; He, L.Z.; Zhao, Z.H.; Zuo, Y.B.; Zhu, Q.F.; Cui, J.Z. Directional Growth of Tin Crystals Controlled by Combined Solute Concentration Gradient Field and Static Magnetic Field. Acta Metall. Sin. 2015, 28, 725–732. [Google Scholar] [CrossRef]
- Asai, S. Rencent development and prospect of electromagnetic processing of materials. Sci. Technol. Adv. Mater. 2000, 1, 191–200. [Google Scholar] [CrossRef]
- Fautrelle, Y.; Wang, J.; Salloum-Abou-Jaoude, G.; Abou-Khalil, L.; Reinhart, G.; Li, X.; Ren, Z.M.; Nguyen-Thi, H. Thermo-Electric-Magnetic Hydrodynamics in Solidification: In Situ Observations and Theory. JOM 2018, 70, 764–771. [Google Scholar] [CrossRef]
- Khine, Y.Y.; Walker, J.S. Thermoelectric magnetohydrodynamic effects during Bridgman semiconductor crystal growth with a uniform axial magnetic field. J. Cryst. Growth 1998, 183, 150–158. [Google Scholar] [CrossRef]
- Li, X.; Fautrelle, Y.; Ren, Z.; Gagnoud, A.; Moreau, R.; Zhang, Y.; Esling, C. Effect of a high magnetic field on the morphological instability and irregularity of the interface of a binary alloy during directional solidification. Acta Mater. 2009, 57, 1689–1701. [Google Scholar] [CrossRef]
- Li, X.; Gagnoud, A.; Ren, Z.; Fautrelle, Y.; Moreau, R. Investigation of thermoelectric magnetic convection and its effect on solidification structure during directional solidification under a low axial magnetic field. Acta Mater. 2009, 57, 2180–2197. [Google Scholar] [CrossRef]
- Li, X.; Zhang, Y.; Fautrelle, Y.; Ren, Z.; Esling, C. Experimental evidence for liquid-solid interface instability caused by the stress in the solid during directional solidification under a strong magnetic field. Acta Mater. 2009, 60, 489–492. [Google Scholar] [CrossRef]
- Inoue, K.; Yasuda, H.; Minami, Y.; Nagira, T.; Yoshiya, M.; Uesugi, K.; Umetani, K. Direct observation of thermoelectric magnetic convection in unidirectionally solidified Al-Cu alloys. J. Iron Steel Res. Int. 2012, 198, 34–39. [Google Scholar] [CrossRef]
- Wang, J.; Fautrelle, Y.; Nguyen-Thi, H.; Salloum Abou Jaoude, G.; Reinhart, G.; Mangelinck-Noël, N.; Kaldre, I. Thermoelectric magnetic flows in melt during directional solidification. Appl. Phys. Lett. 2014, 104, 121916. [Google Scholar] [CrossRef]
- Shen, Z.; Zhou, B.; Zhong, Y.; Dong, L.; Wang, H.; Fan, L.; Zheng, T.; Li, C.; Ren, W.; Xuan, W.; et al. An Electromagnetic Compounding Technique for Counteracting the Thermoelectric Magnetic Effect During Directional Solidification Under a Transverse Static Magnetic Field. Metall. Mater. Trans. A 2018, 49, 3373–3382. [Google Scholar] [CrossRef]
- Wang, J.; Yue, S.; Fautrelle, Y.; Lee, P.D.; Li, X.; Zhong, Y.; Ren, Z. Refinement and growth enhancement of Al2Cu phase during magnetic field assisting directional solidification of hypereutectic Al-Cu alloy. Sci. Rep. 2016, 6, 24585. [Google Scholar] [CrossRef]
- Liu, T.; Miao, L.; Wang, K.; Wang, L.; Sun, J.; Wang, Q. High magnetic-field-induced solute interception among dendrite arms in the mushy zone of a Mn-Sb alloy. J. Appl. Phys. 2018, 124, 045901. [Google Scholar] [CrossRef]
- Zhu, L.; Han, C.; Hou, L.; Gagnoud, A.; Fautrelle, Y.; Ren, Z.; Li, X. Influence of a static magnetic field on the distribution of solute Cu and interdendritic constitutional undercooling in directionally solidified Al-4.5wt.%Cu alloy. Mater. Lett. 2019, 248, 73–77. [Google Scholar] [CrossRef]
- Li, X.; Gagnoud, A.; Fautrelle, Y.; Ren, Z.; Moreau, R.; Zhang, Y.; Esling, C. Dendrite fragmentation and columnar-to-equiaxed transition during directional solidification at lower growth speed under a strong magnetic field. Acta Mater. 2012, 60, 3321–3332. [Google Scholar] [CrossRef]
- Li, X.; Fautrelle, Y.; Ren, Z.; Zhang, Y.; Esling, C. Effect of a high magnetic field on the Al-Al3Ni fiber eutectic during directional solidification. Acta Mater. 2010, 58, 2430–2441. [Google Scholar] [CrossRef]
- Li, C.; Ren, Z.; Shen, Y.; Wang, Q.; Dai, Y.; Wang, H. Faceted growth of primary Al2Cu crystals during directional solidification in high magnetic field. J. Appl. Phys. 2013, 114, 154903. [Google Scholar] [CrossRef]
- He, S.; Shevchenko, N.; Eckert, S. In situ observation of directional solidification in Ga-In alloy under a transverse DC magnetic field. IOP Conf. Ser. Mater. Sci. Eng. 2020, 861, 012025. [Google Scholar] [CrossRef]
- Koe, B.; Abraham, C.; Bailey, C.; Greening, B.; Small, M.; Connolley, T.; Mi, J. A novel electromagnetic apparatus for in-situ synchrotron X-ray imaging study of the separation of phases in metal solidification. HardwareX 2020, 7, e00104. [Google Scholar] [CrossRef] [PubMed]
- Song, Z.; Magdysyuk, O.V.; Sparks, T.; Chiu, Y.-L.; Cai, B. Revealing growth mechanisms of faceted Al2Cu intermetallic compounds via high-speed Synchrotron X-ray tomography. Acta Mater. 2022, 231, 117903. [Google Scholar] [CrossRef]
- Kakeshita, T.; Saburi, T.; Shimizu, K. Effects of hydrostatic pressure and magnetic field on martensitic transformations. Mater. Sci. Eng. A 1999, 273–275, 21–39. [Google Scholar] [CrossRef]
- Magomedov, M.N. On the magnetic-field-induced changes in the parameters of phase transitions. Tech. Phys. Lett. 2002, 28, 73–79. [Google Scholar] [CrossRef]
- Aoki, Y.; Hayashi, S.; Komatsu, H. Liquidus- and eutectic-temperature measurements of Al-rich alloys containing Cu and Si in a magnetic field of 3.5 T. J. Cryst. Growth 1992, 123, 313–316. [Google Scholar] [CrossRef]
- Long, Z.; Wang, J.; Fautrelle, Y.; Li, X. Repeated nucleation behaviors of pure bismuth under a high magnetic field. J. Cryst. Growth 2020, 831, 154746. [Google Scholar] [CrossRef]
- Li, C.; Yang, H.; Ren, Z.; Ren, W.; Wu, Y. Application of differential thermal analysis to investigation of magnetic field effect on solidification of Al-Cu hypereutectic alloy. J. Alloys Compd. 2010, 505, 108–112. [Google Scholar] [CrossRef]
- Li, C.; Guo, R.; Yuan, Z.; Xuan, W.; Ren, Z.; Zhong, Y.; Li, X.; Wang, H.; Wang, Q. Magnetic-field dependence of nucleation undercoolings in non-magnetic metallic melts. Philos. Mag. Lett. 2015, 95, 37–43. [Google Scholar] [CrossRef]
- Guo, R.; Shuai, S.; Zhao, R.; Li, C.; Li, X.; Zhong, Y.; Wang, J.; Ren, Z. Enhanced Degradation in Grain Refinement of Inoculated 2024 Al Alloy in Steady Magnetic field. Metall. Mater. Trans. A 2020, 51, 4584–4591. [Google Scholar] [CrossRef]
- Huang, C.; Shuai, S.; Li, C.; Wang, J.; Wang, J.; Shi, L.; Li, S.; Nan, R.; Ren, Z. Magnetic field-induced variation of solid/liquid interfacial energy of solid Al2Cu and Al-Cu eutectic melt. J. Alloys Compd. 2023, 941, 168977. [Google Scholar] [CrossRef]
- Wang, L.; Long, Z.; Hou, L.; Yan, S.; Sun, B.; Li, X. Effects of a high magnetic field on single-phase interface evolution, additional interfacial energy and nucleation undercooling in Al-based alloy. J. Mater. Sci. Technol. 2023, 154, 43–53. [Google Scholar] [CrossRef]
- Liu, C.; Zhong, Y.; Shen, Z.; Zheng, T.; Dong, L.; Ren, W.; Lei, Z.; Ren, Z. Effect of an axial high static magnetic field on the crystal orientation and magnetic property of Fe-4.5wt.%Si alloy during bulk solidification. Mater. Lett. 2019, 247, 189–192. [Google Scholar] [CrossRef]
- Morikawa, H.; Sassa, K.; Asai, S. Control of precipitating phase alignment and crystal orientation by imposition of a high magnetic field. Mater. Trans. JIM 1998, 39, 814–818. [Google Scholar] [CrossRef]
- Dong, S.; Liu, T.; Dong, M.; Wang, S.; Wang, W.; Wang, K.; Wang, Q. Crystallographic orientation of primary and eutectic phases in a hypoeutectic Mn-Sb alloy induced by solidification in high magnetic fields. J. Appl. Crystallogr. 2019, 52, 945–950. [Google Scholar] [CrossRef]
- Li, X.; Fautrelle, Y.; Moreau, R.; Ren, Z. EBSD study of the morphology and orientation of the primary and eutectic phases in Al-Cu alloys during solidification under a strong magnetic field. J. Appl. Crystallogr. 2016, 49, 139–148. [Google Scholar] [CrossRef]
- Han, Y.; Ban, C.; Guo, S.; Liu, X.; Ba, Q.; Cui, J. Alignment behavior of primary Al3Fe phase in Al-Fe alloy under a high magnetic field. Mater. Lett. 2007, 61, 983–986. [Google Scholar] [CrossRef]
- Wang, C.; Wang, Q.; Wang, Z.; Li, H.; Nakajima, K.; He, J. Phase alignment and crystal orientation of Al3Ni in Al-Ni alloy by imposition of a uniform high magnetic field. J. Cryst. Growth 2008, 310, 1256–1263. [Google Scholar] [CrossRef]
- Luo, D.; Guo, J.; Yan, Z.; Li, T. Effect of high magnetic fields on the solidification microstructure of an Al-Mn alloy. Rare Met. 2009, 28, 302–308. [Google Scholar] [CrossRef]
- Mikelson, A.E.; Karklin, Y.K. Control of crystallization processes by means of magnetic fields. J. Cryst. Growth 1981, 52, 524–529. [Google Scholar] [CrossRef]
- Sugiyama, T.; Tahashi, M.; Sassa, K.; Asai, S. The Control of Crystal Orientation in Non-magnetic Metals by Imposition of a High Magnetic Field. ISIJ Int. 2003, 43, 855–861. [Google Scholar] [CrossRef]
- Lu, Y.; Dong, Y.; Guo, S.; Jiang, L.; Kang, H.; Wang, T.; Wen, B.; Wang, Z.; Jie, J.; Cao, Z.; et al. A Promising New Class of High-Temperature Alloys: Eutectic High-Entropy Alloys. Sci. Rep. 2014, 4, 6200. [Google Scholar] [CrossRef] [PubMed]
- Shi, P.; Li, R.; Li, Y.; Wen, Y.; Zhong, Y.; Ren, W.; Shen, Z.; Zheng, T.; Peng, J.; Liang, X.; et al. Hierarchical crack buffering triples ductility in eutectic herringbone high-entropy alloys. Science 2021, 373, 912–918. [Google Scholar] [CrossRef] [PubMed]
- Shi, P.; Ren, W.; Zheng, T.; Ren, Z.; Hou, X.; Peng, J.; Hu, P.; Gao, Y.; Zhong, Y.; Liaw, P.K. Enhanced strength-ductility synergy in ultrafine-grained eutectic high-entropy alloys by inheriting microstructural lamellae. Nat. Commun. 2019, 10, 489. [Google Scholar] [CrossRef]
- Wang, J.; Long, Z.; Jiang, P.; Fautrelle, Y.; Li, X. Microstructure, Crystallographic Orientation and Mechanical Property in AlCoCrFeNi2.1 Eutectic High-Entropy Alloy Under Magnetic Field-Assisted Directional Solidification. Metall. Mater. Trans. A 2020, 51, 3504–3517. [Google Scholar] [CrossRef]
- Deng, N.; Wang, J.; Wang, J.; He, Y.; Lan, Z.; Zhao, R.; Beaugon, E.; Li, J. Microstructure and properties of AlCoCrCuFeNi high-entropy alloy solidified under high magnetic field. Mater. Lett. 2021, 285, 129182. [Google Scholar] [CrossRef]
- Frank-Rotsch, C.; Dropka, N.; Kießling, F.M.; Rudolph, P. Semiconductor Crystal Growth under the Influence of Magnetic Fields. Cryst. Res. Technol. 2019, 55, 1900115. [Google Scholar] [CrossRef]
- Li, Q.; Xia, Z.; Guo, Y.; Shen, Z.; Zheng, T.; Ding, B.; Zhong, Y. Carbides Modification and Mechanical Properties Enhancement of Cr12MoV Die Steel by Magnetically Controlled Electroslag Remelting. Metall. Mater. Trans. B 2021, 52, 1495–1507. [Google Scholar] [CrossRef]


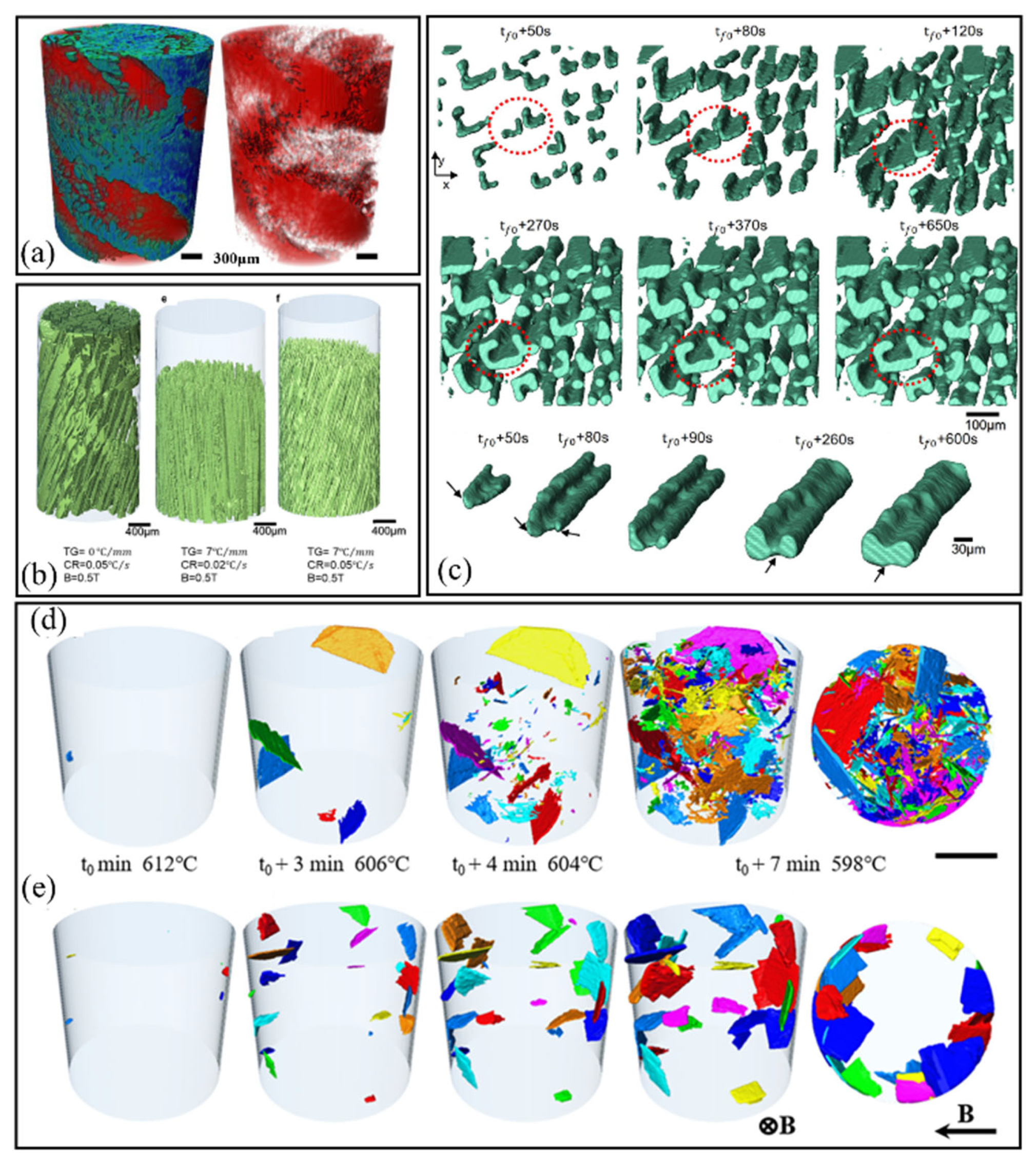

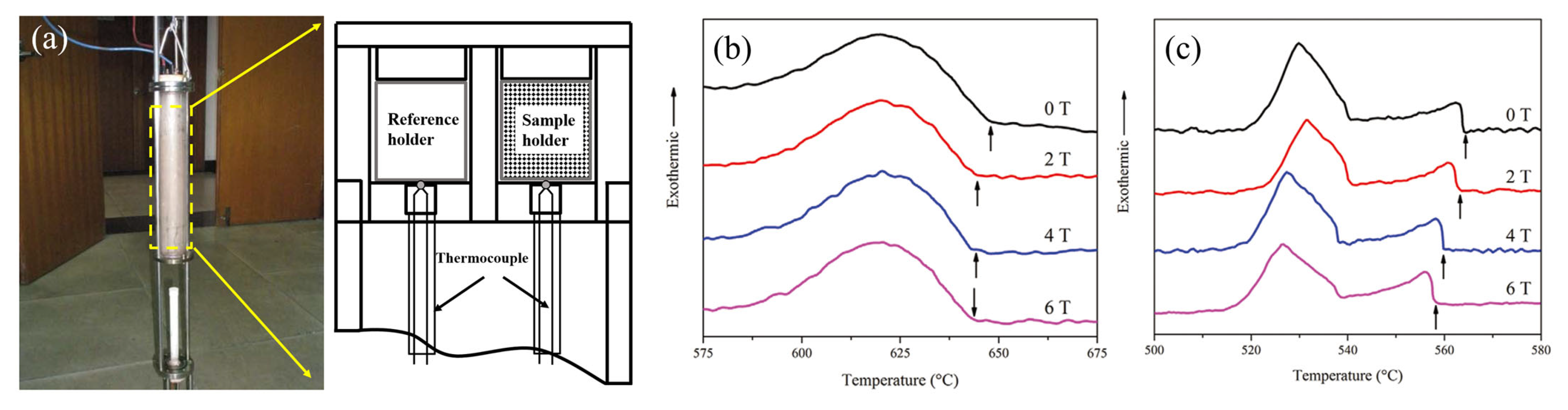

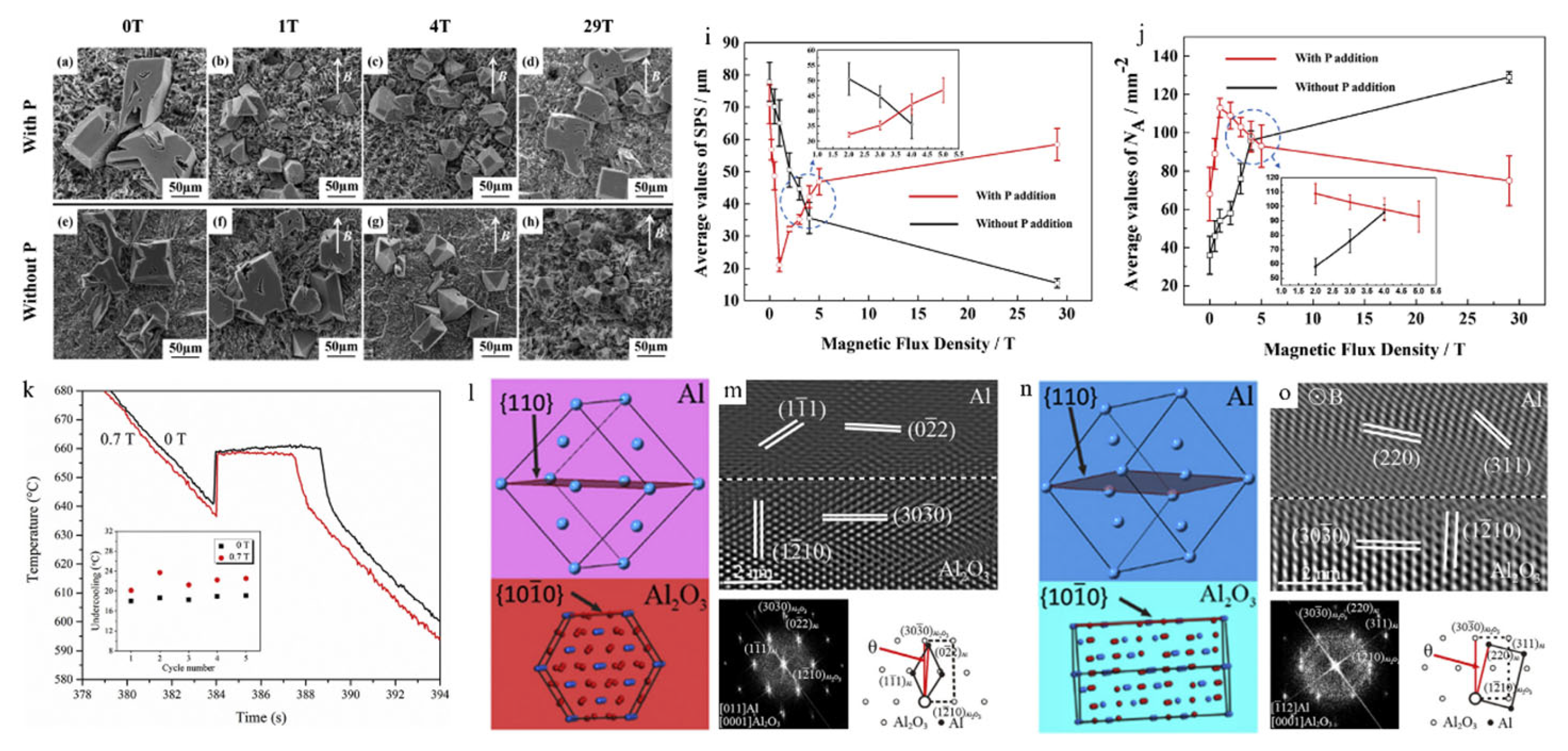
| ETAs | B (T) | Research Content | References |
|---|---|---|---|
| Al-Cu | 0–10 | Eutectic spacing; microstructure evolution, orientation of Al2Cu; interface shape | [14,15,16,17] |
| Nb-Fe | 1–5 | Phase separation | [18] |
| Cu-Ag | 0–200 mT, 0–31.2 | TEM convection in the melt; microstructure, electrical resistivity, magnetoresistance | [19,20] |
| Al-Si | 0–12 | Distribution of primary silicon under uniform and gradient magnetic field; arm spacing, eutectic lamellar spacing; refinement of primary silicon | [21,22,23] |
| Al-Mg | 0, 8.8 | Solute segregation | [24] |
| Al-Fe | 0–12 | Distribution of primary phase | [25,26,27,28] |
| Al-Ni | 0–2 | Microstructural evolution | [29] |
| Al-Zn | 0, 5 | Dendrite morphology and growth orientation | [30] |
| Sn-Bi | 0–0.55 | TEM convection in the melt; microstructural evolution and solute distribution; refinement of arm spacing | [31,32,33] |
| Pb-Sn | 0–12 | Convection and macrosegregation; TEM convection and microstructural evolution; dendrite morphology | [34,35,36] |
| Zn-Mg | 0–12 | Orientation of primary Zn-rich crystals; the morphology, size, and distribution of the primary MgZn2 crystals | [37,38] |
| Mg-Nd | 0, 1 | Phase formation | [39] |
| Co-B | 0–4T | Morphology and magnetic alignment of the primary α-Co phase | [40,41] |
| Co-Sn | 0–12 | Nucleation behavior | [42] |
| Mn-Sb | 0–11.5 | Orientation of both the primary and eutectic MnSb crystals | [43] |
| Bi-Mn | 0–10 | Growth of eutectic Bi/MnBi | [44] |
| Al-Si-Fe | 0, 0.07 | Precipitation behavior of iron intermetallic compounds | [45] |
| Al-Cu-Ag | 0–6 | Microstructural evolution | [46] |
| Al-Cu-Si | 0–0.5 | Evolution of microstructure and crystallization | [47] |
| NiAl-Cr(Mo)-Hf | 0–6 | Microstructural evolution | [48] |
| Materials | Crystals | B (T) | Orientation | References |
|---|---|---|---|---|
| Al-Cu | Al2Cu | 1–29 | c axis//B | [80,121] |
| Al-Fe | Al3Fe | 5 | <542>//B | [122] |
| Al-Ni | Al3Ni | 10 | c axis//B | [123] |
| Al-Mn | Al6Mn | 10 | c axis//B | [124] |
| Mg-Zn/Cd-Zn | primary Zn | 12 | c axis//B | [38,125] |
| Mn-Sb | MnSb | 3–11.5 | c axisB | [43,120] |
| Bi-Mn | MnBi | 2 | c axis//B | [44] |
| Al-Si-Fe | Al9Si15Fe | 5 | Long axis of crystal⊥B | [119] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, H.; Lin, W.; Feng, M.; Zheng, T.; Zhou, B.; Zhong, Y. Review on Eutectic-Type Alloys Solidified under Static Magnetic Field. Crystals 2023, 13, 891. https://doi.org/10.3390/cryst13060891
Cai H, Lin W, Feng M, Zheng T, Zhou B, Zhong Y. Review on Eutectic-Type Alloys Solidified under Static Magnetic Field. Crystals. 2023; 13(6):891. https://doi.org/10.3390/cryst13060891
Chicago/Turabian StyleCai, Hao, Wenhao Lin, Meilong Feng, Tianxiang Zheng, Bangfei Zhou, and Yunbo Zhong. 2023. "Review on Eutectic-Type Alloys Solidified under Static Magnetic Field" Crystals 13, no. 6: 891. https://doi.org/10.3390/cryst13060891
APA StyleCai, H., Lin, W., Feng, M., Zheng, T., Zhou, B., & Zhong, Y. (2023). Review on Eutectic-Type Alloys Solidified under Static Magnetic Field. Crystals, 13(6), 891. https://doi.org/10.3390/cryst13060891







