Reactive Spark Plasma Sintering and Thermoelectric Properties of Zintl Semiconducting Ca14Si19 Compound
Abstract
:1. Introduction
2. Material and Methods
2.1. Synthesis of CaSi
2.2. Sample Characterization
3. Results and Discussion
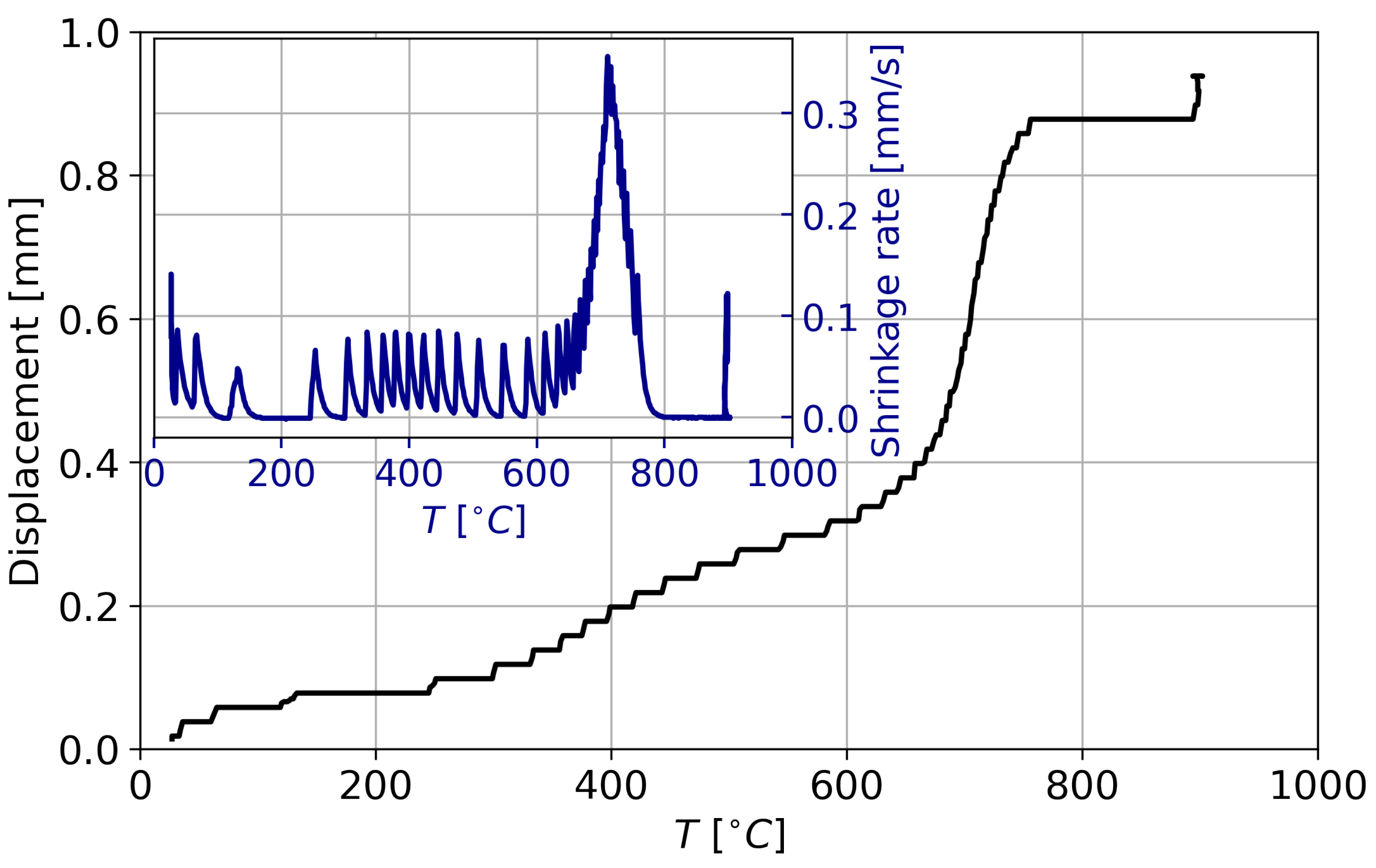
3.1. Sintering Process and Phase Stability of CaSi
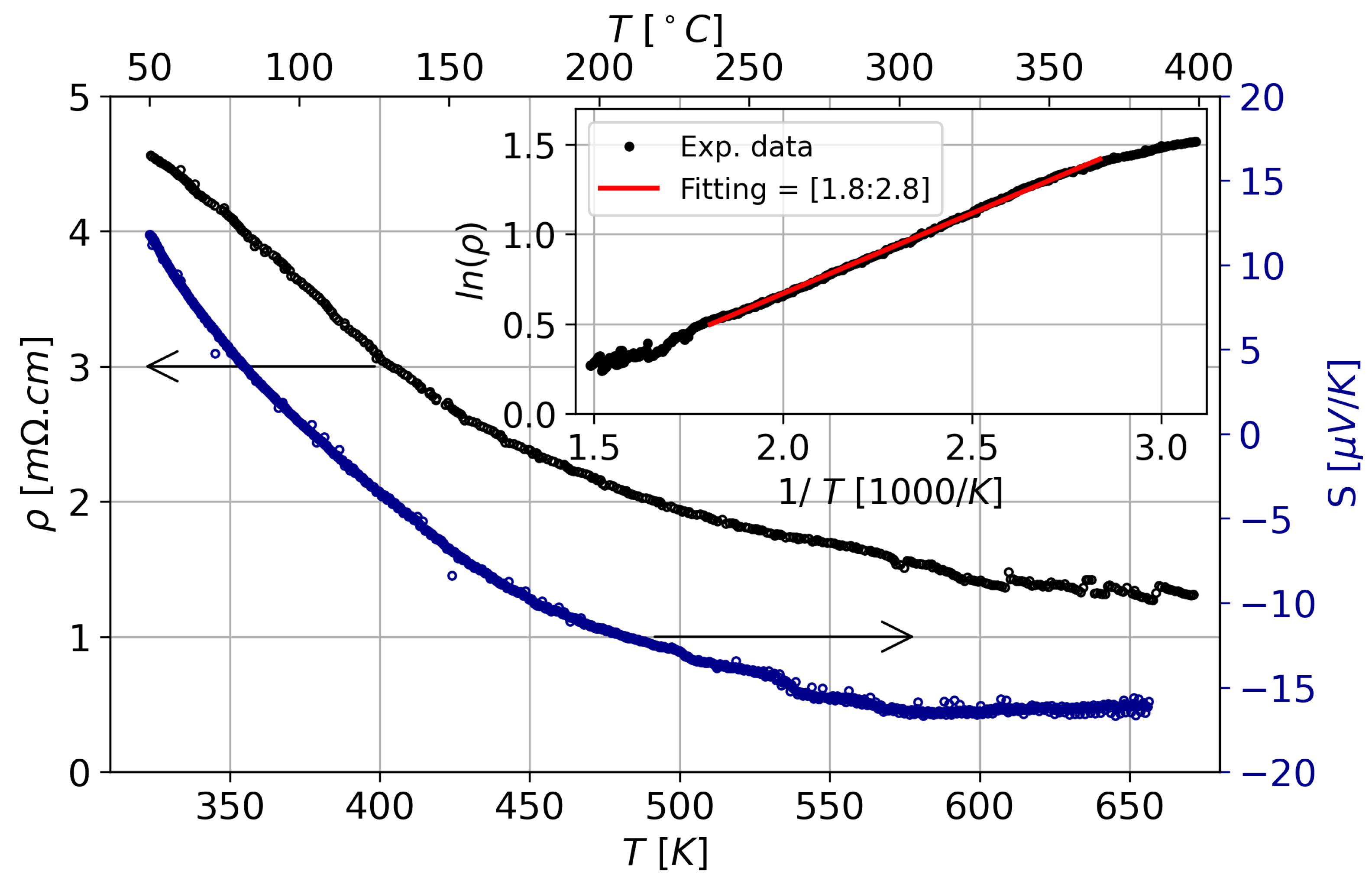
3.2. Thermoelectric and Mechanical Properties
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Buchal, C. The challenge of discussing energy facts and climate change. EPJ Web Conf. 2020, 246, 00003. [Google Scholar] [CrossRef]
- Kalghatgi, G. Is it really the end of internal combustion engines and petroleum in transport? Appl. Energy 2018, 225, 965–974. [Google Scholar] [CrossRef]
- Seebeck, T.J. Magnetische Polarisation der Metalle und Erze durch Temperatur-Differenz; Abhandlungen Der Deutschen Akademie Der Wissenschaften Zu Berlin, 1822–1823; W. Engelmann: Leipzig, Germany, 1895; pp. 265–373. [Google Scholar]
- Peltier, J. Nouvelles expériences sur la caloricité des courants électriques. Annal. Chim. Phys. 1834, 56, 371–386. [Google Scholar]
- Ong, K.; Jiang, L.; Lai, K. Thermoelectric Energy Conversion; Comprehensive Energy System; Elsevier: Amsterdam, The Netherlands, 2018. [Google Scholar] [CrossRef]
- Beretta, D.; Neophytou, N.; Hodges, J.; Kanatzidis, M.; Narducci, D.; Martin-Gonzalez, M.; Beekman, M.; Balke, B.; Cerretti, G.; Tremel, W.; et al. Thermoelectrics: From history, a window to the future. Mater. Sci. Eng. R Rep. 2019, 138, 210–255. [Google Scholar]
- Vining, C. An inconvenient truth about thermoelectrics. Nat. Mater. 2009, 8, 83–85. [Google Scholar] [CrossRef]
- Ioffe, A. Semiconductor Thermoelements and Thermoelectric Cooling; Infosearch Ltd.: London, UK, 1957. [Google Scholar]
- Terasaki, I. Introduction to thermoelectricity. In Materials For Energy Conversion Devices; Woodhead Publishing: Cambridge, UK, 2005; pp. 339–357. [Google Scholar]
- Uher, C. Skutterudites: Prospective novel thermoelectrics. Semiconduct. Semimet. 2001, 69, 139–253. [Google Scholar]
- Slack, G.A. New Materials and Performance Limits for Thermoelectric Cooling. In CRC Handbook of Thermoelectrics; CRC: Boca Raton, FL, USA, 1995; Chapter 34; pp. 407–440. [Google Scholar]
- Takabatake, T.; Suekuni, K.; Nakayama, T.; Kaneshita, E. Phonon-glass electron-crystal thermoelectric clathrates: Experiments and theory. RMP 2014, 86, 669. [Google Scholar] [CrossRef]
- Snyder, G.; Toberer, E. Complex thermoelectric materials. Nat. Mater. 2008, 7, 105–114. [Google Scholar] [CrossRef]
- Toberer, E.; May, A.; Snyder, G. Zintl chemistry for designing high-efficiency thermoelectric materials. Chem. Mater. 2010, 22, 624–634. [Google Scholar] [CrossRef]
- Fleischer, M. The abundance and distribution of the chemical elements in the earth’s crust. J. Chem. Educ. 1954, 31, 446. [Google Scholar] [CrossRef]
- Goldsmid, H.; Douglas, R. The use of semiconductors in thermoelectric refrigeration. Br. J. Appl. Phys. 1954, 5, 386. [Google Scholar] [CrossRef]
- Bulusu, A.; Walker, D. Review of electronic transport models for thermoelectric materials. Superlattices Microstruct. 2008, 44, 1–36. [Google Scholar] [CrossRef]
- Tervo, J.; Manninen, A.; Ilola, R.; Hänninen, H. State-of-the-Art of Thermoelectric Materials Processing; VTT Working Papers: 124; VTT Technical Research Center of Finland: Espoo, Finland, 2009; ISBN 978-951-38-7184-0. [Google Scholar]
- Witting, I.; Chasapis, T.; Ricci, F.; Peters, M.; Heinz, N.; Hautier, G.; Snyder, G. The thermoelectric properties of bismuth telluride. Adv. Electron. Mater. 2019, 5, 1800904. [Google Scholar] [CrossRef]
- Kuhlmann, A. The second most abundant element in the earth’s crust. JOM 1963, 15, 502–505. [Google Scholar] [CrossRef]
- Currao, A.; Wengert, S.; Nesper, R.; Curda, J.; Hillebrecht, H. Ca14Si19—A Zintl Phase with a Novel Twodimensional Silicon Framework. Z. Anorg. Allg. Chem. 1996, 622, 501–508. [Google Scholar] [CrossRef]
- Currao, A. Synthese, Struktur und Eigenschaften von Zintlphasen der Erdalkali-und Alkali-Erdalkalimetalle mit Silicium. 1996. Available online: https://www.research-collection.ethz.ch/bitstream/handle/20.500.11850/142715/1/eth-40372-01.pdf (accessed on 1 December 2022).
- Manfrinetti, P.; Fornasini, M.; Palenzona, A. The phase diagram of the Ca–Si system. Intermetallics 2000, 8, 223–228. [Google Scholar] [CrossRef]
- Canepa, F.; Napoletano, M.; Manfrinetti, P.; Palenzona, A. Heat capacity and thermodynamic properties of some Ca silicides. J. Alloys Compd. 2000, 299, 20–23. [Google Scholar] [CrossRef]
- Brutti, S.; Ciccioli, A.; Balducci, G.; Gigli, G.; Manfrinetti, P.; Napoletano, M. Thermodynamic stabilities of intermediate phases in the Ca–Si system. J. Alloys Compd. 2001, 317, 525–531. [Google Scholar] [CrossRef]
- Gröbner, J.; Chumak, I.; Schmid-Fetzer, R. Experimental study of ternary Ca–Mg–Si phase equilibria and thermodynamic assessment of Ca–Si and Ca–Mg–Si systems. Intermetallics 2003, 11, 1065–1074. [Google Scholar] [CrossRef]
- Wen, C.; Kato, A.; Nonomura, T.; Tatsuoka, H. Phase selection during calcium silicide formation for layered and powder growth. J. Alloys Compd. 2011, 509, 4583–4587. [Google Scholar] [CrossRef]
- Tao, X.; Yang, J.; Xi, L.; Ouyang, Y. First-principles investigation of the thermo-physical properties of Ca3Si4. J. Solid State Chem. 2012, 194, 179–187. [Google Scholar] [CrossRef]
- Yaokawa, R.; Nakano, H.; Ohashi, M. Growth of CaSi2 single-phase polycrystalline ingots using the phase relationship between CaSi2 and associated phases. Acta Mater. 2014, 81, 41–49. [Google Scholar] [CrossRef] [Green Version]
- Eckerlin, P.; Meyer, H.; Wölfel, E. Die Kristallstruktur von CaSn und CaGe. Z. Anorg. Allg. Chem. 1955, 281, 322–328. [Google Scholar] [CrossRef]
- Nedumk, athil, R.; Benson, D.E.; Grins, J.; Spektor, K.; Haussermann, U. The 3R polymorph of CaSi2. J. Solid State Chem. 2015, 222, 18–24. [Google Scholar]
- Galkin, N.G.; Galkin, K.G.; Goroshko, D.L.; Dotsenko, S.A.; Kropachev, O.V.; Chernev, I.M.; Subbotin, E.Y.; Migas, D.B.; Fogarassy, Z.; Pecz, B. Ca silicide films—Promising materials for silicon optoelectronics. Jpn. J. Appl. Phys. 2023, 62, SD0803. [Google Scholar] [CrossRef]
- Bordet, P.; Affronte, M.; Sanfilippo, S.; Nunez-Regueiro, M.; Laborde, O.; Olcese, G.L.; Palenzona, A.; Le Floch, S.; Levy, D.; Hanfl, M. Structural phase transitions in CaSi2 under high pressure. Phys. Rev. B. 2000, 62, 11392–11397. [Google Scholar] [CrossRef]
- Imai, M.; Kikegawa, T. Phase Transitions of Alkaline-Earth-Metal Disilicides MAESi2 (MAE) Ca, Sr, and Ba) at High Pressures and High Temperatures. Chem. Mater. 2003, 15, 2543–2551. [Google Scholar] [CrossRef]
- Evers, J.; Oehlinger, G.; Weiss, A. Effect of pressure on the Structures of Divalent Metal Disilicides MSi2, (M = Ca, Eu, Sr). J. Solid State Chem. 1977, 20, 173–181. [Google Scholar] [CrossRef]
- Affronte, M.; Laborde, O.; Olcese, G.L.; Palenzona, A. Low temperature properties of calcium mono- and disilicides. J. Alloys Compd. 1998, 274, 68–73. [Google Scholar] [CrossRef]
- Schwarz, U.; Wosylus, A.; Rosner, H.; Schnelle, W.; Ormeci, A.; Meier, K.; Baranov, A.; Nickals, M.; Leipe, S.; Muller, C.J.; et al. Dumbbells of Five-Connected Silicon Atoms and Superconductivity in the Binary Silicides MSi3 (M = Ca, Y, Lu). J. Am. Chem. Soc. 2012, 134, 13558–13561. [Google Scholar] [CrossRef] [PubMed]
- Wosylus, A.; Prots, Y.; Burkhardt, U.; Schnelle, W.; Schwarz, U. High-pressure synthesis of the electron-excess compound CaSi6. Sci. Technol. Adv. Mater. 2007, 8, 383–388. [Google Scholar] [CrossRef]
- Moll, A.; Viennois, R.; Hermet, P.; Haidoux, A.; Bantignies, J.; Beaudhuin, M. Stability and properties of the friendly environmental Zintl phases: Ca3Si4 and Ca14Si19. Acta Mater. 2017, 125, 490–497. [Google Scholar] [CrossRef]
- Lebegue, S.; Arnaud, B.; Alouani, M. Calculated quasiparticle and optical properties of orthorhombic and cubic Ca2Si. Phys. Rev. B 2005, 72, 085103. [Google Scholar] [CrossRef]
- Yang, Z.; Shi, D.; Wen, B.; Melnick, R.; Yao, S.; Li, T. First-principle studies of Ca–X (X=Si,Ge,Sn,Pb) intermetallic compounds. J. Solid State Chem. 2010, 183, 136–143. [Google Scholar] [CrossRef]
- Migas, D.; Shaposhnikov, V.; Filonov, A.; Dorozhkin, N.; Borisenko, V. New semiconducting silicide Ca3Si4. J. Phys. Condens. Matter 2007, 19, 346207. [Google Scholar] [CrossRef]
- Wen, C.; Nonomura, T.; Warashina, Y.; Kubota, Y.; Nakamura, T.; Hayakawa, Y.; Tanaka, M.; Isobe, K.; Tatsuoka, H. Synthesis of single-phase polycrystalline Ca2Si powder and sintered compacts. Int. J. Mater. Res. 2011, 102, 401–405. [Google Scholar] [CrossRef]
- Wen, C.; Nonomura, T.; Isobe, K.; Kubota, Y.; Nakamura, T.; Hayakawa, Y.; Kato, A.; Tatsuoka, H. Effect of Na Addition on Electric Properties of Ca2Si Sintered Compacts. IOP Conf. Ser. Mater. Sci. Eng. 2011, 18, 142014. [Google Scholar] [CrossRef]
- Jun, C.; Yinye, Y.; Yi, L.; Quan, X.; Jinmin, Z. Selective Growth of Ca2Si Film or Ca5Si3 Film in Ca-Si System by R.F MS by Annealing. In Proceedings of the 2012 International Conference on Manipulation, Manufacturing and Measurement on the Nanoscale (3M-NANO), Xi’an, China, 29 August–1 September 2012; pp. 75–79. [Google Scholar]
- Inaba, T.; Kato, A.; Miura, K.; Akasaka, M.; Iida, T.; Momose, Y.; Tatssuoka, H. Preparation and electrical properties of Ca5Si3 and Sr5Si3 powders. Thin Solid Films 2007, 515, 8226–8229. [Google Scholar] [CrossRef]
- Uhera, M.; Akiyama, K.; Shimizu, T.; Matsushima, M.; Uchida, H.; Kimura, Y.; Funakubo, H. Preparation of Ca-Si Films on (001) Al2O3 Substrates by an RF Magnetron Sputtering Method and Their Electrical Properties. J. Electron. Mater. 2016, 45, 3121–3126. [Google Scholar] [CrossRef]
- Imamura, Y.; Muta, H.; Kurosaki, K.; Yamanaka, S. Thermoelectric properties of calcium silicides. In Proceedings of the 2006 25th International on Thermoelectrics, Vienna, Austria, 6–10 August 2006; pp. 535–538. [Google Scholar]
- Snyder, G.J. Zintl Phases: Recent Developments in Thermoelectrics and Future Outlook. Thermoelectr. Mater. Devices 2016, 1. [Google Scholar] [CrossRef]
- Dudina, D.V.; Mukherjee, A.K. Reactive spark plasma sintering: Successes and challenges of nanomaterial synthesis. J. Nanomat. 2013, 2013, 625218. [Google Scholar] [CrossRef]
- Mukasyan, A.S.; Rogachev, A.S.; Moskovskikh, D.O.; Yermekova, Z.S. Reactive spark plasma sintering of exothermic systems: A critical review. Ceram. Int. 2022, 48, 2988–2998. [Google Scholar] [CrossRef]
- Munir, Z.; Anselmi-Tamburini, U.; Ohyanagi, M. The effect of electric field and pressure on the synthesis and consolidation of materials: A review of the spark plasma sintering method. J. Mater. Sci. 2006, 41, 763–777. [Google Scholar] [CrossRef]
- Hu, Z.-Y.; Zhang, Z.-H.; Cheng, X.-W.; Wang, F.-C.; Zhang, Y.-F.; Li, S.-L. A review of multi-physical fields induced phenomena and effects in spark plasma sintering: Fundamentals and applications. Mater. Des. 2020, 191, 108662. [Google Scholar]
- Manière, C.; Nigito, E.; Durand, L.; Weibel, A.; Beynet, Y.; Estournes, C. Spark plasma sintering and complex shapes: The deformed interfaces approach. Powder Technol. 2017, 320, 340–345. [Google Scholar] [CrossRef]
- Elissalde, C.; Chung, U.-C.; Josse, M.; Goglio, G.; Suchomel, M.R.; Majimel, J.; Weibel, A.; Soubie, F.; Flaureau, A.; Fregeac, A.; et al. Single-step sintering of zirconia ceramics using hydroxide precursors and Spark Plasma Sintering below 400 °C. Scr. Mater. 2019, 168, 134–138. [Google Scholar] [CrossRef]
- de Beauvoir, T.H.; Ghomari, Z.; Chevallier, G.; Flaureau, A.; Weibel, A.; Elissalde, C.; Mauvy, F.; Chaim, R.; Estournès, C. Flash Spark Plasma Sintering of 3YSZ: Modified sintering pathway and impact on grain boundary formation. J. Eur. Cer. Soc. 2021, 41, 7762–7770. [Google Scholar] [CrossRef]
- Rahaman, M. Sintering of Ceramics; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Abbassi, L.; Mesguich, D.; Coulomb, L.; Chevallier, G.; Aries, R.; Estournès, C.; Flahaut, E.; Viennois, R.; Beaudhuin, M. In-situ reactive synthesis of dense nanostructured β-FeSi2 by Spark Plasma Sintering. J. Alloys Compd. 2022, 902, 163683. [Google Scholar] [CrossRef]
- Lyu, J.; Kashkarov, E.B.; Travitzky, N.; Syrtanov, M.S.; Lider, A.M. Sintering of MAX-phase materials by spark plasma and other methods. J. Mater. Sci. 2021, 56, 1980–2015. [Google Scholar] [CrossRef]
- Gild, J.; Kaufmann, K.; Vecchio, K.; Luo, J. Reactive flash spark plasma sintering of high-entropy ultrahigh temperature ceramics. Scr. Mater. 2019, 170, 106–110. [Google Scholar] [CrossRef]
- Rodriguez-Carvajal, J.; Roisnel, T. FullProf-98 and WinPLOTR: New Windows 95/NT Applications for Diffraction Commission for Powder Diffraction; International Union of Crystallography: Chester, UK, 1998. [Google Scholar]
- Pauw, L. A method of measuring the resistivity and Hall coefficient on lamellae of arbitrary shape. Philips Tech. Rev. 1958, 20, 220–224. [Google Scholar]
- Blundell, S.; Blundell, K. Concepts in Thermal Physics; Oxford University Press: Oxford, UK, 2010. [Google Scholar]
- Ali, D.O.A.; Fabbiani, M.; Koza, M.M.; Beaudhuin, M.; Viennois, R. Inelastic neutron scattering study of the lattice dynamics of the green semiconducting calcium silicides for thermoelectric applications. 2022; Unpublished Work. [Google Scholar]
- Bandhari, C.M.; Rowe, D.M. Optimization of Carrier Concentration. CRC Handbook of Thermoelectrics; CRC: Boca Raton, FL, USA, 1995; Chapter 5. [Google Scholar]
- Borisenko, V.E. Semiconducting Silicides; Springer Series in Material Science, 39; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]

| Specimen | a = b [Å] | c [Å] | V [Å] |
|---|---|---|---|
| After Arc Melting | 8.6712(3) | 68.4789(24) | 4458.1(3) |
| RSPS-Specimen 1 | 8.6710(4) | 68.4761(34) | 4459.7(4) |
| RSPS-Specimen 2 | 8.6698(2) | 68.4707(19) | 4456.4(2) |
| Atoms | x | y | z |
|---|---|---|---|
| Ca(6b) | 0 | 0 | 0 |
| Ca(12c) | 0 | 0 | 0.5552(1) |
| Ca(12c) | 0 | 0 | 0.6101(1) |
| Ca(18e) | 0.2505(8) | 0 | 0.2500(0) |
| Ca(36f) | 0.0071(6) | 0.2488(7) | 0.1869(5) |
| Si(12c) | 0 | 0 | 0.6527(2) |
| Si(12c) | 0 | 0 | 0.7184(2) |
| Si(18e) | 0.5785(12) | 0 | 0.2500(0) |
| Si(36f) | 0.0679(10) | 0.3081(8) | 0.1426(8) |
| Si(36f) | 0.3846(7) | 0.0609(8) | 0.0533(9) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, D.O.A.; Fabbiani, M.; Coulomb, L.; Bosc, S.; Villeroy, B.; Estournès, C.; Estournès, C.; Koza, M.M.; Beaudhuin, M.; Viennois, R. Reactive Spark Plasma Sintering and Thermoelectric Properties of Zintl Semiconducting Ca14Si19 Compound. Crystals 2023, 13, 262. https://doi.org/10.3390/cryst13020262
Ali DOA, Fabbiani M, Coulomb L, Bosc S, Villeroy B, Estournès C, Estournès C, Koza MM, Beaudhuin M, Viennois R. Reactive Spark Plasma Sintering and Thermoelectric Properties of Zintl Semiconducting Ca14Si19 Compound. Crystals. 2023; 13(2):262. https://doi.org/10.3390/cryst13020262
Chicago/Turabian StyleAli, Doaa Omar A., Marco Fabbiani, Loïc Coulomb, Simon Bosc, Benjamin Villeroy, Camille Estournès, Claude Estournès, Michael Marek Koza, Mickaël Beaudhuin, and Romain Viennois. 2023. "Reactive Spark Plasma Sintering and Thermoelectric Properties of Zintl Semiconducting Ca14Si19 Compound" Crystals 13, no. 2: 262. https://doi.org/10.3390/cryst13020262
APA StyleAli, D. O. A., Fabbiani, M., Coulomb, L., Bosc, S., Villeroy, B., Estournès, C., Estournès, C., Koza, M. M., Beaudhuin, M., & Viennois, R. (2023). Reactive Spark Plasma Sintering and Thermoelectric Properties of Zintl Semiconducting Ca14Si19 Compound. Crystals, 13(2), 262. https://doi.org/10.3390/cryst13020262








