First-Principles Calculations of Structural and Mechanical Properties of Cu–Ni Alloys

Abstract

Nanostructured Cu–Ni alloys have become the focus of public attention due to their better corrosion resistance and high hardness in experimental measurements. First-principles calculation based on the density functional theory (DFT) has been confirmed as an effective tool and used to illustrate the mechanical properties of these alloys. In this paper, the DFT has been employed to calculate the mechanical properties of Cu–Ni alloys, including bulk modulus, shear modulus, Young’s modulus, anisotropic index, Poisson’s ratio, average velocity, and B/G. We find that the Ni-rich Cu–Ni alloys have relatively higher mechanical parameters, and the Cu-rich alloys have smaller mechanical parameters, which is consistent with previous experiments. This provides an idea for us to design alloys to improve alloy strength.

1. Introduction

Copper and copper-based alloys are commonly used in manufacturing, meaning they are indispensable metals in society. Cu is known to have excellent electrical conductivity, good ductility, and high thermal conductivity, which is an advantage when it is deformed in terms of the geometry structure of devices and microwires [1]. In order to improve the hardness of Cu to meet certain applications, researchers suggest reliable mechanisms that cause mechanical hardening: (1) solid solution strengthening, (2) precipitation hardening [2].

One of the most attractive Cu-based alloys are Cu–Ni binary compounds. Cu–Ni alloys are considered to possess good hardness and corrosion resistance [2,3,4]. Some Cu–Ni alloys have good electrocatalytic [5] and electrical [6] properties in conducting electricity. Other interesting characteristics of Cu–Ni alloys, such as thermal conductivity reduction in nanostructuration [4] and ferromagnetic features in Ni-rich Cu–Ni deposits [7], are revealed. The deposited films of nanostructured Cu-rich Cu–Ni have better corrosion protection than pure Cu, and films have a high hardness [2]. The mechanical properties are widely revealed in experiments for nanostructured Cu–Ni films; they find that Young’s modulus increases with the increasing Ni concentration, and Young’s modulus decreases with the decreasing Cu concentration [8,9,10].

Recently, the Cu–X alloys for Cu–Al, Cu–Sc, Cu–Zn, and Cu–Ce were discussed in formation energy by VASP, which shows that Cu–Ce displays high mechanical energy and strong bonds [11]. The charge density difference and Mulliken population of Mg–Gd–Cu alloys were calculated by first-principles calculations, where the Cu–Gd terminated interface is probably the most stable interface [12]. Cu–Zn₄ alloy has a high Young’s modulus with lower elastic anisotropy, which is rationally recommended for improving the stiffness of Al–Cu–Zn alloys based on first-principles calculations [13]. It can be seen that the first-principles calculation is one of the most important tools to compute mechanical properties, aiming to improve stiffness. Cu–Ni-based alloys have high elasticity and high electrical conductivity [14,15,16], with the addition of Co improving the conductivity [14] and the addition of Sn enhancing the mechanical stability of alloys [15,16]. The high stiffness and corrosion protection found in experiments with Cu–Ni alloys has brought them wide attention. Those features of the mechanical properties of Cu–Ni alloys can also be obtained from calculations, but have been less reported previously.

Therefore, we consider first-principles calculations to discuss the mechanical properties of Cu–Ni alloys in nanostructuration, trying to support and promote the understanding of these alloys.

2. Computational Details

First-principles calculations based on density functional theory (DFT) are executed by the CASTEP code [17], where the GGA-PBE is chosen as the exchange-correlation energy functional and potential [18]. Total energy for cutoff is set to 440 eV to ensure the energy tolerances. K-point mesh for Monkhorst–Pack grid is set to 12 × 12 × 12; the actual spacing for mesh is 0.004 nm⁻¹. In geometry optimization processing, the detailed convergence tolerance for atom is evinced by setting energy to 5.0 × 10⁻⁶ eV/atom, max. force to 0.01 eV/Å, max. stress to 0.02 GPa, and max. displacement to 5.0 × 10⁻⁵ nm, where the max. iteration is 100 to ensure the energy convergence. The Broyden–Fletcher–Goldfarb–Shanno (BFGS) method is applied to mathematical algorithm for convergence calculation [19].

As shown in Figure 1, three Cu–Ni alloys are implanted in this paper, containing Cu_0.5Ni_0.5, Ni_0.92Cu_0.08, and Cu_0.95Ni_0.05 with same space group $Fm\overline{3}m$. Red points for k-point and green dash for Brillouin edge are marked, respectively. The vectors demonstrate the direction in reciprocal space by $\overrightarrow{\mathrm{g}}1$, $\overrightarrow{\mathrm{g}}2$, $\overrightarrow{\mathrm{g}}3$. The results of geometry optimization are listed in

Table 1. Optimized lattice parameter for Cu–Ni alloys with experiments [2,9,10].

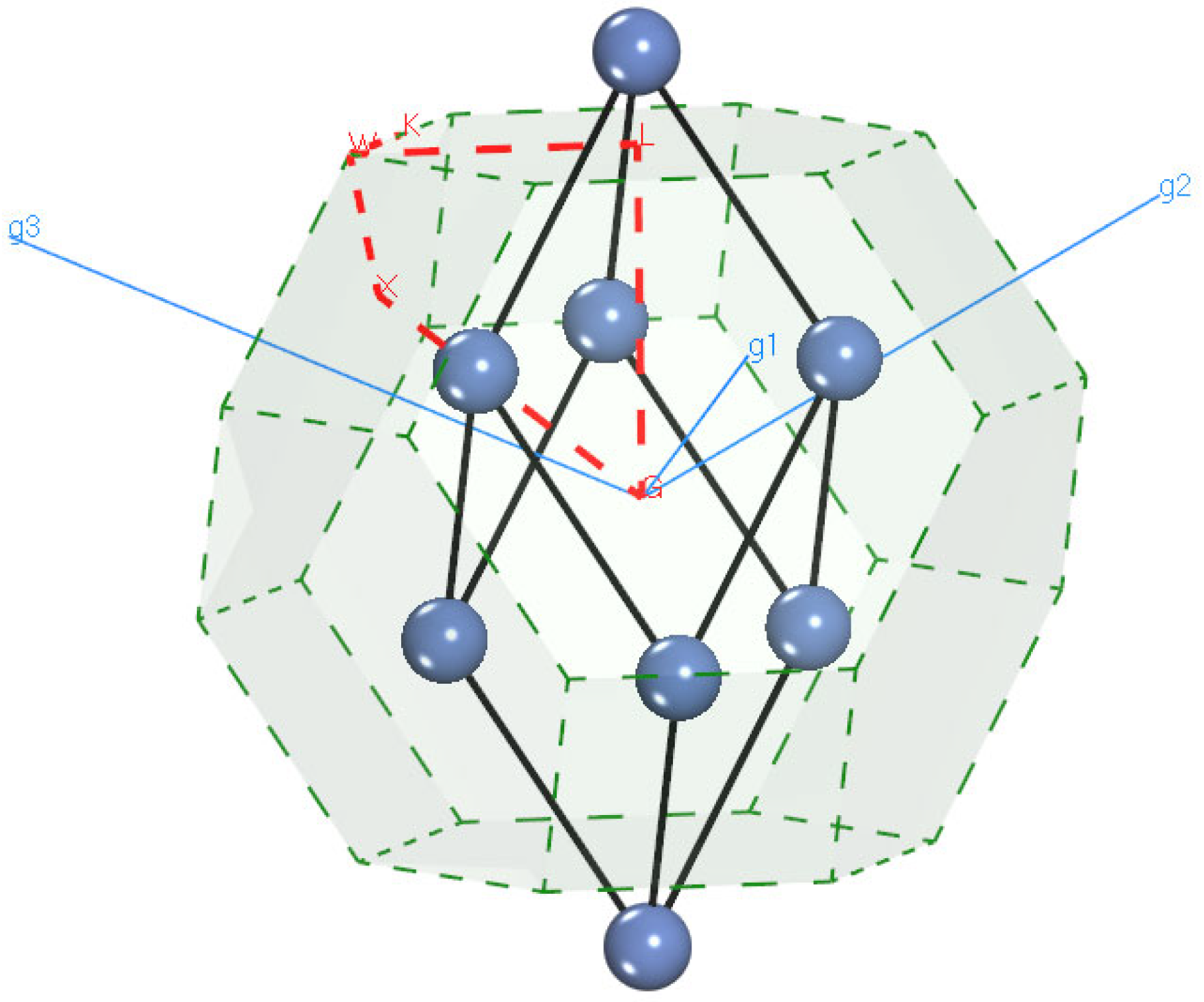
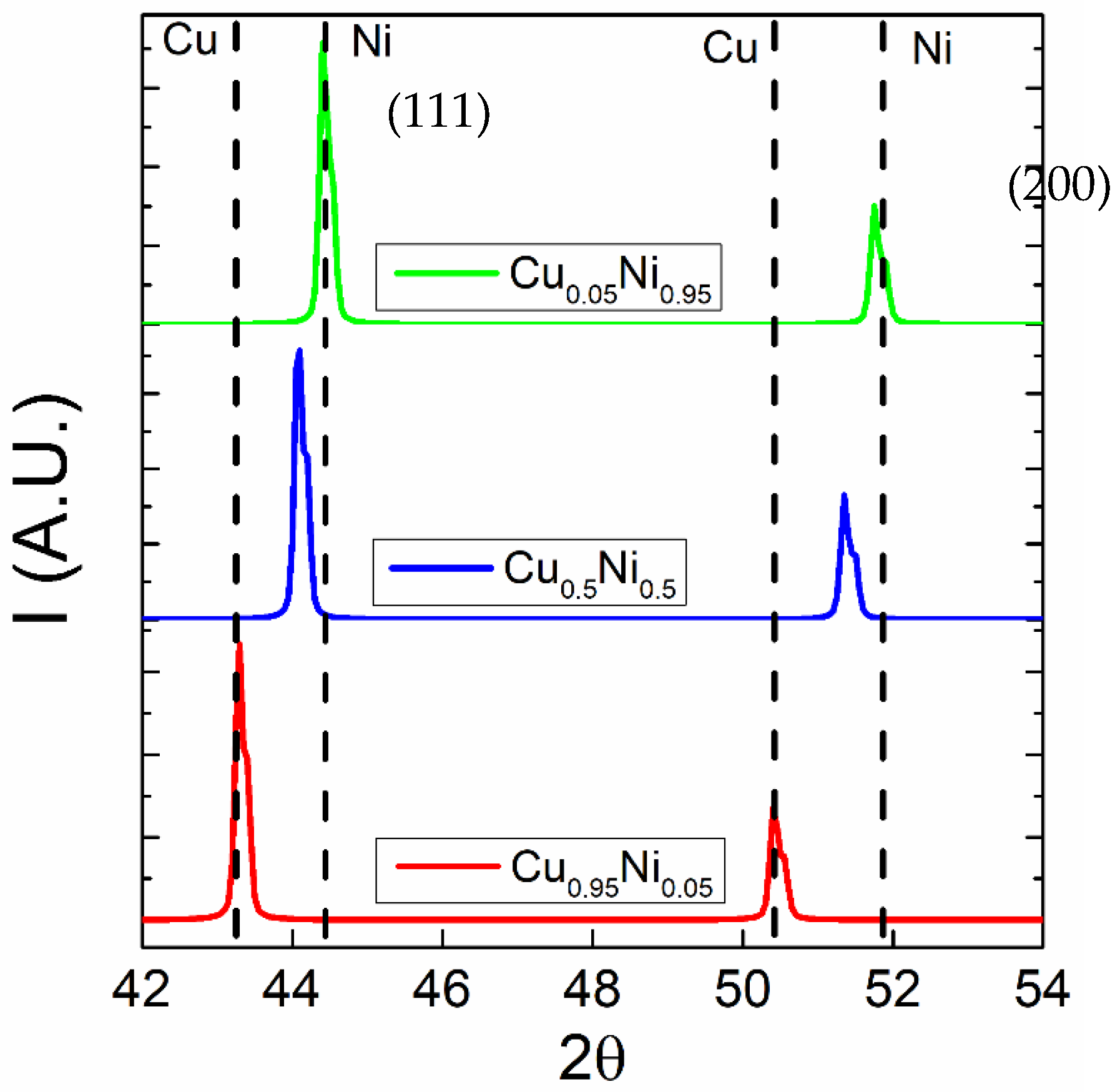
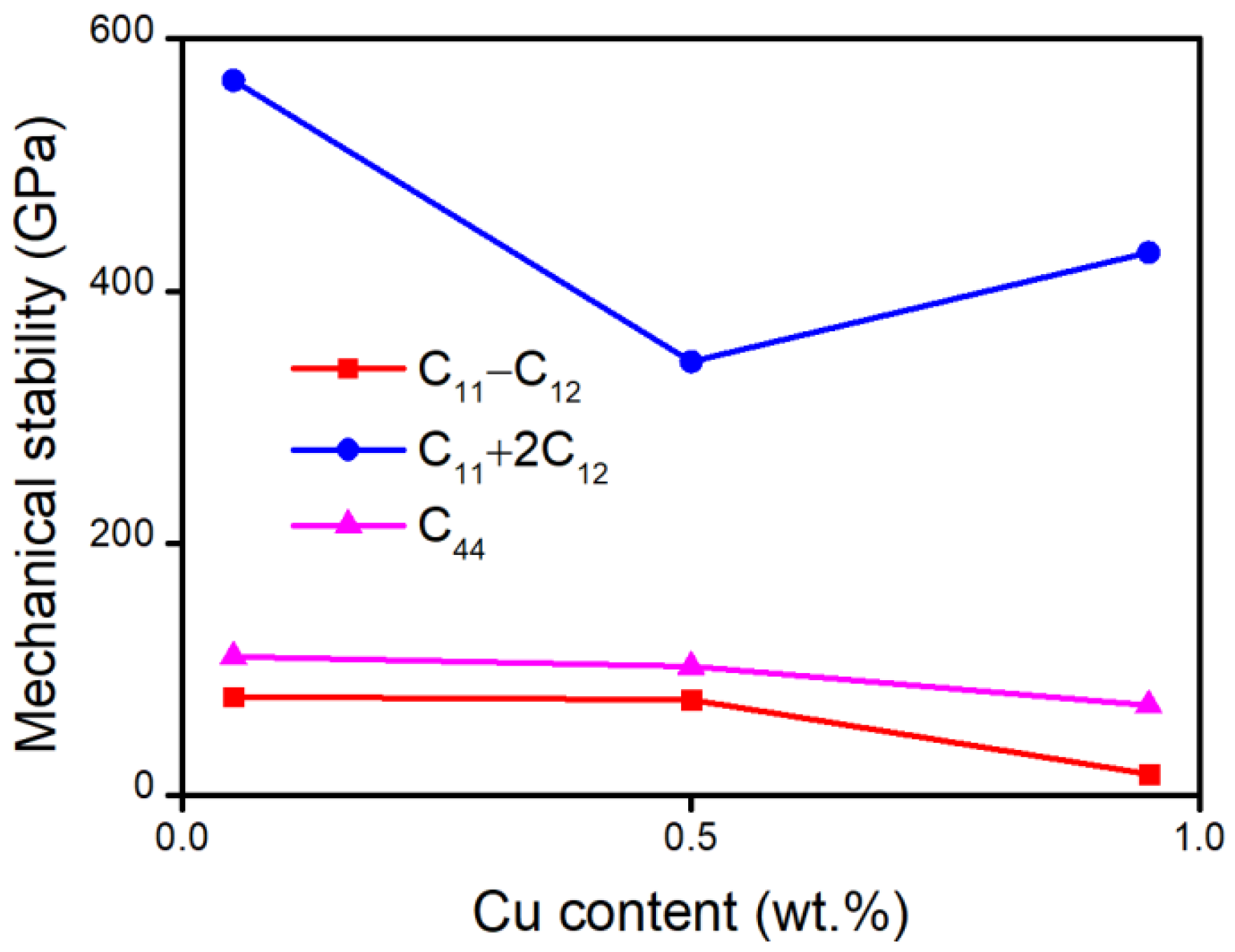
Composition	Lattice Parameter a (nm)	Density (g·cm−3)	Volume (nm3)
Cu0.08Ni0.92	0.35302	8.9223	0.010999
Cu0.5Ni0.5	0.35557	9.0315	0.011239
Cu0.95Ni0.05	3.6174	8.8827	0.011834
Cu0.97Ni0.03	0.35836 [2]
Cu0.46Ni0.54	0.3560 2 [2]
Cu0.55N0.45	0.3561 [9]
Cu0.13Ni0.87	0.3534 [9]
Cu100Ni0	0.36148 [10]
Cu0Ni100	0.35232 [10]

. It can be seen that the large Cu concentration has the larger lattice parameters, and the small atom percentage of Ni concentration has the smaller lattice parameters, which are consistent with experiments. Lattice parameters error is less than 2% compared with XRD data [2,9,10]. Figure 2 shows the simulated XRD patterns of Cu–Ni alloys based on pseudo-Voigt peak shape profile [20], where the peak broadening accounting for instrument broadening is described by Caglioti equation [21] by the U = 0.05, V = 0.002, and W = 0.002; the [UVW] is the direction of incident of the electron beam. It can be seen that the XRD patterns of Cu–Ni alloys are similar; the peak for (111) and (200) reflections shift towards larger angles as the Ni contents increase. The peak position and peak tendency for Cu–Ni alloys are in great agreement with experiments [2,9,10], conforming to the observed particle shapes and crystallite sizes. Hence, these structures are considered to further compute the mechanical properties with GGA-PBE functional.

3. Mechanical Properties

The elastic constants are important to understand the physical properties and engineering applications [22,23,24]. Calculated elastic constant $C_{\rho \sigma }$ can be solved by energy density $u$ with tensors $s_{\rho }$$s_{\sigma }$, which was first noted by Born and Huang [25]:

$$u=\frac{1}{2}{\displaystyle \sum _{\rho \sigma }{C}_{\rho \sigma }{s}_{\rho }{s}_{\sigma }}$$

(1)

where the $\rho$, $\sigma$ are tensor notations in Voigt’s way. Then, the famous Hooke’s law can be listed:

$$S_{\rho }=\frac{\partial u}{\partial s_{\rho }}={\displaystyle \sum _{\sigma }{C}_{\rho \sigma }{s}_{\sigma }}$$

(2)

The $S_{\rho }$ is elastic stress. When the elastic constants are obtained, the mechanical properties of the crystal can be solved by the elastic constant matrix. The Voigt, Reuss, and Hill [26,27,28] methods are popular approaches for mechanical properties including bulk modulus B, shear modulus G, average velocity v_m, Young’s modulus E, ratio B/G, and Poisson’s ratio $v$. In the cubic system, the equation can be simple:

$$B_V=\frac{C_{11}+2C_{12}}{3}$$

(3)

$$G_V=\frac{C_{11}-C_{12}+3C_{44}}{5}$$

(4)

$$B_R=\frac{1}{3S_{11}+6S_{12}}$$

(5)

Average value for Hill’s way is widely used to describe the mechanical properties, i.e.:

$$B=B_{VRH}=\frac{B_V+B_R}{2}$$

(6)

$$G=G_{VRH}=\frac{G_V+G_R}{2}$$

(7)

The average velocity for the crystal can be solved by [13]:

$$v_m={\left[\frac{1}{3}\left(\frac{2}{v_t^3}+\frac{1}{v_l^3}\right)\right]}^{-1/3}$$

(8)

$$v_l={\left(\frac{3B+4G}{3\rho }\right)}^{1/2}$$

(9)

$$v_t={\left(\frac{G}{\rho }\right)}^{1/2}$$

(10)

Other mechanical properties for Young’s modulus E, anisotropic index A_U, and Poisson’s ratio v are expressed [13]:

$$E=\frac{9BG}{3B+G}$$

(11)

$$A_U=5\frac{G_v}{G_R}-\frac{B_v}{B_R}-6$$

(12)

$$v=\frac{3B-2G}{2(3B+G)}$$

(13)

The ratio of B/G has been an adopted criterion to judge the ductility and brittleness of solids; if B/G > 1.75, the solid behaves in a ductile manner, otherwise it behaves in a brittle manner [29]. Based on DFT, the energy density $u$ can be easily obtained. The calculated mechanical properties are shown in

Table 2. Calculated mechanical properties of Cu–Ni alloys, $C_{\rho \sigma }$ is elastic constant, B is bulk modulus, G is shear modulus, v_m is average velocity, E is Young’s modulus, and $v$ is Poisson’s ratio.

	C11	C12	C44	B	G	E	AU	$\mathit{v}$	vm	B/G
Cu0.05Ni0.95	241.1	163.0	110.0	189.0	72.7	193.3	1.405	0.330	3145.7	2.60
Cu0.5Ni0.5	165.2	89.4	102.2	114.6	68.7	171.7	1.278	0.250	3002.2	1.67
Cu0.95Ni0.05	154.8	137.9	71.4	143.5	32.1	89.6	7.884	0.396	2011.8	4.47
Cu0.97Ni0.03						111 [2]
Cu0.46Ni0.54						163 [2]
Cu0.55N0.45						172 [9]
Cu0.13Ni0.87						195 [9]
Cu100Ni0						158 [10]

, solving from the Equations (3) to (13).

Firstly, for different works, the Cu-rich Cu content in Cu–Ni alloys has a smaller Young’s modulus in their respective results, whatever the simulation or experimental measurements. Our calculated Young’s modulus is less small than that of these other experiments, which is caused by the slight difference in the approaching process. It is reasonable and meaningful to further discuss the mechanical properties in calculation for deep consideration.

In Figure 3a, elastic constants refer to the deformation resistance of the crystal in anisotropy. In Voigt’s way, the subscript of C₁₁ represents the deformation resistance in the x-axis for Cu–Ni alloys. The calculated elastic constant for C₁₁ reduces with the increasing Cu content, indicating that the deformation resistance in the x-axis for Cu–Ni is becoming weak. Similarly, C₄₄ represents the deformation resistance in the x,y-axis becoming weak, while C₁₂ represents the deformation resistance in the y-axis to stress in the x-axis decreasing first, then increasing. The Cu content in the crystal has a great effect on deformation resistance in the x-axis. In Figure 3b, the popular moduli for materials are shown in different Cu content. Bulk modulus has a similar tendency as C_12, showing the total deformation resistance of alloys. Shear modulus G and Young’s modulus E decrease in Cu-rich Cu content, predicting the fluctuation of hardness.

In Figure 4a, for the red curve, there is a typical transition from ductile to brittle. In Cu-rich Cu_0.95Ni_0.05 and Ni-rich Cu_0.05Ni_0.85 alloys, they are greatly ductile with the B/G > 1.75; meanwhile, the Cu-rich alloys have the higher ductility. On the other hand, the Cu_0.5Ni_0.5 alloys are brittle due to the B/G = 1.67. It shows that the right ratio for the binary compound of Cu–Ni systems is beneficial to improve the brittleness of pure metal. For the blue curve, the anisotropic index has the same tendency, where the Cu_0.5Ni_0.5 alloy with the smallest numerical index presented the lowest anisotropy. The low anisotropy with higher Young’s modulus shows that the Cu_0.5Ni_0.5 alloy has a great stiffness.

For Poisson’s ratio $v$ in Figure 4a, we can obtain the same results as B/G. When the Poisson’s ratio v > 0.26, the alloys are ductile; otherwise, it behaves in a brittle way [13]. That is, the Cu_0.5Ni_0.5 alloy is brittle. In Figure 4b, the average velocity decreases with the increasing Cu content; the significant drop can be found in the Cu_0.5Ni_0.5 position. It shows that the Cu_0.5Ni_0.5 alloys have a good elastic strength by loading elastic wave velocity, almost comparable with pure copper.

Elastic constant can be applied in a mechanical stability criterion, to reveal the mechanical stability of a crystal. For the cubic system, the criterion can be written simply [30]:

$$C_{11}-C_{12}>0, C_{11}+2C_{12}>0, C_{44}>0$$

(14)

We put the elastic constants from Table 2 in the mechanical stability criterion, and the detailed results are shown in Figure 5; it can be seen that the alloys are mechanically stable.

4. Conclusions

First-principles calculations-based density functional theory is used in this paper; the lattice crystal and mechanical properties for Cu–Ni alloys are good, illustrated in the stiffness compared with the experiment for nanostructured Cu–Ni films. Firstly, we employ the pseudo-Voigt way to simulate the XRD results, showing the crystal is a reliable structure. Then, for the mechanical properties we computed, the main results are included as a list.

• Based on DFT, we calculated elastic constants, bulk modulus, shear modulus, Young’s modulus, anisotropic index A_U, Poisson’s ratio v, average velocity, and B/G in this paper. It improves and supports the results for the experiment.
• Cu-rich and Ni-rich Cu–Ni alloys are ductile; the Ni-rich alloy has the highest uniaxial deformation resistance due to having the largest Young’s modulus.
• Cu_0.5Ni_0.5 as the most suitable binary compound is predicted to have great stiffness in the Cu–Ni system, due to the brittleness and low anisotropy.